Investigation on the Performance of CO2 Absorption in Ceramic Hollow-Fiber Gas/Liquid Membrane Contactors
Abstract
1. Introduction
2. Theoretical Formulations
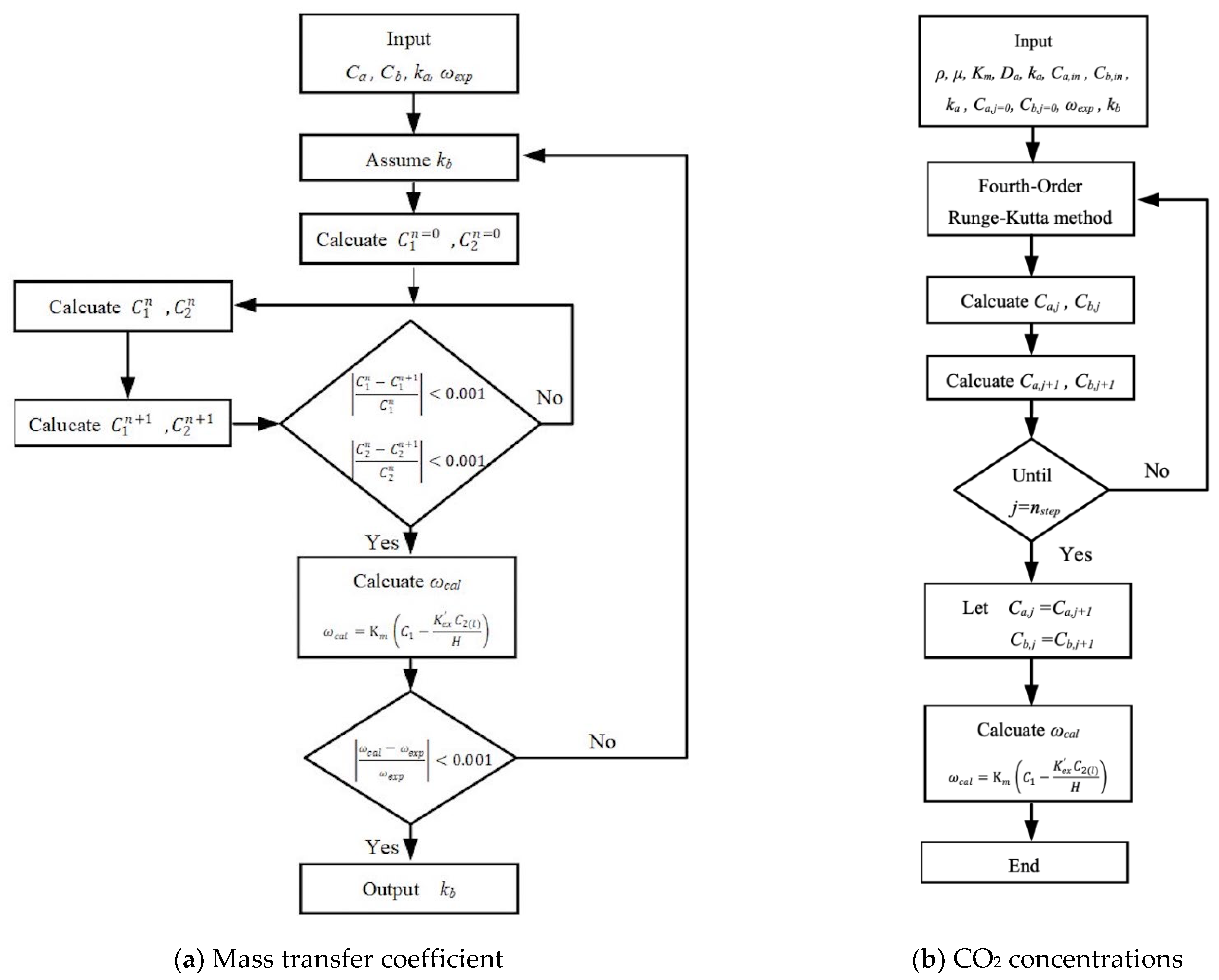
3. Numerical Solutions
4. Enhancement Factor
5. Experimental Runs
5.1. Apparatus and Procedure
5.2. Chemicals and Materials
6. Results and Discussions
6.1. CO2 Absorption Rate Enhancement
6.2. Mass Transfer Enhancement Factor
6.3. Energy Consumption Increment
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| CO2 concentration (mol m−3) | |
| Equivalent hydraulic diameter of channel (m), | |
| Deviation of experimental results from the theoretical predictions | |
| Membrane coefficient based on the Knudsen diffusion model () | |
| Membrane coefficient based on the molecular diffusion model () | |
| Membrane permeation coefficient () | |
| Diffusion coefficient of CO2 in N2 (m2/s) | |
| Diffusion coefficient of CO2 in MEA (m2/s) | |
| Diffusion coefficient of air and vapor in the membrane (m2/s) | |
| Fanning friction factor | |
| Henry’s constant | |
| Hydraulic dissipate energy (J kg−1), | |
| Absorption rate enhancement | |
| Power consumption increment | |
| Mass transfer coefficient in the CO2/N2 feed stream (m s−1) | |
| Mass transfer coefficient of the MEA absorbent stream (m s−1) | |
| Equilibrium constant | |
| Reduced equilibrium constant | |
| Overall mass transfer coefficient of the membrane (m s−1) | |
| Mass transfer of carbon dioxide () | |
| Friction loss of CO2 (J kg−1), | |
| Channel length (m) | |
| Molecular weight of water (kg mol−1) | |
| Absorption rate () | |
| Number of experimental measurements | |
| Number of iterations | |
| Saturation vapor pressure at membrane surface in CO2/N2 feed flow side (Pa) | |
| Saturation vapor pressure at membrane surface in the MEA absorbent flow side (Pa) | |
| Volumetric flow rate of the CO2/N2 feed stream (m3 s−1) | |
| Volumetric flow rate of the absorbent feed stream (m3 s−1) | |
| Transversal coordinate (m) | |
| Free surface radius (m) | |
| Fiber inside radius (m) | |
| Membrane pore radius (m) | |
| Fiber outside radius (m) | |
| Shell outside radius (m) | |
| Gas constant (8.314 J mol−1 K−1) | |
| Reynolds number | |
| Precision index of an experimental measurements of molar flux (mol m−2 s−1) | |
| Mean value of (mol m−2 s−1) | |
| Dimensionless Schmidt number | |
| Enhanced Sherwood number | |
| Schmidt number for laminar flow | |
| Average velocity () | |
| Natural log mean CO2 mole fraction in the membrane | |
| Averaged velocity of fluid (m/s) | |
| Axial coordinate along the flow direction (m) | |
| Greek letters | |
| Mass transfer enhancement factor | |
| Aspect ratio of MEA absorbent channel | |
| Concentration polarization coefficients | |
| Membrane thickness (µm) | |
| Membrane porosity | |
| Density (), | |
| Membrane tortuosity | |
| Packing density | |
| Absorption flux () | |
| Subscripts | |
| 1 | Membrane surface on gas side |
| Liquid phase on membrane surface on MEA side | |
| Gas phase on membrane surface on MEA side | |
| a | In the /N2 feed stream |
| b | In the MEA absorbent stream |
| cal | Calculated results |
| exp | Experimental results |
| in | At the Inlet |
| m | In the membrane |
| out | At the Outlet |
| theo | Theoretical predictions |
References
- Dowell, N.M.; Fennell, P.S.; Shah, N.; Maitland, G.C. The role of CO2 capture and utilization in mitigating climate change. Nat. Clim. Chang. 2017, 7, 243–249. [Google Scholar] [CrossRef]
- Mangalapally, H.P.; Notz, R.; Hoch, S.; Asprion, N.; Sieder, G.; Garcia, H.; Hasse, H. Pilot plant experimental studies of post combustion CO2 capture by reactive absorption with MEA and new solvents. Energy Procedia 2009, 1, 963–970. [Google Scholar] [CrossRef]
- Eide-Haugmo, I.; Lepaumier, H.; Einbu, A.; Vernstad, K.; da Silva, E.F.; Svendsen, H.F. Chemical stability and biodegradability of new solvents for CO2 capture. Energy Procedia 2011, 4, 1636–1637. [Google Scholar] [CrossRef]
- Li, R.; Xu, J.; Wang, L.; Li, J.; Sun, X. Reduction of VOC emissions by a membrane-based gas absorption process. J. Environ. Sci. 2009, 21, 1096–1102. [Google Scholar] [CrossRef] [PubMed]
- Li, J.L.; Chen, B.H. Review of CO2 absorption using chemical solvents in hollow fiber membrane contactors. Sep. Purif. Technol. 2005, 41, 109–122. [Google Scholar] [CrossRef]
- Yeon, S.H.; Lee, K.S.; Sea, B.; Park, Y.I.; Lee, K.H. Application of pilot-scale membrane contactor hybrid system for removal of carbon dioxide from flue gas. J. Membr. Sci. 2005, 257, 156–160. [Google Scholar] [CrossRef]
- Al-Marzouqi, M.H.; El-Naas, M.H.; Marzouk, S.A.M.; Al-Zarooni, M.A.; Abdullatif, N.; Faiz, R. Modeling of CO2 absorption in membrane contactors. Sep. Purif. Technol. 2008, 59, 286–293. [Google Scholar] [CrossRef]
- Wang, M.; Lawal, A.; Stephenson, P.; Sidders, J.; Ramshaw, C. Post-combustion CO2 capture with chemical absorption: A state-of-the-art review. Chem. Eng. Res. Des. 2011, 89, 1609–1624. [Google Scholar] [CrossRef]
- Rochelle, G.T. Amine scrubbing for CO2 capture. Science 2009, 325, 1652–1654. [Google Scholar] [CrossRef]
- Liu, H.; Gao, H.; Idem, R.; Tontiwachwuthikul, P.; Liang, Z. Analysis of CO2 solubility and absorption heat into 1-dimethylamino-2-propanol solution. Chem. Eng. Sci. 2017, 170, 3–15. [Google Scholar] [CrossRef]
- Harbou, I.V.; Imle, M.; Hasse, H. Modeling and simulation of reactive absorption of CO2 with MEA: Results for four different packing on two different scales. Chem. Eng. Sci. 2014, 105, 179–190. [Google Scholar] [CrossRef]
- Zhang, Z.E.; Yan, Y.F.; Zhang, L.; Ju, S.X. Hollow fiber membrane contactor absorption of CO2 from the flue gas: Review and perspective. Glob. Nest Int. J. 2014, 16, 354–374. [Google Scholar]
- Zhang, Z.E.; Yan, Y.F.; Zhang, L.; Ju, S.X. Numerical simulation and analysis of CO2 removal in a polypropylene hollow fiber membrane contactor. Int. J. Chem. Eng. 2014, 2014, 56–62. [Google Scholar] [CrossRef]
- Wang, W.P.; Lin, H.T.; Ho, C.D. An Analytical Study of Laminar Co-Current Flow Gas Absorption through a Parallel-Plate Gas-Liquid Membrane Contactor. J. Membr. Sci. 2006, 278, 181–189. [Google Scholar] [CrossRef]
- Qi, Z.; Cussler, E.L. Microporous hollow fibers for gas absorption. II. Mass transfer across the membrane. J. Membr. Sci. 1985, 23, 333–345. [Google Scholar] [CrossRef]
- Boributh, S.; Jiraratananon, R.; Li, K. Analytical solutions for membrane wetting calculations based on log-normal and normal distribution functions for CO2 absorption by a hollow fiber membrane contactor. J. Membr. Sci. 2013, 429, 459–472. [Google Scholar] [CrossRef]
- Karoor, S.; Sirkar, K.K. Gas absorption studies in microporous hollow fiber membrane modules. Ind. Eng. Chem. Res. 1993, 32, 674–684. [Google Scholar] [CrossRef]
- Bernardo, P.; Drioli, E.; Golemme, G. Membrane gas separation: A review/state of the art. Ind. Eng. Chem. Res. 2009, 48, 4638–4663. [Google Scholar] [CrossRef]
- Ramachandran, N.; Aboudheir, A.; Idem, R.; Tontiwachwuthikul, P. Kinetics of the absorption of CO2 into mixed aqueous loaded solutions of monoethanolamine and methyldiethanolamine. Ind. Eng. Chem. Res. 2006, 45, 2608–2616. [Google Scholar] [CrossRef]
- Hamimour, N.; Sandall, O.C. Absorption of carbon dioxide into aqueous methyldiethanolamine. Chem. Eng. Sci. 1984, 39, 1791–1796. [Google Scholar] [CrossRef]
- Tobiesen, F.A.; Svendsen, H.F. Study of a modified amine-based regeneration unit. Ind. Eng. Chem. Res. 2006, 45, 2489–2496. [Google Scholar] [CrossRef]
- Rongwong, W.; Assabumrungrat, S.; Jiraratananon, R. Rate based modeling for CO2 absorption using monoethanolamine solution in a hollow fiber membrane contactor. J. Membr. Sci. 2013, 429, 396–408. [Google Scholar] [CrossRef]
- Faiz, R.; Al-Marzouqi, M. CO2 removal from natural gas at high pressure using membrane contactors: Model validation and membrane parametric studies. J. Membr. Sci. 2010, 365, 232–241. [Google Scholar] [CrossRef]
- Rezakazemi, M.; Sadrzadeh, M.; Matsuura, T. Thermally stable polymers for advanced high-performance gas separation membranes. Prog. Energy Combust. Sci. 2018, 66, 1–41. [Google Scholar] [CrossRef]
- Belaissaoui, B.; Favre, E. Evaluation of a dense skin hollow fiber gas-liquid membrane contactor for high pressure removal of CO2 from syngas using Selexol as the absorbent. Chem. Eng. Sci. 2018, 184, 186–199. [Google Scholar] [CrossRef]
- Lin, Y.F.; Ko, C.C.; Chen, C.H.; Tung, K.L.; Chang, K.S.; Chung, T.W. Sol-gel preparation of polymethylsilsesquioxane aerogel membranes for CO2 absorption fluxes in membrane contactors. Appl. Energy 2014, 129, 25–31. [Google Scholar] [CrossRef]
- Lin, Y.F.; Kuo, J.W. Mesoporous bis(trimethoxysilyl)hexane (BTMSH)/tetraethyl orthosilicate (TEOS)-based hybrid silica aerogel membranes for CO2 capture. Chem. Eng. J. 2016, 300, 29–35. [Google Scholar] [CrossRef]
- Yang, M.C.; Cussler, E.L. Designing hollow-fiber contactor. AIChE J. 1896, 32, 1910–1915. [Google Scholar] [CrossRef]
- Costello, M.J.; Fane, A.G.; Hogan, P.A.; Schofield, R.W. The effect of shell side hydrodynamics on the performance of axial flow hollow fiber modules. J. Membr. Sci. 1993, 80, 1–11. [Google Scholar] [CrossRef]
- Lipnizki, F.; Field, R.W. Mass transfer performance for hollow fiber modules with shell-side axial feed flow: Using an engineering approach to develop a framework. J. Membr. Sci. 2001, 193, 195–208. [Google Scholar] [CrossRef]
- Chen, V.; Hlavacek, M. Application of Voronoi tessellation for modeling randomly packed hollow-fiber bundles. AIChE J. 1994, 40, 606–612. [Google Scholar] [CrossRef]
- Roger, J.D.; Long, R. Modeling hollow fiber membrane contactors using film theory, Voronoi tessellations, and facilitation factors for systems with interface reactions. J. Membr. Sci. 1997, 134, 1–17. [Google Scholar] [CrossRef]
- Zhang, Z.E.; Yan, Y.F.; Zhang, L.; Ju, S.X.; Chen, Y.X.; Ran, J.Y.; Pu, G.; Qin, C.L. Theoretical Study on CO2 Absorption from Biogas by Membrane Contactors. Ind. Eng. Chem. Res. 2014, 53, 14075–14083. [Google Scholar] [CrossRef]
- Lee, H.J.; Park, Y.G.; Kim, M.K.; Lee, S.H.; Park, J.H. Study on CO2 absorption performance of lab-scale ceramic hollow fiber membrane contactor by gas/liquid flow direction and module design. Sep. Purif. Technol. 2019, 220, 189–196. [Google Scholar] [CrossRef]
- Zheng, J.M.; Xu, Y.Y.; Xu, Z.K. Shell side mass transfer characteristics in a parallel flow hollow fiber membrane module. Sep. Sci. Tech. 2003, 6, 1247–1267. [Google Scholar] [CrossRef]
- Cooney, D.O.; Jackson, C.C. Gas absorption in a hollow fiber device. Chem. Eng. Comm. 1989, 79, 153–163. [Google Scholar] [CrossRef]
- Happel, J. Viscous flow relative to arrays of cylinders. AIChE J. 1959, 5, 174–177. [Google Scholar] [CrossRef]
- Srisurichan, S.; Jiraratananon, R.; Fane, A.G. Mass transfer mechanisms and transport resistances in direct contact membrane distillation process. J. Membr. Sci. 2006, 277, 186–194. [Google Scholar] [CrossRef]
- Lawson, K.W.; Lloyd, D.R. Membrane distillation. J. Membr. Sci. 1997, 124, 1–25. [Google Scholar] [CrossRef]
- Ding, Z.W.; Ma, R.Y.; Fane, A.G. A new model for mass transfer in direct contact membrane distillation. Desalination 2003, 151, 217–227. [Google Scholar] [CrossRef]
- Iversen, S.B.; Bhatia, V.K.; Dam-Jphasen, K.; Jonson, G. Characterization of microporous membranes for use in membrane contactors. J. Membr. Sci. 1997, 130, 205–217. [Google Scholar] [CrossRef]
- Bhattacharya, S.; Hwang, S.T. Concentration polarization, separation factor, and Peclet number in membrane processes. J. Membr. Sci. 1997, 132, 73–90. [Google Scholar] [CrossRef]
- Zheng, Q.; Dong, L.; Chen, J.; Gao, G.; Fei, W. Absorption solubility calculation and process simulation for CO2 capture. J. Chem. Ind. Eng. 2010, 61, 1740–1746. [Google Scholar]
- Bandini, S.; Gostoli, C.; Sarti, G.C. Role of heat and mass transfer in membrane distillation process. Desalination 1991, 81, 91–106. [Google Scholar] [CrossRef]
- Lou, J.C.; Vanneste, J.; DeCaluwe, S.C.; Cath, T.Y.; Tilton, N. Computational fluid dynamics simulations of polarization phenomena in direct contact membrane distillation. J. Membr. Sci. 2019, 591, 117150–117167. [Google Scholar] [CrossRef]
- Shakaib, M.; Hasani, S.M.F.; Mahmood, M. CFD modeling for flow and mass transfer in spacer-obstructed membrane feed channels. J. Membr. Sci. 2009, 326, 270–284. [Google Scholar] [CrossRef]
- Lin, S.H.; Tung, K.L.; Chang, H.W.; Lee, K.R. Influence of Fluorocarbon Fat-Membrane Hydrophobicity on Carbon Dioxide Recovery. Chemosphere 2009, 75, 1410–1416. [Google Scholar] [CrossRef]
- Ciulla, G.; D’Amico, A.; Brano, V.L. Evaluation of building heating loads with dimensional analysis: Application of the Buckingham π theorem. Energy Build. 2017, 154, 479–490. [Google Scholar] [CrossRef]
- Welty, J.R.; Wicks, C.E.; Wilson, R.E. Fundamentals of Momentum, Heat, and Mass Transfer, 3rd ed.; John Wiley & Sons: New York, NY, USA, 1984. [Google Scholar]
- Hu, C.; Wang, M.S.; Chen, C.H.; Chen, Y.R.; Huang, P.H.; Tung, K.L. Phosphorus-doped g-C3N4 integrated photocatalytic membrane reactor for wastewater treatment. J. Membr. Sci. 2019, 580, 1–11. [Google Scholar] [CrossRef]
- Chen, C.H.; Meng, L.; Tung, K.L.; Lin, Y.S. Effect of substrate curvature on microstructure and gas permeability of hollow fiber MFI zeolite membranes. AIChE J. 2018, 64, 3419–3428. [Google Scholar] [CrossRef]
- Moffat, R.J. Describing the uncertainties in experimental results. Exp. Therm. Fluid Sci. 1988, 1, 3–17. [Google Scholar] [CrossRef]
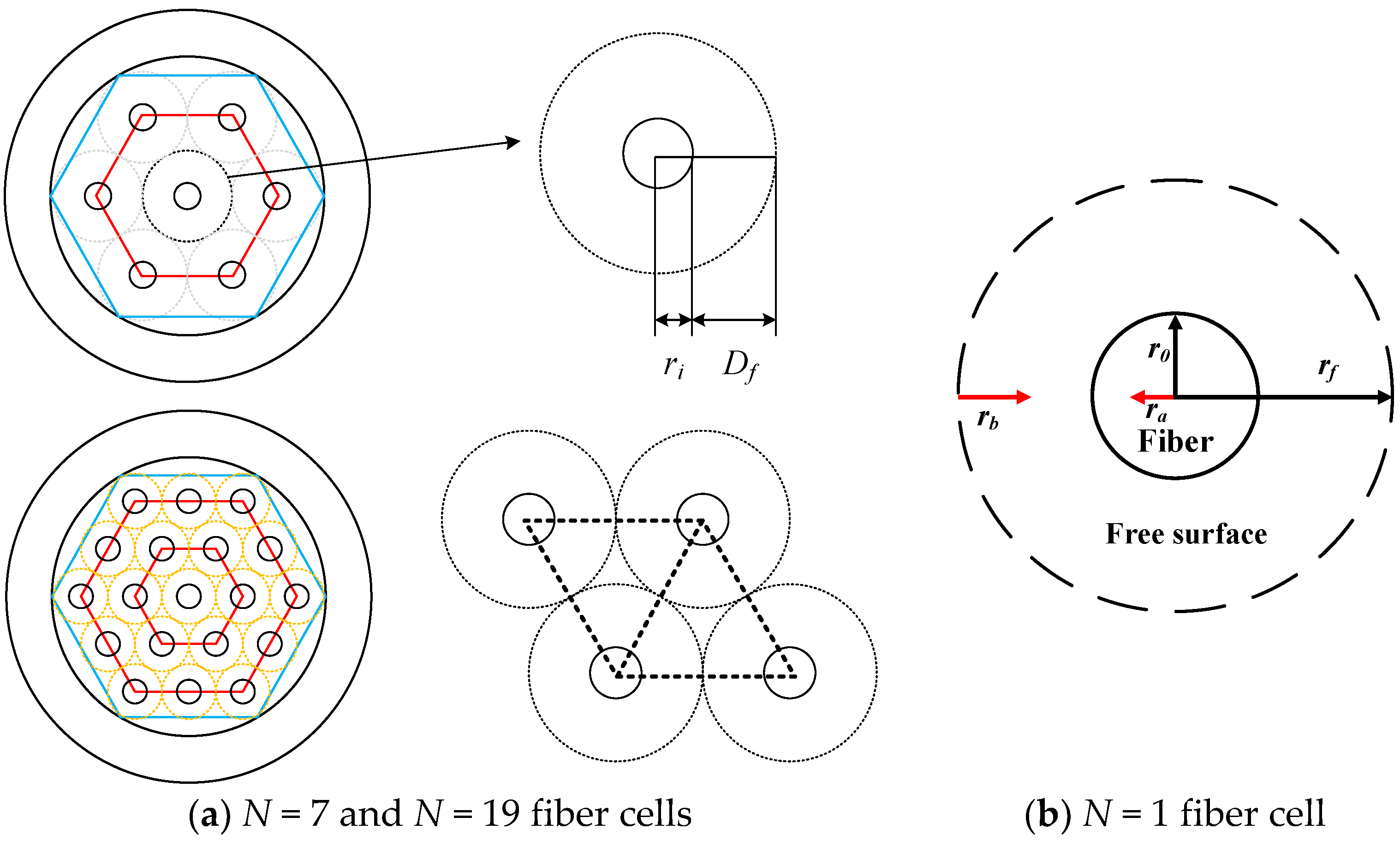
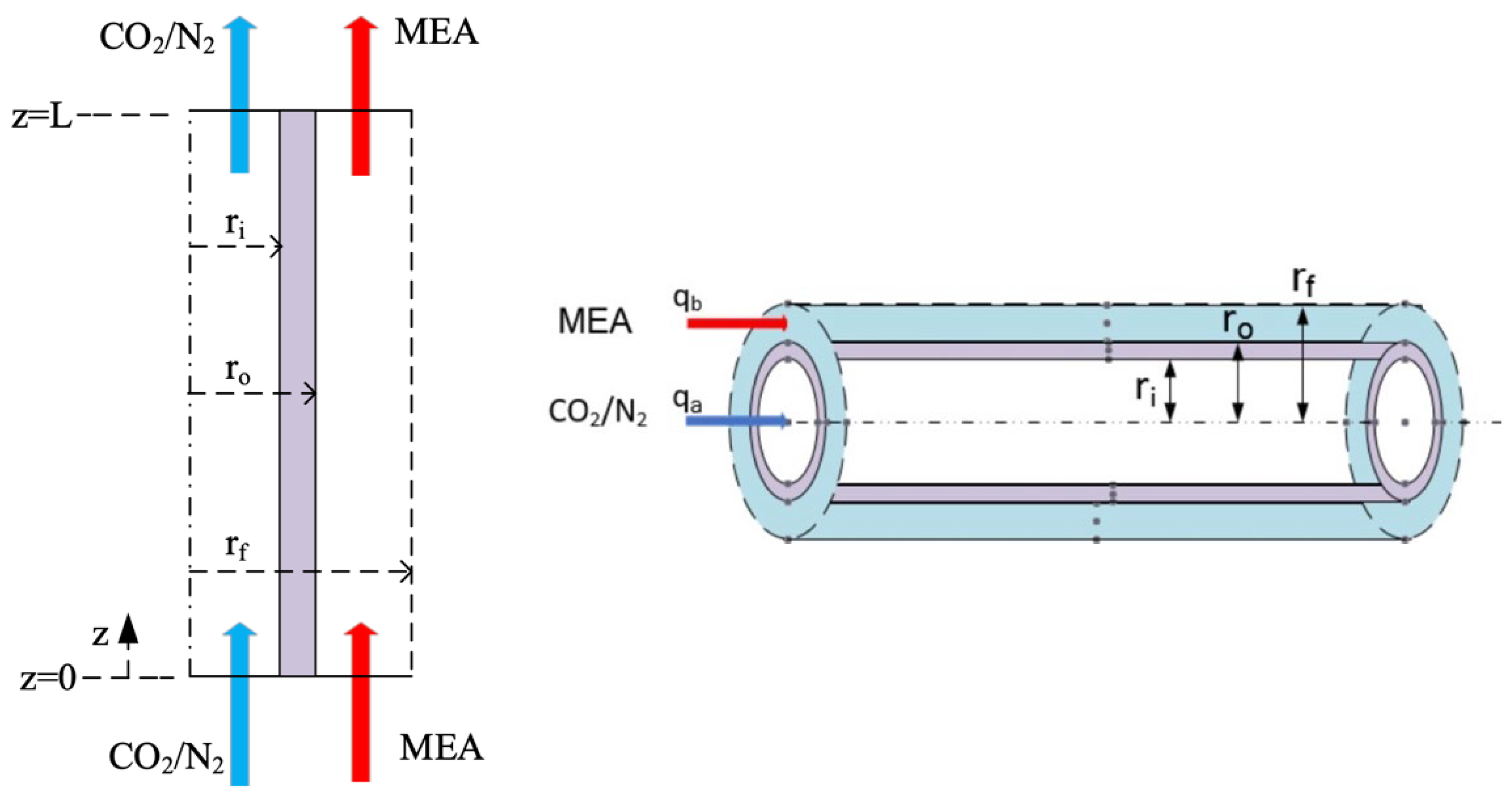
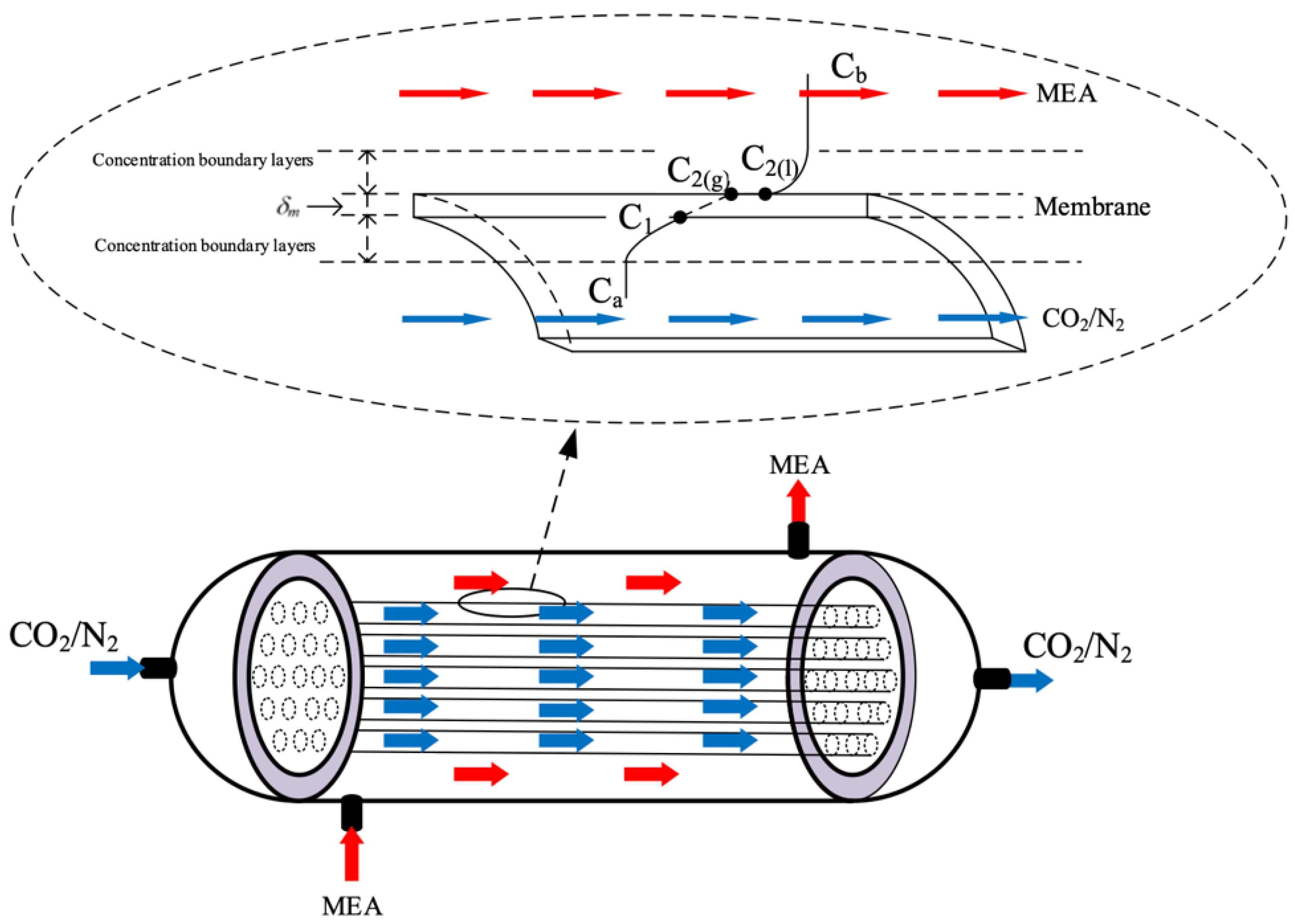
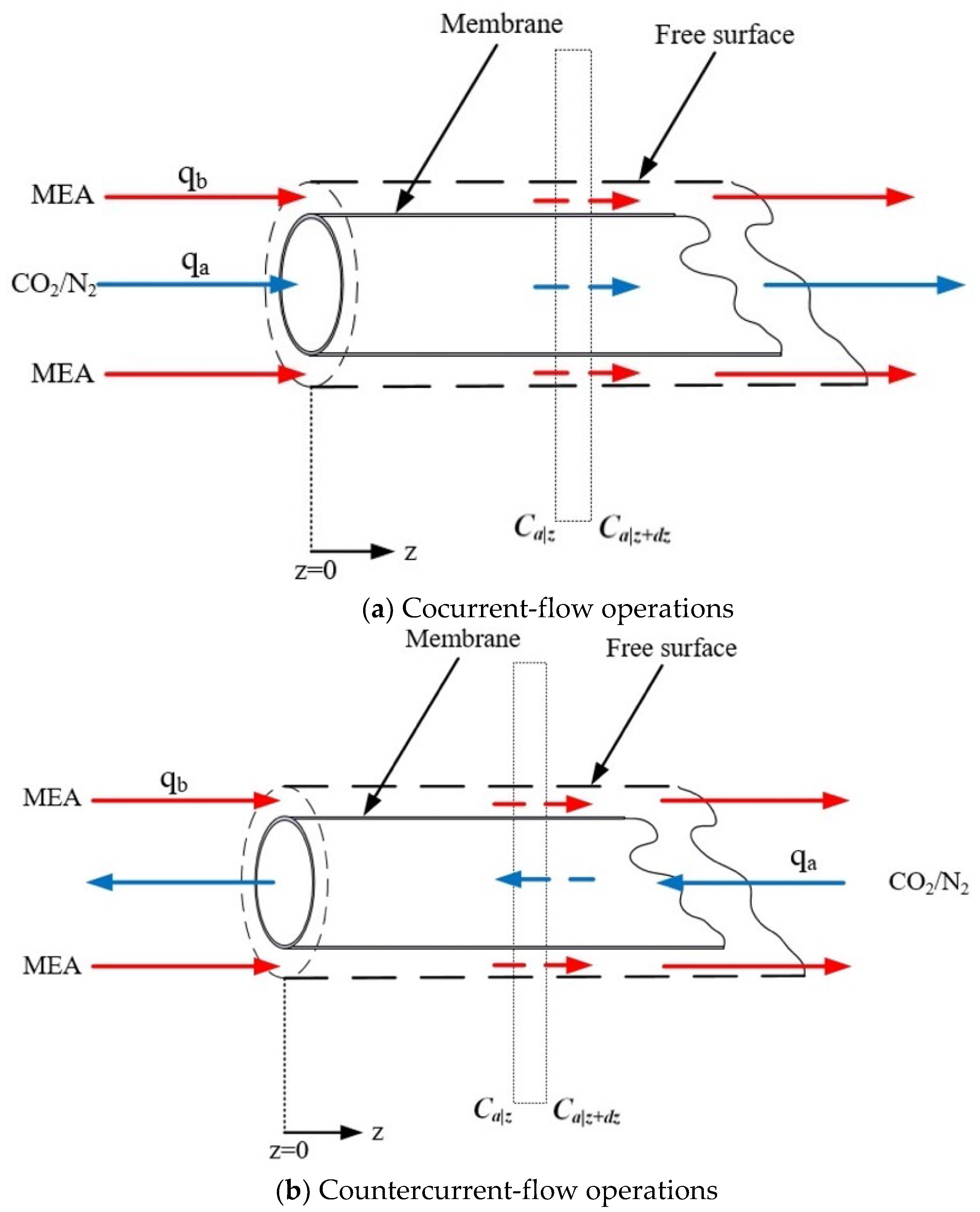



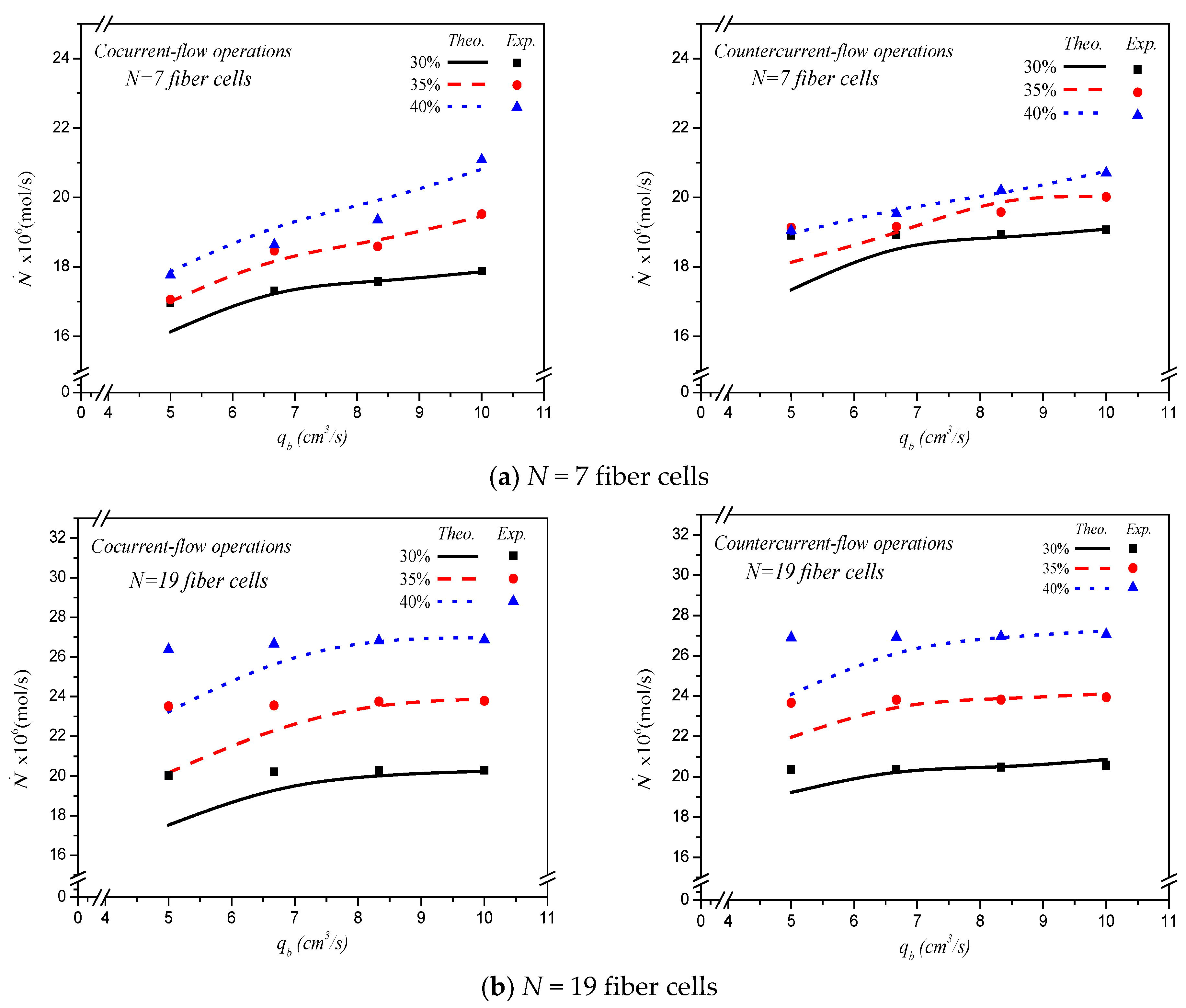


| Single-Fiber Cell | Multiple-Fiber Cells | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| N = 7 | N = 19 | ||||||||
| 30 | 5.00 | 2.47 | 4.84 | 16.13 | 4.94 | 553.1 | 19.23 | 5.53 | 609.9 |
| 6.67 | 3.05 | 1.55 | 17.41 | 0.65 | 471.1 | 20.43 | 0.29 | 540.1 | |
| 8.33 | 3.12 | 3.20 | 17.57 | 0.03 | 463.3 | 20.44 | 0.22 | 543.8 | |
| 10.0 | 3.29 | 9.42 | 17.86 | 0.1 | 442.8 | 20.87 | 1.42 | 515.4 | |
| 35 | 5.00 | 2.41 | 0.61 | 17.00 | 0.34 | 606.0 | 21.96 | 7.19 | 737.7 |
| 6.67 | 2.97 | 10.9 | 18.31 | 0.82 | 516.2 | 23.69 | 0.50 | 655.6 | |
| 8.33 | 2.99 | 1.44 | 18.72 | 0.71 | 527.1 | 23.86 | 0.19 | 694.3 | |
| 10.0 | 3.06 | 0.41 | 19.46 | 0.29 | 536.0 | 24.11 | 0.74 | 680.1 | |
| 40 | 5.00 | 2.26 | 1.53 | 17.87 | 0.59 | 692.3 | 24.08 | 10.5 | 930.5 |
| 6.67 | 2.83 | 0.96 | 19.25 | 3.28 | 580.3 | 26.42 | 1.88 | 815.0 | |
| 8.33 | 2.87 | 0.19 | 19.86 | 2.63 | 591.5 | 26.94 | 0.05 | 838.3 | |
| 10.0 | 2.91 | 0.86 | 20.81 | 1.31 | 614.6 | 27.24 | 0.69 | 826.1 | |
| Single-Fiber Cell | Multiple-Fiber Cells | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| N = 7 | N = 19 | ||||||||
| 30 | 5.00 | 2.47 | 4.84 | 17.34 | 8.27 | 602.0 | 19.23 | 5.53 | 678.6 |
| 6.67 | 3.05 | 1.55 | 18.51 | 2.13 | 506.9 | 20.43 | 0.29 | 569.9 | |
| 8.33 | 3.12 | 3.20 | 18.85 | 0.47 | 504.2 | 20.44 | 0.22 | 555.1 | |
| 10.0 | 3.29 | 9.42 | 19.08 | 0.09 | 480.0 | 20.87 | 1.42 | 534.4 | |
| 35 | 5.00 | 2.41 | 0.61 | 18.13 | 5.23 | 652.3 | 21.96 | 7.19 | 811.2 |
| 6.67 | 2.97 | 10.9 | 18.99 | 0.84 | 539.4 | 23.69 | 0.50 | 697.7 | |
| 8.33 | 2.99 | 1.44 | 19.86 | 1.47 | 564.2 | 23.86 | 0.19 | 698.0 | |
| 10.0 | 3.06 | 0.41 | 20.06 | 0.02 | 555.6 | 24.11 | 0.74 | 687.9 | |
| 40 | 5.00 | 2.26 | 1.53 | 18.95 | 0.46 | 738.5 | 24.08 | 10.5 | 965.5 |
| 6.67 | 2.83 | 0.96 | 19.63 | 0.50 | 593.7 | 26.42 | 1.88 | 833.6 | |
| 8.33 | 2.87 | 0.19 | 20.13 | 0.36 | 601.4 | 26.94 | 0.05 | 838.7 | |
| 10.0 | 2.91 | 0.86 | 20.76 | 0.23 | 613.4 | 27.24 | 0.69 | 836.1 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ho, C.-D.; Chang, H.; Chen, Y.-H.; Chew, T.L.; Ke, J.-W. Investigation on the Performance of CO2 Absorption in Ceramic Hollow-Fiber Gas/Liquid Membrane Contactors. Membranes 2023, 13, 249. https://doi.org/10.3390/membranes13020249
Ho C-D, Chang H, Chen Y-H, Chew TL, Ke J-W. Investigation on the Performance of CO2 Absorption in Ceramic Hollow-Fiber Gas/Liquid Membrane Contactors. Membranes. 2023; 13(2):249. https://doi.org/10.3390/membranes13020249
Chicago/Turabian StyleHo, Chii-Dong, Hsuan Chang, Yu-Han Chen, Thiam Leng Chew, and Jui-Wei Ke. 2023. "Investigation on the Performance of CO2 Absorption in Ceramic Hollow-Fiber Gas/Liquid Membrane Contactors" Membranes 13, no. 2: 249. https://doi.org/10.3390/membranes13020249
APA StyleHo, C.-D., Chang, H., Chen, Y.-H., Chew, T. L., & Ke, J.-W. (2023). Investigation on the Performance of CO2 Absorption in Ceramic Hollow-Fiber Gas/Liquid Membrane Contactors. Membranes, 13(2), 249. https://doi.org/10.3390/membranes13020249








