Numerical Simulation of Membrane Separation Characteristics of Supercritical Carbon Dioxide and Water
Abstract
:1. Introduction
2. Numerical Simulation of Membrane Absorption and Separation Process
2.1. Design of Membrane Separator
2.2. Numerical Model
2.2.1. Single-Phase Porous Medium
2.2.2. The Porous Medium Model
2.2.3. Mathematical Model of Water Absorption
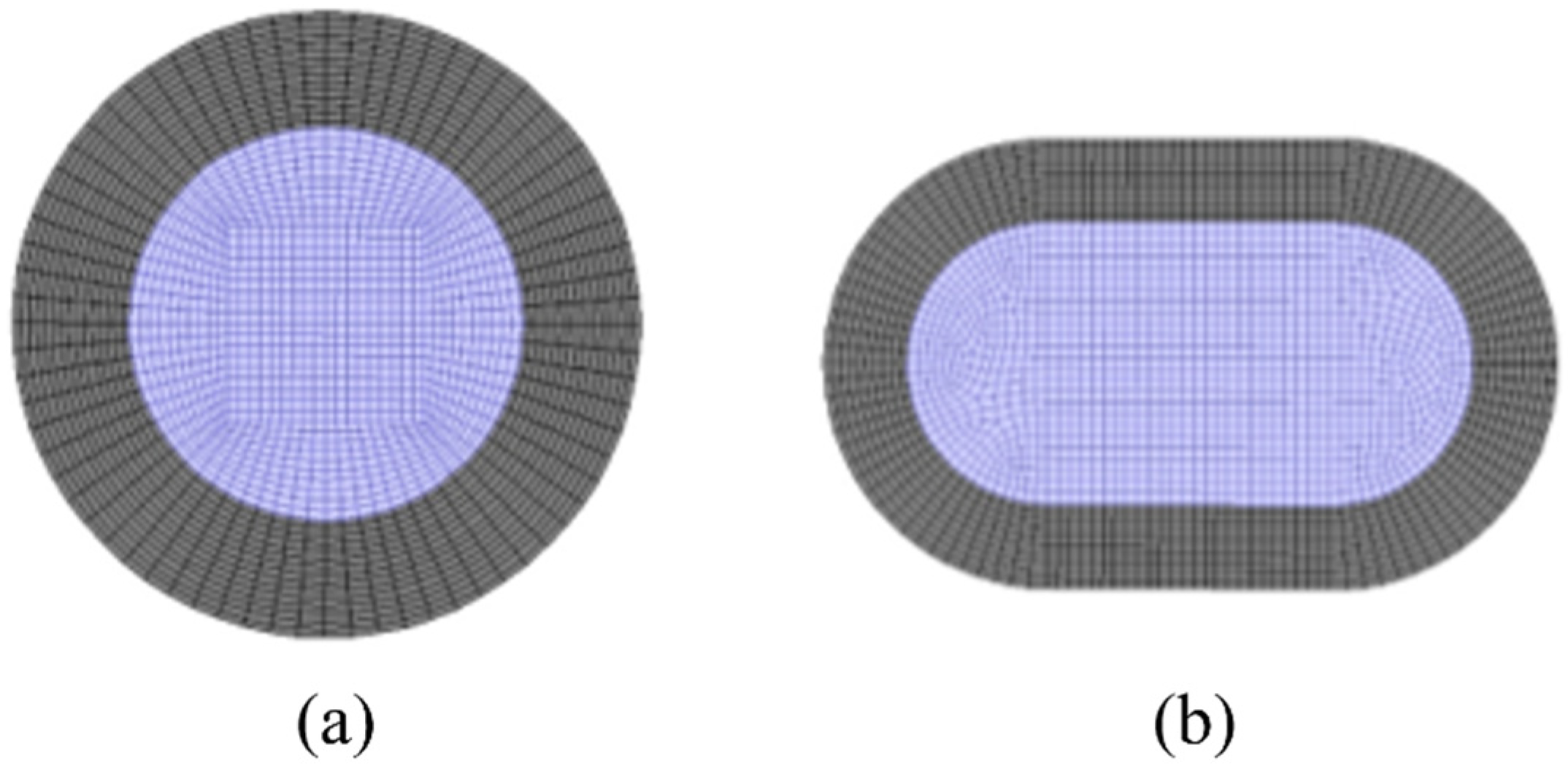
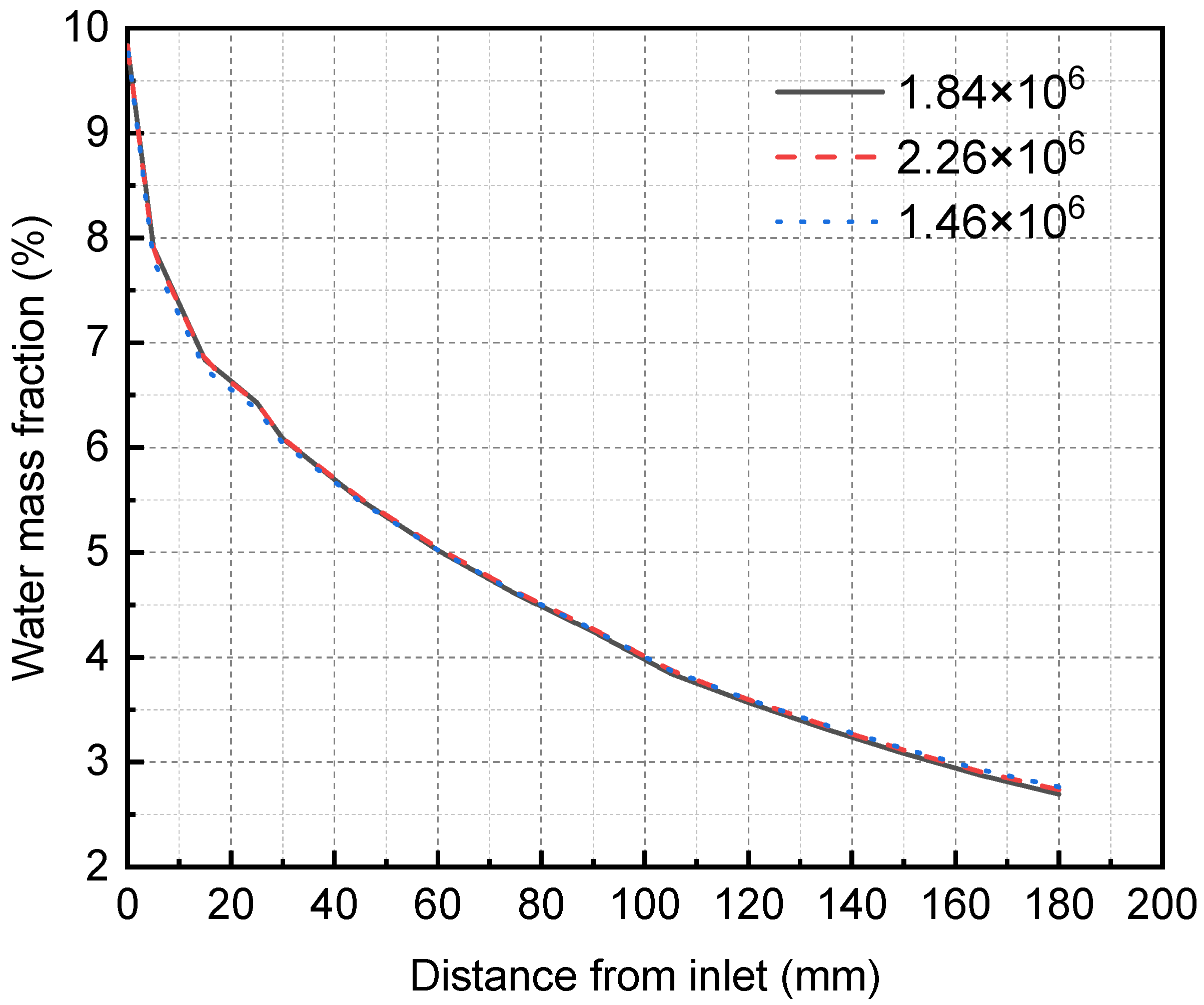
2.3. Grid Division and Boundary Conditions
2.3.1. Simulation of Membrane Tube Structure Dimensions
2.3.2. Grid Division of Membrane Separator
2.3.3. Boundary Conditions
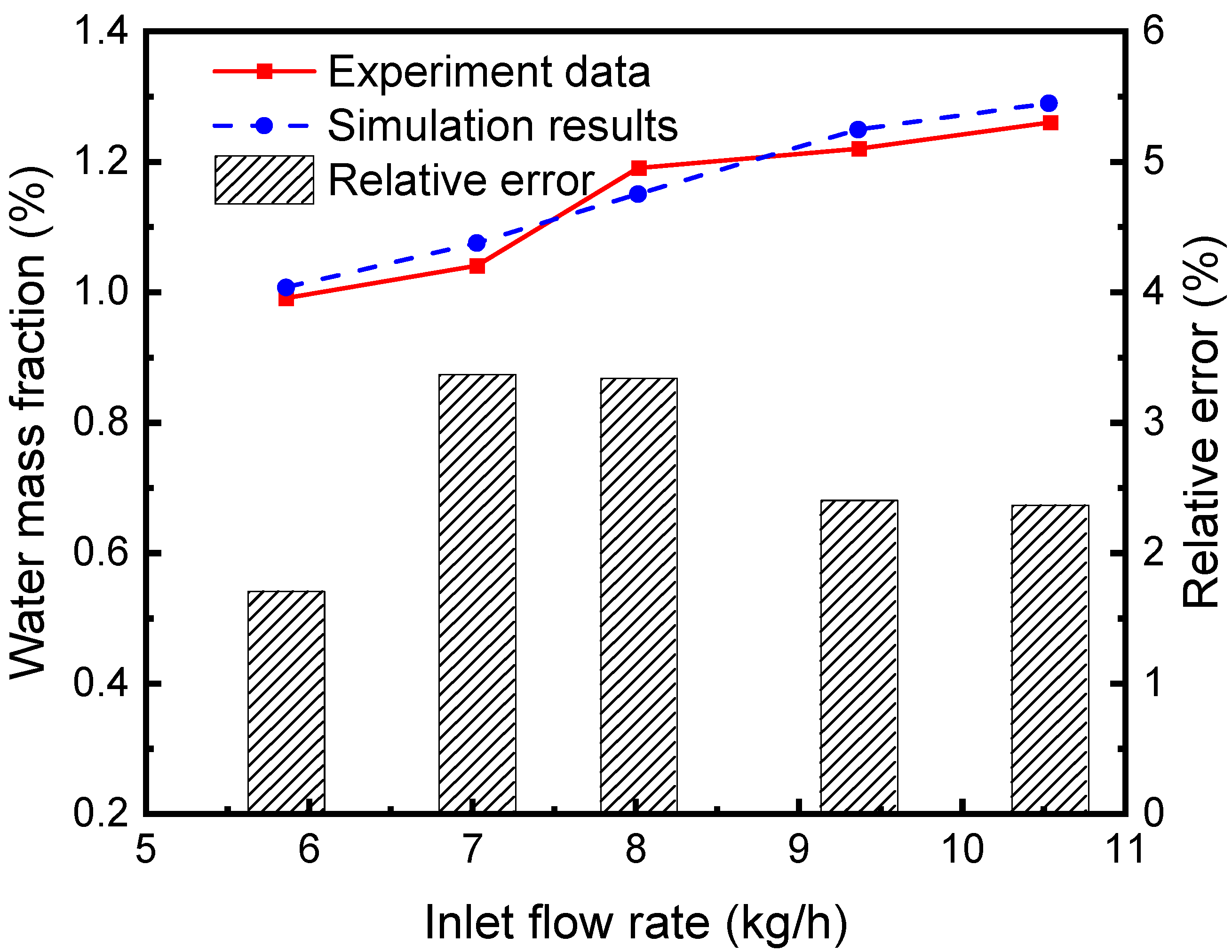
2.4. Model Validation
3. Simulation Results and Analysis
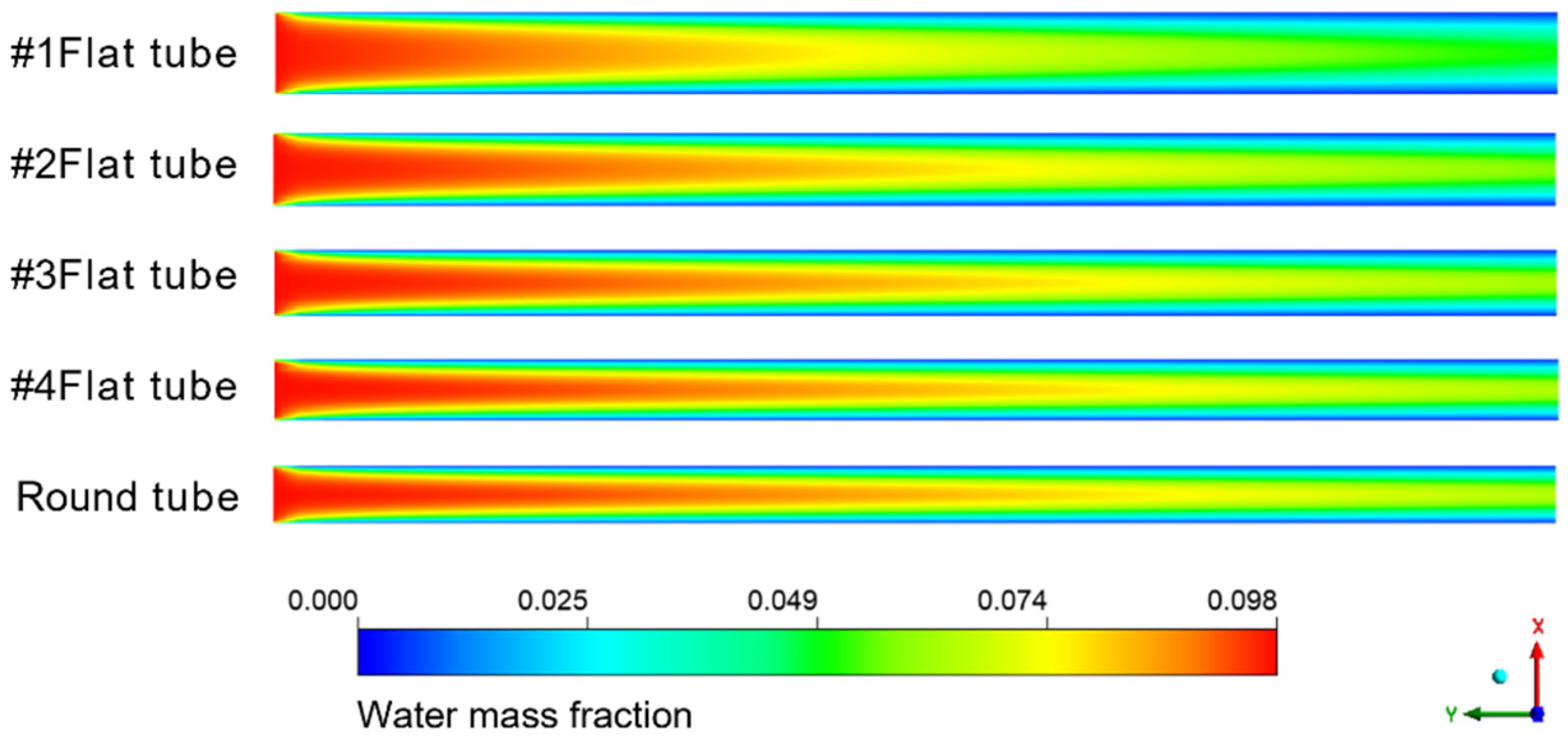
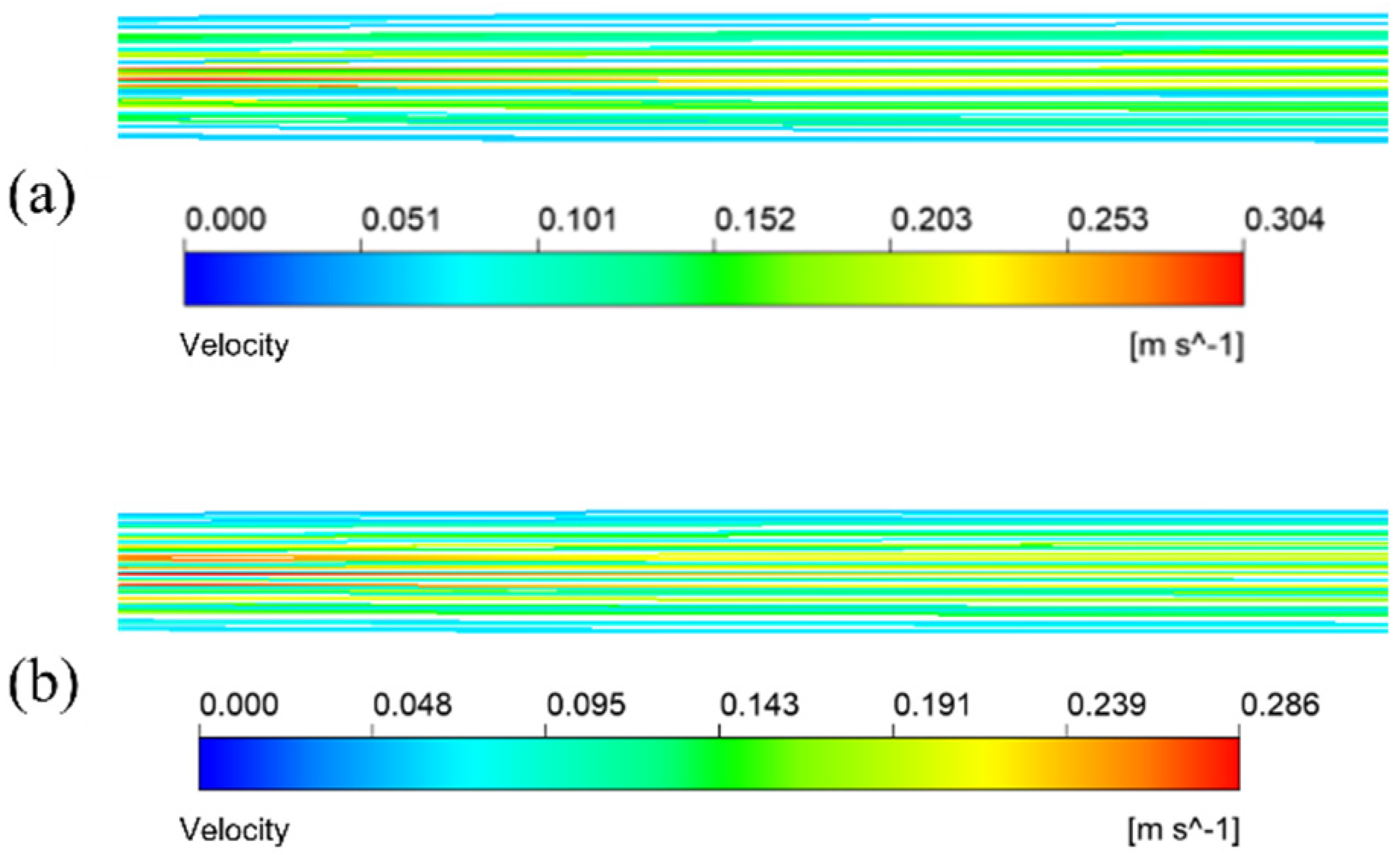
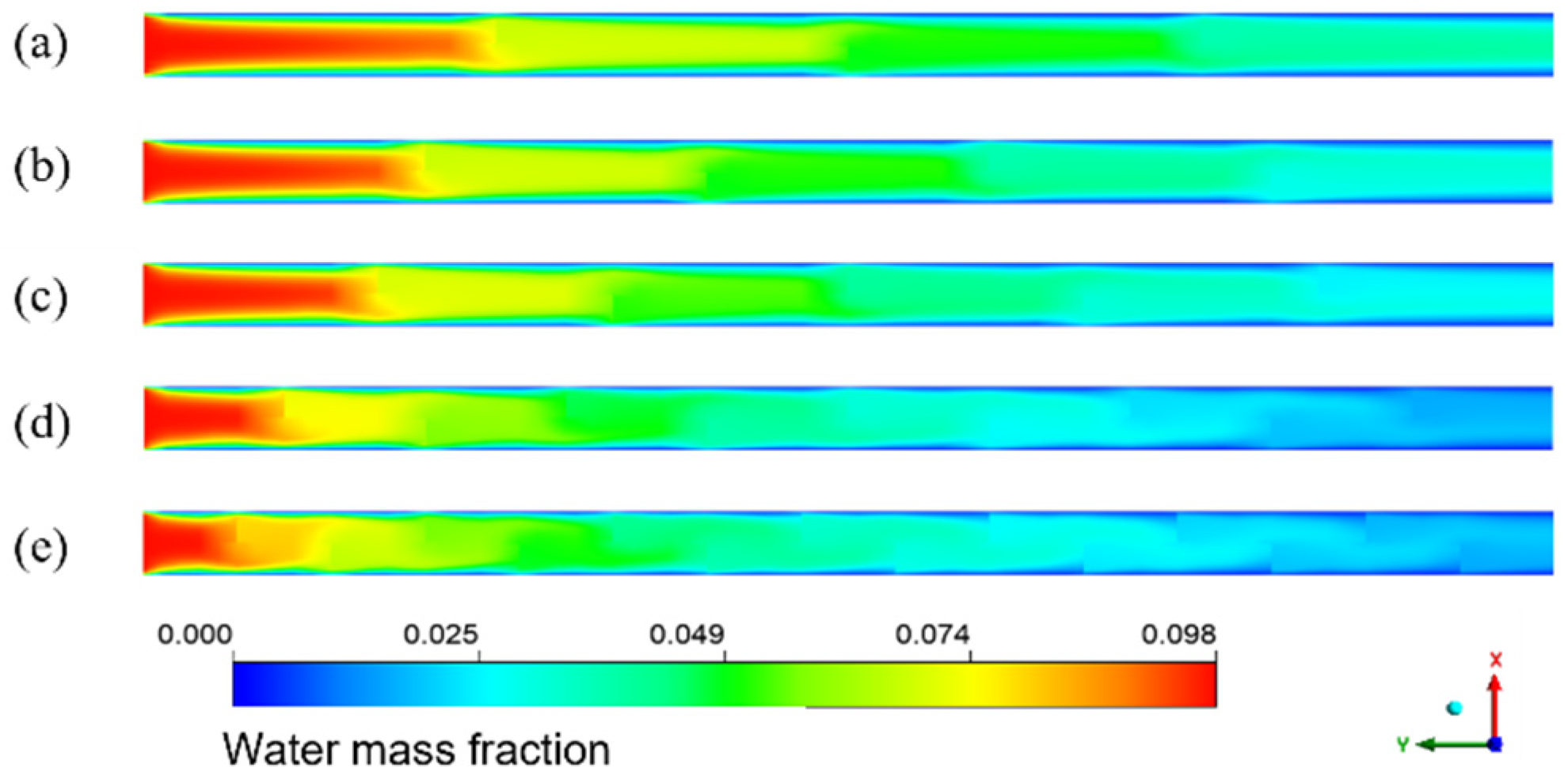
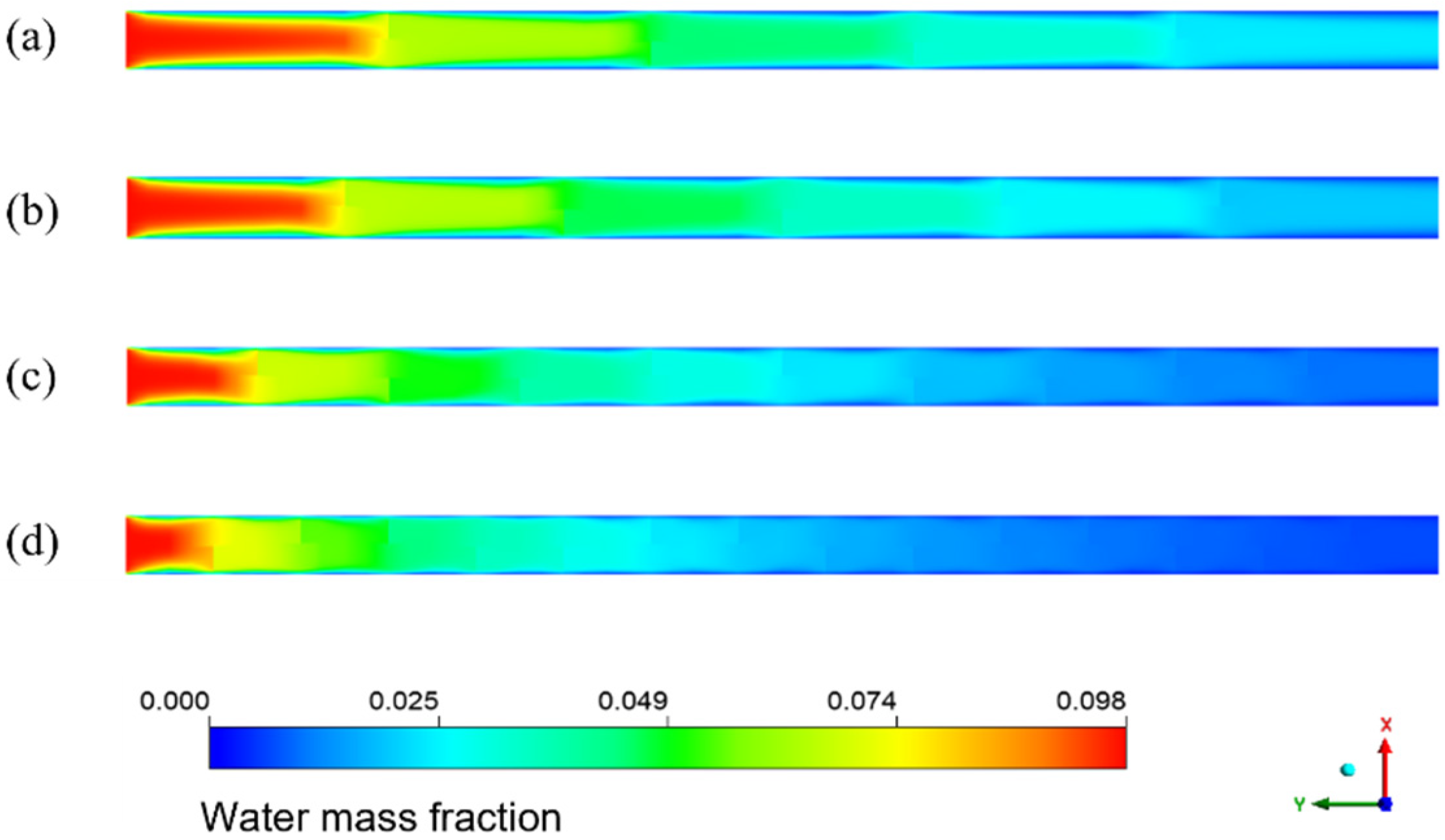
3.1. Flow Field Analysis
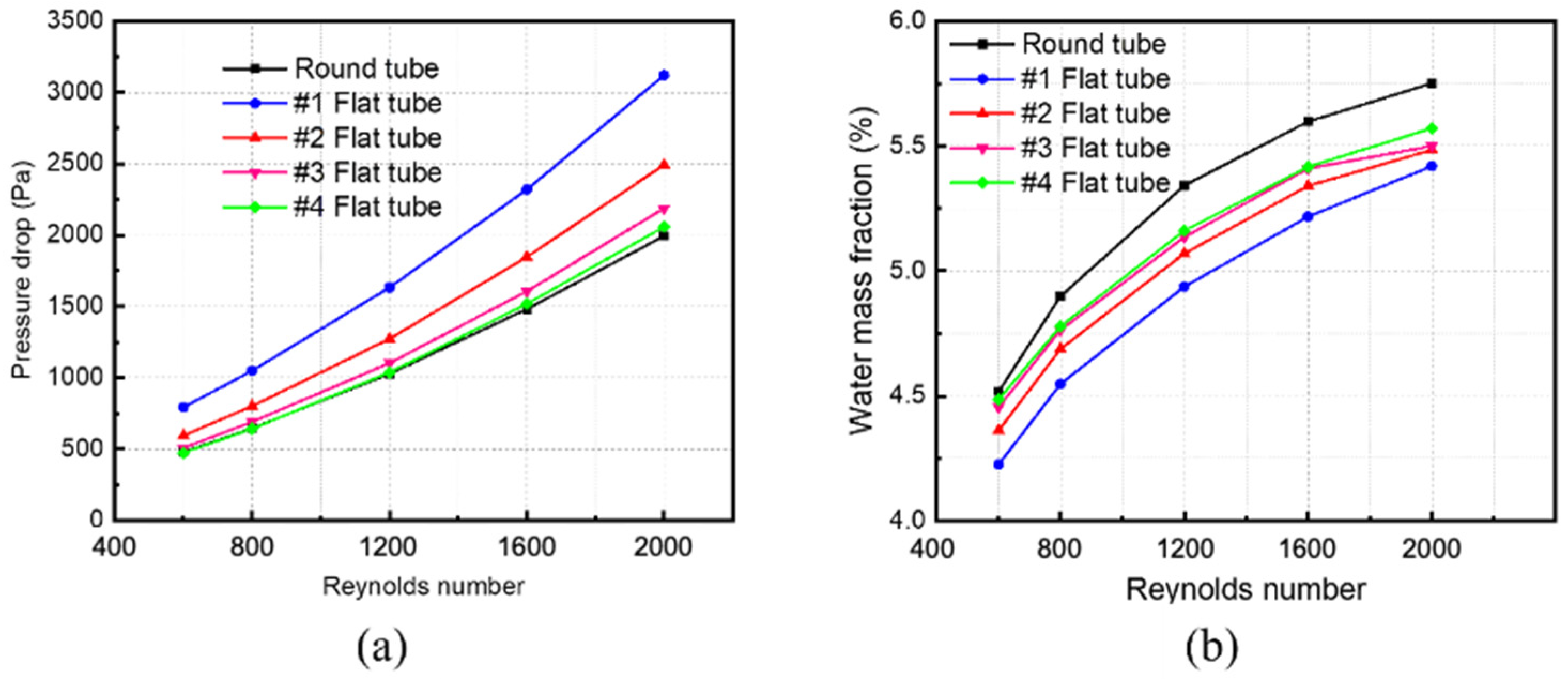
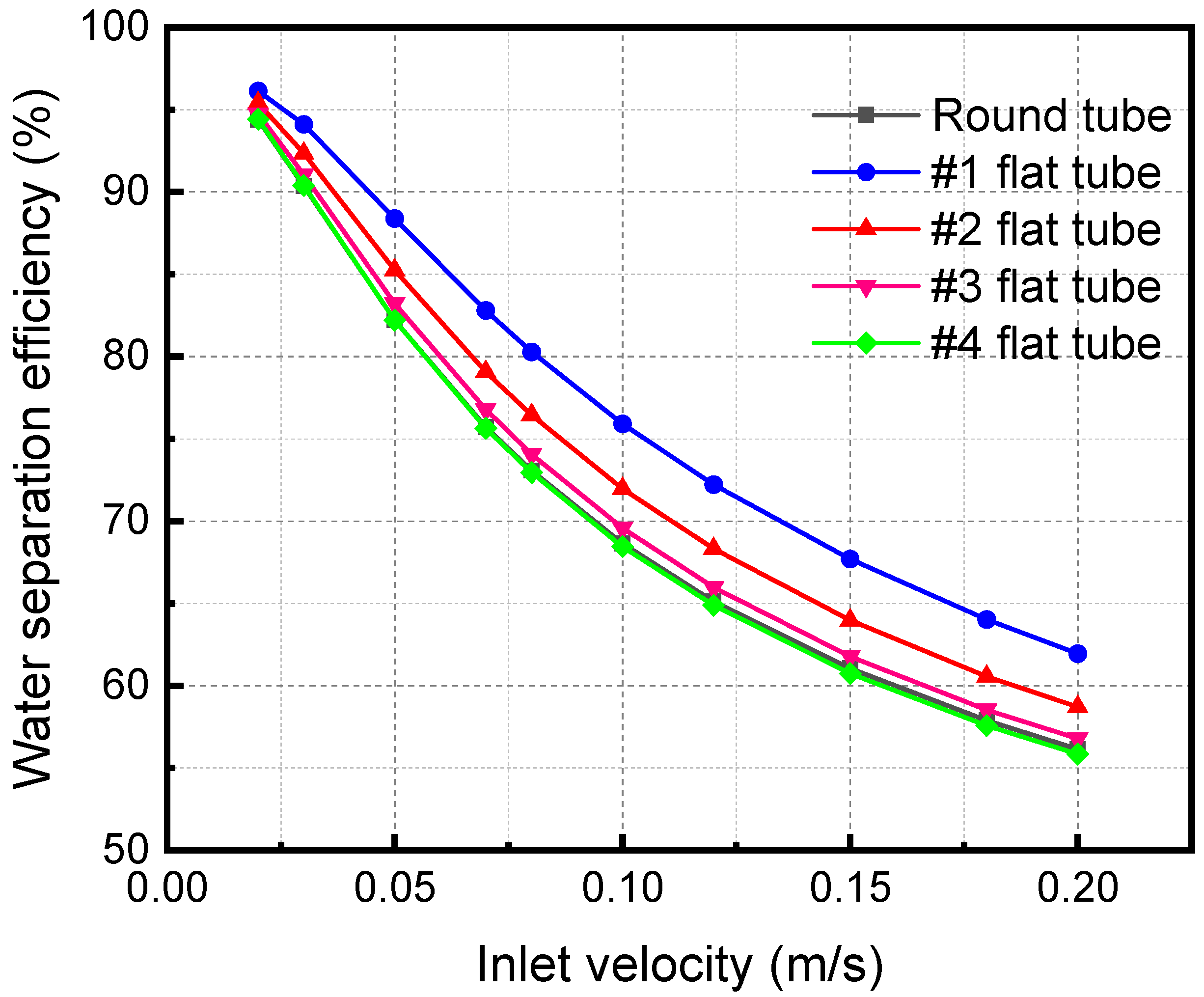
3.2. The Influence of Reynolds Number on Separation Efficiency
3.3. Effect of Mixture Inlet Velocity
3.4. The Influence of Membrane Tube Length
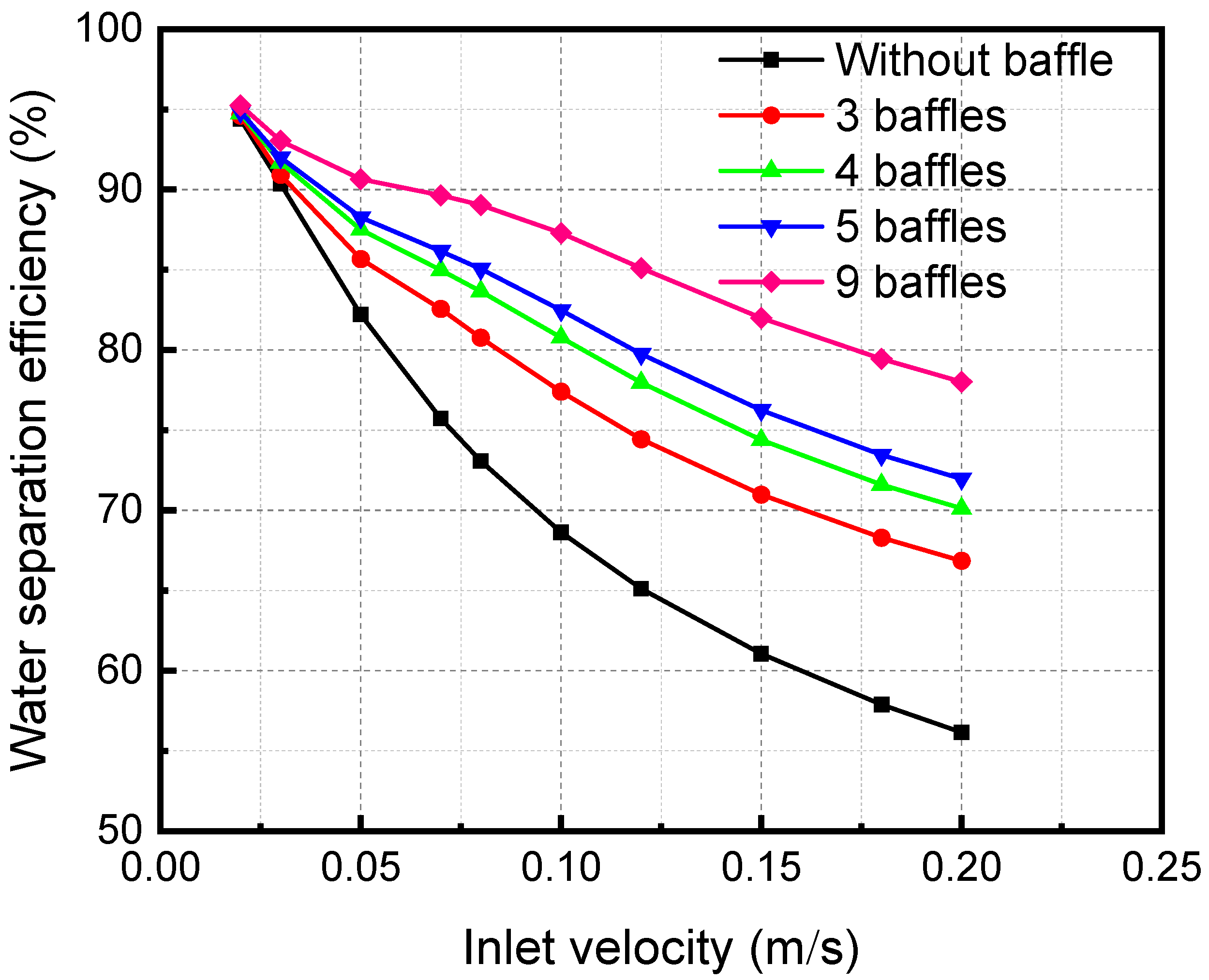
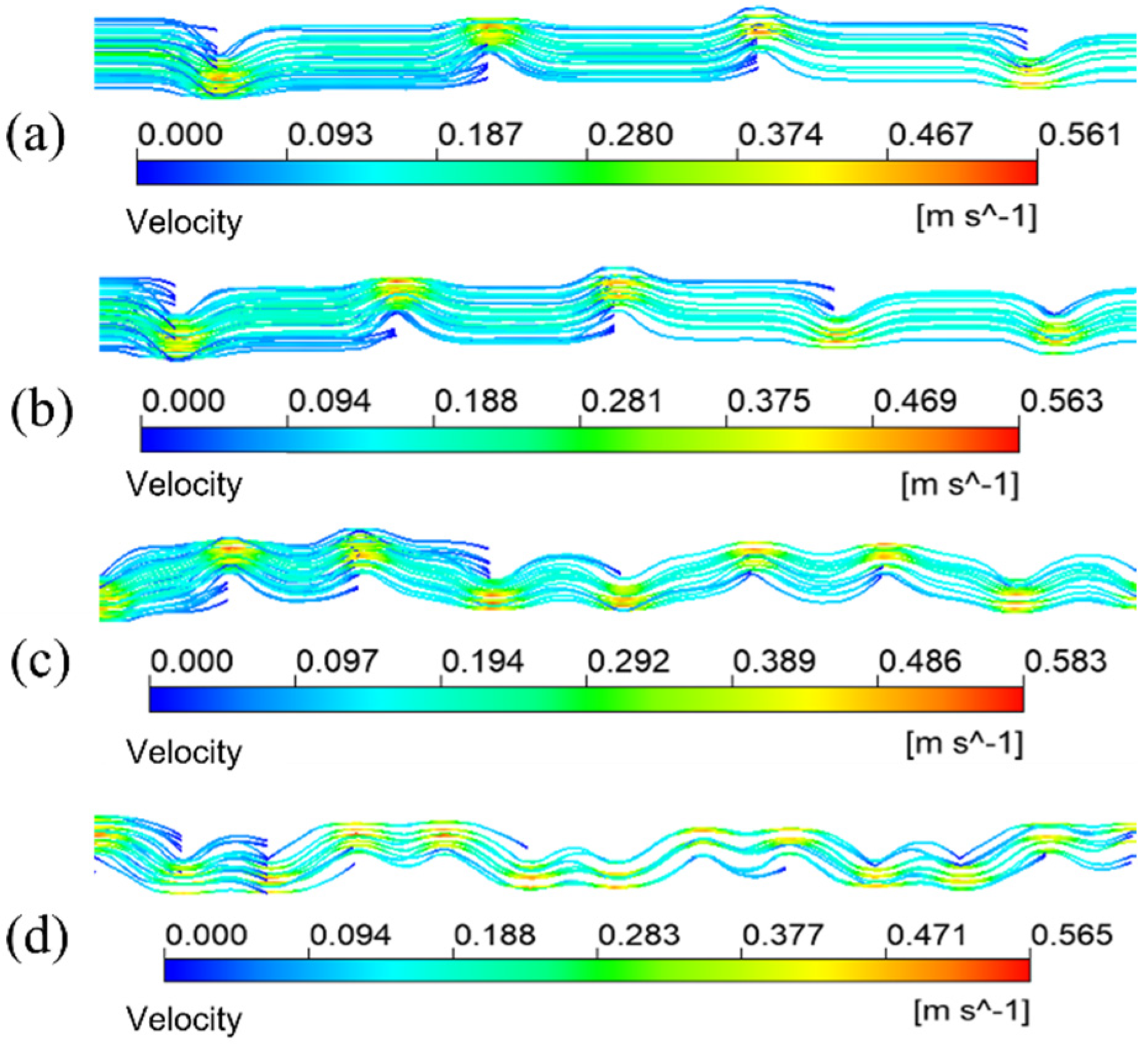
4. Numerical Simulation of Separation Efficiency with Bow Baffle
4.1. The Influence of Bow Baffle
4.2. Influence of Spiral Bow Baffle
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Dubey, A.; Arora, A. Advancements in carbon capture technologies: A review. J. Clean. Prod. 2022, 373, 133932. [Google Scholar] [CrossRef]
- Owebor, K.; Diemuodeke, E.O.; Briggs, T.A. Thermo-economic and environmental analysis of integrated power plant with carbon capture and storage technology. Energy 2022, 240, 122748. [Google Scholar] [CrossRef]
- Nogara, J.; Zarrouk, S.J. Corrosion in geothermal environment: Part 1: Fluids and their impact. Renew. Sust. Energ. Rev. 2017, 82, 1333–1346. [Google Scholar] [CrossRef]
- Qiao, Z.; Tang, Y.; Zhang, L.; Pan, C.; Romero, C.E.; Wang, X.; Charles, J.; Si, F.; Maya, C.R. Design and performance analysis of a supercritical CO2 (sCO2)-water separator for power generation systems using hot sCO2 from geothermal reservoirs. Geothermics 2019, 81, 123–132. [Google Scholar] [CrossRef]
- Qiao, Z.; Tang, Y.; Zhang, L.; Pan, C.; Romero, C.E.; Wang, X.; Charles, J.; Si, F.; Maya, C.R. Performance analysis and optimization design of an axial-flow vane separator for supercritical CO2 (sCO2)-water mixtures from geothermal reservoirs. Int. J. Energy Res. 2019, 43, 2327–2342. [Google Scholar] [CrossRef]
- Wang, Z.; Zhou, Q.; Guo, H.; Yang, P.; Lu, W. Determination of water solubility in supercritical CO2 from 313.15 to 473.15K and from 10 to 50MPa by in-situ quantitative Raman spectroscopy. Fluid Phase Equilib. 2018, 476, 170–178. [Google Scholar] [CrossRef]
- Pan, L.; Freifeld, B.; Doughty, C.; Zakem, S.; Sheu, M.; Cutright, B.; Terrall, T. Fully coupled wellbore-reservoir modeling of geothermal heat extraction using CO2 as the working fluid. Geothermics 2015, 52, 100–113. [Google Scholar] [CrossRef]
- Ravanchi, M.T.; Kaghazchi, T.; Kargari, A. Application of membrane separation processes in petrochemical industry: A review. Desalination 2009, 235, 199–244. [Google Scholar] [CrossRef]
- Aneke, M.; Wang, M. Potential for improving the energy efficiency of cryogenic air separation unit (ASU) using binary heat recovery cycles. Appl. Therm. Eng. 2015, 81, 223–231. [Google Scholar] [CrossRef]
- Aghel, B.; Heidaryan, E.; Sahraie, S.; Nazari, M. Optimization of monoethanolamine for CO2 absorption in a microchannel reactor. J. CO2 Util. 2018, 28, 264–273. [Google Scholar] [CrossRef]
- Shamu, A.; Miedema, H.; Metz, S.J.; Borneman, Z.; Nijmeijer, K. Mass transfer studies on the dehydration of supercritical carbon dioxide using dense polymeric membranes. Sep. Purif. Technol. 2019, 209, 229–237. [Google Scholar] [CrossRef]
- Qiao, Z.; Cao, Y.; Tang, Y.; Si, F. Numerical analysis of membrane–absorption separation for supercritical carbon dioxide and water mixture of plume geothermal power generation systems. Energy Conv. Manag. 2020, 208, 112609. [Google Scholar] [CrossRef]
- Chua, H.; Toh, H.; Malek, A.; Ng, K.; Srinivasan, K. Improved thermodynamic property fields of LiBr-H2O solutions. Int. J. Refrig. 2000, 23, 412–429. [Google Scholar] [CrossRef]
- Rajabzadeh, S.; Yoshimoto, S.; Teramoto, M.; Al-Marzouqi, M.; Matsuyama, H. CO2 absorption by using PVDF hollow fiber membrane contactors with various membrane structures. Sep. Purif. Technol. 2009, 69, 210–220. [Google Scholar] [CrossRef]
- Li, J.; Chen, B. Review of CO2 absorption using chemical solvents in hollow fiber membrane contactor. Sep. Purif. Technol. 2005, 41, 109–122. [Google Scholar] [CrossRef]
- Ouyang, Y.; Zhang, L. Conjugate heat and mass transfer in a skewed flow hollow fiber membrane bank used for liquid desiccant air dehumidification. Int. J. Heat Mass Transf. 2016, 93, 23–40. [Google Scholar] [CrossRef]
- Yoshimune, M.; Haraya, K. CO2/CH4 mixed gas separation using carbon hollow fiber membranes. Energy Procedia 2013, 37, 1109–1116. [Google Scholar] [CrossRef]
- Moulin, P.; Rouch, J.; Serra, C.; Clifton, M.; Aptel, P. Mass transfer improvement by secondary flows: Dean vortices in coiled tubular membranes. J. Membr. Sci. 1996, 114, 235–244. [Google Scholar] [CrossRef]
- Wu, S.; Lin, Y.; Hwang, K.; Cheng, T.; Tung, K. High-efficiency hollow fiber arrangement design to enhance filtration performance by CFD simulation. Chem. Eng. Process. 2018, 125, 87–96. [Google Scholar] [CrossRef]
- Quek, V.; Shah, N.; Chachuat, B. Modeling for design and operation of high-pressure membrane contactors in natural gas sweetening. Chem. Eng. Res. Des. 2018, 132, 1005–1019. [Google Scholar] [CrossRef]
- Kang, G.; Chan, Z.; Saleh, S.M.; Cao, Y. Removal of high concentration CO2 from natural gas using high pressure membrane contactors. Int. J. Greenh. Gas Control 2017, 60, 1–9. [Google Scholar] [CrossRef]
- Li, Z.; Tsotsis, T. Methanol synthesis in a high-pressure membrane reactor with liquid sweep. J. Membr. Sci. 2019, 570–571, 103–111. [Google Scholar] [CrossRef]
- Mansourizadeh, A.; Ismail, A. Hollow fiber gas-liquid membrane contactors for acid gas capture: A review. J. Hazard. Mater. 2009, 171, 38–53. [Google Scholar] [CrossRef]
- Lim, K.; Wang, P.; An, H.; Yu, S. Computational Studies for the Design Parameters of Hollow Fibre Membrane Module. J. Membr. Sci. 2017, 529, 263–273. [Google Scholar] [CrossRef]
- Ma, C.; Liu, Y.; Li, F.; Shen, C.; Huang, M.; Wang, Z.; Cao, C.; Zhou, Q.; Sheng, Y.; Sand, W. CFD simulations of fiber-fiber interaction in a hollow fiber membrane bundle: Fiber distance and position matters. Sep. Purif. Technol. 2018, 209, 707–713. [Google Scholar] [CrossRef]
- Wotzka, A.; Jorabchi, M.; Wohlrab, S. Separation of H2O/CO2 Mixtures by MFI Membranes: Experiment and Monte Carlo Study. Membranes 2021, 11, 439. [Google Scholar] [CrossRef]
- Higashi, Y. NIST Thermodynamic and Transport Properties of Refrigerants and Refrigerant Mixtures (REFPROP). Netsu Bussei 2000, 14, 1575–1577. [Google Scholar]
- Conde, M.R. Properties of aqueous solutions of lithium and calcium chlorides: Formulations for use in air conditioning equipment design. Int. J. Therm. Sci. 2004, 43, 367–382. [Google Scholar] [CrossRef]
- Levy, E.; Wang, X.; Pan, C.; Romero, C.E.; Maya, C.R. Use of hot supercritical CO2 produced from a geothermal reservoir to generate electric power in a gas turbine power generation system. J. CO2 Util. 2018, 23, 20–28. [Google Scholar] [CrossRef]
- Zhang, L.; Huang, S.; Chi, J.; Pei, L. Conjugate heat and mass transfer in a hollow fiber membrane module for liquid desiccant air dehumidification: A free surface model approach. Int. J. Heat Mass Transf. 2012, 55, 3789–3799. [Google Scholar] [CrossRef]







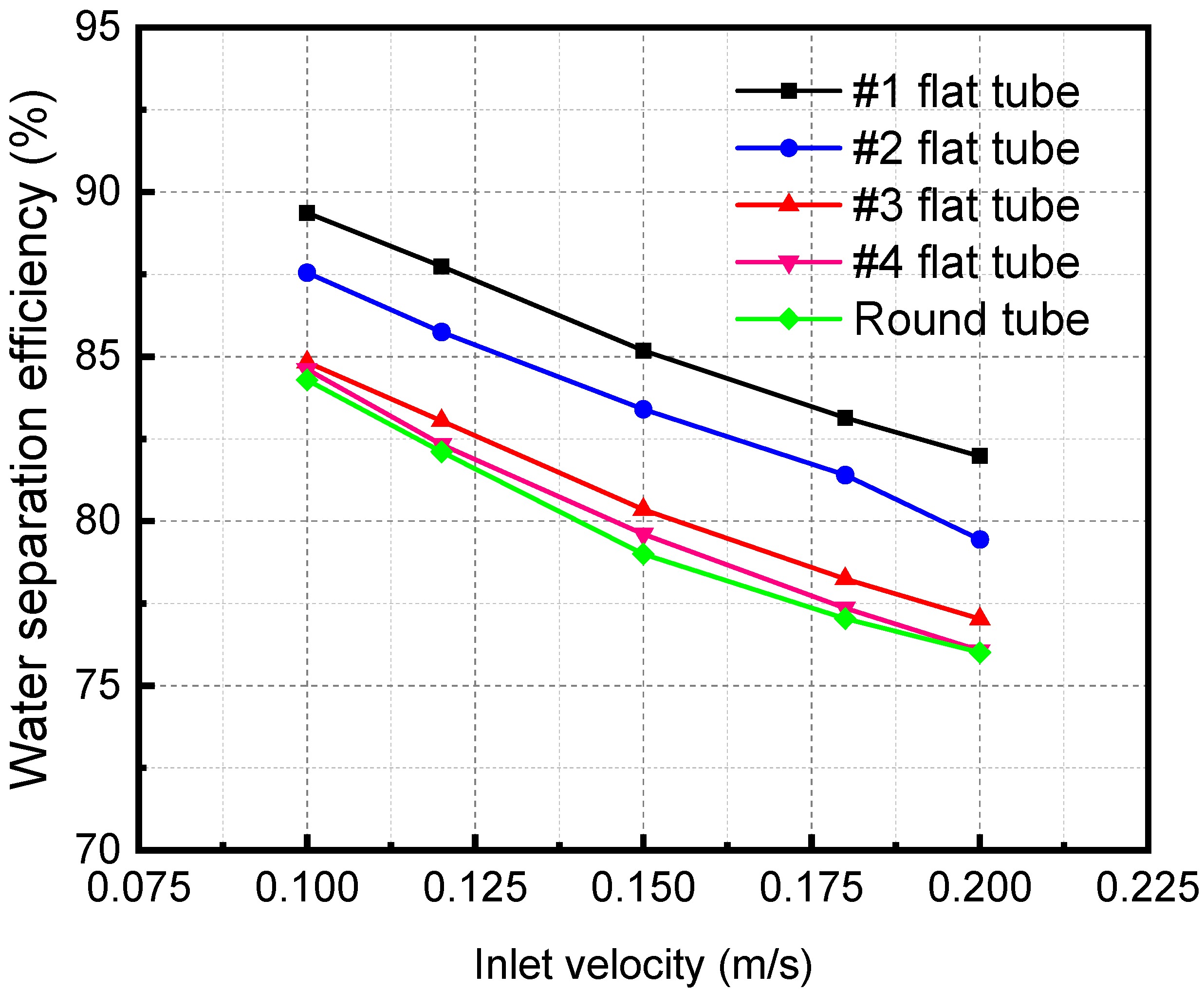
| Name | Round Tube | #1 Flat Tube | #2 Flat Tube | #3 Flat Tube | #4 Flat Tube |
|---|---|---|---|---|---|
| Length, l/mm | 180 | 180 | 180 | 180 | 180 |
| Width, w/mm | 1.60 | 2.05 | 1.88 | 1.76 | 1.67 |
| Height, h/mm | 1.60 | 1.20 | 1.30 | 1.40 | 1.50 |
| Flatness, e/h | 1.00 | 1.71 | 1.45 | 1.26 | 1.11 |
| Hydraulic diameter, d/mm | 1.00 | 0.88 | 0.94 | 0.98 | 1.00 |
| Film thickness, σ/mm | 0.3 | 0.3 | 0.3 | 0.3 | 0.3 |
| Circulating sectional area, S/mm2 | 0.79 | 0.79 | 0.79 | 0.79 | 0.79 |
| Parameter Name | Numerical Value |
|---|---|
| Mixture inlet temperature, Tm,i/°C | 160 |
| Mixture inlet pressure, pm,i/MPa | 20 |
| LiBr solution inlet temperature, Ts,i/°C | 160 |
| LiBr solution inlet pressure, ps,i/MPa | 20 |
| LiBr solution inlet concentration, Xs,i/wt% | 65 |
| SCO2 mass fraction in the mixture, Xs,c/wt% | 90.17 |
| Water mass fraction in the mixture, Xw/wt% | 9.83 |
| Water diffusion coefficient in sCO2, Dw,sc/(m2/s) | 7.69 × 10−8 |
| Water diffusion coefficient in LiBr solution, Dw,s/(m2/s) | 3 × 10−9 |
| LiBr solution dynamic viscosity, μs/(Pa∙s) | 5 × 10−3 |
| LiBr solution density, ρs/(kg/m3) | 1638 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qiao, Z.; Pan, Y.; Tang, Y.; Cao, Y.; Si, F. Numerical Simulation of Membrane Separation Characteristics of Supercritical Carbon Dioxide and Water. Membranes 2023, 13, 892. https://doi.org/10.3390/membranes13120892
Qiao Z, Pan Y, Tang Y, Cao Y, Si F. Numerical Simulation of Membrane Separation Characteristics of Supercritical Carbon Dioxide and Water. Membranes. 2023; 13(12):892. https://doi.org/10.3390/membranes13120892
Chicago/Turabian StyleQiao, Zongliang, Yue Pan, Youfei Tang, Yue Cao, and Fengqi Si. 2023. "Numerical Simulation of Membrane Separation Characteristics of Supercritical Carbon Dioxide and Water" Membranes 13, no. 12: 892. https://doi.org/10.3390/membranes13120892
APA StyleQiao, Z., Pan, Y., Tang, Y., Cao, Y., & Si, F. (2023). Numerical Simulation of Membrane Separation Characteristics of Supercritical Carbon Dioxide and Water. Membranes, 13(12), 892. https://doi.org/10.3390/membranes13120892







