Performance Comparison of Alternative Hollow-Fiber Modules for Hemodialysis by Means of a CFD-Based Model
Abstract
1. Introduction
2. Materials and Methods
2.1. Computational Model
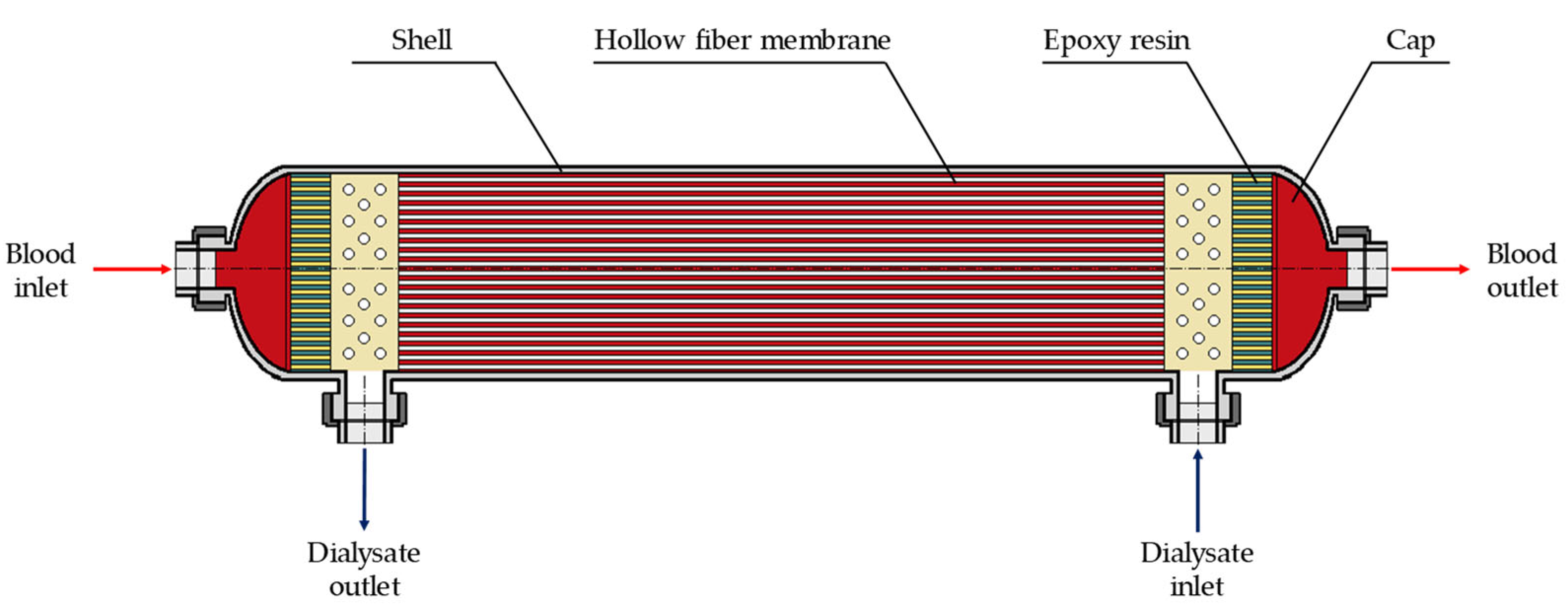
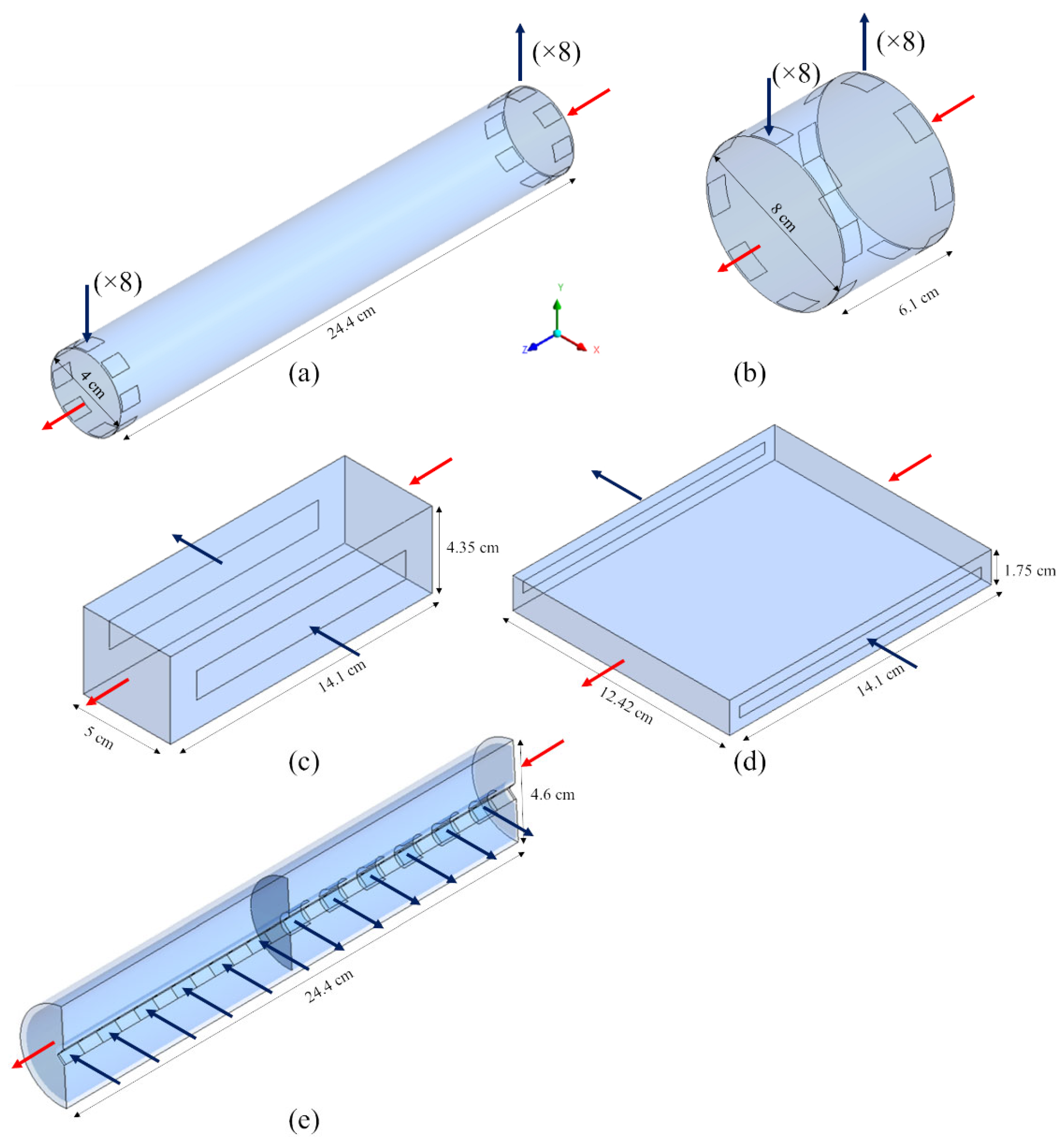
2.2. Geometries Investigated
2.3. Computational Grids
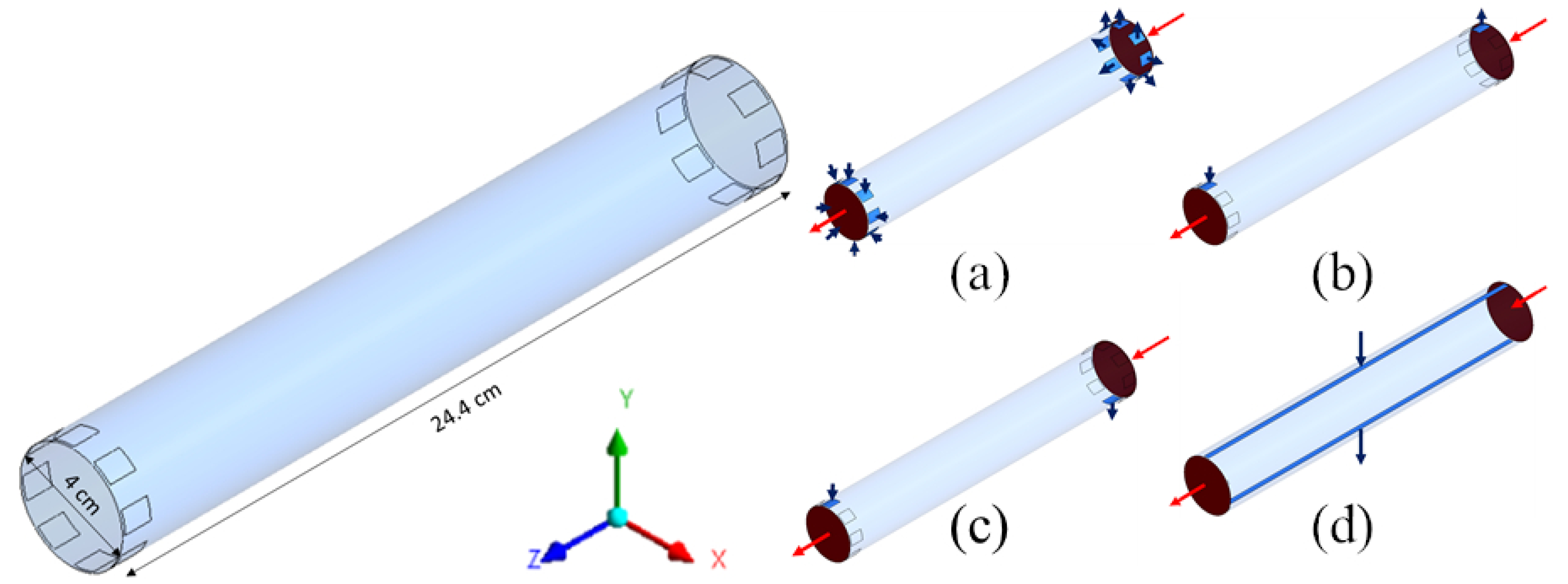
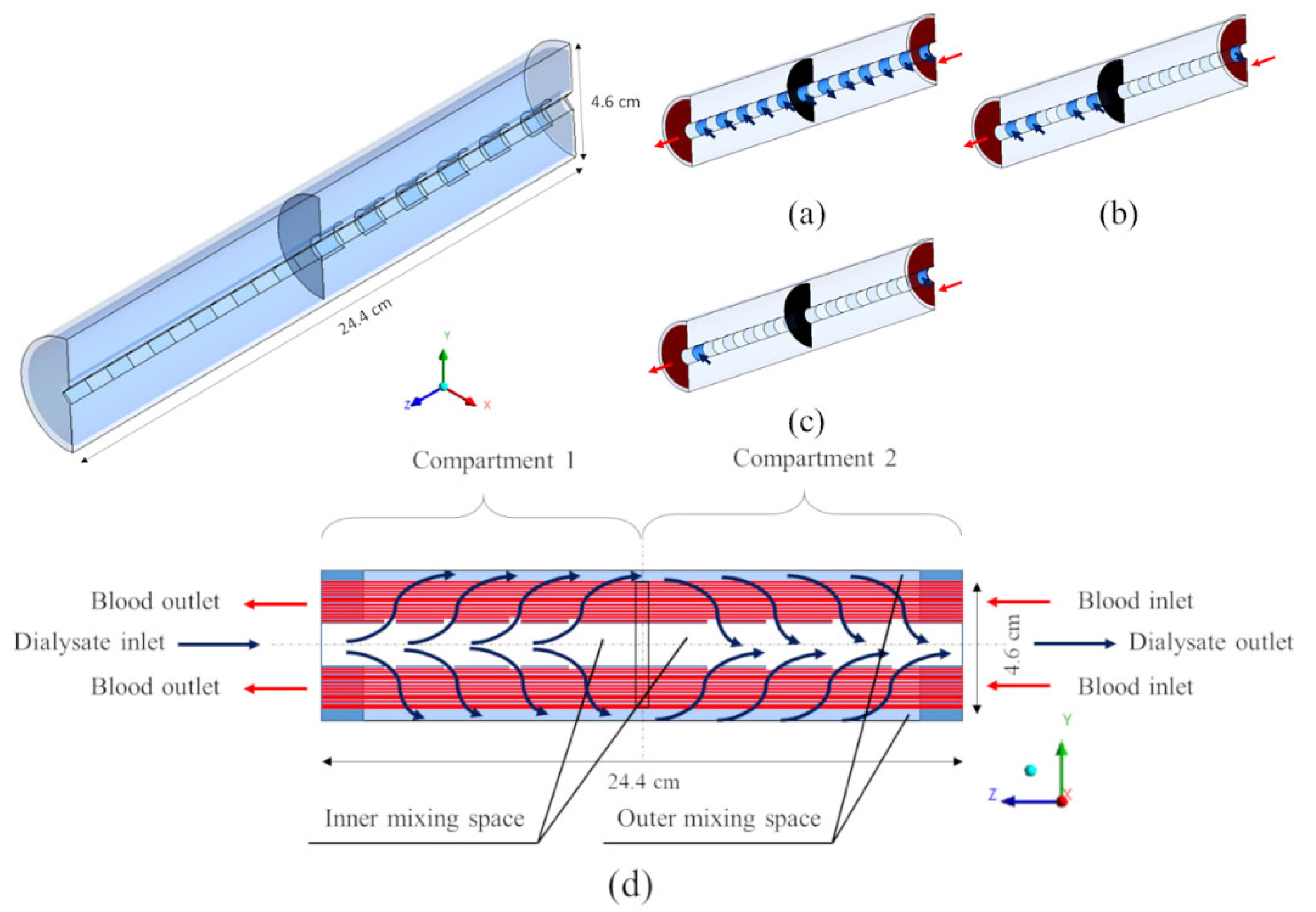
2.4. Inlet–Outlet Configurations
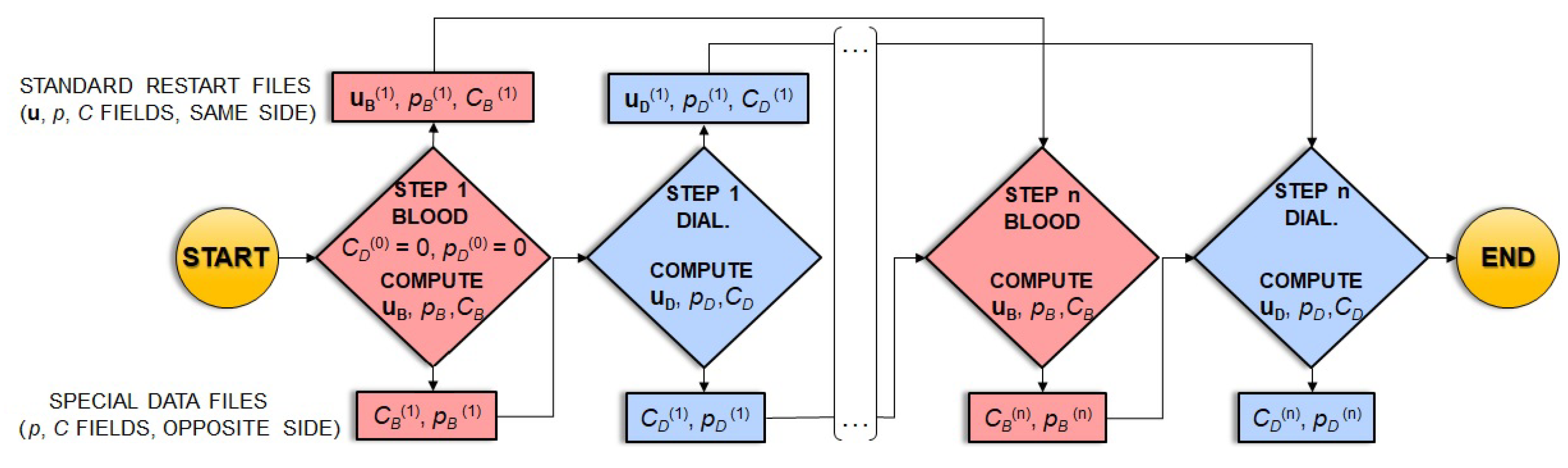
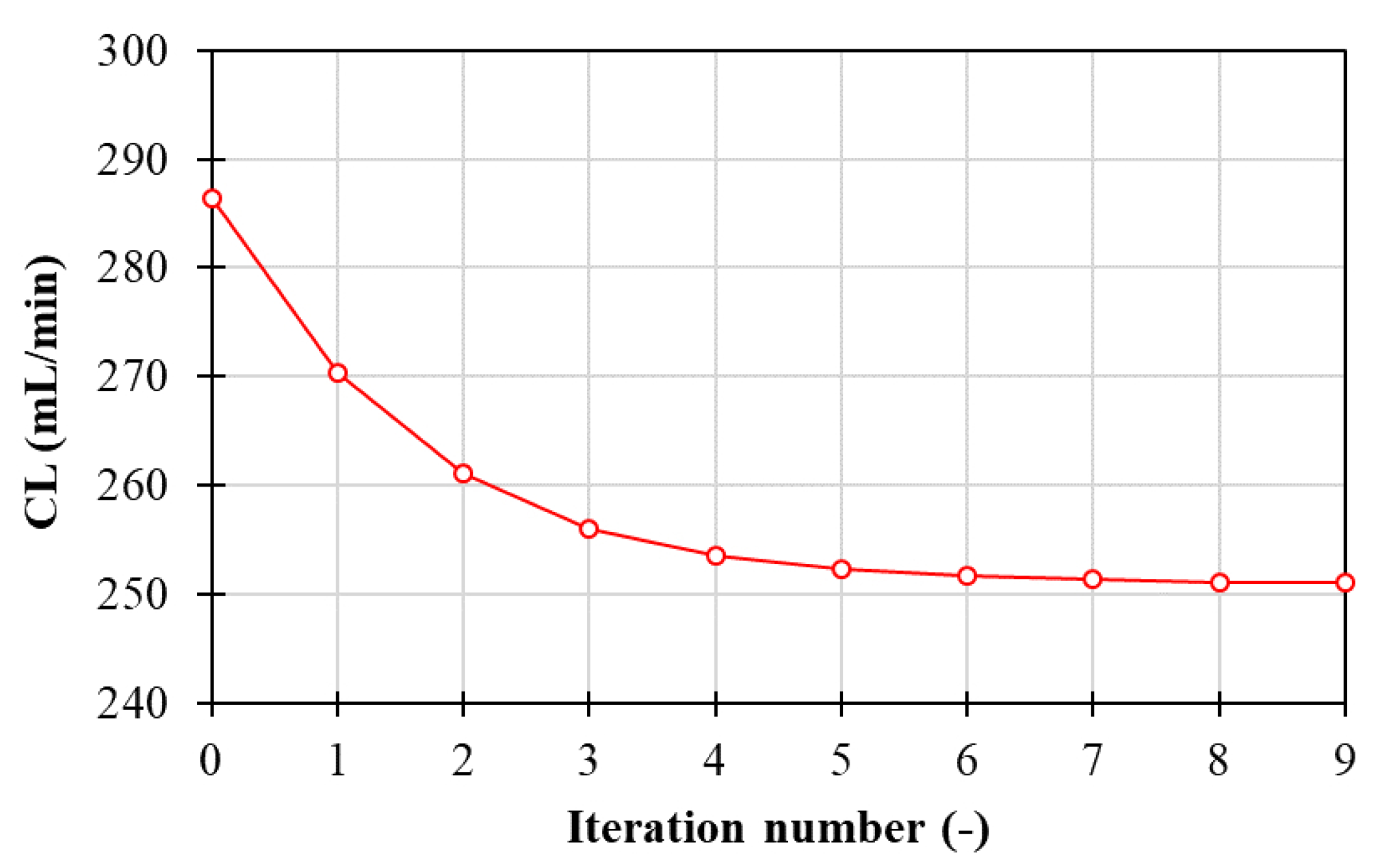
2.5. Simulation Strategy
3. Results and Discussion
3.1. Model Validation
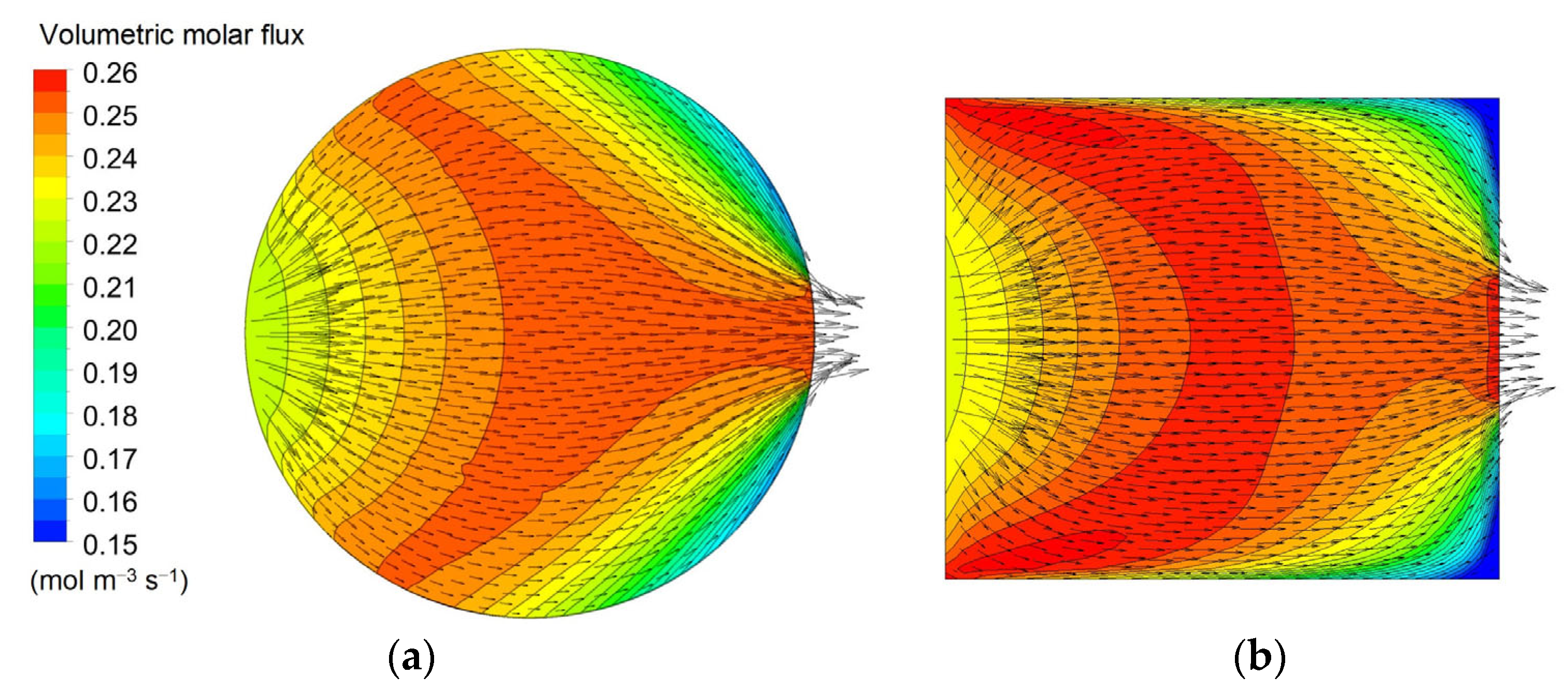
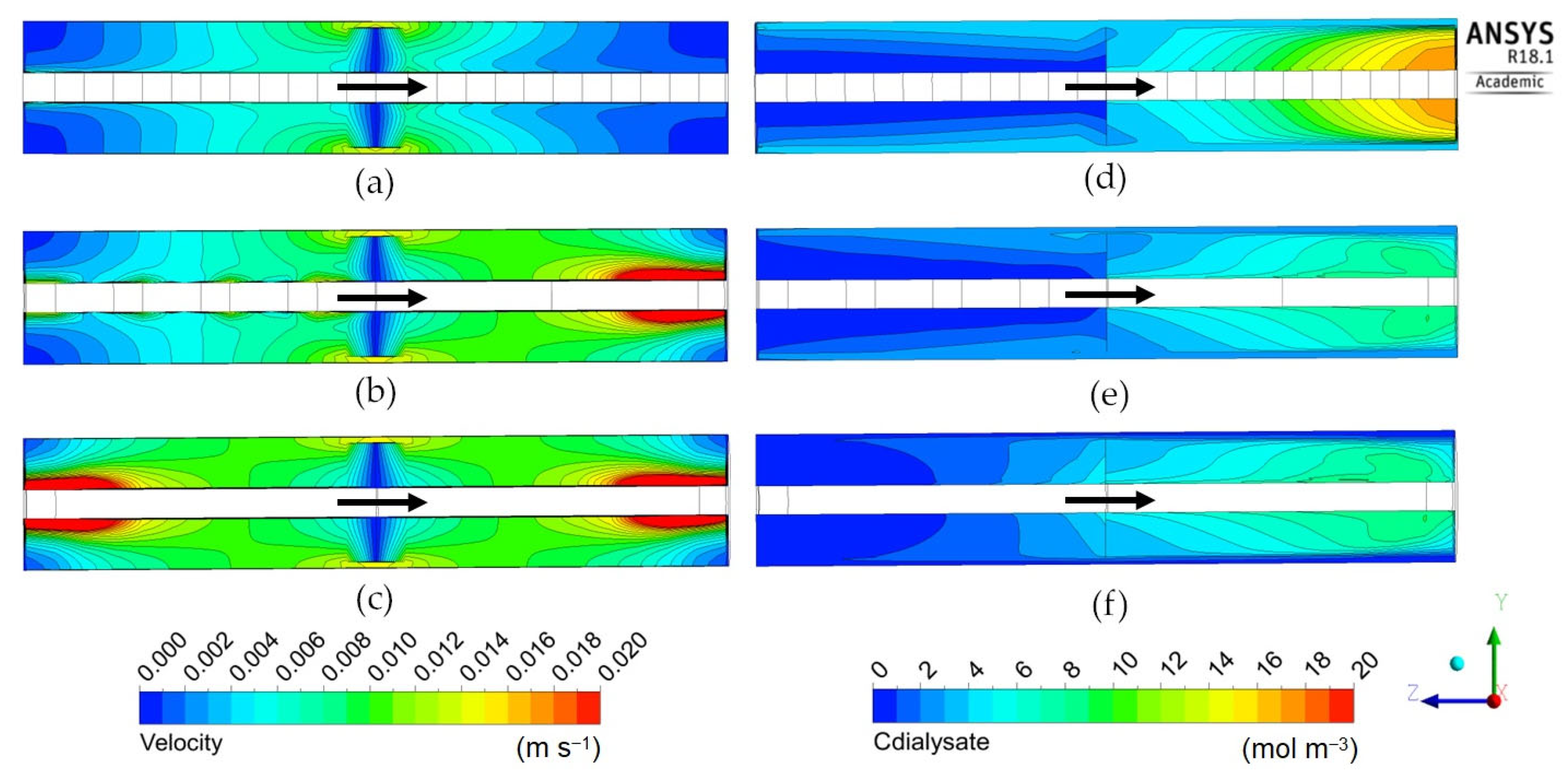
3.2. Comparison of Model Predictions
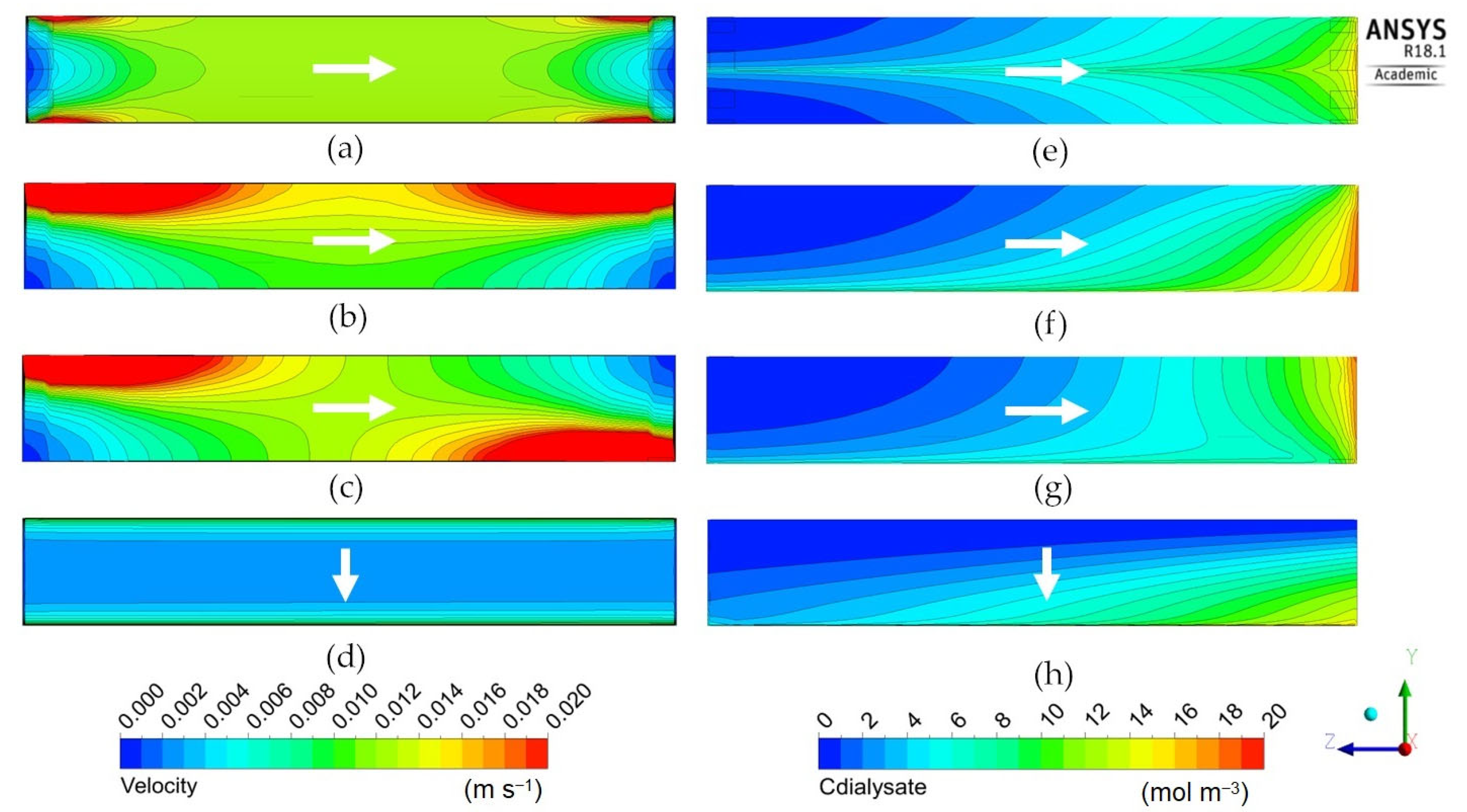
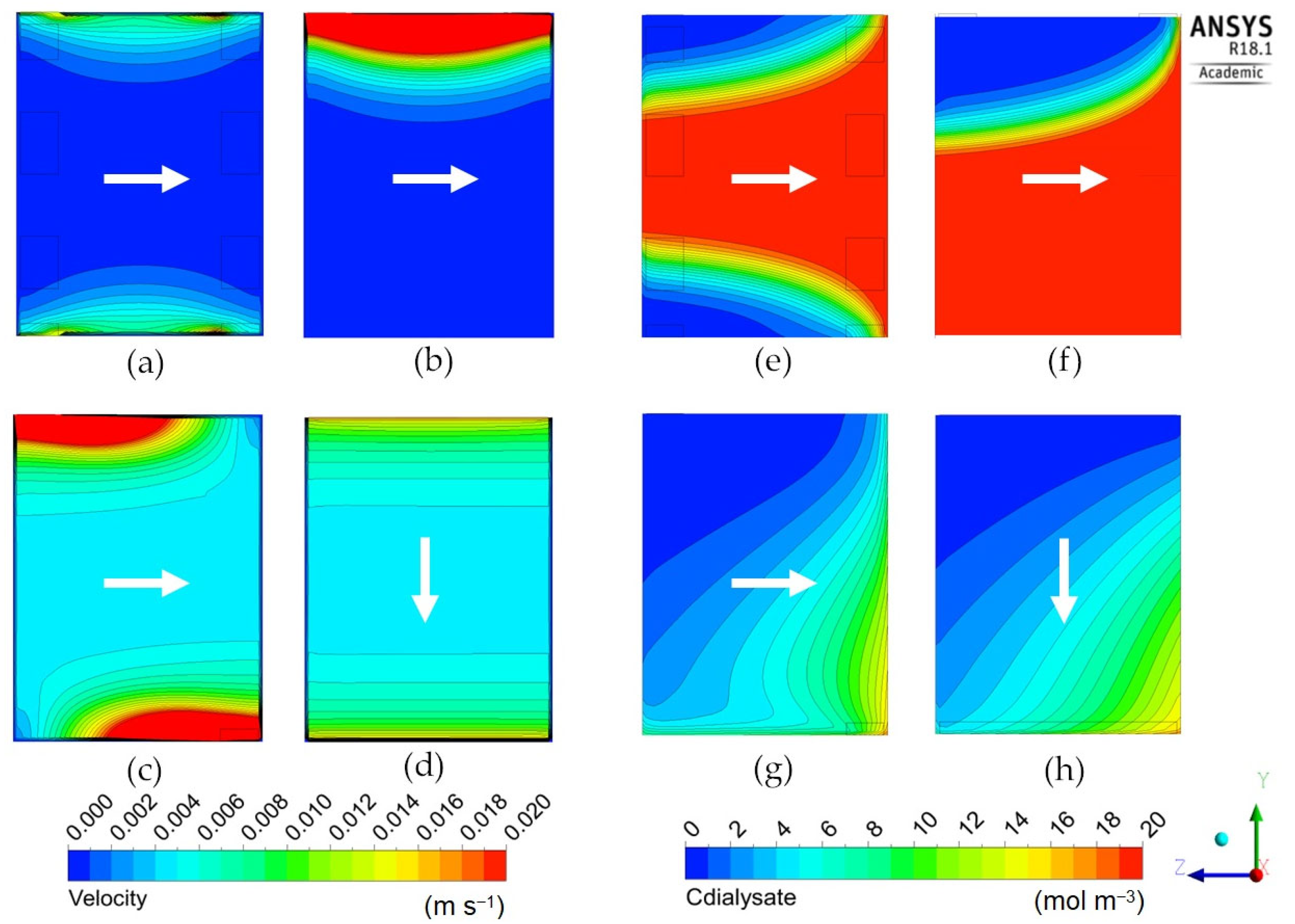
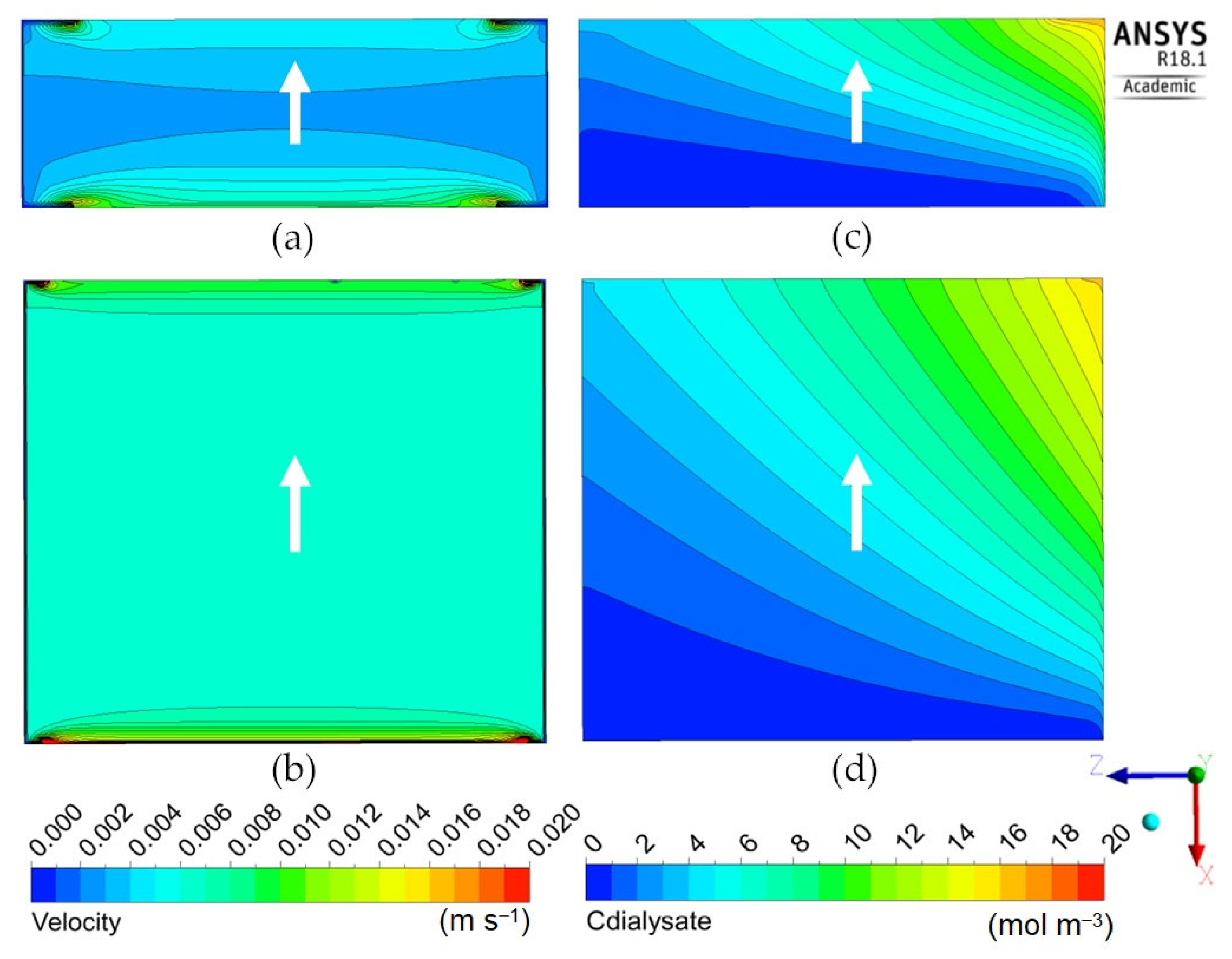
3.3. Dialysate Velocity and Solute Concentration Distributions
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Misra, M. Basic mechanisms governing solute and fluid transport in hemodialysis. Hemodial. Int. 2008, 12, 25–28. [Google Scholar] [CrossRef]
- Sam, R. Hemodialysis: Diffusion and Ultrafiltration. Austin J. Nephrol. Hypertens. 2014, 1, 1010. [Google Scholar]
- Ronco, C.; Fabris, A.; Feriani, M. Hemodialysis Fluid Composition. In Replacement of Renal Function by Dialysis; Jacobs, C., Kjellstrand, C.M., Koch, K.M., Winchester, J.F., Eds.; Springer: Dordrecht, The Netherlands, 1996; pp. 256–276. [Google Scholar]
- Ronco, C.; Clark, W.R. Haemodialysis membranes. Nat. Rev. Nephrol. 2018, 14, 394–410. [Google Scholar] [CrossRef] [PubMed]
- Azar, A.T.; Canaud, B. Hemodialysis System. In Modeling and Control of Dialysis Systems; Azar, A.T., Ed.; Springer: Berlin/Heidelberg, Germany, 2013; pp. 144–147. [Google Scholar]
- Ronco, C.; Ghezzi, P.M.; Brendolan, A.; Crepaldi, C.; La Greca, G. The haemodialysis system: Basic mechanisms of water and solute transport in extracorporeal renal replacement therapies. Nephrol. Dial. Transplant. 1998, 13, 3–9. [Google Scholar] [CrossRef]
- Ross, S.M.; Uvelli, D.A.; Babb, A.L. One-Dimensional Mathematical Model of Transmembrane Diffusional and Convective Mass Transfer in a Hemodialyzer. Am. Soc. Mech. Eng. 1974, 96, 63–64. [Google Scholar]
- Villarroel, F.; Klein, E.; Holland, F. Solute flux in hemodialysis and hemofiltration membranes. Trans. Am. Soc. Artif. Intern. Organs 1977, 23, 225–232. [Google Scholar] [CrossRef] [PubMed]
- Sargent, J.A.; Gotch, F.A. Principles and Biophysics of Dialysis. In Replacement of Renal Function by Dialysis; Springer: Dordrecht, The Netherlands, 1979; pp. 38–68. [Google Scholar]
- Jaffrin, M.Y.; Ding, L.; Laurent, J.M. Simultaneous Convective and Diffusive Mass Transfers in a Hemodialyser. J. Biomech. Eng. 1990, 112, 212–219. [Google Scholar] [CrossRef]
- Jaffrin, M.Y. Convective Mass Transfer in Hemodialysis. Artif. Organs 1995, 19, 1162–1171. [Google Scholar] [CrossRef]
- Waniewski, J. Mathematical modeling of fluid and solute transport in hemodialysis and peritoneal dialysis. J. Memb. Sci. 2006, 274, 24–37. [Google Scholar] [CrossRef]
- Chang, Y.L.; Lee, C.J. Solute transport characteristics in hemodiafiltration. J. Memb. Sci. 1988, 39, 99–111. [Google Scholar] [CrossRef]
- Jaffrin, M.Y.; Gupta, B.B.; Malbrancq, J.M. A one-dimensional model of simultaneous hemodialysis and ultrafiltration with highly permeable membranes. J. Biomech. Eng. 1981, 103, 261–266. [Google Scholar] [CrossRef]
- Legallais, C.; Catapano, G.; Von Harten, B.; Baurmeister, U. A theoretical model to predict the in vitro performance of hemodiafilters. J. Memb. Sci. 2000, 168, 3–15. [Google Scholar] [CrossRef]
- Eloot, S.; D’Asseler, Y.; De Bondt, P.; Verdonck, P. Combining SPECT medical imaging and computational fluid dynamics for analyzing blood and dialysate flow in hemodialyzers. Int. J. Artif. Organs 2005, 28, 739–749. [Google Scholar] [CrossRef] [PubMed]
- Osuga, T.; Obata, T.; Ikehira, H.; Tanada, S.; Sasaki, Y.; Naito, H. Dialysate pressure isobars in a hollow-fiber dialyzer determined from magnetic resonance imaging and numerical simulation of dialysate flow. Artif. Organs 1998, 22, 907–909. [Google Scholar] [CrossRef] [PubMed]
- Abaci, H.E.; Altinkaya, S.A. Modeling of hemodialysis operation. Ann. Biomed. Eng. 2010, 38, 3347–3362. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Donato, D.; Boschetti-de-Fierro, A.; Zweigart, C.; Kolb, M.; Eloot, S.; Storr, M.; Krause, B.; Leypoldt, K.; Segers, P. Optimization of dialyzer design to maximize solute removal with a two- dimensional transport model. J. Memb. Sci. 2017, 541, 519–528. [Google Scholar] [CrossRef]
- Lemanski, J.; Lipscomb, G.G. Effect of shell-side flows on hollow-fiber membrane device performance. AIChE J. 1995, 41, 2322–2326. [Google Scholar] [CrossRef]
- Liao, Z.; Poh, C.K.; Huang, Z.; Hardy, P.A.; Clark, W.R.; Gao, D. A Numerical and Experimental Study of Mass Transfer in the Artificial Kidney. J. Biomech. Eng. 2003, 125, 472. [Google Scholar] [CrossRef]
- Ding, W.; Li, W.; Sun, S.; Zhou, X.; Hardy, P.A.; Ahmad, S.; Gao, D. Three-Dimensional Simulation of Mass Transfer in Artificial Kidneys. Artif. Organs 2015, 39, E79–E89. [Google Scholar] [CrossRef]
- Ding, W.; He, L.; Zhao, G.; Luo, X.; Zhou, M.; Gao, D. Effect of Distribution Tabs on Mass Transfer of Artificial Kidney. AIChE J. 2004, 50, 786–790. [Google Scholar] [CrossRef]
- Cancilla, N.; Gurreri, L.; Marotta, G.; Ciofalo, M.; Cipollina, A.; Tamburini, A.; Micale, G. A porous media CFD model for the simulation of hemodialysis in hollow fiber membrane modules. J. Memb. Sci. 2022, 646, 120219. [Google Scholar] [CrossRef]
- Cancilla, N.; Gurreri, L.; Marotta, G.; Ciofalo, M.; Cipollina, A.; Tamburini, A.; Micale, G. CFD prediction of shell-side flow and mass transfer in regular fiber arrays. Int. J. Heat Mass Transf. 2021, 168, 120855. [Google Scholar] [CrossRef]
- Schoner, P.; Plucinski, P.; Nitsch, W.; Daiminger, U. Mass transfer in the shell side of cross flow hollow fiber modules. Chem. Eng. Sci. 1998, 53, 2319–2326. [Google Scholar] [CrossRef]
- Bowry, S.K. Dialysis membranes today. Int. J. Artif. Organs 2002, 25, 447–460. [Google Scholar] [CrossRef] [PubMed]
- Baskurt, O.K.; Hardeman, M.R.; Rampling, M.W.; Meiselman, H.J. Handbook of Hemorheology and Hemodynamics; IOS Press: Amsterdam, The Netherlands, 2007. [Google Scholar]
- Middleman, S. Transport Phenomena in the Cardiovascular System; Wiley-Interscience Series on Biomedical Engineering; Wiley-Interscience: Hoboken, NJ, USA, 1972. [Google Scholar]
- Dionne, K.E.; Cain, B.M.; Li, R.H.; Bell, W.J.; Doherty, E.J.; Rein, D.H.; Lysaght, M.J.; Gentile, F.T. Transport characterization of membranes for immunoisolation. Biomaterials 1996, 17, 257–266. [Google Scholar] [CrossRef]
- Karabelas, A.J.; Kostoglou, M.; Moschona, A.; Sioutopoulos, D.C. Method development for experimental determination οf key fluid-mechanical parameters of haemo-catharsis modules. J. Memb. Sci. 2020, 611, 118353. [Google Scholar] [CrossRef]
- Kostoglou, M.; Moschona, A.; Karabelas, A.J.; Sioutopoulos, D.C. Implementation and validation of an innovative method for fluid mechanical characterization of haemo-catharsis modules. J. Memb. Sci. 2021, 624, 119117. [Google Scholar] [CrossRef]
- Frank, A.; Lipscomb, G.G.; Dennis, M. Visualization of concentration fields in hemodialyzers by computed tomography. J. Memb. Sci. 2000, 175, 239–251. [Google Scholar] [CrossRef]

| Membrane Data | |
|---|---|
| Internal diameter of the hollow fibers, di (μm) | 200 ± 20 |
| Thickness of the hollow fibers, s (μm) | 30 ± 5 |
| Diffusive permeability (for urea), kM for urea (m s−1) | (1.1 ± 0.2) × 10−5 |
| Hydraulic permeability, Lp (m s−1 Pa−1) | (6.6 ± 0.4) × 10−11 |
| Geometry | Total Number of FV | Number of FV in the Cross-Sectional Plane |
|---|---|---|
| Long cylindrical | 280,800 | 10,800 |
| Short cylindrical | 302,400 | 8400 |
| Thick rectangular | 301,050 | 10,035 (zy plane) |
| Flat rectangular | 354,816 | 2772 (zy plane) |
| Coaxial cylindrical | 299,520 | 11,520 |
| Fluid | Density (kg m−3) | Viscosity (Pa s) | Diffusivity of Urea (m2 s−1) | Inlet Flow Rate (mL min−1) | Inlet Concentration (mol m−3) |
|---|---|---|---|---|---|
| Blood | 1000 | 3.50 × 10−3 | 7.4 × 10−10 | 300 | 20 |
| Dialysate | 1000 | 7.62 × 10−4 | 1.8 × 10−9 | 500 | 0 |
| Solute | QB,i (mL min−1) | Exp. 1 (mL min−1) | Model (mL min−1) | Error |
|---|---|---|---|---|
| Urea | 200 | 191 ± 7.0 | 192 | 0.5% |
| 300 | 249 ± 10 | 255 | 2.4% | |
| 400 | 294 ± 14 | 294 | 0% | |
| B12 vitamin | 200 | 147 ± 8.0 | 144 | 2.0% |
| 300 | 164 ± 12 | 168 | 2.4% | |
| 400 | 186 ± 16 | 182 | 2.2% |
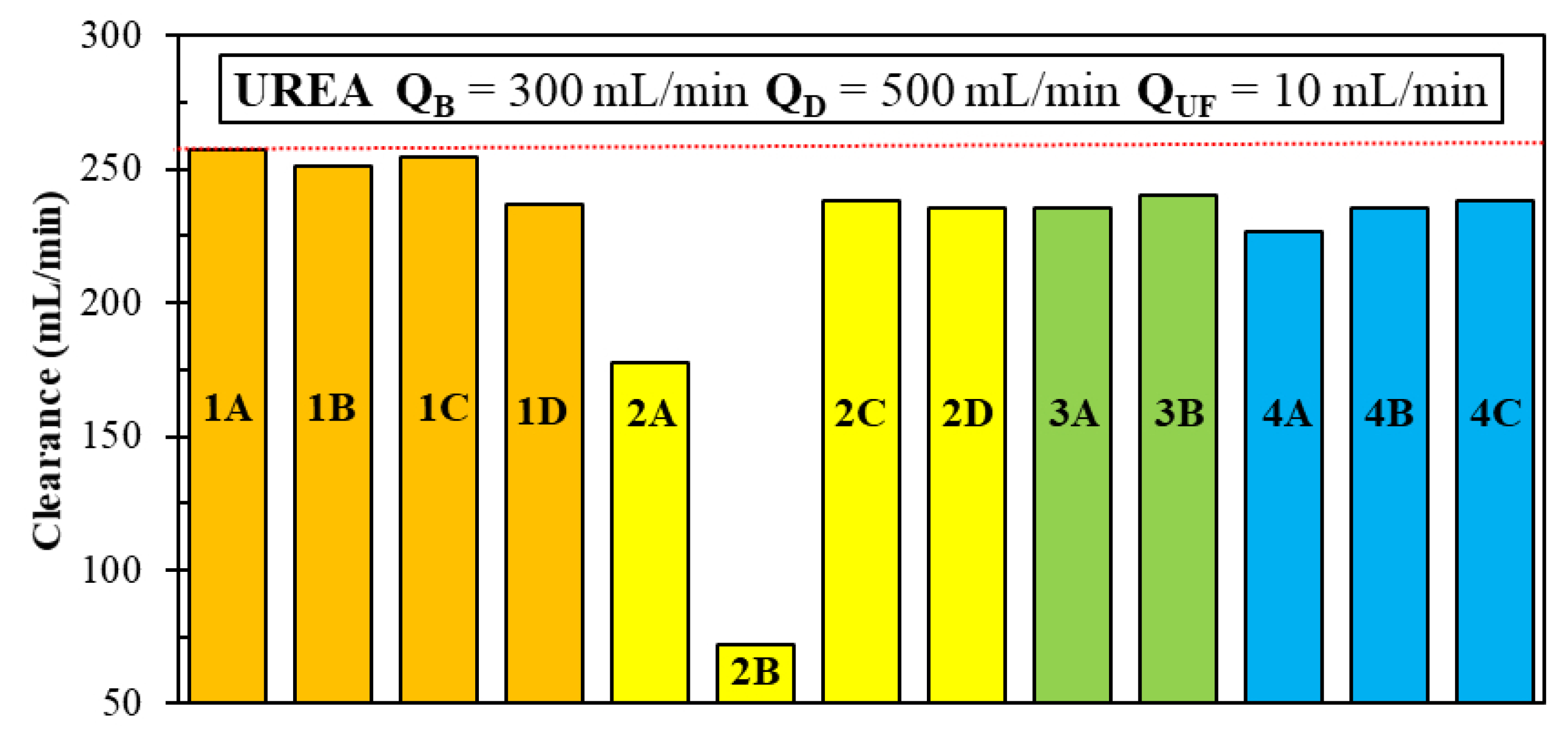
| Long Cylindrical | ||||||
| Case | Notes | ΔpB (Pa) | ΔpD (Pa) | 〈ShD〉 | CL (mL/min) | UF (%) |
| 1A | 8 inlets/8 outlets | 9833 | 4510 | 14.1 | 257 | 4.10 |
| 1B | 1 inlet/1 outlet s.s. | 9833 | 16,280 | 17.5 | 251 | 4.70 |
| 1C | 1 inlet/1 outlet o.s. | 9833 | 16,320 | 18.2 | 255 | 4.30 |
| 1D | Slit inlet/outlet | 9833 | 1812 | 19.8 | 237 | 3.64 |
| Short Cylindrical | ||||||
| Case | Notes | ΔpB (Pa) | ΔpD (Pa) | 〈ShD〉 | CL (mL/min) | UF (%) |
| 2A | 8 inlets/8 outlets | 635.0 | 917.5 | 15.0 | 178 | 3.64 |
| 2B | 1 inlet/1 outlet s.s. | 635.0 | 7134 | 13.4 | 72 | 12.8 |
| 2C | 1 inlet/1 outlet o.s. | 635.0 | 10,942 | 22.5 | 238 | 2.21 |
| 2D | Slit inlet/outlet | 635.0 | 6026 | 22.7 | 236 | 2.28 |
| Rectangular | ||||||
| Case | Notes | ΔpB (Pa) | ΔpD (Pa) | 〈ShD〉 | CL (mL/min) | UF (%) |
| 3A | Thick | 3347 | 2204 | 20.6 | 235 | 2.55 |
| 3B | Flat | 3347 | 10,352 | 24.9 | 240 | 3.43 |
| Coaxial Cylindrical | ||||||
| Case | Notes | ΔpB (Pa) | ΔpD (Pa) | 〈ShD〉 | CL (mL/min) | UF (%) |
| 4A | 6 inlets/6 outlets | 8132 | 1694 | 16.6 | 227 | 4.29 |
| 4B | 4 inlets/1 outlet | 8132 | 3857 | 16.3 | 236 | 3.42 |
| 4C | 1 inlet/1 outlet | 8132 | 5614 | 15.9 | 238 | 3.80 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cancilla, N.; Gurreri, L.; Marotta, G.; Ciofalo, M.; Cipollina, A.; Tamburini, A.; Micale, G. Performance Comparison of Alternative Hollow-Fiber Modules for Hemodialysis by Means of a CFD-Based Model. Membranes 2022, 12, 118. https://doi.org/10.3390/membranes12020118
Cancilla N, Gurreri L, Marotta G, Ciofalo M, Cipollina A, Tamburini A, Micale G. Performance Comparison of Alternative Hollow-Fiber Modules for Hemodialysis by Means of a CFD-Based Model. Membranes. 2022; 12(2):118. https://doi.org/10.3390/membranes12020118
Chicago/Turabian StyleCancilla, Nunzio, Luigi Gurreri, Gaspare Marotta, Michele Ciofalo, Andrea Cipollina, Alessandro Tamburini, and Giorgio Micale. 2022. "Performance Comparison of Alternative Hollow-Fiber Modules for Hemodialysis by Means of a CFD-Based Model" Membranes 12, no. 2: 118. https://doi.org/10.3390/membranes12020118
APA StyleCancilla, N., Gurreri, L., Marotta, G., Ciofalo, M., Cipollina, A., Tamburini, A., & Micale, G. (2022). Performance Comparison of Alternative Hollow-Fiber Modules for Hemodialysis by Means of a CFD-Based Model. Membranes, 12(2), 118. https://doi.org/10.3390/membranes12020118










