Effect of Packing Nonuniformity at the Fiber Bundle–Case Interface on Performance of Hollow Fiber Membrane Gas Separation Modules
Abstract
1. Introduction
2. Simulation Methodology
2.1. Theoretical Background
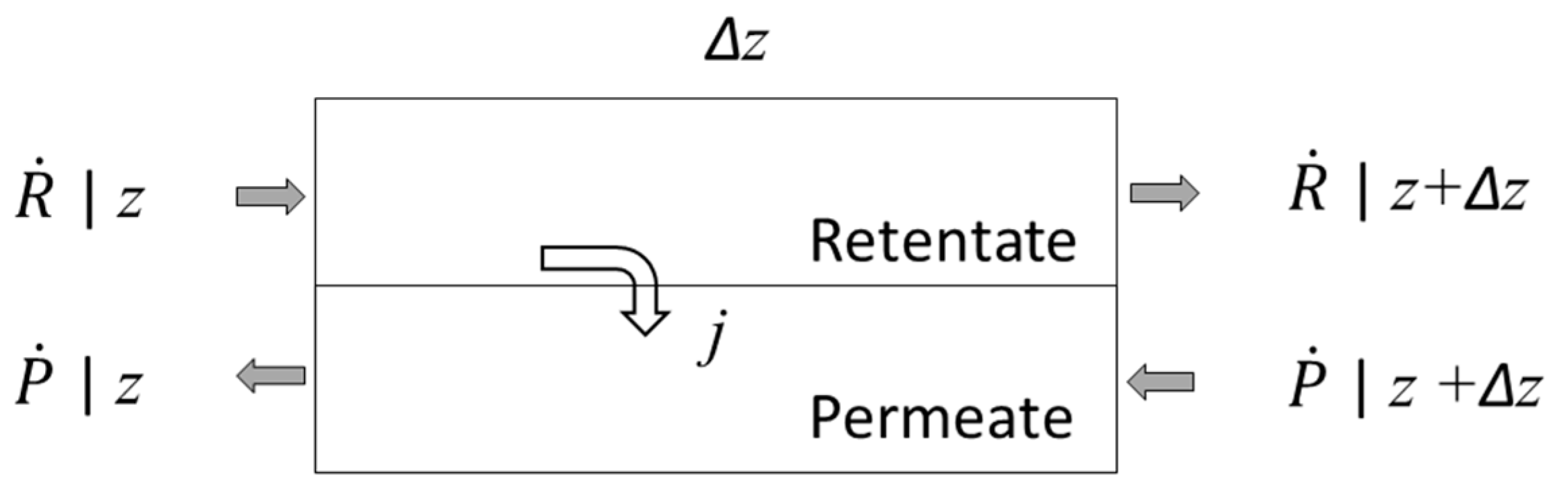
2.1.1. Governing Equations
2.1.2. Constitutive Laws
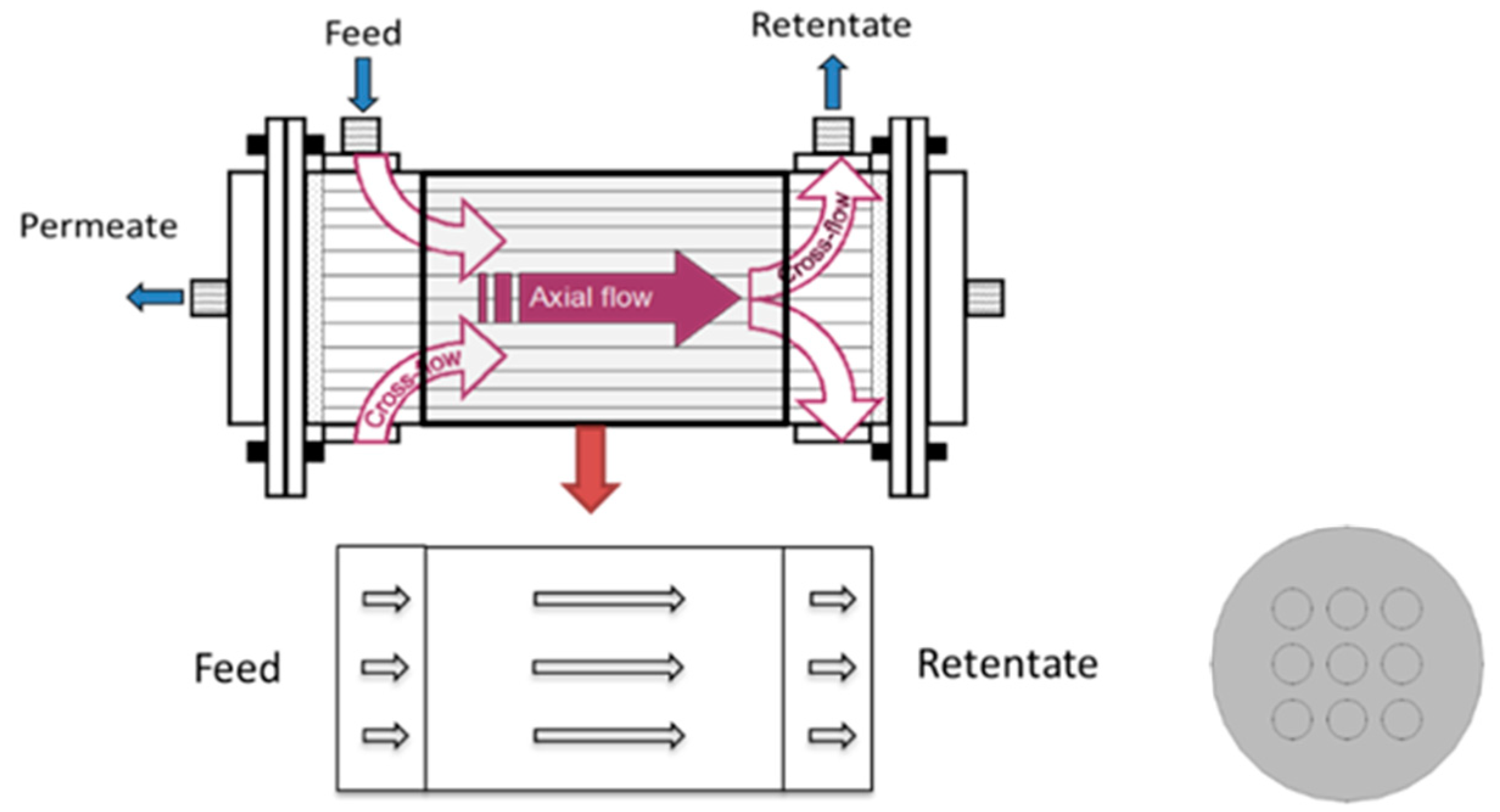
2.2. Numerical Setup
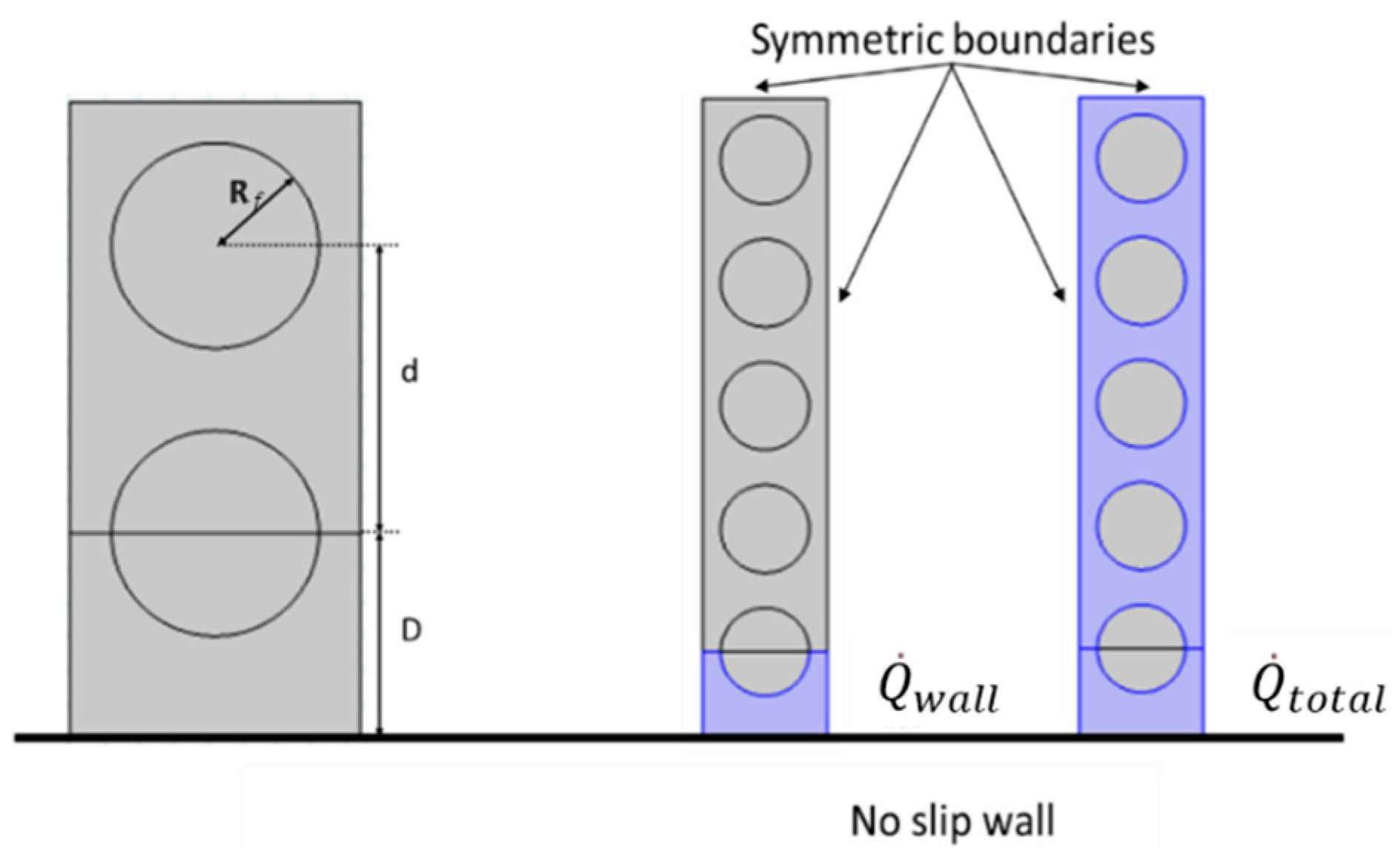
2.2.1. Domain Setup and Boundary Conditions
2.2.2. Numerical Methodology
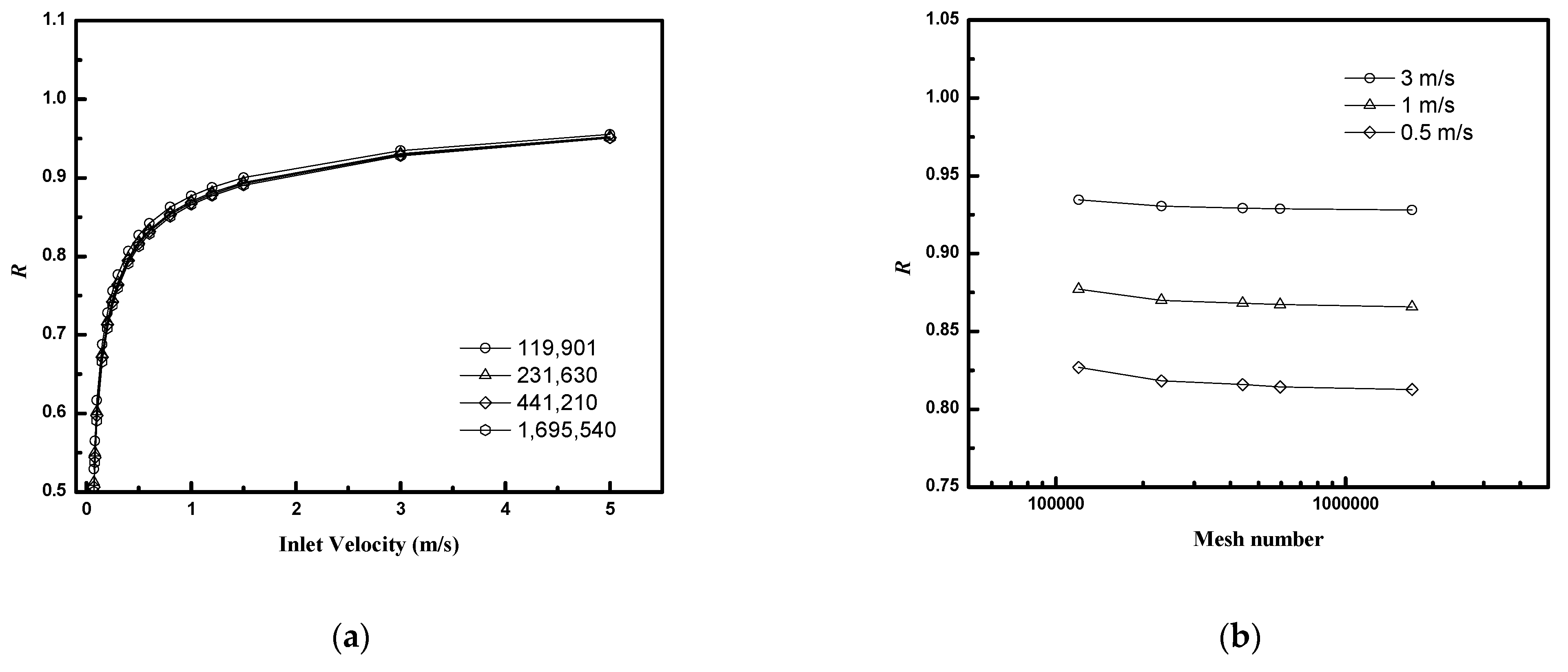
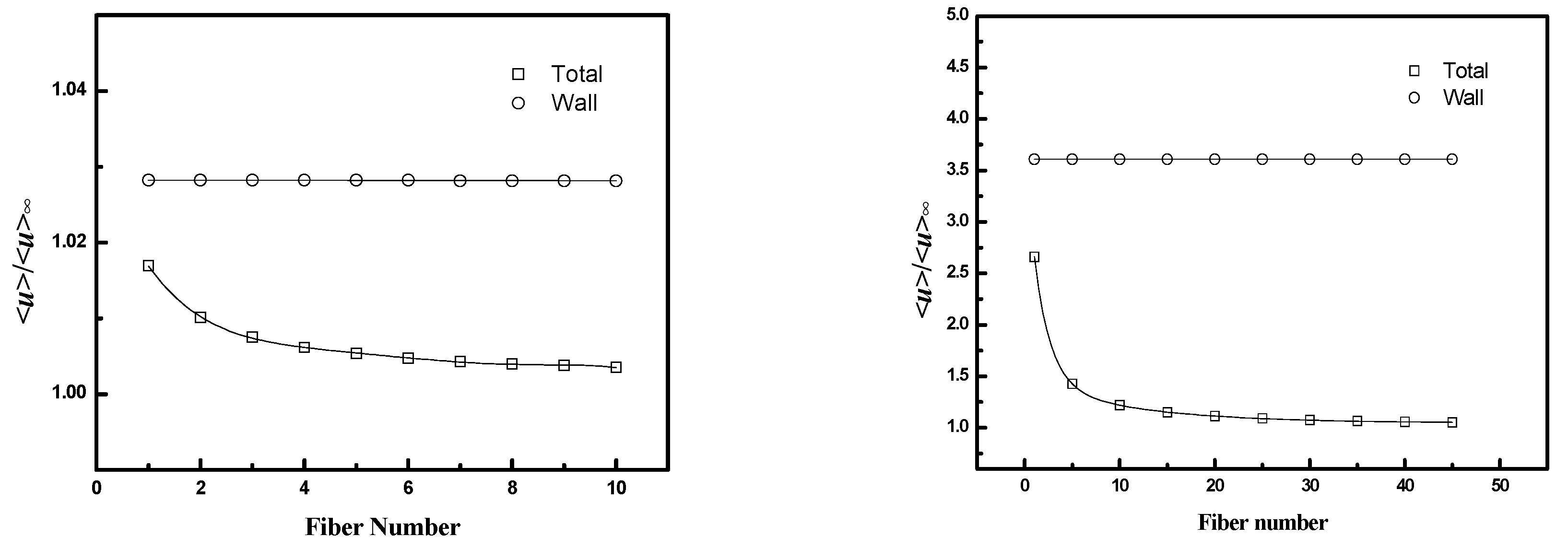
2.2.3. Mesh Independence Studies
2.2.4. Darcy’s Permeability
2.2.5. Ideal Counter-Current Module
2.2.6. Design Space Explored
3. Results and Discussion
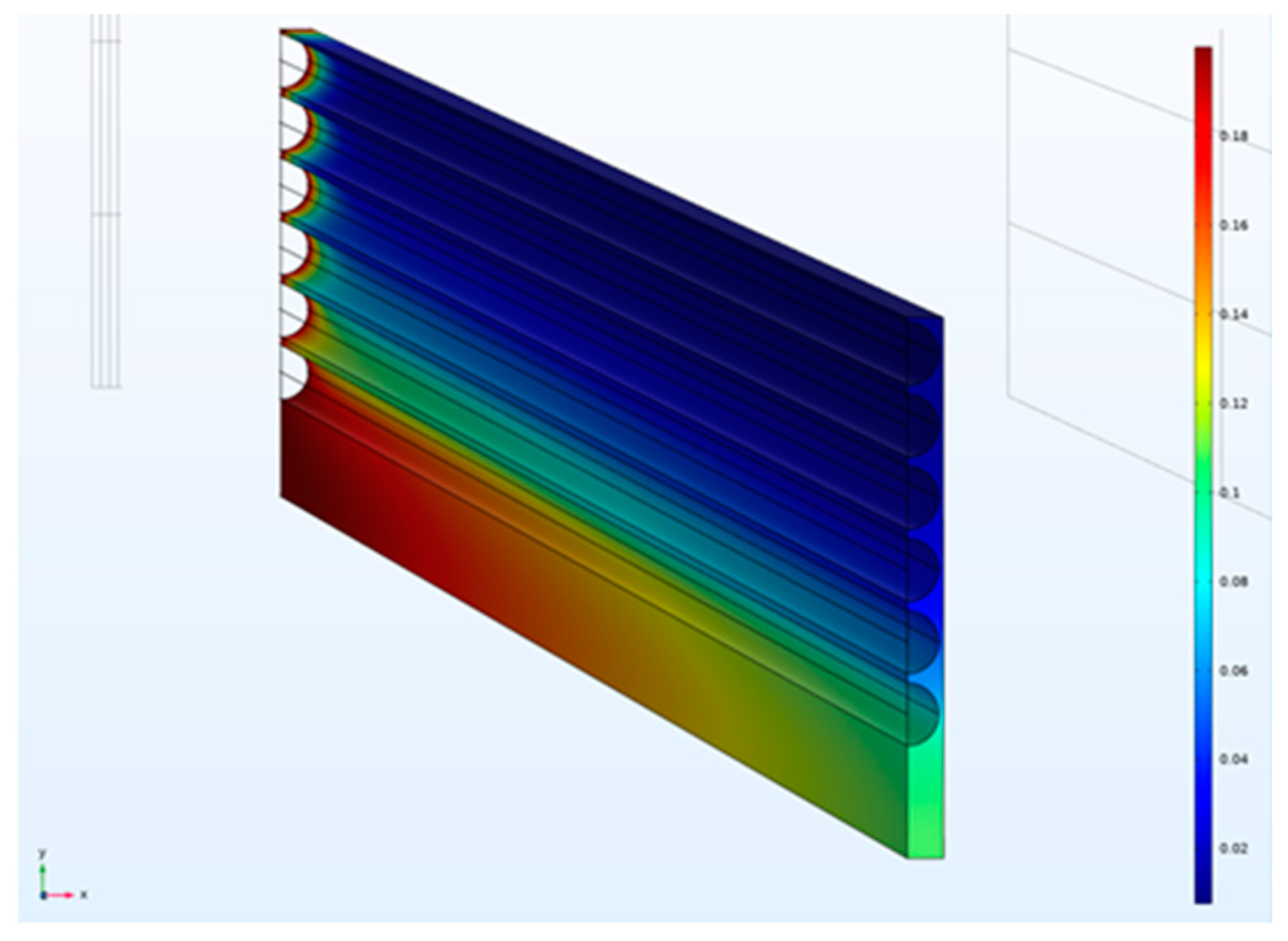
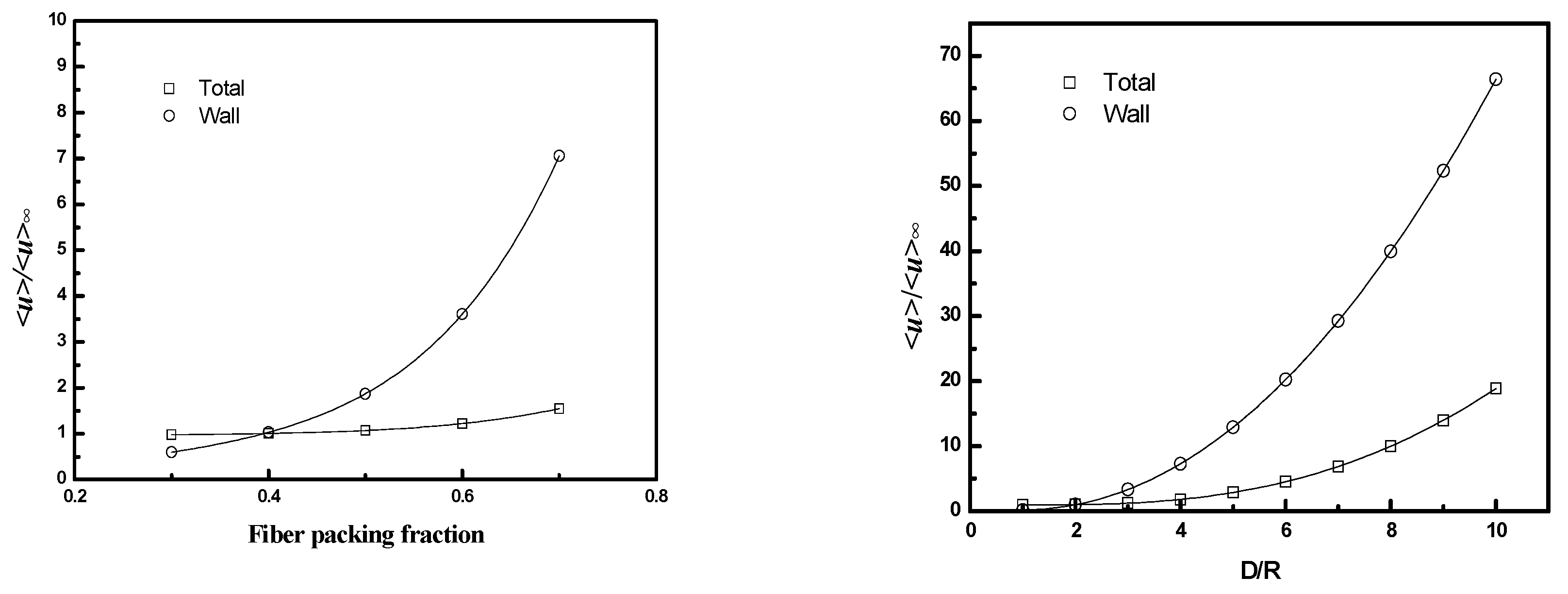
3.1. Planar Square Configuration (Velocity Distribution)
3.2. Planar Equilateral Triangular Configuration (Velocity Distribution)
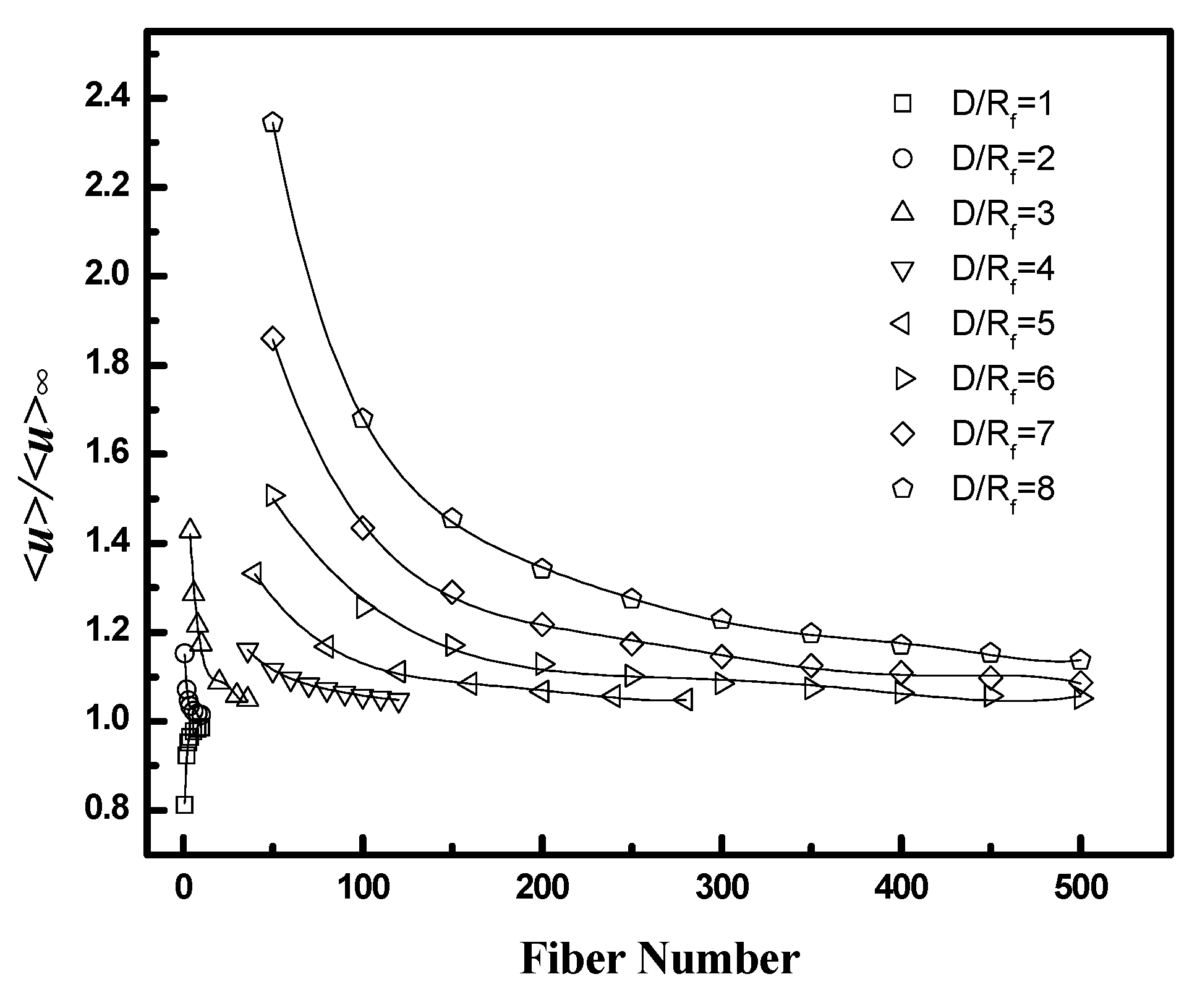
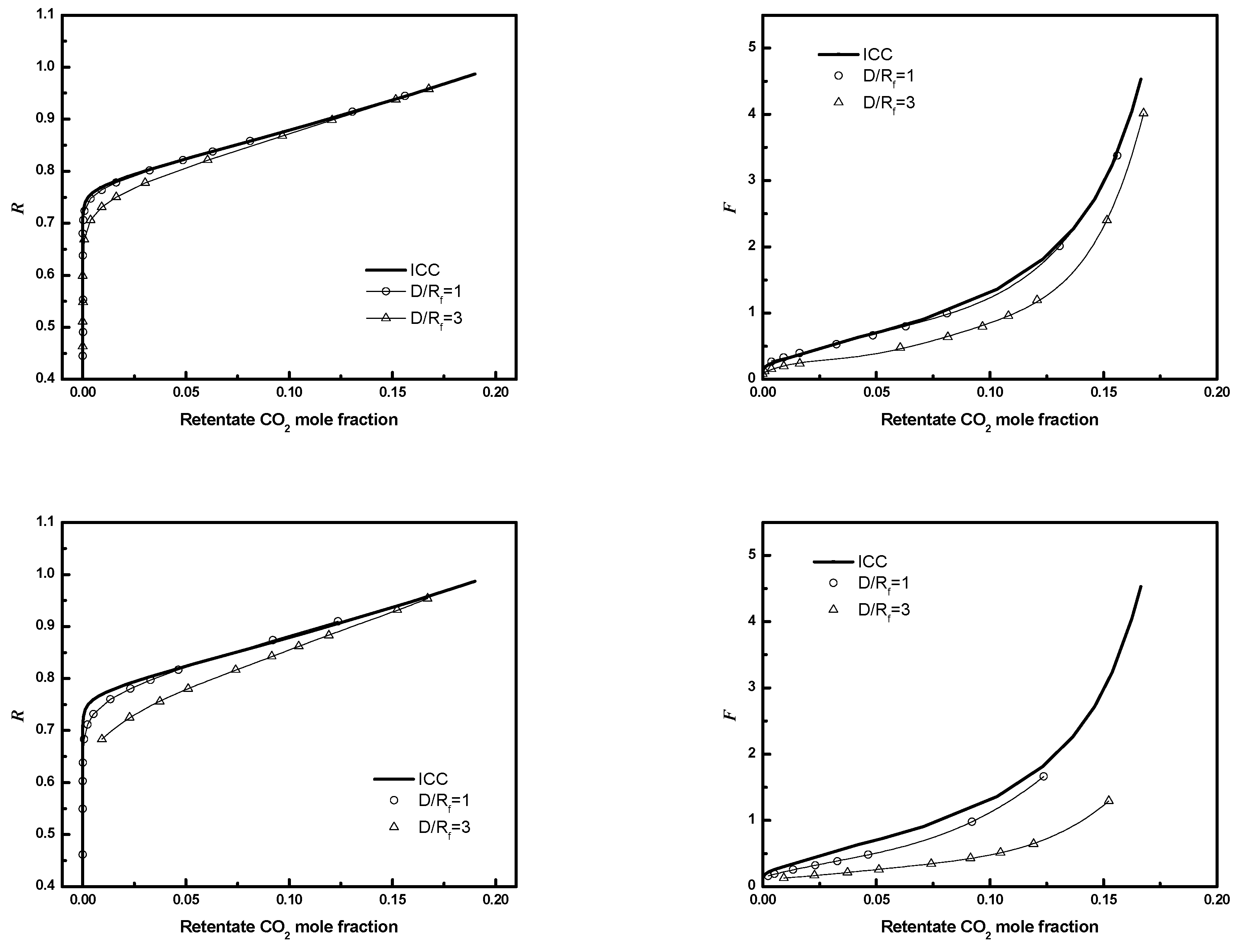
3.3. Planar Square Configuration
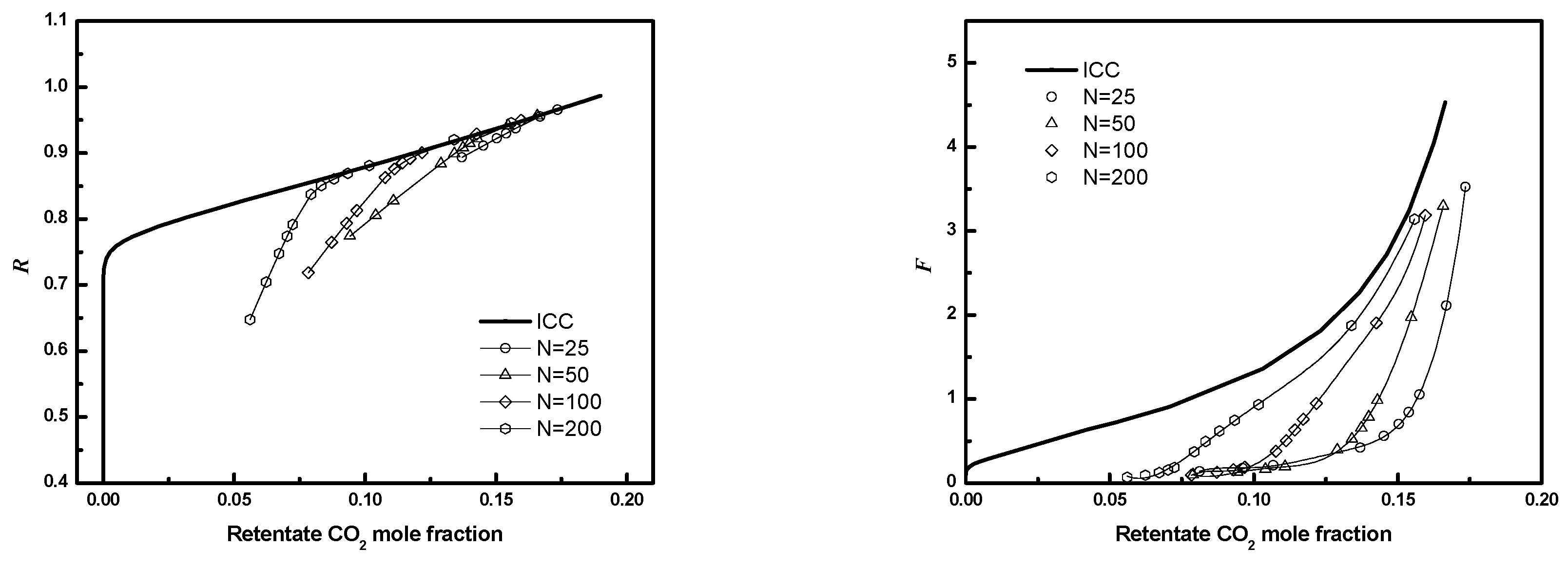
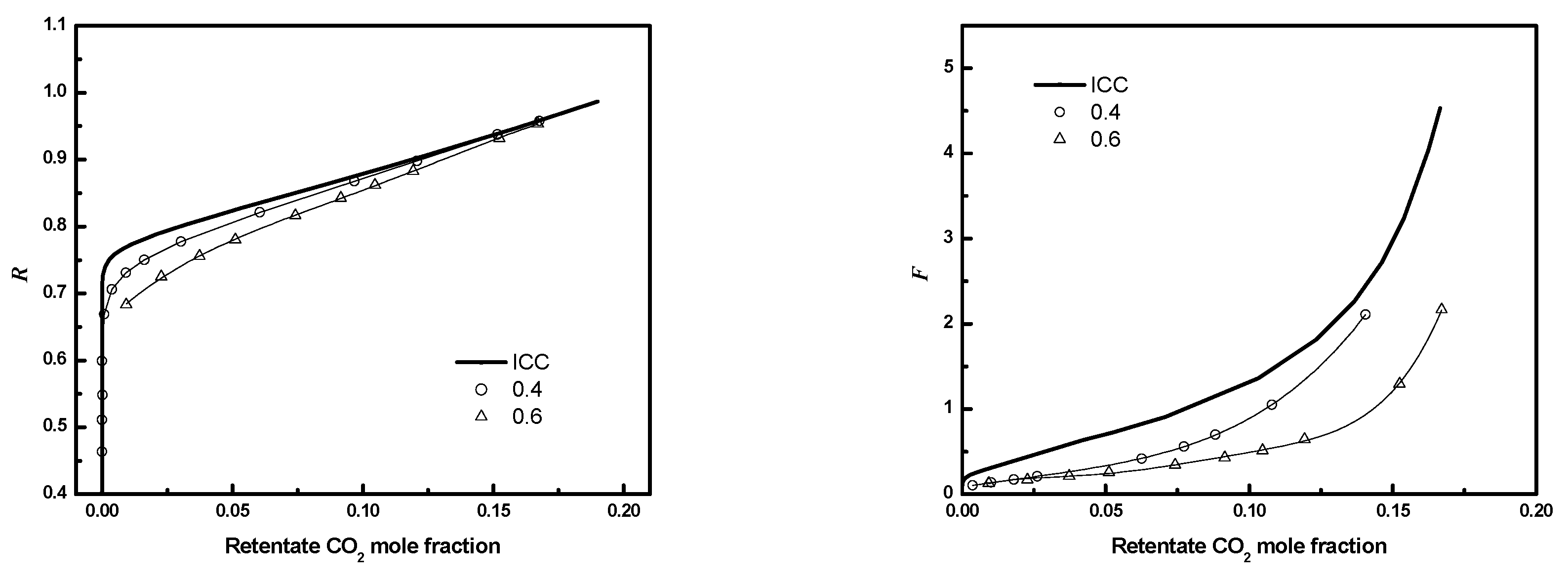
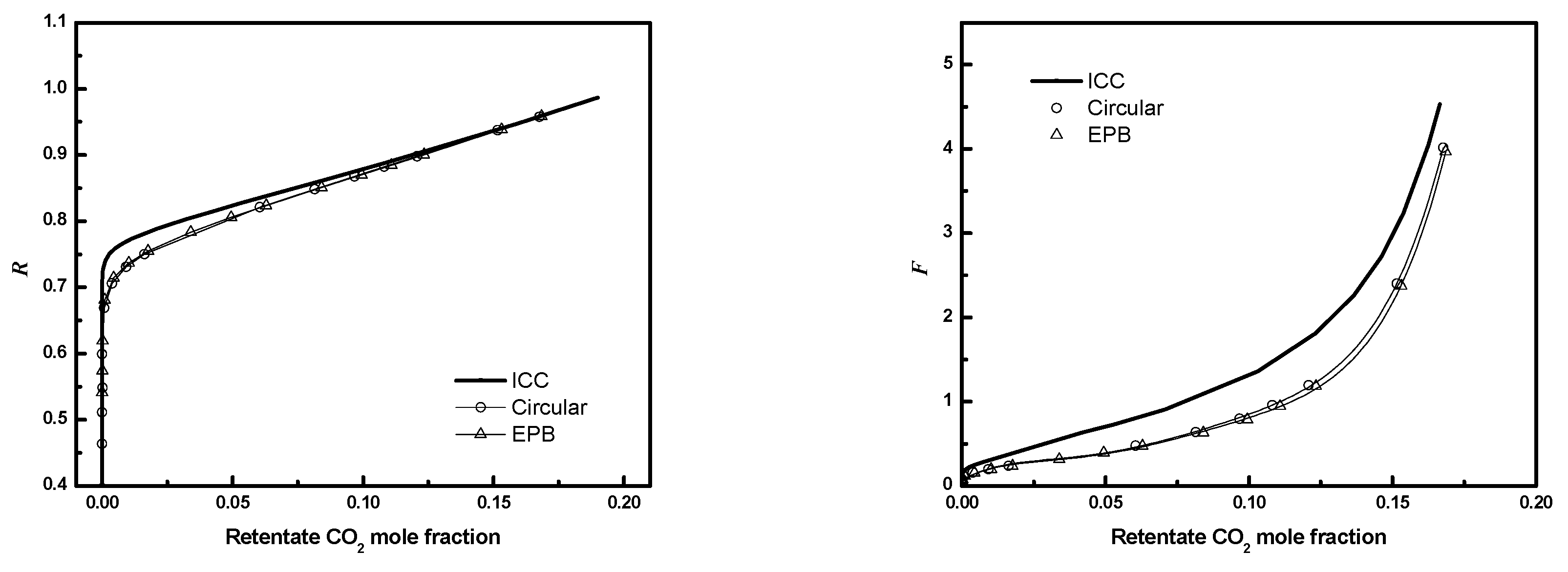
3.4. Circular Square Bundle
3.5. Circular Triangular Bundle
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Disclaimer
References
- Friedlingstein, P.; O'Sullivan, M.; Jones, M.W.; Andrew, R.M.; Hauck, J.; Olsen, A.; Peters, G.P.; Peters, W.; Pongratz, J.; Sitch, S.; et al. Global Carbon Budget 2020. Earth Syst. Sci. Data 2020, 12, 3269–3340. [Google Scholar] [CrossRef]
- Allen, M. Framing and Context; Intergovernmental Panel on Climate Change: Geneva, Switzerland, 2018; Chapter 1. [Google Scholar]
- Merkel, T.C.; Lin, H.; Wei, X.; Baker, R. Power plant post-combustion carbon dioxide capture: An opportunity for membranes. J. Membr. Sci. 2010, 359, 126–139. [Google Scholar] [CrossRef]
- Wu, H.; Li, Q.; Sheng, M.; Wang, Z.; Zhao, S.; Wang, J.; Mao, S.; Wang, D.; Guo, B.; Ye, N.; et al. Membrane technology for CO2 capture: From pilot-scale investigation of two-stage plant to actual system design. J. Membr. Sci. 2021, 624, 119137. [Google Scholar] [CrossRef]
- Lipscomb, G.G.; Sonalkar, S. Sources of non-ideal flow distribution and their effect on the performance of hollow fiber gas separation modules. Sep. Purif. Rev. 2004, 33, 41–76. [Google Scholar] [CrossRef]
- Rautenbach, R.; Struck, A.; Roks, M.F.M. A variation in fiber properties affects the performance of defect-free hollow fiber membrane modules for air separation. J. Membr. Sci. 1998, 150, 31–41. [Google Scholar] [CrossRef]
- Lemanski, J.; Lipscomb, G.G. Effect of fiber variation on the performance of counter-current hollow fiber gas separation modules. J. Membr. Sci. 2000, 167, 241–252. [Google Scholar] [CrossRef]
- Liu, B.; Lipscomb, G.G.; Jensvold, J. Effect of fiber variation on staged membrane gas separation module performance. AIChE J. 2001, 47, 2206–2219. [Google Scholar] [CrossRef]
- Park, J.K.; Chang, H.N. Flow distribution in the fiber lumen side of a hollow fiber module. AIChE J. 1986, 32, 1937–1947. [Google Scholar] [CrossRef]
- Noda, I.; Brown-West, D.G.; Gryte, C.C. Effect of flow maldistribution on hollow fiber dialysis—Experimental studies. J. Membr. Sci. 1979, 5, 209–225. [Google Scholar] [CrossRef]
- Lemanski, J.; Lipscomb, G.G. Effect of shell-side flows on hollow-fiber membrane device performance. AIChE J. 1995, 41, 2322–2326. [Google Scholar] [CrossRef]
- Labecki, M.; Piret, J.M.; Bowen, B.D. Two-dimensional analysis of fluid flow in hollow-fibre modules. Chem. Eng. Sci. 1995, 50, 3369–3384. [Google Scholar] [CrossRef]
- Bao, L.; Liu, B.; Lipscomb, G.G. Entry mass transfer in axial flows through randomly packed fiber bundles. AIChE J. 1999, 45, 2346–2356. [Google Scholar] [CrossRef]
- Lemanski, J.; Lipscomb, G.G. Effect of shell-side flows on the performance of hollow-fiber gas separation modules. J. Membr. Sci. 2001, 195, 215–228. [Google Scholar] [CrossRef]
- Bao, L.; Lipscomb, G.G. Mass transfer in axial flows through randomly packed fiber bundles with constant wall concentration. J. Membr. Sci. 2002, 204, 207–220. [Google Scholar] [CrossRef]
- Wang, Y.; Chen, F.; Wang, Y.; Luo, G.; Dai, Y. Effect of random packing on shell-side flow and mass transfer in hollow fiber module described by normal distribution function. J. Membr. Sci. 2003, 216, 81–93. [Google Scholar] [CrossRef]
- Kim, J.C.; Kim, J.H.; Sung, J.; Kim, H.C.; Kang, E.; Lee, S.H.; Kim, J.K.; Kim, H.C.; Min, B.G.; Ronco, C. Effects of arterial port design on blood flow distribution in hemodialyzers. Blood Purif. 2009, 28, 260–267. [Google Scholar] [CrossRef] [PubMed]
- Frank, A.; Lipscomb, G.G.; Dennis, M. Visualization of concentration fields in hemodialyzers by computed tomography. J. Membr. Sci. 2000, 175, 239–251. [Google Scholar] [CrossRef]
- Ding, W.; He, L.; Zhao, G.; Luo, X.; Zhou, M.; Gao, D. Effect of distribution tabs on mass transfer of artificial kidney. AIChE J. 2004, 50, 786–790. [Google Scholar] [CrossRef]
- Hao, P.; Lipscomb, G.G. The effect of sweep uniformity on gas dehydration module performance. In Membrane Gas Separation; Yampolskii, Y., Freeman, B., Eds.; Wiley: Hoboken, NJ, USA, 2010; pp. 333–353. [Google Scholar]
- Rivero, J.R.; Panagakos, G.; Lieber, A.; Hornbostel, K. Hollow fiber membrane contactors for post-combustion carbon capture: A review of modeling approaches. Membranes 2020, 10, 382. [Google Scholar] [CrossRef] [PubMed]
- Baker, R.W. Membrane Technology and Applications, 3rd ed.; Wiley: Chichester, UK, 2012. [Google Scholar]
- Sangani, A.S.; Yao, C. Transport processes in random arrays of cylinders. II. Viscous flow. Phys. Fluids 1988, 31, 2435–2444. [Google Scholar] [CrossRef]

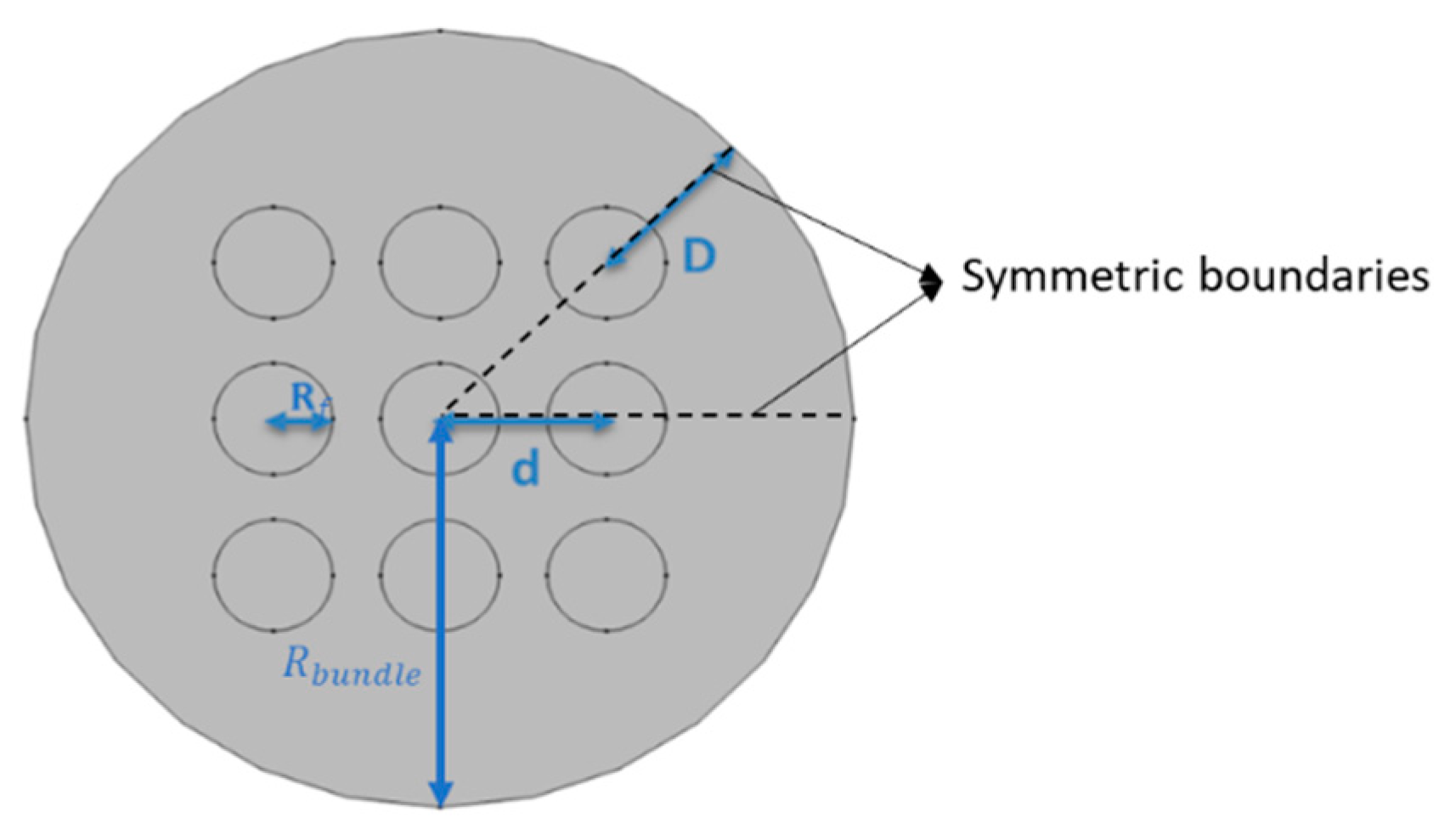


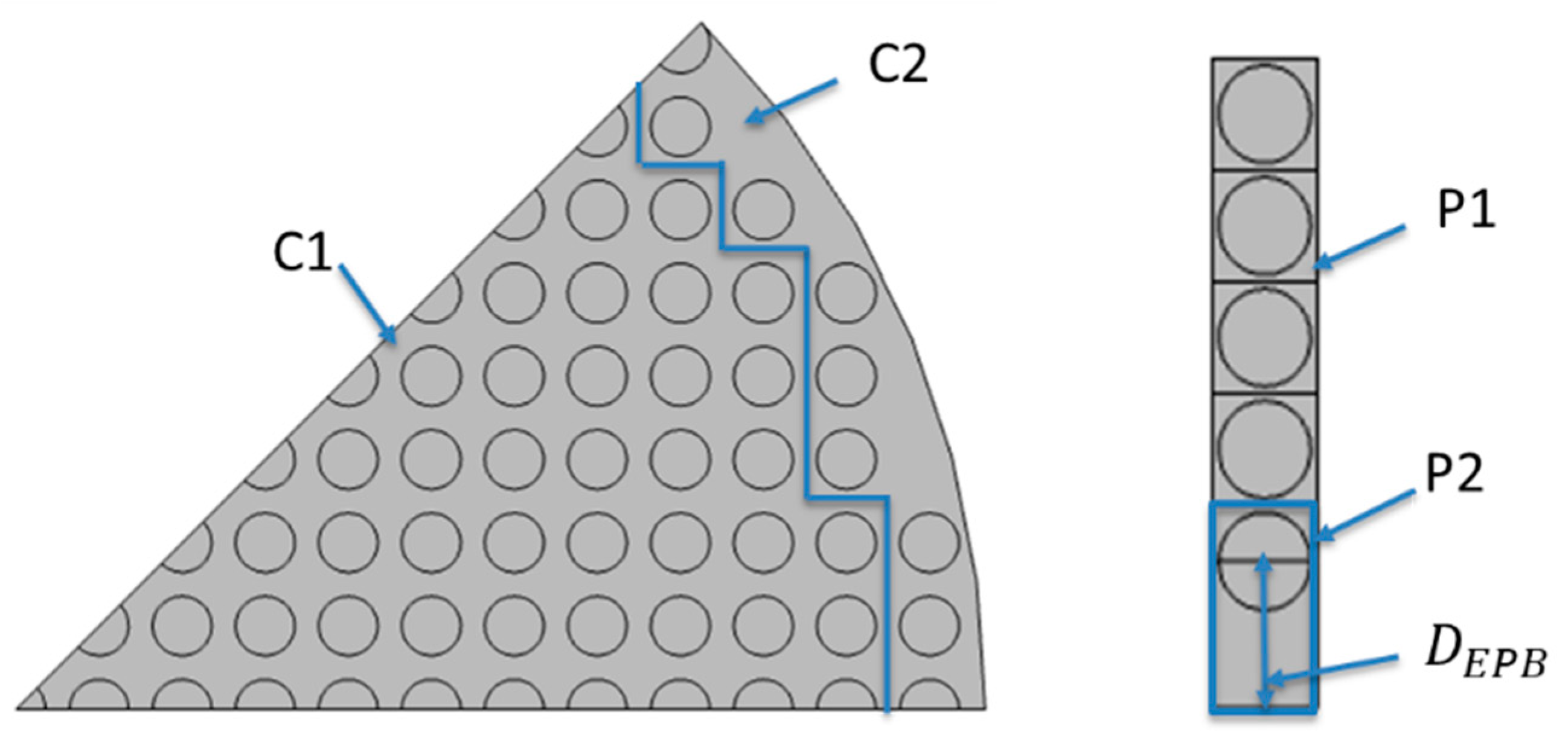






| Symbol | Definition |
|---|---|
| fiber radius | |
| fiber bundle radius | |
| distance between fibers | |
| distance from wall to center of fiber closest to wall | |
| fiber packing fraction [21] |
| Bao [15] | Sangani [23] | ||
|---|---|---|---|
| 0.3 | 0.234 | 0.235 | 0.235 |
| 0.4 | 0.0984 | - | - |
| 0.5 | 0.0445 | 0.0444 | 0.0445 |
| 0.6 | 0.0203 | - | - |
| 0.7 | 0.0095 | 0.0094 | 0.0094 |
| Parameter | Value |
|---|---|
| Feed CO2 mole fraction | 0.2 |
| Fiber length (L) | 0.15 m |
| Fiber outside diameter, OD | 3.00 × 10−4 m |
| Selectivity (α) | 75 |
| 1500 GPU | |
| 20 GPU | |
| 2 atm | |
| 0 atm | |
| 1.60 × 10−5 m2s−1 | |
| Operating temperature, T | 298 K |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, L.; Panagakos, G.; Lipscomb, G. Effect of Packing Nonuniformity at the Fiber Bundle–Case Interface on Performance of Hollow Fiber Membrane Gas Separation Modules. Membranes 2022, 12, 1139. https://doi.org/10.3390/membranes12111139
Sun L, Panagakos G, Lipscomb G. Effect of Packing Nonuniformity at the Fiber Bundle–Case Interface on Performance of Hollow Fiber Membrane Gas Separation Modules. Membranes. 2022; 12(11):1139. https://doi.org/10.3390/membranes12111139
Chicago/Turabian StyleSun, Lili, Grigorios Panagakos, and Glenn Lipscomb. 2022. "Effect of Packing Nonuniformity at the Fiber Bundle–Case Interface on Performance of Hollow Fiber Membrane Gas Separation Modules" Membranes 12, no. 11: 1139. https://doi.org/10.3390/membranes12111139
APA StyleSun, L., Panagakos, G., & Lipscomb, G. (2022). Effect of Packing Nonuniformity at the Fiber Bundle–Case Interface on Performance of Hollow Fiber Membrane Gas Separation Modules. Membranes, 12(11), 1139. https://doi.org/10.3390/membranes12111139









