Design of Multi-Layer Graphene Membrane with Descending Pore Size for 100% Water Desalination by Simulation Using ReaxFF
Abstract
:1. Introduction
2. Materials and Methods
2.1. Molecular Dynamics Simulation
2.2. Investigations of the Properties of the Membrane with Recommended Pores
3. Results
3.1. Water Permeability
3.2. Salt Rejection
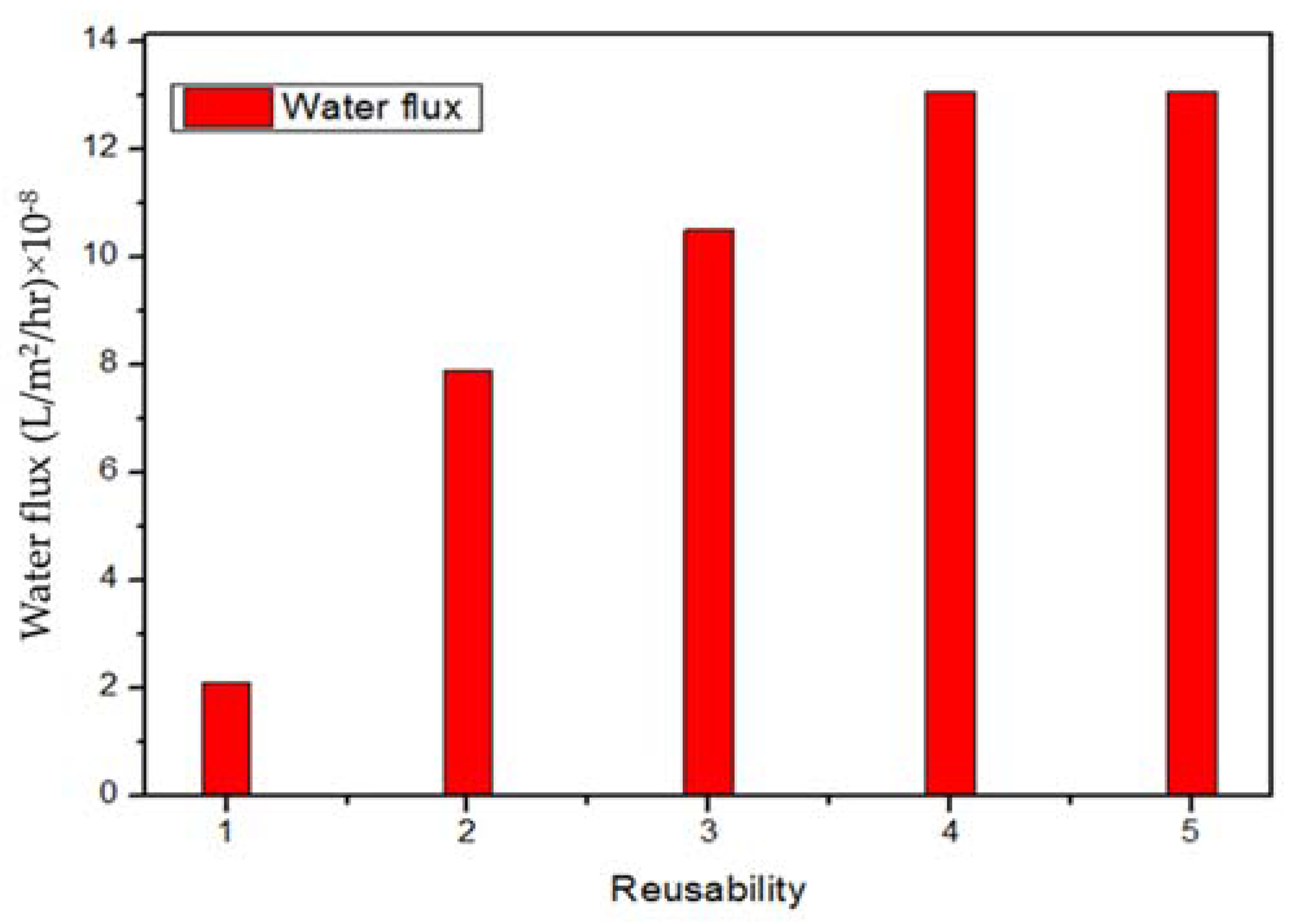
3.3. Water Permeability Profile after Several Cycles
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Homaeigohar, S.; Elbahri, M. Graphene membranes for water desalination. NPG Asia Mater. 2017, 9, e427. [Google Scholar] [CrossRef] [Green Version]
- Cath, T.Y.; Childress, A.E.; Elimelech, M. Forward osmosis: Principles, applications, and recent developments. J. Membr. Sci. 2006, 281, 70–87. [Google Scholar] [CrossRef]
- Qin, M.; Deshmukh, A.; Epsztein, R.; Patel, S.K.; Owoseni, O.M.; Walker, W.S.; Elimelech, M. Comparison of energy consumption in desalination by capacitive deionization and reverse osmosis. Desalination 2019, 455, 100–114. [Google Scholar] [CrossRef]
- Cohen-Tanugi, D.; Grossman, J.C. Water desalination across nanoporous graphene. Nano Lett. 2012, 12, 3602–3608. [Google Scholar] [CrossRef] [PubMed]
- You, Y.; Sahajwalla, V.; Yoshimura, M.; Joshi, R.K. Graphene and graphene oxide for desalination. Nanoscale 2016, 8, 117–119. [Google Scholar] [CrossRef]
- Goh, P.S.; Ismail, A.F.; Ng, B.C. Carbon nanotubes for desalination: Performance evaluation and current hurdles. Desalination 2013, 308, 2–14. [Google Scholar] [CrossRef]
- Qiu, H.; Xue, M.; Shen, C.; Zhang, Z.; Guo, W. Graphynes for Water Desalination and Gas Separation. Adv. Mater. 2019, 31, e1803772. [Google Scholar] [CrossRef]
- Lockheed Martin Corporation. Perforene™ Membrane. 2016. Available online: https://www.lockheedmartin.com/en-us/products/perforene-graphene-membrane.html (accessed on 16 July 2013).
- Pendergast, M.M.; Hoek, E.M. A review of water treatment membrane nanotechnologies. Energy Environ. Sci. 2011, 4, 1946–1971. [Google Scholar] [CrossRef] [Green Version]
- Yao, Y.; Chakraborty, S.; Dhar, A.; Sangani, C.B.; Duan, Y.T.; Vekariya, R.L. Graphene, an epoch-making material in RFID technology: A detailed overview. New J. Chem. 2021, 45, 18700–18721. [Google Scholar] [CrossRef]
- Ibrahim, Q.; Akbarzadeh, R.; Gharbia, S. The electronic properties and water desalination performance of a photocatalytic TiO2/MoS2 nanocomposites bilayer membrane: A molecular dynamic simulation. J. Mol. Modeling 2022, 28, 61. [Google Scholar] [CrossRef]
- Boretti, A.; Al-Zubaidy, S.; Vaclavikova, M.; Al-Abri, M.; Castelletto, S.; Mikhalovsky, S. Outlook for graphene-based desalination membranes. Npj Clean Water 2018, 1, 5. [Google Scholar] [CrossRef] [Green Version]
- Nguyen, C.T.; Beskok, A. Charged nanoporous graphene membranes for water desalination. Phys. Chem. Chem. Phys. 2019, 21, 9483–9494. [Google Scholar] [CrossRef] [PubMed]
- Hosseini, M.; Azamat, J.; Erfan-Niya, H. Water desalination through fluorine-functionalized nanoporous graphene oxide membranes. Mater. Chem. Phys. 2019, 223, 277–286. [Google Scholar] [CrossRef]
- Fischbein, M.D.; Drndić, M. Electron beam nanosculpting of suspended graphene sheets. Appl. Phys. Lett. 2008, 93, 113107. [Google Scholar] [CrossRef] [Green Version]
- Garaj, S.; Hubbard, W.; Reina, A.; Kong, J.; Branton, D.; Golovchenko, J.A. Graphene as a subnanometre trans-electrode membrane. Nature 2010, 467, 190–193. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Merchant, C.A.; Healy, K.; Wanunu, M.; Ray, V.; Peterman, N.; Bartel, J.; Fischbein, M.D.; Venta, K.; Luo, Z.; Johnson, A.T.C.; et al. DNA Translocation through Graphene Nanopores. Nano Lett. 2010, 10, 2915–2921. [Google Scholar] [CrossRef] [PubMed]
- Lu, N.; Wang, J.; Floresca, H.C.; Kim, M.J. In Situ studies on the shrinkage and expansion of graphene nanopores under electron beam irradiation at temperatures in the range of 400–1200 °C. Carbon 2012, 50, 2961–2965. [Google Scholar] [CrossRef]
- Rikhtehgaran, S.; Lohrasebi, A. Multilayer nanoporous graphene as a water purification membrane. J. Nanosci. Nanotechnol. 2018, 18, 5799–5803. [Google Scholar] [CrossRef]
- Ibrahim, Q.; Akbarzadeh, R. A photocatalytic TiO2/graphene bilayer membrane design for water desalination: A molecular dynamic simulation. J. Mol. Modeling 2020, 26, 165. [Google Scholar] [CrossRef]
- Durán, J.M. Computer Simulations in Science and Engineering: Concepts-Practices-Perspectives; Springer: Berlin/Heidelberg, Germany, 2018. [Google Scholar]
- Van Duin, A.C.T.; Dasgupta, S.; Lorant, F.; Goddard, W.A. ReaxFF: A Reactive force field for hydrocarbons. J. Phys. Chem. A 2001, 105, 9396–9409. [Google Scholar] [CrossRef]
- Lemak, A.S.; Balabaev, N.K. On the Berendsen thermostat. Mol. Simul. 1994, 13, 177–187. [Google Scholar] [CrossRef]
- Scm.com. Search—Amsterdam Modeling Suite: Making Computational Chemistry Work for You Software for Chemistry & Materials. Available online: https://www.scm.com/search.php?cat=&search=forcefield (accessed on 8 April 2020).
- Drioli, E.; Giorno, L. (Eds.) Comprehensive Membrane Science and Engineering; Academic Press: Cambridge, MA, USA, 2010; Volume 1, Newnes. [Google Scholar]
- Sun, J.; Hu, C.; Liu, Z.; Liu, H.; Qu, J. Surface charge and hydrophilicity improvement of graphene membranes via modification of pore surface oxygen-containing groups to enhance permeability and selectivity. Carbon 2019, 145, 140–148. [Google Scholar] [CrossRef]
- Dougherty, R.C. Temperature and pressure dependence of hydrogen bond strength: A perturbation molecular orbital approach. J. Chem. Phys. 1998, 109, 7372–7378. [Google Scholar] [CrossRef]
- Gogoi, A.; Konch, T.J.; Raidongia, K.; Reddy, K.A. Water and salt dynamics in multilayer graphene oxide (GO) membrane: Role of lateral sheet dimensions. J. Membr. Sci. 2018, 563, 785–793. [Google Scholar] [CrossRef]
- Dahanayaka, M.; Liu, B.; Hu, Z.; Pei, Q.-X.; Chen, Z.; Law, A.W.-K.; Zhou, K. Graphene membranes with nanoslits for seawater desalination via forward osmosis. Phys. Chem. Chem. Phys. 2017, 19, 30551–30561. [Google Scholar] [CrossRef] [PubMed]
- Cohen-Tanugi, D.; Lin, L.C.; Grossman, J.C. Multilayer nanoporous graphene membranes for water desalination. Nano Lett. 2016, 16, 1027–1033. [Google Scholar] [CrossRef] [Green Version]
- Arkles, B. Hydrophobicity, hydrophilicity and silane surface modification. Gelest. Inc. 2011, 215, 547–1015. [Google Scholar]
- Lee, B.; Kim, Y.; Lee, H.; Yi, J. Synthesis of functionalized porous silicas via templating method as heavy metal ion adsorbents: The introduction of surface hydrophilicity onto the surface of adsorbents. Microporous Mesoporous Mater. 2001, 50, 77–90. [Google Scholar] [CrossRef]
- Narasimhan, T.N. Of wetting and osmotic transport. Phys. Today 2010, 63, 60. [Google Scholar] [CrossRef]
- Amin, M.; Hafez, A.; Shaaban, A.; Abdelmonem, N.; Hanafy, M. Removal of Reactive Dyes from Dye-house Effluent Using Nanofiltration Membrane. Egypt. Soc. Chem. Eng. 2011, 37, 1–20. [Google Scholar]
- Mukherjee, A.; Faruque, H.M.R.; Islam, M.S.; Bhuiyan, A.G.; Hashimoto, A. Permeability Analysis of Pure Water across Nano Porous Graphene. In Proceedings of the 2019 International Conference on Electrical, Computer and Communication Engineering (ECCE), Cox’sBazar, Bangladesh, 7–9 February 2019; IEEE: Cox’sBazar, Bangladesh, 2019; pp. 1–4. [Google Scholar]
- Jahanshahi, D.; Vahid, B.; Azamat, J. Computational study on the ability of functionalized graphene nanosheet for nitrate removal from water. Chem. Phys. 2018, 511, 20–26. [Google Scholar] [CrossRef]
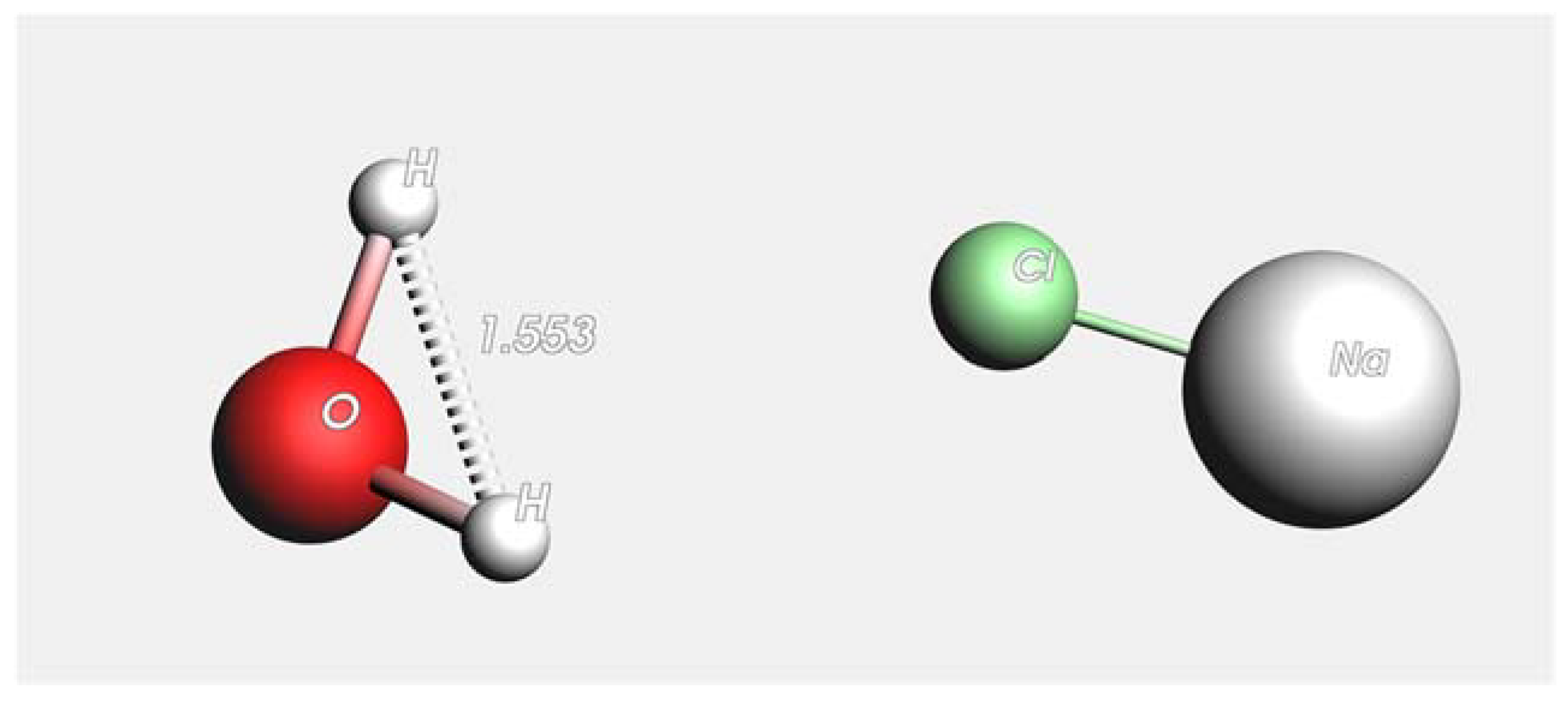

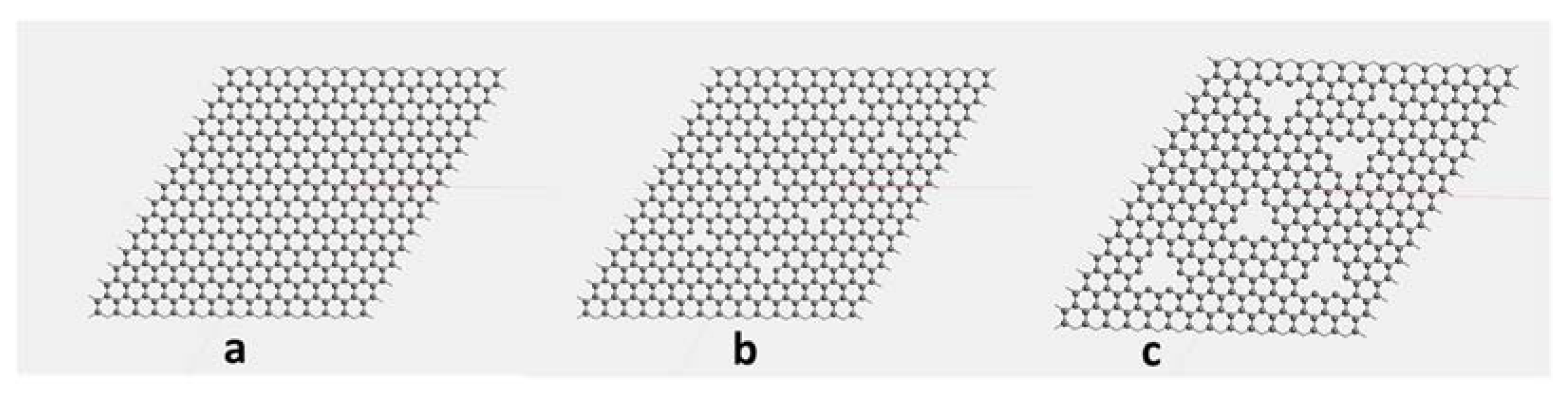
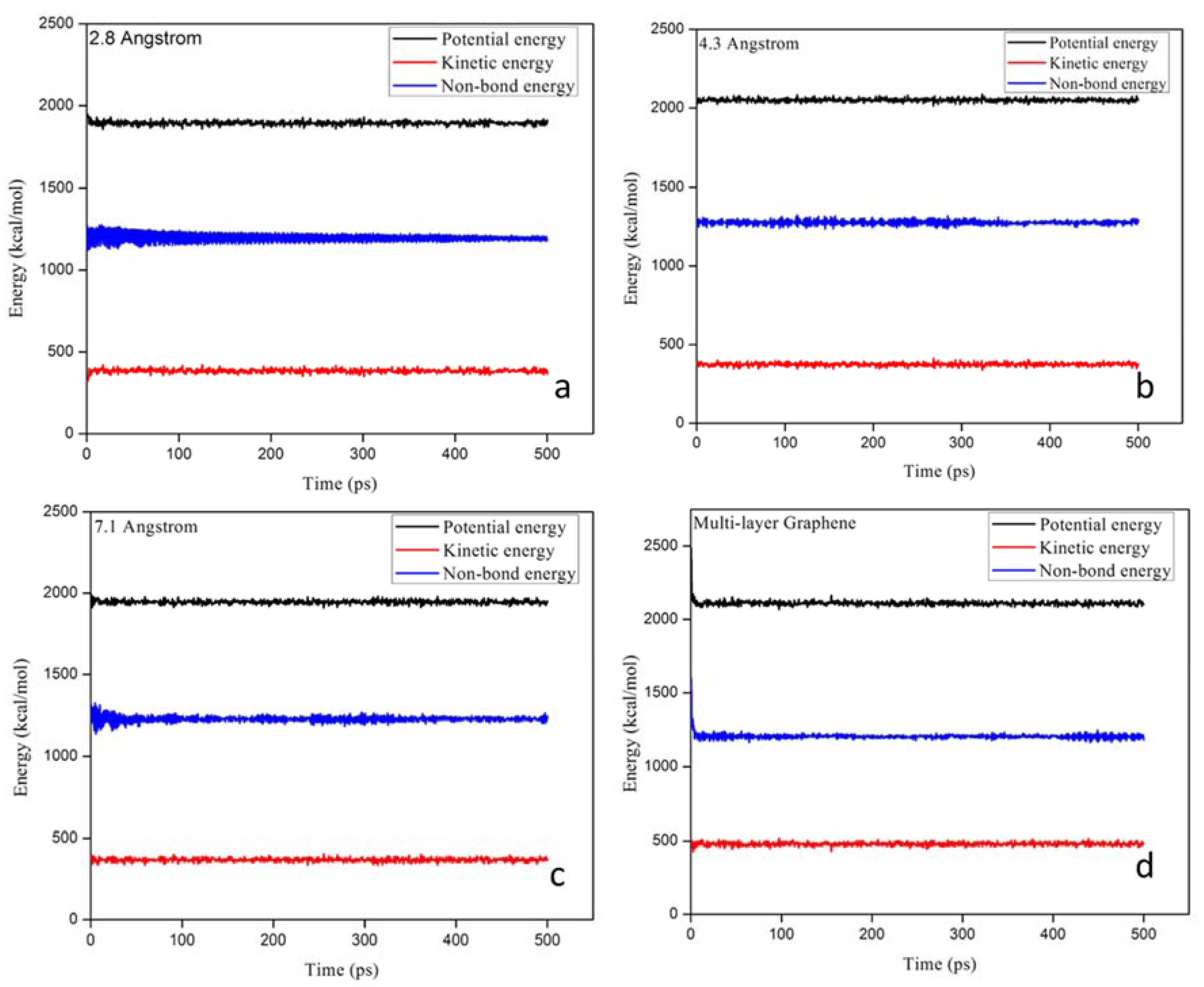


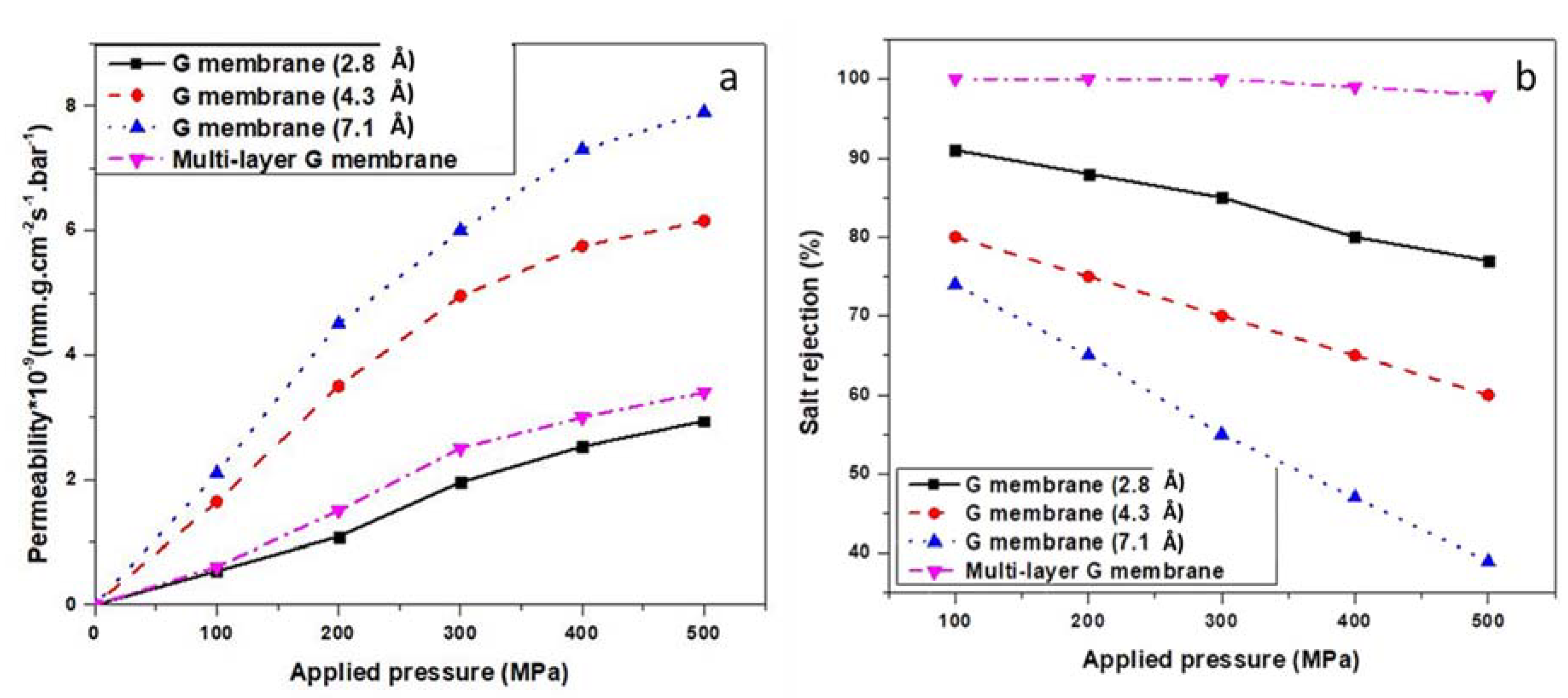
| Membrane Layer | Pore Size (Å) | Potential Energy (kcal/mol) | Kinetic Energy (kcal/mol) | Non-Bond Energy (kcal/mol) | |||
|---|---|---|---|---|---|---|---|
| Initial | Under Pressure | Initial | Under Pressure | Initial | Under Pressure | ||
| Single layer | 2.8 | 1698 | 1912 | 384 | 365 | 1270 | 1190 |
| Single layer | 4.3 | 2039 | 2049 | 374 | 375 | 1500 | 1290 |
| Single layer | 7.1 | 2038 | 1945 | 368 | 369 | 1310 | 1240 |
| Multi-layer | 7.1, 4.3, 2.8 | 2578 | 2102 | 480 | 487 | 1588 | 1221 |
| Simulation Method | Applied Pressure (MPa) | Pore Size (Å) | Salt Rejection (%) | Water Permeability | Material | References |
|---|---|---|---|---|---|---|
| NPT Berendsen | 50 | 2.8 | 95 (285 NaCl molecules blocked) | 0.347 × 10−9 (mm.g.cm−2s−1.bar−1) 106 H2O molecules filtered | Single layer graphene | This paper |
| NPT Berendsen | 500 | 2.8 | 77 (231 NaCl molecules blocked) | 2.94 × 10−9 (mm.g.cm−2s−1.bar−1) 900 H2O molecules filtered | Single layer graphene | This paper |
| NPT Berendsen | 500 | 4.3 | 60 (180 NaCl molecules blocked) | 6.154 × 10−9 (mm.g.cm−2s−1.bar−1) 2430 H2O molecules filtered | Single layer graphene | This paper |
| NPT Berendsen | 500 | 7.1 | 39 (117 NaCl molecules blocked) | 7.9 × 10−9 (mm.g.cm−2s−1.bar−1) 2850 H2O molecules filtered | Single layer graphene | This paper |
| NPT Berendsen | 100 | 2.8 | 91 (273 NaCl molecules blocked) | 0.541 × 10−9 (mm.g.cm−2s−1.bar−1) 180 H2O molecules filtered | Single layer graphene | This paper |
| NPT Berendsen | 100 | 7.1, 4.3, 2.8 | 100 (All NaCl molecules blocked) | 0.61 × 10−9 (mm.g.cm−2s−1.bar−1) 197 H2O molecules filtered | Multi-layer graphene membrane | This paper |
| NVE integration | 1.1 × 10−4 | 3 | Not reported | Only 1 H2O molecules passed | Graphene | [35] |
| NVE integration | 240 | 6 | Not reported | 24 H2O molecules passed | Graphene | [35] |
| NVE integration | 140 | 8 | Not reported | 56 H2O molecules passed | Graphene | [35] |
| NVT ensemble | 100 | Not reported | 5 ions permeated | 900–1000 (water molecules filtered) | Graphene | [36] |
| NVT ensemble | Rigid piston | 3.3 | 98.35 | 31.9 (g/s cm2) | Three graphene layer membrane | [19] |
| NVT ensemble | Rigid piston | 4 | 68.4 | 50.5 (g/s·cm2) | Three graphene layer membrane | [19] |
| NVT ensemble | Rigid piston | 5 | 55 | 55.7 (g/s·cm2) | Three graphene layer membrane | [19] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ibrahim, Q.; Akbarzadeh, R.; Gharbia, S.S.; Ndungu, P.G. Design of Multi-Layer Graphene Membrane with Descending Pore Size for 100% Water Desalination by Simulation Using ReaxFF. Membranes 2022, 12, 1038. https://doi.org/10.3390/membranes12111038
Ibrahim Q, Akbarzadeh R, Gharbia SS, Ndungu PG. Design of Multi-Layer Graphene Membrane with Descending Pore Size for 100% Water Desalination by Simulation Using ReaxFF. Membranes. 2022; 12(11):1038. https://doi.org/10.3390/membranes12111038
Chicago/Turabian StyleIbrahim, Qusai, Rokhsareh Akbarzadeh, Salem S. Gharbia, and Patrick Gathura Ndungu. 2022. "Design of Multi-Layer Graphene Membrane with Descending Pore Size for 100% Water Desalination by Simulation Using ReaxFF" Membranes 12, no. 11: 1038. https://doi.org/10.3390/membranes12111038
APA StyleIbrahim, Q., Akbarzadeh, R., Gharbia, S. S., & Ndungu, P. G. (2022). Design of Multi-Layer Graphene Membrane with Descending Pore Size for 100% Water Desalination by Simulation Using ReaxFF. Membranes, 12(11), 1038. https://doi.org/10.3390/membranes12111038









