Multiphysics Modeling and Analysis of a Solar Desalination Process Based on Vacuum Membrane Distillation
Abstract
1. Introduction
2. Materials and Methods
2.1. Exergy Balance
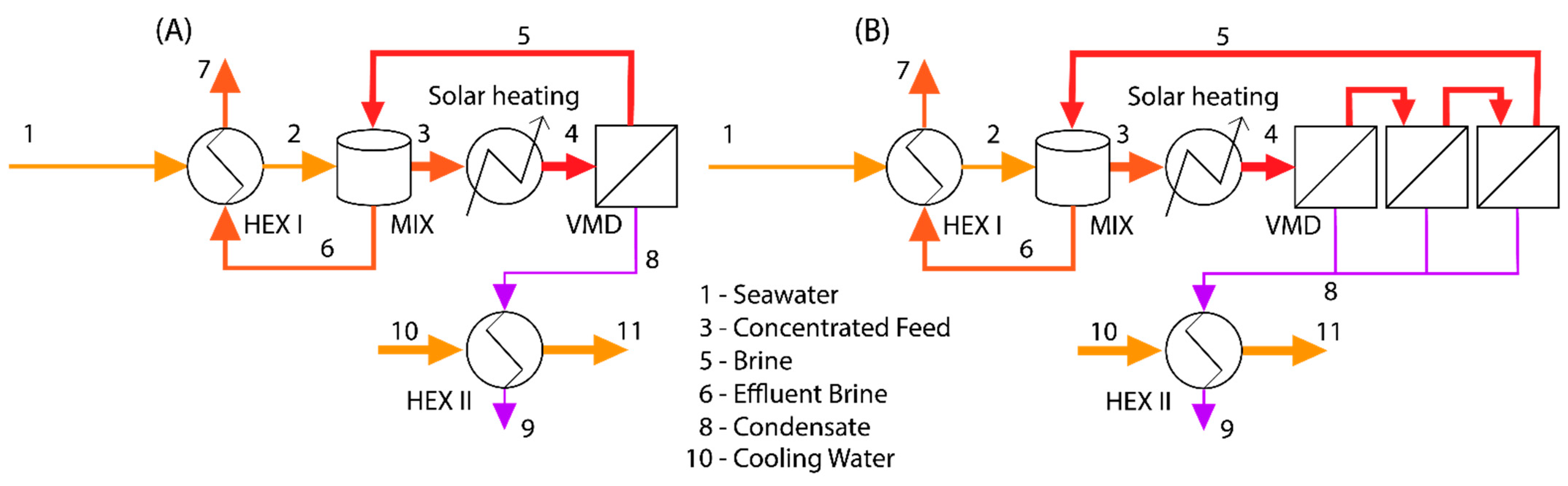
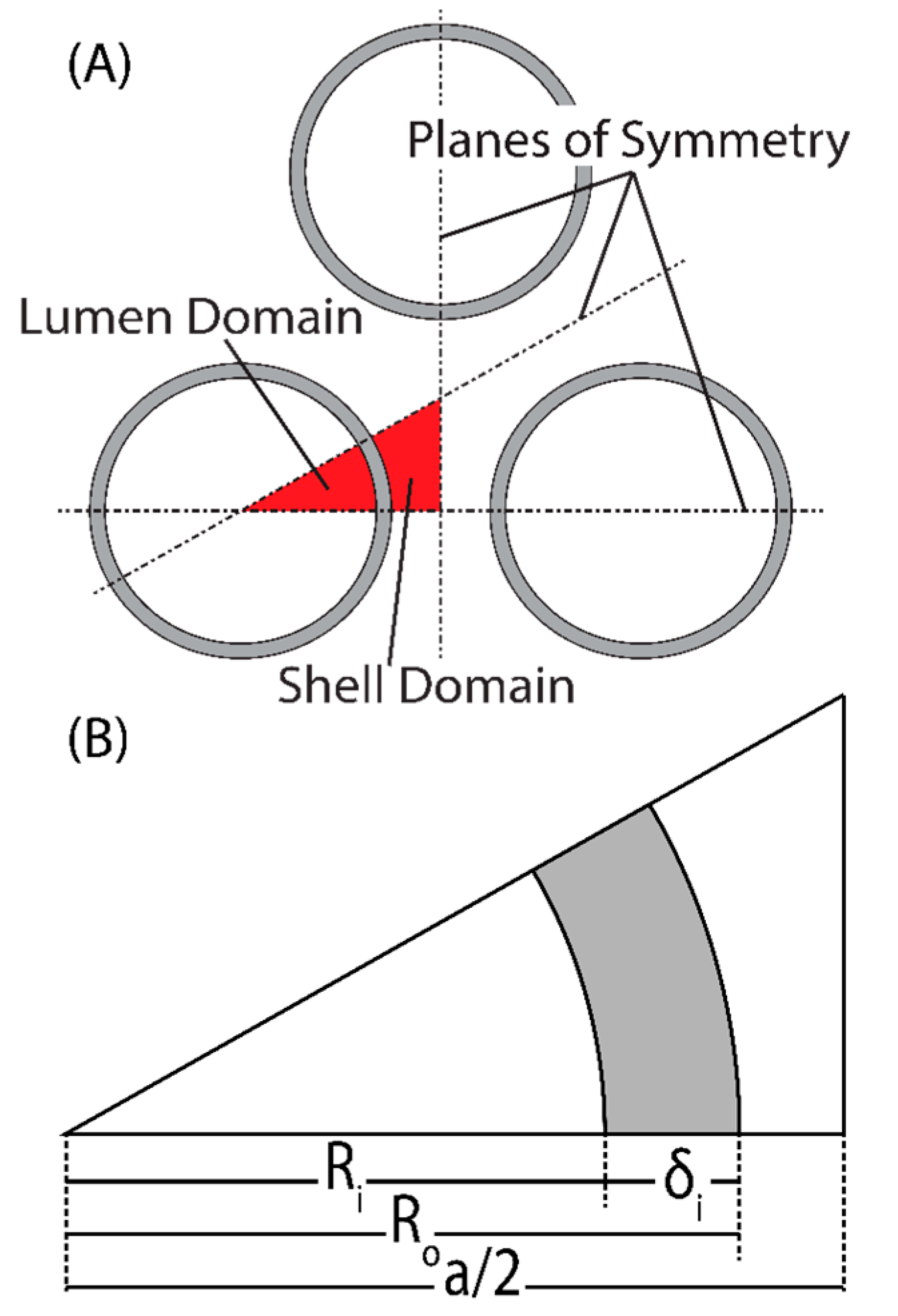
2.2. Geometry Definition
2.3. Momentum Transfer Governing Equations and Boundary Conditions
2.4. Mass Transfer Governing Equations and Boundary Conditions
2.5. Heat-Transfer-Governing Equations and Boundary Conditions
3. Results
3.1. Effects of Different Membrane Parameters
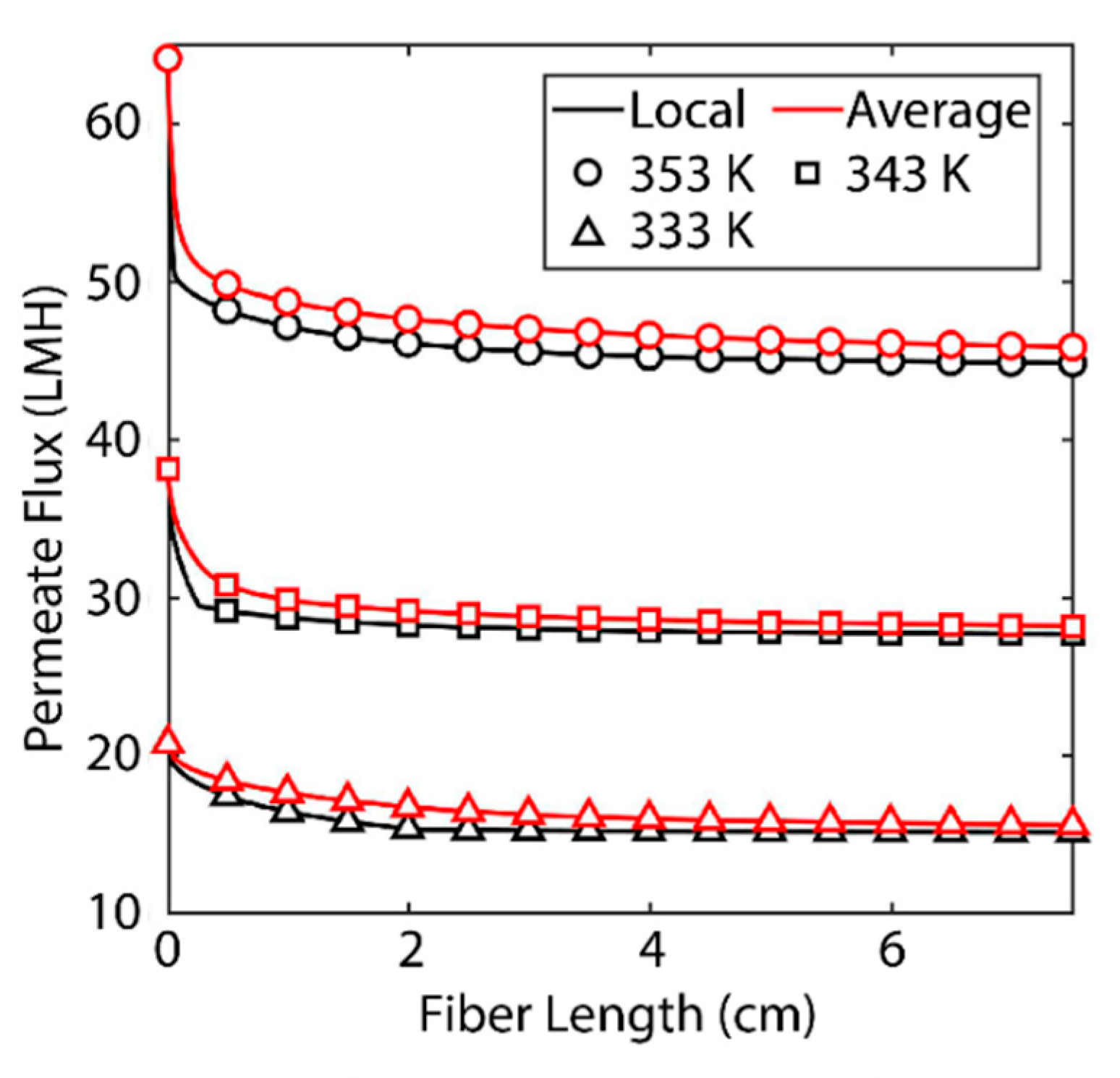
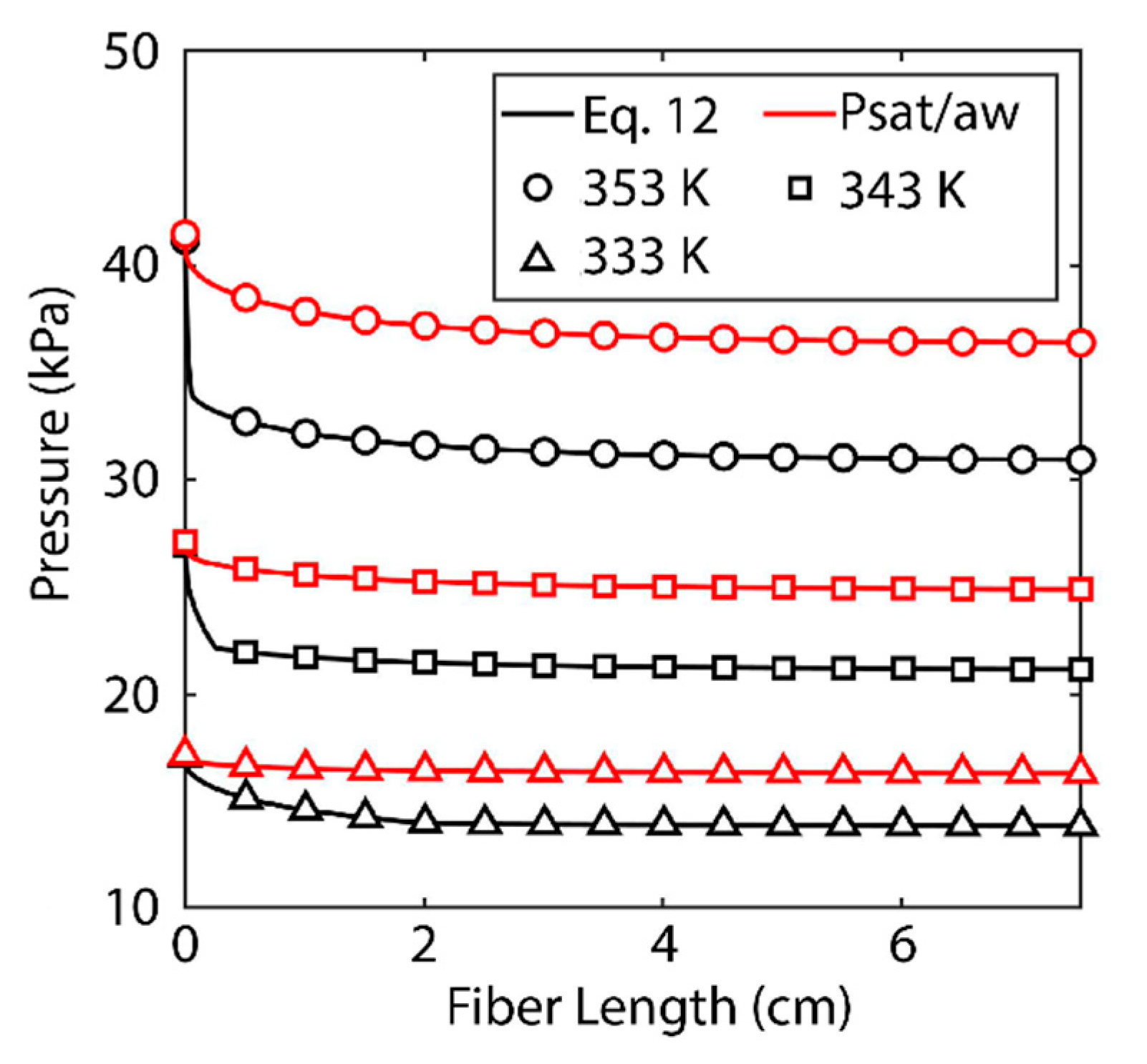
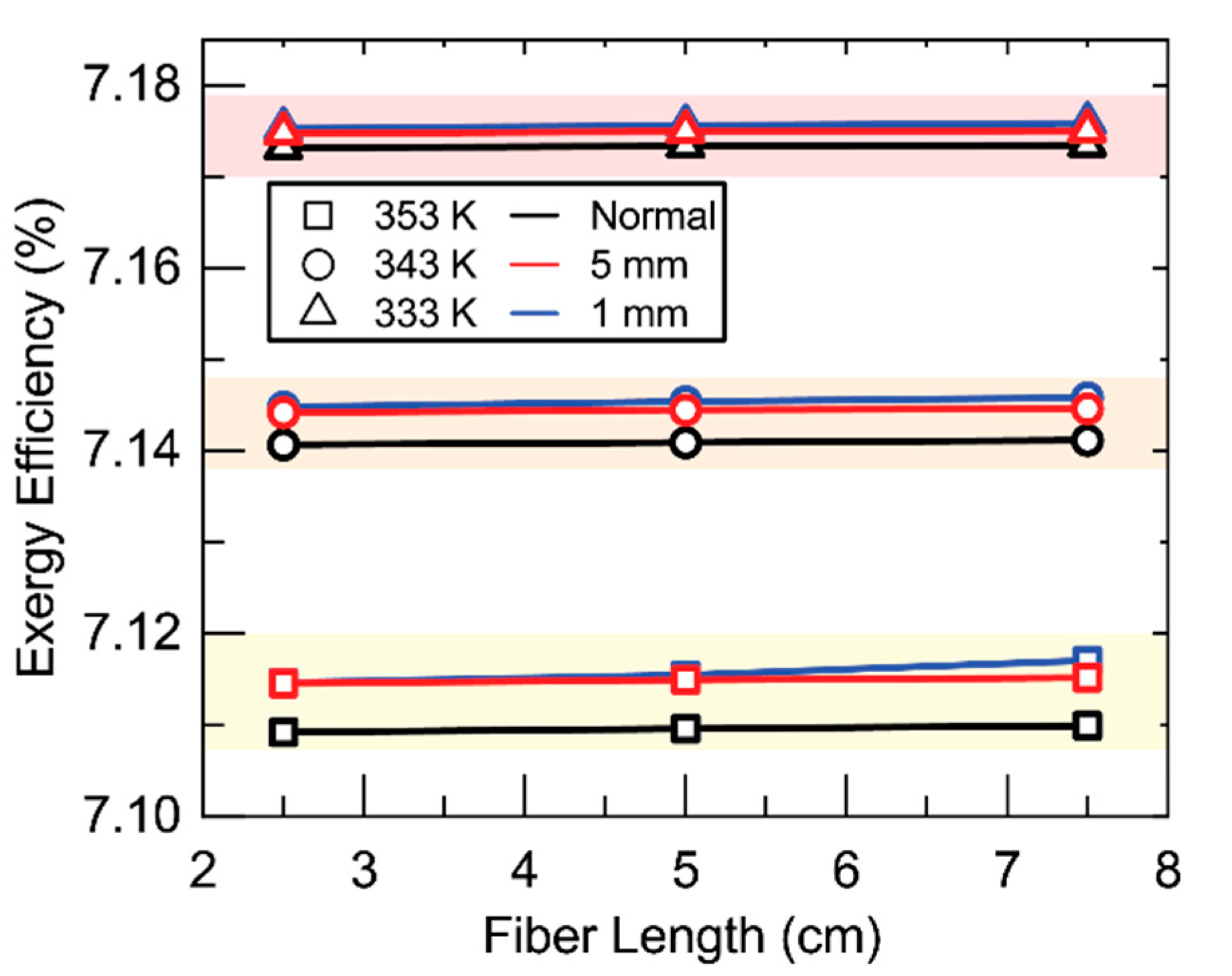
3.1.1. Effect of Fiber Length
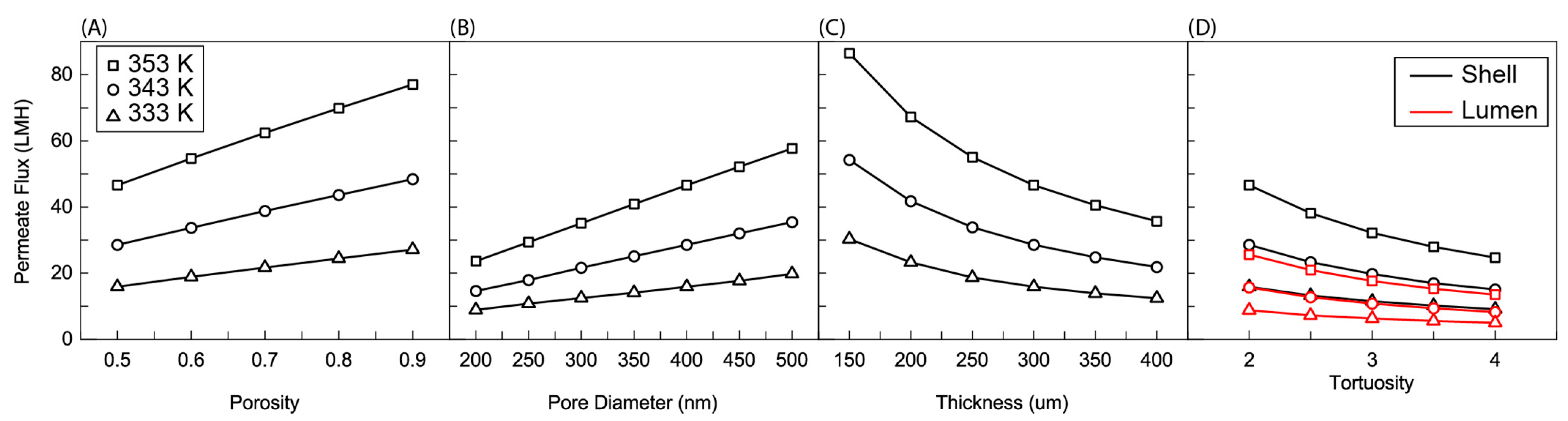
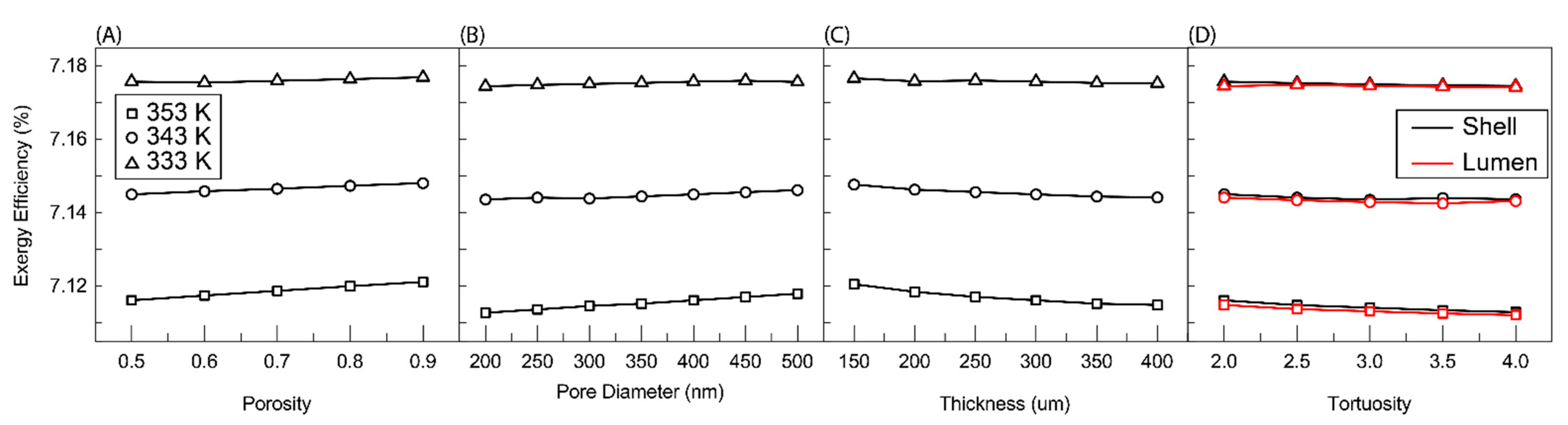
3.1.2. Effect of Porosity
3.1.3. Effect of Pore Diameter
3.1.4. Effect of Thickness
3.1.5. Effect of Tortuosity
3.2. Limiting Phenomena
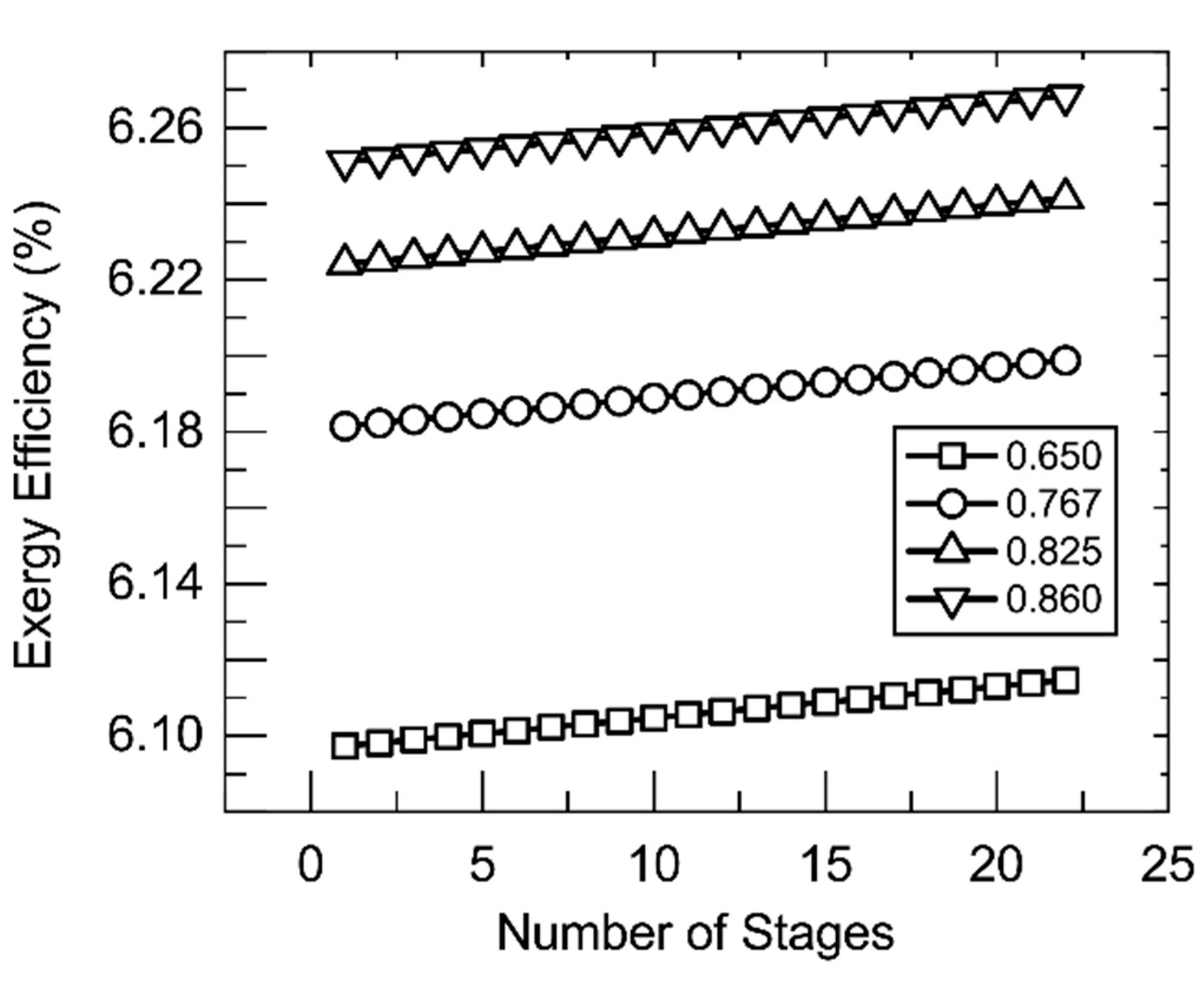
3.3. Effect of Baffling Design
3.4. Exergy Efficiency
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Terms | Definition |
| Membrane thickness | |
| Membrane porosity | |
| Exergy efficiency | |
| Membrane permeability | |
| Dynamic viscosity of fluid | |
| Dynamic viscosity of the feed stream | |
| Specific Gibbs free energy of the dead state | |
| Density of feed stream | |
| Membrane tortuosity | |
| Mass fraction of component | |
| Mass fraction of salt at the inlet | |
| Fiber spacing parameter | |
| Activity coefficient of water | |
| Total non-flow exergy | |
| Specific flow exergy of stream | |
| Knudsen diffusion coefficient | |
| Poiseuille diffusion coefficient | |
| Heat capacity of fluid | |
| Total diffusion coefficient | |
| Mean pore diameter | |
| Mixture average diffusion coefficient of component “i” | |
| Diffusion coefficient for salt in water | |
| Kinetic energy | |
| Potential energy | |
| Total exergy loss due to system irreversibility | |
| Solar exergy flux | |
| Total Thermal Exergy Flux | |
| Specific enthalpy of dead state | |
| Specific enthalpy of stream “i” | |
| Convective heat transfer coefficient | |
| Heat of vaporization of water | |
| Identity tensor | |
| Mixture diffusion correction term | |
| Diffusive flux of component in the feed stream | |
| Thermal conductivity of feed stream | |
| Length of membrane module | |
| Mass flowrate of stream | |
| Molar mass of component in membrane feed | |
| Mean molar mass of the feed stream | |
| Number of fibers in a module | |
| Normal vector | |
| Mass flux of water vapor across membrane | |
| Total Number of Streams entering and leaving | |
| Total mass flux of component “i” within the membrane feed stream | |
| Total mass flux of water within the membrane feed stream | |
| Dead state pressure | |
| Pressure of the feed stream | |
| Transmembrane change in pressure | |
| Average pressure within the membrane | |
| Saturation pressure of water | |
| Vacuum pressure | |
| Volumetric flowrate | |
| Conductive heat flux in the membrane feed stream | |
| Transmembrane heat flux | |
| Solar energy | |
| Radial spatial variable | |
| Ideal gas constant | |
| Inner radius of the membrane | |
| Specific Gas constant for component “k” | |
| Outer radius of membrane | |
| Specific entropy of stream “i” | |
| Dead state-specific entropy | |
| Entropy | |
| Dead State temperature | |
| Feed stream temperature | |
| Feed temperature at inlet | |
| Feed stream temperature at membrane interface | |
| Temperature of the Sun | |
| Feed velocity | |
| Feed velocity at inlet | |
| Internal Energy | |
| Volume | |
| Mechanical Power | |
| Mole fraction of component k | |
| Dead state mole fraction of component k | |
| Mole fraction of salt in feed stream | |
| Axial spatial variable |
References
- Kummu, M.; Guillaume, J.H.A.; De Moel, H.; Eisner, S.; Flörke, M.; Porkka, M.; Siebert, S.; Veldkamp, T.I.E.; Ward, P.J. The world’s road to water scarcity: Shortage and stress in the 20th century and pathways towards sustainability. Sci. Rep. 2016, 6, 38495. [Google Scholar] [CrossRef]
- Ng, P.J.H.; Teo, C. Singapore’s water challenges past to present. Int. J. Water Resour. Dev. 2019, 36, 269–277. [Google Scholar] [CrossRef]
- El-Nashar, A.M.; Samad, M. The solar desalination plant in Abu Dhabi: 13 years of performance and operation history. Renew. Energy 1998, 14, 263–274. [Google Scholar] [CrossRef]
- Ghaffour, N.; Bundschuh, J.; Mahmoudi, H.; Goosen, M.F. Renewable energy-driven desalination technologies: A comprehensive review on challenges and potential applications of integrated systems. Desalination 2015, 356, 94–114. [Google Scholar] [CrossRef]
- Peñate, B.; Rodríguez, M.D.L.G. Current trends and future prospects in the design of seawater reverse osmosis desalination technology. Desalination 2012, 284, 1–8. [Google Scholar] [CrossRef]
- Aboelmaaref, M.M.; Zayed, M.E.; Zhao, J.; Li, W.; Askalany, A.A.; Ahmed, M.S.; Ehab, S.A. Hybrid Solar Desalination Systems Driven by Parabolic Trough and Parabolic Dish CSP Technologies: Technology Categorization, Thermodynamic Performance and Economical Assessment. Energy Convers. Manag. 2020, 220, 33. [Google Scholar] [CrossRef]
- Deshmukh, A.; Boo, C.; Karanikola, V.; Lin, S.; Straub, A.P.; Tong, T.; Warsinger, D.M.; Elimelech, M. Membrane distillation at the water-energy nexus: Limits, opportunities, and challenges. Energy Environ. Sci. 2018, 11, 1177–1196. [Google Scholar] [CrossRef]
- Wang, P.; Chung, T.-S. Recent advances in membrane distillation processes: Membrane development, configuration design and application exploring. J. Membr. Sci. 2015, 474, 39–56. [Google Scholar] [CrossRef]
- Drioli, E.; Ali, A.; Macedonio, F. Membrane distillation: Recent developments and perspectives. Desalination 2015, 356, 56–84. [Google Scholar] [CrossRef]
- Ghaleni, M.M.; Al Balushi, A.; Bavarian, M.; Nejati, S. Omniphobic Hollow Fiber Membranes for Water Recovery and Desalination. ACS Appl. Polym. Mater. 2020, 2, 3034–3038. [Google Scholar] [CrossRef]
- Warsinger, D.M.; Swaminathan, J.; Guillen-Burrieza, E.; Arafat, H.A. Scaling and fouling in membrane distillation for desalination applications: A review. Desalination 2015, 356, 294–313. [Google Scholar] [CrossRef]
- Li, Q.; Omar, A.; Cha-Umpong, W.; Liu, Q.; Li, X.; Wen, J.; Wang, Y.; Razmjou, A.; Guan, J.; Taylor, R.A. The potential of hollow fiber vacuum multi-effect membrane distillation for brine treatment. Appl. Energy 2020, 276, 115437. [Google Scholar] [CrossRef]
- El Kadi, K.; Janajreh, I.; Hashaikeh, R. Numerical simulation and evaluation of spacer-filled direct contact membrane distillation module. Appl. Water Sci. 2020, 10, 1–17. [Google Scholar] [CrossRef]
- Lou, Y.; Gogar, R.; Hao, P.; Lipscomb, G.; Amo, K.; Kniep, J. Simulation of net spacers in membrane modules for carbon dioxide capture. Sep. Sci. Technol. 2016, 52, 168–185. [Google Scholar] [CrossRef]
- Wang, Z.; Horseman, T.; Straub, A.P.; Yip, N.Y.; Li, D.; Elimelech, M.; Lin, S. Pathways and challenges for efficient solar-thermal desalination. Sci. Adv. 2019, 5, eaax0763. [Google Scholar] [CrossRef] [PubMed]
- Demirel, Y. Nonequilibrium Thermodynamics, 2nd ed.; Elsevier B.V.: Amsterdam, The Netherlands, 2007. [Google Scholar]
- Signorato, F.; Morciano, M.; Bergamasco, L.; Fasano, M.; Asinari, P. Exergy analysis of solar desalination systems based on passive multi-effect membrane distillation. Energy Rep. 2020, 6, 445–454. [Google Scholar] [CrossRef]
- Kirk-Othmer Encyclopedia of Chemical Technology, 5th ed.; John Wiley & Sons: Hoboken, NJ, USA, 2004; Volume 13.
- Holmgren, M. X Steam, Thermodynamic Properties of Water and Steam. Available online: https://www.mathworks.com/matlabcentral/fileexchange/9817-x-steam-thermodynamic-properties-of-water-and-steam (accessed on 16 April 2021).
- Nayar, K.G.; Sharqawy, M.H.; Banchik, L.D. Thermophysical properties of seawater: A review and new correlations that include pressure dependence. Desalination 2016, 390, 1–24. [Google Scholar] [CrossRef]
- Sharqawy, M.H.; Lienhard, J.H.; Zubair, S.M. Thermophysical properties of seawater: A review of existing correlations and data. Desalination Water Treat. 2010, 16, 354–380. [Google Scholar] [CrossRef]
- Zhang, J.; Li, J.-D.; Duke, M.; Hoang, M.; Xie, Z.; Groth, A.; Tun, C.; Gray, S. Modelling of vacuum membrane distillation. J. Membr. Sci. 2013, 434, 1–9. [Google Scholar] [CrossRef]
- Alklaibi, A.M.; Lior, N. Membrane-distillation desalination: Status and potential. Desalination 2005, 171, 111–131. [Google Scholar] [CrossRef]
- Alkhudhiri, A.; Darwish, N.; Hilal, N. Membrane distillation: A comprehensive review. Desalination 2012, 287, 2–18. [Google Scholar] [CrossRef]
- Wilkes, J.O. Fluid Mechanics for Chemical Engineers with Microfluidics and CFD, 2nd ed.; Pearson Education Inc.: Upper Saddle River, NJ, USA, 2006. [Google Scholar]
- Bird, R.B.; Stewart, W.E.; Lightfoot, E.N. Transport Phenomena; John Wiley & Sons: Hoboken, NJ, USA, 1960. [Google Scholar]
- Zhang, Y.; Peng, Y.; Ji, S.; Wang, S. Numerical simulation of 3D hollow-fiber vacuum membrane distillation by computational fluid dynamics. Chem. Eng. Sci. 2016, 152, 172–185. [Google Scholar] [CrossRef]
- Abu-Zeid, M.A.E.-R.; Zhang, Y.; Dong, H.; Zhang, L.; Chen, H.-L.; Hou, L. A comprehensive review of vacuum membrane distillation technique. Desalination 2015, 356, 1–14. [Google Scholar] [CrossRef]
- Lawson, K.W.; Lloyd, D.R. Membrane distillation. J. Membr. Sci. 1997, 124, 1–25. [Google Scholar] [CrossRef]
- Schofield, R.W. Membrane Distillation. Ph.D. Thesis, Univeristy of New South Wales, Sydney, Australia, 1989. [Google Scholar]
- Chemical Reaction Engineering Module User’s Guide, COMSOL Multiphysics® v. 5.4; COMSOL AB: Stockholm, Sweden, 2018.
- Millero, F.J.; Feistel, R.; Wright, D.G.; McDougall, T.J. The composition of Standard Seawater and the definition of the Reference-Composition Salinity Scale. Deep. Sea Res. Part I Oceanogr. Res. Pap. 2008, 55, 50–72. [Google Scholar] [CrossRef]
- Vitagliano, V.; Lyons, P.A. Diffusion Coefficients for Aqueous Solutions of Sodium Chloride and Barium Chloride. J. Am. Chem. Soc. 1956, 78, 1549–1552. [Google Scholar] [CrossRef]
- Ghaleni, M.M.; Bavarian, M.; Nejati, S. Model-guided design of high-performance membrane distillation modules for water desalination. J. Membr. Sci. 2018, 563, 794–803. [Google Scholar] [CrossRef]
- Alkhudhiri, A.; Hilal, N. Membrane Distillation—Principles, Applications, Configurations, Design, and Implementation. In Emerging Technologies for Sustainable Desalination Handbook; Gude, V.G., Ed.; Butterworth-Heinemann: Oxford, UK, 2008. [Google Scholar]
- Blumm, J.; Lindemann, A. Characterization of the thermophysical properties of molten plymers and liquids using the flash technique. High Temp. Press. 2003, 35/36, 627–632. [Google Scholar] [CrossRef]
- Chang, Y.; Ooi, B.; Ahmad, A.; Leo, C.; Low, S. Vacuum membrane distillation for desalination: Scaling phenomena of brackish water at elevated temperature. Sep. Purif. Technol. 2021, 254, 117572. [Google Scholar] [CrossRef]
- Reverter, J.A.; Talo, S.; Alday, J. Las Palmas III—The success story of brine staging. Desalination 2001, 138, 207–217. [Google Scholar] [CrossRef]
- Magara, Y.; Kawasaki, M.; Sekino, M.; Yamamura, H. Development of reverse osmosis membrane seawater desalination in Japan. Water Sci. Technol. 2000, 41, 1–8. [Google Scholar] [CrossRef]
- Tong, T.; Elimelech, M. The Global Rise of Zero Liquid Discharge for Wastewater Management: Drivers, Technologies, and Future Directions. Environ. Sci. Technol. 2016, 50, 6846–6855. [Google Scholar] [CrossRef] [PubMed]
- Salmón, I.R.; Luis, P. Membrane crystallization via membrane distillation. Chem. Eng. Process. Process. Intensif. 2018, 123, 258–271. [Google Scholar] [CrossRef]


| Parameter | Value | Parameter | Value |
|---|---|---|---|
| 333–353 K | 200–500 nm | ||
| 5 kPa | 2–4 | ||
| 5 m s−1 | 0.5–0.9 | ||
| 350 μm | 2.5–7.5 cm | ||
| 150–400 μm | 0.35 |
| Scheme | Mass Flow Rate (kg h−1) | Mass Fraction of Salt % | Temperature (K) |
|---|---|---|---|
| 1 | 1 | 3.5 | 288 |
| 2 | 1 | 3.5 | 307.2 |
| 3 | 2041.3 | 10 | 352.8 |
| 4 | 2041.3 | 10 | 353 |
| 5 | 2040.7 | 10.003 | 352.8 |
| 6 | 0.35 | 10 | 352.8 |
| 7 | 0.35 | 10 | 298 |
| 8 | 0.65 | 0 | 350.5 |
| 9 | 0.65 | 0 | 306 |
| 10 | 50 | 3.5 | 288 |
| 11 | 50 | 3.5 | 295.8 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shuldes, B.N.; Bavarian, M.; Nejati, S. Multiphysics Modeling and Analysis of a Solar Desalination Process Based on Vacuum Membrane Distillation. Membranes 2021, 11, 386. https://doi.org/10.3390/membranes11060386
Shuldes BN, Bavarian M, Nejati S. Multiphysics Modeling and Analysis of a Solar Desalination Process Based on Vacuum Membrane Distillation. Membranes. 2021; 11(6):386. https://doi.org/10.3390/membranes11060386
Chicago/Turabian StyleShuldes, Benjamin N., Mona Bavarian, and Siamak Nejati. 2021. "Multiphysics Modeling and Analysis of a Solar Desalination Process Based on Vacuum Membrane Distillation" Membranes 11, no. 6: 386. https://doi.org/10.3390/membranes11060386
APA StyleShuldes, B. N., Bavarian, M., & Nejati, S. (2021). Multiphysics Modeling and Analysis of a Solar Desalination Process Based on Vacuum Membrane Distillation. Membranes, 11(6), 386. https://doi.org/10.3390/membranes11060386







