Interphase Surface Stability in Liquid-Liquid Membrane Contactors Based on Track-Etched Membranes
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
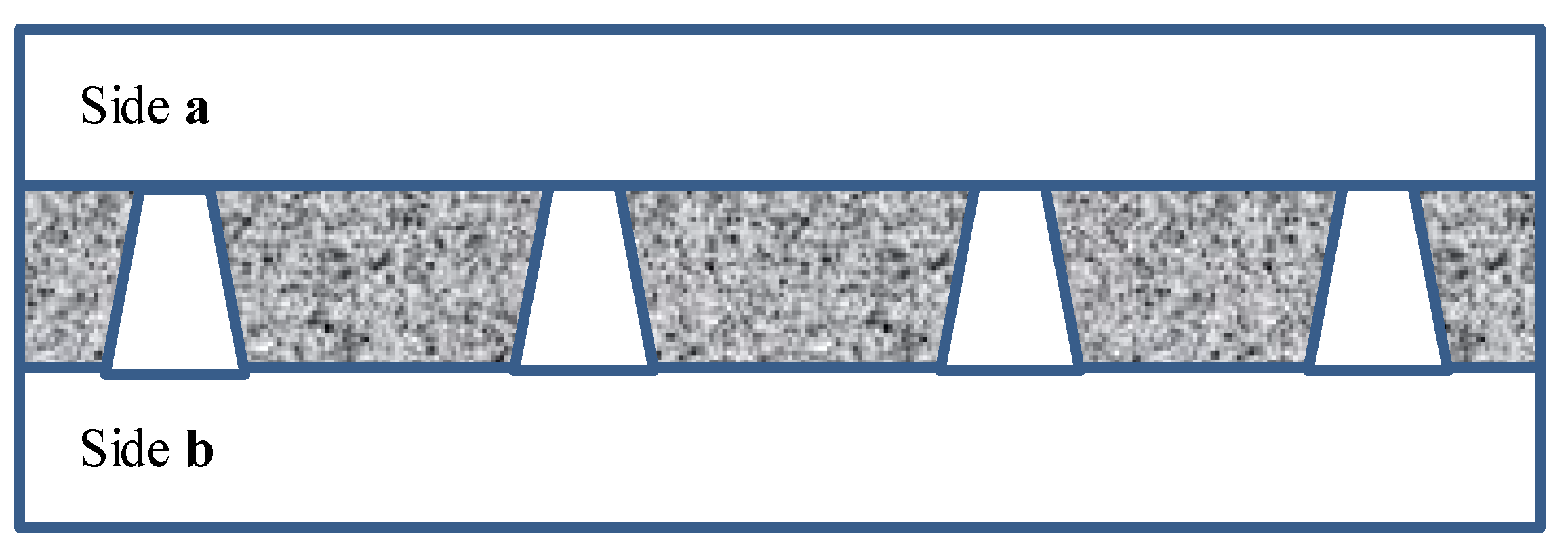
2.2. Track-Etched Membranes
2.3. Membrane Characterization
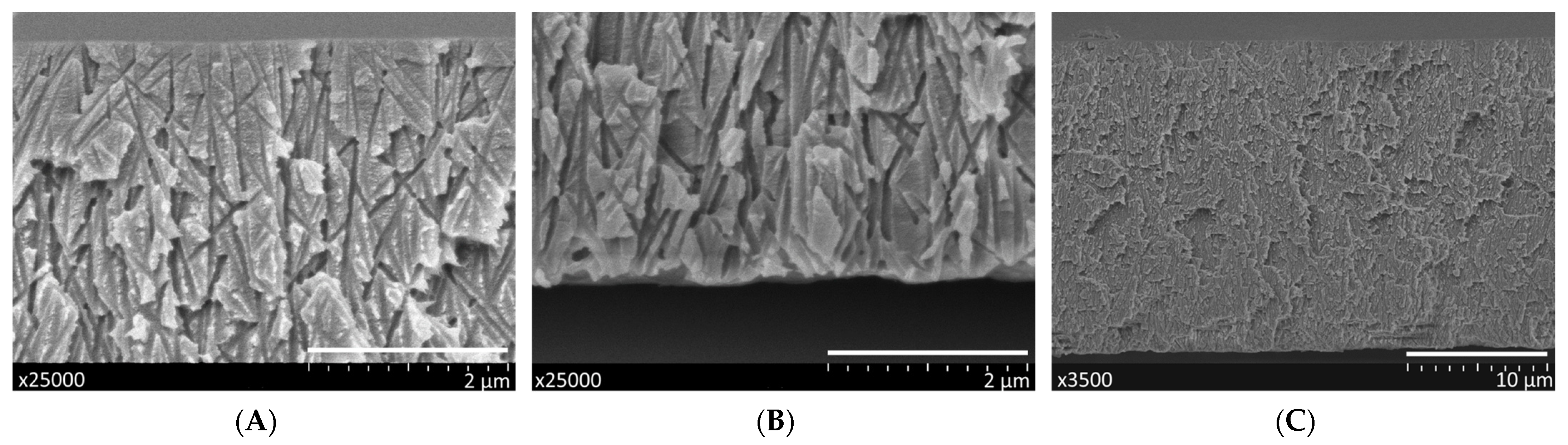
2.3.1. Scanning Electron Microscopy
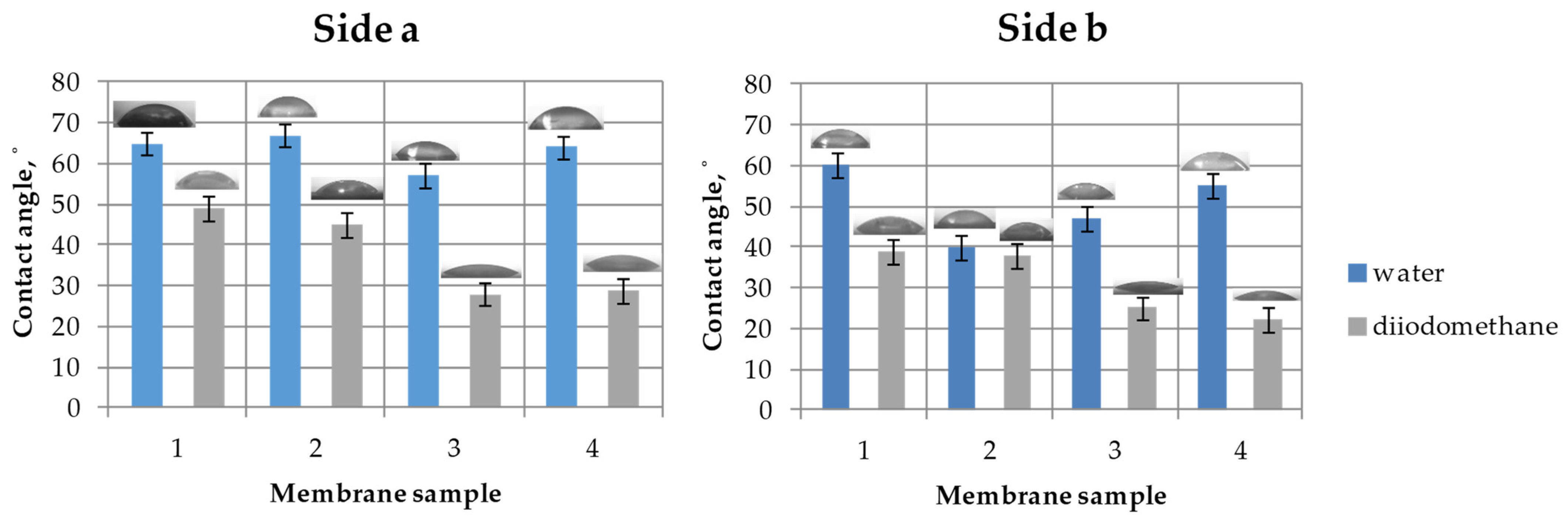
2.3.2. Surface Properties of the Membranes
2.3.3. Dynamic Light Scattering
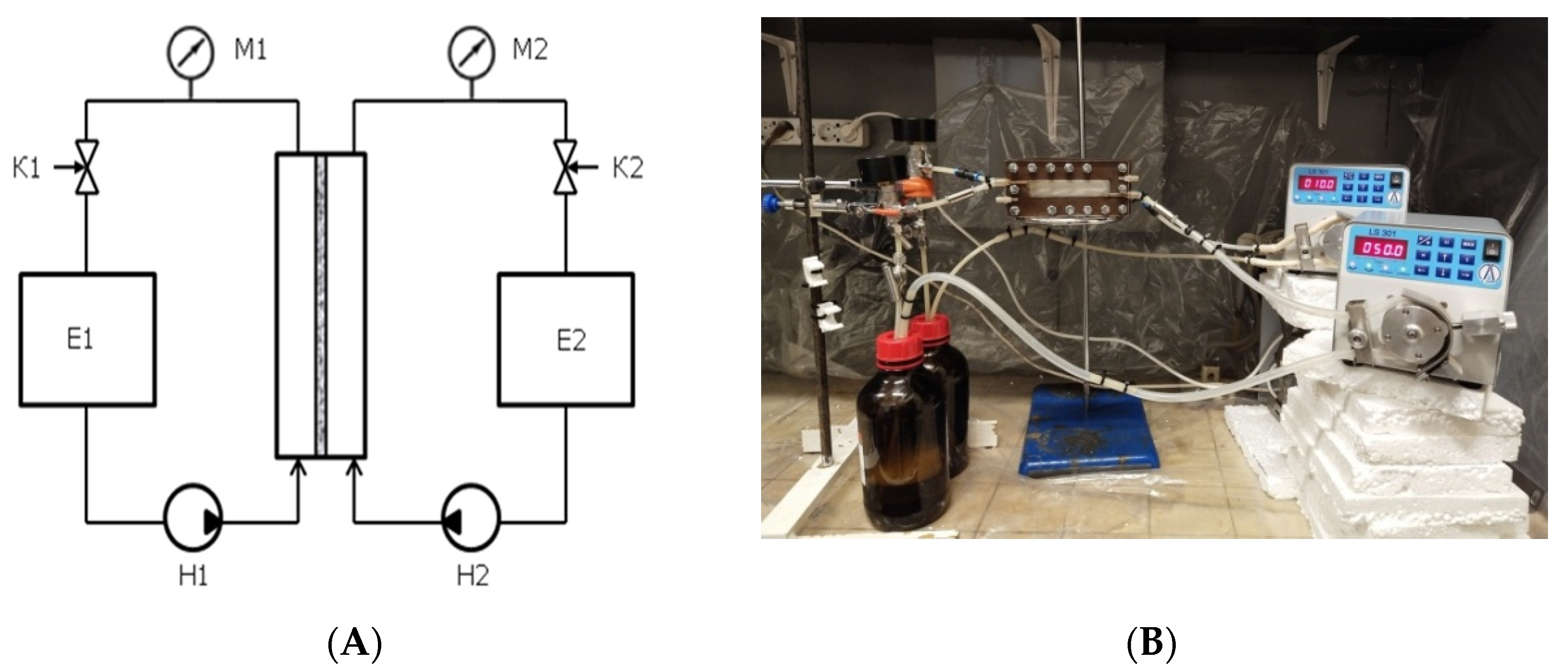
2.4. Liquid–Liquid Membrane Contactor
3. Results and Discussion
3.1. Track-Etched Membranes
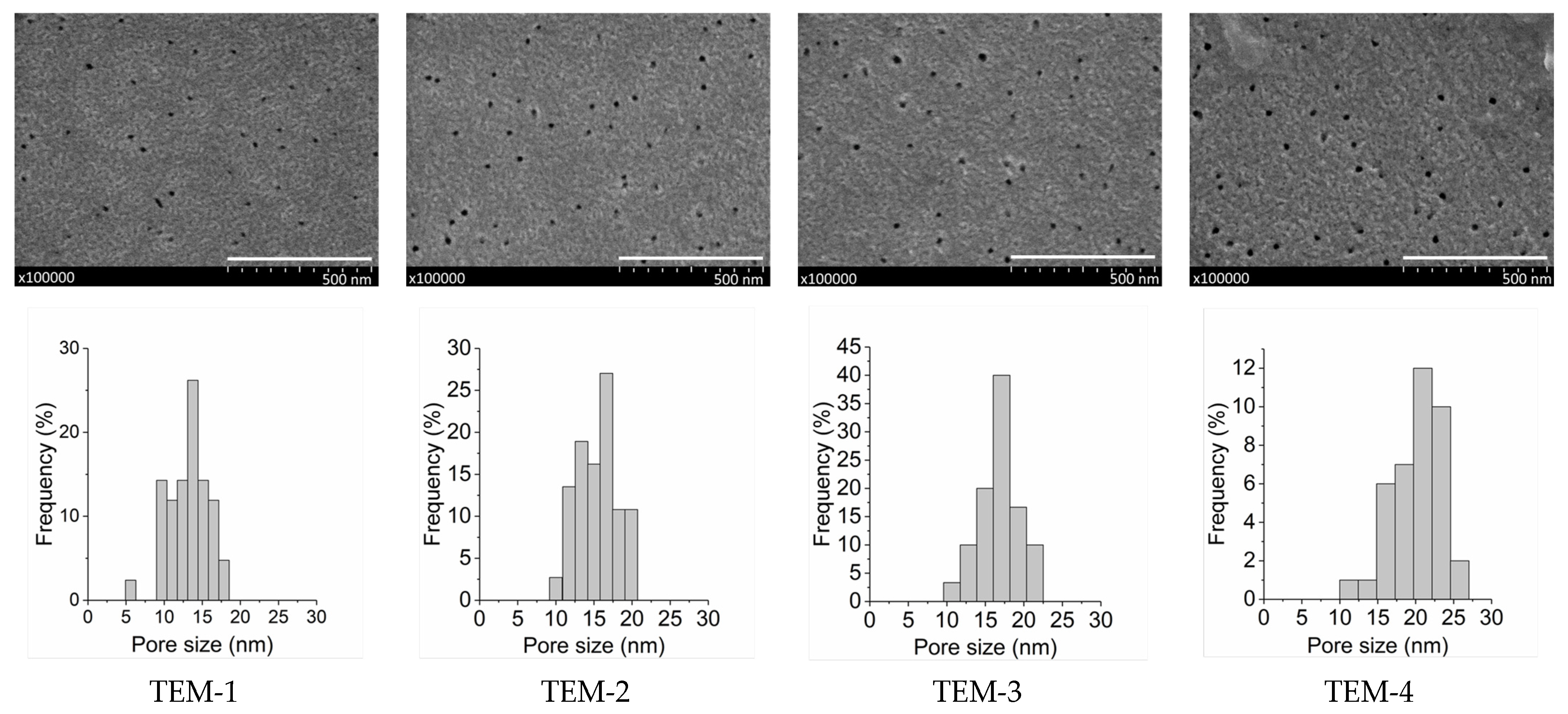
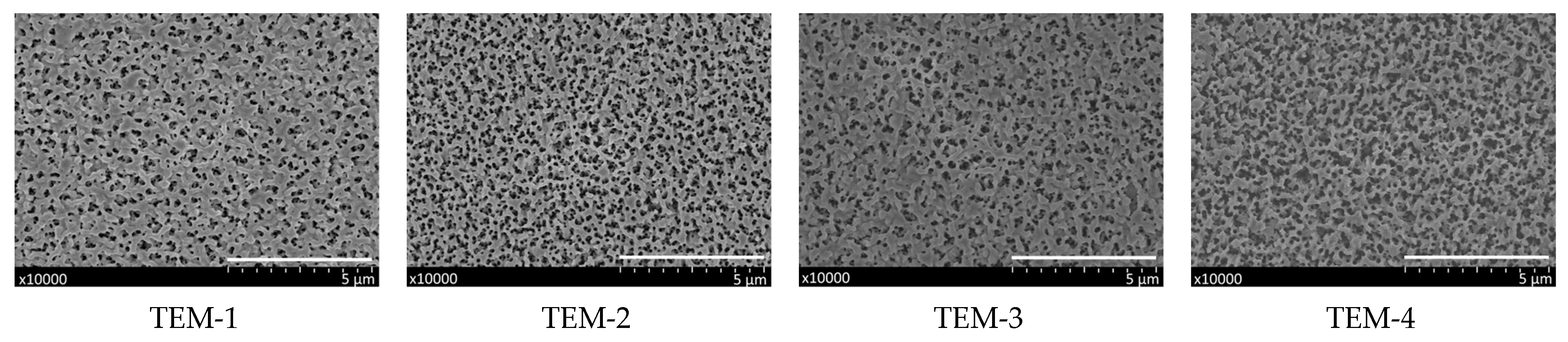
3.1.1. Characterization of Track-Etched Membranes Using SEM
3.1.2. Surface Properties of the Membranes
3.2. Liquid–Liquid Membrane Contactor System
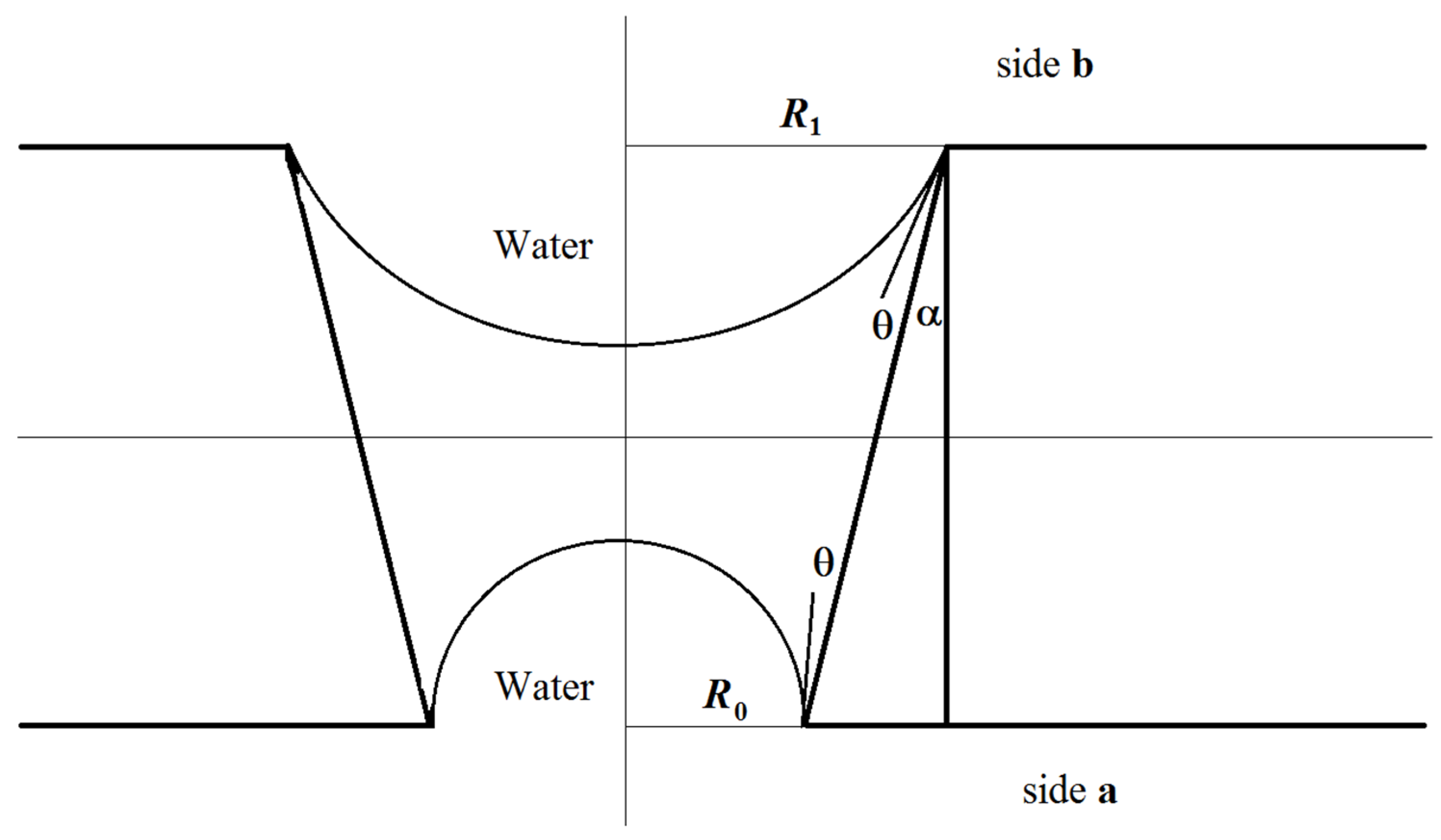
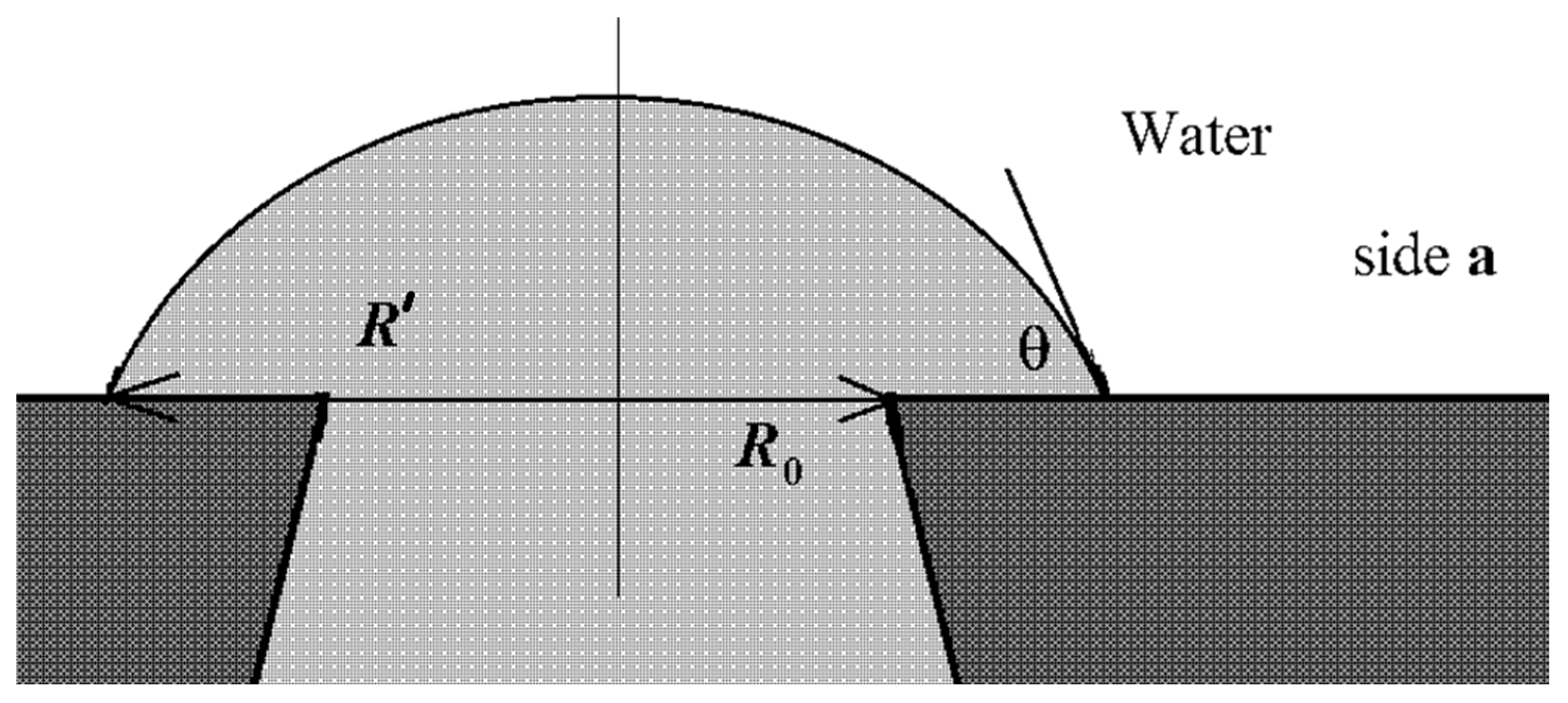
3.2.1. Influence of the Porous Structure of the Membrane on the Stability of the Phase Contact Interface
3.2.2. Influence of a Drop in Pressure between Phases on the Interphase Stability
3.2.3. Influence of the Parameters of the Membrane-Contactor System on the Stability of the Liquid–Liquid Interface
3.2.4. Discussion of Obtained Results
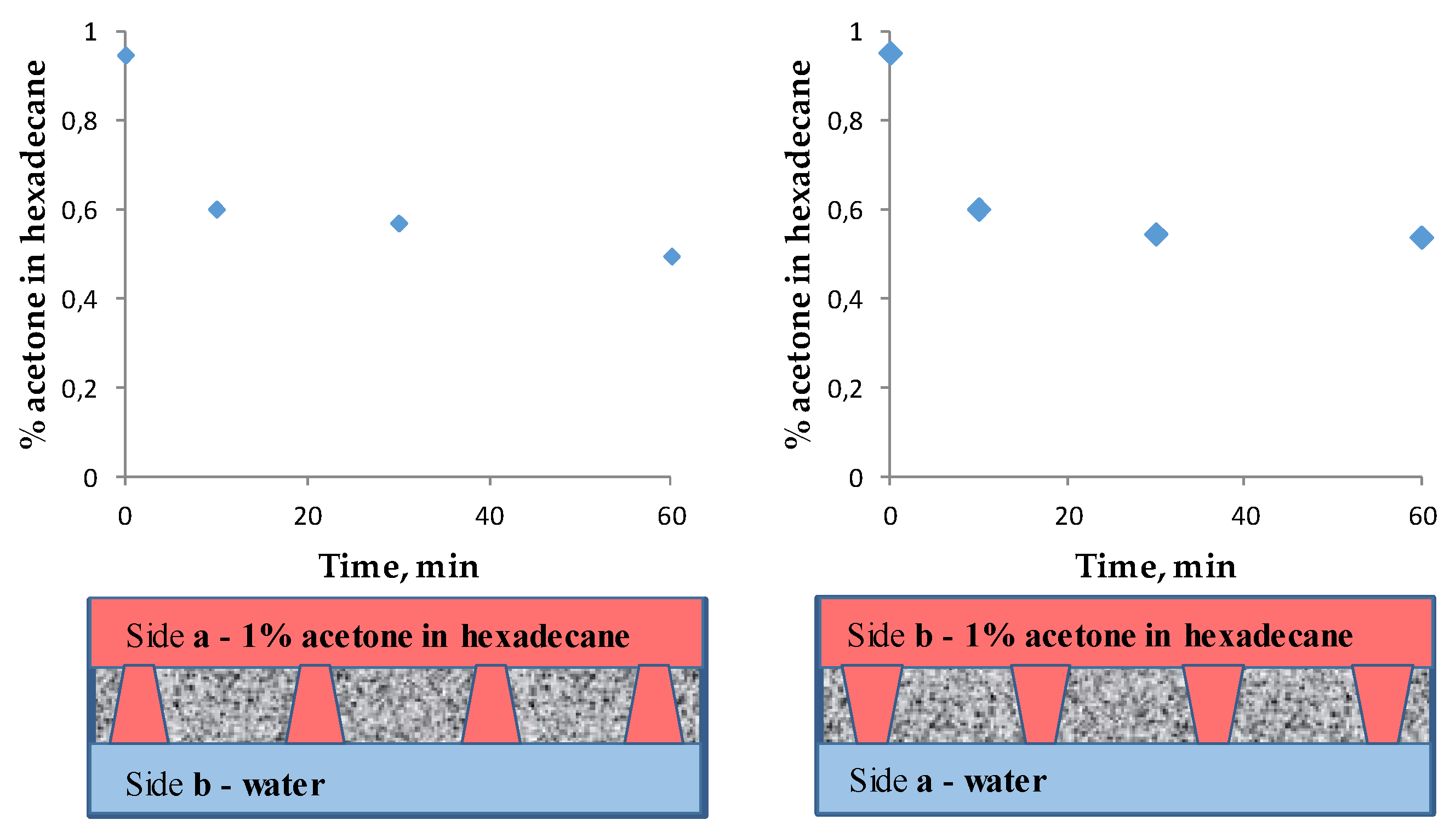
3.3. Extraction of Acetone from the Organic Phase to the Aqueous Phase in the Membrane Contactor
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Baker, R.W. Membrane Technology and Applications, 3rd ed.; John Wiley and Sons: Hoboken, NJ, USA, 2012; 576p. [Google Scholar]
- Russian Membrane Society. Terminology. Membr. Membr. Technol. 2013, 3, 74–82. [Google Scholar] [CrossRef]
- Zhao, S.; Feron, P.H.M.; Deng, L.; Favre, E.; Chabanon, E.; Yan, S.; Hou, J.; Chen, V.; Qi, H. Status and progress of membrane contactors in post-combustion carbon capture: A state-of-the-art review of new developments. J. Membr. Sci. 2016, 511, 180–206. [Google Scholar] [CrossRef]
- Obstals, F.; Vorobii, M.; Riedel, T.; de los Santos Pereira, A.; Bruns, M.; Singh, S.; Rodriguez-Emmenegger, C. Improving Hemocompatibility of Membranes for Extracorporeal Membrane Oxygenators by Grafting Nonthrombogenic Polymer Brushes. Macromol. Biosci. 2018, 18, 1700359. [Google Scholar] [CrossRef]
- Zheng, Z.; Wang, W.; Huang, X.; Fan, W.; Li, L. Surface modification of polysulfone hollow fiber membrane for extracorporeal membrane oxygenator using low-temperature plasma treatment. Plasma Proc. Polym. 2018, 15, 1700122. [Google Scholar] [CrossRef]
- Li, S.; Pyrzynski, T.J.; Klinghoffer, N.B.; Tamale, T.; Zhong, Y.; Aderhold, J.L.; Zhou, S.J.; Meyer, H.S.; Ding, Y.; Bikson, B. Scale-up of PEEK hollow fiber membrane contactor for post-combustion CO2 capture. J. Membr. Sci. 2017, 527, 92–101. [Google Scholar] [CrossRef] [Green Version]
- Tantikhajorngosol, P.; Laosiripojana, N.; Jiraratananon, R.; Assabumrungrat, S. Physical absorption of CO2 and H2S from synthetic biogas at elevated pressures using hollow fiber membrane contactors: The effects of Henry’s constants and gas diffusivities. Int. J. Heat Mass Transf. 2019, 128, 1136–1148. [Google Scholar] [CrossRef]
- Henares, M.; Ferrero, P.; San-Valero, P.; Martínez-Soria, V.; Izquierdo, M. Performance of a polypropylene membrane contactor for the recovery of dissolved methane from anaerobic effluents: Mass transfer evaluation, long-term operation and cleaning strategies. J. Membr. Sci. 2018, 563, 926–937. [Google Scholar] [CrossRef]
- Martić, I.; Maslarević, A.; Mladenović, S.; Lukić, U.; Budimir, S. Water deoxygenation using hollow fiber membrane module with nitrogen as inert gas. Desalin. Water Treat. 2015, 54, 1563–1567. [Google Scholar] [CrossRef]
- Ghasem, N.; Al-Marzouqi, M.; Ismail, Z. Gas–liquid membrane contactor for ethylene/ethane separation by aqueous silver nitrate solution. Sep. Purif. Technol. 2014, 127, 140–148. [Google Scholar] [CrossRef]
- Xu, Y.; Goh, K.; Wang, R.; Bae, T.-H. A review on polymer-based membranes for gas-liquid membrane contacting processes: Current challenges and future direction. Sep. Purif. Technol. 2019, 229, 115791. [Google Scholar] [CrossRef]
- Goh, P.S.; Naim, R.; Rahbari-Sisakht, M.; Ismail, A.F. Modification of membrane hydrophobicity in membrane contactors for environmental remediation. Sep. Purif. Technol. 2019, 227, 115721. [Google Scholar] [CrossRef]
- Bazhenov, S.; Bildyukevich, A.; Volkov, A. Gas-liquid hollow fiber membrane contactors for different applications. Fibers 2018, 6, 76. [Google Scholar] [CrossRef] [Green Version]
- Bazhenov, S.D.; Lyubimova, E.S. Gas–liquid membrane contactors for carbon dioxide capture from gaseous streams. Pet. Chem. 2016, 56, 889–914. [Google Scholar] [CrossRef]
- Winter, D.; Koschikowski, J.; Gross, F.; Maucher, D.; Düver, D.; Jositz, M.; Mann, T.; Hagedorn, A. Comparative analysis of full-scale membrane distillation contactors—Methods and modules. J. Membr. Sci. 2017, 524, 758–771. [Google Scholar] [CrossRef]
- Darestani, M.; Haigh, V.; Couperthwaite, S.J.; Millar, G.J.; Nghiem, L.D. Hollow fibre membrane contactors for ammonia recovery: Current status and future developments. J. Environ. Chem. Eng. 2017, 5, 1349–1359. [Google Scholar] [CrossRef] [Green Version]
- Aligwe, P.A.; Sirkar, K.K.; Canlas, C.J. Hollow fiber gas membrane-based removal and recovery of ammonia from water in three different scales and types of modules. Sep. Purif. Technol. 2019, 224, 580–590. [Google Scholar] [CrossRef]
- Xu, X.; Martin, G.J.; Kentish, S.E. Enhanced CO2 bio-utilization with a liquid–liquid membrane contactor in a bench-scale microalgae raceway pond. J. CO2 Util. 2019, 34, 207–214. [Google Scholar] [CrossRef] [Green Version]
- Rehman, W.U.; Muhammad, A.; Khan, Q.A.; Younas, M.; Rezakazemi, M. Pomegranate juice concentration using osmotic distillation with membrane contactor. Sep. Purif. Technol. 2019, 224, 481–489. [Google Scholar] [CrossRef]
- Pabby, A.K.; Sastre, A.M. State-of-the-art review on hollow fibre contactor technology and membrane-based extraction processes. J. Membr. Sci. 2013, 430, 263–303. [Google Scholar] [CrossRef]
- Kiani, A.; Bhave, R.R.; Sirkar, K.K. Solvent extraction with immobilized interfaces in a microporous hydrophobic membrane. J. Membr. Sci. 1984, 20, 125–145. [Google Scholar] [CrossRef]
- D’elia, N.A.; Dahuron, L.; Cussler, E.L. Liquid-liquid extractions with microporous hollow fibers. J. Membr. Sci. 1986, 29, 309–319. [Google Scholar] [CrossRef]
- Song, J.; Huang, T.; Qiu, H.; Niu, X.; Li, X.M.; Xie, Y.; He, T. A critical review on membrane extraction with improved stability: Potential application for recycling metals from city mine. Desalination 2018, 440, 18–38. [Google Scholar] [CrossRef]
- De Souza Moraes, L.; de Araujo Kronemberger, F.; Ferraz, H.C.; Habert, A.C. Liquid–liquid extraction of succinic acid using a hollow fiber membrane contactor. J. Ind. Eng. Chem. 2015, 21, 206–211. [Google Scholar] [CrossRef]
- Moreno, T.; Tallon, S.J.; Catchpole, O.J. Supercritical CO2 extraction of 1-butanol and acetone from aqueous solutions using a hollow-fiber membrane contactor. Chem. Eng. Technol. 2014, 37, 1861–1872. [Google Scholar] [CrossRef]
- Shojaee Nasirabadi, P.; Saljoughi, E.; Mousavi, S.M. Membrane processes used for removal of pharmaceuticals, hormones, endocrine disruptors and their metabolites from wastewaters: A review. Desalin. Water Treat. 2016, 57, 24146–24175. [Google Scholar] [CrossRef]
- Hylton, K.; Sangwan, M.; Mitra, S. Microscale membrane extraction of diverse antibiotics from water. Anal. Chim. Acta 2009, 653, 116–120. [Google Scholar] [CrossRef]
- Yahaya, G.O.; Hamad, F.; Bahamdan, A.; Tammana, V.V.; Hamad, E.Z. Supported ionic liquid membrane and liquid–liquid extraction using membrane for removal of sulfur compounds from diesel/crude oil. Fuel Proc. Technol. 2013, 113, 123–129. [Google Scholar] [CrossRef]
- Ferreira, A.R.; Neves, L.A.; Ribeiro, J.C.; Lopes, F.M.; Coutinho, J.A.; Coelhoso, I.M.; Crespo, J.G. Thiols’ extraction from “jet-fuel” assisted by ionic liquids in hollow fibre membrane contactors. J. Membr. Sci. 2015, 477, 66–73. [Google Scholar] [CrossRef] [Green Version]
- Amelio, A.; Loise, L.; Azhandeh, R.; Darvishmanesh, S.; Calabró, V.; Degrève, J.; Luis, P.; Van der Bruggen, B. Purification of biodiesel using a membrane contactor: Liquid–liquid extraction. Fuel Proc. Technol. 2016, 142, 352–360. [Google Scholar] [CrossRef]
- Prasad, R.; Kiani, A.; Bhave, R.R.; Sirkar, K.K. Further studies on solvent extraction with immobilized interfaces in a microporous hydrophobic membrane. J. Membr. Sci. 1986, 26, 79–97. [Google Scholar] [CrossRef]
- Prasad, R.; Sirkar, K.K. Microporous Membrane Solvent Extraction. Sep. Sci. Technol. 1987, 22, 619–640. [Google Scholar] [CrossRef]
- Prasad, R.; Sirkar, K.K. Solvent extraction with microporous hydrophilic and composite membranes. AIChE J. 1987, 33, 1057–1066. [Google Scholar] [CrossRef]
- Feng, S.; Zhong, Z.; Wang, Y.; Xing, W.; Drioli, E. Progress and perspectives in PTFE membrane: Preparation, modification, and applications. J. Membr. Sci. 2018, 549, 332–349. [Google Scholar] [CrossRef]
- Himma, N.F.; Anisah, S.; Prasetya, N.; Wenten, I.G. Advances in preparation, modification, and application of polypropylene membrane. J. Polym. Eng. 2016, 36, 329–362. [Google Scholar] [CrossRef]
- Guillen, G.R.; Pan, Y.; Li, M.; Hoek, E.M. Preparation and Characterization of Membranes Formed by Nonsolvent Induced Phase Separation: A Review. Ind. Eng. Chem. Res. 2011, 50, 3798–3817. [Google Scholar] [CrossRef]
- Thakur, V.K.; Voicu, S.I. Recent advances in cellulose and chitosan based membranes for water purification: A concise review. Carbohydr. Polym. 2016, 146, 148–165. [Google Scholar] [CrossRef]
- Apel, P.Y. Fabrication of functional micro- and nanoporous materials from polymers modified by swift heavy ions. Radiat. Phys. Chem. 2019, 159, 25–34. [Google Scholar] [CrossRef]
- Yeszhanov, A.B.; Korolkov, I.V.; Dosmagambetova, S.S.; Zdorovets, M.V.; Güven, O. Recent progress in the membrane distillation and impact of track-etched membranes. Polymer 2021, 13, 2520. [Google Scholar] [CrossRef]
- Kislyi, A.G.; Butylskii, D.Y.; Mareev, S.A.; Nikonenko, V.V. Model of Competitive Ion Transfer in an Electro-Baromembrane System with Track-Etched Membrane. Membr. Membr. Technol. 2021, 3, 131–138. [Google Scholar] [CrossRef]
- Butylskii, D.Y.; Pismenskaya, N.D.; Apel, P.Y.; Sabbatovskiy, K.G.; Nikonenko, V.V. Highly selective separation of singly charged cations by countercurrent electromigration with a track-etched membrane. J. Membr. Sci. 2021, 635, 119449. [Google Scholar] [CrossRef]
- Armstrong, J.A.; Bernal, E.E.L.; Yaroshchuk, A.; Bruening, M.L. Separation of Ions Using Polyelectrolyte-Modified Nanoporous Track-Etched Membranes. Langmuir 2013, 29, 10287–10296. [Google Scholar] [CrossRef] [PubMed]
- Tang, C.; Yaroshchuk, A.; Bruening, M.L. Flow through negatively charged, nanoporous membranes separates Li+ and K+ due to induced electromigration. Chem. Commun. 2020, 56, 10954–10957. [Google Scholar] [CrossRef] [PubMed]
- Apel, P.Y.; Korchev, Y.E.; Siwy, Z.; Spohr, R.; Yoshida, M. Diode-like single-ion track membrane prepared by electro-stopping. Nucl. Instrum. Meth. Phys. Res. B. 2001, 184, 337–346. [Google Scholar] [CrossRef]
- Apel, P.Y.; Blonskaya, I.V.; Dmitriev, S.N.; Mamonova, T.I.; Orelovitch, O.L.; Sartowska, B.; Yamauchi, Y. Surfactant-controlled etching of ion track nanopores and its practical applications in membrane technology. Radiat. Meas. 2008, 43, 552–559. [Google Scholar] [CrossRef]
- NIST TRC TDE Standart Reference Database 103a; Aspen Properties®; TIPS RAS: Moscow, Russia, 2021.
- Orelovitch, O.L.; Apel, P.Y.; Sartowska, B. New methods of track membrane treatment in the preparation of samples for further observation with scanning electron microscopy. J. Microsc. 2006, 224, 100–103. [Google Scholar] [CrossRef]
- Owens, D.K.; Wendt, R.C. Estimation of the surface free energy of polymers. J. Appl. Polym. Sci. 1969, 13, 1741–1747. [Google Scholar] [CrossRef]
- Fowkes, F.M. Attractive forces at interfaces. Ind. Eng. Chem. 1964, 56, 40–52. [Google Scholar] [CrossRef]
- Hulterström, A.K.; Berglund, A.; Ruyter, I.E. Wettability, water sorption and water solubility of seven silicone elastomers used for maxillofacial prostheses. J. Mater. Sci. Mater. Med. 2008, 19, 225–231. [Google Scholar] [CrossRef]
- Arikan, E.; Holtmannspötter, J.; Zimmer, F.; Hofmann, T.; Gudladt, H.J. The role of chemical surface modification for structural adhesive bonding on polymers-Washability of chemical functionalization without reducing adhesion. Int. J. Adhes. Adhes. 2019, 95, 102409. [Google Scholar] [CrossRef]
- Apel, P.Y.; Blonskaya, I.V.; Orelovitch, O.L.; Ramirez, P.; Sartowska, B.A. Effect of nanopore geometry on ion current rectification. Nanotechnology 2011, 22, 175302. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Volkov, V.V.; Lebedeva, V.I.; Petrova, I.V.; Bobyl, A.V.; Konnikov, S.G.; Roldughin, V.I.; van Erkel, J.; Tereshchenko, G.F. Adlayers of palladium particles and their aggregates on porous polypropylene hollow fiber membranes as hydrogenization contractors/reactors. Adv. Colloid Interface Sci. 2011, 164, 144–155. [Google Scholar] [CrossRef]
- Kobayashi, I.; Yasuno, M.; Iwamoto, S.; Shono, A.; Satoh, K.; Nakajima, M. Microscopic observation of emulsion droplet formation from a polycarbonate membrane. Colloids Surf. A Physicochem. Eng. Asp. 2002, 207, 185–196. [Google Scholar] [CrossRef]
- Vladisavljević, G.T. Preparation of microemulsions and nanoemulsions by membrane emulsification. Coll. Surf. A Physicochem. Eng. Asp. 2019, 579, 123709. [Google Scholar] [CrossRef]
- Vladisavljević, G.T. Fabrication of nanoemulsions by membrane emulsification. Nanoemulsions 2018, 287–346. [Google Scholar] [CrossRef]
- Kuzmanović, B.; Kuipers, N.J.; de Haan, A.B.; Kwant, G. Reactive extraction of alcohols from apolar hydrocarbons with aqueous solutions. Tsinghua Sci. Technol. 2006, 11, 222–227. [Google Scholar] [CrossRef]
- Zhu, Z.; Bai, W.; Qi, P.; Dai, Y.; Wang, Y.; Cui, P.; Gao, J. Liquid liquid equilibrium data for the separation of acetone from n-heptane using four imidazolium-based ionic liquids. J. Chem. Eng. Data. 2019, 64, 1202–1208. [Google Scholar] [CrossRef]

| Property | Aqueous Phase | Organic Phase | |
|---|---|---|---|
| Water | Hexadecane | 1-Pentanol | |
| Molar mass, g/mole | 18 | 226.45 | 88.15 |
| Normal boiling point, °C | 100 | 286.8 | 137.9 |
| Viscosity at 25 °C, mPa·s | 0.895 | 3.08 | 3.36 |
| Surface tension at 25 °C, mN/m | 71.98 | 27.15 | 25.3 |
| Interfacial tension of the water–liquid interface at 25 °C, mN/m | - | 55.2 1 | 4.5 |
| Sample | Time of Chemical Etching, min | Average Pore Diameter on the Selective Side Determined by SEM, nm | Thickness, μm | Volume Porosity, % |
|---|---|---|---|---|
| TEM-1 | 3 | 12.5 ± 0.3 * | 23.7 | 14 |
| TEM-2 | 3.5 | 14.7 ± 0.4 | 22.8 | 17 |
| TEM-3 | 4 | 15.7 ± 0.5 | 22.7 | 22 |
| TEM-4 | 4.5 | 19.0 ± 0.5 | 22.6 | 32 |
| Sample | Surface Energy, mJ/m2 | |||||
|---|---|---|---|---|---|---|
| Side a | Side b | |||||
| γd | γp | γ | γd | γp | γ | |
| TEM-1 | 26 | 15 | 41 | 30 | 17 | 47 |
| TEM-2 | 28 | 13 | 41 | 28 | 31 | 59 |
| TEM-3 | 35 | 16 | 51 | 34 | 23 | 57 |
| TEM-4 | 35 | 12 | 47 | 36 | 17 | 53 |
| Sample | Interphase Surface Stability | |||
|---|---|---|---|---|
 |  |  |  | |
| TEM-1 | - | + | + | + |
| TEM-2 | - | + | + | + |
| TEM-3 | - | - | - | - |
| TEM-4 | - | - | - | - |
| Water Overpressure, kPa | Interphase Surface Stability | |||
|---|---|---|---|---|
| TEM-1 | TEM-2 | |||
 |  |  |  | |
| 3 | + | + | + | + |
| 5 | - | + | + | + |
| 7 | - | + | - | + |
| 10 | n/a | - | - | + |
| 13 | n/a | n/a | n/a | - |
| Side a—Hexadecane Side b—Water | Side a—Water Side b—Hexadecane | ||||||
|---|---|---|---|---|---|---|---|
| The linear velocity of water = 2.4 cm/s; the linear velocity of hexadecane varies | The linear velocity of water varies; the linear velocity of hexadecane = 2.6 cm/s | The linear velocity of water = 2.4 cm/s; the linear velocity of hexadecane varies | The linear velocity of water varies; the linear velocity of hexadecane = 2.6 cm/s | ||||
| The linear velocity of hexadecane | DLS | The linear velocity of hexadecane | DLS | The linear velocity of hexadecane | DLS | The linear velocity of hexadecane | DLS |
| 1.6 | - | 1.6 | - | 1.6 | - | 1.6 | - |
| 1.9 | - | 1.9 | - | 1.9 | - | 1.9 | - |
| 3 | - | 3 | - | 3 | - | 3 | - |
| 3.3 | - | 3.3 | - | 3.3 | - | 3.3 | - |
| 3.9 | hexadecane drops (176 nm) in water | 3.9 | hexadecane drops (176 nm) in water | 3.9 | hexadecane drops (176 nm) in water | 3.9 | hexadecane drops (176 nm) in water |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bazhenov, S.; Kristavchuk, O.; Kostyanaya, M.; Belogorlov, A.; Ashimov, R.; Apel, P. Interphase Surface Stability in Liquid-Liquid Membrane Contactors Based on Track-Etched Membranes. Membranes 2021, 11, 949. https://doi.org/10.3390/membranes11120949
Bazhenov S, Kristavchuk O, Kostyanaya M, Belogorlov A, Ashimov R, Apel P. Interphase Surface Stability in Liquid-Liquid Membrane Contactors Based on Track-Etched Membranes. Membranes. 2021; 11(12):949. https://doi.org/10.3390/membranes11120949
Chicago/Turabian StyleBazhenov, Stepan, Olga Kristavchuk, Margarita Kostyanaya, Anton Belogorlov, Ruslan Ashimov, and Pavel Apel. 2021. "Interphase Surface Stability in Liquid-Liquid Membrane Contactors Based on Track-Etched Membranes" Membranes 11, no. 12: 949. https://doi.org/10.3390/membranes11120949
APA StyleBazhenov, S., Kristavchuk, O., Kostyanaya, M., Belogorlov, A., Ashimov, R., & Apel, P. (2021). Interphase Surface Stability in Liquid-Liquid Membrane Contactors Based on Track-Etched Membranes. Membranes, 11(12), 949. https://doi.org/10.3390/membranes11120949








