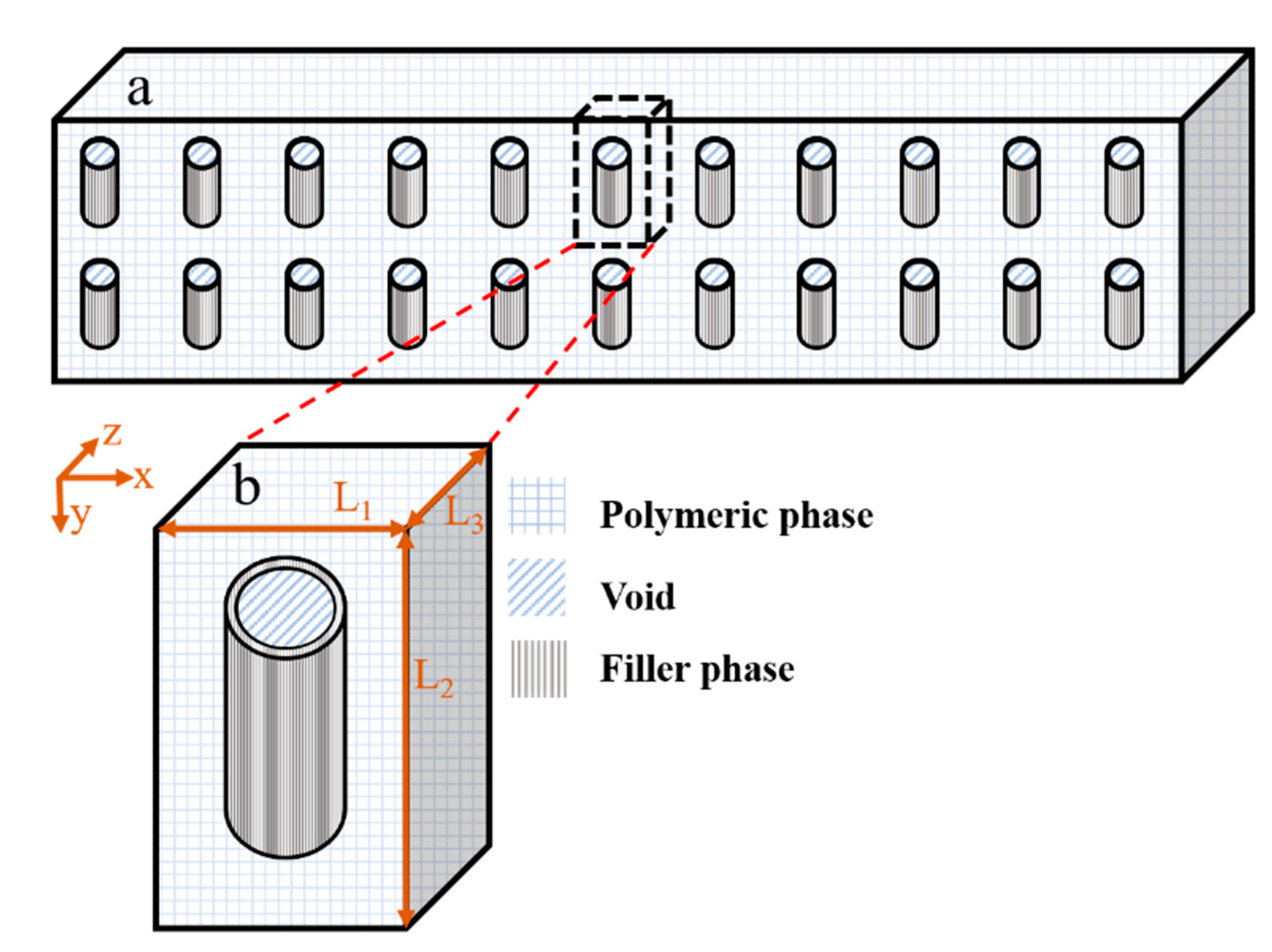
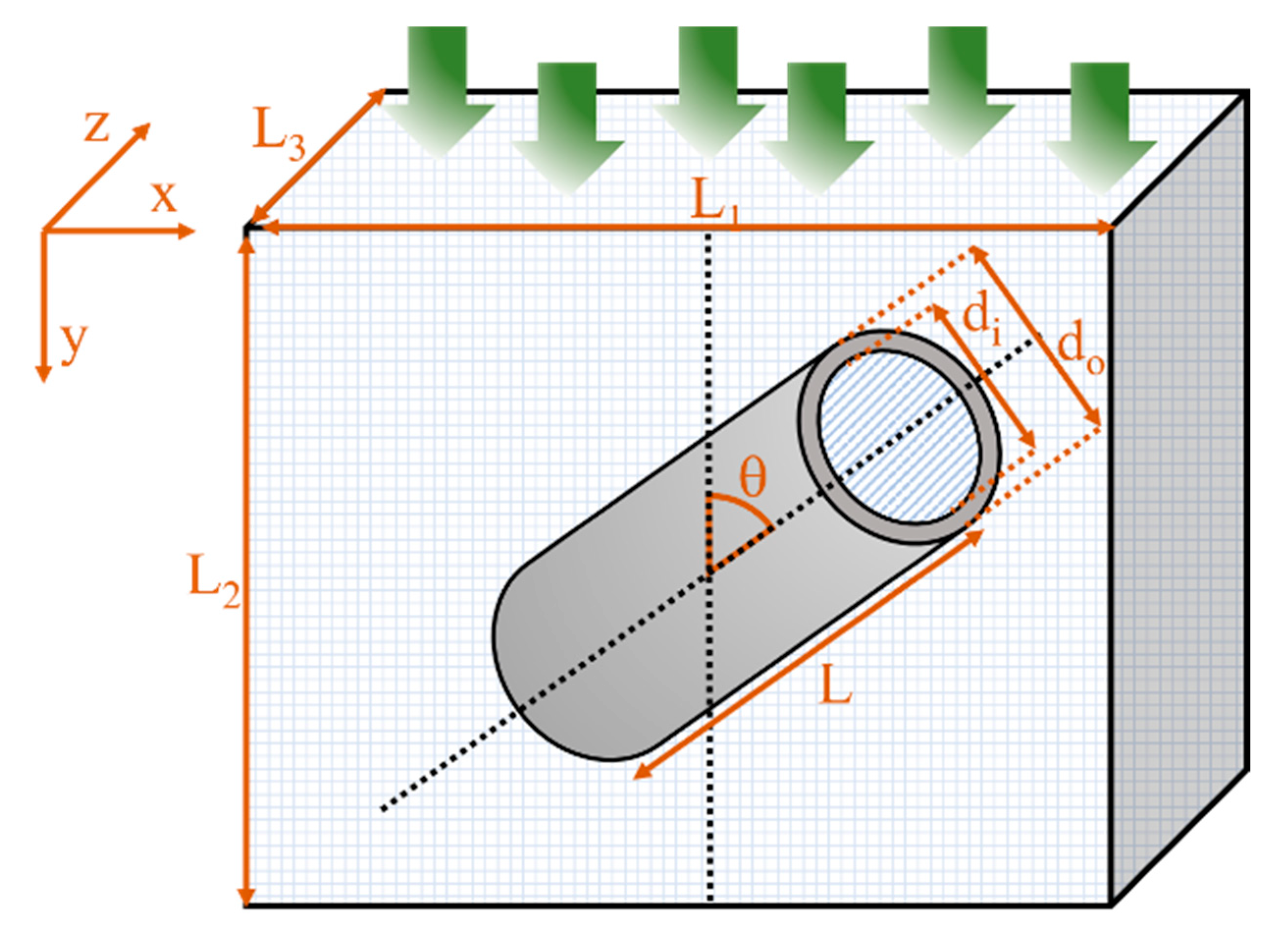
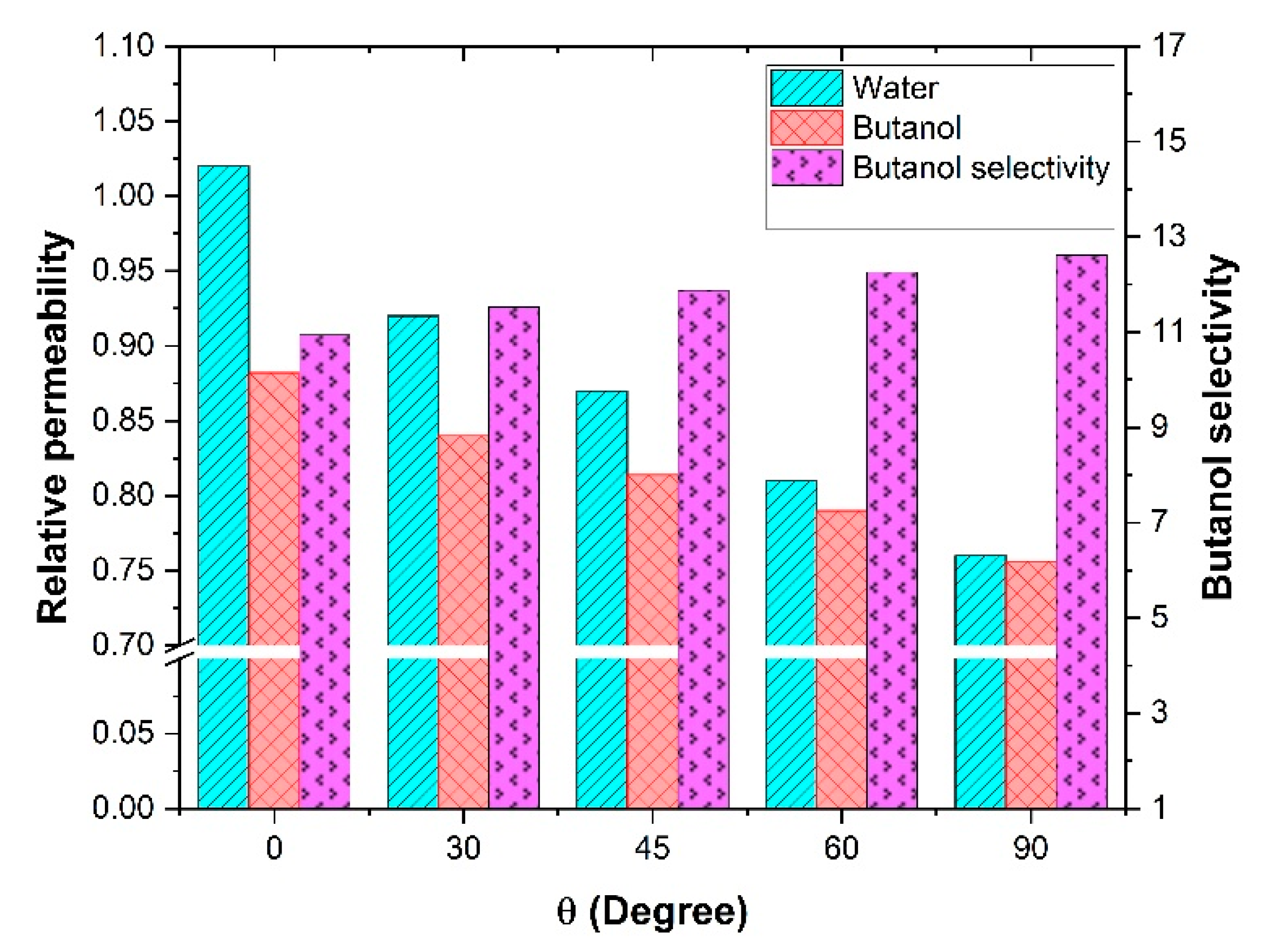
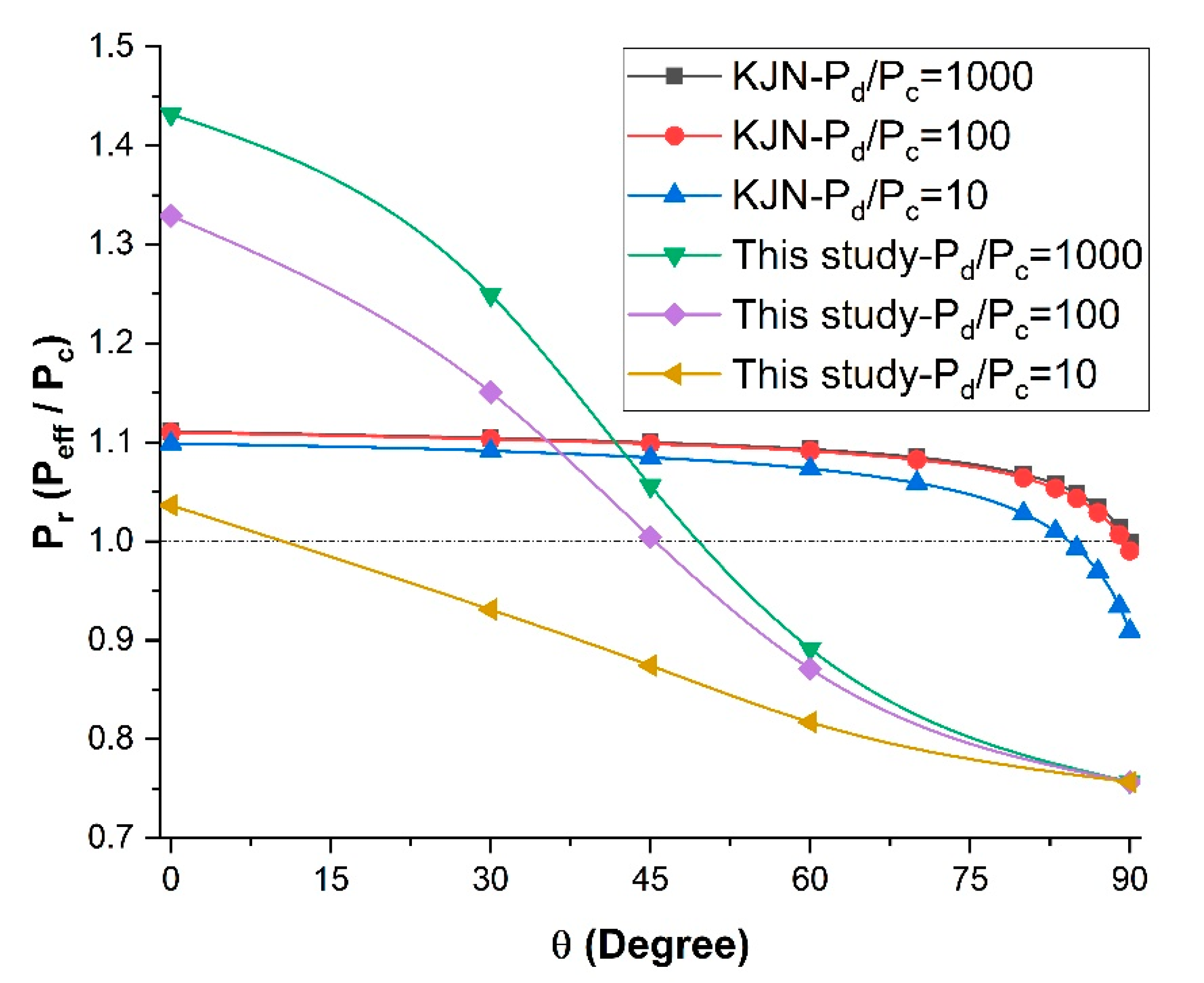
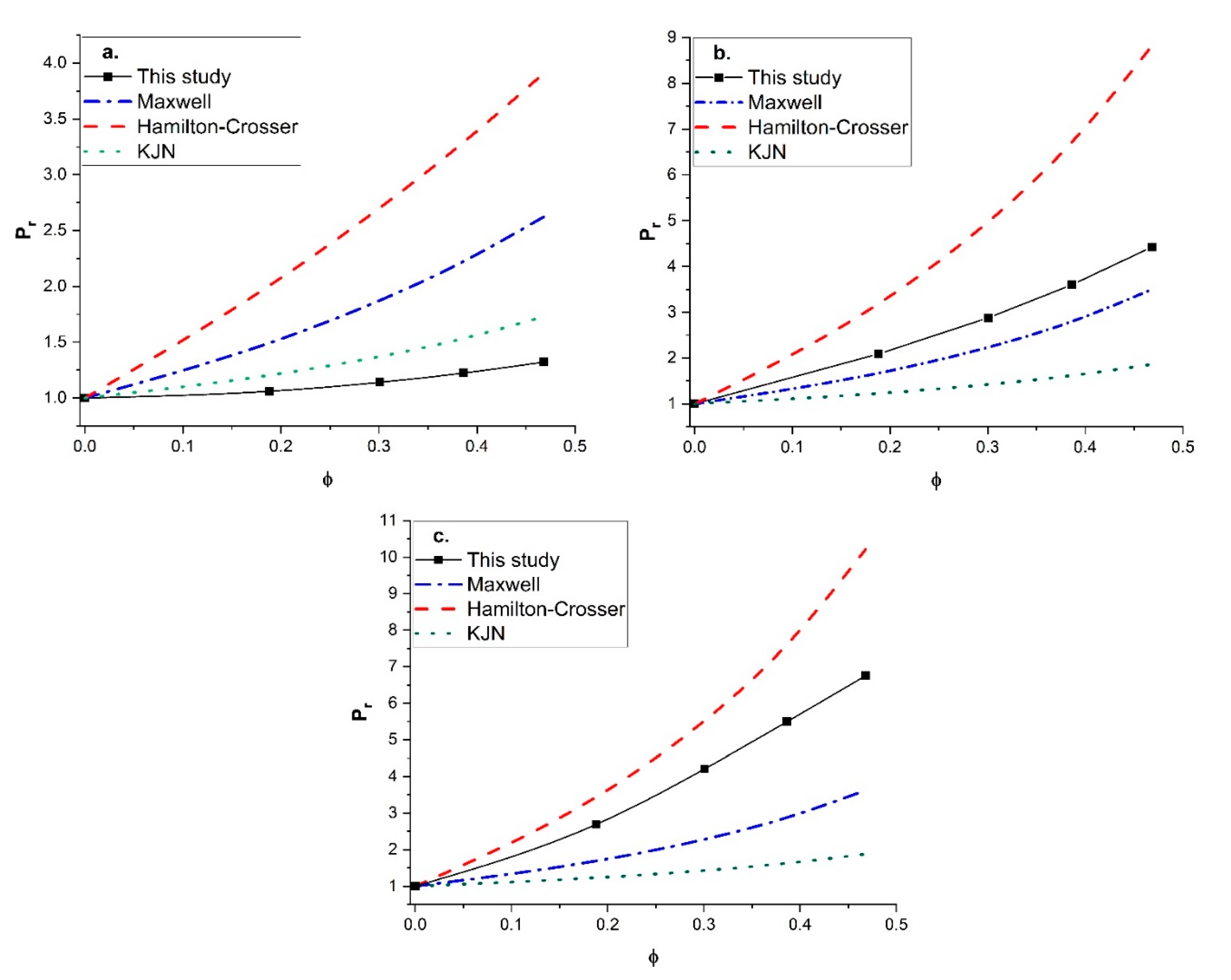
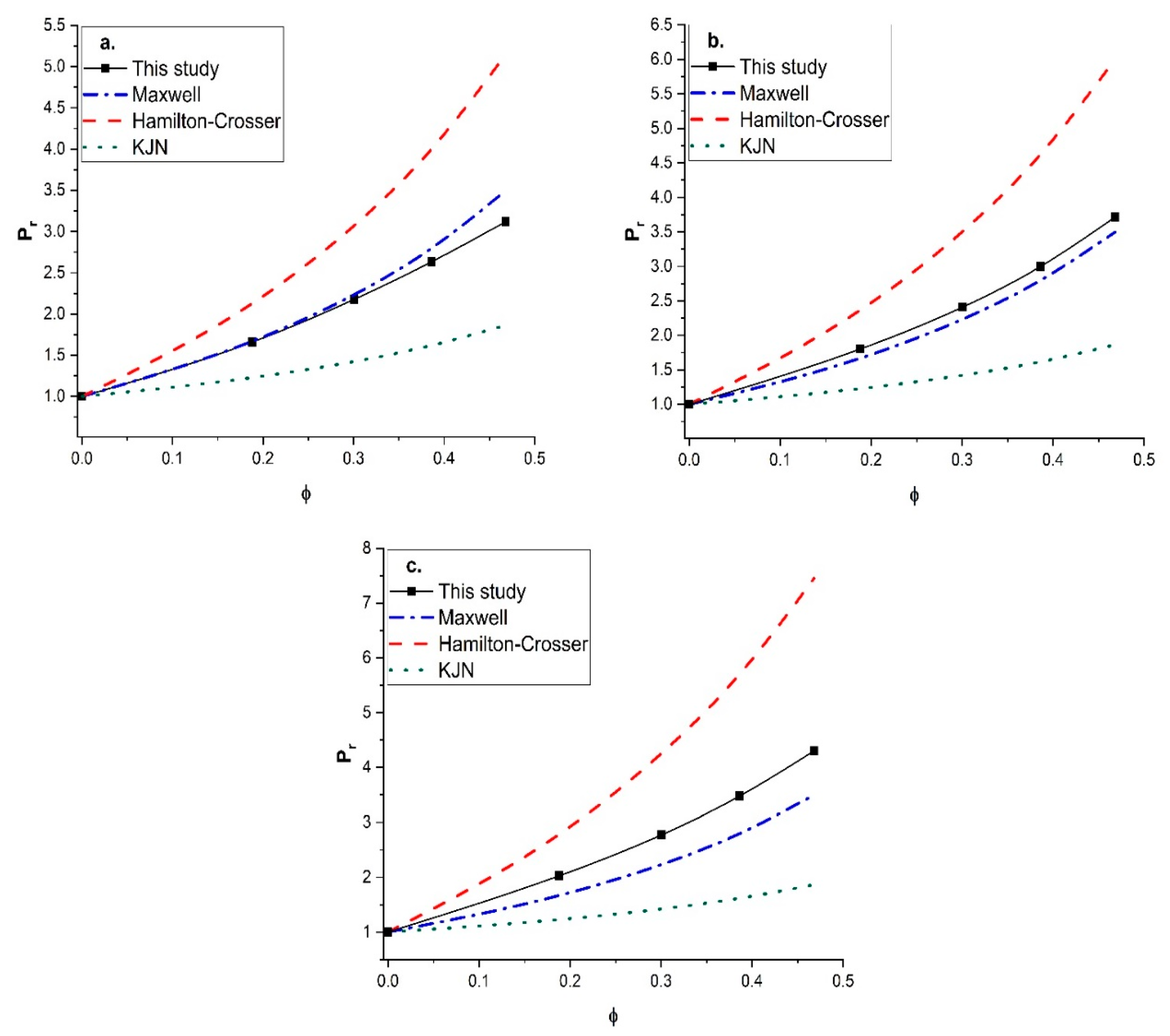
1. Introduction
The use of membranes in separation processes for chemical, petrochemical, and environmental applications has significantly increased in recent years. The main advantages of using membranes in industrial separation processes are the much lower energy requirements and the smaller plant footprint compared to conventional separation processes. In addition, given their high stability, efficiency, and ease of processing, employing membranes in industrial processes leads to lower environmental impact and cost [
1,
2]. Polymeric membranes are currently used commercially in separation processes such as pervaporation [
3,
4] and gas separation [
5]. In gas separation processes, cellulose acetate membranes were employed by Cynara, Separex, and GMS to remove carbon dioxide from natural gas by the mid-1980s and further developed by involving polyimide hollow-fiber membranes [
6]. At about the same time, GENERON introduced the first membrane system to separate nitrogen from air using poly(4-methyl-1-pentene) (TPX) membranes. Due to the collaboration of other companies such as Dupont and Air Liquide, over 10,000 similar systems have already been installed worldwide. However, many gas processes such as oxygen separation or pervaporation applications such as alcohol separation and dehydration still require better membranes to become more commercially viable [
2,
6].
Improving the separation performances of a membrane-based process at both laboratory and industrial scales depends largely on the chemical, mechanical, and permeation properties of the membrane. Even though polymeric membrane materials are continuously improved [
7], polymeric membranes mostly suffer from the well-known trade-off between the separation factor and permeating flux [
4,
8,
9]. In an attempt to improve the separation factor and permeating flux of polymeric membranes, many researchers are now directing their efforts to developing mixed-matrix membranes (MMMs). It has been reported that MMMs, which are made by embedding a proper organic or inorganic filler in the polymer matrix, can combine the advantages of the higher selectivity of the filler particles and the ease of processing of polymers [
3,
4,
10,
11,
12]. Different filler materials, such as activated carbons [
4], carbon nanotubes (CNTs) [
13], zeolites [
14], and metal-organic frameworks (MOFs) [
15,
16], have been incorporated within the matrix of polymers to make MMMs.
Among all types of fillers, nanotubes are considered emerging nanostructured materials for their potential to enhance the separation performance of membranes for numerous applications. Since the discovery of carbon nanotubes (CNTs), mixed-matrix membranes incorporating different single-wall and multi-wall carbon nanotubes [
17,
18] have shown their potential due to their exceptional transport properties and their physical compatibility with the polymeric membrane. Although the mass transport properties of CNTs are appealing, the ability to mass-produce and fabricate defect-free mixed-matrix membranes using CNTs is still challenging and limits their applications to large-scale industrial processes. To address these problems, other nanotubes such as titania [
19], halloysite nanotubes [
20,
21], organosilicon [
22], and aluminosilicate [
23,
24] nanotubes have been investigated. Novel techniques have been developed for the synthesis of nanotubes as well as to manipulate their dimension [
25,
26] and to modify their surface functionality [
27,
28]. Recent numerical studies on nanotubes have suggested that they possess mass transport rates up to three orders of magnitude larger than other materials with similar channel sizes, such as zeolites. The mass transport rates were also found to be considerably larger than the one predicted based on the Knudsen diffusion [
29,
30]. However, most nanotubes have an impermeable side wall that causes the orientation of nanotubes to greatly impact the achievable mass transport, as the permeant can only diffuse in the nanotube axial direction [
31,
32,
33]. Since Skoulidas et al.’s [
34] atomistic simulations on vertically aligned CNTs demonstrated the extremely high transport rate and permeability of light gases, aligning nanotubes within the membrane has received considerable attention for the development of membrane-based separation technologies.
Although there are numerous types of nanotubes and polymers available, a rational choice of both phases toward the preparation of MMMs is necessary. Therefore, theoretical predictions of the separation performance from the pure species permeation properties in these MMMs become increasingly valuable. Up to now, different theoretical models have been developed to predict the performance of both ideal and non-ideal MMMs based on their polymer-particle interface morphology [
35,
36]. Different models, including the modified Maxwell model proposed by Vu et al. [
37], the modified Lewis−Nielsen model [
2,
36], the modified Pal model [
36], as well as the original and modified Felske model [
35], have been developed to estimate the effective permeability of non-ideal MMMs. Generally, these models can predict the permeability and the selectivity for the most common MMM morphologies over a large range of filler loading [
2]. However, several additional parameters such as particle pore size and distribution, permeability of species in the rigidified or void layer, filler-pore blockage ratio, as well as the thickness of the non-ideal phase should be taken into account. These parameters are most sensitive to operating conditions and, since there are no reliable methods yet to measure these parameters, their estimation and determination remain a significant challenge [
1]. Concentration gradient-driven molecular dynamics simulations, although currently limited to short simulation times, can contribute to elucidating the interaction between the polymer and the filler and estimate transport properties near and at the filler-polymer interphase region [
38].
Even though the ideal polymer-particle interface is generally difficult to achieve, the non-ideality can be negligible in some MMMs depending on the particle size and intrinsic properties of polymer and particle materials [
1,
2]. Numerous analytical models have been developed for estimating the effective permeability of ideal MMMs with spherical or near-spherical fillers such as activated carbons, zeolites, or metal-organic frameworks (MOFs) [
1,
2,
8,
39]. The Maxwell model [
40] is a well-known correlation to predict effective permeability in terms of the permeability of the dispersed and continuous phases and the filler volume fraction:
where
Peff is the effective permeability of the mixed-matrix membrane,
Pc is the permeability of the polymer matrix (continuous phase),
Pd is the permeability of the filler (dispersed phase),
φ is the volume fraction of the filler 0 ≤
φ ≤ 1, and
n is the shape factor of the filler. Considering the original Maxwell model for near-spherical fillers, the shape factor
n is taken as
n = 3.
The Maxwell correlation can predict the effective permeability of MMMs for spherical and near-spherical fillers fairly accurately up to the intermediate filler volume fraction [
1]. However, the Maxwell correlation is not well adapted for predicting the effective permeability of particles that deviate significantly from traditional geometry such as for MMMs incorporating tubular fillers, as it is difficult to specify the shape factor. A modified Maxwell model, also known as the Hamilton-Crosser model [
41], was suggested for the prediction of effective permeability by substituting the semi-empirical shape factor in the Maxwell equation using Equations (2) and (3).
where
ψ represents the sphericity of nanotubes and
g is an empirical parameter taken as unity, as in the original paper by Hamilton and Crosser [
41].
L and
do are the length and outer diameter of the nanotubes, respectively. Although the Hamilton-Crosser model can estimate permeation in composite membranes containing nanotubes by using a shape factor, the assumption that the fillers have an isotropic permeability makes it inconsistent with the high mass transport in the axial direction of the nanotubes. Furthermore, the Hamilton-Crosser model assumes that the fillers are randomly oriented, whereas nanotubes could be well aligned in the mixed-matrix membranes [
13].
The Kang-Jones-Nair (KJN) model [
30] is among the few analytical models that were proposed to estimate effective permeability considering the orientation of the nanotubes and their permeability in the axial direction (Equations (4)–(6)).
where
Vt is the total volume,
Vd is the dispersed phase volume based on the length and outside diameter of the nanotube, and
θ is the orientation with respect to the axis parallel to the main migration direction and nanotube aspect ratio
α, which is the length over the outer diameter ratio (
L/
do).
Even though the KJN model can account for the orientation and aspect ratio of the nanotubes, a one-dimensional (1D) mass transfer was assumed for its derivation. This approximation may not represent adequately the three-dimensional (3D) mass transport phenomena occurring in MMMs and consequently reduces the reliability of the KJN model. In addition, the KJN model cannot be used for completely or partially impermeable filler particles.
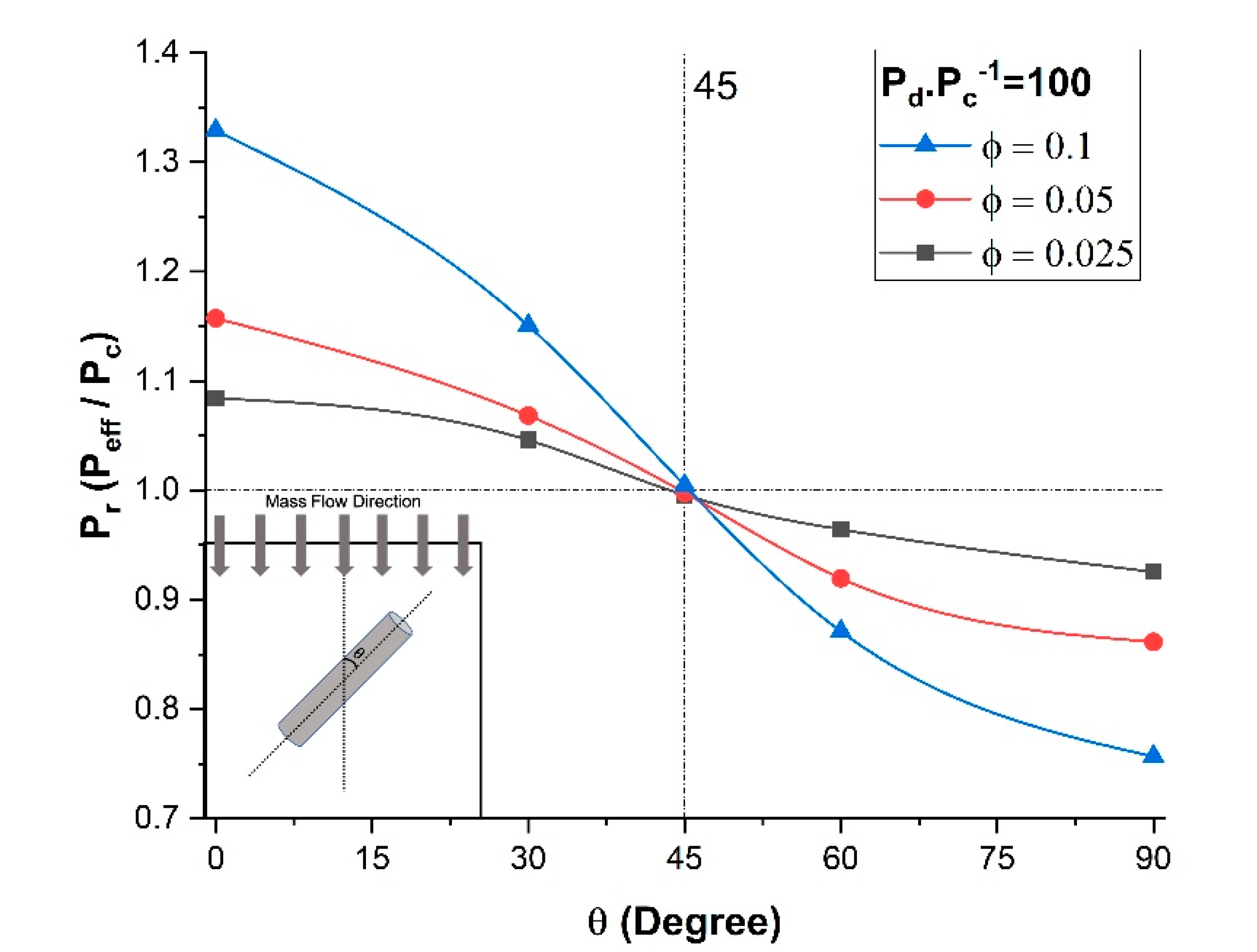
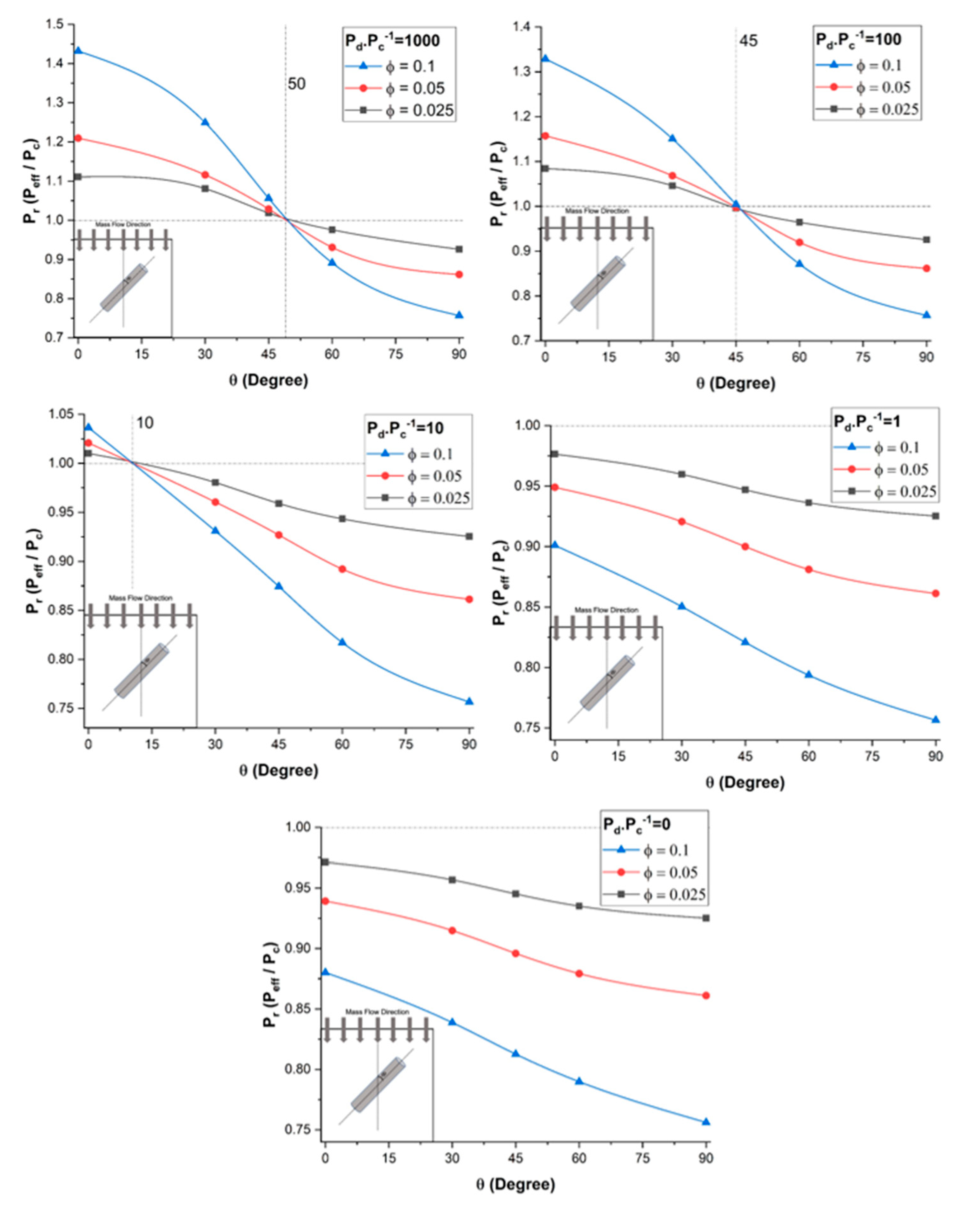
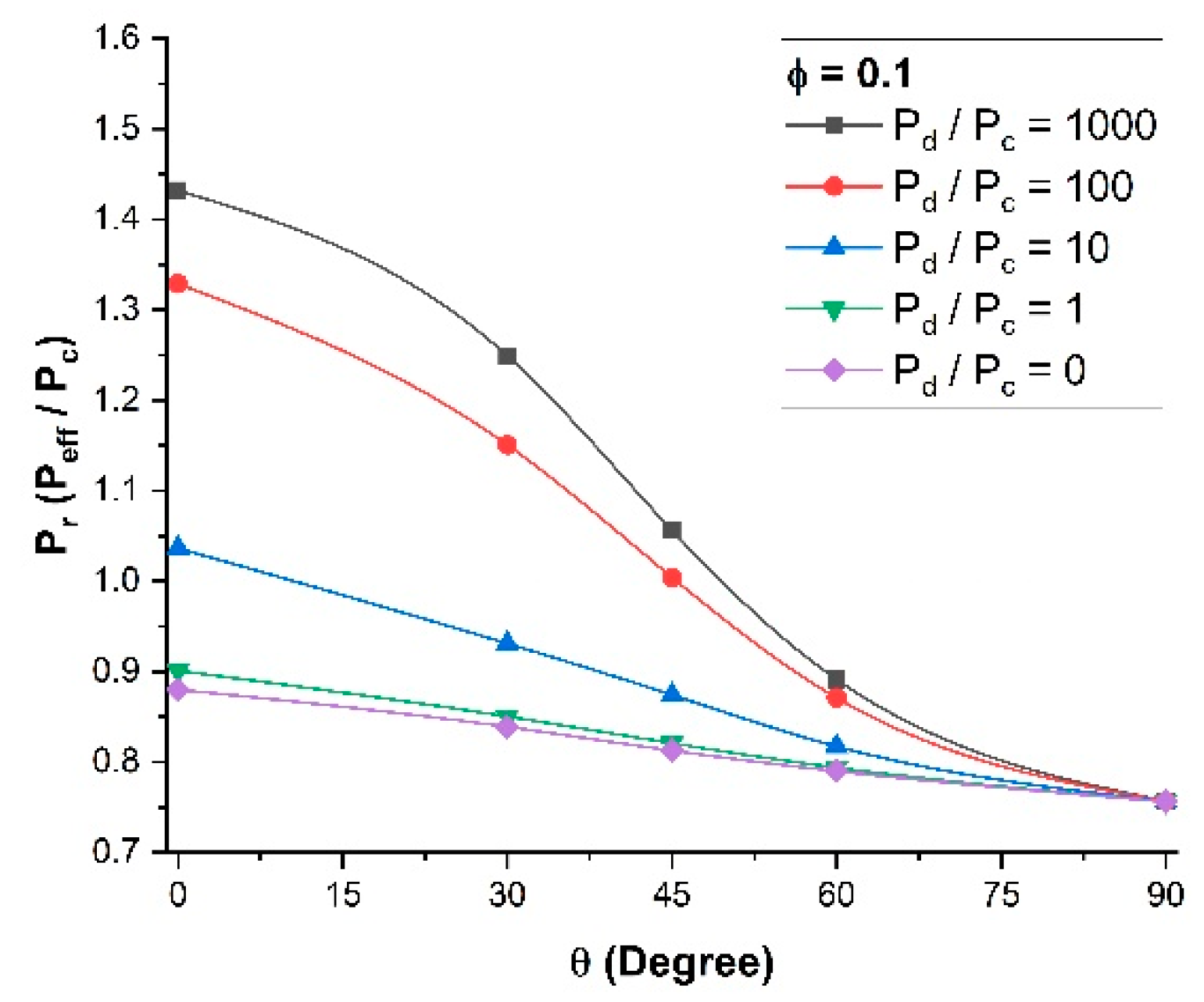
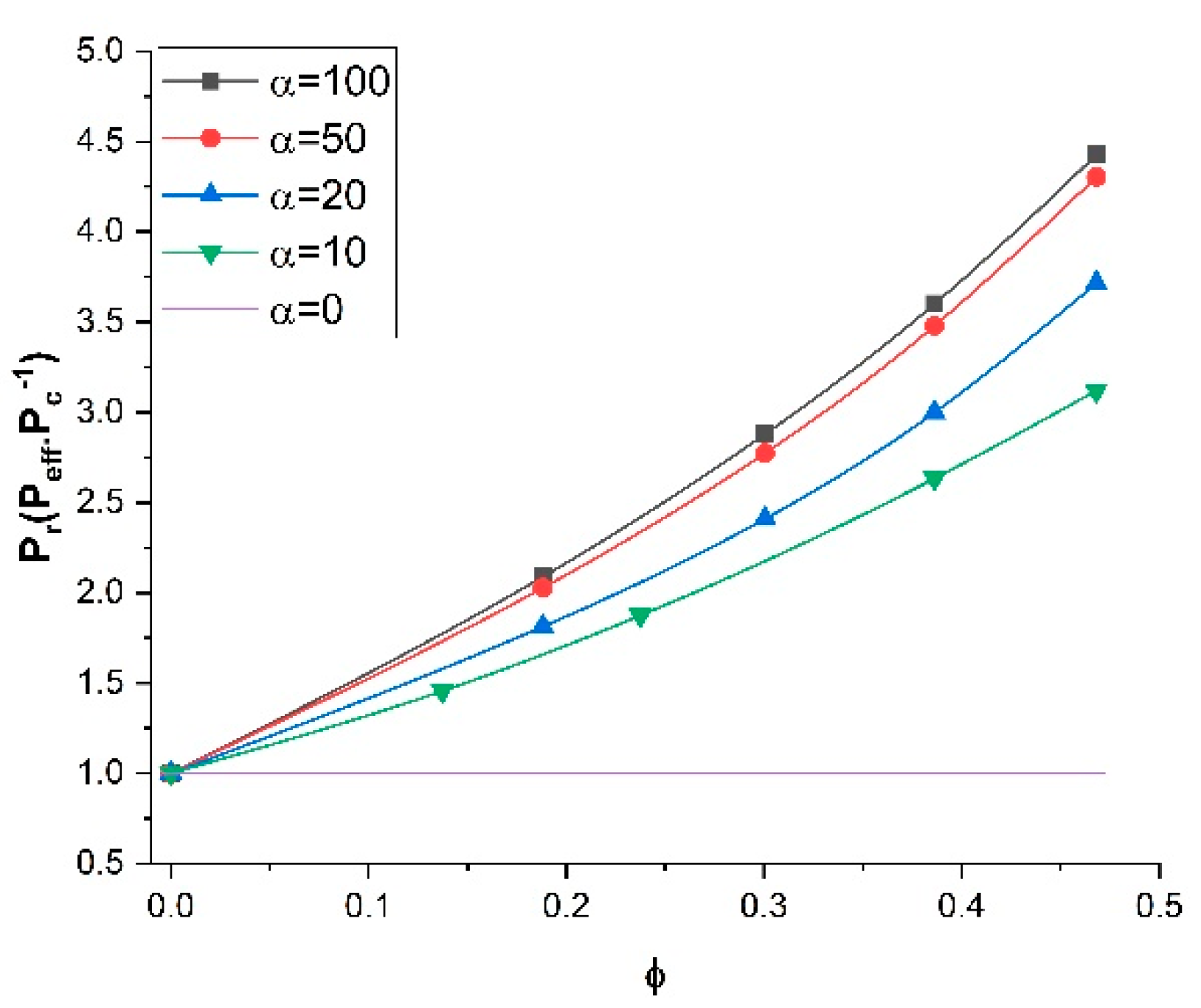
To assess the degree of accuracy of the previously mentioned models for the estimation of effective permeability, the three-dimensional Fick’s diffusion equation was solved numerically to determine the steady-state permeation flux of a mixed-matrix membrane containing nanotubes. The steady-state permeation flux allows for the calculation of a given MMM’s effective permeability. The numerical investigation allows the effects of the filler orientation, the length-to-diameter aspect ratio, and the permeability ratio between the continuous and dispersed phases on the effective permeability to be studied. The results were compared with the Maxwell, Hamilton-Crosser, and KJN models and provided data to potentially develop a better empirical predictive model. There were numerous studies in the literature using different types of fillers, where the effective permeability did not follow the expected trends, and many reasons were provided to explain these discrepancies. The relative permeability of ideal MMMs, calculated numerically as in this investigation and used as a benchmark, would be very helpful in identifying the sources of these non-idealities. The objective of this paper is therefore to investigate the impact of using nanotubes as fillers in MMMs on the permeability of a migrating species under ideal filler distribution. Since nanotubes such as CNTs are increasingly used in MMMs in various separation processes, including liquid and gas separation and pervaporation, it is very important to understand fundamentally how the permeability changes with different operating parameters. The data obtained in this investigation can also serve to develop an empirical model for MMM permeability.