Influence of Spacer Design and Module Geometry on the Filtration Performance during Skim Milk Microfiltration with Flat Sheet and Spiral-Wound Membranes
Abstract
:1. Introduction
2. Materials and Methods
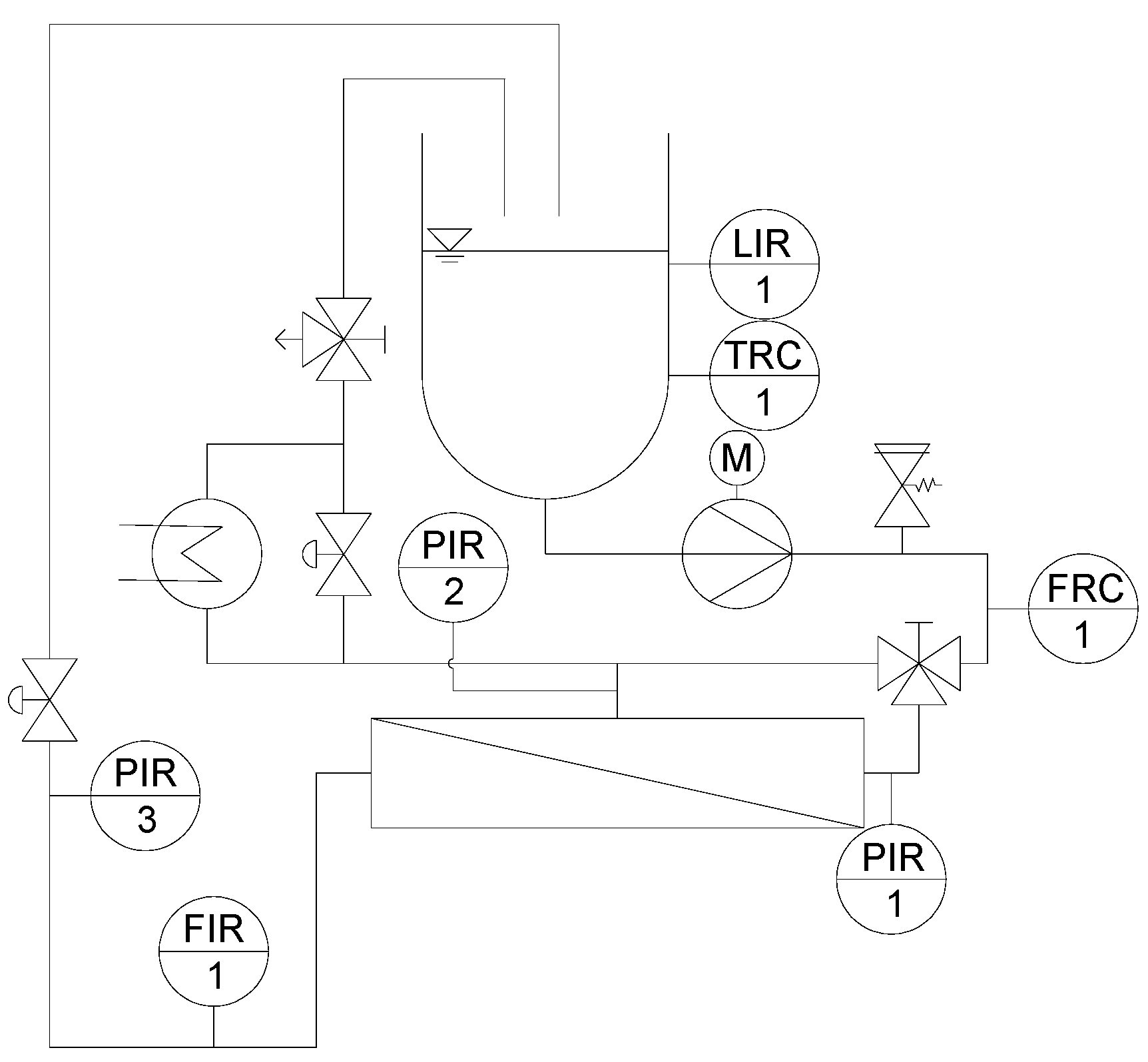
2.1. Crossflow Filtration Rigs and Experimental Procedure
2.1.1. Flat Sheet Test Cell and Experimental Design
2.1.2. Pilot-Scale Filtration Plant for SWM and Experimental Design
2.2. Filtration Fluid Skim Milk and Calculations
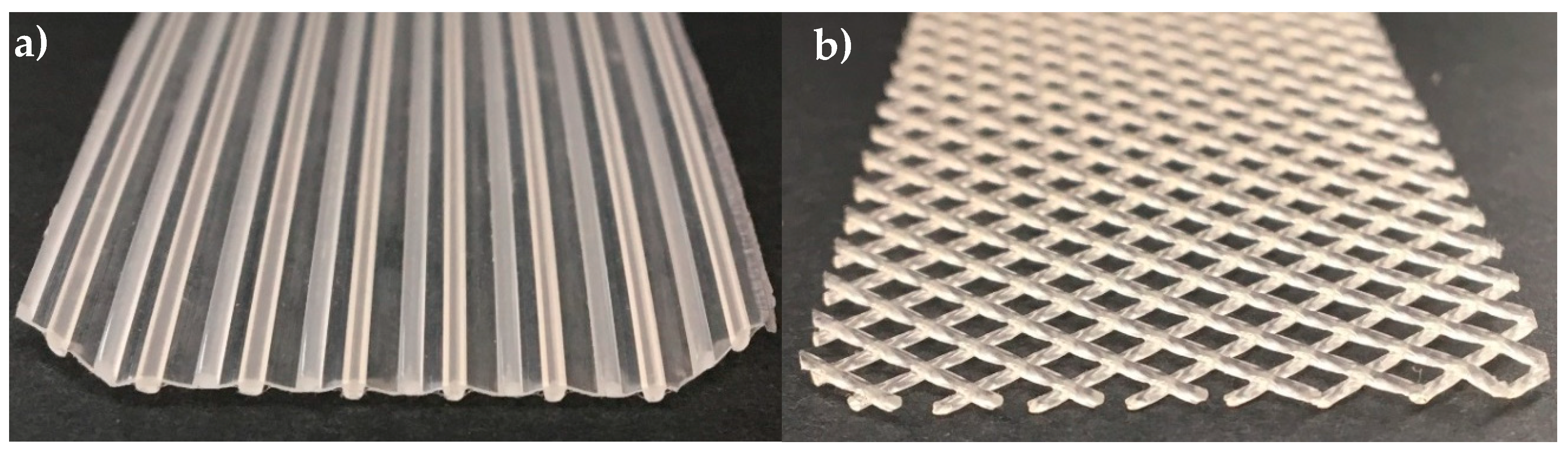
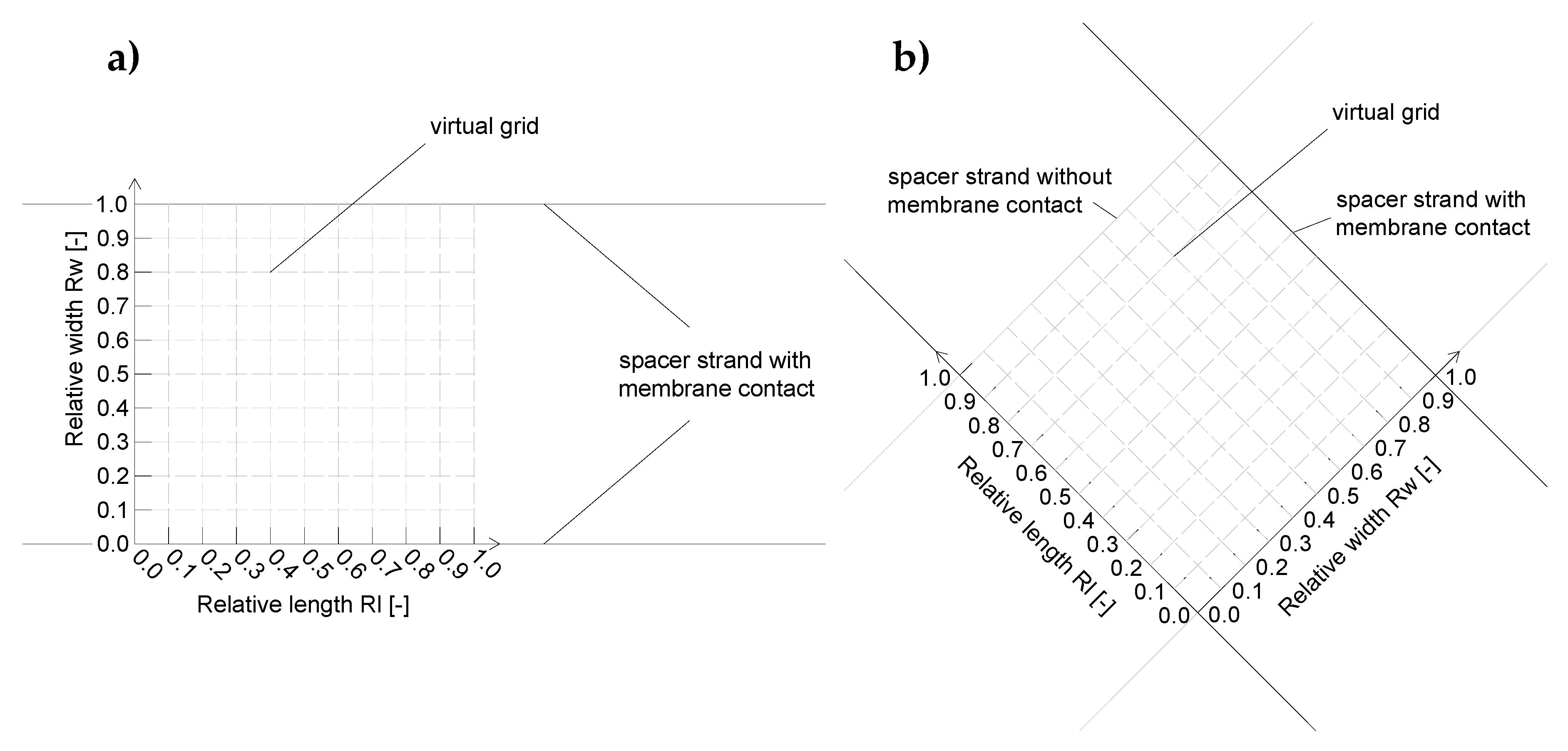
2.3. Spacer Design
2.4. Quantification of Deposited Protein on Membranes
2.5. Membrane Staining and Image Evaluation
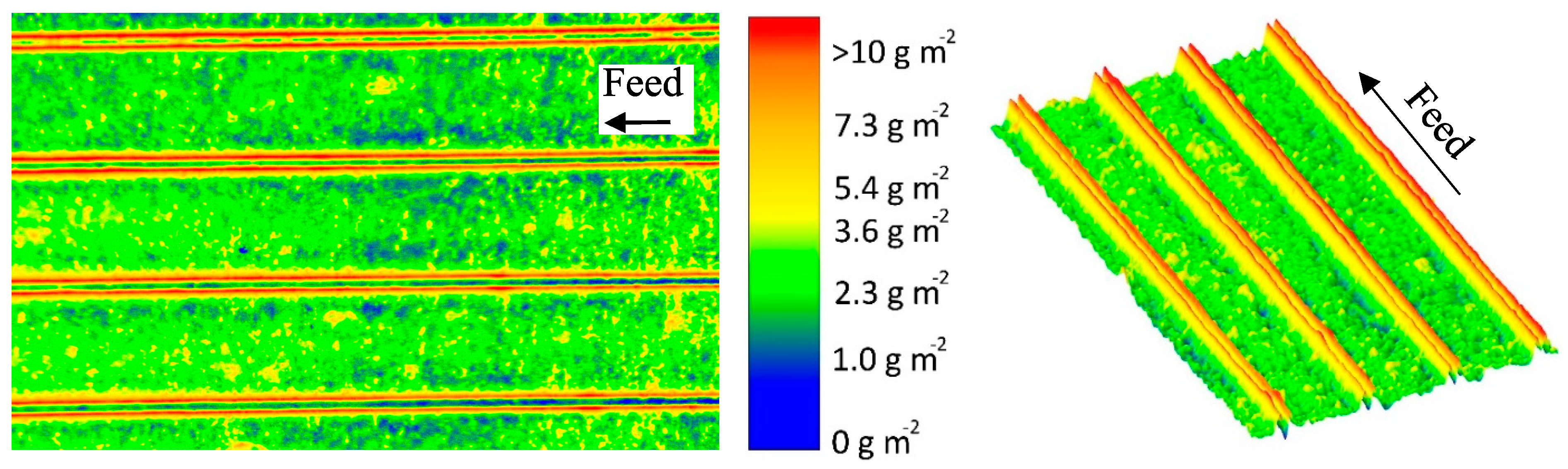
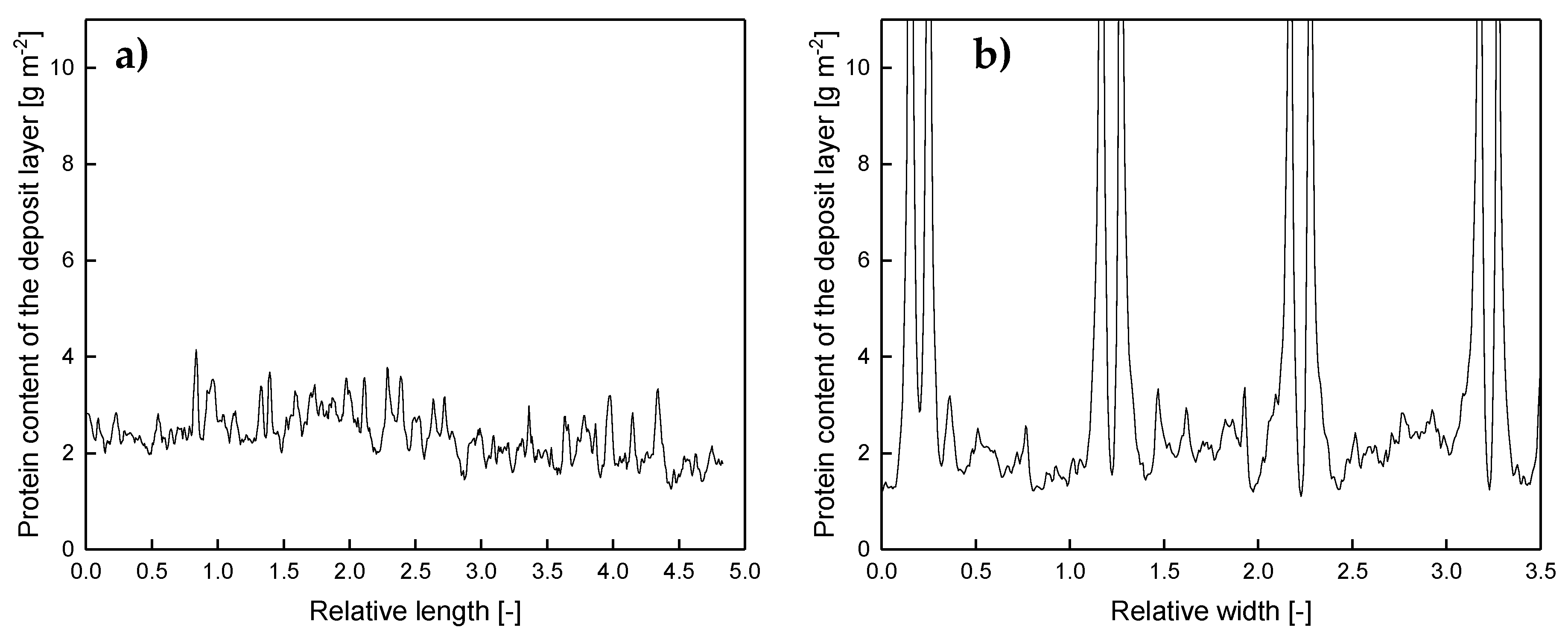
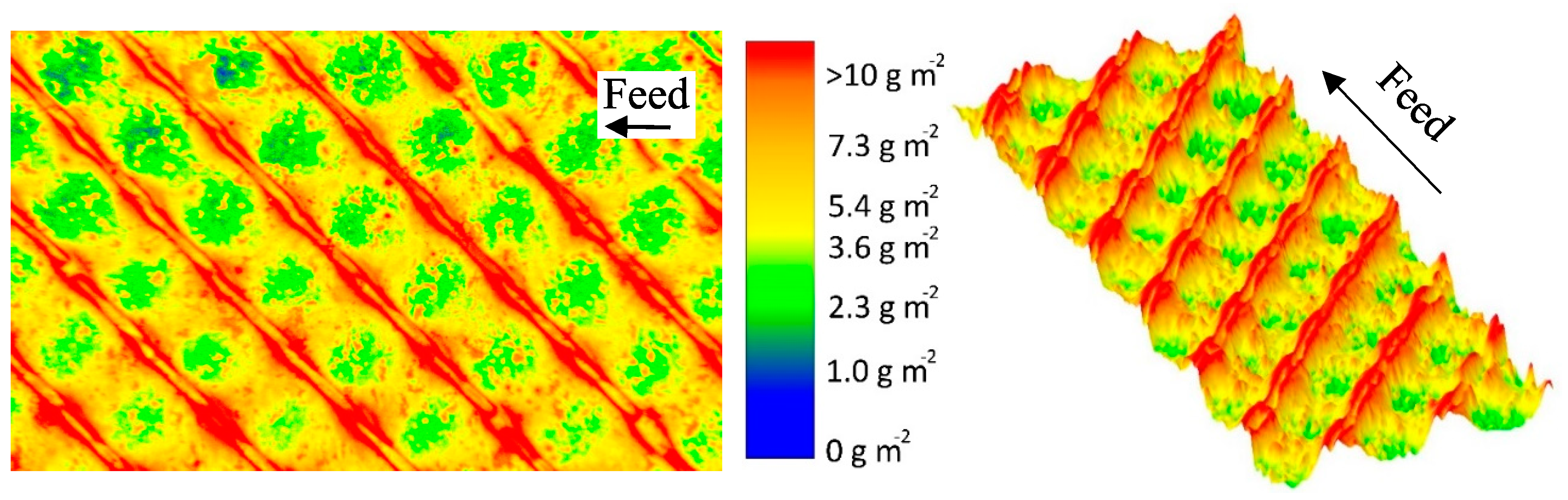
2.5.1. Visualization of the Deposit Layer Distribution in a False Color and a Topographic Image
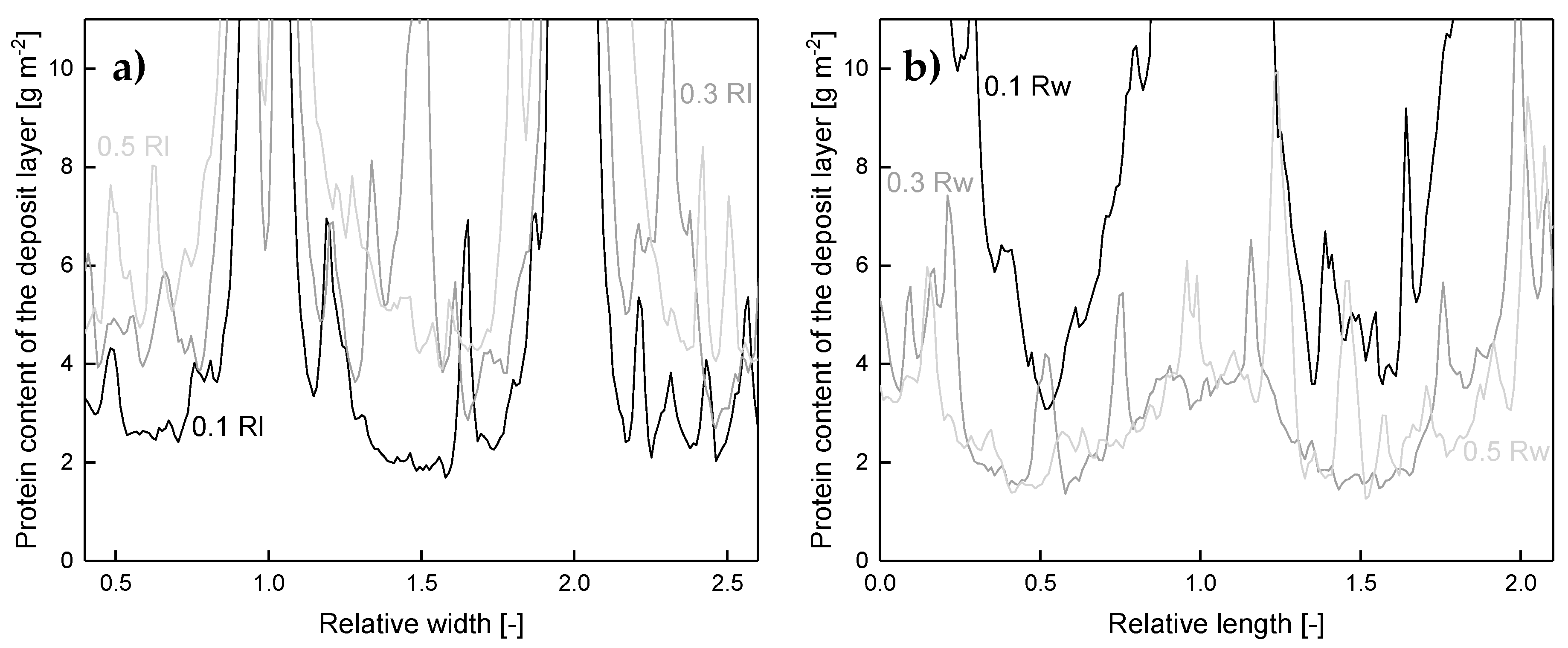
2.5.2. Position-Resolved Evaluation of the Deposited Protein
2.6. Data Plotting and Fitting
3. Results and Discussion
3.1. Deposit Layer Formation Caused by a Parallel Spacer
3.2. Deposit Layer Formation Caused by a Diamond-Shaped Spacer
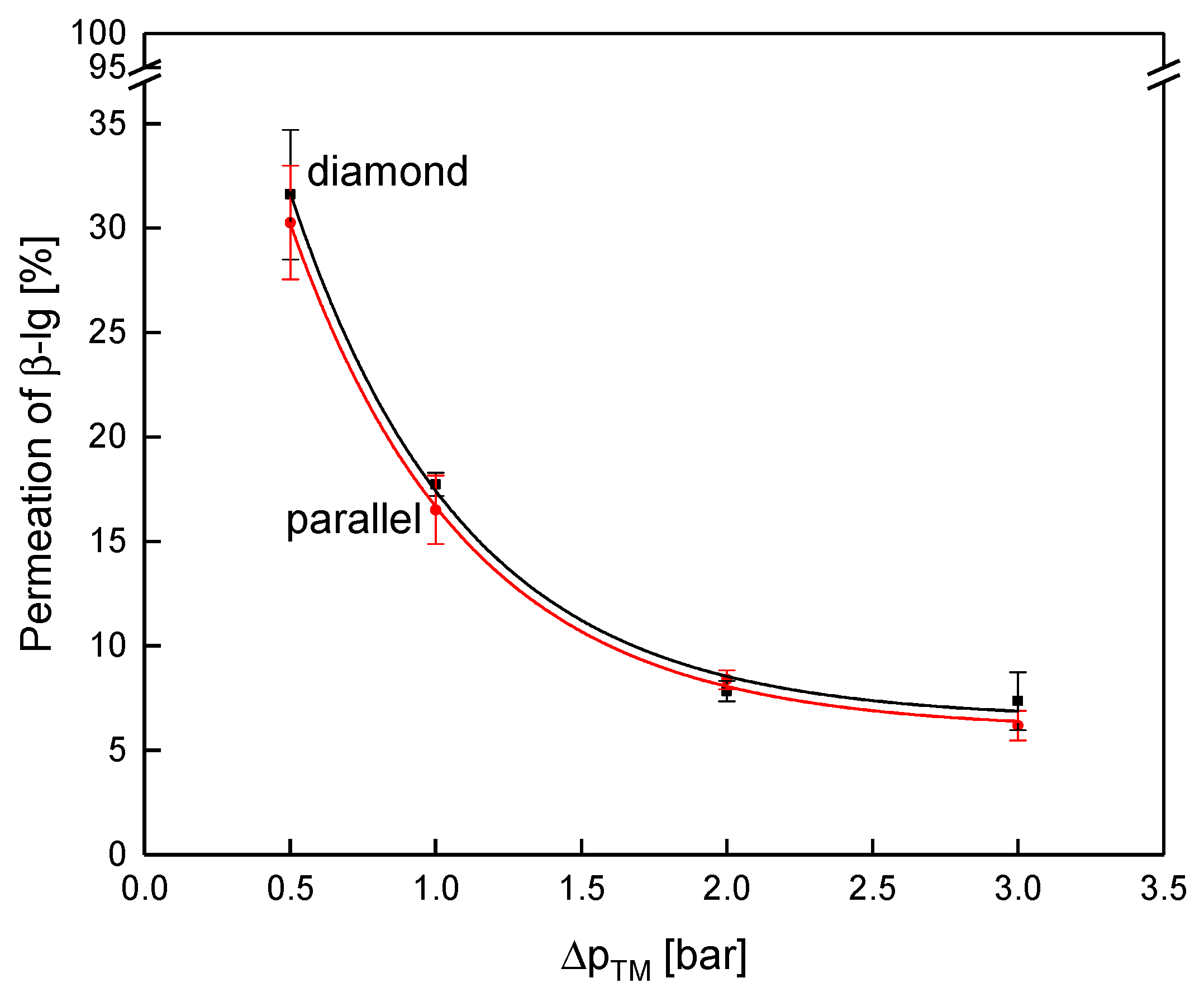
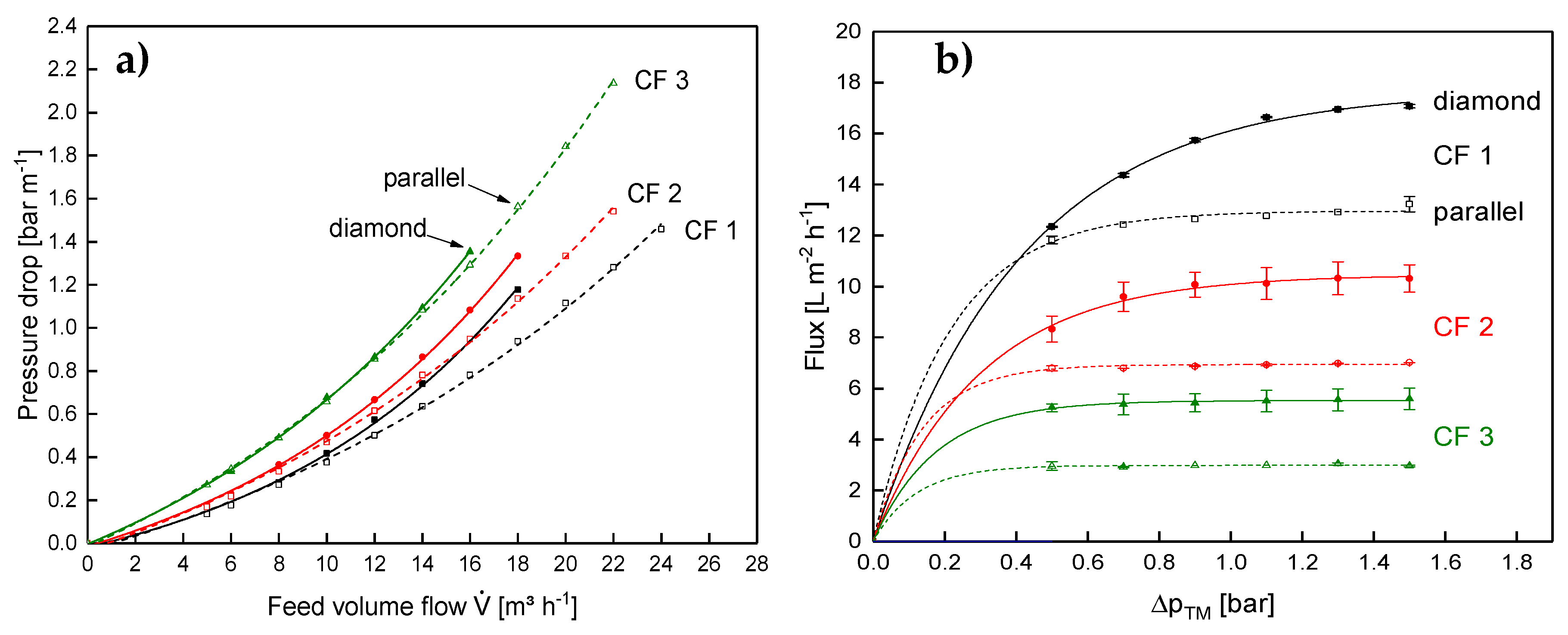
3.3. Filtration Performance of the Diamond and the Parallel Spacer
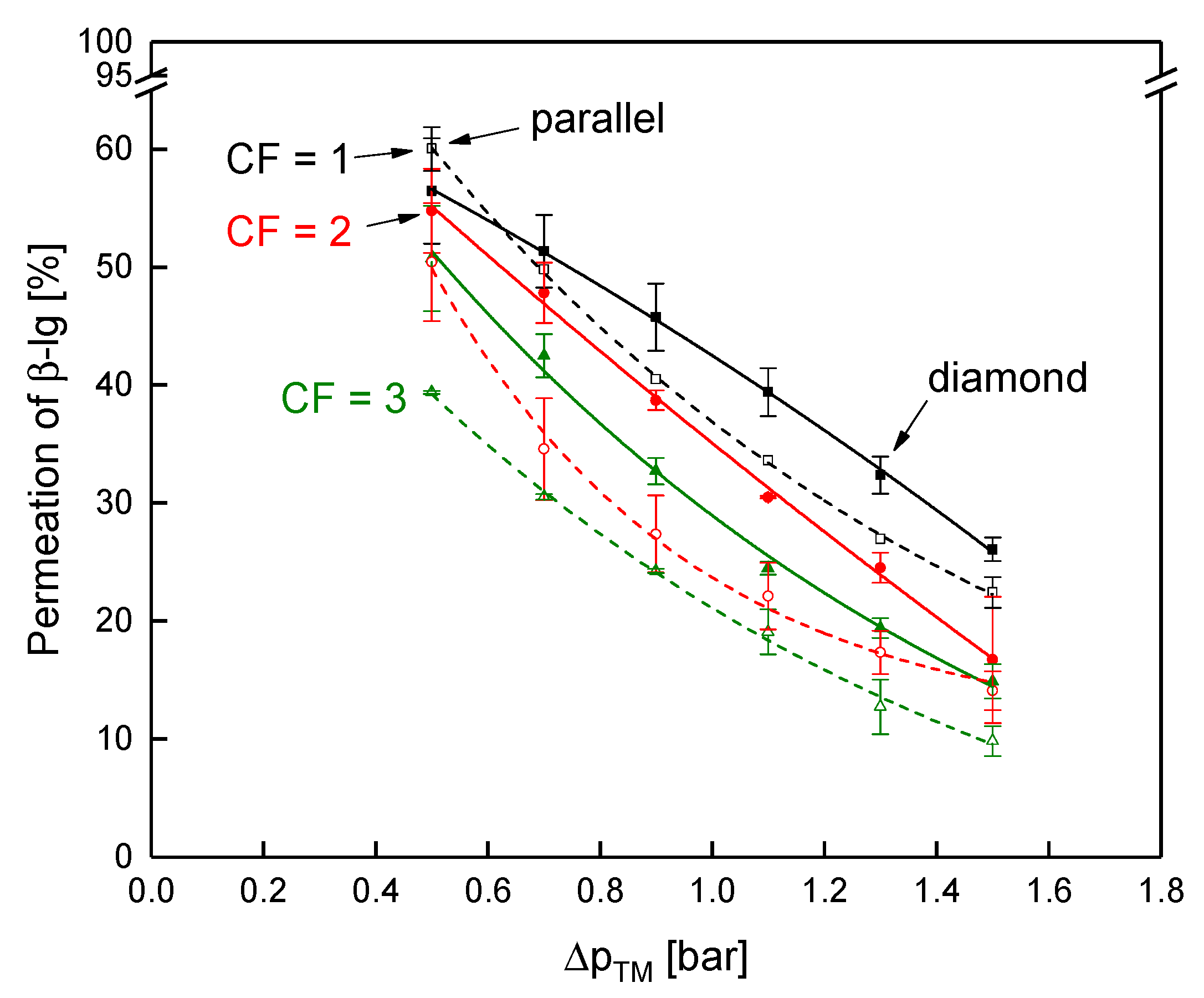
3.4. Filtration Performance of the Diamond and the Parallel Spacer in a Spiral-Wound Membrane at Varying Concentration Factors
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Bacchin, P. A possible link between critical and limiting flux for colloidal systems: Consideration of critical deposit formation along a membrane. J. Membr. Sci. 2004, 228, 237–241. [Google Scholar] [CrossRef] [Green Version]
- Vrouwenvelder, J.S.; Manolarakis, S.A.; van der Hoek, J.P.; van Paassen, J.A.M.; van der Meer, W.G.J.; van Agtmaal, J.M.C.; Prummel, H.D.M.; Kruithof, J.C.; van Loosdrecht, M.C.M. Quantitative biofouling diagnosis in full scale nanofiltration and reverse osmosis installations. Water Res. 2008, 42, 4856–4868. [Google Scholar] [CrossRef] [PubMed]
- Kühnl, W.; Piry, A.; Kaufmann, V.; Grein, T.; Ripperger, S.; Kulozik, U. Impact of colloidal interactions on the flux in cross-flow microfiltration of milk at different pH values: A surface energy approach. J. Membr. Sci. 2010, 352, 107–115. [Google Scholar] [CrossRef]
- Field, R.W.; Pearce, G.K. Critical, sustainable and threshold fluxes for membrane filtration with water industry applications. Adv. Colloid Interface Sci. 2011, 164, 38–44. [Google Scholar] [CrossRef] [PubMed]
- Meyer, P.; Hartinger, M.; Sigler, S.; Kulozik, U. Concentration of Milk and Whey by Membrane Technologies in Alternative Cascade Modes. Food Bioprocess Technol. 2017, 10, 674–686. [Google Scholar] [CrossRef]
- Qu, P.; Gésan-Guiziou, G.; Bouchoux, A. Dead-end filtration of sponge-like colloids: The case of casein micelle. J. Membr. Sci. 2012, 417-418, 10–19. [Google Scholar] [CrossRef] [Green Version]
- Davis, R.H.; Leighton, D.T. Shear-induced transport of a particle layer along a porous wall. Chem. Eng. Sci. 1987, 42, 275–281. [Google Scholar] [CrossRef]
- von der Schulenburg, D.; Vrouwenvelder, J.S.; Creber, S.; van Loosdrecht, M.; Johns, M.L. Nuclear magnetic resonance microscopy studies of membrane biofouling. J. Membr. Sci. 2008, 323, 37–44. [Google Scholar] [CrossRef]
- Hartinger, M.; Heidebrecht, H.-J.; Schiffer, S.; Dumpler, J.; Kulozik, U. Technical Concepts for the Investigation of Spatial Effects in Spiral-Wound Microfiltration Membranes. Membranes 2019, 9, 80. [Google Scholar] [CrossRef] [Green Version]
- Da Costa, A.R.; Fane, A.G.; Wiley, D.E. Spacer characterization and pressure drop modelling in spacer-filled channels for ultrafiltration. J. Membr. Sci. 1994, 87, 79–98. [Google Scholar] [CrossRef]
- Schwinge, J.; Neal, P.R.; Wiley, D.E.; Fletcher, D.F.; Fane, A.G. Spiral wound modules and spacers. J. Membr. Sci. 2004, 242, 129–153. [Google Scholar] [CrossRef]
- Arunkumar, A.; Singh, N.; Schutsky, E.G.; Peck, M.; Swanson, R.K.; Borys, M.C.; Li, Z.J. Effect of channel-induced shear on biologics during ultrafiltration/diafiltration (UF/DF). J. Membr. Sci. 2016, 514, 671–683. [Google Scholar] [CrossRef]
- Binabaji, E.; Ma, J.; Rao, S.; Zydney, A.L. Ultrafiltration of highly concentrated antibody solutions: Experiments and modeling for the effects of module and buffer conditions. Biotechnol. Prog. 2016, 32, 692–701. [Google Scholar] [CrossRef] [PubMed]
- Hartinger, M.; Kulozik, U. Milk protein fractionation by spiral-wound microfiltration membranes in diafiltration mode - Influence of feed protein concentration and composition on the filtration performance. Int. Dairy J. 2019, 104606. [Google Scholar] [CrossRef]
- de Carvalho, I.C. Über das Fließverhalten von Ultrafiltrationskonzentraten aus Milch und dessen Abhängigkeit von der Wärmebehandlung und der Tiefkühllagerung. Ph.D. Thesis, Technische Universität München, München, Germany, July 1986. [Google Scholar]
- Koutsou, C.P.; Yiantsios, S.G.; Karabelas, A.J. A numerical and experimental study of mass transfer in spacer-filled channels: Effects of spacer geometrical characteristics and Schmidt number. J. Membr. Sci. 2009, 326, 234–251. [Google Scholar] [CrossRef]
- Vrouwenvelder, J.S.; Graf von der Schulenburg, D.A.; Kruithof, J.C.; Johns, M.L.; van Loosdrecht, M.C.M. Biofouling of spiral-wound nanofiltration and reverse osmosis membranes: A feed spacer problem. Water Res. 2009, 43, 583–594. [Google Scholar] [CrossRef]
- Gu, B.; Adjiman, C.S.; Xu, X.Y. The effect of feed spacer geometry on membrane performance and concentration polarisation based on 3D CFD simulations. J. Membr. Sci. 2017, 527, 78–91. [Google Scholar] [CrossRef]
- Hartinger, M.; Napiwotzki, J.; Schmid, E.-M.; Kurz, F.; Kulozik, U. Semi-quantitative, spatially resolved analysis of protein deposit layers on membrane surfaces. MethodsX 2020, 7, 100780. [Google Scholar] [CrossRef]
- Hartinger, M.; Heidebrecht, H.-J.; Schiffer, S.; Dumpler, J.; Kulozik, U. Milk protein fractionation by means of spiral-wound microfiltration membranes: Effect of the pressure adjustment mode and temperature on flux and protein permeation. Foods 2019, 8, 180. [Google Scholar] [CrossRef] [Green Version]
- Dumpler, J.; Wohlschläger, H.; Kulozik, U. Dissociation and coagulation of caseins and whey proteins in concentrated skim milk heated by direct steam injection. Dairy Sci. Technol. 2017, 96, 807–826. [Google Scholar] [CrossRef]
- Jimenez-Lopez, A.; Leconte, N.; Dehainault, O.; Geneste, C.; Fromont, L.; Gésan-Guiziou, G. Role of milk constituents on critical conditions and deposit structure in skimmilk microfiltration (0.1 μm). Sep. Purif. Technol. 2008, 61, 33–43. [Google Scholar] [CrossRef]
- Kavianipour, O.; Ingram, G.D.; Vuthaluru, H.B. Investigation into the effectiveness of feed spacer configurations for reverse osmosis membrane modules using Computational Fluid Dynamics. J. Membr. Sci. 2017, 526, 156–171. [Google Scholar] [CrossRef] [Green Version]
- Grosse-Gorgemann, A.; Hahne, W.; Fiebig, M. Influence of the rib heights on oscillations, heat transfer and pressure drop in laminar channel flow. In Proceedings of the Eurotherm 31 “Vortices and Heat Transfer”, Bochum, Germany, 24–26 May 1993. [Google Scholar]
- Fiebig, M. Vortex Generators for Compact Heat Exchangers. J. Enh. Heat Transf. 1995, 2, 43–61. [Google Scholar] [CrossRef]
- Li, F.; Meindersma, W.; de Haan, A.B.; Reith, T. Novel spacers for mass transfer enhancement in membrane separations. J. Membr. Sci. 2005, 253, 1–12. [Google Scholar] [CrossRef]
- Beckman, S.L.; Barbano, D.M. Effect of microfiltration concentration factor on serum protein removal from skim milk using spiral-wound polymeric membranes. J. Dairy Sci. 2013, 96, 6199–6212. [Google Scholar] [CrossRef]
- Samuelsson, G.; Dejmek, P.; Trägårdh, G.; Paulsson, M. Minimizing whey protein retention in cross-flow microfiltration of skim milk. Int. Dairy J. 1997, 7, 237–242. [Google Scholar] [CrossRef]
- Steinhauer, T.; Kühnl, W.; Kulozik, U. Impact of Protein Interactions and Transmembrane Pressure on Physical Properties of Filter Cakes Formed during Filtrations of Skim Milk. Procedia Food Sci. 2011, 1, 886–892. [Google Scholar] [CrossRef] [Green Version]
- Karabelas, A.J.; Koutsou, C.P.; Sioutopoulos, D.C. Comprehensive performance assessment of spacers in spiral-wound membrane modules accounting for compressibility effects. J. Membr. Sci. 2018, 549, 602–615. [Google Scholar] [CrossRef]



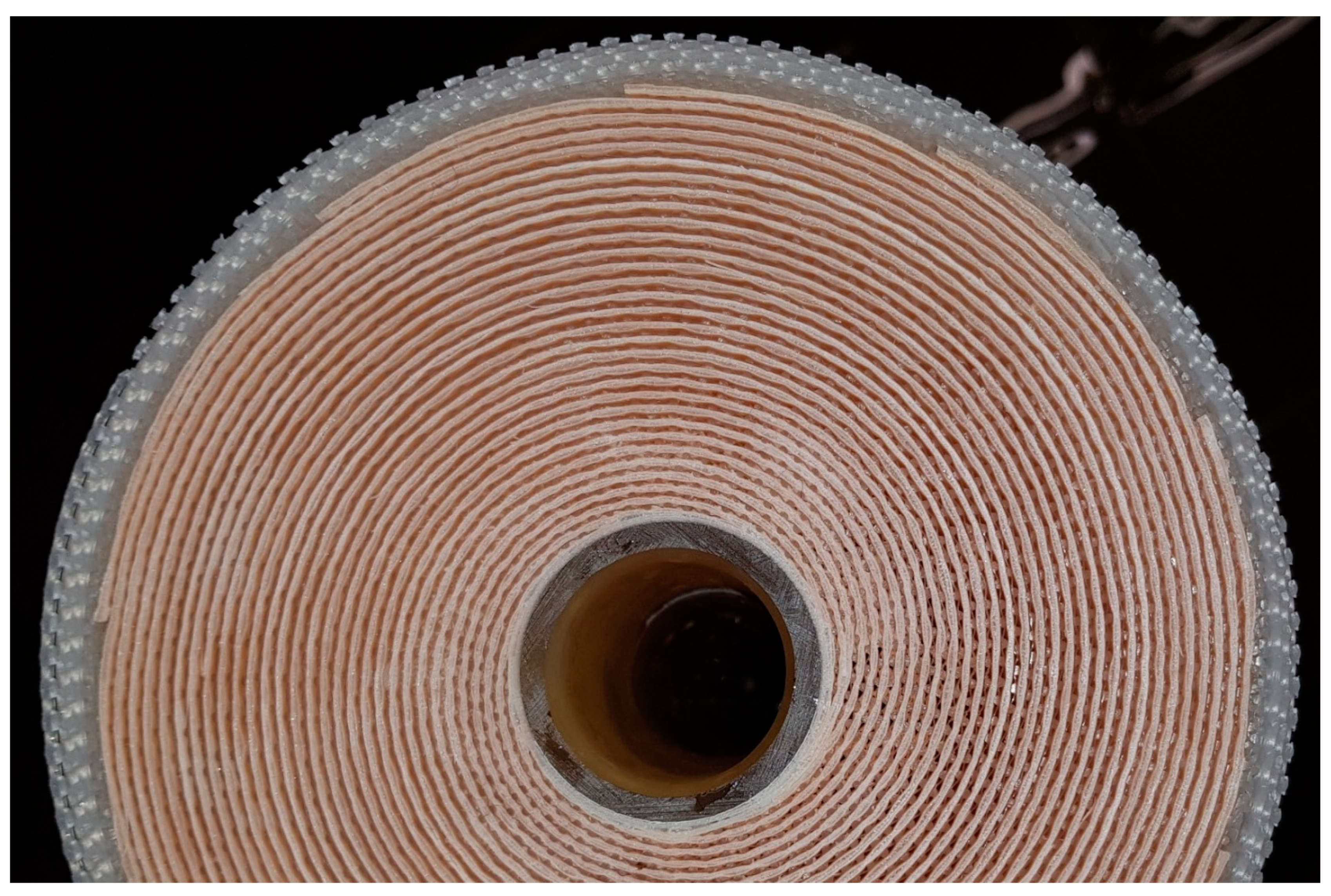
| Length [m] | Active Membrane Area [m2] | |
|---|---|---|
| Test cell | 0.2 | 8.00 × 10−3 |
| SWM | 0.96 | 16.35 |
| Mean Value [g L−1] | Standard Deviation [g L−1] | Mean Value [g L−1] | Standard Deviation [g L−1] | ||
|---|---|---|---|---|---|
| κ-casein | 12.21 | 1.05 | αS1-casein | 14.20 | 2.02 |
| β-casein | 41.46 | 2.26 | αS2-casein | 36.22 | 0.42 |
| α-la | 4.58 | 0.03 | β-lg | 12.96 | 0.33 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hartinger, M.; Napiwotzki, J.; Schmid, E.-M.; Hoffmann, D.; Kurz, F.; Kulozik, U. Influence of Spacer Design and Module Geometry on the Filtration Performance during Skim Milk Microfiltration with Flat Sheet and Spiral-Wound Membranes. Membranes 2020, 10, 57. https://doi.org/10.3390/membranes10040057
Hartinger M, Napiwotzki J, Schmid E-M, Hoffmann D, Kurz F, Kulozik U. Influence of Spacer Design and Module Geometry on the Filtration Performance during Skim Milk Microfiltration with Flat Sheet and Spiral-Wound Membranes. Membranes. 2020; 10(4):57. https://doi.org/10.3390/membranes10040057
Chicago/Turabian StyleHartinger, Martin, Jonas Napiwotzki, Eva-Maria Schmid, Dominik Hoffmann, Franziska Kurz, and Ulrich Kulozik. 2020. "Influence of Spacer Design and Module Geometry on the Filtration Performance during Skim Milk Microfiltration with Flat Sheet and Spiral-Wound Membranes" Membranes 10, no. 4: 57. https://doi.org/10.3390/membranes10040057
APA StyleHartinger, M., Napiwotzki, J., Schmid, E.-M., Hoffmann, D., Kurz, F., & Kulozik, U. (2020). Influence of Spacer Design and Module Geometry on the Filtration Performance during Skim Milk Microfiltration with Flat Sheet and Spiral-Wound Membranes. Membranes, 10(4), 57. https://doi.org/10.3390/membranes10040057




