Oxygen Depletion and the Role of Cellular Antioxidants in FLASH Radiotherapy: Mechanistic Insights from Monte Carlo Radiation-Chemical Modeling
Abstract
1. Introduction
1.1. On the FLASH Effect
1.2. Water Radiolysis in Cellular Environments
1.3. Investigating the Role of Cellular Antioxidants in FLASH-RT
2. Materials and Methods
2.1. Determining the Effects of Dose Rates Using the ‘Instantaneous Pulse’ (Dirac) Model
2.2. Monte Carlo Multi-Track Chemistry Simulations Using the IONLYS-IRT Code
2.3. Our Irradiated Cell Water Model: Proposed Chemical Reaction Scheme
- -
- -
- Carbon-based biomolecules (RH), such as DNA and RNA (present in much lower concentrations than proteins and lipids), proteins (the primary components by weight in most cells), free amino acids, free nucleotides, and lipids (mainly in cell membranes), typically occur at a concentration of [RH] ~ 1 M [62,130]. This concentration has been previously utilized in the literature (e.g., see [62,67]). In our simulations, 1 M denotes the ‘oxidizable’ substrate concentration in a living system, as defined by Qian and Buettner [130]. Nevertheless, a 1 M concentration of bio-organic molecules may appear high when averaged across an entire cell. To account for some cellular heterogeneity, we also tested a 0.5 M concentration, both for comparison and to evaluate the sensitivity of the results to [RH] variations.
- -
- For simplicity, as noted above, our model does not distinguish between these macromolecular constituents. We acknowledge that using a single RH species poorly captures variations in radiation-induced cellular damage, particularly the differences between DNA and lipids. To improve accuracy, we are currently developing a more detailed compartmental cell model akin to those proposed by Hu et al. [65] and Babbs and Steiner [106].
- -
- Under the specific conditions of FLASH irradiation employed in this study—a ~30 Gy dose delivered with 300-MeV protons (LET ~ 0.3 keV/μm) at an instantaneous dose rate of ~106–107 Gy/s [68,69]—we estimate an initial bio-radical (R●) concentration of ~2.5 μM. These bio-radicals originate from the ‘direct’ ionization of RH, followed by the deprotonation of the resulting radical cations (RH●+). This concentration estimate is derived from direct action yields reported in previous studies [40,62,65].
| Symbol | Reaction | k | References |
|---|---|---|---|
| (R1) | RH + e−aq → (RH)•− | 1.5 × 109 | [61,131,132] |
| (R2) | RH + H• → RH(+H)• | 8 × 107 | [61,131,132,133] |
| (R3) | O2 + e−aq → O2•− | 1.9 × 1010 | [74] |
| (R4) | O2 + H• → HO2• → O2•− + H+ (pKa = 4.8) c | 2.1 × 1010 | [60,74] |
| (R5) | O2•− + O2•− + 2H+ → O2 + H2O2 d | 4 × 109 | [82,92,134] |
| (R6) | H2O2 + H2O2 → 2H2O + O2 e | 2 × 107 | [82] |
| (1) | RH + •OH → R• + H2O | 5 × 108 | [56,57,61,74,132] |
| (2) | R• + O2 → ROO• | 2 × 109 | [75,76,77,78] |
| (R7) | R• + R• → R–R | 108 | [62,106] |
| (R8) | R• + ROO• → ROOR | 5 × 107 | [106] |
| (R9) | ROO• + ROO• → products | 105 | [62,65,75] |
| (R10) | ROO• + RH → ROOH + R• | 1.3 × 103 | [62,65,75] |
| (R11) | ROO• + O2•− + H+ → ROOH + O2 | 5 × 107 | [82,106,135] |
| (R12) | e−aq + ROOH → RO• + OH− | 1010 | [56] |
| (R13) | RO• + RH → ROH + R• | 5 × 104 f | [57] |
| (5) | ROO• + GSH → GS• + ROOH g | 5 × 104 | [77,106] |
| (R14) | R• + GSH → GS• + RH | 5.6 × 106 | [65] |
| (R15) | GSH + e−aq + H+ → G• + H2S | 4.5 × 109 | [74] |
| (R16) | GSH + H• → GS• + H2 h | 1.8 × 109 | [136] |
| (R17) | GSH + •OH → GS• + H2O | 1.4 × 1010 | [74,106,137,138] |
| (R18) | GS• + O2 → GSOO• | 2 × 109 | [65,139,140,141] |
| (R19) | GSOO• → GS• + O2 | 6.2 × 105 | [65,108,130] |
| (R20) | GSOO• + GSH → GSO• + GSOH | 2 × 106 | [108] |
| (R21) | GS• + GS• → GSSG i | 7.5 × 108 | [82,139,142] |
| (R22) | GS• + GSH → GSSG•− + H+ | 3.5 × 108 | [65,136,137,142] |
| (R23) | GSSG•− + O2 → GSSG + O2•− | 5.1 × 108 | [82,139,140,141] |
| (R24) | GSSG + e−aq → GSSG•− | 3.7 × 109 | [74] |
| (R25) | GSSG + H• → GSH + GS• | 1010 | [74] |
| (R26) | GSSG + •OH → GSSG•+ + OH− | 1.7 × 1010 | [136] |
| (R27) | GSSG•+ + GSSG•+ → GSSG2+ + GSSG | 2.5 × 109 | [136] |
| (R28) | GS• + •NO → GSNO | 3 × 109 | [142] |
| (R29) | GS• + GSNO → GSSG + •NO | 1.7 × 109 | [142] |
| (R30) | GS• + AH− → GSH + A•− j,k | 6 × 108 | [65,82,139,140] |
| (R31) | R• + AH− → RH + A•− | 107 | [65] |
| (7) | ROO• + AH− → ROOH + A•− | 2.2 × 106 | [63,76,77,109,113,114,115] |
| (R32) | RO• + AH− → ROH + A•− | 1.6 × 109 | [114,115] |
| (R33) | AH− + O2•− + H+ → A•− + H2O2 | 2.7 × 105 | [82,114,115,116,139] |
| (R34) | AH− + •OH → A•− + H2O | 1.1 × 1010 | [74,82,114,115] |
| (R35) | A•− + A•− + H+ → AH− + A l | 2.8 × 105 | [113,114,115] |
| (R36) | A•− + O2•− + 2H+ → A + H2O2 | 2.6 × 108 | [113,114] |
| (R37) | •OH + TOH → TO• + H2O m | 1010 | [106] |
| (R38) | R• + TOH → RH + TO• | 2.5 × 106 | [65,106] |
| (R39) | ROO• + TOH → ROOH + TO• | 5 × 105 | [56,65,82,99,101,106] |
| (R40) | TO• + AH− → TOH + A•− | 1.3 × 107 | [114] |
| (3) | NO• + O2•− → ONOO− n | 1.9 × 1010 | [95,96,97,98,143,144,145] |
| (R41) | NO• + •OH → HNO2 | 1010 | [82] |
| (R42) | R• + •NO → RNO | 2 × 109 | [129] |
| (4) | ROO• + •NO → ROONO | 2 × 109 o | [98,99,100,101,102] |
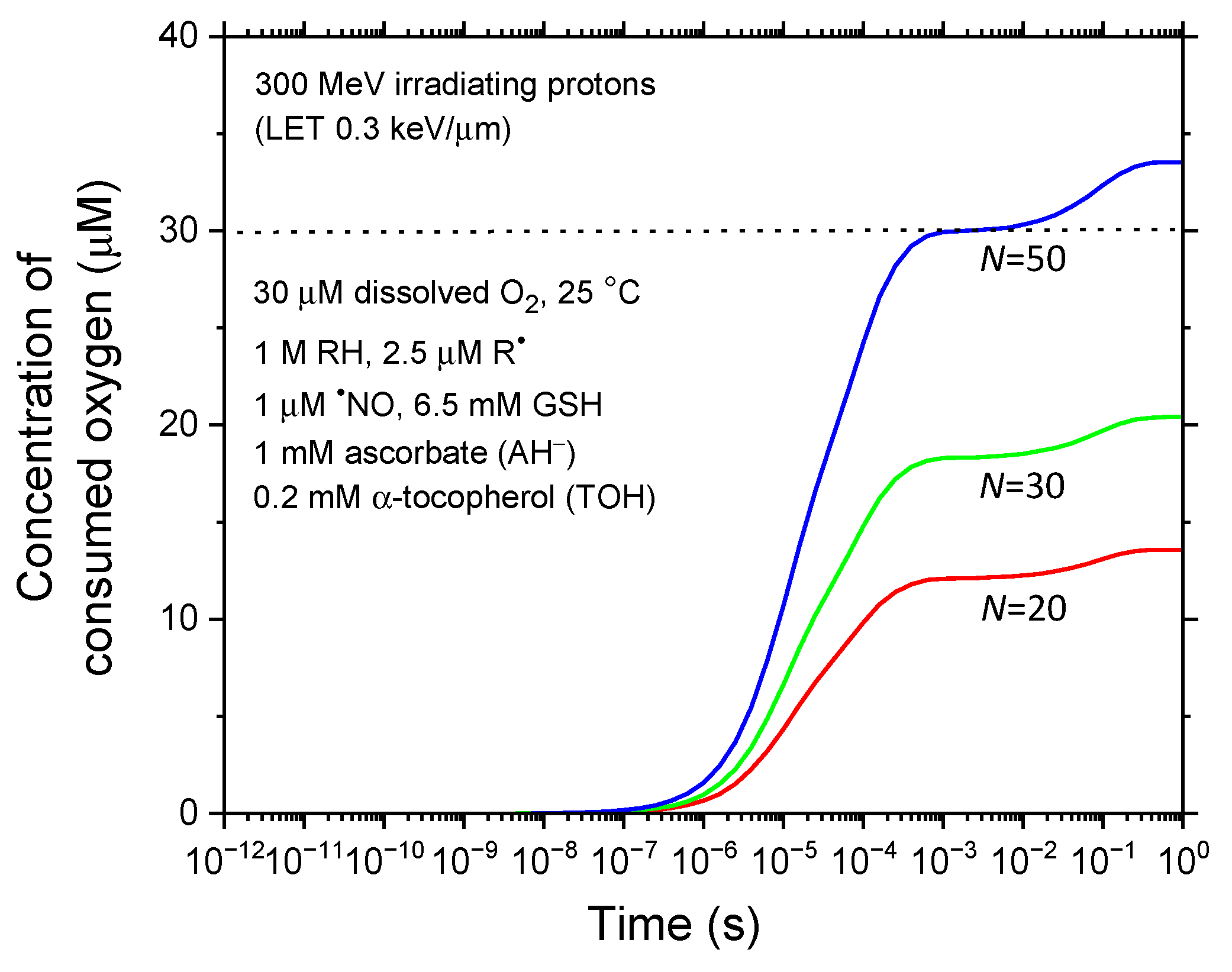
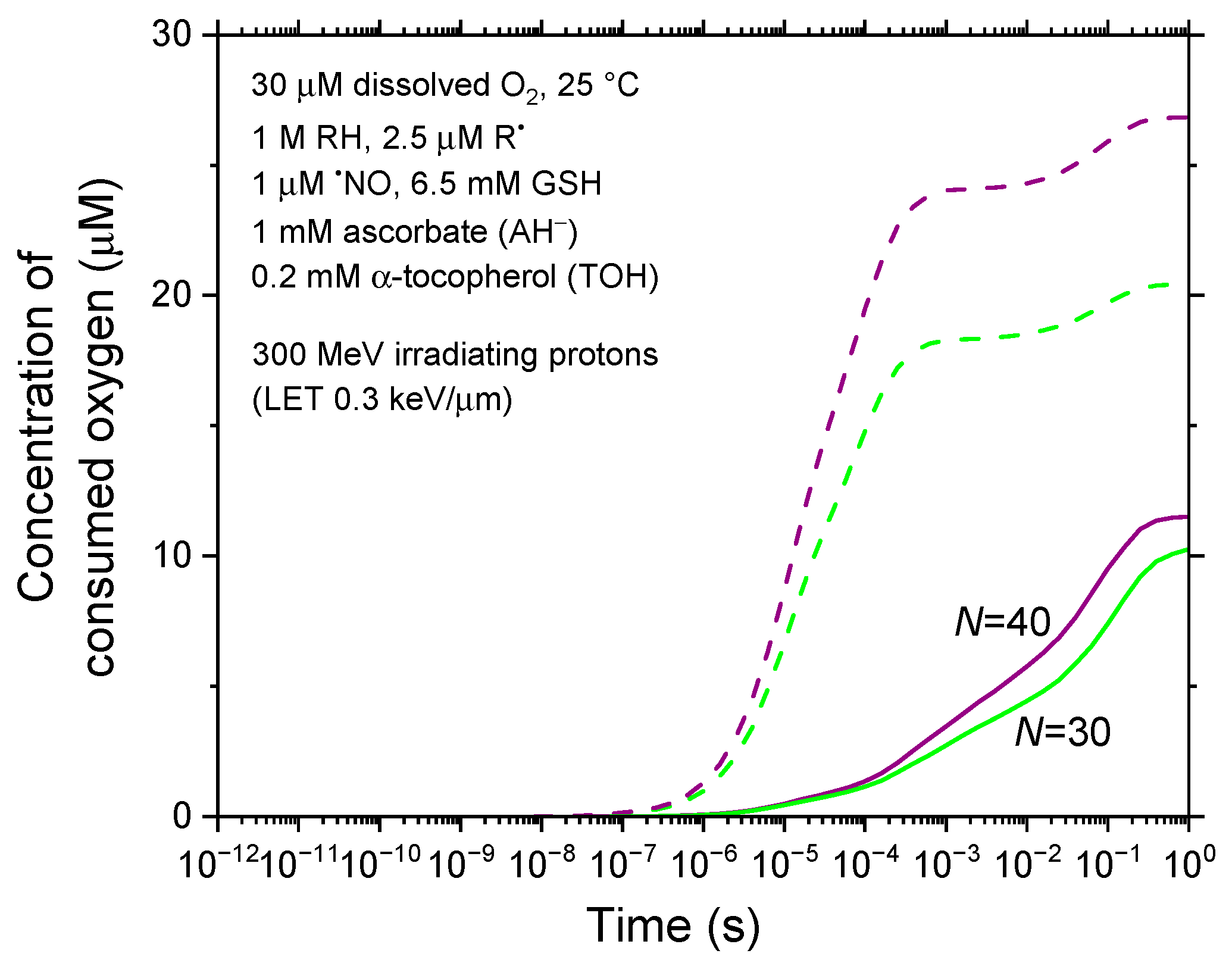
3. Results and Discussion
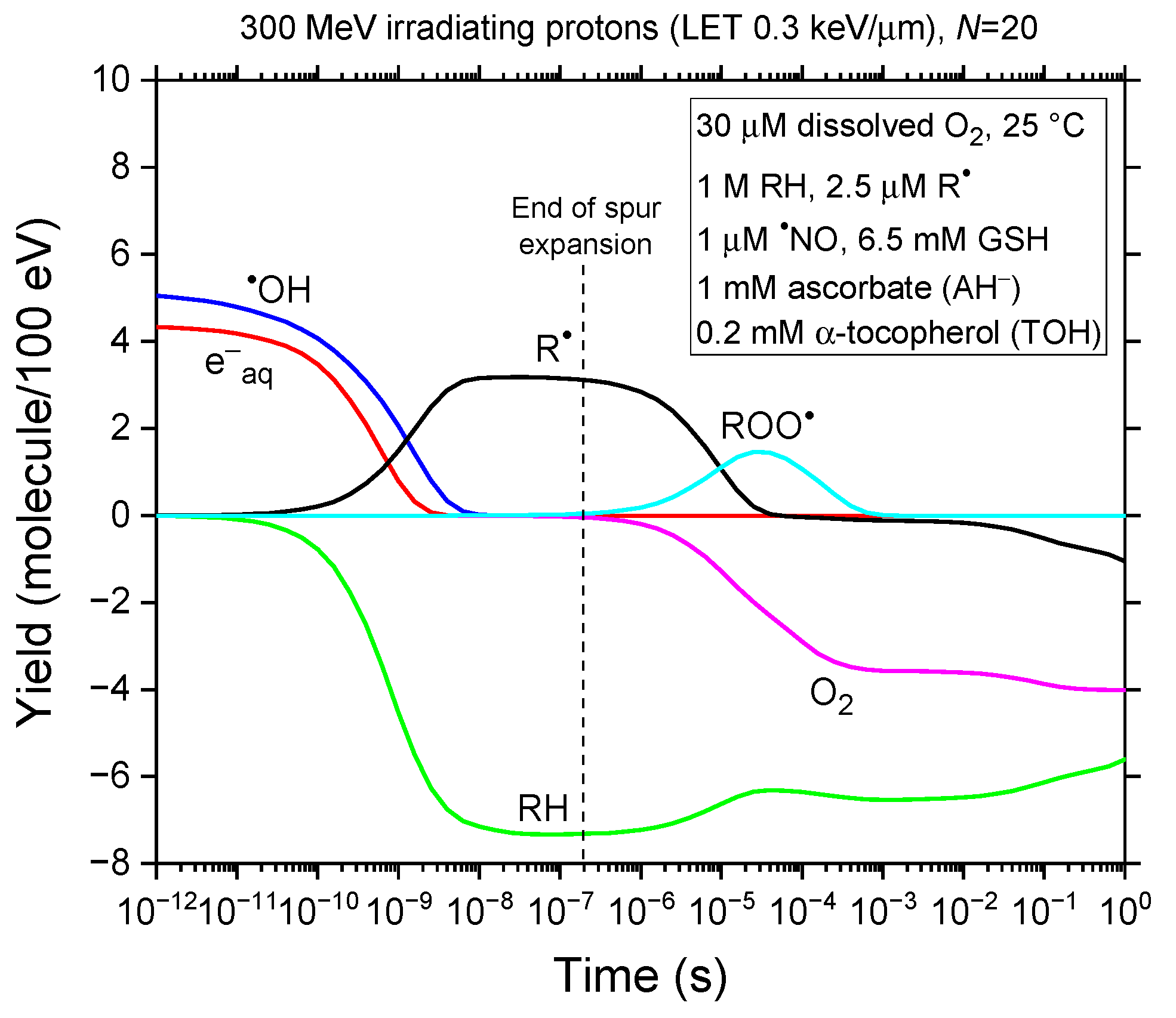
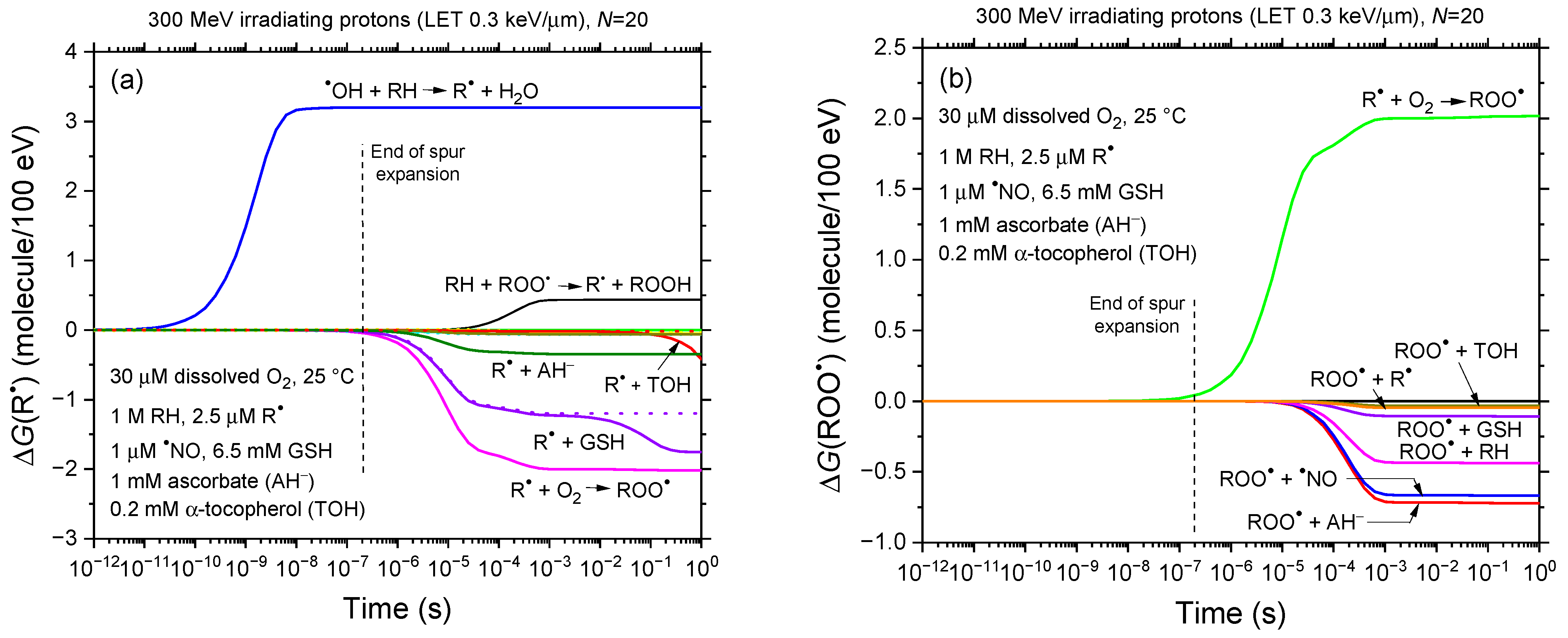
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Halperin, E.C.; Brady, L.W.; Perez, C.A.; Wazer, D.E. Perez & Brady’s Principles and Practice of Radiation Oncology; Lippincott Williams & Wilkins: Philadelphia, PA, USA, 2013. [Google Scholar]
- Favaudon, V.; Caplier, L.; Monceau, V.; Pouzoulet, F.; Sayarath, M.; Fouillade, C.; Poupon, M.-F.; Brito, I.; Hupé, P.; Bourhis, J.; et al. Ultrahigh dose-rate FLASH irradiation increases the differential response between normal and tumor tissue in mice. Sci. Transl. Med. 2014, 6, 245ra93. [Google Scholar] [CrossRef]
- Favaudon, V.; Fouillade, C.; Vozenin, M.-C. Ultrahigh dose-rate, “flash” irradiation minimizes the side-effects of radiotherapy. Cancer Radiother. 2015, 19, 526–531. [Google Scholar] [CrossRef] [PubMed]
- Favaudon, V.; Fouillade, C.; Vozenin, M.-C. La radiothérapie FLASH pour épargner les tissus sains. Med. Sci. 2015, 31, 121–123. [Google Scholar] [CrossRef]
- Wilson, J.D.; Hammond, E.M.; Higgins, G.S.; Petersson, K. Ultra-high dose rate (FLASH) radiotherapy: Silver bullet or fool’s gold? Front. Oncol. 2020, 9, 1563. [Google Scholar] [CrossRef]
- Esplen, N.; Mendonca, M.S.; Bazalova-Carter, M. Physics and biology of ultrahigh dose-rate (FLASH) radiotherapy: A topical review. Phys. Med. Biol. 2020, 65, 23TR03. [Google Scholar] [CrossRef] [PubMed]
- Marcu, L.G.; Bezak, E.; Peukert, D.D.; Wilson, P. Translational research in FLASH radiotherapy—From radiobiological mechanisms to in vivo results. Biomedicines 2021, 9, 181. [Google Scholar] [CrossRef]
- Gao, Y.; Liu, R.; Chang, C.-W.; Charyyev, S.; Zhou, J.; Bradley, J.D.; Liu, T.; Yang, X. A potential revolution in cancer treatment: A topical review of FLASH radiotherapy. J. Appl. Clin. Med. Phys. 2022, 23, e13790. [Google Scholar] [CrossRef]
- Matuszak, N.; Suchorska, W.M.; Milecki, P.; Kruszyna-Mochalska, M.; Misiarz, A.; Pracz, J.; Malicki, J. FLASH radiotherapy: An emerging approach in radiation therapy. Rep. Pract. Oncol. Radiother. 2022, 27, 344–351. [Google Scholar] [CrossRef] [PubMed]
- Bogaerts, E.; Macaeva, E.; Isebaert, S.; Haustermans, K. Potential molecular mechanisms behind the ultra-high dose rate “FLASH” effect. Int. J. Mol. Sci. 2022, 23, 12109. [Google Scholar] [CrossRef]
- Borghini, A.; Labate, L.; Piccinini, S.; Panaino, C.M.V.; Andreassi, M.G.; Gizzi, L.A. FLASH radiotherapy: Expectations, challenges, and current knowledge. Int. J. Mol. Sci. 2024, 25, 2546. [Google Scholar] [CrossRef]
- Tang, R.; Yin, J.; Liu, Y.; Xue, J. FLASH radiotherapy: A new milestone in the field of cancer radiotherapy. Cancer Lett. 2024, 587, 216651. [Google Scholar] [CrossRef] [PubMed]
- Bondy, S.C. FLASH radiotherapy versus conventional cancer therapy: Promises, paradoxes and problems. Int. J. Transl. Med. 2024, 4, 559–569. [Google Scholar] [CrossRef]
- Chow, J.C.L.; Ruda, H.E. Mechanisms of action in FLASH radiotherapy: A comprehensive review of physicochemical and biological processes on cancerous and normal cells. Cells 2024, 13, 835. [Google Scholar] [CrossRef]
- Ma, Y.; Zhang, W.; Zhao, Z.; Lv, J.; Chen, J.; Yan, X.; Lin, X.; Zhang, J.; Wang, B.; Gao, S.; et al. Current views on mechanisms of the FLASH effect in cancer radiotherapy. Natl. Sci. Rev. 2024, 11, nwae350. [Google Scholar] [CrossRef]
- Yan, O.; Wang, S.; Wang, Q.; Wang, X. FLASH radiotherapy: Mechanisms of biological effects and the therapeutic potential in cancer. Biomolecules 2024, 14, 754. [Google Scholar] [CrossRef] [PubMed]
- Scarmelotto, A.; Delprat, V.; Michiels, C.; Lucas, S.; Heuskin, A.-C. The oxygen puzzle in FLASH radiotherapy: A comprehensive review and experimental outlook. Clin. Transl. Radiat. Oncol. 2024, 49, 100860. [Google Scholar] [CrossRef] [PubMed]
- Shiraishi, Y.; Matsuya, Y.; Fukunaga, H. Possible mechanisms and simulation modeling of FLASH radiotherapy. Radiol. Phys. Technol. 2024, 17, 11–23. [Google Scholar] [CrossRef]
- Li, M.; Zhou, S.; Dong, G.; Wang, C. Emergence of FLASH-radiotherapy across the last 50 years (Review). Oncol. Lett. 2024, 28, 602. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, H.; Hu, J.; Chai, J.; Luan, J.; Li, J.; Xu, Q. FLASH radiotherapy: Mechanisms, nanotherapeutic strategy and future development. Nanoscale Adv. 2025, 7, 711–721. [Google Scholar] [CrossRef]
- Manring, H.R.; Fleming, J.L.; Meng, W.; Gamez, M.E.; Blakaj, D.M.; Chakravarti, A. FLASH radiotherapy: From in vivo data to clinical translation. Hematol. Oncol. Clin. N. Am. 2025, 39, 237–255. [Google Scholar] [CrossRef]
- Friedl, A.A.; Prise, K.M.; Butterworth, K.T.; Montay-Gruel, P.; Favaudon, V. Radiobiology of the FLASH effect. Med. Phys. 2022, 49, 1993–2013. [Google Scholar] [CrossRef] [PubMed]
- Hageman, E.; Che, P.-P.; Dahele, M.; Slotman, B.J.; Sminia, P. Radiobiological aspects of FLASH radiotherapy. Biomolecules 2022, 12, 1376. [Google Scholar] [CrossRef] [PubMed]
- Limoli, C.L.; Vozenin, M.-C. Reinventing radiobiology in the light of FLASH radiotherapy. Annu. Rev. Cancer Biol. 2023, 7, 1–21. [Google Scholar] [CrossRef]
- Rezaee, M.; Iordachita, I.; Wong, J.W. Ultrahigh dose-rate (FLASH) x-ray irradiator for pre-clinical laboratory research. Phys. Med. Biol. 2021, 66, 095006. [Google Scholar] [CrossRef]
- Montay-Gruel, P.; Corde, S.; Laissue, J.A.; Bazalova-Carter, M. FLASH radiotherapy with photon beams. Med. Phys. 2022, 49, 2055–2067. [Google Scholar] [CrossRef]
- Gao, F.; Yang, Y.; Zhu, H.; Wang, J.; Xiao, D.; Zhou, Z.; Dai, T.; Zhang, Y.; Feng, G.; Li, J.; et al. First demonstration of the FLASH effect with ultrahigh dose rate high-energy X-rays. Radiother. Oncol. 2022, 166, 44–50. [Google Scholar] [CrossRef]
- Yang, Y.; Wang, J.; Gao, F.; Liu, Z.; Dai, T.; Zhang, H.; Zhu, H.; Wang, T.; Xiao, D.; Zhou, Z.; et al. FLASH radiotherapy using high-energy X-rays: Current status of PARTER platform in FLASH research. Radiother. Oncol. 2024, 190, 109967. [Google Scholar] [CrossRef]
- Buonanno, M.; Grilj, V.; Brenner, D.J. Biological effects in normal cells exposed to FLASH dose rate protons. Radiother. Oncol. 2019, 139, 51–55. [Google Scholar] [CrossRef]
- Hughes, J.R.; Parsons, J.L. FLASH radiotherapy: Current knowledge and future insights using proton-beam therapy. Int. J. Mol. Sci. 2020, 21, 6492. [Google Scholar] [CrossRef]
- Diffenderfer, E.S.; Sørensen, B.S.; Mazal, A.; Carlson, D.J. The current status of preclinical proton FLASH radiation and future directions. Med. Phys. 2022, 49, 2039–2054. [Google Scholar] [CrossRef]
- Guo, Z.; Buonanno, M.; Harken, A.; Zhou, G.; Hei, T.K. Mitochondrial damage response and fate of normal cells exposed to FLASH irradiation with protons. Radiat. Res. 2022, 197, 569–582. [Google Scholar] [CrossRef] [PubMed]
- Mascia, A.E.; Daugherty, E.C.; Zhang, Y.; Lee, E.; Xiao, Z.; Sertorio, M.; Woo, J.; Backus, L.R.; McDonald, J.M.; McCann, C.; et al. Proton FLASH radiotherapy for the treatment of symptomatic bone metastases: The FAST-01 nonrandomized trial. JAMA Oncol. 2023, 9, 62–69. [Google Scholar] [CrossRef]
- Tessonnier, T.; Mein, S.; Walsh, D.W.M.; Schuhmacher, N.; Liew, H.; Cee, R.; Galonska, M.; Scheloske, S.; Schömers, C.; Weber, U.; et al. FLASH dose rate helium ion beams: First in vitro investigations. Int. J. Radiat. Oncol. Biol. Phys. 2021, 111, 1011–1022. [Google Scholar] [CrossRef] [PubMed]
- Weber, U.; Scifoni, E.; Durante, M. FLASH radiotherapy with carbon ion beams. Med. Phys. 2022, 49, 1974–1992. [Google Scholar] [CrossRef] [PubMed]
- Tinganelli, W.; Weber, U.; Puspitasari, A.; Simoniello, P.; Abdollahi, A.; Oppermann, J.; Schuy, C.; Horst, F.; Helm, A.; Fournier, C.; et al. FLASH with carbon ions: Tumor control, normal tissue sparing, and distal metastasis in a mouse osteosarcoma model. Radiother. Oncol. 2022, 175, 185–190. [Google Scholar] [CrossRef]
- Zakaria, A.M.; Colangelo, N.W.; Meesungnoen, J.; Azzam, E.I.; Plourde, M.-É.; Jay-Gerin, J.-P. Ultra-high dose-rate, pulsed (FLASH) radiotherapy with carbon ions: Generation of early, transient, highly oxygenated conditions in the tumor environment. Radiat. Res. 2020, 194, 587–593. [Google Scholar] [CrossRef]
- Bourhis, J.; Sozzi, W.J.; Jorge, P.G.; Gaide, O.; Bailat, C.; Duclos, F.; Patin, D.; Ozsahin, M.; Bochud, F.; Germond, J.-F.; et al. Treatment of a first patient with FLASH-radiotherapy. Radiother. Oncol. 2019, 139, 18–22. [Google Scholar] [CrossRef]
- Gaide, O.; Herrera, F.; Sozzi, W.J.; Jorge, P.G.; Kinj, R.; Bailat, C.; Duclos, F.; Bochud, F.; Germond, J.-F.; Gondré, M.; et al. Comparison of ultra-high versus conventional dose rate radiotherapy in a patient with cutaneous lymphoma. Radiother. Oncol. 2022, 174, 87–91. [Google Scholar] [CrossRef]
- Spitz, D.R.; Buettner, G.R.; Petronek, M.S.; St-Aubin, J.J.; Flynn, R.T.; Waldron, T.J.; Limoli, C.L. An integrated physico-chemical approach for explaining the differential impact of FLASH versus conventional dose rate irradiation on cancer and normal tissue responses. Radiother. Oncol. 2019, 139, 23–27. [Google Scholar] [CrossRef]
- Pratx, G.; Kapp, D.S. Ultra-high-dose-rate FLASH irradiation may spare hypoxic stem cell niches in normal tissues. Int. J. Radiat. Oncol. Biol. Phys. 2019, 105, 190–192. [Google Scholar] [CrossRef]
- Montay-Gruel, P.; Acharya, M.M.; Petersson, K.; Alikhani, L.; Yakkala, C.; Allen, B.D.; Ollivier, J.; Petit, B.; Gonçalves Jorge, P.; Syage, A.R.; et al. Long-term neurocognitive benefits of FLASH radiotherapy driven by reduced reactive oxygen species. Proc. Natl. Acad. Sci. USA 2019, 116, 10943–10951. [Google Scholar] [CrossRef]
- Pratx, G.; Kapp, D.S. A computational model of radiolytic oxygen depletion during FLASH irradiation and its effect on the oxygen enhancement ratio. Phys. Med. Biol. 2019, 64, 185005. [Google Scholar] [CrossRef]
- Adrian, G.; Konradsson, E.; Lempart, M.; Bäck, S.; Ceberg, C.; Petersson, K. The FLASH effect depends on oxygen concentration. Br. J. Radiol. 2020, 93, 20190702. [Google Scholar] [CrossRef]
- Petersson, K.; Adrian, G.; Butterworth, K.; McMahon, S.J. A quantitative analysis of the role of oxygen tension in FLASH radiation therapy. Int. J. Radiat. Oncol. Biol. Phys. 2020, 107, 539–547. [Google Scholar] [CrossRef] [PubMed]
- Rothwell, B.C.; Kirkby, N.F.; Merchant, M.J.; Chadwick, A.L.; Lowe, M.; Mackay, R.I.; Hendry, J.H.; Kirkby, K.J. Determining the parameter space for effective oxygen depletion for FLASH radiation therapy. Phys. Med. Biol. 2021, 66, 055020. [Google Scholar] [CrossRef]
- Favaudon, V.; Labarbe, R.; Limoli, C.L. Model studies of the role of oxygen in the FLASH effect. Med. Phys. 2022, 49, 2068–2081. [Google Scholar] [CrossRef] [PubMed]
- Dewey, D.L.; Boag, J.W. Modification of the oxygen effect when bacteria are given large pulses of radiation. Nature 1959, 183, 1450–1451. [Google Scholar] [CrossRef]
- Weiss, H.; Epp, E.R.; Heslin, J.M.; Ling, C.C.; Santomasso, A. Oxygen depletion in cells irradiated at ultra-high dose-rates and at conventional dose-rates. Int. J. Radiat. Biol. 1974, 26, 17–29. [Google Scholar] [CrossRef]
- Epp, E.R.; Weiss, H.; Ling, C.C. Irradiation of cells by single and double pulses of high intensity radiation: Oxygen sensitization and diffusion kinetics. Curr. Top. Radiat. Res. Q 1976, 11, 201–250, Corpus ID: 21836789. [Google Scholar]
- Wilson, P.; Jones, B.; Yokoi, T.; Hill, M.; Vojnovic, B. Revisiting the ultra-high dose rate effect: Implications for charged particle radiotherapy using protons and light ions. Br. J. Radiol. 2012, 85, e933–e939. [Google Scholar] [CrossRef]
- Cao, X.; Zhang, R.; Esipova, T.V.; Allu, S.R.; Ashraf, R.; Rahman, M.; Gunn, J.R.; Bruza, P.; Gladstone, D.J.; Williams, B.B.; et al. Quantification of oxygen depletion during FLASH irradiation in vitro and in vivo. Int. J. Radiat. Oncol. Biol. Phys. 2021, 111, 240–248. [Google Scholar] [CrossRef] [PubMed]
- Jansen, J.; Knoll, J.; Beyreuther, E.; Pawelke, J.; Skuza, R.; Hanley, R.; Brons, S.; Pagliari, F.; Seco, J. Does FLASH deplete oxygen? Experimental evaluation for photons, protons, and carbon ions. Med. Phys. 2021, 48, 3982–3990. [Google Scholar] [CrossRef]
- El Khatib, M.; van Slyke, A.L.; Velalopoulou, A.; Kim, M.M.; Shoniyozov, K.; Allu, S.R.; Diffenderfer, E.S.; Busch, T.M.; Wiersma, R.D.; Koch, C.J.; et al. Ultrafast tracking of oxygen dynamics during proton FLASH. Int. J. Radiat. Oncol. Biol. Phys. 2022, 113, 624–634. [Google Scholar] [CrossRef] [PubMed]
- Grilj, V.; Leavitt, R.J.; El Khatib, M.; Paisley, R.; Franco-Perez, J.; Petit, B.; Ballesteros-Zebadua, P.; Vozenin, M.-C. In vivo measurements of change in tissue oxygen level during irradiation reveal novel dose rate dependence. Radiother. Oncol. 2024, 201, 110539. [Google Scholar] [CrossRef] [PubMed]
- Bensasson, R.V.; Land, E.J.; Truscott, T.G. Excited States and Free Radicals in Biology and Medicine. Contributions from Flash Photolysis and Pulse Radiolysis; Oxford University Press: Oxford, UK, 1993. [Google Scholar]
- Spinks, J.W.T.; Woods, R.J. An Introduction to Radiation Chemistry; Wiley: New York, NY, USA, 1990. [Google Scholar]
- LaVerne, J.A. Radiation chemical effects of heavy ions. In Charged Particle and Photon Interactions with Matter: Chemical, Physicochemical, and Biological Consequences with Applications; Mozumder, A., Hatano, Y., Eds.; Marcel Dekker: New York, NY, USA, 2004; pp. 403–429. [Google Scholar]
- Meesungnoen, J.; Jay-Gerin, J.-P. Radiation chemistry of liquid water with heavy ions: Monte Carlo simulations studies. In Charged Particle and Photon Interactions with Matter: Recent Advances, Applications, and Interfaces; Hatano, Y., Katsumura, Y., Mozumder, A., Eds.; Taylor & Francis: Boca Raton, FL, USA, 2011; pp. 355–400. [Google Scholar]
- Bielski, B.H.J.; Cabelli, D.E.; Arudi, R.L.; Ross, A.B. Reactivity of HO2/O2− radicals in aqueous solution. J. Phys. Chem. Ref. Data 1985, 14, 1041–1100. [Google Scholar] [CrossRef]
- Michaels, H.B.; Hunt, J.W. A model for radiation damage in cells by direct effect and by indirect effect: A radiation chemistry approach. Radiat. Res. 1978, 74, 23–34. [Google Scholar] [CrossRef]
- Labarbe, R.; Hotoiu, L.; Barbier, J.; Favaudon, V. A physicochemical model of reaction kinetics supports peroxyl radical recombination as the main determinant of the FLASH effect. Radiother. Oncol. 2020, 153, 303–310. [Google Scholar] [CrossRef]
- Wardman, P. Approaches to modeling chemical reaction pathways in radiobiology. Int. J. Radiat. Biol. 2022, 98, 1399–1413. [Google Scholar] [CrossRef]
- Wardman, P. Radiotherapy using high-intensity pulsed radiation beams (FLASH): A radiation-chemical perspective. Radiat. Res. 2020, 194, 607–617. [Google Scholar] [CrossRef]
- Hu, A.; Qiu, R.; Li, W.B.; Zhou, W.; Wu, Z.; Zhang, H.; Li, J. Radical recombination and antioxidants: A hypothesis on the FLASH effect mechanism. Int. J. Radiat. Biol. 2023, 99, 620–628. [Google Scholar] [CrossRef]
- Hu, A.; Zhou, W.; Qiu, R.; Wei, S.; Wu, Z.; Zhang, H.; Li, J. Computational model of radiation oxygen effect with Monte Carlo simulation: Effects of antioxidants and peroxyl radicals. Int. J. Radiat. Biol. 2024, 100, 595–608. [Google Scholar] [CrossRef] [PubMed]
- Tan, H.S.; Teo, K.B.K.; Dong, L.; Friberg, A.; Koumenis, C.; Diffenderfer, E.; Zou, J.W. Modeling ultra-high dose rate electron and proton FLASH effect with the physicochemical approach. Phys. Med. Biol. 2023, 68, 145013. [Google Scholar] [CrossRef]
- Sultana, A.; Alanazi, A.; Meesungnoen, J.; Jay-Gerin, J.-P. On the transient radiolytic oxygen depletion in the ultra-high (FLASH) dose-rate radiolysis of water in a cell-like environment: Effect of e−aq and ●OH competing scavengers. Radiat. Res. 2022, 197, 566–567. [Google Scholar] [CrossRef]
- Alanazi, A.; Meesungnoen, J.; Jay-Gerin, J.-P. A computer modeling study of water radiolysis at high dose rates. Relevance to FLASH radiotherapy. Radiat. Res. 2021, 195, 149–162. [Google Scholar] [CrossRef]
- Lai, Y.; Jia, X.; Chi, Y. Modeling the effect of oxygen on the chemical stage of water radiolysis using GPU-based microscopic Monte Carlo simulations, with an application in FLASH radiotherapy. Phys. Med. Biol. 2021, 66, 025004. [Google Scholar] [CrossRef]
- Zhu, H.; Li, J.; Deng, X.; Qiu, R.; Wu, Z.; Zhang, H. Modeling of cellular response after FLASH irradiation: A quantitative analysis based on the radiolytic oxygen depletion hypothesis. Phys. Med. Biol. 2021, 66, 185009. [Google Scholar] [CrossRef]
- Boscolo, D.; Scifoni, E.; Durante, M.; Krämer, M.; Fuss, M.C. May oxygen depletion explain the FLASH effect? A chemical track structure analysis. Radiother. Oncol. 2021, 162, 68–75. [Google Scholar] [CrossRef]
- Farokhi, F.; Shirani, B.; Fattori, S.; Asgarian, M.A.; Cuttone, G.; Jia, S.B.; Petringa, G.; Sciuto, A.; Cirrone, G.A.P. Effects of the oxygen depletion in FLASH irradiation investigated through Geant4-DNA toolkit. Radiat. Phys. Chem. 2023, 212, 111184. [Google Scholar] [CrossRef]
- Buxton, G.V.; Greenstock, C.L.; Helman, W.P.; Ross, A.B. Critical review of rate constants for reactions of hydrated electrons, hydrogen atoms and hydroxyl radicals (●OH/●O−) in aqueous solution. J. Phys. Chem. Ref. Data 1988, 17, 513–886. [Google Scholar] [CrossRef]
- von Sonntag, C. Free-Radical-Induced DNA Damage and Its Repair: A Chemical Perspective; Springer: Berlin/Heidelberg, Germany, 2006; pp. 159–194. [Google Scholar]
- Alfassi, Z.B.; Huie, R.E.; Neta, P. Kinetic studies of organic peroxyl radicals in aqueous solutions and mixed solvents. In Peroxyl Radicals; Alfassi, Z.B., Ed.; Wiley: Chichester, UK, 1997; pp. 235–281. [Google Scholar]
- Neta, P.; Huie, R.E.; Ross, A.B. Rate constants for reactions of peroxyl radicals in fluid solutions. J. Phys. Chem. Ref. Data 1990, 19, 413–513. [Google Scholar] [CrossRef]
- Ingold, K.U. Peroxy radicals. Acc. Chem. Res. 1969, 2, 1–9. [Google Scholar] [CrossRef]
- Alfassi, Z.B. (Ed.) Peroxyl Radicals; Wiley: Chichester, UK, 1997. [Google Scholar]
- Yin, H.; Xu, L.; Porter, N.A. Free radical lipid peroxidation: Mechanisms and analysis. Chem. Rev. 2011, 111, 5944–5972. [Google Scholar] [CrossRef] [PubMed]
- Azzam, E.I.; Jay-Gerin, J.-P.; Pain, D. Ionizing radiation-induced metabolic oxidative stress and prolonged cell injury. Cancer Lett. 2012, 327, 48–60. [Google Scholar] [CrossRef] [PubMed]
- Halliwell, B.; Gutteridge, J.M.C. Free Radicals in Biology and Medicine; Oxford University Press: Oxford, UK, 2015. [Google Scholar]
- Robert, G.; Wagner, J.R.; Cadet, J. Oxidatively generated tandem DNA modifications by pyrimidinyl and 2-deoxyribosyl peroxyl radicals. Free Radic. Biol. Med. 2023, 196, 22–36. [Google Scholar] [CrossRef]
- Hall, E.J.; Giaccia, A.J. Radiobiology for the Radiologist, 8th ed.; Wolters Kluwer: Philadelphia, PA, USA, 2019. [Google Scholar]
- Baatout, S. (Ed.) Radiobiology Textbook; Belgian Nuclear Research Centre (SCK CEN): Mol, Belgium; Springer: Cham, Switzerland, 2023. [Google Scholar] [CrossRef]
- Kuppermann, A. Diffusion kinetics in radiation chemistry. In Actions Chimiques et Biologiques des Radiations; Haïssinsky, M., Ed.; Masson: Paris, France, 1961; Volume 5, pp. 85–166. [Google Scholar]
- Bepari, M.I.; Meesungnoen, J.; Jay-Gerin, J.-P. Early and transient formation of highly acidic pH spikes in water radiolysis under the combined effect of high dose rate and high linear energy transfer. Radiation 2023, 3, 165–182. [Google Scholar] [CrossRef]
- Baikalov, A.; Abolfath, R.; Schüler, E.; Mohan, R.; Wilkens, J.J.; Bartzsch, S. Intertrack interaction at ultra-high dose rates and its role in the FLASH effect. Front. Phys. 2023, 11, 1215422. [Google Scholar] [CrossRef]
- Furchgott, R.S.; Zawadki, J.V. The obligatory role of endothelial cells in the relaxation of arterial smooth muscle by acetylcholine. Nature 1980, 288, 373–376. [Google Scholar] [CrossRef]
- Moncada, S.; Palmer, R.M.J.; Higgs, E.A. Nitric oxide: Physiology, pathophysiology, and pharmacology. Pharmacol. Rev. 1991, 43, 109–142. [Google Scholar]
- Fukuto, J.M.; Wink, D.A. Nitric oxide (NO): Formation and biological roles in mammalian systems. In Metal Ions in Biological Systems; Sigel, A., Sigel, H., Eds.; Marcel Dekker: New York, NY, USA, 1999; Volume 36, pp. 547–595. [Google Scholar]
- Jay-Gerin, J.-P.; Ferradini, C. Are there protective enzymatic pathways to regulate high local nitric oxide (●NO) concentrations in cells under stress conditions? Biochimie 2000, 82, 161–166. [Google Scholar] [CrossRef]
- Toledo, J.C., Jr.; Augusto, O. Connecting the chemical and biological properties of nitric oxide. Chem. Res. Toxicol. 2012, 25, 975–989. [Google Scholar] [CrossRef]
- Belenichev, I.; Popazova, O.; Bukhtiyarova, N.; Savchenko, D.; Oksenych, V.; Kamyshnyi, O. Modulating nitric oxide: Implications for cytotoxicity and cytoprotection. Antioxidants 2024, 13, 504. [Google Scholar] [CrossRef] [PubMed]
- Beckman, J.S. Ischaemic injury mediator. Nature 1990, 345, 27–28. [Google Scholar] [CrossRef] [PubMed]
- Goldstein, S.; Czapski, G. The reaction of NO● with O2●− and HO2●: A pulse radiolysis study. Free Radic. Biol. Med. 1995, 19, 505–510. [Google Scholar] [CrossRef]
- Kissner, R.; Nauser, T.; Bugnon, P.; Lye, P.G.; Koppenol, W.H. Formation and properties of peroxynitrite as studied by laser flash photolysis, high-pressure stopped-flow technique, and pulse radiolysis. Chem. Res. Toxicol. 1997, 10, 1285–1292. [Google Scholar] [CrossRef] [PubMed]
- Padmaja, S.; Huie, R.E. The reaction of nitric oxide with organic peroxyl radicals. Biochem. Biophys. Res. Commun. 1993, 195, 539–544. [Google Scholar] [CrossRef]
- Hogg, N.; Kalyanaraman, B. Nitric oxide and lipid peroxidation. Biochim. Biophys. Acta 1999, 1411, 378–384. [Google Scholar] [CrossRef]
- Korytowski, W.; Zareba, M.; Girotti, A.W. Inhibition of free radical-mediated cholesterol peroxidation by diazeniumdiolate-derived nitric oxide: Effect of release rate on mechanism of action in a membrane system. Chem. Res. Toxicol. 2000, 13, 1265–1274. [Google Scholar] [CrossRef]
- Darley-Usmar, V.M.; Patel, R.P.; O’Donnell, V.B.; Freeman, B.A. Antioxidant actions of nitric oxide. In Nitric Oxide. Biology and Pathobiology; Ignarro, L.J., Ed.; Academic Press: San Diego, CA, USA, 2000; Chapter 17; pp. 265–276. [Google Scholar]
- Rubbo, H.; Radi, R.; Anselmi, D.; Kirk, M.; Barnes, S.; Butler, J.; Eiserich, J.P.; Freeman, B.A. Nitric oxide reaction with lipid peroxyl radical spares α-tocopherol during lipid peroxidation. J. Biol. Chem. 2000, 275, 10812–10818. [Google Scholar] [CrossRef]
- Boveris, A.; Poderoso, J.J. Regulation of oxygen metabolism by nitric oxide. In Nitric Oxide: Biology and Pathobiology; Ignarro, L.J., Ed.; Academic Press: San Diego, CA, USA, 2000; Chapter 23; pp. 355–368. [Google Scholar]
- Hall, C.N.; Garthwaite, J. What is the real physiological NO concentration in vivo? Nitric Oxide 2009, 21, 92–103. [Google Scholar] [CrossRef]
- Kennedy, L.; Sandhu, J.K.; Harper, M.-E.; Cuperlovic-Culf, M. Role of glutathione in cancer: From mechanisms to therapies. Biomolecules 2020, 10, 1429. [Google Scholar] [CrossRef]
- Babbs, C.F.; Steiner, M.G. Simulation of free radical reactions in biology and medicine: A new two-compartment kinetic model of intracellular lipid peroxidation. Free Radic. Biol. Med. 1990, 8, 471–485. [Google Scholar] [CrossRef] [PubMed]
- Wahlländer, A.; Soboll, S.; Sies, H.; Linke, I.; Müller, M. Hepatic mitochondrial and cytosolic glutathione content and the subcellular distribution of GHS-S-transferases. FEBS Lett. 1979, 97, 138–140. [Google Scholar] [CrossRef] [PubMed]
- Arteel, G.E.; Briviba, K.; Sies, H. Mechanisms of antioxidant defense against nitric oxide/peroxynitrite. In Nitric Oxide: Biology and Pathobiology; Ignarro, L.J., Ed.; Academic Press: San Diego, CA, USA, 2000; Chapter 22; pp. 343–354. [Google Scholar]
- Robert, G.; Wagner, J.R. Scavenging of alkylperoxyl radicals by addition to ascorbate: An alternative mechanism to electron transfer. Antioxidants 2024, 13, 1194. [Google Scholar] [CrossRef]
- Jackson, T.S.; Xu, A.; Vita, J.A.; Keaney, J.F., Jr. Ascorbate prevents the interaction of superoxide and nitric oxide only at very high physiological concentrations. Circ. Res. 1998, 83, 916–922. [Google Scholar] [CrossRef]
- Shen, J.; Griffiths, P.T.; Campbell, S.J.; Utinger, B.; Kalberer, M.; Paulson, S.E. Ascorbate oxidation by iron, copper and reactive oxygen species: Review, model development, and derivation of key rate constants. Sci. Rep. 2021, 11, 7417. [Google Scholar] [CrossRef]
- Tu, Y.-J.; Njus, D.; Schlegel, H.B. A theoretical study of ascorbic acid oxidation and HOO●/O2●− radical scavenging. Org. Biomol. Chem. 2017, 15, 4417–4431. [Google Scholar] [CrossRef]
- Bielski, B.H.J. Chemistry of ascorbic acid radicals. In Ascorbic Acid: Chemistry, Metabolism, and Uses; Seib, P., Tolbert, B.M., Eds.; American Chemical Society: Washington, DC, USA, 1982; Chapter 4; pp. 81–100. [Google Scholar] [CrossRef]
- Njus, D.; Kelley, P.M.; Tu, Y.-J.; Schlegel, H.B. Ascorbic acid: The chemistry underlying its antioxidant properties. Free. Radic. Biol. Med. 2020, 159, 37–43. [Google Scholar] [CrossRef]
- Buettner, G.R.; Jurkiewicz, B.A. Catalytic metals, ascorbate and free radicals: Combinations to avoid. Radiat. Res. 1996, 145, 532–541. [Google Scholar] [CrossRef]
- Wardman, P. Some applications of radiation chemistry to biochemistry and radiobiology. In Radiation Chemistry: Principles and Applications; Farhataziz, Rodgers, M.A.J., Eds.; VCH Publishers: New York, NY, USA, 1987; pp. 565–599. [Google Scholar]
- Priyadarsini, K.I. Redox reactions of antioxidants: Contributions from radiation chemistry of aqueous solutions. In Charged Particle and Photon Interactions with Matter: Recent Advances, Applications, and Interfaces; Hatano, Y., Katsumura, Y., Mozumder, A., Eds.; Taylor & Francis: Boca Raton, FL, USA, 2011; pp. 595–622. [Google Scholar]
- Packer, J.E.; Slater, T.F.; Willson, R.L. Direct observation of a free radical interaction between vitamin E and vitamin C. Nature 1979, 278, 737–738. [Google Scholar] [CrossRef]
- Forni, L.G. Free radical reactions involving fatty acids: The pulse radiolysis approach. In Membrane Lipid Oxidation; Vigo-Pelfrey, C., Ed.; CRC Press: Boca Raton, FL, USA, 1990; Volume 1, pp. 15–32. [Google Scholar]
- Penabeï, S.; Meesungnoen, J.; Jay-Gerin, J.-P. Assessment of cystamine’s radioprotective/antioxidant ability under high-dose-rate irradiation: A Monte Carlo multi-track chemistry simulation study. Antioxidants 2023, 12, 776. [Google Scholar] [CrossRef]
- Mustaree, S.; Meesungnoen, J.; Butarbutar, S.L.; Causey, P.; Stuart, C.R.; Jay-Gerin, J.-P. Self-radiolysis of tritiated water. 3. The ●OH scavenging effect of bromide ions on the yield of H2O2 in the radiolysis of water by 60Co γ-rays and tritium β-particles at room temperature. RSC Adv. 2014, 4, 43572–43581. [Google Scholar] [CrossRef]
- The Dosimetry of Pulsed Radiation; ICRU Report No. 34; International Commission on Radiation Units and Measurements: Bethesda, MD, USA, 1982.
- Cobut, V.; Frongillo, Y.; Patau, J.P.; Goulet, T.; Fraser, M.-J.; Jay-Gerin, J.-P. Monte Carlo simulation of fast electron and proton tracks in liquid water–I. Physical and physicochemical aspects. Radiat. Phys. Chem. 1998, 51, 229–243. [Google Scholar] [CrossRef]
- Frongillo, Y.; Goulet, T.; Fraser, M.-J.; Cobut, V.; Patau, J.P.; Jay-Gerin, J.-P. Monte Carlo simulation of fast electron and proton tracks in liquid water–II. Nonhomogeneous chemistry. Radiat. Phys. Chem. 1998, 51, 245–254. [Google Scholar] [CrossRef]
- Tachiya, M. Theory of diffusion-controlled reactions: Formulation of the bulk reaction rate in terms of the pair probability. Radiat. Phys. Chem. 1983, 21, 167–175. [Google Scholar] [CrossRef]
- Pimblott, S.M.; Pilling, M.J.; Green, N.J.B. Stochastic models of spur kinetics in water. Radiat. Phys. Chem. 1991, 37, 377–388. [Google Scholar] [CrossRef]
- Pimblott, S.M.; Green, N.J.B. Recent advances in the kinetics of radiolytic processes. In Research in Chemical Kinetics; Compton, R.G., Hancock, G., Eds.; Elsevier: Amsterdam, The Netherlands, 1995; Volume 3, pp. 117–174. [Google Scholar] [CrossRef]
- Goulet, T.; Fraser, M.-J.; Frongillo, Y.; Jay-Gerin, J.-P. On the validity of the independent reaction times approximation for the description of the nonhomogeneous kinetics of liquid water radiolysis. Radiat. Phys. Chem. 1998, 51, 85–91. [Google Scholar] [CrossRef]
- Wardman, P. Radiation-chemical perspective of the radiobiology of pulsed (high dose-rate) radiation (FLASH): A postscript. Radiat. Res. 2024, 201, 87–91. [Google Scholar] [CrossRef]
- Qian, S.Y.; Buettner, G.R. Iron and dioxygen chemistry is an important route to initiation of biological free radical oxidations: An electron paramagnetic resonance spin trapping study. Free Radic. Biol. Med. 1999, 26, 1447–1456. [Google Scholar] [CrossRef] [PubMed]
- von Sonntag, C. The chemistry of free-radical-mediated DNA damage. In Physical and Chemical Mechanisms in Molecular Radiation Biology; Glass, W.A., Varma, M.N., Eds.; Plenum Press: New York, NY, USA, 1991; pp. 287–321. [Google Scholar]
- Mee, L.K. Radiation chemistry of biopolymers. In Radiation Chemistry: Principles and Applications; Farhataziz, Rodgers, M.A.J., Eds.; VCH Publishers: New York, NY, USA, 1987; pp. 477–499. [Google Scholar]
- Anbar, M.; Farhataziz; Ross, A.B. Selected Specific Rates of Reactions of Transients from Water in Aqueous Solution. II. Hydrogen Atom; Report No. NERDS-NBS 51; US Government Printing Office: Washington, DC, USA, 1975. [Google Scholar]
- Fielden, E.M.; Roberts, P.B.; Bray, R.C.; Lowe, D.J.; Mautner, G.N.; Rotilio, G.; Calabrese, L. The mechanism of action of superoxide dismutase from pulse radiolysis and electron paramagnetic resonance. Evidence that only half the active sites function in catalysis. Biochem. J. 1974, 139, 49–60. [Google Scholar] [CrossRef]
- Bothe, E.; Schuchmann, M.N.; Schulte-Frohlinde, D.; von Sonntag, C. Hydroxyl radical-induced oxidation of ethanol in oxygenated aqueous solutions. A pulse radiolysis and product study. Z. Naturforsch. B 1983, 38, 212–219. [Google Scholar] [CrossRef]
- Meesat, R.; Sanguanmith, S.; Meesungnoen, J.; Lepage, M.; Khalil, A.; Jay-Gerin, J.-P. Utilization of the ferrous sulfate (Fricke) dosimeter for evaluating the radioprotective potential of cystamine: Experiment and Monte Carlo simulation. Radiat. Res. 2012, 177, 813–826. [Google Scholar] [CrossRef] [PubMed]
- Abedinzadeh, Z.; Gardès-Albert, M.; Ferradini, C. Reactions of ●OH and Br2●− radicals with glutathione. A radiolysis study. Radiat. Phys. Chem. 1992, 40, 551–558. [Google Scholar] [CrossRef]
- Mezyk, S.P. Rate constant determination for the reaction of hydroxyl and glutathione thiyl radicals with glutathione in aqueous solution. J. Phys. Chem. 1996, 100, 8861–8866. [Google Scholar] [CrossRef]
- Wardman, P. Thiyl radicals in biology: Their role as a ‘molecular switch’ central to cellular oxidative stress. In S-Centered Radicals; Alfassi, Z.B., Ed.; Wiley: Chichester, UK, 1999; pp. 289–309. [Google Scholar]
- Wardman, P.; von Sonntag, C. Kinetic factors that control the fate of thiyl radicals in cells. Methods Enzymol. 1995, 251, 31–45. [Google Scholar] [CrossRef]
- Nauser, T.; Koppenol, W.H.; Schöneich, C. Protein thiyl radical reactions and product formation: A kinetic simulation. Free Radic. Biol. Med. 2015, 80, 158–163. [Google Scholar] [CrossRef]
- Madej, E.; Folkes, L.K.; Wardman, P.; Czapski, G.; Goldstein, S. Thiyl radicals react with nitric oxide to form S-nitrosothiols with rate constants near the diffusion-controlled limit. Free Radic. Biol. Med. 2008, 44, 2013–2018. [Google Scholar] [CrossRef]
- Huie, R.E.; Padmaja, S. The reaction of NO with superoxide. Free Radic. Res. Commun. 1993, 18, 195–199. [Google Scholar] [CrossRef] [PubMed]
- Bartlett, D.; Church, D.F.; Bounds, P.L.; Koppenol, W.H. The kinetics of the oxidation of L-ascorbic acid by peroxynitrite. Free Radic. Biol. Med. 1995, 18, 85–92. [Google Scholar] [CrossRef]
- Keszler, A.; Zhang, Y.; Hogg, N. Reaction between nitric oxide, glutathione, and oxygen in the presence and absence of protein: How are S-nitrosothiols formed? Free Radic. Biol. Med. 2010, 48, 55–64. [Google Scholar] [CrossRef]
- Sanguanmith, S.; Meesungnoen, J.; Muroya, Y.; Lin, M.; Katsumura, Y.; Jay-Gerin, J.-P. On the spur lifetime and its temperature dependence in the low linear energy transfer radiolysis of water. Phys. Chem. Chem. Phys. 2012, 14, 16731–16736. [Google Scholar] [CrossRef]
- Hummel, A. Radiation Chemistry: The Chemical Effects of Ionizing Radiation and Their Applications; Interfaculty Reactor Institute-Technische Universiteit Delft (IRI-DUT): Delft, The Netherlands, 1995. [Google Scholar]
- Schardt, D.; Elsässer, T.; Schulz-Ertner, D. Heavy-ion tumor therapy: Physical and radiobiological benefits. Rev. Mod. Phys. 2010, 82, 383–425. [Google Scholar] [CrossRef]
- Baverstock, K.F.; Burns, W.G. Primary production of oxygen from irradiated water as an explanation for decreased radiobiological oxygen enhancement at high LET. Nature 1976, 260, 316–318. [Google Scholar] [CrossRef] [PubMed]
- Pimblott, S.M.; LaVerne, J.A. Effects of track structure on the ion radiolysis of the Fricke dosimeter. J. Phys. Chem. A 2002, 106, 9420–9427. [Google Scholar] [CrossRef]
- Negendank, W.; Edelmann, L. The State of Water in the Cell; Scanning Microscopy International: Chicago, IL, USA, 1988. [Google Scholar]
- Swietach, P.; Vaughan-Jones, R.D. Relationship between intracellular pH and proton mobility in rat and guinea-pig ventricular myocytes. J. Physiol. 2005, 566, 793–806. [Google Scholar] [CrossRef]
- Islam, M.M.; Kanike, V.; Meesungnoen, J.; Lertnaisat, P.; Katsumura, Y.; Jay-Gerin, J.-P. In situ generation of ultrafast transient “acid spikes” in the 10B(n,α)7Li radiolysis of water. Chem. Phys. Lett. 2018, 693, 210–215. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rabeya, I.; Meesungnoen, J.; Jay-Gerin, J.-P. Oxygen Depletion and the Role of Cellular Antioxidants in FLASH Radiotherapy: Mechanistic Insights from Monte Carlo Radiation-Chemical Modeling. Antioxidants 2025, 14, 406. https://doi.org/10.3390/antiox14040406
Rabeya I, Meesungnoen J, Jay-Gerin J-P. Oxygen Depletion and the Role of Cellular Antioxidants in FLASH Radiotherapy: Mechanistic Insights from Monte Carlo Radiation-Chemical Modeling. Antioxidants. 2025; 14(4):406. https://doi.org/10.3390/antiox14040406
Chicago/Turabian StyleRabeya, Israth, Jintana Meesungnoen, and Jean-Paul Jay-Gerin. 2025. "Oxygen Depletion and the Role of Cellular Antioxidants in FLASH Radiotherapy: Mechanistic Insights from Monte Carlo Radiation-Chemical Modeling" Antioxidants 14, no. 4: 406. https://doi.org/10.3390/antiox14040406
APA StyleRabeya, I., Meesungnoen, J., & Jay-Gerin, J.-P. (2025). Oxygen Depletion and the Role of Cellular Antioxidants in FLASH Radiotherapy: Mechanistic Insights from Monte Carlo Radiation-Chemical Modeling. Antioxidants, 14(4), 406. https://doi.org/10.3390/antiox14040406







