Experimental Studies of Ethyl Acetate Saponification Using Different Reactor Systems: The Effect of Volume Flow Rate on Reactor Performance and Pressure Drop
Abstract
:1. Introduction
- ○
- The T-shaped reactor with circular cross sections,
- ○
- The interdigital microreactor with parallel multilamination,
- ○
- The chicane microreactor (the Y-micromixer coupled with the chicane mixing channel).
2. Materials and Methods
2.1. Setup Composition
2.2. Analytical Procedure
2.3. Kinetic Study
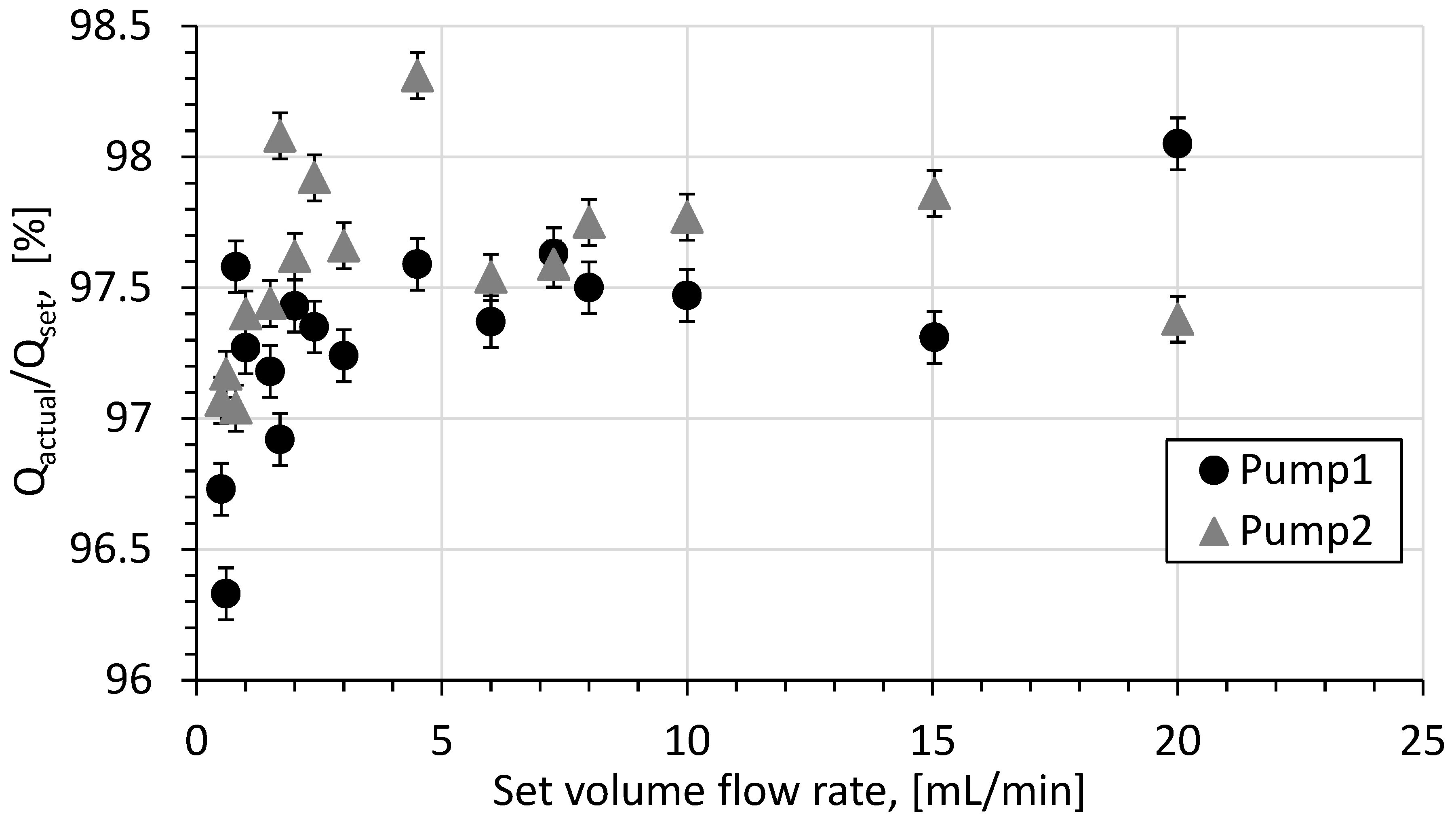
2.4. Choice and Calibration of Pumps
3. Results and Discussion
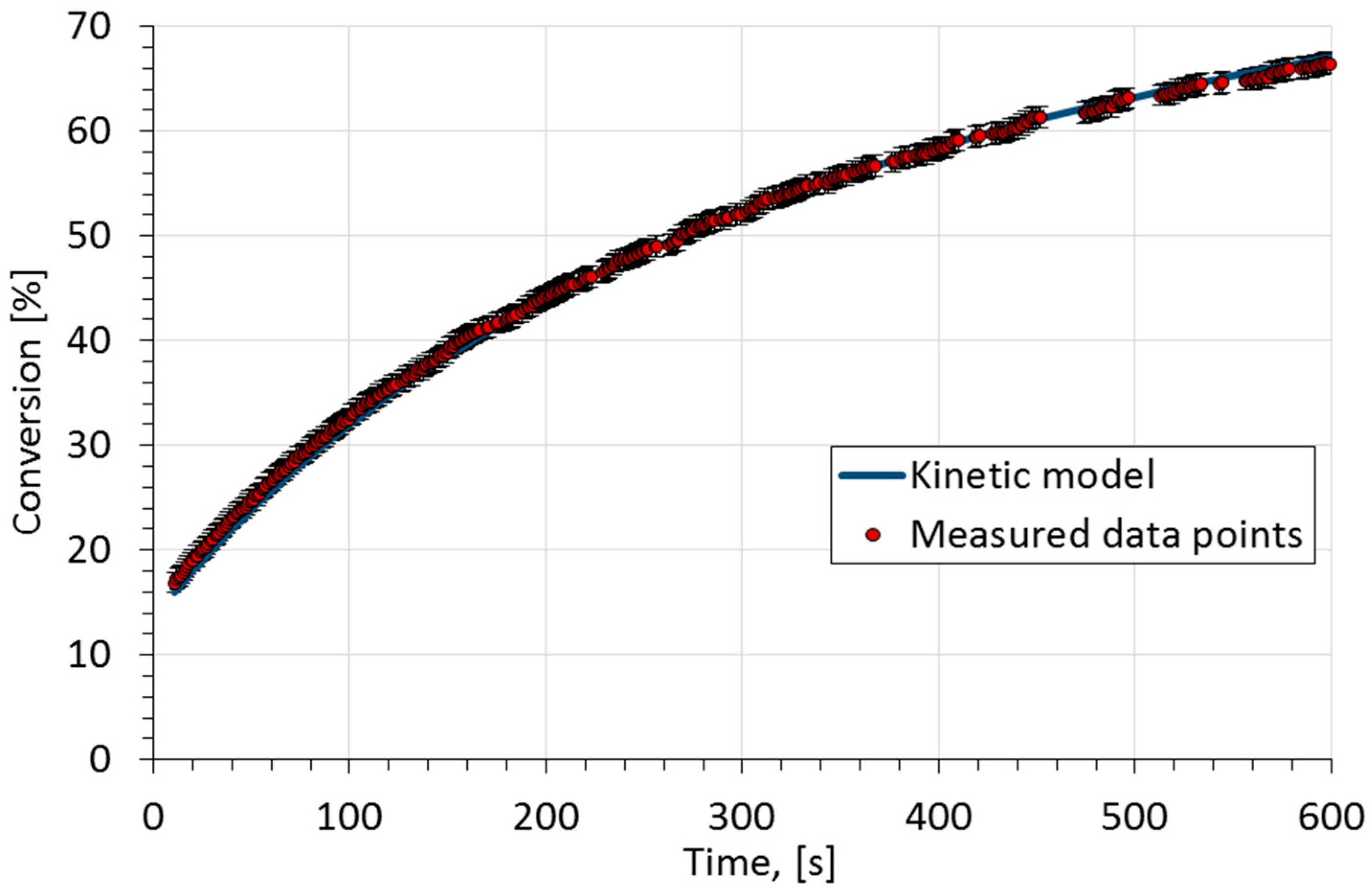
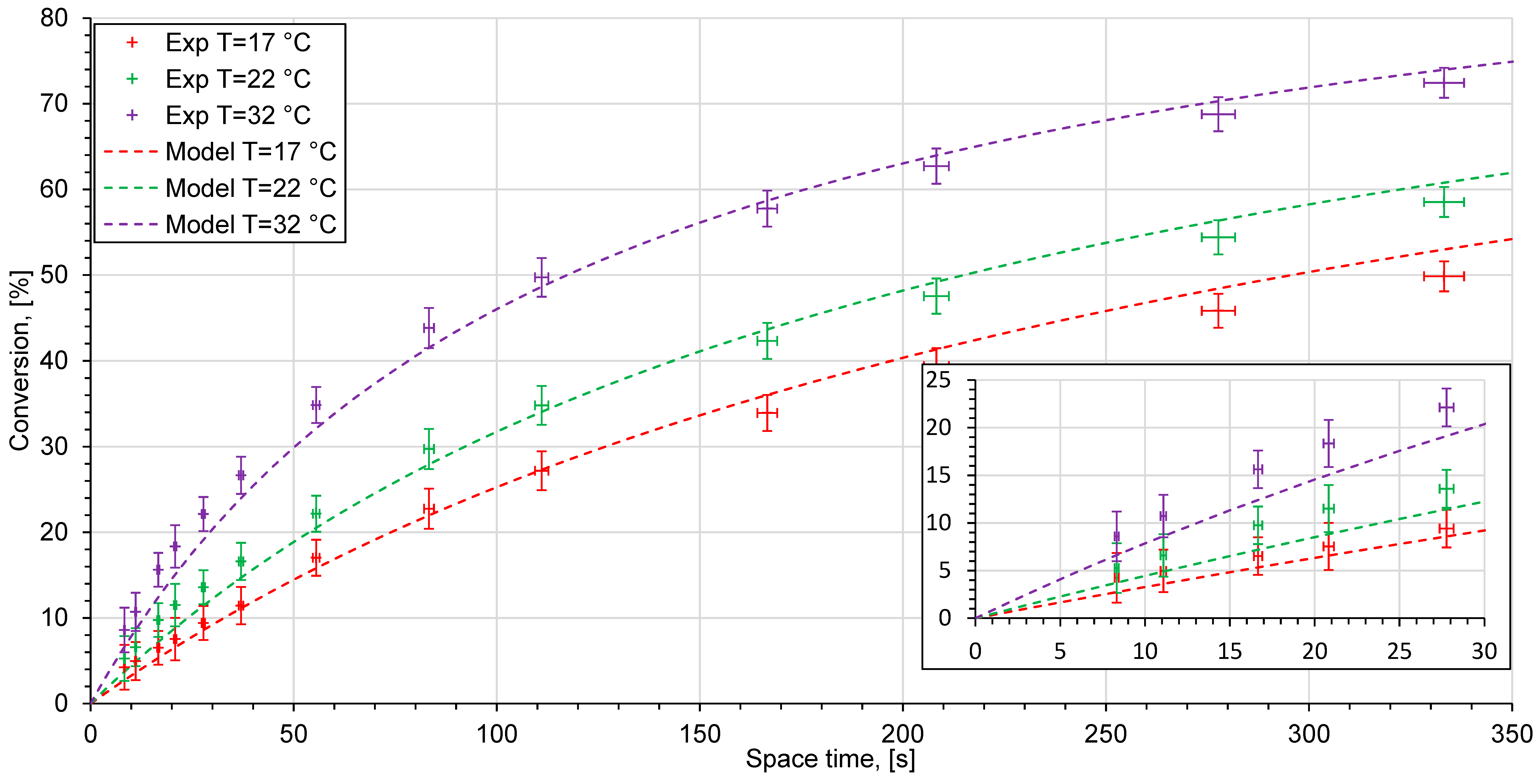
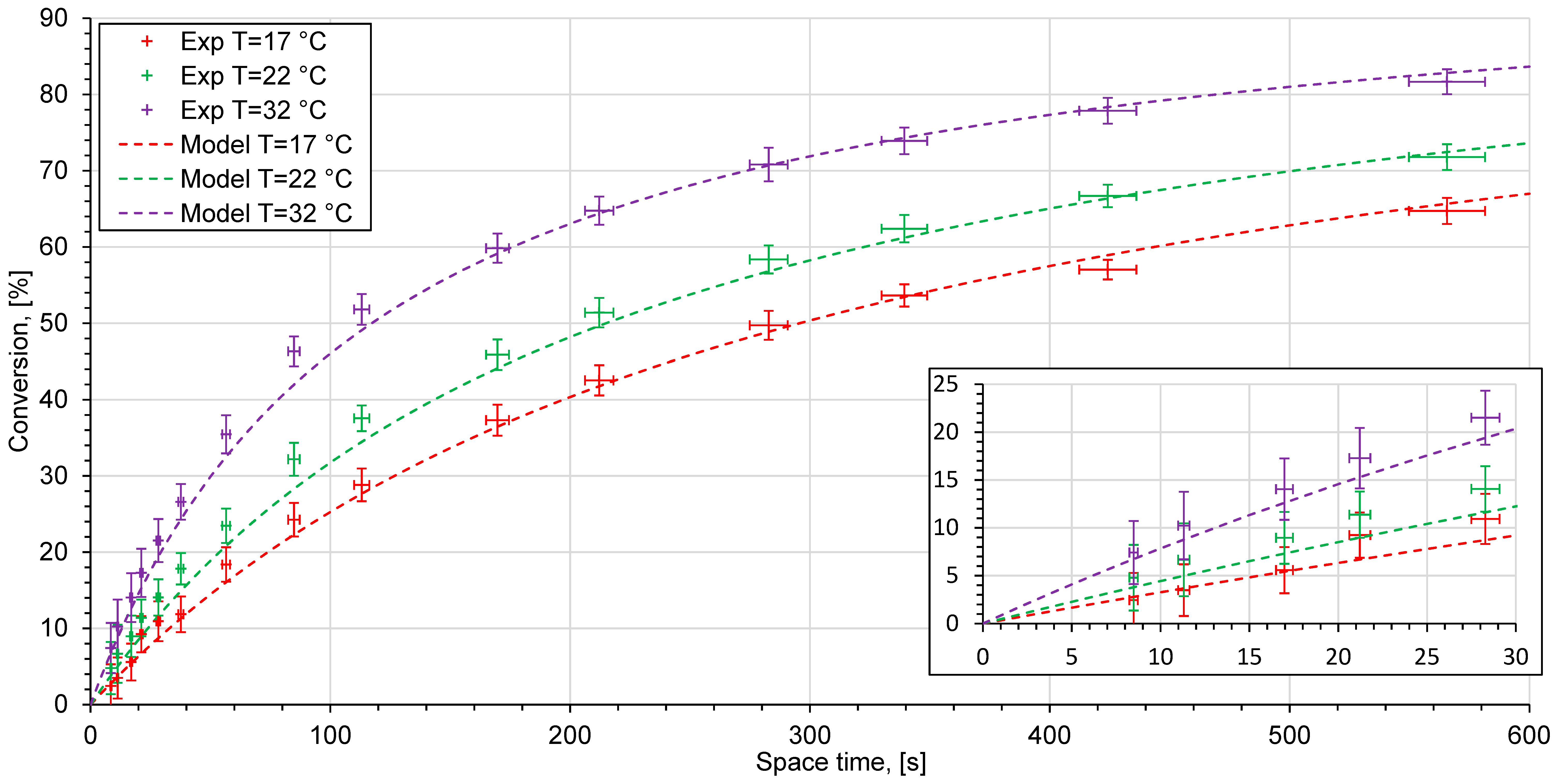
3.1. The Effect of Volume Flow Rate and Temperature on Conversion
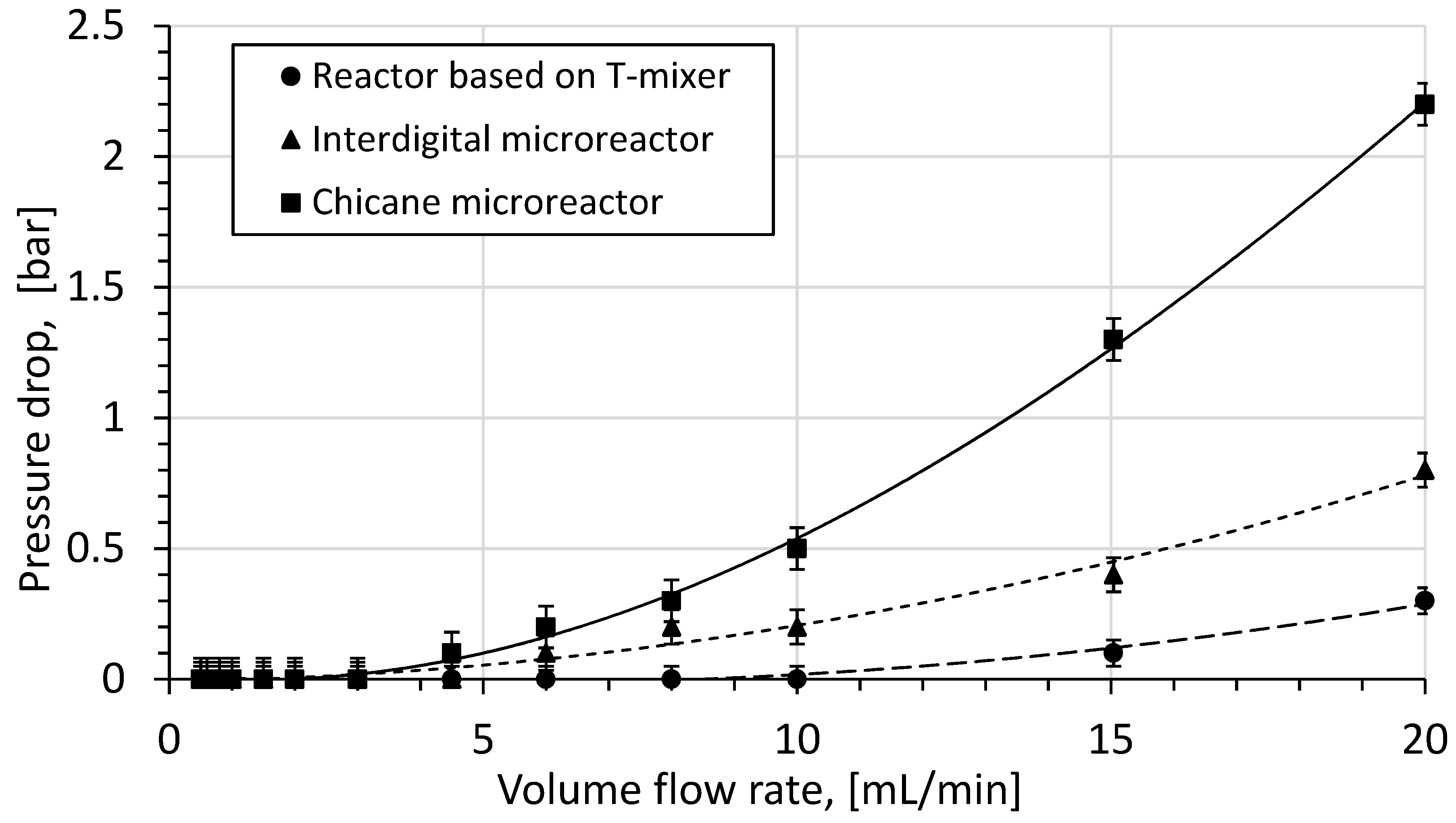
3.2. Effect of Volume Flow Rate on Pressure Drop
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Reschetilowski, W. Microreactors in Preparative Chemistry: Practical Aspects in Bioprocessing, Nanotechnology, Catalysis and More; Wiley-VCH: Weinheim, Germany, 2013; ISBN 978-3-527-33282-3. [Google Scholar]
- Schwarz, S.; Borovinskaya, E.S.; Reschetilowski, W. Base catalyzed ethanolysis of soybean oil in microreactors: Experiments and kinetic modeling. Chem. Eng. Sci. 2013, 104, 610–618. [Google Scholar] [CrossRef]
- Sun, J.; Ju, J.; Ji, L.; Zhang, L.; Xu, N. Synthesis of Biodiesel in Capillary Microreactors. Ind. Eng. Chem. Res. 2008, 47, 1398–1403. [Google Scholar] [CrossRef]
- Wen, Z.; Yu, X.; Tu, S.T.; Yan, J.; Dahlquist, E. Intensification of biodiesel synthesis using zigzag micro-channel reactors. Bioresour. Technol. 2009, 100, 3054–3060. [Google Scholar] [CrossRef] [PubMed]
- Pennemann, H.; Watts, P.; Haswell, S.J.; Hessel, V.; Löwe, H. Benchmarking of Microreactor Applications. Organ. Process Res. Dev. 2004, 8, 422–439. [Google Scholar] [CrossRef]
- Jähnisch, K.; Baerns, M.; Hessel, V.; Ehrfeld, W.; Haverkamp, V.; Löwe, H.; Wille, C.; Guber, A. Direct fluorination of toluene using elemental fluorine in gas/liquid microreactors. J. Fluor. Chem. 2000, 105, 117–128. [Google Scholar] [CrossRef]
- Drott, J.; Lindström, K.; Rosengren, L.; Laurell, T. Porous silicon as the carrier matrix in microstructured enzyme reactors yielding high enzyme activities. J. Micromech. Microeng. 1997, 7, 14–23. [Google Scholar] [CrossRef]
- Ahmed-Omer, B.; Brandt, J.C.; Wirth, T. Advanced organic synthesis using microreactor technology. Org. Biomol. Chem. 2007, 5, 733–740. [Google Scholar] [CrossRef]
- Borovinskaya, E.S.; Reschetilowski, W. Perspectives of heterogeneous process intensification in microreactors. Russ. J. Gen. Chem. 2012, 82, 2108–2115. [Google Scholar] [CrossRef]
- Yoshida, J.I.; Nagaki, A.; Iwasaki, T.; Suga, S. Enhancement of Chemical Selectivity by Microreactors. Chem. Eng. Technol. 2005, 28, 259–266. [Google Scholar] [CrossRef]
- Wörz, O.; Jäckel, K.P.; Richter, T.; Wolf, A. Microreactors, a new efficient tool for optimum reactor design. Chem. Eng. Sci. 2001, 56, 1029–1033. [Google Scholar] [CrossRef]
- Murphy, E.R.; Martinelli, J.R.; Zaborenko, N.; Buchwald, S.L.; Jensen, K.F. Accelerating reactions with microreactors at elevated temperatures and pressures: Profiling aminocarbonylation reactions. Angew. Chem. Int. Ed. Engl. 2007, 46, 1734–1737. [Google Scholar] [CrossRef] [PubMed]
- Yoshida, J.; Nagaki, A.; Yamada, T. Flash chemistry: Fast chemical synthesis by using microreactors. Chemistry 2008, 14, 7450–7459. [Google Scholar] [CrossRef] [PubMed]
- Roberge, D.M.; Gottsponer, M.; Eyholzer, M.; Kockmann, N. Industrial design, scale-up, and use of microreactors. Chem. Today 2009, 27, 8–11. [Google Scholar]
- Kockmann, N.; Gottsponer, M.; Roberge, D.M. Scale-up concept of single-channel microreactors from process development to industrial production. Chem. Eng. J. 2011, 167, 718–726. [Google Scholar] [CrossRef]
- Nguyen, N. Micromixers: Fundamentals, Design and Fabrication, 2nd ed.; William Andrew: Norwich, UK, 2011; ISBN 978-1-4377-3520-8. [Google Scholar]
- Kockmann, N.; Kiefer, T.; Engler, M.; Woias, P. Convective mixing and chemical reactions in microchannels with high flow rates. Sens. Actuators B Chem. 2006, 117, 495–508. [Google Scholar] [CrossRef]
- Kockmann, N. Transport Phenomena in Micro Process Engineering; Springer: Berlin/Heidelberg, Germany, 2008; ISBN 978-3-540-74616-4. [Google Scholar]
- Bothe, D.; Stemich, C.; Warnecke, H.J. Computation of scales and quality of mixing in a T-shaped microreactor. Comput. Chem. Eng. 2008, 32, 108–114. [Google Scholar] [CrossRef]
- Levenspiel, O. Chemical Reaction Engineering, 3rd ed.; John Wiley & Sons: New York, NY, USA, 1998; ISBN 978-0471254249. [Google Scholar]
- Borovinskaya, E.S.; Sabaditsch, D.; Reschetilowski, W. Base-Catalyzed Ethanolysis of Waste Cooking Oil in a Micro/Millireactor System: Flow and Reaction Analysis. Chem. Eng. Technol. 2018, 42, 495–505. [Google Scholar] [CrossRef]
- Robertson, K. Using flow technologies to direct the synthesis and assembly of materials in solution. Chem. Cent. J. 2017, 11, 4. [Google Scholar] [CrossRef]
- Niu, X.Z.; Lee, Y.K. Efficient spatial-temporal chaotic mixing in microchannels. J. Micromech. Microeng. 2003, 13, 454–462. [Google Scholar] [CrossRef]
- Hessel, V.; Hardt, S.; Löwe, H.; Schönfeld, F. Laminar mixing in different interdigital micromixers: I. Experimental characterization. AIChE J. 2003, 49, 566–577. [Google Scholar] [CrossRef]
- Schwesinger, N.; Frank, T.; Wurmus, H. A modular microfluid system with an integrated micromixer. J. Micromech. Microeng. 1996, 6, 99–102. [Google Scholar] [CrossRef]
- Knight, J.B.; Vishwanath, A.; Brody, J.P.; Austin, R.H. Hydrodynamic Focusing on a Silicon Chip: Mixing Nanoliters in Microseconds. Phys. Rev. Lett. 1998, 80, 3863–3866. [Google Scholar] [CrossRef]
- Stroock, A.D.; Dertinger, S.K.; Ajdari, A.; Mezić, I.; Stone, H.A.; Whitesides, G.M. Chaotic mixer for microchannels. Science 2002, 295, 647–651. [Google Scholar] [CrossRef] [PubMed]
- Hessel, V.; Löwe, H.; Schönfeld, F. Micromixers—A review on passive and active mixing principles. Chem. Eng. Sci. 2005, 60, 2479–2501. [Google Scholar] [CrossRef]
- Villermaux, J. Micromixing phenomena in stirred reactors. In Encyclopedia Fluid Mechanics; Gulf Publishing Company: Houston, TX, USA, 1986. [Google Scholar]
- Löb, P.; Drese, K.S.; Hessel, V.; Hardt, S.; Hofmann, C.; Löwe, H.; Schenk, R.; Schönfeld, F.; Werner, B. Steering of Liquid Mixing Speed in Interdigital Micro Mixers—From Very Fast to Deliberately Slow Mixing. Chem. Eng. Technol. 2004, 27, 340–345. [Google Scholar] [CrossRef]
- Schönfeld, F.; Hessel, V.; Hofmann, C. An optimised split-and-recombine micro-mixer with uniform chaotic mixing. Lab Chip 2004, 4, 65–69. [Google Scholar] [CrossRef]
- Zhang, Z.; Zhao, P.; Xiao, G.; Lin, M.; Cao, X. Focusing-enhanced mixing in microfluidic channels. Biomicrofluidics 2008, 2, 14101. [Google Scholar] [CrossRef]
- Wong, S.H.; Ward, M.C.; Wharton, C.W. Micro T-mixer as a rapid mixing micromixer. Sens. Actuators B Chem. 2004, 100, 359–379. [Google Scholar] [CrossRef]
- Bothe, D.; Stemich, C.; Warnecke, H.-J. Fluid mixing in a T-shaped micro-mixer. Chem. Eng. Sci. 2006, 61, 2950–2958. [Google Scholar] [CrossRef]
- Gobby, D.; Angeli, P.; Gavriilidis, A. Mixing characteristics of T-type microfluidic mixers. J. Micromech. Microeng. 2001, 11, 126–132. [Google Scholar] [CrossRef]
- Rudyak, V.; Minakov, A. Modeling and Optimization of Y-Type Micromixers. Micromachines 2014, 5, 886–912. [Google Scholar] [CrossRef]
- Yang, J.; Qi, L.; Chen, Y.; Ma, H. Design and Fabrication of a Three Dimensional Spiral Micromixer. Chin. J. Chem. 2013, 31, 209–214. [Google Scholar] [CrossRef]
- Schwolow, S.; Hollmann, J.; Schenkel, B.; Röder, T. Application-Oriented Analysis of Mixing Performance in Microreactors. Organ. Process Res. Dev. 2012, 16, 1513–1522. [Google Scholar] [CrossRef]
- Mengeaud, V.; Josserand, J.; Girault, H.H. Mixing Processes of a Zigzag Microchannel: Finite Element Simulations and Optical Study. Anal. Chem. 2002, 64, 4279–4286. [Google Scholar] [CrossRef]
- Bhagat, A.A.; Peterson, E.T.; Papautsky, I. A passive planar micromixer with obstructions for mixing at low Reynolds numbers. J. Micromech. Microeng. 2007, 17, 1017–1024. [Google Scholar] [CrossRef]
- Khaydarov, V.; Borovinskaya, E.S.; Reaschetilowski, W. Numerical and Experimental Investigations of a Micromixer with Chicane Mixing Geometry. Appl. Sci. 2018, 8, 2458. [Google Scholar] [CrossRef]
- Hossain, S.; Ansari, M.A.; Kim, K.Y. Evaluation of the mixing performance of three passive micromixers. Chem. Eng. J. 2009, 150, 492–501. [Google Scholar] [CrossRef]
- Holvey, C.P.; Roberge, D.M.; Gottsponer, M.; Kockmann, N.; Macchi, A. Pressure drop and mixing in single phase microreactors: Simplified designs of micromixers. Chem. Eng. Process. 2011, 50, 1069–1075. [Google Scholar] [CrossRef]
- Falk, L.; Commenge, J.M. Performance comparison of micromixers. Chem. Eng. Sci. 2010, 65, 405–411. [Google Scholar] [CrossRef]
- Das, K.; Sahoo, P.; Sai Baba, M.; Murali, N.; Swaminathan, P. Kinetic studies on saponification of ethyl acetate using an innovative conductivity-monitoring instrument with a pulsating sensor. Int. J. Chem. Kinet. 2011, 43, 648–656. [Google Scholar] [CrossRef]
- Petek, A.; Krajnc, M. The enthalpy and entropy of activation for ethyl acetate saponification. Int. J. Chem. Kinet. 2012, 44, 692–698. [Google Scholar] [CrossRef]
- Iorio, C.S.; Perfetti, C.; Dubois, F. Fast Mixing in Microchannels by Input Modulation: A Numerical and Experimental Study. ASME Proc./Micromixers 2011, 165–169. [Google Scholar] [CrossRef]
- Caro, C.G. The Mechanics of the Circulation; Cambridge University Press: Cambridge, MA, USA, 2011; ISBN 0-19-263323-6. [Google Scholar]
- DeMello, A.J. Control and detection of chemical reactions in microfluidic systems. Nature 2006, 442, 394–402. [Google Scholar] [CrossRef] [PubMed]
- Bothe, D.; Lojewski, A.; Warnecke, H.-J. Computational analysis of an instantaneous chemical reaction in a T-microreactor. AIChE J. 2010, 56, 1406–1415. [Google Scholar] [CrossRef]
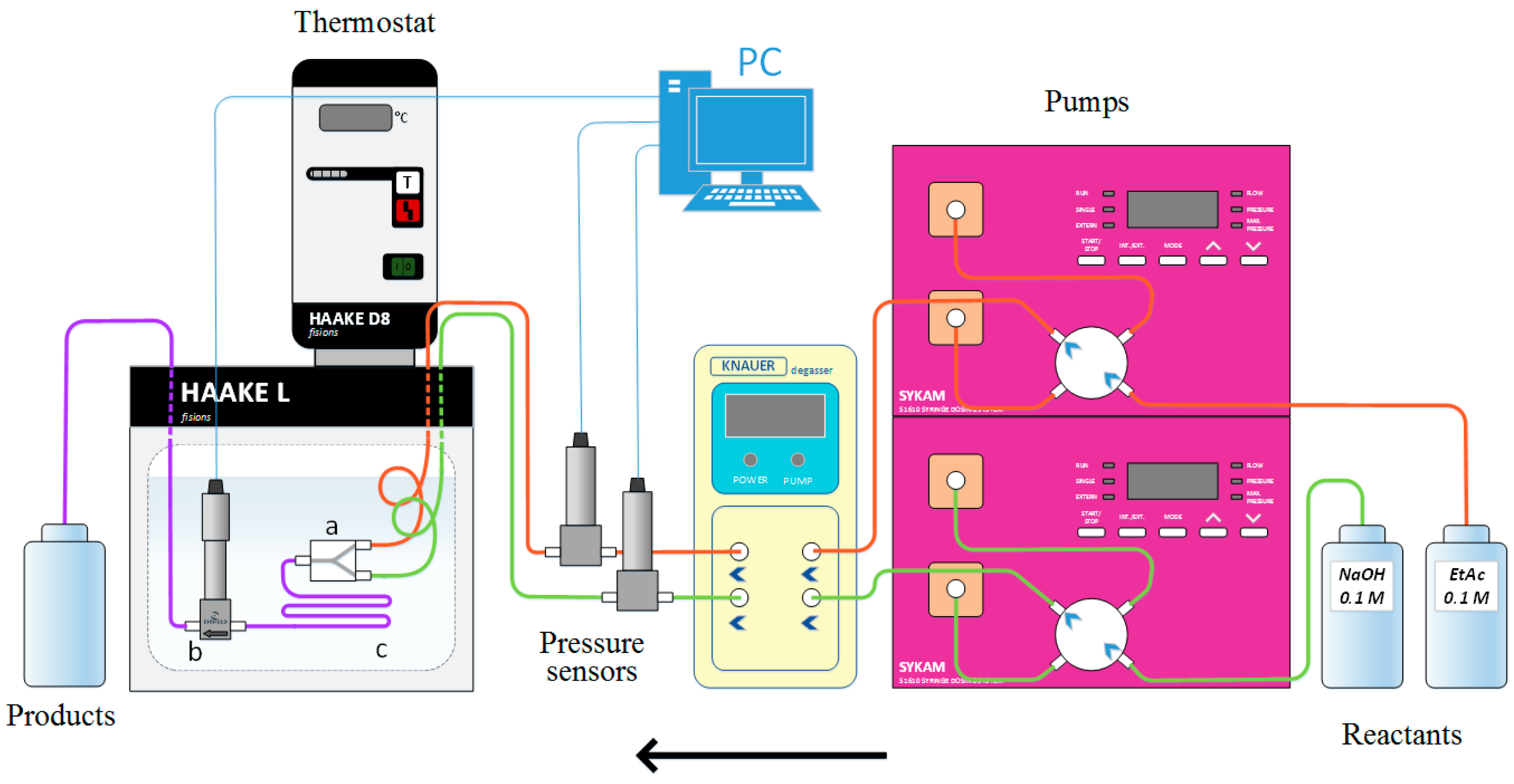



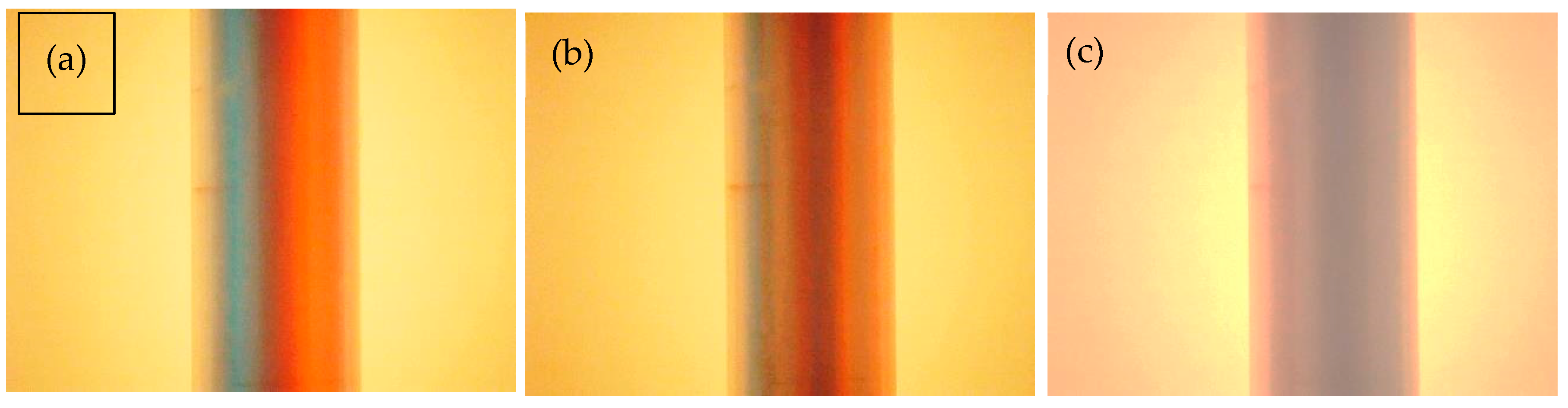
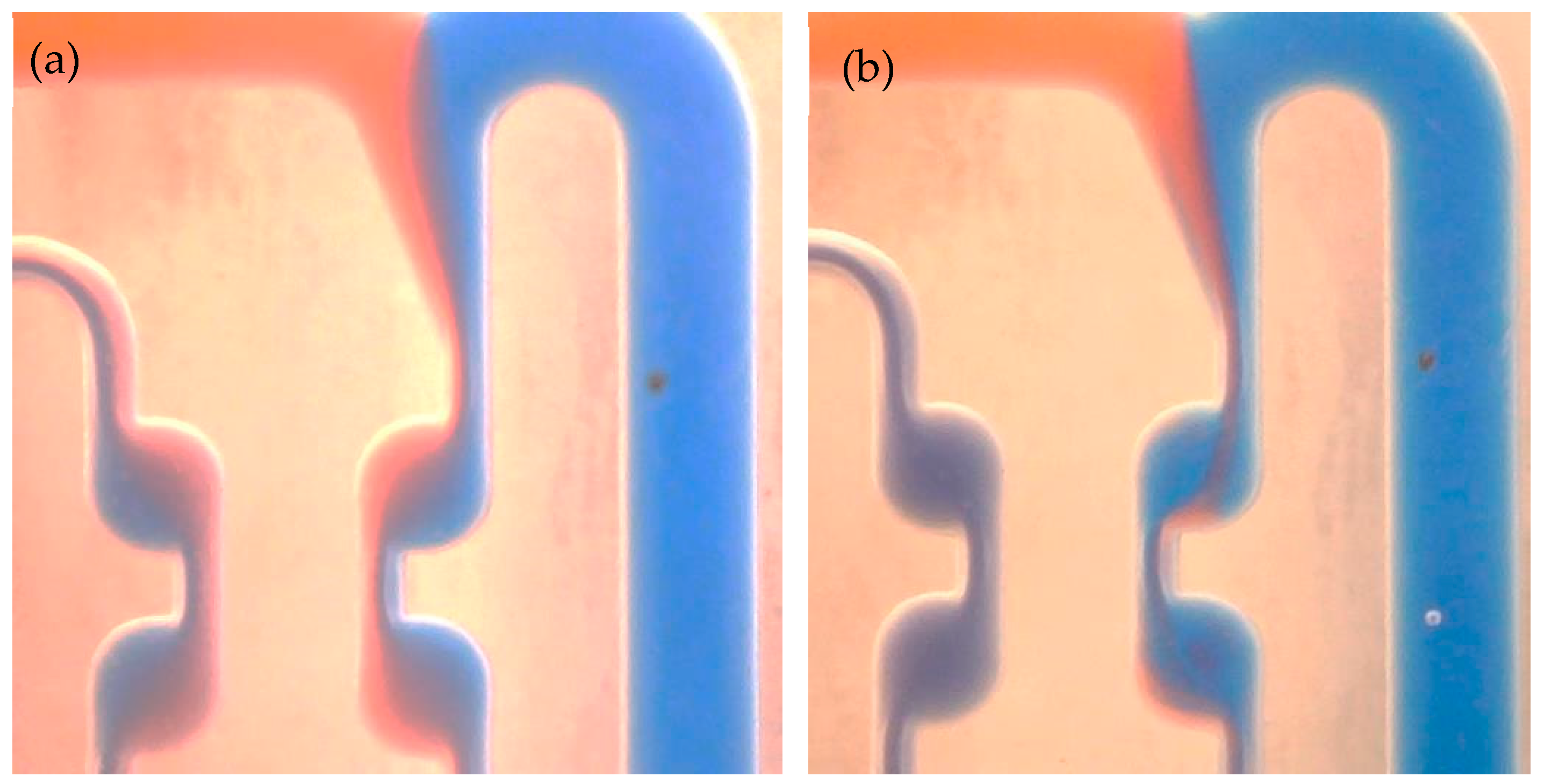
| Name and Supplier | Mixing Principle | Material | Volume, mL |
|---|---|---|---|
| T-shaped reactor | T-mixing | Stainless steel | 0.05 |
| Swagelok Corp | |||
| Interdigital microreactor | Parallel multilamination | Stainless steel | 0.10 |
| Ehrfeld Mikrotechnik BTS | |||
| Chicane microreactor XXL-S-01 | Chaotic advection within the chicane mixing channel | Borosilicate glass | 1.80 |
| Little Things Factory GmbH |
| Temperature (°C) | G0 (mS/cm) | G100 (mS/cm) |
|---|---|---|
| 17 | 11.41 ± 0.05 | 3.75 ± 0.04 |
| 22 | 11.20 ± 0.04 | 3.75 ± 0.04 |
| 32 | 10.85 ± 0.05 | 3.75 ± 0.04 |
| Measurement | Reaction Rate Constant, (L/(mol·s)) | ||
|---|---|---|---|
| 17 °C | 22 °C | 32 °C | |
| 1 | 0.0603 | 0.0990 | 0.1549 |
| 2 | 0.0617 | 0.0967 | 0.1605 |
| 3 | 0.0616 | 0.0950 | 0.1596 |
| 4 | 0.0716 | 0.0986 | 0.1824 |
| 5 | 0.0721 | 0.0997 | 0.1821 |
| 6 | 0.0687 | 0.0963 | 0.1722 |
| Average value | 0.0660 | 0.0976 | 0.1686 |
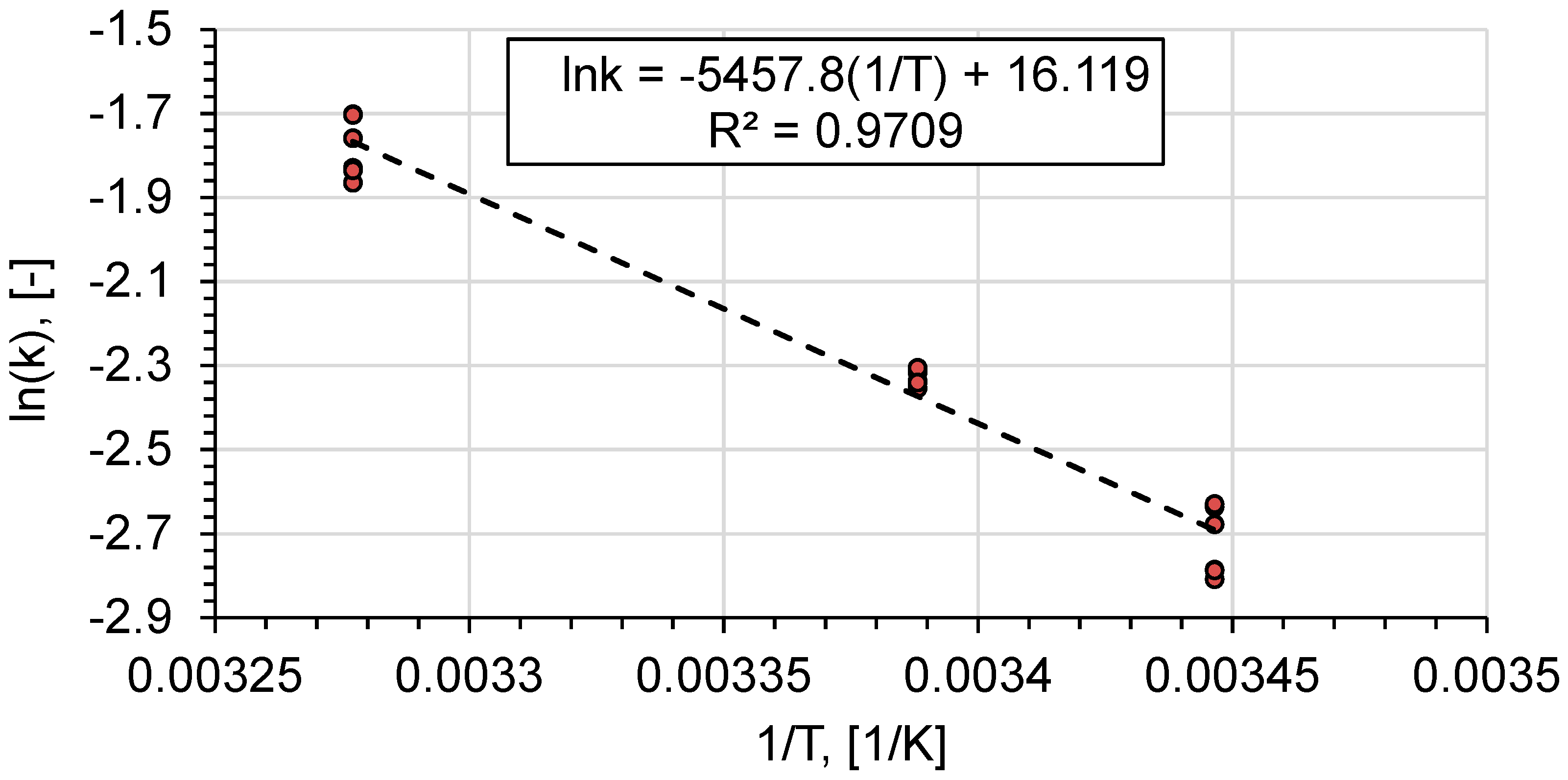
| Activation Energy (kJ/mol) | Pre-Exponential Factor (L/(mol·s)) | Reaction Rate Constant (L/(mol·s)) at | Source | ||
|---|---|---|---|---|---|
| 17 °C | 22 °C | 32 °C | |||
| 41.40 | 2.18 × 10 6 | 0.077 | 0.103 | 0.178 | [45] |
| 48.00 | 2.86 × 10 7 | 0.065 | 0.091 | 0.173 | [46] |
| 45.38 | 1.00 × 10 6 | 0.068 | 0.093 | 0.171 | present study |
| Name | Manufacturer | Type |
|---|---|---|
| REGLO-CPF | Cole-Parmer GmbH | Piston pump |
| NE-300 | New Era Pump Systems Inc. | Single syringe pump |
| S 1610 | SYKAM Chromatographie Vertriebs GmbH | Continuous two-syringe pumping system |
| Setup Based on | a | b | R2 |
|---|---|---|---|
| T-shaped reactor | 0.0010 | 0.0006 | 0.89 |
| Interdigital microreactor | 0.0050 | 0.0016 | 0.98 |
| Chicanes microreactor | 0.0030 | 0.0055 | 1.00 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Borovinskaya, E.; Khaydarov, V.; Strehle, N.; Musaev, A.; Reschetilowski, W. Experimental Studies of Ethyl Acetate Saponification Using Different Reactor Systems: The Effect of Volume Flow Rate on Reactor Performance and Pressure Drop. Appl. Sci. 2019, 9, 532. https://doi.org/10.3390/app9030532
Borovinskaya E, Khaydarov V, Strehle N, Musaev A, Reschetilowski W. Experimental Studies of Ethyl Acetate Saponification Using Different Reactor Systems: The Effect of Volume Flow Rate on Reactor Performance and Pressure Drop. Applied Sciences. 2019; 9(3):532. https://doi.org/10.3390/app9030532
Chicago/Turabian StyleBorovinskaya, Ekaterina, Valentin Khaydarov, Nicole Strehle, Alexander Musaev, and Wladimir Reschetilowski. 2019. "Experimental Studies of Ethyl Acetate Saponification Using Different Reactor Systems: The Effect of Volume Flow Rate on Reactor Performance and Pressure Drop" Applied Sciences 9, no. 3: 532. https://doi.org/10.3390/app9030532
APA StyleBorovinskaya, E., Khaydarov, V., Strehle, N., Musaev, A., & Reschetilowski, W. (2019). Experimental Studies of Ethyl Acetate Saponification Using Different Reactor Systems: The Effect of Volume Flow Rate on Reactor Performance and Pressure Drop. Applied Sciences, 9(3), 532. https://doi.org/10.3390/app9030532






