Theoretical Calculations of the Multistep Reaction Mechanism Involved in Asparagine Pyrolysis Supported by Degree of Rate Control and Thermodynamic Control Analyses
Abstract
:1. Introduction
2. Results and Discussion
2.1. The Formation of Maleimide and Succinimide Products: Mechanism of Polymerization-Rupture
2.1.1. Optimization and Thermodynamic Properties
2.1.2. Degree of Rate Control and Thermodynamic Control
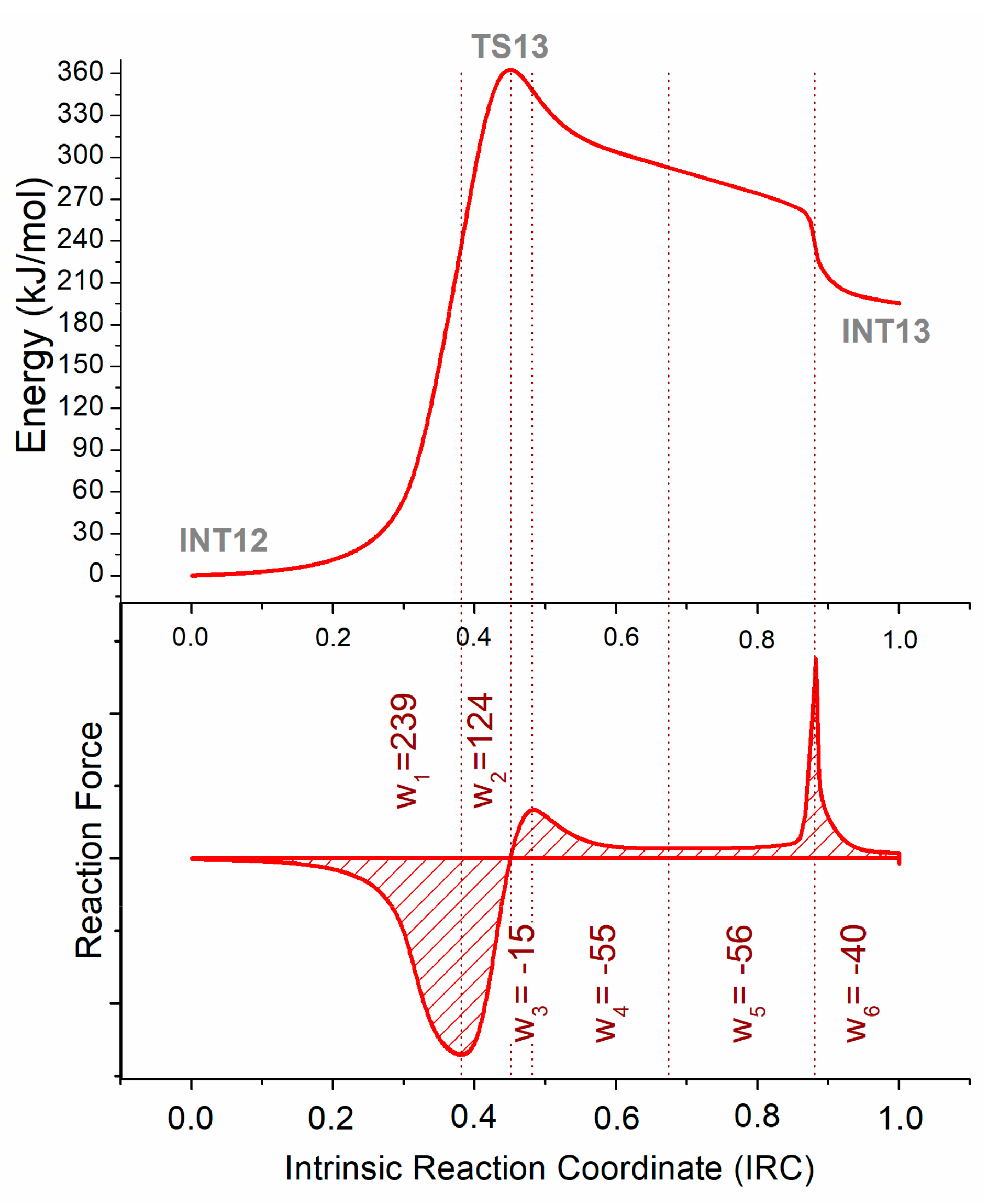
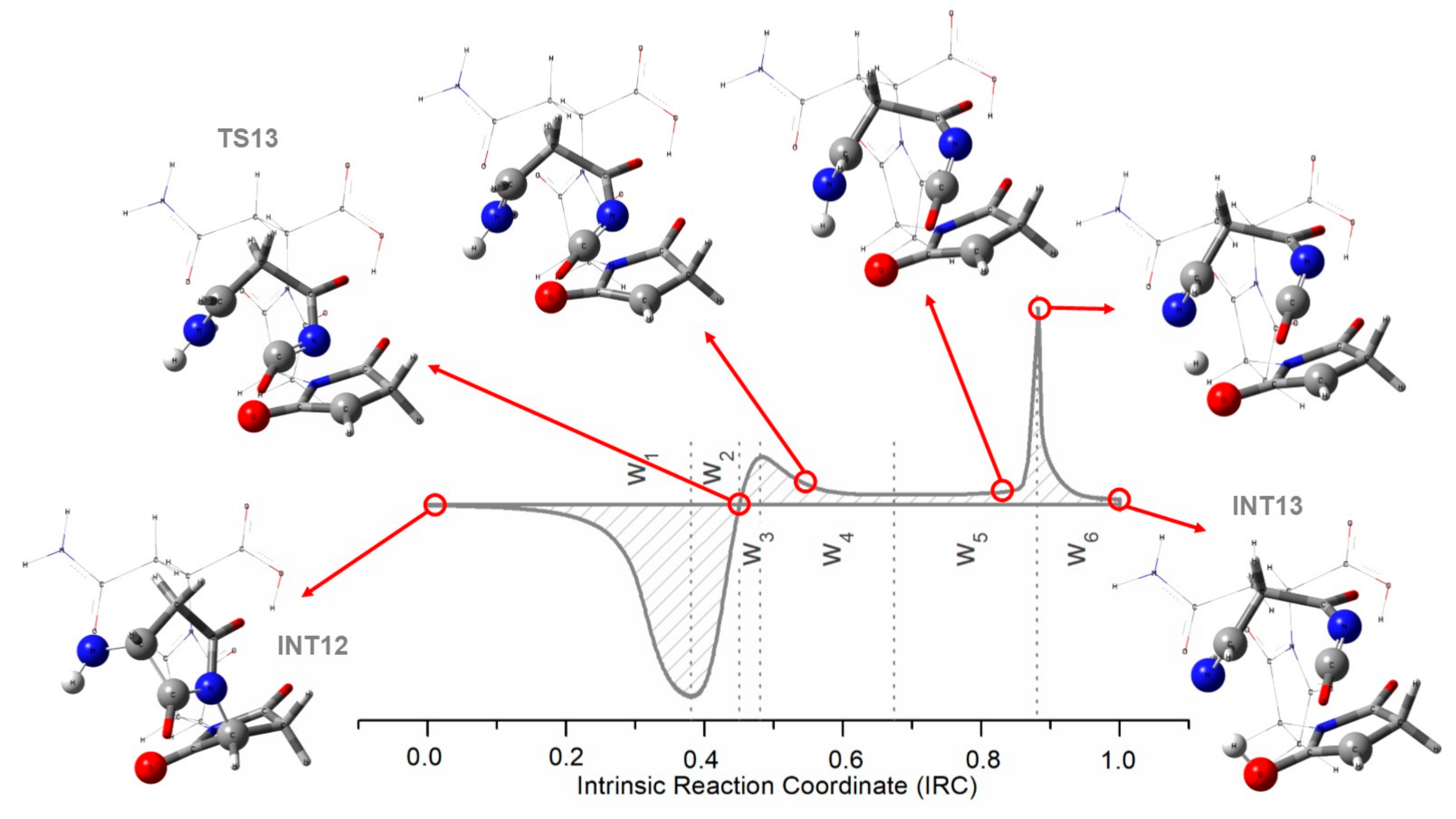
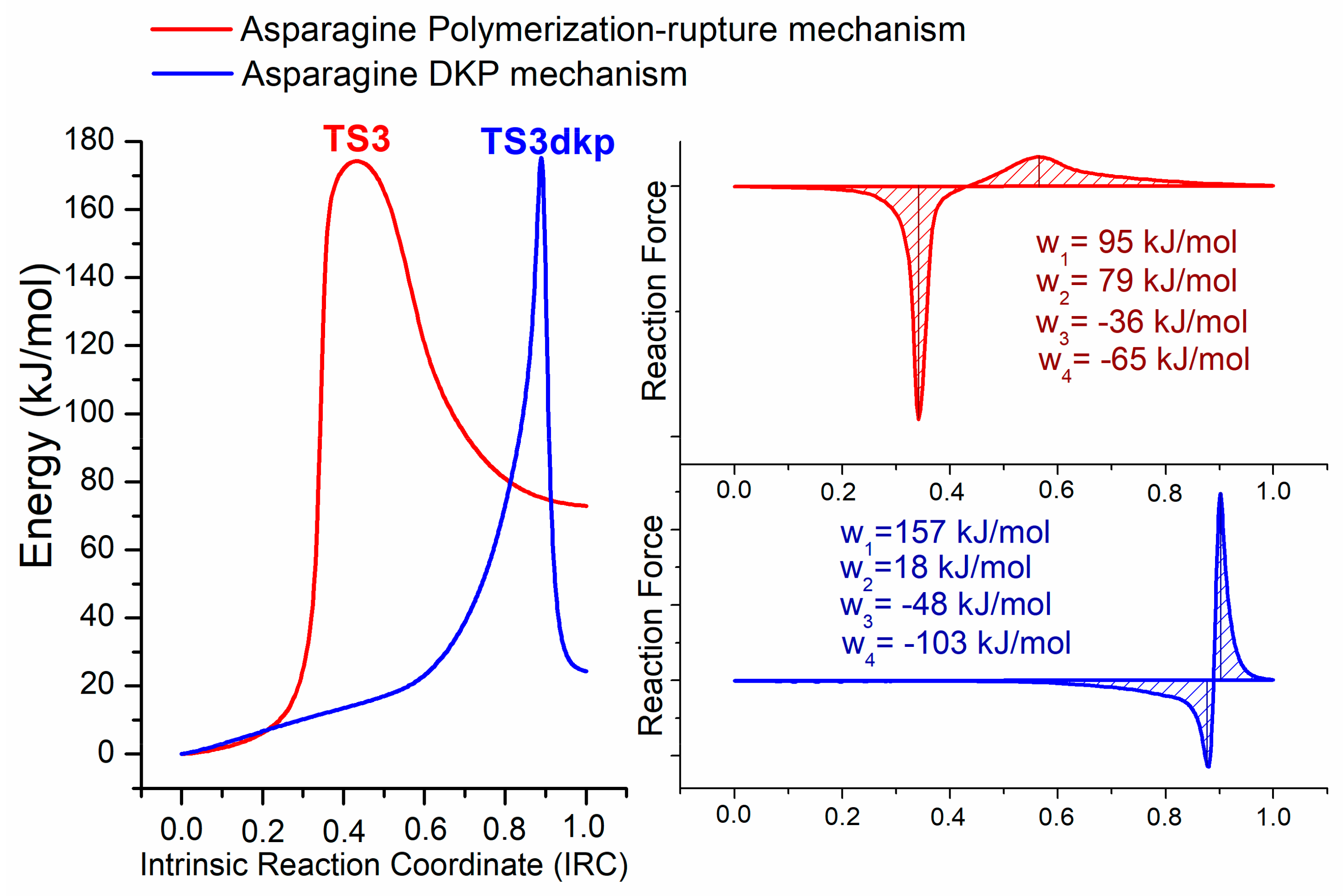
2.1.3. Reaction Force Analysis
2.1.4. NBO Analysis
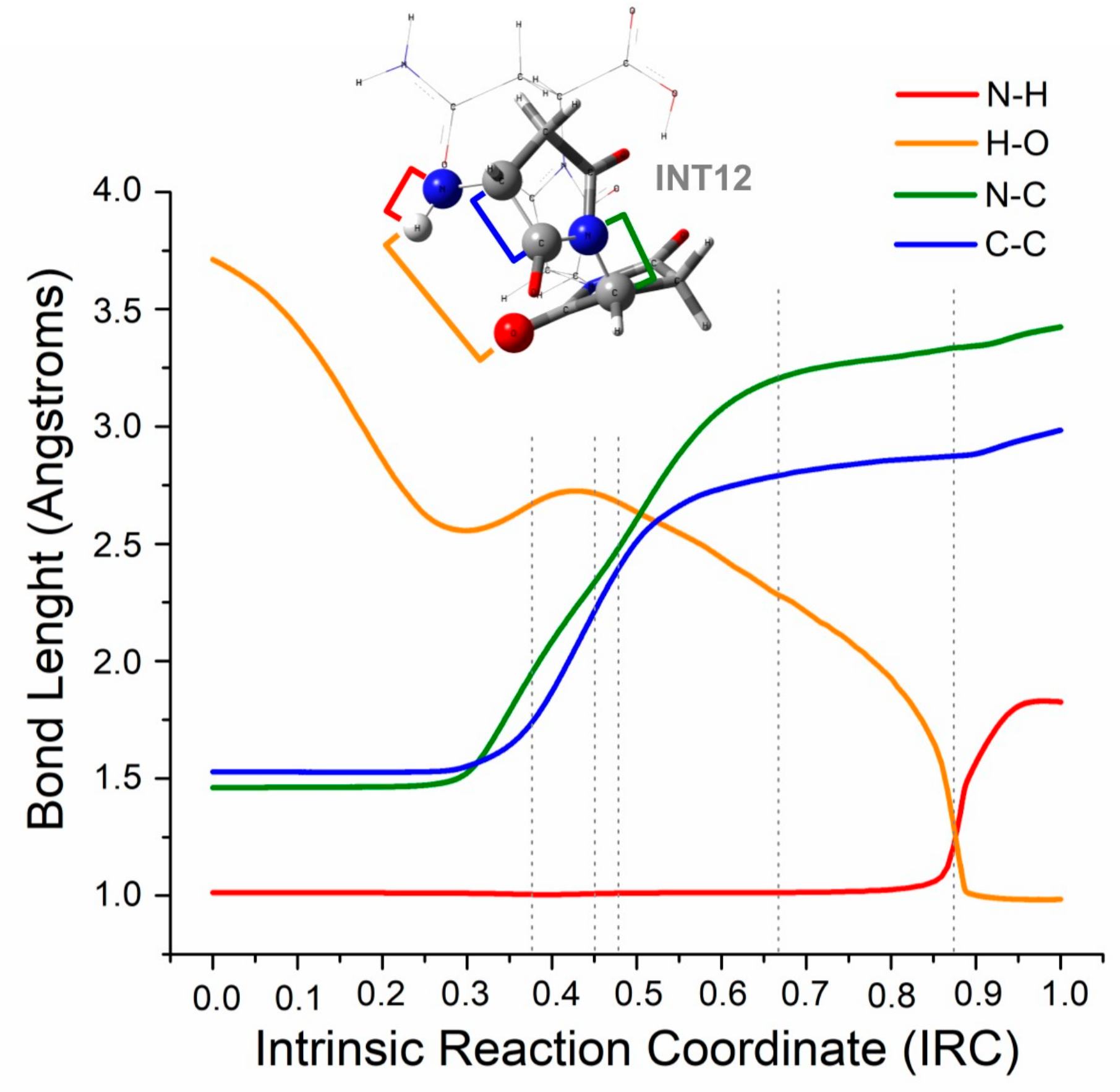
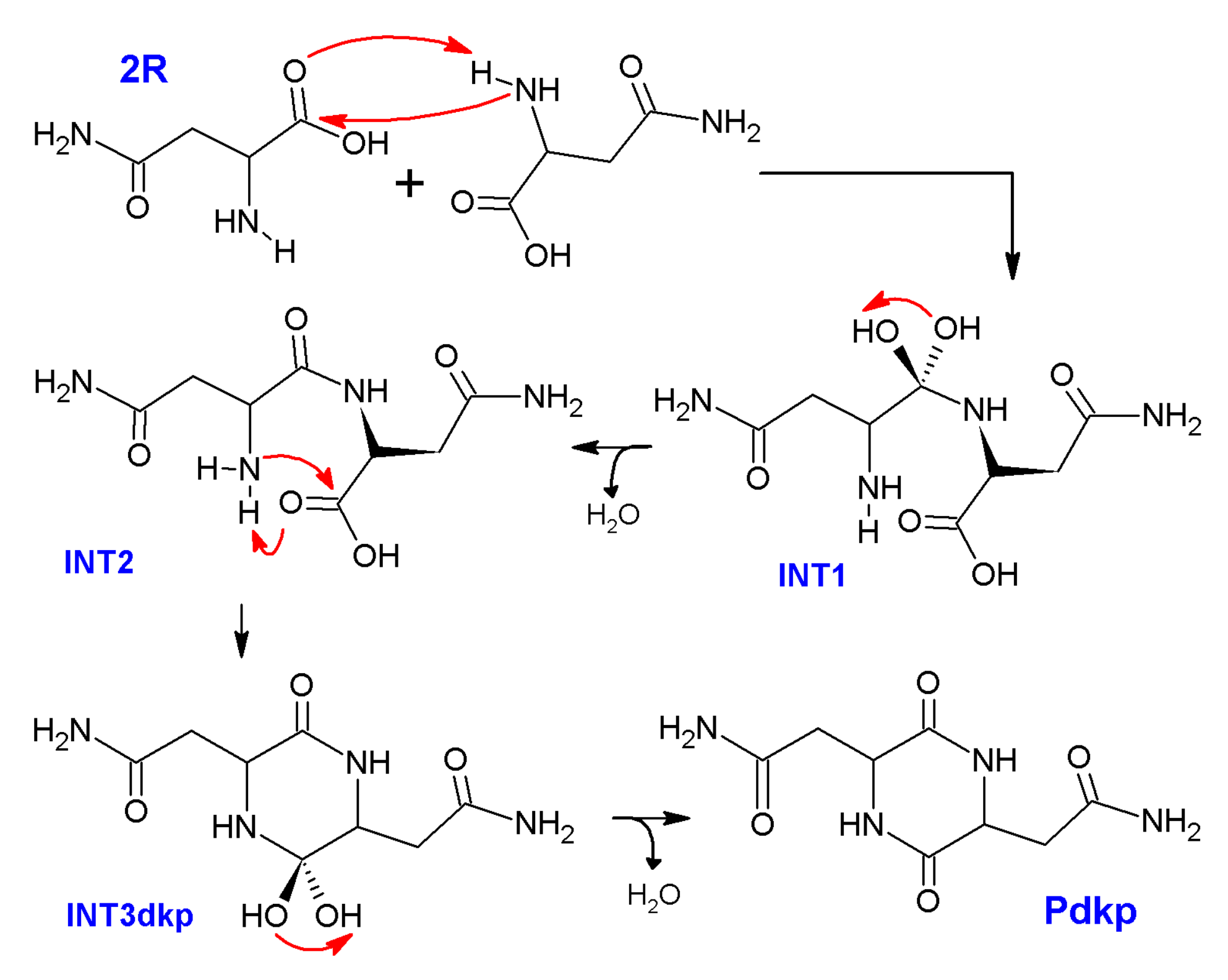
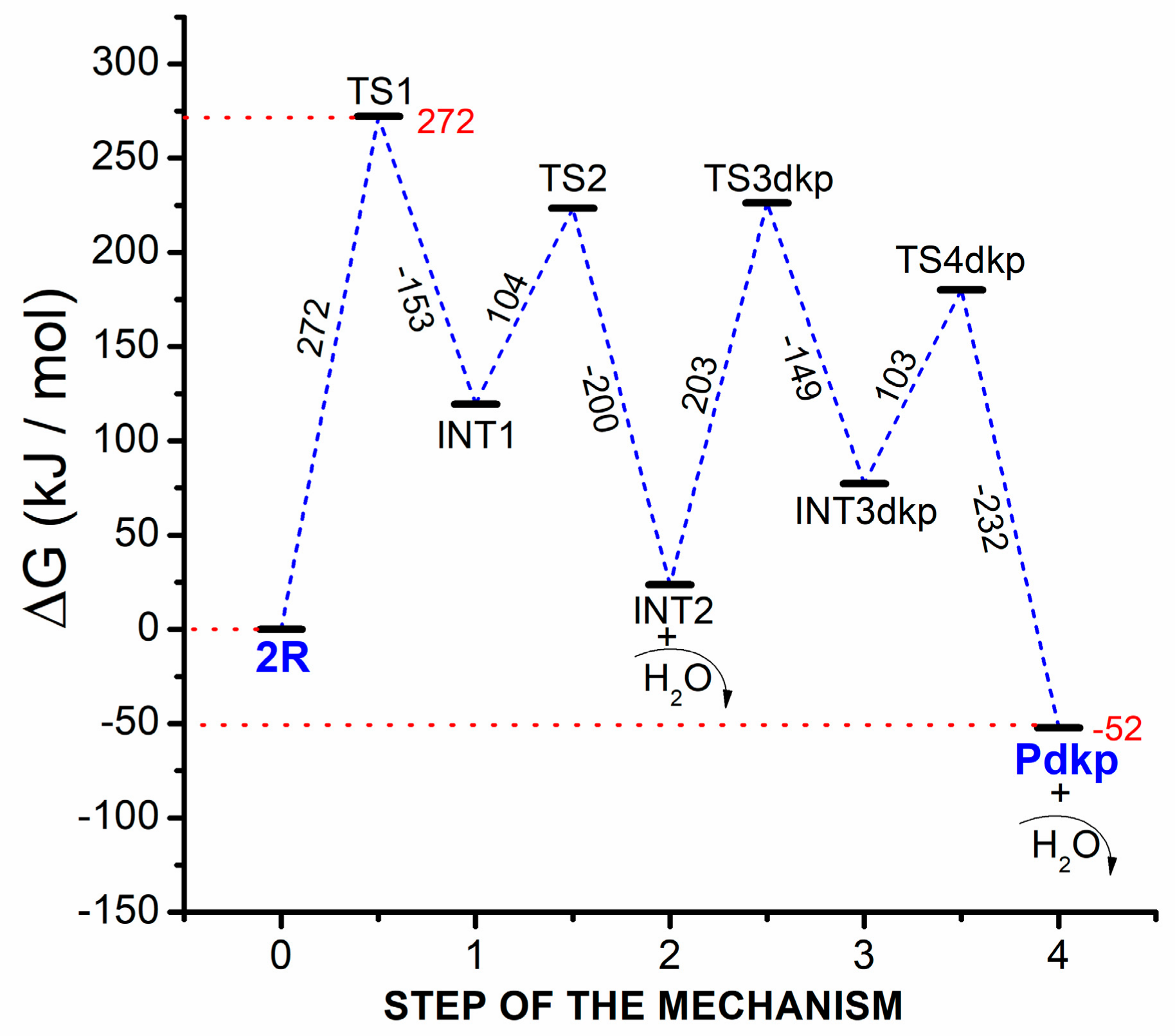
2.2. Theoretical Comparison: Why Does the Pyrolysis of Asparagine Not Produce the 2,5-Diketopiperazine Product?
Reaction Force Analysis on the Cyclization Process
3. Computational Methodology
3.1. Optimization of Structures and Determination of Thermodynamic Properties
3.2. Determination of Degrees of Rate Control and the Rate-Determinant Process
3.3. Reaction Force Analysis
3.4. NBO Analysis
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| MDPI | Multidisciplinary Digital Publishing Institute |
| DFT | Density Functional Theory |
| DKP | Diketopiperazine |
| TS | Transition State |
| INT | Intermediate |
| R | Reactive |
| P | Product |
| Res | Residues |
| NBO | Natural Bond Orbital |
References
- Moldoveanu, S.C. Pyrolysis of Organic Molecules with Applications to Health and Environmental Issues-Techniques and Instrumentation in Analytical Chemistry; Elsevier: Amsterdam, The Netherlands, 2010; Volume 28. [Google Scholar]
- Irwin, W.J. Analytical pyrolysis-an overview. J. Anal. Appl. Pyrolysis 1979, 1, 3–25. [Google Scholar] [CrossRef]
- Wang, S.; Dai, G.; Yang, H.; Luo, Z. Lignocellulosic biomass pyrolysis mechanism: A state-of-the-art review. Prog. Energy Combust. Sci. 2017, 62, 33–86. [Google Scholar] [CrossRef]
- Brea, O.; Loroño, M.; Marquez, E.; Mora, J.R.; Cordova, T.; Chuchani, G. Theoretical study of methoxy group influence in the gas-phase elimination kinetics of methoxyalkyl chlorides. Int. J. Quantum. Chem. 2012, 112, 2504–2514. [Google Scholar] [CrossRef]
- Julio, L.L.; Mora, J.R.; Maldonado, A.; Chuchani, G. Gas-phase elimination kinetics of selected aliphatic α,β-unsaturated aldehydes catalyzed by hydrogen chloride. J. Phys. Org. Chem. 2015, 28, 261–265. [Google Scholar] [CrossRef]
- Lezama, J.; Márquez, E.; Mora, J.R.; Córdova, T.; Chuchani, G. Theoretical calculations on the mechanisms of the gas phase elimination kinetics of chlorocyclohexane, 3-chlorocyclohexene and 4-chlorocyclohexene. J. Mol. Struct. THEOCHEM 2009, 916, 17–22. [Google Scholar] [CrossRef]
- Mora, J.R.; Loroño, M.; Cordova, T.; Chuchani, G. Ab initio calculations of the gas-phase elimination kinetics of ethyl oxamate, ethyl oxanilate, and ethyl N,N-dimethyl oxamate. J. Phys. Org. Chem. 2006, 19, 503–511. [Google Scholar] [CrossRef]
- Choi, S.S.; Ko, J.E. Dimerization reactions of amino acids by pyrolysis. J. Anal. Appl. Pyrolysis 2010, 89, 74–86. [Google Scholar] [CrossRef]
- Sharma, R.K.; Geoffrey Chan, W.; Seeman, J.I.; Hajaligol, M.R. Formation of low molecular weight heterocycles and polycyclic aromatic compounds (PACs) in the pyrolysis of α-amino acids. J. Anal. Appl. Pyrolysis 2003, 66, 97–121. [Google Scholar] [CrossRef]
- Chiavari, G.; Galletti, G.C. Pyrolysis-gas chromatography/mass spectrometry of amino acids. J. Anal. Appl. Pyrolysis 1992, 24, 123–137. [Google Scholar] [CrossRef]
- Kan, T.; Strezov, V.; Evans, T.J. Lignocellulosic biomass pyrolysis: A review of product properties and effects of pyrolysis parameters. Renew. Sustain. Energy Rev. 2016, 57, 1126–1140. [Google Scholar] [CrossRef]
- Sharma, R.K.; Chan, W.G.; Wang, J.; Waymack, B.E.; Wooten, J.B.; Seeman, J.I.; Hajaligol, M.R. On the role of peptides in the pyrolysis of amino acids. J. Anal. Appl. Pyrolysis 2004, 72, 153–163. [Google Scholar] [CrossRef]
- Ratcliff, A.; Medley, E.E.; Simmonds, P.G. Pyrolysis of amino acids. Mechanistic considerations. J. Org. Chem. 1974, 39, 1481–1490. [Google Scholar] [CrossRef] [PubMed]
- Funes-Ardoiz, I.; Paton, R.S. GoodVibes; Version 2.0.3; Zenode: Geneva, Switzerland, 2018. [Google Scholar]
- Uhe, A.; Kozuch, S.; Shaik, S. Software news and update automatic analysis of computed catalytic cycles. J. Comput. Chem. 2010, 32, 978–985. [Google Scholar] [CrossRef] [PubMed]
- Cervantes, C.; Mora, J.R.; Rincón, L.; Rodríguez, V. Theoretical study of the mechanism of 2,5-diketopiperazine formation during pyrolysis of proline. Mol. Phys. 2019. [Google Scholar] [CrossRef]
- Jones, R.O. Density functional theory: Its origins, rise to prominence, and future. Rev. Mod. Phys. 2015, 87, 897. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 16; Revision A. 03; Gaussian Inc.: Wallingford, CT, USA, 2016. [Google Scholar]
- Chai, J.-D.; Head-Gordon, M. Long-range corrected hybrid density functionals with improved dispersion corrections. Phys. Chem. Chem. Phys. 2008, 10, 6615–6620. [Google Scholar] [CrossRef] [PubMed]
- Krishnan, R.; Binkley, J.S.; Seeger, R.; Pople, J.A. Self-consistent molecular orbital methods. XX. A basis set for correlated wave functions. J. Chem. Phys. 1980, 72, 650–654. [Google Scholar] [CrossRef]
- Frisch, M.J.; Pople, J.A.; Binkley, J.S. Self-consistent molecular orbital methods 25. Supplementary functions for Gaussian basis sets. J. Chem. Phys. 1984, 80, 3265–3269. [Google Scholar] [CrossRef]
- Grimme, S. Supramolecular binding thermodynamics by dispersion-corrected density functional theory. Chem. A. Eur. J. 2012, 18, 9955–9964. [Google Scholar] [CrossRef] [PubMed]
- Fukui, K. The path of chemical reactions-The IRC Approach. Acc. Chem. Res. 1981, 14, 363–368. [Google Scholar] [CrossRef]
- Toro-Labbé, A.; Gutiérrez-Oliva, S.; Murray, J.S.; Politzer, P. The reaction force and the transition region of a reaction. J. Mol. Model. 2009, 15, 707–710. [Google Scholar] [CrossRef] [PubMed]
- Politzer, P.; Toro-Labeé, A.; Gutiérrez-Oliva, S.; Herrera, B.; Jaque, P.; Concha, M.C.; Murray, J.S. The reaction force: Three key points along an intrinsic reaction coordinate. J. Chem. Sci. 2005, 117, 467–472. [Google Scholar] [CrossRef]
- Martínez, J.; Toro-Labbé, A. The reaction force. A scalar property to characterize reaction mechanisms. J. Math. Chem. 2009, 45, 911–927. [Google Scholar] [CrossRef]
- Jaque, P.; Toro-Labbé, A.; Politzer, P.; Geerlings, P. Reaction force constant and projected force constants of vibrational modes along the path of an intramolecular proton transfer reaction. Chem. Phys. Lett. 2008, 456, 135–140. [Google Scholar] [CrossRef]
- Foster, J.P.; Weinhold, F. Natural Hybrid Orbitals. J. Am. Chem. Soc. 1980, 102, 7211–7218. [Google Scholar] [CrossRef]
- Mora, J.R.; Cervantes, C.; Marquez, E. New insight into the chloroacetanilide herbicide degradation mechanism through a nucleophilic attack of hydrogen sulfide. Int. J. Mol. Sci. 2018, 19, 1–13. [Google Scholar] [CrossRef] [PubMed]
- Wiberg, K.B. Application of the pople-santry-segal CNDO method to the cyclopropylcarbinyl and cyclobutyl cation and to bicyclobutane. Tetrahedron 1968, 24, 1083–1096. [Google Scholar] [CrossRef]
- Moyano, A.; Pericàs, M.A.; Valentí, E. A theoretical study on the mechanism of the thermal and the acid-catalyzed decarboxylation of 2-oxetanones (beta-Lactones). J. Org. Chem. 1989, 54, 573–582. [Google Scholar] [CrossRef]
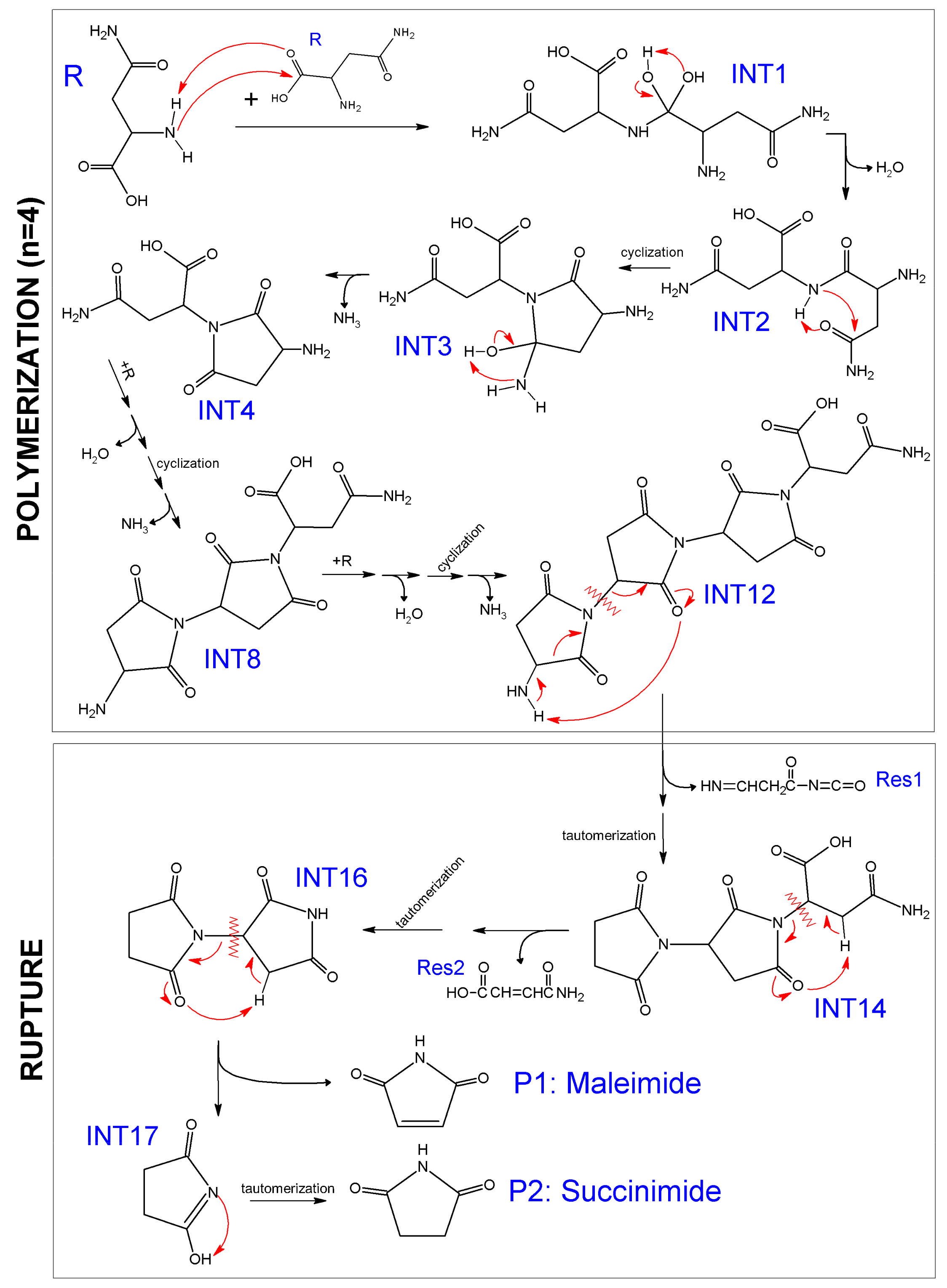
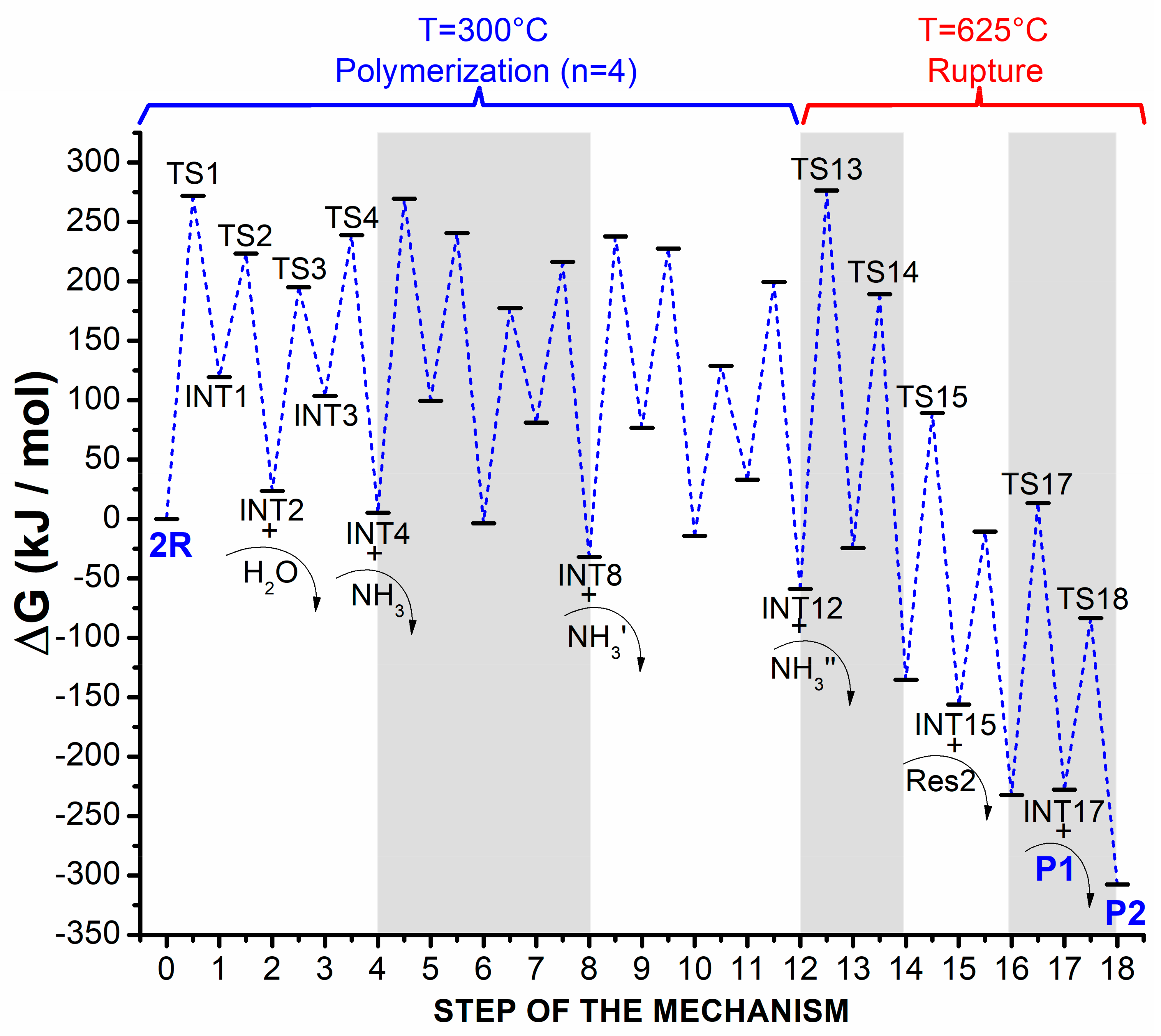


| T (°C) | i | X | Y | ΔGi (kJ mol−1) | Normalized Gibbs Free Energy, GY (kJ mol−1) |
|---|---|---|---|---|---|
| 300 | -- | -- | 2 R | -- | 0 * |
| 1 | 2 R | TS1 | 272 | 272 | |
| −1 | TS1 | INT1 | −153 | 119 | |
| 2 | INT1 | TS2 | 104 | 223 | |
| −2 | TS2 | INT2 + H2O | −200 | 24 | |
| 3 | INT2 | TS3 | 171 | 195 | |
| −3 | TS3 | INT3 | −91 | 104 | |
| 4 | INT3 | TS4 | 135 | 239 | |
| −4 | TS4 | INT4 + NH3 | −233 | 5 | |
| 5 | INT4+R’ | TS5 | 264 | 270 | |
| −5 | TS5 | INT5 | −170 | 100 | |
| 6 | INT5 | TS6 | 141 | 241 | |
| −6 | TS6 | INT6 + H2O’ | −244 | −4 | |
| 7 | INT6 | TS7 | 181 | 177 | |
| −7 | TS7 | INT7 | −96 | 81 | |
| 8 | INT7 | TS8 | 135 | 216 | |
| −8 | TS8 | INT8 + NH3′ | −248 | −32 | |
| 9 | INT8+R” | TS9 | 270 | 238 | |
| −9 | TS9 | INT9 | −161 | 77 | |
| 10 | INT9 | TS10 | 151 | 228 | |
| −10 | TS10 | INT10 + H2O” | −242 | −14 | |
| 11 | INT10 | TS11 | 143 | 129 | |
| −11 | TS11 | INT11 | −96 | 33 | |
| 12 | INT11 | TS12 | 167 | 200 | |
| −12 | TS12 | INT12 + NH3” | −259 | −59 | |
| 625 | −12 | TS12 | INT12 + NH3” | -- | −59 * |
| 13 | INT12 | TS13 | 336 | 276 | |
| −13 | TS13 | INT13 + Res1 | −301 | −24 | |
| 14 | INT13 | TS14 | 214 | 189 | |
| −14 | TS14 | INT14 | −325 | −135 | |
| 15 | INT14 | TS15 | 225 | 89 | |
| −15 | TS15 | INT15 + Res2 | −245 | −156 | |
| 16 | INT15 | TS16 | 146 | −10 | |
| −16 | TS16 | INT16 | −222 | −232 | |
| 17 | INT16 | TS17 | 246 | 14 | |
| −17 | TS17 | INT17 + P1 | −241 | −228 | |
| 18 | INT17 | TS18 | 145 | −83 | |
| −18 | TS18 | P2 | −224 | −307 |
| step i | Mini | |||
|---|---|---|---|---|
| 1 | 2 R | 7.02 × 10 −4 | TS1 | 1.97 × 10 −4 |
| 2 | INT1 | 5.70 10 −11 | TS2 | 2.93 × 10 −7 |
| 3 | INT2 + H2O | 2.12 × 10 −5 | TS3 | 6.77 × 10 −9 |
| 4 | INT3 | 4.66 × 10 −10 | TS4 | 2.41 × 10 −6 |
| 5 | INT4 + NH3 | 2.42 × 10 −4 | TS5 | 2.15 × 10 −4 |
| 6 | INT5 | 5.83 × 10 −10 | TS6 | 4.49 × 10 −6 |
| 7 | INT6 + H2O’ | 5.77 × 10 −4 | TS7 | 1.93 × 10 −9 |
| 8 | INT7 | 6.86 × 10 −9 | TS8 | 3.54 × 10 −7 |
| 9 | INT8 + NH3′ | 2.56 × 10 −2 | TS9 | 1.52 × 10 −4 |
| 10 | INT9 | 1.2210 −8 | TS10 | 3.82 × 10 −5 |
| 11 | INT10 + H2O” | 2.35 × 10 −3 | TS11 | 7.64 × 10 −11 |
| 12 | INT11 | 4.27 × 10 −6 | TS12 | 9.70 × 10 −7 |
| 13 * | INT12 + NH3” | 9.71 × 10 −1 | TS13 | 9.99 × 10 −1 |
| 14 | INT13 + Res1 | 7.72 × 10 −8 | TS14 | 8.41 × 10 −6 |
| 15 | INT14 | 3.41 × 10 −7 | TS15 | 3.41 × 10 −7 |
| 16 | INT15 + Res2 | 2.29 × 10 −10 | TS16 | 9.50 × 10 −12 |
| 17 | INT16 | 5.88 × 10 −6 | TS17 | 5.85 × 10 −6 |
| 18 | INT17 + P1 | 1.67 × 10 −8 | TS18 | 2.12 × 10 −11 |
| - | P2 | --- | --- | --- |
| 1 | 2 R | 1.00 × 100 | TS1 | 1.00 × 100 |
| 2 | INT1 | 3.69 × 10−5 | TS2 | 1.55 × 10−15 |
| 3 | INT2+H2O | 6.55 × 10−5 | TS3dkp | 5.75 × 10−7 |
| 4 | INT3dkp | 4.16 × 10−9 | TS4dkp | 1.59 × 10−12 |
| - | Pdkp+H2O | --- | --- | --- |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cervantes, C.; Mora, J.R.; Marquez, E.; Torres, J.; Rincón, L.; Mendez, M.A.; Alcázar, J.J. Theoretical Calculations of the Multistep Reaction Mechanism Involved in Asparagine Pyrolysis Supported by Degree of Rate Control and Thermodynamic Control Analyses. Appl. Sci. 2019, 9, 4847. https://doi.org/10.3390/app9224847
Cervantes C, Mora JR, Marquez E, Torres J, Rincón L, Mendez MA, Alcázar JJ. Theoretical Calculations of the Multistep Reaction Mechanism Involved in Asparagine Pyrolysis Supported by Degree of Rate Control and Thermodynamic Control Analyses. Applied Sciences. 2019; 9(22):4847. https://doi.org/10.3390/app9224847
Chicago/Turabian StyleCervantes, Cristian, Jose R. Mora, Edgar Marquez, Javier Torres, Luis Rincón, Miguel A. Mendez, and Jackson J. Alcázar. 2019. "Theoretical Calculations of the Multistep Reaction Mechanism Involved in Asparagine Pyrolysis Supported by Degree of Rate Control and Thermodynamic Control Analyses" Applied Sciences 9, no. 22: 4847. https://doi.org/10.3390/app9224847
APA StyleCervantes, C., Mora, J. R., Marquez, E., Torres, J., Rincón, L., Mendez, M. A., & Alcázar, J. J. (2019). Theoretical Calculations of the Multistep Reaction Mechanism Involved in Asparagine Pyrolysis Supported by Degree of Rate Control and Thermodynamic Control Analyses. Applied Sciences, 9(22), 4847. https://doi.org/10.3390/app9224847






