Stress-Controlled Frequency Tuning and Parametric Amplification of the Vibrations of Coupled Nanomembranes
Abstract
:1. Introduction
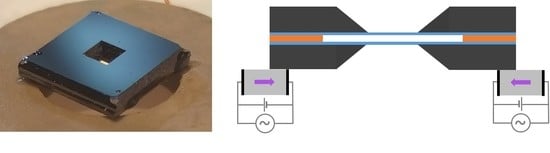
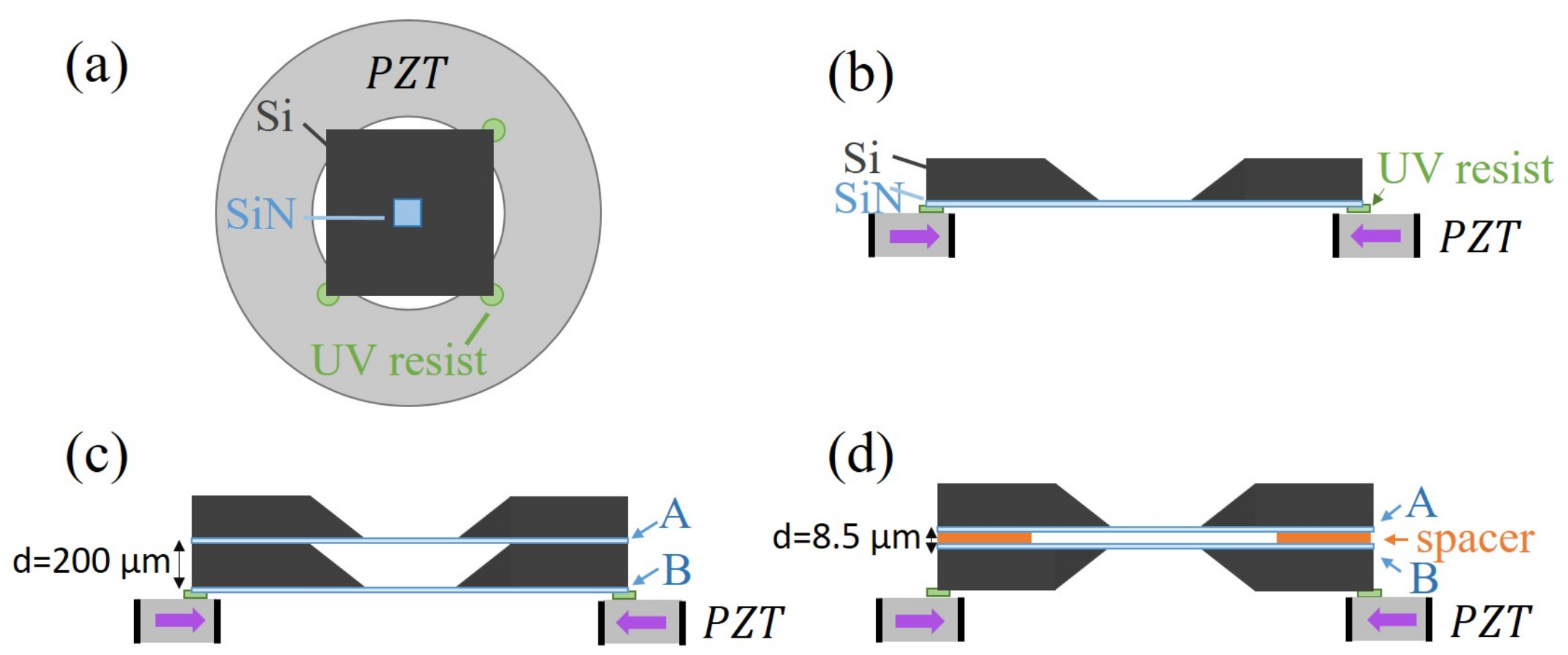
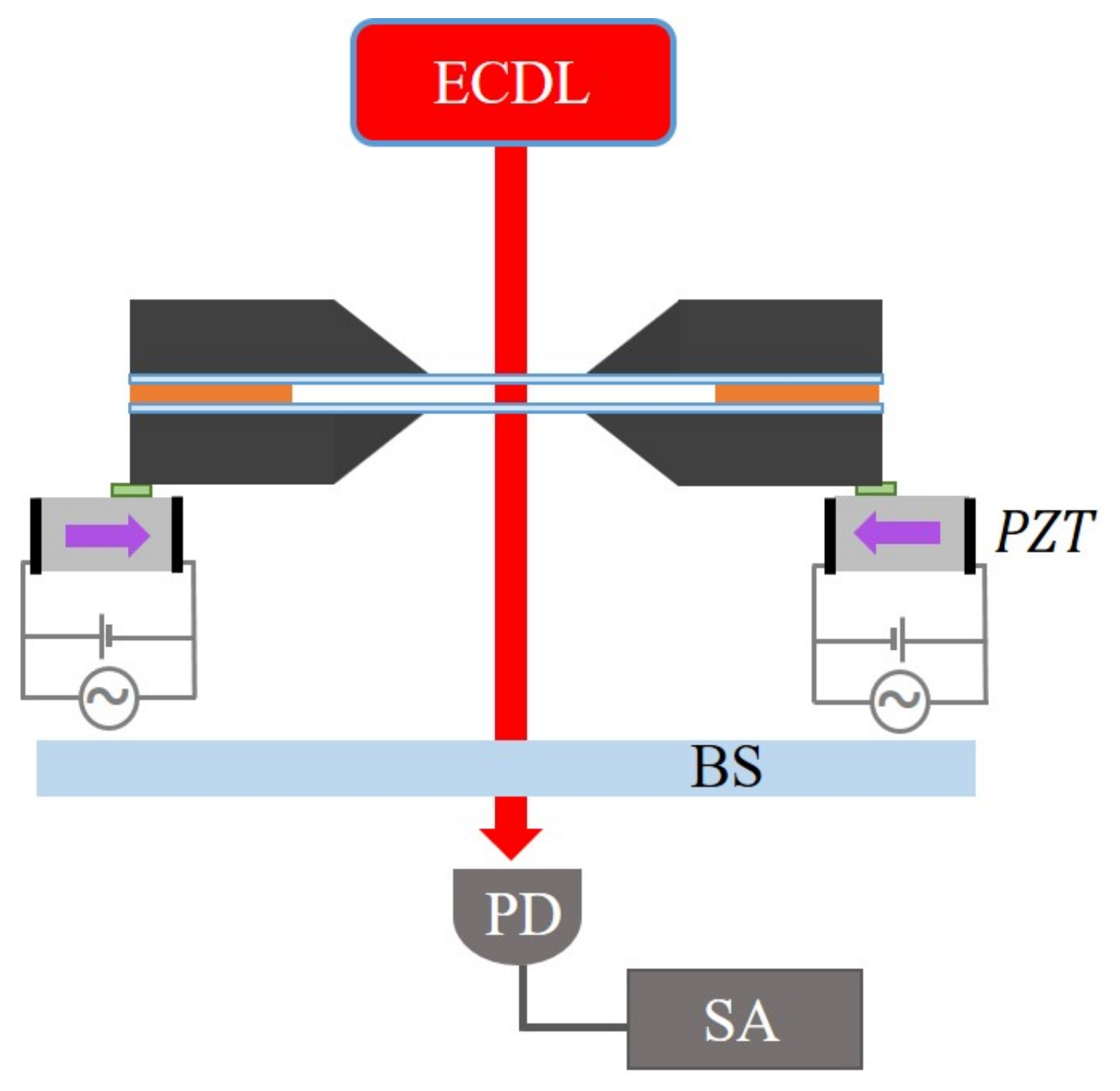
2. Electromechanical Resonators and Experimental Setup
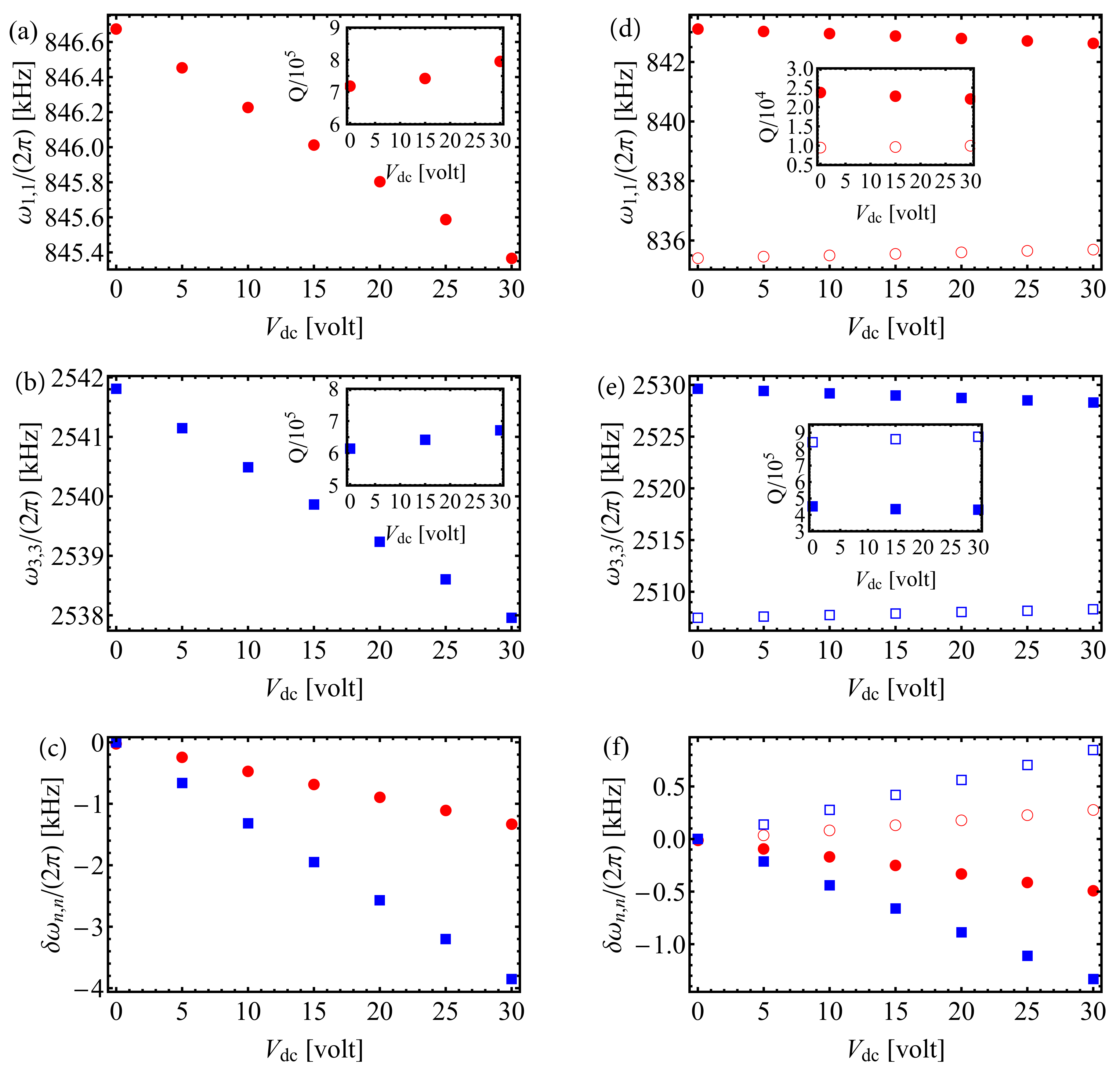
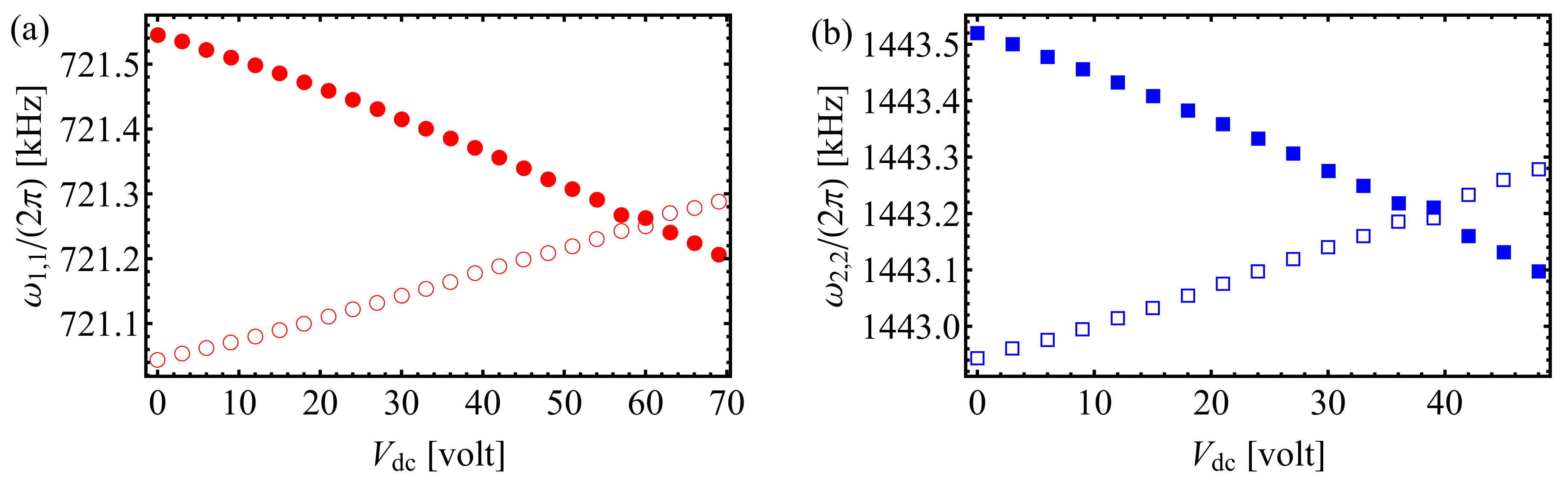
3. Mechanical Frequency Tuning
4. Parametric Amplification of the Thermal Fluctuations
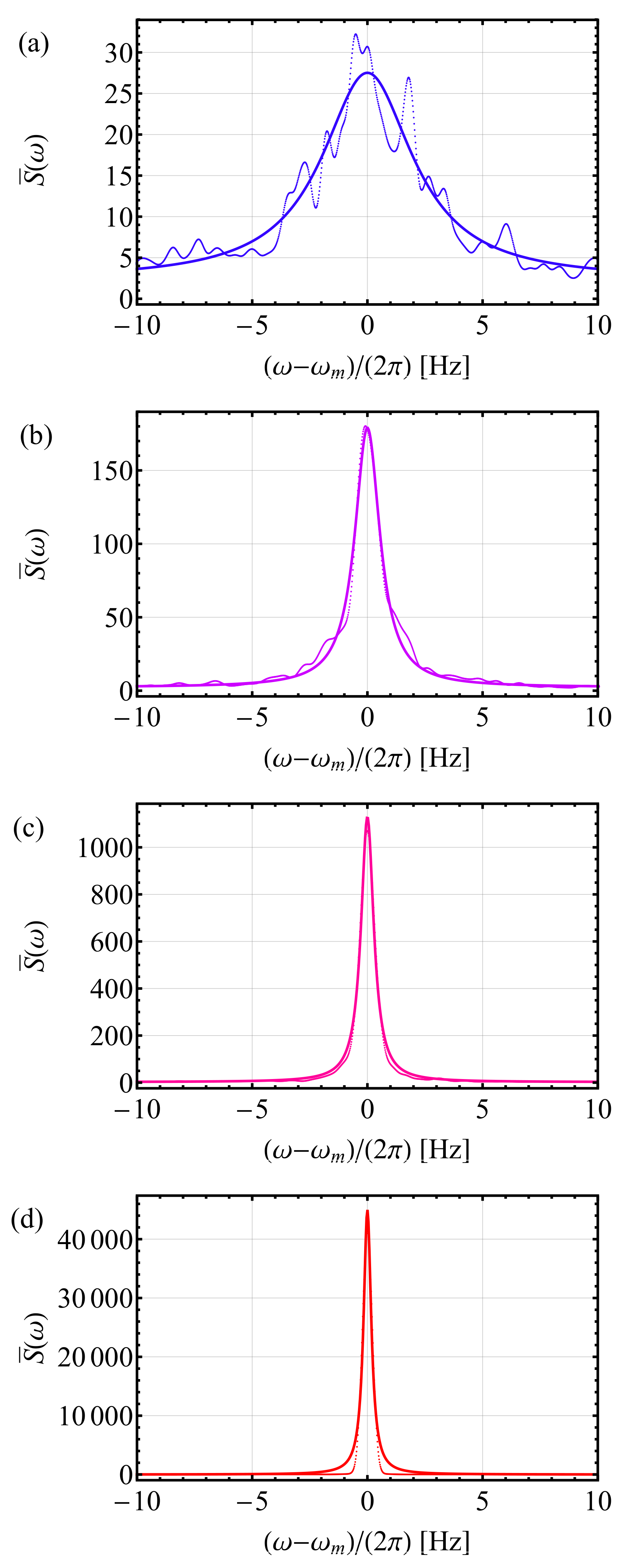
4.1. Theoretical Model
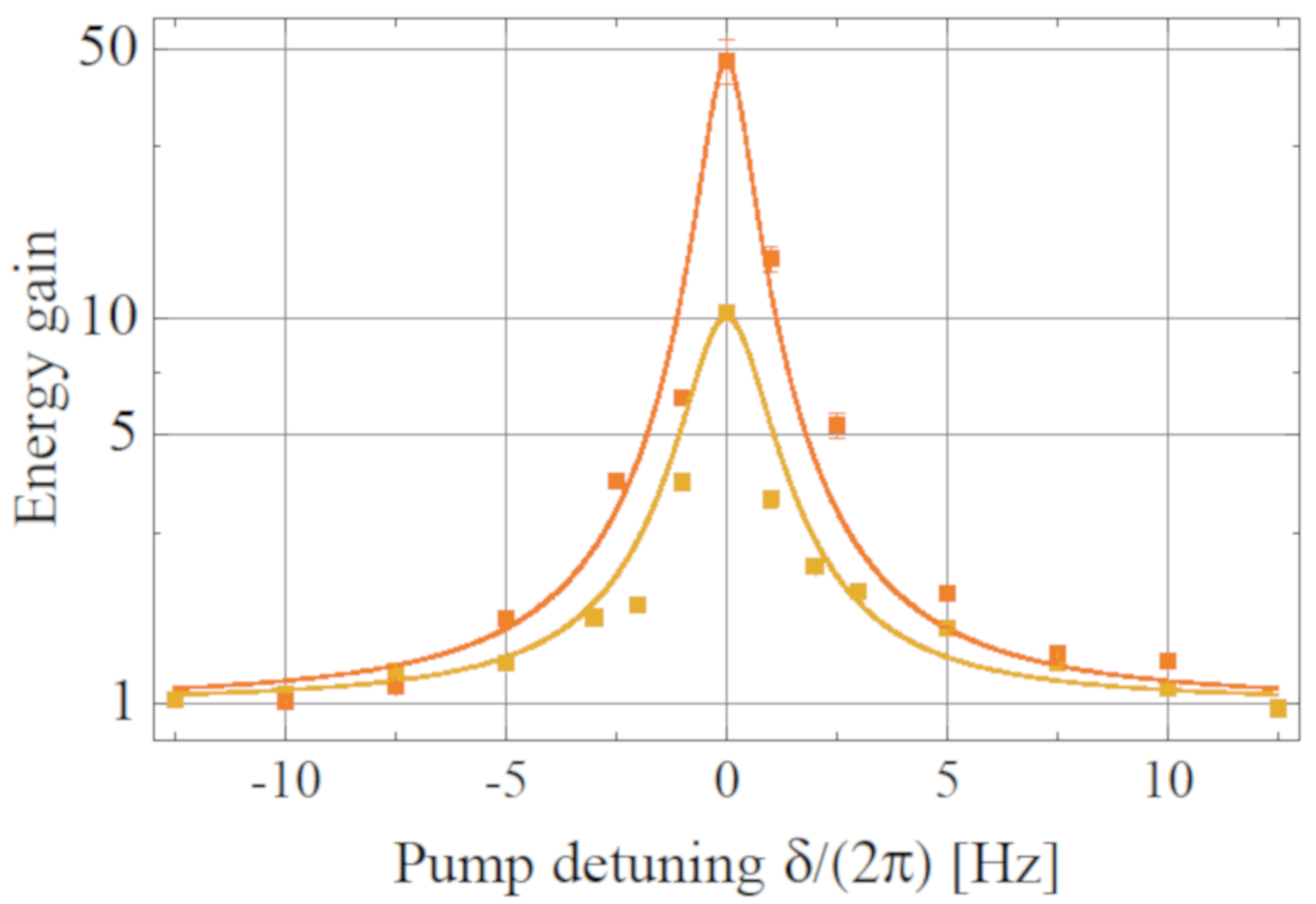
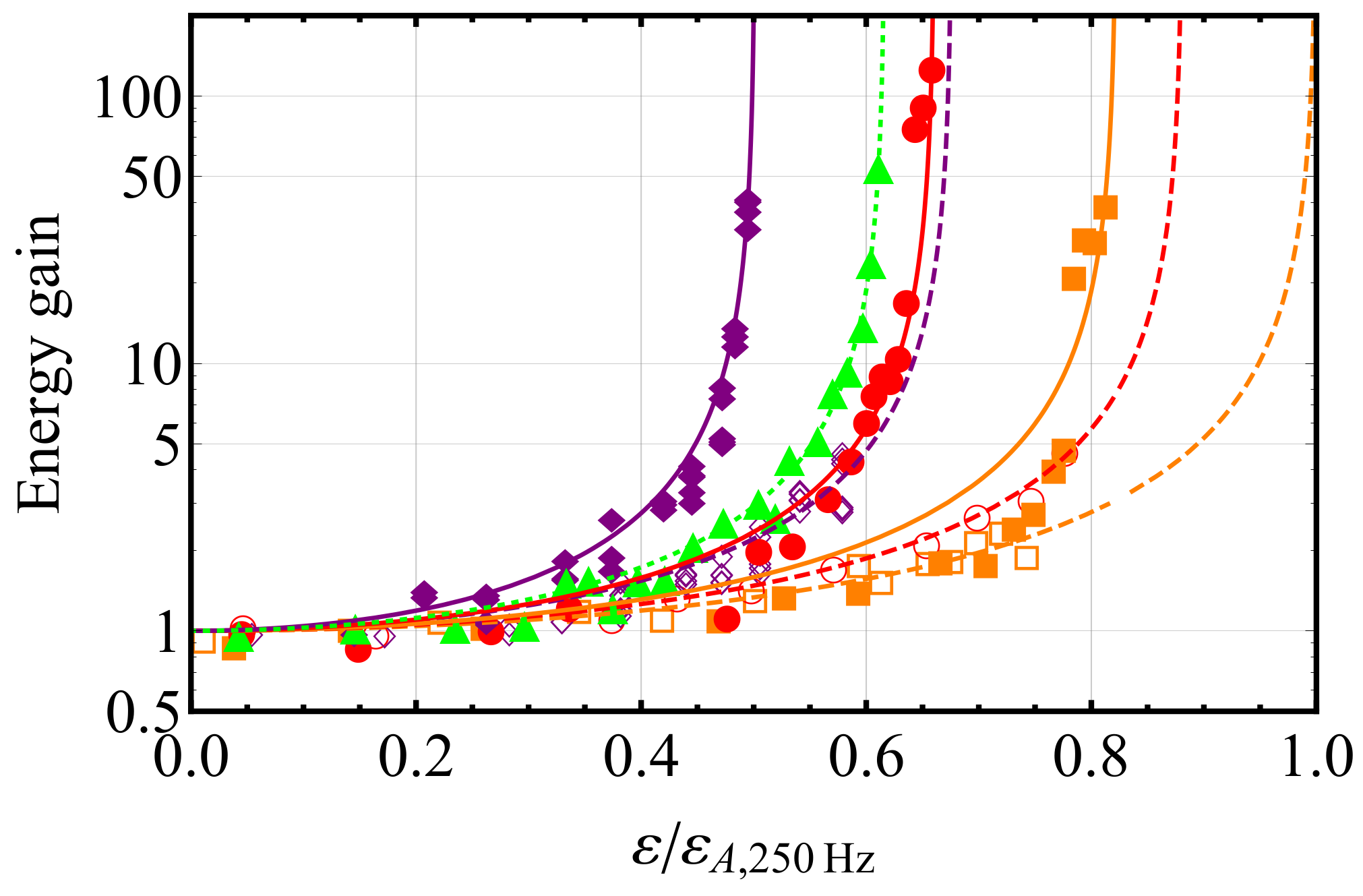
4.2. Experimental Results
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
References
- Ekinci, K.L.; Roukes, M.L. Nanoelectromechanical systems. Rev. Sci. Instrum. 2005, 76, 061101. [Google Scholar] [CrossRef]
- Li, M.; Tang, H.X.; Roukes, M.L. Ultra-sensitive NEMS-based cantilevers for sensing, scanned probe and very high-frequency applications. Nat. Nanotechnol. 2007, 2, 114. [Google Scholar] [CrossRef] [PubMed]
- Kozinsky, I.; Postma, H.W.C.; Bargatin, I.; Roukes, M.L. Tuning nonlinearity, dynamic range, and frequency of nanomechanical resonators. Appl. Phys. Lett. 2006, 88, 253101. [Google Scholar] [CrossRef]
- Jun, S.C.; Huang, X.M.H.; Manolidis, M.; Zorman, C.A.; Mehregany, M.; Hone, J. Electrothermal tuning of Al–SiC nanomechanical resonators. Nanotechnology 2006, 17, 1506–1511. [Google Scholar] [CrossRef]
- Hüttel, A.K.; Steele, G.A.; Witkamp, B.; Poot, M.; Kouwenhoven, L.P.; van der Zant, H.S.J. Carbon Nanotubes as Ultrahigh Quality Factor Mechanical Resonators. Nano Lett. 2009, 9, 2547–2552. [Google Scholar] [CrossRef] [PubMed]
- Barton, R.A.; Storch, I.R.; Adiga, V.P.; Sakakibara, R.; Cipriany, B.R.; Ilic, B.; Wang, S.P.; Ong, P.; McEuen, P.L.; Parpia, J.M.; et al. Photothermal Self-Oscillation and Laser Cooling of Graphene Optomechanical Systems. Nano Lett. 2012, 12, 4681–4686. [Google Scholar] [CrossRef] [PubMed]
- Okamoto, H.; Kamada, T.; Onomitsu, K.; Mahboob, I.; Yamaguchi, H. Optical Tuning of Coupled Micromechanical Resonators. Appl. Phys. Express 2009, 2, 062202. [Google Scholar] [CrossRef]
- Jöckel, A.; Rakher, M.T.; Korppi, M.; Camerer, S.; Hunger, D.; Mader, M.; Treutlein, P. Spectroscopy of mechanical dissipation in micro-mechanical membranes. Appl. Phys. Lett. 2011, 99, 143109. [Google Scholar] [CrossRef]
- Inoue, T.; Anno, Y.; Imakita, Y.; Takei, K.; Arie, T.; Akita, S. Resonance Control of a Graphene Drum Resonator in a Nonlinear Regime by a Standing Wave of Light. ACS Omega 2017, 2, 5792–5797. [Google Scholar] [CrossRef] [PubMed]
- Verbridge, S.S.; Shapiro, D.F.; Craighead, H.G.; Parpia, J.M. Macroscopic Tuning of Nanomechanics: Substrate Bending for Reversible Control of Frequency and Quality Factor of Nanostring Resonators. Nano Lett. 2007, 7, 1728–1735. [Google Scholar] [CrossRef] [PubMed]
- St-Gelais, R.; Bernard, S.; Reinhardt, C.; Sankey, J.C. Swept-Frequency Drumhead Optomechanical Resonators. ACS Photon. 2019, 6, 525–530. [Google Scholar] [CrossRef]
- Almog, R.; Zaitsev, S.; Shtempluck, O.; Buks, E. Signal amplification in a nanomechanical Duffing resonator via stochastic resonance. Appl. Phys. Lett. 2007, 90, 013508. [Google Scholar] [CrossRef]
- Antoni, T.; Makles, K.; Braive, R.; Briant, T.; Cohadon, P.F.; Sagnes, I.; Robert-Philip, I.; Heidmann, A. Nonlinear mechanics with suspended nanomembranes. EPL Europhys. Lett. 2012, 100, 68005. [Google Scholar] [CrossRef]
- Thomas, O.; Mathieu, F.; Mansfield, W.; Huang, C.; Trolier-McKinstry, S.; Nicu, L. Efficient parametric amplification in micro-resonators with integrated piezoelectric actuation and sensing capabilities. Appl. Phys. Lett. 2013, 102, 163504. [Google Scholar] [CrossRef]
- Buks, E.; Roukes, M.L. Electrically tunable collective response in a coupled micromechanical array. J. Microelectromech. Syst. 2002, 11, 802–807. [Google Scholar] [CrossRef]
- Sage, E.; Sansa, M.; Fostner, S.; Defoort, M.; Gély, M.; Naik, A.K.; Morel, R.; Duraffourg, L.; Roukes, M.L.; Alava, T.; et al. Single-particle mass spectrometry with arrays of frequency-addressed nanomechanical resonators. Nat. Commun. 2018, 9, 3283. [Google Scholar] [CrossRef] [PubMed]
- Zhao, C.; Zhou, X.; Pandit, M.; Sobreviela, G.; Du, S.; Zou, X.; Seshia, A. Toward High-Resolution Inertial Sensors Employing Parametric Modulation in Coupled Micromechanical Resonators. Phys. Rev. Appl. 2019, 12, 044005. [Google Scholar] [CrossRef]
- Shim, S.B.; Imboden, M.; Mohanty, P. Synchronized Oscillation in Coupled Nanomechanical Oscillators. Science 2007, 316, 95–99. [Google Scholar] [CrossRef] [PubMed]
- Matheny, M.H.; Grau, M.; Villanueva, L.G.; Karabalin, R.B.; Cross, M.C.; Roukes, M.L. Phase Synchronization of Two Anharmonic Nanomechanical Oscillators. Phys. Rev. Lett. 2014, 112, 014101. [Google Scholar] [CrossRef] [PubMed]
- Deng, G.W.; Zhu, D.; Wang, X.H.; Zou, C.L.; Wang, J.T.; Li, H.O.; Cao, G.; Liu, D.; Li, Y.; Xiao, M.; et al. Strongly Coupled Nanotube Electromechanical Resonators. Nano Lett. 2016, 16, 5456–5462. [Google Scholar] [CrossRef] [PubMed]
- Luo, G.; Zhang, Z.Z.; Deng, G.W.; Li, H.O.; Cao, G.; Xiao, M.; Guo, G.C.; Tian, L.; Guo, G.P. Strong indirect coupling between graphene-based mechanical resonators via a phonon cavity. Nat. Commun. 2018, 9, 383. [Google Scholar] [CrossRef] [PubMed]
- Matheny, M.H.; Emenheiser, J.; Fon, W.; Chapman, A.; Salova, A.; Rohden, M.; Li, J.; Hudoba de Badyn, M.; Pósfai, M.; Duenas-Osorio, L.; et al. Exotic states in a simple network of nanoelectromechanical oscillators. Science 2019, 363. [Google Scholar] [CrossRef] [PubMed]
- Bagheri, M.; Poot, M.; Li, M.; Pernice, W.P.H.; Tang, H.X. Dynamic manipulation of nanomechanical resonators in the high-amplitude regime and non-volatile mechanical memory operation. Nat. Nanotechnol. 2011, 6, 726–732. [Google Scholar] [CrossRef] [PubMed]
- Mahboob, I.; Nishiguchi, K.; Okamoto, H.; Yamaguchi, H. Phonon-cavity electromechanics. Nat. Phys. 2012, 8, 503. [Google Scholar] [CrossRef]
- Faust, T.; Rieger, J.; Seitner, M.J.; Kotthaus, J.P.; Weig, E.M. Coherent control of a classical nanomechanical two-level system. Nat. Phys. 2013, 9, 485. [Google Scholar] [CrossRef]
- Okamoto, H.; Gourgout, A.; Chang, C.Y.; Onomitsu, K.; Mahboob, I.; Chang, E.Y.; Yamaguchi, H. Coherent phonon manipulation in coupled mechanical resonators. Nat. Phys. 2013, 9, 480. [Google Scholar] [CrossRef]
- Mahboob, I.; Okamoto, H.; Onomitsu, K.; Yamaguchi, H. Two-Mode Thermal-Noise Squeezing in an Electromechanical Resonator. Phys. Rev. Lett. 2014, 113, 167203. [Google Scholar] [CrossRef] [PubMed]
- Mathew, J.P.; Patel, R.N.; Borah, A.; Vijay, R.; Deshmukh, M.M. Dynamical strong coupling and parametric amplification of mechanical modes of graphene drums. Nat. Nanotechnol. 2016, 11, 747. [Google Scholar] [CrossRef] [PubMed]
- Chen, C.; Zanette, D.H.; Czaplewski, D.A.; Shaw, S.; López, D. Direct observation of coherent energy transfer in nonlinear micromechanical oscillators. Nat. Commun. 2017, 8, 15523. [Google Scholar] [CrossRef] [PubMed]
- Yang, F.; Rochau, F.; Huber, J.S.; Brieussel, A.; Rastelli, G.; Weig, E.M.; Scheer, E. Spatial Modulation of Nonlinear Flexural Vibrations of Membrane Resonators. Phys. Rev. Lett. 2019, 122, 154301. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.Z.; Song, X.X.; Luo, G.; Su, Z.J.; Wang, K.L.; Cao, G.; Li, H.O.; Xiao, M.; Guo, G.C.; Tian, L.; et al. Coherent Phonon Dynamics in Spatially-Separated Graphene Mechanical Resonators. arXiv 2019, arXiv:1909.11311. [Google Scholar]
- Thompson, J.D.; Zwickl, B.M.; Jayich, A.M.; Marquardt, F.; Girvin, S.M.; Harris, J.G.E. Strong dispersive coupling of a high-finesse cavity to a micromechanical membrane. Nature 2008, 452, 72–75. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wilson, D.J.; Regal, C.A.; Papp, S.B.; Kimble, H.J. Cavity Optomechanics with Stoichiometric SiN Films. Phys. Rev. Lett. 2009, 103, 207204. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Purdy, T.P.; Peterson, R.W.; Regal, C.A. Observation of Radiation Pressure Shot Noise on a Macroscopic Object. Science 2013, 339, 801–804. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bagci, T.; Simonsen, A.; Schmid, S.; Villanueva, L.G.; Zeuthen, E.; Appel, J.; Taylor, J.M.; Sørensen, A.; Usami, K.; Schliesser, A.; et al. Optical detection of radio waves through a nanomechanical transducer. Nature 2014, 507, 81. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Andrews, R.W.; Peterson, R.W.; Purdy, T.P.; Cicak, K.; Simmonds, R.W.; Regal, C.A.; Lehnert, K.W. Bidirectional and efficient conversion between microwave and optical light. Nat. Phys. 2014, 10, 321. [Google Scholar] [CrossRef] [Green Version]
- Yuan, M.; Singh, V.; Blanter, Y.M.; Steele, G.A. Large cooperativity and microkelvin cooling with a three-dimensional optomechanical cavity. Nat. Commun. 2015, 6, 8491. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Xu, H.; Mason, D.; Jiang, L.; Harris, J.G.E. Topological energy transfer in an optomechanical system with exceptional points. Nature 2016, 80, 537. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Rossi, M.; Mason, D.; Chen, J.; Tsaturyan, Y.; Schliesser, A. Measurement-based quantum control of mechanical motion. Nature 2018, 563, 53–58. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Nair, B.; Naesby, A.; Dantan, A. Optomechanical characterization of silicon nitride membrane arrays. Opt. Lett. 2017, 42, 1341–1344. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Piergentili, P.; Catalini, L.; Bawaj, M.; Zippilli, S.; Malossi, N.; Natali, R.; Vitali, D.; Giuseppe, G.D. Two-membrane cavity optomechanics. N. J. Phys. 2018, 20, 083024. [Google Scholar] [CrossRef]
- Gärtner, C.; Moura, J.P.; Haaxman, W.; Norte, R.A.; Gröblacher, S. Integrated Optomechanical Arrays of Two High Reflectivity SiN Membranes. Nano Lett. 2018, 18, 7171–7175. [Google Scholar] [CrossRef] [PubMed]
- Wei, X.; Sheng, J.; Yang, C.; Wu, Y.; Wu, H. Controllable two-membrane-in-the-middle cavity optomechanical system. Phys. Rev. A 2019, 99, 023851. [Google Scholar] [CrossRef] [Green Version]
- Xuereb, A.; Genes, C.; Dantan, A. Strong Coupling and Long-Range Collective Interactions in Optomechanical Arrays. Phys. Rev. Lett. 2012, 109, 223601. [Google Scholar] [CrossRef] [PubMed]
- Xuereb, A.; Genes, C.; Dantan, A. Collectively enhanced optomechanical coupling in periodic arrays of scatterers. Phys. Rev. A 2013, 88, 053803. [Google Scholar] [CrossRef]
- Seok, H.; Buchmann, L.F.; Singh, S.; Meystre, P. Optically mediated nonlinear quantum optomechanics. Phys. Rev. A 2012, 86, 063829. [Google Scholar] [CrossRef] [Green Version]
- Kipf, T.; Agarwal, G.S. Superradiance and collective gain in multimode optomechanics. Phys. Rev. A 2014, 90, 053808. [Google Scholar] [CrossRef] [Green Version]
- Bemani, F.; Motazedifard, A.; Roknizadeh, R.; Naderi, M.H.; Vitali, D. Synchronization dynamics of two nanomechanical membranes within a Fabry-Perot cavity. Phys. Rev. A 2017, 96, 023805. [Google Scholar] [CrossRef] [Green Version]
- Xuereb, A.; Genes, C.; Pupillo, G.; Paternostro, M.; Dantan, A. Reconfigurable Long-Range Phonon Dynamics in Optomechanical Arrays. Phys. Rev. Lett. 2014, 112, 133604. [Google Scholar] [CrossRef] [PubMed]
- Xuereb, A.; Imparato, A.; Dantan, A. Heat transport in harmonic oscillator systems with thermal baths: application to optomechanical arrays. N. J. Phys. 2015, 17, 055013. [Google Scholar] [CrossRef] [Green Version]
- Bhattacharya, M.; Meystre, P. Multiple membrane cavity optomechanics. Phys. Rev. A 2008, 78, 041801. [Google Scholar] [CrossRef] [Green Version]
- Hartmann, M.J.; Plenio, M.B. Steady State Entanglement in the Mechanical Vibrations of Two Dielectric Membranes. Phys. Rev. Lett. 2008, 101, 200503. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Nielsen, W.H.P.; Tsaturyan, Y.; Møller, C.B.; Polzik, E.S.; Schliesser, A. Multimode optomechanical system in the quantum regime. Proc. Natl. Acad. Sci. USA 2017, 114, 62. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Patil, Y.S.; Chakram, S.; Chang, L.; Vengalattore, M. Thermomechanical Two-Mode Squeezing in an Ultrahigh-Q Membrane Resonator. Phys. Rev. Lett. 2015, 115, 017202. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Pontin, A.; Bonaldi, M.; Borrielli, A.; Marconi, L.; Marino, F.; Pandraud, G.; Prodi, G.A.; Sarro, P.M.; Serra, E.; Marin, F. Dynamical Two-Mode Squeezing of Thermal Fluctuations in a Cavity Optomechanical System. Phys. Rev. Lett. 2016, 116, 103601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wu, S.; Sheng, J.; Zhang, X.; Wu, Y.; Wu, H. Parametric excitation of a SiN membrane via piezoelectricity. AIP Adv. 2018, 8, 015209. [Google Scholar] [CrossRef]
- Naserbakht, S.; Naesby, A.; Dantan, A. Electromechanics in vertically coupled nanomembranes. Appl. Phys. Lett. 2019, 115, 061105. [Google Scholar] [CrossRef] [Green Version]
- Naesby, A.; Naserbakht, S.; Dantan, A. Effects of pressure on suspended micromechanical membrane arrays. Appl. Phys. Lett. 2017, 111, 201103. [Google Scholar] [CrossRef] [Green Version]
- Naserbakht, S.; Dantan, A. Squeeze film pressure sensors based on SiN membrane sandwiches. Sens. Actuators A Phys. 2019, 298, 111588. [Google Scholar] [CrossRef] [Green Version]
- Rugar, D.; Grütter, P. Mechanical parametric amplification and thermomechanical noise squeezing. Phys. Rev. Lett. 1991, 67, 699–702. [Google Scholar] [CrossRef] [PubMed]
- Midolo, L.; Schliesser, A.; Fiore, A. Nano-opto-electro-mechanical systems. Nat. Nanotechnol. 2018, 13, 11–18. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Turner, K.L.; Miller, S.A.; Hartwell, P.G.; MacDonald, N.C.; Strogatz, S.H.; Adams, S.G. Five parametric resonances in a microelectromechanical system. Nature 1998, 396, 149–152. [Google Scholar] [CrossRef]
- Carr, D.W.; Evoy, S.; Sekaric, L.; Craighead, H.G.; Parpia, J.M. Parametric amplification in a torsional microresonator. Appl. Phys. Lett. 2000, 77, 1545–1547. [Google Scholar] [CrossRef] [Green Version]
- Mahboob, I.; Yamaguchi, H. Piezoelectrically pumped parametric amplification and Q enhancement in an electromechanical oscillator. Appl. Phys. Lett. 2008, 92, 173109. [Google Scholar] [CrossRef]
- Karabalin, R.B.; Masmanidis, S.C.; Roukes, M.L. Efficient parametric amplification in high and very high frequency piezoelectric nanoelectromechanical systems. Appl. Phys. Lett. 2010, 97, 183101. [Google Scholar] [CrossRef] [Green Version]
- Seitner, M.J.; Abdi, M.; Ridolfo, A.; Hartmann, M.J.; Weig, E.M. Parametric Oscillation, Frequency Mixing, and Injection Locking of Strongly Coupled Nanomechanical Resonator Modes. Phys. Rev. Lett. 2017, 118, 254301. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Huber, J.S.; Rastelli, G.; Seitner, M.; Maximilian, J.; Köbl, J.; Belzig, W.; Dykman, M.I.; Weig, E.M. Squeezing of thermal fluctuations in a driven nanomechanical resonator. arXiv 2019, arXiv:1903.07601. [Google Scholar]
- Bothner, D.; Yanai, S.; Iniguez-Rabago, A.; Yuan, M.; Blanter, Y.M.; Steele, G.A. Cavity electromechanics with parametric mechanical driving. arXiv 2019, arXiv:1908.08496. [Google Scholar]
- Szorkovszky, A.; Doherty, A.C.; Harris, G.I.; Bowen, W.P. Mechanical Squeezing via Parametric Amplification and Weak Measurement. Phys. Rev. Lett. 2011, 107, 213603. [Google Scholar] [CrossRef] [PubMed]
- Farace, A.; Giovannetti, V. Enhancing quantum effects via periodic modulations in optomechanical systems. Phys. Rev. A 2012, 86, 013820. [Google Scholar] [CrossRef] [Green Version]
- Szorkovszky, A.; Brawley, G.A.; Doherty, A.C.; Bowen, W.P. Strong Thermomechanical Squeezing via Weak Measurement. Phys. Rev. Lett. 2013, 110, 184301. [Google Scholar] [CrossRef] [PubMed]
- Lemonde, M.A.; Didier, N.; Clerk, A.A. Enhanced nonlinear interactions in quantum optomechanics via mechanical amplification. Nat. Commun. 2016, 7, 11338. [Google Scholar] [CrossRef] [PubMed]
- Levitan, B.A.; Metelmann, A.; Clerk, A.A. Optomechanics with two-phonon driving. N. J. Phys. 2016, 18, 093014. [Google Scholar] [CrossRef]
- Norcada Inc. Available online: www.norcada.com (accessed on 12 November 2019).
- Naesby, A.; Dantan, A. Microcavities with suspended subwavelength structured mirrors. Opt. Express 2018, 26, 29886–29894. [Google Scholar] [CrossRef] [PubMed] [Green Version]

© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Naserbakht, S.; Naesby, A.; Dantan, A. Stress-Controlled Frequency Tuning and Parametric Amplification of the Vibrations of Coupled Nanomembranes. Appl. Sci. 2019, 9, 4845. https://doi.org/10.3390/app9224845
Naserbakht S, Naesby A, Dantan A. Stress-Controlled Frequency Tuning and Parametric Amplification of the Vibrations of Coupled Nanomembranes. Applied Sciences. 2019; 9(22):4845. https://doi.org/10.3390/app9224845
Chicago/Turabian StyleNaserbakht, Sepideh, Andreas Naesby, and Aurélien Dantan. 2019. "Stress-Controlled Frequency Tuning and Parametric Amplification of the Vibrations of Coupled Nanomembranes" Applied Sciences 9, no. 22: 4845. https://doi.org/10.3390/app9224845
APA StyleNaserbakht, S., Naesby, A., & Dantan, A. (2019). Stress-Controlled Frequency Tuning and Parametric Amplification of the Vibrations of Coupled Nanomembranes. Applied Sciences, 9(22), 4845. https://doi.org/10.3390/app9224845