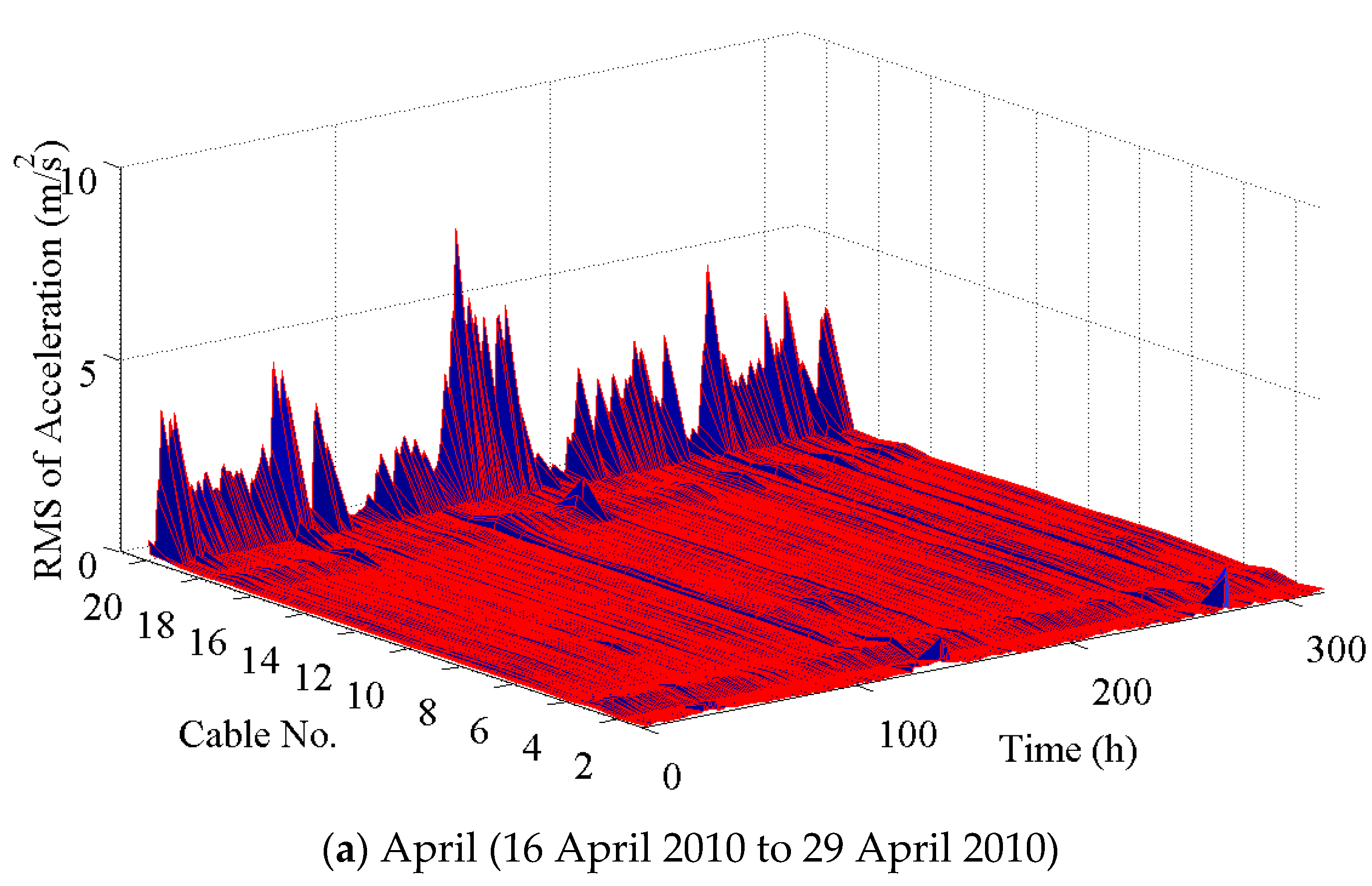
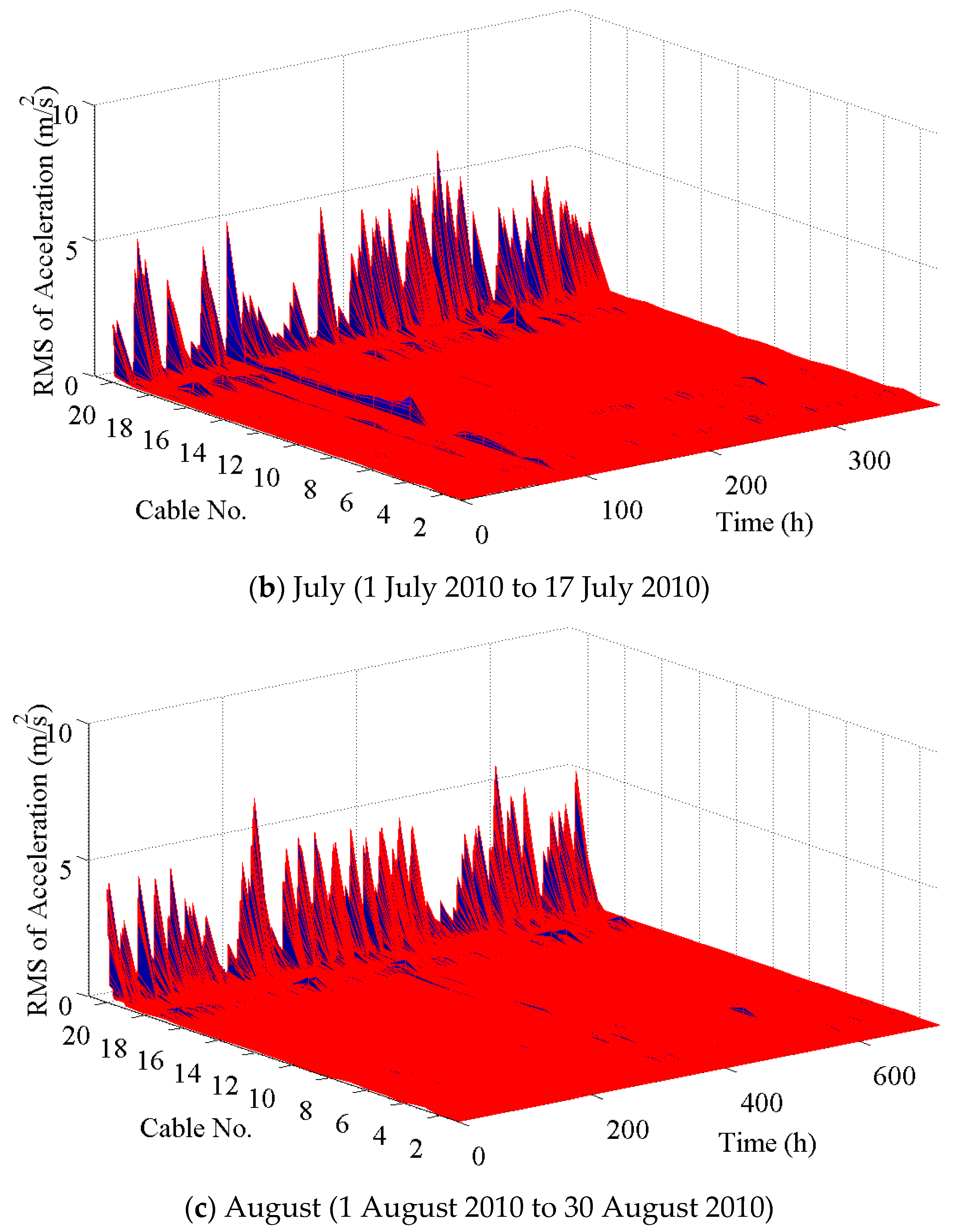
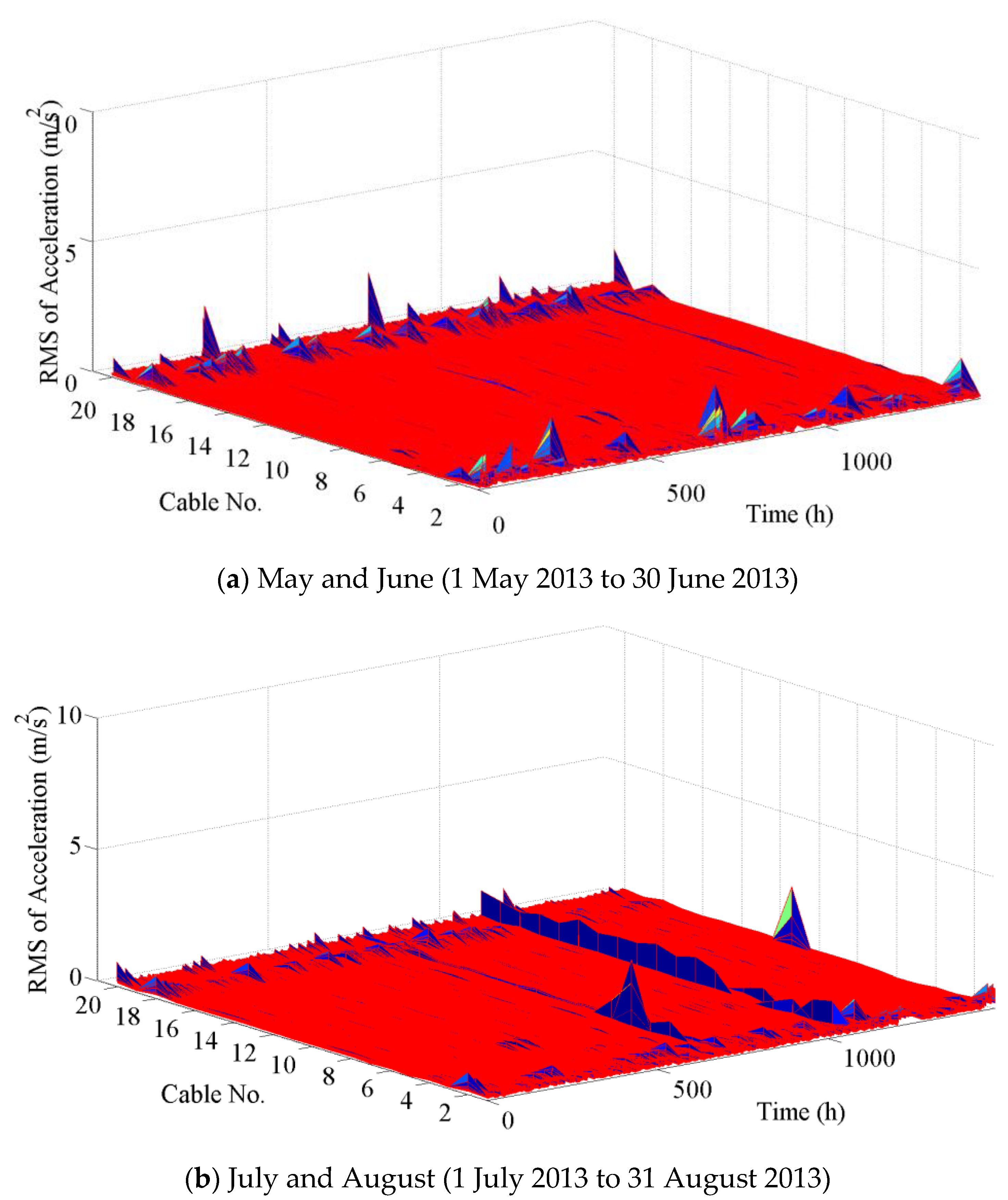
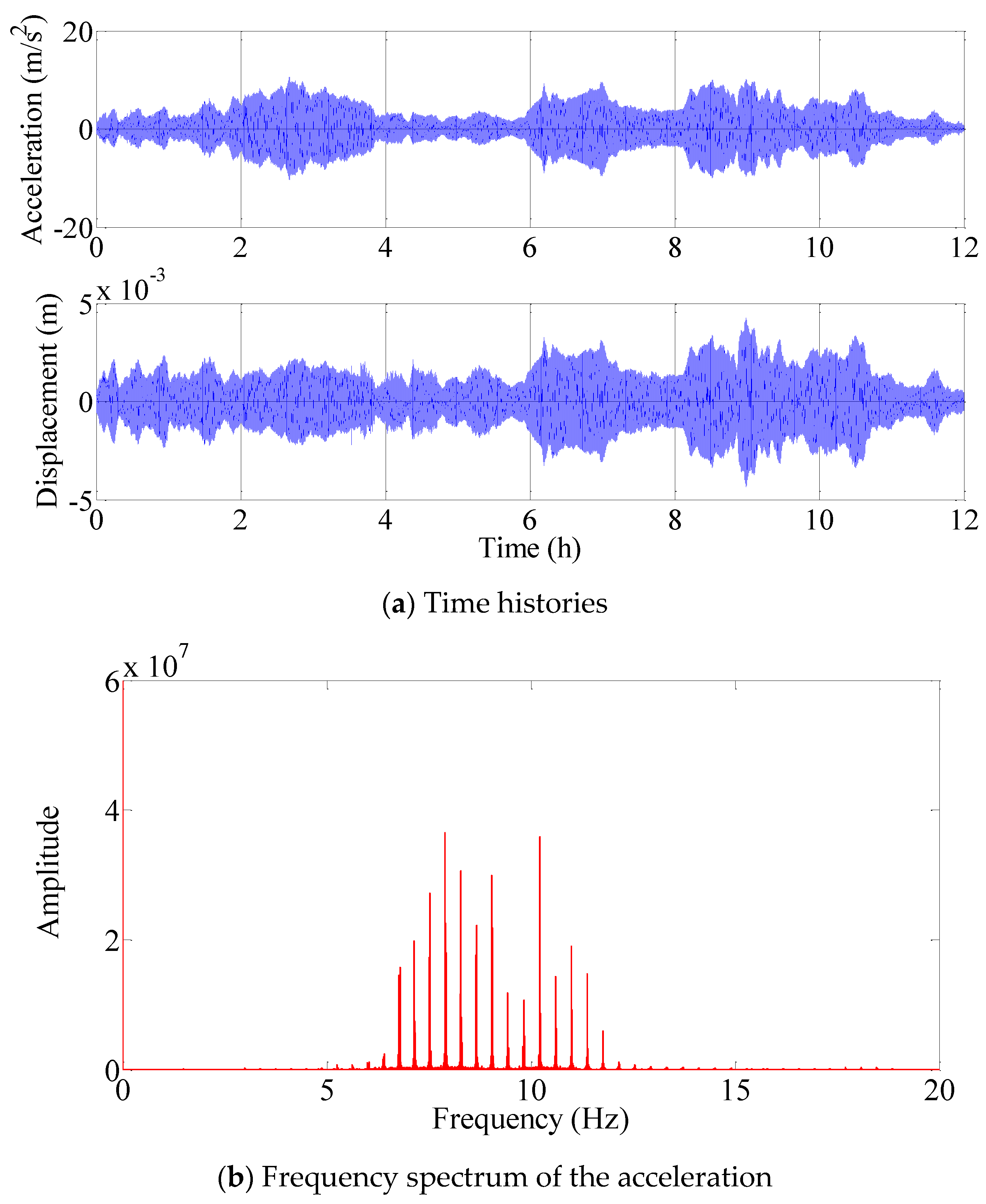
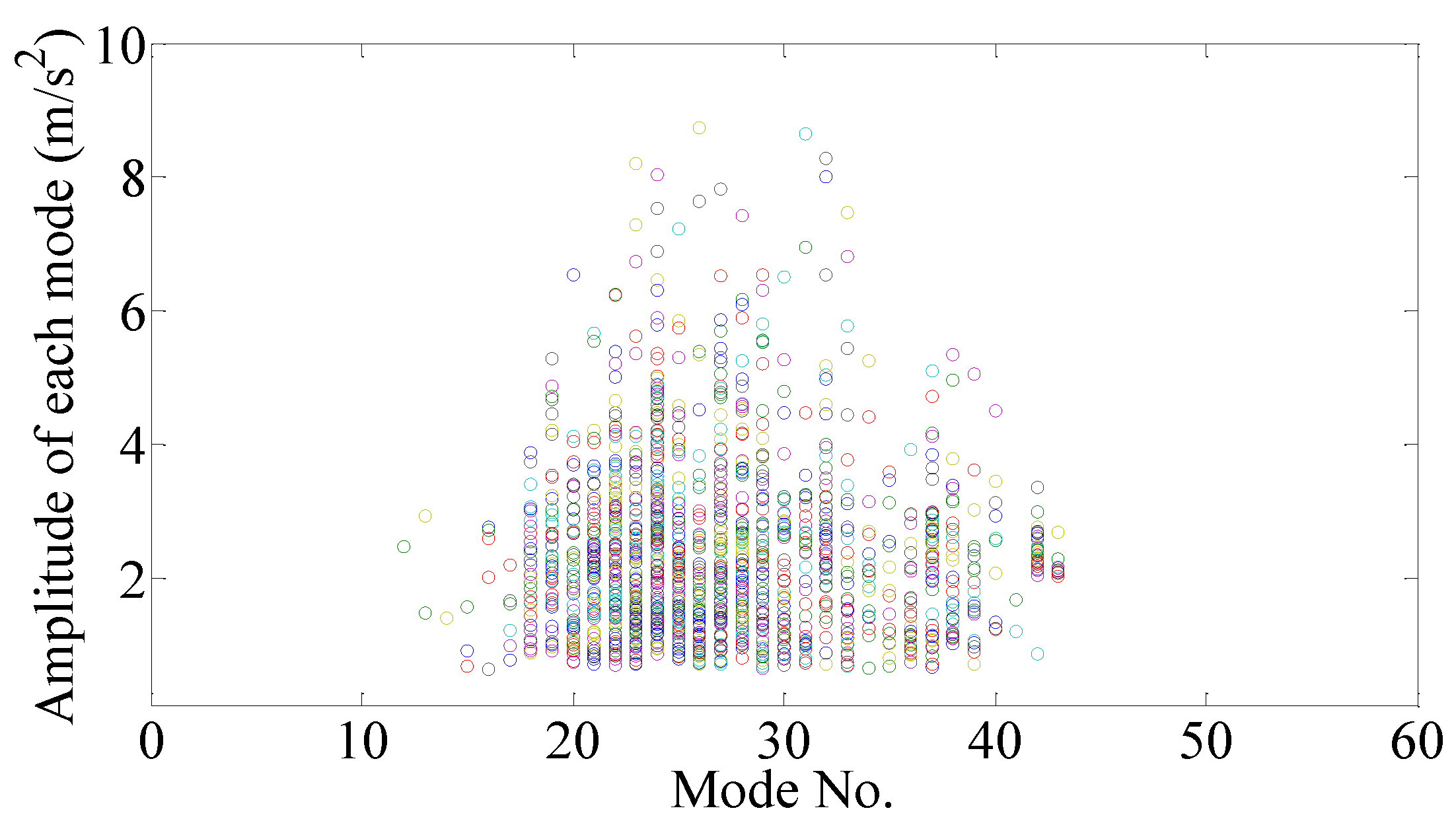
4.1. Vibration Amplitude Versus Wind Characteristics
The wind data of two anemometers installed on the upstream and downstream sides of the mid-span section of the deck were collected to investigate the wind characteristics and their correlation with the cable response. The wind data of two anemometers are denoted as “incoming flow” and “wake flow”, respectively. It should be noted that “incoming flow” and “wake flow” do not absolutely indicate the inflow and wake flow and that they are only used to distinguish the wind data measured by the two anemometers.
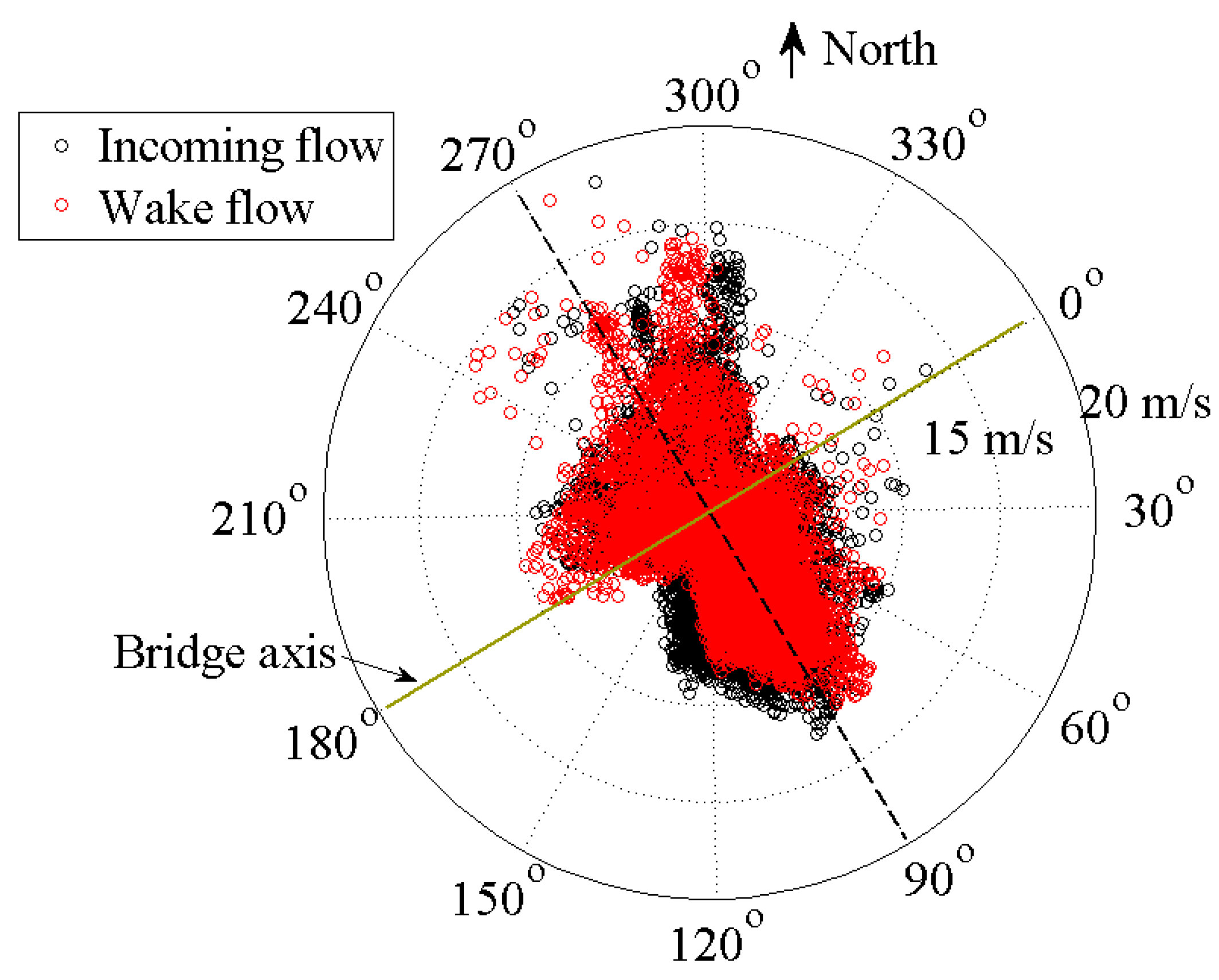
Figure 7 shows 10 min mean wind speeds and directions of incoming and wake flows in April, July, and August, measured by the two anemometers, respectively. It is found that the data measured by two anemometers are close to each other for most of the samples, and the 10 min mean wind speeds are almost less than 15 m/s, with only several points larger than 15 m/s. The wind direction between the flow and the longitudinal bridge axis shows that the wind is nearly perpendicular to the bridge axis when the wind blows from the south.
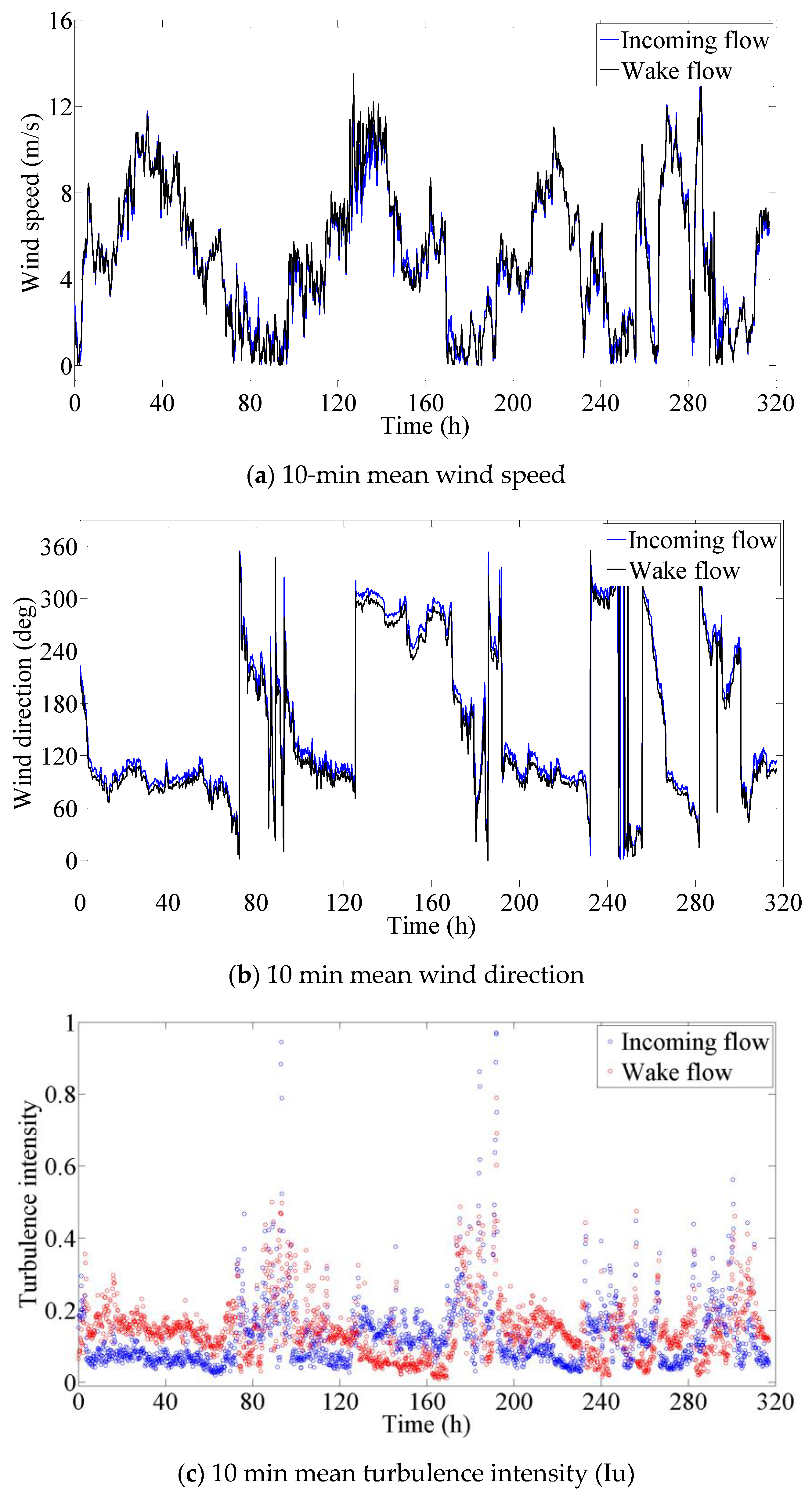
Figure 8 shows the time histories of 10 min mean wind speeds, directions, and turbulence intensities of incoming and wake flows measured by two anemometers in April (16 April 2010 to 29 April 2010). The 10 min mean wind speeds are primarily located in the range of 3 to 12 m/s, as shown in
Figure 8a. It was found that, while 10 min mean wind speed histories are close to each other, there is a smaller phase difference of the wind direction between the incoming flow and wake flow, as shown in
Figure 8b. The largest difference between two anemometers happens in the turbulence intensity (Iu) of the stream-wise direction, as shown in
Figure 8c, because one anemometer is located in the incoming flow and the other is inevitably in the wake flow when the flow is nearly perpendicular to the bridge axis. The turbulence intensity in the incoming flow is certainly less than that in the wake flow. The larger numerical values of the turbulence intensities measured by two anemometers alternately change with time. Whatever the incoming flow or wake flow, the turbulence intensities are mainly less than 0.2. The large value of the turbulence intensity is mainly induced by a small mean wind speed, as shown in
Figure 8a.
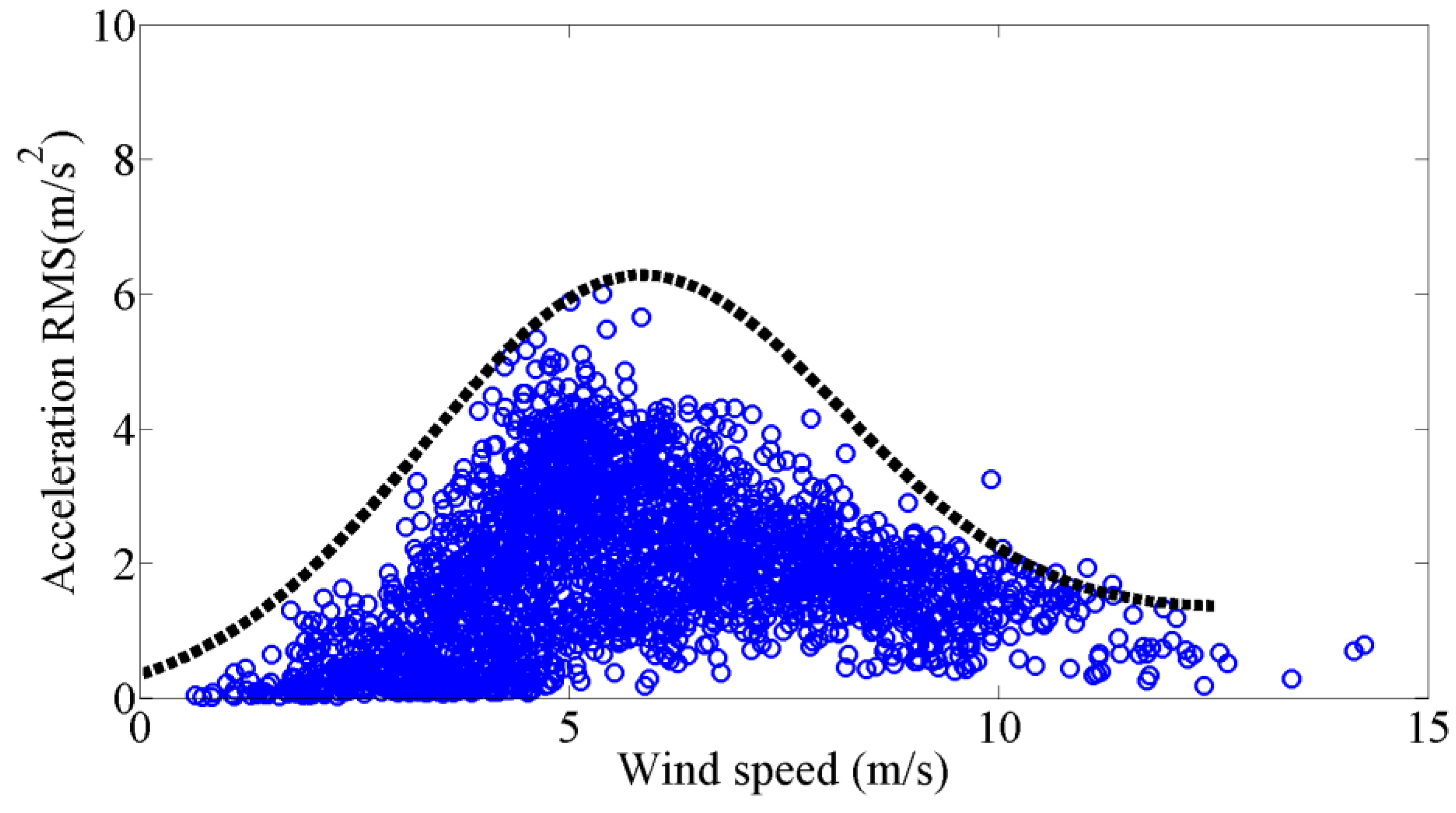
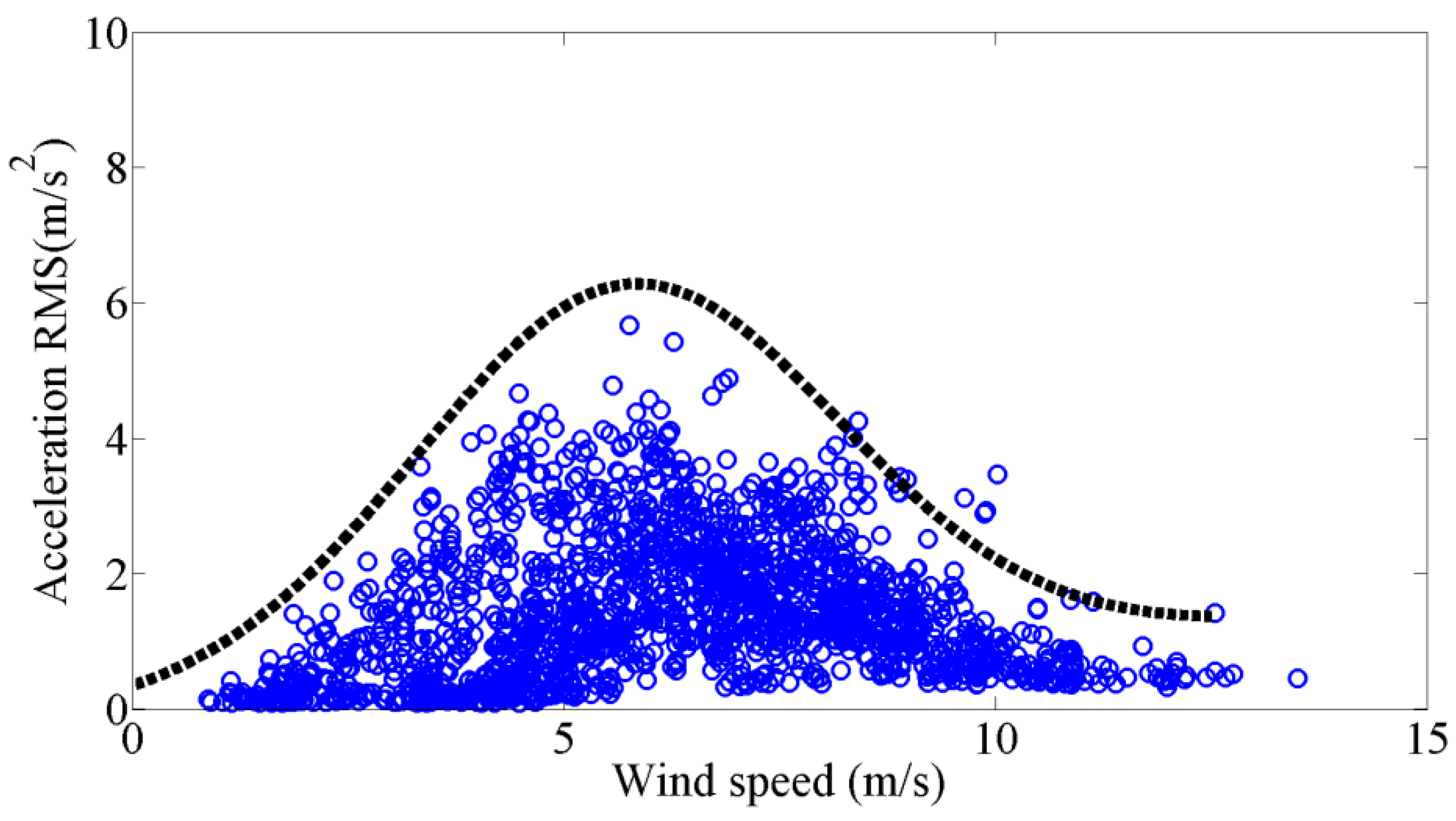
Figure 9 shows the relationship between the acceleration RMSs of 10 min for the cable CAC20 and the corresponding 10 min mean wind speed in three months of April, July, and August. It is found that the vibration amplitude initially increases with the mean wind speed, reaches the largest amplitude at the mean wind speed of approximately 4 to 6 m/s, and then decreases with the mean wind speed. The large amplitude is mainly located in the range of 3 to 12 m/s.
According to the characteristics of the distribution of the vibration amplitude with the mean wind speed, the relationship between the maximum vibration amplitude and the mean wind speed can be fitted and denoted by the following form of expression:
where
is the mean wind speed,
is fitted to be 0.1,
is 5.75 m/s,
is 0.07 m/s, and the factor
is 1200. The first term of the right side of Equation (1) is a linear function, which means the forced vibration component increases with the mean wind speed. The second term of the right side is a modified function of normal distribution, which has a factor of
to meet the statistical samples. The additional data of September and October of 2010 was employed to validate the presented relationship between the vibration amplitude and the mean wind speed, as shown in
Figure 10. It is suggested that the maximum vibration amplitude at different mean wind speeds can be reflected by the presented relationship.
To reveal the correlation between the cable vibration and the velocity, the mean wind speed was often changed into the reduced velocity, i.e.,
, where
is the
nth modal frequency and
is the diameter of the cable. As a multimode vibration in the present study, the reduced velocity
was calculated based on the dominant mode frequency that had the maximum vibration amplitude in all vibration participative modes.
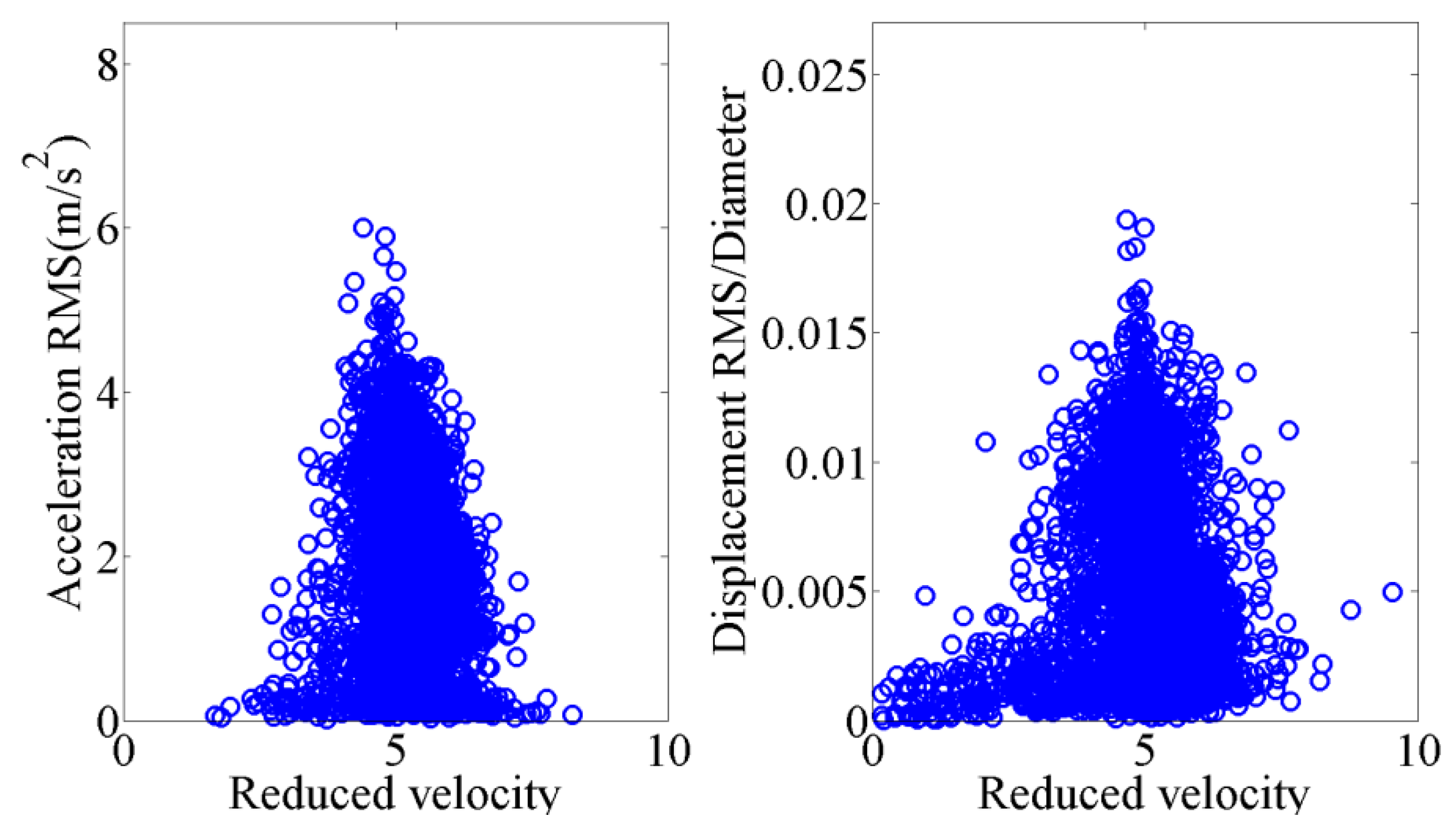
Figure 11a shows the correlation of the 10 min acceleration RMSs and the corresponding 10 min mean reduced velocity. Next, the relationship of the dimensionless vibration amplitude with the reduced velocity is obtained, as shown in
Figure 11b. The results indicate that the large vibration amplitudes are mainly located in the region near the reduced velocity of five. This phenomenon is similar to the feature of a vortex-induced vibration of a stayed cable observed by Zuo and Jones [
6].
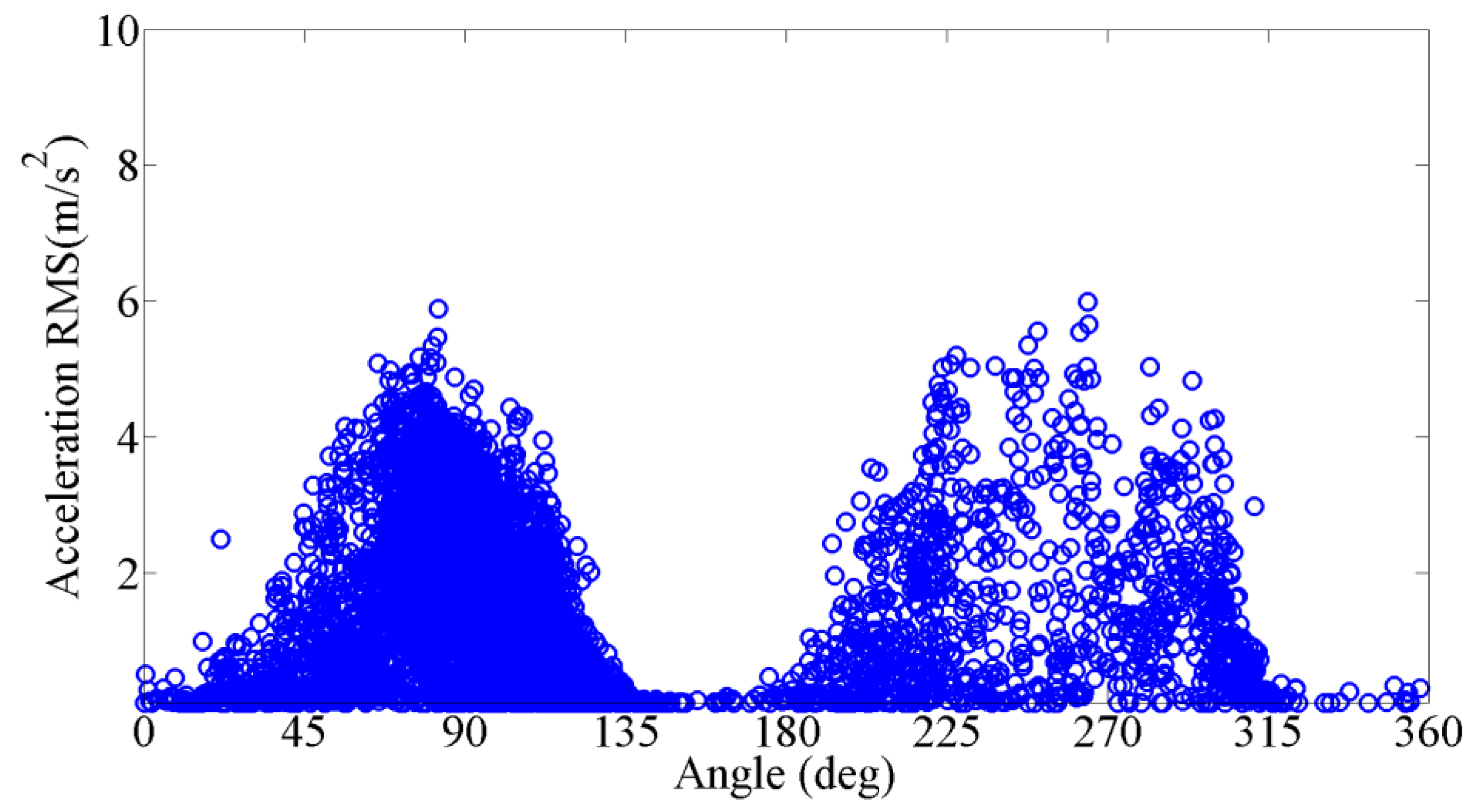
Figure 12 shows the relationship of the RMS of the acceleration of the CAC20 cable and the corresponding mean wind direction in April, July, and August. It is found that the large amplitudes mainly happen at the wind direction nearly perpendicular to the bridge. The large amplitudes are more concentrated, with a wind direction near 90 degrees (deg), and are distributed wider when the wind direction nears 270 degrees. The reason is believed to be that the wind direction is mainly concentrated in the direction perpendicular to the bridge axis when the wind blows from the south; and the wind direction covers more angles when the wind comes from the north, as shown in
Figure 7.
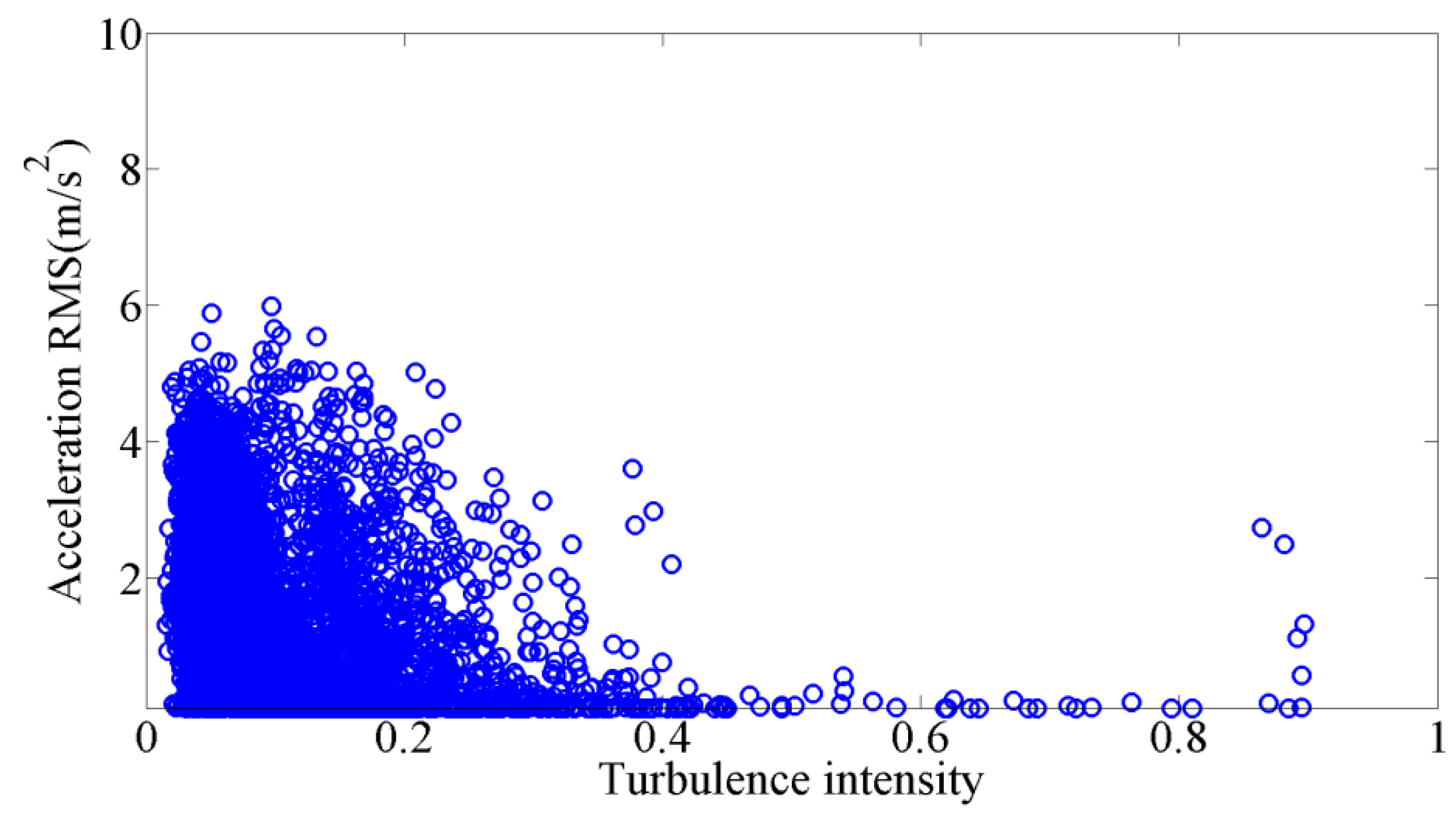
Figure 13 shows the relationship of the RMS of the acceleration of the CAC20 cable and the corresponding turbulence intensity in three months. The large vibration amplitudes mainly occur when the turbulence intensity is less than 20%.
4.2. Estimation Method of the Participative Modes of VIVs
The mean wind speed profile acting on the stay cables was not uniform, because the investigated cable-stayed bridge was constructed in the atmospheric boundary layer. According to the log law, the mean wind speed profile in the atmospheric boundary layer can be defined as the following:
where
is the reference height above the ground,
is the arbitrary height above the ground,
is the mean wind speed at the height
,
is the mean wind speed at the height,
, and
is the terrain roughness index, equal to 0.12, 0.16, 0.22, and 0.30 for A, B, C, and D terrains, respectively, according to the Chinese Standard GB 5009-2001 (National Standard of the People’s Republic of China, 2001 version, see [
16]).
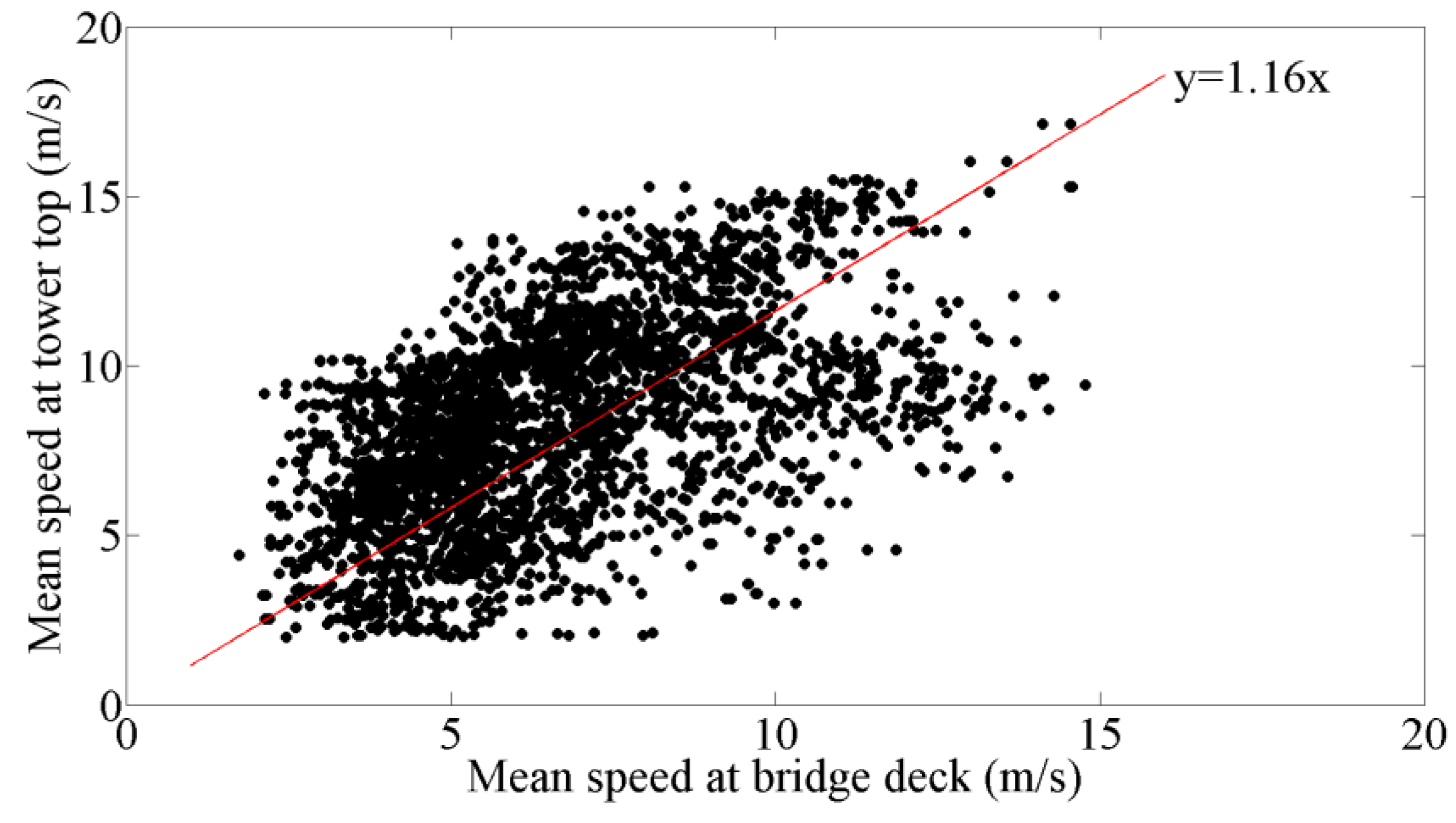
According to the wind data measured by the anemometers installed at the tower top and bridge deck, the correlation of the mean wind speeds between the tower top and deck level of the bridge was obtained, as shown in
Figure 14. The mean wind speed on the tower top is nearly 1.16 times that on the bridge deck level, according to the fitting line.
In the present study, the height of the deck level is 59.0 m above the ground, and the corresponding height of the reference height (i.e., the deck anemometer installing height) is 65.0 m above the ground. The height of the tower top is 151.0 m above the bridge deck, and the corresponding height, , of the anemometer of the tower top is 212.1 m, with the anemometer 2.1 m above the tower top. According to Equation (2), the terrain roughness index, , can be calculated as 0.125, which is close to 0.12 because the investigated bridge is constructed near the sea; therefore, the terrain of the bridge is close to a type of A terrain. Next, according to by the anemometer at the deck level, the mean wind speed at an arbitrary height can be calculated by Equation (2).
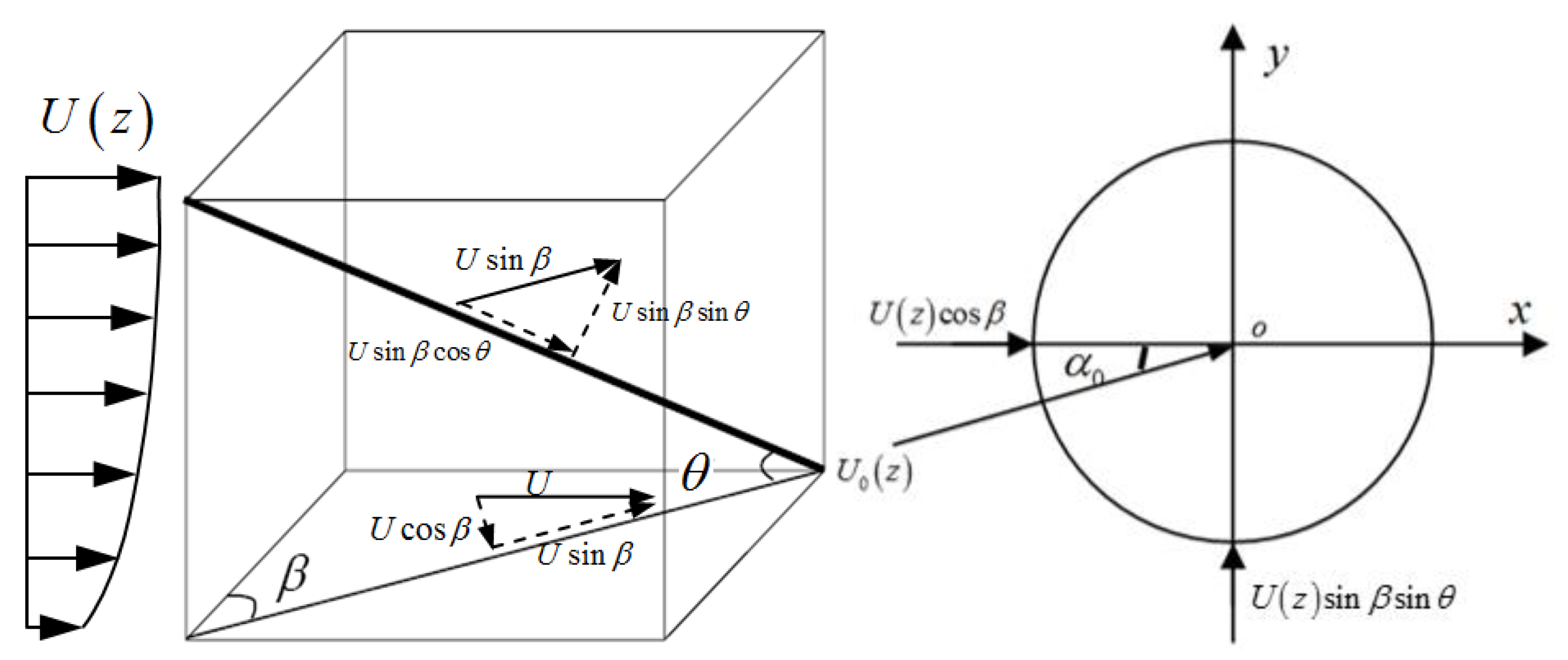
Actually, the wind is not perpendicularly acting on the stay cable plane, as shown in
Figure 15, and the yaw angle of the stay cable plane is
. The inclination angle of the stay cable is
. The incoming velocity is decomposed into two components that are perpendicular to the cable spanwise direction:
and
. The axial flow component
is neglected in the present study. The relative velocity
and the attack of angle
are subsequently obtained, as follows:
Because the Reynolds number varies at different heights of the wind velocity profile, the Strouhal number,
, can be estimated by using the following formula [
17]:
where,
is the cable diameter at the height of z (in this study, it is constant along the cables),
is the Reynolds number depending on z,
is the coefficient of kinematic viscosity, and
is the incoming relative velocity.
The vortex-shedding frequency,
, of a long cable can be expressed as the following:
According to the velocities at the upper and lower ends of the stay cable, the vortex-shedding frequencies of two ends of the stay cable, and , can be calculated from Equation (6). For a long flexible stay cable of a real cable-stayed bridge, high modal frequencies are usually the multiple of its natural frequency. If the fundamental natural frequency is known, the participative modes of VIVs can be estimated from the vortex-shedding-frequency range, [, ].
4.3. Comparison of the Estimated Participative Modes with the Measured Vibration Modes of VIVs
The data from April 2010 were first employed to estimate the participative modes of VIVs, and the estimated participative modes were subsequently compared with the measured vibration modes of VIVs. The preprocessing of the data was performed in advance, and the data with the acceleration amplitude of less than 2 m/s
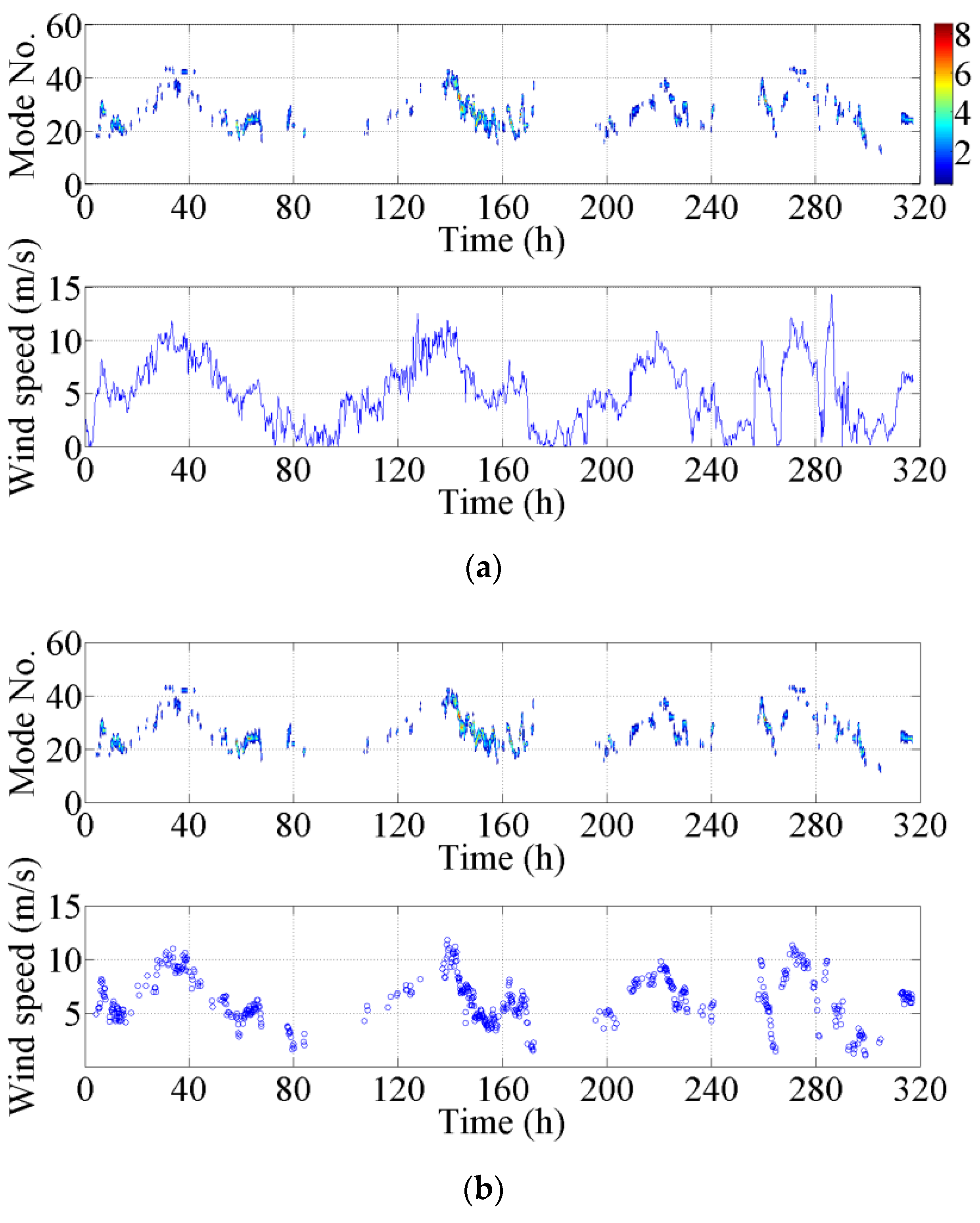
2 were deleted first. The time history of the measured vibration modes was shown in the upper sub-figure of
Figure 16. The colors indicate the participative mode numbers. The lower sub-figure of
Figure 16a is the time history of the mean wind speed measured by only one anemometer at the bridge deck level, because the mean wind speeds are close to each other for two anemometers, as shown in
Figure 8a.
Figure 16a shows that the mean wind speeds corresponding to the acceleration amplitudes less than 2 m/s
2 are notably small. These parts of the mean wind speeds are subsequently deleted, as shown in
Figure 16b. It is determined that the shapes of the time histories of the measured vibration modes and the mean wind speeds are similar to each other. The magnitude of the vibration mode increases or decreases with the mean wind speed.
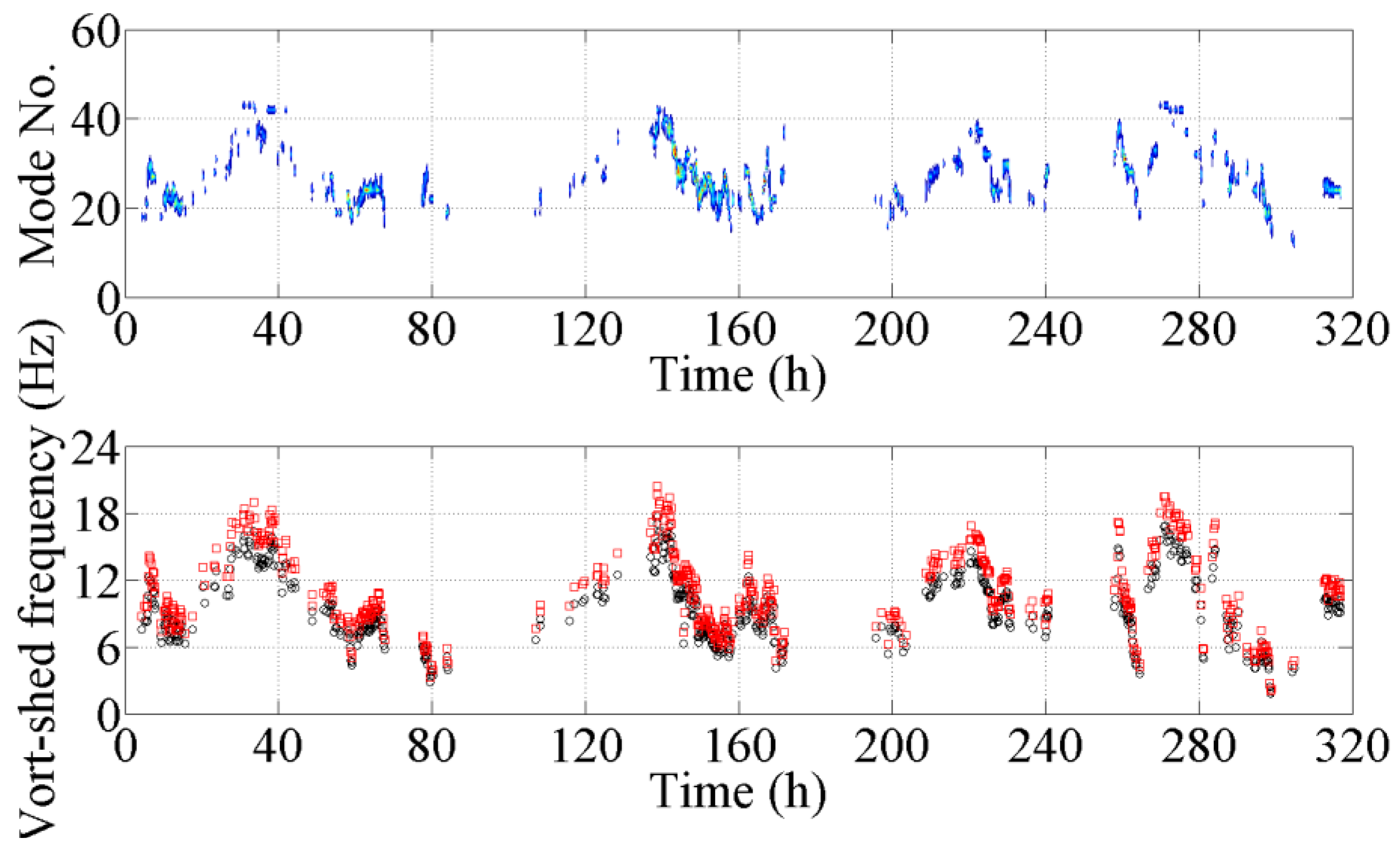
According the present estimation method for the participative modes, the lower and upper ends of the velocities were first obtained according to Equation (2) and the lower sub-figure of
Figure 16b. Then, the vortex-shedding-frequency range, [
,
], was calculated by using Equation (6), as shown in
Figure 17. Similar to the results in
Figure 16, the vortex-shedding-frequency range changing with time has a similar trend to that of the measured vibration modes, varying with time.
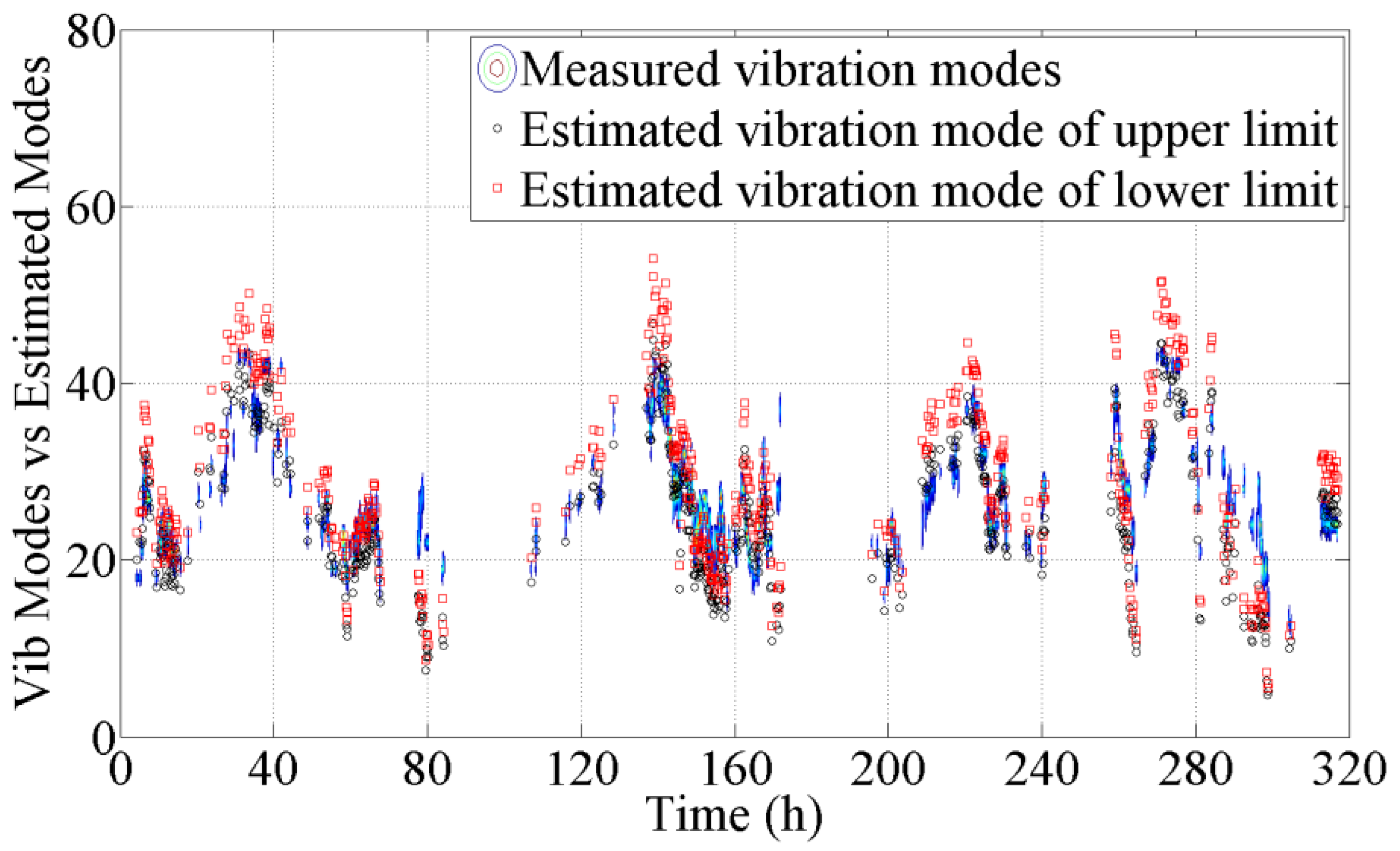
After they were combined with the fundamental natural frequency of the cable CAC20, the estimated participative vibration modes were then calculated and compared with the measured vibration modes in April, as shown in
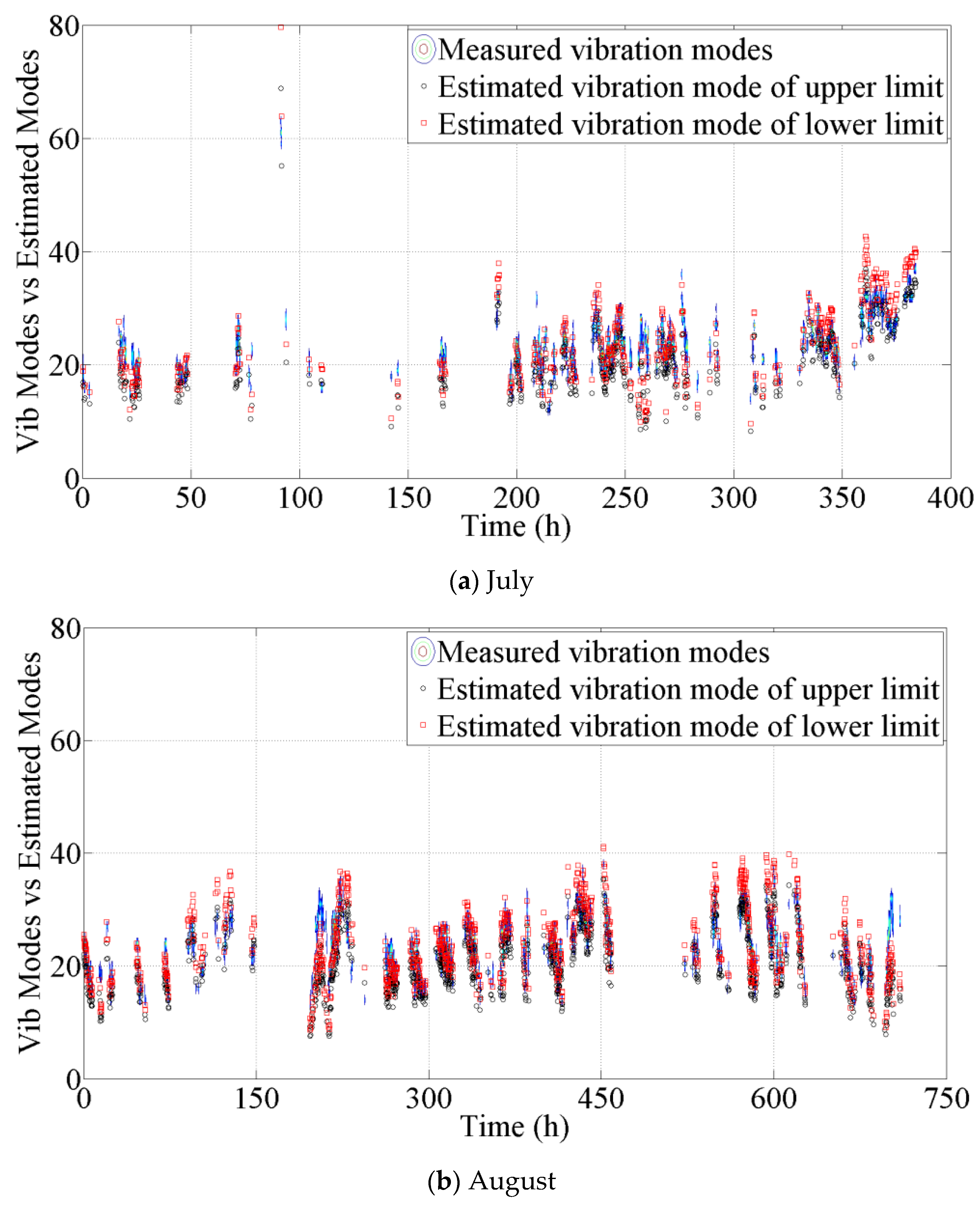
Figure 18. The results indicate that the estimated vibrations are close to the measured vibration modes. Simultaneously, the presented method was validated by the data in July and August, and the same conclusion can be obtained from the comparison shown in
Figure 19. Therefore, the results finally denote that the monitoring vibration of the stay cable is a VIV. The validity and reliability of the present estimated method for the participative vibration modes of VIVs are also approved by the above results.