Plasmonic Jackiw-Rebbi Modes in Graphene Waveguide Arrays
Abstract
1. Introduction
2. Materials and Methods
2.1. Coupled-Mode Equations
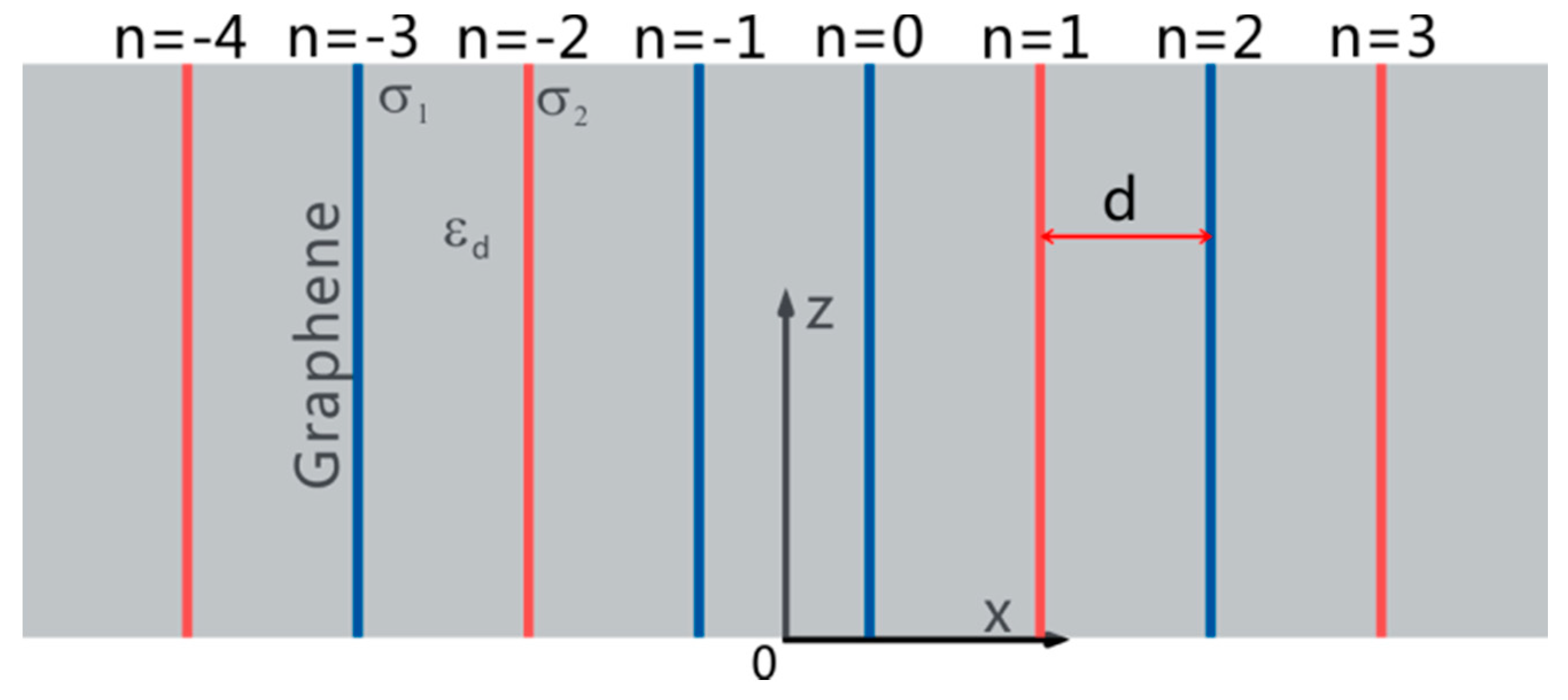
2.2. Graphene Waveguide Arrays
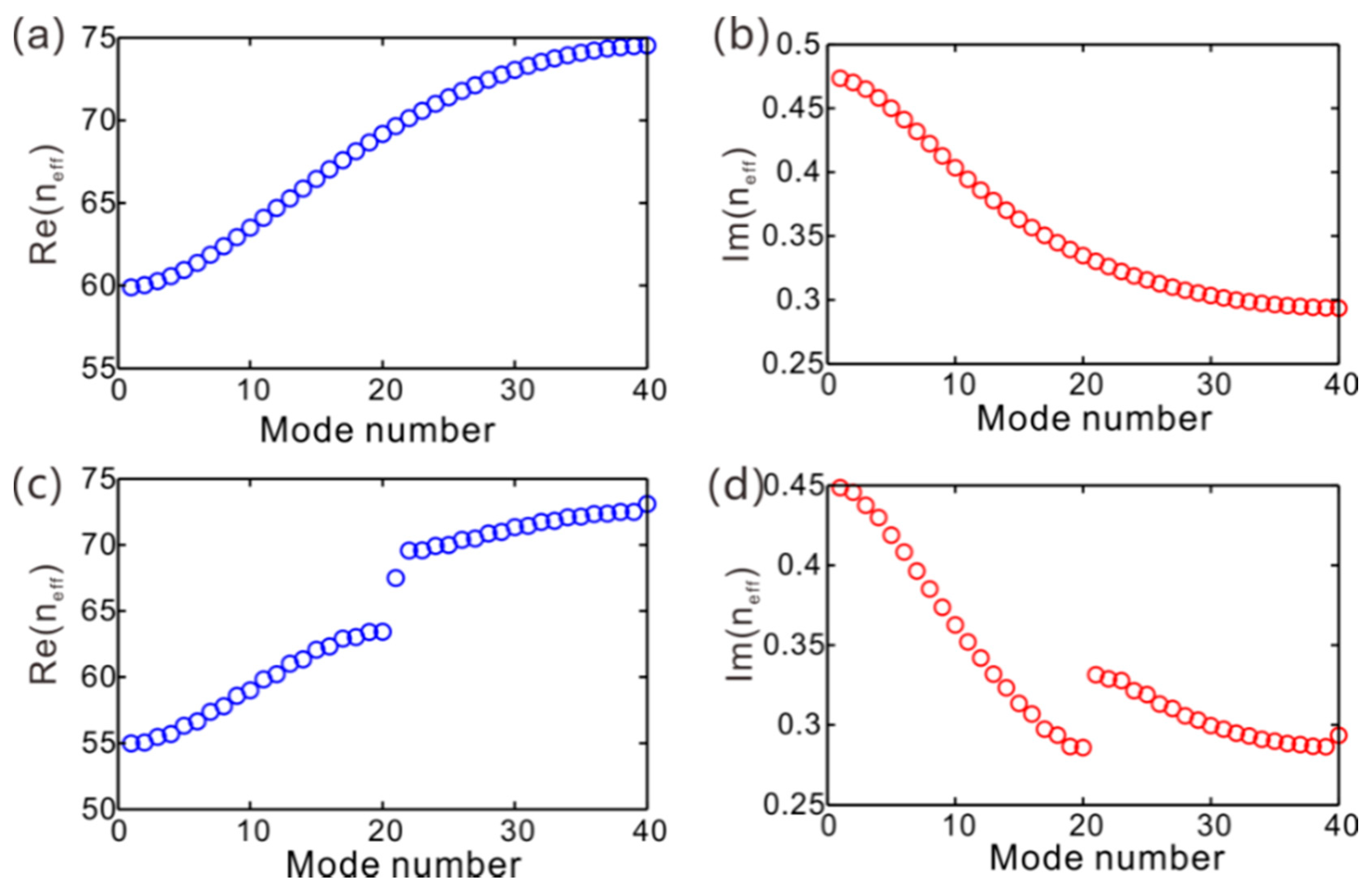
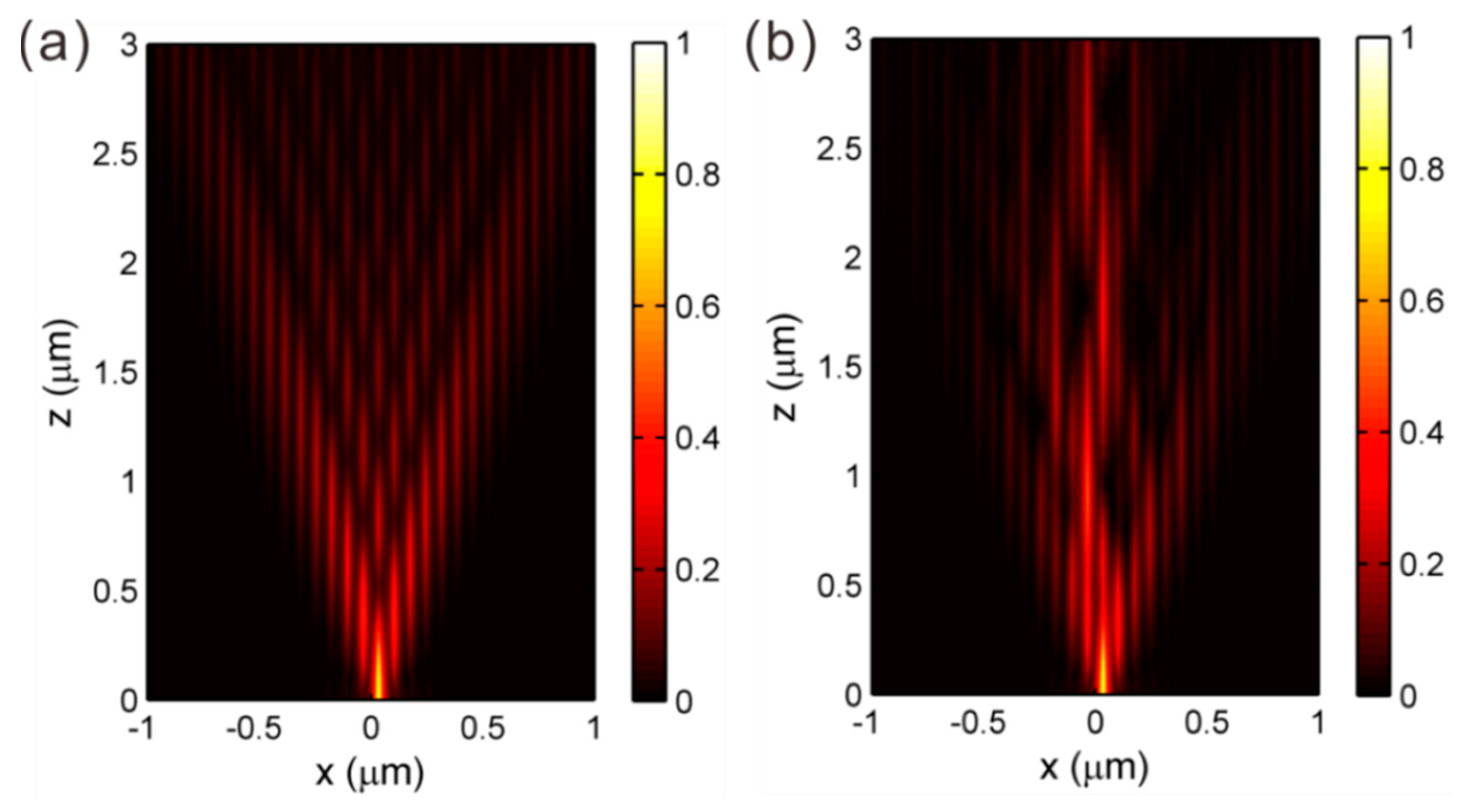
3. Results
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Maier, S.A. Plasmonics: Fundamentals and Applications; Springer: Berlin, Germany, 2007. [Google Scholar]
- Ding, F.; Bozhevolnyi, S.I. A Review of Unidirectional Surface Plasmon Polariton Metacouplers. IEEE J. Sel. Top. Quantum Electron. 2019, 25, 4600611. [Google Scholar] [CrossRef]
- Ke, S.; Liu, J.; Liu, Q.; Zhao, D.; Liu, W. Strong absorption near exceptional points in plasmonic waveguide arrays. Opt. Quantum Electron. 2018, 50, 318. [Google Scholar] [CrossRef]
- Ding, F.; Deshpande, R.; Bozhevolnyi, S.I. Bifunctional Gap-Plasmon Metasurfaces for Visible Light: Polarization-Controlled Unidirectional Surface Plasmon Excitation and Beam Steering at Normal Incidence. Light Sci. Appl. 2018, 7, 17178. [Google Scholar] [CrossRef] [PubMed]
- Bao, Q.; Loh, K.P. Graphene photonics, plasmonics, and broadband optoelectronic devices. ACS Nano 2012, 6, 3677–3694. [Google Scholar] [CrossRef] [PubMed]
- Chen, P.Y.; Al, A. Atomically thin surface cloak using graphene monolayers. ACS Nano 2011, 5, 5855–5863. [Google Scholar] [CrossRef] [PubMed]
- Huang, H.; Ke, S.; Wang, B.; Long, H.; Wang, K.; Lu, P. Numerical study on plasmonic absorption enhancement by a rippled graphene sheet. J. Lightwave Technol. 2017, 35, 320–324. [Google Scholar] [CrossRef]
- Deng, H.; Ye, F.; Malomed, B.A.; Chen, X.; Panoiu, N.C. Optically and electrically tunable Dirac points and Zitterbewegung in graphene-based photonic superlattices. Phys. Rev. B 2015, 91, 201402. [Google Scholar] [CrossRef]
- Liu, J.X.; Park, S.; Nowak, D.; Tian, M.C.; Wu, Y.; Long, H.; Wang, K.; Wang, B.; Lu, P. Near-Field Characterization of Graphene Plasmons by Photo-Induced Force Microscopy. Laser Photonics Rev. 2018, 12, 1800040. [Google Scholar] [CrossRef]
- Ke, S.; Zhao, D.; Liu, Q.; Liu, W. Adiabatic transfer of surface plasmons in non-Hermitian graphene waveguides. Opt. Quantum Electron. 2018, 50, 393. [Google Scholar] [CrossRef]
- Salunkhe, R.R.; Lee, Y.H.; Chang, K.H.; Li, J.M.; Simon, P.; Tang, J.; Torad, N.L.; Hu, C.C.; Yamauchi, Y. Nanoarchitectured graphene-based supercapacitors for next-generation energy-storage applications. Chem. Eur. J. 2014, 20, 13838–13852. [Google Scholar] [CrossRef]
- Tang, J.; Yamauchi, Y. MOF morphologies in control. Nat. Chem. 2016, 8, 638–639. [Google Scholar] [CrossRef] [PubMed]
- Salunkhe, R.R.; Hsu, S.; Wu, K.C.W.; Yamauchi, Y. Large-Scale Synthesis of Reduced Graphene Oxides with.Uniformly Coated Polyaniline for Supercapacitor Applications. ChemSusChem 2014, 7, 1551–1556. [Google Scholar] [CrossRef] [PubMed]
- Tan, H.; Tang, J.; Henzie, J.; Li, Y.; Xu, X.; Chen, T.; Wang, Z.; Wang, J.; Ide, Y.; Bando, Y.; et al. Assembly of Hollow Carbon Nanospheres on Graphene Nanosheets and Creation of Iron−Nitrogen-Doped Porous Carbon for Oxygen Reduction. ACS Nano 2018, 12, 5674–5683. [Google Scholar] [CrossRef] [PubMed]
- Ni, G.X.; Wang, L.; Goldflam, M.D.; Wagner, M.; Fe, Z.; McLeo, A.S.; Liu, M.K.; Keilman, F.; Özyilmaz, B.; Castro, A.H.; et al. Ultrafast optical switching of infrared plasmon polaritons in high-mobility graphene. Nat. Photonics 2016, 10, 244–247. [Google Scholar] [CrossRef]
- Li, M.; Xie, H.; Cao, W.; Luo, S.; Tan, J.; Feng, Y.; Du, B.; Zhang, W.; Li, Y.; Zhang, Q.; et al. Photoelectron Holographic Interferometry to Probe the Longitudinal Momentum Offset at the Tunnel Exit. Phys. Rev. Lett. 2019, 122, 183202. [Google Scholar] [CrossRef] [PubMed]
- Tan, J.; Zhou, Y.; He, M.; Chen, Y.; Ke, Q.; Liang, J.; Zhu, X.; Li, M.; Lu, P. Determination of the Ionization Time Using Attosecond Photoelectron Interferometry. Phys. Rev. Lett. 2018, 121, 253203. [Google Scholar] [CrossRef] [PubMed]
- Zhai, C.; Zhang, Y.; Zhang, Q. Characterizing the ellipticity of an isolated attosecond pulse. Opt. Commun. 2019, 437, 104–109. [Google Scholar] [CrossRef]
- Kou, Y.; Förstner, J. Discrete plasmonic solitons in graphene-coated nanowire arrays. Opt. Express 2016, 24, 4714–4721. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Wang, Z.; Wang, B.; Long, H.; Wang, K.; Lu, P. Surface plasmonic lattice solitons in semi-infinite graphene sheet arrays. J. Lightwave Technol. 2017, 35, 2960–2965. [Google Scholar] [CrossRef]
- Zhao, D.; Wang, Z.; Long, H.; Wang, K.; Wang, B.; Lu, P. Optical bistability in defective photonic multilayers doped by graphene. Opt. Quantum Electron. 2017, 49, 163. [Google Scholar] [CrossRef]
- Li, L.; Lan, P.; Zhu, X.; Huang, T.; Zhang, Q.; Lein, M.; Lu, P. Reciprocal-Space-Trajectory Perspective on High-Harmonic Generation in Solids. Phys. Rev. Lett. 2019, 122, 193901. [Google Scholar] [CrossRef] [PubMed]
- Kinyua, D.M.; Niu, L.; Long, H.; Wang, K.; Wang, B. Tuning the photoinduced charge transfer from CdTe quantum dots to ZnO nanofilms through Ga doping. Opt. Mater. 2019, 96, 109311. [Google Scholar] [CrossRef]
- Wang, B.; Zhang, X.; Garcı´a-Vidal, F.J.; Yuan, X.; Teng, J. Strong Coupling of Surface Plasmon Polaritons in Monolayer Graphene Sheet Arrays. Phy. Rev. Lett. 2012, 109, 073901. [Google Scholar] [CrossRef] [PubMed]
- Ke, S.; Wang, B.; Qin, C.; Long, H.; Wang, K.; Lu, P. Exceptional points and asymmetric mode switching in plasmonic waveguides. J. Lightwave Technol. 2016, 34, 5258–5262. [Google Scholar] [CrossRef]
- Ke, S.; Wang, B.; Long, H.; Wang, K.; Lu, P. Topological mode switching in a graphene doublet with exceptional points. Opt. Quantum Electron. 2017, 49, 224. [Google Scholar] [CrossRef]
- Li, J.; Fu, J.; Liao, Q.; Ke, S. Exceptional points in chiral metasurface based on graphene strip arrays. J. Opt. Soc. Am. B 2019, 36, 2492–2498. [Google Scholar] [CrossRef]
- Qin, C.; Wang, B.; Long, H.; Wang, K.; Lu, P. Nonreciprocal phase shift and mode modulation in dynamic graphene waveguides. J. Lightwave Technol. 2016, 34, 3877–3883. [Google Scholar]
- Zhao, D.; Liu, F.; Meng, P.; Wen, J.; Xu, S.; Li, Z.; Zhong, D. Reflection Enhancement and Giant Lateral Shift in Defective Photonic Crystals with Graphene. Appl. Sci. 2019, 9, 2141. [Google Scholar] [CrossRef]
- Liu, Q.; Ke, S.; Liu, W. Mode conversion and absorption in an optical waveguide under cascaded complex modulations. Opt. Quantum Electron. 2018, 50, 356. [Google Scholar] [CrossRef]
- Zhao, D.; Zhong, D.; Hu, Y.; Ke, S.; Liu, W. Imaginary modulation inducing giant spatial Goos–Hänchen shifts in one-dimensional defective photonic lattices. Opt. Quantum Electron. 2019, 51, 113. [Google Scholar] [CrossRef]
- Wang, F.; Qin, C.Z.; Wang, B.; Long, H.; Wang, K.; Lu, P.X. Rabi oscillations of plasmonic supermodes in graphene multilayer arrays. IEEE J. Sel. Top. Quantum Electron. 2017, 23, 4600105. [Google Scholar] [CrossRef]
- Wang, S.; Wang, B.; Qin, C.; Wang, K.; Long, H. Rabi oscillations of optical modes in a waveguide with dynamic modulation. Opt. Quantum Electron. 2017, 49, 389. [Google Scholar] [CrossRef]
- Qin, C.; Zhou, F.; Peng, Y.; Sounas, D.; Zhu, X.; Wang, B.; Dong, J.; Zhang, X.; Alù, A.; Lu, P. Spectrum Control through Discrete Frequency Diffraction in the Presence of Photonic Gauge Potentials. Phys. Rev. Lett. 2018, 120, 133901. [Google Scholar] [CrossRef]
- Lu, L.; Joannopoulos, J.D.; Soljačić, M. Topological photonics. Nat. Photonics 2014, 8, 821–829. [Google Scholar] [CrossRef]
- Peng, Y.G.; Qin, C.Z.; Zhao, D.G.; Shen, Y.X.; Xu, X.Y.; Bao, M.; Jia, H.; Zhu, X.F. Experimental demonstration of anomalous Floquet topological insulator for sound. Nat. Commun. 2016, 7, 13368. [Google Scholar] [CrossRef] [PubMed]
- Ke, S.; Zhao, D.; Liu, Q.; Wu, S.; Wang, B.; Lu, P. Optical imaginary directional couplers. J. Lightwave Technol. 2018, 36, 2510–2516. [Google Scholar] [CrossRef]
- Ke, S.; Zhao, D.; Liu, J.; Liu, Q.; Liao, Q.; Wang, B.; Lu, P. Topological bound modes in anti-PT-symmetric optical waveguide arrays. Opt. Express 2019, 27, 13858–13870. [Google Scholar] [CrossRef]
- Deng, H.; Chen, X.; Panoiu, N.C.; Ye, F. Topological surface plasmons in superlattices with changing sign of the average permittivity. Opt. Lett. 2016, 41, 4281–4284. [Google Scholar] [CrossRef]
- Deng, H.; Chen, Y.; Panoiu, N.C.; Malomed, B.A.; Ye, F. Surface modes in plasmonic Bragg fibers with negative average permittivity. Opt. Express 2018, 26, 2559–2568. [Google Scholar] [CrossRef]
- Blanco-Redondo, A.; Andrea, I.; Collins, M.J.; Harari, G.; Lumer, Y.; Rechtsman, M.C.; Eggleton, B.J.; Segev, M. Topological optical waveguiding in silicon and the transition between topological and trivial defect states. Phys. Rev. Lett. 2016, 116, 163901. [Google Scholar] [CrossRef]
- Cheng, Q.; Pan, Y.; Wang, Q.; Li, T.; Zhu, S. Topologically protected interface mode in plasmonic waveguide arrays. Laser Photonics Rev. 2015, 9, 392–398. [Google Scholar] [CrossRef]
- Pocock, S.R.; Xiao, X.; Huidobro, P.A.; Giannini, V. Topological plasmonic chain with retardation and radiative effects. ACS Photonics 2018, 5, 2271–2279. [Google Scholar] [CrossRef]
- Ling, C.W.; Xiao, M.; Chan, C.T.; Yu, S.F.; Fung, K.H. Topological edge plasmon modes between diatomic chains of plasmonic nanoparticles. Opt. Express 2015, 23, 2021–2031. [Google Scholar] [CrossRef] [PubMed]
- Ge, L.; Wang, L.; Xiao, M.; Wen, W.; Chan, C.T.; Han, D. Topological edge modes in multilayer graphene systems. Opt. Express 2015, 23, 21585–21595. [Google Scholar] [CrossRef] [PubMed]
- Ke, S.; Wang, B.; Long, H.; Wang, K.; Lu, P. Topological edge modes in non-Hermitian plasmonic waveguide arrays. Opt. Express 2017, 25, 11132–11143. [Google Scholar] [CrossRef] [PubMed]
- Meng, P.; Zhao, D.; Zhong, D.; Liu, W. Topological plasmonic modes in graphene-coated nanowire arrays. Opt. Quantum Electron. 2019, 51, 156. [Google Scholar] [CrossRef]
- Wang, F.; Ke, S.; Qin, C.; Wang, B.; Long, H.; Wang, K.; Lu, P. Topological interface modes in graphene multilayer arrays. Opt. Laser Technol. 2018, 103, 272–278. [Google Scholar] [CrossRef]
- Ge, L.; Liu, L.; Xiao, M.; Du, G.; Shi, L.; Han, D.; Chan, C.T.; Zi, J. Topological phase transition and interface states in hybrid plasmonic-photonic systems. J. Opt. 2017, 19, 06LT02. [Google Scholar] [CrossRef]
- Jackiw, R.; Rebbi, C. Solitons with fermion number ½. Phys. Rev. D 1976, 13, 3398. [Google Scholar] [CrossRef]
- Truong, X.T.; Biancalana, F. Linear and nonlinear photonic Jackiw-Rebbi states in waveguide arrays. Phys. Rev. A 2017, 96, 013831. [Google Scholar]
- Tran, T.X.; Duong, D.C.; Fabio, B. Interaction Between Dirac Solitons and Jackiw–Rebbi States in Binary Waveguide Arrays. J. Lightwave Technol. 2017, 35, 5092–5097. [Google Scholar] [CrossRef]
- Alexey, A.G.; Dmitry, V.Z.; Alexey, P.S.; Alexander, B.K.; Maxim, A.G. Photonic Jackiw-Rebbi states in all-dielectric structures controlled by bianisotropy. Phys. Rev. B 2019, 99, 205122. [Google Scholar]
- Charles, A.D.; Guillaume, W. Topological collective plasmons in bipartite chains of metallic nanoparticles. Phys. Rev. B 2017, 95, 125426. [Google Scholar]
- Wang, H.; Kong, W.; Zhang, P.; Li, Z.; Zhong, D. Coherent Perfect Absorption Laser Points in One-Dimensional Anti-Parity–Time-Symmetric Photonic Crystals. Appl. Sci. 2019, 9, 2738. [Google Scholar] [CrossRef]



© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, C.; Zhang, P.; Zhao, D.; Guo, H.; Huang, M.; Ke, S. Plasmonic Jackiw-Rebbi Modes in Graphene Waveguide Arrays. Appl. Sci. 2019, 9, 4152. https://doi.org/10.3390/app9194152
Xu C, Zhang P, Zhao D, Guo H, Huang M, Ke S. Plasmonic Jackiw-Rebbi Modes in Graphene Waveguide Arrays. Applied Sciences. 2019; 9(19):4152. https://doi.org/10.3390/app9194152
Chicago/Turabian StyleXu, Chunyan, Pu Zhang, Dong Zhao, Huang Guo, Mingqiang Huang, and Shaolin Ke. 2019. "Plasmonic Jackiw-Rebbi Modes in Graphene Waveguide Arrays" Applied Sciences 9, no. 19: 4152. https://doi.org/10.3390/app9194152
APA StyleXu, C., Zhang, P., Zhao, D., Guo, H., Huang, M., & Ke, S. (2019). Plasmonic Jackiw-Rebbi Modes in Graphene Waveguide Arrays. Applied Sciences, 9(19), 4152. https://doi.org/10.3390/app9194152





