Correlation between Coda Wave and Stresses in Uni-Axial Compression Concrete
Abstract
1. Introduction
2. Correlation Model of Sound Velocity and Stress in Concrete
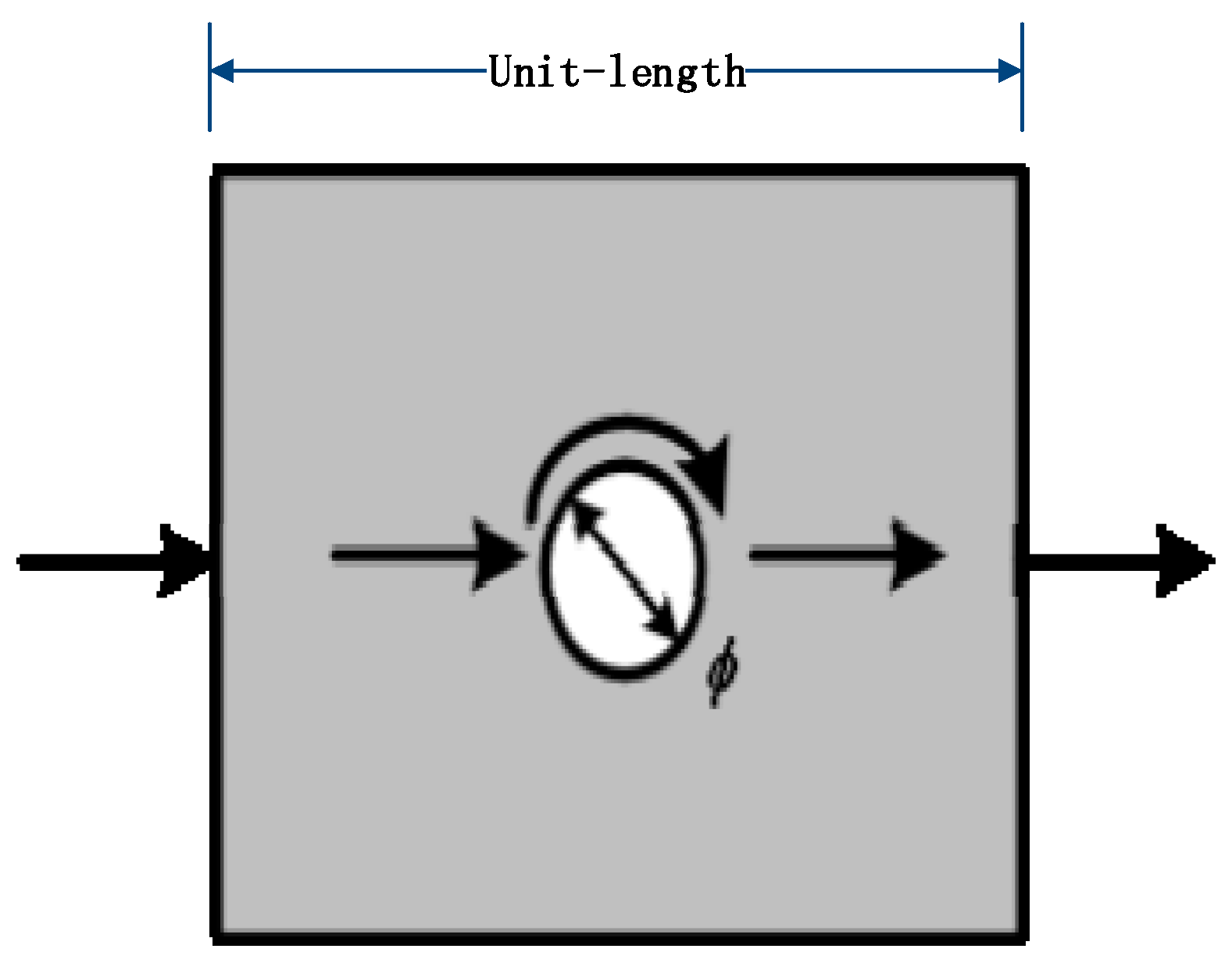
2.1. Sound Velocity in Concrete without Stress Action
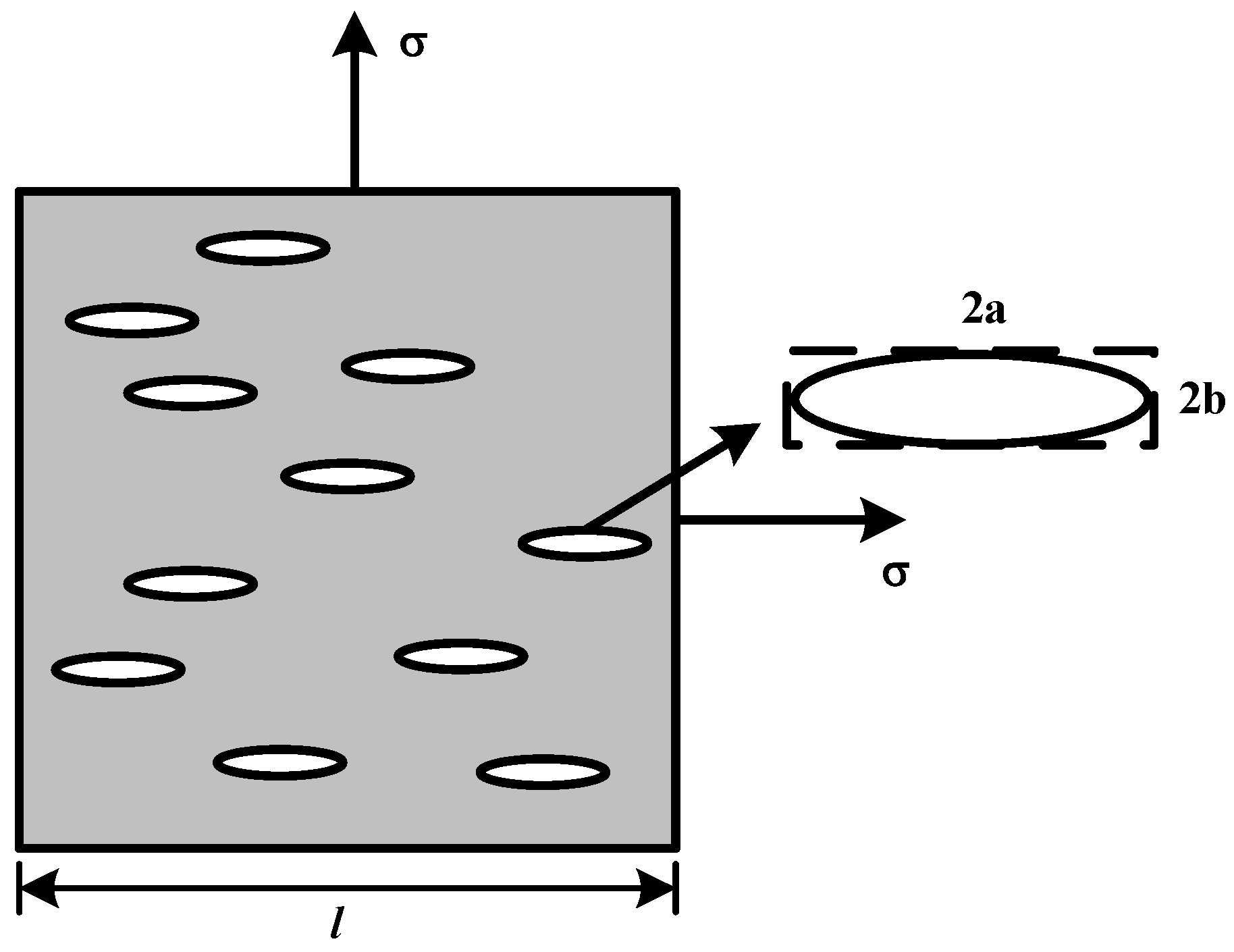
2.2. Crack Deformation under Uni-Axial Stress
2.3. Sound Velocity Change Induced by Uni-Axial Stress
3. Experimental Investigation
3.1. Experimental System and Sensors
3.2. Specimen Arrangement
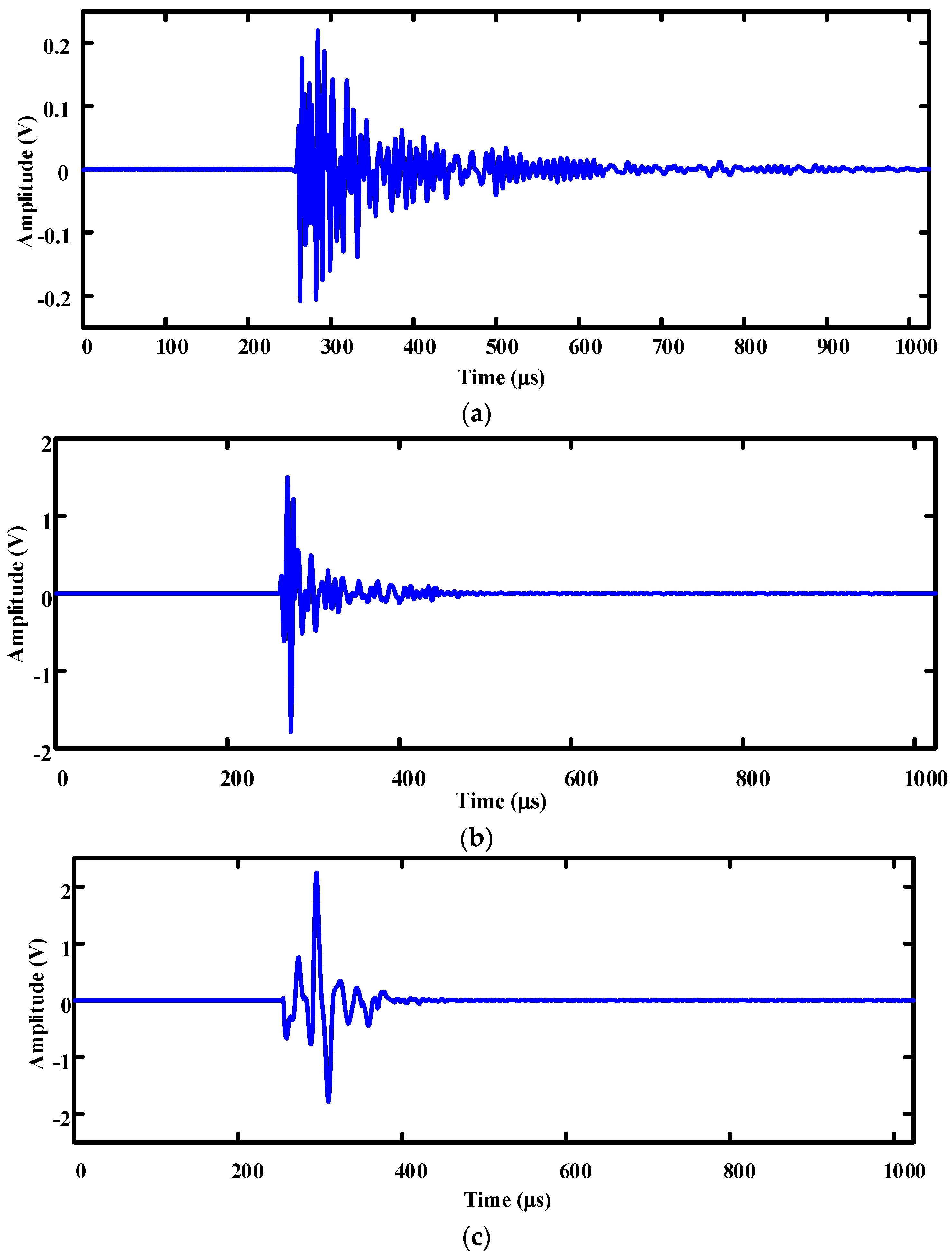
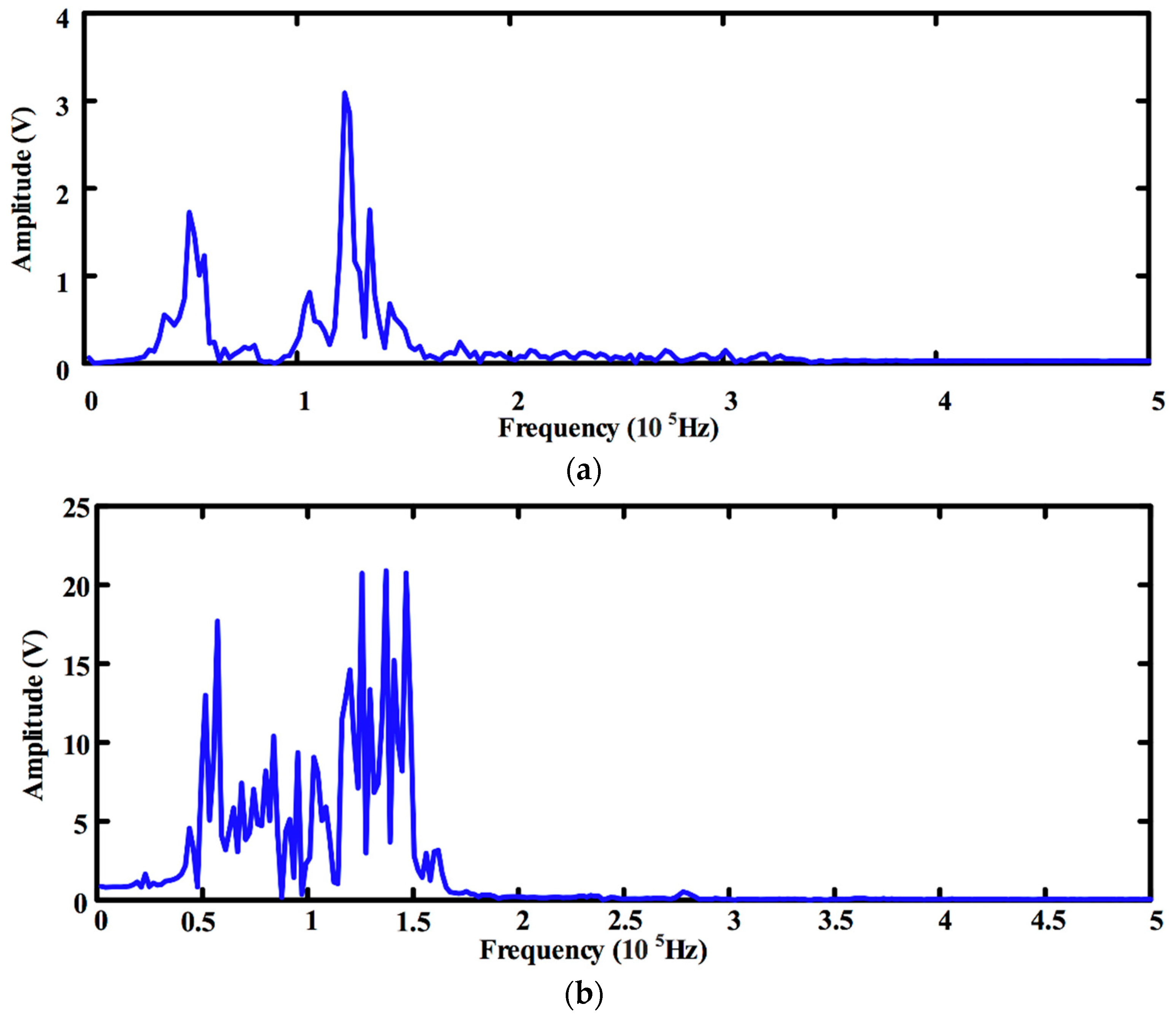
3.3. Propagating Regularity of the Coda Wave in Concrete
3.3.1. Frequency Effect
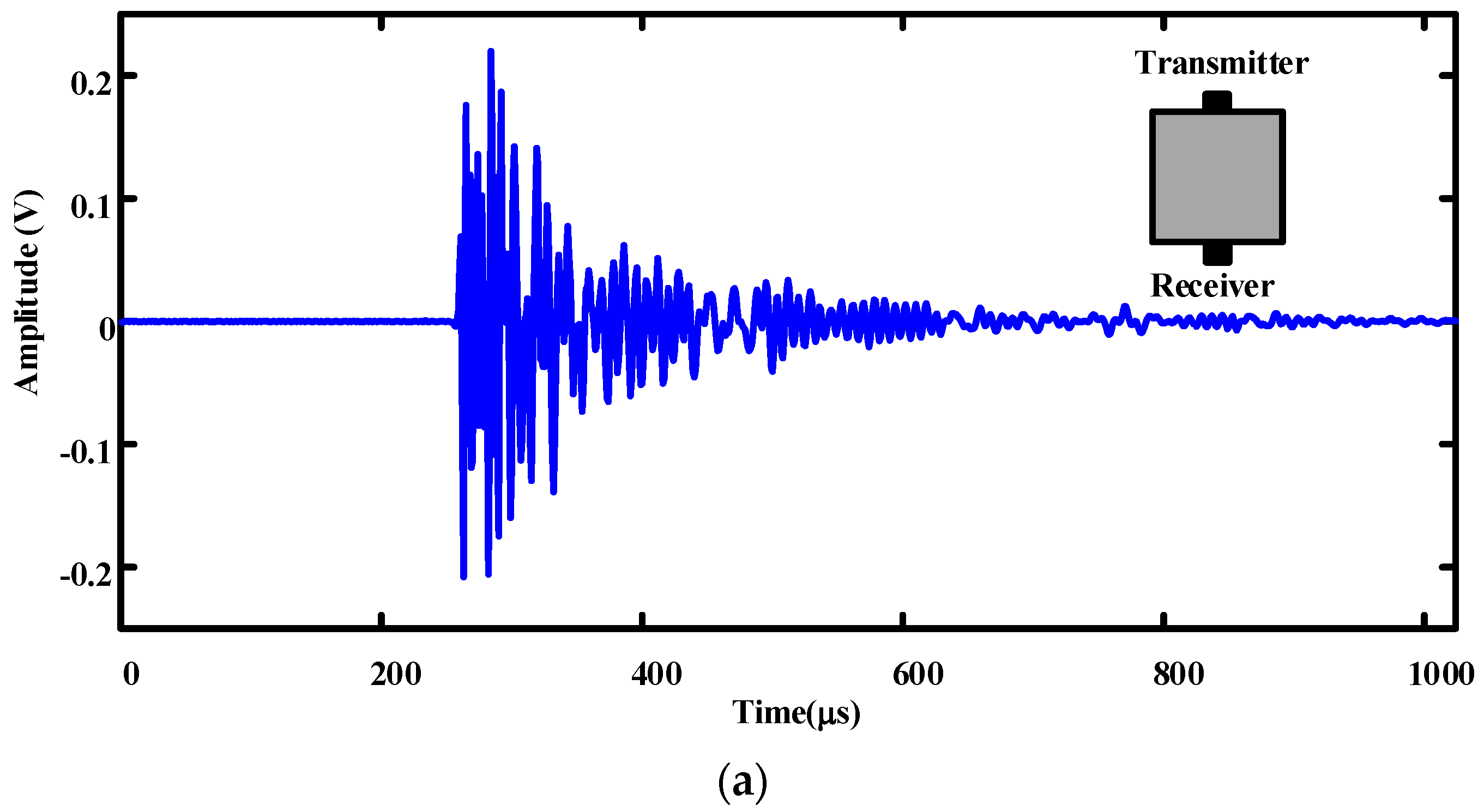
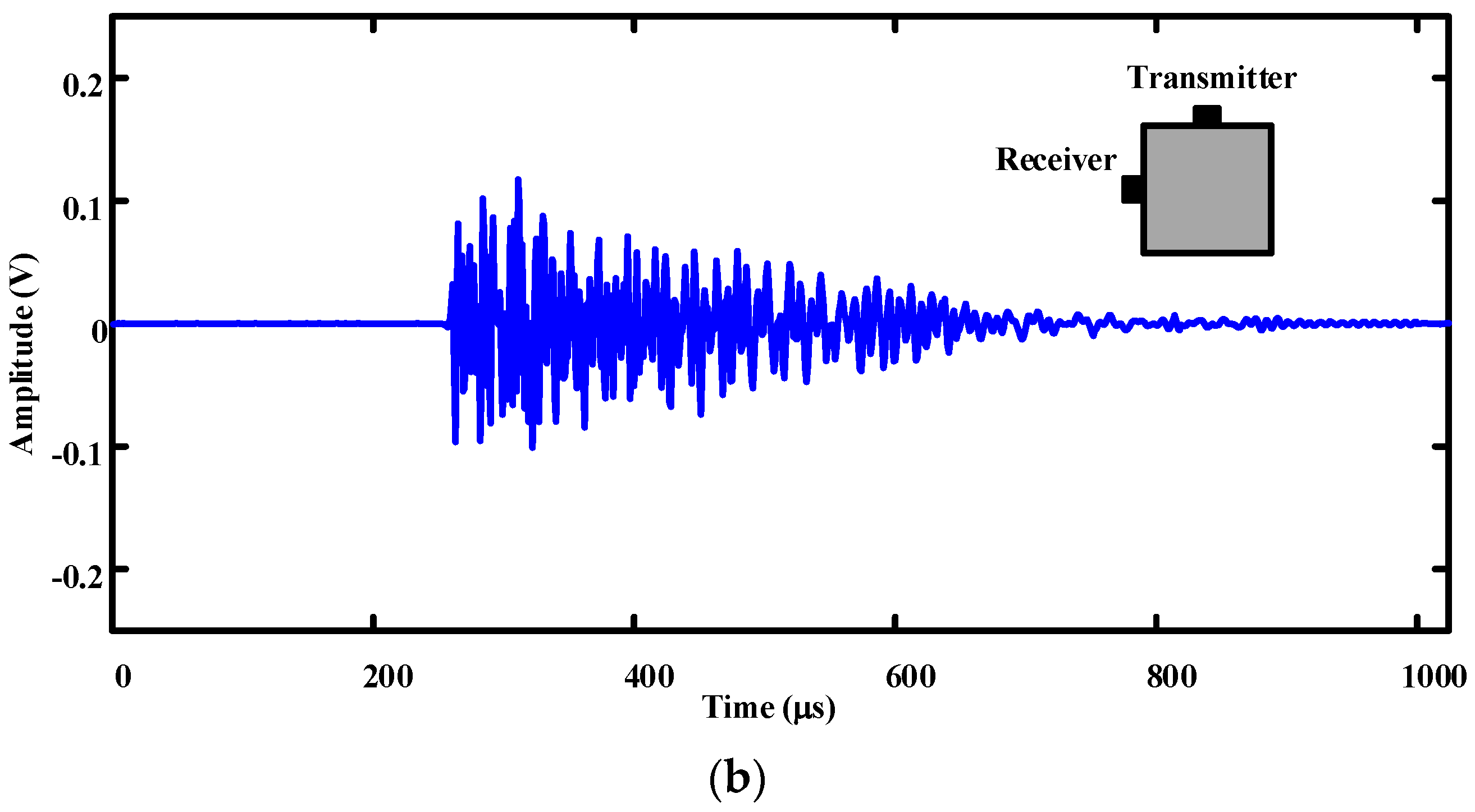
3.3.2. Influence of the Relative Position of Transmitters and Receivers
3.4. The Coda Wave Test under Uni-Axial Stress
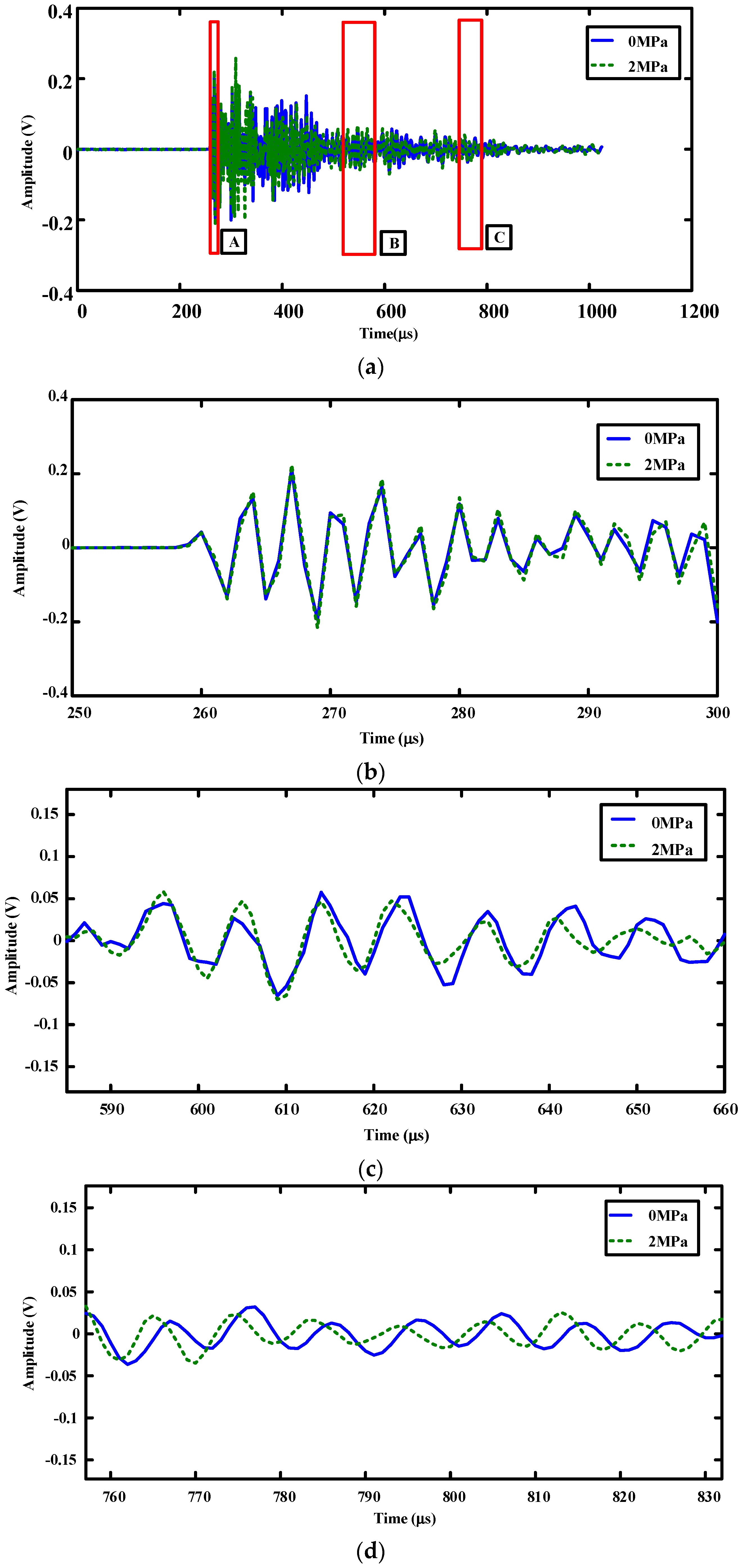
3.4.1. CWI Analysis Method
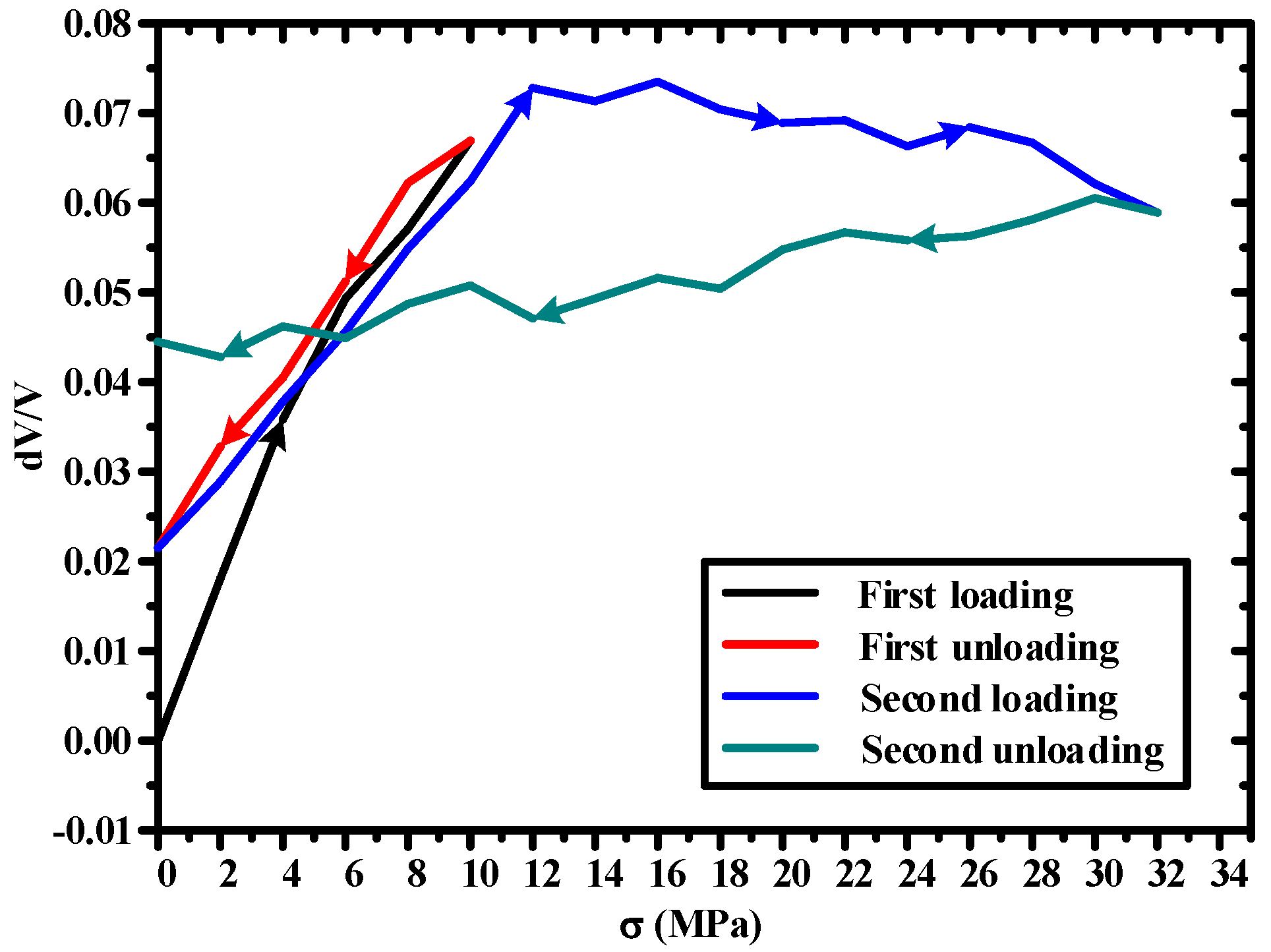
3.4.2. Relation between Stress and Coda Wave Velocity
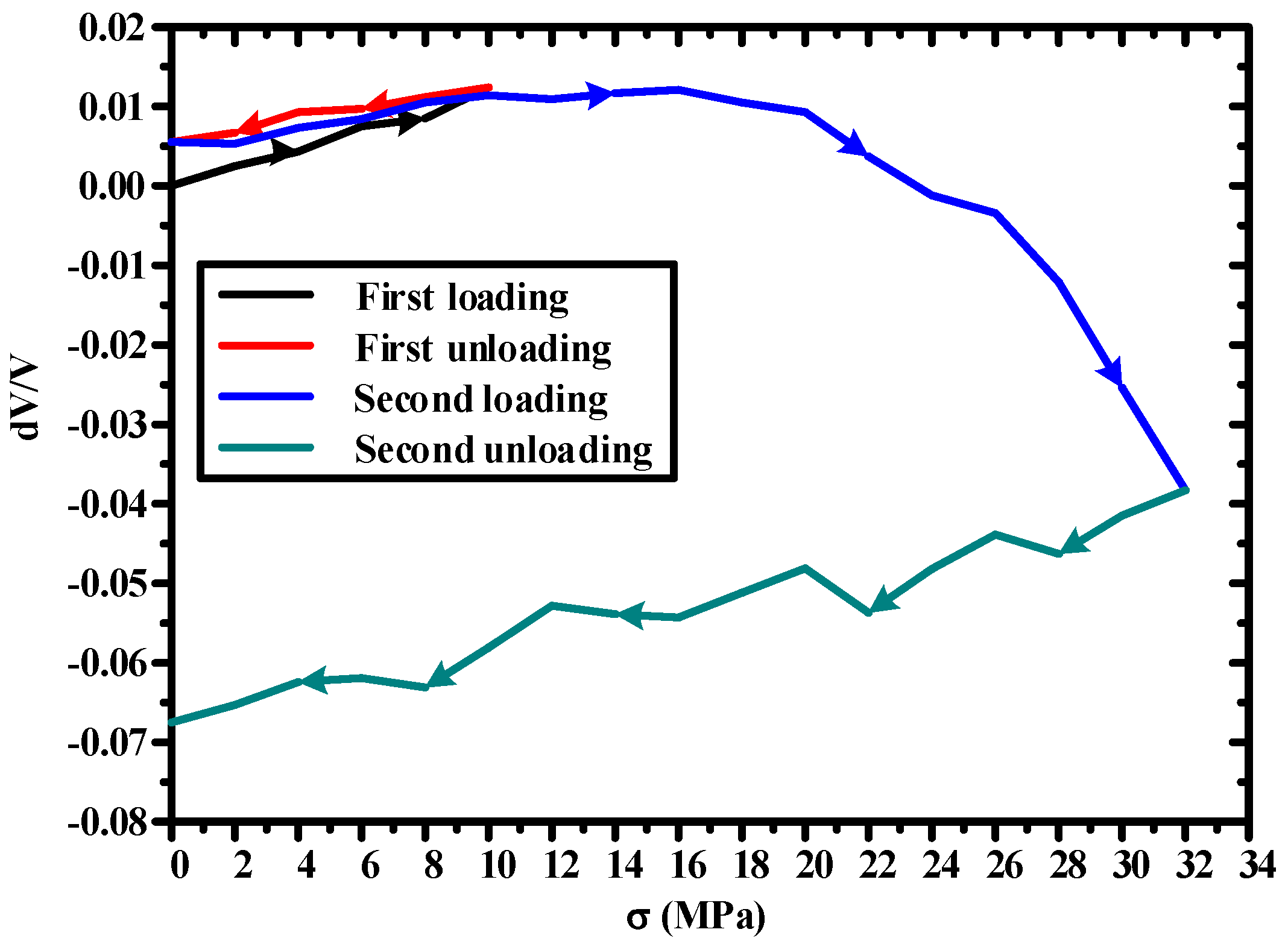
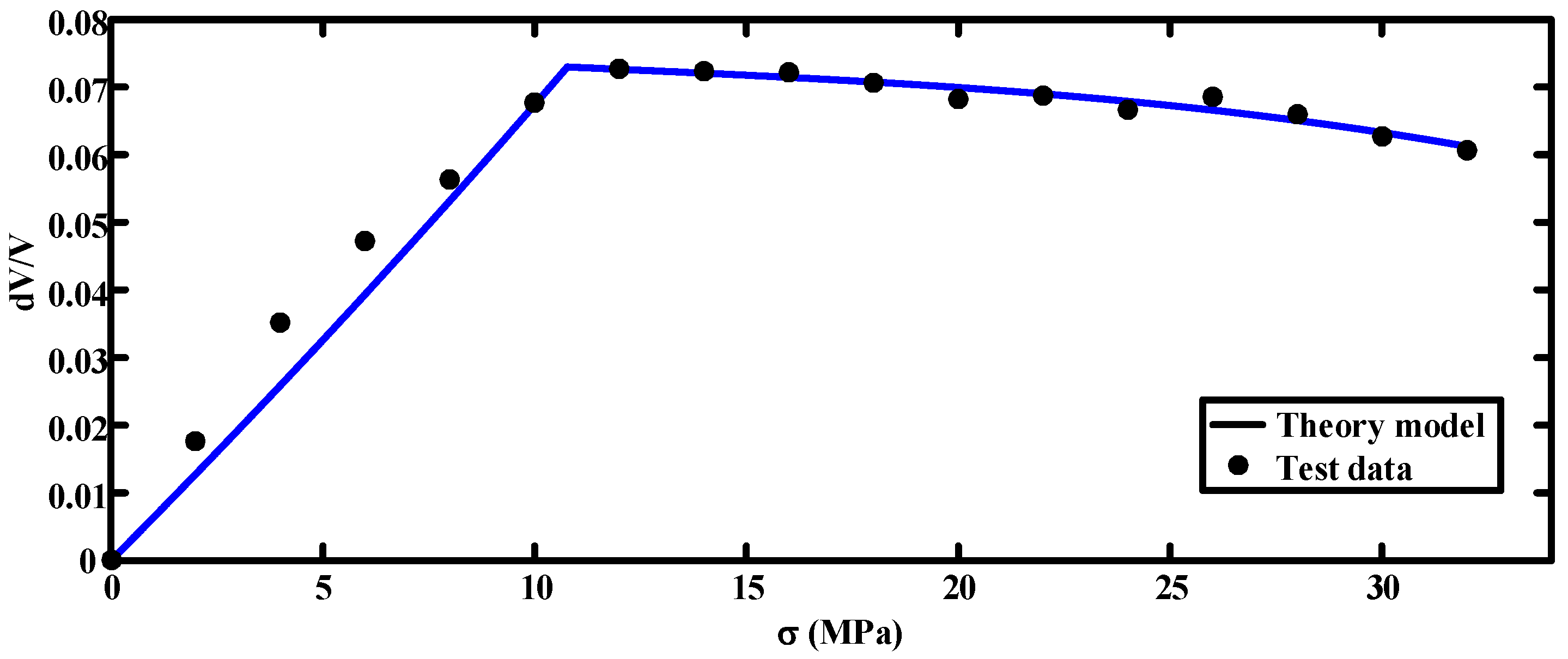
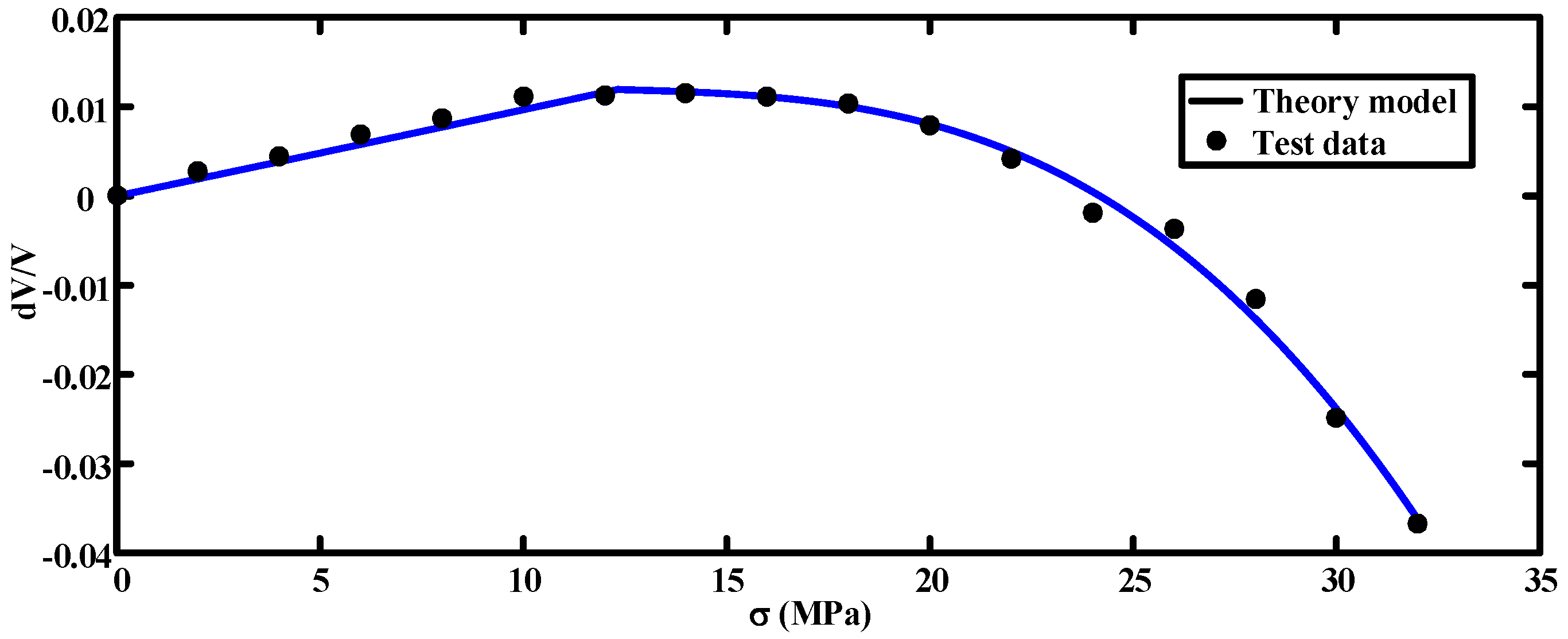
3.4.3. Data Processing and Parameter Fitting
4. Discussion
- Step 1.
- Conduction of field test or laboratory experiment on unloaded concrete to obtain the initial waveform of the coda wave;
- Step 2.
- Calibration or regression of the parameters in Equation (22);
- Step 3.
- Conduction of field test on loaded concrete structures to obtain the waveform of the coda wave;
- Step 4.
- Analysis of the obtained coda wave by the wave expansion method to determine the relative change between the sound velocity ;
- Step 5.
- Determine the stress (or stress increment ) using Equation (22) with calibrated parameters.
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| acoustic slowness; | |
| sound velocity; | |
| , and | velocity of the waves propagating in the elastic matrix, concrete with cracks, and air, respectively; |
| , , and | acoustic slowness in the elastic matrix, concrete with cracks, and air, respectively; |
| porosity of concrete; | |
| stress in concrete under an applied load; | |
| total deformation of concrete; | |
| deformation of elastic concrete matrix; | |
| closed deformation of microcracks; | |
| extended deformation of microcracks; | |
| elastic modulus of concrete; | |
| shear modulus of concrete; | |
| elastic constant of concrete, ; | |
| Poisson’s ratio of concrete; | |
| fracture toughness of concrete; | |
| crack density parameter in unit volume concrete; | |
| a0 | semi-major axis of concrete microcrack; |
| b0 | semi-minor axis of concrete microcrack; |
| and | orientation parameters of crack; |
| critical stress of crack closure or expansion; | |
| proportional to the stress ; | |
| U | displacement of crack in the direction of the major axis; |
| V | displacement of crack in the direction of the minor axis; |
| displacement of point A in the direction of the major axis; | |
| displacement of point B in the direction of the major axis; | |
| displacement of point A in the direction of the minor axis; | |
| displacement of point B in the direction of the minor axis; | |
| displacement of point A in the direction of the y axis; | |
| displacement of point B in the direction of the x axis; | |
| closure displacement in the direction of the y axis; | |
| closure displacement in the direction of the y axis; | |
| expansion displacement in the direction of the x axis; | |
| kN | intensity of crack in concrete without an applied load; |
| closure deformation of concrete caused by stress σ; | |
| expansion deformation of concrete caused by stress σ; | |
| volume strain of the crack closure; | |
| volume strain of the crack expansion; | |
| ka, kb1 and kb2 | undetermined coefficients; |
| density parameter of the horizontal equivalent cracks in concrete; | |
| density parameter of the extended equivalent cracks in concrete; | |
| a | semi-length of microcrack in concrete; |
| b | semi-width of microcrack in concrete; |
| increment of acoustic slowness caused by microcracks; | |
| S1 | acoustic slowness caused by microcrack, when the sound wave passes through the element in the minor axis direction of the crack; |
| S2 | acoustic slowness caused by microcrack, when the sound wave passes through the element in the major axis direction of the crack; |
| increment of concrete acoustic slowness caused by a set of parallel cracks; | |
| shape correction coefficient of an elliptical crack in a rectangular crack; | |
| and | increment of the acoustic slowness caused by crack closure and expansion, respectively; |
| ks | parameter related to the shape and density of crack, ; |
| , , , , , and | undetermined parameters related to cracks, , , , , , ; |
| increment of sound velocity; | |
| i = 1, 2, … | denotes axial and horizontal directions. |
References
- Porco, F.; Fiore, A.; Porco, G.; Uva, G. Monitoring and safety for prestressed bridge girders by SOFO sensors. J. Civ. Struct. Heal. Monit. 2012, 3, 3–18. [Google Scholar] [CrossRef]
- Zhang, F.P.; Qiu, Z.G. Analysis of measuring existing stresses in concrete structure by hole drilling core surface strain gauge method. Mater. Res. Innov. 2011, 15, s601–s604. [Google Scholar] [CrossRef]
- Trautner, C.A.; Mcginnis, M.J.; Pessiki, S.P. The incremental core drilling method to determine in-situ stresses in concrete. ACI Mater. J. 2011, 8, 85–94. [Google Scholar]
- McCann, D.M.; Forde, M.C. Review of NDT methods in the assessment of concrete and masonry structures. NDT E Int. 2001, 34, 71–84. [Google Scholar] [CrossRef]
- Invernizzi, S.; Lacidogna, G.; Carpinteri, A. Numerical models for the assessment of historical masonry structures and materials, monitored by acoustic emission. Appl. Sci. 2016, 6, 102. [Google Scholar] [CrossRef]
- Planès, T.; Larose, E. A review of ultrasonic Coda Wave Interferometry in concrete. Cem. Concr. Res. 2013, 53, 248–255. [Google Scholar] [CrossRef]
- Hertlein, B.H. Stress wave testing of concrete: A 25-year review and a peek into the future. Constr. Build. Mater. 2013, 38, 1240–1245. [Google Scholar] [CrossRef]
- Mutlib, N.K.; Baharom, S.B.; El-Shafie, A.; Nuawi, M.Z. Ultrasonic health monitoring in structural engineering: Buildings and bridges. Struct. Control Heal. Monit. 2016, 23, 409–422. [Google Scholar] [CrossRef]
- Niederleithinger, E.; Taffe, A. Early stage elastic wave velocity of concrete piles. Cem. Concr. Compos. 2006, 28, 317–320. [Google Scholar] [CrossRef]
- Aggelis, D.G.; Shiotani, T.; Philippidis, T.P.; Polyzos, D. Stress wave scattering: An enemy or friend of non-destructive testing of Concrete? J. Solid Mech. Mater. Eng. 2008, 2, 397–408. [Google Scholar] [CrossRef]
- Snieder, R. The theory of coda wave interferometry. Pure Appl. Geophys. 2006, 163, 455–473. [Google Scholar] [CrossRef]
- Aki, K. Analysis of the seismic coda of local earthquakes as scattered waves. J. Geophys. Res. 1969, 74, 615–631. [Google Scholar] [CrossRef]
- Snieder, R.; Grêt, A.; Douma, H.; Scales, J. Coda wave interferometry for estimating nonlinear behavior in seismic velocity. Science 2002, 295, 2253–2255. [Google Scholar] [CrossRef] [PubMed]
- Lobkis, O.I.; Weaver, R.L. Coda-wave interferometry in finite solids: Recovery of P-to-S conversion rates in an elastodynamic billiard. Phys. Rev. Lett. 2003, 90, 254302. [Google Scholar] [CrossRef] [PubMed]
- Larose, E.; Hall, S. Monitoring stress related velocity variation in concrete with a 2 × 10−5 relative resolution using diffuse ultrasound. J. Acoust. Soc. Am. 2009, 125, 1853–1856. [Google Scholar] [CrossRef] [PubMed]
- Larose, E.; de Rosny, J.; Margerin, L.; Anache, D.; Gouedard, P.; Campillo, M.; van Tiggelen, B. Observation of multiple scattering of kHz vibrations in a concrete structure and application to monitoring weak changes. Phys. Rev. E 2006, 73, 016609. [Google Scholar] [CrossRef] [PubMed]
- Schurr, D.P.; Kim, J.Y.; Sabra, K.G.; Jacobs, L.J. Damage detection in concrete using coda wave interferometry. NDT E Int. 2011, 44, 728–735. [Google Scholar] [CrossRef]
- Lillamand, I.; Chaix, J.F.; Ploix, M.A.; Garnier, V. Acoustoelastic effect in concrete material under uni-axial compressive loading. NDT E Int. 2010, 43, 655–660. [Google Scholar] [CrossRef]
- Niederleithinger, E.; Sens-Schönfelder, C.; Grothe, S.; Wiggenhauser, H. Coda Wave Interferometry used to localize compressional load effects in a concrete specimen. In Proceedings of the EWSHM-7th European Workshop on Structural Health Monitoring, Nates, France, 1 July 2014. [Google Scholar]
- Stähler, S.C.; Sensschönfelder, C.; Niederleithinger, E. Monitoring stress changes in a concrete bridge with coda wave interferometry. J. Acoust. Soc. Am. 2011, 129, 1945–1952. [Google Scholar] [CrossRef] [PubMed]
- Chronopoulos, D. Wave steering effects in anisotropic composite structures: Direct calculation of the energy skew angle through a finite element scheme. Ultrasonics 2017, 73, 43–48. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Fu, L.Y.; Zhang, L.; Wei, W.; Guan, X. Finite difference modeling of ultrasonic propagation (coda waves) in digital porous cores with un-split convolutional PML and rotated staggered grid. J. Appl. Geophys. 2014, 104, 75–89. [Google Scholar] [CrossRef]
- Walsh, J.B. The effect of cracks on the compressibility of rock. J. Geophys. Res. 1965, 70, 381–389. [Google Scholar] [CrossRef]
- Minato, S.; Tsuji, T.; Ohmi, S.; Matsuoka, T. Monitoring seismic velocity change caused by the 2011 Tohoku-oki earthquake using ambient noise records. Geophys. Res. Lett. 2012, 39, L09309–L09314. [Google Scholar] [CrossRef]


| Center Frequency (kHz) | Size (mm) | Weight (g) | Operating Temperature Range (°C) | Frequency Range (kHz) | Resonance Frequency (kHz) |
|---|---|---|---|---|---|
| 300 | 8 × 8 | 2 | −65~177 | 150~400 | 300 |
| 150 | 23 × 19 | 27 | −45~125 | 50~200 | 150 |
| 50 | 23 × 19 | 33 | −45~125 | 35~80 | 50 |
| Composition | Cement | Sand | Aggregate | Water | Admixture |
|---|---|---|---|---|---|
| Dosage per m3 (kg) | 420 | 622 | 1155 | 210 | 2.10 (1%) |
| Model Parameter | Fitted Value | Correlation Coefficient R |
|---|---|---|
| 6.314 × 10−3 | 0.9359 | |
| 2.395 × 10−4 | 0.9109 | |
| 5.454 × 10−7 | ||
| 10.77 |
| Model Parameter | Fitted Value | Correlation Coefficient R |
|---|---|---|
| 9.58 × 10−4 | 0.9237 | |
| 1.326 × 10−4 | 0.9915 | |
| 6.147 × 10−6 | ||
| 12.33 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, J.; Han, B.; Xie, H.-B.; Zhu, L.; Zheng, G.; Wang, W. Correlation between Coda Wave and Stresses in Uni-Axial Compression Concrete. Appl. Sci. 2018, 8, 1609. https://doi.org/10.3390/app8091609
Zhang J, Han B, Xie H-B, Zhu L, Zheng G, Wang W. Correlation between Coda Wave and Stresses in Uni-Axial Compression Concrete. Applied Sciences. 2018; 8(9):1609. https://doi.org/10.3390/app8091609
Chicago/Turabian StyleZhang, Jinquan, Bing Han, Hui-Bing Xie, Li Zhu, Gang Zheng, and Wenwu Wang. 2018. "Correlation between Coda Wave and Stresses in Uni-Axial Compression Concrete" Applied Sciences 8, no. 9: 1609. https://doi.org/10.3390/app8091609
APA StyleZhang, J., Han, B., Xie, H.-B., Zhu, L., Zheng, G., & Wang, W. (2018). Correlation between Coda Wave and Stresses in Uni-Axial Compression Concrete. Applied Sciences, 8(9), 1609. https://doi.org/10.3390/app8091609





