A Comparative Study on Violent Sloshing with Complex Baffles Using the ISPH Method
Abstract
:Featured Application
Abstract
1. Introduction
2. ISPH Methodology
3. Model Validation and Convergence Analysis
3.1. Model Validations through Experiment
3.2. Model Convergence and CPU Analyses
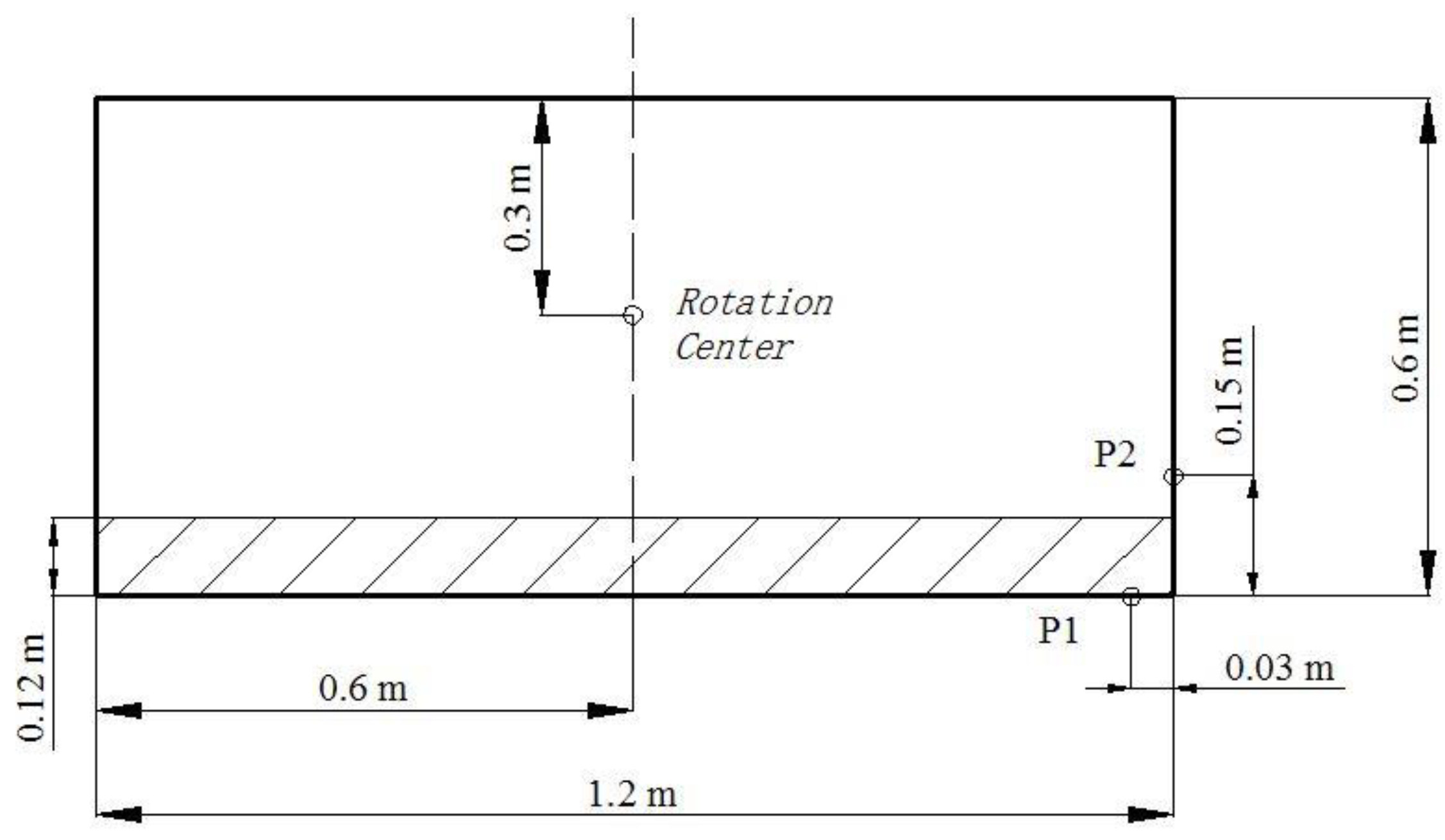
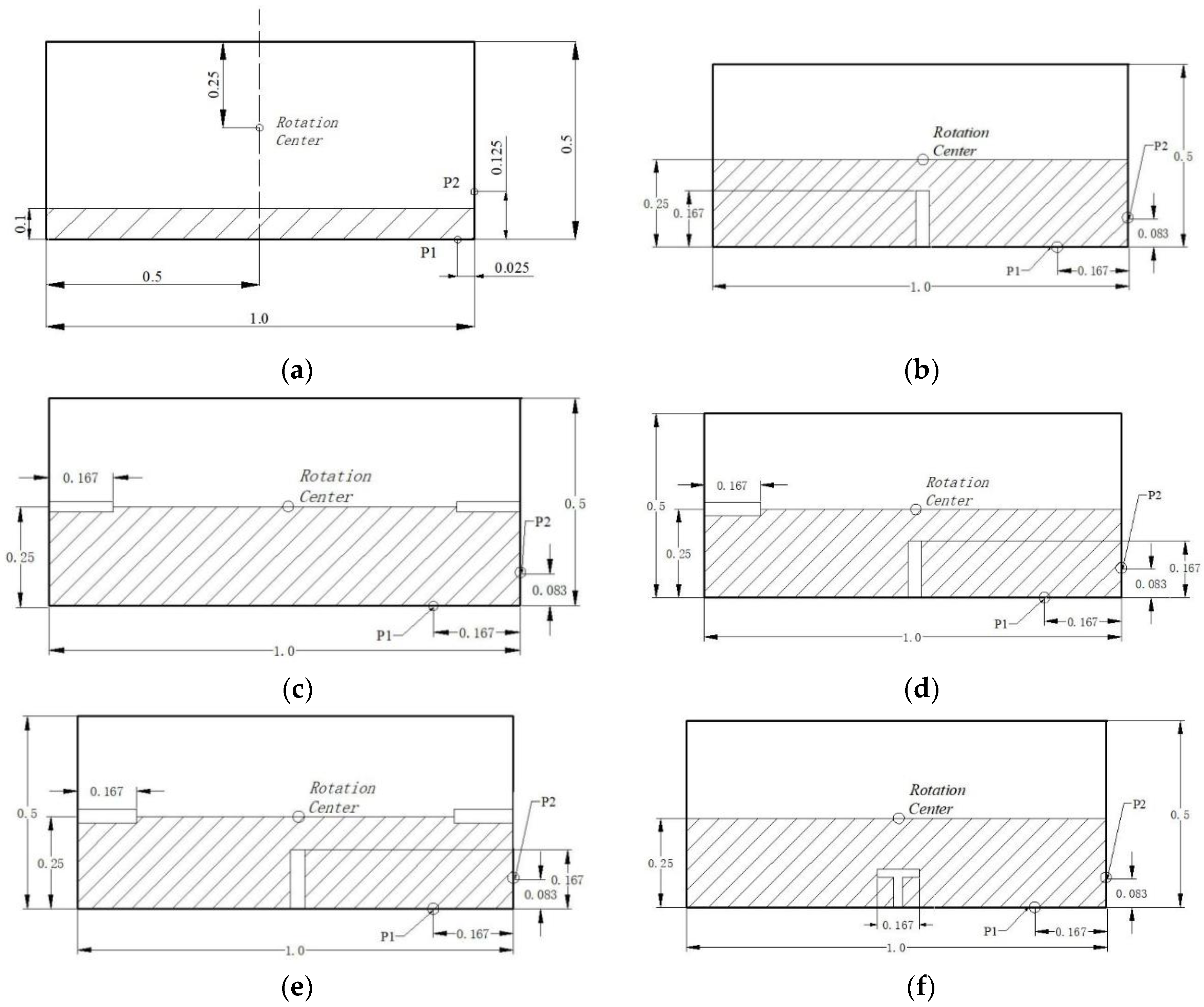
4. Configurations of Different Complex Baffles
5. Analyses and Discussion
5.1. Height of Simple Vertical Baffle
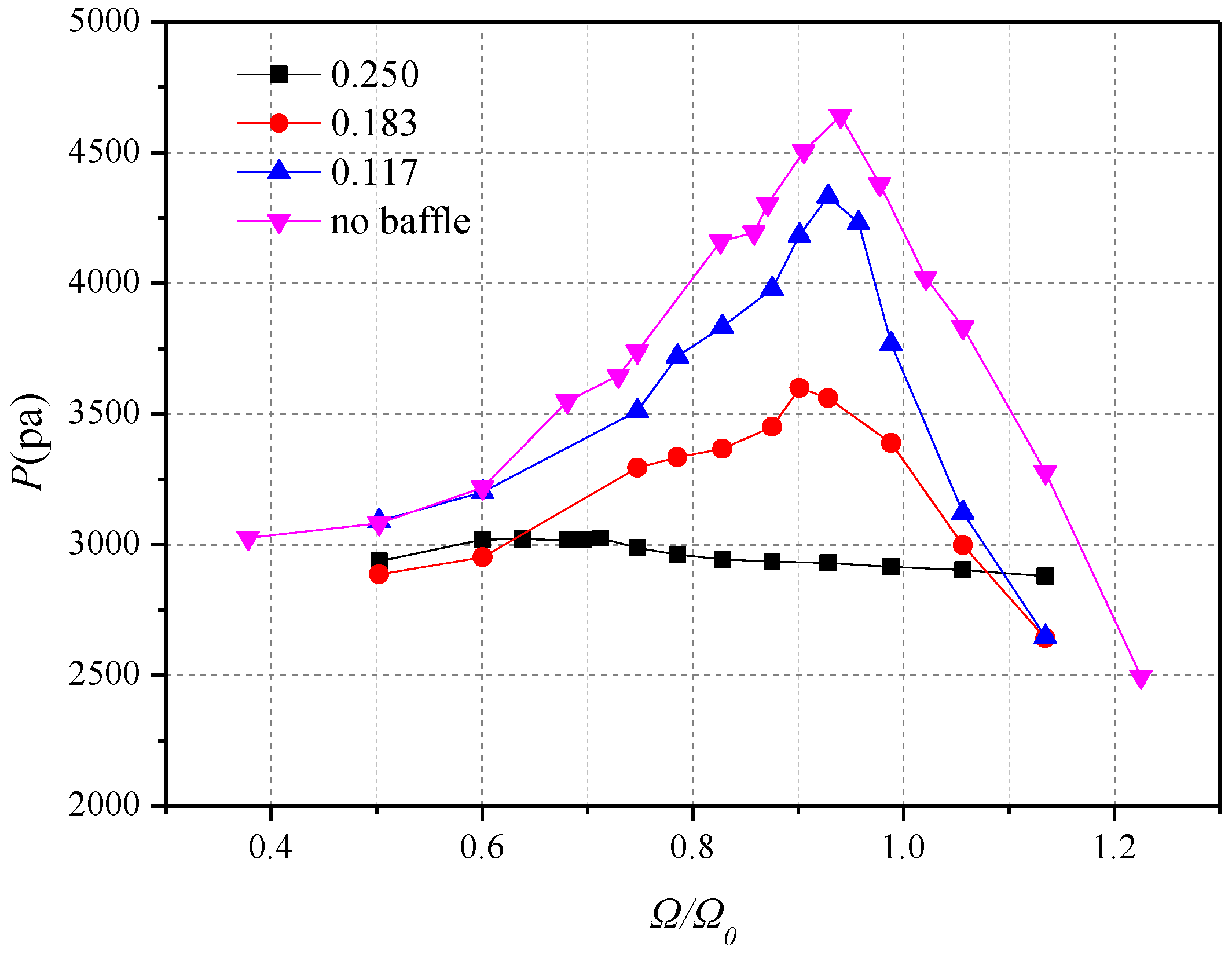
5.2. Position Height of Two Horizontal Baffles
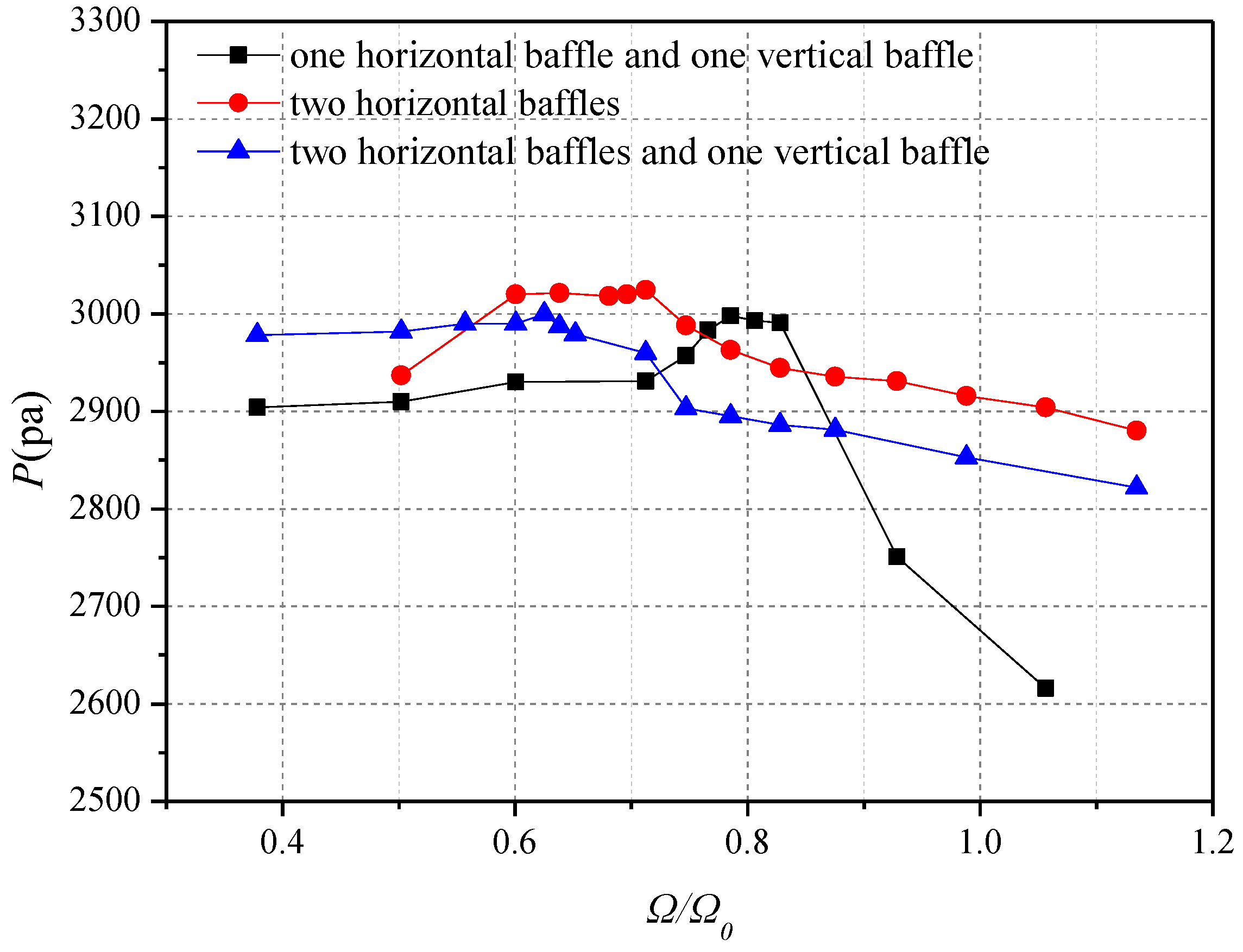
5.3. Combined Vertical and Horizontal Baffles
5.4. T-Shaped Baffle
6. Conclusions
- (1)
- Both of the ISPH and STAR-CCM+ computations have been carried out in 2D. According to previous trials, it seems that not much difference has been generated between the 2D and 3D STAR-CCM+, at least for the water surface and impact pressure in the present sloshing flows;
- (2)
- The compressibility of entrapped air should have played an important role during the violent sloshing process, and a fully two-phase ISPH coupled model would provide an ultimate solution for such a process. The maximum Mach number of all the particles in the experimental validations remains nearly below 1%, which may have partially justified the use of the incompressible SPH model;
- (3)
- Using a full 3-D model compared with a 2-D model could lead to different flow fields when the turbulence model is employed. This could partially explain the numerical disagreement between STAR-CCM+ and ISPH in the model validations. As documented by Alberello et al. [31], the presence of turbulence produces fully three-dimensional flow structures in the breaking region at the tip of the wave crest. In the sloshing applications, the major interest would be the general free surface deformation and macro liquid impact pressure on the tank walls. Therefore, a 2D ISPH model could provide a reasonable engineering approximation;
- (4)
- SPH for the coastal and ocean engineering problems has been mainly used for impulsive wave impact on breakwater, and similar longer simulations are often performed with the more traditional CFD methods such as VOF;
- (5)
- Lastly, the existence of baffles can indeed change the resonance frequency of a sloshing tank and this effect can be quantitatively evaluated by [2,3,4]. However, under the resonance frequency, some nonlinear quantities such as impact pressure may not achieve the maximum but result in a slight phase shift, which has also been observed in [20]. In the present study, we mainly used the maximum impact pressure rather than other resonance quantities.
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Ibrahim, R.A. Liquid Sloshing Dynamics: Theory and Applications; Cambridge University Press: Cambridge, UK, 2005. [Google Scholar]
- Faltinsen, O.M.; Rognebakke, O.F.; Timokha, A.N. Resonant three-dimensional non-linear sloshing in a square-base basin. J. Fluid Mech. 2003, 487, 1–42. [Google Scholar] [CrossRef]
- Faltinsen, O.M.; Rognebakke, O.F.; Timokha, A.N. Resonant three-dimensional non-linear sloshing in a square-base basin. Part 2. Effect of higher modes. J. Fluid Mech. 2005, 523, 199–218. [Google Scholar] [CrossRef]
- Faltinsen, O.M.; Rognebakke, O.F.; Timokha, A.N. Resonant three-dimensional non-linear sloshing in a square-base basin. Part 3. Base ratio perturbations. J. Fluid Mech. 2006, 551, 93–116. [Google Scholar] [CrossRef]
- Huang, S.; Duan, W.Y.; Ma, Q.W. An approximation to energy dissipation in time domain simulation of sloshing waves based on linear potential theory. China Ocean Eng. 2011, 2, 189–200. [Google Scholar] [CrossRef]
- Zhao, D.; Hu, Z.; Chen, G.; Lim, S.; Wang, S. Nonlinear sloshing in rectangular tanks under forced excitation. Int. J. Naval Archit. Ocean Eng. 2017. [Google Scholar] [CrossRef]
- Akyildiz, H.; Unal, E. Experimental investigation of pressure distribution on a rectangular tank due to the liquid sloshing. Ocean Eng. 2005, 32, 1503–1516. [Google Scholar] [CrossRef]
- Wu, G.X.; Ma, Q.W.; Taylor, R.E. Numerical simulation of sloshing waves in a 3D tank based on a finite element method. Appl. Ocean Res. 1998, 20, 337–355. [Google Scholar] [CrossRef]
- Chen, B.F.; Nokes, R. Time-independent finite difference analysis of fully non-linear and viscous fluid sloshing in a rectangular tank. J. Comput. Phys. 2005, 209, 47–81. [Google Scholar] [CrossRef]
- Pan, X.J.; Zhang, H.X.; Lu, Y.T. Numerical simulation of viscous liquid sloshing by moving-particle semi-implicit method. J. Mar. Sci. Appl. 2008, 7, 184–189. [Google Scholar] [CrossRef]
- Koshizuka, S.; Nobe, A.; Oka, Y. Numerical analysis of breaking waves using the moving particle semi-implicit method. Int. J. Numer. Methods Fluids 1998, 26, 751–769. [Google Scholar] [CrossRef]
- Zhou, J.T.; Ma, Q.W. MLPG method based on Rankine source solution for modeling 3D breaking waves. CMES Comput. Model. Eng. Sci. 2010, 56, 179–210. [Google Scholar]
- Liu, M.B.; Liu, G.R. Smoothed particle hydrodynamics (SPH): An overview and recent developments. Arch. Comput. Methods Eng. 2010, 17, 25–76. [Google Scholar] [CrossRef]
- Souto-Iglesias, A.; Delorme, L.; Pérez-Rojas, L.; Abril-Pérez, S. Liquid moment amplitude assessment in sloshing type problems with smooth particle hydrodynamics. Ocean Eng. 2006, 33, 1462–1484. [Google Scholar] [CrossRef]
- Rafiee, A.; Pistani, F.; Thiagarajan, K. Study of liquid sloshing: Numerical and experimental approach. Comput. Mech. 2011, 47, 65–75. [Google Scholar] [CrossRef]
- Marsh, A.; Prakash, M.; Semercigil, E.; Turan, O.F. A study of sloshing absorber geometry for structural control with SPH. J. Fluids Struct. 2011, 27, 1165–1181. [Google Scholar] [CrossRef]
- Shao, J.R.; Li, H.Q.; Liu, G.R.; Liu, M.B. An improved SPH method for modeling liquid sloshing dynamics. Comput. Struct. 2012, 100–101, 18–26. [Google Scholar] [CrossRef]
- Biswal, K.C.; Bhattacharyya, S.K.; Sinha, P.K. Non-linear sloshing in partially liquid filled containers with baffles. Int. J. Numer. Methods Eng. 2006, 68, 317–337. [Google Scholar] [CrossRef]
- Panigrahy, P.K.; Saha, U.K.; Maity, D. Experimental studies on sloshing behavior due to horizontal movement of liquids in baffled tanks. Ocean Eng. 2009, 36, 213–222. [Google Scholar] [CrossRef]
- Xue, M.A.; Lin, P. Numerical study of ring baffle effects on reducing violent liquid sloshing. Comput. Fluids 2011, 52, 116–129. [Google Scholar] [CrossRef]
- Goudarzi, M.A.; Sabbagh-Yazdi, S.R. Analytical and experimental evaluation on the effectiveness of upper mounted baffles with respect to commonly used baffles. Ocean Eng. 2012, 42, 205–217. [Google Scholar] [CrossRef]
- Goudarzi, M.A.; Danesh, P.N. Numerical investigation of a vertically baffled rectangular tank under seismic excitation. J. Fluids Struct. 2016, 61, 450–460. [Google Scholar] [CrossRef]
- Shao, S.D.; Lo, E.Y.M. Incompressible SPH method for simulating newtonian and non-newtonian flows with a free surface. Adv. Water Resour. 2003, 26, 787–800. [Google Scholar] [CrossRef]
- Gotoh, H.; Khayyer, A.; Ikari, H.; Arikawa, T.; Shimosako, K. On enhancement of Incompressible SPH method for simulation of violent sloshing flows. Appl. Ocean Res. 2014, 46, 104–105. [Google Scholar] [CrossRef]
- Khayyer, A.; Gotoh, H. A Multiphase Compressible—Incompressible Particle Method for Water Slamming; International Society of Offshore and Polar Engineers: Kona, HI, USA, 2015. [Google Scholar]
- Zheng, X.; Ma, Q.W.; Duan, W.Y. Incompressible SPH method based on Rankine source solution for violent water wave simulation. J. Comput. Phys. 2014, 276, 291–314. [Google Scholar] [CrossRef] [Green Version]
- Liao, K.P.; Hu, C.H. A coupled FDM-FEM method for free surface flow interaction with thin elastic plate. J. Mar. Sci. Technol. 2013, 18, 1–11. [Google Scholar] [CrossRef]
- Idelsohn, S.R.; Marti, J.; Gotoh, H.; Souto-Iglesias, A.; Oñate, E. Interaction between an elastic structure and free-surface flows: Experimental versus numerical comparisons using the PFEM. Comput. Mech. 2008, 43, 125–132. [Google Scholar] [CrossRef] [Green Version]
- Hwang, S.C.; Park, J.C.; Gotoh, H.; Khayyer, C.; Kang, K.J. Numerical simulations of sloshing flows with elastic baffles by using a particle-based fluid—Structure interaction analysis method. Ocean Eng. 2016, 118, 227–241. [Google Scholar] [CrossRef]
- Hwang, S.C.; Khayyer, A.; Gotoh, H.; Park, J.C. Development of a fully Lagrangian MPS-based coupled method for simulation of fluid—Structure interaction problems. J. Fluids Struct. 2014, 50, 497–511. [Google Scholar] [CrossRef]
- Alberello, A.; Pakodzi, C.; Nelli, F.; Bitner-Gregersen, E.M.; Toffoli, A. Three dimensional velocity field underneath a breaking rogue wave. In Proceedings of the 36th International Conference on Ocean, Offshore and Arctic Engineering (ASME 2017), Trondheim, Norway, 25–30 June 2017. [Google Scholar]
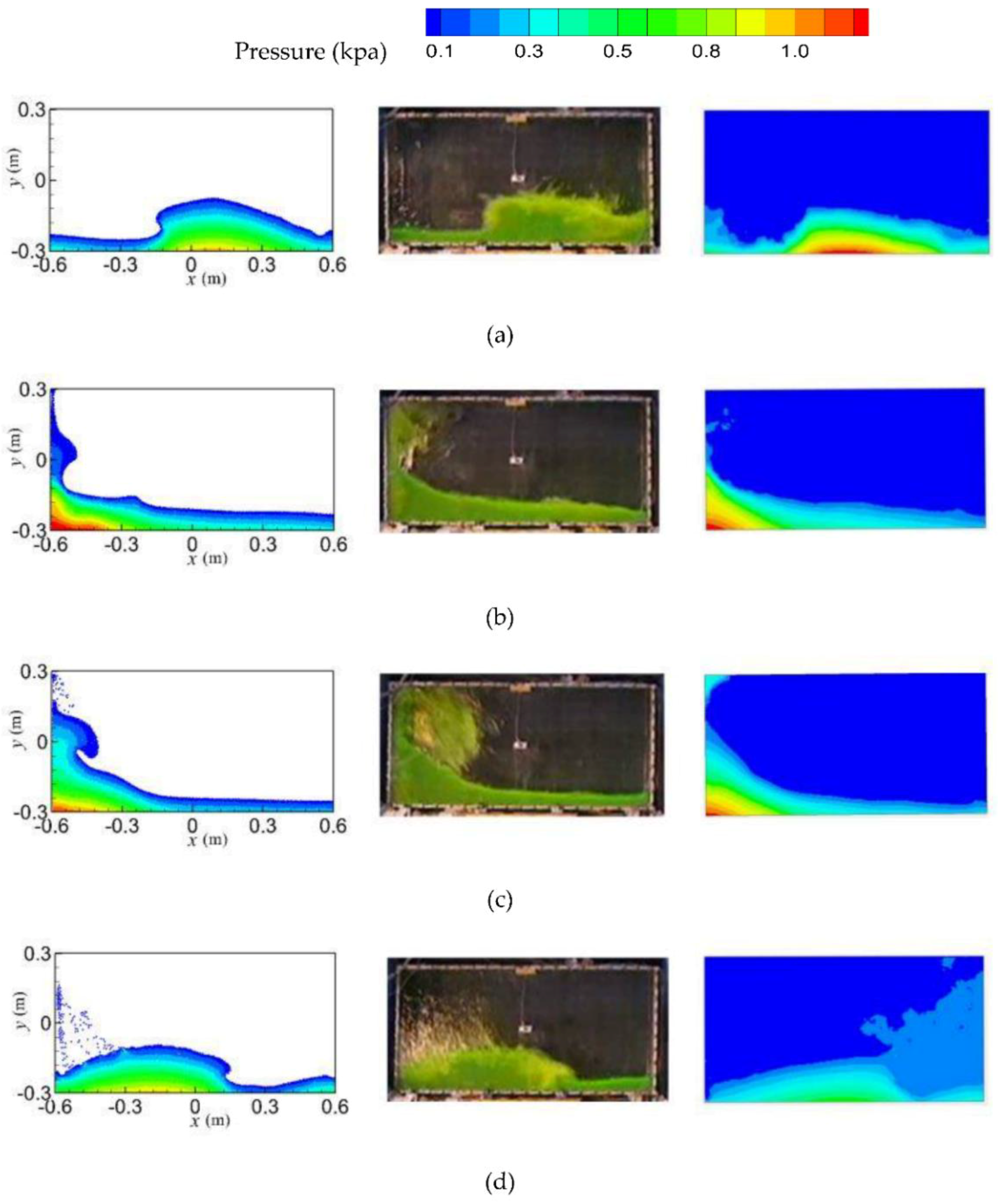
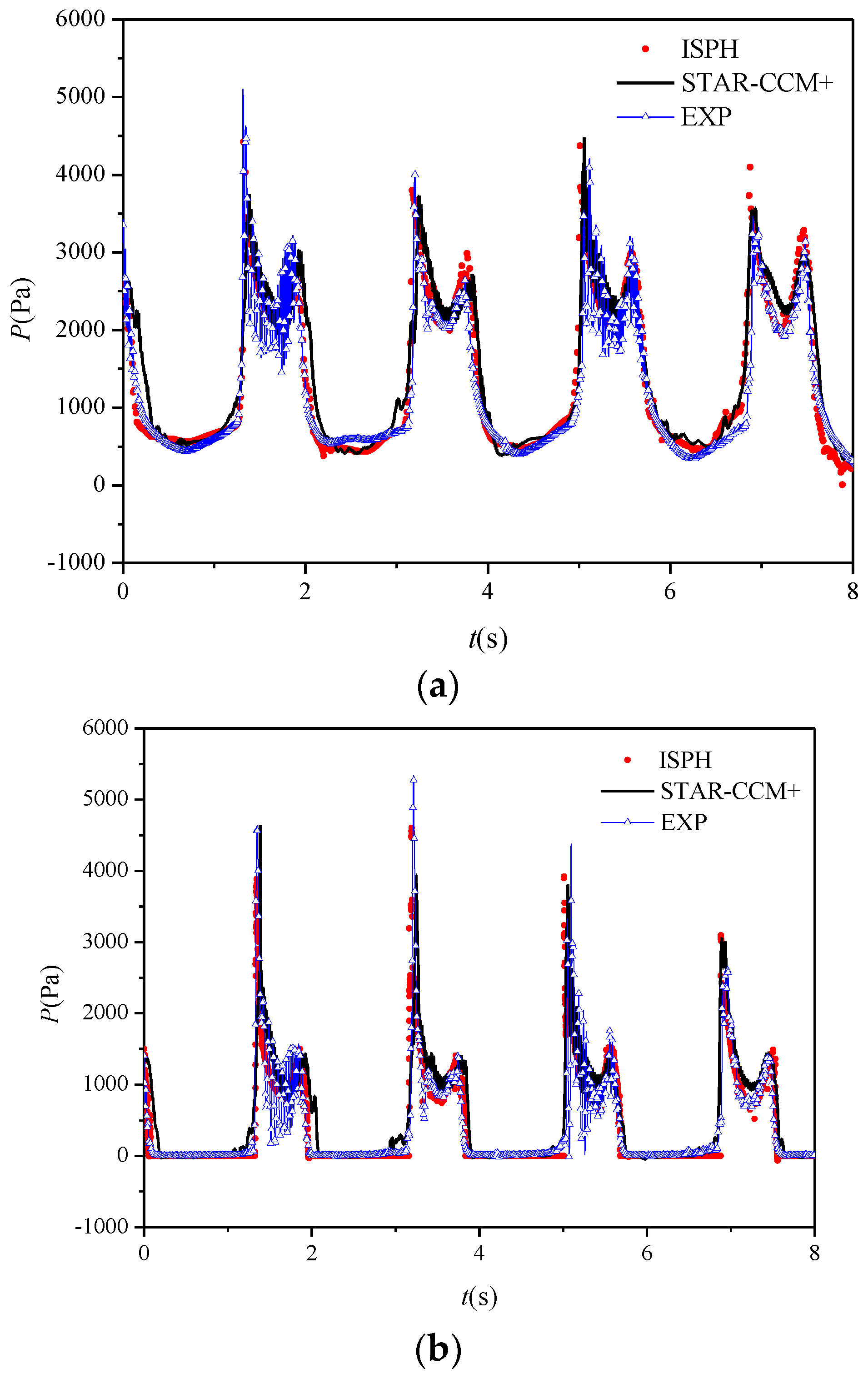




| Models | Grid/Particle No. | Grid/Particle Size (m) | CPU Time (h) | Er (P1) | Er (P2) |
|---|---|---|---|---|---|
| STAR-CCM+ | 11,589 | 0.01 | 11.1 | 2.42% | 3.32% |
| 45,376 | 0.004 | 32.2 | 0.92% | 1.69% | |
| 88,463 | 0.002 | 72.5 | 0.82% | 1.52% | |
| ISPH | 9000 | 0.004 | 1.3 | 1.77% | 1.35% |
| 16,000 | 0.003 | 2.8 | 0.97% | 0.93% | |
| 49,000 | 0.002 | 7.2 | 0.40% | 0.36% |
| Baffle Type | Length | Distance from Bottom | Number of Particle | Excitation Frequency (rad/s) | Ω/Ω0 |
|---|---|---|---|---|---|
| No baffle | ---- | ---- | 22,500 | 1.5–5.0 | 0.3–1.2 |
| One vertical only | 0.083 | ---- | 22,275 | 1.5–5.0 | 0.3–1.2 |
| 0.117 | ---- | 22,165 | |||
| 0.167 | ---- | 22,000 | |||
| 0.217 | ---- | 21,835 | |||
| Two horizontal | 0.167 | 0.117 | 21,825 | 2.0–5.0 | 0.5–1.2 |
| 0.183 | |||||
| 0.250 | |||||
| One vertical and one horizontal | 0.250 | 21,575 | 1.5–5.0 | 0.3–1.2 | |
| One vertical and two horizontal | 0.250 | 21,275 | 1.5–5.0 | 0.3–1.2 | |
| One T-shaped only | 0.083 | ---- | 21,710 | 1.7–4.5 | 0.4–1.1 |
| 0.117 | ---- | 21,600 | |||
| 0.167 | ---- | 21,435 | |||
| 0.217 | ---- | 21,270 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zheng, X.; You, Y.; Ma, Q.; Khayyer, A.; Shao, S. A Comparative Study on Violent Sloshing with Complex Baffles Using the ISPH Method. Appl. Sci. 2018, 8, 904. https://doi.org/10.3390/app8060904
Zheng X, You Y, Ma Q, Khayyer A, Shao S. A Comparative Study on Violent Sloshing with Complex Baffles Using the ISPH Method. Applied Sciences. 2018; 8(6):904. https://doi.org/10.3390/app8060904
Chicago/Turabian StyleZheng, Xing, Yi You, Qingwei Ma, Abbas Khayyer, and Songdong Shao. 2018. "A Comparative Study on Violent Sloshing with Complex Baffles Using the ISPH Method" Applied Sciences 8, no. 6: 904. https://doi.org/10.3390/app8060904
APA StyleZheng, X., You, Y., Ma, Q., Khayyer, A., & Shao, S. (2018). A Comparative Study on Violent Sloshing with Complex Baffles Using the ISPH Method. Applied Sciences, 8(6), 904. https://doi.org/10.3390/app8060904






