Simplified Modeling Strategy for the Thermomechanical Analysis of Massive Reinforced Concrete Structures at an Early Age
Abstract
Featured Application
Abstract
1. Introduction
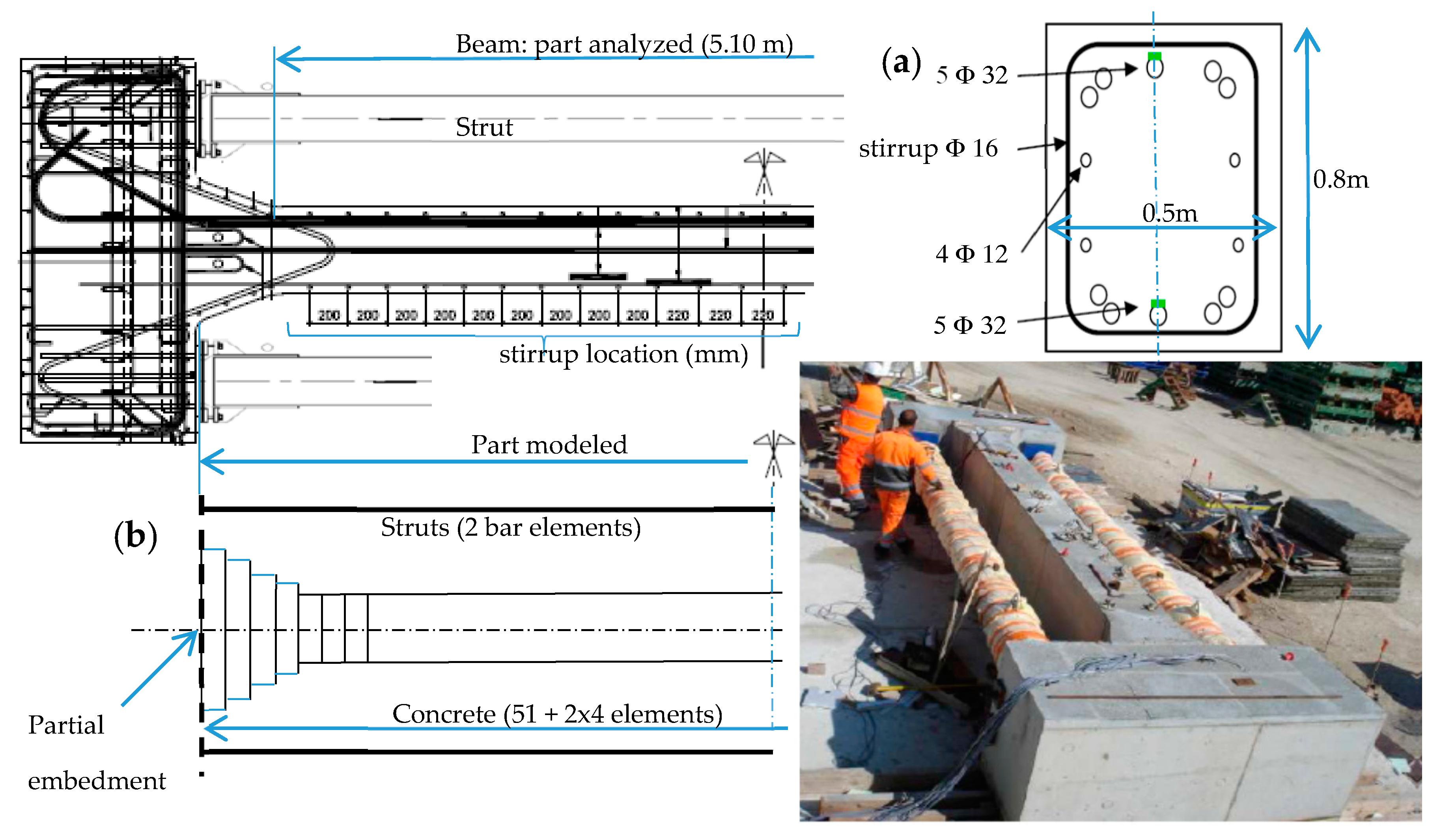
2. The RG8 Test
Blocks Subjected to Restrained Shrinkage
- A central block (5.10 m × 0.80 m × 0.50 m), plus a 0.4-m transition zone on each side with a gradual increase in the width. The reinforcement map is given in Figure 1a; in the cross-section, it includes a combination of 32-mm diameter (10) and 12-mm diameter (4) reinforcements; stirrups are 16 mm in diameter, and they are spaced at 200 mm.
- Two heads (0.9 m × 2.2 m × 0.9 m) serve to support the struts described below;
- Two steel struts fastened on the two heads to restrain strain evolution, as shown in the picture in Figure 1.
3. Models
3.1. Multi-Fiber Beam Modeling
3.2. Models for Concrete
- Concrete maturation;
- Evolution of mechanical performance with maturation;
- Autogenous shrinkage of concrete;
- Basic creep of concrete;
- Thermal deformation of both concrete and steel;
- Elasto-damaging behavior of concrete;
- Elastoplastic behavior of reinforcements;
3.2.1. Early-Age Thermal Model
- the thermal problem was treated independently from the mechanical problem;
- temperature variations were assumed to be identical in each section of the beam (independence with respect to the longitudinal axis x).
- The energy balance equation:
- ξ is the degree of hydration, as given by the Arrhenius equation:
- The external exchange is solved as follows:
3.2.2. Mechanical Model
3.2.3. Thermo-Chemo-Mechanical Coupling
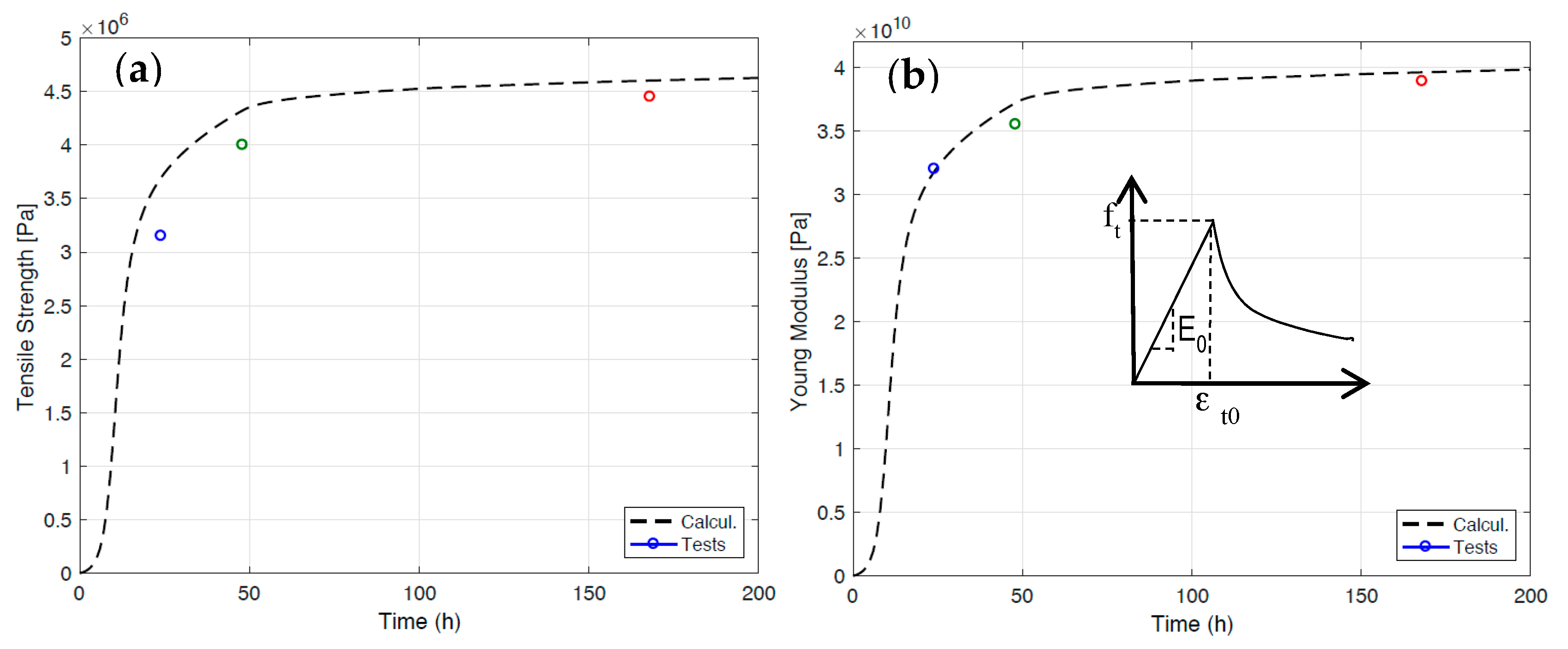
- Young’s modulus: E(M) = ME∞, with E∞ being the value when M = 1 (mature concrete);
- Concrete tensile strength: ft(M) = E(M). ε0t (ε0t is assumed to be constant regardless of the maturity).
3.2.4. Scale Effect
3.2.5. Global Description of the Problem
4. Application to the RG8 Test
4.1. Thermo-Chemo-Mechanical Loading
- From 0 to 120 h, the temperature variation is mostly due to hydration effects. Thanks to the energy balance Equation (1), this evolution has been evaluated; Figure 2a shows good agreement with the in situ experimental measurements both at the center and near the surface of the beam section;
- From 120 h to 700 h (i.e., the time corresponding to strut removal), the temperature variation is mainly due to external temperature evolution (day-night changes). Due to the massiveness of the beam, this change only concerns material close to the surface and, for the sake of simplification, the calculation has been performed with a constant temperature of 12 °C. At the same time, the struts are sensitive to the day-night temperature differential. The cycle lasts 24 h, and in accordance to the measured temperature, the discrepancy is assumed to lie between 9° and 15 °C.
4.1.1. Shrinkage and Creep
- Autogenous shrinkage was calibrated in order to obtain κ∞ (Equation (14)).
- From compression tests loaded at 2 days and 7 days, the basic creep model (Equations (15) and (16)) was also calibrated (see results in Appendix A, Table A1). In order to optimize coverage of the entire test duration, the characteristic times of the three K-V models were: τ1 = 0.1 day, τ2 = 1 day, and τ3 = 10 days.
4.1.2. Maturity and Mechanical Performance of Concrete
4.1.3. Mechanical Loading
4.2. Results
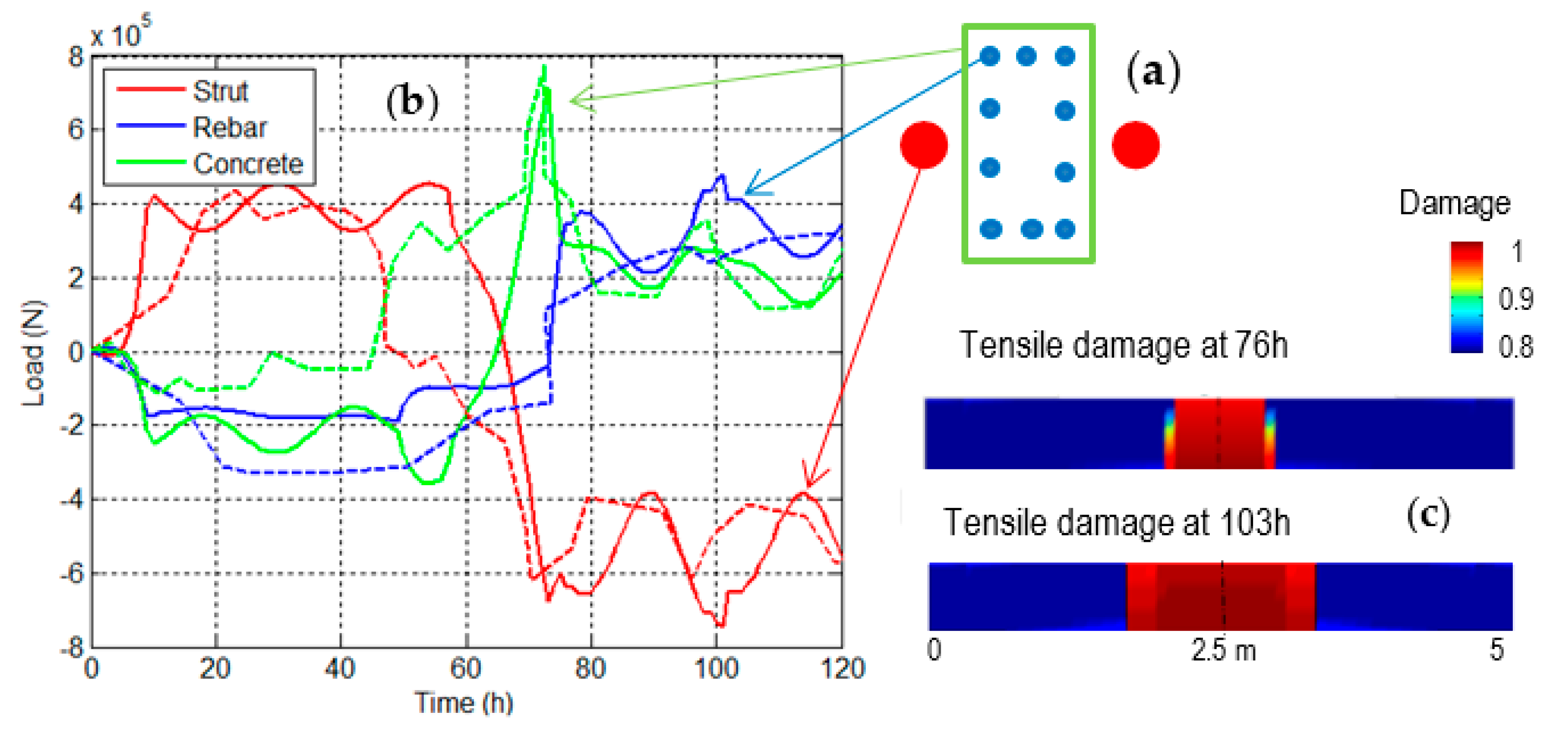
4.2.1. From 0 to 120 h: Maturation and Cracking Period
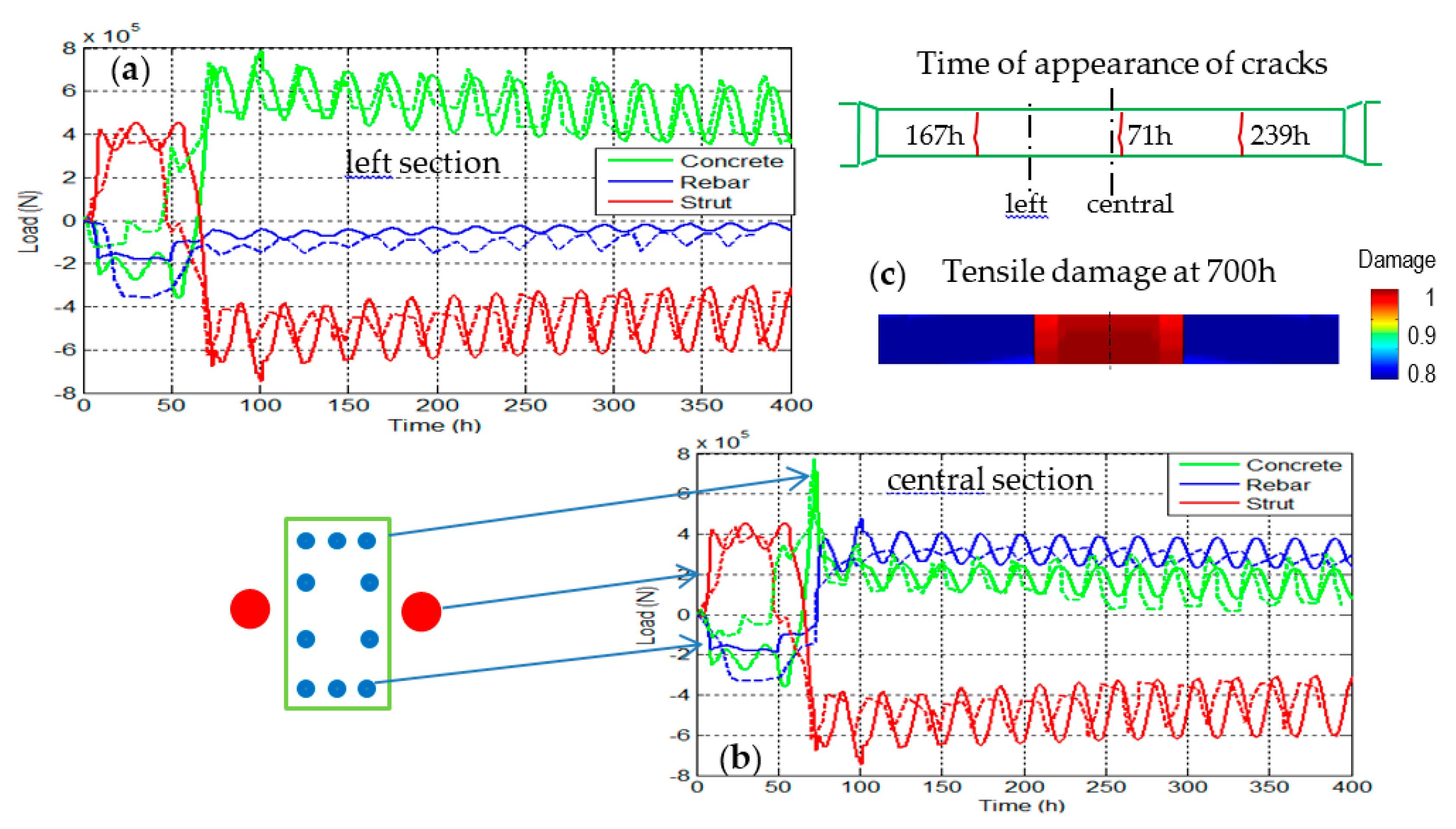
4.2.2. From 0 to 700 h: Restrained Shrinkage
- 3 main cracks are observed during the experiment. The calculation exhibits an initial cracking zone in the beam center at 76 h, as shown in Figure 4c, which is consistent with the first experimental crack appearing at 71 h. At 103 h, the cracking zone extends on both sides at the same time, while the experimental cracks appear on the left side at 167 h and then on the right side at 239 h. It can thus be concluded that the calculation indeed provides for these two cracking phases (left and right of the central zone) but prematurely with respect to the experiment. In the case of the present loading (tension + bending), as demonstrated in [7] for multi-fiber beams, the damage is diffuse and the resultant mesh is insensitive. This diffuse characteristic and the loading mesh symmetry are probably at the origin of the simultaneous appearance of the left and right zones.
- Figure 7a,b display the global responses of all 3 systems (struts, concrete, and rebar). In the central section, once the concrete is cracked, the rebar and struts are the main active systems. In the left section outside the cracking zone, the main active systems are the struts and concrete, as the load supported by the rebar is close to zero. After 120 h, the mean values of these loads are practically constant, and the small cyclic evolution that appears is due to the cyclic day-night temperature variations, which generate a quasi-cyclic load in the struts that is subsequently transmitted to the beam.
4.2.3. Final Stage: 4-Point Bending Loading
- The experimental curve (dashed black line): the beam was only loaded up to the serviceability limit state (SLS).
- The calculated curve for the beam after the restrained shrinkage loading program (blue curve).
- The calculated curve for the same beam considered to be structurally sound (red curve). This loading triggers plasticity on the rebar; the steel behavior considered here is an elastoplastic model with linear hardening (fe = 500 MPa).
- The calculated RG8 curve is in good compliance with the experimental curve.
- The two calculated curves (RG8 and structurally sound beams) are very close during most of the loading evolution.
- A tremendous difference is observed between the initial stiffness values. The initial stiffness is strongly reduced (about 50%) due to concrete cracking produced by the restrained shrinkage. An additional calculation has shown that the first vibration mode is equal to 31.7 Hz instead of 59.2 Hz for the sound beam, which leads to a very different dynamic response (Figure 8c). This decrease must be taken into account in cases of dynamic loading, such as an earthquake.
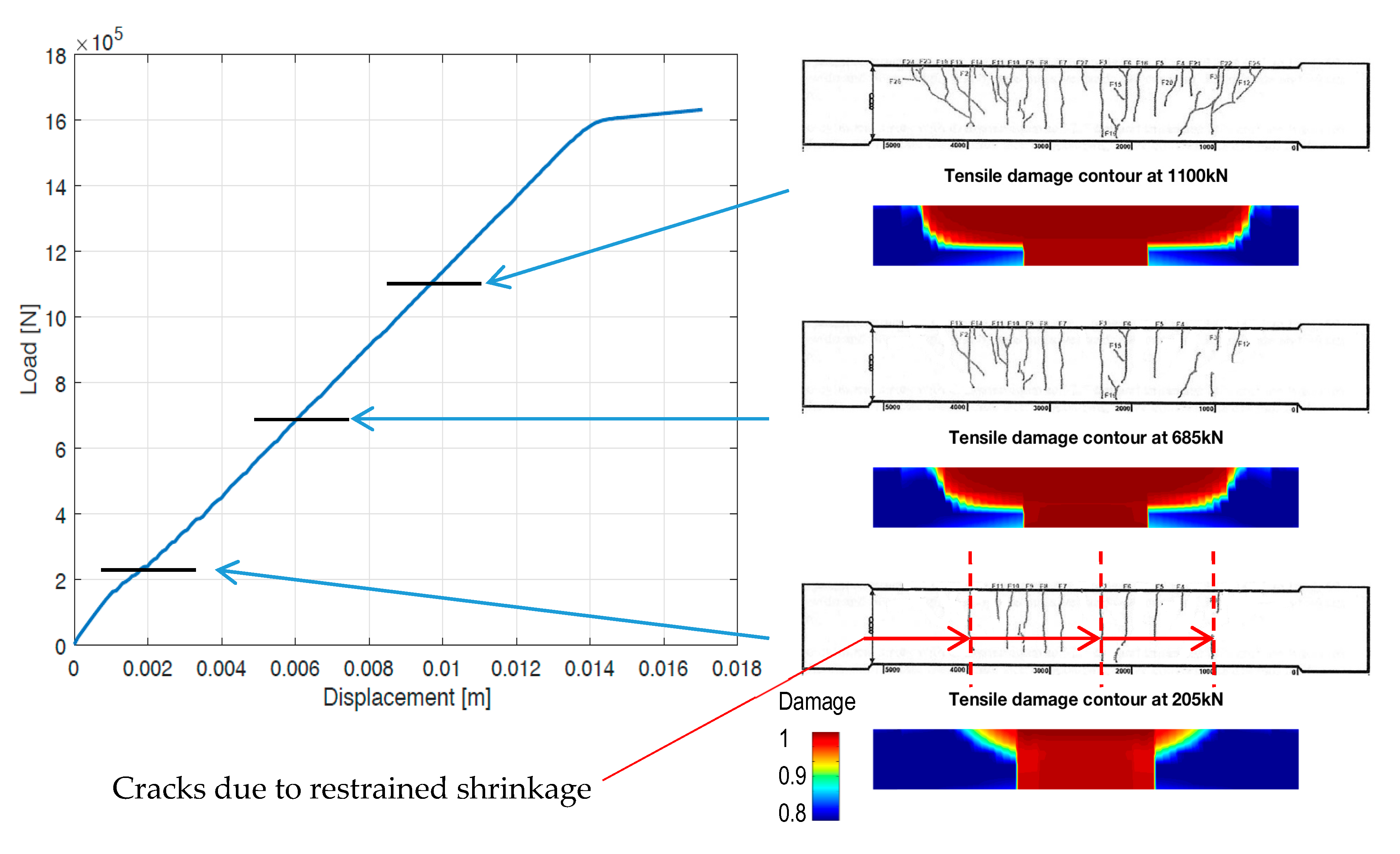
- At a low loading level (205 kN), cracking appears in the center of the beam between cracks due to restrained shrinkage, while the diffuse damage, as developed during the restrained shrinkage phase, is located in the same area and remains relatively unchanged during the first loading phase.
- For the following phases (685 kN and 1100 kN), cracks and damage develop on the upper part of the beam (loading occurs from the bottom up); moreover, the damage contour, which is always diffuse, is quite consistent with the crack pattern.
- The initial stiffness is heavily influenced by the restrained shrinkage treatment. The ultimate beam strength however remains the same, with or without this treatment.
- The calculations have yielded consistent results in terms of both global behavior (load-displacement) and local indicators (damage vs. crack pattern).
5. Conclusions
- On structural durability, such as steel corrosion or the alkali-aggregate reaction, sulfate attack, and freeze-thaw [26].
- On its mechanical response, especially in a dynamic loading, such as an earthquake (in the present case, the first natural frequency was reduced by nearly 50%).
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A
| Concrete at Early-Age | C (KJ/°C·m3) | k | L | h | κ | α | k1bc_∞ | k2bc_∞ | k3bc_∞ |
| (W/mK) | (KJ/m3) | (W/m2K) | (μm/m) | (μm/m°C) | (Gpa) | (Gpa) | (Gpa) | ||
| 2400 | 2.8 | 157,840 | 2 & 10 | 110 | 12 | 3 × 1011 | 9 × 1010 | 2.5 × 1010 | |
| Ai (s−1) | Ap (s−1) | Mp | ζ | ξ0 | ξ∞ | ||||
| 64.85 | 1660 | 0.172 | 75 | 0 | 0.8 | ||||
| Concrete damage model | E∞ (Gpa) | εP | Gf (N/m) | εf | At | Bt | ε/ | Ac | Bc |
| 40 | 0.53 × 10−4 | 60 | 3.5 × 10−4 | 0.815 | 19,250 | 3.75 × 10−4 | 1.5 | 355 | |
| Steel model | Es (GPa) | σy (MPa) | Et (GPa) | α (μm/m°C) | |||||
| 210 | 500 | 5 | 7.5 |
References
- Barre, F.; Bisch, P.; Chauvel, D.; Cortade, J.; Dubois, J.P.; Erlicher, S.; Sellier, A. Control of Cracking in Reinforced Concrete Structures: Research Project CEOS.fr; Mazars, J., Ed.; Wiley: London, UK, 2016. [Google Scholar]
- Buffo-Lacarriere, L.; Rospars, C.; Delaplace, A.; Duong, A.; Jason, L. International Benchmark ConCrack—Synthesis of the Results; Workshop Concrack 2; International Robot Exhibition (IREX): Paris, France, 2011. [Google Scholar]
- Mazars, J. Control of cracking of RC structures: CEOS.fr international benchmark. Eur. J. Environ. Civ. Eng. 2014, 18, 721–844. [Google Scholar] [CrossRef]
- Sellier, A.; Buffo-Lacarrière, L. Towards a simple and unified modelling of basic creep, shrinkage and drying creep of concrete. Eur. J. Environ. Civ. Eng. 2011, 13, 1161–1182. [Google Scholar] [CrossRef]
- Ferreira, D.; Crespo, M.D.; Marí, A.; Bairán, J. Thermo-mechanical simulation of the ConCrack Benchmark RL1 test with a filament beam model. Eng. Struct. 2014, 73, 143–159. [Google Scholar] [CrossRef]
- Briffaut, M.; Benboudjema, F.; Torrenti, J.M.; Nahas, G. Numerical analysis of the thermal active restrained shrinkage ring test to study the early age behaviour of massive concrete structures. Eng. Struct. 2011, 33, 1390–1401. [Google Scholar] [CrossRef]
- Mazars, J.; Grange, S. Simplified Modeling of Reinforced Concrete Structures for Engineering Issues. Comput. Concr. 2015, 16, 683–701. [Google Scholar] [CrossRef]
- Mazars, J.; Pijaudier-Cabot, G.; Saouridis, C. Size effect and continuous damage in cementitious materials. Int. J. Fract. 1991, 51, 159–173. [Google Scholar]
- Swamy, R.; Bandyopadhyay, A.; Stavrides, H. The Ring Method of Measuring Restrained Shrinkage in Mortar and Concrete. Cem. Concr. Aggreg. 1979, 1, 13–20. [Google Scholar]
- Kianoush, M.R.; Acarcan, M.; Ziari, A. Behavior of Base Restrained Reinforced Concrete Walls under Volumetric Change. Eng. Struct. 2008, 30, 1526–1534. [Google Scholar] [CrossRef]
- Raoufi, K.; Pour-Ghaz, M.; Poursaee, A.; Weiss, J. Restrained Shrinkage Cracking in Concrete Elements: Role of Substrate Bond on Crack Development. J. Mater. Civ. Eng. 2011, 23, 895–902. [Google Scholar] [CrossRef]
- Kotronis, P.; Mazars, J. Simplified modelling strategies to simulate the dynamic behaviour of R/C walls. J. Earthq. Eng. 2005, 9, 285–306. [Google Scholar] [CrossRef][Green Version]
- Mazars, J.; Bournazel, J.P. Modelling of damage processes due to volumic variations for maturing and matured concrete. In Concrete from Material to Structure; Malier, Y., Bournazel, J.-P., Eds.; RILEM: Paris, France, 1998; pp. 43–54. [Google Scholar]
- Sciumè, G.; Benboudjema, F.; De Sa, C.; Pesavento, F.; Berthaud, Y.; Schrefler, B. A multi-physics model for concrete at early age applied to repairs problems. Eng. Struct. 2012, 57, 374–387. [Google Scholar] [CrossRef]
- Briffaut, M.; Benboudjema, F.; Torrenti, J.-M.; Nahas, G. Effects of early-age thermal behaviour on damage risks in massive concrete structures. Eur. J. Environ. Civ. Eng. 2012, 16, 589–605. [Google Scholar] [CrossRef]
- Hillerborg, A.; Modeer, M.; Peterssonn, P.E. Analysis of crack formation and growth in concre-te by means of fracture mechanics and finite elements. Cem. Concr. Res. 1976, 6, 773–782. [Google Scholar] [CrossRef]
- Planas, J.; Elices, M.; Guinea, G.V. Measurement of the fracture energy using three-point bend tests: Part 2, influence of bulk energy dissipation. Mater. Struct. 1992, 25, 305–316. [Google Scholar] [CrossRef]
- Bazant, Z.P. Concrete fracture models: Testing and practice. Eng. Fract. Mech. 2002, 69, 165–205. [Google Scholar] [CrossRef]
- Waller, V. Relation Entre Formulation d’un Beton et Propriétés Mécaniques, Formulation et Chaleur D’hydratation, cas de Cendre Volantes. Ph.D. Thesis, Ecole Nationale des Ponts et Chaussées, Paris, France, 1999. [Google Scholar]
- De Schutter, G. Degree of hydration based Kelvin model for the basic creep of early age concrete. Mater. Struct. 1999, 32, 260–265. [Google Scholar] [CrossRef]
- Rossi, P.; Wu, X. Scale effect on concrete in tension. Mater. Struct. 1994, 27, 437–444. [Google Scholar] [CrossRef]
- Sellier, A. Millard, Weakest link and localisation WL2: A method to conciliate probabilistic and energetic scale effects in numerical models. Eur. J. Environ. Civ. Eng. 2014, 18, 1177–1191. [Google Scholar]
- Kuguel, R. A Relation between Theoretical Stress Concentration Factor and Fatigue Notch Factor Deduced from the Concept of Highly Stressed Volume; ASTM: West Conshohocken, PA, USA, 1961; Volume 61, pp. 732–748. [Google Scholar]
- Buffo-Lacarriere, L.; Baron, S.; Barré, F.; Chauvel, D.; Darquennes, A.; Dubois, J.-P.; Gayete, J.; Grondin, F.; Kolani, B.; Torrenti, J.M.; et al. Restrained shrinkage of massive reinforced concrete structures: Results of the project CEOS.fr. Eur. J. Environ. Civ. Eng. 2015, 20, 785–808. [Google Scholar] [CrossRef]
- Cheops Data. Available online: http://www.ceosfr.irex.asso.fr/en/ (accessed on 30 June 2017).
- Tang, S.; Yao, Y.; Andrade, C.; Li, Z.J. Recent durability studies on concrete structure. Cem. Concr. Res. 2015, 78, 143–154. [Google Scholar] [CrossRef]
- Grange, S. ATL4S—A Tool and Language for Simplified Structural Solution Strategy; Internal Report; GEOMAS INSA-Lyon: Villeurbanne, France, 2016. [Google Scholar]




© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mazars, J.; Grange, S.; Briffaut, M. Simplified Modeling Strategy for the Thermomechanical Analysis of Massive Reinforced Concrete Structures at an Early Age. Appl. Sci. 2018, 8, 448. https://doi.org/10.3390/app8030448
Mazars J, Grange S, Briffaut M. Simplified Modeling Strategy for the Thermomechanical Analysis of Massive Reinforced Concrete Structures at an Early Age. Applied Sciences. 2018; 8(3):448. https://doi.org/10.3390/app8030448
Chicago/Turabian StyleMazars, Jacky, Stéphane Grange, and Matthieu Briffaut. 2018. "Simplified Modeling Strategy for the Thermomechanical Analysis of Massive Reinforced Concrete Structures at an Early Age" Applied Sciences 8, no. 3: 448. https://doi.org/10.3390/app8030448
APA StyleMazars, J., Grange, S., & Briffaut, M. (2018). Simplified Modeling Strategy for the Thermomechanical Analysis of Massive Reinforced Concrete Structures at an Early Age. Applied Sciences, 8(3), 448. https://doi.org/10.3390/app8030448





