Decision Optimization for Power Grid Operating Conditions with High- and Low-Voltage Parallel Loops
Abstract
:1. Introduction
2. Complex Network Theory Used for Opening Parallel Loops
2.1. Complex Network Complexity
(a) Structural complexity
(b) Node complexity
(c) Interaction among various complexity factors
2.2. Complex Network Indices
(a) Node degree
(b) Shortest path
(c) Edge betweenness
2.3. Floyd-Warshall Algorithm
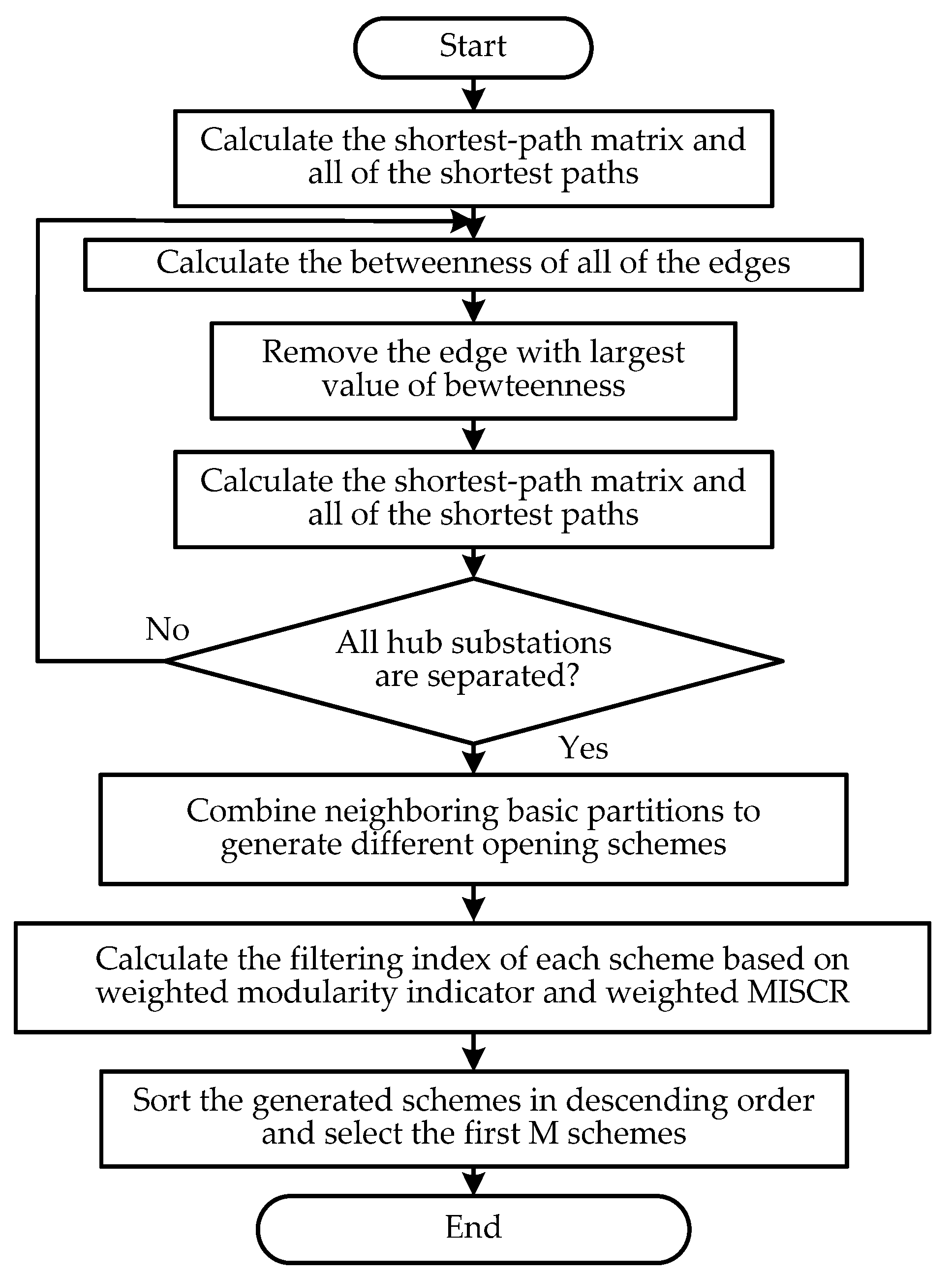
2.4. GN Algorithm
- (a)
- Calculate the betweenness of all edges in the complex network;
- (b)
- Find the edge with the largest betweenness and remove it from the current network; and
- (c)
- Return to step (a) until every node degenerates into a community.
2.5. Modularity Indicator
3. Generation of Parallel Loop Opening Schemes
3.1. Weighted Network and Weighted Modularity Indicator
3.2. Weighted Multi-Infeed Short Circuit Ratio
- (a)
- The weighted factor should not be assigned a value manually;
- (b)
- The weighted factor should represent the interaction between different DC links; and
- (c)
- The weighted factor should represent the contribution to system stability of the specific DC link.
3.3. Generation Process
4. Evaluation for Parallel Loop Opening Schemes
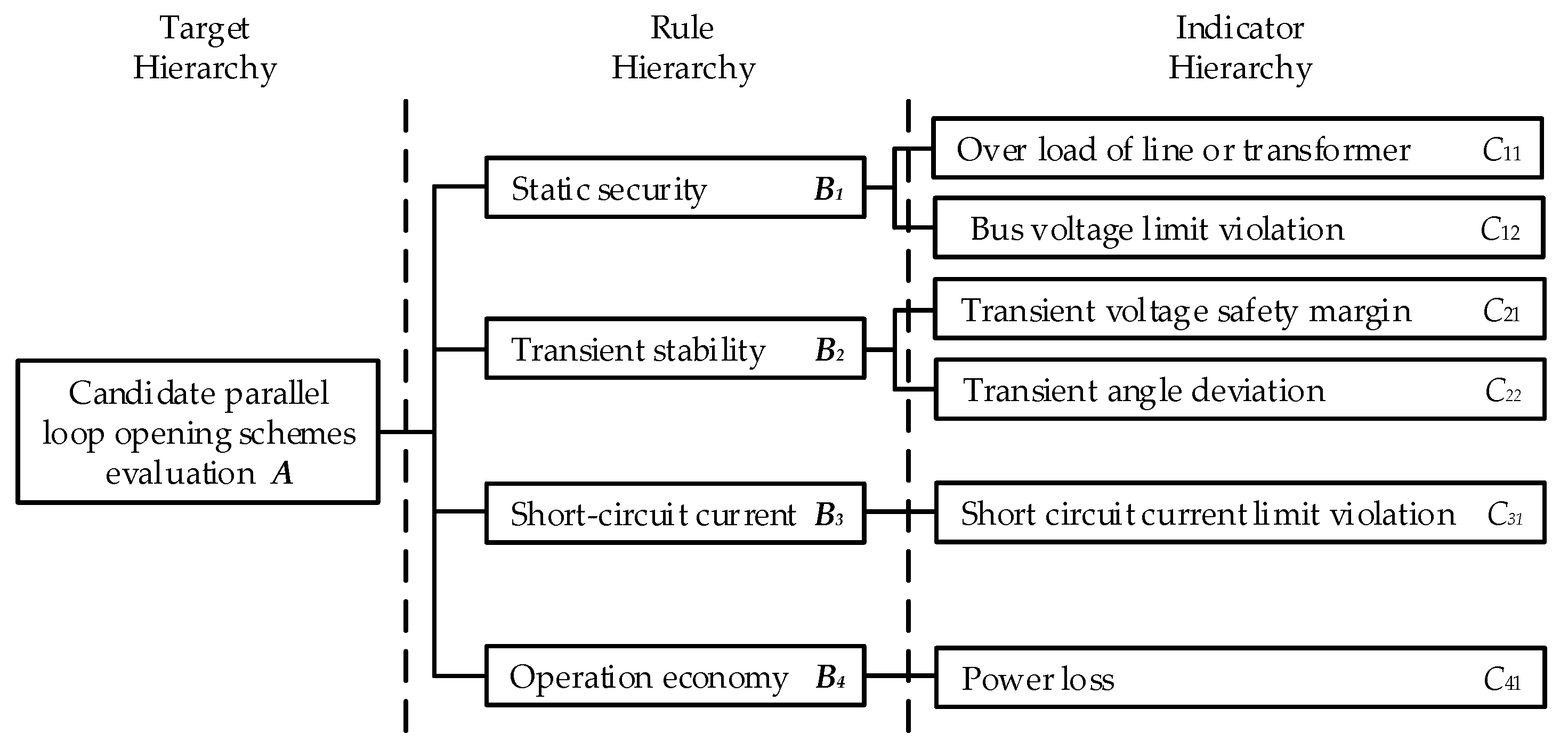
4.1. Comprehensive Evaluation Model
(a) Static security
(b) Transient stability
(c) Short circuit current
(d) Operation economy
4.2. Fuzzy Evaluation Algorithm
5. Case Study
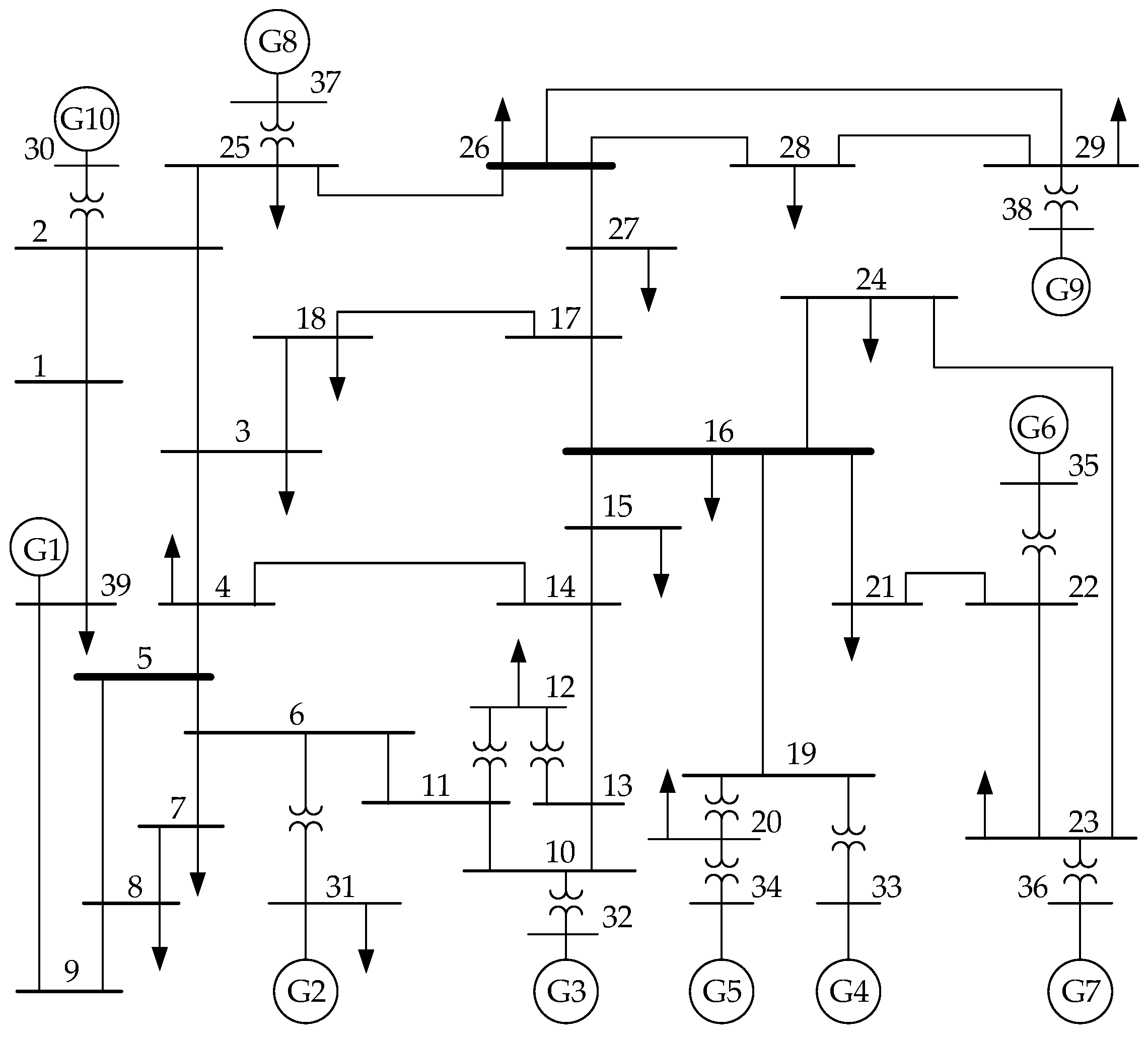
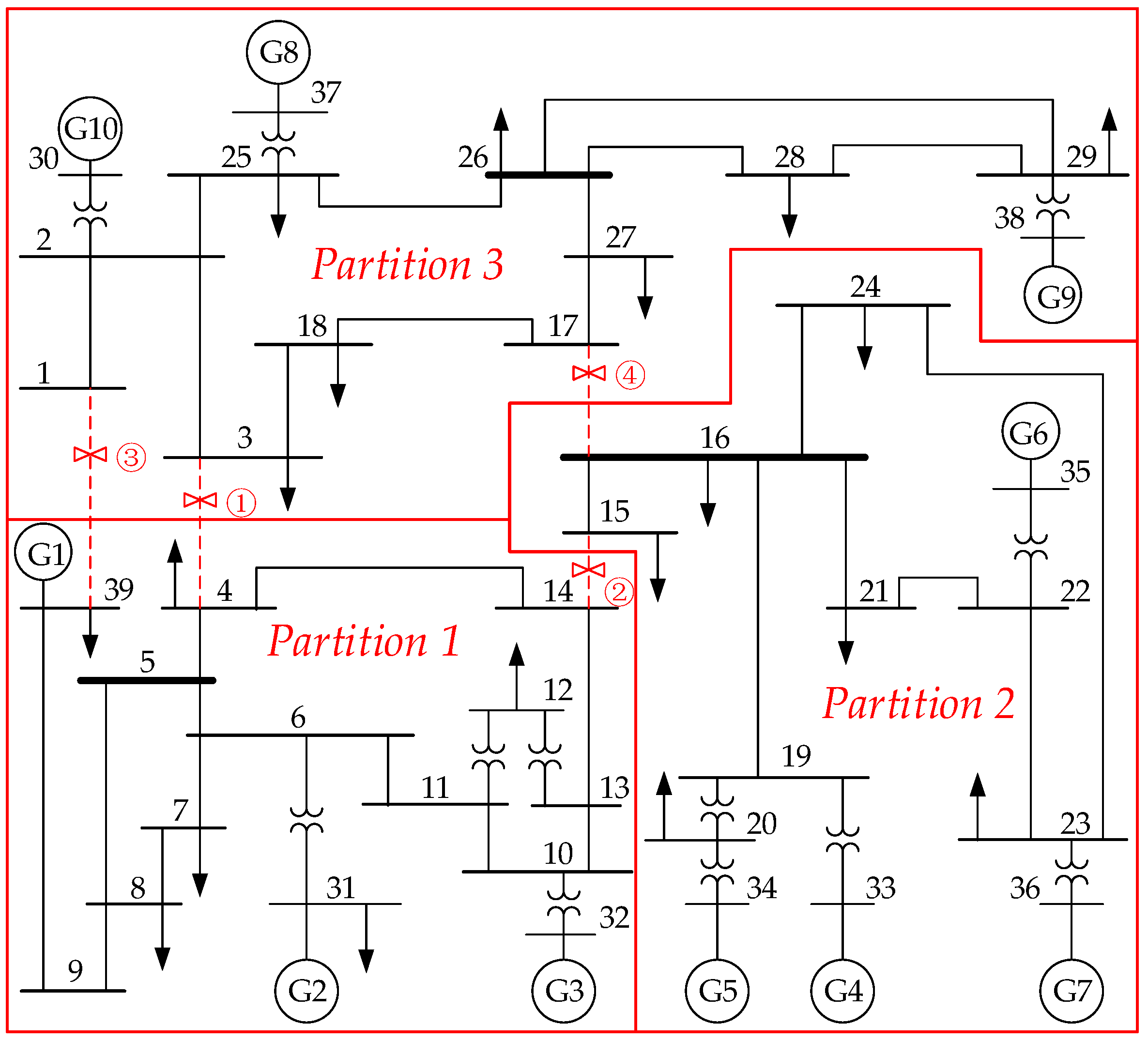
5.1. New England 39-Bus System
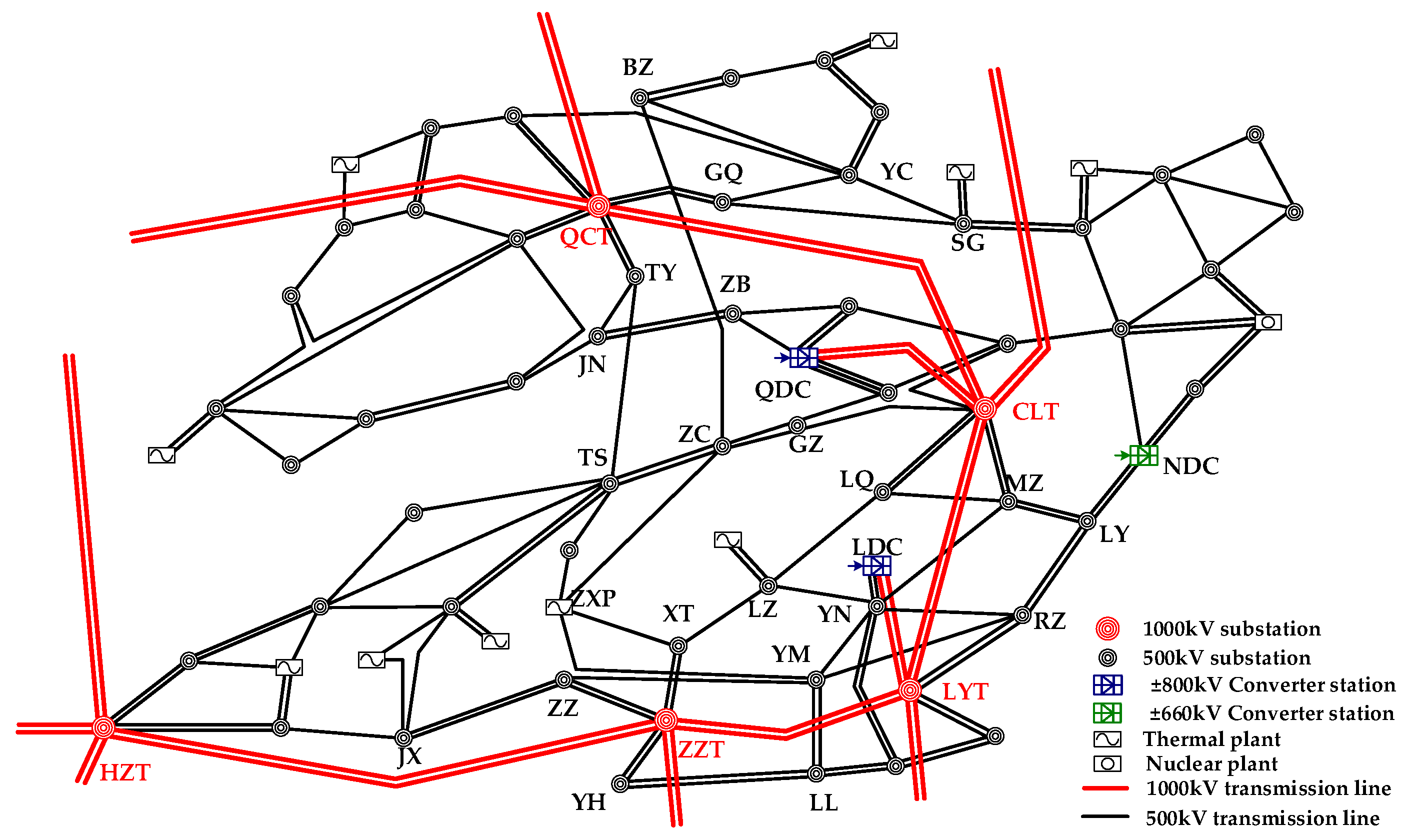
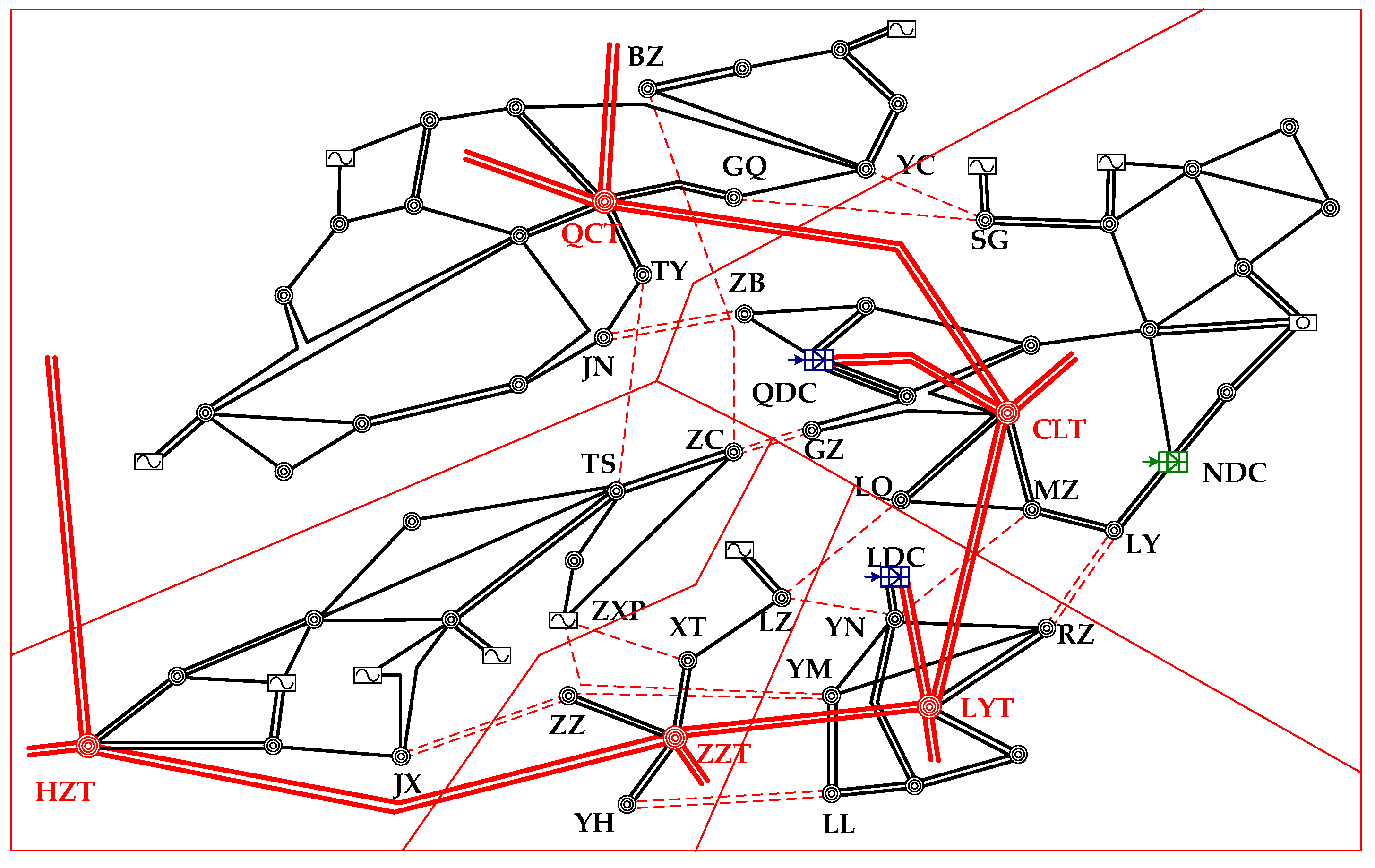
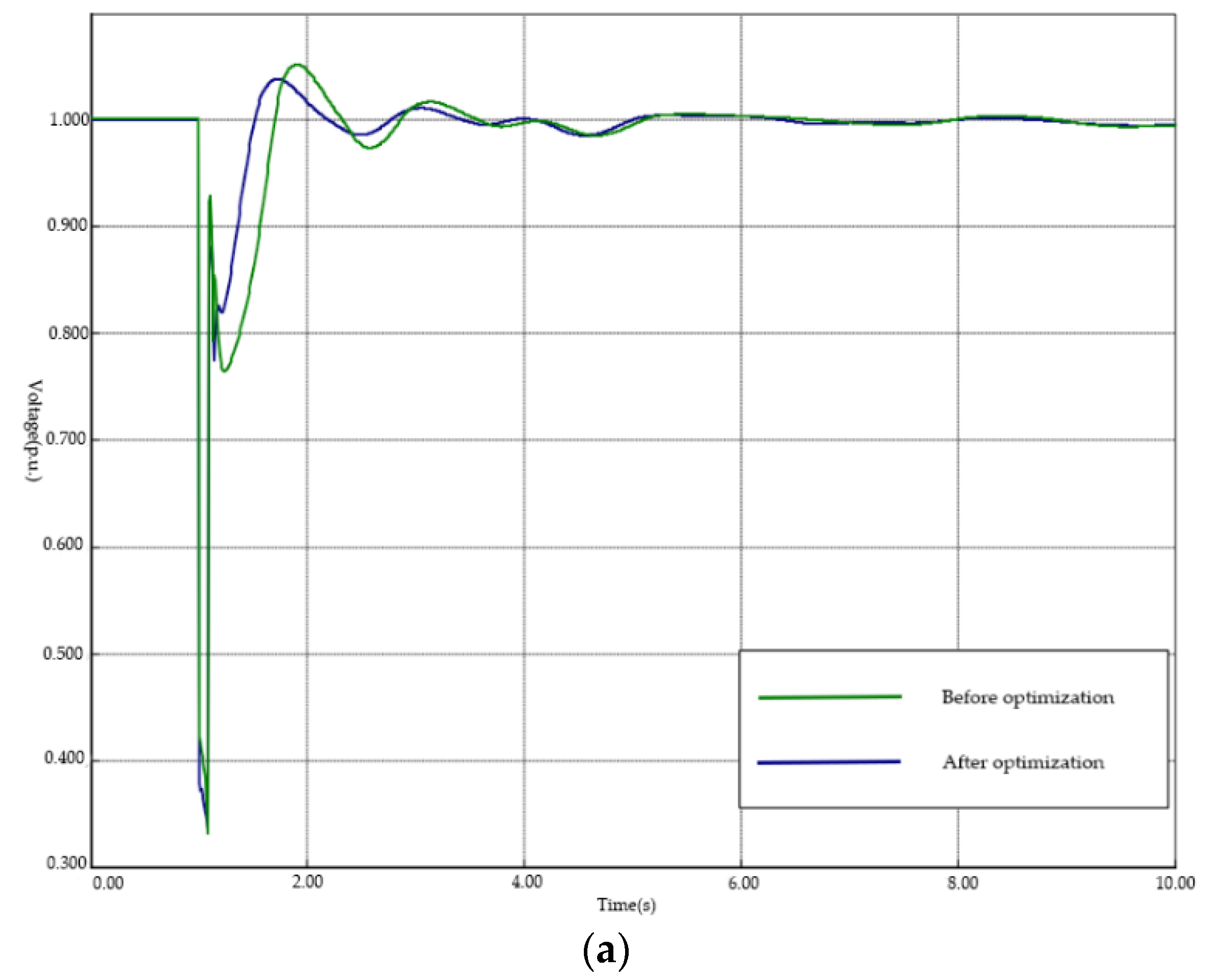
5.2. Actual AC/DC Interconnected Power System
6. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Miller, J.; Balmat, B. Operating problems with parallel flows. IEEE Trans. Power Syst. 1991, 6, 1024–1034. [Google Scholar]
- Huang, D.; Shu, Y.; Ruan, J.; Hu, Y. Ultra high voltage transmission in china: Developments, current status and future prospects. Proc. IEEE. 2009, 97, 555–583. [Google Scholar] [CrossRef]
- Liu, Y.; Fan, R.; Terzija, V. Power system restoration: A literature review from 2006 to 2016. J. Mod. Power Syst. Clean Energy 2016, 4, 332–341. [Google Scholar] [CrossRef]
- Granelli, G.; Montagna, M.; Zanellini, F.; Bresesti, P.; Vailati, R. A genetic algorithm-based procedure to optimize system topology against parallel flows. IEEE Trans Power Syst. 2006, 21, 333–340. [Google Scholar] [CrossRef]
- Makela, O.; Warrington, J.; Morari, M.; Andersson, G. Optimal transmission line switching for large-scale power systems using the alternating direction method of multipliers. In Proceedings of the Power Systems Computation Conference, Wrocław, Poland, 18–22 August 2014. [Google Scholar]
- Hou, L.R.; Chiang, H.D. Toward online line switching method for reducing transmission loss in power systems. In Proceedings of the IEEE Power and Energy Society General Meeting (PESGM), Boston, MA, USA, 17–21 July 2016; pp. 1–5. [Google Scholar]
- Zhao, L.; Zeng, B. Vulnerability analysis of power grids with line switching. IEEE Trans. Power Syst. 2013, 28, 2727–2736. [Google Scholar] [CrossRef]
- Fuller, J.D.; Ramasra, R.; Cha, A. Fast heuristics for transmission-line switching. IEEE Trans. Power Syst. 2012, 27, 1377–1386. [Google Scholar] [CrossRef]
- Li, M.; Luh, P.B.; Michel, L.D.; Zhao, Q.; Luo, X. Corrective line switching with security constraints for the base and contingency cases. IEEE Trans. Power Syst. 2012, 27, 125–133. [Google Scholar] [CrossRef]
- Wu, J.; Cheung, K.W. On selection of transmission line candidates for optimal transmission switching in large power networks. In Proceedings of the IEEE Power & Energy Society General Meeting, Vancouver, BC, Canada, 21–25 July 2013; pp. 1–5. [Google Scholar]
- Bacher, R.; Glavitsch, H. Network topology optimization with security constraints. IEEE Trans. Power Syst. 1986, 1, 103–111. [Google Scholar] [CrossRef]
- Bacher, R.; Glavitsch, H. Loss reduction by network switching. IEEE Trans. Power Syst. 1988, 3, 447–454. [Google Scholar] [CrossRef]
- Shao, W.; Vittal, V. A new algorithm for relieving overloads and voltage violations by transmission line and bus-bar switching. In Proceedings of the 2004 IEEE PES Power Systems Conference and Exposition, NewYork, NY, USA, 10–13 October 2004; pp. 322–327. [Google Scholar]
- Arya, L.D.; Choube, S.C.; Kotharib, D.P. Line switching for alleviating overloads under line outage condition taking bus voltage limits into account. Electr. Power Energy Syst. 2000, 22, 213–221. [Google Scholar] [CrossRef]
- Chen, L.; Tozyo, H.; Tada, Y.; Okamoto, H.; Tanabe, R. Reconfiguration of transmission systems with transient stability constraints. Proceeding of the 2000 IEEE Power Engineering Society Winter Meeting, Singapore, 23–27 January 2000; pp. 1320–1324. [Google Scholar]
- Zhang, N.; Ye, H.; Liu, Y. Decision support for choosing optimal electromagnetic loop circuit opening scheme based on analytic hierarchy process and multi-level fuzzy comprehensive evaluation. Eng. Intell. Syst. Electr. Eng. Commun. 2008, 16, 183–191. [Google Scholar]
- Yang, D.; Zhao, K.; Liu, Y. Coordinated optimization for controlling short circuit current and multi-infeed dc interaction. J. Mod. Power Syst. Clean Energy 2014, 2, 374–384. [Google Scholar] [CrossRef]
- Cormen, T.H.; Leiserson, C.E.; Rivest, R.L.; Stein, C. Introduction to Algorithms; The MIT Press: Cambridge, MA, USA, 2009. [Google Scholar]
- Girvan, M.; Newman, M.E.J. Community structure in social and biological networks. Proc. Natl. Acad. Sci. USA 2002, 99, 7821–7826. [Google Scholar] [CrossRef] [PubMed]
- Newman, M.E.J.; Girvan, M. Finding and evaluating community structure in networks. Phys. Rev. E Stat. Nonlinear Soft Matter Phys. 2004, 69, 026113. [Google Scholar] [CrossRef] [PubMed]
- Santo, F.; Marc, B. Resolution limit in community detection. Proc. Natl. Acad. Sci. USA 2007, 104, 36–41. [Google Scholar]
- Clauset, A.; Newman, M.E.J.; Moore, C. Finding community structure in very large networks. Phys. Rev. E Stat. Nonlinear Soft Matter Phys. 2004, 70, 066111. [Google Scholar] [CrossRef] [PubMed]
- Newman, M.E.J. Analysis of weighted networks. Phys. Rev. E Stat. Nonlinear Soft Matter Phys. 2004, 70, 056131. [Google Scholar] [CrossRef] [PubMed]
- CIGRE. Working Group B4.41. In Systems with Multiple dc Infeed; CIGRE: Paris, France, 2008. [Google Scholar]
- Lin, W.; Tang, Y.; Bu, G.; Shao, Y. Voltage stability analysis of multi-infeed ac/dc power system based on multi-infeed short circuit ratio. Proceeding of the 2010 Power System Technology International Conference (POWERCON), Hangzhou, China, 24–28 October 2010; pp. 1–6. [Google Scholar]
- Zhang, W.; Liu, Y. Multi-objective reactive power and voltage control based on fuzzy optimization strategy and fuzzy adaptive particle swarm. Int. J. Electr. Power Energy Syst. 2008, 30, 525–532. [Google Scholar] [CrossRef]
- Liang, Z.; Yang, K.; Sun, Y.; Yuan, J.; Zhang, H.; Zhang, Z. Decision support for choice optimal power generation projects: Fuzzy comprehensive evaluation model based on the electricity market. Energy Policy 2006, 34, 3359–3364. [Google Scholar] [CrossRef]
- Liu, Z. Global Energy Interconnection; Academic Press: Pittsburgh, PA, USA, 2015. [Google Scholar]
- Wu, D.; Zhang, N.; Kang, C.; Ge, Y.; Xie, Z.; Huang, J. Techno-economic analysis of contingency reserve allocation scheme for combined UHV DC and AC recieving -end power system. CSEE J. Power Energy Syst. 2016, 2, 62–70. [Google Scholar] [CrossRef]

| Branch | Edge Betweenness | |||
|---|---|---|---|---|
| First Partition | Second Partition | Third Partition | Forth Partition | |
| bus1–bus2 | 1.2581 | 1.6293 | 4.0836 | 0.3300 |
| bus1–bus39 | 1.1759 | 1.9265 | 4.9039 | switched off |
| bus2–bus3 | 1.4398 | 0.7426 | 2.0915 | 0.3789 |
| bus2–bus25 | 0.6875 | 0.4990 | 0.7762 | 0.1663 |
| bus3–bus4 | 2.0273 | switched off | switched off | switched off |
| bus3–bus18 | 0.7607 | 0.6539 | 1.7082 | 0.3870 |
| bus4–bus5 | 1.3723 | 0.9619 | 0.8849 | 0.2309 |
| bus4–bus14 | 1.1245 | 1.0598 | 0.6075 | 0.1680 |
| bus5–bus6 | 0.1591 | 0.1382 | 0.1799 | 0.0469 |
| bus5–bus8 | 0.7860 | 0.8421 | 1.6057 | 0.2695 |
| bus6–bus7 | 0.3135 | 0.3503 | 0.6177 | 0.1475 |
| bus6–bus11 | 0.2222 | 0.1975 | 0.6502 | 0.1399 |
| bus7–bus8 | 0.1755 | 0.2124 | 0.3832 | 0.0693 |
| bus8–bus9 | 0.8730 | 1.4367 | 3.3099 | 0.3637 |
| bus9–bus39 | 0.9508 | 1.8014 | 4.7288 | 0.2502 |
| bus10–bus11 | 0.0907 | 0.1209 | 0.1857 | 0.0518 |
| bus10–bus13 | 0.1555 | 0.1598 | 0.0302 | 0.0302 |
| bus13–bus14 | 0.5983 | 0.5678 | 0.2636 | 0.0913 |
| bus14–bus15 | 2.0250 | 2.8089 | switched off | switched off |
| bus15–bus16 | 0.8499 | 1.2654 | 0.2550 | 0.1511 |
| bus16–bus17 | 1.6069 | 2.3926 | 2.6247 | 1.2498 |
| bus16–bus19 | 0.5283 | 0.5283 | 0.5283 | 0.3131 |
| bus16–bus21 | 0.6627 | 0.6627 | 0.6627 | 0.3651 |
| bus16–bus24 | 0.2895 | 0.2895 | 0.2895 | 0.1595 |
| bus17–bus18 | 0.4444 | 0.4938 | 1.0287 | 0.3045 |
| bus17–bus27 | 1.0930 | 1.3706 | 0.7807 | 0.7807 |
| bus21–bus22 | 0.3646 | 0.3646 | 0.3646 | 0.2103 |
| bus22–bus23 | 0.0289 | 0.0289 | 0.0289 | 0.0289 |
| bus23–bus24 | 0.9118 | 0.9118 | 0.9118 | 0.5260 |
| bus25–bus26 | 1.8826 | 1.5904 | 2.1747 | 0.7465 |
| bus26–bus27 | 0.7679 | 1.0337 | 0.7679 | 0.6054 |
| bus26–bus28 | 1.2375 | 1.2375 | 1.2375 | 0.7139 |
| bus26–bus29 | 1.6317 | 1.6317 | 1.6317 | 0.9414 |
| bus28–bus29 | 0.0152 | 0.0152 | 0.0152 | 0.0152 |
| No. | Candidate Opening Scheme | ξ | P |
|---|---|---|---|
| 1 | Partition 1, Partition 2, Partition 3 | 0.5775 | 0.5304 |
| 2 | Partition 1, Partition 2 + Partition 3 | 0.5327 | 0.5102 |
| 3 | Partition 1 + Partition 2, Partition 3 | 0.5101 | 0.4854 |
| 4 | Partition 1 + Partition 3, Partition 2 | 0.5091 | 0.4603 |
| 5 | Open line 2–25, line 14–15 and line 3–18 | 0.4566 | 0.4517 |
| 6 | Open line 1–2, line 3–4, line 14–15 and line 16–17 | 0.4891 | 0.4633 |
| Partition Times | Switched-Off Line | Edge Betweenness |
|---|---|---|
| 1 | BZ-ZC | 5.6125 |
| 2 | TY-TS | 6.3311 |
| 3 | LZ-LQ | 9.2143 |
| 4 | YN-MZ | 7.4017 |
| 5 | ZC-GZ | 8.1541 |
| 6 | RZ-LY | 10.3012 |
| 7 | SG-GQ | 4.2884 |
| 8 | SG-YC | 3.1251 |
| 9 | JN-ZB | 5.3021 |
| 10 | ZXP-YM | 1.2185 |
| 11 | ZXP-XT | 1.8547 |
| 12 | JX-ZZ | 2.5622 |
| 13 | ZZ-YM | 1.0221 |
| 14 | LZ-YN | 0.9836 |
| 15 | YH-LL | 1.3647 |
| Inverter Station | MISCR |
|---|---|
| QDC-L | 3.39 |
| QDC-H | 3.88 |
| LDC-L | 3.40 |
| LDC-H | 3.84 |
| NDC | 4.32 |
| No. | Candidate Opening Scheme | Q′ | M′ | ξ | P |
|---|---|---|---|---|---|
| 1 | QCT+CLT, LYT+ZZT+HZT | 0.6306 | 0.7681 | 0.69935 | 0.5698 |
| 2 | QCT, CLT, LYT+ZZT+HZT | 0.6507 | 0.6528 | 0.65175 | 0.5417 |
| 3 | QCT+HZT+ZZT, CLT+ LYT | 0.5291 | 0.6823 | 0.6057 | 0.5206 |
| 4 | QCT+HZT, CLT+ LYT +ZZT | 0.5213 | 0.7214 | 0.62135 | 0.5257 |
| 5 | Open lines of YC-SG, GQ-SG, JN-ZB, ZC-GZ, XT-LZ, ZXP-YM, ZZ-YM, YH-LL | 0.5118 | 0.6924 | 0.6021 | 0.4798 |
| 6 | Open lines of TY-TS, TS-ZC, ZC-ZXP, LZ-LQ, YN-MZ, RZ-LY | 0.4987 | 0.7422 | 0.62045 | 0.5175 |
| 7 | Original Network | 0.4533 | 0.7825 | 0.6179 | 0.5023 |
| Inverter Station | MISCR |
|---|---|
| QDC-L | 3.50 |
| QDC-H | 3.90 |
| LDC-L | 3.61 |
| LDC-H | 3.88 |
| NDC | 4.30 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, D.; Zhao, K.; Tian, H.; Liu, Y. Decision Optimization for Power Grid Operating Conditions with High- and Low-Voltage Parallel Loops. Appl. Sci. 2017, 7, 487. https://doi.org/10.3390/app7050487
Yang D, Zhao K, Tian H, Liu Y. Decision Optimization for Power Grid Operating Conditions with High- and Low-Voltage Parallel Loops. Applied Sciences. 2017; 7(5):487. https://doi.org/10.3390/app7050487
Chicago/Turabian StyleYang, Dong, Kang Zhao, Hao Tian, and Yutian Liu. 2017. "Decision Optimization for Power Grid Operating Conditions with High- and Low-Voltage Parallel Loops" Applied Sciences 7, no. 5: 487. https://doi.org/10.3390/app7050487
APA StyleYang, D., Zhao, K., Tian, H., & Liu, Y. (2017). Decision Optimization for Power Grid Operating Conditions with High- and Low-Voltage Parallel Loops. Applied Sciences, 7(5), 487. https://doi.org/10.3390/app7050487






