Inverse Identification of the Frequency-Dependent Mechanical Parameters of a Viscoelastic Core Layer Based on the Vibration Response
Abstract
:1. Introduction
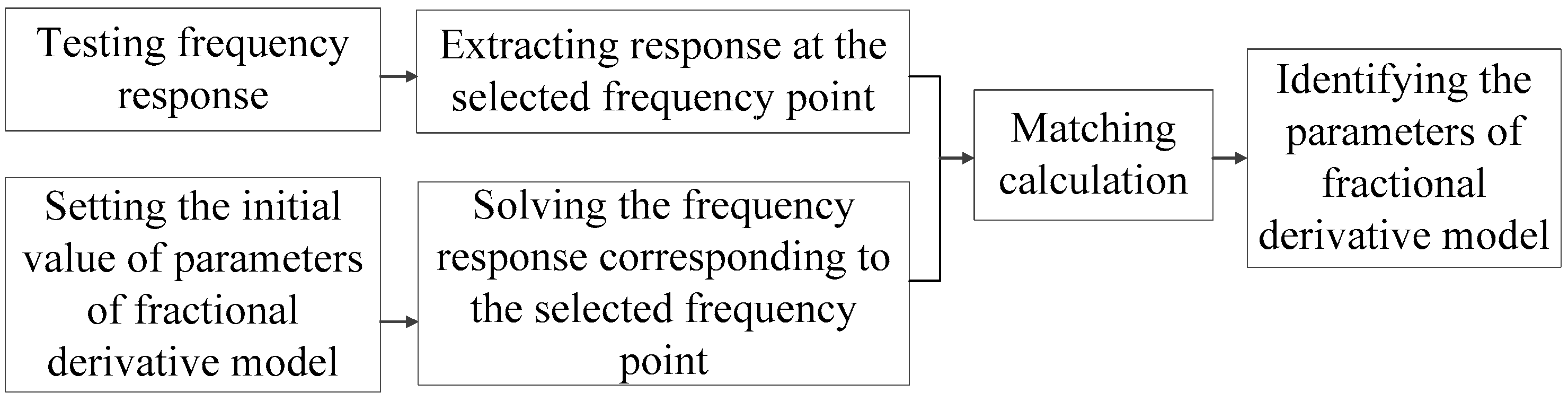
2. The Principle of Inverse Identification
3. Frequency Response Calculation of the CLD Plate Considering Two Kinds of Damping
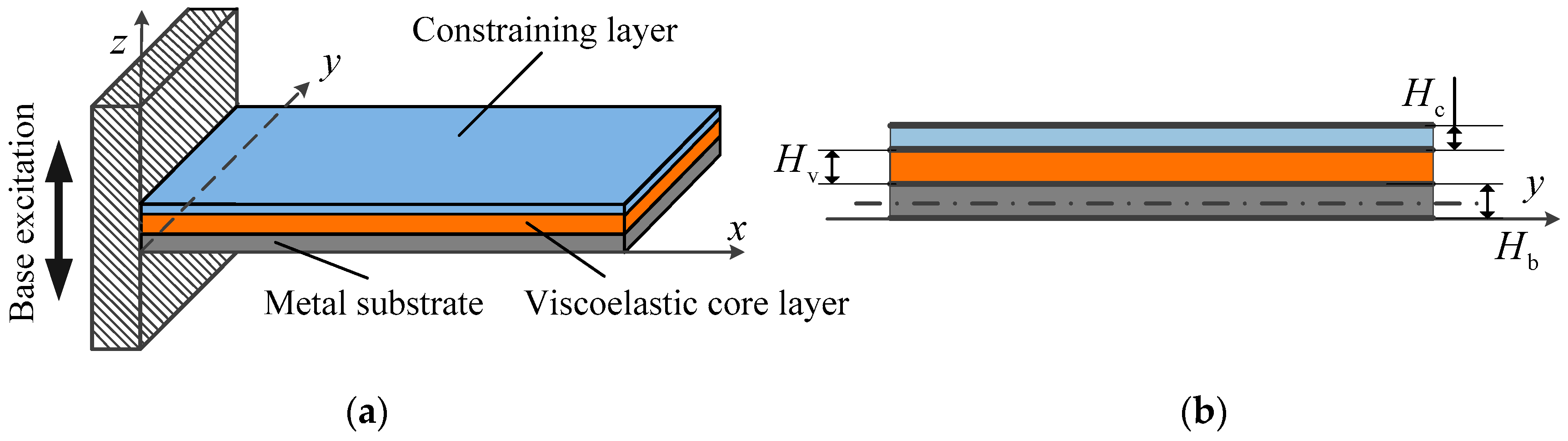
3.1. Derivation of Dynamic Equation of the CLD Plate Under Base Excitation
3.2. Derivation of the Frequency Response
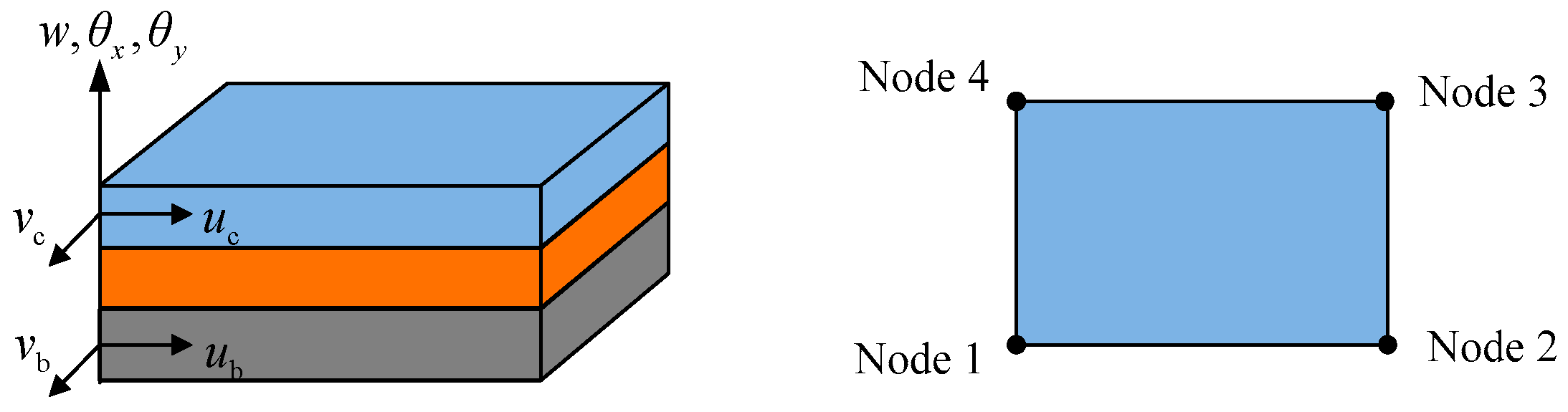
3.3. Derivation of the Mass Matrix and Stiffness Matrix
- (1)
- Dividing the composite thin plate into several elements.
- (2)
- Solving the contribution of each layer to the stiffness and mass matrices of the whole CLD element. The contribution of each layer to the element stiffness matrix can be expressed as:The contribution of each layer to the element mass matrix can be expressed as:where, , , , and are the strain matrices; , , , , , , , , and are the shape function vectors of the element; and , , and refer to the densities of the constraining layer, viscoelastic core layer, and metal substrate, respectively. Symbols , , and represent the elastic matrices of the constraining layer, metal substrate, and viscoelastic core layer, respectively, which are given by:where and are the Young’s modulus of the metal substrate and the constraining layer, respectively; and , , and are the Poisson's ratio of the constraining layer, metal substrate, and viscoelastic core layer, respectively. Here, they are set as invariables.
- (3)
- Determining the mass and stiffness matrices of the whole element. The contribution of each layer to the element stiffness and mass matrices is added, thus forming the stiffness and mass matrices of the CLD element:
- (4)
- Assembling the element stiffness and mass matrices, and forming the total complex stiffness matrix and the total mass matrix M on the basis of the consideration of the cantilever constraints.
3.4. Analysis Examples
4. Matching Calculation Based on the Sensitivity Method
5. Case Study
5.1. Specimens and Frequency Response Test of the CLD Plate
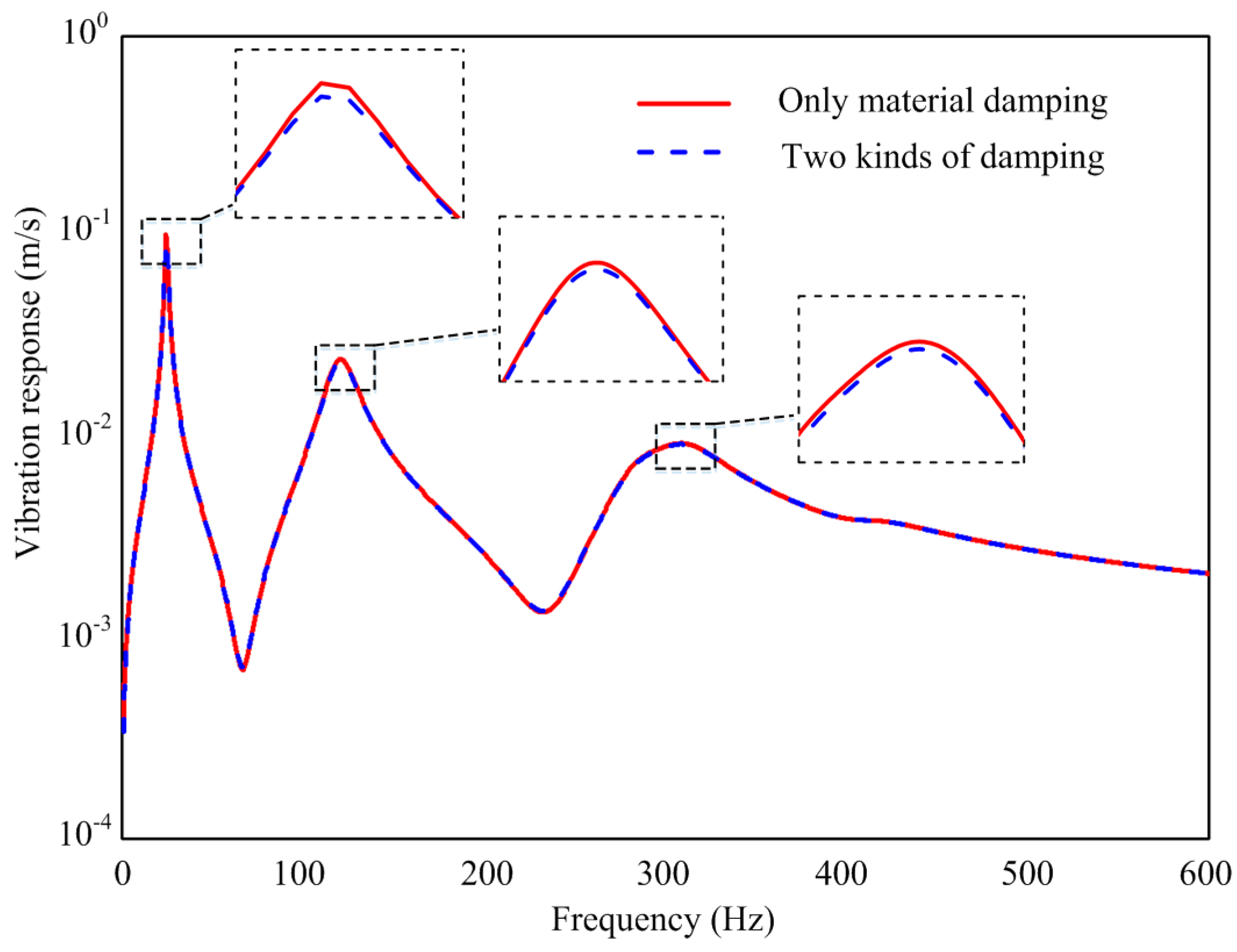
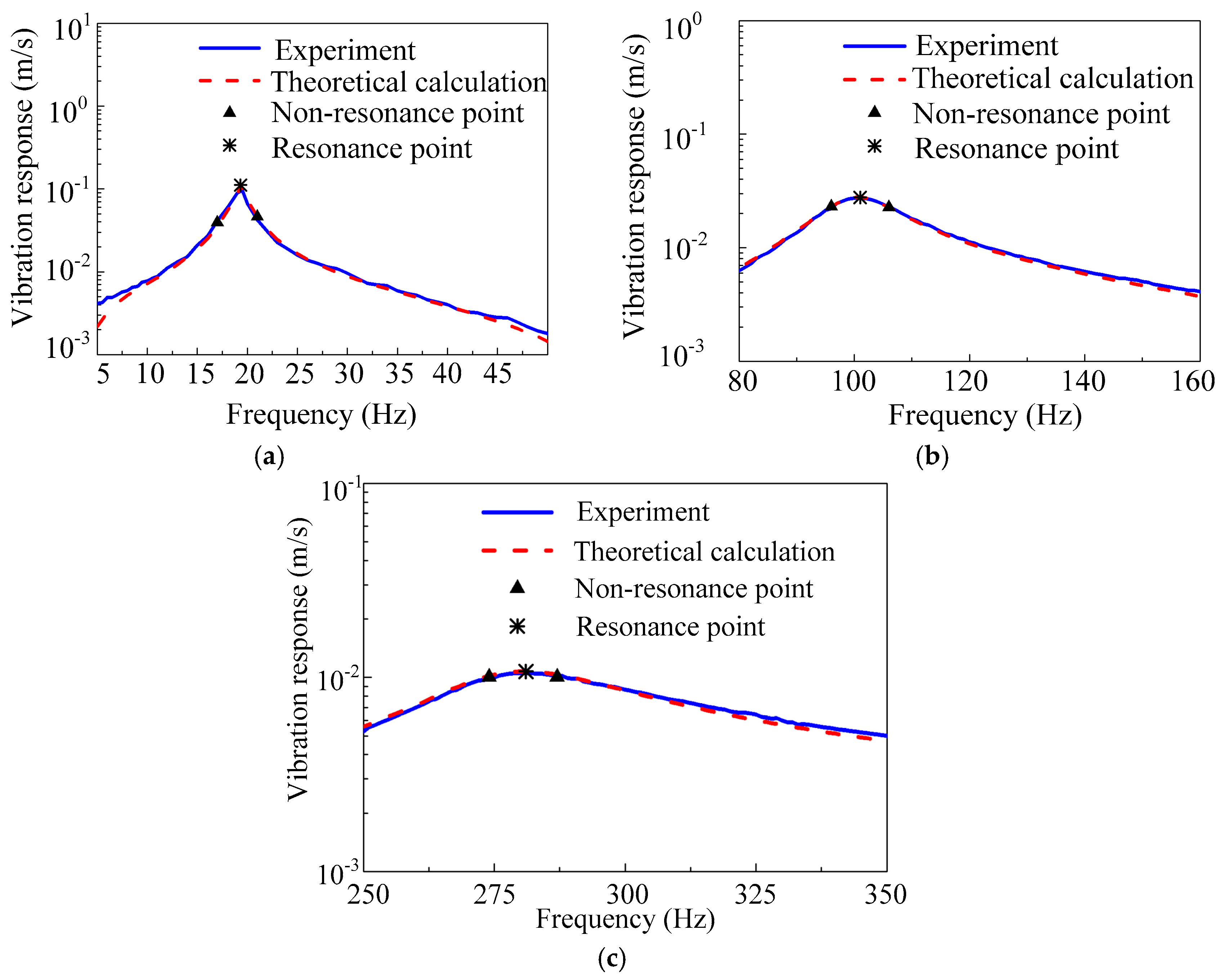
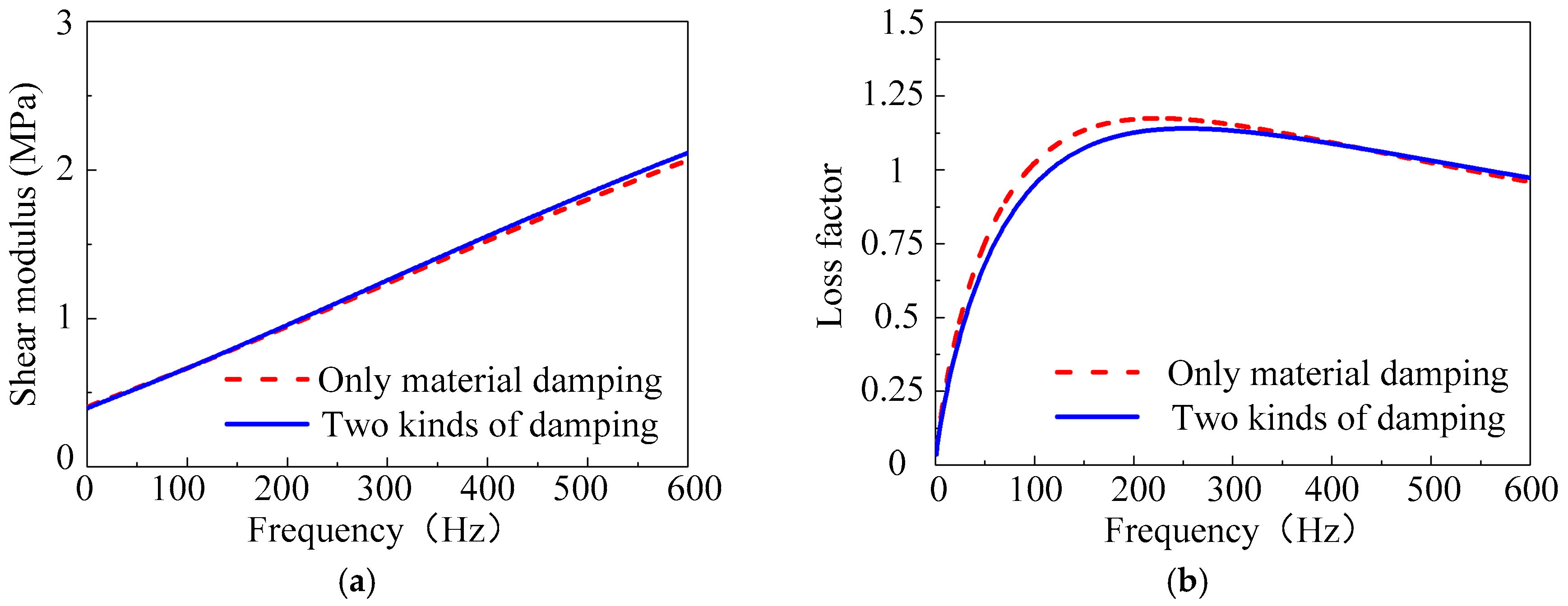
5.2. Parameter Identification of the Viscoelastic Core Layer
6. Conclusions
- (1)
- In the created vibration response analysis model of the CLD plate, both the material damping and the remaining equivalent viscous damping were considered. This kind of damping model is consistent with the real environment of the CLD plate, which is helpful to improve the identification accuracy of the material parameters of the viscoelastic core layer.
- (2)
- The method in which the sweeping frequency response under base excitation is mainly used to identify the mechanical parameters of the viscoelastic core layer was proposed. This identification method avoids the difference caused by the inaccurate location of the exciting point in the hammer test. On the other hand, it can also reduce the interference of noise seen in the identification results to the maximum extent.
- (3)
- Taking the minimum differences between the theoretical and experimental values of the vibration response as the objective function, a matching calculation based on sensitivity was proposed. On the premise of obtaining a reasonable initial value, the parameters of the viscoelastic core layer to be identified can quickly converge.
- (4)
- The CLD plate with ZN_1 viscoelastic material as the core layer was chosen to demonstrate the developed method. The identified viscoelastic mechanical parameters were substituted into the analysis model and the predicted vibration response was compared with the experimental values. The rationality of the identification results was verified.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Moreira, R.A.S. Structural Dynamics and Viscoelastic Passive Damping Treatments. In Modern Mechanical Engineering; Springer: Heidelberg, Berlin, 2014; pp. 89–107. [Google Scholar]
- Lu, J.; Xiang, Y.; Huang, Y.; Li, X.; Ni, Q. Transfer matrix method for analyzing vibration and damping characteristics of rotational shell with passive constrained layer damping treatment. Acta Mech. Solida Sin. 2010, 23, 297–311. [Google Scholar] [CrossRef]
- Balmes, E.; Corus, M.; Baumhauer, S.; Jean, P.; Lombard, J.-P. Constrained viscoelastic damping, test/analysis correlation on an aircraft engine. In Structural Dynamics; Springer: Heidelberg, Berlin, 2011; Volume 3, pp. 1177–1185. [Google Scholar]
- Kumar, A.; Panda, S. Design of a 1–3 viscoelastic composite layer for improved free/constrained layer passive damping treatment of structural vibration. Compos. B Eng. 2016, 96, 204–214. [Google Scholar] [CrossRef]
- Barkanov, E.; Skukis, E.; Petitjean, B. Characterisation of viscoelastic layers in sandwich panels via an inverse technique. J. Sound Vib. 2009, 327, 402–412. [Google Scholar] [CrossRef]
- Martinez-Agirre, M.; Elejabarrieta, M.J. Dynamic characterization of high damping viscoelastic materials from vibration test data. J. Sound Vib. 2011, 330, 3930–3943. [Google Scholar] [CrossRef]
- Cortés, F.; Elejabarrieta, M.J. An approximate numerical method for the complex eigenproblem in systems characterised by a structural damping matrix. J. Sound Vib. 2006, 296, 166–182. [Google Scholar] [CrossRef]
- Hau, L.C.; Fung, E.H.K. Effect of ACLD treatment configuration on damping performance of a flexible beam. J. Sound Vib. 2004, 269, 549–567. [Google Scholar] [CrossRef]
- Roy, H.; Dutt, J.K. Dynamics of polymer and polymer composite rotors—An operator based finite element approach. Appl. Math. Model. 2016, 40, 1754–1768. [Google Scholar] [CrossRef]
- Lampoh, K.; Charpentier, I.; El Mostafa, D. Eigenmode sensitivity of damped sandwich structures. C. R. Méc. 2014, 342, 700–705. [Google Scholar] [CrossRef]
- Schmidt, A.; Gaul, L. Finite element formulation of viscoelastic constitutive equations using fractional time derivatives. Nonlinear Dyn. 2002, 29, 37–55. [Google Scholar] [CrossRef]
- Cortés, F.; Elejabarrieta, M.J. Homogenised finite element for transient dynamic analysis of unconstrained layer damping beams involving fractional derivative models. Comput. Mech. 2007, 40, 313–324. [Google Scholar] [CrossRef]
- Shi, Y.; Sol, H.; Hua, H. Material parameter identification of sandwich beams by an inverse method. J. Sound Vib. 2006, 290, 1234–1255. [Google Scholar] [CrossRef]
- Kim, S.Y.; Lee, D.H. Identification of fractional-derivative-model parameters of viscoelastic materials from measured FRFs. J. Sound Vib. 2009, 324, 570–586. [Google Scholar] [CrossRef]
- Bagley, R.L.; Torvik, P.J. A theoretical basis for the application of fractional calculus to viscoelasticity. J. Rheol. 1983, 27, 201–210. [Google Scholar] [CrossRef]
- Shin, Y.S.; Maurer, G.J. Vibration response of constrained viscoelastically damped plates: Analysis and experiments. Finite Elem. Anal. Des. 1991, 7, 291–297. [Google Scholar] [CrossRef]
- Li, L.; Hu, Y.; Wang, X. Harmonic response calculation of viscoelastic structures using classical normal modes: An iterative method. Comput. Struct. 2014, 133, 39–50. [Google Scholar] [CrossRef]
- Huang, Z.; Qin, Z.; Chu, F. A comparative study of finite element modeling techniques for dynamic analysis of elastic-viscoelastic-elastic sandwich structures. J. Sandw. Struct. Mater. 2016, 18, 531–551. [Google Scholar] [CrossRef]


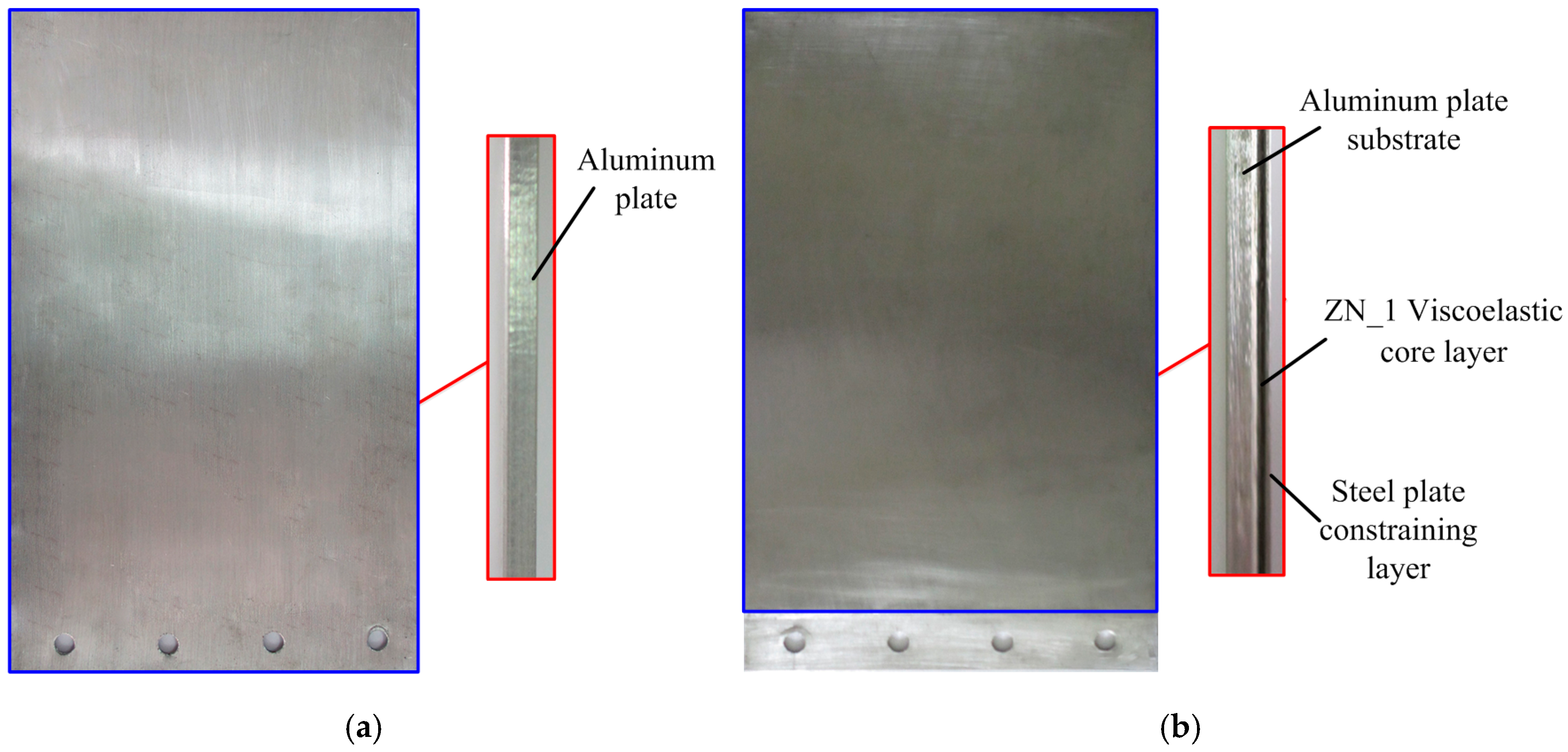
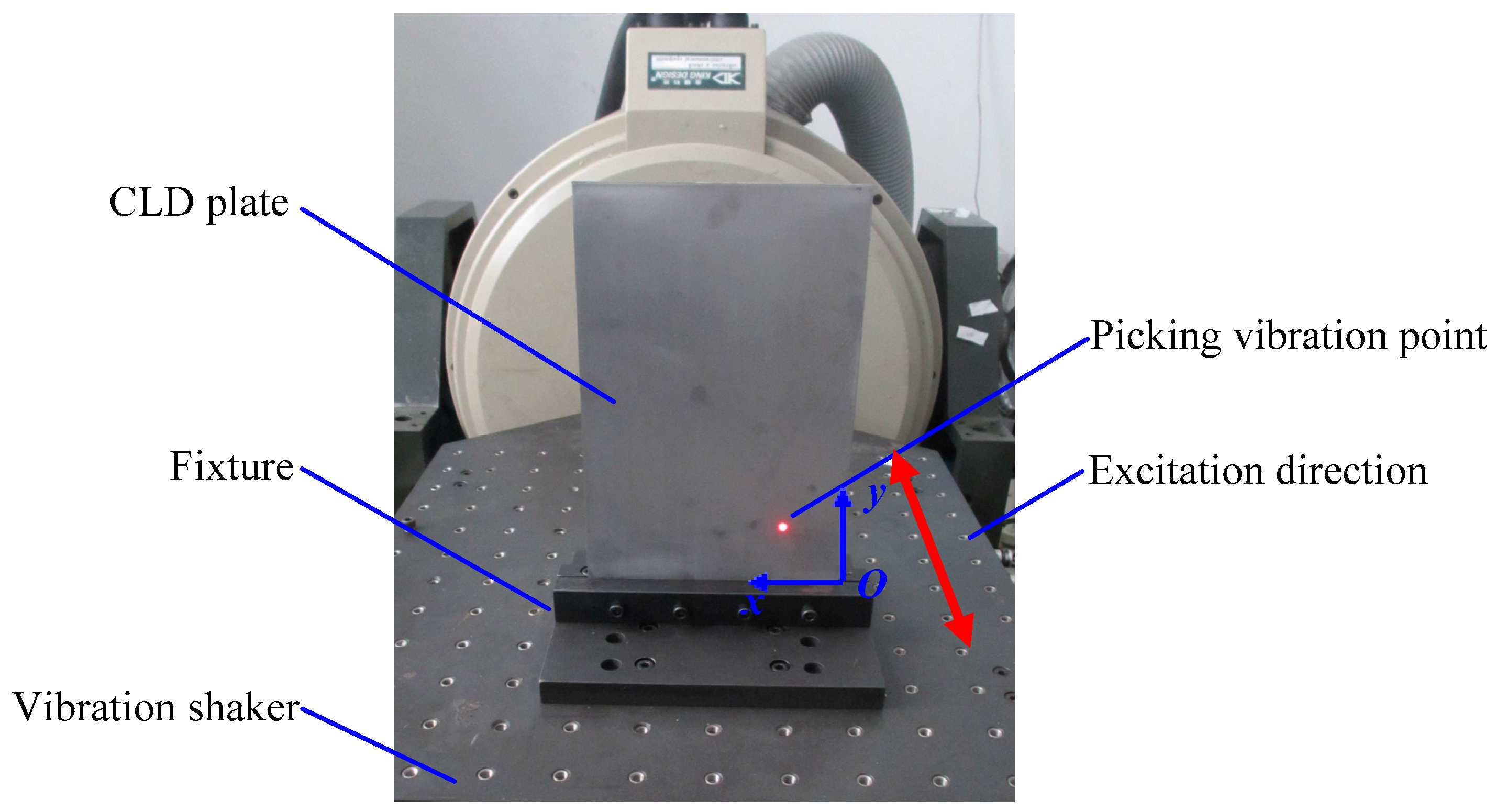
| Type of Materials | Length (mm) | Width (mm) | Thickness (mm) | Storage Modulus (GPa) | Densith (kg/m3) | Poisson‘s Ratio | Loss Factor |
|---|---|---|---|---|---|---|---|
| Aluminum plate substrate | 300.2 | 199.9 | 1.9 | 6.89 | 2700 | 0.3 | 0 |
| Steel constraining layer | 300.2 | 199.9 | 0.3 | 209 | 7890 | 0.3 | 0 |
| Viscoelastic core layer ZN_1 | 300.2 | 199.9 | 0.5 | - | 789.5 | 0.49 | - |
| Number | Name |
|---|---|
| 1 | KINGDESIGN EM-1000F vibration shaker |
| 2 | LMS SCADAS mobile front-end |
| 3 | PCB 8206-001 54627 modal hammer |
| 4 | Polytec PDV-100 laser vibrometer |
| 5 | LMS.Testlab workstation |
| Order | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
|---|---|---|---|---|---|---|---|---|
| Modal damping ratio | 0.29 | 0.13 | 0.18 | 0.21 | 0.11 | 0.07 | 0.09 | 0.04 |
| Parameters | ||||
|---|---|---|---|---|
| Identified value | 0.27 MPa | 4.1 MPa | 1.21 × | 0.83 |
| Frequency Point (Hz) | Experiment () | Theoretical Calculation () | Difference |
|---|---|---|---|
| 18.4 | 0.0726 | 0.0708 | 2.48 |
| 19.3 * | 0.1112 | 0.1128 | 1.44 |
| 20.2 | 0.0705 | 0.0729 | 3.40 |
| 94.3 | 0.0197 | 0.0206 | 4.57 |
| 100.5 * | 0.0279 | 0.0276 | 1.08 |
| 105.0 | 0.0240 | 0.0229 | 4.58 |
| 264.8 | 0.0082 | 0.0086 | 4.87 |
| 281.1 * | 0.0107 | 0.0109 | 1.58 |
| 295.2 | 0.0092 | 0.0088 | 4.99 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, W.; Wang, Z.; Liu, R.; Yan, X. Inverse Identification of the Frequency-Dependent Mechanical Parameters of a Viscoelastic Core Layer Based on the Vibration Response. Appl. Sci. 2017, 7, 455. https://doi.org/10.3390/app7050455
Sun W, Wang Z, Liu R, Yan X. Inverse Identification of the Frequency-Dependent Mechanical Parameters of a Viscoelastic Core Layer Based on the Vibration Response. Applied Sciences. 2017; 7(5):455. https://doi.org/10.3390/app7050455
Chicago/Turabian StyleSun, Wei, Zhuo Wang, Rong Liu, and Xianfei Yan. 2017. "Inverse Identification of the Frequency-Dependent Mechanical Parameters of a Viscoelastic Core Layer Based on the Vibration Response" Applied Sciences 7, no. 5: 455. https://doi.org/10.3390/app7050455
APA StyleSun, W., Wang, Z., Liu, R., & Yan, X. (2017). Inverse Identification of the Frequency-Dependent Mechanical Parameters of a Viscoelastic Core Layer Based on the Vibration Response. Applied Sciences, 7(5), 455. https://doi.org/10.3390/app7050455





