Analysis Method of Articulated Torque of Heavy-Duty Six-Legged Robot under Its Quadrangular Gait
Abstract
:1. Introduction
2. Walking Ways of Heavy-Duty Six-Legged Robot under Quadrangular Gait
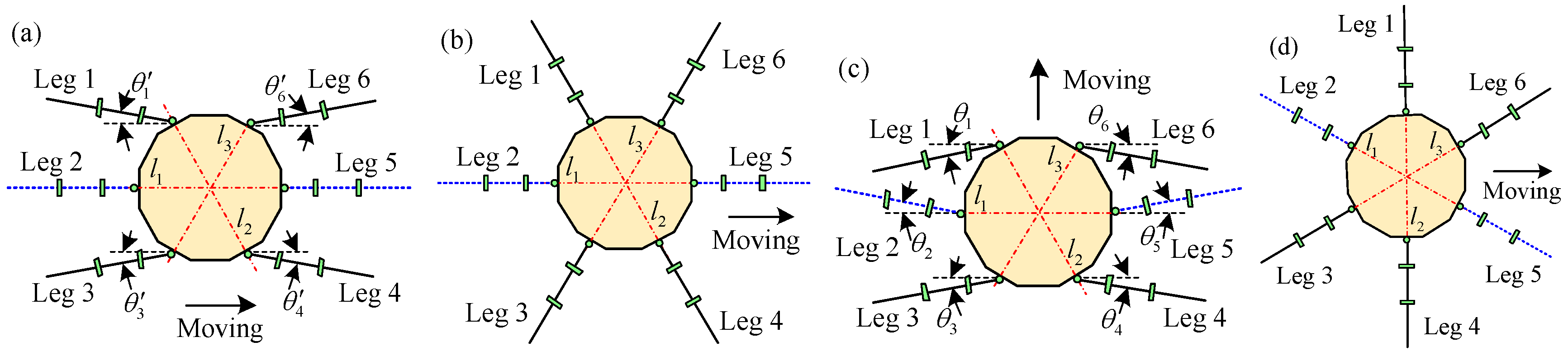
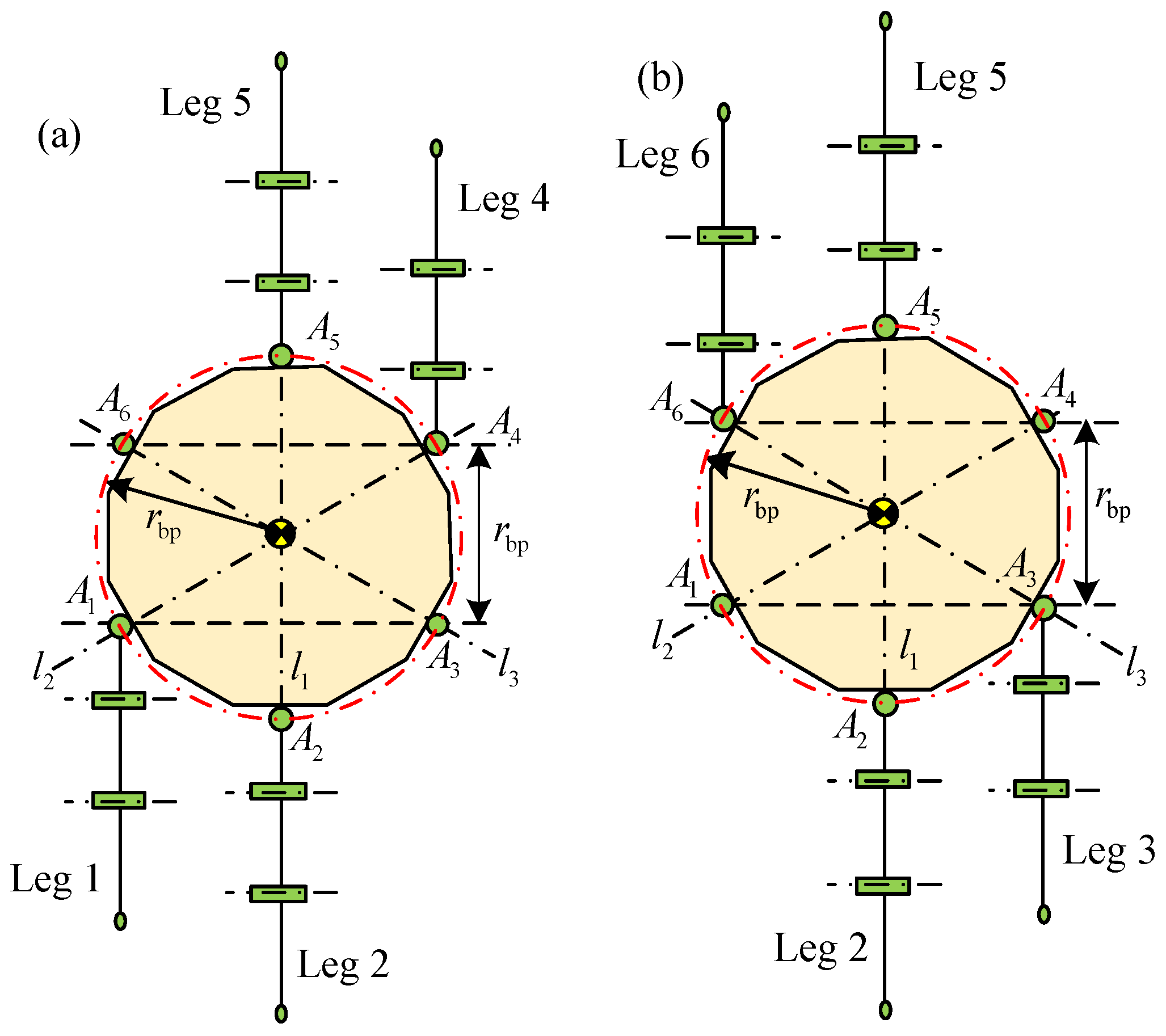
2.1. Typical Walking Ways of Robot under Quadrangular Gait
2.2. Support Phase Analysis of Crab-Type Quadrangular Gait
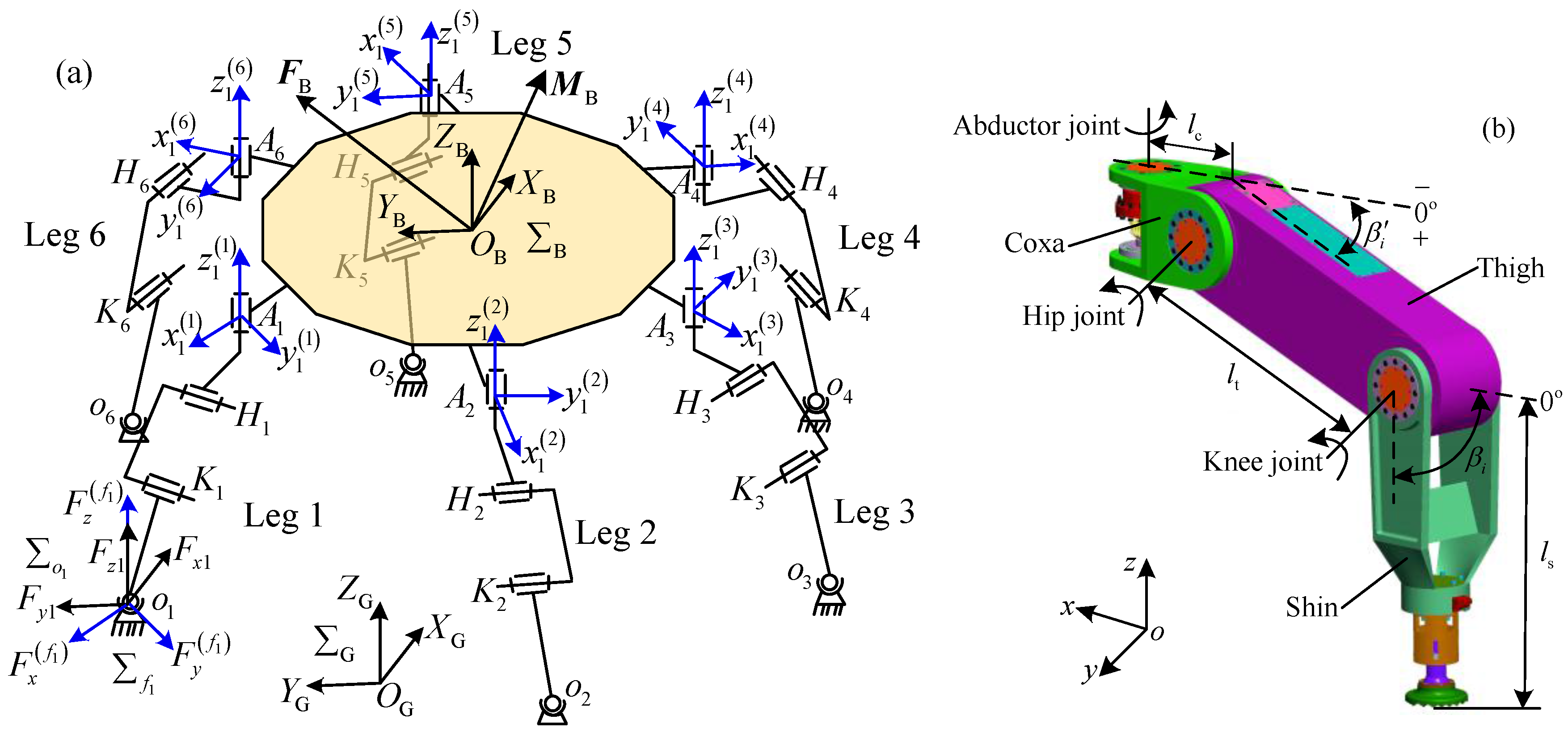
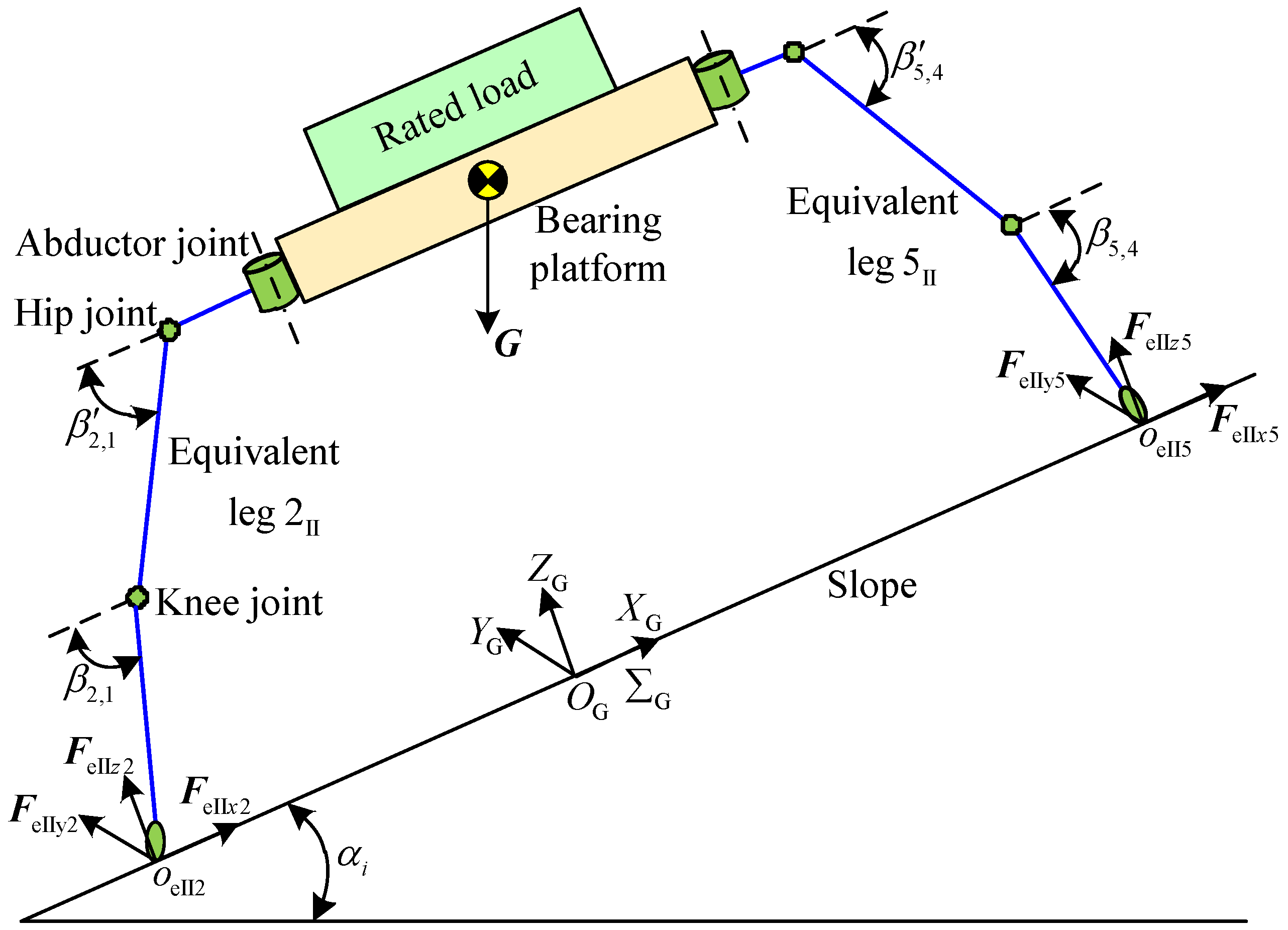
3. Static Torque Analysis of Hip Joint and Knee Joint
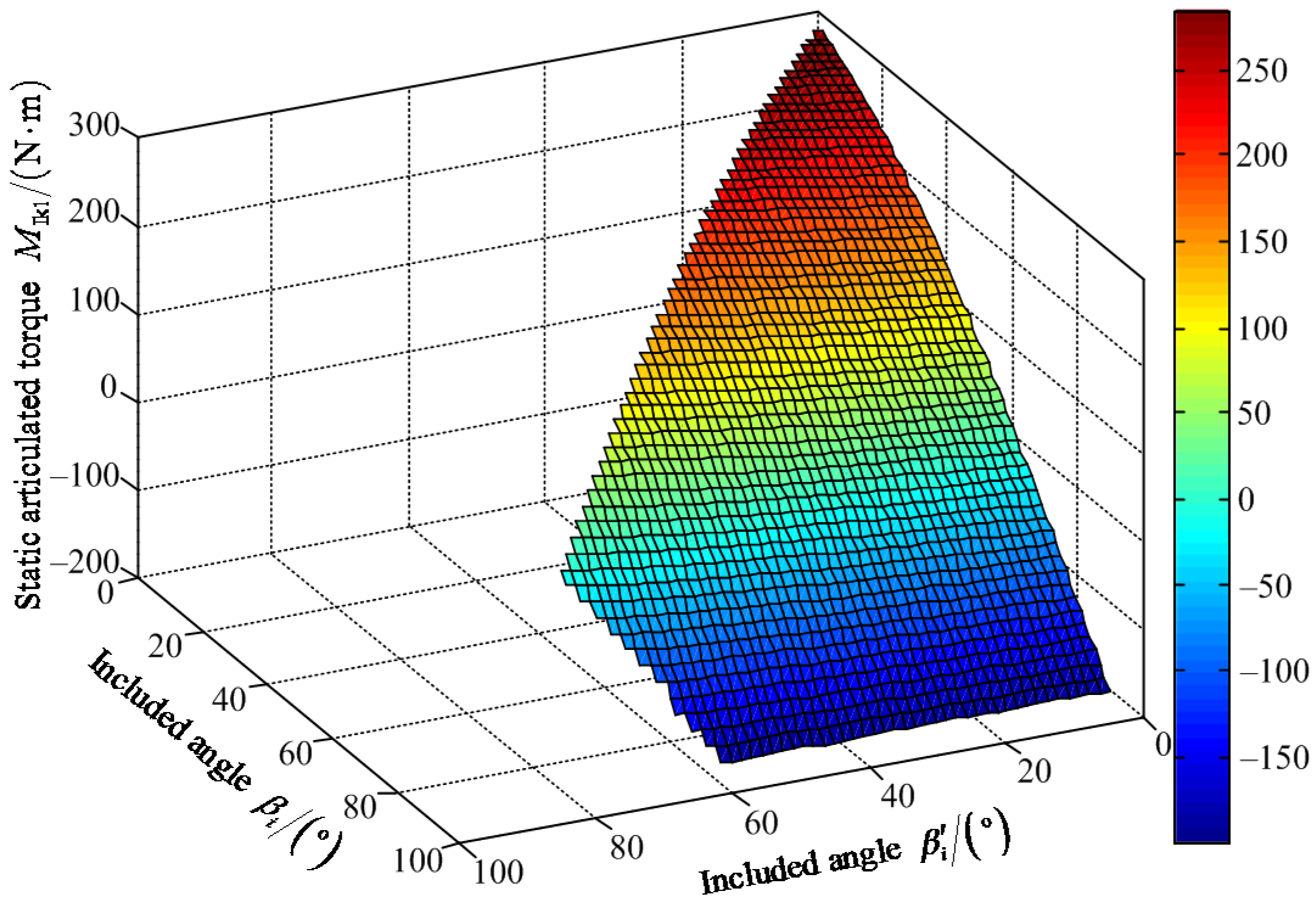
3.1. Analysis of Static Articulated Torques under Working Condition I
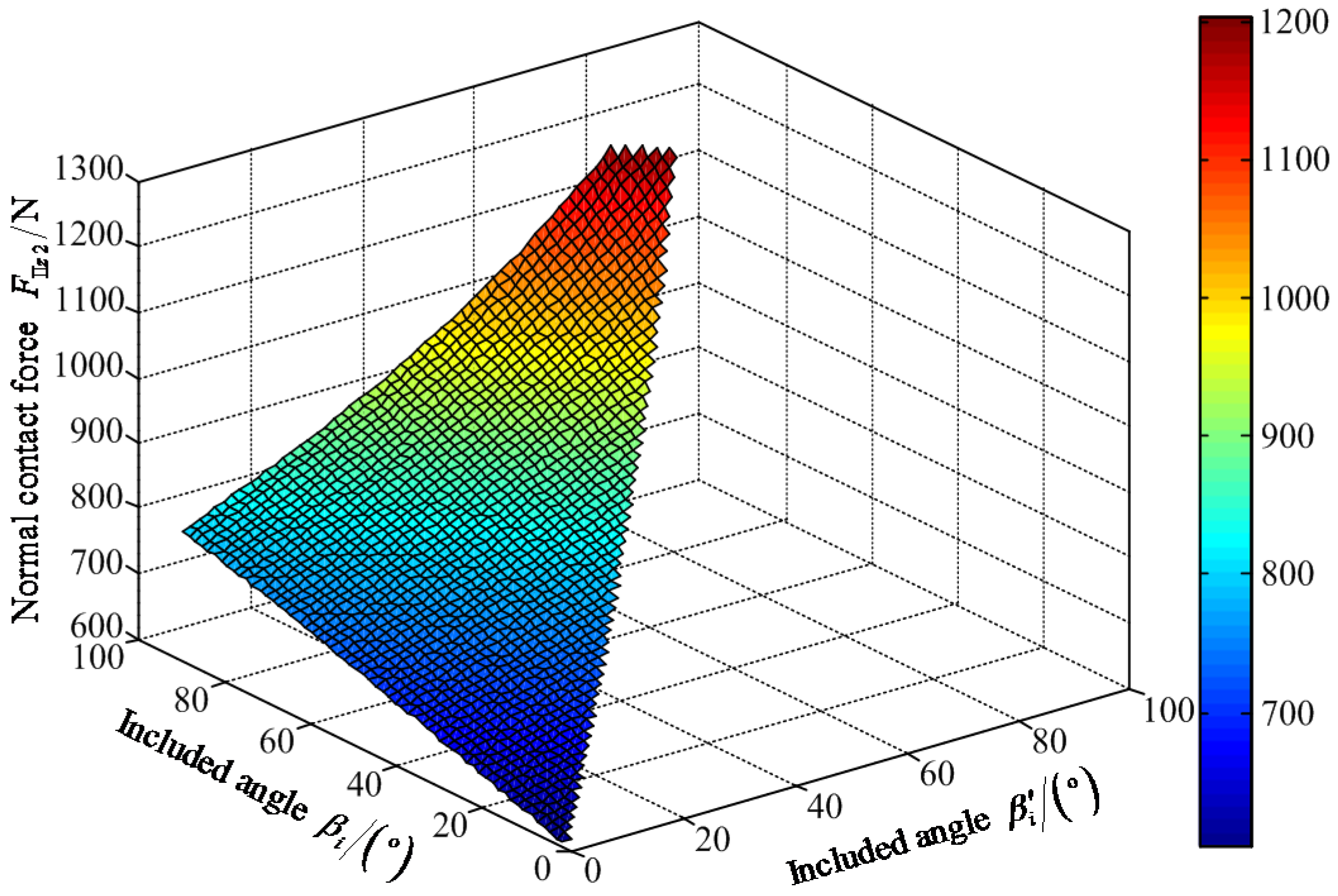
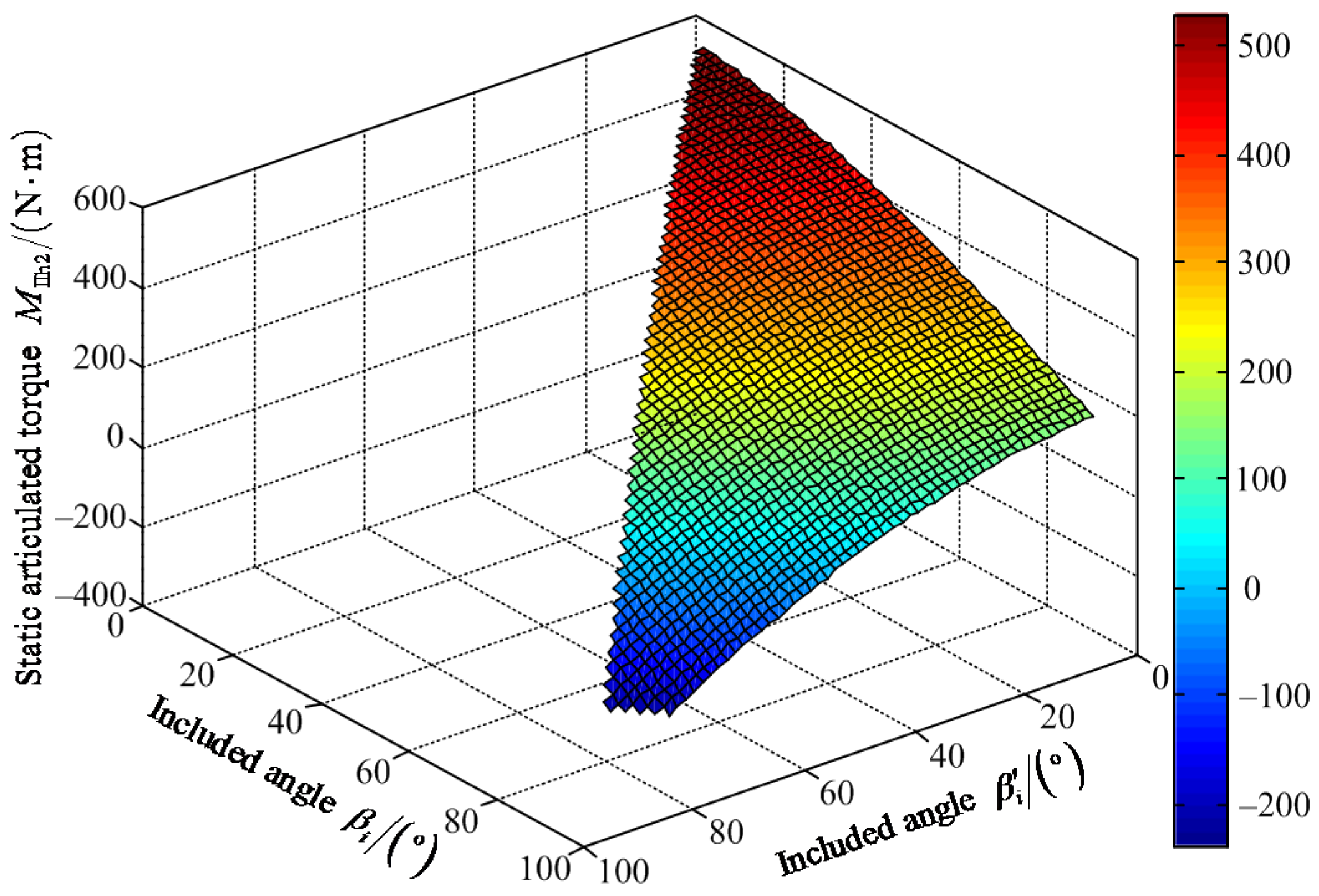
3.2. Analysis of Static Articulated Torques under Working Condition II
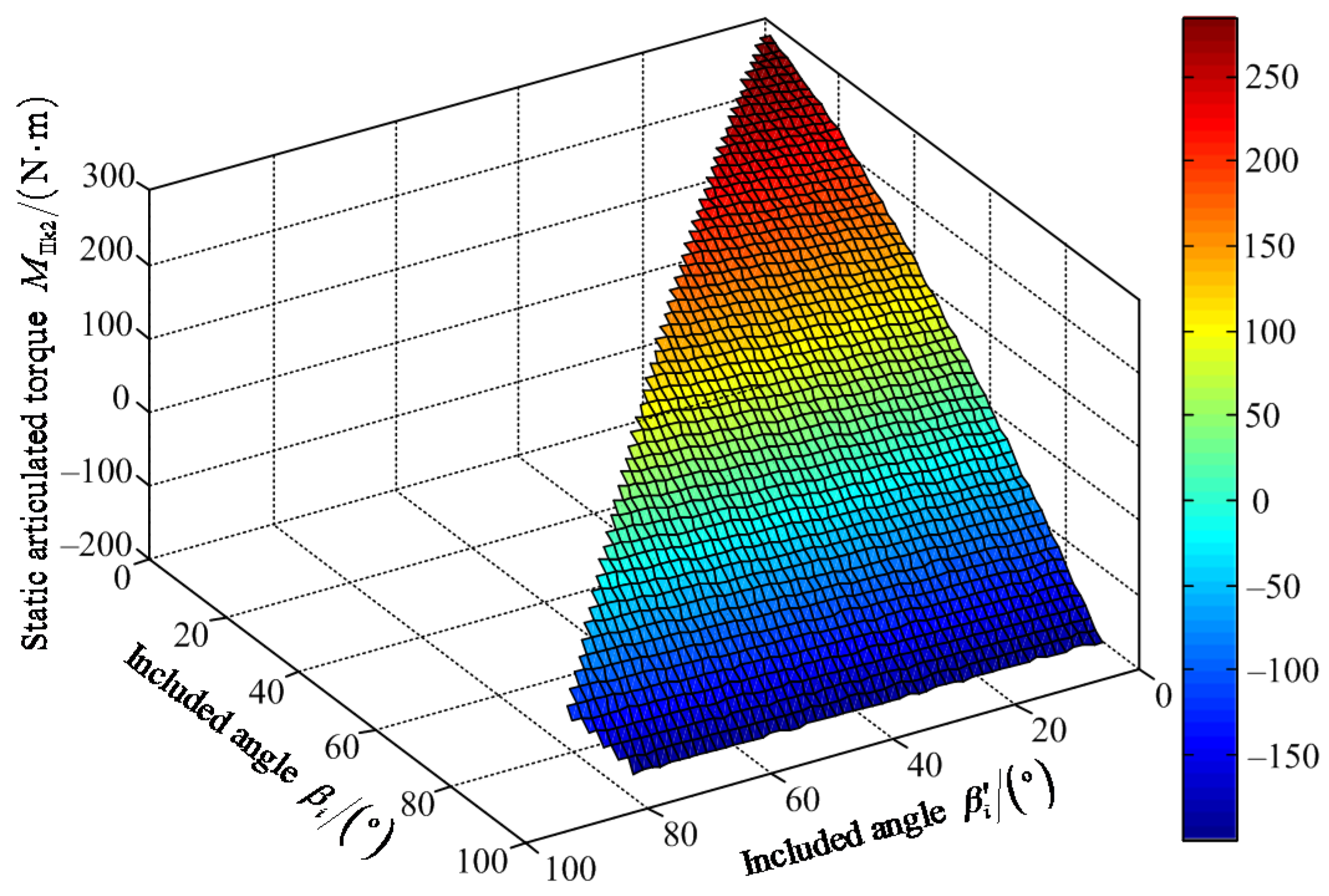
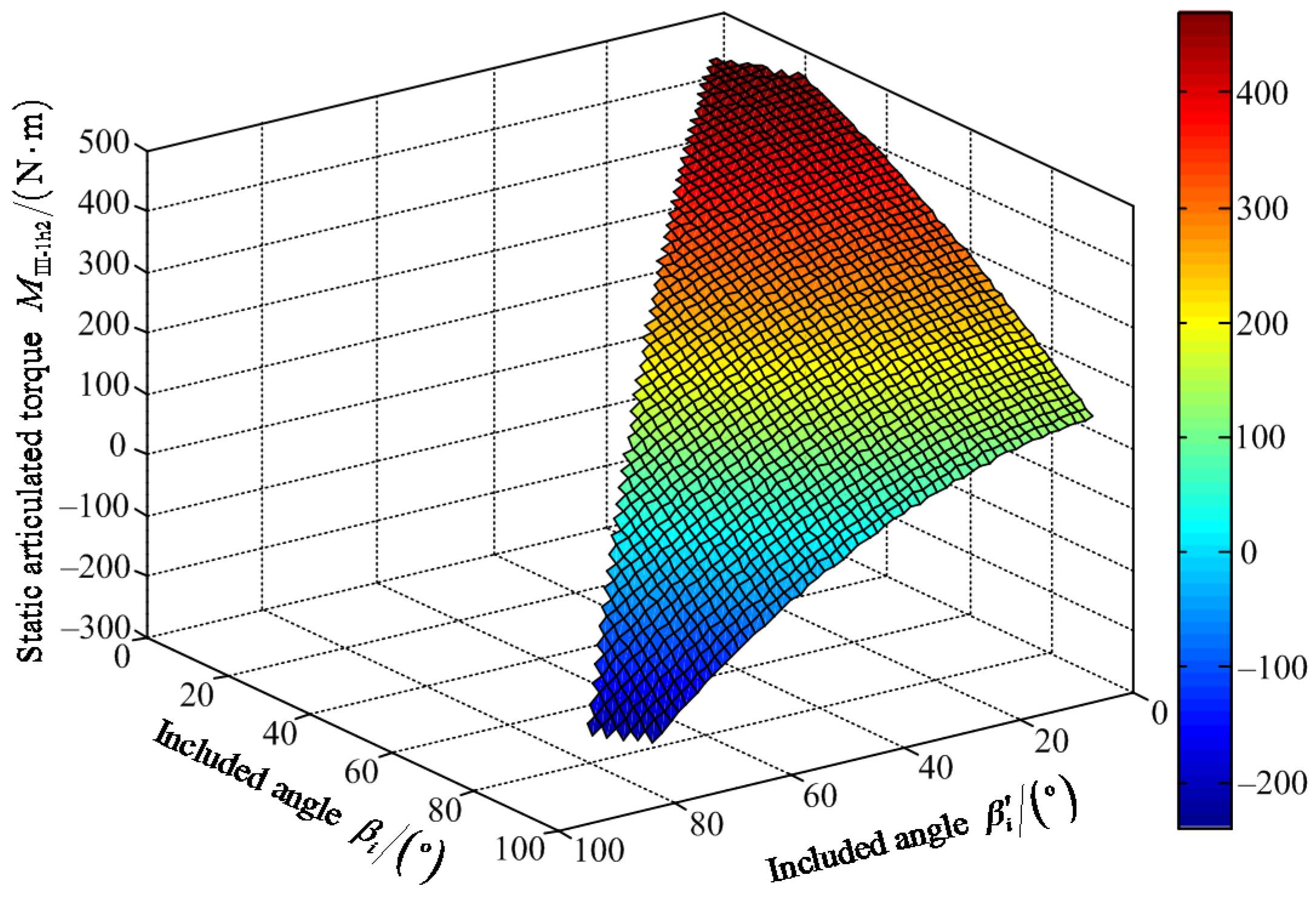
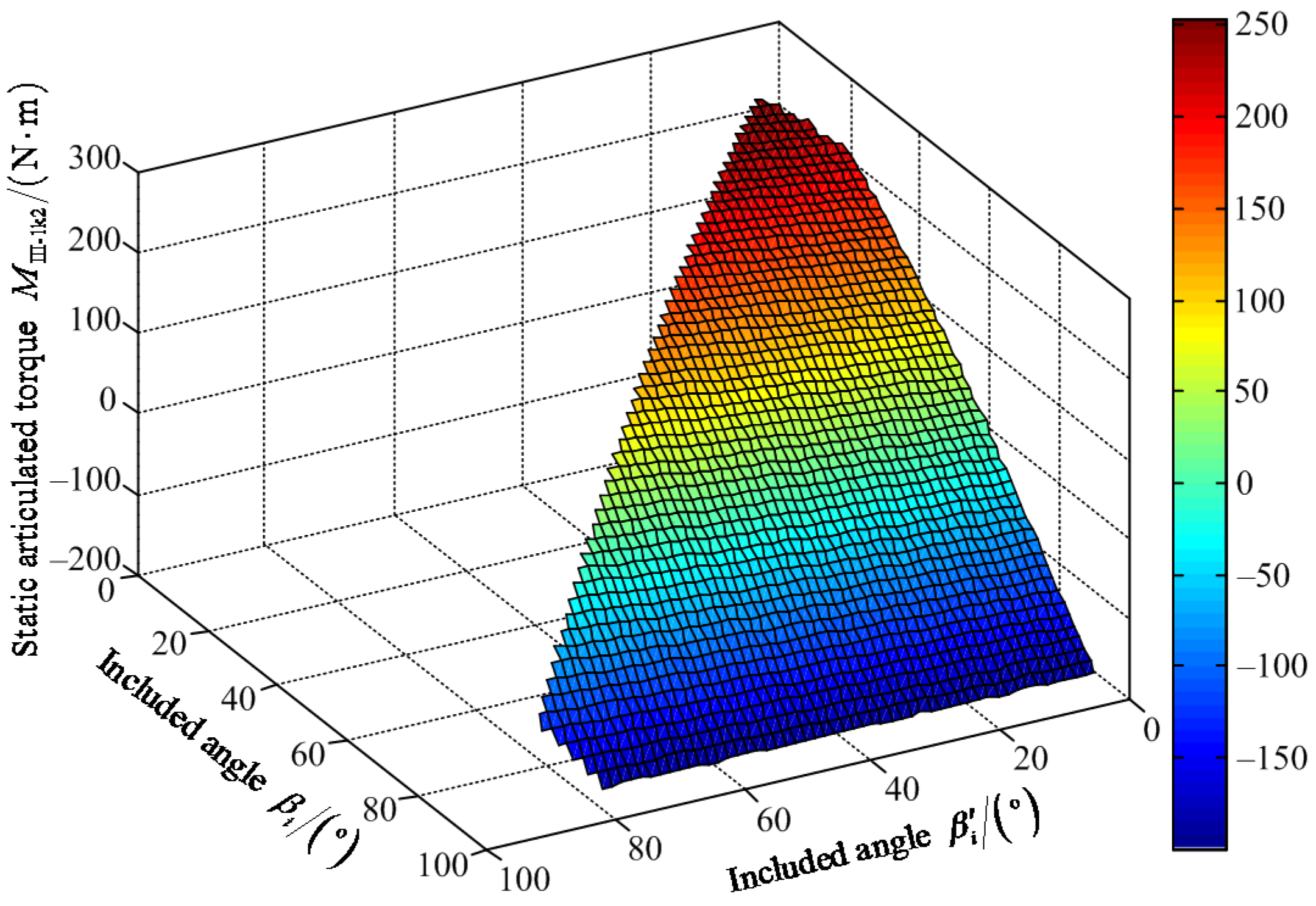
3.3. Analysis of Static Articulated Torques under Working Condition III
3.3.1. Analysis of Static Articulated Torques under Working Condition III-1
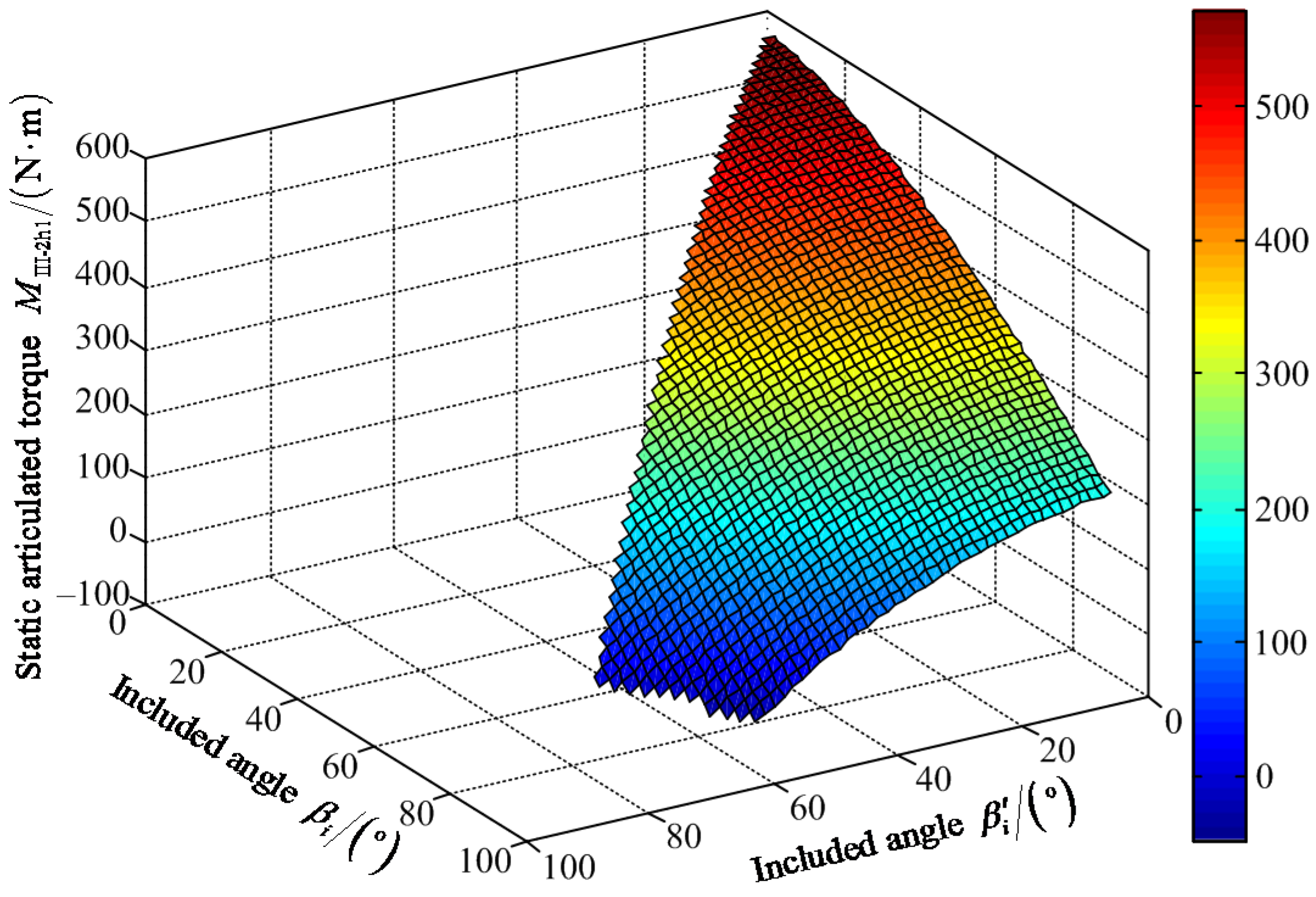
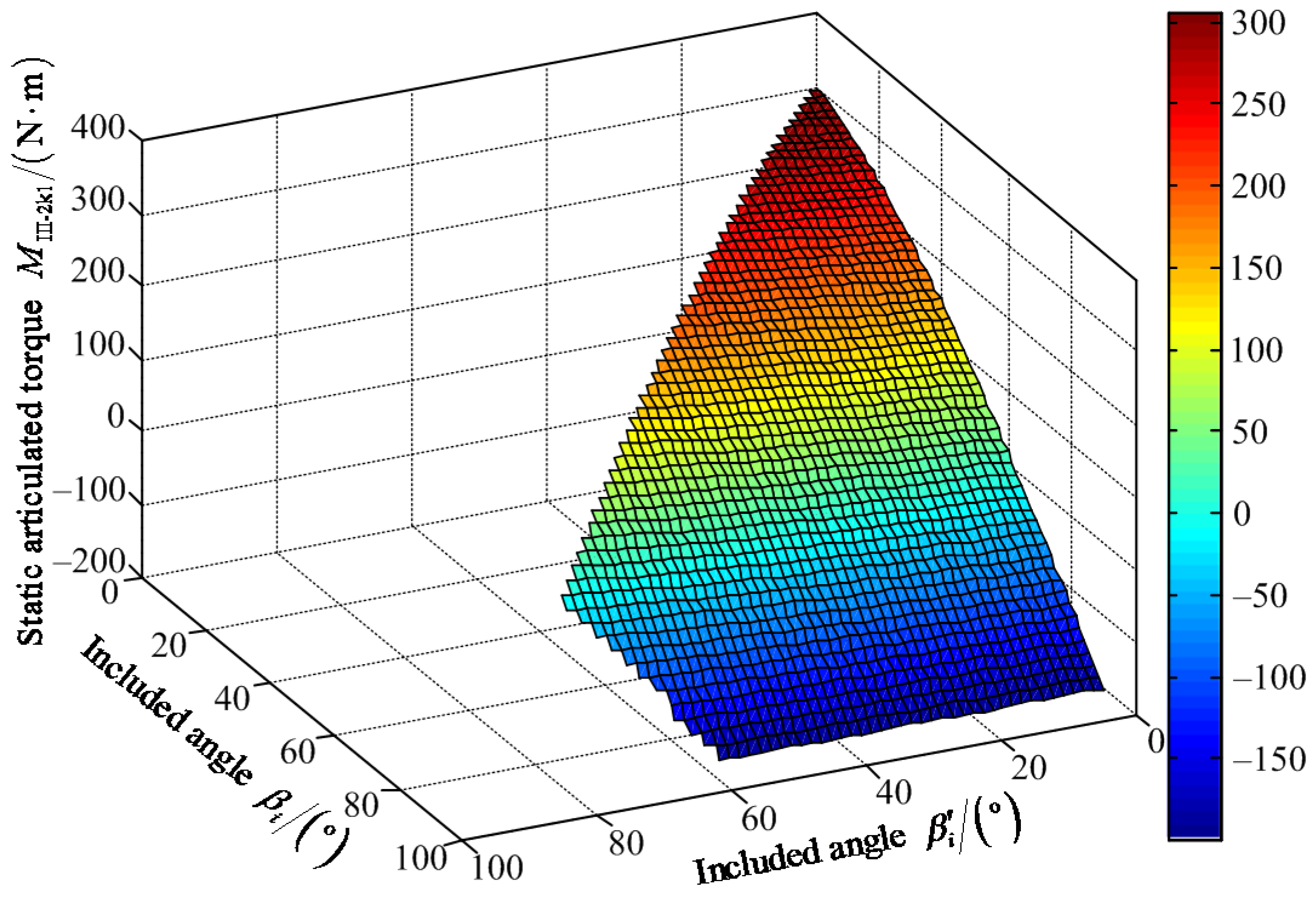
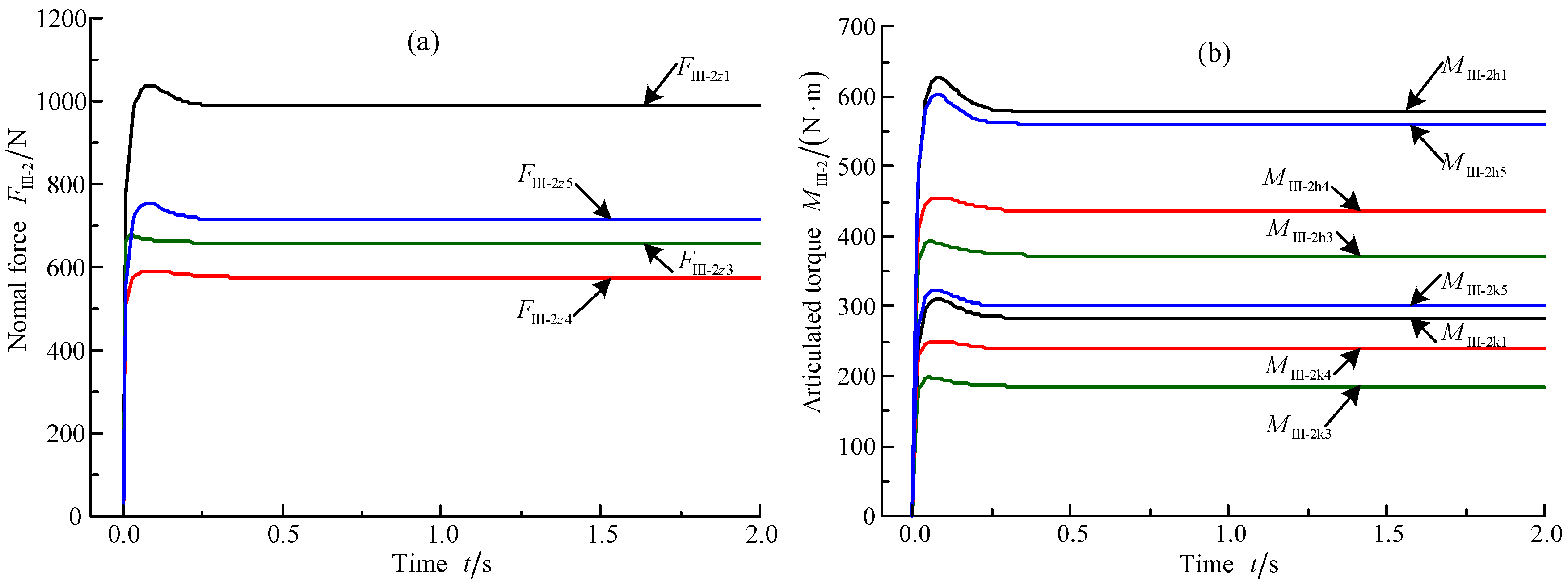
3.3.2. Analysis of Static Articulated Torques under Working Condition III-2
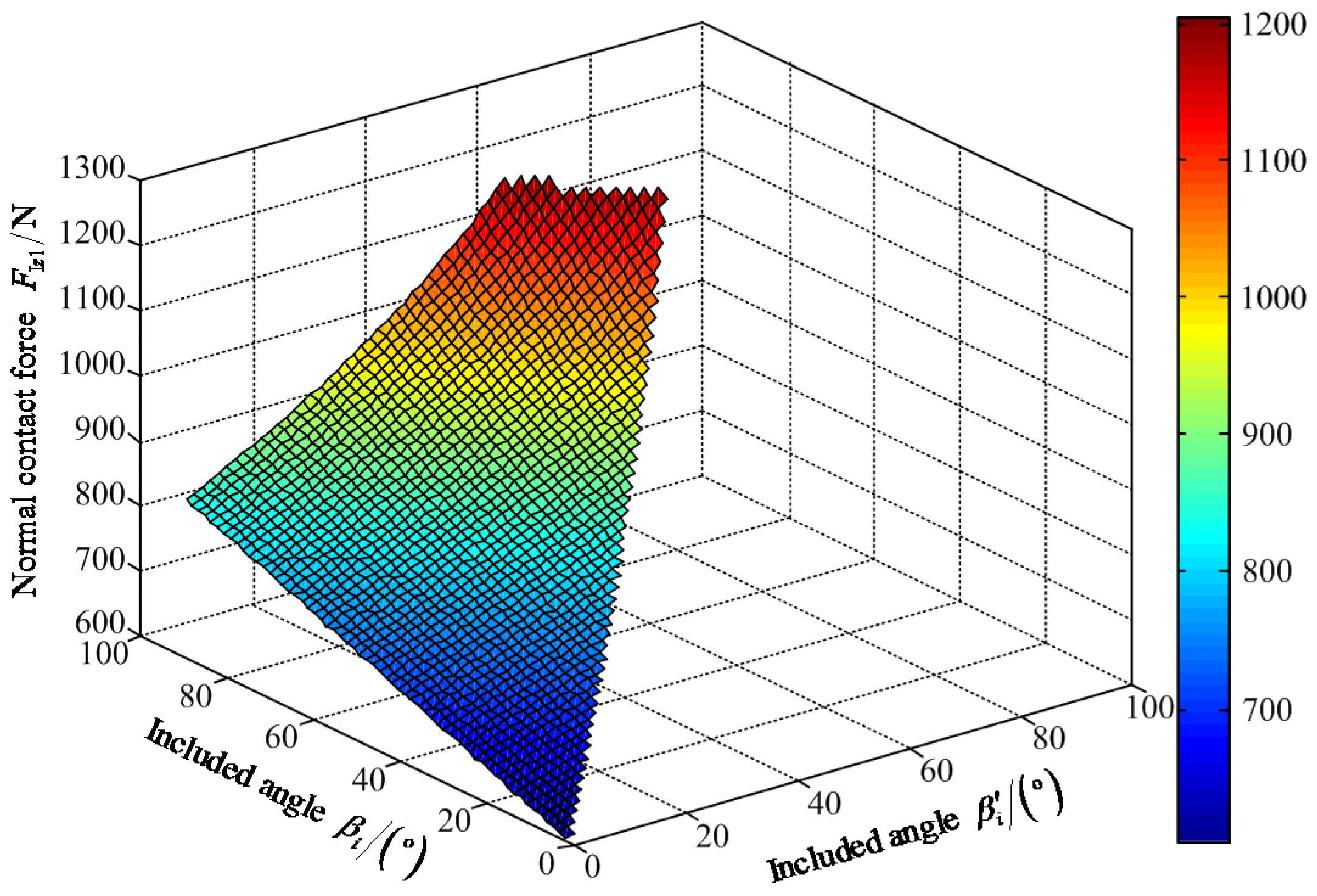
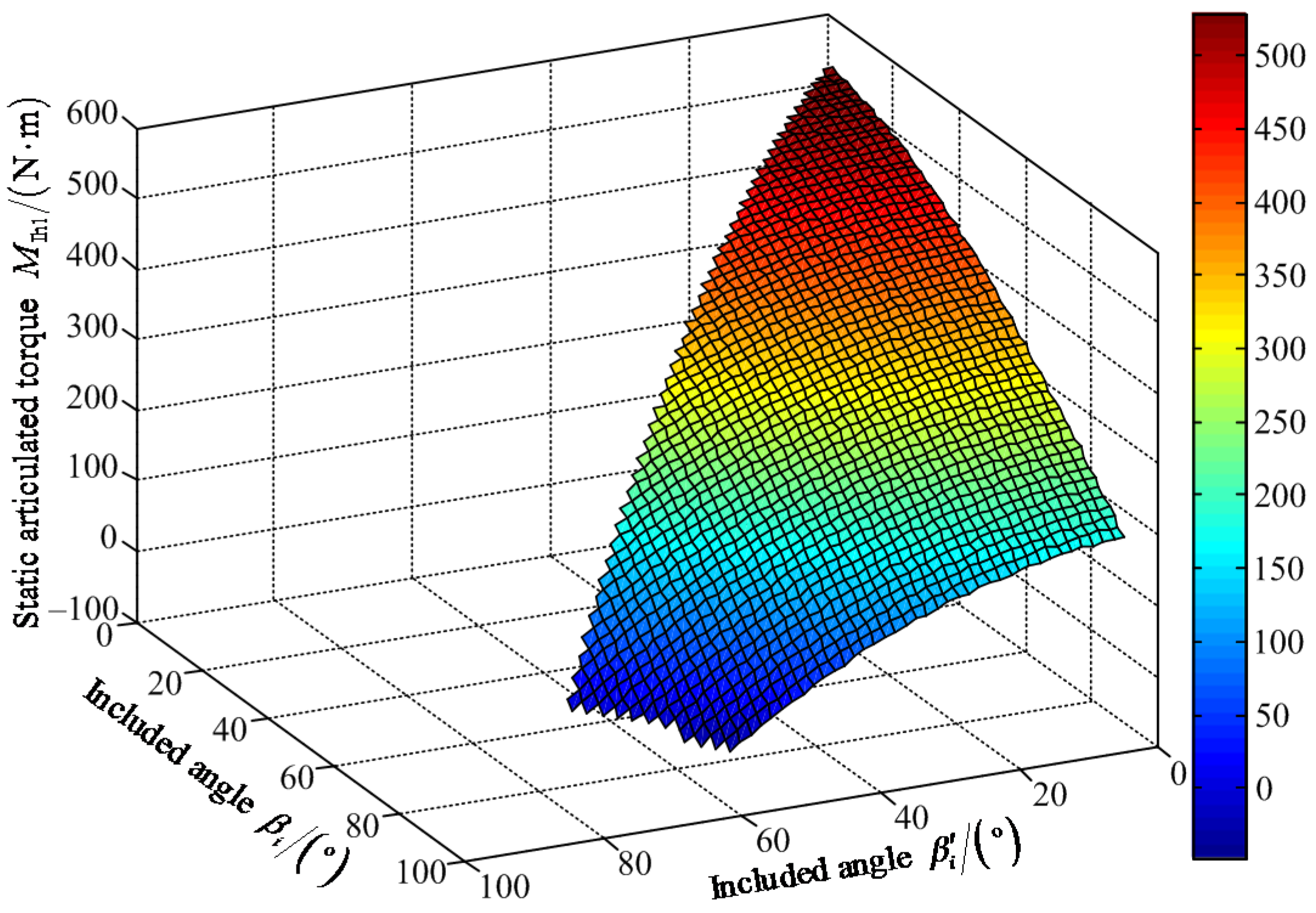
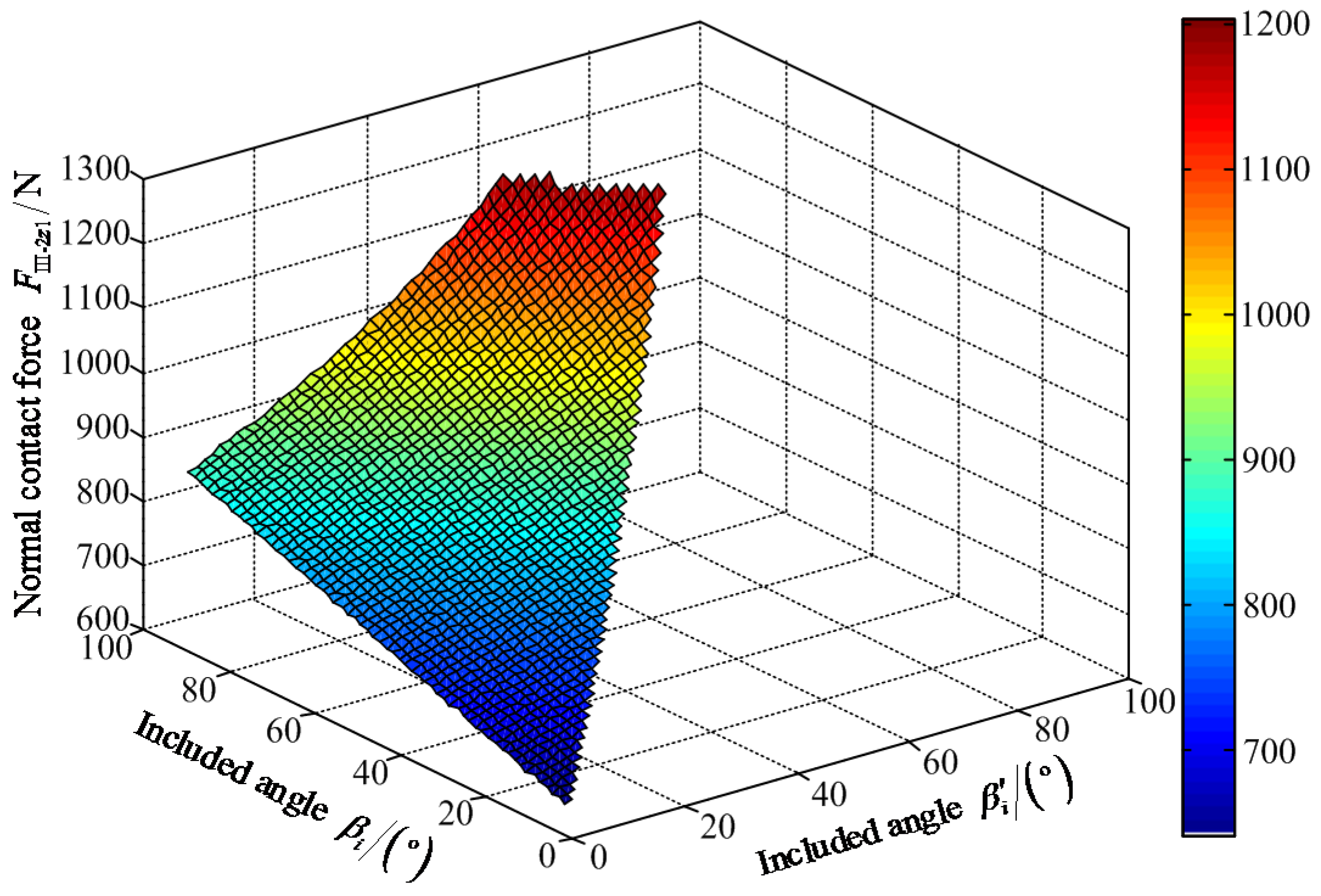
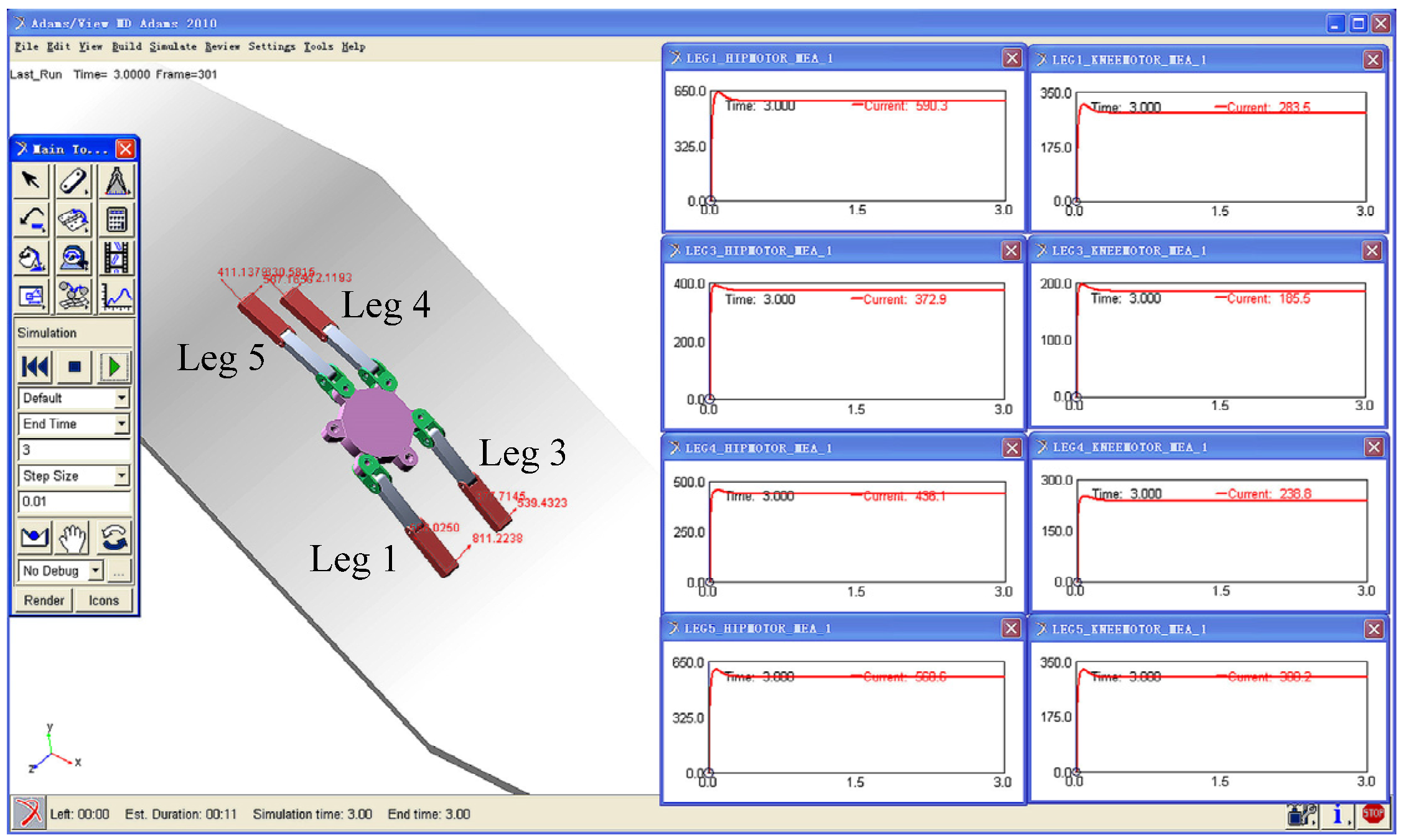
4. Simulation Analysis
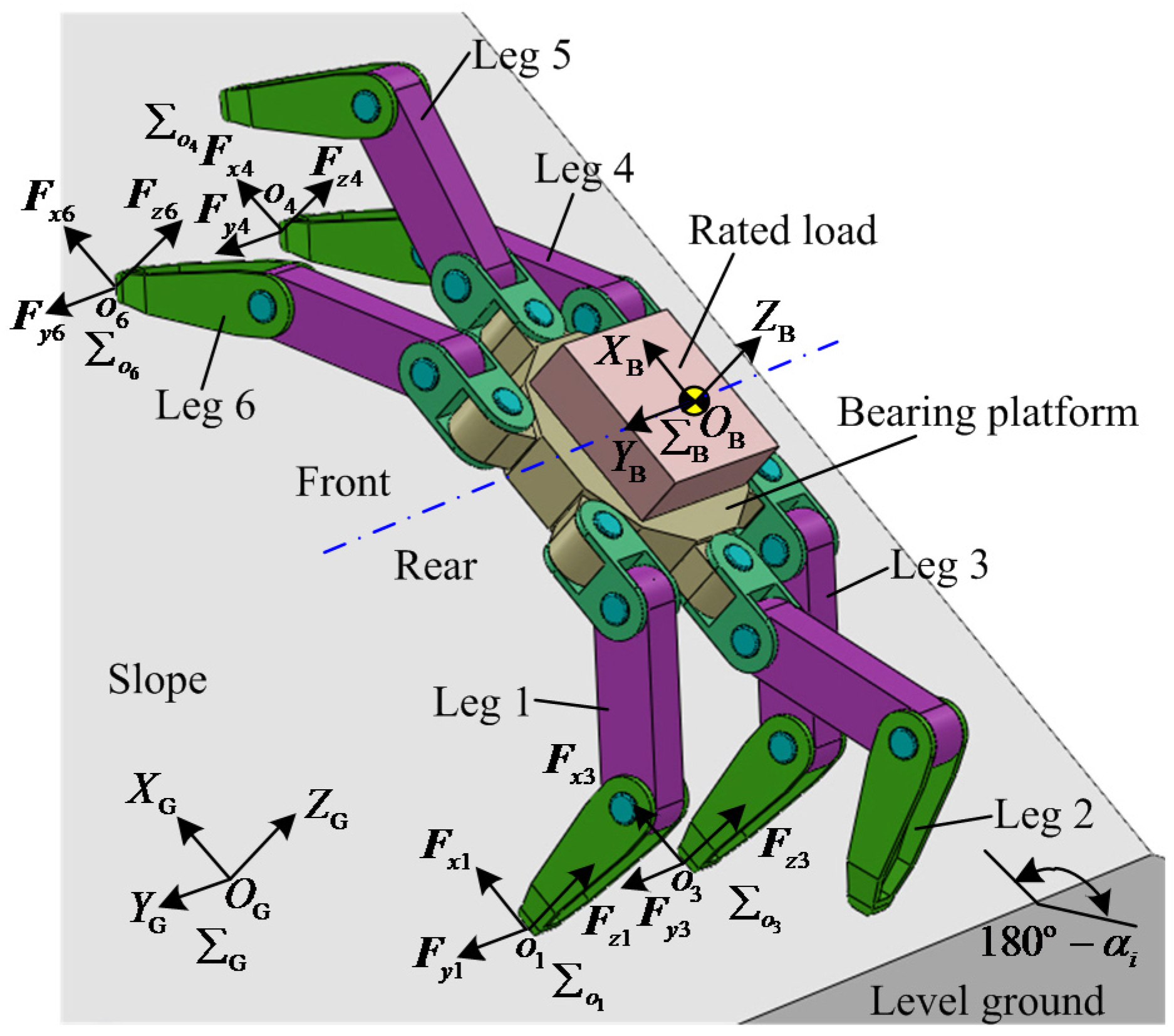
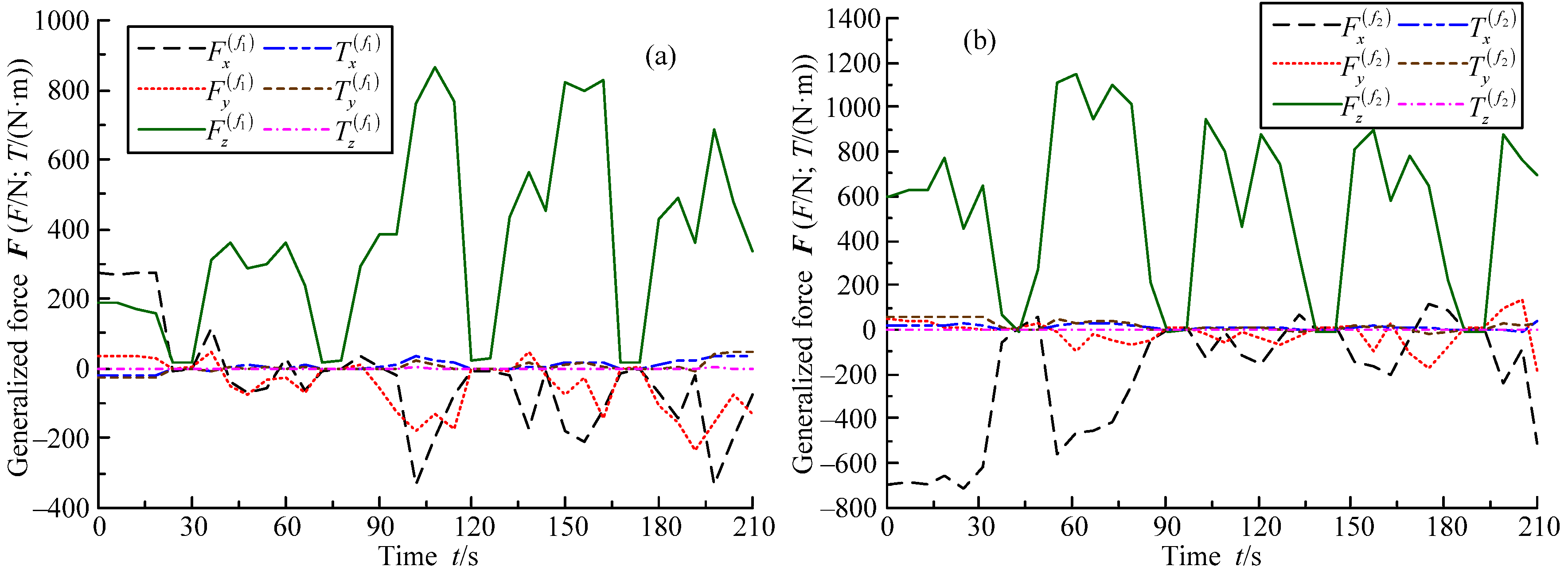
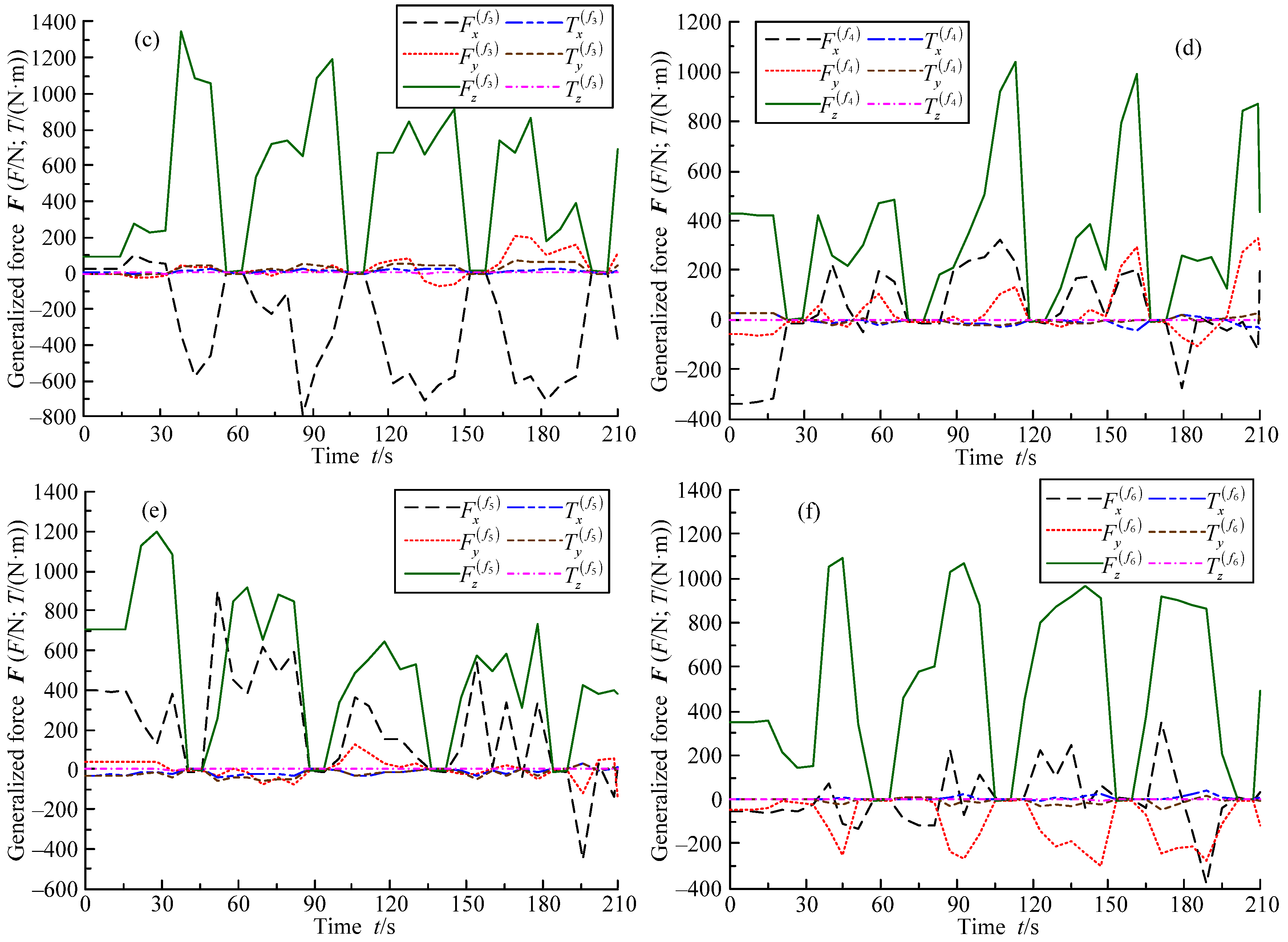
5. Applications and Experiments
6. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Wang, D.L.; Leung, H.; Kurian, A.P.; Kim, H.-J.; Yoon, H. A deconvolutive neural network for speech classification with applications to home service robot. IEEE Trans. Instrum. Meas. 2010, 59, 3237–3243. [Google Scholar] [CrossRef]
- Krotkov, E.P.; Simmons, R.G.; Whittaker, W.L. Ambler: Performance of a six-legged planetary rover. Acta Astronaut. 1995, 35, 75–81. [Google Scholar] [CrossRef]
- Wilcox, B.H.; Litwin, T.; Biesiadecki, J.; Matthews, J.; Heverly, M.; Morrison, J.; Townsend, J.; Ahmad, N.; Sirota, A.; Cooper, B. ATHLETE: A cargo handling and manipulation robot for the moon. J. Field Robot. 2007, 24, 421–434. [Google Scholar] [CrossRef]
- Luk, B.L.; Cooke, D.S.; Galt, S.; Collie, A.A.; Chen, S. Intelligent legged climbing service robot for remote maintenance applications in hazardous environments. Robot. Auton. Syst. 2005, 53, 142–152. [Google Scholar] [CrossRef]
- Irawan, A.; Nonami, K. Compliant walking control for hydraulic driven hexapod robot on rough terrain. J. Robot. Mechatron. 2011, 23, 149–162. [Google Scholar]
- Roy, S.S.; Pratihar, D.K. Effects of turning gait parameters on energy consumption and stability of a six-legged walking robot. Robot. Auton. Syst. 2012, 60, 72–82. [Google Scholar] [CrossRef]
- Hyon, S.H.; Emura, T. Energy-preserving control of a passive one-legged running robot. Adv. Robot. 2004, 18, 357–381. [Google Scholar] [CrossRef]
- Raibert, M.; Blankespoor, K.; Nelson, G.; Playter, R.; the BigDog Team. BigDog, the rough-terrain quadruped robot. In Proceedings of the 17th World Congress, International Federation of Automatic Control, Seoul, Korea, 6–11 July 2008.
- Silva, M.F.; Machado, J.A.T. A historical perspective of legged robots. J. Vib. Control 2007, 13, 1447–1486. [Google Scholar] [CrossRef]
- Liu, J.; Tan, M.; Zhao, X.G. Legged robots—An overview. Trans. Inst. Meas. Control 2007, 29, 185–202. [Google Scholar] [CrossRef]
- Erden, M.S.; Leblebicioğlu, K. Free gait generation with reinforcement learning for a six-legged robot. Robot. Auton. Syst. 2008, 56, 199–212. [Google Scholar] [CrossRef]
- Kurashige, K.; Fukuda, T.; Hoshino, H. Reusing primitive and acquired motion knowledge for gait generation of a six-legged robot using genetic programming. J. Intell. Robot. Syst. 2003, 38, 121–134. [Google Scholar] [CrossRef]
- Zhang, X.L.; E, M.C.; Zeng, X.Y.; Zheng, H.J. Adaptive walking of a quadrupedal robot based on layered biological reflexes. Chin. J. Mech. Eng. 2012, 25, 654–664. [Google Scholar] [CrossRef]
- Irawan, A.; Nonami, K. Optimal impedance control based on body inertia for a hydraulically driven hexapod robot walking on uneven and extremely soft terrain. J. Field Robot. 2011, 28, 690–713. [Google Scholar] [CrossRef]
- Luk, B.L.; Galt, S.; Chen, S. Using genetic algorithms to establish efficient walking gaits for an eight legged robot. Int. J. Syst. Sci. 2001, 32, 703–713. [Google Scholar] [CrossRef]
- Tan, G.Z.; He, H.; Sloman, A. Global optimal path planning for mobile robot based on improved Dijkstra algorithm and ant system algorithm. J. Cent. South Univ. Technol. 2006, 13, 80–86. [Google Scholar] [CrossRef]
- Sintov, A.; Avramovich, T.; Shapiro, A. Design and motion planning of an autonomous climbing robot with claws. Robot. Auton. Syst. 2011, 59, 1008–1019. [Google Scholar] [CrossRef]
- Erden, M.S.; Leblebicioğlu, K. Torque distribution in a six-legged robot. IEEE Trans. Robot. 2007, 23, 179–186. [Google Scholar] [CrossRef]
- Roy, S.S.; Singh, A.K.; Pratihar, D.K. Estimation of optimal feet forces and joint torques for on-line control of six-legged robot. Robot. Comput. Integr. Manuf. 2011, 27, 910–917. [Google Scholar] [CrossRef]
- Santos, P.G.D.; Estremera, J.; Garcia, E.; Armada, M. Including joint torques and power consumption in the stability margin of walking robots. Auton. Robot. 2005, 18, 43–57. [Google Scholar] [CrossRef]
- Silva, M.F.; Machado, J.A.T.; Lopes, A.M. Fractional order control of a hexapod robot. Nonlinear Dyn. 2004, 38, 417–433. [Google Scholar] [CrossRef]
- Mahapatra, A.; Roy, S.S. Computer aided dynamic simulation of six-legged robot. Int. J. Recent Trends Eng. 2009, 2, 146–151. [Google Scholar]
- Wang, G.; Zhang, L.X.; Chen, D.L.; Liu, D.F.; Chen, X. Modeling and simulation of multi-legged walking machine prototype. In Proceedings of the 2009 International Conference on Measuring Technology and Mechatronics Automation, Zhangjiajie, China, 11–12 April 2009.
- Gao, H.B.; Zhuang, H.C.; Deng, Z.Q.; Ding, L.; Liu, Z. Transmission mode research on the joints of a multi-legged walking robot. Appl. Mech. Mater. 2012, 151, 518–522. [Google Scholar] [CrossRef]
- Gao, H.B.; Zhuang, H.C.; Li, Z.G.; Deng, Z.Q.; Ding, L.; Liu, Z. Optimization and experimental research on a new-type short cylindrical cup-shaped harmonic reducer. J. Cent. South Univ. Technol. 2012, 19, 1869–1882. [Google Scholar] [CrossRef]
- Zhuang, H.C.; Gao, H.B.; Deng, Z.Q.; Ding, L.; Liu, Z. Method for analyzing articulated rotating speeds of heavy-duty six-legged robot. J. Mech. Eng. 2013, 49, 44–52. [Google Scholar] [CrossRef]
- Zhuang, H.C.; Gao, H.B.; Deng, Z.Q.; Ding, L.; Liu, Z. A review of heavy-duty legged robots. Sci. China Technol. Sci. 2014, 57, 298–314. [Google Scholar] [CrossRef]

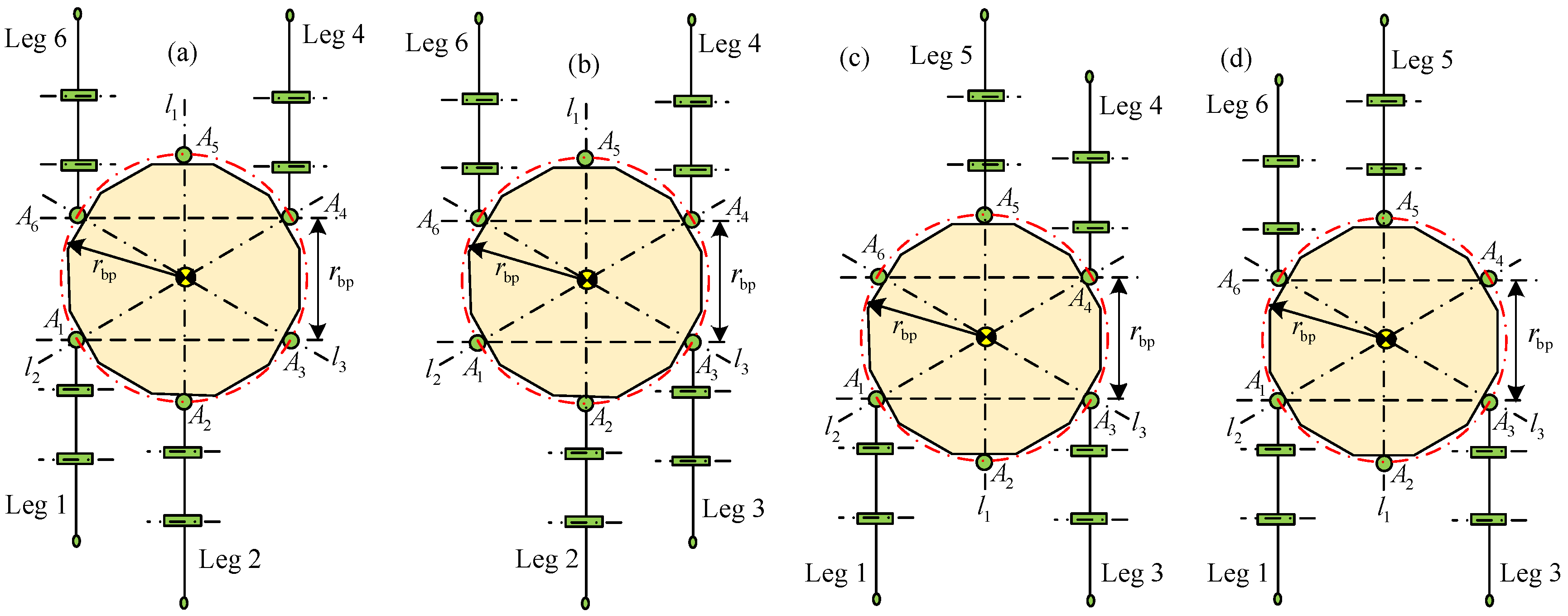
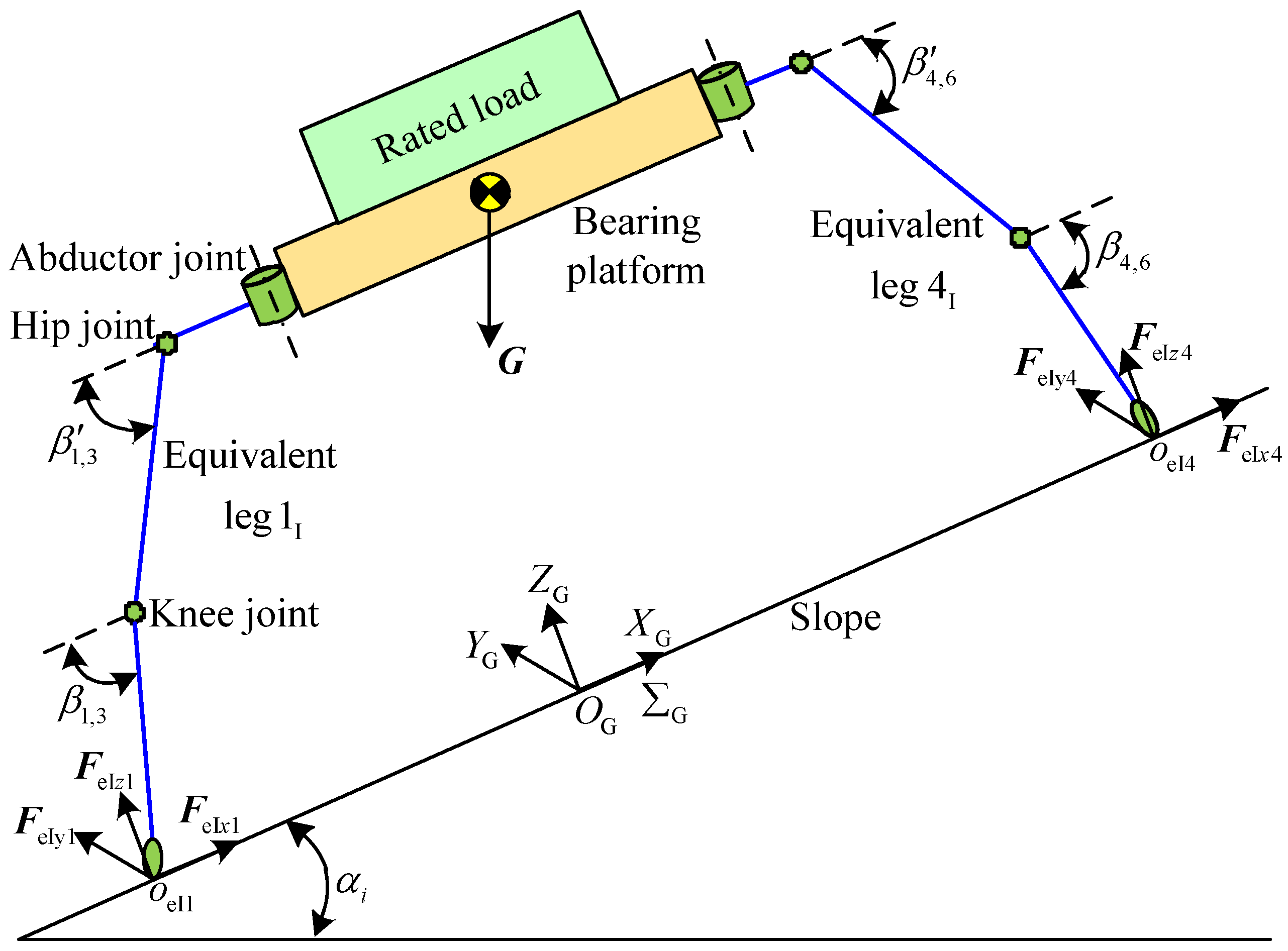




| No. | Phase | 1/3 Gait Legs | 2/3 Gait Legs | 3/3 Gait Legs | No. | Phase | 1/3 Gait Legs | 2/3 Gait Legs | 3/3 Gait Legs |
|---|---|---|---|---|---|---|---|---|---|
| 1 | Transfer | 1, 5 | 2, 4 | 3, 6 | 10 | Transfer | 2, 5 | 3, 6 | 1, 4 |
| Support | 2, 3, 4, 6 | 1, 3, 5, 6 | 1, 2, 4, 5 | Support | 1, 3, 4, 6 | 1, 2, 4, 5 | 2, 3, 5, 6 | ||
| 2 | Transfer | 1, 5 | 3, 6 | 2, 4 | 11 | Transfer | 2, 4 | 3, 6 | 1, 5 |
| Support | 2, 3, 4, 6 | 1, 2, 4, 5 | 1, 3, 5, 6 | Support | 1, 3, 5, 6 | 1, 2, 4, 5 | 2, 3, 4, 6 | ||
| 3 | Transfer | 1, 4 | 2, 5 | 3, 6 | 12 | Transfer | 2, 4 | 1, 5 | 3, 6 |
| Support | 2, 3, 5, 6 | 1, 3, 4, 6 | 1, 2, 4, 5 | Support | 1, 3, 5, 6 | 2, 3, 4, 6 | 1, 2, 4, 5 | ||
| 4 | Transfer | 1, 4 | 2, 6 | 3, 5 | 13 | Transfer | 3, 6 | 2, 5 | 1, 4 |
| Support | 2, 3, 5, 6 | 1, 3, 4, 5 | 1, 2, 4, 6 | Support | 1, 2, 4, 5 | 1, 3, 4, 6 | 2, 3, 5, 6 | ||
| 5 | Transfer | 1, 4 | 3, 6 | 2, 5 | 14 | Transfer | 3, 6 | 2, 4 | 1, 5 |
| Support | 2, 3, 5, 6 | 1, 2, 4, 5 | 1, 3, 4, 6 | Support | 1, 2, 4, 5 | 1, 3, 5, 6 | 2, 3, 4, 6 | ||
| 6 | Transfer | 1, 4 | 3, 5 | 2, 6 | 15 | Transfer | 3, 6 | 1, 5 | 2, 4 |
| Support | 2, 3, 5, 6 | 1, 2, 4, 6 | 1, 3, 4, 5 | Support | 1, 2, 4, 5 | 2, 3, 4, 6 | 1, 3, 5, 6 | ||
| 7 | Transfer | 2, 6 | 3, 5 | 1, 4 | 16 | Transfer | 3, 6 | 1, 4 | 2, 5 |
| Support | 1, 3, 4, 5 | 1, 2, 4, 6 | 2, 3, 5, 6 | Support | 1, 2, 4, 5 | 2, 3, 5, 6 | 1, 3, 4, 6 | ||
| 8 | Transfer | 2, 6 | 1, 4 | 3, 5 | 17 | Transfer | 3, 5 | 1, 4 | 2, 6 |
| Support | 1, 3, 4, 5 | 2, 3, 5, 6 | 1, 2, 4, 6 | Support | 1, 2, 4, 6 | 2, 3, 5, 6 | 1, 3, 4, 5 | ||
| 9 | Transfer | 2, 5 | 1, 4 | 3, 6 | 18 | Transfer | 3, 5 | 2, 6 | 1, 4 |
| Support | 1, 3, 4, 6 | 2, 3, 5, 6 | 1, 2, 4, 5 | Support | 1, 2, 4, 6 | 1, 3, 4, 5 | 2, 3, 5, 6 |
| No. | Max. Static Torque of Hip Joint | Max. Static Torque of Knee Joint | Range | ||
|---|---|---|---|---|---|
| Pose | Value/N·m | Pose | Value/N·m | ||
| I | βi′ = 0° | 529.80 | βi′ = 0° | 285.00 | 0° ≤ βi′ ≤ βi ≤ 90° |
| βi = 0° | βi = 0° | 0° ≤ βi′ + βi ≤ 147° | |||
| II | βi′ = 0° | 529.80 | βi′ = 0° | 285.00 | 0° ≤ βi′ ≤ βi ≤ 90° |
| βi = 0° | βi = 0° | 0° ≤ βi′ + βi ≤ 167° | |||
| III-1 | βi′ = 8° | 470.30 | βi′ = 9° | 252.20 | 0° ≤ βi′ ≤ βi ≤ 90° |
| βi = 9° | βi = 9° | 17° ≤ βi′ + βi ≤ 167° | |||
| III-2 | βi′ = 0° | 589.50 | βi′ = 0° | 305.30 | 0° ≤ βi′ ≤ βi ≤ 90° |
| βi = 0° | βi = 0° | 0° ≤ βi′ + βi ≤ 147° | |||
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhuang, H.-C.; Gao, H.-B.; Deng, Z.-Q. Analysis Method of Articulated Torque of Heavy-Duty Six-Legged Robot under Its Quadrangular Gait. Appl. Sci. 2016, 6, 323. https://doi.org/10.3390/app6110323
Zhuang H-C, Gao H-B, Deng Z-Q. Analysis Method of Articulated Torque of Heavy-Duty Six-Legged Robot under Its Quadrangular Gait. Applied Sciences. 2016; 6(11):323. https://doi.org/10.3390/app6110323
Chicago/Turabian StyleZhuang, Hong-Chao, Hai-Bo Gao, and Zong-Quan Deng. 2016. "Analysis Method of Articulated Torque of Heavy-Duty Six-Legged Robot under Its Quadrangular Gait" Applied Sciences 6, no. 11: 323. https://doi.org/10.3390/app6110323
APA StyleZhuang, H.-C., Gao, H.-B., & Deng, Z.-Q. (2016). Analysis Method of Articulated Torque of Heavy-Duty Six-Legged Robot under Its Quadrangular Gait. Applied Sciences, 6(11), 323. https://doi.org/10.3390/app6110323






