Elastic Properties and Stability of Physisorbed Graphene
Abstract
:1. Introduction
2. Teachings of Hooke’s Law and the Kirchhoff-Love Theory
3. Teachings of Phonon Dispersion Laws
4. Results of Calculations
5. Experimental Nanomechanical Measurements
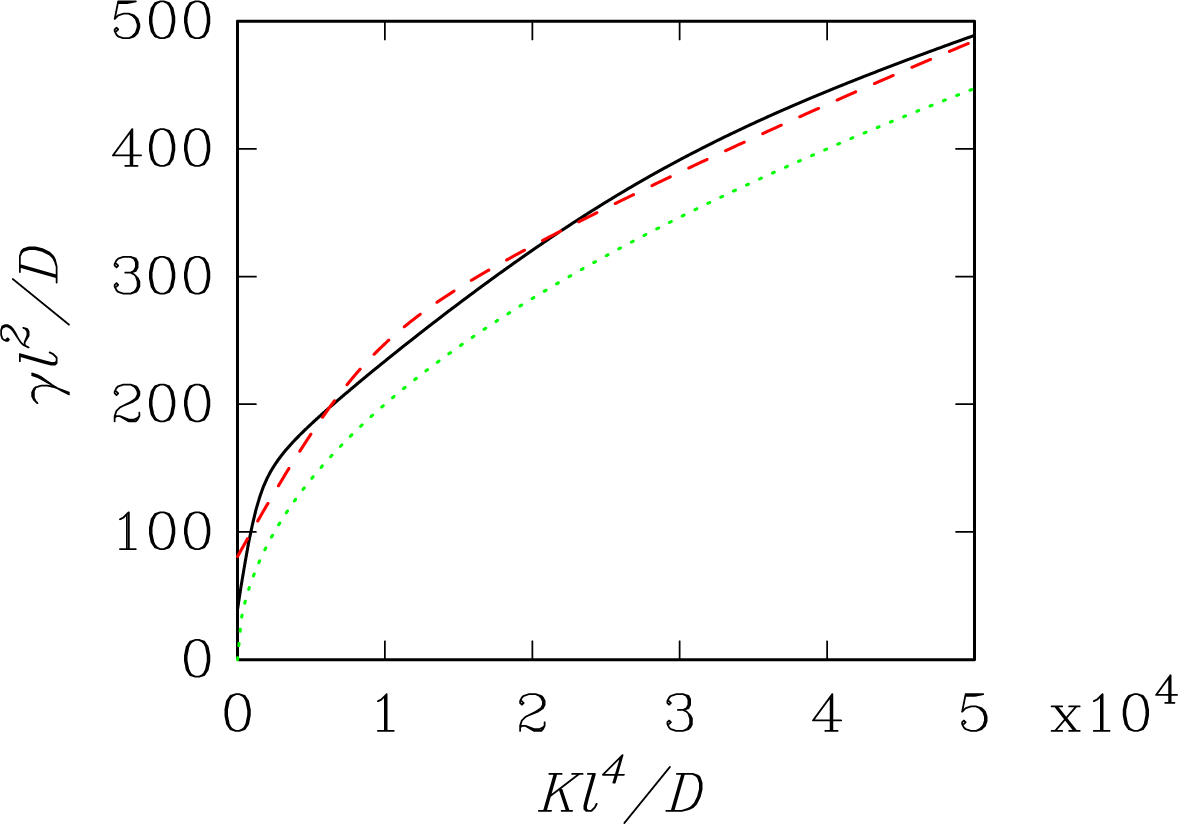
6. Buckling Instability
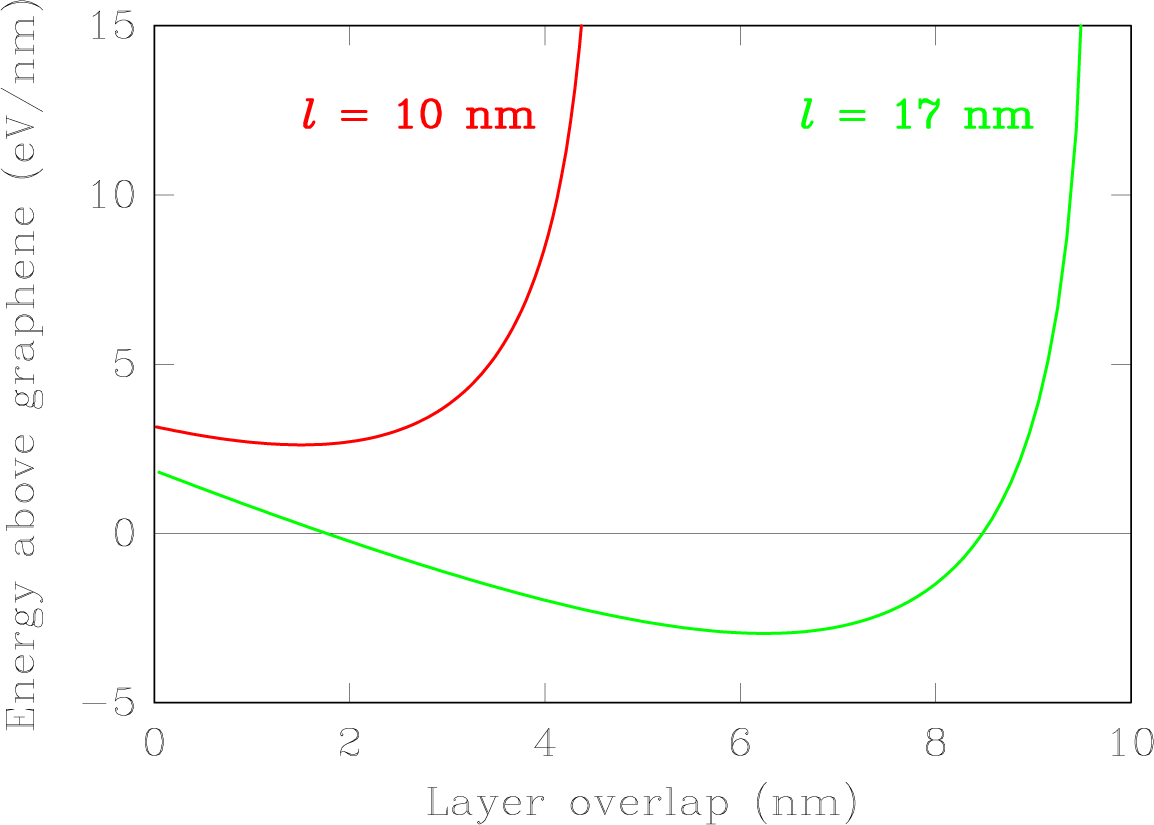
7. A Buckling Mode of Non-Supported Graphene: Scrolling
8. Nonlocal, Nonlinear and Temperature Effects
9. Conclusions
Conflicts of Interest
Appendix: Force Constant of the Girifalco Interaction Potential
| Substrate | h (nm) | a (meV nm4) | b (meV nm10) | z0 (nm) |
|---|---|---|---|---|
| gr(001) | 0.3354 | 4.14 | 6.37 × 103 | 0.338 |
| h-BN(001) | 0.3331 | 3.25 | 5.49 × 103 | 0.343 |
Acknowledgments
References
- Mermin, N.D. Crystalline order in two dimensions. Phys. Rev. B 1968, 176, 250–254. [Google Scholar]
- Meyer, J.C.; Geim, A.K.; Katsnelson, M.I.; Novoselov, K.S.; Booth, T.J.; Roth, S. The structure of suspended graphene sheets. Nature 2007, 446, 60–63. [Google Scholar]
- Fasolino, A.; Los, J.H.; Katsnelson, M.I. Intrinsic ripples in graphene. Nat. Mater 2007, 6, 858–861. [Google Scholar]
- Xu, Z.; Buehler, M. Geometry controls conformation of graphene sheets: Membranes, ribbons, and scrolls. ACS Nano 2010, 4, 3869–3876. [Google Scholar]
- Zhu, W.; Low, T.; Perebeinos, V.; Bol, A.A.; Zhu, Y.; Yan, H.; Tersoff, J.; Avouris, P. Structure and electronic transport in graphene wrinkles. Nano Lett 2012, 12, 3431–3436. [Google Scholar]
- Farjam, M.; Rai-Tabar, H. Comment on “Band structure engineering of graphene by strain: First-principles calculations”. Phys. Rev. B 2009, 80, 167401.1–167401.3. [Google Scholar]
- Li, Y.; Jiang, X.; Liu, Z.; Liu, Z. Strain effects in graphene and graphene nanoribbons: The underlying mechanism. Nano Res 2010, 3, 545–556. [Google Scholar]
- Peres, N.M.R. Colloquium: The transport properties of graphene: An introduction. Rev. Mod. Phys 2010, 82, 2673–2700. [Google Scholar]
- Liu, N.; Pan, Z.; Fu, L.; Zhang, C.; Dai, B.; Liu, Z. The origin of wrinkles on transferred graphene. Nano Res 2011, 4, 996–1004. [Google Scholar]
- Mohiuddin, T.M.G.; Lombardo, A.; Nair, R.R.; Bonetti, A.; Savini, G.; Jali, R.; Bonini, N.; Basko, D.M.; Galiotis, C.; Marzari, N.; et al. Uniaxial strain in graphene by Raman spetrosopy: G peak splitting, Gruneisen parameters and sample orientation. Phys. Rev. B 2009, 79, 205433.1–205433.8. [Google Scholar]
- Ferralis, N. Probing mechanical properties of graphene with Raman spectroscopy. J. Mater. Sci 2010, 45, 5135–5149. [Google Scholar]
- He, R.; Zhao, L.; Petrone, N.; Kim, K.S.; Roth, M.; Hone, J.; Kim, P.; Pasupathy, A.; Pinczuk, A. Large physisorption strain in chemical vapor deposition of graphene on copper substrates. Nano Lett 2012, 12, 2408–2413. [Google Scholar]
- Frank, O.; Mohr, M.; Maultzsch, J.; Thomsen, C.; Riaz, I.; Jalil, R.; Novoselov, K.S.; Tsoukleri, G.; Parthenios, J.; Papagelis, K.; Kavan, L.; Galiotis, C. Raman 2D-band splitting in graphene: Theory and experiment. ACS Nano 2011, 5, 2231–2239. [Google Scholar]
- Popov, V.N.; Lambin, Ph. Theoretical 2D Raman band of strained graphene. Phys. Rev. B 2013, 87, 155425.1–155425.7. [Google Scholar]
- Huang, Y.; Wu, J.; Hwang, K.C. Thickness of graphene and single-wall carbon nanotubes. Phys. Rev. B 2006, 74, 245413.1–245413.9. [Google Scholar]
- Lu, Q.; Huang, R. Nonlinear mechanics of single-atomic-layer graphene sheets. Int. J. Appl. Mechan 2009, 1, 443–447. [Google Scholar]
- Marenć, E.; Ibrahimbegovic, A.; Sorić, J.; Guidault, P.A. Homogenized elastic properties of graphene for small deformations. Materials 2013, 6, 3764–3782. [Google Scholar]
- Tapaszto, L.; Dumitrica, T.; Kim, S.J.; Nemes-Incze, P.; Hwang, C.; Biro, L.P. Breakdown of continuum mechanics for nanometre-wavelength rippling of graphene. Nat. Phys 2012, 8, 739–742. [Google Scholar]
- Marianetti, C.A.; Yevick, H.G. Failure mechanisms of graphene under tension. Phys. Rev. Lett 2010, 105, 245502.1–245502.4. [Google Scholar]
- Bosak, A.; Krisch, M.; Mohr, M.; Maultzsch, J.; Thomsen, C. Elasticity of single-crystalline graphite: Inelastic x-ray scattering study. Phys. Rev. B 2007, 75, 153408.1–153408.4. [Google Scholar]
- Politano, A.; Raimondo Marino, A.; Campi, D.; Farías, D.; Miranda, R.; Chiarello, G. Elastic properties of a macroscopic graphene sample from phonon dispersion measurements. Carbon 2012, 50, 4903–4910. [Google Scholar]
- Michel, K.H.; Verberck, B. Theory of the elastic constants of graphite and graphene. Phys. Stat. Sol. (b) 2008, 245, 2177–2180. [Google Scholar]
- Lazzeri, M.; Attaccalite, C.; Wirtz, L.; Mauri, F. Impact of the electron-electron correlation on phonon dispersion: Failure of LDA and GGA DFT functionals in graphene and graphite. Phys. Rev. B 2008, 78, 081406R.1–081406R.4. [Google Scholar]
- Robertson, D.H.; Brenner, D.W.; Mintmire, J.W. Energetics of nanoscale graphitic tubules. Phys. Rev. B 1992, 45, 12592–12595. [Google Scholar]
- Arroyo, M.; Belytschko, T. Finite crystal elasticity of carbon nanotubes based on the exponential Cauchy-Born rule. Phys. Rev. B 2004, 69(115415), 1–11541511. [Google Scholar]
- Lu, Q.; Arroyo, M.; Huang, R. Elastic bending modulus of monolayer graphene. J. Phys. D 2009, 42, 102002.1–102002.5. [Google Scholar]
- Zakharchenko, K.V.; Katsnelson, M.I.; Fasolino, A. Finite temperature lattice properties of graphene beyond the quasiharmonic approximation. Phys. Rev. Lett 2009, 102, 046808.1–046808.4. [Google Scholar]
- Kalosakas, G.; Lathiotakis, N.N.; Galiotis, C.; Papagelis, K. In-plane force fields and elastic properties of graphene. J. Appl. Phys 2013, 113, 134307.1–134307.7. [Google Scholar]
- Tsai, J.L.; Tu, J.F. Characterizing mechanical properties of graphite using molecular dynamics simulation. Mater. Des 2010, 31, 194–199. [Google Scholar]
- Hernandez, E.; Goze, C.; Bernier, P.; Rubio, A. Elastic properties of C and Bx Cy Nz composite nanotubes. Phys. Rev. Lett 1998, 80, 4502–4505. [Google Scholar]
- Xin, Z.; Jianjun, Z.; Zhong-can, O.Y. Strain energy and Young modulus of single-wall carbon nanotubes calculated from energy-band theory. Phys. Rev. B 2000, 62, 13692–13696. [Google Scholar]
- Popov, V.N.; University of Sofia, Sofia, Bulgaria. Private communication. 2012.
- Adams, G.B.; Sankey, O.F.; Page, J.B.; O’Keeffe, M.; Drabold, D.A. Energetics of large fullerenes: Balls, tubes, and capsules. Science 1992, 256, 1792. [Google Scholar]
- Van Lier, G.; van Alsenoy, C.; van Doren, V.; Geerlings, P. Ab initio study of the elastic properties of single-walled carbon nanotubes and graphene. Chem. Phys. Lett 2000, 326, 181–185. [Google Scholar]
- Kudin, K.N.; Scuseria, G.E.; Yakobson, B.I. C2F, BN, and C nanoshell elasticity from ab initio computations. Phys. Rev. B 2001, 64(235406), 1–23540610. [Google Scholar]
- Sanchez-Portal, D.; Artacho, E.; Soler, J.M.; Rubio, A.; Ordejon, P. Ab initio structural, elastic, and vibrational properties of carbon nanotubes. Phys. Rev. B 1999, 59, 12678–12688. [Google Scholar]
- Koskinen, P.; Kit, O.O. Approximate modeling of spherical membranes. Phys. Rev. B 2010, 82, 235420.1–235420.5. [Google Scholar]
- Leenaerts, O.; Peelaers, H.; Hernández-Nieves, A.D.; Partoens, B.; Peeters, F.M. First-principles investigation of graphene uoride and graphane. Phys. Rev. B 2010, 82, 195436.1–195436.5. [Google Scholar]
- Wei, X.; Fragneaud, B.; Marianetti, C.A.; Kysar, J.W. Nonlinear elastic behavior of graphene: Ab initio calculations to continuum description. Phys. Rev. B 2009, 80, 205407.1–205407.8. [Google Scholar]
- Shao, T.; Wen, B.; Melnik, R.; Yao, S.; Kawazoe, Y.; Tian, Y. Temperature dependent elastic constants and ultimate strength of graphene and graphyne. J. Chem. Phys 2012, 137, 194901.1–194901.8. [Google Scholar]
- Wagner, P.; Ivanovskaya, V.V.; Rayson, M.J.; Briddon, P.R.; Ewels, C.P. Mechanical properties of nanosheets and nanotubes investigated using a new geometry independent volume definition. J. Phys 2013, 25, 155302.1–155302.5. [Google Scholar]
- Liu, X.; Metcalf, T.H.; Robinson, J.T.; Houston, B.H.; Scarpa, F. Shear modulus of monolayer graphene prepared by chemical vapor deposition. Nano Lett 2012, 12, 1013–1017. [Google Scholar]
- Lee, G.H.; Cooper, R.C.; An, S.J.; Lee, S.; van der Zande, A.; Petrone, N.; Hammerberg, A.G.; Lee, C.; Crawford, B.; Oliver, W.; Kysar, J.W.; Hone, J. High-strength chemical-vapor deposited graphene and grain boundaries. Science 2013, 340, 1073–1076. [Google Scholar]
- Kelly, B.T. Physics of Graphite; Applied Science Publishers: London, UK, 1981; Volume 62. [Google Scholar]
- Blakslee, O.L.; Proctor, D.G.; Seldin, E.J.; Spence, G.B.; Weng, T. Elastic constants of compression annealed pyrolytic graphite. J. Appl. Phys 1970, 41, 3373–3382. [Google Scholar]
- Zhang, Z.; Li, T. Determining graphene adhesion via substrate-regulated morphology of graphene. J. Appl. Phys 2011, 110, 083526.1–083526.5. [Google Scholar]
- Yakobson, B.I.; Brabec, C.J.; Bernholc, J. Nanomechanic of carbon tubes: Instabilities beyond linear response. Phys. Rev. Lett 1996, 76, 2511–2514. [Google Scholar]
- Harik, V.M. Mechanics of carbon nanotube: Applicability of the continuum-beam models. Comput. Mater. Sci 2002, 24, 328–342. [Google Scholar]
- Oshima, C.; Nagashima, A. Ultra-thin epitaxial films of graphite and hexagonal boron nitride on solid surfaces. J. Phys 1997, 9, 1–20. [Google Scholar]
- Mohr, M.; Maultzsch, J.; Dobard, E.; Reich, S.; Milosevic, I.; Damnjanovic, M.; Bosak, A.; Krisch, M.; Thomsen, C. The phonon dispersion of graphite by inelastic X-ray scattering. Phys. Rev. B 2007, 76, 035439.1–035439.7. [Google Scholar]
- Jorio, A.; Pimenta, M.A.; Filho, A.G.S.; Saito, R.; Dresselhaus, G.; Dresselhaus, M.S. Characterizing carbon nanotube samples with resonance Raman scattering. New J. Phys 2003, 5, 139.1–139.17. [Google Scholar]
- Jorio, A.; Saito, R.; Hafner, J.H.; Lieber, C.M.; Hunte, M.; McClure, T.; Dresselhaus, G.; Dresselhaus, M.S. Structural (n,m) Determination of Isolated Single-Wall Carbon Nanotubes by Resonant Raman Scattering. Phys. Rev. Lett 2001, 86, 1118–1121. [Google Scholar]
- Meyer, C.; Paillet, M.; Michel, T.; Moreac, A.; Neumann, A.; Duesberg, G.S.; Roth, S.; Sauvajol, J.L. Raman-modes of index-identified free-standing single-walled carbon nanotubes. Phys. Rev. Lett 2005, 95, 217401.1–217401.4. [Google Scholar]
- Liu, K.; Wang, W.; Wu, M.; Xiao, F.; Hong, X.; Aloni, S.; Bai, X.; Wang, E.; Wang, F. Intrinsic radial breathing oscillation in suspended single-walled carbon nanotubes. Phys. Rev. B 2011, 83, 113404.1–113404.4. [Google Scholar]
- Jiang, J.W.; Wang, J.S.; Li, B. Young’s modulus of graphene: A molecular dynamics study. Phys. Rev. B 2009, 80(113405), 1–1134054. [Google Scholar]
- Min, K.; Aluru, N.R. Mechanical properties of graphene under shear deformation. Appl. Phys. Lett 2011, 98, 013113.1–013113.3. [Google Scholar]
- Lucas, A.A.; Lambin, P.; Smalley, R.E. On the energetics of tubular fullerenes. J. Phys. Chem. Solids 1993, 54, 587–593. [Google Scholar]
- Brenner, D.W.; Shenderova, O.A.; Harrison, J.A.; Stuart, S.J.; Ni, B.; Sinnott, S.B. A second generation reactive empirical bond order (REBO) potential energy expression for hydrocarbons. J. Phys 2002, 14, 783–802. [Google Scholar]
- Brenner, D.W. Empirical potential for hydrocarbons for use in simulating the chemical vapor deposition of diamond films. Phys. Rev. B 1990, 42, 9458–9471. [Google Scholar]
- Frank, I.W.; Tanenbauma, D.M.; van der Zande, A.M.; McEuen, P.L. Mechanical properties of suspended graphene sheets. J. Vac. Sci. Technol. B 2007, 25, 2558–2561. [Google Scholar]
- Gómez-Navarro, C.; Burghard, M.; Kern, K. Elastic properties of chemically derived single graphene sheets. Nano Lett 2008, 8, 2045–2049. [Google Scholar]
- Lee, C.; Wei, X.; Kysar, J.W.; Hone, J.H. Measurement of the elastic properties and intrinsic strength of monolayer graphene. Science 2008, 321, 385–387. [Google Scholar]
- Grantab, R.; Shenoy, V.B.; Ruoff, R.S. Anomalous strength characteristics of tilt grain boundaries in graphene. Science 2010, 330, 946–948. [Google Scholar]
- Kotakoski, J.; Meyer, J.C. Mechanical properties of polycrystalline graphene based on a realistic atomistic model. Phys. Rev. B 2012, 85, 195447.1–195447.6. [Google Scholar]
- Wei, Y.; Wu, J.; Yin, H.; Shi, X.; Yang, R.; Dresselhaus, M. The nature of strength enhancement and weakening by pentagonheptagon defects in graphene. Nat. Mater 2012, 11, 759–763. [Google Scholar]
- Awasthi, A.P.; Lagoudas, D.C.; Hammerand, D.C. Modeling of graphene-polymer interfacial mechanical behavior using molecular dynamics. Modell. Simul. Mater. Sci. Eng 2009, 17, 015002.1–015002.37. [Google Scholar]
- Rissanou, A.N.; Harmandaris, V. A molecular dynamics study of polymer/graphene nanocomposites. Macromol. Symp 2013, 331–332, 43–49. [Google Scholar]
- Shames, I.H.; Dym, C.L. Energy and Finite Element Method in Structural Mechanics; New Age International: New Dehli, India, 1995; Volume 421. [Google Scholar]
- Zhang, Y.; Liu, F. Maximum asymmetry in strain induced mechanical instability of graphene: Compression versus tension. Appl. Phys. Lett 2011, 99, 241908.1–241908.3. [Google Scholar]
- Landau, L.D.; Lifshitz, E.M. Theory of Elasticity; Pergamon Press: Oxford, UK, 1970; Volume 96. [Google Scholar]
- Allard, A.; Wirtz, L. Graphene on metallic substrates: Suppression of the Kohn anomalies in the phonon dispersion. Nano Lett 2010, 10, 4335–4540. [Google Scholar]
- Allard, A. Etude ab initio des phonons du graphène sur substrats métalliques. Ph.D. Thesis, Université de Lille, Villeneuve d’Ascq, France, December 2011. [Google Scholar]
- Olsen, T.; Thygesen, K.S. Random phase approximation applied to solids, molecules, and graphene-metal interfaces: From van der Waals to covalent bonding. Phys. Rev. B 2013, 87(075111), 1–07511113. [Google Scholar]
- Yoon, T.; Shin, W.C.; Kim, T.Y.; Mun, J.H.; Kim, T.S.; Cho, B.J. Direct measurement of adhesion energy of monolayer graphene as-grown on copper and its application to renewable transfer process. Nano Lett 2012, 12, 1448–1452. [Google Scholar]
- He, Y.; Chen, W.F.; Yu, W.B.; Ouyang, G.; Yang, G.W. Anomalous interface adhesion of graphene membrane. Sci. Rep 2013, 3, 2660.1–2660.6. [Google Scholar]
- Mao, Y.; Wang, W.L.; Wei, D.; Kaxiras, E.; Sodroski, J.G. Graphene structures at an extreme degree of buckling. ACS Nano 2011, 5, 1395–1400. [Google Scholar]
- Cerda, E.; Mahadevan, L. Geometry and physics of wrinkling. Phys. Rev. Lett 2003, 90, 074302.1–074302.4. [Google Scholar]
- Chen, C.C.; Bao, W.; Theiss, J.; Dames, C.; Lau, C.N.; Cronin, S.B. Raman spectroscopy of ripple formation in suspended graphene. Nano Lett 2009, 9, 4172–4176. [Google Scholar]
- Abramowitz, M.; Stegun, I.A. Handbook of Mathematical Functions; National Bureau of Standards: Washington, DC, USA, 1972; Volume 590. [Google Scholar]
- Wang, Z.; Devel, M. Periodic ripples in suspended graphene. Phys. Rev. B 2011, 83, 125422.1–125422.4. [Google Scholar]
- Shibuta, Y.; Elliott, J.A. Interaction between two graphene sheets with a turbostratic orientational relationship. Chem. Phys. Lett 2011, 512, 146–150. [Google Scholar]
- Polizzotto, C. Nonlocal elasticity and related variational principles. Inte. J. Solids Struct 2001, 38, 7359–7380, and refereces therein. [Google Scholar]
- Eringen, A.C. On differential equations of nonlocal elasticity and solutions of screw dislocation and surface waves. J. Appl. Phys 1983, 54, 4703–4710. [Google Scholar]
- Lu, P.; Zhang, P.Q.; Lee, H.P.; Wang, C.M.; Reddy, J.N. Non-local elastic plate theories. Proc. R. Soc. A 2007, 463, 3225–3240. [Google Scholar]
- Hashemi, S.H.; Samaei, A.T. Buckling analysis of micro/nanoscale plates via nonlocal elasticity theory. Physica E 2011, 43, 1400–1404. [Google Scholar]
- Narendar, S.; Gopalakrishnan, S. Nonlocal flexural wave propagation in a embedded graphene. Int. J. Comput 2012, 6, 29–36. [Google Scholar]
- Pradhan, S.C. Buckling of single layer graphene sheet based on nonlocal elasticity and higher order shear deformation theory. Phys. Lett. A 2009, 373, 4182–4188. [Google Scholar]
- Zhao, H.; Aluru, N.R. Temperature and strain-rate dependent fracture strength of graphene. Appl. Phys. J 2010, 108, 064321.1–064321.5. [Google Scholar]
- Zhang, Y.Y.; Gu, Y.T. Mechanical properties of graphene: Effects of layer number, temperature and isotope. Comput. Mater. Sci 2013, 71, 197–200. [Google Scholar]
- Girifalco, L.A.; Ladd, R.A. Energy of cohesion, compressibility, and the potential energy functions of the graphite system. J. Chem. Phys 1956, 25, 693–697. [Google Scholar]
- Sachs, B.; Wehling, T.O.; Katsnelson, M.I.; Lichtenstein, A.I. Adhesion and electronic structure of graphene on hexagonal boron nitride substrates. Phys. Rev. B 2011, 84, 195414.1–195414.8. [Google Scholar]


| A (N/m) Equation (2) | ν Equation (2) | C (N/m) Equation (4) | g (N/m) Equation (3) | D (eV) Equation (7) | ν′ Equation (7) | Method |
|---|---|---|---|---|---|---|
| 372 | 0.125 | 366 | 163 | Elastic constants of graphite [20]: A = hC11, = C12=C11, , g = h(C11 C22)=2 with h the graphite interlayer distance (0.335 nm) | ||
| 368 | 149 | 1.75 | HREELS phonon dispersion measurements [21] | |||
| 405 | 156 | 1.85 | Phonons dispersion (derived from experimental data) [22] | |||
| 363 | 146 | 1.33 | Phonons dispersion (GW-DFT calculations) [23] radial breathing mode of carbon SWNTs (see text) | |||
| 350 ± 65 | ||||||
| 0.412 | 236 | 83 | 0.85 | 1st generation Brenner potential [24,25] | ||
| 0.91 | 2nd generation Brenner potential [4] | |||||
| 289 | 0.397 | 243 | 1.39 | 2nd generation Brenner potential [16,25,26] | ||
| 355 | 0.15 | 1.1 | Monte-Carlo, empirical bond-order potential [3,27] | |||
| 0.22 | 320 | DFT-derived in-plane force field [28] | ||||
| 0.26 | 312 | 122 | AMBER 3D force field [29] | |||
| 410 | 1.88 | Non-orthogonal tight-binding [30] | ||||
| 0.24 | 358 | 1.10 | Non-orthogonal tight-binding [31] | |||
| 407 | 0.281 | Non-orthogonal tight-binding [32] | ||||
| 1.62 | Non self-consistent ab-initio [33] | |||||
| 377 | Hartree Fock 6-31G* [34] | |||||
| 0.149 | 345 | DFT Gaussian orbitals [35] | ||||
| 370 | 1.53–1.65 | LDA SIESTA [36] | ||||
| 1.61 | 0.56 | DFT tight-binding [37] | ||||
| 0.17 | 336 | DFT ABINIT code[38] | ||||
| 358 | 0.169 | DFT VASP program [39] | ||||
| 361 | 0.184 | 350 | LDA Vienna VASP [40] | |||
| 0.20 | 350 | DFT AIMPRO code [41] | ||||
| 95 | Measurement of torsional modulus [42] | |||||
| 330 ± 15 | Experimental AFM nano-indentation [43] | |||||
| 365 | 0.205 | 350 | 145 | 1.6 | Best fit (see Section 9) |
| References | C11 | C12 | C13 | C33 | C44 | C66 |
|---|---|---|---|---|---|---|
| Reference [45] | 1060(20) | 180(20) | 15(5) | 36.5(10) | 0.18–0.35 | 440(40) |
| Reference [20] | 1109(16) | 139(36) | 0(3) | 38.7(7) | 5.0(3) | 485(10) |
| ct (km/s) | cl(km/s) | α(m2/s) | Phonon band structure |
|---|---|---|---|
| 14.0 | 22.0 | 6.1 × 10−7 | HREELS measurements [21] |
| 14.3 | 23.1 | 6.25 × 10−7 | semi-experimental [22] |
| 13.8 | 21.8 | 5.28 × 10−7 | ab-initio calculations [23] |
| Substrate | ZA phonon frequency at Γ (cm−1) [72] | K(J/m4) | (N/m) | (nm) | |
|---|---|---|---|---|---|
| Co(001) | 295 | 2.35 × 1021 | 49.1 | 0.64 | 0.14 |
| Ni(111) | 240 | 1.56 × 1021 | 40.0 | 0.71 | 0.11 |
| Cu(111) | 45 | 5.48 × 1019 | 7.5 | 1.64 | 0.021 |
| SiO2 | [a] | 1.5 × 1020 | 12 | 1.3 | 0.036 |
| gr(0001) | [b] | 1.08 × 1020 | 10.5 | 1.39 | 0.030 |
| h-BN(0001) | [b] | 7.60 × 1019 | 8.8 | 1.51 | 0.025 |
© 2014 by the authors; licensee MDPI, Basel, Switzerland This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Lambin, P. Elastic Properties and Stability of Physisorbed Graphene. Appl. Sci. 2014, 4, 282-304. https://doi.org/10.3390/app4020282
Lambin P. Elastic Properties and Stability of Physisorbed Graphene. Applied Sciences. 2014; 4(2):282-304. https://doi.org/10.3390/app4020282
Chicago/Turabian StyleLambin, Philippe. 2014. "Elastic Properties and Stability of Physisorbed Graphene" Applied Sciences 4, no. 2: 282-304. https://doi.org/10.3390/app4020282
APA StyleLambin, P. (2014). Elastic Properties and Stability of Physisorbed Graphene. Applied Sciences, 4(2), 282-304. https://doi.org/10.3390/app4020282






