Review of Magnetoelectric Effects on Coaxial Fibers of Ferrites and Ferroelectrics

Abstract

Composites of ferromagnetic and ferroelectric phases are of interest for studies on mechanical strain-mediated coupling between the two phases and for a variety of applications in sensors, energy harvesting, and high-frequency devices. Nanocomposites are of particular importance since their surface area-to-volume ratio, a key factor that determines the strength of magneto-electric (ME) coupling, is much higher than for bulk or thin-film composites. Core–shell nano- and microcomposites of the ferroic phases are the preferred structures, since they are free of any clamping due to substrates that are present in nanobilayers or nanopillars on a substrate. This review concerns recent efforts on ME coupling in coaxial fibers of spinel or hexagonal ferrites for the magnetic phase and PZT or barium titanate for the ferroelectric phase. Several recent studies on the synthesis and ME measurements of fibers with nickel ferrite, nickel zinc ferrite, or cobalt ferrite for the spinel ferrite and M-, Y-, and W-types for the hexagonal ferrites were considered. Fibers synthesized by electrospinning were found to be free of impurity phases and had uniform core and shell structures. Piezo force microscopy (PFM) and scanning microwave microscopy (SMM) measurements of strengths of direct and converse ME effects on individual fibers showed evidence for strong coupling. Results of low-frequency ME voltage coefficient and magneto-dielectric effects on 2D and 3D films of the fibers assembled in a magnetic field, however, were indicative of ME couplings that were weaker than in bulk or thick-film composites. A strong ME interaction was only evident from data on magnetic field-induced variations in the remnant ferroelectric polarization in the discs of the fibers. Follow-up efforts aimed at further enhancement in the strengths of ME coupling in core–shell composites are also discussed in this review.

1. Introduction

Single phase and composite multiferroics have been of significant interest for studies on the nature of cross-coupling between ordered magnetic and electric subsystems and for a variety of devices and sensors [1,2,3,4,5,6,7]. Multiferroicity was first predicted in Cr₂O₃ and then verified by experiments [8]. Although coexisting multiferroic orders occur only at very low temperatures in most of the single phase materials, BiFeO₃ and several hexagonal ferrites are reported to exhibit both magnetic and ferroelectric/anti-ferroelectric orderings at room temperature, but the coupling between the two ferroic orderings is rather weak [9,10]. This review concerns magneto-electric (ME) coupling in multiferroic composites consisting of ferrimagnetic and ferroelectric phases [3,4,5]. The ME effects on such composites arise due to the transfer of mechanical strain produced in one of the two phases in a magnetic or an electric field to the other phase. The piezomagnetic strain in the magnetic phase under an applied magnetic field H leads to variations in the order parameters such as polarization, dielectric permittivity, etc., in the ferroelectric phase and is termed the direct ME (DME) effect [4]. The induced polarization P is related to H by P = αH, where α is ME susceptibility, and is a measure of the strength of the ME coupling in the material. In experiments, however, one may measure the magneto-electric voltage coefficient (MEVC) α_E = δE/δH by applying an AC magnetic field δH and measuring the induced AC electric field δE = δV/t, where δV is the induced voltage and t is the composite thickness. The MEVC is related to α by α_E = (1/ε₀ε_r)α, where ε₀ is the permittivity of free space, and ε_r is the relative permittivity of the material. One may also measure the strength of ME coupling by the converse ME (CME) effect, i.e., piezoelectric strain in an applied E-field resulting in variations in the magnetic order parameter such as the magnetization and anisotropy field.

In order to achieve a strong DME in a composite, one prefers a ferromagnetic material with a high piezomagnetic coupling coefficient q = dλ/dH, where λ is the magnetostriction and high initial permeability for the confinement of applied magnetic fields [4]. A ferroelectric with a large d/ε_r, where d is the piezoelectric coupling coefficient, is preferred. For a strong CME, a ferromagnetic phase with a large λ and a small saturation magnetization M_s and a ferroelectric phase with high d-values are preferred [4]. Numerous composite systems with ferromagnetic phases such as 3D transition metals/alloys, rare earth metals/alloys, spinel and hexagonal ferrites, ferroelectric lead zirconate titanate (PZT), barium titanate (BTO), or lead magnesium niobate–lead titanate (PMN-PT) show strong ME coupling [9,10,11].

The mechanical connectivity between the ferroic phases in composites is one of the key factors that determine the strength of ME interactions [12]. Composites with a variety of connectivity including 0–3 (particles of one of the two phases with no connectivity to itself dispersed in a matrix of the second phase with connectivity to itself in all three dimensions), 1-3 (pillars of one phase in a 3D matrix of the second phase), 2-2 (bilayer or multilayers of the two phases with connectivity to itself in 2D), 3-3 (a bulk composite with 3D connectivity for both phases), etc., were studied in the past [3,4,5,6,7,11,13,14,15,16]. The most effective connectivity was realized in 2-2 composites, which can be formed as thick laminates, thin films, or co-fired ceramics. In 2-2 composites, the issue of leakage current was eliminated, which was the cause of poor ME coupling in bulk composites synthesized by mixing and co-firing the two phases [4].

Another key factor that determines the ME coupling strength is the surface-to-volume ratio for the composites since the coupling originates essentially from the strain transfer at the interface of the two phases. One therefore expects a strong ME coupling in nano- and micro-composites compared with bulk structures. Nanocomposites studied in recent years include ordered arrays [17,18,19,20,21,22], thin films [23,24], core–shell particles [25,26,27], coaxial wires [28,29,30,31,32], nanopillars [33,34,35,36], and core–shell fibers [37,38,39]. Coaxial fibers, in particular, are of interest due to structural features suitable for realizing strong ME effects. In the case of nanobilayer or nanopillar composites on a substrate, the substrate clamping could result in significant reduction in the strengths of ME interactions [17]. Ordered arrays and nanopillars could be fabricated such that the clamping effect is minimized but not completely eliminated, whereas core–shell fibers are free of any such clamping effects [37,38,39,40,41,42,43,44]. Coaxial nanofibers could be fabricated by low-cost techniques such as electrospinning, which have been shown to be ideal for the synthesis of composites with uniform cores and shells and free of interface defects. Nano- and microfiber composites, therefore, are one of the most promising candidates for achieving strong ME coupling and for application in sensors, energy harvesting, etc.

Although there have only been a few reports on core–shell multiferroic composites in recent years, they do provide clear evidence for the potential to miniaturize ME composite structures and also achieve strong cross-coupling in them. This review article on core–shell micro- and nanofiber composites is aimed at highlighting key advances in this category of multiferroic materials and to promote activities in this important topic of interest, in particular, for applications in magnetic sensors for medical imaging and dual electric and magnetic field tunable high-frequency microwave and millimeter wave devices. The focus here is on structures with spinel ferrites or hexagonal ferrites for the ferromagnetic phase and ferroelectric PZT or BTO. Several ferrimagnetic spinel ferrites, including pure and Zn-substituted nickel and cobalt ferrites, have desirable order parameters such as large magnetostriction λ and piezomagnetic coupling coefficient q, and high Curie temperature T_c for use in the composites [45]. Similarly, hexaferrites with large uniaxial or planar magneto-crystalline anisotropy fields are of interest for composites with self-magnetic bias and are preferred for applications in magnetic sensors and energy harvesting [46,47]. Fibers with cobalt ferrite (CFO), nickel ferrite (NFO), Zn-substituted NFO, and M-, Y-, and W-type hexagonal ferrites for the magnetic phase and PZT or BTO for the ferroelectric phase are considered here. Synthesis by electrospinning, structural characterization by X-ray diffraction, electron microscopy, and scanning probe microscopy are described. Characterization of the fibers in terms of ferroic order parameters was carried out in most of the studies covered in this review. Results on the measurements of strengths of DME by low-frequency MEVC in fibers assembled into 2D and 3D films in a magnetic field, static magnetic field H-induced ferroelectric polarization, and piezo force microscopy (PFM) under applied H are provided. Converse ME effects by voltage tuning of ferromagnetic resonance (FMR) and magnetic force microscopy (MFM) under an electric field are discussed. Finally, we conclude with a brief discussion on follow-up studies and avenues for potential device applications for the core–shell fiber composites.

2. Theory

In this section, we review the results of theoretical estimates on the strengths of ME interactions in the coaxial fibers of spinel and hexagonal ferrites and ferroelectrics. Nickel ferrite was considered in the models due to its large magnetostriction λ and piezomagnetic coefficient q = dλ/dH [41]. Although cobalt ferrite has a large λ, the piezomagnetic coefficient q is small due to slow variation in λ under H and is yet to be considered for modeling. In another report, fibers with hexagonal ferrites were considered [47]. Ferrimagnetic hexagonal ferrites contain cubic spinel (S-) blocks and two types of hexagonal blocks, T and R, in their crystal structure. Depending on the arrangement of S, T, and/or R blocks, they are classified into several categories including M-, W-, Y-, U-types, etc. Most of them have either a uniaxial or a planar magneto-crystalline anisotropy field, H_a [46]. The choice for the ferroelectric phase in the fibers was either PZT or barium titanate (BTO), since their composites with ferrites are found to be free of any impurity phases. Both BTO and PZT have a large piezoelectric coefficient d. The important magnetic parameters, saturation magnetization 4πM_s, λ, and q for spinel ferrites and hexagonal ferrites and ferroelectric order parameters, d, and permittivity ε for PZT and BTO, used for theoretical estimates, are given in

Table 1. Room temperature piezoelectric coupling coefficient d₃₃ and dielectric permittivity ε₃₃/ε₀ for PZT and BTO and saturation magnetization 4πM_s, magnetostriction λ, and piezomagnetic coefficient q₃₃ for NFO, and Y- and W-type hexagonal ferrites. The parameters are the highest measured values for magnetic or electric fields along the length of the fiber (direction 3) and magnetostriction or piezoelectric coupling coefficients measured along the same direction. These values are used in the models in [41,47].

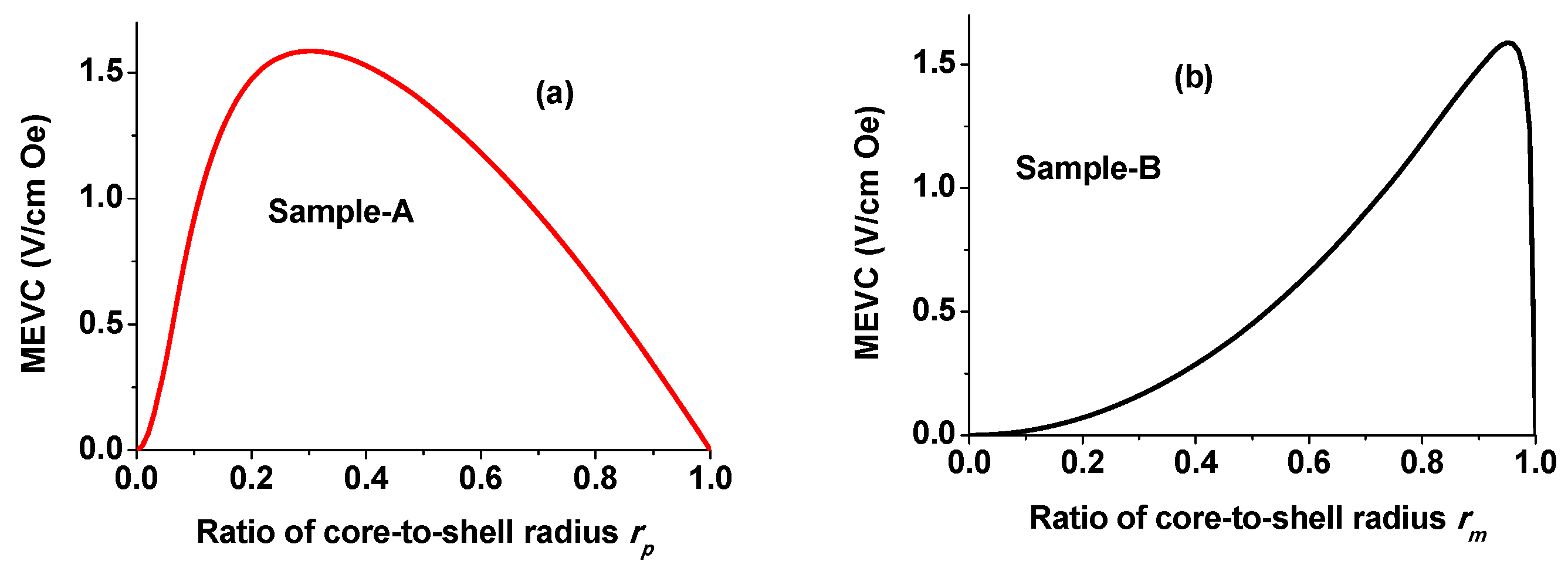
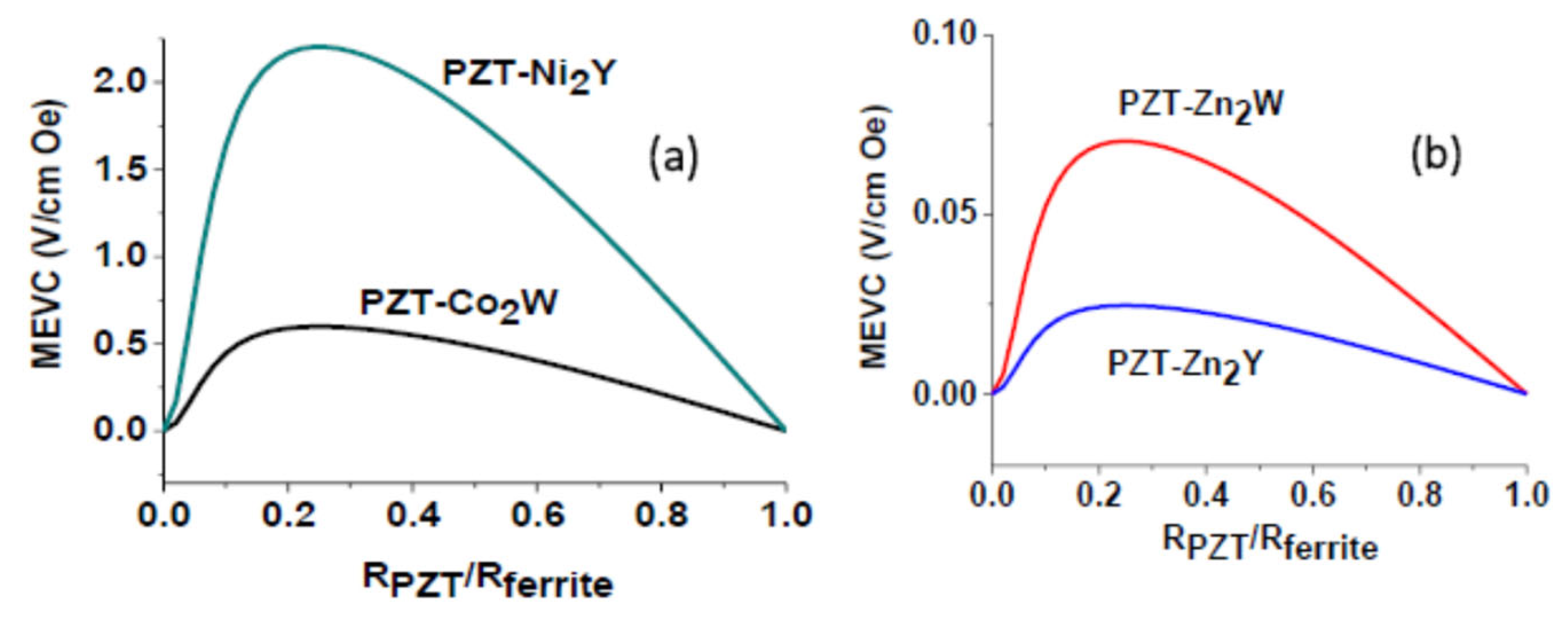
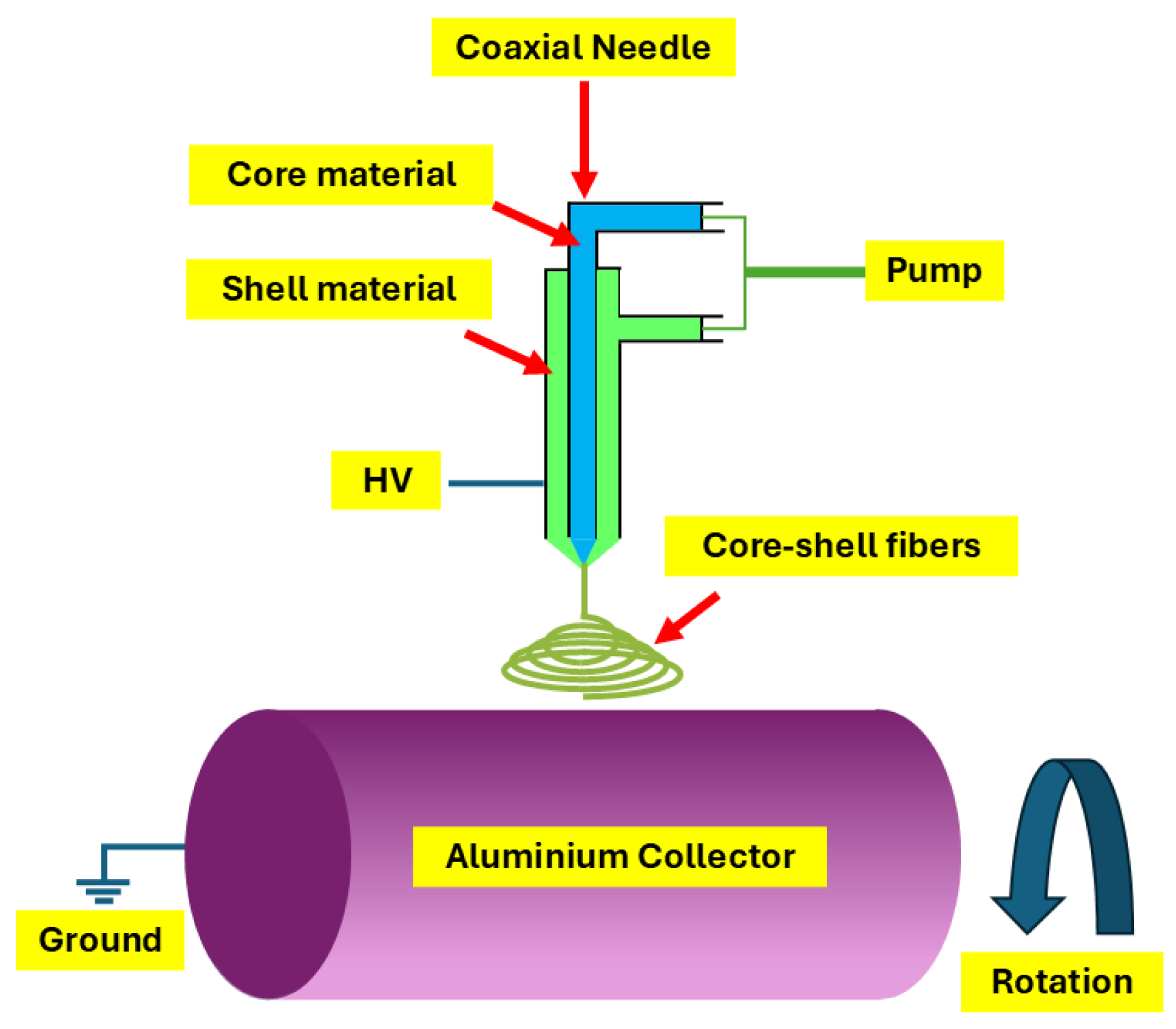
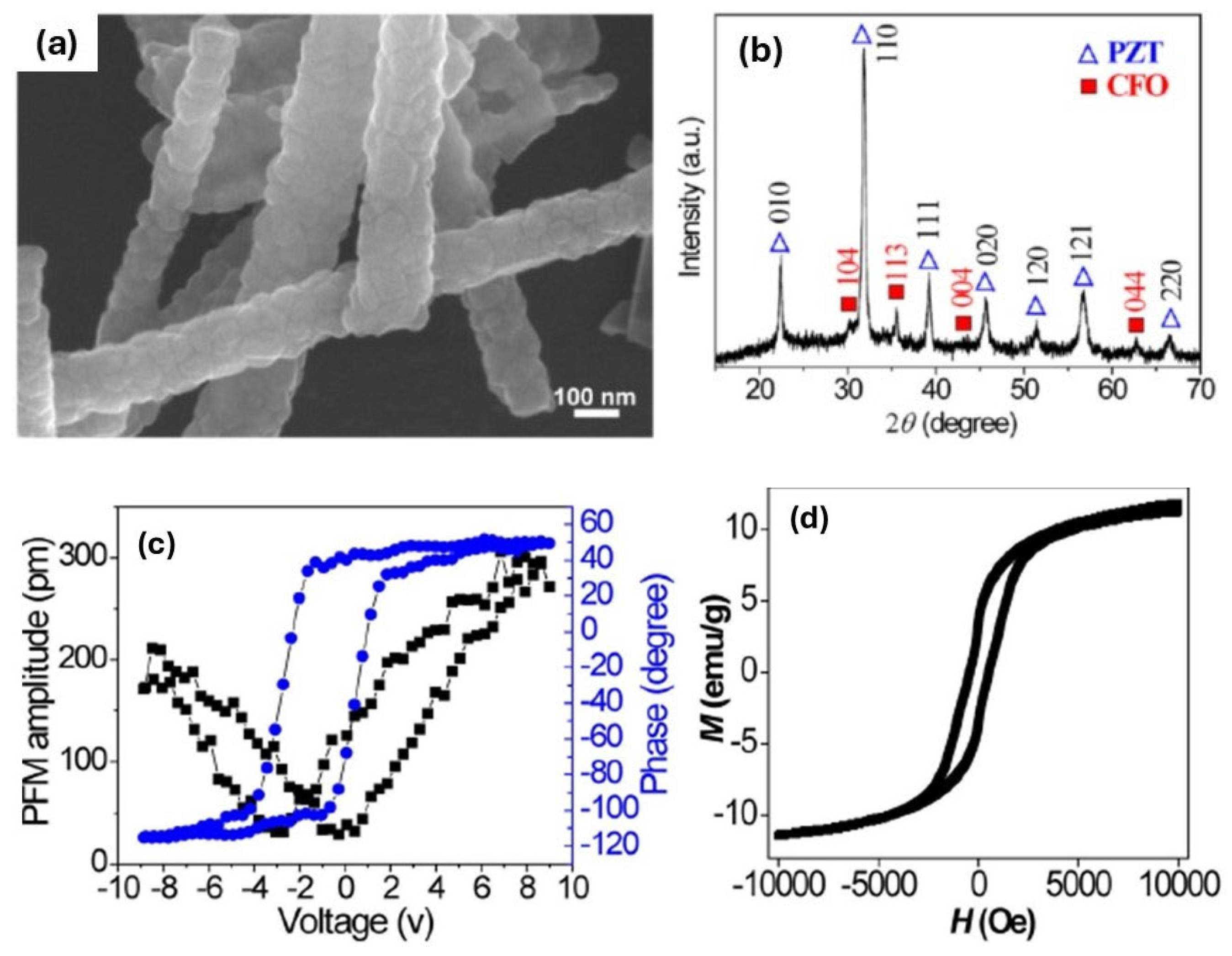
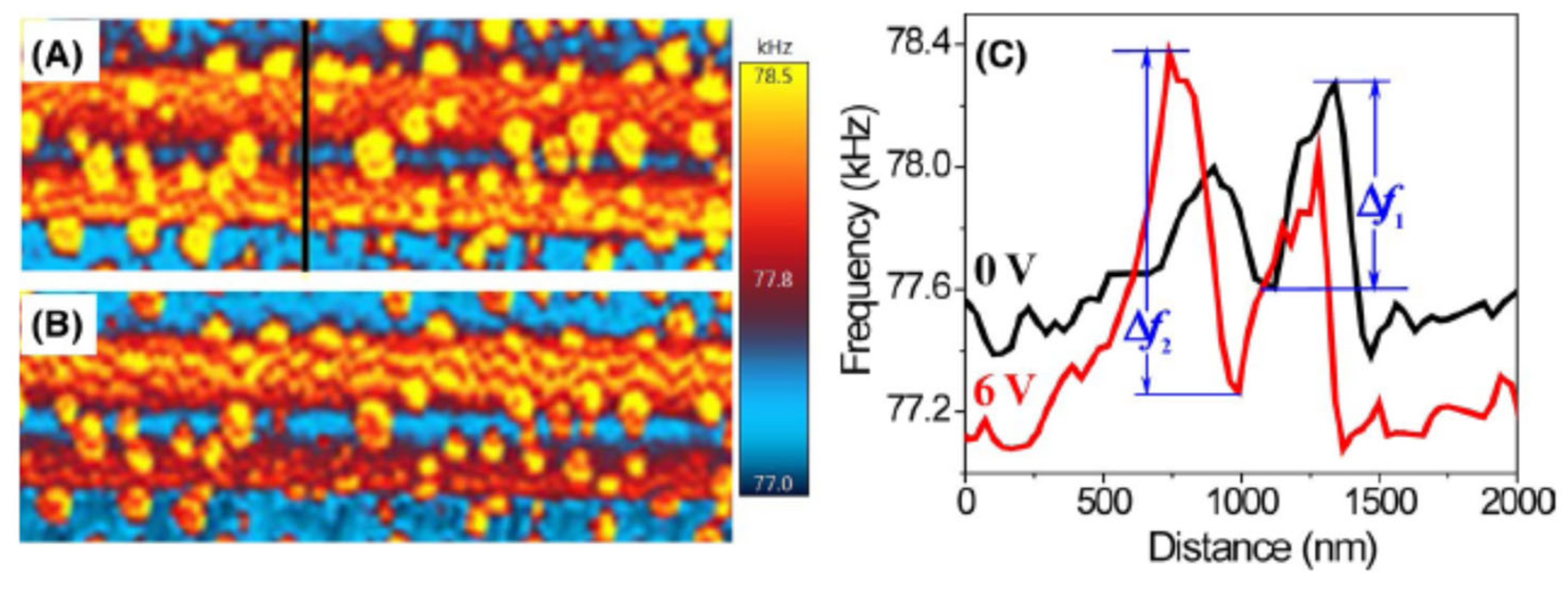
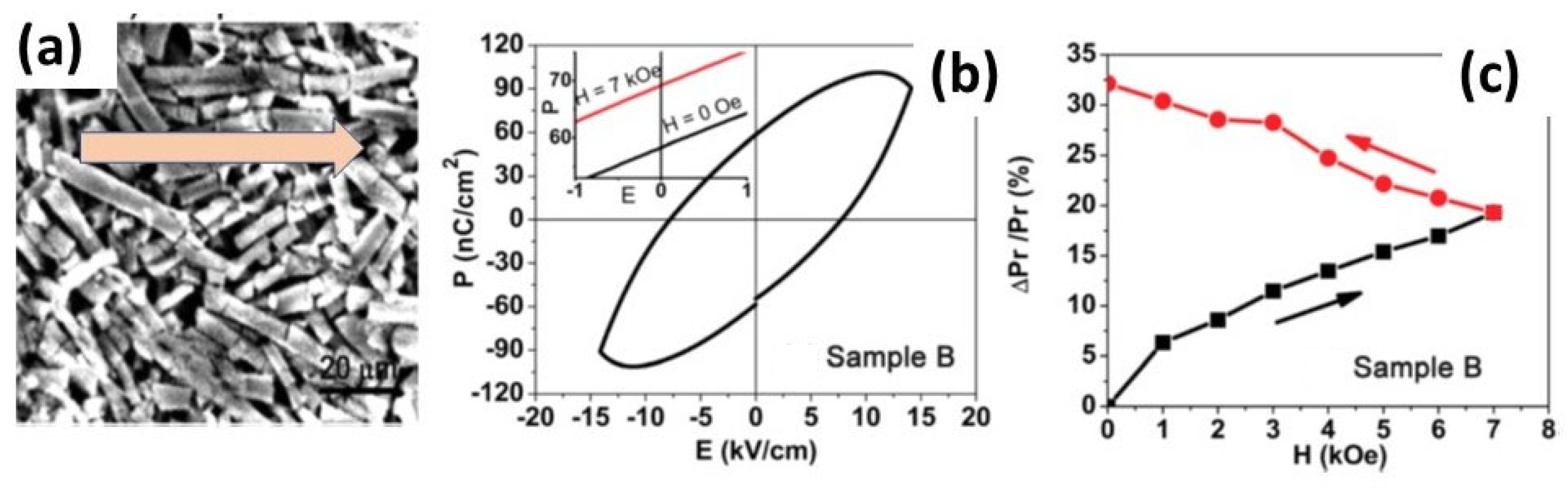
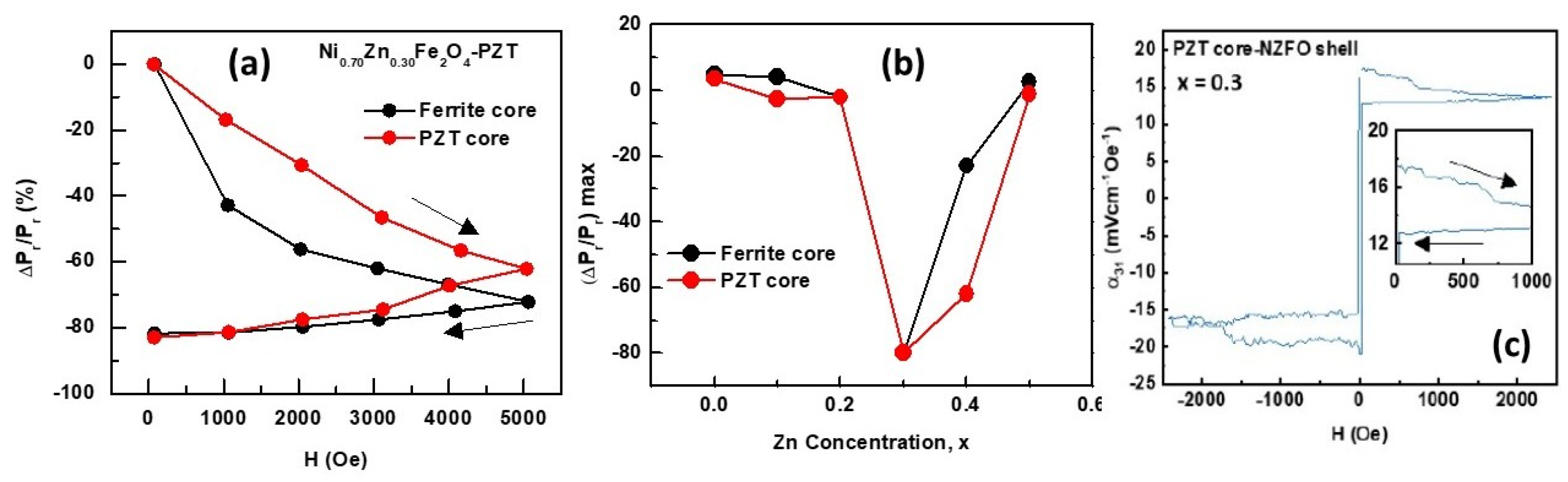
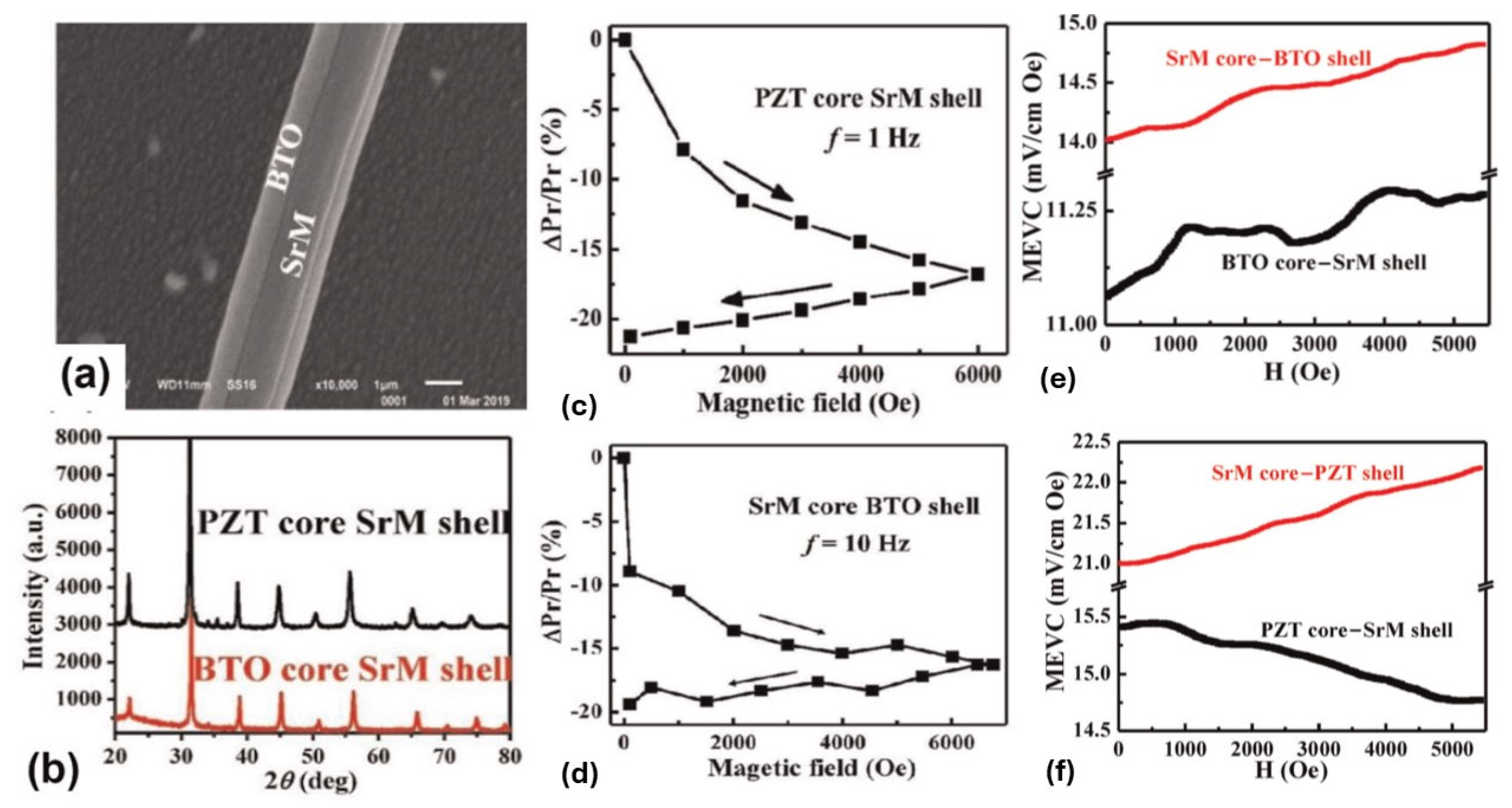
Material	4πMs (kG)	λ (10−6)	q33 (10−12 m/A)	d33 (10−12 m/V)	ε33/ε0
PZT	-			400	1750
BTO	-			260	2500
NFO	3.3	−30	−680		10
Co2W	2.3	−74	−170	-	10
Zn2W	1.5	−15	−20	-	10
Zn2Y	2.58	−10	−7	-	10
Ni2Y	7.51	−19	−625	-	10

. Estimates of strengths of direct ME effects in terms of the low-frequency MEVC for core–shell fibers of NFO-BTO [41] and Y- and W-type hexagonal ferrites and PZT [47] are considered next.

The theories developed are for the direct ME effect on a fiber with a length that is much greater than its radius. It is assumed that the fiber is subjected to a static magnetic field H and an AC magnetic field δH, both along the length of the fiber (direction 3). The resulting piezomagnetic deformation in the ferrite when transferred to the ferroelectric phase will produce an induced electric field δE. Figure 1 shows the estimated MEVC = δE/δH as a function of the ratio of the radius of the core to the radius of the fiber [41]. Figure 1a is for a fiber with a BTO core and an NFO shell (sample A). As r_p = core radius/shell radius increases from zero (i.e., in the absence of a BTO core), the MEVC increases from zero, is expected to reach a maximum value of 1.6 V/cm Oe for r_p = 0.33, and then decreases to zero for r_p = 1 when the fiber is BTO and does not contain a ferrite shell. Similar estimates for a fiber with an NFO core and a BTO shell (sample B) are shown in Figure 1b. A maximum MEVC~1.6 V/cm Oe is expected for this case when the radius ratio is 0.95. The dependence of MEVC in Figure 1 is significantly different from similar estimates for bulk or 2-2 laminate (layered) composites in which a maximum MEVC is predicted for composites with an equal volume fraction or thickness of the two phases [8].

Figure 2 shows the results of similar modeling for fibers with W- or Y-type hexagonal ferrites and PZT [47]. The material parameters in Table 1 are used for theoretical values of MEVC as a function of the ratio of the PZT core to the ferrite shell. MEVC is expected to be the largest for fibers with Ni₂Y and the smallest for fibers withZn₂Y. A maximum MEVC is expected when the ratio of the core-to-shell radius is ≈0.3. Fibers with Zn₂W or Zn₂Y have much lower MEVC compared with their counterparts due to a weak magnetostriction and q-value. Estimates for the hexaferrite core–PZT shell are provided in [47].

The results in Figure 1 and Figure 2 are for a single isolated fiber. A very significant reduction in the measured values of MEVC is expected, in particular, for assemblies of the fibers due to several assumptions in the theory. The theory does not consider the following: (i) The results in Figure 1 and Figure 2 are for material parameters measured in polycrystalline ferroelectric and magnetic phases. For nanomaterials, however, one anticipates much smaller ferroic order parameters. (ii) The theory did not take into account magnetic or electric dipole–dipole interactions. Interactions between ferrite cores or shells will lead to a demagnetizing field that will result in a reduction in the strengths of both DC and AC magnetic fields and a decrease in MEVC. (iii) Porosity or airgaps in the fiber assemblies will also have a similar effect on MEVC. Influences of these factors are considered in detail later in the discussion part (Section 6).

3. Nanofiber Synthesis

There are several techniques, including sol–gel routes [48,49], electrodeposition [50], template assisted synthesis [51], and electrospinning [39,40,41,42,43], that are utilized for the synthesis of coaxial fibers. Electrospinning has several advantages over other competing techniques for the synthesis of core shell fibers, e.g., (i) it is a low-cost synthesis technique compared with lithography or template-assisted processes; (ii) fibers of uniform core and shell structures free of any defects can be prepared; (iii) the technique can be easily scaled up for the large-scale synthesis of nano- and microfibers. In this review, we focus on fibers made by electrospinning. The synthesis in general involves the following steps [52,53]: The first step in the process involves the preparation of the sols for core and shell materials separately with precursors that are soluble in water/alcohol or that are liquid themselves. For ferrite fibers, one generally uses metal acetates or nitrates for the sol. A polymer, usually polyvinylpyrrolidone (PVP), is added to the solutions to make the sol viscous. Second, the sols are loaded onto two separate syringes and dispensed with a syringe pump, as in the setup shown schematically in Figure 3. The viscosity of the sols has to be adjusted for optimum pumping rates and to control the fiber dimensions.

Finally, the sol fed through a dual chamber stainless steel needle is subjected to an electric field of 1.5–2 kV/cm by applying a DC voltage between the needle and a metal drum used to collect the fibers (Figure 3). As the sols exit the needle tip they are electrically charged, and the repulsive Coulomb force leads to the formation of a Tyler cone and eventually to the formation of fibers of the ceramics that are collected on a rotating metal drum. As-spun fibers are dried at room temperature for 24 h and then annealed at a desired temperature in order to complete the formation of ferrites and ferroelectrics [52,53].

4. Spinel Ferrite–Ferroelectric Coaxial Fibers

Studies on fibers with spinel ferrites mainly focus on cobalt ferrite and pure and Zn-substituted nickel ferrite [37,38,39,40,41,42,43,44,54,55,56,57,58,59]. Such spinel ferrite–ferroelectric systems are preferred, since past studies on bulk composites with spinel ferrites and BTO or PZT indicate the absence of any impurities when both phases are co-sintered at high temperatures [4]. Fibers with hexagonal ferrites and PZT or BTO are considered in Section 5.

4.1. Cobalt Ferrite–PZT Core–Shell Nanofibers

CoFe₂O₄ (CFO) is one of the well-studied spinel ferrites in ME composites due to its large magnetostriction (−110 ppm) and high Curie temperature (520 °C) [60]. Ferroelectric lead zirconate titanate, PbZr_xTi_1-xO₃ (PZT), in particular with a morphotropic phase boundary composition of x = 0.52, has the desired large piezoelectric coefficient (d₃₃ = 200–400 pC/N) and high Curie temperature (386 °C) [61,62]. The combination of these two ferroic phases has attracted significant interest in the fabrication of ME materials with a variety of mechanical connectivities [56,57,63,64,65].

The first reported studies on CFO-PZT fibers were carried out by Xie et al. [56,57]. Sols made with metal nitrates or acetates were used to synthesize the fibers by electrospinning and annealed fibers were characterized in terms of ferroic order parameters by PFM and magnetization measurements [56]. In another study on CFO and PbZr_xTi_1-xO₃ with x = 0.2, annealing of the fibers at high temperatures was reported to result in minor deformations in their shape, as seen in Figure 4a [40]. Structural characterization of annealed fibers by X-ray diffraction showed diffraction peaks corresponding to CFO and PZT and the absence of any impurities (Figure 4b).

Liu et al. confirmed the ferroelectric nature of the fibers by PFM measurements [40]. The PFM amplitude versus voltage data and the 180° contrast in the phase angle hysteresis loop in Figure 4c are clear evidence of the piezoelectric nature of the fiber rather than it having originated from electrostatic interactions. The estimated piezoelectric coefficient d₃₃ from the PFM data was 30 pm/V. Measurements of d₃₃ by PFM on CFO-PbZr_0.52Ti_0.48O₃ fibers yielded values ranging from 125 to 157 pm/V, depending on the molar fraction of CFO and PZT [56]. Ferromagnetic characterization on the fiber was performed by measuring the magnetization M with a vibrating sample magnetometer. Figure 4d shows the expected M vs. H hysteresis loop at room temperature with a saturation magnetization of 12 emu/g, which is a smaller than expected value for pure CFO since the fiber also contains nonmagnetic PZT.

Several investigators have reported on the measurements of ME coupling in individual fibers by scanning probe microscopy. Liu et al. [40] carried out MFM of CFO-PZT under an applied voltage to determine the strength of a converse ME (CME) effect. The magnetic domains were imaged (Figure 5A) first by the MFM mode. Then, the fiber was poled electrically by applying a DC voltage to the tip, operating under the PFM mode. This was followed by MFM to determine any change in the magnetization in CFO due to the piezoelectric strain in PZT (Figure 5B). The procedure could be used to determine the strength of the static converse ME coupling from data on the shifts in MFM frequency maps. The profiles in Figure 5C show significant changes in the frequency shifts, ∆f, before and after applying a poling voltage of 6 V to the fiber, and the shift in turn is proportional to the magnetization of CFO. From these data, the estimated ME susceptibility is 1.2 × 10⁻⁸ s/m [40]. Xie et al., on the other hand, reported MEVC = 29.5 V/cm Oe from PFM studies under a static magnetic field [57]. It is one of highest MEVC reported for a composite of CFO and PZT and is two orders of magnitude higher than values reported for bulk or layered composites of CFO and PZT [4].

4.2. CoFe₂O₄-BaTiO₃ Core–Shell Nanofibers

Apart from PZT, another well-studied ferroelectric phase in the composites with CFO is BTO with T_C = 120 °C and d₃₃ = 260 pC/N [64,66,67]. Shvartsman et al. reported on the preparation of CFO-BTO fibers by coating the ferrite particles with a sol of BTO. Upon high temperature annealing, XRD measurements revealed the fiber formation free of any impurity phases [66]. An ME susceptibility of α_C ≈ (2.2 ± 0.1)10⁻¹¹ s m⁻¹ was determined for the ceramic samples of the fibers by the converse ME effect by PFM under an applied H [66].

Fu et al. prepared coaxial fibers of BTO-CFO by electrospinning [67]. Upon annealing at high temperature, the fibers had a room temperature magnetization of 50 emu/g. They utilized PFM measurements on fibers on a substrate and on free-standing fibers to measure the ME coupling. They determined the piezoelectric coupling coefficient and its variation due to an applied H from the slope of PFM displacement vs. V profiles for clamped and free-standing fibers. The MEVCs estimated from the PFM data were 12 V/cm Oe and 35 V/cm Oe, respectively, for fibers under clamping due to a substrate and free-standing fibers.

4.3. NiFe₂O₄-PZT Core–Shell Nanofibers

Nickel ferrite (NFO)-based ME composites have been studied extensively in the past [3,4,5,6,7]. Although the saturation magnetostriction λ_s for NFO is in the order of −30 ppm, which is much smaller than for CFO, the maximum value of the piezomagnetic coupling coefficient q = dλ/dH for NFO is higher than for CFO [4]. Since the low-frequency MEVC is directly proportional to q, one expects a much higher MEVC for NFO- than for CFO-based composites [3,4,5,6,7]. However, there have been very few studies on ME effects on NFO-PZT nanowires or fibers. Liu et al. synthesized core–shell NFO-PZT nanowires by a three-step process involving a sol–gel process to form PZT tubes, followed by electrodeposition of the NiFe alloy to fill the cores of the tubes, and high-temperature annealing to form the wires with an NFO core and a PZT shell [29]. Arrays of the nanowires were characterized in terms of ferroic order parameters.

The first studies on the synthesis and ME characterization of NFO-PZT core–shell fibers were reported by Srinivasulu et al. [39]. Fibers with a PZT core–ferrite shell (sample A) or a ferrite core–PZT shell (sample B) were prepared by electrospinning using sols of NFO and PbZr_0.52Ti_0.48O₃. Fibers, upon annealing at 650 °C, had a core diameter in the range 200–450 nm and a shell thickness of 125–175 nm. XRD data, shown in Figure 6a, were indicative of absence of any impurity phases. The compositions of both NFO and PZT were confirmed by energy dispersive X-ray spectroscopy (EDS). SEM images in Figure 6b,c show fibers with uniform core diameters and shell thicknesses and interfaces free of any defects [39]. Further confirmation of the core and shell structure was obtained from scanning microwave microscope (SMM) images at 2–20 GHz [68]. Figure 6d shows an SMM image of capacitance at 5.7 GHz. The core–shell structure for the fiber is well resolved in the SMM image. Magnetization and ferromagnetic resonance (FMR) measurements of the fibers confirmed the ferromagnetic ordering of NFO in the fibers. Ferroelectric polarization P vs. E for the fibers were indicative of P-values that were one to two orders of magnitude smaller than for bulk or thin films of PZT [39].

Direct ME effects on NFO-PZT fibers were investigated by (i) P vs. E under H in a disk of the fibers pressed in a static magnetic field and (ii) change in the permittivity measured at 20–22 GHz under H in films of the fibers assembled under a magnetic field [39]. For P vs. E under H, films of the fibers were pressed into a disc in a uniform magnetic field of 3 kOe. Partial alignment of the fibers in the H direction was evident from SEM images, as shown in Figure 7a, for fibers with an NFO core and a PZT shell. Measured P vs. E are shown in Figure 7b for H = 0–7 kOe [39]. The fractional change in the remnant polarization ∆P_r/P_r = [P_r (H)—P_r (0)]/P_r (0) versus H, a measure of DME effects on the fibers, is shown in Figure 7c. A hysteresis showing an increase in P_r with increasing H was followed by a further increase in P_r when H was decreased back to zero. A similar behavior, but with a decrease in P_r under H, was reported for a disc containing fibers of a PZT core and an NFO shell [39].

The overall fractional increase in P_r for the NFO-PZT fibers was higher by an order of magnitude or more than reported values for bulk or layered NFO-PZT composites [3,4,5,6,7,11,17]. The strength of DME in NFO-PZT was also measured by the magneto-dielectric effect, i.e., H-induced variation in the real part of relative permittivity ε_r^′ of a film of the composites assembled in a magnetic field gradient produced by a permanent magnet [39]. Figure 8a shows an SEM image of such a film of sample B. Results of the variation of ε_r^′ vs. f for a series of H at 20–22 GHz, well above FMR frequencies for the fibers, are shown in Figure 8b. The procedure for the measurements is described in [39]. The fractional changes in ε_r^′ under H at 21 and 22 GHz are shown in Figure 8c. A decrease in ε_r^′ under H is evident and is much higher than the reported values for single phase multiferroics [1,2].

The converse ME (CME) effects on composites were investigated by measuring the magnetization as a function of H under an electric field or the shift in the FMR resonance field or frequency under E [4]. Liu et al. [68] employed an SMM for FMR in an isolated fiber of an NFO core and a PZT shell, as shown in Figure 9a. The amplitude and phase of the reflected microwave power (scattering matrix S₁₁) from the fiber at 5.4 GHz was recorded as the static magnetic field H applied to the fiber was scanned from 0 to 2 kOe. The FMR at the resonance field H_r manifested as a dip in the amplitude and an 180⁰ phase change in the S₁₁ vs. H profile, as seen in Figure 9b. The FMR profiles were then recorded as a function of applied DC voltage V, and the dependence of H_r on V is shown in Figure 9c. One observes a small increase in H_r as V is increased from 0 to 2 V and is followed by a linear decrease in H_r with increasing V. The voltage-CME coefficient A_v = dH_r/dV= −24 Oe/V was determined from the slope of the linear regime, as shown in Figure 8c [68], and is either comparable or higher than the reported A_v for several ferromagnetic–ferroelectric composites [50].

4.4. NiFe₂O₄-BTO Core–Shell Nanofibers

Barium titanate is one of the preferred ferroelectrics for the replacement of lead-based ferroelectrics in ME nanocomposites [69,70] and was used extensively in the early works on bulk ME composites [71,72]. Sreenivasulu et al. reported on the synthesis of BTO-NFO coaxial fibers by electrospinning, and magneto-electric characterization by H-induced ferroelectric polarization, low-frequency MEVC, and high-frequency magneto-dielectric effects [69]. Fibers with a BTO core–ferrite shell and an NFO core–BTO shell were prepared and annealed at 600–700 °C. The core–-shell structure was confirmed with MFM images. Measurements of P vs. E in the discs of the fibers showed p-values as high as 3 μC/cm² for E = 40 kV/cm. FMR measurements indicated ferromagnetism with a gyromagnetic ratio γ = 3.1 GHz/kOe, and effective magnetization 4πM_eff = 480 G.

Results of ME coupling measurements in H-assembled discs and thin films of the fibers are shown in Figure 10. Magnetic field-induced variation in P_r was measured on the discs of the fibers pressed in a magnetic field. Figure 10a,b show the fractional change in P_r as a function of H. A hysteresis in (ΔP_r/P_r) vs. H and a sign reversal are seen for the disc with fibers of the BTO core–NFO shell and a maximum increase in P_r of 3%. For the disc with fibers of the NFO core–BTO shell, ΔP_r/P_r is positive and increases under H to a maximum of 1.5%. Upon decreasing H back to zero, P_r continues to increase to a maximum of 6.5% at H = 0. The results of MEVC versus H measured at 100 Hz in H-assembled films of BTO-NFO fibers are shown in Figure 10c,d. A rapid increase in MEVC to a maximum as H is increased from zero is followed by a decrease in its value for H > 2 kOe. An 180° phase shift in ME voltage upon reversal of H direction and a hysteresis in MEVC vs. H are evident, as shown in Figure 10. The film with fibers of the NFO core–BTO shell shows a large zero-bias MEVC. Although the H-induced polarization and results of the magneto-dielectric effects [69] provide clear evidence for strong ME coupling in the fibers, the overall magnitude of MEVC in Figure 10 are orders of magnitude smaller than for bulk or layered composites [3,4,5,6,7,71,72], and the possible causes are discussed in Section 6.

4.5. Nickel Zinc Ferrite–PZT Core–Shell Fibers

Zinc-substituted nickel ferrite, Ni_1-x Zn_x Fe₂O₄ (NZFO), and ferroelectrics are well-studied composite systems in the form of thin films, thick films, nanopillars, etc., due to their large magnetostriction and piezomagnetic coupling coefficients [3,65,73,74]. Studies on coaxial fibers of NZFO-PZT were reported very recently by Ge et.al. [59]. Core–shell fibers of NZFO (x = 0.1–0.5)—PZT, prepared by electrospinning with a diameter in the range 1–3 μm and annealed at 700–900 °C, were free of impurity phases and had uniform core and shell structures, as seen in electron and scanning probe microscopy images [59]. Ferromagnetic ordering of NZFO was confirmed by FMR and by magnetization measurements. A gyromagnetic ratio γ = 2.7–3.1 GHz/kOe and effective magnetization 4πM_eff = 0.9–2.9 kG were estimated from FMR profiles, as shown in Figure 11a. The saturation magnetizations for the fibers were in the range M = 5–24 emu/g. The magnetostriction λ₁₁ was measured along the H direction, with a strain gage on the discs of the fibers. Profiles of λ₁₁ vs. H, as in Figure 11b,c for NZFO (x = 0.2)-PZT showed hysteresis and the saturation values of λ₁₁ varied from −5 ppm to −11 ppm, depending on the x-values [59].

The strength of ME coupling in the composites was measured by H-induced variation in P_r and low-frequency MEVC. Estimated ∆P_r/P_r from P vs. E under H, as in Figure 12a,b, in discs of the fibers of NZFO (x = 0.3)-PZT, showed evidence of a giant ME coupling [59]. A “memory effect” was seen in the data, characterized by an increase in the magnitude of ∆P_r/P_r to 80% when H was increased from 0 to 5 kOe and this value was retained when H was decreased back to zero. A similar behavior was evident for samples with either a ferrite core or a PZT core. Low-frequency MEVC measured in fiber discs showed a rapid increase in H, as shown in Figure 12c, and the maximum MEVC values ranged from 6 to 35 mV/cm Oe and were an order of magnitude smaller than reported values for thick film NZFO-PZT [65].

5. Hexagonal Ferrite and Ferroelectric Core–Shell Fibers

Ferrimagnets with hexagonal crystal structures with desirable magnetic parameters are of importance for use in ME composites. Hexaferrites, depending on the arrangement of spinel and hexagonal blocks, are classified into M-, W-, Y-, U-types, etc. Most of them have a very high uniaxial or planar magneto-crystalline anisotropy field, H_a [46]. M-type ferrites such as barium (BaM) and strontium (SrM) hexaferrites, for example, have uniaxial anisotropy fields of 18 to 20 kOe. Although composites with hexaferrites have weak direct ME interactions due to smaller magnetostriction compared with CFO or NFO, they are attractive for use in ME composites due to the high H_a values that could give rise to a strong ME coupling in the absence of an external bias magnetic field. Such zero-bias ME coupling is of importance for composite-based sensors and energy harvesters [75]. This section deals with ME coupling in composites with ferrimagnetic M-, Y-, or W-type hexaferrites and PZT or BTO.

5.1. M-Type Hexaferrite-Based Core–Shell Nanofibers

Liu et.al. reported on the synthesis of coaxial fibers of M-type strontium hexagonal ferrite, SrFe₁₂O₁₉, (SrM), and BTO or PZT [76]. SrM is a good candidate material amongst the M-type hexaferrites due to a large H_a of around 20 kOe [46]. The electrospinning procedure was similar to the ones described earlier for spinel ferrites and ferroelectrics. Fibers with either a ferrite core or a ferroelectric core were made and annealed at 700–1000 °C [76]. An SEM micrograph for the fibers clearly revealed the core–shell structure, as shown in Figure 13a, for a SrM core–BTO shell fiber. XRD data, as shown in Figure 13b, showed strong diffraction peaks for the piezoelectric phase, i.e., PZT/BTO, and relatively weaker peaks for SrM.

The nature of ME coupling was studied by H-induced P on a disc of the fibers annealed at 900 °C [77]. In addition, low-frequency MEVC was measured on 2D and 3D films of the fibers assembled in a magnetic field. The fractional change in P_r for PZT core–SrM shell and SrM core–BTO shell fibers are shown in Figure 13c,d [76]. The data for PZT-SrM showed a decrease in P_r under H and its magnitude increased under H to −17% for H = 6 kOe. Upon decreasing H, P_r continued to decrease further to −22% at H = 0. A similar behavior for H-induced P_r at the same magnitude is evident in Figure 13d for fibers of SrM-BTO. Data on MEVC measured at 100 Hz in 3D films of SrM-BTO and SrM-PZT are shown in Figure 13e,f. A very large zero-bias MEVC, at H = 0, is seen in the data and is due to a large “built-in” bias field provided by the magneto-crystalline anisotropy for SrM. The MEVC increased with increasing H, except for the fibers with a PZT core–SrM shell, which showed a decrease under H. The overall magnitude of the MEVC was comparable to results reported for layered composites of single crystal SrM and PZT [78].

5.2. Y-Type and W-Type Hexaferrite-Based Core–Shell Nanofibers

Y- and W-type hexaferrite have more complex structures relative to M-type ferrites, but the hexagonal symmetry remains [46]. Liu et al. [79] studied coaxial fibers of Y- and W-type ferrites and PZT or BTO. Y-types with the composition Ba₂Ni₂Fe₁₂O₂₂ (Ni₂Y) and Ba₂Zn₂Fe₁₂O₂₂ (Zn₂Y) with in-plane anisotropy fields H_a = 14 kOe and 9 kOe, respectively, were chosen. Both had much lower Curie temperatures compared with SrM [46]. Their choices for W-type ferrites were BaCo₂Fe₁₆O₂₇ (Co₂W) and BaZn₂Fe₁₆O₂₇ (Zn₂W) with uniaxial anisotropy fields H_a = 21 kOe and 12.5 kOe, respectively. Individual fibers of Y- and W-type hexaferrites and coaxial fibers with PZT or BTO were prepared, and their direct ME effects were systematically studied. Individual fibers of Y-type and W-type ferrites made by electrospinning were characterized in terms of crystal structure and magnetic parameters, and the magnetostriction of the fibers was smaller than the vales of the bulk samples [47,79]. The saturation magnetostriction λ_s measured on the annealed disks of the fibers was −10 ppm for both Zn₂W and Co₂W, whereas it was −13 ppm for Ni₂Y and −2.5 ppm for Zn₂Y. Several groups also previously reported on the synthesis and magnetic characterization of pure hexaferrite fibers [80,81,82].

Core–shell fibers of Y- and W-type ferrites and PZT or BTO were annealed at 900–1000 °C and SMM images showed well-formed and defect-free core–shell structures in all of the fiber systems [79]. The saturation magnetization at room temperature for the fibers ranged from a minimum of 2.5 emu/g for Zn₂W-BTO to a maximum of 8 emu/g for Co₂W-PZT. The maximum polarization obtained from P vs. E measurements on the discs of the fibers were in the range 1–2.5 μC/cm². Both 2D and 3D films of the annealed fibers were assembled in a static magnetic field for measurements of MEVC vs. H. Representative data from [79] for 3D films for both DC and AC magnetic fields parallel or perpendicular to the film plane are shown in Figure 14 for Ni₂Y-BTO, Zn₂Y-BTO, Zn₂Y-BTO, Zn₂W-PZT, and Co₂W-PZT.

The data in Figure 14a,b for films with Y-type ferrite and BTO show a large zero-bias MEVC potentially due to a built-in field arising from the magneto-crystalline anisotropy field. For films with Ni₂Y, MEVC is higher for in-plane fields than for out-of-plane fields and it increases under H for both field orientations and there is no hysteresis in MEVC vs. H. For the films with Zn₂Y, the MEVC is higher than for Ni₂Y, although the magnetostriction for Zn₂Y was measured to be much smaller than for Ni₂Y [79]. Since MEVC is directly proportional to the piezomagnetic coefficient q = dλ/dH, one may infer from the data in Figure 14 that q for fibers with Zn₂Y is higher than for Ni₂Y. MEVC for films with fibers of Zn₂W and Co₂W with PZT are shown in Figure 14c,d. Zero-bias ME effects are evident for both films and MEVC increases under H for the film with Zn₂W whereas it decreases under H for films with Co₂W. It is clear from the zero-bias MEVC values in Figure 14 that a large magneto-crystalline anisotropy in the ferrite eliminates the need for an external source of bias magnetic field such as an electromagnet or a solenoid.

6. Discussion

It is clear from the discussion so far that the somewhat very limited efforts on ME composites with core–shell nano- and microfibers of ferromagnetic and ferroelectric phases have been primarily on oxides of ferrimagnets and ferroelectrics, since it is rather easy to synthesize fibers free of chemical and structural impurities by electrospinning. There were many reports on fibers with spinel ferrites and ferroelectrics, whereas very few studies focused on hexagonal ferrites and PZT/BTO. Early studies were limited to structural, magnetic, and ferroelectric characterization of the fibers and were lacking any measurements on the nature of ME interactions. Initial reports on ME interactions in core–shell fibers were on CFO-PZT and CFO-BTO and utilized SPM techniques for ME measurements. Piezo force microscopy studies under H were employed to estimate the strength of DME in single fibers [56,57,64,65,66,67]. An ME susceptibility of 1.2 × 10⁻⁸ s/m was measured by a combination of PFM and MFM in CFO-PZT fibers [40]. Reported values of MEVC varied from 29.5 V/cm Oe for CFO-PZT [57] to 35 V/cm Oe for CFO-BTO [67].

Another spinel ferrite-based fiber system studied in the past was NFO-PZT/BTO. Even though XRD data did not reveal any impurity phases and SEM and SPM images showed defect-free fibers, both the magnetization and ferroelectric polarization were reported to be much smaller than for bulk materials [39,69]. Although DME studies by PFM on single fiber are yet to be reported, converse ME effects by FMR were carried out on NFO-PZT using an SMM, and a voltage-CME coefficient of Av = −24 Oe/V was reported [68]. Low-frequency MEVC results were reported for 2D films of the fibers of both NFO-PZT and NFO-BTO and were orders of magnitude smaller than for bulk or thick-layered composites [39,69]. A much higher MEVC, however, was measured in 3D films of the fiber composites. Similar studies on NZFO-PZT coaxial fibers also reported low MEVC values for fiber films [59].

Films of fibers of hexagonal ferrites and PZT/BTO showed a higher MEVC than their spinal ferrite/ferroelectric counterparts [76,79]. The most significant observation in these hexagonal ferrite-based fiber systems was the large zero-bias MEVC due to a built-in bias field provided by the very high magneto-crystalline anisotropy in the ferrites. Hexaferrite/ferroelectric fibers and fiber assemblies with high zero-bias ME effects have the potential for use in both AC and DC magnetic field sensors and in energy harvesting applications since they eliminate the need for a bias magnetic field.

Next, we compared the MEVC for single core–shell fibers in films and discs of fiber samples, with reported values in

Table 2. MEVC for ferrite/ferroelectric composites with various mechanical connectivities [83,84,85,86,87,88,89,90,91,92,93,94,95].

Material	Connectivity	MEVC (mVcm−1Oe−1)	Reference
Bulk Composites 0.3 CoFe2O4–0.7BiFeO3	0-3	285	[83]
CuFe2O4-PbZr0.53Ti0.47O3	0-3	425	[84]
PZT-CFO	0-3	549	[85]
BTO-CFO	0-3	2540	[86]
PZT-Ni0.83Co0.15Cu0.02Fe1.9O4	0-3	3150	[87]
PZT-NFO	0-3	6700	[88]
PZT- NZFO	0-3	1.3 × 104	[89]
PZT-NFO	0-3	2.9 × 104	[90]
BaM-PZT	0-3	95	[91]
Layered Composites PZT-NFO	2-2	1500	[92]
BTO-CFO	2-2	944,000	[93]
BaM-PZT	2-2	5	[94]
Fiber films
SrM-PZT	1-1	22	[76]
SrM-BTO	1-1	14.7	[76]
Ni2Y-PZT/BTO Zn2Y-PZT/BTO Co2W-PZT/BTO Zn2W-PZT/BTO NFO-BTO NZFO-PZT Single fiber CFO-BTO	1-1 1-1 1-1 1-1 1-1 1-1 1-1	11.5 16.5 12.3 10.4 0.45 20 3.5 × 104	[79] [79] [79] [79] [41] [59] [67]
CFO-PZT	1-1	2.95 × 104	[57]

for composites of various mechanical connectivity including bulk (0-3) and layered (2-2) samples [83,84,85,86,87,88,89,90,91,92,93,94]. It is clear from Table 2 that films of core–shell fibers had the lowest MEVC, whereas measurements on individual coaxial fiber had values comparable to bulk and layered composites. It is also noteworthy that the MEVC measured on individual fibers was in fact higher than the theoretical estimates in Figure 1 and Figure 2.

The relatively small MEVC for films of the fibers compared with theoretical estimates and values for individual fibers could be attributed to the effects of porosity and dipole–dipole interactions in the fiber films. As discussed in the theory section, the model was only valid for isolated fibers. It is essential to consider the effects of (i) both magnetic and electric dipole–dipole interactions between the cores and shells of ferrites and ferroelectrics and (ii) the porosity (or fiber discontinuity) in films. Magnetic dipole–dipole interactions, in particular, between ferrite cores or shells will give rise to demagnetizing effects and reductions in the magnitudes of applied AC and DC magnetic fields and a reduction in MEVC [47]. In fibers with a ferrite core, one anticipates an increase in the dipole–dipole interaction when the thickness of the ferroelectric shell decreases, leading to a further reduction in MEVC [41,47].

Another source of reduction in MEVC in films is the fiber discontinuity (porosity). This can be taken into account by assuming an air inclusion along the fiber axis that will result in a demagnetizing effect and a reduction in the internal magnetic field and a decrease in ME coupling strength. Assuming an air gap of length L_a in a fiber of length L, one can estimate the resulting reduction in MEVC. Figure 15 shows the estimated effective fractional MEVC vs. L_a/L for fibers of the NFO core–BTO shell [41]. A very large reduction in MEVC due to fiber discontinuity is evident from Figure 15. An air gap of 17%, for example, will result in three orders of magnitude reduction in MEVC. Thus, the weakening of the ME coupling strength observed in 2D- and 3D-assembled films of fibers could be primarily due to an air gap (or porosity) in the film.

Although MEVC measurements in the assembled film indicated a weak coupling, a strong or even a “giant ME effect” was evident from H-induced polarization measurements on the discs of the fibers of NFO and NZFO and PZT or BTO, with a fractional change in P_r in NZFO-PZT as high as −80% [59,68,69]. A similar strong DME was evident from ferroelectric polarization under H in discs of fibers of M-, Y-, or W-type hexagonal ferrites and PZT or BTO. Results of similar studies on bulk or layered composites are lacking regarding any meaningful comparison with results for coaxial fiber samples.

Finally, in addition to coaxial ferrite/ferroelectric fibers, there have been several reports in recent years on ME effects on ferrite/ferroelectric core–shell wires, Janus-type fibers, and ferrite fibers embedded in ferroelectric thin films. A template-assisted synthesis of CFO-BTO nanowires was utilized to control the magnetic parameters, in particular the anisotropy of CFO, and the ME coupling strength was probed by measuring the variations in the magnetic properties before and after thermal depolarization of the composite [96]. In the Janus nanofiber, in which half the fiber contains CFO and the remaining half BTO, the ME coupling was studied by second harmonic generation polarimetry under an applied magnetic field [97]. A ferroelectric phase of particular interest for use in ME nanocomposites is polyvinylidene fluoride (PVDF), which is a fluoropolymer. It is corrosion resistant and is ideal for synthesizing flexible ME composite structures. There have been recent reports on the synthesis and characterization of fiber mats and thin-film composites of PVDF and spinel or hexagonal ferrites [70,98,99]. Fiber mats of PVDF with nanoparticles of CFO were investigated in terms of the strength of ME coupling strength by H-induced polarization and the maximum polarization was found to decrease by as much as 10% [97]. In another study, ME coupling was determined by H-induced variation in P_r in films of PVDF-based ferroelectric containing SrM fibers [99]. Fractional variation in P_r ranged from 4% to 9%, depending on the amount of SrM fibers in the films.

7. Follow-Up Research

Future efforts on coaxial fibers of ferrites and ferroelectrics need to focus on studies aimed at the following factors: (i) ME measurements to date on single fibers are rather indirect, utilizing PFM under an applied H or MFM under E in order to come up with an estimate of the variation in ferroic order parameters and ME coefficients. Available data obtained from SPM measurements of MEVC and ME susceptibility on isolated fibers of CFO-BTO and CFO-PZT indicate very strong coupling with values comparable to or exceeding the theoretical estimates. Although direct measurements of MEVC involving the application of AC and DC magnetic fields to a single fiber and measuring the resulting induced voltage may be challenging, such measurements are essential for an understanding of ME coupling in coaxial fibers. (ii) Studies on fibers with Zn-substituted CFO are yet to be reported. Even though CFO has large magnetostriction, the piezomagnetic coupling coefficient q is rather small. One could enhance q-values in CFO with Zn-substitution. Thus, fibers of CZFO-PZT/BTO are expected to show strong ME coupling. (iii) The MEVC very much depends on the volume fraction of the ferrites and ferroelectrics in the fibers. There is a wide distribution in the core radius and the shell thickness in fibers prepared by electrospinning. Studies relating the fiber dimensions to ME coupling are essential. (iv) Modeling of H-induced ferroelectric polarization is also essential to understand the “giant ME coupling” inferred from measurements on fibers discs. (v) Al-substituted M-type Sr and Ba ferrites have much higher q and magneto-crystalline anisotropy fields than BaM or SrM and are good candidate materials to achieve strong zero-bias ME coupling in fibers with ferroelectrics. (vi) In addition to the fiber composites discussed here, there are several Y- and W-type hexaferrites with high magnetostriction that are good candidates for use in fiber ME composites. (vii) Experiments aimed at enhancing ME coupling on a collection of fibers are essential, since any applications related to sensors or energy harvesting will require fiber assembly.

8. Conclusions

This review article on coaxial fibers of ferrites and ferroelectrics provided a comprehensive overview of the efforts during the past decade. There have been very few reports on this topic so far, even though one can anticipate a strong ME coupling in fibers due to (i) the high surface-area-to-volume ratio compared with bulk or layered composites and (ii) the absence of clamping effects related to the presence of a substrate in thin-film or nanopillar structures. Scanning probe microscopy techniques were utilized for investigations on ME coupling in individual fibers. Films of fibers assembled in a magnetic field or disc of the fibers were used for MEVC and H-induced variations in ferroelectric order parameters. PFM under H and FMR under E on individual fibers with spinel ferrite and PZT revealed strong direct and converse ME effects. Collections of fibers with NFO, NiZn-ferrite, or CFO for the ferromagnetic phase and PZT or BTO for the ferroelectric phase in general were found to show a strong direct ME coupling by measurements of static magnetic field-induced variations in the ferroelectric polarization, whereas low-frequency MEVC values were much smaller than the reported values for bulk or thick-film-layered composites. Three-dimensional films of hexagonal ferrites with M-, Y-, or W-type structures and PZT or BTO were reported to show an MEVC much higher than for spinel ferrite/ferroelectric films but an order of magnitude smaller than for thick-layered composites.