Temperature Effect on Service Life of Reinforced Concrete (RC) Structure Under Chemical Erosion: Deterministic and Probabilistic Approach
Abstract
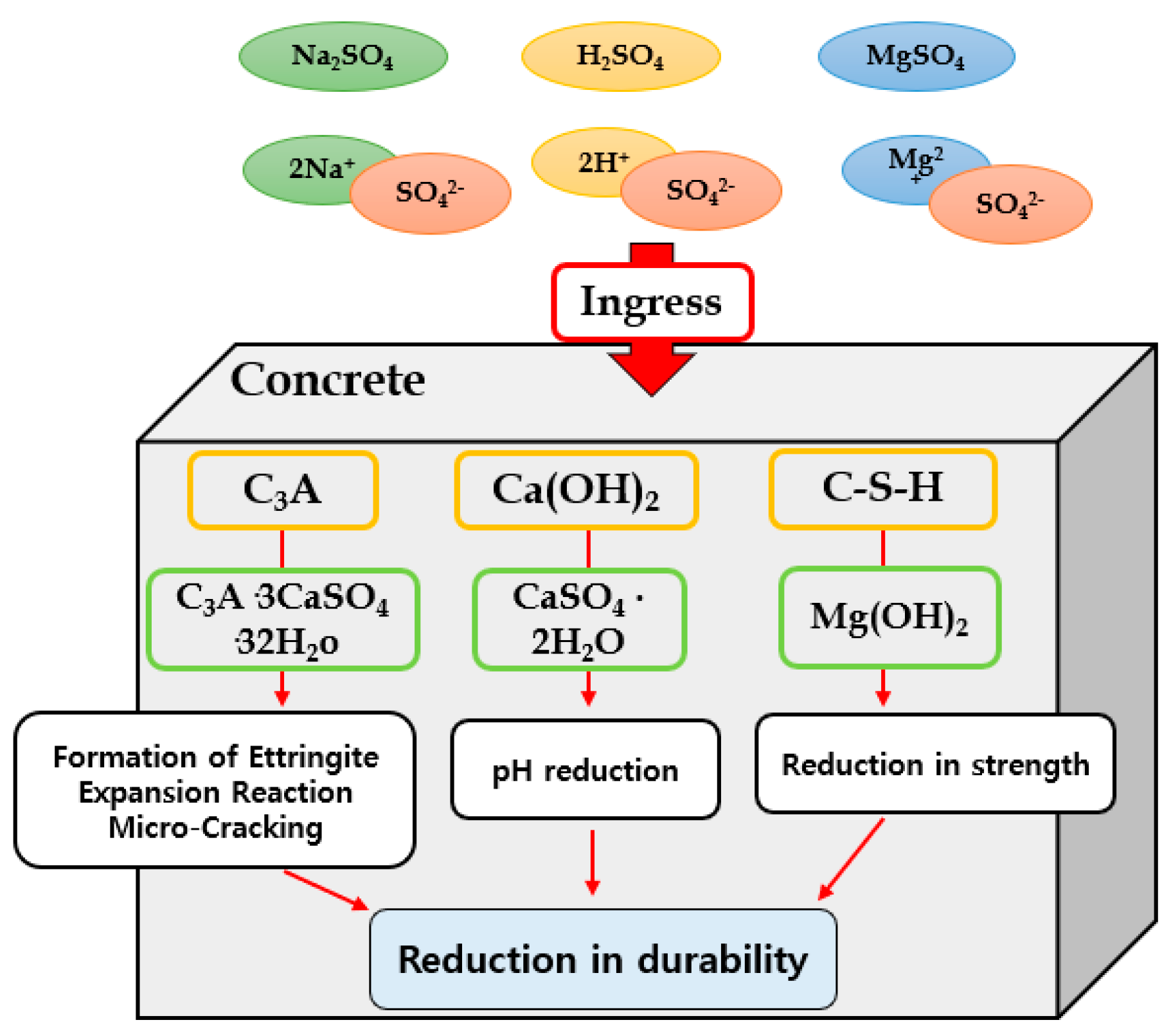
1. Introduction
2. Deterministic and Probabilistic Approach in Service Life Evaluation
3. Evaluation of Service Life Using Different Design Approaches
3.1. Design Parameters and Governing Equations
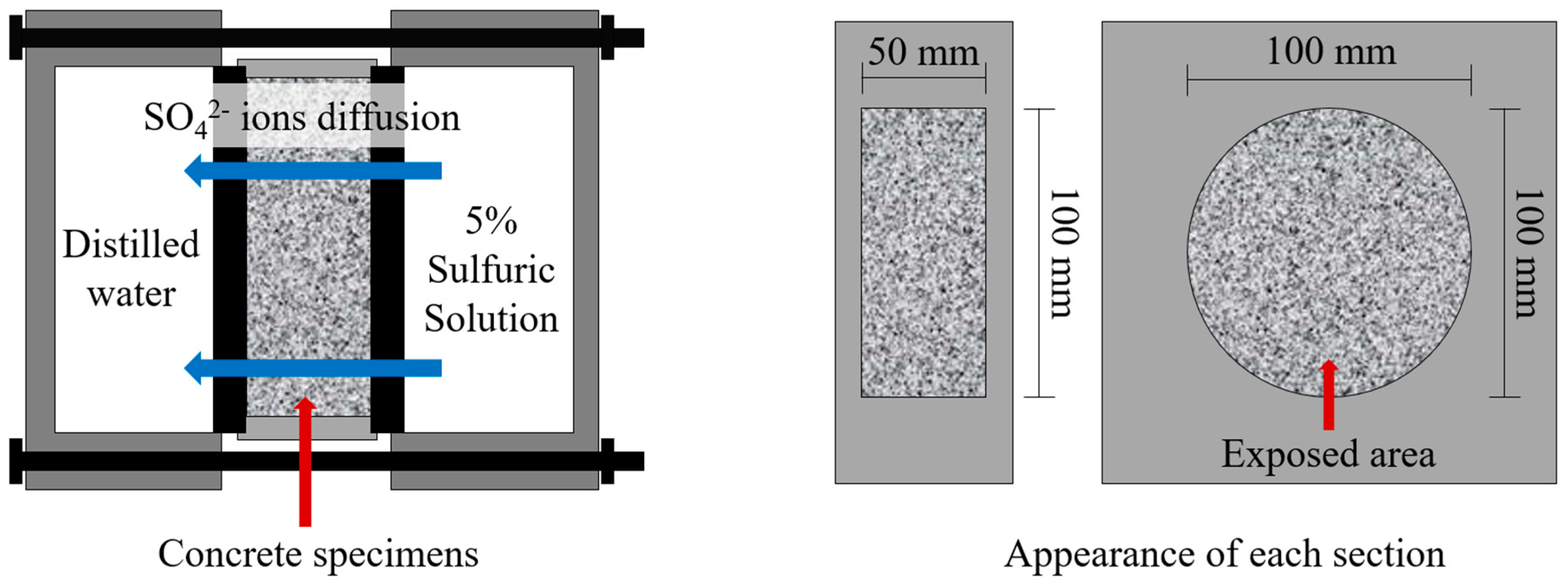
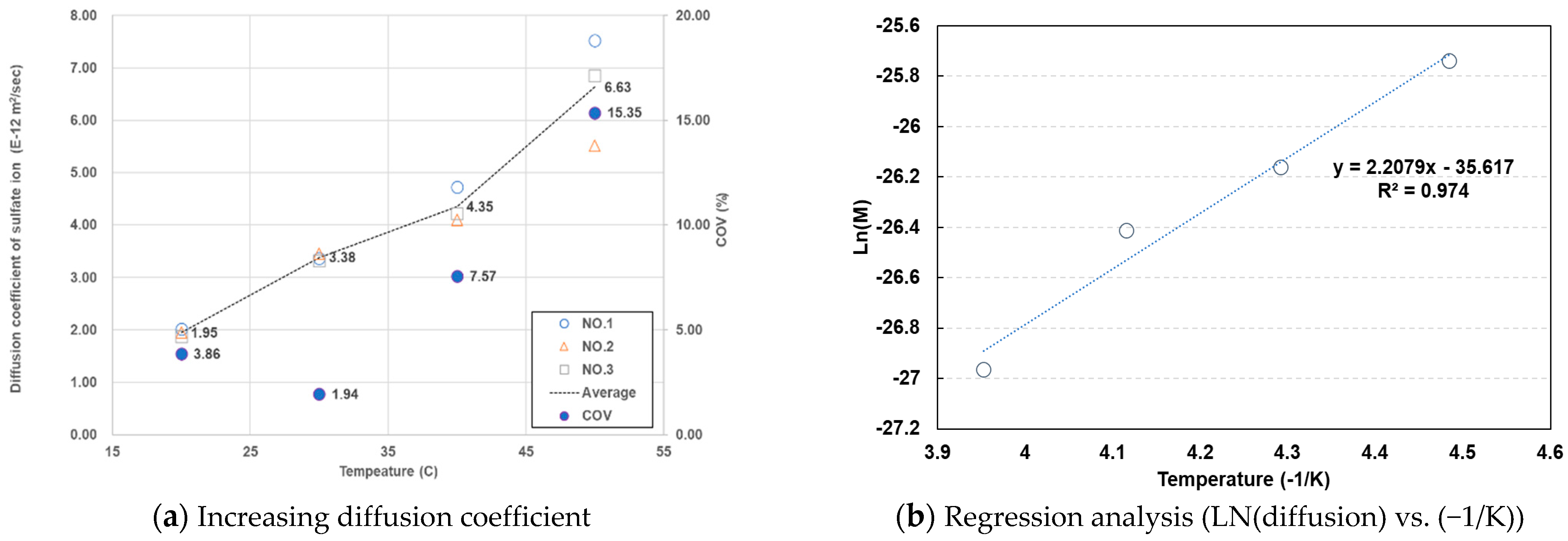
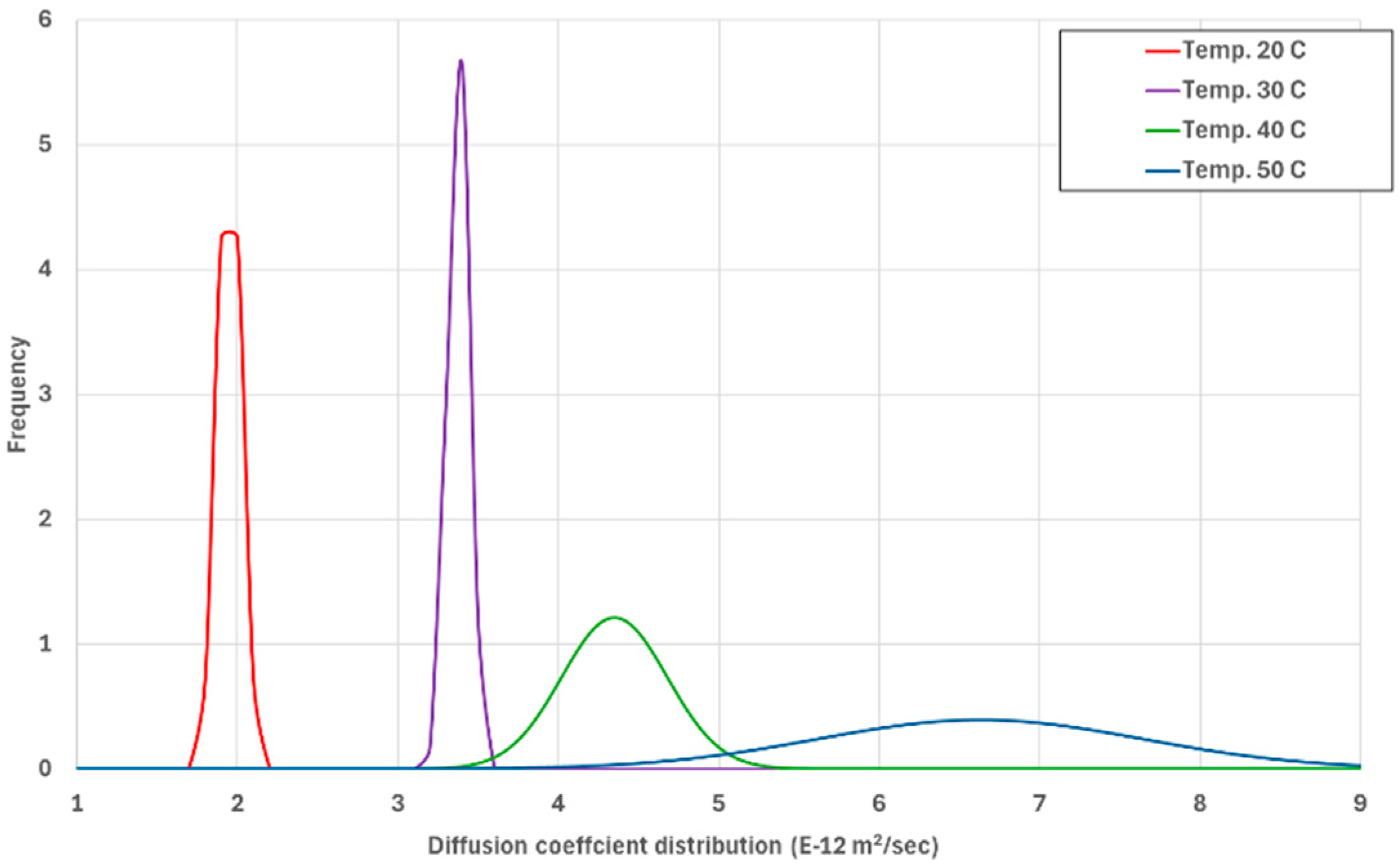
3.2. Diffusion Test with Changing Temperature
3.3. Service Life Variation with Activation Energy-Deterministic Methods
3.4. Service Life Variation with Random Variables (Probabilistic Methods)
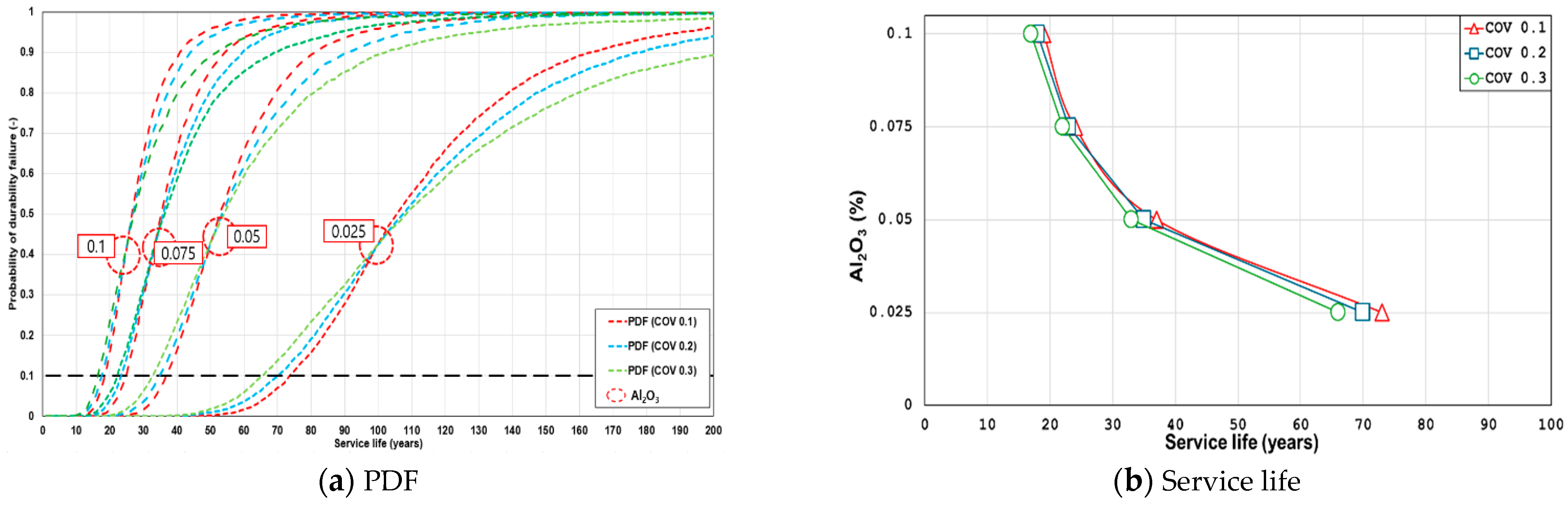
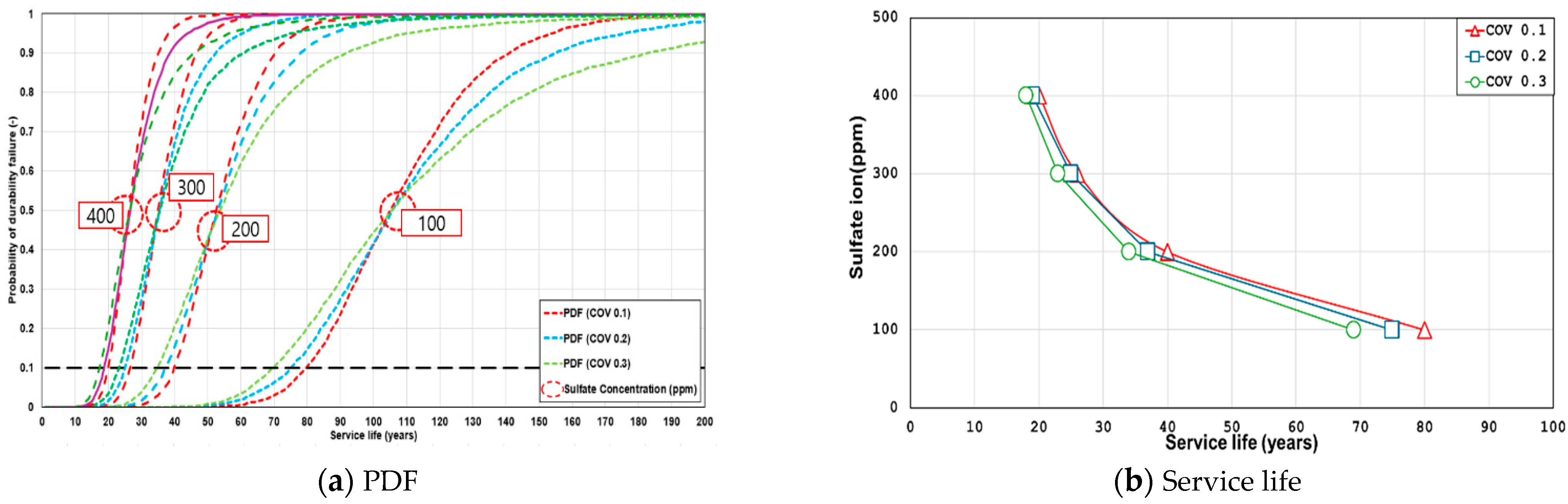
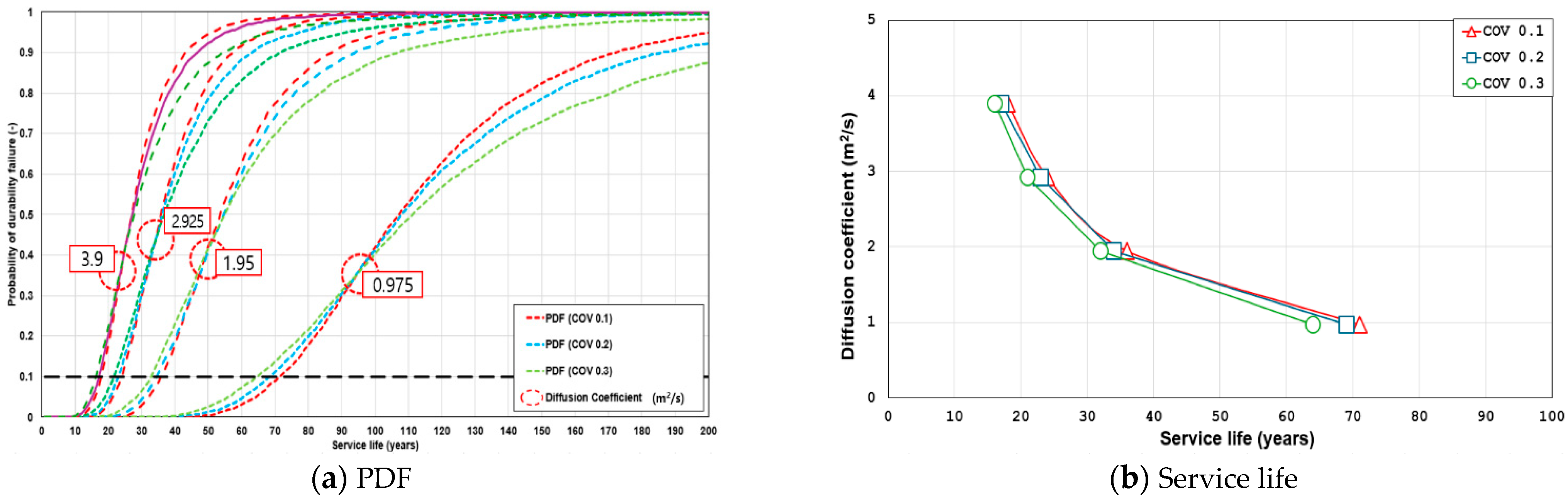
3.4.1. Service Life from the Different Methods Considering Temperature Effect
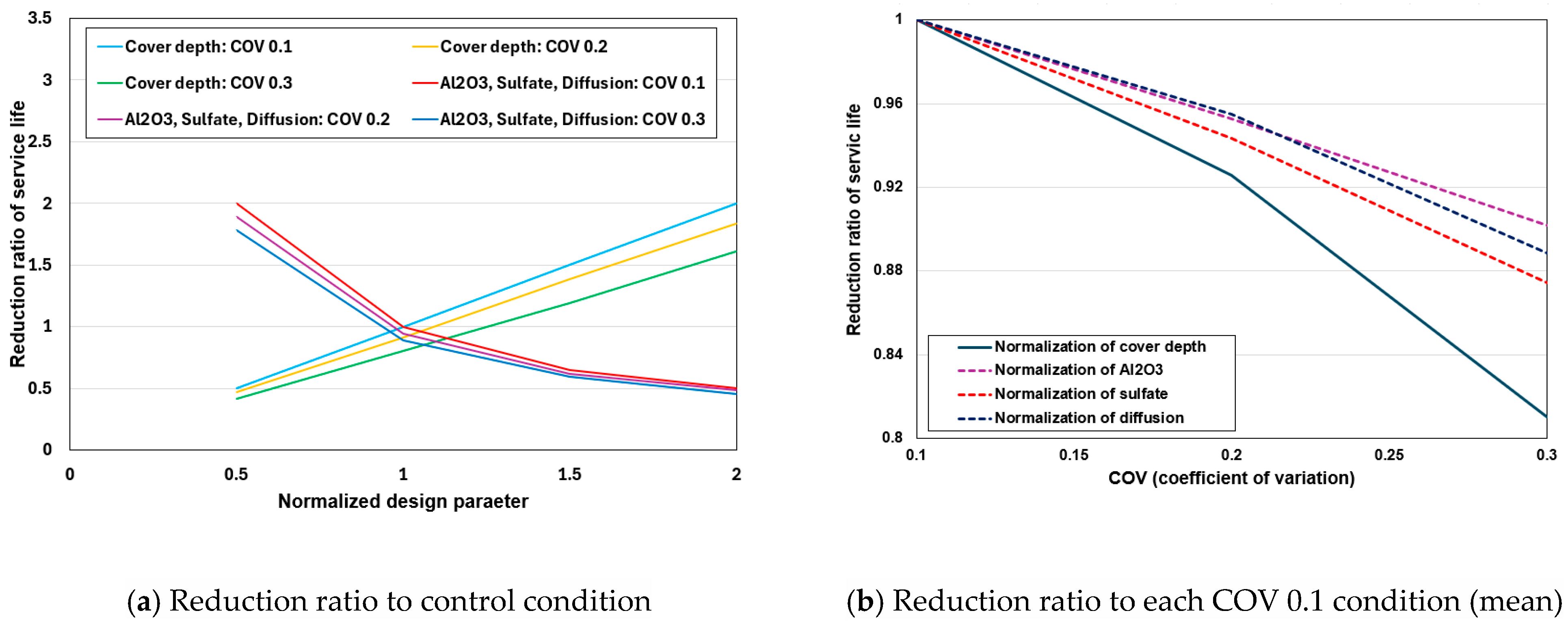
3.4.2. Service Life Simulation with Changing Variation
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- American Concrete Institute. ACI 304.3R-20: Heavyweight Concrete: Measuring, Mixing, Transporting, and Placing; American Concrete Institute: Farmington Hills, MI, USA, 2020. [Google Scholar]
- Soave, F.; Ferrara, L. A durability-based design approach for structural applications with advanced cement-based materials. Case Stud. Constr. Mater. 2025, 22, e04294. [Google Scholar] [CrossRef]
- JSCE. Guidelines for Concrete 16, Standard Specification for Concrete Structures—Materials and Construction; Japan Society of Civil Engineering: Tokyo, Japan, 2016; pp. 81–92. [Google Scholar]
- EN-1992-1-1; Eurocode 2: Design of Concrete Structures. European Committee for Standardization (Comité Européen de Normalisation, CEN): Brussels, Belgium, 2004; pp. 8–22.
- ACI 201R-08; Guide to Durable Concrete. American Concrete Institute (ACI) Committee-201: Detroit, MI, USA, 2008; pp. 26–28.
- Qin, S.; Zou, D.; Liu, T.; Jivkov, A. A chemo-transport-damage model for concrete under external sulfate attack. Cem. Concr. Res. 2020, 132, 106048. [Google Scholar] [CrossRef]
- Sotiriadis, K.; Mácová, P.; Mazur Anton, A.S.; Viani, A.; Tolstoy, P.M.; Tsivilis, S. Long-term thaumasite sulfate attack on portland limestone cement concrete: A multi-technique analytical approach for assessing phase assemblage. Cem. Concr. Res. 2020, 130, 105995. [Google Scholar] [CrossRef]
- Wang, D.; Guan, F.; Feng, C.; Mathivanan, K.; Zhang, R.; Sand, W. Review on microbially influenced concrete corrosion. Microorganisms 2023, 11, 2076. [Google Scholar] [CrossRef]
- Zhang, M.; Yang, L.M.; Guo, J.J.; Liu, W.L.; Chen, H.L. Mechanical properties and service life prediction of modified concrete attacked by sulfate corrosion. Adv. Civ. Eng. 2018, 2018, 8907363. [Google Scholar] [CrossRef]
- Rasheed, P.A.; Nayar, S.K.; Barsoum, I.; Alfantazi, A. Degradation of concrete structures in nuclear power plants: A review of the major causes and possible preventive measures. Energies 2022, 15, 8011. [Google Scholar] [CrossRef]
- Wu, L.; Huang, G.; Liu, W.V. Methods to evaluate resistance of cement-based materials against microbially induced corrosion: A state-of-the-art review. Cem. Concr. Compos. 2021, 123, 104208. [Google Scholar] [CrossRef]
- Thomas, M.D.A.; Bentz, E.C. Computer Program for Predicting the Service Life and Life-Cycle Costs of Reinforced Concrete Exposed to Chlorides; Life365 Manual; SFA: Lovettsville, VA, USA, 2002; pp. 12–56. [Google Scholar]
- Damrongwiriyanupap, N.; Limkatanyu, S.; Xi, Y. A thermo-hygro-coupled model for chloride penetration in concrete structures. Adv. Mater. Sci. Eng. 2015, 2015, 682940. [Google Scholar] [CrossRef]
- Park, S.S.; Kwon, S.J.; Jung, S.H. Analysis technique for chloride penetration in cracked concrete using equivalent diffusion and permeation. Constr. Build. Mater. 2012, 29, 183–192. [Google Scholar] [CrossRef]
- Yoon, H.S.; Yang, K.H.; Kwon, S.J. Service life of sewage culvert with bacteria coating from waste sludge and negative pressure method. J. Mater. Cycles Waste Manag. 2022, 24, 582–594. [Google Scholar] [CrossRef]
- Yoon, H.S.; Yang, K.H.; Lee, K.M.; Kwon, S.J. Service life evaluation for RC sewer structure repaired with bacteria mixed coating: Through probabilistic and deterministic method. Materials 2021, 14, 5424. [Google Scholar] [CrossRef]
- Atkinson, A.; Hearne, J.A. Mechanistic model for the durability of concrete barriers exposed to sulphate-bearing groundwaters. MRS OPL 1989, 176, 149. [Google Scholar] [CrossRef]
- Andrade, C.; Díez, J.M.; Alonso, C. Mathematical modeling of a concrete surface “skin effect” on diffusion in chloride contaminated media. Adv. Cem. Based Mater. 1997, 6, 39–44. [Google Scholar] [CrossRef]
- Care, S. Effect of temperature on porosity and on chloride diffusion in cement pastes. Constr. Build. Mater. 2008, 22, 1560–1573. [Google Scholar] [CrossRef]
- Samson, E.; Marchand, J. Modeling the effect of temperature on ionic transport in cementitious materials. Cem. Concr. Res. 2007, 37, 455–468. [Google Scholar] [CrossRef]
- Dousti, A.; Rashetnia, R.; Ahmadi, B.; Shekarchi, M. Influence of exposure temperature on chloride diffusion in concretes incorporating silica fume or natural zeolite. Constr. Build. Mater. 2013, 49, 393–399. [Google Scholar] [CrossRef]
- Kwon, S.J.; Na, U.J.; Park, S.S.; Jung, S.H. Service life prediction of concrete wharves with early-aged crack: Probabilistic approach for chloride diffusion. Struct. Saf. 2009, 31, 75–83. [Google Scholar] [CrossRef]
- Saassouh, B.; Lounis, Z. Probabilistic modeling of chloride-induced corrosion in concrete structures using first-and second-order reliability methods. Cem. Concr. Compos. 2012, 34, 1082–1093. [Google Scholar] [CrossRef]
- Jiang, C.; Gu, X.; Huang, Q.; Zhang, W. Carbonation depth predictions in concrete bridges under changing climate conditions and increasing traffic loads. Cem. Concr. Compos. 2018, 93, 140–154. [Google Scholar] [CrossRef]
- Saetta, A.V.; Scotta, R.V.; Vitaliani, R.V. Analysis of chloride diffusion into partially saturated concrete. Mater. J. 1993, 90, 441–451. [Google Scholar]
- Page, C.; Short, N.R.; EI Tarras, A. Diffusion of chloride ions in hardened cement pastes. Cem. Concr. Res. 1981, 11, 395–406. [Google Scholar] [CrossRef]
- Detwiler, R.J.; Kjellsen, K.O.; Gjorv, O.E. Resistance to chloride intrusion of corrosion cured at different temperatures-closure. ACI Mater. J. 1991, 88, 677–679. [Google Scholar]
- Dhir, R.K.; Jones, M.R.; Elghaly, A.E. PFA Concrete: Exposure temperature effects on chloride diffusion. Cem. Concr. Res. 1993, 23, 1105–1114. [Google Scholar] [CrossRef]
- So, H.S.; Choi, S.H.; Seo, J.S.; So, S.Y.; Seo, K.S. Influence of temperature on chloride ion diffusion of concrete. J. Korea Concr. Inst. 2014, 26, 71–78. (In Korean) [Google Scholar] [CrossRef]
- Yang, K.H.; Kwon, S.J.; Hwang, J.W.; Yoon, Y.S. Temperature effect on strength and chloride migration in nuclear power plant concrete. Constr. Build. Mater. 2023, 405, 13345. [Google Scholar] [CrossRef]
- Goto, S.; Roy, D.M. Diffusion of ions through hardened cement pastes. Cem. Concr. Res. 1981, 11, 751–757. [Google Scholar] [CrossRef]
- Yuan, Q.; Shi, C.; Schutter, G.D.; Audenaert, K. Effect of temperature on transport of chloride ions in concrete. In Concrete Repair, Rehabilitation and Retrofitting II; CRC Press: Boca Raton, FL, USA, 2008; pp. 177–178. [Google Scholar]
- Hwang, J.W.; Kwon, S.J. Temperature-dependent Diffusion Coefficient of Chloride Ion in UAE Concrete. J. Kor. Inst. Struct. Maint. Inspect. 2024, 28, 48–54. (In Korean) [Google Scholar]
- Ha, J.S.; Kim, S.Y.; Al Suwaidi, J.K.; Beeley, P. Development of a framework for establishment of risk-informed safety goals for nuclear power plants operation in the UAE. In Proceedings of the PSAM 2014—Probabilistic Safety Assessment and Management, Honolulu, HI, USA, 22–27 June 2014. [Google Scholar]
- An, K.H. Experimental investigation of sulfate attack on concrete in UAE’s nuclear power plants. In Proceedings of the Transactions of the Korean Nuclear Society Autumn Meeting, Changwon, Republic of Korea, 24–25 October 2024. [Google Scholar]
- Yoon, H.S.; Yang, K.H. Evaluation of effectiveness of concrete coated with bacterial glycocalix under simulated sewage environments. J. Rec. Const. Resour. 2020, 8, 97–104. (In Korean) [Google Scholar]
- Clifton, J.R.; Frohnsdorff, G.J.; Ferraris, C.C. Standards for Evaluating the Susceptibility of Cement-Based Materials to External Sulfate Attack. 1999, 29, 821–830. Available online: https://www.nist.gov/publications/standards-evaluating-susceptibility-cement-based-materials-external-sulfate-attack?pub_id=860169 (accessed on 19 March 2025).




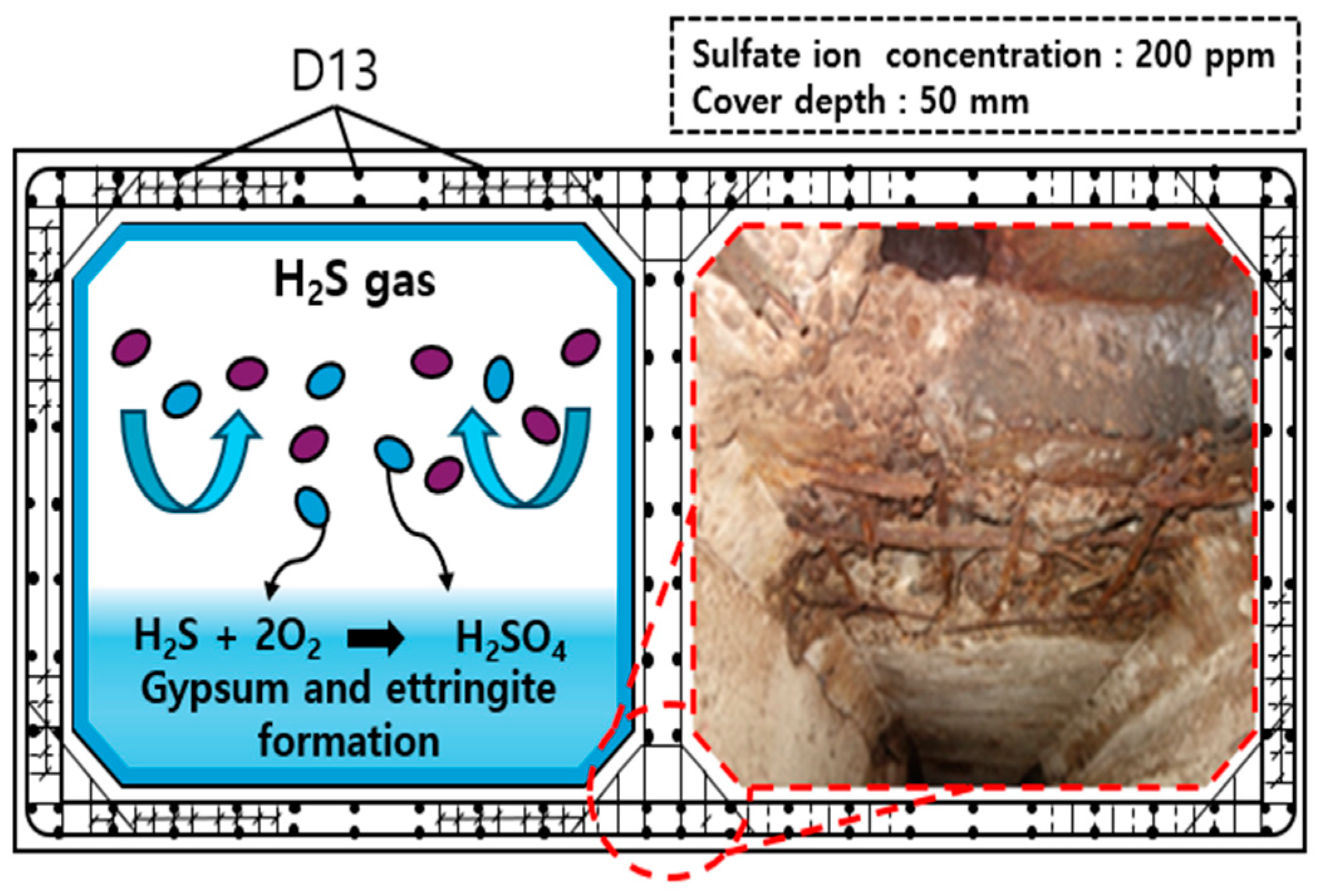


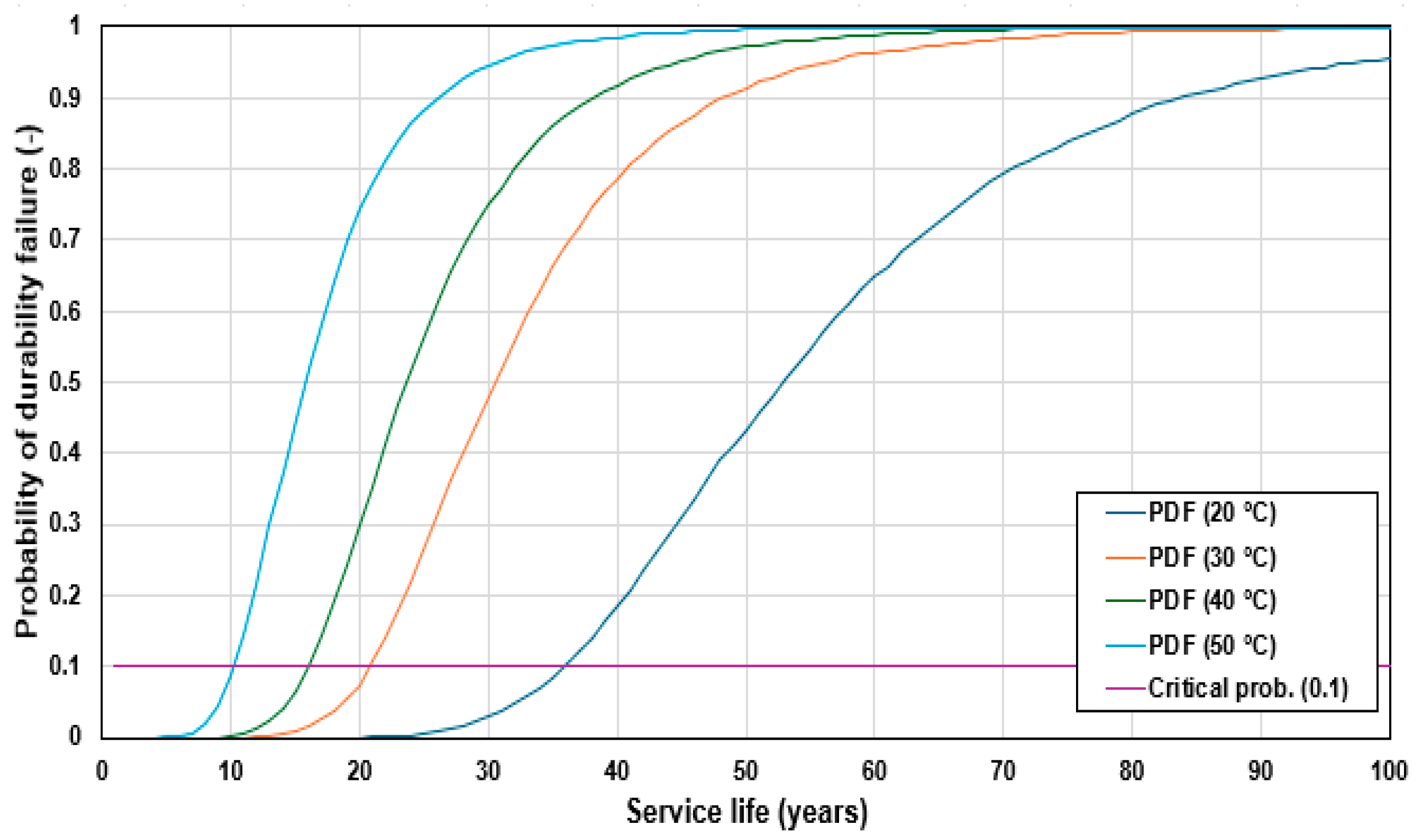
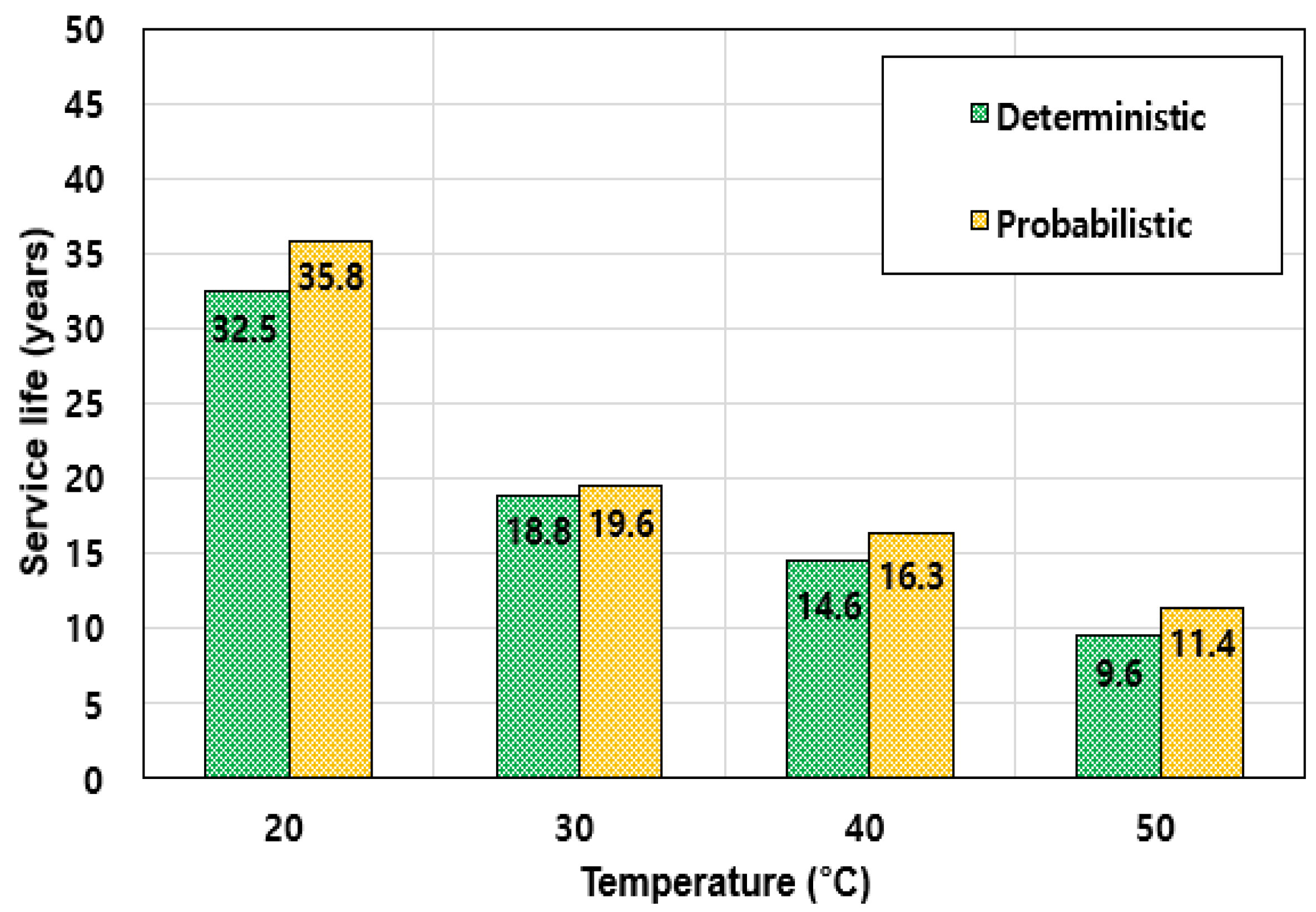
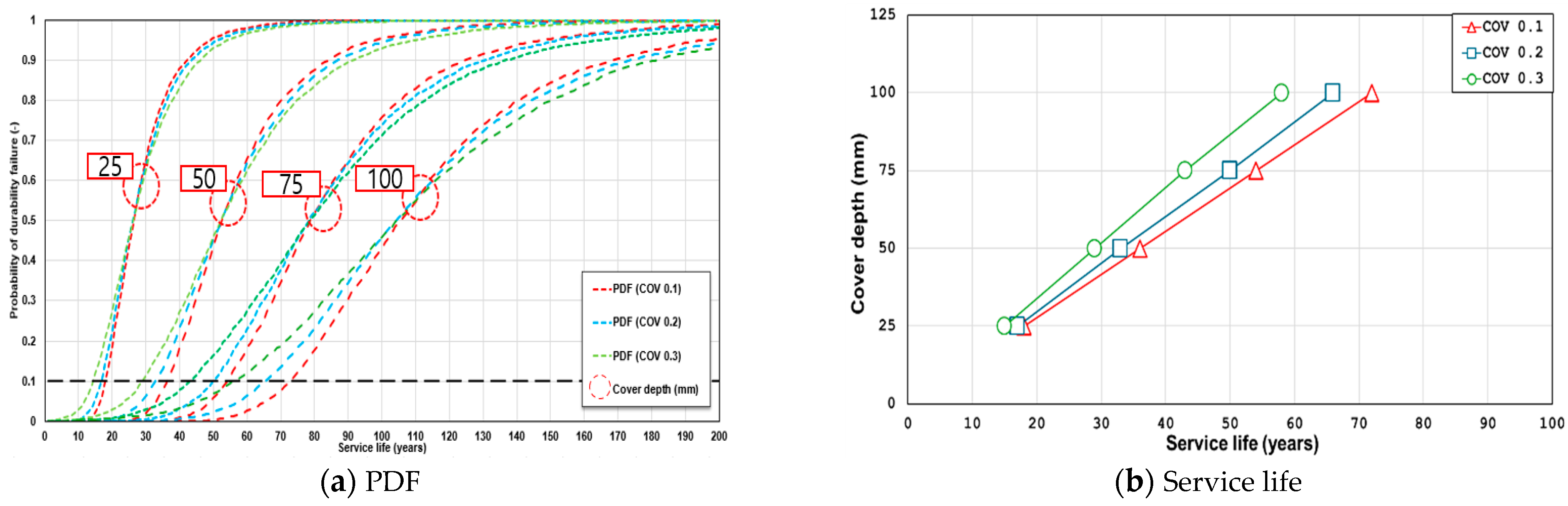
| Uncertainties | Engineering Limitations | Definition |
|---|---|---|
| Physical | - Concrete cover depth - Concentration of sulfate concentration - Diffusion coefficient of sulfate and cement compositions - Local condition (roughness) | Inherent random nature of a basic variables |
| Statistical | Limited sample size | Assumption for probability density function. |
| Model | - Simplified equation of deterioration depth in steady state - Assumption of material properties (Al2O3 and fracture energy of cracking) - Assumption of non-correlated variables | Governing mechanism for linear deterioration depth with period |
| Decision | - The period that deterioration depth exceeds the cover depth | Definition of durability failure criteria |
| W/B | Strength (Mpa) | Material (kg/m3) | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Water | Cement | GGBFS * | SF ** | Crushed Sand | Dune Sand | Coarse Agg | HWRA (Type F) | ||
| 0.4 | 40 | 147 | 113 | 244 | 19 | 707 | 230 | 938 | 4.1 |
| Temp. (°C) | ×10−12 m2/s | COV (%) | |||
|---|---|---|---|---|---|
| No.1 | No.2 | No.3 | Mean | ||
| 20 | 2.02 | 1.95 | 1.87 | 1.95 | 3.86 |
| 30 | 3.37 | 3.45 | 3.32 | 3.38 | 1.94 |
| 40 | 4.72 | 4.10 | 4.22 | 4.35 | 7.57 |
| 50 | 7.52 | 5.52 | 6.85 | 6.63 | 15.35 |
| Types | Deterioration Depth Method | Probabilistic Method | |
|---|---|---|---|
| Mean | Normal Distribution/COV | ||
| Cover depth (mm) | 50 | 50 | 0.12 |
| Al2O3 (%) | 0.05 | 0.05 | 0.15 |
| Average sulfate concentration (ppm) | 200 | 200 | 0.25 |
| Diffusion coefficient (10−12·m2/s) | 1.95 | 20 °C 1.95 | 0.04 |
| 30 °C 3.38 | 0.02 | ||
| 40 °C 4.35 | 0.08 | ||
| 50 °C 6.63 | 0.15 | ||
| Elastic modulus (Mpa) | 26,000 | 26,000 | - |
| Poisson’s ratio (-) | 0.17 | 0.17 | - |
| Roughness coefficient (-) | 0.25 | 0.25 | - |
| Concrete fracture energy ) | 10 | 10 | - |
| 1.8 | 1.8 | - | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, K.-H.; Lee, H.-W.; Alkaabi, A.K.; Kwon, S.-J. Temperature Effect on Service Life of Reinforced Concrete (RC) Structure Under Chemical Erosion: Deterministic and Probabilistic Approach. Appl. Sci. 2025, 15, 4816. https://doi.org/10.3390/app15094816
Yang K-H, Lee H-W, Alkaabi AK, Kwon S-J. Temperature Effect on Service Life of Reinforced Concrete (RC) Structure Under Chemical Erosion: Deterministic and Probabilistic Approach. Applied Sciences. 2025; 15(9):4816. https://doi.org/10.3390/app15094816
Chicago/Turabian StyleYang, Keun-Hyeok, Hyeon-Woo Lee, Ahmed K. Alkaabi, and Seung-Jun Kwon. 2025. "Temperature Effect on Service Life of Reinforced Concrete (RC) Structure Under Chemical Erosion: Deterministic and Probabilistic Approach" Applied Sciences 15, no. 9: 4816. https://doi.org/10.3390/app15094816
APA StyleYang, K.-H., Lee, H.-W., Alkaabi, A. K., & Kwon, S.-J. (2025). Temperature Effect on Service Life of Reinforced Concrete (RC) Structure Under Chemical Erosion: Deterministic and Probabilistic Approach. Applied Sciences, 15(9), 4816. https://doi.org/10.3390/app15094816








