Normalized Residual Displacements for Single-Degree-of-Freedom Systems Subjected to Mainshock–Aftershock Sequences

Abstract

Post-earthquake structural rehabilitation faces critical challenges from aftershock-induced cumulative damage, particularly through residual displacement accumulation that compromises structural realignment feasibility. While residual displacements serve as pivotal indicators for repair-or-replace decisions, the amplification effects of aftershocks on such displacements remain systematically underexplored. This study investigates residual displacement demands of bilinear single-degree-of-freedom (SDOF) systems subjected to mainshock–aftershock sequences. A novel metric is proposed, defined as the maximum residual displacement considering both isolated mainshock and full sequence scenarios, normalized against peak inelastic displacements (termed residual displacement ratio) for predictive analysis. The influence of sequence characteristics (duration, frequency content, aftershock intensity) and structural properties (post-yield stiffness ratio, displacement ductility, natural period) on residual displacement ratios is evaluated. Statistical analysis reveals that aftershocks amplify mainshock-induced residual displacements in the statistical mean sense, with an observed maximum increase reaching up to 72%. The mainshock with stronger aftershocks tends to result in larger residual displacement ratios. A constant-ductility residual displacement ratio response spectrum is finally developed for the repairability assessment of structures against mainshock–aftershock sequences in terms of residual displacements.

1. Introduction

Seismic reconnaissance surveys [1,2,3,4] consistently reveal that conventional structures lacking self-centering mechanisms often sustain excessive residual displacements following strong earthquake shaking, even when collapse is prevented. The large permanent deformations frequently necessitate structural demolition due to two critical factors: (1) pronounced visual deformations that jeopardize structural functionality and (2) technical challenges and economical impracticality in rehabilitating significantly tilted structures. The 1995 Kobe earthquake [2] exemplifies this phenomenon, where 88 reinforced concrete piers with tilt angles exceeding 1° were irreparable and required replacement despite minimal structural damage. Such observations have elevated residual displacement as a pivotal performance metric in modern performance-based earthquake engineering frameworks.

Extensive research has been conducted on post-earthquake residual displacements of structures that can be simplified to single-degree-of-freedom (SDOF) oscillators. Some studies [5,6,7,8,9,10,11,12,13] have focused on analyzing the factors that influence residual displacement response, while others [14,15,16,17,18,19,20,21,22,23] have aimed to develop computational models for estimating residual displacements. For instance, Macrae and Kawashima [5] and Kawashima et al. [6] identified the post-yield stiffness ratio as the dominant factor governing residual displacements and proposed a constant-ductility residual displacement spectrum for seismic design. Borzi et al. [7] observed that the residual displacement of oscillators with softening behavior (negative post-yield stiffness) is more significant than in the case of elastic–perfectly plastic and hardening SDOF systems. Ruiz-García and Miranda [14,15] derived simplified equations to estimate residuals considering site conditions, natural periods, and lateral strength, extending their framework to soft-soil site motions. Liossatou and Fardis [9,10] linked hysteresis loop shapes to residual displacement magnitudes. Their analyses revealed that the ratio of residual to maximum inelastic displacement remains approximately constant, even for ground motions containing velocity pulses. Madhu Girija and Gupta [18], Harikrishnan and Gupta [19], and Saifullah and Gupta [20] generated several ensembles of ground motions based on the control variate technique to isolate the effect of different seismological parameters on structural response. A predictive model was developed for structures with bilinear and pinching hysteretic characteristics. Liu et al. [21] investigated the constant-damage residual displacement ratios of SDOF systems with different damage levels.

While these prior works advanced the understanding of residual displacement mechanics, a fundamental limitation persists: existing models are predominantly calibrated to single-earthquake scenarios. In reality, mainshock-damaged structures often endure aftershocks that exacerbate residual displacements through cumulative damage. Studies in [14,15,16,17,18,19,20,21,22,23], though pioneering in methodology, neither explicitly account for ground motion sequences nor evaluate interactions between structural damage progression (e.g., ductility) and aftershock properties (e.g., duration, intensity ratios, spectral compatibility). This critical gap between conventional analytical assumptions and real seismic scenarios highlights the necessity to account for sequential ground motions in evaluating post-earthquake residual displacements.

To the knowledge of the authors, the present research on seismic sequences remains focused on sequence characteristics [24,25,26], displacement ductility [27,28,29,30,31], seismic vulnerability [25,32,33,34,35,36,37], cumulative damage [38,39,40], and structural resilience [41,42,43], with residual displacement evaluation receiving scant attention. Notably, Amiri et al. [44,45,46] pioneered the prediction of residual displacement demands of structures against mainshock–aftershock sequences. However, the residual displacement demands of seismic sequences were defined as the permanent shift that remains in the structure after the complete sequential ground shaking in their work, without considering the offset induced by the mainshock in the middle of the sequences. In reality, the mainshock-damaged structures cannot be repaired prior to the subsequent aftershocks due to the short time between the mainshock and its aftershock [39]. Consequently, both the residual displacement induced by the mainshock and that induced by the complete earthquake sequence should be worthy of attention. Furthermore, the extent to which aftershocks amplify residual displacements through cumulative effects has not been quantitatively examined.

The objective of this study is to statistically estimate the residual displacement demands of SDOF oscillators when subjected to mainshock–aftershock sequences. A comparison considering mainshock only and complete mainshock–aftershock sequences is presented to highlight the significance of aftershocks on residual displacement estimation. The residual displacement demands of mainshock–aftershock sequences are newly defined as the maximum value of the mainshock-induced offset and sequence-induced offset. The effect of the ground motion duration, frequency content, relative aftershock intensity, post-yield stiffness ratio, and displacement ductility is examined. A constant-ductility residual displacement ratio response spectrum is developed to provide a more reliable estimate of residual displacement demands of bilinear SDOF oscillators when subjected to mainshock–aftershock sequences.

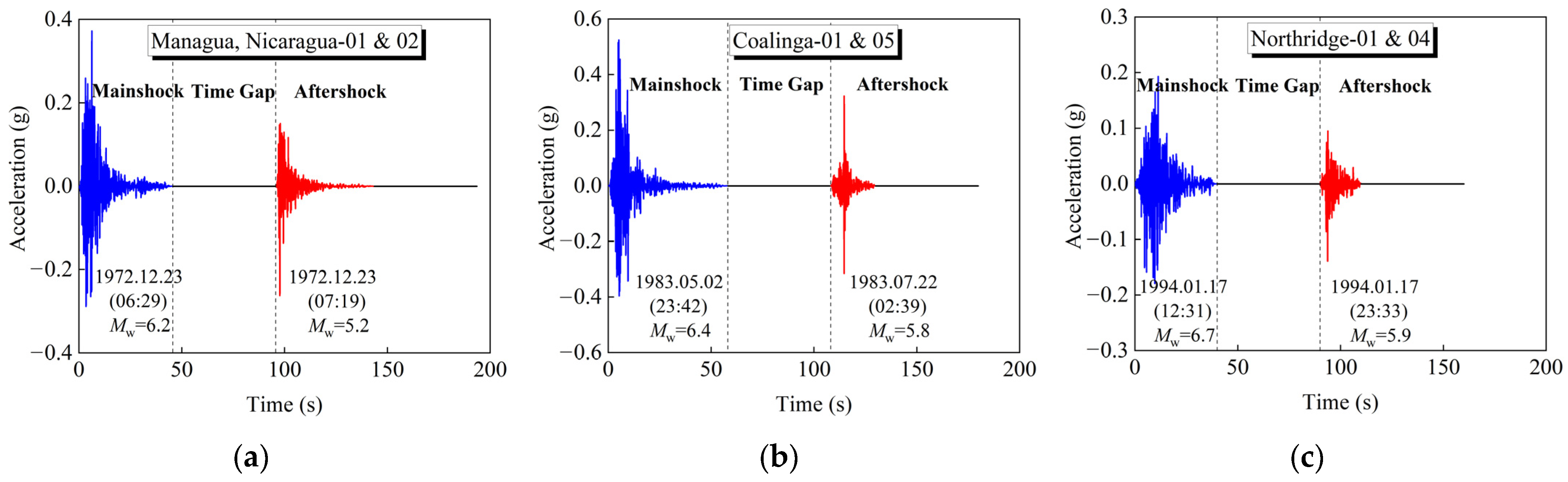
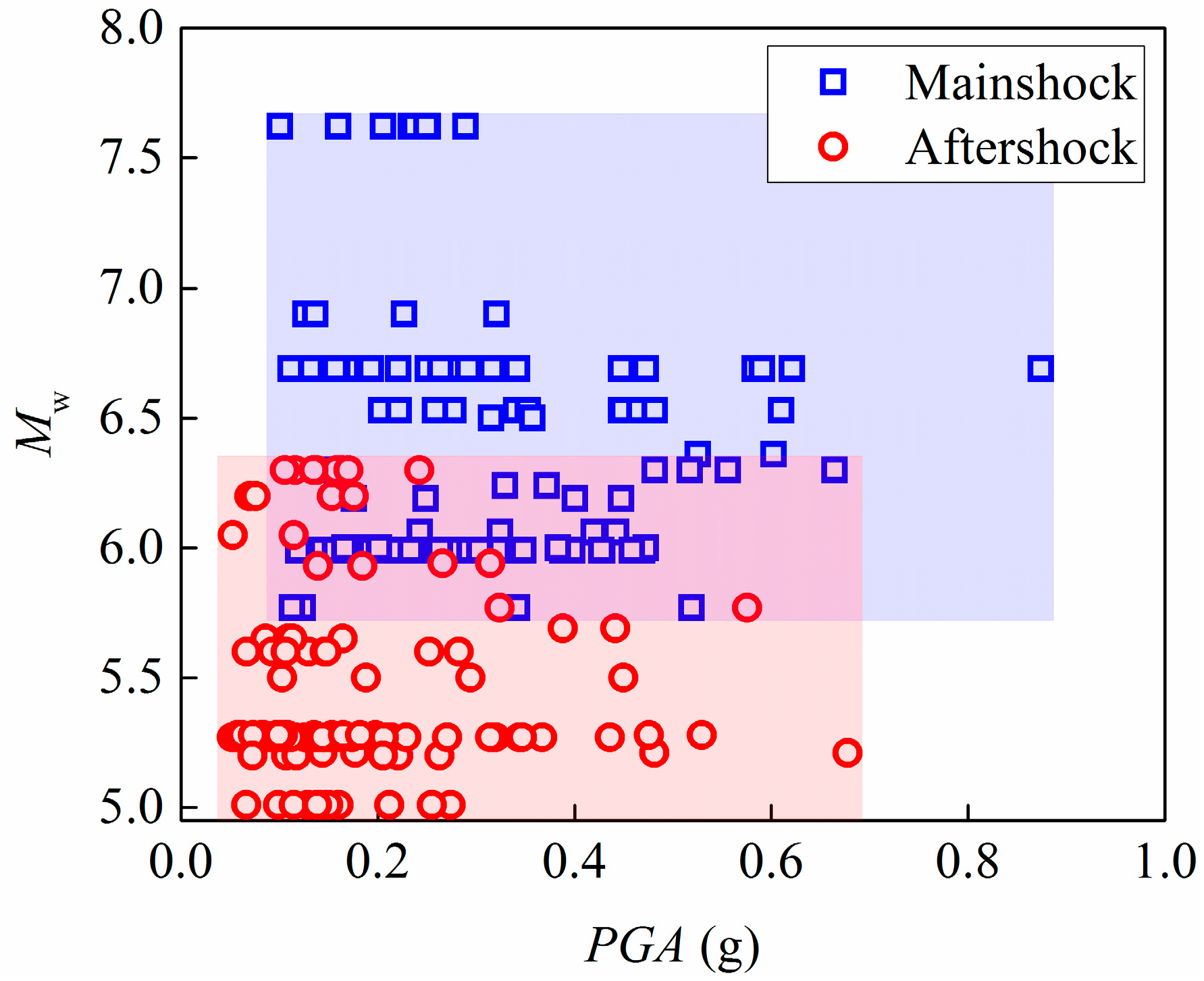
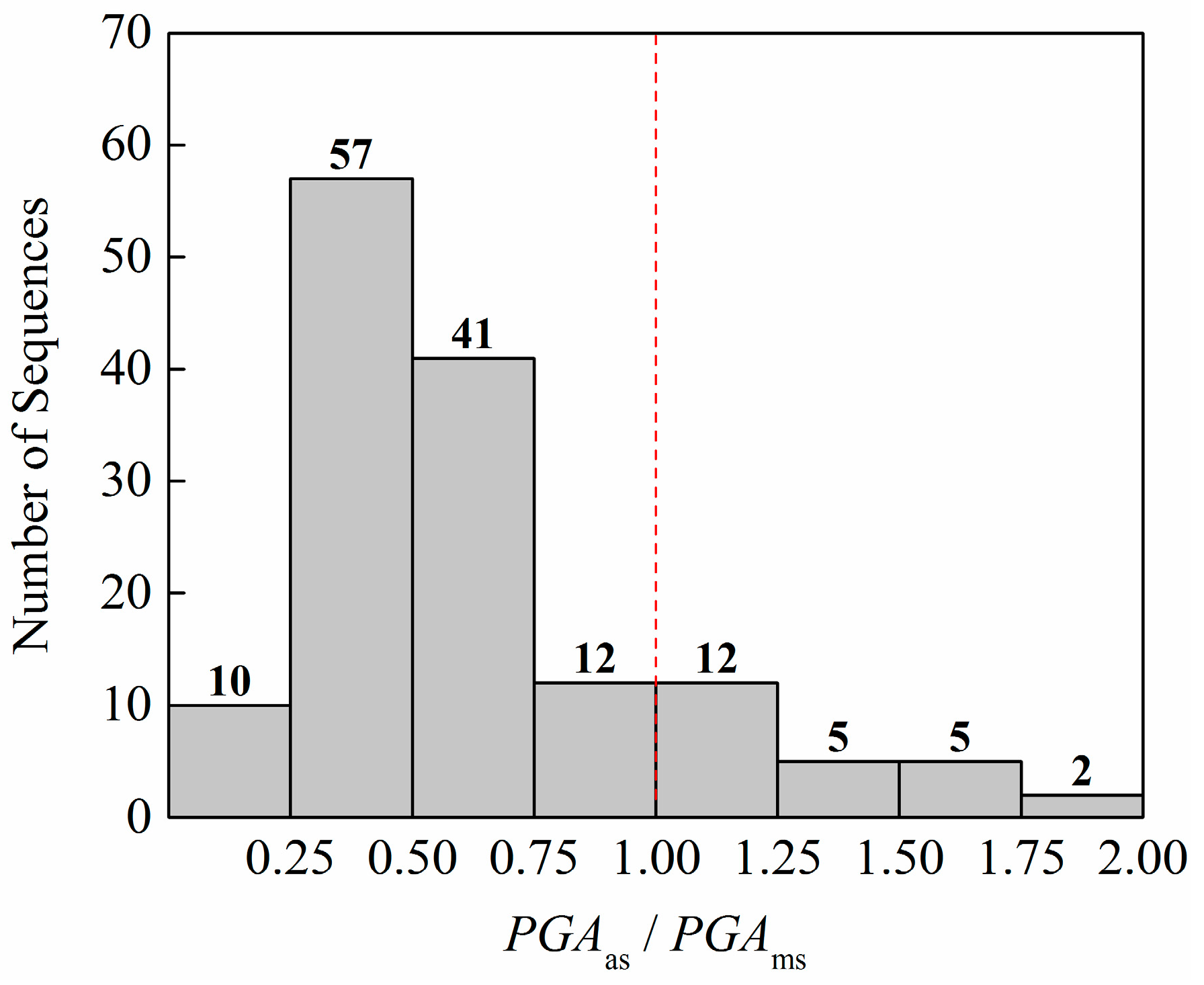
2. Mainshock–Aftershock Sequences

Previous studies [24,25,26] have shown that the characteristics, such as amplitudes, duration, and frequency contents, of the mainshock are seismologically distinct from those of their aftershocks. These distinctions originate from physical mechanisms governing stress redistribution and fault zone adjustments following the mainshock rupture. Crucially, such physics-based correlations between mainshocks and aftershocks are inherently difficult to replicate in artificial sequences generated through repeated scaling or stochastic superposition methods [29,30]. Empirical evidence highlights systematic flaws in synthetic approaches. Ruiz-Garcia and Negrete-Manriquez [29] and Goda and Taylor [30] demonstrated that synthetic sequences artificially enforce spectral compatibility between aftershocks and mainshock-damaged structures, thereby triggering unphysical resonance effects. Such computational artifacts evidently amplify structural responses compared to real sequences, distorting risk evaluations. To overcome these limitations, this study intentionally adopts as-recorded sequences. It should be noted that while individual as-recorded sequences exhibit scatter due to natural seismological variability, their collective behavior adheres to deterministic physical principles.

The statistics of mainshock and aftershock scenarios indicate that, in general, a strong mainshock triggers a series of aftershocks with smaller magnitudes. However, a portion of the aftershock ground motion has not been recorded at that time for numerous reasons (e.g., seismometer failure, shock too slight to be detected), thus resulting in the as-recorded dataset being incomplete [30]. In addition, a strong aftershock is more inclined to aggravate the damage to the structure than a small aftershock [39,40]. For simplicity, this study mainly focuses on the case of the mainshock with the strongest aftershock, hereafter referred to as the mainshock–aftershock sequence.

In this investigation, the mainshock–aftershock sequences are selected from the Pacific Earthquake Engineering Research Center (PEER) ground motion database [47]. All earthquake records meet the following criteria: (1) the moment-magnitude (M_w) of the mainshock is no less than 5.5, and the M_w of the aftershock is no less than 5.0; (2) the peak ground acceleration (PGA) of the mainshock is no less than 0.10 g, and the PGA of the aftershock is no less than 0.05 g; (3) ground motions recorded on soft soil sites (i.e., the average shear-wave velocity v_s,30 is no less than 180 m/s in the upper 30 m of the site profile) or exhibiting distinct velocity pulse characteristics are excluded; (4) recording stations are located in free-field sites or within first-floor low-rise buildings where soil–structure interaction effects can be neglected.

According to the aforementioned criteria, an ensemble of 144 mainshock–aftershock sequences (considering both horizontal components) recorded from 12 earthquake events is selected in this study. Each sequence comprises a mainshock paired with its predominant aftershock. The details of all seismic sequences, including v_s,30, M_w, PGA, distances to horizontal projection of rupture (R_rup) and peak ground velocity (PGV), are summarized in Appendix A. To precisely determine residual displacements under seismic sequences, a 50 s zero-acceleration interval is inserted both between the mainshock–aftershock transition and at the sequence termination. This ensures that structural vibrations fully subside before displacement measurements. Figure 1 illustrates three samples of as-recorded mainshock–aftershock sequences (the 1972 Managua earthquake, the 1983 Coalinga earthquake, and the 1994 Northridge earthquake) after padding zero-acceleration time history. Using the processed earthquake sequences, the nonlinear time–history analysis (NTHA) can be performed to calculate the residual displacement response.

Figure 2 illustrates the M_w and PGA distributions for mainshock–aftershock pairs. Two key observations emerge: (1) The mainshocks exhibit significantly higher M_w compared to their associated primary aftershocks, with limited overlap in the range of 5.8–6.3. Further statistical analysis shows that the mean magnitude difference between mainshocks and primary aftershocks is about 0.9. (2) Despite differing PGA selection thresholds, both mainshocks and aftershocks exhibit comparable PGA ranges.

To explore the relation between the mainshock and its aftershock PGA, the relative intensity ratio κ is firstly defined as follows:

κ = PGA_as/PGA_ms

(1)

where subscripts ‘ms’ and ‘as’ denote the mainshock and aftershock, respectively.

Figure 3 displays the distribution of relative intensity ratios κ for the selected seismic sequences. The histogram reveals that the κ values span a broad range from 0.18 to 1.89, reflecting substantial aftershock variability relative to mainshocks. Overall, 83% of seismic sequences exhibit κ < 1, confirming the predominance of mainshock-dominant intensity patterns. However, a non-negligible subset (17%, n = 24/144) demonstrates κ ≥ 1, suggesting potential aftershock amplification phenomena in specific tectonic settings.

Figure 4 compares the mean pseudo-acceleration response spectra of mainshocks only, aftershocks only, and mainshock–aftershock sequences. Note that the acceleration time histories are respectively normalized by their PGA to anchor the zero-period spectral ordinate for a standard spectral shape. It can be seen that the mainshock spectra are higher than the aftershock spectra for the period T > 0.1 s, attributable to the significant difference in magnitude between mainshocks and aftershocks [30]. In addition, the sequence spectra demonstrate near-complete overlap with mainshock spectra, indicating negligible aftershock-induced spectral modifications in elastic systems.

3. Residual Displacement Ratios

To establish a rational normalization framework for residual displacements, the correlation between residual displacements and elastic spectra as well as peak inelastic displacements is examined. The nonlinear behavior of structural systems is modeled using an elastoplastic hysteresis idealization with a tangent stiffness-proportional damping ratio of 5%. The displacement response is computed using the constant-ductility method, ensuring that the analyses preserve uniform post-yield deformation levels across all ground motions.

3.1. Residual Displacements for Mainshock–Aftershock Sequences

Under intense sequential ground motions, structures typically retain unrecoverable residual displacements following either the mainshock or the complete seismic sequence. Figure 5 exemplifies the displacement response of a typical elastoplastic SDOF oscillator with the natural period T = 1.0 s and peak displacement ductility μ = 4 subjected to three earthquake sequence samples (as previously shown in Figure 1). Three typical relationships between the mainshock-induced residual displacement (d_r,ms) and the sequence-induced residual displacement (d_r,seq) are summarized as follows:

(1)

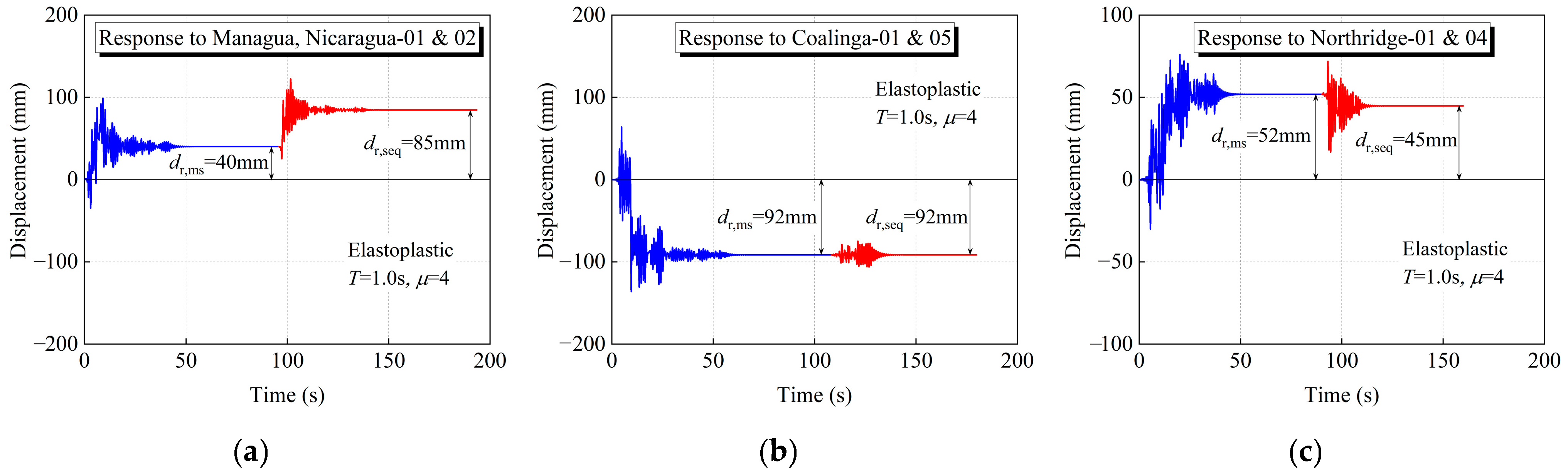
d_r,seq > d_r,ms: indicates that the aftershock further deteriorates the structural repairability. In such cases, d_r,seq serves as the governing parameter for post-earthquake rehabilitation assessments.

(2)

d_r,seq = d_r,ms: suggests negligible aftershock impact on repairability thresholds. In this case, both d_r,ms and d_r,seq must comply with prescribed allowable limits.

(3)

d_r,seq < d_r,ms: while partial displacement recovery may occur, immediate post-mainshock residual displacement d_r,ms remains critical for emergency response operations (personnel evacuation, supply delivery) and secondary disaster mitigation.

Under sequential seismic excitation, a more reliable and conservative approach for residual displacement estimation involves prioritizing the maximum offset recorded during either the mainshock or the subsequent aftershock. Consequently, it is desirable to define the residual displacement for mainshock–aftershock sequences as follows:

d_r = max{d_r,ms, d_r,seq}

(2)

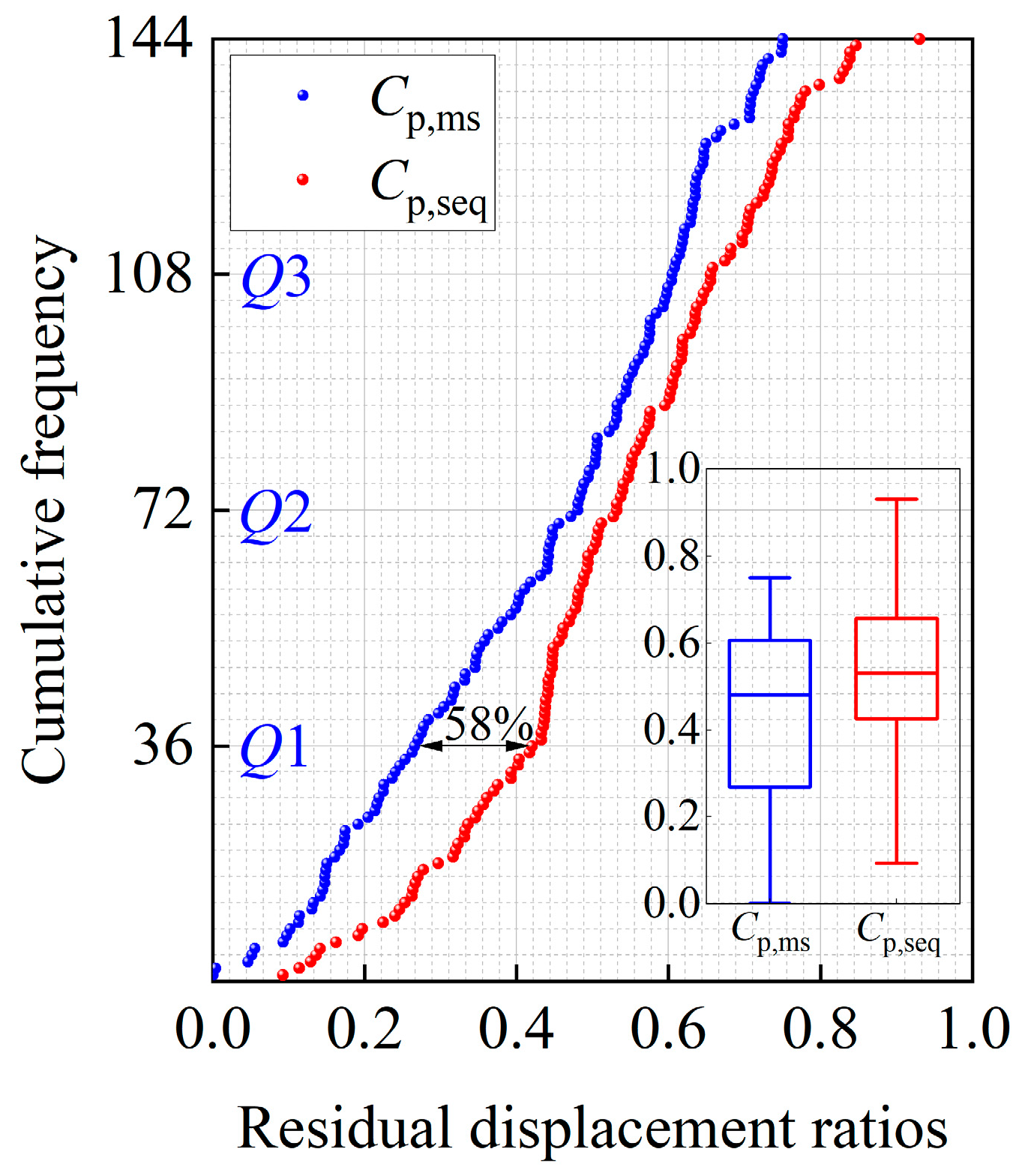
3.2. Definition of Residual Displacement Ratios

In view of the significant variability in residual displacements (with a coefficient of variation reaching up to 1.5 in most cases [13]), several indicators, such as elastic spectral displacements and peak inelastic displacements, which exhibit relatively high correlation with residual displacements, are used to normalize the residual displacements. By employing such normalization, the high uncertainty of residual displacements associated with ground motions can be excluded feasibly. Furthermore, such normalization facilitates the estimation of residual displacements in practical engineering design.

To this end, the correlation between residual displacements and elastic spectral as well as peak inelastic displacements is first examined for mainshock–aftershock sequence excitation. To provide a visual insight, the scatter density plots of residual displacements (d_r) versus elastic spectral displacements (S_d) and peak inelastic displacements (d_m) are presented in Figure 6, where a large ensemble of 151,200 samples (144 sequences × 30 natural periods (T = 0.1–3.0 s) × 5 levels of displacement ductility (μ = 2–6) × 7 relative intensity ratios (κ = 1/4, 1/3, 1/2, 2/3, 4/5, 1, and 4/3) generated by the elastoplastic hysteretic system under all mainshock–aftershock sequences are considered for illustration.

A comparison of Figure 6a,b reveals a stronger correlation between residual displacements and peak inelastic displacements (the Pearson correlation coefficient R = 0.88) than with elastic spectral displacements (R = 0.71), suggesting that structures experiencing large transient peak inelastic displacement is more susceptible to sustaining excessive permanent residual displacement when subjected to sequential ground motions. This observation motivates the adoption of peak inelastic displacements as the normalization basis. The residual displacement ratio for mainshock–aftershock sequences is therefore defined as follows:

$$C_{\mathrm{r},\mathrm{seq}}={\displaystyle \frac{d_{\mathrm{r}}}{d_{\mathrm{m},\mathrm{seq}}}}={\displaystyle \frac{\max \{d_{\mathrm{r},\mathrm{ms}} , d_{\mathrm{r},\mathrm{seq}}\}}{\max \{d_{\mathrm{m},\mathrm{ms}} , d_{\mathrm{m},\mathrm{seq}}\}}}$$

(3)

For the special case of κ = 0, the above definition can be converted to the residual displacement ratios under single earthquake record excitation (mainshock only), that is written as follows:

$$C_{\mathrm{r},\mathrm{ms}}={\displaystyle \frac{d_{\mathrm{r},\mathrm{ms}}}{d_{\mathrm{m},\mathrm{ms}}}}$$

(4)

where d_m,ms and d_m,seq are respectively the peak inelastic displacement under mainshock only and mainshock–aftershock sequence.

3.3. Analysis Methodology

The methodological framework of displacement-based seismic design has been profoundly shaped by advancements in constant-ductility spectral analysis. Seminal works by Newmark and Hall [48] established the theoretical basis for relating structural displacement ductility demands to spectral characteristics, a paradigm further refined through the nonlinear static procedures of Fajfar [49]. Recent extensions of these principles—such as Beyer et al.’s [50] adaptation for masonry structures, Katsanos and Sextos’ [51] exploration of period-dependent inelastic spectral ordinates, and da Silva et al.’s [52] strength–ductility relationship formulations—have collectively reinforced the validity of constant-ductility spectra as a robust tool for displacement demand quantification.

In this investigation, the constant-ductility approach is adopted in computing residual displacement demands of SDOF oscillators. The tangent stiffness-proportional damping model with an initial damping ratio of 5% is employed in NTHA. The bilinear hysteresis model is selected as shown in Figure 7. This model provides a valid approximation for simulating steel-framed structural systems. As shown in the figure, the initial elastic stiffness k_i can be determined in terms of the yield displacement d_y and the yield force F_y, that is written as follows:

$$k_{\mathrm{i}}={\displaystyle \frac{F_{\mathrm{y}}}{d_{\mathrm{y}}}}$$

(5)

The displacement ductility demand μ can be defined as follows:

$$\mu ={\displaystyle \frac{d_{\mathrm{m}}}{d_{\mathrm{y}}}}$$

(6)

where d_m is the maximum displacement that the structure reaches.

To reflect the strain hardening or softening after yielding, the post-yield stiffness ratio is defined as follows:

$$r={\displaystyle \frac{k_{\mathrm{p}}}{k_{\mathrm{i}}}}$$

(7)

where k_p is the slope of the second branch of the skeleton force–displacement relation.

The study also considers bilinear SDOF oscillators incorporating five post-yield stiffness ratios (r = 0.00, 0.01, 0.03, 0.05, and 0.10), 30 natural periods (T = 0.1–3.0 s, with an interval of 0.1 s), and five levels of displacement ductility (μ = 2–6, with an interval of 1). To examine the effect of aftershock intensity, eight levels of relative intensity ratio (κ = 1/5, 1/4, 1/3, 1/2, 2/3, 4/5, 1, and 4/3) are considered in scaling sequence ground motions.

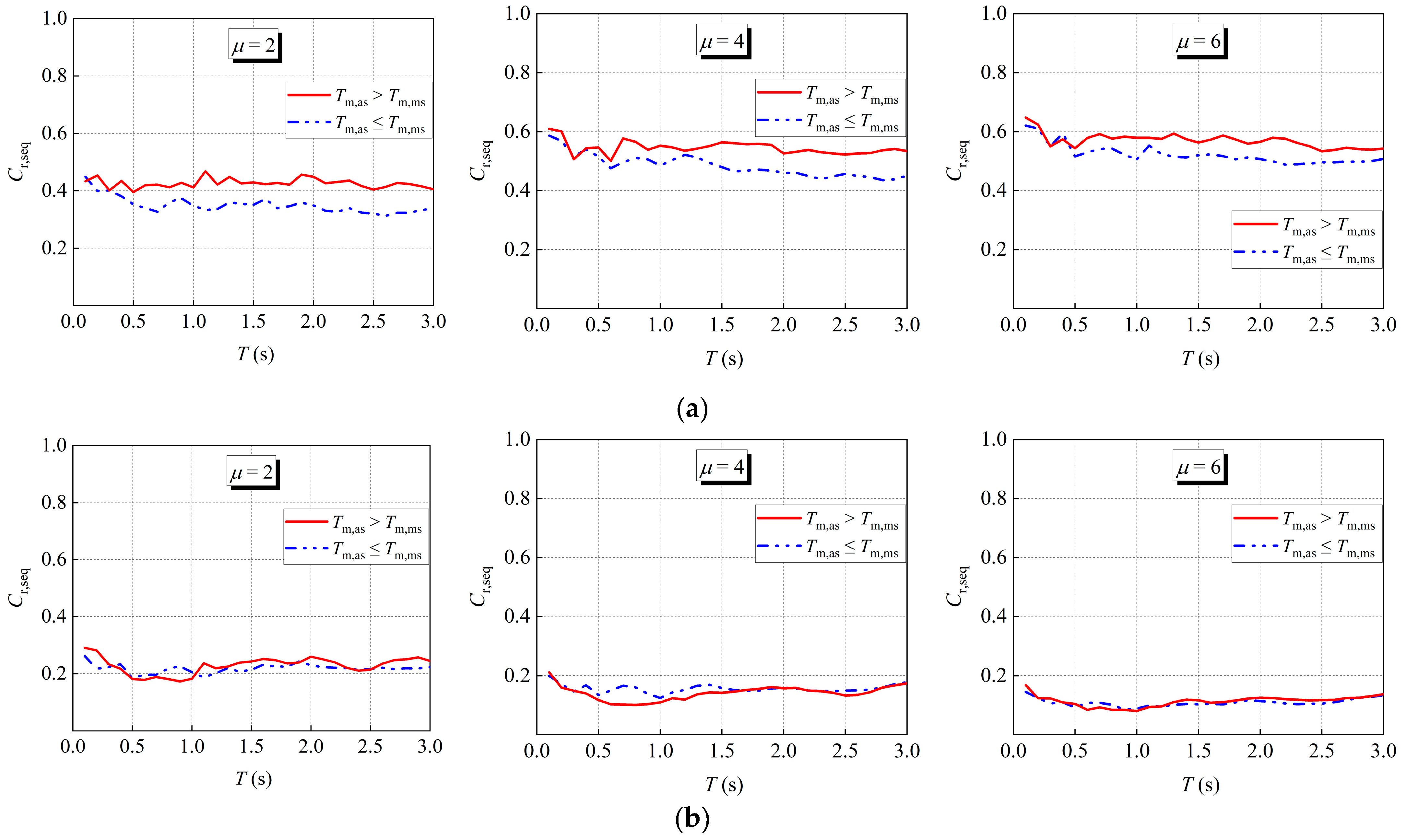
4. Effect of Aftershocks on Residual Displacements

It is worth noting that the residual-to-peak-inelastic displacement ratio as defined in Equation (4) has been extensively adopted for estimating residual displacements under single earthquake records [8,10,11,12,17,18,19,21], and several empirical equations and response spectra have also been developed and incorporated into design specifications as guidelines for structural seismic design. However, it is not clear whether such equations or response spectra can be used to accurately estimate the residual displacement of structures when subjected to mainshock–aftershock sequences. To this end, it is necessary to investigate the effect of aftershocks on residual displacement demands.

With or without considering the aftershock excitation, the residual displacement ratios can be computed from Equation (3) (i.e., C_r,seq) and Equation (4) (i.e., C_r,ms), respectively. Then the effect of aftershocks can be readily examined by comparing C_r,ms with C_r,seq. As an illustration, 144 C_r,ms and C_r,seq samples are computed for a typical elastoplastic system (i.e., T = 0.5 s, μ = 4) subjected to mainshock records with or without aftershock ground motions shown in Appendix A. Then the cumulative frequency as well as the boxplot of 144 C_r,ms and C_r,seq samples is presented in Figure 8. A comparison between C_r,ms (blue dot) and C_r,seq (red dot) cumulative frequency plots shows that the C_r,seq values are larger than the C_r,ms values across all quantiles. Particularly, the difference between the first quartile (Q1) of C_r,ms and C_r,seq samples is up to 58%. This implies that the aftershock can significantly increase the residual displacement ratios.

To have a quantitative insight into the effect of aftershocks on residual displacement ratios, the ratios of the sample mean C_r,seq to C_r,ms (i.e., C_r,seq/C_r,ms) are shown in Figure 9, in which Figure 9a corresponds to different levels of displacement ductility, Figure 9b to different aftershock intensity, and Figure 9c to different levels of post-yield stiffness ratio.

It is seen that the ratios C_r,seq/C_r,ms are always larger than the unit for all displacement ductility, relative intensity ratios, and post-yield stiffness ratios considered in this study, which thus implies that the aftershock can amplify the residual displacement ratios in the mean sense. Another observation, worthy to be noted, is that the effect of aftershocks largely depends on relative intensity ratios and post-yield stiffness ratios, especially for intense aftershock excitation and oscillators with high post-yield stiffness. For instance, for the structure with r = 0.10, the aftershock can amplify the residual displacement ratios by at most 72%.

In view of the significant effect of aftershocks on residual displacement ratios, it is obvious that conventional single-earthquake-record residual displacement spectra would underestimate demands for the situation of seismic sequence excitation. Therefore, a more reliable model for residual displacement estimation considering aftershocks needs to be developed. For this purpose, the residual displacement ratios of structures undergoing mainshock–aftershock sequences should be first statistically analyzed to investigate the influence of sequence characteristics and structural parameters.

5. Statistical Analysis of Residual Displacement Ratios

5.1. Effect of Ground Motion Duration

The duration of earthquake ground motion is a key parameter for characterizing seismic records, with its impact on the inelastic response of structures having been systematically quantified through prior studies [53,54]. However, the specific influence of ground motion duration—particularly in sequential earthquakes—on the residual displacement response of structures remains insufficiently explored, despite its critical implications for post-earthquake functionality and repair strategies.

In this section, the concept of significant duration, as defined by Trifunac and Brady [55], is adopted to quantify the time interval of strong shaking during an earthquake. Mathematically, it is determined by first normalizing the cumulative squared acceleration of the ground motion a(t):

$$F\left(t\right)={\displaystyle \frac{{\displaystyle {\int }_0^{t}a{\left(\tau \right)}^{2}d\tau }}{{\displaystyle {\int }_0^{t_{end}}a{\left(\tau \right)}^{2}d\tau }}}$$

(8)

where F(t) represents the fraction of total energy released up to time t.

The 5–95% significant duration D_s is the time interval between the first arrival at 5% (t₅) and 95% (t₉₅) of the normalized cumulative squared acceleration F(t), that is written as follows:

$$D_{\mathrm{s}}=t_{95}-t_5$$

(9)

According to the significant duration of mainshock (D_s,ms) and aftershock (D_s,as) determined in terms of Equations (8) and (9), all the selected mainshock–aftershock sequences are divided into four subsets, including: (a) long mainshock and long aftershock group (D_s,ms ≥ 8 s & D_s,as ≥ 8 s), abbreviated by Long-Long, containing 50 sequences; (b) long mainshock and short aftershock group (D_s,ms ≥ 8 s & D_s,as < 8 s), abbreviated by Long-Short, containing 47 sequences; (c) short mainshock and long aftershock group (D_s,ms < 8 s & D_s,as ≥ 8 s), abbreviated by Short-Long, containing six sequences; (d) short mainshock and short aftershock group (D_s,ms < 8 s & D_s,as < 8 s), abbreviated by Short-Short, containing 41 sequences. To ensure the validity of statistical results, the Short-Short group with a sample size that is too small is excluded from the analysis.

Figure 10 shows the residual displacement ratios C_r,seq for three groups of mainshock–aftershock sequences. It is seen that the spectral shape as well as the ordinate of C_r,seq is not significantly influenced by the sequence duration for post-yield stiffness ratios larger than zero. Significant duration seems to affect the amplitude of C_r,seq for zero post-yield stiffness ratio, particularly for natural periods between 0.5 and 2.5 s. As can be seen, the longer the duration of mainshocks and aftershocks, the larger the residual displacement ratios. This observation, however, does not necessarily apply to other period ranges. For instance, for r = 0.00 and T > 2.5 s, the amplitude of C_r,seq computed from the Short-Short group is larger than that from the Long-Long group. In general, it is concluded that the significant duration has a slighter effect on hardening structures than on elastic–perfectly plastic structures.

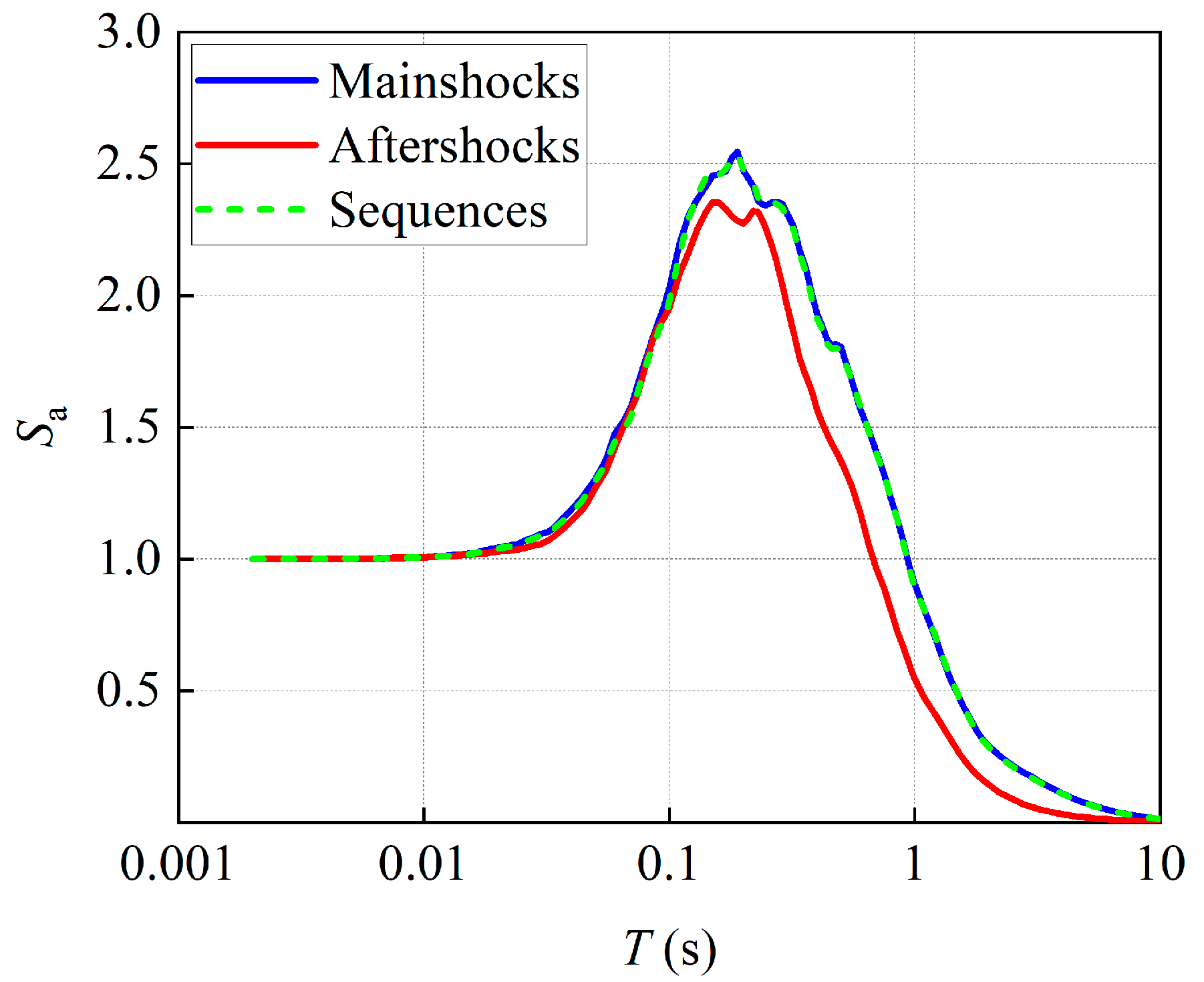
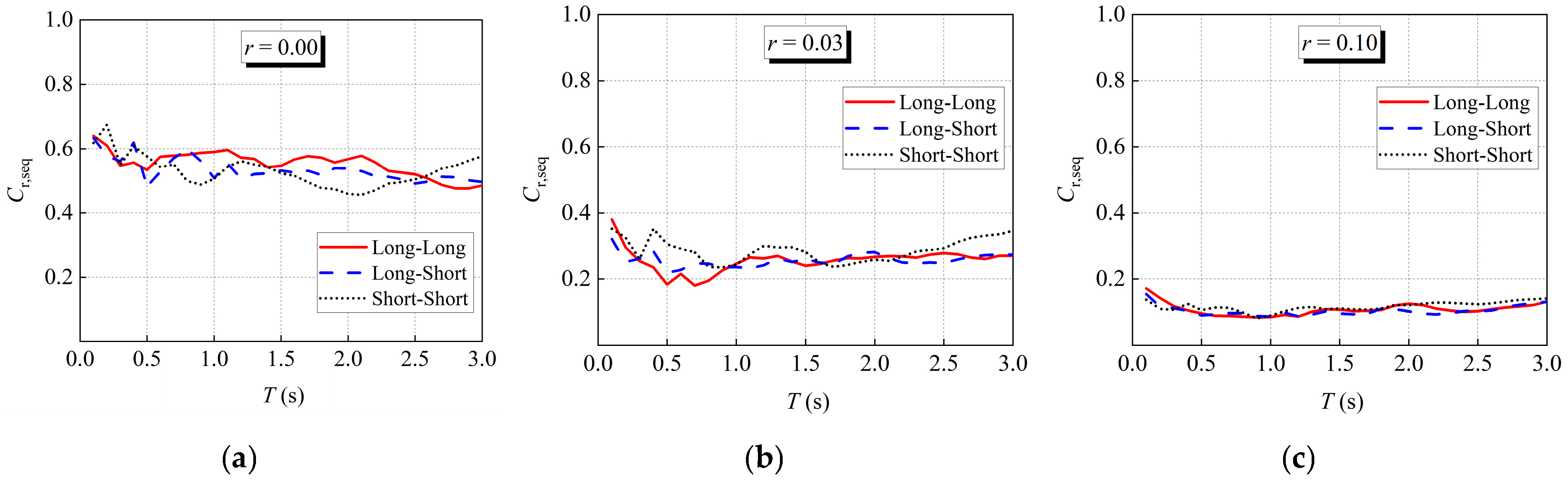
5.2. Effect of Frequency Content

The frequency content of mainshocks and aftershocks may exhibit differences due to variations in their source mechanisms (e.g., rupture complexity and scale), propagation-path effects (e.g., attenuation or scattering), and post-seismic stress redistribution. Site-specific amplification can further modify these spectral signatures. Empirical studies [25,29,56] have explicitly identified the significant difference in frequency content between the mainshock and the aftershock. However, the issue concerning the effect of such difference on residual displacements has not been examined. To this end, the definition of the mean period proposed by Rathje et al. [57] is adopted to quantify the frequency content of sequences. In accordance with the relation between the mean period of mainshock (T_m,ms) and aftershock (T_m,as), all the seismic sequences are divided into two subgroups: (a) the mean period of aftershock is longer than that of mainshock (T_m,as > T_m,ms), corresponding to 52 seismic sequences; (b) the mean period of aftershock is shorter than that of mainshock (T_m,as < T_m,ms), corresponding to 92 seismic sequences.

The residual displacement ratios, C_r,seq, corresponding to the two subgroups are compared in Figure 11, where two levels of post-yield stiffness ratios (r = 0.0 and 0.1) are considered. As can be observed from Figure 11a, except for several short natural periods, seismic sequences with T_m,as > T_m,ms generally produce larger C_r,seq than those with T_m,as < T_m,ms. This is largely attributed to the fact that the fundamental vibration period of structures will generally be elongated against strong mainshock, and T_m,as > T_m,ms greatly increases the possibility of the resonant response of damaged structures to aftershock. Such observation, however, does not hold true for high levels of post-yield stiffness ratio. As shown in Figure 11b, the C_r,seq has less to do with frequency contents for r = 0.10.

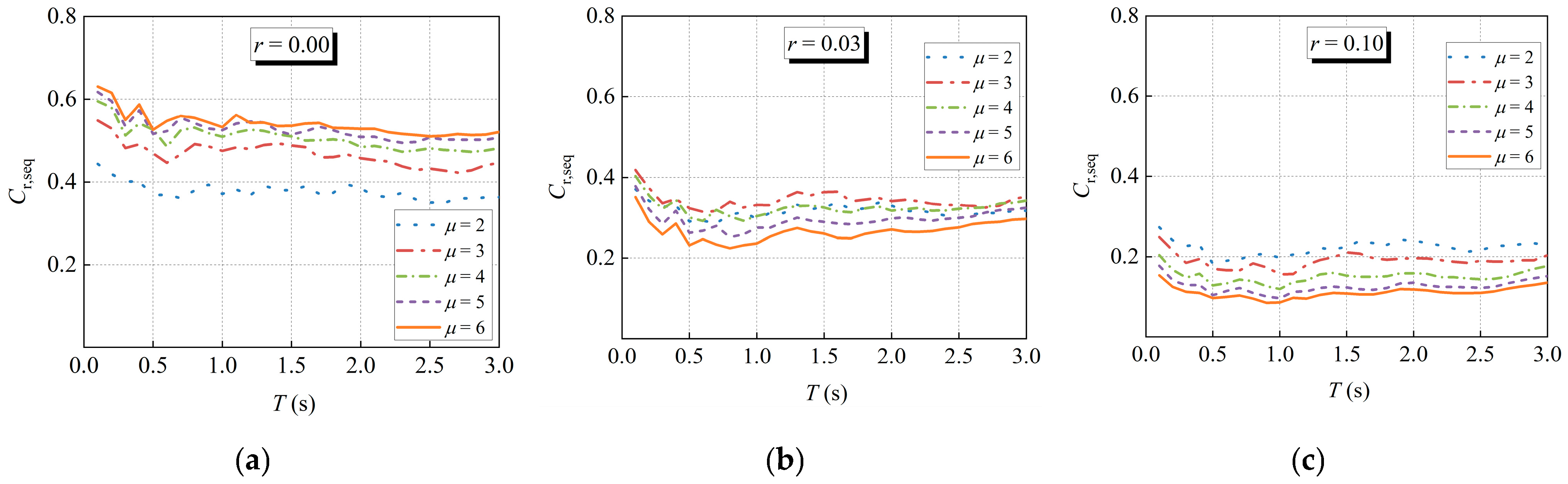
5.3. Effect of Aftershock Intensity

Past earthquake events have shown that the aftershock ground motion with various intensities occurs following the mainshock. It is thus significant to investigate the effect of aftershock ground motion intensity on residual displacement ratios.

Figure 12 shows the C_r,seq of systems when subjected to seismic sequences with different intensities of aftershock ground motions (for brevity, only five cases, κ = 1/3, 1/2, 2/3, 1.0, and 4/3, are presented). It is seen that the effect of aftershock intensity depends on the post-yield stiffness ratio. For zero post-yield stiffness ratio, the C_r,seq increases with the increase in κ, indicating that the sequences with stronger aftershock ground motions generally produce larger residual displacement ratios. However, for a higher post-yield stiffness ratio (e.g., r ≥ 0.03), the C_r,seq are relatively close to each other for different levels of κ. This observation can be explained by the fact that the yield limit of the structure with high post-yield stiffness is far away from the zero-acceleration (or force) axis, thus resulting in an oscillation being restricted within the loading–unloading branch.

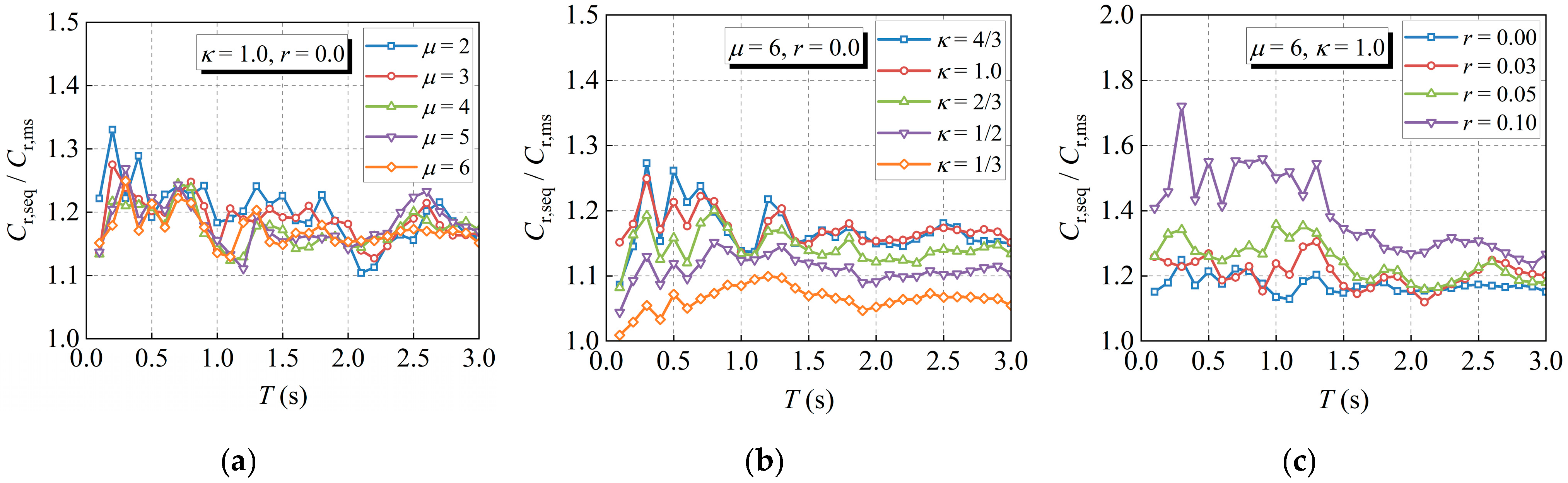
5.4. Effect of Post-Yield Stiffness

Figure 13 presents the mean residual displacement ratios for five levels of post-yield stiffness ratio. Due to space limitations, only the typical case of μ = 4 and κ = 1.0 is illustrated; other combinations exhibit similar trends. As can be observed, increasing the post-yield stiffness ratio can significantly reduce the residual displacement ratios over the whole period range. For instance, a mere increase in r from 0 to 1% will lead to a decrease of approximately 16% in C_r,seq.

5.5. Effect of Displacement Ductility

Figure 14 illustrates the mean residual displacement ratios for different levels of displacement ductility. It is obvious that the influence of displacement ductility on residual displacement ratios strongly depends on the post-yield stiffness ratio. For a zero post-yield stiffness ratio, the mean C_r,seq increases and saturates as μ increases. On the contrary, for positive post-yield stiffness ratio (r > 0), the mean C_r,seq decreases with the increase in μ.

This indicates that for elastic–perfectly plastic oscillators, the larger the displacement ductility, the higher the proportion of residual to peak inelastic displacements reaches. However, for hardening oscillators, the larger the displacement ductility, the lower the proportion of residual to peak inelastic displacements reaches.

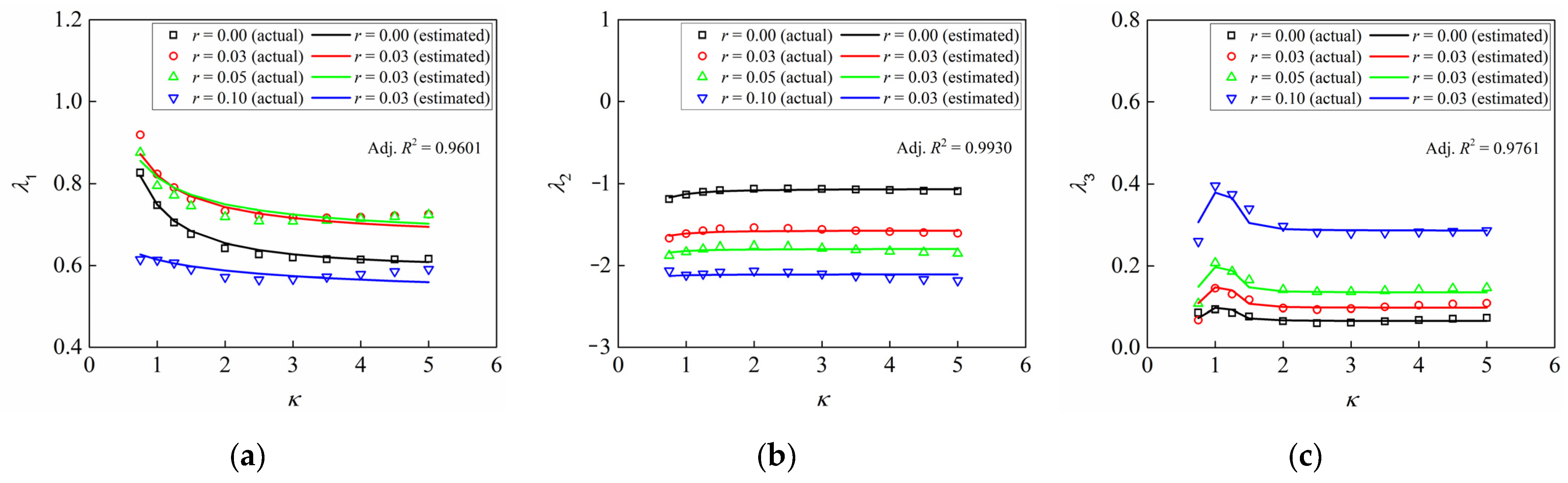
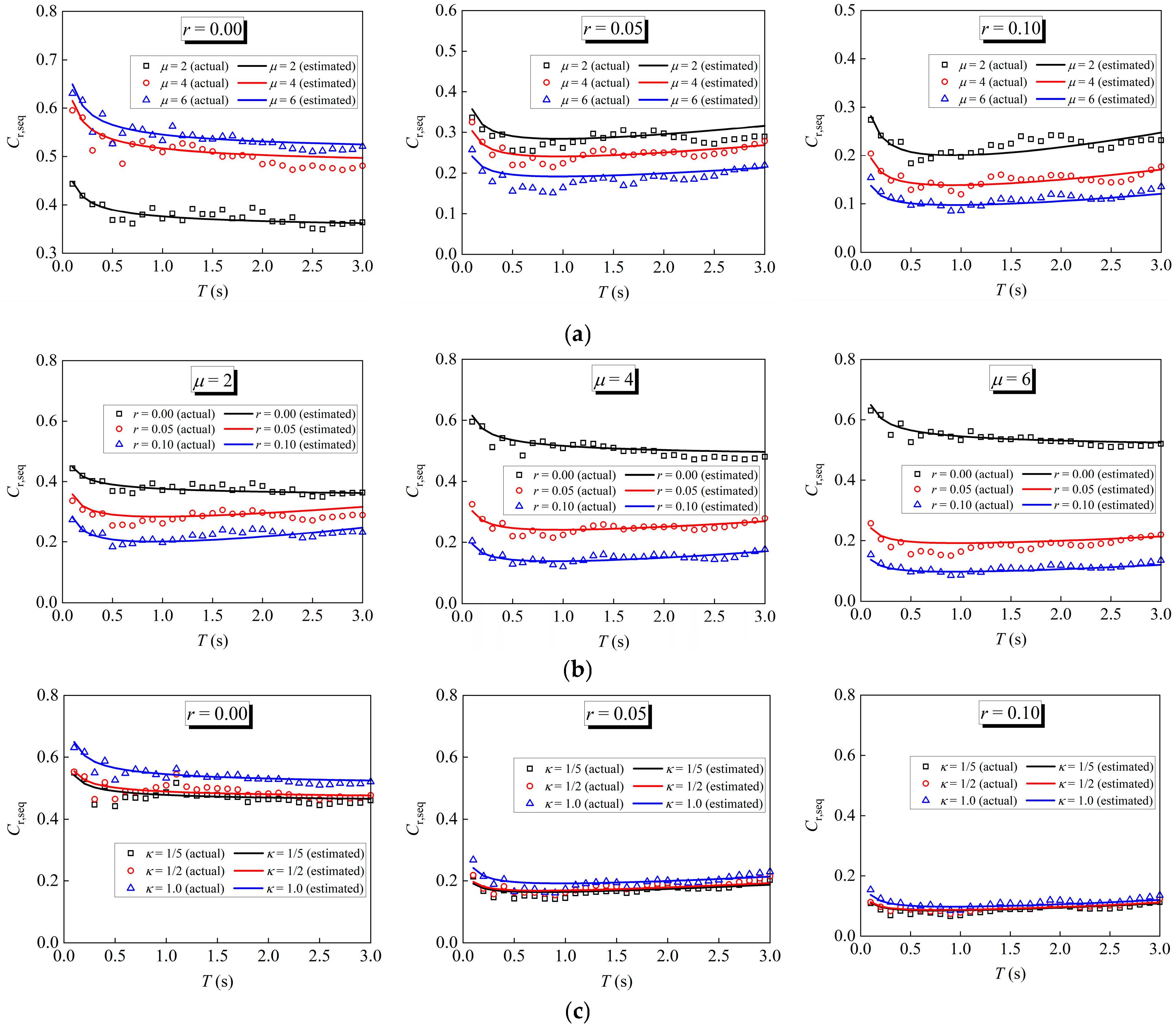
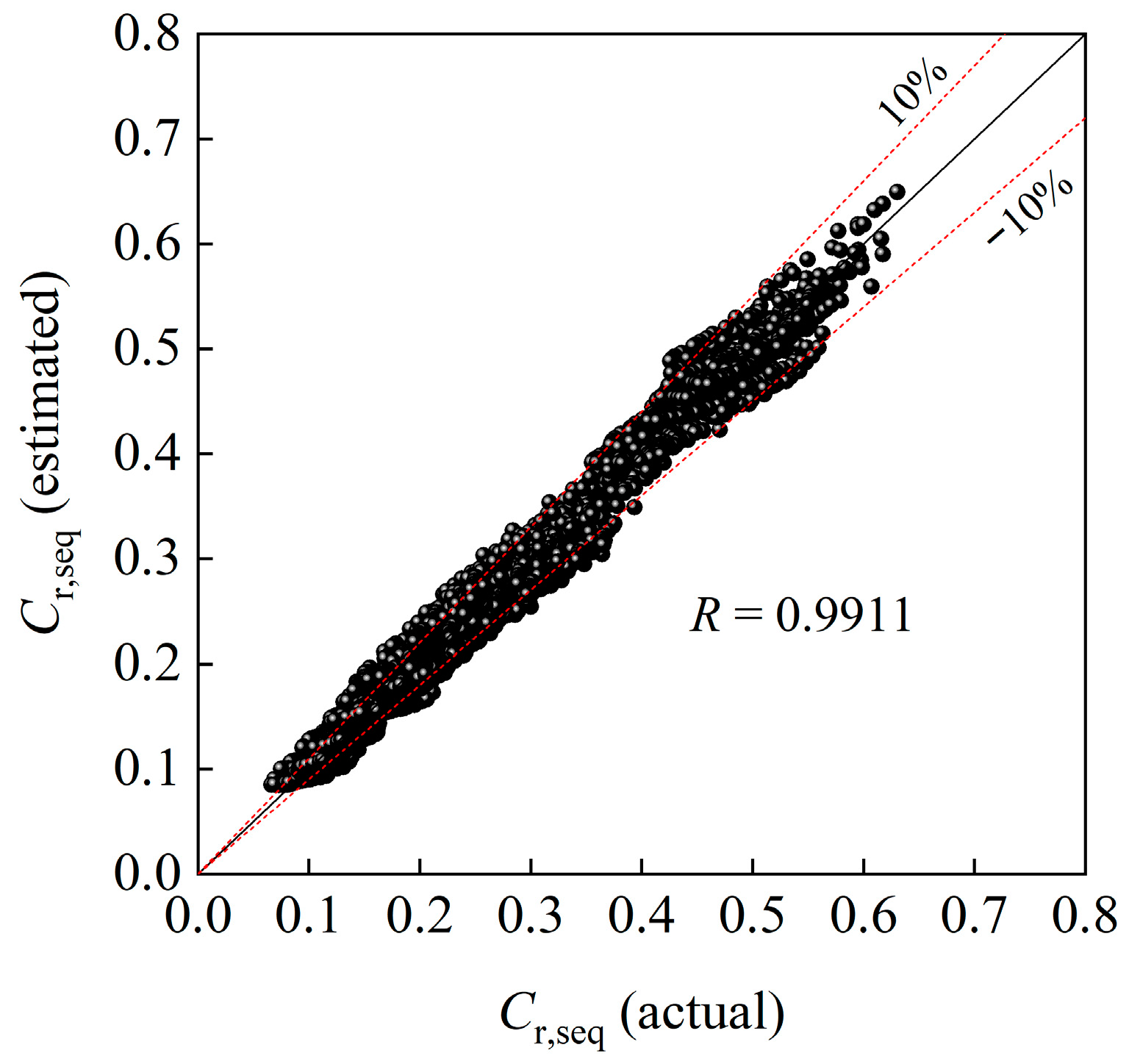
6. Constant-Ductility Residual Displacement Ratio Spectra

6.1. Establishment of Response Spectra

To enable practical estimation of residual displacement demands under mainshock–aftershock sequences, this study proposes a residual displacement ratio response spectrum. As demonstrated by the parametric analysis, the sequence-induced residual displacement ratio, C_r,seq, largely depends on the post-yield stiffness ratio r, displacement ductility μ, relative intensity ratio κ, and natural period T. To mathematically characterize this multivariate dependence while considering parameter coupling effects, a basic functional form, as presented in the following Equation (10), is adopted:

$$C_{\mathrm{r},\mathrm{seq}}=\left(\mu -1\right)\left(1-r\right)Q\left(r,\mu ,\kappa ,T\right)$$

(10)

where the introduction of terms (μ − 1) and (1 − r) is to satisfy the fundamental condition of C_r,seq(μ = 1) = 0 and C_r,seq(r = 1) = 0, since the elastic oscillators do not sustain residual displacements after earthquake excitation. The term Q(r, μ, κ, T) represents the adjustment coefficient related to r, μ, κ, and T.

To capture the dependence of Q(r, μ, κ, T) on these parameters, the following functional form, as shown in Equation (11), is selected after testing many expressions. The test is conducted with the help of the nonlinear curve fit module in the 1st Opt software (version 15.0), where the Levenberg–Marquardt algorithm is utilized to estimate the regression coefficients. Note that the adjusted determination coefficient (Adj. R²) is adopted as the preliminary criterion to evaluate the goodness-of-fit of the different regression equations. The equation that maximizes the adjusted determination coefficient and possesses the simplified function form is considered a good candidate for the best regression model.

$$Q\left(r,\mu ,\kappa ,T\right)={\lambda }_1{\mu }^{{\lambda }_2}\left[{\left(1+2r\right)}^T+{\displaystyle \frac{{\lambda }_3}{\sqrt{T}}}\right]$$

(11)

where λ₁, λ₂, and λ₃ are the regression coefficients, of which the estimated values are listed in

Table 1. Estimated values for λ₁.

r	κ
	1/5	1/4	1/3	1/2	2/3	4/5	1.0	4/3
0.00	0.6165	0.6144	0.6193	0.6421	0.6763	0.7047	0.7472	0.8267
0.03	0.7247	0.7182	0.7169	0.7327	0.7619	0.7903	0.8231	0.9193
0.05	0.7235	0.7143	0.7074	0.7185	0.7450	0.7715	0.7949	0.8758
0.10	0.5908	0.5786	0.5665	0.5714	0.5909	0.6065	0.6134	0.6147

,

Table 2. Estimated values for λ₂.

r	κ
	1/5	1/4	1/3	1/2	2/3	4/5	1.0	4/3
0.00	−1.0923	−1.0786	−1.0646	−1.0612	−1.081	−1.1013	−1.1339	−1.1895
0.03	−1.6102	−1.5896	−1.5618	−1.5391	−1.5535	−1.5780	−1.6143	−1.6699
0.05	−1.8504	−1.8255	−1.7907	−1.7615	−1.7734	−1.8002	−1.8338	−1.878
0.10	−2.1852	−2.15	−2.1017	−2.0675	−2.0808	−2.1039	−2.1168	−2.0651

and

Table 3. Estimated values for λ₃.

r	κ
	1/5	1/4	1/3	1/2	2/3	4/5	1.0	4/3
0.00	0.0731	0.0679	0.0615	0.0649	0.0762	0.0851	0.0938	0.0856
0.03	0.1084	0.1033	0.0952	0.0965	0.1168	0.1307	0.145	0.0676
0.05	0.1460	0.1416	0.1366	0.1416	0.1654	0.1862	0.2071	0.1075
0.10	0.2858	0.2826	0.2793	0.2969	0.3395	0.3745	0.3961	0.2596

.

Using Equations (10) and (11) together with Table 1, Table 2 and Table 3, the residual displacement ratios under mainshock–aftershock sequences can be predicted conveniently. It should be noted that if r and κ are not exactly equal to the values in the table but a certain value between given points, then the parameters λ₁, λ₂, and λ₃ can be determined approximately by the linear interpolation. As an alternative, the discrete data in tables can be used to develop the appropriate functional forms of λ₁, λ₂, and λ₃ via regression analysis, which can facilitate the practical application. To this end, the discrete estimates λ₁, λ₂, and λ₃ are plotted against r and κ to identify the potential trend.

Figure 15 shows the tendency of λ₁, λ₂, and λ₃ with respect to κ for different levels of r. It can be observed that the dependence of λ₁, λ₂, and λ₃ on r and κ may be characterized by the following equations:

$${\lambda }_1=0.5873+4.0321 r-53.6939 r^2+0.1609 {\kappa }^{10r-1.2635}$$

(12)

$${\lambda }_2=-2.3448+1.2790 \exp \left(-16.9347 r\right){\displaystyle \frac{{\kappa }^2}{{\kappa }^2+0.0534}}$$

(13)

$${\lambda }_3=0.0656+0.5632 r+16.3878 r^2+{\displaystyle \frac{0.0511+r}{1+{\left(6.9219\kappa -7.7095\right)}^2}}$$

(14)

The fitted curves of λ₁, λ₂, and λ₃ together with the corresponding adjusted determination coefficients are also presented in Figure 15. As can be seen, the proposed models can provide accurate estimates of λ₁, λ₂, and λ₃.

To verify the validity of the proposed residual displacement ratio response spectra, a comparison of statistical mean C_r,seq and those predicted using Equations (10)–(14) is shown in Figure 16. As can be observed, the proposed equations can reasonably describe the dependence of C_r,seq on such independent variables, particularly for the inversion of the effect of displacement ductility on C_r,seq (Figure 16a), which resulted from post-yield stiffness ratios increasing, as well as the insensitivity of C_r,seq on κ with the increase in post-yield stiffness ratios (Figure 16b).

Figure 17 illustrates the scatter plots of the actual and fitted residual displacement ratios for all cases under consideration. The correlation coefficients between the actual and fitted results are also provided in Figure 17 for examining the global goodness of fit. As can be observed, the proposed response spectra can provide reasonably accurate estimates of mean residual displacement ratios for bilinear oscillators subjected to mainshock–aftershock sequences.

6.2. Comparison with Existing Models

It is noteworthy that Madhu Girija and Gupta [18] developed a residual displacement ratio prediction model for bilinear SDOF oscillators. This model, however, is based on the statistical analysis of structural responses to single earthquake events. With the objective of validating the reliability of the proposed sequence-based model and emphasizing the necessity of incorporating aftershock effects, a comparison of their model with that proposed in this study is compelling.

Figure 18 presents a comparison of residual displacement ratios computed from the Madhu Girija–Gupta model with those from the sequence-based model proposed in this study. The results demonstrate consistent underestimation of residual displacement ratio response spectra by the conventional single-event model compared to the proposed sequence-based approach under low (μ = 2), moderate (μ = 4), and high (μ = 6) ductility demands. This discrepancy, ranging from 5% to 22% depending on structural periods, quantitatively verifies that neglecting aftershock effects leads to non-conservative residual displacement predictions, thereby justifying the critical need for sequence-dependent modeling.

6.3. Limitations and Future Work

While the proposed residual displacement spectra provide a practical framework for estimating post-earthquake displacements under sequential ground motions, the following limitations warrant explicit acknowledgment:

(1)

Simplified Structural Idealization: the spectra are formulated under the assumption of bilinear hysteretic behavior, which inherently disregards critical nonlinear characteristics such as strength degradation, stiffness deterioration, and pinch effects. While the bilinear hysteresis assumption remains applicable to structures with stable energy-dissipating mechanisms (e.g., ductile steel systems), it may lead to biased predictions of residual displacements in systems exhibiting complex hysteresis, such as reinforced concrete structures.

(2)

Temporal and Socio-Resource Considerations: the current framework focuses solely on the mechanical aspects of structural repairability and does not explicitly account for temporal factors, such as the interval between the mainshock and aftershock or the timeline of post-event resource allocation. While the spectra aid in prioritizing structures requiring urgent post-mainshock intervention (e.g., those exceeding displacement thresholds under aftershocks), the model overlooks socio-temporal dependencies critical to community-level recovery, including aftershock probability decay rates, repair resource availability, and reconstruction schedules.

To address these gaps, future research will focus on the following:

(1)

Quantifying residual displacement demands for structures with complex hysteresis (e.g., strength degradation and pinch effects) under sequential excitations.

(2)

Integrating probabilistic aftershock timing models with socio-economic recovery metrics (e.g., infrastructure interdependencies, resource mobilization curves) to link mechanical damage thresholds to functional repairability timelines.

7. Conclusions

A comprehensive study was implemented to investigate the influence of aftershocks on residual displacement ratios by comparing the seismic response of bilinear oscillators under mainshock-only and mainshock–aftershock sequences. The effect of ground motion sequence characteristics and structural parameters is examined based on the statistical analysis of nonlinear time–history analysis results. Finally, the constant-ductility residual displacement ratio response spectra are developed for simple SDOF oscillators subjected to mainshock–aftershock sequences. The main conclusions are summarized as follows:

(1)

The aftershock can significantly amplify the residual displacement ratios in a statistical mean sense. Conventional single-record-based models exhibit non-conservative predictions, underestimating residual displacement ratios by 3–42% across natural periods, thereby validating the necessity of sequence-dependent analysis.

(2)

Given the identical mainshock, seismic sequences with stronger aftershocks tend to result in larger residual displacement ratios. Such observation is particularly true for systems with low levels of post-yield stiffness ratio.

(3)

Sequences combining high-frequency mainshock with low-frequency aftershock (mean period T_m,ms < T_m,as) generate 5–40% higher residual displacement ratios than reverse configurations (i.e., T_m,ms > T_m,as) for elastic–perfectly plastic systems, highlighting spectral incompatibility risks.

(4)

For elastic–perfectly plastic systems, the larger the displacement ductility, the higher the residual displacement ratios. However, for hardening oscillators (r > 0), the larger the displacement ductility, the lower the residual displacement ratios.

(5)

The proposed response spectra, which can reasonably account for the parametric dependencies of residual displacement ratios on post-yield stiffness ratios, displacement ductility, natural periods, and relative intensity ratios, provide a reliable and rapid tool for repairability assessment of structures against mainshock–aftershock sequences in terms of residual displacements.

Based on the findings, the following practical recommendations are proposed to guide when aftershock effects should be prioritized in seismic design:

(1)

Oscillators with softening behavior (r ≤ 3%) require explicit consideration of aftershocks, as absolute residual displacements escalate disproportionately.

(2)

Structures located in regions prone to sequences with strong aftershocks (κ ≥ 1/2) require explicit sequence-based evaluation to avoid underestimation.

(3)

Regions prone to spectrally incompatible sequences (e.g., high-frequency mainshock followed by low-frequency aftershock) warrant sequence-specific assessments to mitigate residual displacement amplifications.