Mechanism of Mining-Induced Dynamic Loading in Shallow Coal Seams Crossing Maoliang Terrain
Abstract
1. Introduction
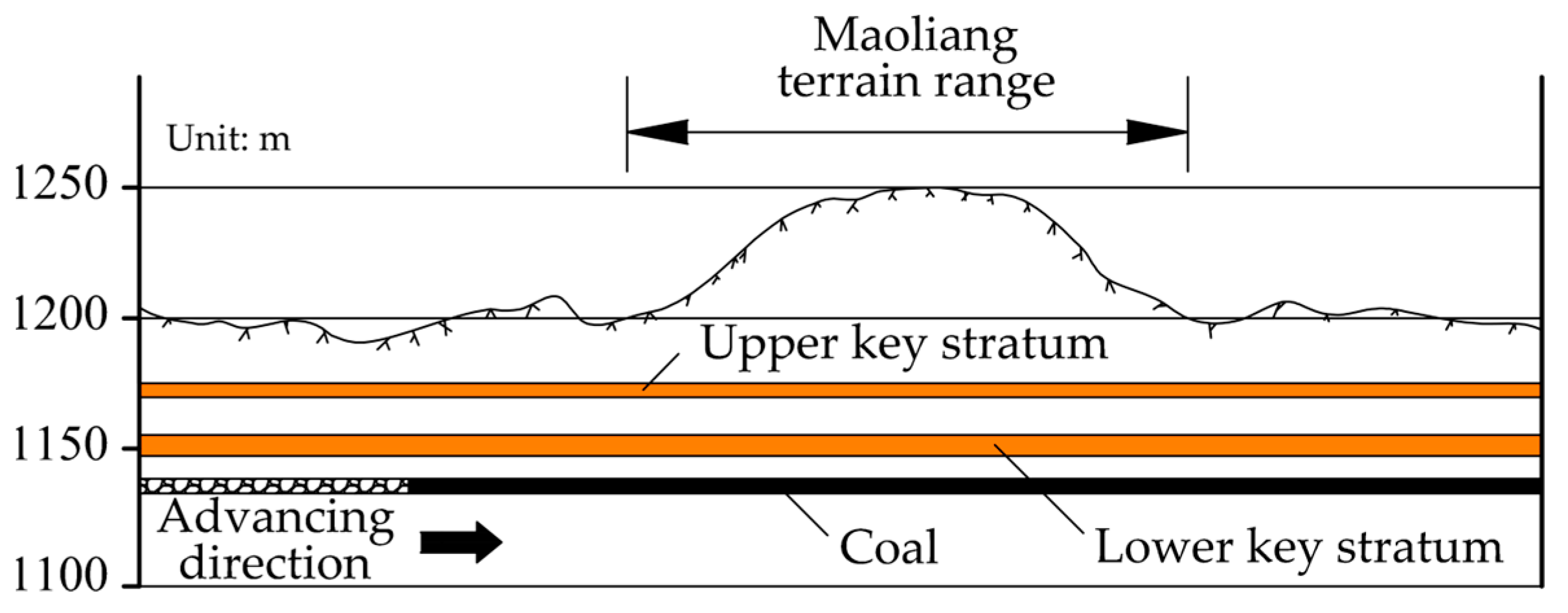
2. Working Face Overview
3. Strata Stress Distribution Characteristics
3.1. Mechanical Model Analysis
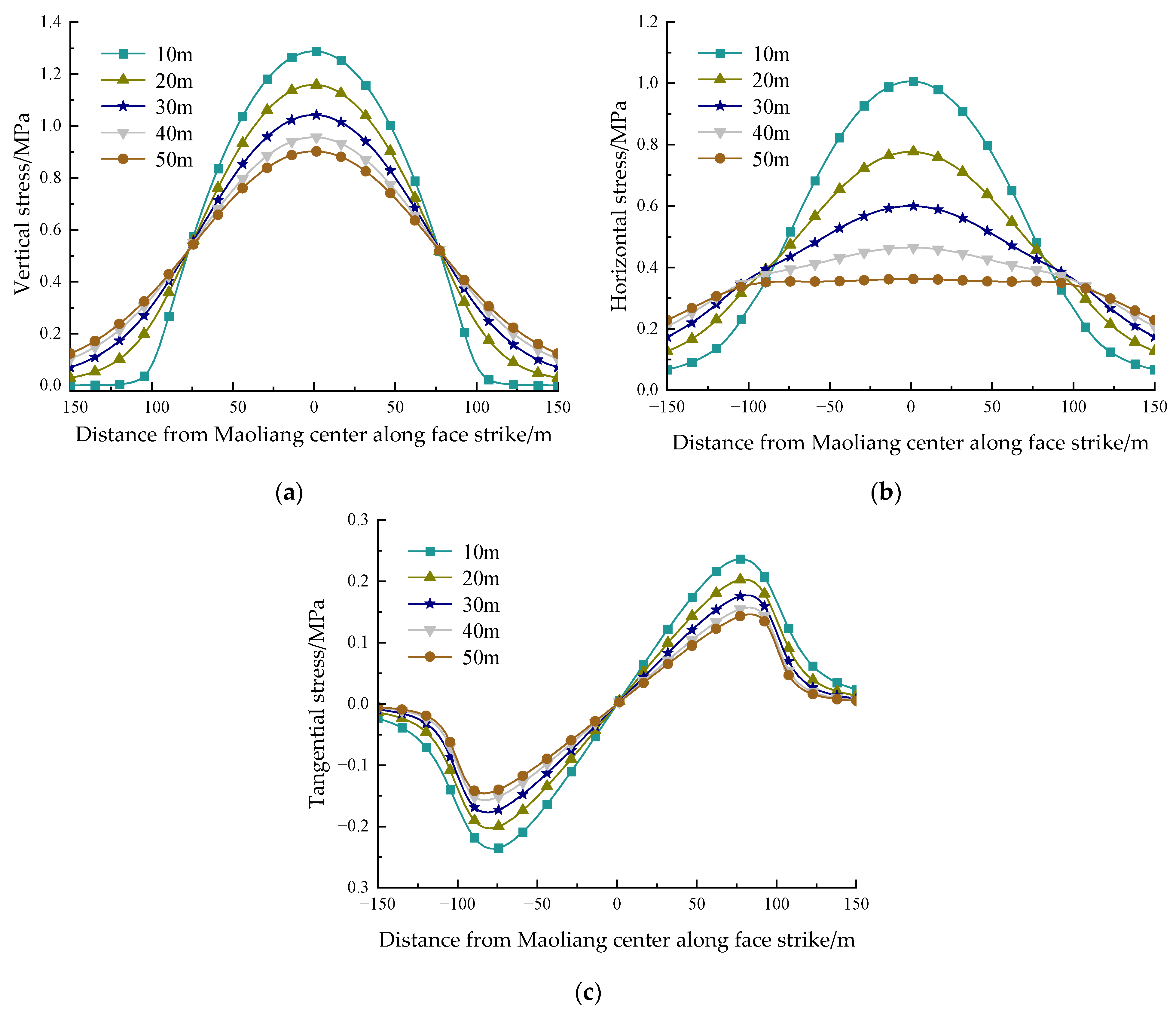
3.2. Strata Stress Distribution Patterns
4. Strata Pressure Manifestation Mechanism
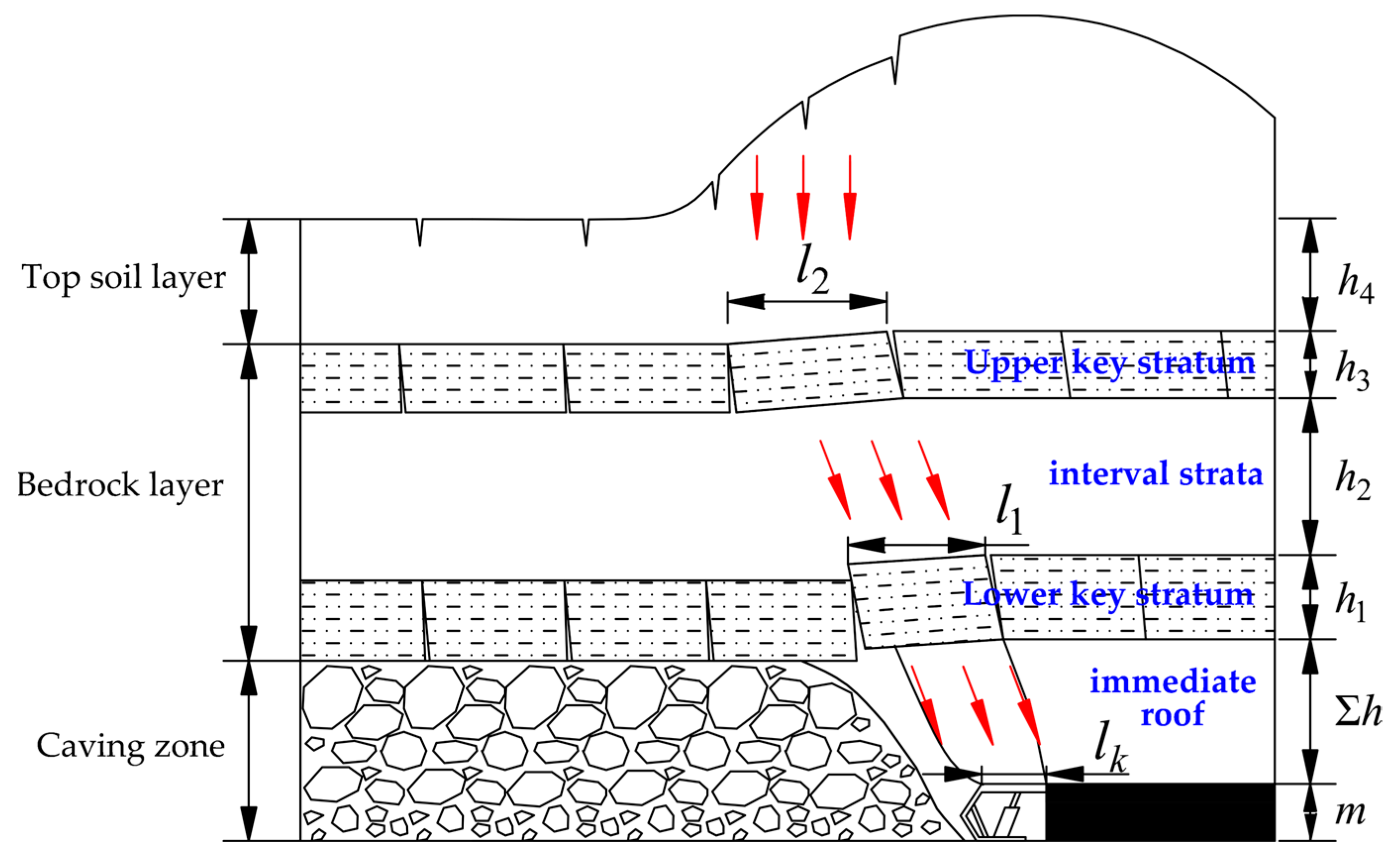
4.1. Evolution Characteristics of Overburden Structures
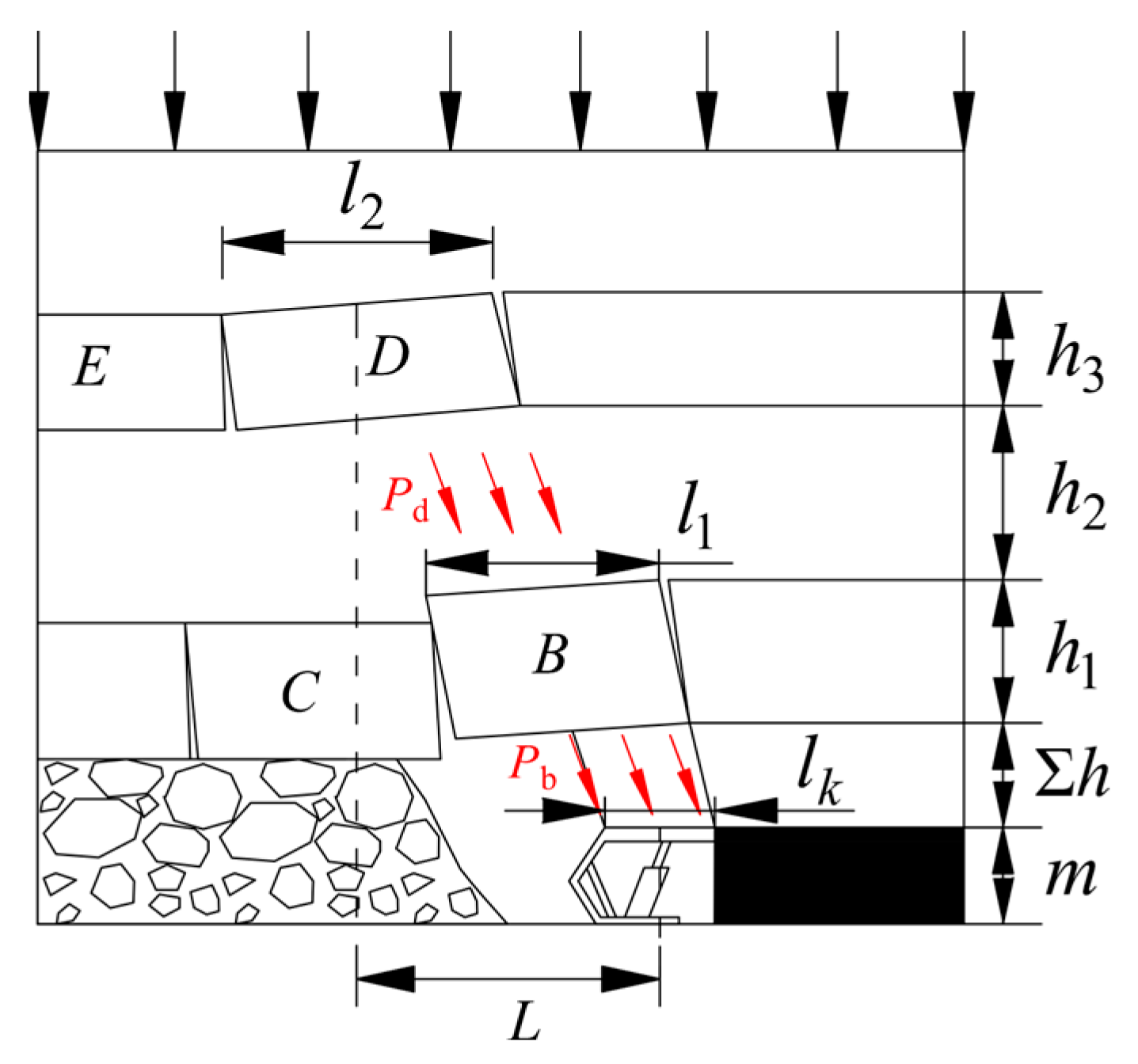
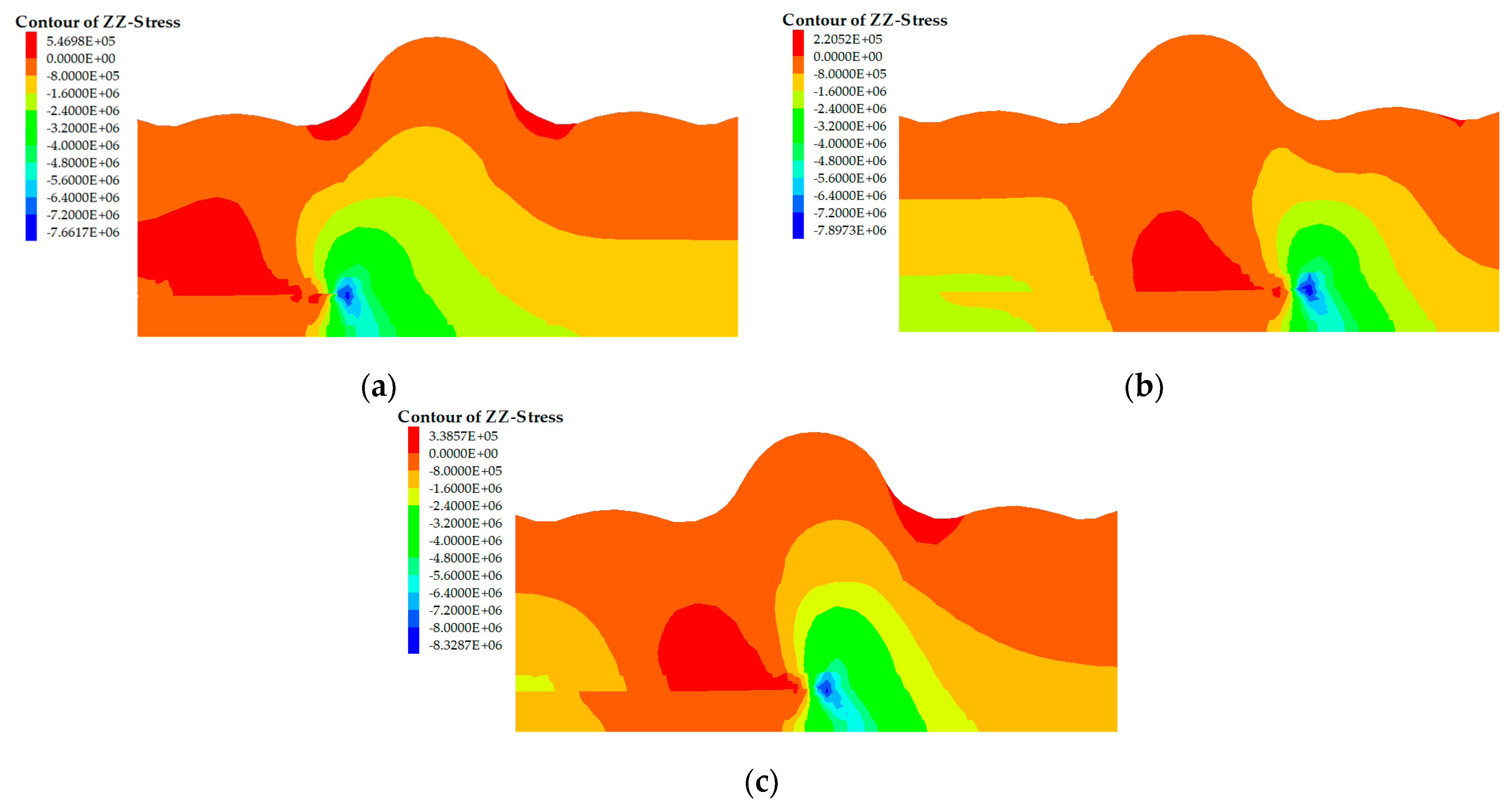
4.2. Dynamic Roof Failure and Stress Transfer Mechanisms
5. Strata Pressure Evolution Laws
6. Engineering Case Validation
6.1. Calculation of Maximum Support Working Resistance
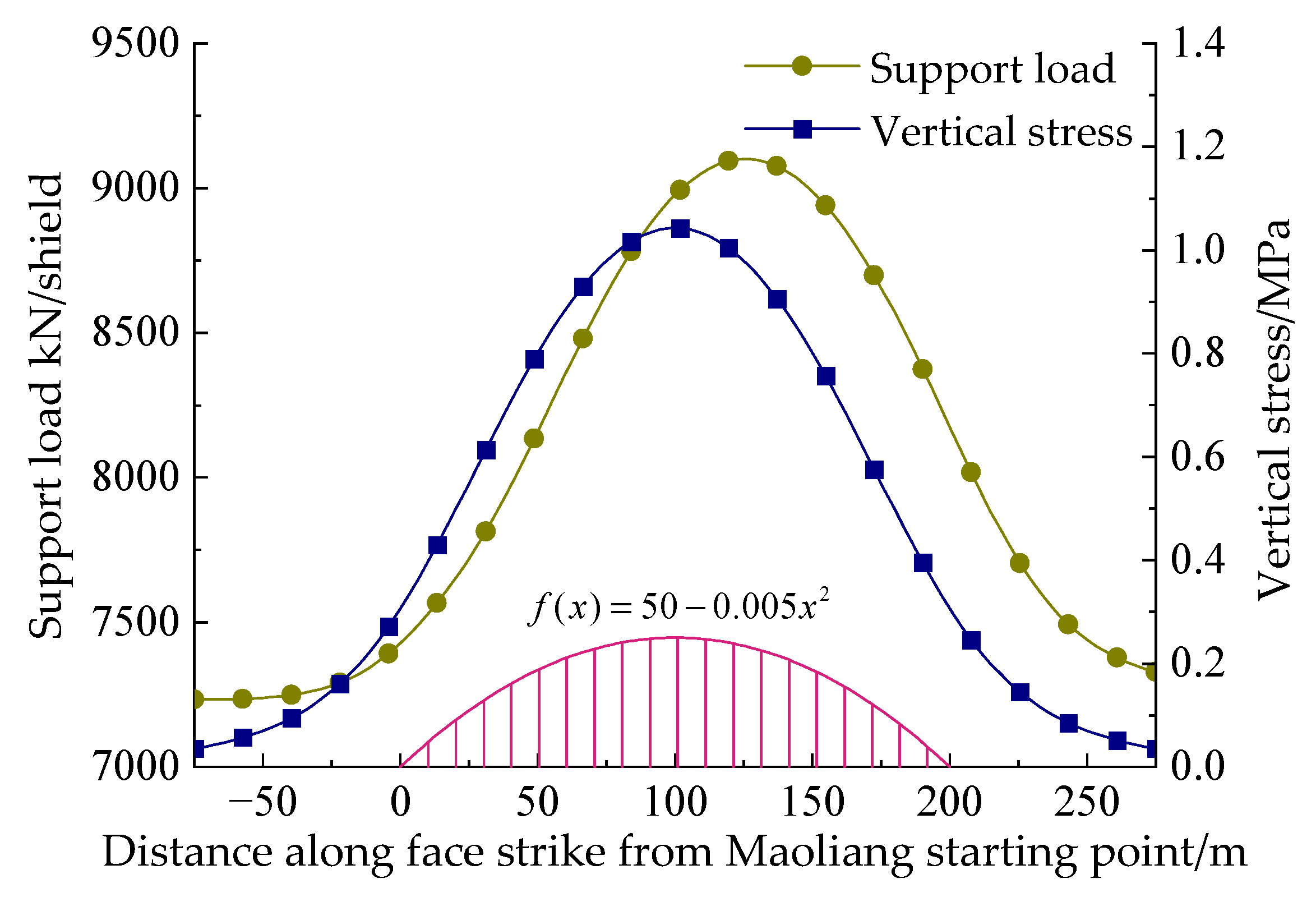
6.2. Dynamic Variation Patterns of Support Loads
- Spatiotemporal differences in key stratum fracturing
- 2.
- Synergistic deformation effect between soil and bedrock
- 3.
- Hierarchical adjustment of stress transfer paths
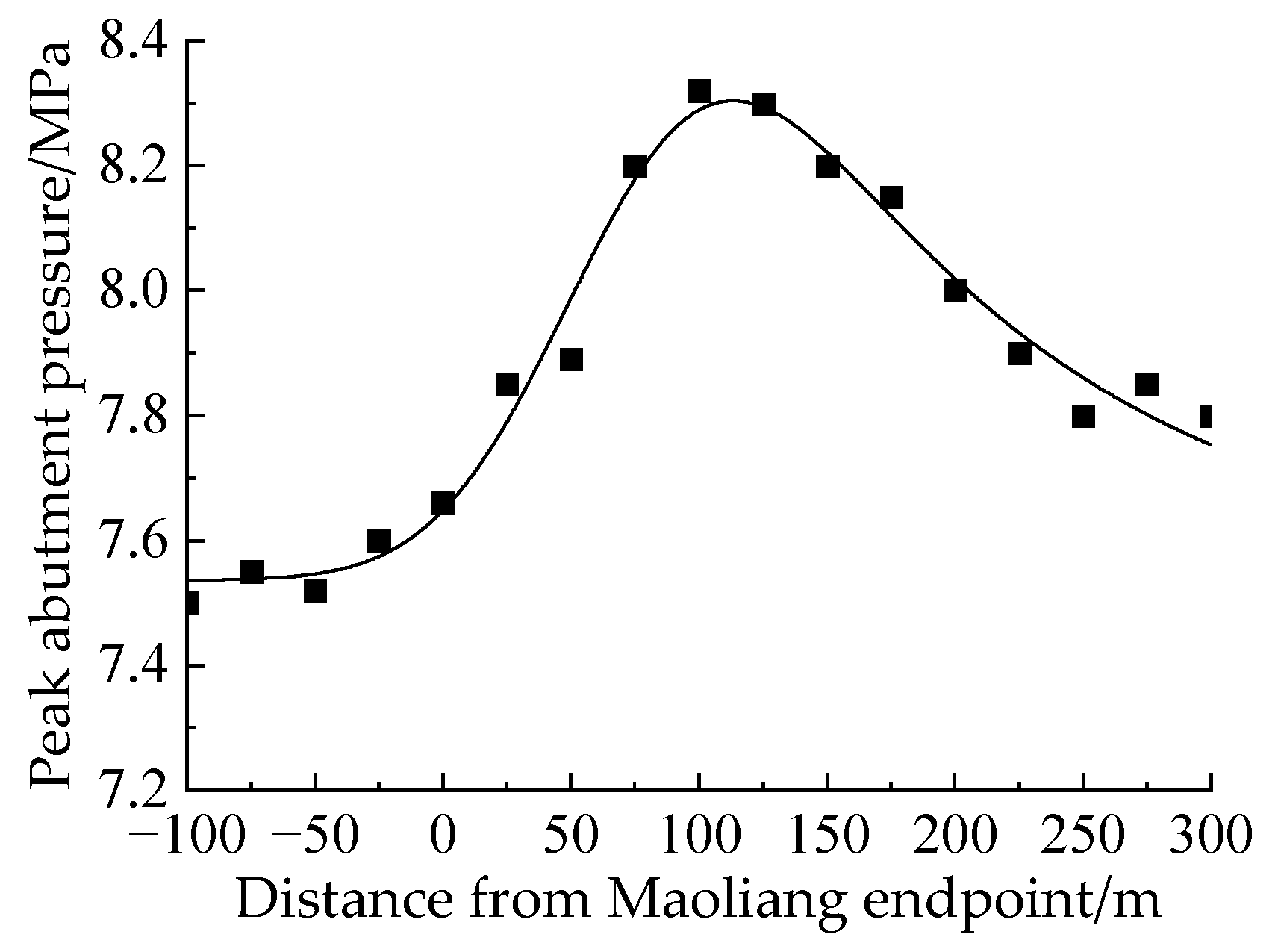
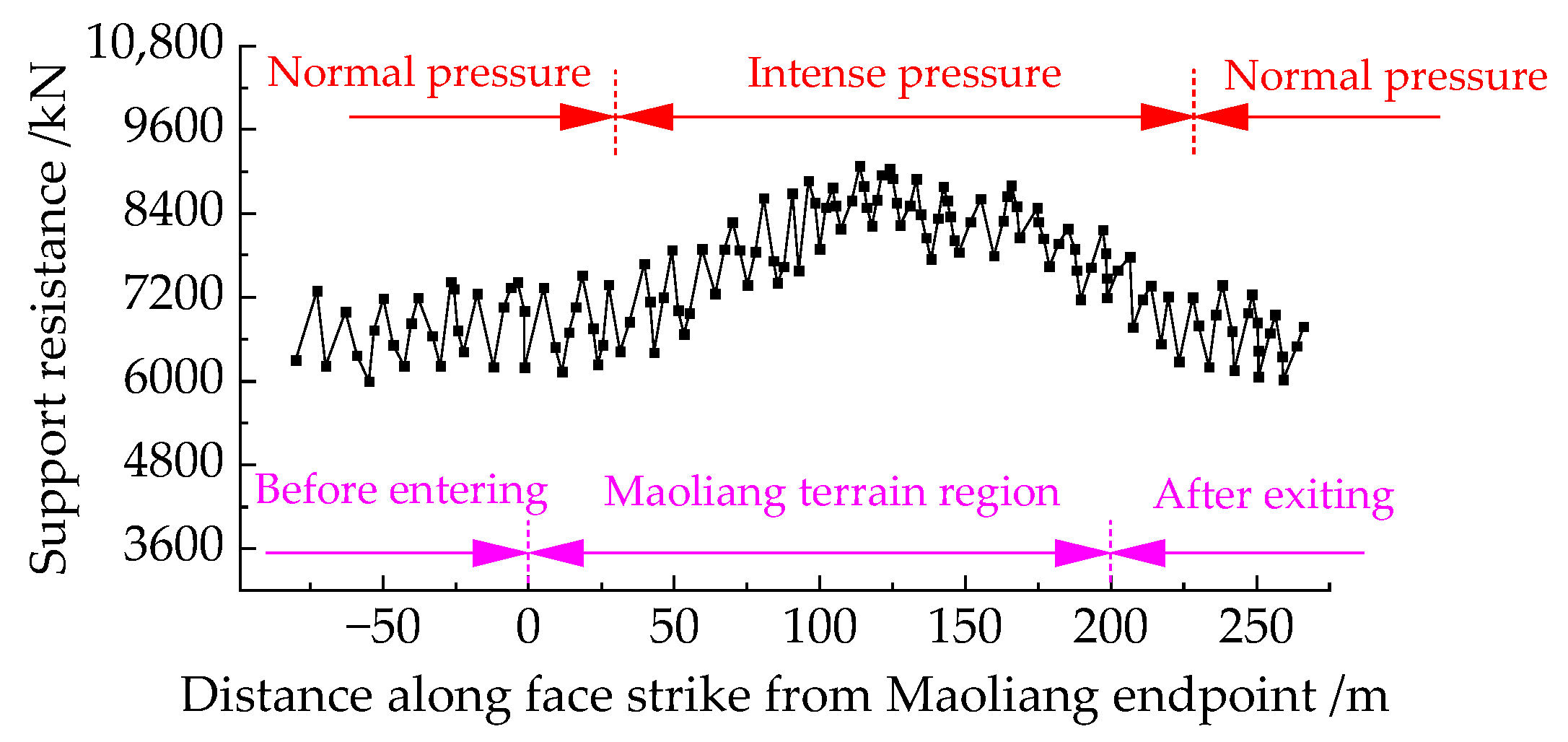
6.3. Field Monitoring Analysis of Support Working Resistance
7. Discussion
8. Conclusions
- (1)
- By constructing a static load stress transmission mechanical model for the Maoliang terrain, the stress distribution pattern of the strata under the Maoliang load was determined. Under the Maoliang load, both the vertical and horizontal stresses in the underlying strata show symmetrical peak distributions, reaching their maximum at the Maoliang center and significantly decreasing toward both sides. As the burial depth increases, the influence of the load on these stresses gradually diminishes.
- (2)
- A mechanical model of the overburden structure during mining in the Maoliang terrain zone was established, revealing the evolution characteristics of the overburden structure and stress transmission patterns in the working face. The Maoliang load has a significant impact on the peak support pressure and the support load ahead of the coal face, with a noticeable delay. The mining-induced disturbance exacerbates the failure and instability of the key overburden layers, leading to a significant increase in support load and more intense mining pressure.
- (3)
- Based on the roof stress transmission mechanical model, a calculation method for the support resistance in the Maoliang terrain zone was developed. The maximum support working resistance for the 30206 working face is 8974 kN, with an error of 3.25% compared to the theoretical calculation result of 9266 kN. Compared to the situation before entering the Maoliang zone, the support load increased by 28.04%. The range of strong mining pressure extends from 25 m to 225 m from the Maoliang endpoint, lagging behind the Maoliang load by approximately 25 m.
- (4)
- Future research directions will primarily focus on the following aspects: First, to deeply investigate the transmission laws of Maoliang terrain loads under complex geological conditions and their influences on mining pressure, and to construct more accurate mechanical models by incorporating more geological factors. Second, to expand the scope of field measurements, studies should be conducted in different mining areas to validate and refine the research results, thereby enhancing their universality. Third, advanced monitoring technologies and numerical simulation methods should be integrated for the real-time monitoring and simulation of overburden structure changes and stress distribution during mining, to provide more effective technical support for mining pressure control.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wang, S.; Wei, J.; Song, S.; Hou, E.; Sun, T. Study on overburden and surface damage characteristics of shallow-buried coal seam mining in loess gully area. Coal Sci. Technol. 2022, 50, 1–9. [Google Scholar]
- Song, S.; Ruan, H.; Wei, J.; Niu, R.; Cheng, X.; Chen, B. The Influence of the Key Characteristics of Overburden Rock Structure on the Development Height of Water-Conducting Fracture in Yushenfu Coal Mine Area, China. Appl. Sci. 2024, 14, 10537. [Google Scholar] [CrossRef]
- Zhang, D.; Fan, G.; Wang, X. Characteristics and stability of slope movement response to underground mining of shallow coal seams away from gullies. Int. J. Min. Sci. Technol. 2012, 22, 47–50. [Google Scholar] [CrossRef]
- Xu, G.; Zhang, Z.; Zhang, C.; Fan, Z.; Lu, Z.; Huang, Z.; Lu, C.; Xue, J.; Wang, C.; Chen, F.; et al. Review on roof disaster and prevention technology for coal mining face in China. J. Min. Strat. Control. Eng. 2024, 6, 16–41. [Google Scholar]
- Song, G.; Zhang, B.; Fu, X.; Du, F.; Bi, Z.; Wang, Y.; Yang, Y. Formation mechanism and application of “main control Layer-weak layer” composite structure in shallow coal seam. J. Min. Saf. Eng. 2021, 38, 286–294. [Google Scholar]
- Wang, J.; Xu, J.; Yang, S.; Wang, Z. Development of strata movement and its control in underground mining: In memory of 40 years of Voussoir Beam Theory proposed by Academician Minggao Qian. Coal Sci. Technol. 2023, 51, 80–94. [Google Scholar]
- Zhao, B.; Guo, Y.; Sun, H.; Yang, X.; Wang, J.; Zhao, Y. Stability research on the water-resistant strata under mining in nearly shallow coal seam based on location of main key stratum. J. Min. Saf. Eng. 2022, 39, 653–662. [Google Scholar]
- Hou, Z. Study on key stratum in shallow seam. J. China Coal Soc. 1999, 24, 359–363. [Google Scholar]
- Huang, Q. Ground pressure behavior and definition of shallow seams. Chin. J. Rock Mech. Eng. 2002, 21, 1174–1177. [Google Scholar]
- Huang, Q.; Qian, M.; Shi, P. Structural analysis of main roof stability during periodic weighting in longwall face. J. China Coal Soc. 1999, 24, 581–585. [Google Scholar]
- Huang, Q.; Wang, X.; He, Y.; Li, K.; Li, J.; Liu, J.; Wang, S. Activated roof structure and support dynamic load inshallow-buried close coal seam mining. J. Min. Saf. Eng. 2022, 39, 857–866. [Google Scholar]
- Zhang, J.; He, Y. Research on the fracture evolution law and combined bearing structure load of shallow buried coal seam group. Coal Sci. Technol. 2023, 51, 65–76. [Google Scholar]
- Ren, Y. Presentation and verification of “cantilever beam-articulated rock beam” composite structure in shallow buried working face. J. China Coal Soc. 2019, 44, 1–8. [Google Scholar]
- Shang, T.; Wang, J.; Liu, Y.; Suo, Y.; Wang, Z.; Fu, S.; Zhang, F. A Study on the Instability Mechanisms of Coal Pillars in Shallow Coal Seams Group Mining. Adv. Civ. Eng. 2023, 2023, 4646450. [Google Scholar] [CrossRef]
- Lai, X.; Zhu, H.; Guo, Z.; Gu, H.; Li, X.; Zhao, Q.; Shan, P. Breaking dynamic load mechanism and application of high key strata in gully area of shallow coal seam. J. Min. Saf. Eng. 2024, 41, 879–888. [Google Scholar]
- Lai, X.; Qiao, H.; Shan, P.; Wu, L.; Zhu, H.; Zhao, H.; Dai, Z. Evolution characteristics of water flowing fracture in shallow buried coal seam in water abundance gully valley area. Coal Sci. Technol. 2024, 52, 1–11. [Google Scholar]
- Liu, C.; Li, J.; Zhao, J.; Chen, Y.; Zhang, H.; Yu, X. Dynamic strata pressure mechanism and distribution characteristics of overburden air leakage fissures in the condition of shallow thick coal seam in gully area. J. Min. Saf. Eng. 2023, 40, 965–971. [Google Scholar]
- Zhang, J.; Long, J.; Yang, T.; Wang, B.; Zhou, F. Study on dynamic loading mechanism of mining in gully area of shallow coal seam. J. Min. Saf. Eng. 2019, 36, 1222–1227. [Google Scholar]
- Xie, X.; Hou, E.; Zhao, B.; Feng, D.; Hou, P. Investigating the Evolution Law and Fracture Mechanism of Overlying Coal-Bearing Strata Caused by Shallow Multi-Seam Mining in a Gully Area. Appl. Sci. 2025, 15, 2649. [Google Scholar] [CrossRef]
- Fu, Y.; Li, C.; He, Y. Research on the Movement of Overlying Strata in Shallow Coal Seams with High Mining Heights and Ultralong Working Faces. Appl. Sci. 2024, 14, 4685. [Google Scholar] [CrossRef]
- Wang, X.F.; Zhang, D.S.; Zhang, C.G.; Fan, G.W. Mechanism of mining-induced slope movement for gullies overlaying shallow coal seams. J. Mt. Sci. 2013, 10, 388–397. [Google Scholar] [CrossRef]
- Wang, B.; Liu, H.; Liu, D.; Zhang, J.; Lin, H. Study on Deformation Mechanism and Surrounding Rock Strata Control in End-Mining Retracement Roadway in Closely Spaced Coal Seams. Appl. Sci. 2025, 15, 436. [Google Scholar] [CrossRef]
- Huang, Q.; Wang, X.; Hu, J.; Zhou, H.; Li, J.; He, Y. Activated roof structure and dynamic load of support under goaf of shallow-buried close coal seams in loess hilly area. J. Min. Saf. Eng. 2023, 40, 983–990. [Google Scholar]
- Di, S.; Wang, J.; Li, D.; Qi, X.; Wang, Y. Influence of hill topography on ground pressure feature of shallow buried 7.0 m working face. China Saf. Sci. J. 2018, 28, 164–169. [Google Scholar]
- Wang, Y.; Zhang, X.; Xu, Q. Study on strata behavior characteristics of fully mechanized mining face at near shallow buried coal seam with superimposed static and dynamic loading in hill terrain. J. Saf. Sci. Technol. 2017, 13, 115–121. [Google Scholar]
- Wang, X.; Zhang, D.; Fan, G.; Zhang, C. Underground pressure characteristics analysis in back-gully mining of shallow coal seam under a bedrock gully slope. Min. Sci. Technol. 2011, 21, 23–27. [Google Scholar]
- Zhao, Y.; Wang, S.; Zou, Y.; Wang, X.; Huang, B.; Zhang, X. Pressure-Arching Characteristics of Fractured Strata Structure during Shallow Horizontal Coal Mining. Teh. Vjesn. 2018, 25, 1457–1466. [Google Scholar]
- Yang, D. Analysis on fracture mechanics theory of roof cutting instability mechanism with large mining height face in shallow coal seam. Front. Earth Sci. 2023, 10, 900144. [Google Scholar] [CrossRef]
- Du, F.; Yuan, R.; Zheng, J.; Song, G. Mechanism of abnormal strata pressure of mining under coal pillar in close distance shallow coal seams. J. China Coal Soc. 2017, 42, 24–29. [Google Scholar]
- Zhao, Y.; Wang, S.; Hagan, P.; Guo, W. Evolution Characteristics of Pressure-Arch and Elastic Energy during Shallow Horizontal Coal Mining. Teh. Vjesn. 2018, 25, 867–875. [Google Scholar]
- Hu, S.; Yu, T. Study on overlying rock movement and mine pressure behavior in shallow-buried close coal multi-seam mining. Alex. Eng. J. 2024, 105, 578–587. [Google Scholar] [CrossRef]
- Liu, Y.; Kong, D.; Li, P.; Wen, Z.; Li, F.; Zuo, Y.; Wu, G. The migration and evolution law of overlying strata and the instability and failure characteristics of end face roof under the condition of ascending mining in close distance coal seam: Case study. Eng. Fail. Anal. 2024, 165, 108809. [Google Scholar]
- Zhang, J.; Sun, J.; He, Y.; Zhang, Y.; Pang, H.; Wu, H.; Zhang, J.; Peng, B. Study on floor stress distribution law and roadway layout of contiguous coal seams. Coal Sci. Technol. 2024, 52, 11–22. [Google Scholar]
- Yuan, Y.; Yuan, C.F.; Zhu, C.; Liu, H.X.; Wang, S.Z. Study on the disaster reduction mechanism of presplitting blasting and reasonable blasting parameters for shallowly buried remnant pillars. Energy Sci. Eng. 2019, 7, 2884–2894. [Google Scholar] [CrossRef]
- Lan, T.; Liu, Y.; Yuan, Y.; Liu, H.; Liu, H.; Zhang, S.; Wang, S. Mine pressure behavior law of isolated island working face under extremely close goaf in shallow coal seam. Sci. Rep. 2023, 13, 20576. [Google Scholar] [CrossRef]
- Ren, Y. Influence of surface mountain load on mining pressure of shallow buried deep working face. Min. Saf. Environ. Prot. 2020, 47, 77–81+87. [Google Scholar]
- Ju, J.; Xu, J. Surface stepped subsidence related to top-coal caving longwall mining of extremely thick coal seam under shallow cover. Int. J. Rock Mech. Min. Sci. 2015, 78, 27–35. [Google Scholar] [CrossRef]
- Li, L.; Wu, G.; Liu, Q. Study on Overburden Movement and Fissure Evolution Law of Protective Layer Mining in Shallow Coal Seam. Energies 2022, 15, 1831. [Google Scholar] [CrossRef]
- Makarov, V.; Perov, M.; Bilan, T.; Novoseltsev, O.; Zaporozhets, A. Technological State of Coal Mining in Ukraine. In Geomining: Studies in Systems, Decision and Control; Springer: Cham, Switzerland, 2024; Volume 224, pp. 31–34. [Google Scholar]
- Makarov, V.; Kaplin, M.; Bilan, T.; Perov, M.; Zaporozhets, A. Forecasting the Technological Development of the Coal Industry. In Geomining: Studies in Systems, Decision and Control; Springer: Cham, Switzerland, 2024; Volume 224, pp. 165–177. [Google Scholar]


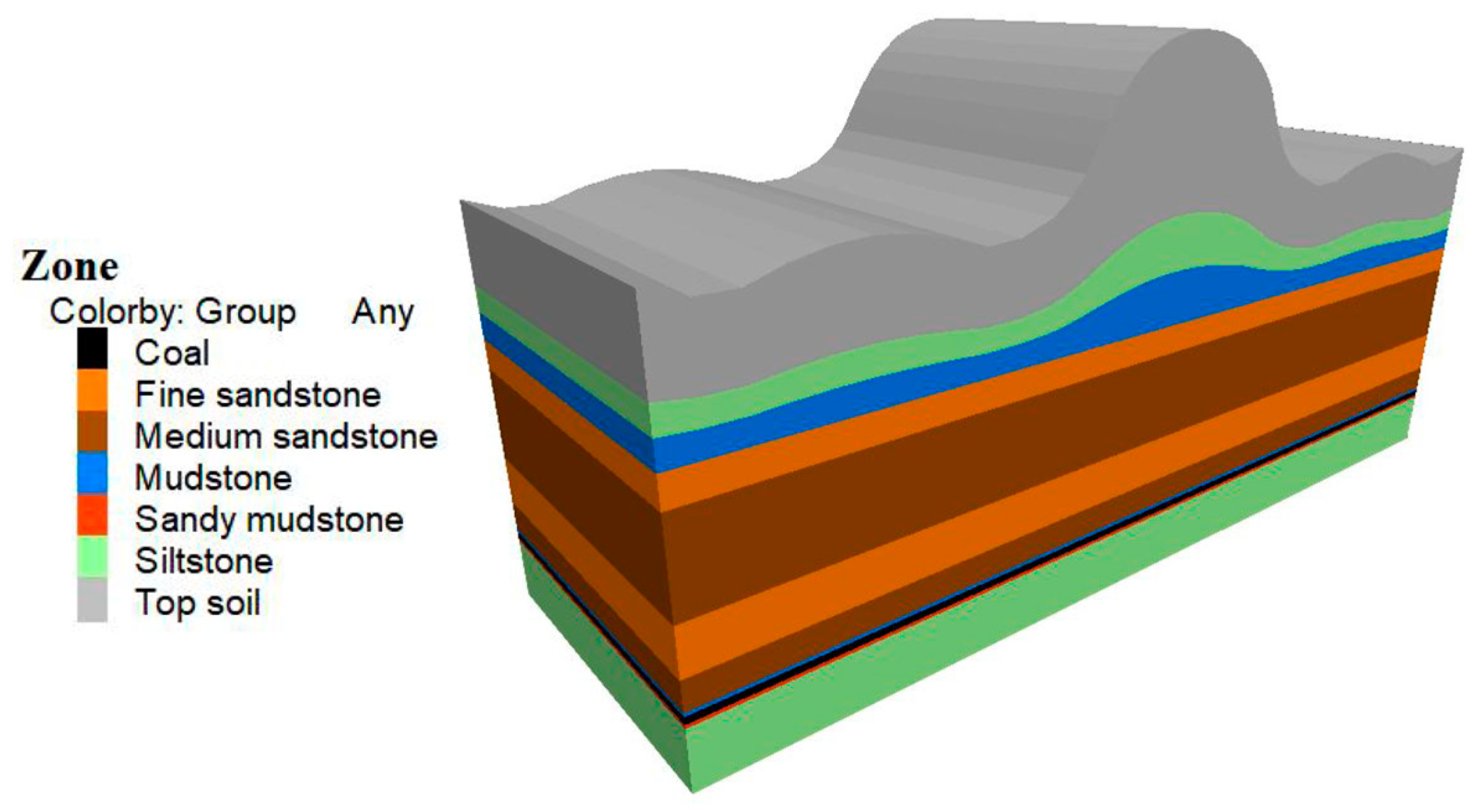
| Rock Stratum | Thickness (m) | Unit Weight (kN/m3) | Physical and Mechanical Parameters | ||||
|---|---|---|---|---|---|---|---|
| Bulk Modulus (GPa) | Shear Modulus (GPa) | Cohesion (MPa) | Internal Friction Angle (°) | Tensile Strength (MPa) | |||
| Top soil | 10.4 | 20 | 0.8 | 0.6 | 0.05 | 12 | 0.05 |
| Siltstone | 6.5 | 26 | 14.6 | 10.5 | 10.20 | 36 | 2.40 |
| Mudstone | 8.4 | 24 | 10.3 | 8.2 | 7.60 | 32 | 1.50 |
| Fine sandstone | 7.6 | 26 | 13.0 | 9.8 | 9.50 | 34 | 1.60 |
| Medium sandstone | 25.2 | 25 | 12.6 | 9.4 | 9.00 | 32 | 1.90 |
| Fine sandstone | 13.2 | 26 | 13.0 | 9.8 | 9.50 | 34 | 1.60 |
| Medium sandstone | 8.2 | 25 | 12.6 | 9.4 | 9.00 | 32 | 1.90 |
| Mudstone | 0.3 | 22 | 7.2 | 3.6 | 2.20 | 28 | 1.20 |
| Coal | 2.2 | 16 | 6.8 | 3.2 | 2.10 | 25 | 1.00 |
| Sandy mudstone | 0.4 | 25 | 11.8 | 8.0 | 8.00 | 31 | 1.60 |
| Siltstone | 19.5 | 26 | 14.6 | 10.5 | 10.20 | 36 | 2.40 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, J.; Qin, G.; Yang, T.; Wang, B.; He, Y.; Gao, S. Mechanism of Mining-Induced Dynamic Loading in Shallow Coal Seams Crossing Maoliang Terrain. Appl. Sci. 2025, 15, 4550. https://doi.org/10.3390/app15084550
Zhang J, Qin G, Yang T, Wang B, He Y, Gao S. Mechanism of Mining-Induced Dynamic Loading in Shallow Coal Seams Crossing Maoliang Terrain. Applied Sciences. 2025; 15(8):4550. https://doi.org/10.3390/app15084550
Chicago/Turabian StyleZhang, Jie, Guang Qin, Tao Yang, Bin Wang, Yifeng He, and Shoushi Gao. 2025. "Mechanism of Mining-Induced Dynamic Loading in Shallow Coal Seams Crossing Maoliang Terrain" Applied Sciences 15, no. 8: 4550. https://doi.org/10.3390/app15084550
APA StyleZhang, J., Qin, G., Yang, T., Wang, B., He, Y., & Gao, S. (2025). Mechanism of Mining-Induced Dynamic Loading in Shallow Coal Seams Crossing Maoliang Terrain. Applied Sciences, 15(8), 4550. https://doi.org/10.3390/app15084550






