Stability Analysis of the Backfill Roof with Basket Weave Drift Layout in Underhand Drift-and-Fill Mining
Abstract
1. Introduction
2. Numerical Simulation with FLAC3D
2.1. Numerical Model
2.2. The Iterative Search Method for Assessing the Strength Requirement of Backfill Roof
3. Numerical Results
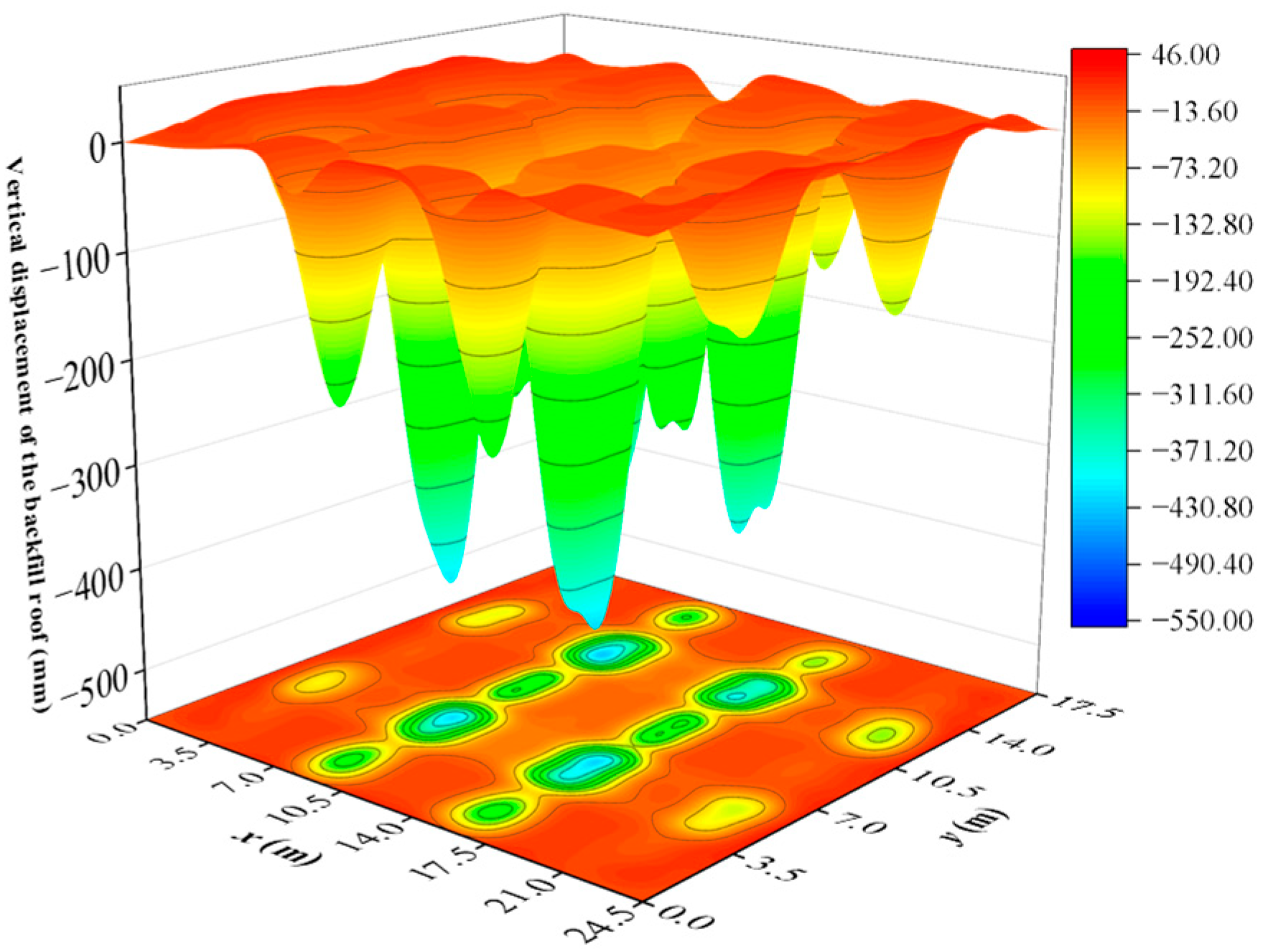
3.1. Displacement Distribution Characteristics of the Backfill Roof
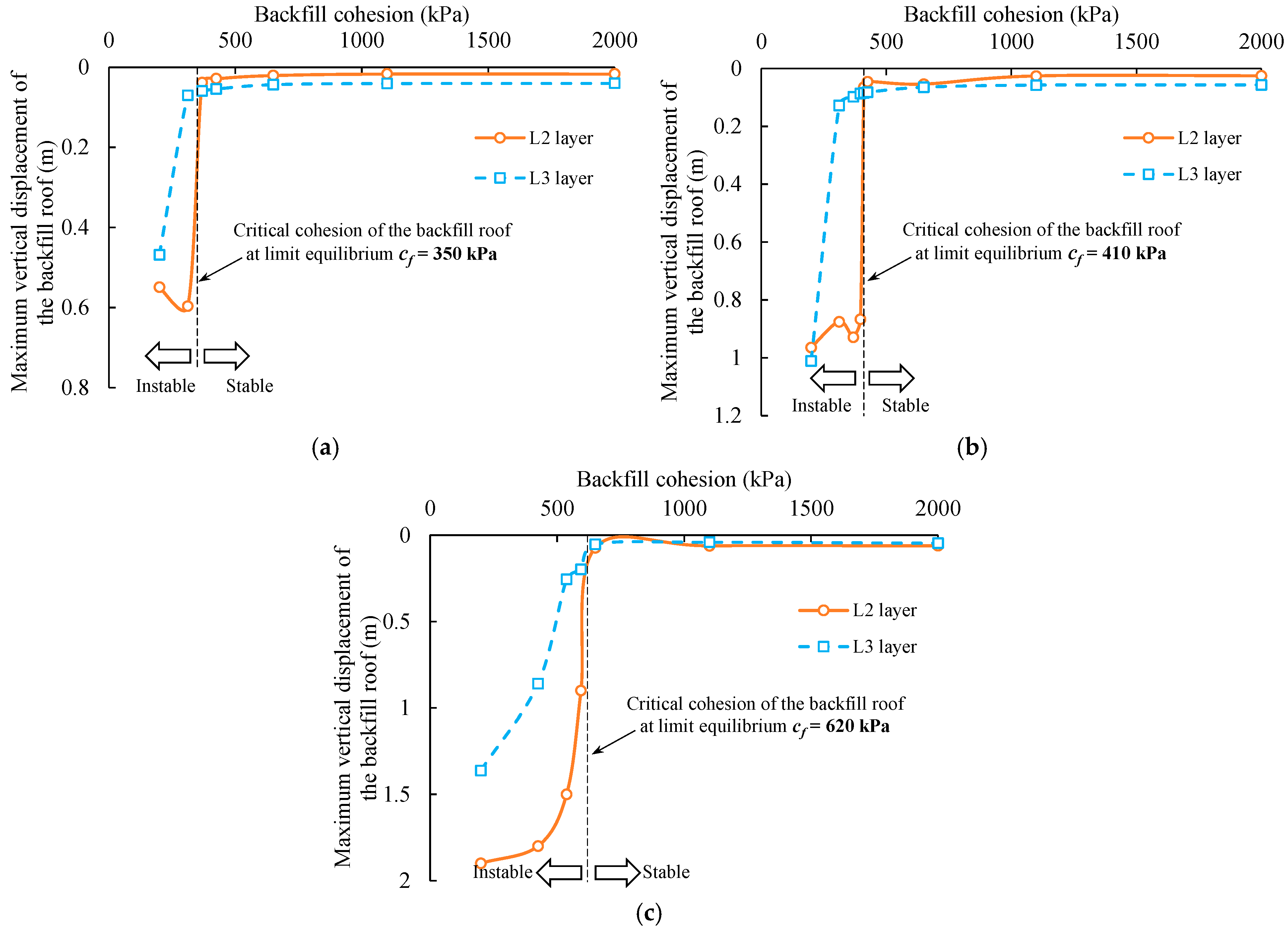
3.2. Strength Requirements of the Backfill Roof with the Basket Weave Drift Layout
4. Discussion
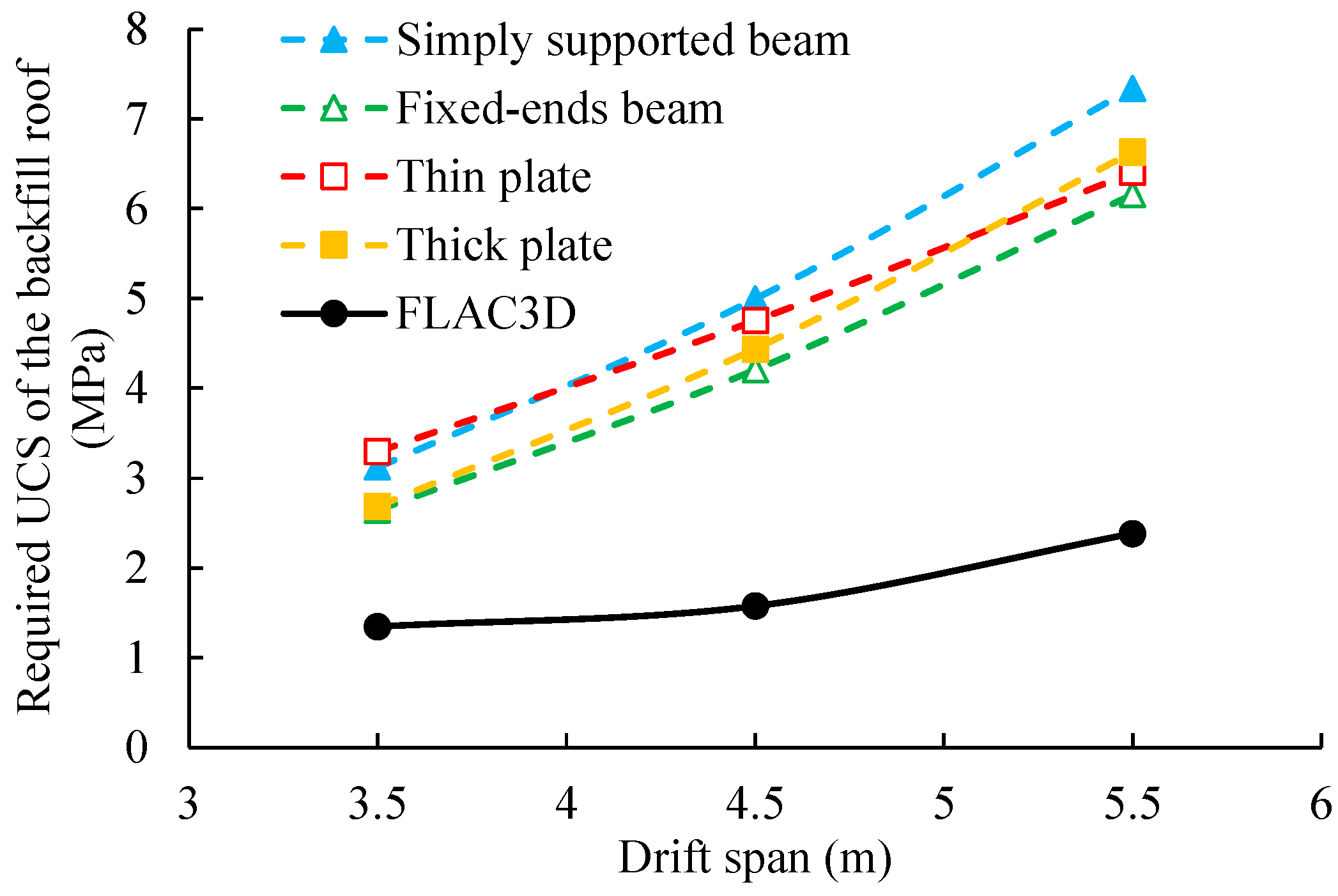
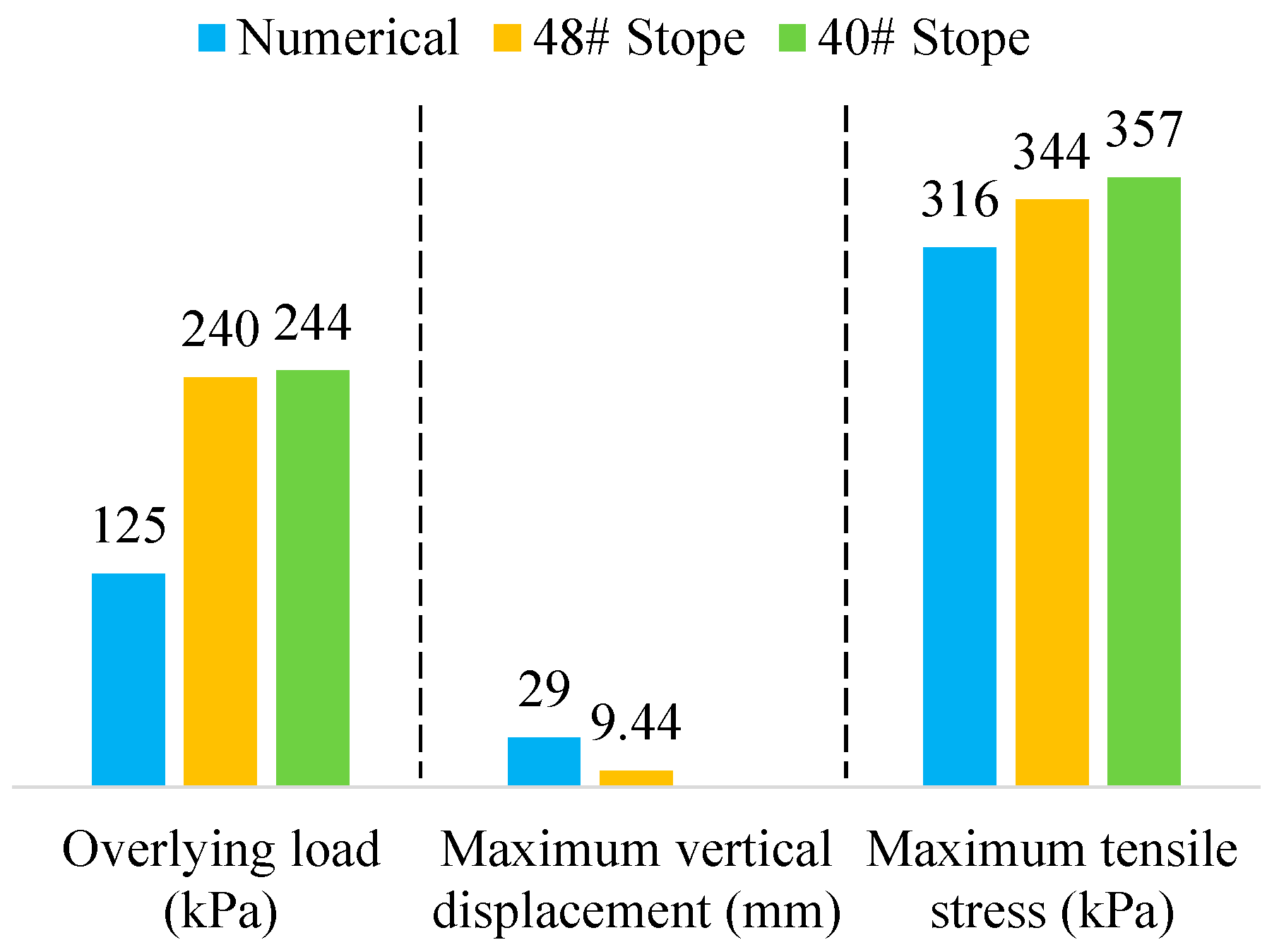
4.1. Comparison of the Numerical Results with Analytical Solutions
4.2. Limitations
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Darling, P. SME Mining Engineering Handbook, 3rd ed.; Society for Mining, Metallurgy, and Exploration, Inc.: Denver, CO, USA, 2011. [Google Scholar]
- Hustrulid, W.A.; Bullock, R.L. Underground Mining Methods: Engineering Fundamentals and International Case Studies; Society for Mining, Metallurgy, and Exploration, Inc.: Littleton, CO, USA, 2001. [Google Scholar]
- Kumar, D.; Singh, U.K.; Singh, G.S.P. Laboratory characterization of cemented rock fill for underhand cut and fill method of mining. J. Inst. Eng. India Ser. D 2016, 97, 193–203. [Google Scholar] [CrossRef]
- Wang, X.; Guo, P.; Huang, W.; Chen, Z.; Chen, Q.; Zhao, K. Stability calculation of backfill roof in downward roadway under multifactor influence. Rock Soil Mech. 2022, 43, 3453–3462. [Google Scholar] [CrossRef]
- Wang, J.; Qiao, D.; Li, G.; Liu, Y.; Zhang, X. Strength model and its application of downward drift filling roof based on thick plate theory. J. China Coal Soc. 2022, 48, 28–36. [Google Scholar] [CrossRef]
- Williams, T.J.; Brady, T.M.; Bayer, D.C.; Bren, M.J.; Pakalnis, R.C.; Marjerison, J.A.; Langston, R.B. Underhand cut and fill mining as practiced in three deep hard rock mines in the United States. In Proceedings of the 109th Canadian Institute of Mining Annual General Meeting, Montreal, QC, Canada, 29 April–2 May 2007. [Google Scholar]
- Guo, S.; Fall, M.; Haruna, S. Interface shear behavior of cementing underground mine backfill. Int. J. Geomech. 2020, 20, 04020230. [Google Scholar] [CrossRef]
- Lu, X. Research on Optimization of Stoping Drifts Parameters by Downward Cemented Filling Method in Jinchuan No. 2 Mining Area. Master’s Thesis, Wuhan University of Science and Technology, Wuhan, China, 2022. [Google Scholar]
- Sun, C.; Luo, L.; Liang, Y. Application of downward horizontal layered drift cementing andfilling mining method in Maoping lead zine mine. China Min. Mag. 2023, 32, 382–385. [Google Scholar]
- Li, M.; Mo, J.; Zhou, C. Stability analysis of full tailing paste filling roof in Wushan Copper Mine. Copp. Eng. 2023, 182, 126–130. [Google Scholar]
- Liu, G.; Li, L.; Yang, X.; Guo, L. Required strength estimation of a cemented backfill with the front wall exposed and back wall pressured. Int. J. Min. Miner. Eng. 2018, 9, 1–20. [Google Scholar] [CrossRef]
- Wang, R.; Zeng, F.; Li, L. Stability analyses of side-exposed backfill considering mine depth and extraction of adjacent stope. Int. J. Rock Mech. Min. Sci. 2021, 142, 104735. [Google Scholar] [CrossRef]
- Ma, Q.; Liu, G.; Yang, X.; Guo, L.; Andy, F. Stability of base-exposed backfill roof considering interfaces between adjacent drifts in underhand drift-and-fill mining. J. Rock Mech. Geotech. Eng. 2025. accepted. [Google Scholar]
- Fan, C.; Li, L.; Liu, G.; Yang, X.; Guo, L.; Tang, J. Analytical solution for estimating the minimum required strength of sill mat by considering failure due to rock wall closure. J. Rock Mech. Geotech. Eng. 2024, 17, 996–1007. [Google Scholar] [CrossRef]
- Zhao, K.; Liang, N.; Zeng, P.; Wang, W.; Gong, C.; Xiong, L.; Liu, H. Research on optimization and numerical simulation of layout scheme of mining approach in downward slicing and filling method. Appl. Sci. 2023, 13, 8688. [Google Scholar] [CrossRef]
- Gao, T.; Sun, W.; Liu, Z.; Cheng, H. Investigation on fracture characteristics and failure pattern of inclined layered cemented tailings backfill. Constr. Build. Mater. 2022, 343, 128110. [Google Scholar] [CrossRef]
- Zhu, B.; Gao, T.; Li, Y.; Ge, C.; Zhang, B.; Sun, W. Study on stability of inclined layered cemented backfill in downward drift. Min. Res. Dev. 2024, 44, 1–12. [Google Scholar]
- Itasca. FLAC3D-Fast Lagrangian Analysis of Continua, User’s Guide; Itasca Consulting Group: Minneapolis, MN, USA, 2019. [Google Scholar]
- Liu, G.; Li, L.; Yang, X.; Guo, L. Stability analyses of vertically exposed cemented backfill: A revisit to Mitchell’s physical model tests. Int. J. Min. Sci. Technol. 2016, 26, 1135–1144. [Google Scholar] [CrossRef]
- Guo, L.; Peng, X.; Zhao, Y.; Liu, G.; Tang, G.; Pan, A. Experimental study on direct tensile properties of cemented paste backfill. Front. Mater. 2022, 9, 864264. [Google Scholar] [CrossRef]
- Grabinsky, M.W.F.; Jafari, M.A.; Pan, A.N. Cemented paste backfill (CPB) material properties for undercut analysis. Mining 2022, 2, 103–122. [Google Scholar] [CrossRef]
- Talibe Keita, A.M.; Jahanbakhshzadeh, A.; Li, L. Numerical analysis of the stability of arched sill mats made of cemented backfill. Int. J. Rock Mech. Min. Sci. 2021, 140, 104667. [Google Scholar] [CrossRef]
- Pagé, P.; Li, L.; Yang, P.; Simon, R. Numerical investigation of the stability of a base-exposed sill mat made of cemented backfill. Int. J. Rock Mech. Min. Sci. 2019, 114, 195–207. [Google Scholar] [CrossRef]
- Xing, C.; Gao, M.; Qiu, Y.; Yu, L.; Zhang, Y.; Xue, C.; Peng, K. Study of deep-mine ore rock failure mechanism using downward approachfilling mining method by numerical simulation. Gold 2024, 45, 58–63. [Google Scholar]
- Wang, K.; Liu, L.; He, J.; Li, X.; Ran, J. Numerical simulation of approach rock stability under interlocking vertical mining of adjacent layered approaches. Min. Metall. 2024, 33, 503–507. [Google Scholar]
- Caceres, C.A. Effect of Delayed Backfill on Open Stope Mining Methods. Master’s Thesis, The University of British Columbia, Vancouver, BC, Canada, 2005. [Google Scholar]
- Lin, H.; Yang, Y.; Chen, C.; Ding, C. Influence of mining sequence of branch on stope pressure behaviour on continuous mining and continuous backfilling. Processes 2024, 12, 442. [Google Scholar] [CrossRef]
- Caceres, C.; Moffat, R.; Pakalnis, R. Evaluation of flexural failure of sill mats using classical beam theory and numerical models. Int. J. Rock Mech. Min. Sci. 2017, 99, 21–27. [Google Scholar] [CrossRef]
- Han, B. Reliability Analysis of Filling Body in Jinchuan No.2 Mining Area and Optimization of Mining Ground Pressure Control in 1 # Ore Body. Ph.D. Thesis, Central South University, Changsha, China, 2004. [Google Scholar]
- Mitchell, R.J. Sill mat evaluation using centrifuge models. Int. J. Min. Sci. Technol. 1991, 13, 301–313. [Google Scholar] [CrossRef]
- Huang, Y.; Sun, H.; Liu, W. Discussion on the mechanics model of underhand drift-and-fill mining. Nonferrous Met. 1999, 51, 1–4. [Google Scholar]
- Hu, Y.; Han, B.; Zhang, B.; Li, K. Force analysis and strength determination of the cemented paste backfill roof in underhand drift cut-and-fill stopping. Appl. Sci. 2023, 13, 855. [Google Scholar] [CrossRef]
- Fan, C.; Li, L.; Yang, X.; Liu, G.; Guo, L.; Tang, J. Analytical solution for determining wall closure associated with stope excavation underneath sill mat constructed by cemented backfill. J. Rock Mech. Geotech. Eng. 2024, 17, 983–995. [Google Scholar] [CrossRef]
- Dong, L.; Zhang, Y.; Chen, Z.; Kou, Y.; Pei, Z. Dynamic stress characterization and instability risk identification using multisource acoustic signals in cut-and-fill stopes. Sci. Rep. 2024, 14, 16499. [Google Scholar] [CrossRef]
- Hao, J.; Chen, A.; Li, X.; Bian, H.; Shi, Y.; Wang, X.; Zhao, J.; Liu, H. A case study of pillar extraction techniques based on strip-filling and second-mining method. Front. Earth Sci. 2022, 10, 1051245. [Google Scholar] [CrossRef]
- Chen, S.; Jin, A.; Zhao, Y.; Li, H.; Wang, J. Mechanical properties and deformation mechanism of stratified cemented tailings backfill under unconfined compression. Constr. Build. Mater. 2022, 335, 127205. [Google Scholar] [CrossRef]

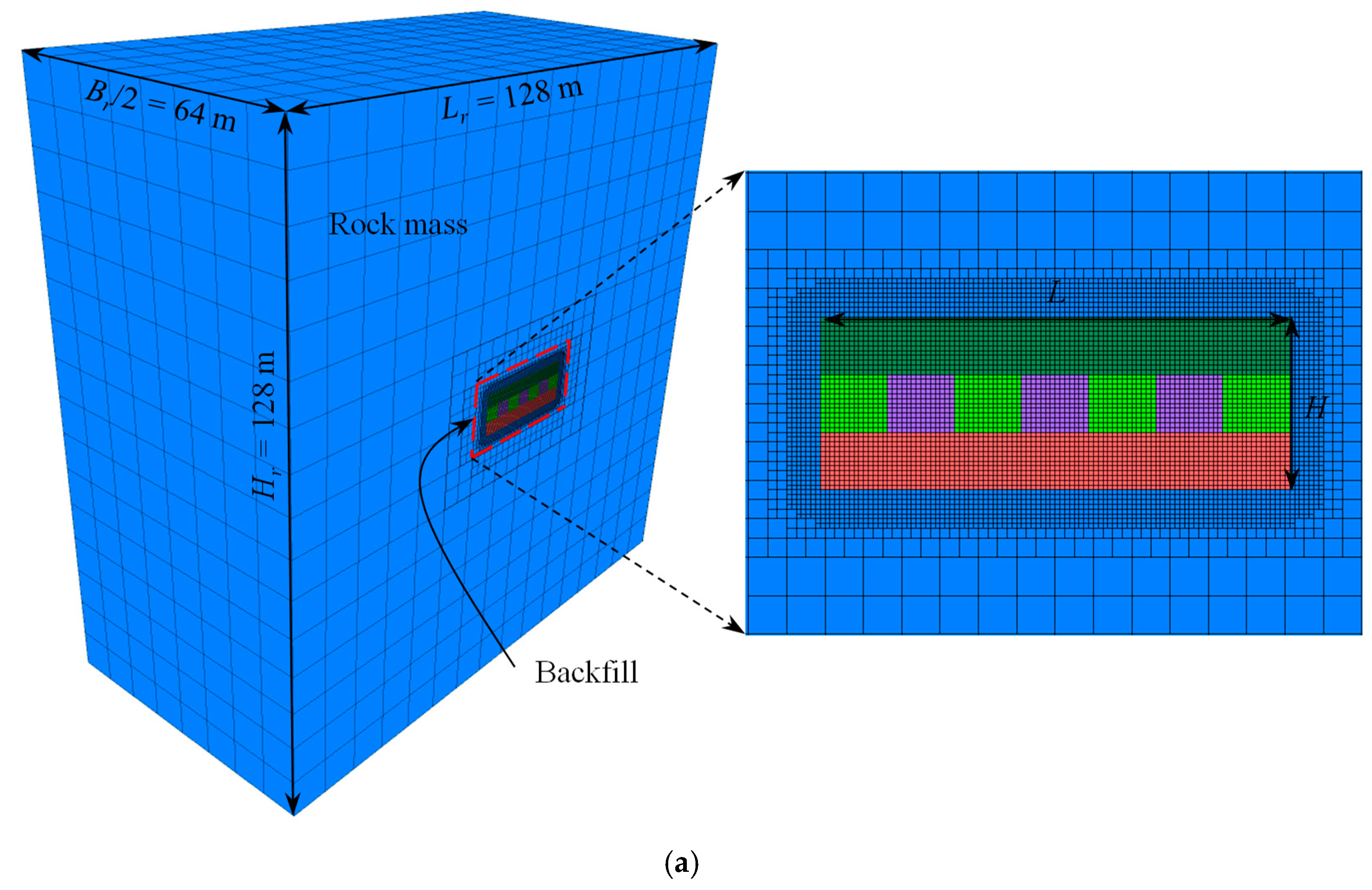
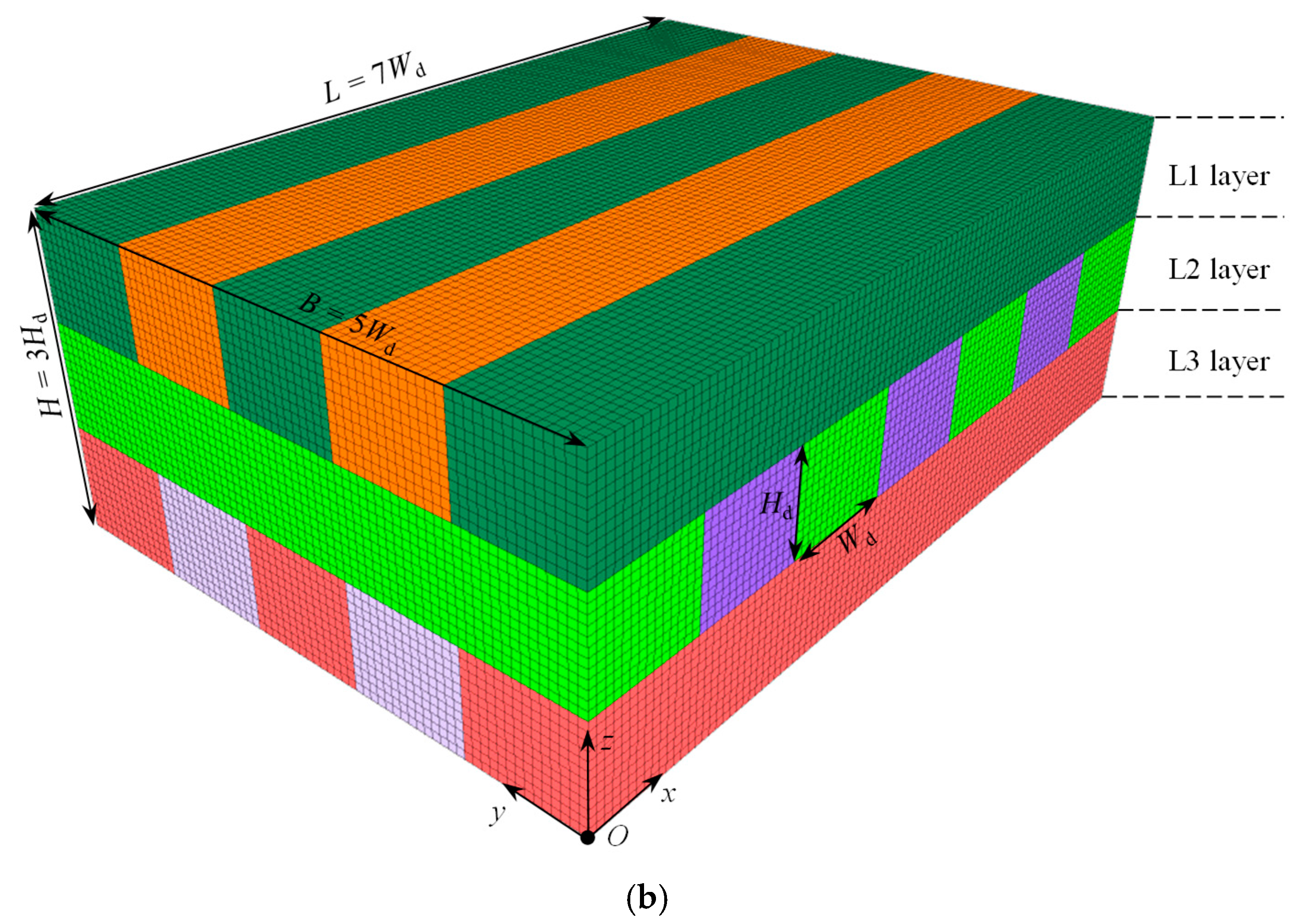
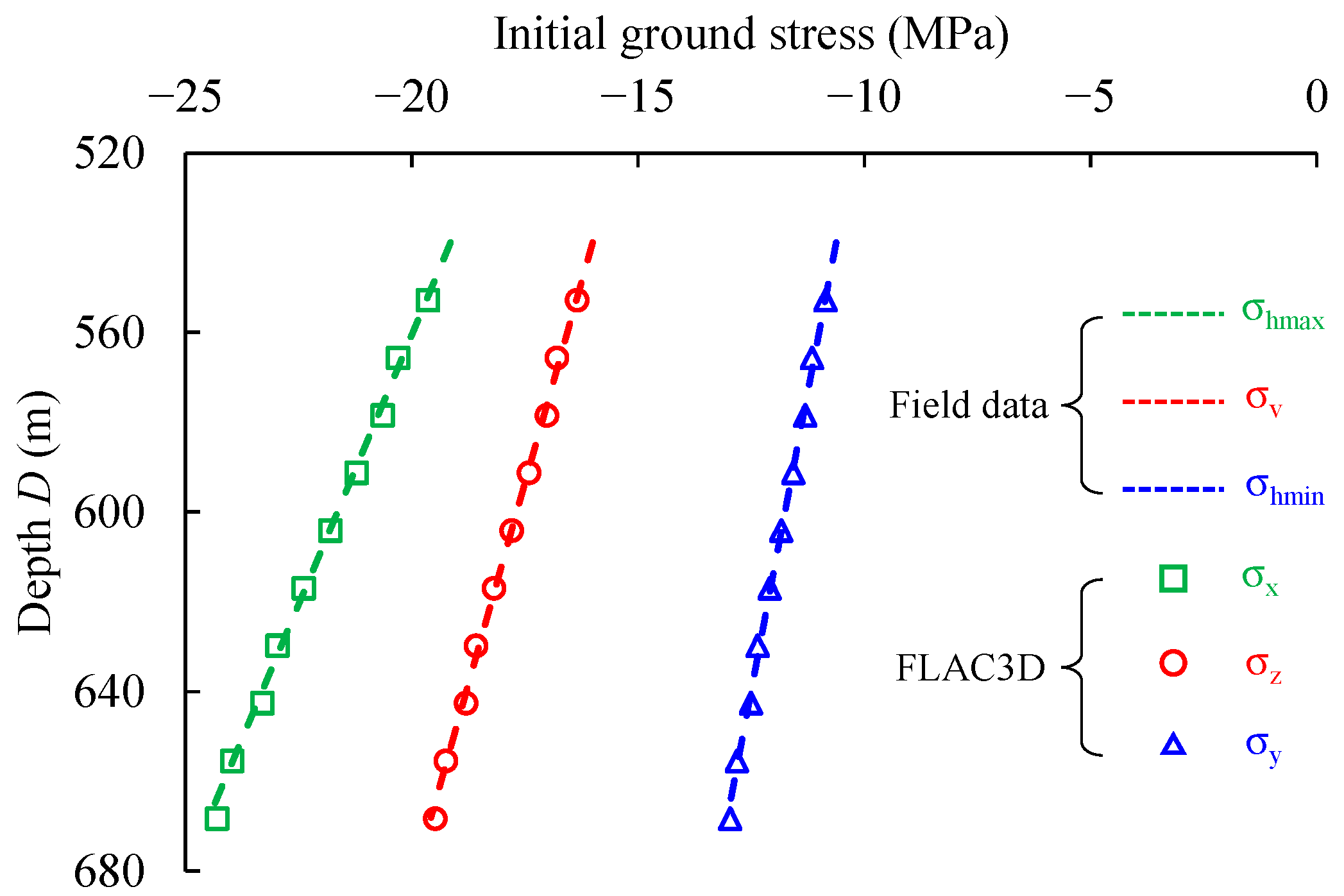
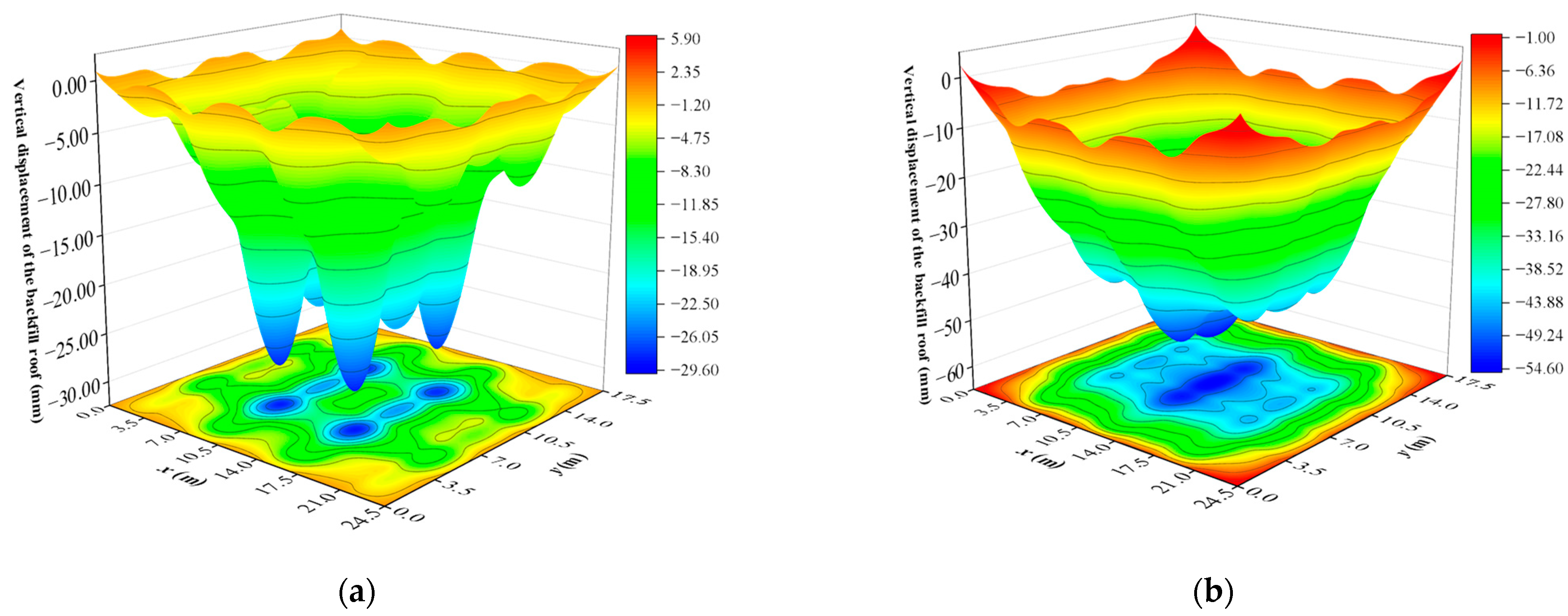
| Material | Density (kg⋅m−3) | Posson’s Ratio | Friction Angle (°) | Cohesion (MPa) | Young’s Modulus (GPa) | Tensile Strength (MPa) |
|---|---|---|---|---|---|---|
| Rock mass | 2800 | 0.3 | 35 | 1.98 | 10 | 1.25 |
| Backfill | 1780 | 0.27 | 33 | / | 0.315 | / |
| No. | Backfill Cohesion (kPa) | Roof Stability | Backfill Cohesion for Next (kPa) | Note |
|---|---|---|---|---|
| 1 | 200 | unstable | 2000 | confirm the lower bound |
| 2 | 2000 | stable | 1100 = (200 + 2000) ÷ 2 | confirm the upper bound |
| 3 | 1100 | stable | 650 = (200 + 1100) ÷ 2 | current range [200, 1100] |
| 4 | 650 | stable | 425 = (200 + 650) ÷ 2 | current range [200, 650] |
| 5 | 425 | stable | 312.5 = (200 + 425) ÷ 2 | current range [200, 425] |
| 6 | 312.5 | unstable | 368.75 = (312.5 + 425) ÷ 2 | current range [312.5, 425] |
| 7 | 368.75 | stable | 340.625 = (312.5 + 368.75) ÷ 2 | current range [312.5, 368.75] |
| 8 | 340.625 | stable | … |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ma, Q.; Liu, G.; Yang, X.; Song, W. Stability Analysis of the Backfill Roof with Basket Weave Drift Layout in Underhand Drift-and-Fill Mining. Appl. Sci. 2025, 15, 4487. https://doi.org/10.3390/app15084487
Ma Q, Liu G, Yang X, Song W. Stability Analysis of the Backfill Roof with Basket Weave Drift Layout in Underhand Drift-and-Fill Mining. Applied Sciences. 2025; 15(8):4487. https://doi.org/10.3390/app15084487
Chicago/Turabian StyleMa, Qinghai, Guangsheng Liu, Xiaocong Yang, and Weidong Song. 2025. "Stability Analysis of the Backfill Roof with Basket Weave Drift Layout in Underhand Drift-and-Fill Mining" Applied Sciences 15, no. 8: 4487. https://doi.org/10.3390/app15084487
APA StyleMa, Q., Liu, G., Yang, X., & Song, W. (2025). Stability Analysis of the Backfill Roof with Basket Weave Drift Layout in Underhand Drift-and-Fill Mining. Applied Sciences, 15(8), 4487. https://doi.org/10.3390/app15084487






