A Novel Earth-System Spatial Grid Model: ISEA4H-ESSG for Multi-Layer Geoscience Data Integration and Analysis
Abstract
1. Introduction
1.1. Background and Motivation
1.2. Current Status of Multi-Layer Observational Data in the Earth System
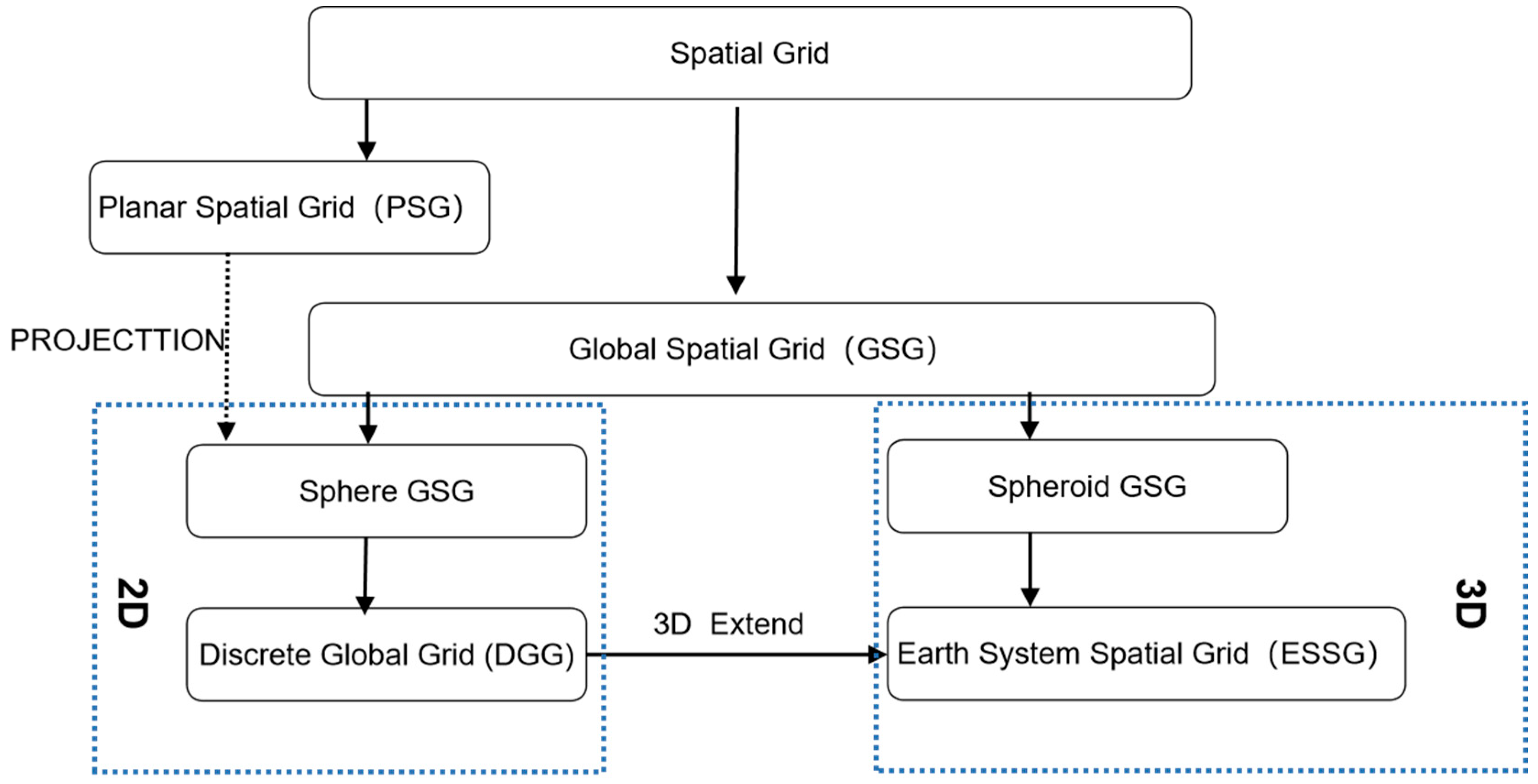
1.3. Current Research Status of Earth Spatial Grid
- A.
- Latitude–Longitude Grids
- B.
- Regular Polyhedron-Based Grids
- Latitude–longitude spheroidal grids: Extend 2D grids radially but suffer from polar distortion [24].
- Cubed-sphere grids: Use cubic projections for uniform coverage, suited for atmospheric modeling [25].
- Yin–Yang Grid: Designed for mantle convection with overlapping domains [26].
- Adaptive Mesh Refinement (AMR): Dynamically adjusts resolution for localized phenomena [27].
- Sphere Degenerated Octree Grid (SDOG): Employs octree subdivision for 3D Earth modeling but lacks flexible layering [28].
2. Materials and Methods
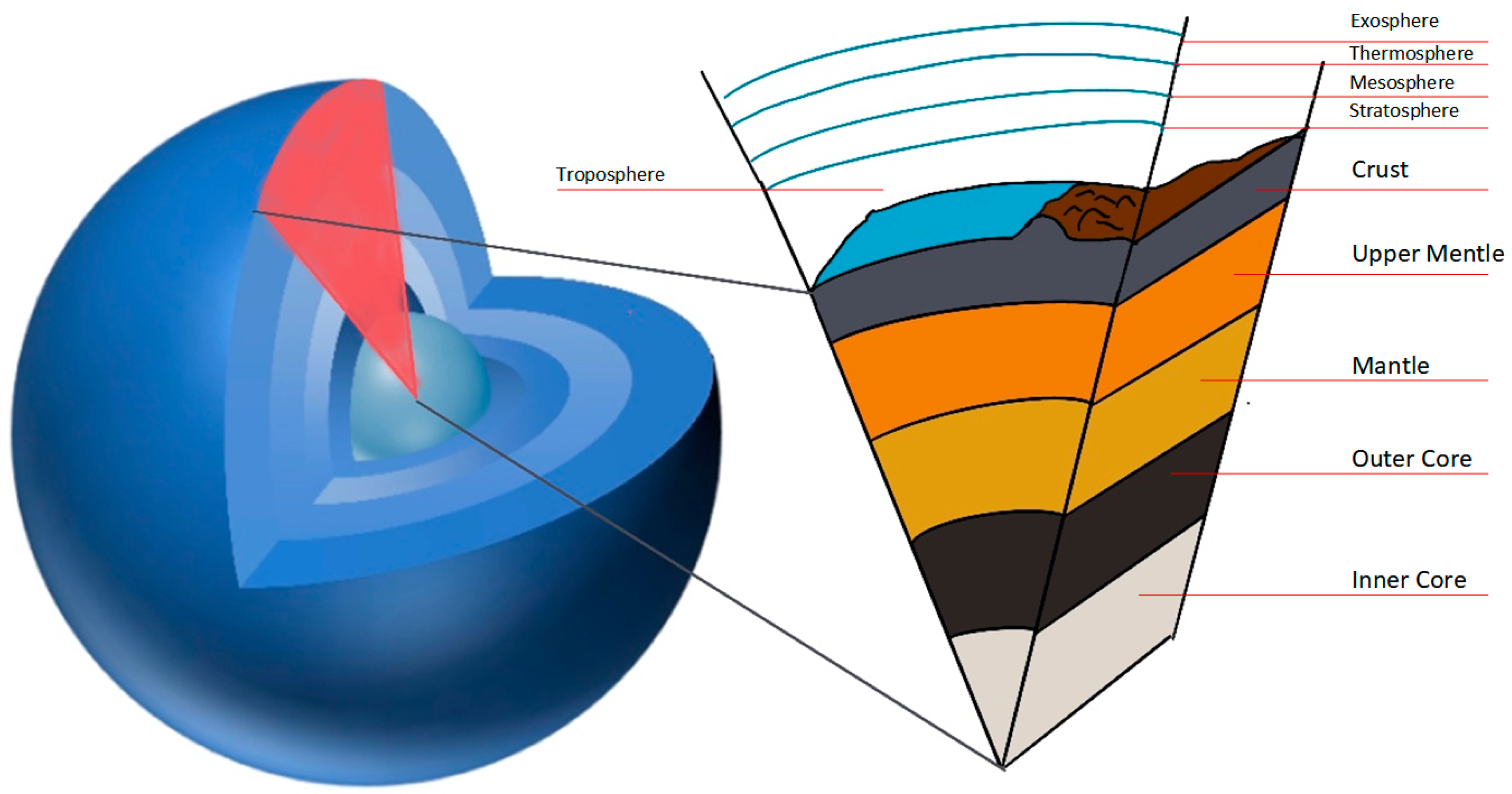
2.1. Requirements for the Earth-System Spatial Grid
2.1.1. Definition of the Earth-System Spatiotemporal Grid
2.1.2. Principles and Requirements for the Earth-System Spatial Grid
- (1)
- Stratified Spherical Coverage Criterion
- (2)
- Geographic Consistency Criterion
- (3)
- Multi-Scale Dynamic Adaptability Criterion
- (4)
- Global Seamless Partitioning Criterion
- (5)
- Encoding Uniqueness and Efficiency Criterion
- (6)
- Data Fusion and Multi-Source Compatibility Criterion
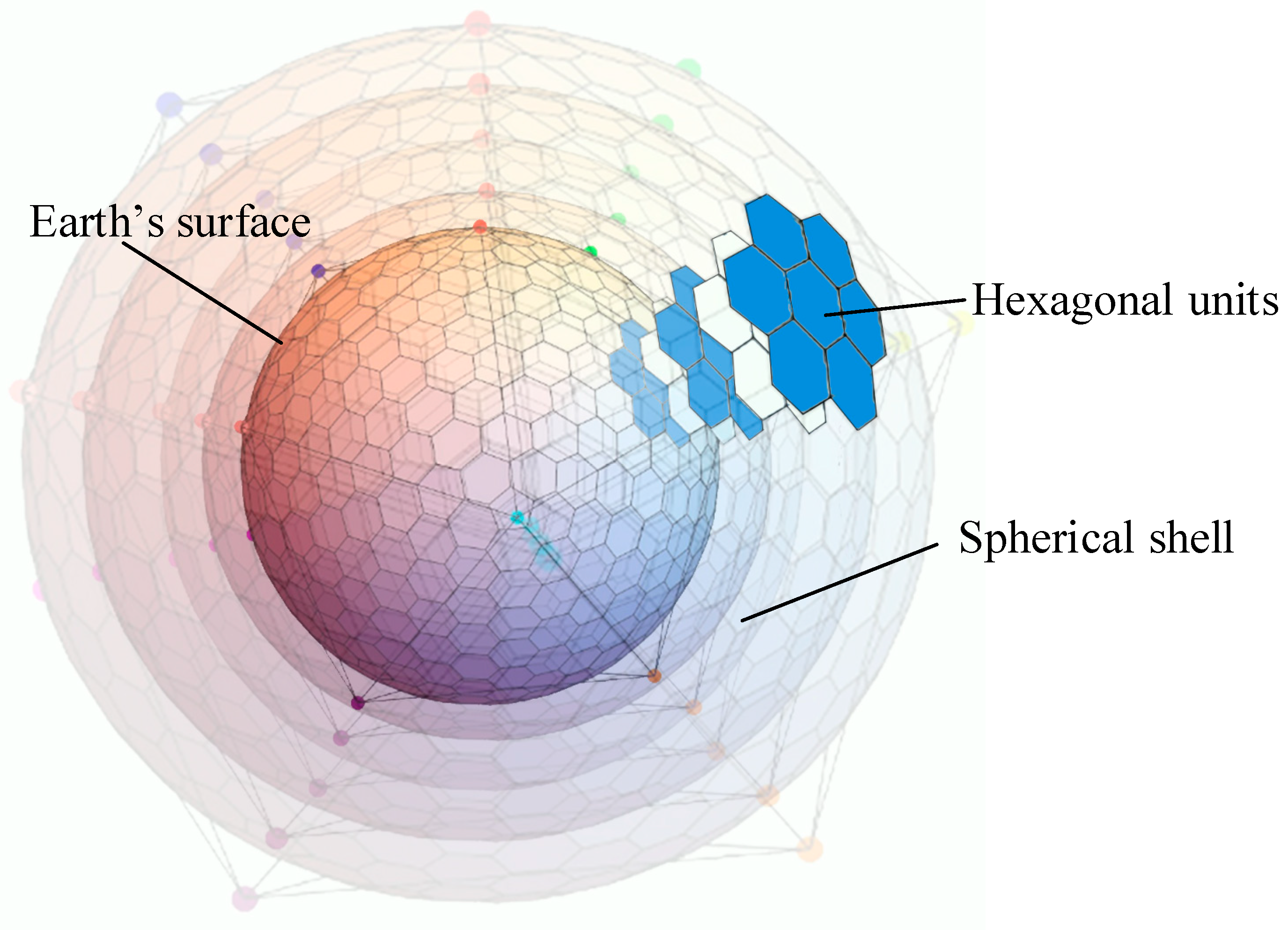
2.2. ISEA4H Subdivision Model of the Temporal and Spatial Grid of the Earth System’s Layers
2.2.1. Basic Concept
2.2.2. Design Concept of the ISEA4H-ESSG Subdivision Model of the Temporal and Spatial Grid of the Earth System’s Layers
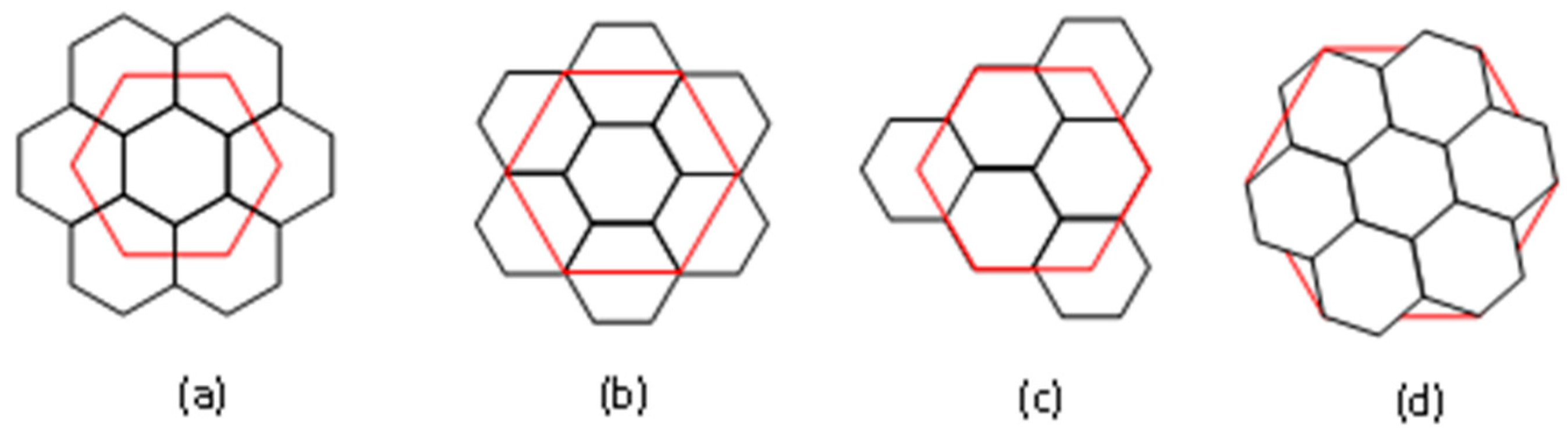
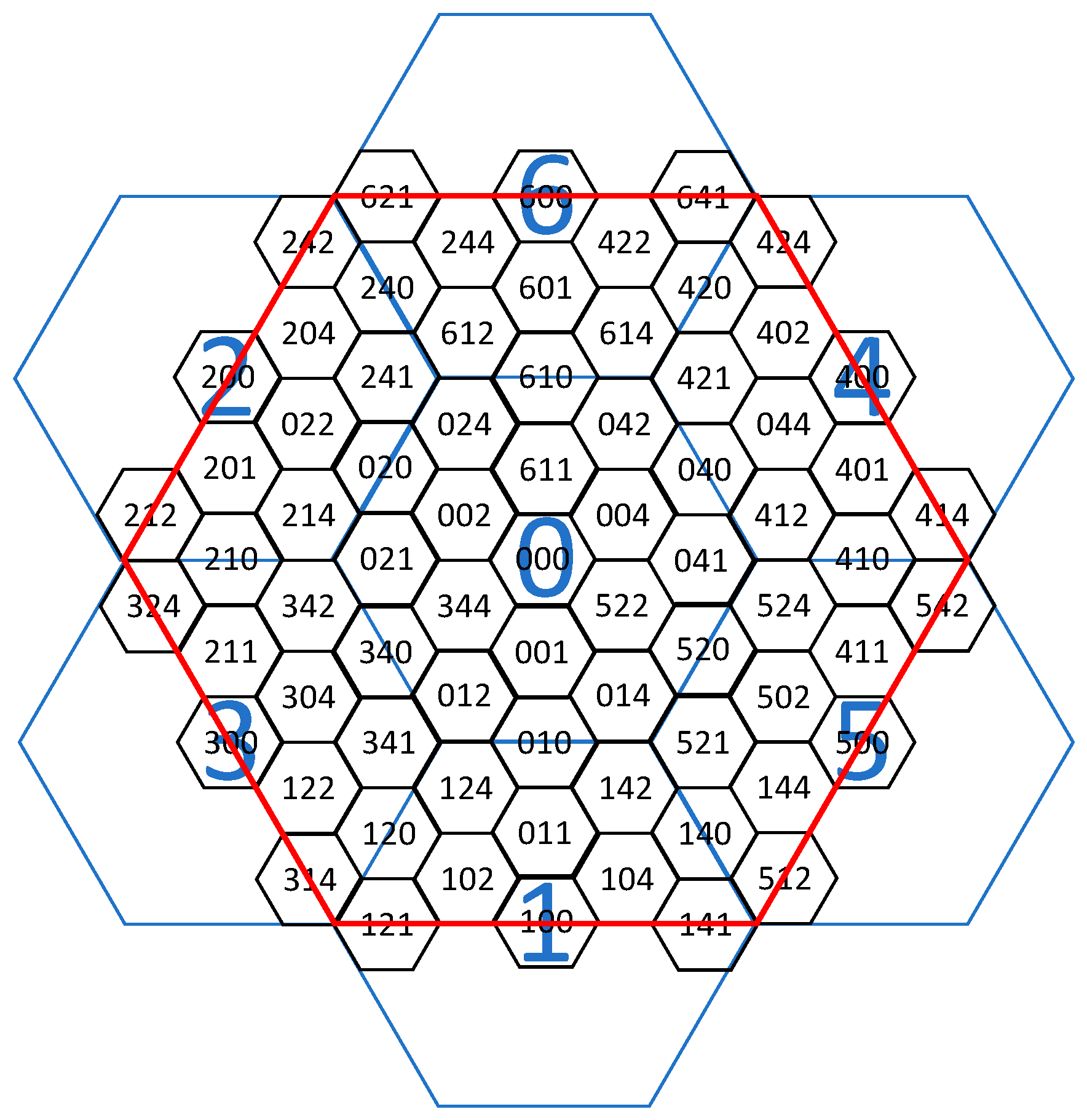
2.2.3. Subdivision Mechanism of the ISEA4H-ESSG Temporal and Spatial Grid of the Earth System’s Layers
- (1)
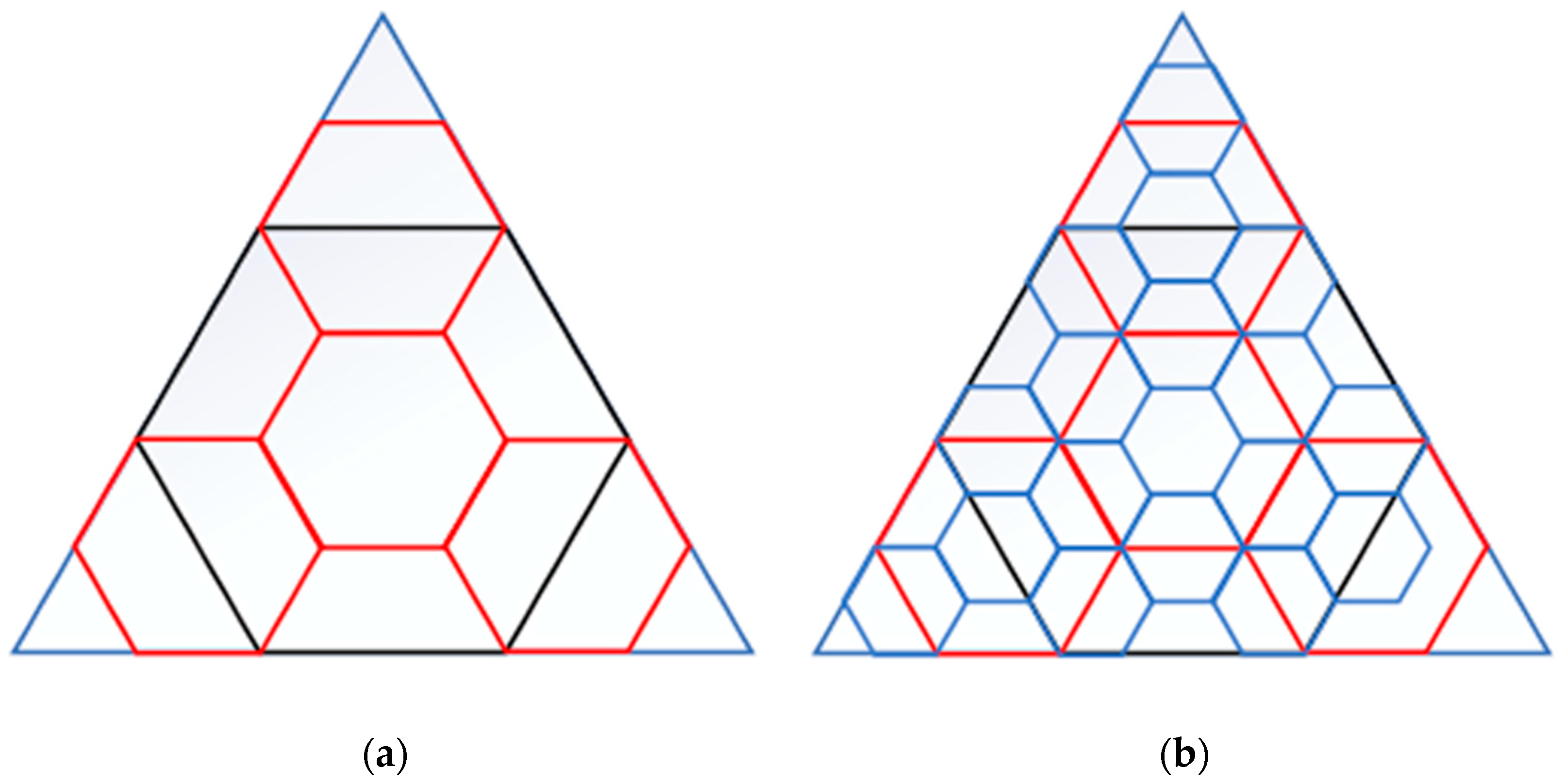
- Subdivision of the Four-Aperture Hexagonal Global Discrete Grid
- (2)
- Degenerate Subdivision
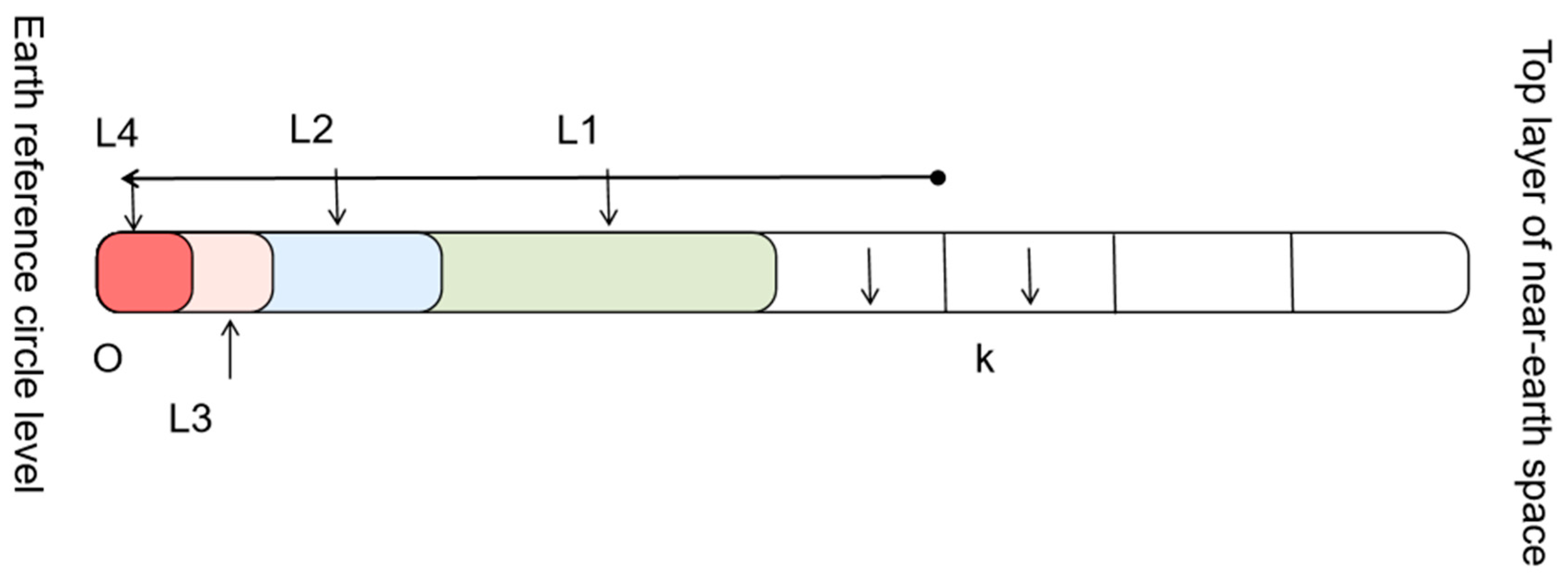
2.2.4. Subdivision Model of the Layer Surface of the ISEA4H-ESSG Temporal and Spatial Grid of the Earth System’s Layers
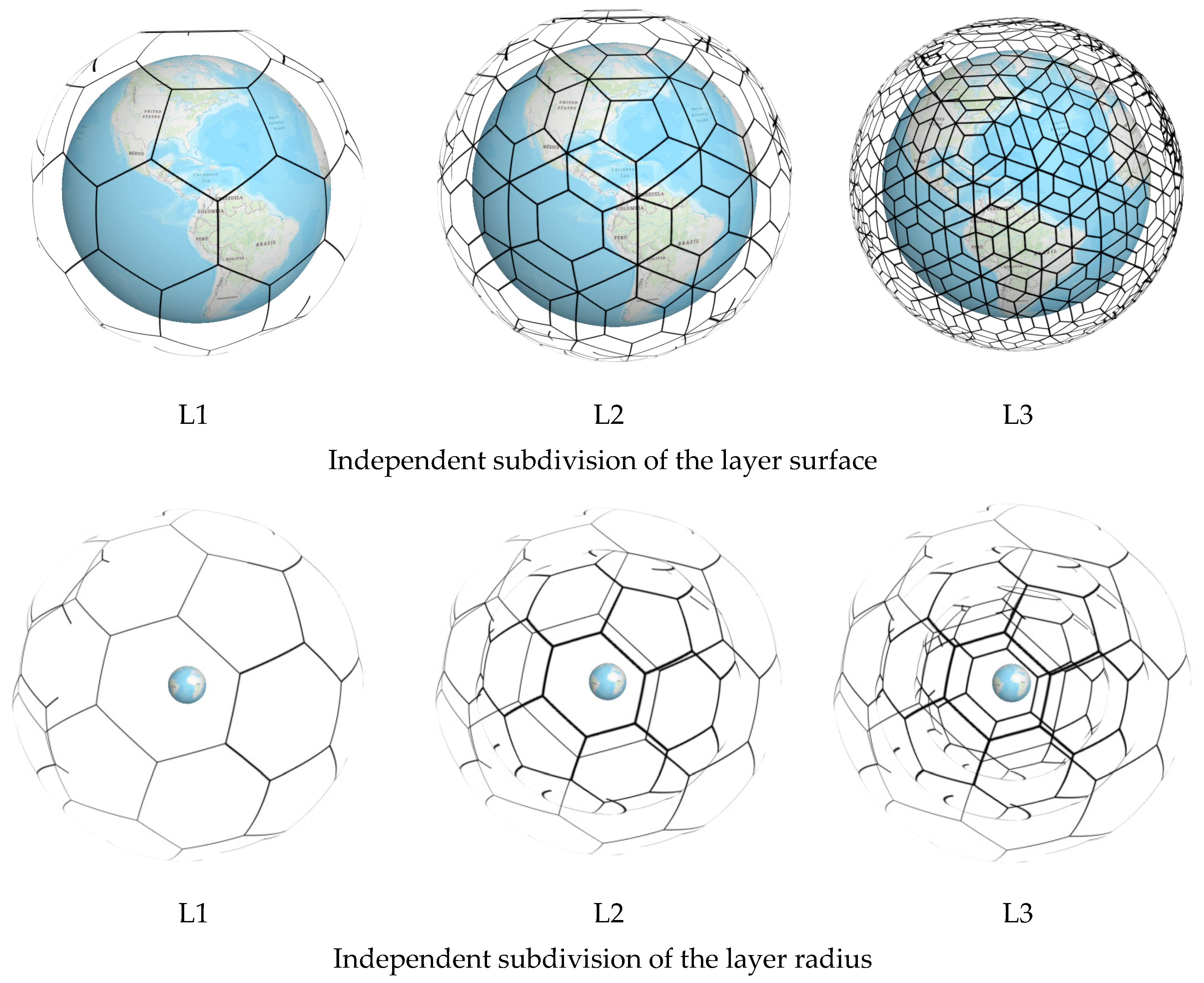
2.2.5. The ISEA4H-ESSG Layered Block Subdivision Model of the Earth-System Spatiotemporal Grid
2.3. Encoding of the Temporal and Spatial Grid of the Earth System’s Layers
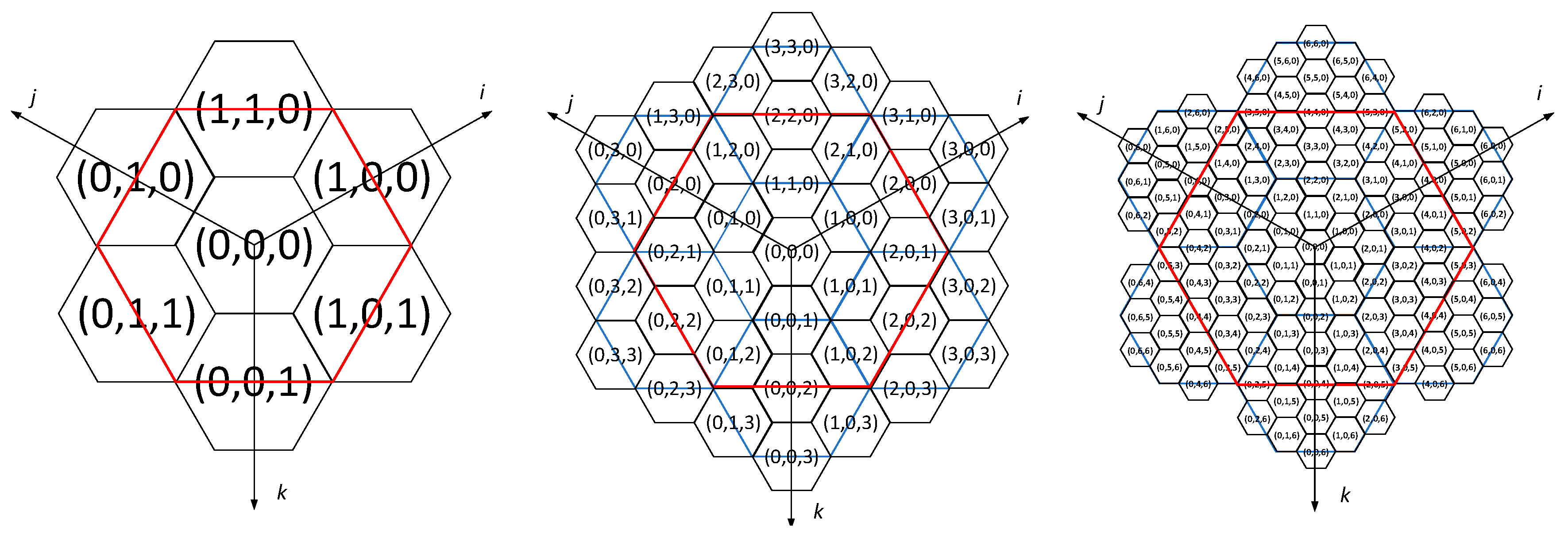
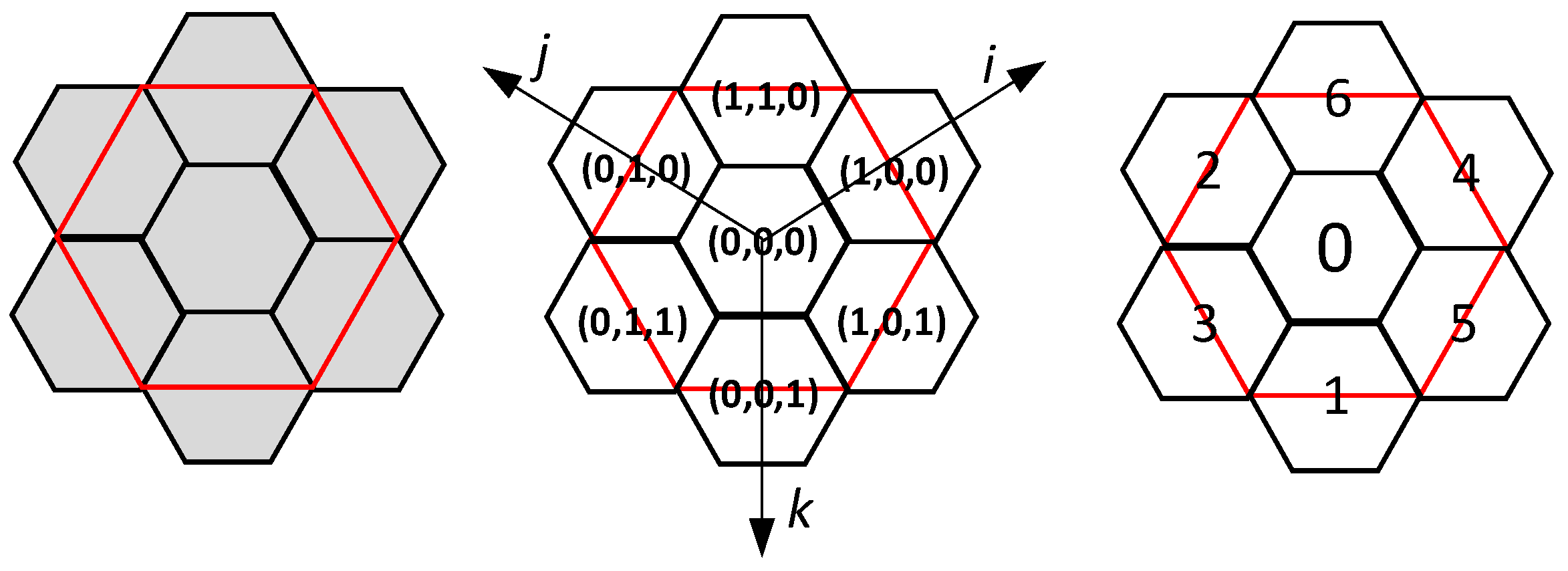
2.3.1. ISEA4H-ESSG Encoding Structure
- Encoding of the Layer Surface
- 2.
- Encoding of the Layer Radius
- 3.
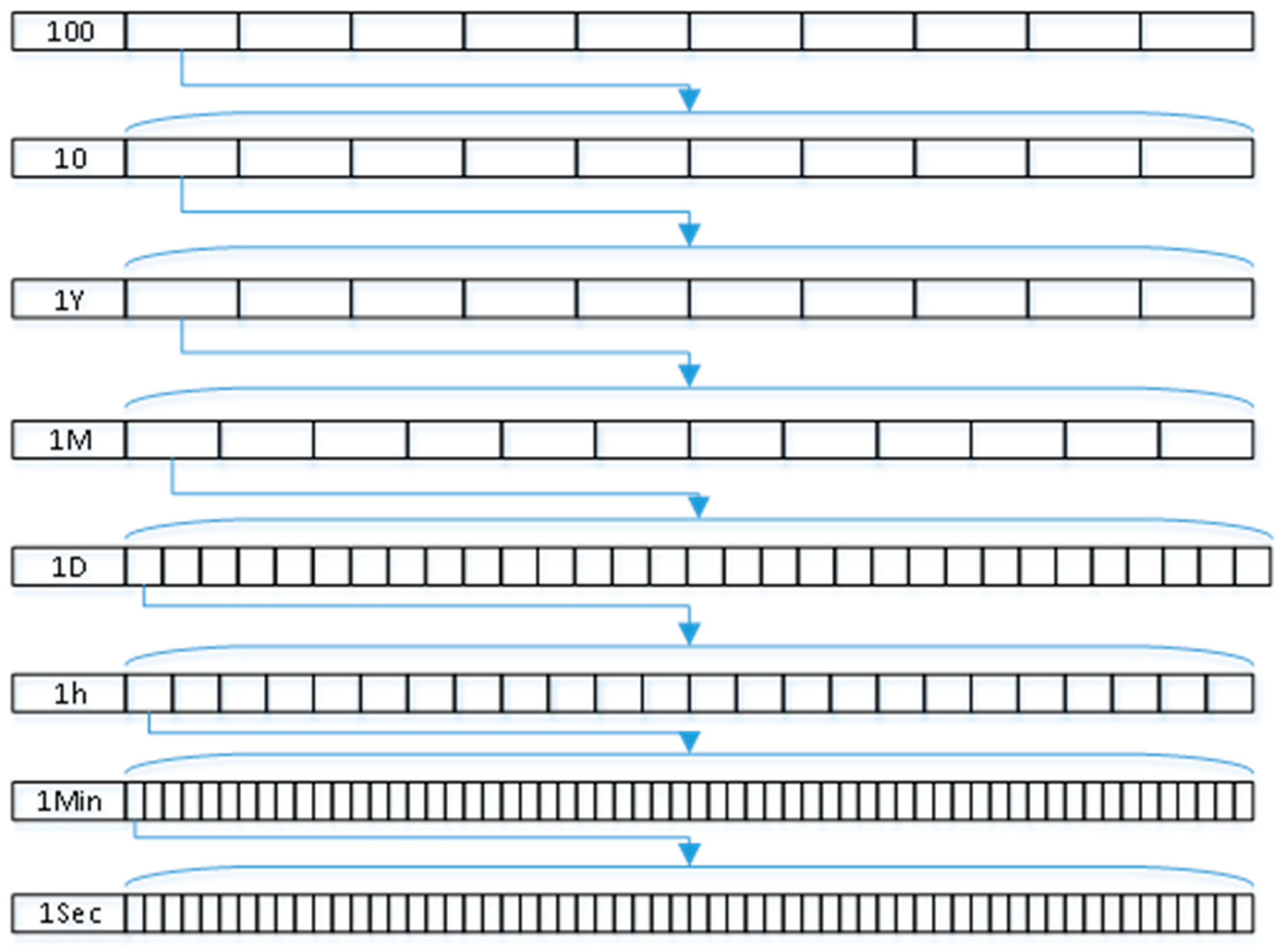
- Temporal Subdivision and Encoding
2.3.2. Encoding Structure
3. Results and Discussion
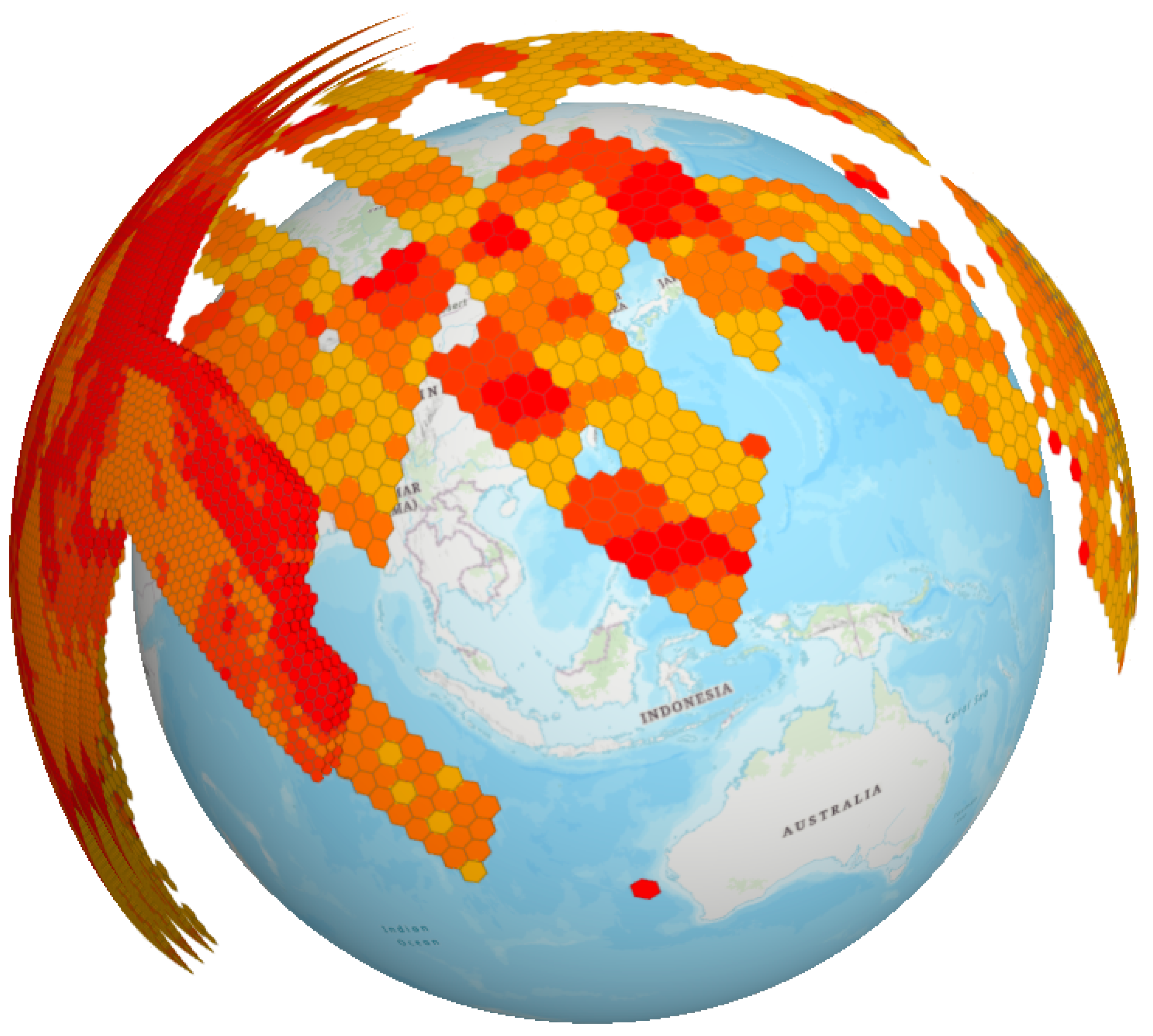
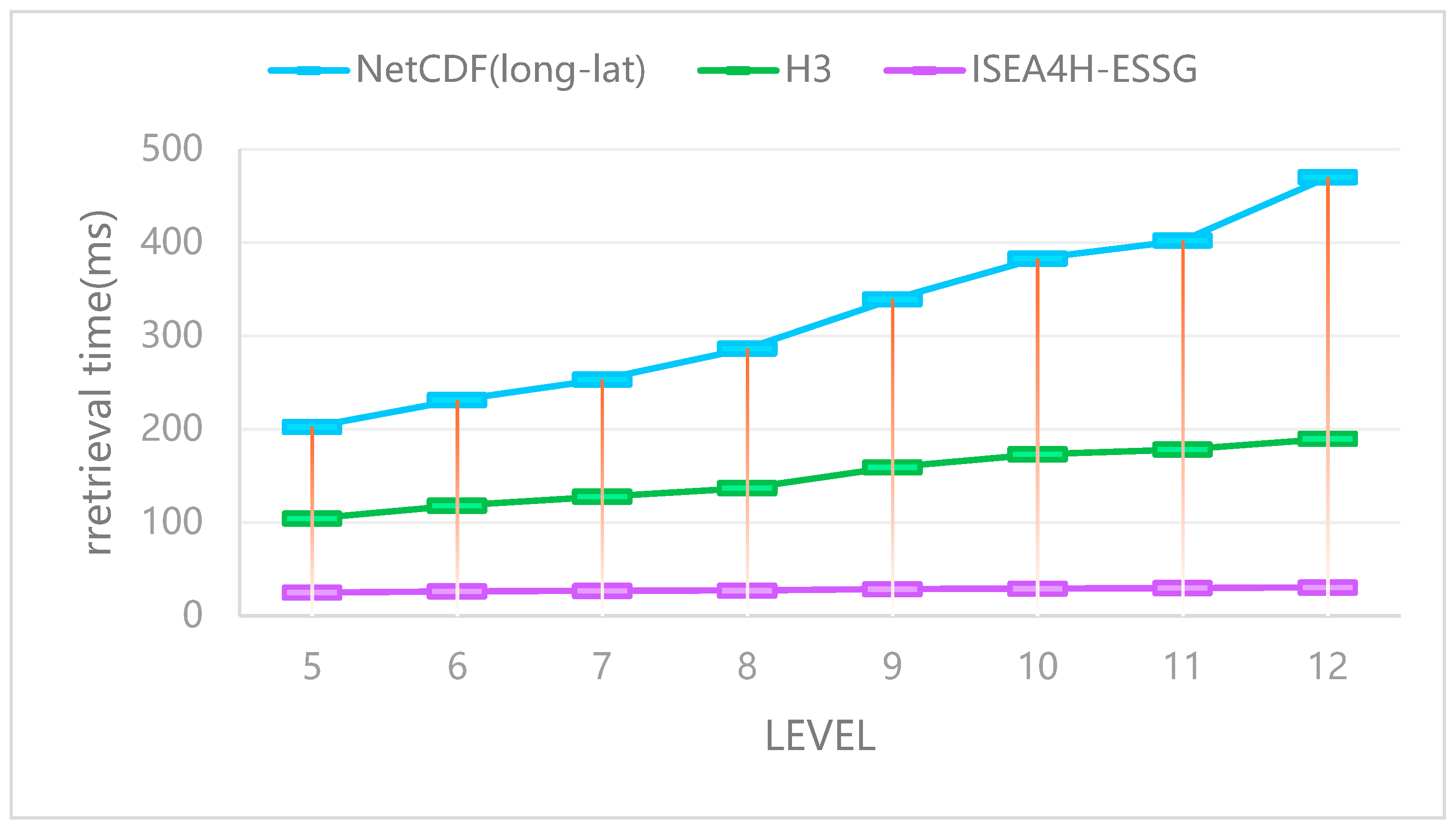
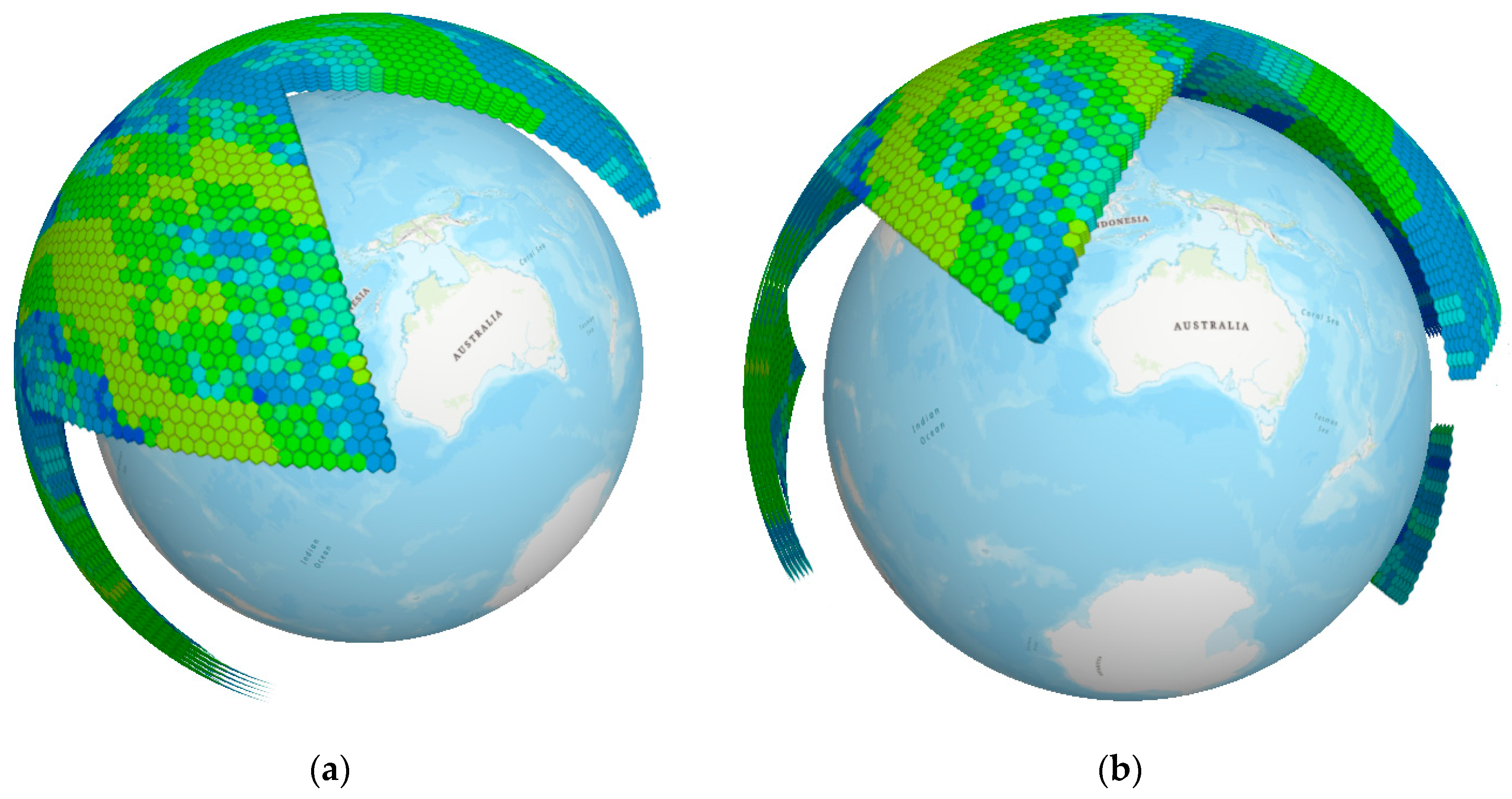
3.1. Three-Dimensional Modeling of the Ionosphere
3.2. Formatting of Mathematical Components
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| ESSG | Earth-System Stratified Grid |
| ISEA4H | Icosahedral Snyder Equal-Area Aperture 4 Hexagon Discrete Global Grid |
| DGGS | Discrete Global Grid Systems |
| NCEP | National Centers for Environmental Prediction |
| GNSS-TEC | Global Navigation Satellite System-Total Electron Content |
| IRI | International Reference Ionosphere |
| COSPAR | Committee on Space Research |
| URSI | International Union of Radio Science |
| NASA | National Aeronautics and Space Administration |
| GRIB | GRIdded Binary |
| SDOG | Sphere Degenerated Octree Grid |
| AMR | Adaptive Mesh Refinement |
| QTM | Quaternary Triangular Mesh |
| SQT | Sphere Quad Tree |
| H3 | Uber’s open-source hexagonal grid system |
| rHEALPix | Refined Hierarchical Equal-Area iso-Latitude Pixelization |
| OHQS | Optimized Hexagonal Quadtree Structure |
| GTOPO30 | Global Topography at 30 arc-second resolution |
| ETOPO5 | Earth Topography at 5 arc-minute resolution |
| GTED | Global Terrain Elevation Data |
References
- Goodchild, M.F. Geographic information systems. In Research Methods in Geography; Gomez, B., Jones, J.P., III, Eds.; Wiley-Blackwell: Chichester, UK, 2010; pp. 376–391. [Google Scholar]
- Pulinets, S.; Ouzounov, D. Lithosphere–Atmosphere–Ionosphere Coupling (LAIC) model–An unified concept for earthquake precursors validation. J. Asian Earth Sci. 2011, 41, 371–382. [Google Scholar]
- Goodchild, M.F.; Guo, H.; Annoni, A.; Bian, L.; de Bie, K.; Campbell, F.; Craglia, M.; Ehlers, M.; van Genderen, J.; Jackson, D.; et al. Next-generation digital earth. Proc. Natl. Acad. Sci. USA 2012, 109, 11088–11094. [Google Scholar]
- Barmin, I.V.; Kulagin, V.P.; Savinykh, V.P.; Tsvetkov, V.Y. Near-Earth space as an object of global monitoring. Sol. Syst. Res. 2014, 48, 531–535. [Google Scholar] [CrossRef]
- Nwankwo, V.U.; Jibiri, N.N.; Kio, M.T. The impact of space radiation environment on satellites operation in near-Earth space. In Satellites Missions and Technologies for Geosciences; IntechOpen: London, UK, 2020; Volume 1, pp. 73–90. [Google Scholar]
- Yu, J.; Wu, L.; Zi, G.; Guo, Z. SDOG-based multi-scale 3D modeling and visualization on global lithosphere. Sci. China Earth Sci. 2012, 55, 1012–1020. [Google Scholar]
- Battrick, B. Global Earth Observation System of Systems GEOSS: 10-Year Implementation Plan Reference Document; Ad-Hoc Group on Earth Observations; Final Draft; ESA Publication Division: Noordwijk, The Netherlands, 2005. [Google Scholar]
- Nativi, S.; Mazzetti, P.; Saarenmaa, H.; Kerr, J.; Tuama, É.Ó. Biodiversity and climate change use scenarios framework for the GEOSS interoperability pilot process. Ecol. Inform. 2009, 4, 23–33. [Google Scholar]
- Bensana, E.; Lemaitre, M.; Verfaillie, G.J.C. Earth observation satellite management. Constraints 1999, 4, 293–299. [Google Scholar]
- Sahr, K.; White, D.; Kimerling, A.J. Discrete Global Grid System. Cartogr. Geogr. Inf. Sci. 2003, 30, 121–134. [Google Scholar]
- Yao, X.; Li, G.; Xia, J.; Ben, J.; Cao, Q.; Zhao, L.; Ma, Y.; Zhang, L.; Zhu, D. Enabling the Big Earth Observation Data via Cloud Computing and DGGS: Opportunities and Challenges. Remote Sens. 2019, 12, 62. [Google Scholar] [CrossRef]
- Purss, M.B.J.; Gibb, R.; Samavati, F.; Peterson, P.; Ben, J. The OGC® Discrete Global Grid System core standard: A framework for rapid geospatial integration. In Proceedings of the 2016 IEEE International Geoscience and Remote Sensing Symposium (IGARSS), Beijing, China, 10–15 July 2016. [Google Scholar]
- Goodchild, M.F. The Application of Advanced Information Technology in Assessing Environmental Impacts; Soil Science Society of America: Madison, WI, USA, 1996. [Google Scholar]
- Goodchild, M.F.; Kimerling, A.J.E. Discrete Global Grids: A Web Book; National Center for Geographic Information and Analysis: New Yor, NY, USA, 2002. [Google Scholar]
- Miliaresis, G.; Argialas, D. Segmentation of physiographic features from the global digital elevation model/GTOPO30. Comput. Geosci. 1999, 25, 715–728. [Google Scholar] [CrossRef]
- Arabelos, D. Intercomparisons of the global DTMs ETOPO5, TerrainBase and JGP95E. Phys. Chem. Earth Part A Solid Earth Geodesy 2000, 25, 89–93. [Google Scholar]
- Purss, M.B.; Peterson, P.R.; Strobl, P.; Dow, C.; Sabeur, Z.A.; Gibb, R.G.; Ben, J. Datacubes: A Discrete Global Grid Systems Perspective. Cartogr. Int. J. Geogr. Inf. Geovis. 2019, 54, 63–71. [Google Scholar]
- Sun, W.; Cui, M.; Zhao, X.; Gao, Y. A global discrete grid modeling method based on the spherical degenerate quadtree. In Proceedings of the 2008 International Workshop on Education Technology and Training & 2008 International Workshop on Geoscience and Remote Sensing, Shanghai, China, 21–22 December 2008. [Google Scholar]
- Dutton, G. Encoding and handling geospatial data with hierarchical triangular meshes. In Proceedings of the 7th International Symposium on Spatial Data Handling, Delft, The Netherlands, 12–16 August 1996. [Google Scholar]
- Ottoson, P.; Hauska, H. Ellipsoidal quadtrees for indexing of global geographical data. Int. J. Geogr. Inf. Sci. 2002, 16, 213–226. [Google Scholar]
- Gibb, R. The rHEALPix discrete global grid system. In IOP Conference Series: Earth and Environmental Science; IOP Publishing: Bristol, UK, 2016. [Google Scholar]
- Zhao, L.; Li, G.; Yao, X.; Ma, Y.; Cao, Q. An optimized hexagonal quadtree encoding and operation scheme for icosahedral hexagonal discrete global grid systems. Int. J. Digit. Earth 2022, 15, 975–1000. [Google Scholar] [CrossRef]
- Brodsky, I. H3: Uber’s Hexagonal Hierarchical Spatial Index. 2018. Available online: https://eng.uber.com/h3/ (accessed on 22 June 2019).
- Ullrich, P.A.; Lauritzen, P.H.; Jablonowski, C. Geometrically Exact Conservative Remapping (GECoRe): Regular latitude–longitude and cubed-sphere grids. Mon. Weather. Rev. 2009, 137, 1721–1741. [Google Scholar] [CrossRef]
- Béjar, R.; Lacasta, J.; Lopez-Pellicer, F.J.; Nogueras-Iso, J. Discrete Global Grid Systems with quadrangular cells as reference frameworks for the current generation of Earth observation data cubes. Environ. Model. Softw. 2023, 162, 105656. [Google Scholar]
- Kageyama, A.; Sato, T.J.G. Geophysics, Geosystems, “Yin-Yang grid”: An overset grid in spherical geometry. Geochem. Geophys. Geosyst. 2004, 5. [Google Scholar] [CrossRef]
- Bryan, G.L.; Norman, M.L.; O’Shea, B.W.; Abel, T.; Wise, J.H.; Turk, M.J.; Reynolds, D.R.; Collins, D.C.; Wang, P.; Skillman, S.W.; et al. Enzo: An adaptive mesh refinement code for astrophysics. Astrophys. J. Suppl. Ser. 2014, 211, 19. [Google Scholar]
- Yu, J.; Wu, L.; Li, Z.; Li, X. An SDOG-based intrinsic method for three-dimensional modelling of large-scale spatial objects. Ann. GIS 2012, 18, 267–278. [Google Scholar]
- Di, L.; Chen, A.; Yang, W.; Liu, Y.; Wei, Y.; Mehrotra, P.; Hu, C.; Williams, D. The development of a geospatial data Grid by integrating OGC Web services with Globus-based Grid technology. Concurr. Comput. Pract. Exp. 2008, 20, 1617–1635. [Google Scholar] [CrossRef]
- Goodchild, M.F. Integrating GIS and remote sensing for vegetation analysis and modeling: Methodological issues. J. Veg. Sci. 1994, 5, 615–626. [Google Scholar]
- Kimerling, J.A.; Sahr, K.; White, D.; Song, L. Comparing geometrical properties of global grids. Cartogr. Geogr. Inf. Sci. 1999, 26, 271–288. [Google Scholar]
- Ben, J.; Tong, X.-C.; Zhang, Y.-S.; Zhang, H. Discrete global grid systems: Generating algorithm and software model. In Proceedings of the Geoinformatics 2006: Geospatial Information Technology, Wuhan, China, 28–29 October 2006. [Google Scholar]
- Zhao, L.; Li, G.; Yao, X.; Ma, Y. Code Operation Scheme for the Icosahedral Hexagonal Discrete Global Grid System. Geo-Inf. Sci. 2023, 25, 239–251. [Google Scholar]
- Fu, C.C.; Jhuang, H.K.; Ho, Y.Y.; Tsai, T.C.; Lee, L.C.; Lin, C.H.; Lin, C.R.; Walia, V.; Lee, I.T. A Study of Lithosphere–Ionosphere Seismic Precursors from Detecting Gamma-Ray and Total Electron Content Anomalies Prior to the 2018 ML6. 2 Hualien Earthquake in Eastern Taiwan. Remote Sens. 2025, 17, 188. [Google Scholar]
- De Santis, A.; Jian, L.; Piersanti, M.; Shen, X.; Xiong, C.; Zhima, Z. Near-earth electromagnetic environment and natural hazards disturbances. Front. Environ. Sci. 2023, 11, 1307941. [Google Scholar]
- Saha, S.; Moorthi, S.; Pan, H.-L.; Wu, X.; Wang, J.; Nadiga, S.; Tripp, P.; Kistler, R.; Woollen, J.; Behringer, D.; et al. The NCEP climate forecast system reanalysis. Bull. Am. Meteorol. Soc. 2010, 91, 1015–1058. [Google Scholar]
- Ma, Y.; Li, G.; Yao, X.; Cao, Q.; Zhao, L.; Wang, S.; Zhang, L. A Precision Evaluation Index System for Remote Sensing Data Sampling Based on Hexagonal Discrete Grids. Int. J. Geo-Inf. 2021, 10, 194. [Google Scholar]




| Grid Type | Structural Features | Representative Models | Advantages and Limitations | Typical Applications |
|---|---|---|---|---|
| Triangular Grids | Based on octahedrons/icosahedrons; hierarchical but geometrically complex | QTM (Quaternary Triangular Mesh) [19] SQT (Sphere Quad Tree) [20] | Pros: Hierarchical continuity Cons: Complex neighborhood algorithms, irreducible geometric distortion | Global terrain modeling Spatial indexing |
| Quadrilateral Grids | Merged triangular units; simplified adjacency relations | Octahedral rhombus subdivision Quadtree-extended models | Pros: Simplified neighborhood operations Cons: Limited flexibility, minor high-latitude distortion | Data visualization Hierarchical analysis |
| Hexagonal Grids | High adjacency symmetry; optimal coverage efficiency | H3 (seven-aperture) rHEALPix (refined Hierarchical Equal-Area iso-Latitude Pixelization) [21] OHQS (Optimized Hexagonal Quadtree Structure) [22] | Pros: Dynamic modeling performance Cons: High encoding complexity, projection approximation required | Global environmental sampling Multi-resolution platforms |
| Angle with the i-axis | 0 | |||
| Binary system | 000 | 001 | 010 | 100 |
| 0 | 1 | 2 | 4 |
| Temporal Resolution | Start Encoding | End Encoding |
|---|---|---|
| 100 y | 1 | 10 |
| 10 | 11 | 110 |
| 1 y | 111 | 1110 |
| 1 m | 1111 | 13110 |
| 1 d | 13111 | 385110 |
| 1 h | 385111 | 9313110 |
| 1 min | 9313111 | 544993110 |
| 1 s | 544993111 | 32685793110 |
| Composition of the Layer Body Encoding | Code Element of the Layer Body | Data Type |
|---|---|---|
| Subdivision identification of the layer body | Code of the layer-surface unit | String |
| Code of the subdivision level of the layer radius | String | |
| Code of the layer-radius unit | String |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ma, Y.; Li, G.; Zhao, L.; Yao, X. A Novel Earth-System Spatial Grid Model: ISEA4H-ESSG for Multi-Layer Geoscience Data Integration and Analysis. Appl. Sci. 2025, 15, 3703. https://doi.org/10.3390/app15073703
Ma Y, Li G, Zhao L, Yao X. A Novel Earth-System Spatial Grid Model: ISEA4H-ESSG for Multi-Layer Geoscience Data Integration and Analysis. Applied Sciences. 2025; 15(7):3703. https://doi.org/10.3390/app15073703
Chicago/Turabian StyleMa, Yue, Guoqing Li, Long Zhao, and Xiaochuang Yao. 2025. "A Novel Earth-System Spatial Grid Model: ISEA4H-ESSG for Multi-Layer Geoscience Data Integration and Analysis" Applied Sciences 15, no. 7: 3703. https://doi.org/10.3390/app15073703
APA StyleMa, Y., Li, G., Zhao, L., & Yao, X. (2025). A Novel Earth-System Spatial Grid Model: ISEA4H-ESSG for Multi-Layer Geoscience Data Integration and Analysis. Applied Sciences, 15(7), 3703. https://doi.org/10.3390/app15073703








