Extra-Tidal Members and Dynamics of the Open Cluster NGC 6705
Abstract
1. Introduction
2. Data and Method
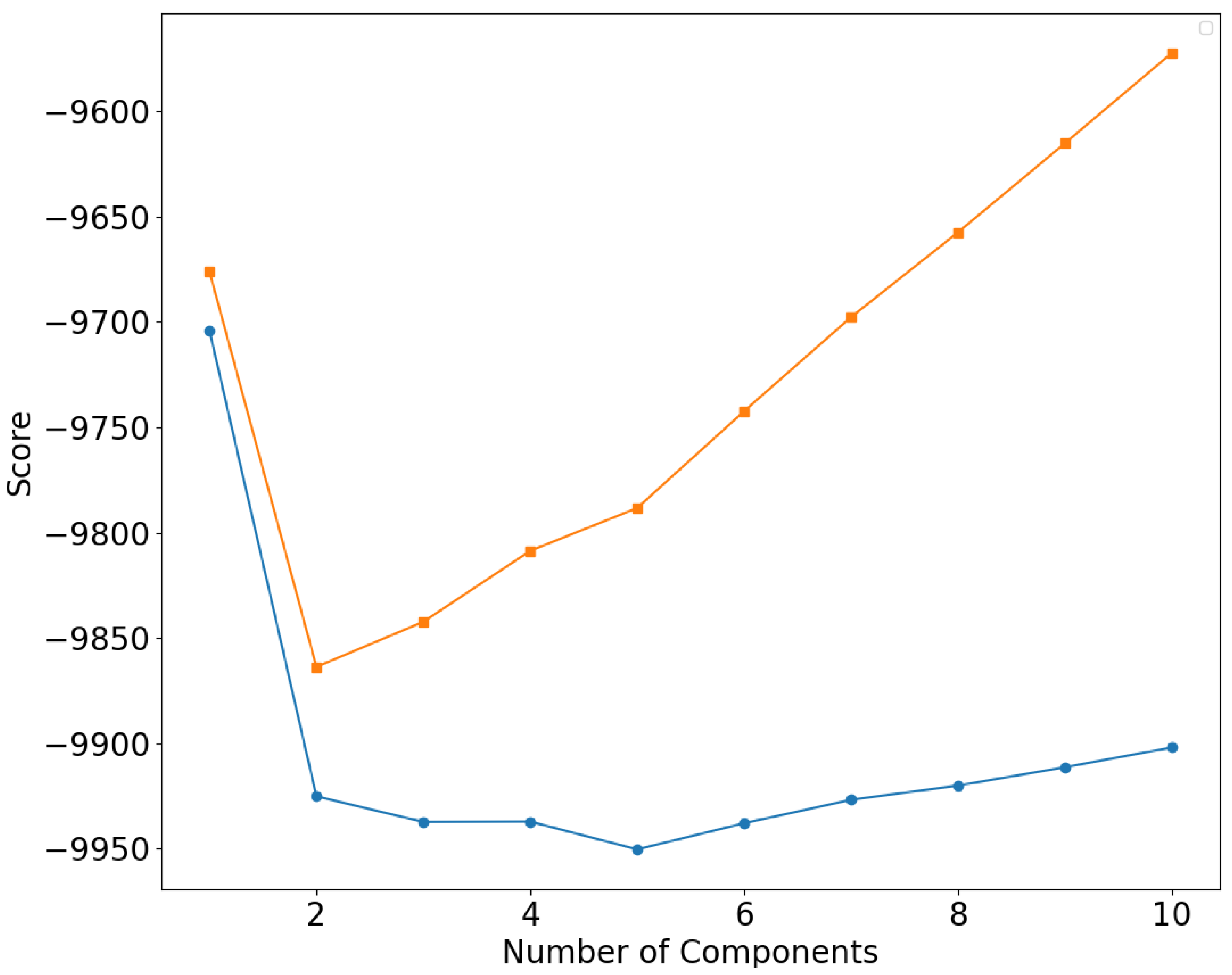
2.1. Sample Selection
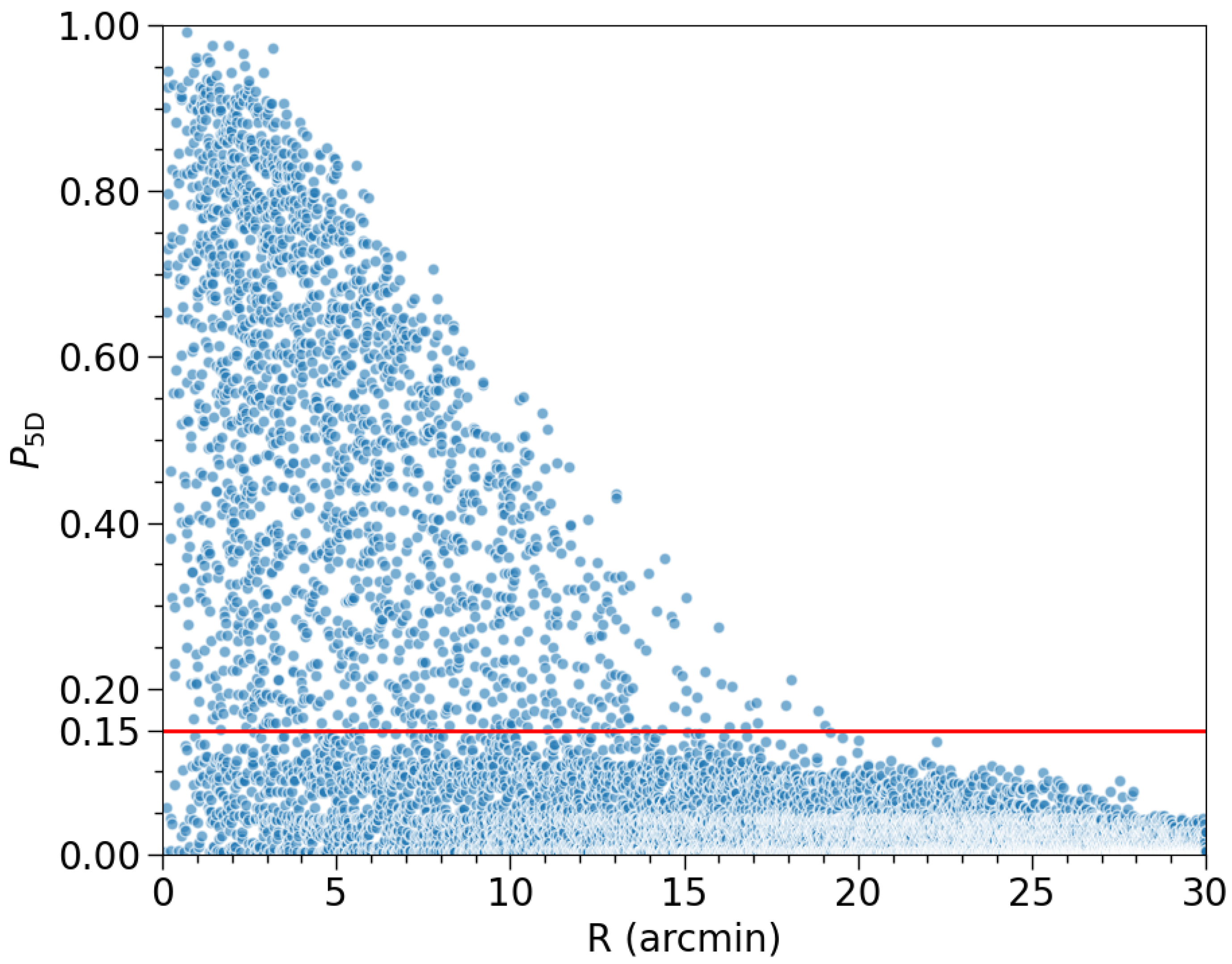
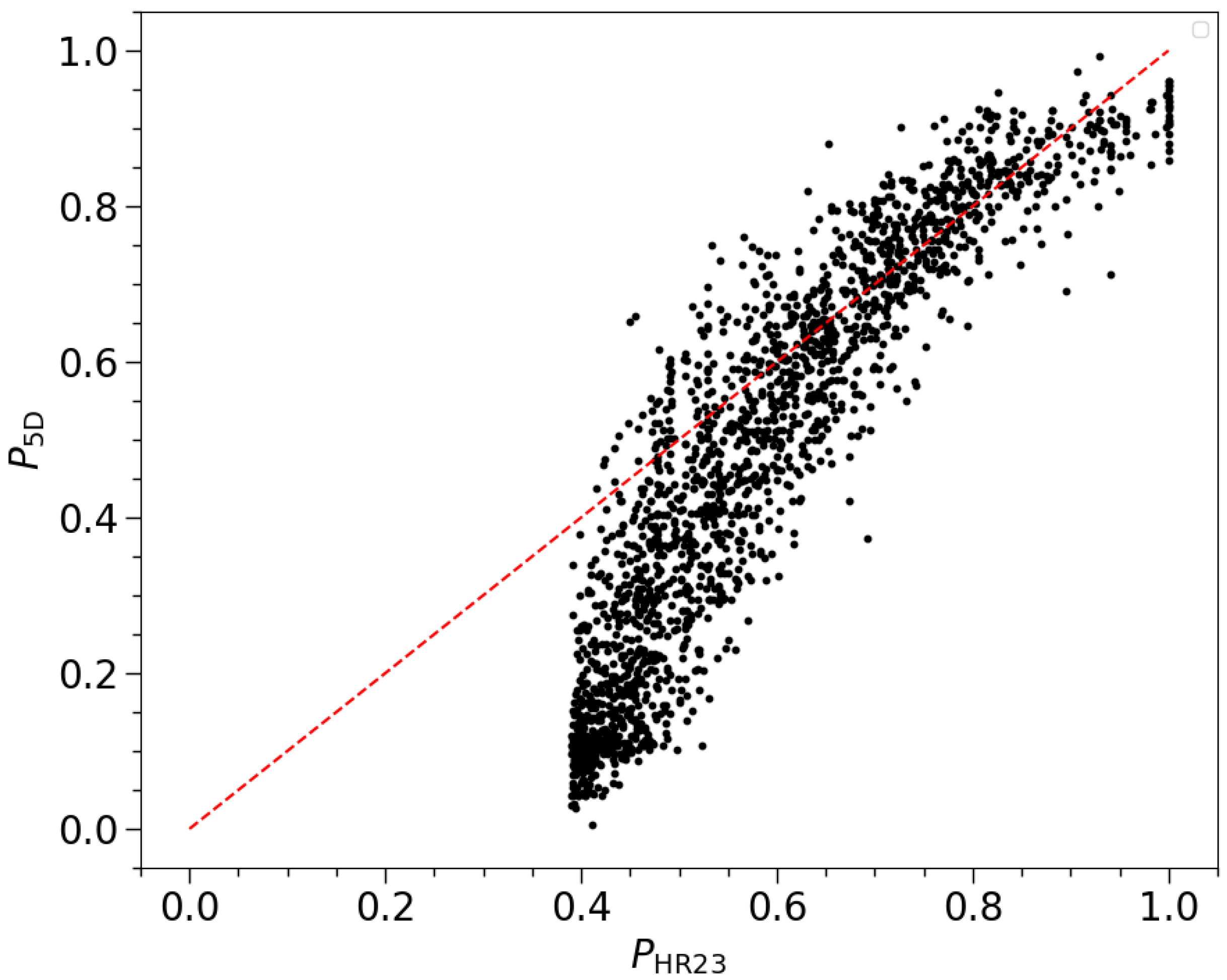
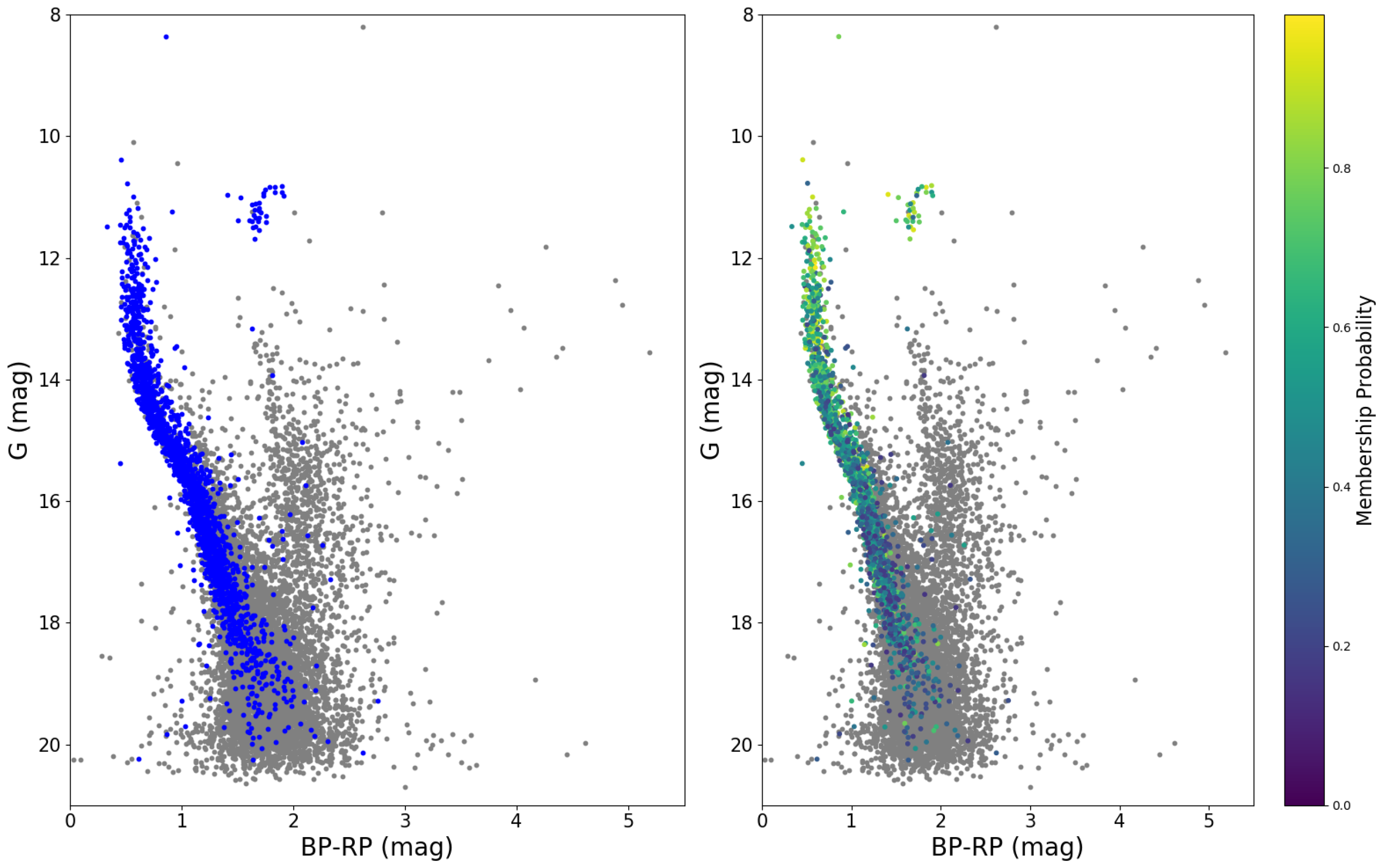
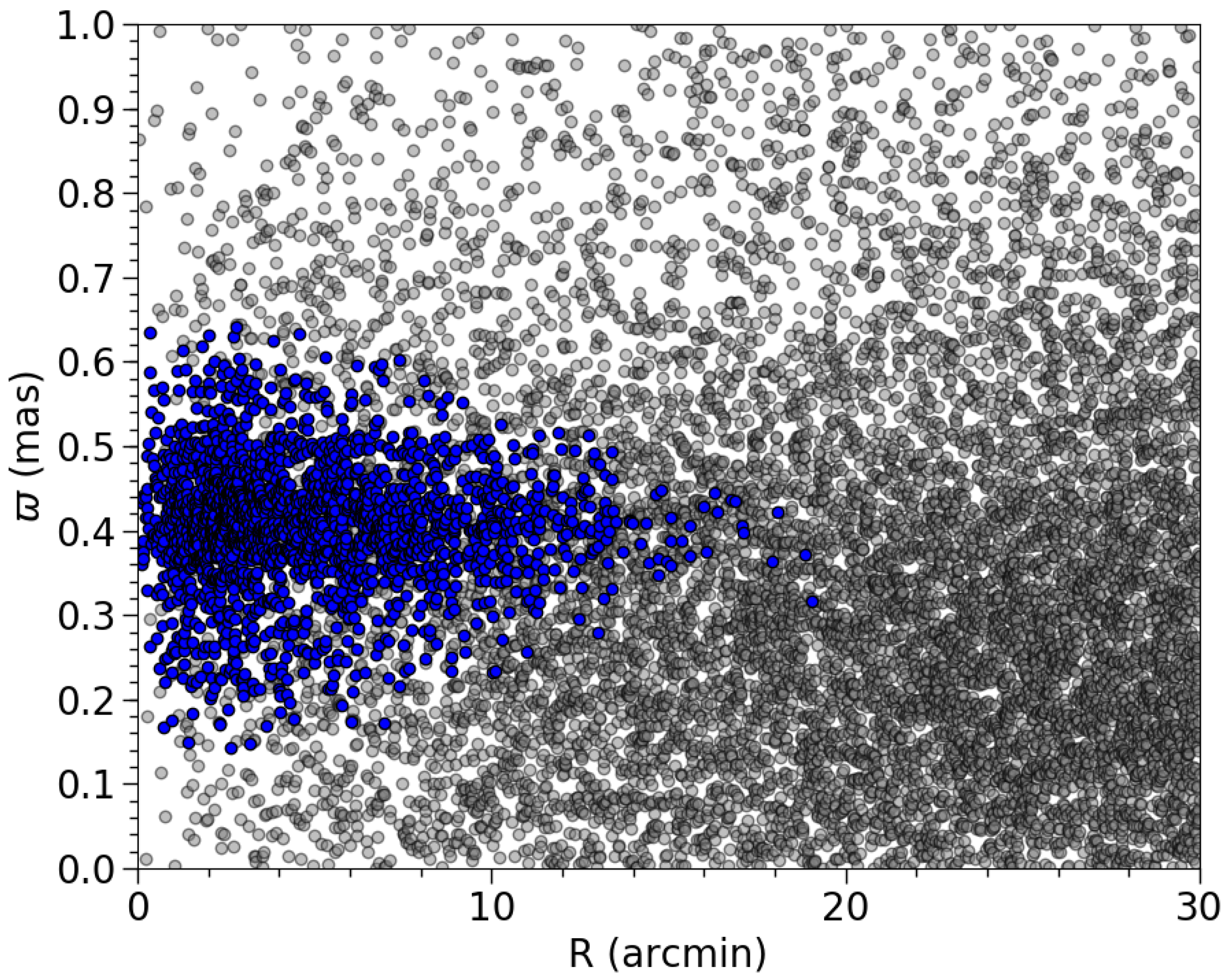
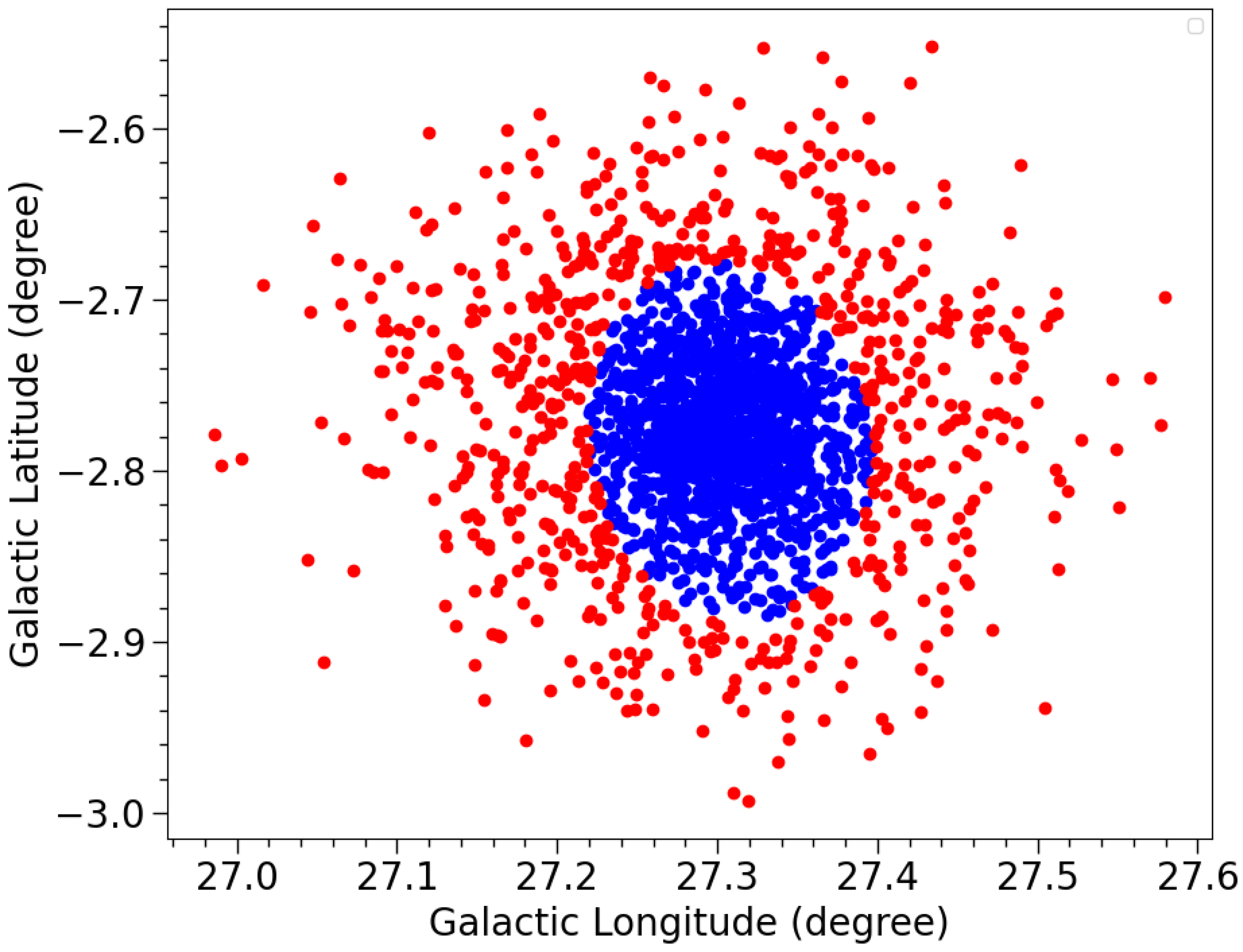
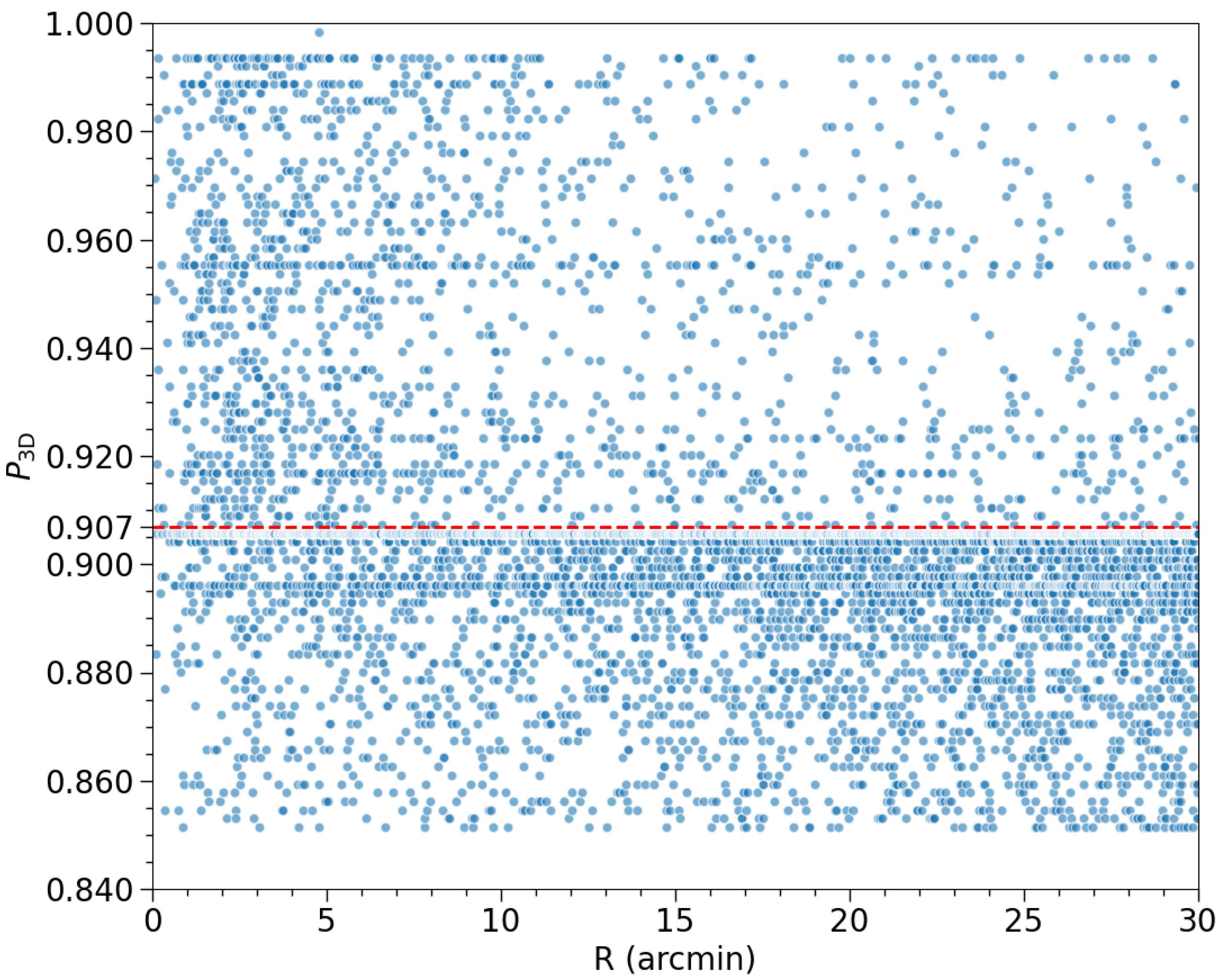
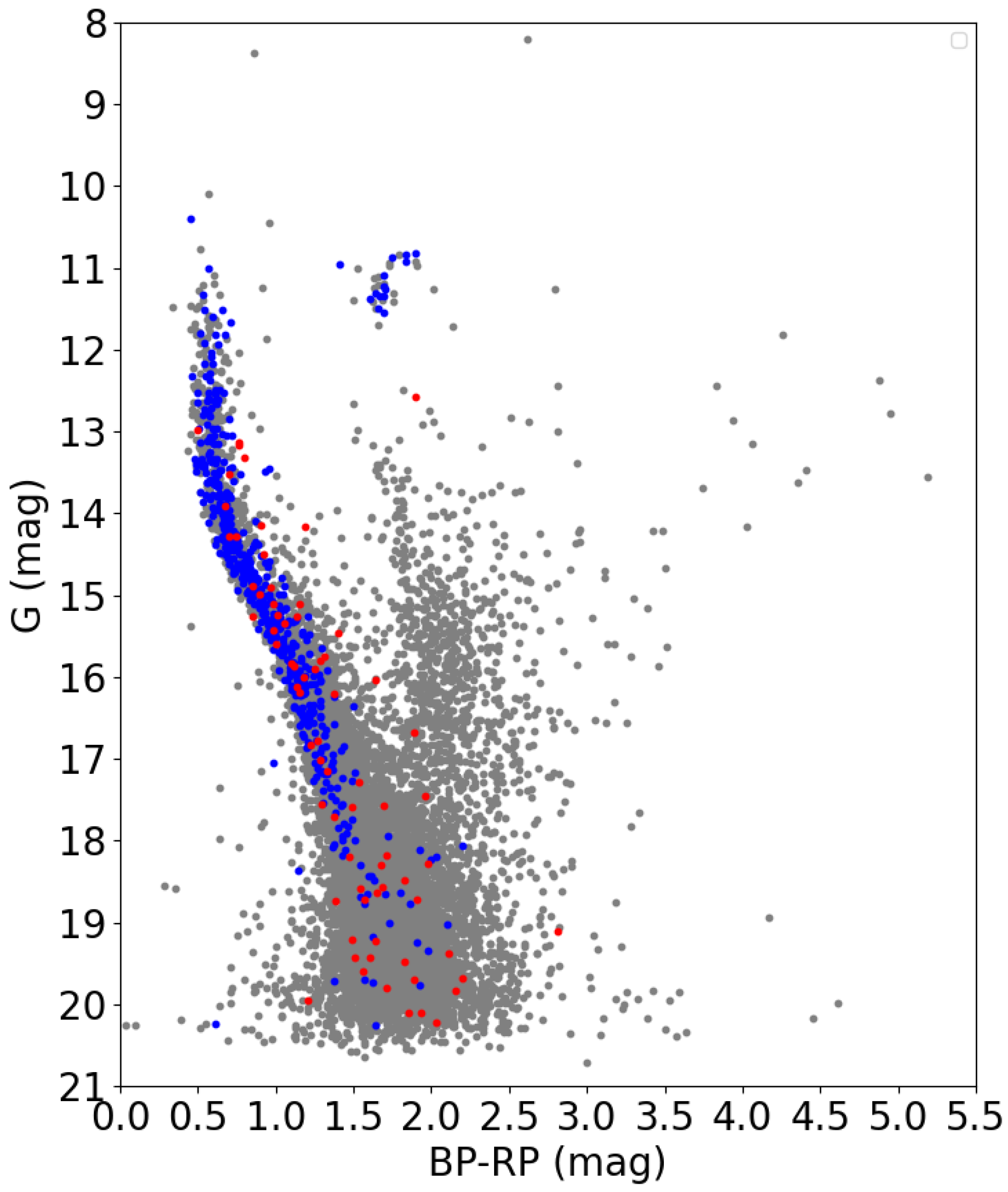
2.2. Membership Determination
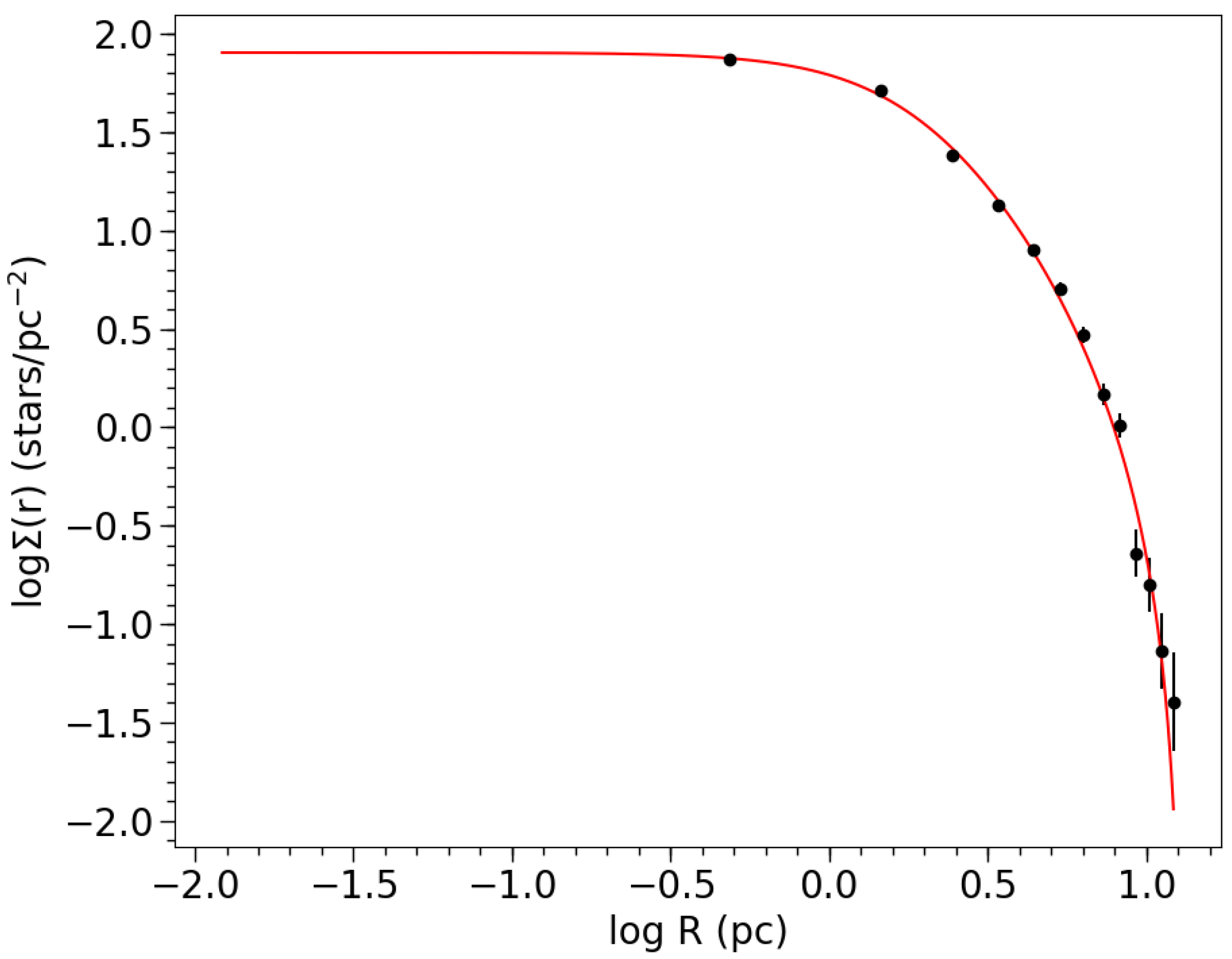
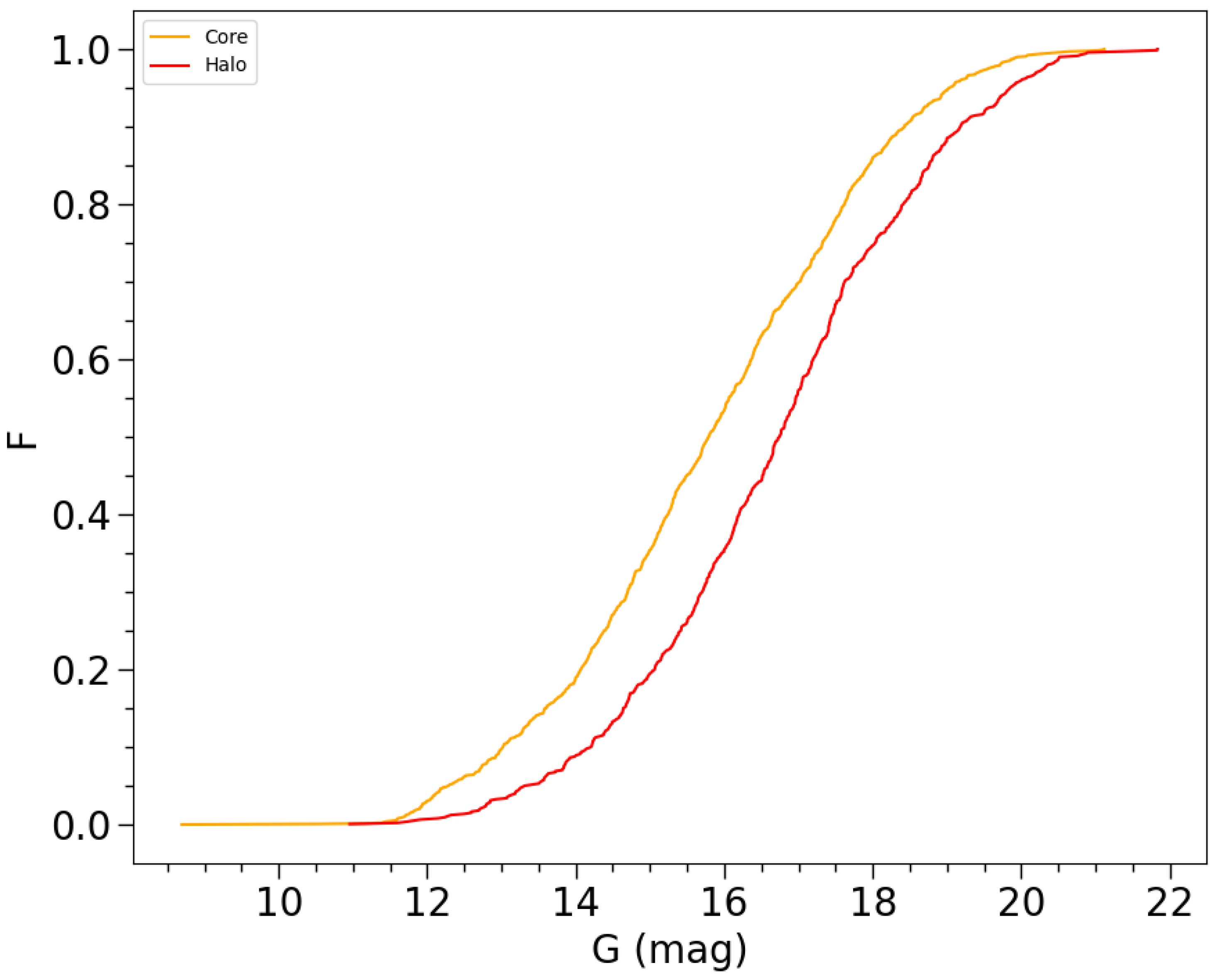
3. Structural Parameters and Dynamical State
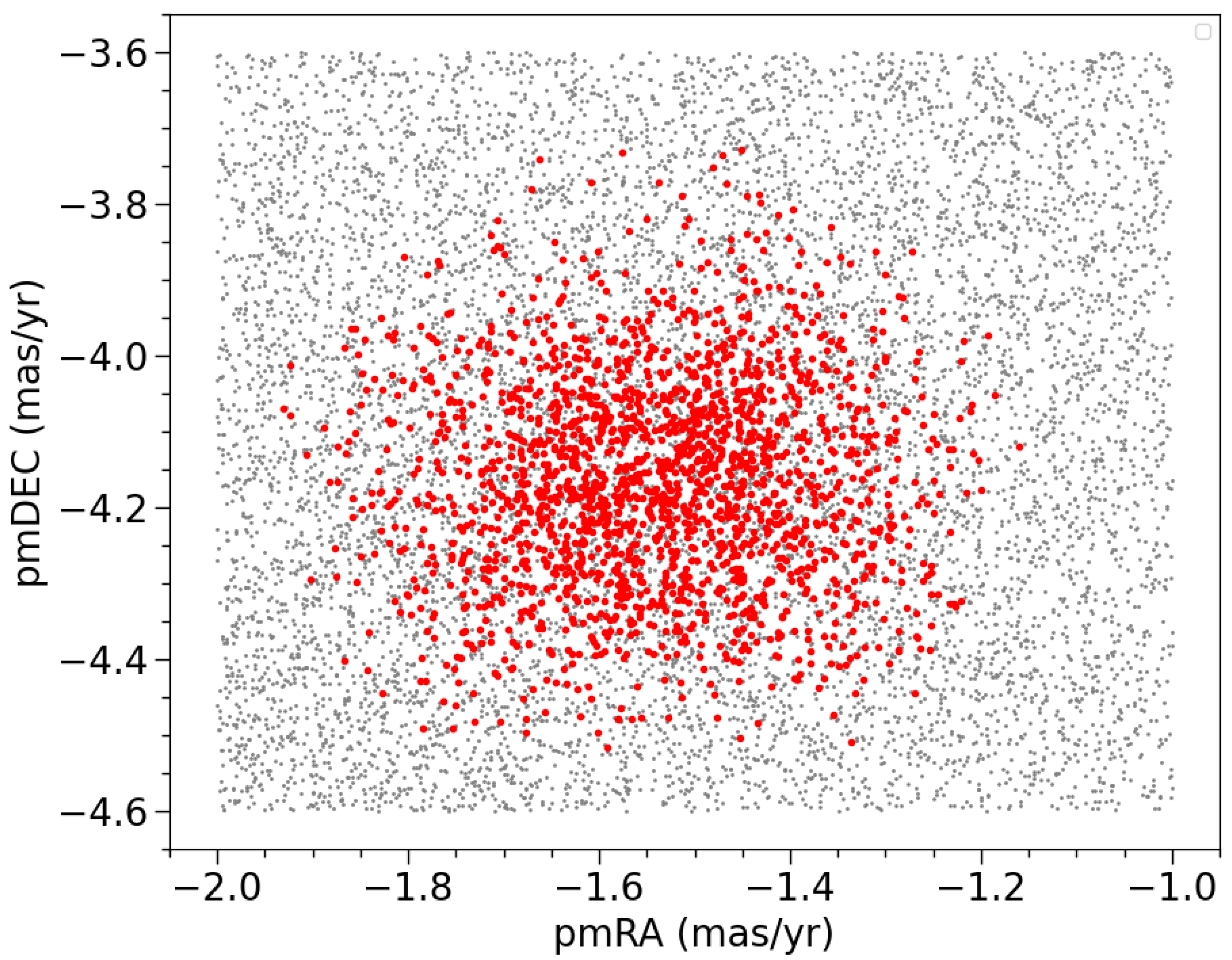
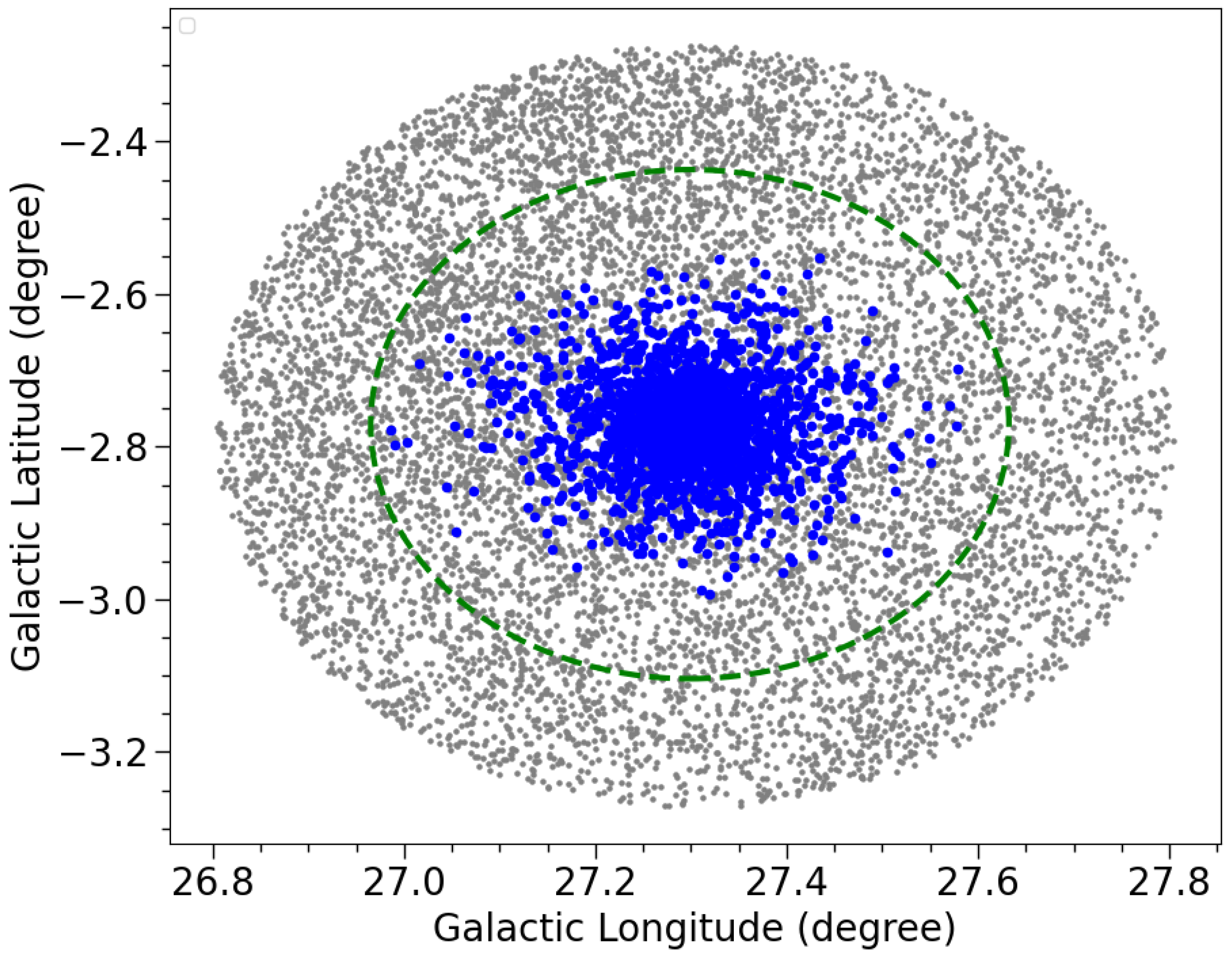
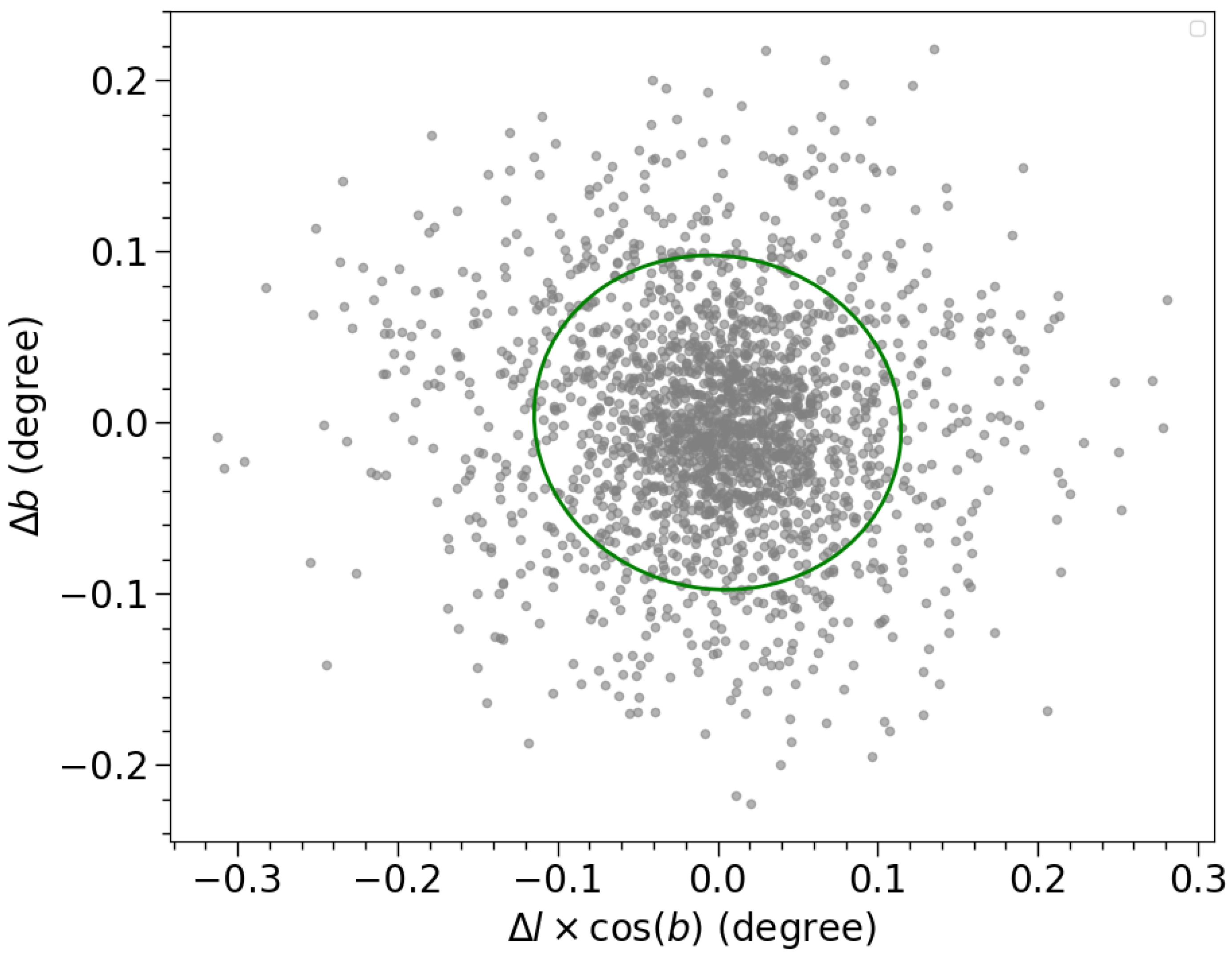
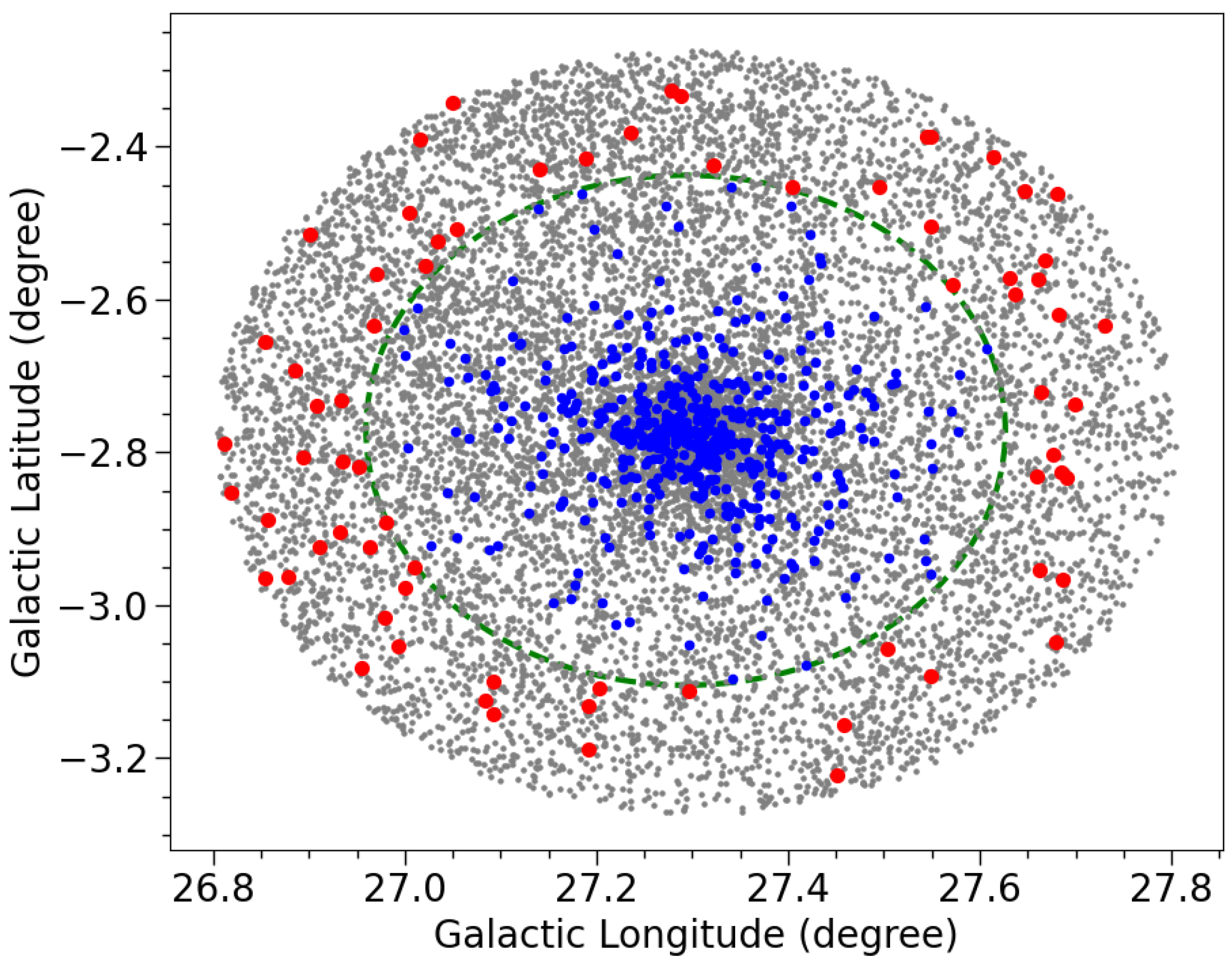
4. Extra-Tidal Members
5. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kharchenko, N.; Piskunov, A.; Röser, S.; Schilbach, E.; Scholz, R.D. Astrophysical parameters of Galactic open clusters. Astron. Astrophys. 2005, 438, 1163–1173. [Google Scholar] [CrossRef]
- Kalirai, J.S.; Richer, H.B. Star clusters as laboratories for stellar and dynamical evolution. Philos. Trans. R. Soc. Math. Phys. Eng. Sci. 2010, 368, 755–782. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Friel, E. The old open clusters of the Milky Way. Annu. Rev. Astron. Astrophys. 1995, 33, 381–414. [Google Scholar] [CrossRef]
- Cantat-Gaudin, T.; Jordi, C.; Vallenari, A.; Bragaglia, A.; Balaguer-Núñez, L.; Soubiran, C.; Bossini, D.; Moitinho, A.; Castro-Ginard, A.; Krone-Martins, A.; et al. A Gaia DR2 view of the open cluster population in the Milky Way. Astron. Astrophys. 2018, 618, A93. [Google Scholar] [CrossRef]
- Cantat-Gaudin, T.; Anders, F.; Castro-Ginard, A.; Jordi, C.; Romero-Gómez, M.; Soubiran, C.; Casamiquela, L.; Tarricq, Y.; Moitinho, A.; Vallenari, A.; et al. Painting a portrait of the Galactic disc with its stellar clusters. Astron. Astrophys. 2020, 640, A1. [Google Scholar] [CrossRef]
- Dias, W.S.; Monteiro, H.; Moitinho, A.; Lépine, J.R.; Carraro, G.; Paunzen, E.; Alessi, B.; Villela, L. Updated parameters of 1743 open clusters based on Gaia DR2. Mon. Not. R. Astron. Soc. 2021, 504, 356–371. [Google Scholar] [CrossRef]
- Hunt, E.L.; Reffert, S. Improving the open cluster census-II. An all-sky cluster catalogue with Gaia DR3. Astron. Astrophys. 2023, 673, A114. [Google Scholar] [CrossRef]
- Magrini, L.; Randich, S.; Romano, D.; Friel, E.; Bragaglia, A.; Smiljanic, R.; Jacobson, H.; Vallenari, A.; Tosi, M.; Spina, L.; et al. The Gaia-ESO Survey: Abundance ratios in the inner-disk open clusters Trumpler 20, NGC 4815, NGC 6705. Astron. Astrophys. 2014, 563, A44. [Google Scholar] [CrossRef]
- Tang, B.; Geisler, D.; Friel, E.; Villanova, S.; Smiljanic, R.; Casey, A.; Randich, S.; Magrini, L.; San Roman, I.; Muñoz, C.; et al. The Gaia-ESO survey: The inner disk intermediate-age open cluster NGC 6802. Astron. Astrophys. 2017, 601, A56. [Google Scholar] [CrossRef][Green Version]
- Overbeek, J.; Friel, E.; Donati, P.; Smiljanic, R.; Jacobson, H.; Hatzidimitriou, D.; Held, E.V.; Magrini, L.; Bragaglia, A.; Randich, S.; et al. The Gaia-ESO Survey: The inner disk, intermediate-age open cluster Trumpler 23. Astron. Astrophys. 2017, 598, A68. [Google Scholar] [CrossRef]
- Santos, J.; Bonatto, C.; Bica, E. Structure and stellar content analysis of the open cluster M 11 with 2MASS photometry. Astron. Astrophys. 2005, 442, 201–209. [Google Scholar]
- Bonatto, C.; Bica, E. Mass segregation in M 67 with 2MASS. Astron. Astrophys. 2003, 405, 525–530. [Google Scholar]
- Sagar, R.; Pandey, A.; Mohan, V. A study of spatial structure of galactic open star clusters. Astron. Astrophys. 2002, 383, 153–162. [Google Scholar]
- Bonatto, C.; Bica, E. Old open clusters in the inner Galaxy: FSR 1744, FSR 89 and FSR 31. Astron. Astrophys. 2007, 473, 445–455. [Google Scholar] [CrossRef][Green Version]
- Bonatto, C.; Bica, E. Open clusters in dense fields: The importance of field-star decontamination for NGC 5715, Lyngå 4, Lyngå 9, Trumpler 23, Trumpler 26 and Czernik 37. Mon. Not. R. Astron. Soc. 2007, 377, 1301–1323. [Google Scholar] [CrossRef]
- Tarricq, Y.; Soubiran, C.; Casamiquela, L.; Castro-Ginard, A.; Olivares, J.; Miret-Roig, N.; Galli, P. Structural parameters of 389 local open clusters. Astron. Astrophys. 2022, 659, A59. [Google Scholar]
- Prusti, T.; De Bruijne, J.; Brown, A.G.; Vallenari, A.; Babusiaux, C.; Bailer-Jones, C.; Bastian, U.; Biermann, M.; Evans, D.W.; Eyer, L.; et al. The gaia mission. Astron. Astrophys. 2016, 595, A1. [Google Scholar]
- Vallenari, A.; Brown, A.G.; Prusti, T.; De Bruijne, J.H.; Arenou, F.; Babusiaux, C.; Biermann, M.; Creevey, O.L.; Ducourant, C.; Evans, D.W.; et al. Gaia data release 3-summary of the content and survey properties. Astron. Astrophys. 2023, 674, A1. [Google Scholar]
- Gao, X. A clustering (DBSCAN+ GMM) investigation of the young open cluster NGC 6649. Mon. Not. R. Astron. Soc. 2024, 527, 1784–1793. [Google Scholar] [CrossRef]
- Gao, X. Discovery of Tidal Tails around the Old Open Cluster NGC 2506. Astrophys. J. 2020, 894, 48. [Google Scholar]
- Gao, X.; Zhu, C. The extended halo and tidal tails of the old open cluster NGC 2112. Publ. Astron. Soc. Jpn. 2024, 76, psae085. [Google Scholar] [CrossRef]
- Kos, J. Tidal tails of open clusters. Astron. Astrophys. 2024, 691, A28. [Google Scholar] [CrossRef]
- Solomon, S.; McNamara, B. M11-A study of its inner and outer regions. Astron. J. 1980, 85, 432–437. [Google Scholar] [CrossRef]
- Casamiquela, L.; Balaguer-Núnez, L.; Jordi, C.; Masana, E. Physical parameters of NGC6705 (M11) open cluster using Strömgren photometry. In Highlights of Spanish Astrophysics VIII: Proceedings of the XI Scientific Meeting of the Spanish Astronomical Society, Teruel, Spain, 8–12 September 2014; Spanish Astronomical Society: Barcelona, Spain, 2015; p. 596. [Google Scholar]
- Carbajo-Hijarrubia, J.; Casamiquela, L.; Carrera, R.; Balaguer-Núñez, L.; Jordi, C.; Anders, F.; Gallart, C.; Pancino, E.; Drazdauskas, A.; Stonkutė, E.; et al. OCCASO-V. Chemical-abundance trends with Galactocentric distance and age. Astron. Astrophys. 2024, 687, A239. [Google Scholar] [CrossRef]
- Bragaglia, A.; D’Orazi, V.; Magrini, L.; Baratella, M.; Bensby, T.; Martell, S.; Randich, S.; Tautvaišienė, G.; Alfaro, E.J.; Morbidelli, L.; et al. The Gaia-ESO Survey: No sign of multiple stellar populations in open clusters from their sodium and oxygen abundances. Astron. Astrophys. 2024, 687, A124. [Google Scholar] [CrossRef]
- Ester, M.; Kriegel, H.P.; Sander, J.; Xu, X. A density-based algorithm for discovering clusters in large spatial databases with noise. In Proceedings of the Second International Conference on Knowledge Discovery and Data Mining, Portland, OR, USA, 2–4 August 1996; pp. 226–231. [Google Scholar]
- Agarwal, M.; Rao, K.K.; Vaidya, K.; Bhattacharya, S. ML-MOC: Machine learning (kNN and GMM) based membership determination for open clusters. Mon. Not. R. Astron. Soc. 2021, 502, 2582–2599. [Google Scholar] [CrossRef]
- Gao, X.H.; Xu, S.K.; Chen, L. 3D cluster members and near-infrared distance of open cluster NGC 6819. Res. Astron. Astrophys. 2015, 15, 2193. [Google Scholar] [CrossRef]
- Gao, X. Spatial structure and dynamical state of the old open cluster Collinder 261 based on a clustering method. Publ. Astron. Soc. Jpn. 2023, 75, 82–89. [Google Scholar] [CrossRef]
- Bhattacharya, S.; Mahulkar, V.; Pandaokar, S.; Singh, P.K. Morphology of open clusters NGC 1857 and Czernik 20 using clustering algorithms. Astron. Comput. 2017, 18, 1–7. [Google Scholar] [CrossRef]
- Gao, X.; Fang, D. A clustering study of the old open cluster Trumpler 19. Astrophys. Space Sci. 2023, 368, 73. [Google Scholar] [CrossRef]
- King, I. The structure of star clusters. I. an empirical density law. Astron. J. 1962, 67, 471. [Google Scholar]
- Just, A.; Piskunov, A.E.; Klos, J.H.; Kovaleva, D.A.; Polyachenko, E.V. Global survey of star clusters in the Milky Way-VII. Tidal parameters and mass function. Astron. Astrophys. 2023, 672, A187. [Google Scholar]
- Jolliffe, I.T. Principal Component Analysis for Special Types of Data; Springer: Berlin/Heidelberg, Germany, 2002. [Google Scholar]
- Cottaar, M.; Hénault-Brunet, V. Binary-corrected velocity dispersions from single-and multi-epoch radial velocities: Massive stars in R136 as a test case. Astron. Astrophys. 2014, 562, A20. [Google Scholar]
- Piatti, A.E. Binary star sequence in the outskirts of the disrupting Galactic open cluster UBC 274. Astron. Astrophys. 2020, 639, A55. [Google Scholar] [CrossRef]




| Age (Gyr) | Distance (pc) | [Fe/H] | AV (mag) | E (B-V) | Bibliography |
|---|---|---|---|---|---|
| 0.200 | 1900 | 0.42 | Solomon and McNamara 1980 [23] | ||
| 2000 | −0.2 ± 0.4 | 0.42 | Casamiquela et al. 2015 [24] | ||
| 0.288 | 1888 | 0.043 | 1.457 | Dias et al. 2021 [6] | |
| 0.310 | 2233 | 1.3 | Hunt and Reffert 2023 [7] | ||
| 0.309 | 2203 | 0.114 | Carbajo-Hijarrubia et al. 2024 [25] | ||
| 0.309 | 2203 | 0.114 | 1.2 | Bragaglia et al. 2024 [26] |
| Component | Stars | Central Positions | pmRA | pmDEC | e | ||||
|---|---|---|---|---|---|---|---|---|---|
| Core | 1279 | (l,b) = (,) | −1.543 | −4.167 | 0.89 | ||||
| Halo | 690 | (l,b) = (,) | −1.539 | −4.163 | 0.80 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, C.; Gao, X. Extra-Tidal Members and Dynamics of the Open Cluster NGC 6705. Appl. Sci. 2025, 15, 3488. https://doi.org/10.3390/app15073488
Zhou C, Gao X. Extra-Tidal Members and Dynamics of the Open Cluster NGC 6705. Applied Sciences. 2025; 15(7):3488. https://doi.org/10.3390/app15073488
Chicago/Turabian StyleZhou, Chen, and Xinhua Gao. 2025. "Extra-Tidal Members and Dynamics of the Open Cluster NGC 6705" Applied Sciences 15, no. 7: 3488. https://doi.org/10.3390/app15073488
APA StyleZhou, C., & Gao, X. (2025). Extra-Tidal Members and Dynamics of the Open Cluster NGC 6705. Applied Sciences, 15(7), 3488. https://doi.org/10.3390/app15073488





