Experimental Study on Horizontal Pipeline Transportation Characteristics of Coarse Particle

Abstract

In dredging operations, the efficient transportation of dredged materials presents a significant and intricate challenge. This study focuses on the motion and resistance characteristics of coarse-grained dredged materials during pipeline conveyance. A specialized simulation experiment platform was developed to investigate the horizontal pipeline transport of coarse-grained materials. The experimental design encompassed varying particle diameters, material volume concentrations, and mixed average flow rates to analyze the motion and resistance characteristics of these materials in horizontal pipelines. Three distinct particle beds were identified based on different coarse particle motion states. This study statistically analyzed the impact of the particle diameter and material volume concentration on the transport efficiency of coarse particle populations. The key findings indicate that the mixed mean flow rate significantly influences the transportation efficiency of coarse particle groups, whereas the particle diameter and material volume concentration have a minimal effect. Specifically, coarse particles with a diameter of 0.9 mm demonstrated optimal water flow following, and higher mixed mean flow rates correlated with increased transportation efficiency of the coarse particle group. The transition speed of the coarse particle group flow type was notably affected by the material volume concentration and particle diameter, exhibiting a linear relationship. Therefore, when the particle size of the dredged material increases or the concentration increases, the average flow rate of the mixture is appropriately increased to ensure that the flow pattern of the dredged material in the pipeline remains in a non-homogeneous suspended flow pattern, thereby improving the efficiency and stability of the transportation system. By optimizing the conveying characteristics of coarse-grained materials, the pipeline conveying efficiency can be improved and the risk of pipeline wear and clogging can be reduced, thus lowering engineering costs and energy consumption and promoting technological innovation in related industries. In addition, this research can enhance engineering safety, reduce resource waste and environmental pollution, promote sustainable development, and provide important theoretical support and practical guidance for emerging fields such as deep-sea mining and environmental engineering.

1. Introduction

Dredging is a long-established construction technique that involves the excavation of soil and rock from the bottoms of rivers and seas using either manual labor or machinery and transporting the excavated materials to designated areas [1]. Due to the complex construction environment and the varying skill levels of construction personnel, high-concentration delivery is often not feasible during the construction process, resulting in relatively high construction costs [2]. Currently, dredging projects commonly utilize methods such as pipeline hydraulic transport, land transportation, and waterway transportation for the movement of dredged materials. Among these, pipeline hydraulic transport is widely used in large-scale dredging operations due to its advantages of high efficiency, low cost, and low energy consumption [3].

Therefore, studying the motion of the solid–liquid two-phase flow of coarse-grained dredged materials in horizontal pipelines is of significant reference value and practical importance for improving the hydraulic transport efficiency of pipelines and promoting applications.

Currently, most studies rely primarily on experiments. Early theoretical models were based on macro-experimental summaries of pipeline transport characteristics. Wasp [4,5] used a two-phase flow model, treating fine particles and water as the carrier, while considering large-diameter coarse particles as the transport object. They fully took into account the flow patterns of solid–liquid two-phase flow to derive the resistance loss of the two-phase carrier and the resistance loss of bed-load sediment transport.

Several studies from the Saskatchewan Research Council have continuously improved the two-phase flow model and proposed the new model [6,7]. The model is based on the Coulomb friction assumption, placing the particles causing friction in the lower layer while other particles are uniformly suspended in the pipeline. The model considers various factors, such as the pipe diameter, particle size, flow velocity, etc., and has high computational accuracy.

Kaushal and Tomita [8,9] proposed a complex concentration distribution calculation model [10] that comprehensively considers the effects of the particle size distribution and volume concentration, which can significantly improve prediction accuracy and fit a larger particle size distribution. The most comprehensive model currently available internationally is the Delft head loss and critical velocity framework proposed by Miedema [11,12,13]. This model framework provides a more detailed description of the flow types of materials transported inside the pipeline and comprehensively considers the types of energy loss and the effects of interfacial forces. The model has a wider range of applicability and can be used for more complex pipeline layouts.

Cou, Zou [14], and others used high-speed cameras and particle imaging velocimetry technology to conduct a more intuitive analysis of the particle bed layer. They pointed out that the velocity distribution of particles in the cross-section is logarithmic and particles in the center of the cross-section of the pipeline flow are the largest, and those close to the top of the pipe wall flow are the smallest. Zhang [15] analyzed the particle motion behavior using methods such as acoustic emission, pressure pulsation, and high-speed imaging, revealing the formation mechanism of the minimum conveying speed, realizing the identification of complex flow patterns and quantitative detection of the solid mass flow rate/concentration across flow patterns, and establishing an intelligent prediction model for the solid mass flow rate in the horizontal pipe pneumatic conveying process.

In summary, the research of most scholars and institutions mainly relies on experimental research methods. Early theoretical models were based on macroscopic experimental pipeline transportation characteristics, with an insufficient theoretical basis. Current research still faces problems such as small parameter ranges and difficulty in meeting accuracy requirements.

This study focuses on the challenges associated with pipeline conveyance and the transfer of coarse-grained dredged materials during dredging operations, with a particular emphasis on investigating the motion characteristics of coarse particle groups in horizontal pipeline hydraulic conveying systems. The research begins by establishing an energy theory model for solid–liquid two-phase flow, which is subsequently validated through experimental observations. By analyzing the motion states of coarse particles, the study systematically examines the influence of the mixed average velocity, material volume concentration, and particle size on the motion characteristics of coarse particles during pipeline hydraulic conveying. Based on the findings, the research provides practical recommendations for optimizing flow parameters in the pipeline hydraulic conveying of coarse particles, offering valuable insights for enhancing the efficiency and effectiveness of dredging construction processes.

2. Methodology

2.1. Theory of Solid–Liquid Two Phase Flow Model

In the realm of dredging engineering, the physical properties of the solid–liquid two-phase flow, which results from the mixture of coarse dredging materials and water, significantly influence the dynamics of the two-phase flow [16]. These properties are determined collectively by both the solid and liquid components. The characteristics pertinent to the mixture and its hydraulic transportation can be described by the following formula:

$${\gamma }_m={\displaystyle \frac{G_m}{{\varnothing }_m}}$$

(1)

where ${\gamma }_m$ represents the bulk density of slurry; $G_m$ represents the total weight of the slurry; ${\varnothing }_m$ represents the total volume of slurry. This physical quantity reflects, to some extent, the concentration of the solid–liquid two-phase flow.

The settling velocity of solid particles in a liquid is a critical physical parameter that characterizes the behavior of two-phase flow. This velocity significantly influences the formation and evolution of flow patterns within the pipeline. The settling velocity is primarily determined by factors such as the density of the particles, their diameter, the viscosity and density of the liquid, and the local gravitational acceleration. According to Stokes’ law, when the settling velocity of particles is low and the relative velocity between the particles and the liquid is minimal, the settling velocity of the particles can be calculated using the following formula [17]:

$$\omega =\sqrt{{\displaystyle \frac{4}{3}}gd\left({\displaystyle \frac{{\rho }_s-\rho }{\rho }}\right)/C_D}$$

(2)

where $\omega$ represent the settling velocity of particles, $g$ represent the gravitational acceleration, ${\rho }_s$ represent the density of particles, $\rho$ represent the density of liquids, $d$ represent the diameter of particles, and $C_D$ represent the drag coefficient.

The spatial volume concentration $C_{vs}$ is the volume occupied by the solid ${\varnothing }_s$ divided by the total mixture volume ${\varnothing }_m$ of the pipeline section.

$$C_{vs}={\displaystyle \frac{{\varnothing }_s}{{\varnothing }_m}}$$

(3)

The transport volume concentration $C_{vt}$ is the solids volume flow rate $Q_s$ divided by the total mixture volume flow rate $Q_m$.

$$C_{vt}={\displaystyle \frac{Q_s}{Q_m}}$$

(4)

For a certain control volume, if a certain linear velocity $V_{ls}$ is given and the volumetric spatial concentration $C_{vs}$ and slip velocity $V_{sl}$ are known, the volumetric transport concentration $C_{vt}$ can be determined by the following equation:

$$C_{vt}=\left(1-{\displaystyle \frac{V_{sl}}{V_{ls}}}\right)·C_{vs}=\left(1-\xi \right)·C_{vs}$$

(5)

In the field of pipeline hydraulic transportation, there is a difference in velocity between liquid and solid phases. Slip velocity is the difference in velocity between one phase and another, while linear velocity is the flow velocity inside the fluid pipeline.

$$V_{\mathrm{sl}}=V_l-V_s=V_{ls}-V_s=V_{ls}·\left(1-{\displaystyle \frac{V_s}{V_{ls}}}\right)=V_{ls}·\left(1-\xi \right)$$

(6)

where $V_{sl}$ represents the slip velocity; $V_l$ represents the liquid phase velocity; $V_s$ represents the solid velocity; $V_{sl}$ represents the linear velocity of the fluid inside the pipeline; $\xi$ represents the slip ratio, which is the ratio of the slip velocity $V_{sl}$ to the linear velocity $V_{ls}$. The magnitude of the slip ratio is more related to the development of flow patterns between flows in the pipe [18]. Generally speaking, when the slip ratio is close to 1, it means that the velocities of the two phases are close to each other, the relative motion is small, and the closer the flow pattern in the pipeline is to the homogeneous suspension flow; when the slip ratio is less than 1, it means that the velocity of the gas phase or the liquid phase is greater, the relative motion is more obvious, and the closer the flow pattern in the pipeline is to the sedimentary bed type.

In this paper, due to the small overall velocity range and low volume concentration of solids, the velocity of the liquid was approximately taken as the average mixing velocity of the two-phase flow inside the entire pipe.

Moreover, in accordance with practical industrial applications, the particles with a diameter less than 0.2 mm are classified as “fine”, while those with a diameter greater than 0.2 mm are categorized as “coarse”.

2.2. Flow Morphology of Coarse Particle Group in Pipes

Based on the varying flow states of coarse particles within a pipeline [11,18], the flow patterns of coarse particle groups are typically classified into five distinct categories.

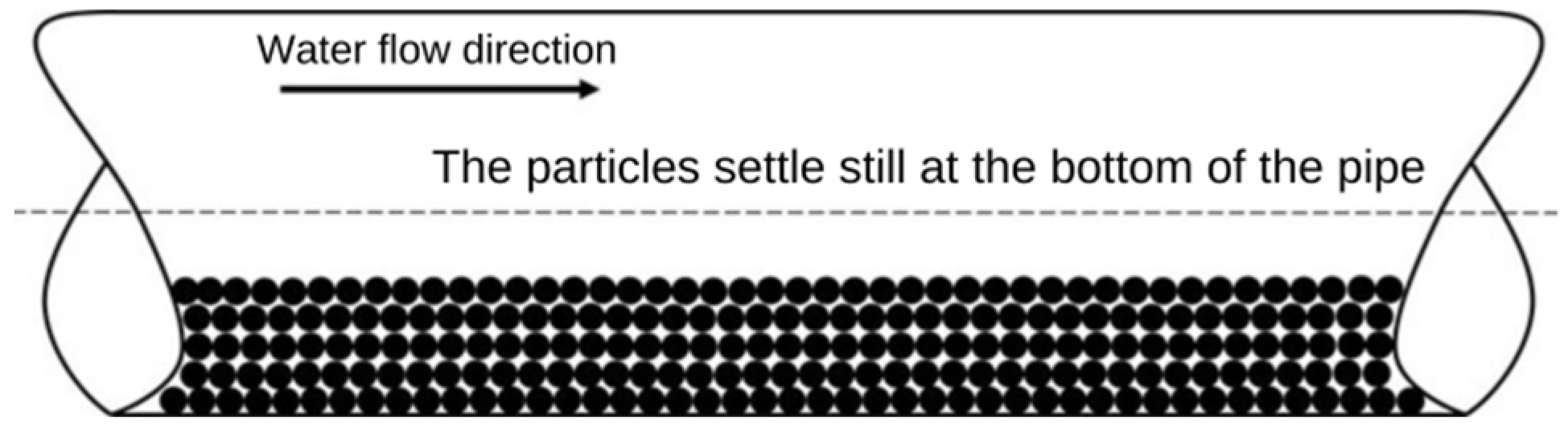
• Sedimentary Bed Type: In this type, particles accumulate statically at the bottom of the pipeline, forming a sedimentary bed, as illustrated in Figure 1.
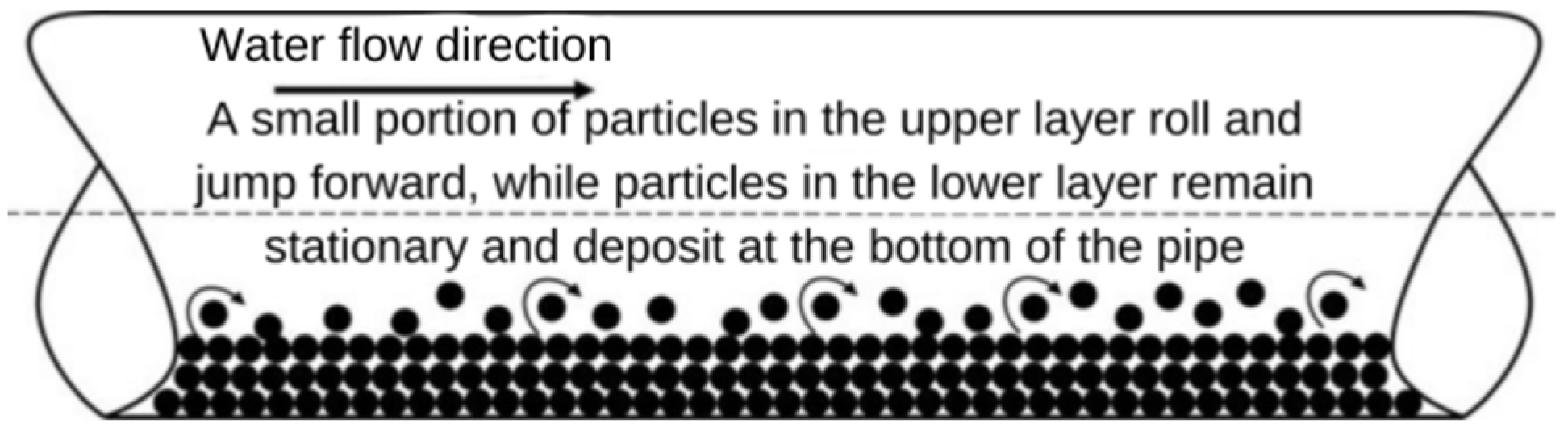
• Sedimentary Bed Type with Jumping/Rolling Particles: Here, some particles detach from the sedimentary bed and move forward by rolling or jumping, as depicted in Figure 2.
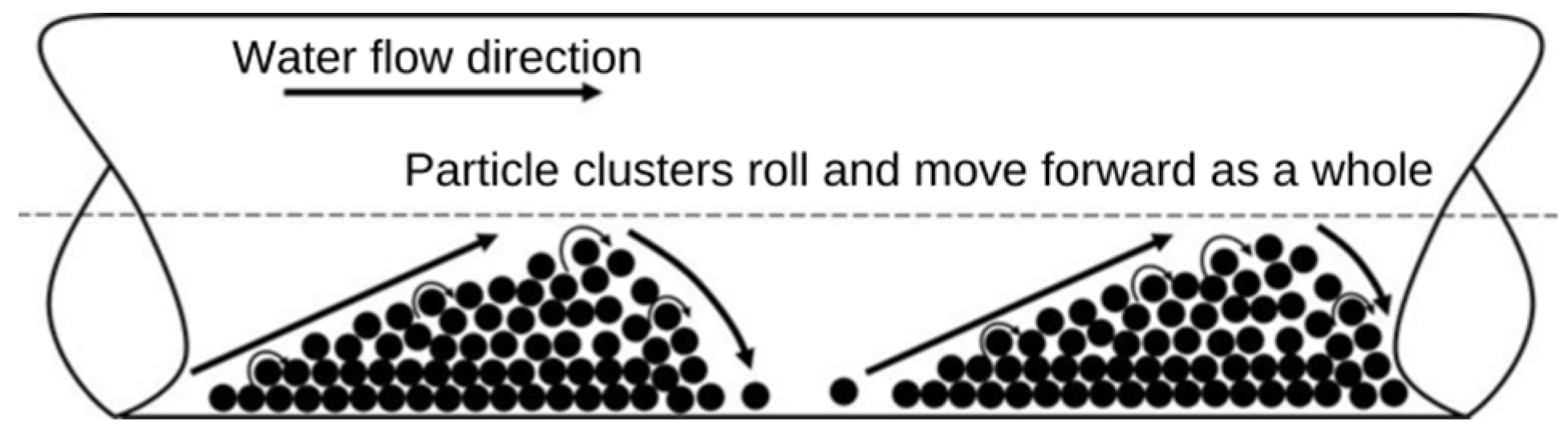
• Nudging Movement: In this category, all particles participate in the two-phase flow movement, with the solid particles’ motion dominated by nudging. This type is further divided into the following:
  • Intermittent Nudging (sand dune): as shown in Figure 3.
  • Continuous Nudging (sliding bed): as shown in Figure 4.
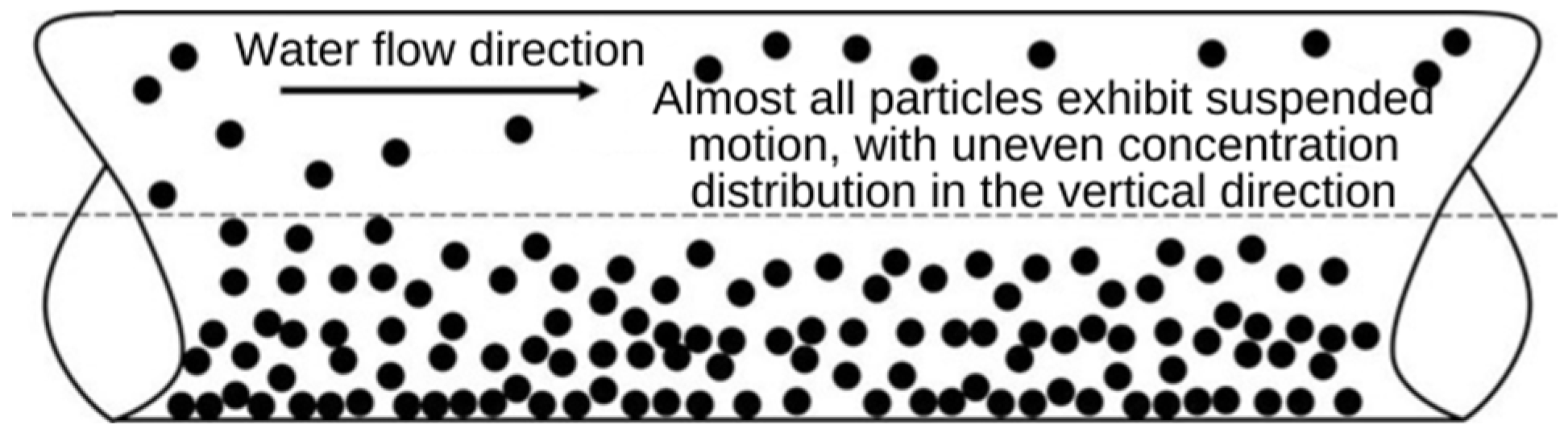
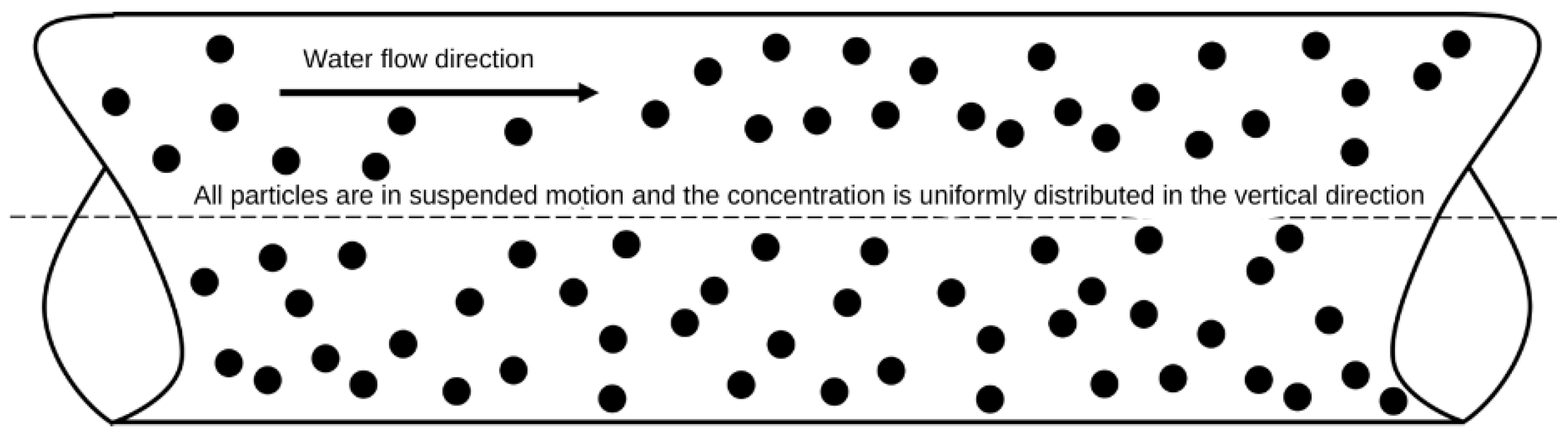
• Non-Homogeneous Flow Suspension Movement: In this state, all solid particles are suspended and moving, but the concentration distribution of particles within the group is non-uniform. The fluid is treated as a non-Newtonian fluid, as illustrated in Figure 5.
• Pseudo-Homogeneous Flow Suspension Movement: Here, all particles are suspended and moving, with a relatively uniform concentration distribution. The two-phase flow fluid is considered a single fluid, behaving as a Newtonian fluid, as shown in Figure 6.

These classifications help in understanding and analyzing the complex dynamics of coarse particle groups in pipeline flows, providing a framework for optimizing transport efficiency and stability in dredging and similar operations.

Figure 1. Schematic diagram of sedimentary bed type.

Figure 1. Schematic diagram of sedimentary bed type.

Figure 2. Schematic diagram of sedimentary bed with jumping and rolling particles.

Figure 2. Schematic diagram of sedimentary bed with jumping and rolling particles.

Figure 3. Schematic diagram of intermittent pushing (sand dune) movement flow pattern.

Figure 3. Schematic diagram of intermittent pushing (sand dune) movement flow pattern.

Figure 4. Schematic diagram of continuous sliding bed motion flow pattern.

Figure 4. Schematic diagram of continuous sliding bed motion flow pattern.

Figure 5. Schematic diagram of non-uniform flow suspended motion flow pattern.

Figure 5. Schematic diagram of non-uniform flow suspended motion flow pattern.

Figure 6. Schematic diagram of the flow pattern of pseudo-homogeneous flow suspension motion.

Figure 6. Schematic diagram of the flow pattern of pseudo-homogeneous flow suspension motion.

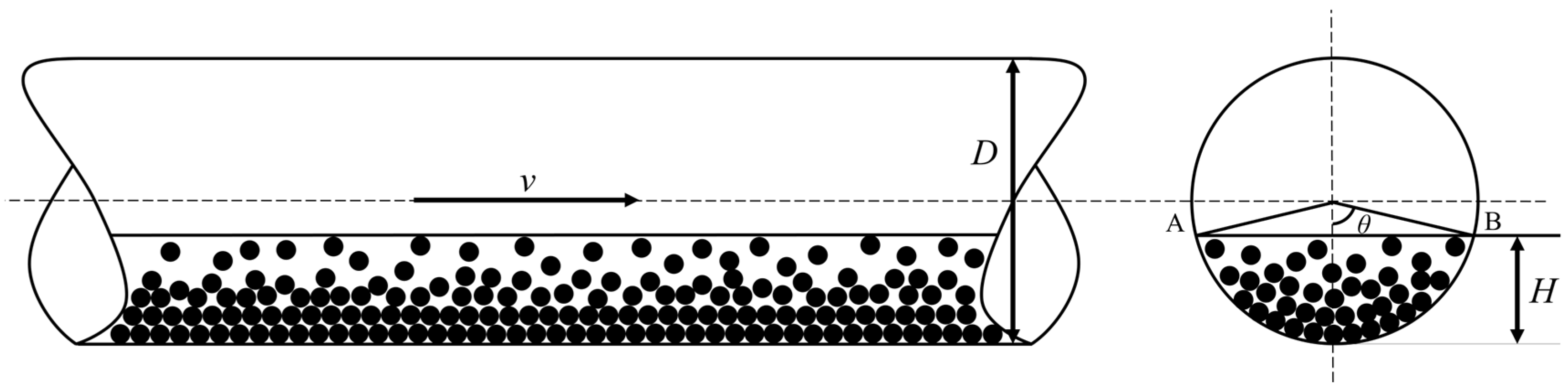
2.3. Granular Beds

After a series of images were captured using a high-speed camera, the images were analyzed frame by frame with the aid of particle image velocimetry (PIV) [19,20] to measure and record the heights of the tubes containing different types of moving particle beds. Simultaneously, the movement state of the coarse particle population in the tubes was determined by observing the particle dynamics in the experiments.

For a circular pipe, the in-pipe heights of particle layers with different motion types do not intuitively reflect the overall flow behavior of the coarse particle population [21]. By applying the geometric proportionality relationship within the pipe, the percentage of the cross-sectional area occupied by particle layers of different motion states was calculated, illustrating the impact of varying in-pipe particle bed states on the flow pattern.

Figure 7 presents a schematic diagram for calculating the cross-sectional area of the granular layer in the pipe. The granular layer’s cross-sectional area is determined using the height of the granular layer in the pipe and the pipe diameter, as shown in Equation (7).

$$S=\left\{\begin{array}{cc}{\mathit{cos}}^{-1}\left({\displaystyle \frac{r-H}{r}}\right)\cdot r^2-(r-H)\cdot \sqrt{(r^2-(r-H{)}^2{)}^2}& H\le r\\ (\pi -{\mathit{cos}}^{-1}\left({\displaystyle \frac{H-r}{r}}\right))\cdot r^2+(H-r)\cdot \sqrt{(r^2-(H-r{)}^2{)}^2}& H\ge r\end{array}\right.$$

(7)

where $r$ is the inner diameter of the pipe, $r$ = $D/2$, and $H$ is the height of the pipe in the granular layer.

The following is an example of analytical extraction for each bed height for coarse particles with a particle diameter of 0.9 mm (average mixing velocity of $V_m$ = 0.76 m/s and material volume concentration $C_v$ = 15%).

The motion state of the particle bed layer can be divided into a stationary deposition layer, a nudging motion layer, and a suspension motion layer; the clear water layer is represented by a blank area; the motion state of the particles and the bed layer is compared frame by frame with the image acquired using a high-speed camera to determine the bed height of each bed layer; and subsequently, the obtained height of each bed layer is integrated into Equation (7) so as to obtain the percentage of the pipeline cross-sectional area that is occupied by the corresponding motion state of the coarse particles. This is used to illustrate the effect of each type of particle bed on the flow pattern of the coarse particle population.

3. Experimental Preparation

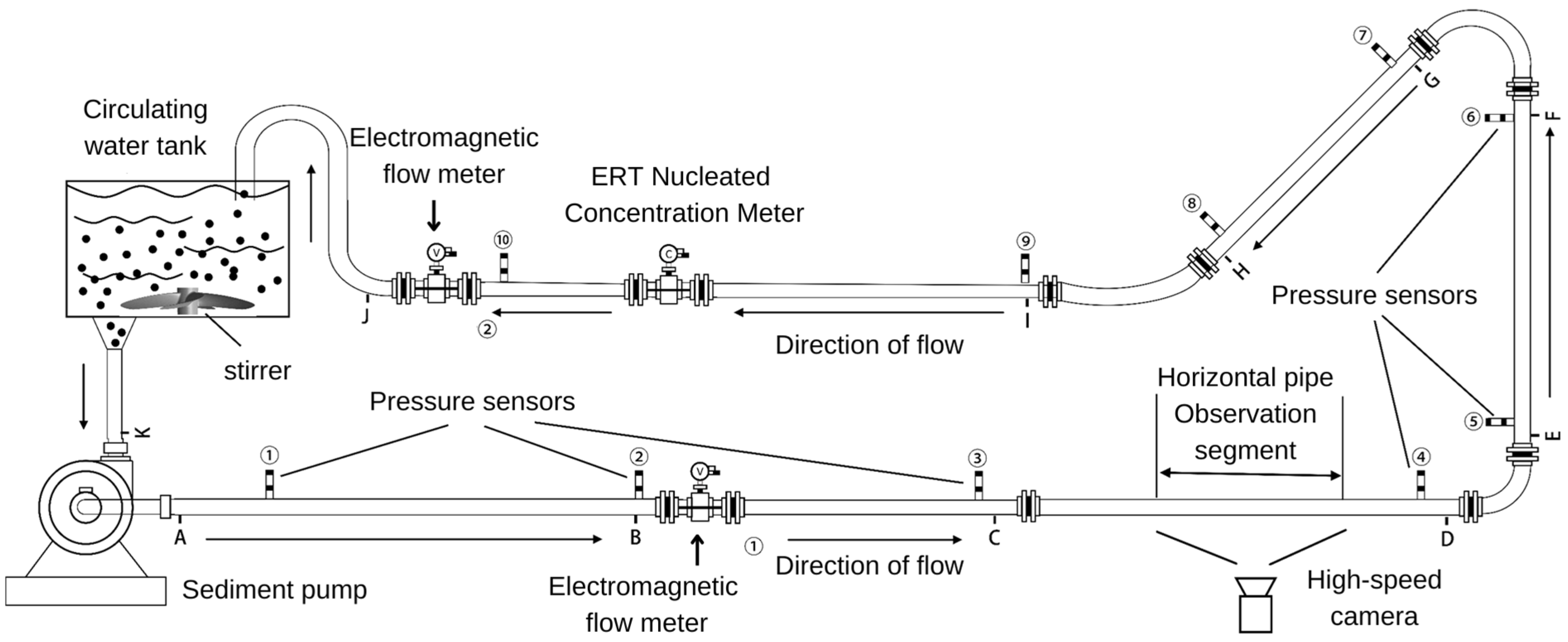
3.1. Experiment Setup

The experimental material is a slurry consisting of three different grain sizes of quartz sand and water, and its physical properties are similar to those of dredged material. The density of the quartz sand was 2650 kg/m³, and the average grain size was 0.9, 1.5, and 2.5 mm, respectively.

The main equipment for the experiments were circulation pipes, power equipment, data monitoring equipment, and filming equipment. As shown in Figure 8, this experiment included a circulating pipeline system that simulates sediment pipeline transportation. The system is mainly divided into a circulating water tank, an initial development section (AB), a fully developed section (BC), a horizontal observation section (CD), a vertical observation section (EF), an inclined observation section (GH), a reflux section (IJ), and an outlet section (JK). The pipeline circulation system is provided with the kinetic energy required for the movement inside the pipeline by the sediment pump. After the sediment pump is started, the mixture of sediment and water in the sediment tank enters the initial development stage AB; after a certain distance of flow development, it enters the fully developed stage (BC), at which point the water phase in the pipeline reached laminar flow state. Subsequently, it enters the horizontal observation section (CD), vertical observation section (EF), and inclined observation section (GH) in sequence, which can be used to physically observe the movement state of sediment particles inside the pipe. Finally, it enters the reflux section (IK) and the outlet section (JK) to return to the mud and sand tank, completing the circulation of the entire circulation pipeline system.

As shown in Figure 8, the main structural dimensions of the circulating pipeline system are a total length of 24.6 m, an initial development section + outlet section (AB + JK) with a total length of 3.5 + 5.5 = 9 m, a fully developed section (BC) with a length of 1.8 m, a horizontal observation section (CD) with a length of 3.1 m, a vertical observation section (EF) with a length of 1.6 m, an inclined observation section (GH) with a length of 1.8 m, a reflux section (IJ) with a length of 3.5 m, and other bent pipe sections with a total length of 2.4 m.

Among them, the pipes are all made of transparent polymer polyvinyl chloride (PVC) material with an inner diameter of 45 mm, which has the advantages of easy observation and high strength.

The power equipment used in this experiment is a variable frequency-controlled sediment pump, and the mixing device is a skewed blade slurry mixer. The specifications of the sediment pump are flow rate of 40 m³/h, head of 12 m, power of 4 kW, and speed of 1480 r/min. The three-phase motor model matched with the sediment pump is G-112B, power of 80 W, speed of 2550 r/min, and air volume of 229 m³/h. The mixer used is the BLD-10 mixer produced by Jiangsu Yingma Transmission Equipment Co., Ltd. (Changzhou, China). Its specifications are mixer power of 0.75 kW, speed of 850 rpm, mixing rod length of 600 mm, impeller diameter of 300 mm, and double-bladed wheel mixing.

The monitoring parameters of this experiment include pipeline pressure, flow rate, concentration, etc. Pressure sensor using xingyi Sensor Manufacturing Co., Ltd. (langfang, China).production CYYZ51A-H-12-A1-14-B-G flat membrane pressure transmitter, used to monitor the real-time pressure value of the pipeline. The specifications of the pressure transmitter are range of 0~1 MPa, output of 4~20 mA, and connection of M20×1.5. Accuracy level is 0.25; power supply is 9~36 VDC. The electromagnetic flowmeter is a KEFT-type electromagnetic flowmeter produced by Shanghai Kent Instrument Co., Ltd. (Shanghai, China). It is installed in the inlet in fully developed section and outlet section of the circulation system to monitor the mixture flow velocity, Vm, in the pipeline, as shown in Figure 8. Its specification parameters are power supply of 220 V/24 V, (4~20) mA current output, pulse output, RS-485 (custom protocol), RS-485 (ModBus protocol), error of ±0.5% R (V ≥ 0.5 m/s), ±2.5 mm/s (V < 0.5 m/s), and pipeline diameter of DN45.

The ERT nuclear-free concentration meter is used to measure the volume concentration of a constant volume inside a pipeline using the CWLL ERT concentration meter produced by Wuhan Green Forest System Technology Co., Ltd. (Wuhan, China).

The filming equipment used is the SA-Z high-speed camera (Photron, Yonezawa City, Japan), mainly used to capture the flow pattern of sediment in the pipeline during the experimental process. The photos are saved every 0.01 s during the filming process. The specifications are as follows: pixel size of 1024 × 1024, shooting frame rate of 5000 fps, shortest exposure time of 159 nsec, pixel size of 20 µm.

3.2. Design of Experiments

This experiment uses sensors such as pressure and flow rate, as well as high-speed cameras, to study the motion characteristics of coarse particle groups. Through macroscopic experimental phenomena, different coarse particle flow patterns in the pipe are defined. With the help of particle image velocimetry (PIV) technology, the influence of factors such as mixed average flow rate, material volume concentration, and particle size on the transition speed of coarse particle flow patterns was analyzed and statistically analyzed.

The experiments were carried out using the experimental sand prepared in advance as the solid phase. Its density = 2650 kg/m³, with water as the liquid phase, in which the quartz sand has three groups of average particle size, 0.9 mm, 1.5 mm, and 2.5 mm, each group of particle size materials for the three concentrations of 10%, 15%, and 20% for the experiments. The groups of experimental initial conditions are shown in

Table 1. Experimental conditions for hydraulic transportation of coarse particle pipelines.

Experimental Group Number	Average Particle Size d (mm)	Feed Concentration Cv	Range of Average Flow Velocity Variation Vm (m/s)
1	0.9	10%	0.49~4.00
2	0.9	15%	0.49~4.00
3	0.9	20%	0.49~4.00
4	1.5	10%	0.56~4.00
5	1.5	15%	0.56~4.00
6	1.5	20%	0.56~4.00
7	2.5	10%	0.64~4.00
8	2.5	15%	0.64~4.00
9	2.5	20%	0.64~4.00

.

(1)

Pre-Experiment Preparation Stage.

Before the official operation of the experimental equipment, there is need to perform the main preparatory work: (1) Establish the initial parameters of the sensor calibration—in the circulating water tank filled with water, start the sediment pump, which is stabilized after the initial value of the sensor is set to 0; (2) Establish the initial parameters of the sensor calibration—a certain volume of the corresponding particle size of the quartz sand is loaded into the circulating water tank. Refill the water to start the stirrer to fully mix the samples to measure the concentration and modulation to the target concentration; (3) Set up a good high-speed camera shooting position, control the length of the pipe in the shooting screen for 400 mm, according to the actual light intensity to adjust the camera’s exposure. Set the high-speed camera shooting frequency to 5000 fps, that is, every 0.01 s, to maintain a frame of coarse particles in the group of motion images.

(2)

Equipment operation stage.

Set the rotational speed of the sediment pump in accordance with the experimental program; control the flow rate in the pipe to reach the preset value and gradually increase the flow rate in the pipe, so that the flow of the two-phase flow in the pipe is a continuous change, and ensure that the flow of the two-phase flow in the pipe reaches a stable level before increasing the flow rate. The macroscopic experimental phenomena and the speed at which the coarse particle group appears to have obvious flow transformation are recorded and counted in the experimental process.

(3)

Experimental data collection and screening.

After the system is stabilized, the upper computer of the control platform is used to record the experimental data of each sensor for a period of time during the stable operation. In addition, due to the limited saving capacity of the high-speed camera, it is necessary to wait until the system is stabilized to record the shooting of motion images, shooting the flow in the pipe for a period of time during the stabilization phase of the system, and saving a picture every 0.01 s. The system can be used to record the flow in the pipe for a period of time. Since there is a certain randomness in the motion state of coarse particles during the pipeline conveying process, it is necessary to filter and reject the relevant data and images accordingly after exporting the experimental data and images.

4. Results and Discussion

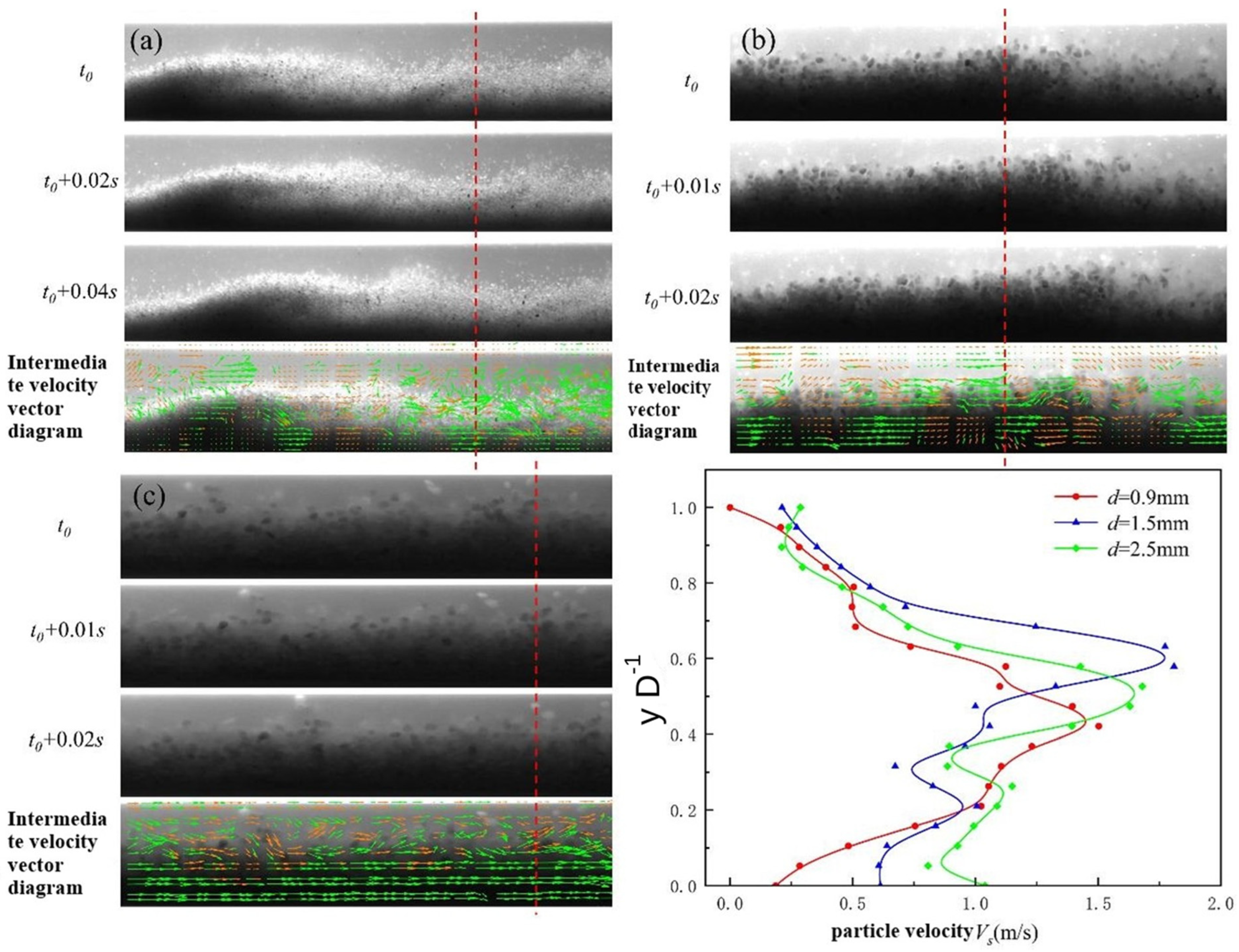
4.1. Velocity Distribution Characteristics of Different Coarse Particle Group Flow Patterns

Section 2.2 describes the five flow regimes in detail; however, during the experimental process, the flow patterns primarily manifested as the sedimentation bed type with jumping rolling particles, displacement motion, and heterogeneous flow suspension motion state. The sedimentation bed type and pseudo-homogeneous flow suspension motion state were found to be extreme and less representative. Therefore, the following analysis focuses on summarizing the flow patterns of the coarse particle group in the three intermediate states mentioned above.

(1)

Sedimentary bed type with jumping and rolling particles

Figure 9a–c present schematic diagrams illustrating the velocity distribution extraction process for three different particle sizes, with the velocity distribution diagrams for the three types of particles displayed in the lower right corner. Under this flow pattern, a sedimentary bed of coarse particles forms within the pipeline. Particles near the centerline of the pipeline exhibit flipping, jumping, and rolling motions. The coarse particles do not fully occupy the entire pipeline, and for the majority of the time, there is no particle movement in the upper section of the pipeline. The lower part of the pipeline consists of areas where coarse particles are deposited, with particle velocities approaching zero. The maximum particle velocity is observed near and just below the centerline of the pipeline.

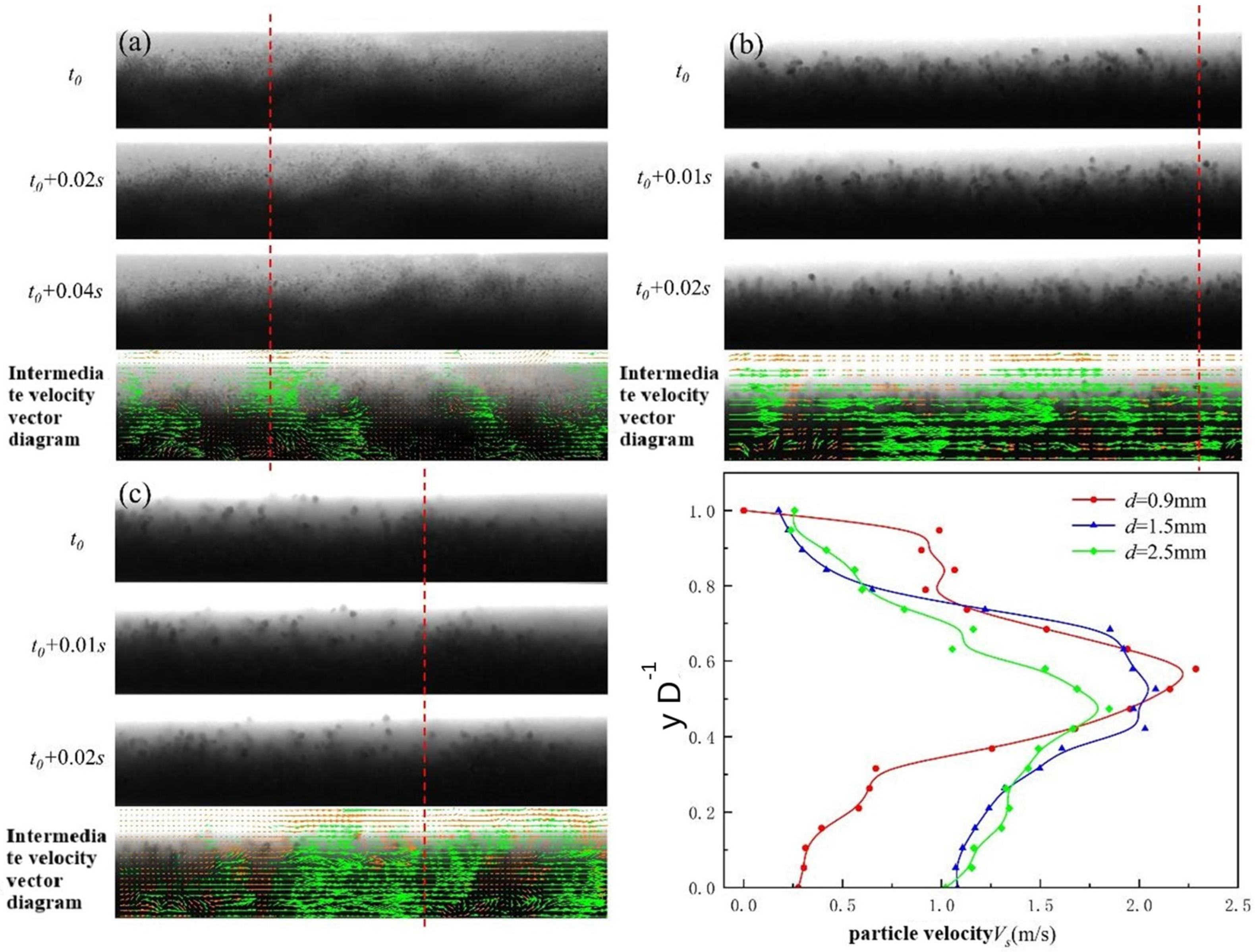
(2)

Moving Movement

Figure 10a–c illustrate schematic diagrams of the velocity distribution extraction process for three different particle sizes exhibiting translational motion. Under this flow pattern, the lower section of the pipeline and the coarse particles near the centerline experience tight pushing motion, although the velocity of the coarse particles at the centerline is higher than that at the bottom. A certain amount of coarse particle suspension motion occurs in the upper part of the pipeline, where particle movement is faster and the distribution of coarse particles is more uniform compared to the previous flow pattern. However, a no-particle zone remains at the top. Observing the velocity distribution map, it is evident that the velocity in the upper half of the pipeline rapidly decreases, approaching zero, while in the lower half, the particle velocity fluctuates and does not reach zero. The highest velocity is located at the centerline, and the velocity distribution follows a logarithmic profile.

(3)

Heterogeneous flow suspension motion

Figure 11a–c show schematic diagrams of the velocity distribution extraction process for three different particle sizes exhibiting heterogeneous flow suspension motion. In this flow pattern, the upper section of the pipeline is primarily characterized by suspended motion, with coarse particles almost filling the entire pipeline. The maximum particle velocity remains concentrated near the centerline of the pipeline, and the velocity distribution characteristics are similar to those observed in the bed type of translational motion. However, with an increase in velocity, the proportion of coarse particles also increases, causing the high-speed particle region near the centerline to expand, resulting in a higher overall flow velocity. The highest particle velocity is observed in the central area of the pipeline, while the velocity of the coarse particles in the upper and lower halves of the pipeline gradually decreases as the distance from the centerline increases.

By comprehensively comparing the characteristics of the three types of coarse particle flow patterns mentioned above, the reasons for their occurrence are analyzed from the perspectives of particle forces, collisions, and the influence of water flow on the coarse particles.

Influence of the boundary layer near the pipe wall: the logarithmic velocity distribution of coarse particles is primarily influenced by the boundary layer near the pipe wall. Fluid molecules in this boundary layer are affected by the wall, and coarse particles, driven by the inertia of the water flow, tend to align with the velocity distribution characteristics of the water. Simultaneously, the peak particle velocity is slightly shifted downward due to the influence of the particles’ own weight.

The balance between the lifting force provided by the water flow and the gravity of the particles plays a crucial role in determining particle behavior: the direction of the drag force exerted by the water flow on coarse particles is relatively random. As the water flow speed increases and the drag force becomes stronger, coarse particles are more likely to be lifted by the water flow, leading to a more even dispersion of particles throughout the pipe. This reduction in collision and friction allows coarse particles to more easily suspend or move.

The degree of collision and friction between coarse particles varies across different flow patterns: in the sedimentation bed flow pattern, the collision and friction between particles are at their highest, which weakens the influence of the water flow drag force and facilitates particle settling. In contrast, suspended coarse particles are less affected by particle collision friction and only need to maintain a balance between their own gravity and the upward force created by the water flow drag force in order to sustain their motion state.

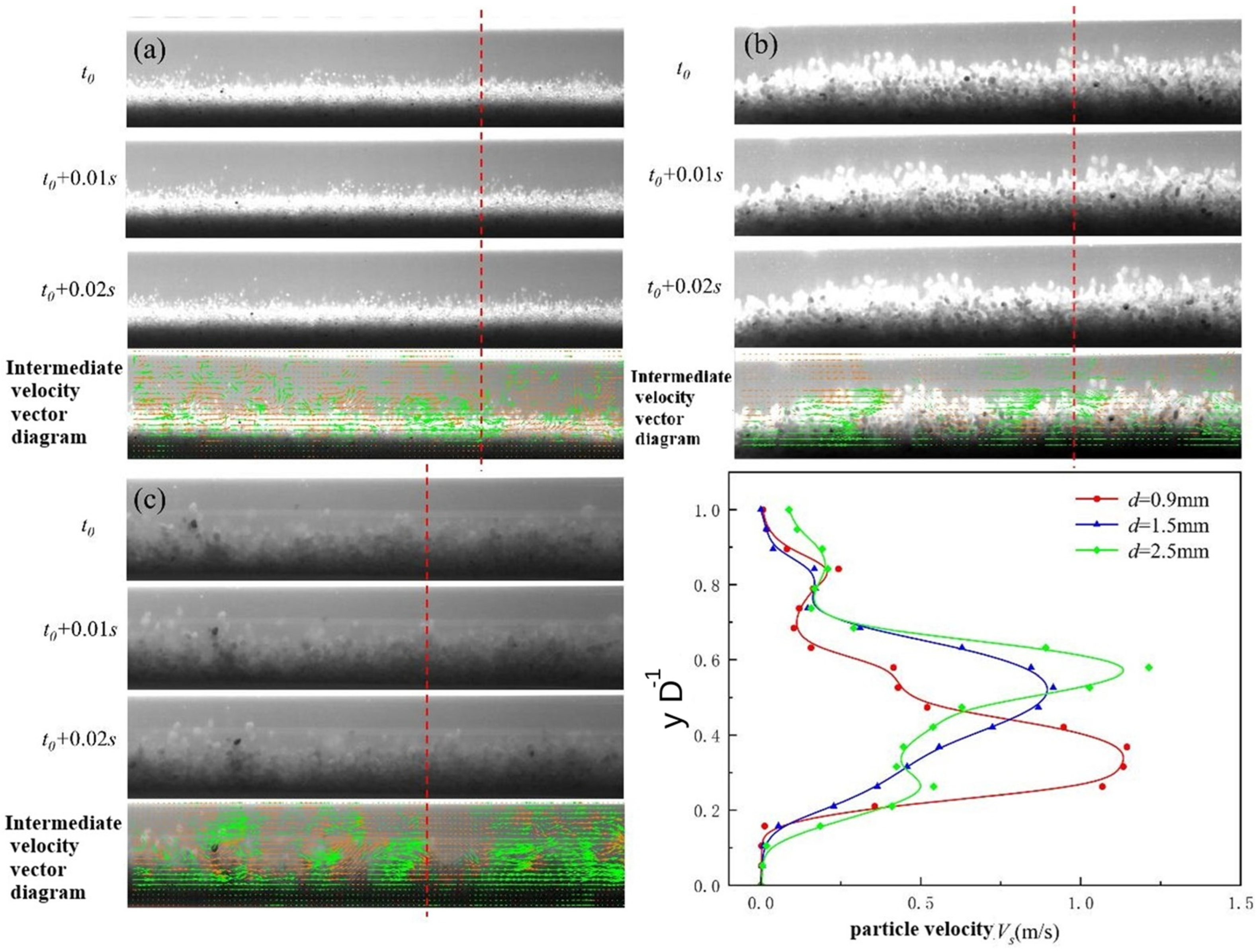
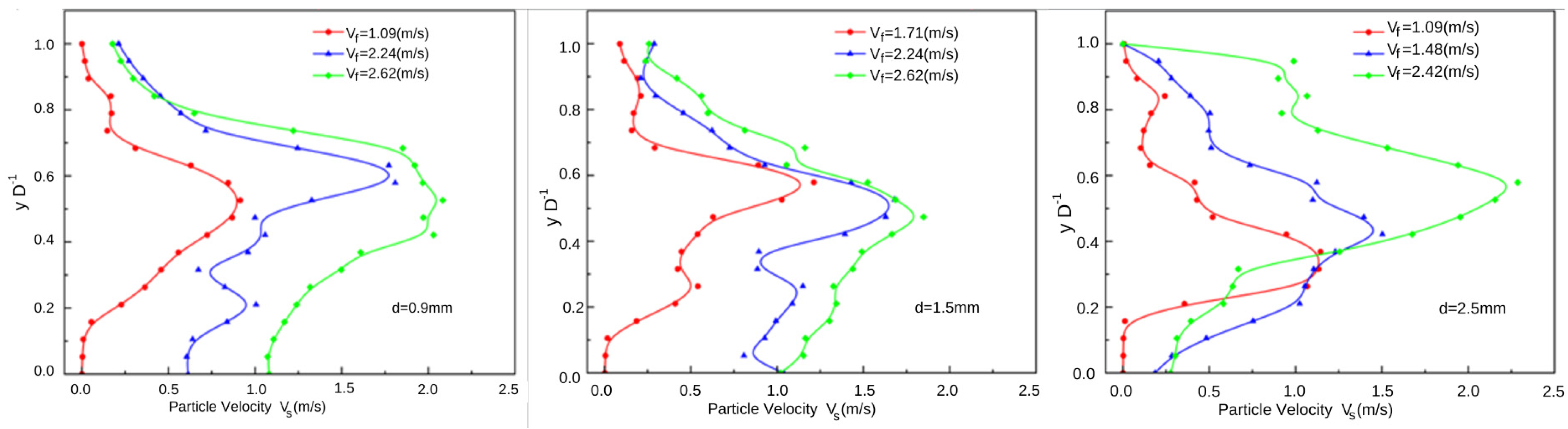
4.2. The Influence of Particle Diameter on the Velocity Distribution of Coarse Particle Groups

As shown in Figure 12, it can be observed that for coarse particle groups of the same size, an increase in water flow velocity leads to a continuous increase in both the overall velocity and the maximum velocity of the coarse particles. Simultaneously, the particle groups with higher velocities near the centerline of the pipeline become more pronounced. Notably, the 0.9 mm coarse particle exhibits a unique behavior: as the water flow velocity increases, the maximum velocity of the coarse particles shifts vertically, a phenomenon not observed in the two larger coarse particle groups.

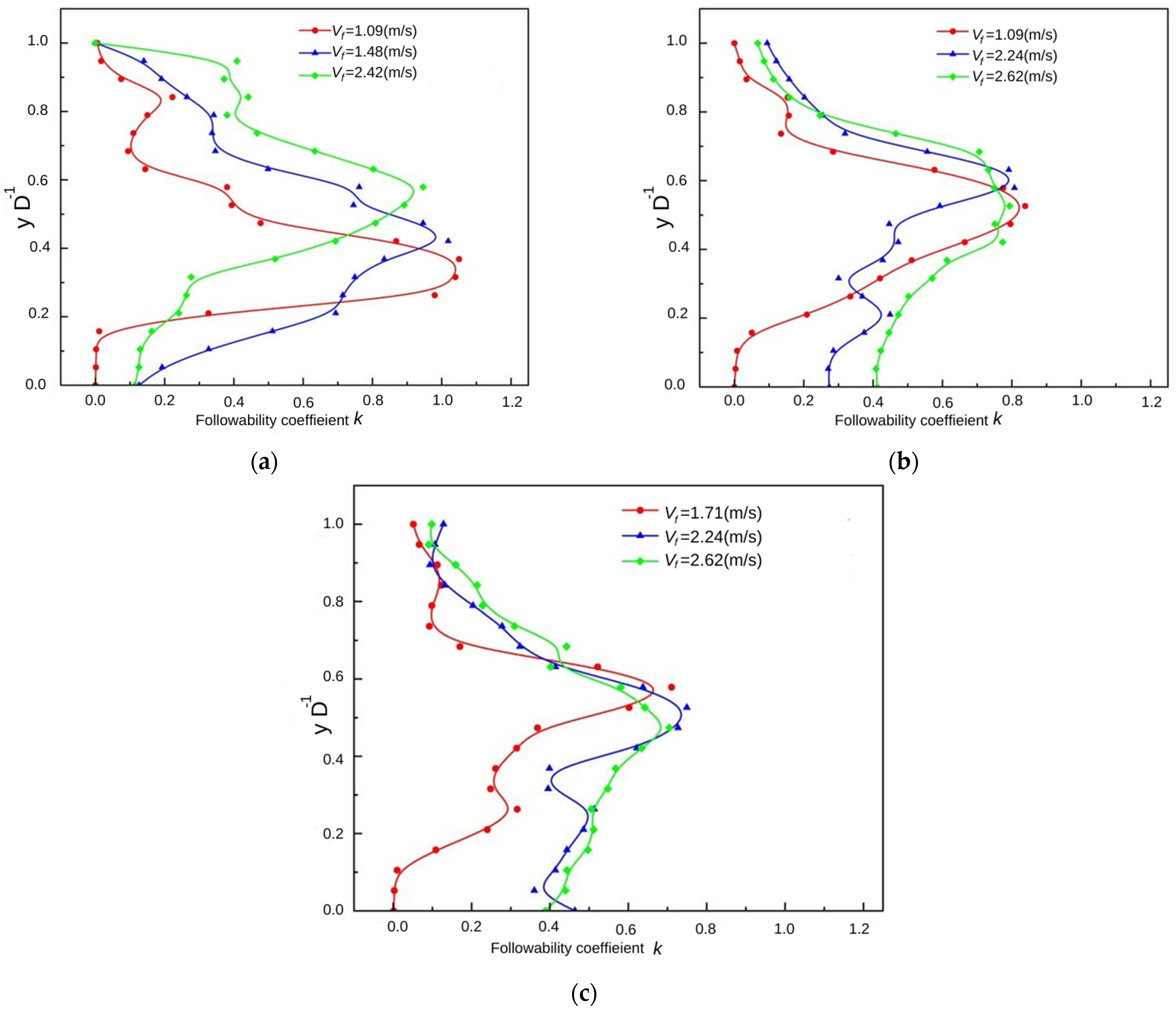
4.3. Analysis of Coarse Particle Flow Characteristics

In order to better analyze and compare the followability of coarse particles with water flow, a followability coefficient kk is introduced to characterize the followability characteristics of coarse particles in relation to water flow. The followability coefficient of particles is defined as the ratio of particle flow velocity to clean water flow velocity, as shown in Equation (7).

$$k={\displaystyle \frac{V_s}{V_l}}$$

(8)

where k represents the particle followability coefficient, $V_s$ represents the particle flow rate, and $V_l$ represents the clear water flow rate.

By using Equation (7) and the previous data, the following relationships of three different particle sizes of coarse particles with water flow can be obtained, as shown in Figure 13. Among them, the horizontal axis of the coordinate axis represents the particle’s followability coefficient k, and the vertical axis of the coordinate axis represents the ratio of the actual height in the vertical direction inside the pipe to the pipe diameter $y·D^{-1}$.

As shown in Figure 13, for coarse particles with a diameter of 0.9 mm, the increase in water flow velocity causes the maximum velocity of the particles to shift vertically, indicating that as the water flow velocity increases, the particles are gradually lifted by the water flow. For coarse particles with diameters of 1.5 mm and 2.5 mm, the maximum velocity of the particles consistently occurs near the centerline of the pipeline. The smaller the particle size, the larger both the maximum and overall values of the particle’s followability coefficient kk. Additionally, the overall span of the followability coefficient kk for coarse particles with a diameter of 0.9 mm is much larger than that for coarse particles with a diameter of 2.5 mm. This suggests that the followability of particles varies significantly across different areas of the pipeline, with particles near the centerline being more easily accelerated by the water flow.

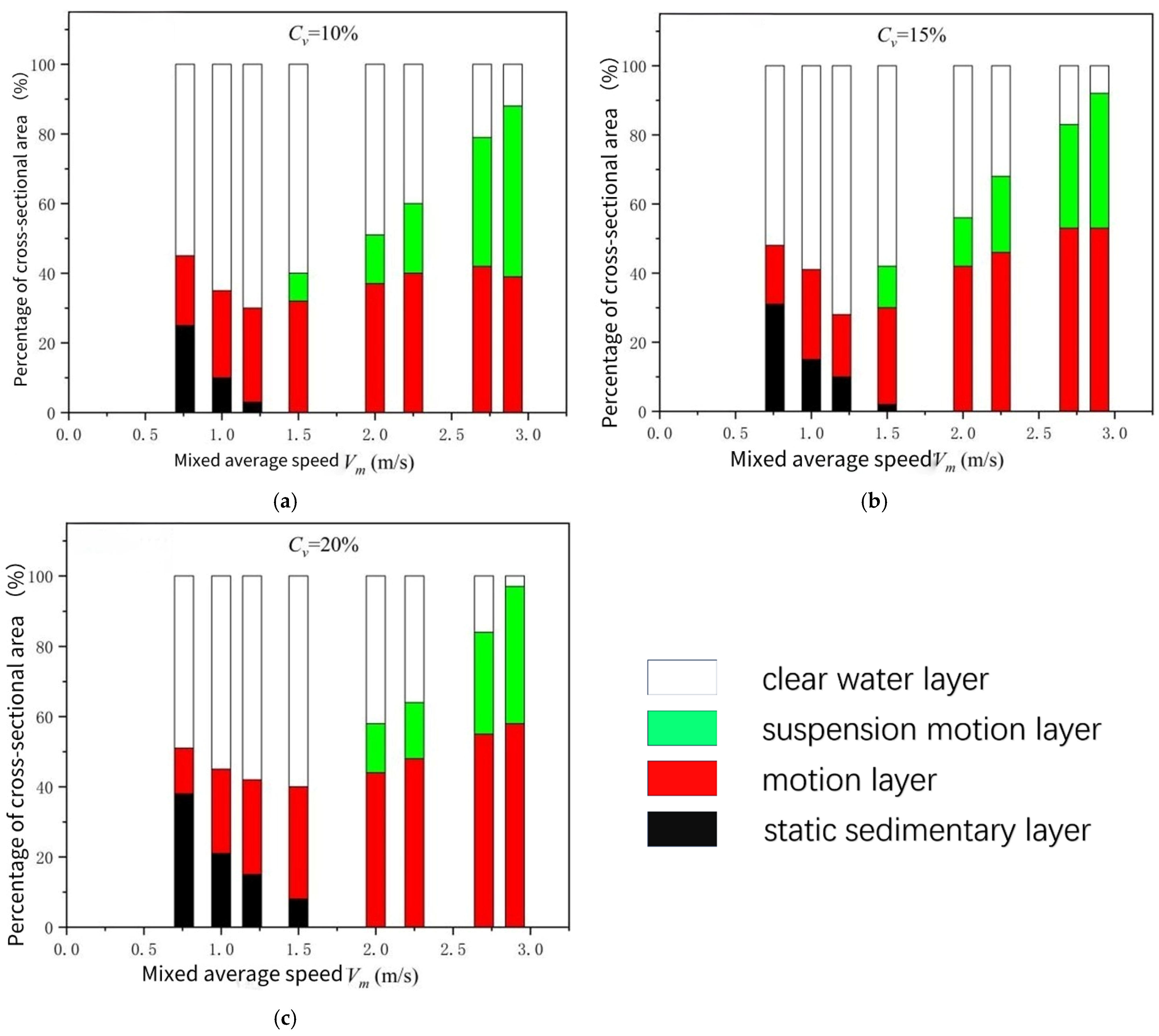
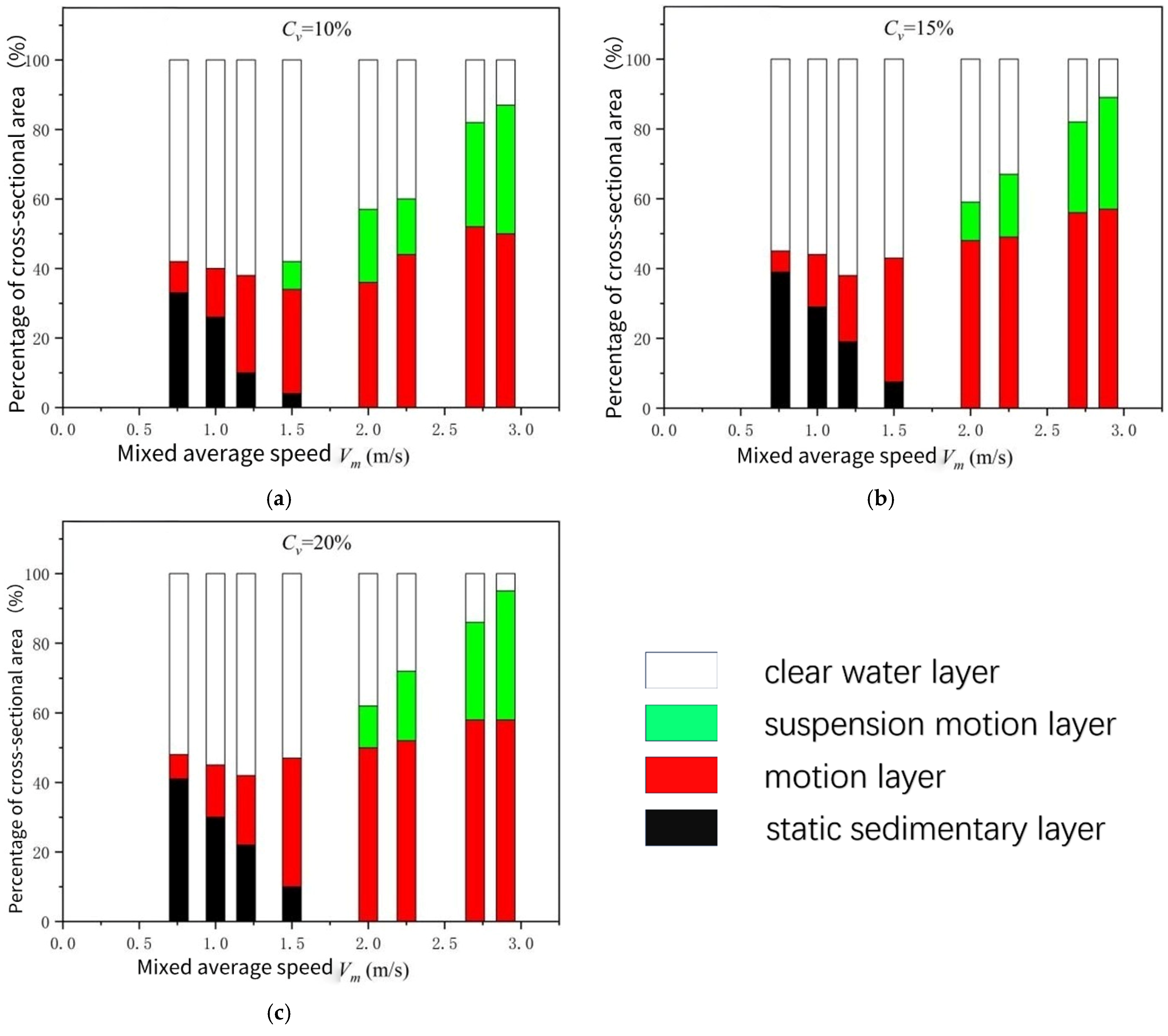
4.4. The Influence of Particle Bed Types on the Flow Pattern of Coarse Particle Clusters in Various Motion States

The following text summarizes the percentage distribution of each moving particle layer within the pipeline cross-sectional area for three distinct particle sizes, analyzed under varying material volume concentrations and mixed average velocities, as depicted in Figure 14, Figure 15 and Figure 16. In these figures, the particle bed layers corresponding to different coarse particle motion states are illustrated using differently colored bar graphs. The horizontal axis denotes the mixed average velocity of the coarse particle group, while the vertical axis represents the percentage of the particle bed layer relative to the pipeline cross-sectional area.

As shown in Figure 14, for particles with a diameter of 0.9 mm, a stationary deposition layer is present at low mixed average velocities. As the material volume concentration increases, the proportion of the stationary deposition layer also rises, with no suspending movement layer observed. When the velocity reaches 1.5 m/s, the suspending movement layer begins to emerge. As the velocity continues to increase, the proportion of the suspending movement layer also grows.

As shown in Figure 15, for particles with a diameter of 1.5 mm, the stationary deposition layer occupies a substantial proportion at low mixed average velocities. Even when the velocity reaches 1.5 m/s, a stationary deposition layer persists at a material volume concentration of 15% in the two-phase flow. With further increases in the mixed average velocity, the proportions of both the nudging motion layer and the suspension motion layer gradually increase.

As illustrated in Figure 16, for particles with a diameter of 2.5 mm, a stationary sedimentary layer persists when the mixed average velocity is below 1.5 m/s. When the velocity reaches 2.0 m/s, the proportion of the clear-water layer drops below 50%, and the transporting movement layer becomes dominant. As the mixed average velocity increases further, the proportions of both the transporting movement layer and the suspending movement layer rise, while the clear-water layer proportion decreases.

By analyzing the image, it can be observed that an increase in the material volume concentration and a change in particle size mostly result in an increase in the cross-sectional area of the stationary sedimentary layer in the low-speed region (V_m < 1.75 m/s). At this point, the average mixing velocity required for the coarse particle group to enter the translational flow pattern is greater, and the transition of the coarse particle group flow pattern is delayed. The proportion of the cross-sectional area of the suspended motion layer for the three particle sizes increases with the increase in the average mixing velocity, but the increase in the cross-sectional area of the 0.9 mm coarse particle is more significant. In the high-speed region (V_m > 2.5 m/s), the proportion of the cross-sectional area of the displacement motion layer for the 0.9 mm coarse particle decreases with the increase in the average mixing velocity, but the proportion of the cross-sectional area of the displacement motion layer for the other two large particle sizes is relatively stable. Reflected in the transition of the coarse particle group flow pattern, it can be found that the coarse particle group flow pattern with a diameter of 0.9 mm can quickly complete the transition from a translational motion flow pattern to a non-homogeneous flow suspension motion flow pattern, while the other two types of coarse particle group flow patterns with larger particle sizes still mainly rely on translational motion.

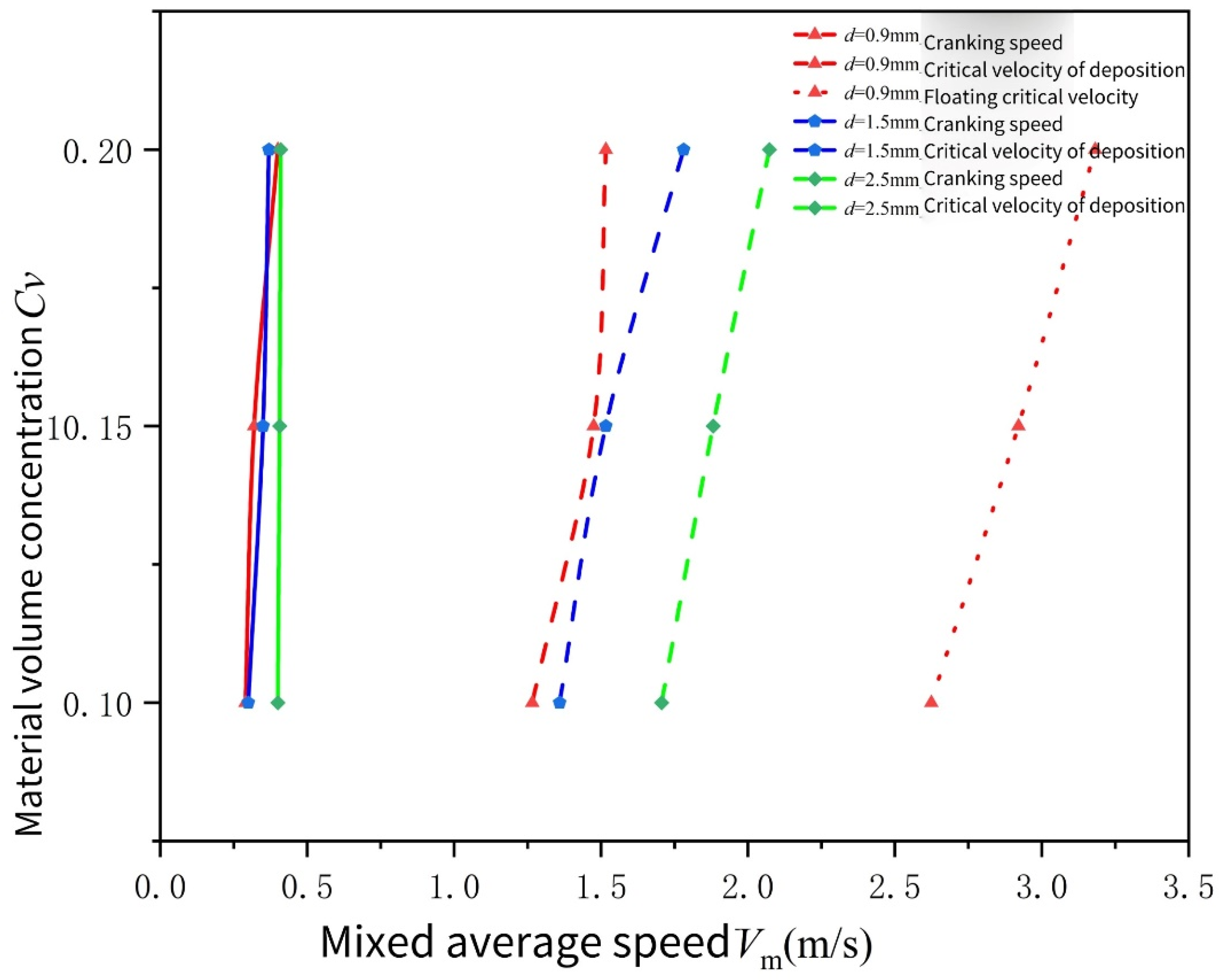
4.5. Statistical Analysis of Coarse Particle Flow Transitions

By summarizing and analyzing the motion characteristics of particle layers in different motion states and observing and recording phenomena in experiments, the various velocities at which coarse particles undergo motion state transitions under different particle sizes (0.9 mm, 1.5 mm, and 2.5 mm) and material volume concentrations (10%, 15%, and 20%) were summarized, as shown in Figure 17. The horizontal coordinate of the graph indicates the average speed of mixing, and the vertical coordinate indicates the material volume concentration. The red line is the velocity profile of each kinematic state transition for a particle size of 0.9 mm, the blue line is the velocity profile of each kinematic state transition for a particle size of 1.5 mm, and the green line is the velocity profile of each kinematic state transition for a particle size of 2.5 mm.

The starting speed V_cd, sedimentation critical speed V_d, and floating critical speed V_B, respectively, indicate the speed at which particles in the pipeline begin to move, the speed at which all particles in the pipeline begin to move without stationary sedimentation particles, and the speed at which coarse particles exceeding 50% of the pipeline section begin to suspend, corresponding to the transition of the coarse particle group flow pattern from a sedimentation bed type to a sedimentation bed type with jumping and rolling particles, and then to translational and heterogeneous flow suspension flow patterns. Due to the limitation of the velocity range during the experiment, particles with diameters of 1.5 mm and 2.5 mm did not reach the critical velocity for floating, and therefore are not shown in Figure 17.

It can be observed that the range of particle starting velocity variation is relatively narrow, consistently below 0.5 m/s, while the range of sedimentation critical velocity variation is considerably wider. The sedimentation critical velocity is highly sensitive to changes in particle diameter and material volume concentration. Specifically, larger particle diameters and higher concentrations result in greater sedimentation critical velocities for the coarse particle group, necessitating higher speeds for the transition of the coarse particle group flow pattern to translational motion. For a 0.9 mm coarse particle group, the critical velocity of planktonic particles is significantly higher, exceeding 2.5 m/s, and it increases linearly with rising material volume concentration, demonstrating a strong linear correlation.

The above phenomenon indicates that the initial conditions of particle motion are not significantly influenced by the particle diameter and material volume concentration, whereas the mixed average flow rate has a more pronounced effect on particle initiation. The critical sedimentation velocity exhibits a linear correlation with both the material volume concentration and particle diameter. Specifically, larger particle diameters and higher material volume concentrations make it more challenging for the coarse particle group to transition from a sedimentation bed with jumping and rolling particles to translational motion. Within the experimental scope of this study, only the 0.9 mm coarse particle group achieved the transition to a non-homogeneous flow suspension motion flow pattern, with its critical floating velocity demonstrating a clear linear relationship with the material volume concentration.

5. Conclusions

This paper takes the pipeline hydraulic conveying of dredged material in the field of dredging construction as the research and application background, adopts the method of experimental research, designs and builds the experimental platform of horizontal pipeline hydraulic conveying of coarse particles on its own, and analyzes the effects of the particle diameter and material volume concentration on the velocity distribution of the coarse particle group flow pattern and the particle bed by combining the experimental images, data, and the observed macroscopic phenomena. The main conclusions are as follows:

(1)

In horizontal circular pipe flow, the velocity distribution of each flow type has an overall symmetrical distribution, with the highest velocity in the central region, while the velocity of coarse particles in the upper and lower regions of the pipeline gradually decreases with an increasing distance from the centerline of the pipeline. There is a significant difference in the followability of particles with water flow, and particles near the centerline of the pipeline are more susceptible to the acceleration of water flow. The 0.9 mm diameter particles are more likely to complete the transition of the coarse particle group flow pattern.

(2)

The granular bed exhibits three main motion states: static sedimentation, displacement, and suspension under different operating conditions, with the static sedimentation layer accounting for a larger proportion in the low-speed region; The particles in the moving layer are tightly arranged and pushed forward as a whole through rolling or horizontal movement. The suspended moving layer mainly appears under high flow velocity conditions, and the particle distribution inside the pipe is relatively uniform. In addition, an increase in the material volume concentration and an increase in particle size will both cause the expansion of static sedimentary layers and delay the transition of flow patterns during translational motion. Therefore, when transferring dredged material via pipeline hydraulic conveyance, the concentration of dredged material should not be too high in order to avoid the emergence of an excessively thick sediment layer.

(3)

The range of particle starting speed variation is relatively small and always below 0.5 m/s. It is greatly affected by the average mixing speed, and the critical sedimentation speed is sensitive to changes in particle diameter and material volume concentration, showing a certain linear correlation as a whole. The transition of the coarse particle group flow pattern from the sedimentation bed type to translational motion is closely related to the particle diameter and material volume concentration. Coarse particle groups with a low material volume concentration and small particle diameter are more likely to complete the flow pattern transition. Therefore, when transferring dredged material via pipeline hydraulic conveyance, the water flow rate can be increased to achieve a reduction in the concentration of dredged material, thus avoiding blockage of the pipeline.

(4)

The range of the material particle size and the range of the maximum flow rate are limited in this study, and future research can further expand the range of the material particle size and the range of the maximum flow rate, as well as increase the number of experimental groups, so as to more comprehensively analyze the influence of relevant factors on the particle motion state. In view of the complexity of the pipeline arrangement in the actual dredging construction, future research can focus on exploring the mechanism of the influence of various factors on the movement and resistance characteristics of coarse particles in the pipeline under complex pipeline conditions. In addition, the influence of other factors such as pipe material and pipe geometry on the flow pattern and hydraulic gradient of the coarse particle population can also be studied in depth. It is recommended to combine numerical simulation methods to systematically analyze the motion characteristics of the coarse particle population under different parameter combinations, which will help optimize the conveying process, improve the conveying efficiency, and reduce energy consumption. The expansion of the above research directions can provide more comprehensive theoretical support and practical guidance for the theoretical research and engineering application of horizontal pipeline conveying of coarse particles.