Analysis of the Hourglass–Spindle-Shaped Trajectory Generated by the Collision of Circularly Polarized Laser Pulses with Electrons
Abstract
1. Introduction
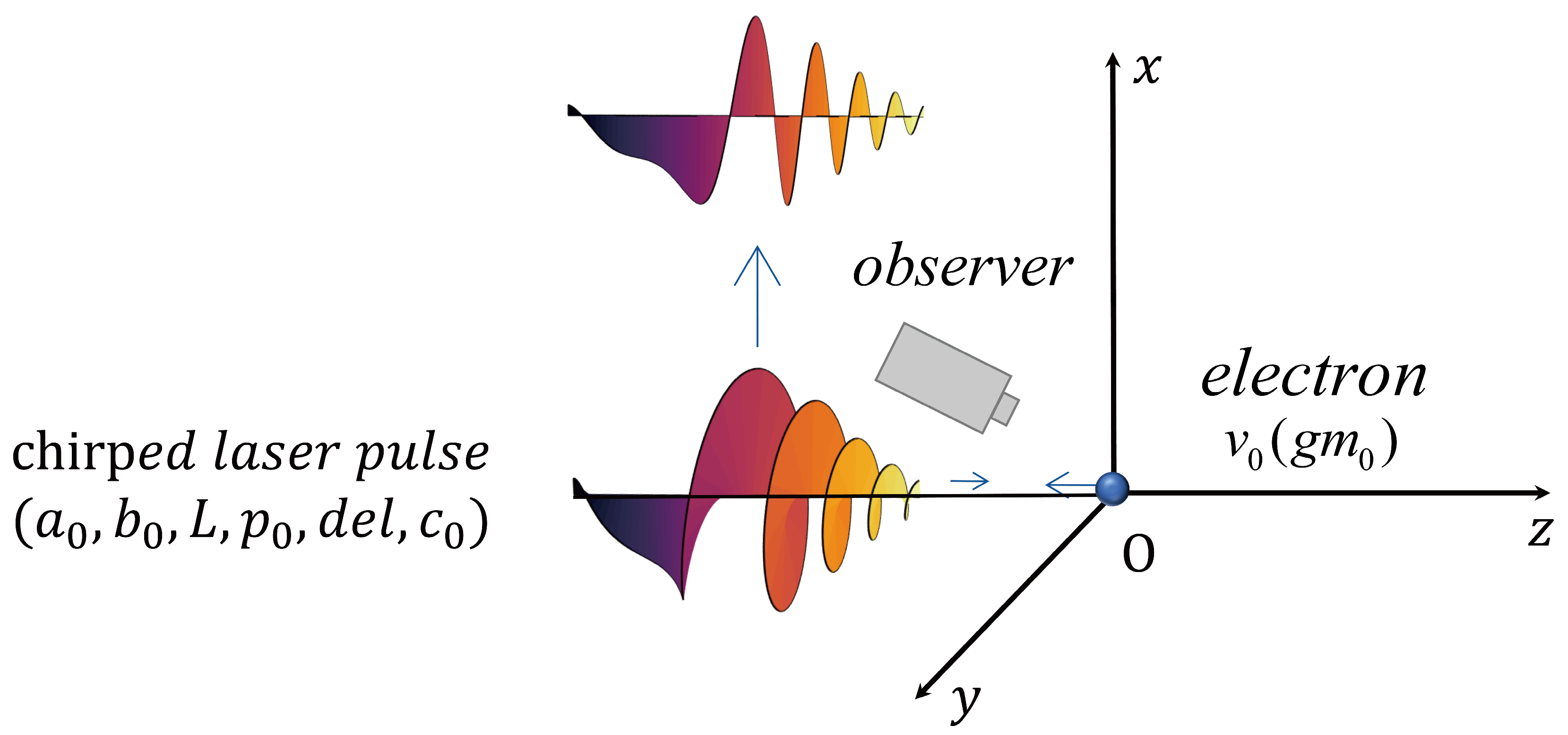
2. Materials and Methods
3. Results
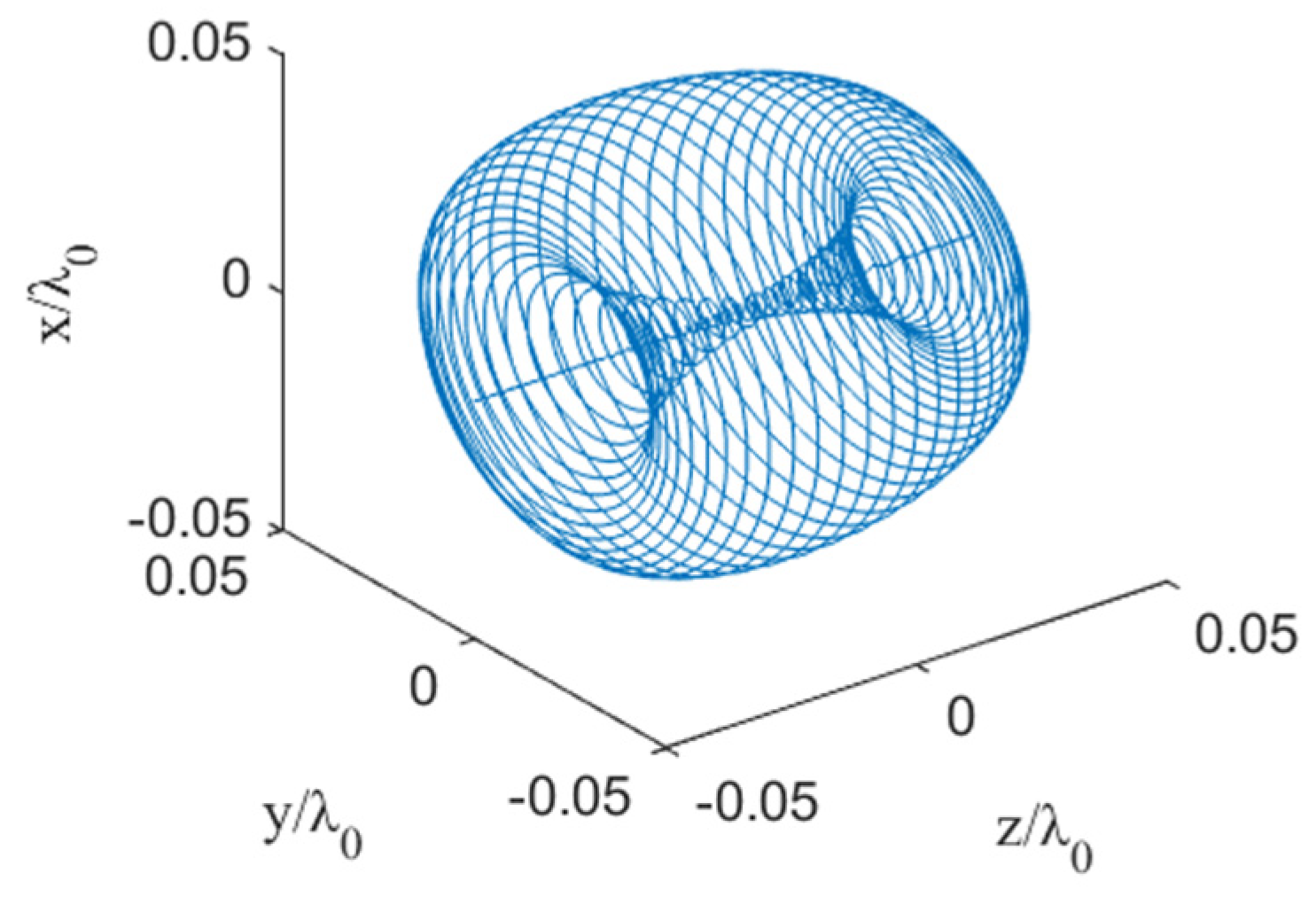
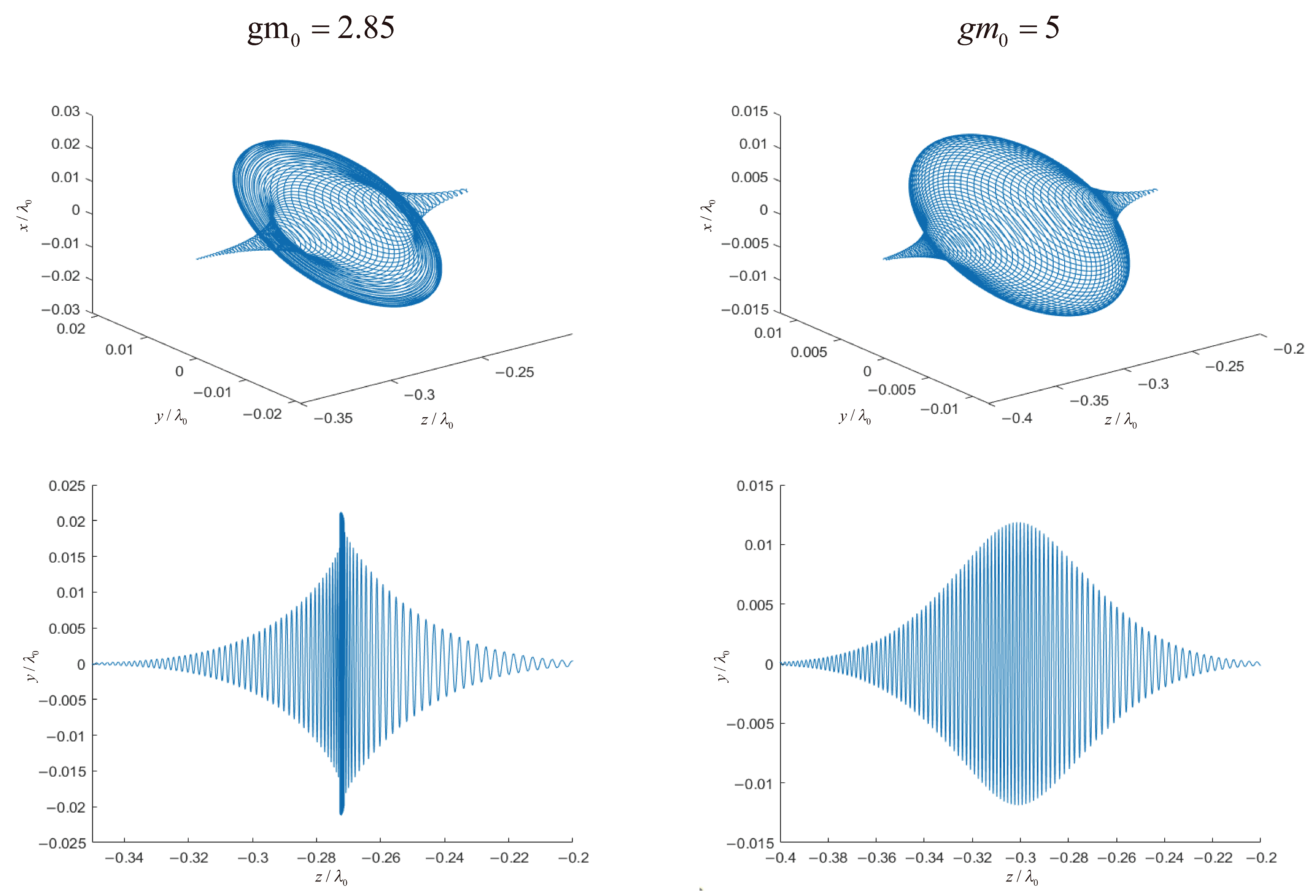
3.1. Motion Trajectory Analysis
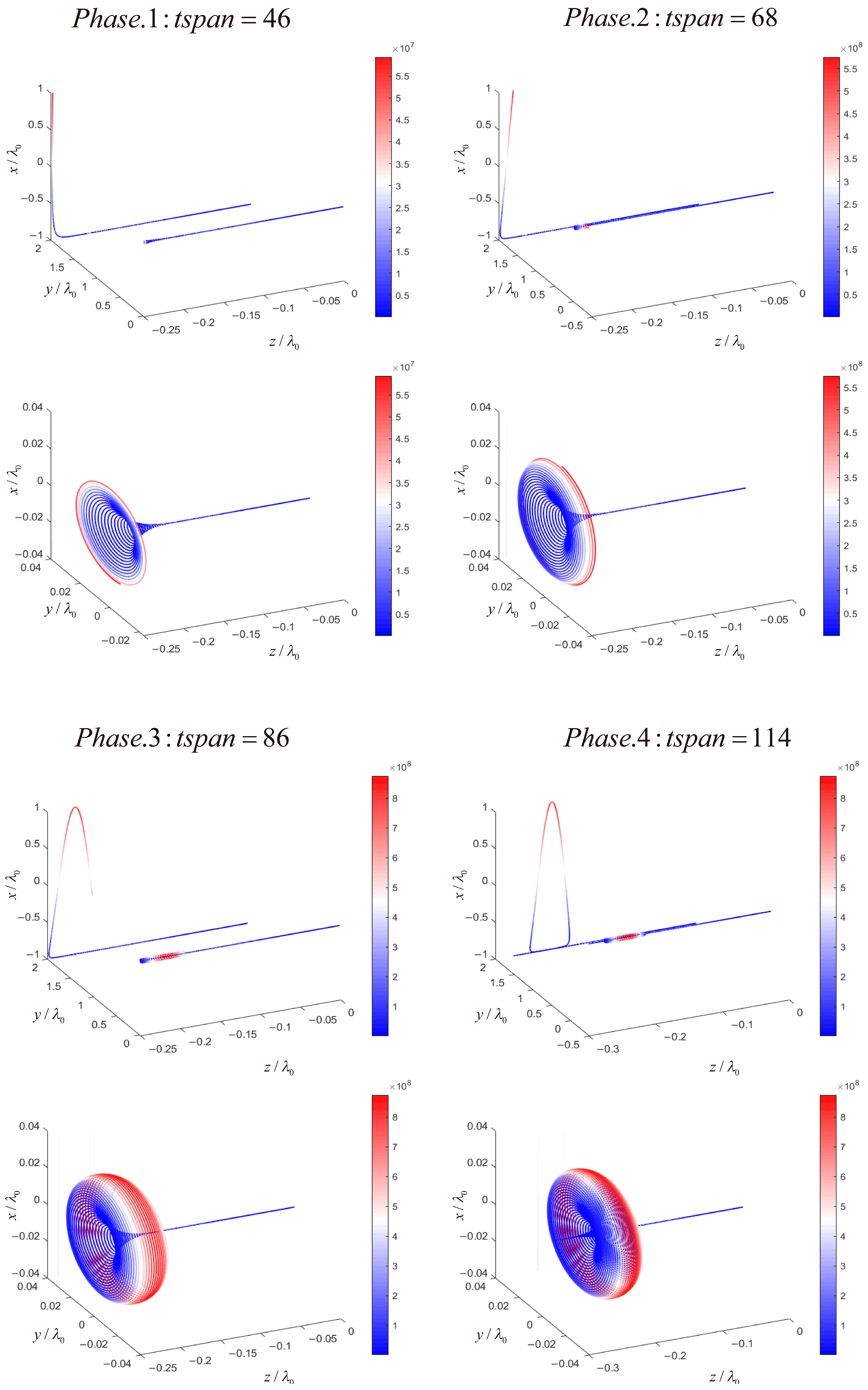
3.1.1. The Coupling Analysis of Motion Trajectory and Radiation Power
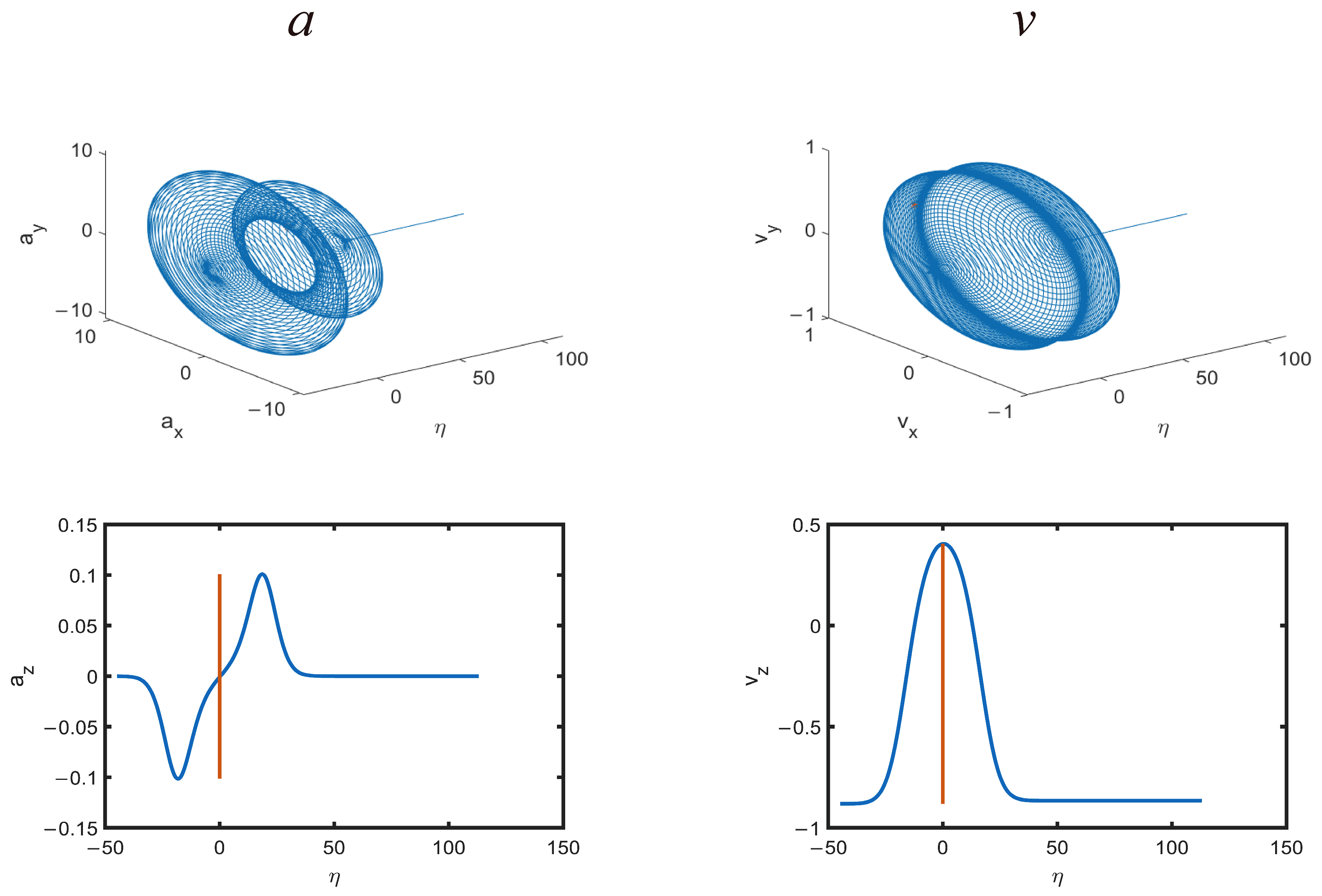
3.1.2. Further Conclusion
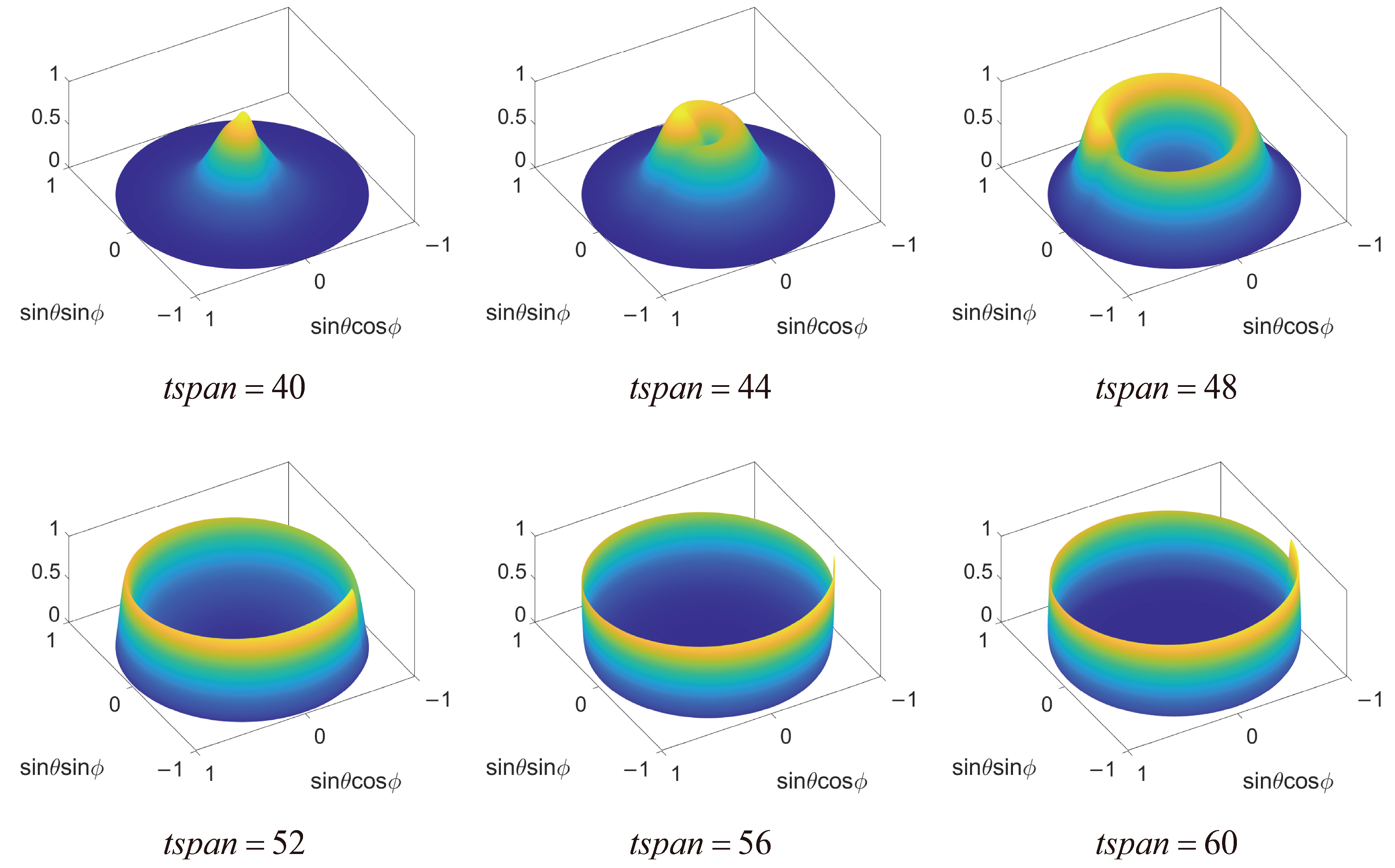
3.2. Radiation Spatial Distribution Variation
3.3. Time and Frequency Spectrum Distribution of Radiation
3.4. Further Study
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Sarachik, E.S.; Schappert, G.T. Classical theory of the scattering of intense laser radiation by free electrons. Phys. Rev. D 1970, 1, 2738. [Google Scholar] [CrossRef]
- Salamin, Y.I.; Keitel, C.H. Electron acceleration by a tightly focused laser beam. Phys. Rev. Lett. 2002, 88, 095005. [Google Scholar] [CrossRef]
- He, Y.; Yakup, B.; Bake, M.A. Linearly Polarized γ Photon Generation from Unpolarized Electron Bunch Interacting with Laser. Appl. Sci. 2025, 15, 481. [Google Scholar] [CrossRef]
- Esarey, E.; Ride, S.K.; Sprangle, P. Nonlinear Thomson scattering of intense laser pulses from beams and plasmas. Phys. Rev. E 1993, 48, 3003. [Google Scholar] [CrossRef]
- Zhang, Y.; Yang, Q.; Wang, J.; Gong, X.; Tian, Y. Zeptosecond-Yoctosecond Pulses Generated by Nonlinear Inverse Thomson Scattering: Modulation and Spatiotemporal Properties. Appl. Sci. 2024, 14, 7038. [Google Scholar] [CrossRef]
- Lee, K.; Cha, Y.H.; Shin, M.S.; Kim, B.H.; Kim, D. Temporal and spatial characterization of harmonics structures of relativistic nonlinear Thomson scattering. Opt. Express 2003, 11, 309–316. [Google Scholar] [CrossRef] [PubMed]
- Compton, A.H. A quantum theory of the scattering of X-rays by light elements. Phys. Rev. 1923, 21, 483. [Google Scholar] [CrossRef]
- Lau, Y.Y.; He, F.; Umstadter, D.P.; Kowalczyk, R. Nonlinear Thomson scattering: A tutorial. Phys. Plasmas 2003, 10, 2155–2162. [Google Scholar] [CrossRef]
- Glenzer, S.H.; Alley, W.E.; Estabrook, K.G.; De Groot, J.S.; Haines, M.G.; Hammer, J.H.; Jadaud, J.-P.; MacGowan, B.J.; Moody, J.D.; Rozmus, W.; et al. Thomson scattering from laser plasmas. Phys. Plasmas 1999, 6, 2117–2128. [Google Scholar] [CrossRef]
- Herold, H. Compton and Thomson scattering in strong magnetic fields. Phys. Rev. D 1979, 19, 2868. [Google Scholar] [CrossRef]
- Maine, P.; Strickland, D.; Bado, P.; Pessot, M.; Mourou, G. Generation of ultrahigh peak power pulses by chirped pulse amplification. IEEE J. Quantum Electron. 1988, 24, 398–403. [Google Scholar] [CrossRef]
- Ross, I.; Matousek, P.; Towrie, M.; Langley, A.; Collier, J. The prospects for ultrashort pulse duration and ultrahigh intensity using optical parametric chirped pulse amplifiers. Opt. Commun. 1997, 144, 125–133. [Google Scholar] [CrossRef]
- Kim, H.Y.; Garg, M.; Mandal, S.; Seiffert, L.; Fennel, T.; Goulielmakis, E. Attosecond field emission. Nature 2023, 613, 662–666. [Google Scholar] [CrossRef] [PubMed]
- Calegari, F.; Ayuso, D.; Trabattoni, A.; Belshaw, L.; De Camillis, S.; Anumula, S.; Frassetto, F.; Poletto, L.; Palacios, A.; Decleva, P.; et al. Ultrafast electron dynamics in phenylalanine initiated by attosecond pulses. Science 2014, 346, 336–339. [Google Scholar] [CrossRef]
- Zeng, L.; Wang, X.; Liang, Y.; Yi, H.; Zhang, W.; Yang, X. Chirped-Pulse Amplification in an Echo-Enabled Harmonic-Generation Free-Electron Laser. Appl. Sci. 2023, 13, 10292. [Google Scholar] [CrossRef]
- Witting, T.; Osolodkov, M.; Schell, F.; Morales, F.; Patchkovskii, S.; Šušnjar, P.; Cavalcante, F.H.M.; Menoni, C.S.; Schulz, C.P.; Furch, F.J.; et al. Generation and characterization of isolated attosecond pulses at 100 kHz repetition rate. Optica 2022, 9, 145–151. [Google Scholar] [CrossRef]
- Gaál, P.; Gilinger, T.; Nagyillés, B.; Nagymihály, R.; Seres, I.; Kovács, Á.; Füle, M.; Karnok, M.; Balázs, P.; Novák, T.; et al. A Versatile 100 Hz Laser System with Few-Cycle and TeraWatt Pulses for Applications. Appl. Sci. 2024, 14, 10649. [Google Scholar] [CrossRef]
- Paul, P.M.; Toma, E.S.; Breger, P.; Mullot, G.; Augé, F.; Balcou, P.; Muller, H.G.; Agostini, P. Observation of a train of attosecond pulses from high harmonic generation. Science 2001, 292, 1689–1692. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, C.; Li, K.; Li, L.; Tian, Y. Spatial radiation features of Thomson scattering from electron in circularly polarized tightly focused laser beams. Laser Phys. Lett. 2020, 18, 015303. [Google Scholar] [CrossRef]
- Salamin, Y.I.; Faisal, F.H.M. Harmonic generation by superintense light scattering from relativistic electrons. Phys. Rev. A 1996, 54, 4383. [Google Scholar] [CrossRef]
- Yariv, A. Quantum Electronics, 2nd ed.; John Wiley & Sons: New York, NY, USA, 1975; p. 123. [Google Scholar]
- Barton, J.P.; Alexander, D.R. Alexander. Fifth-order corrected electromagnetic field components for a fundamental Gaussian beam. J. Appl. Phys. 1989, 66, 2800–2802. [Google Scholar] [CrossRef]
- Zhang, S.Y. Accurate correction field of circularly polarized laser and its acceleration effect. J. At. Mol. Sci. 2010, 1, 308–317. [Google Scholar]
- Jackson, J.D.; Ronald, F.F. Classical Electrodynamics; Wiley: Hoboken, NJ, USA, 1999; pp. 841–842. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, J.; Xu, J.; Wang, Z.; Zheng, Q.; Tian, Y. Analysis of the Hourglass–Spindle-Shaped Trajectory Generated by the Collision of Circularly Polarized Laser Pulses with Electrons. Appl. Sci. 2025, 15, 3013. https://doi.org/10.3390/app15063013
Li J, Xu J, Wang Z, Zheng Q, Tian Y. Analysis of the Hourglass–Spindle-Shaped Trajectory Generated by the Collision of Circularly Polarized Laser Pulses with Electrons. Applied Sciences. 2025; 15(6):3013. https://doi.org/10.3390/app15063013
Chicago/Turabian StyleLi, Jiachen, Junyuan Xu, Zi Wang, Qianmin Zheng, and Youwei Tian. 2025. "Analysis of the Hourglass–Spindle-Shaped Trajectory Generated by the Collision of Circularly Polarized Laser Pulses with Electrons" Applied Sciences 15, no. 6: 3013. https://doi.org/10.3390/app15063013
APA StyleLi, J., Xu, J., Wang, Z., Zheng, Q., & Tian, Y. (2025). Analysis of the Hourglass–Spindle-Shaped Trajectory Generated by the Collision of Circularly Polarized Laser Pulses with Electrons. Applied Sciences, 15(6), 3013. https://doi.org/10.3390/app15063013





