Effect of Fissure Penetration Degrees on Spalling Process and Feature of Surrounding Rock in Rectangular Tunnel Under True Triaxial Loading
Abstract
1. Introduction
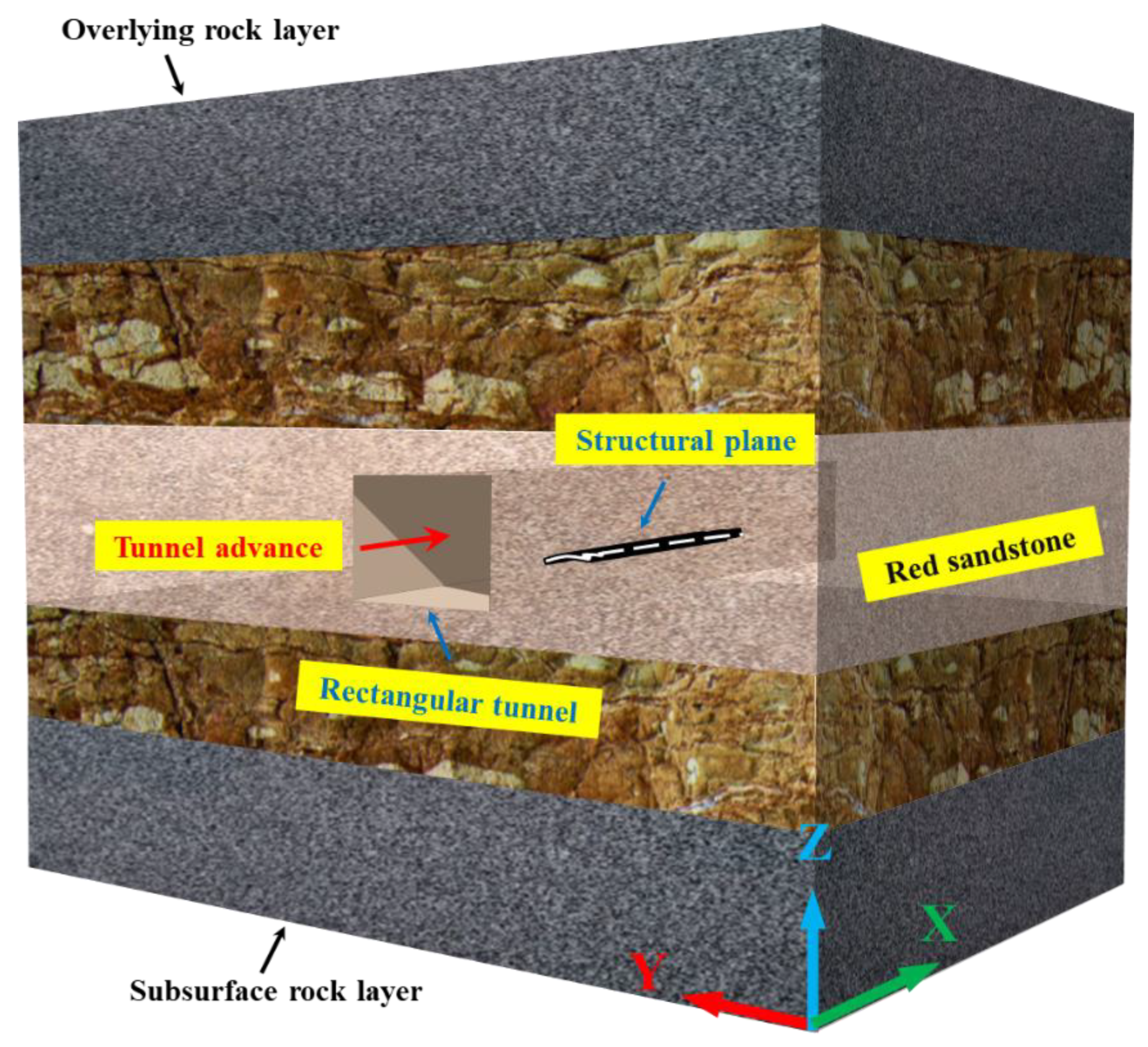
- Based on previous studies, most research has used circular holes and cubic specimens to simulate the failure of circular tunnels, but there is limited research on rectangular tunnels. As the use of rectangular tunnels and shafts increases in mining and deep engineering projects, it is essential to investigate spalling in the surrounding rock of rectangular tunnels.
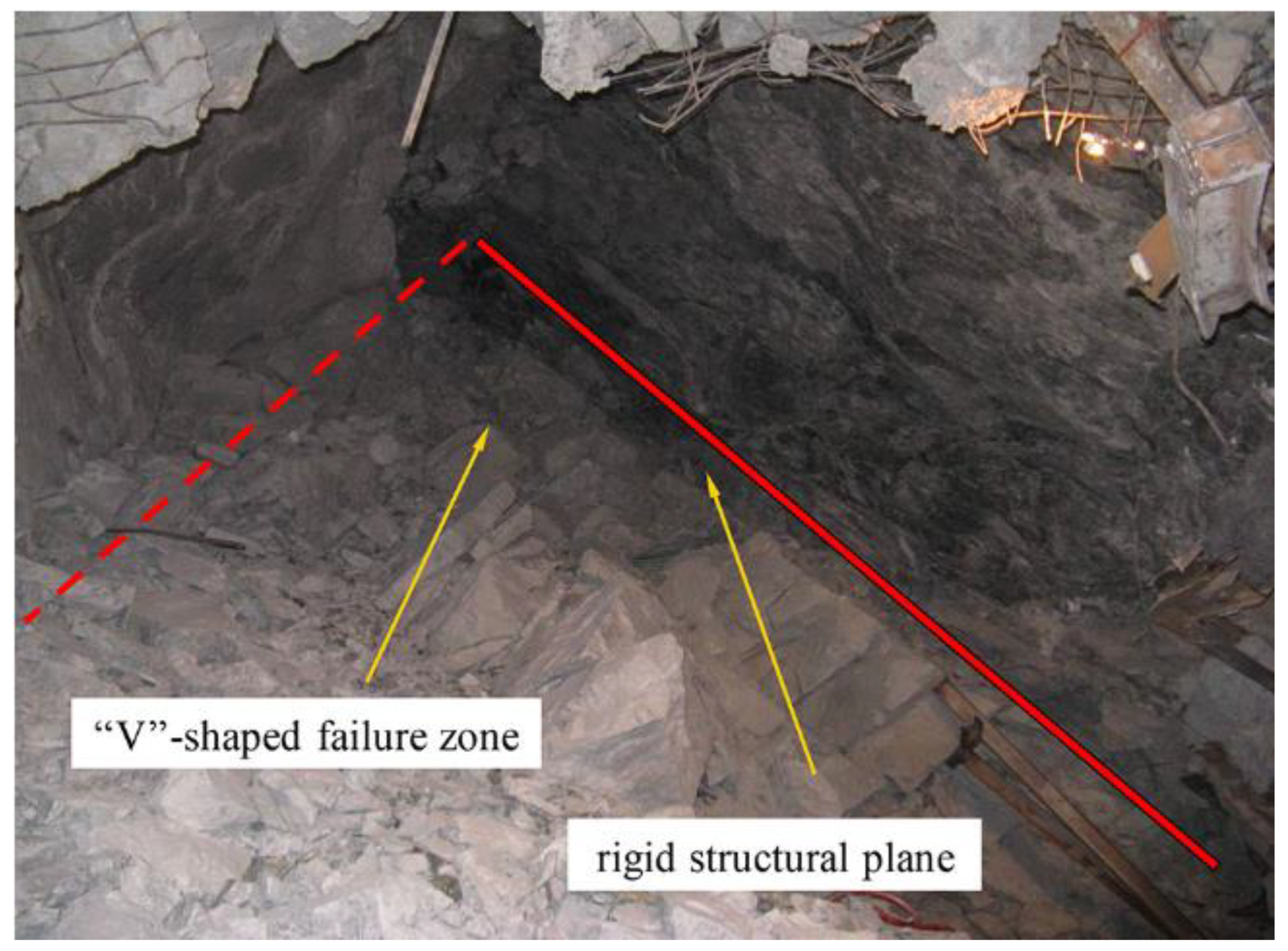
- The spalling failure process in deep mine tunnels is inevitably influenced by complex geological conditions, including bedding, discontinuous joints, and faults. Many rock mass engineering disasters result from the expansion and interconnection of fractures in the rock mass [40,41,42,43]. While much research has focused on mining-related factors such as the stress state, stress path, and loading rate, and their influence on spalling failure and surrounding rock characteristics, the geological environment of deep tunnels has received less attention.
2. Experimental Methods
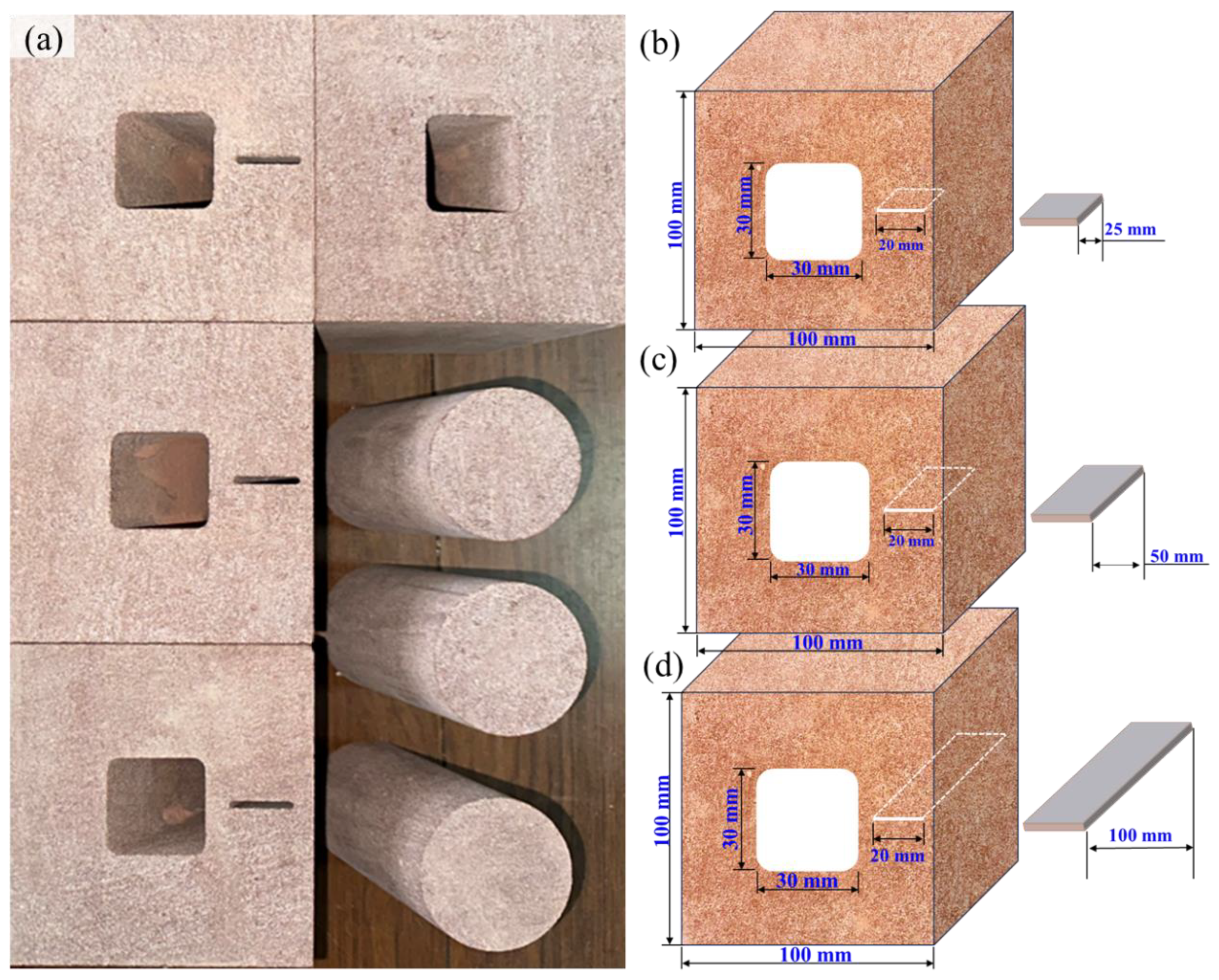
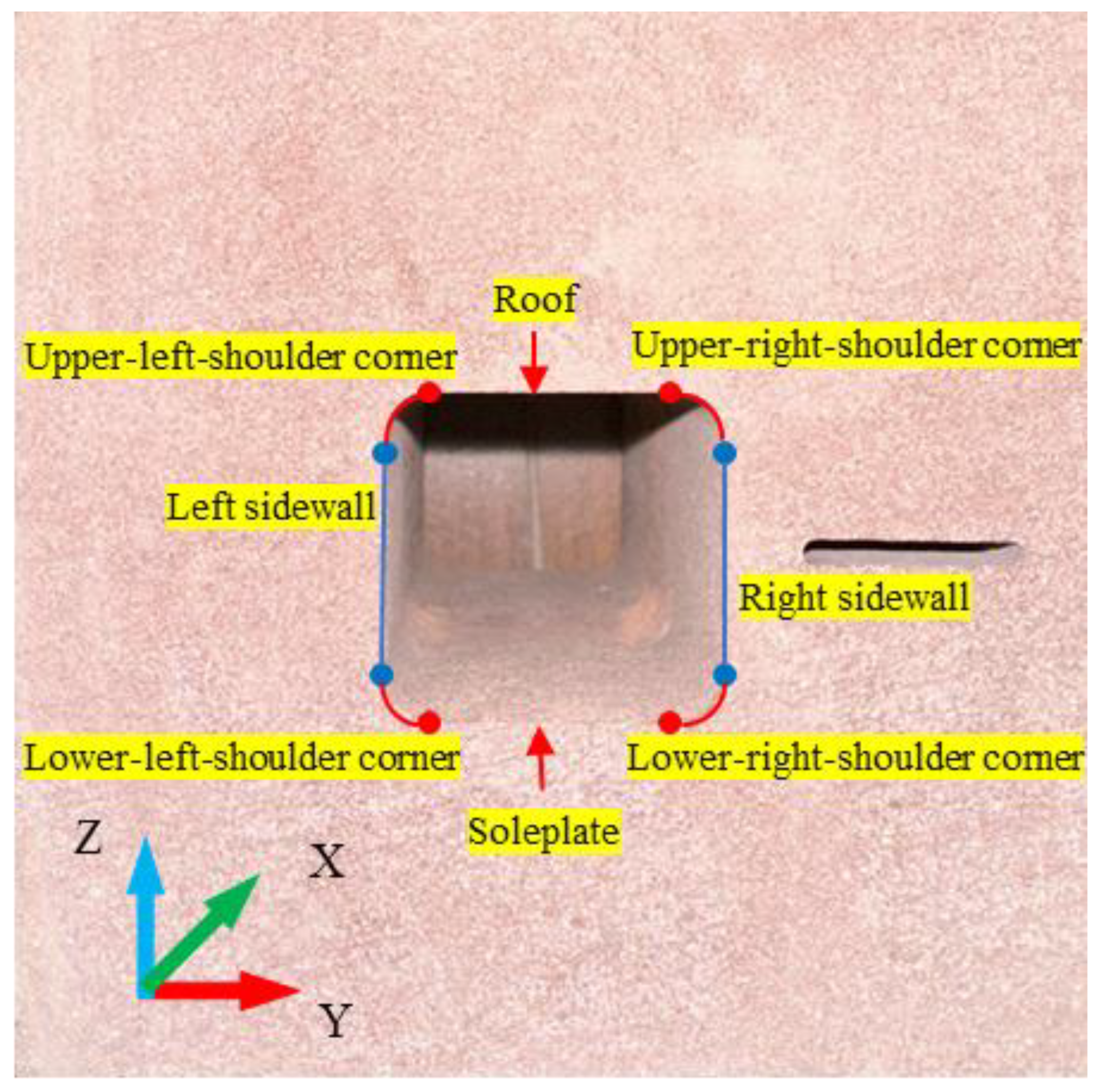
2.1. Specimen Design and Preparation
2.2. Testing Machines
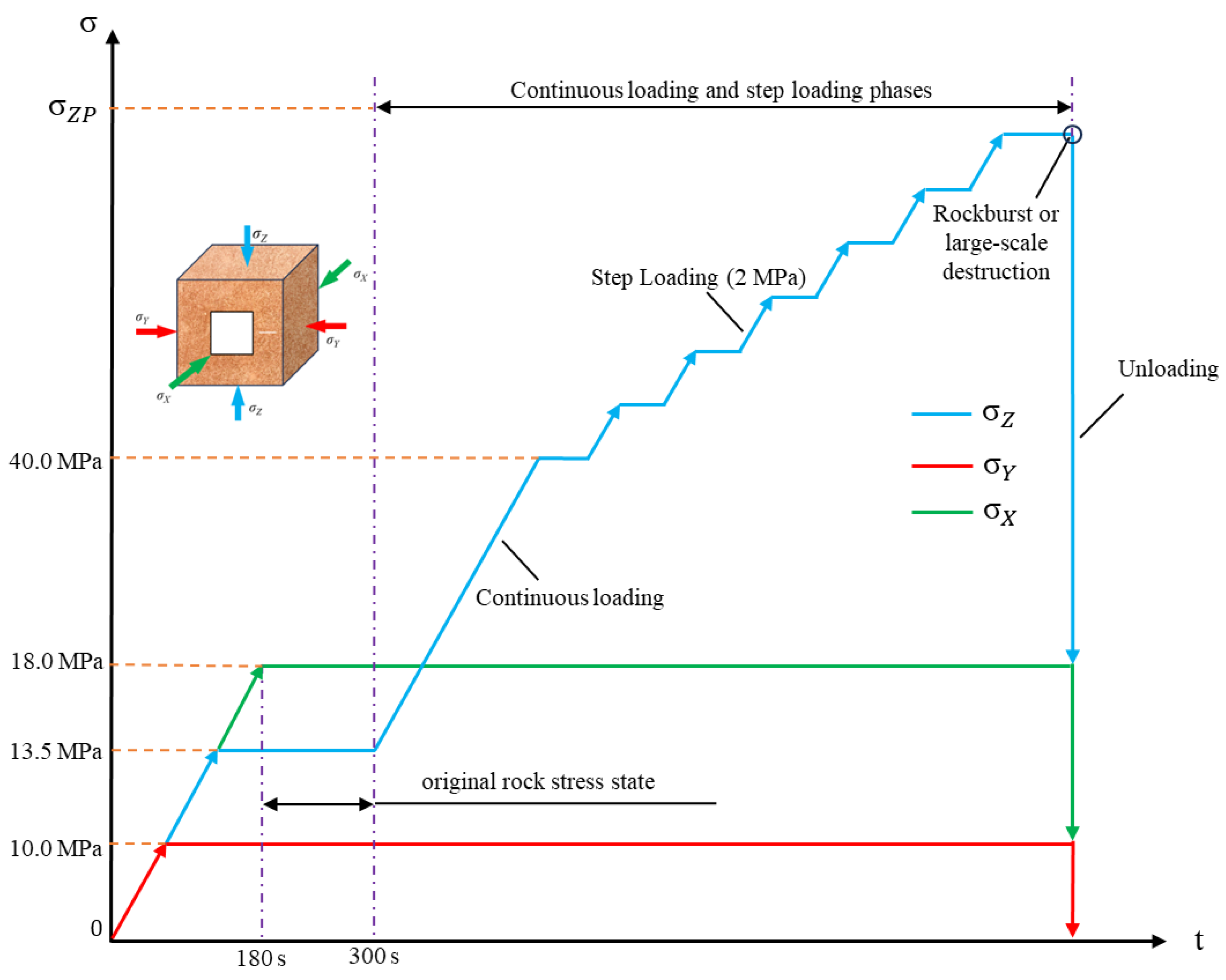
2.3. Testing Method
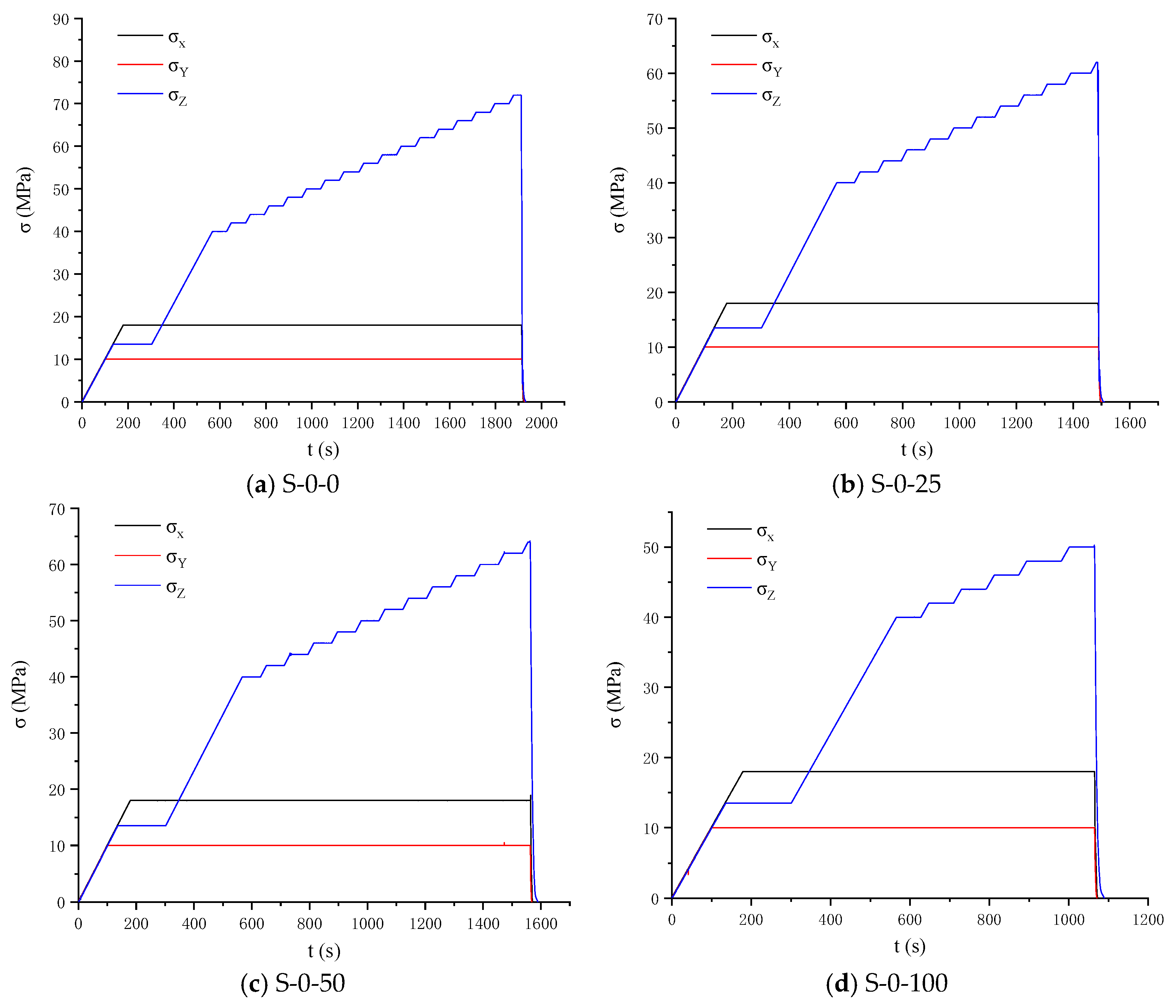
3. Test Results
3.1. Results of Uniaxial Compression Tests
3.2. Stress-Time Curves
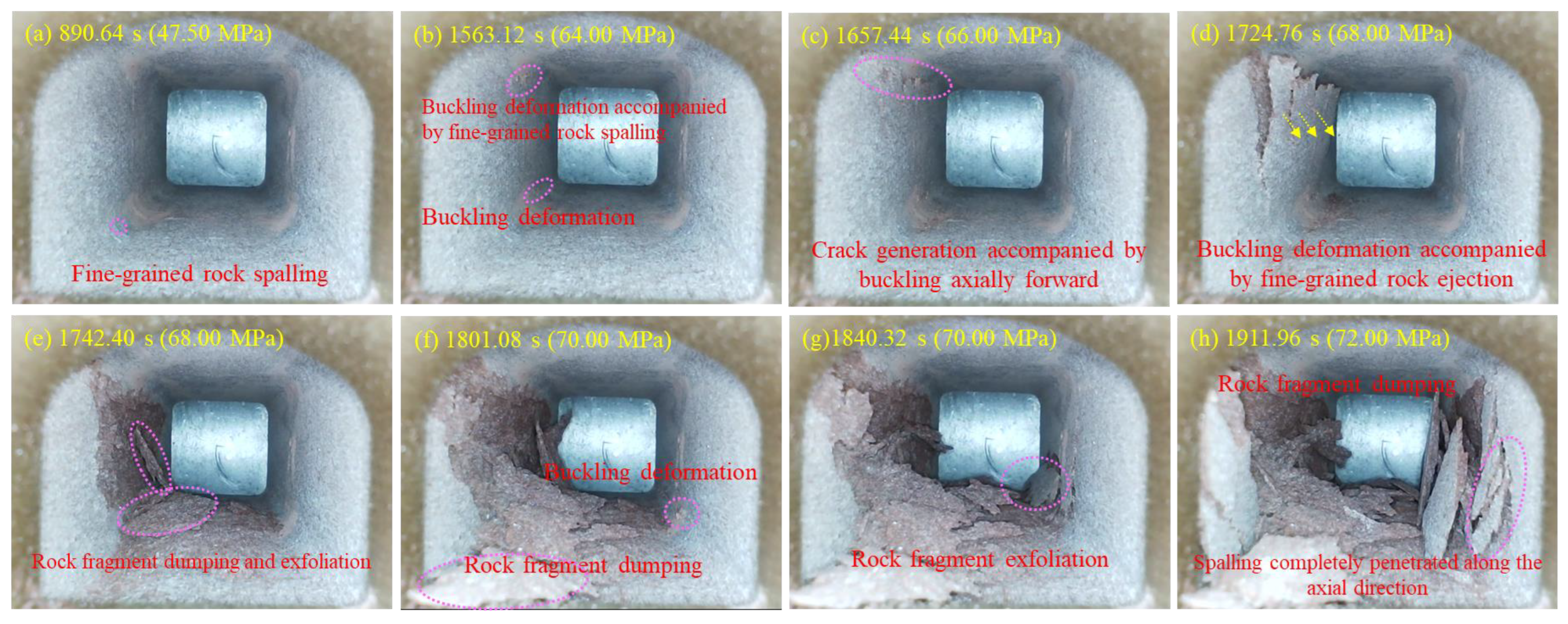
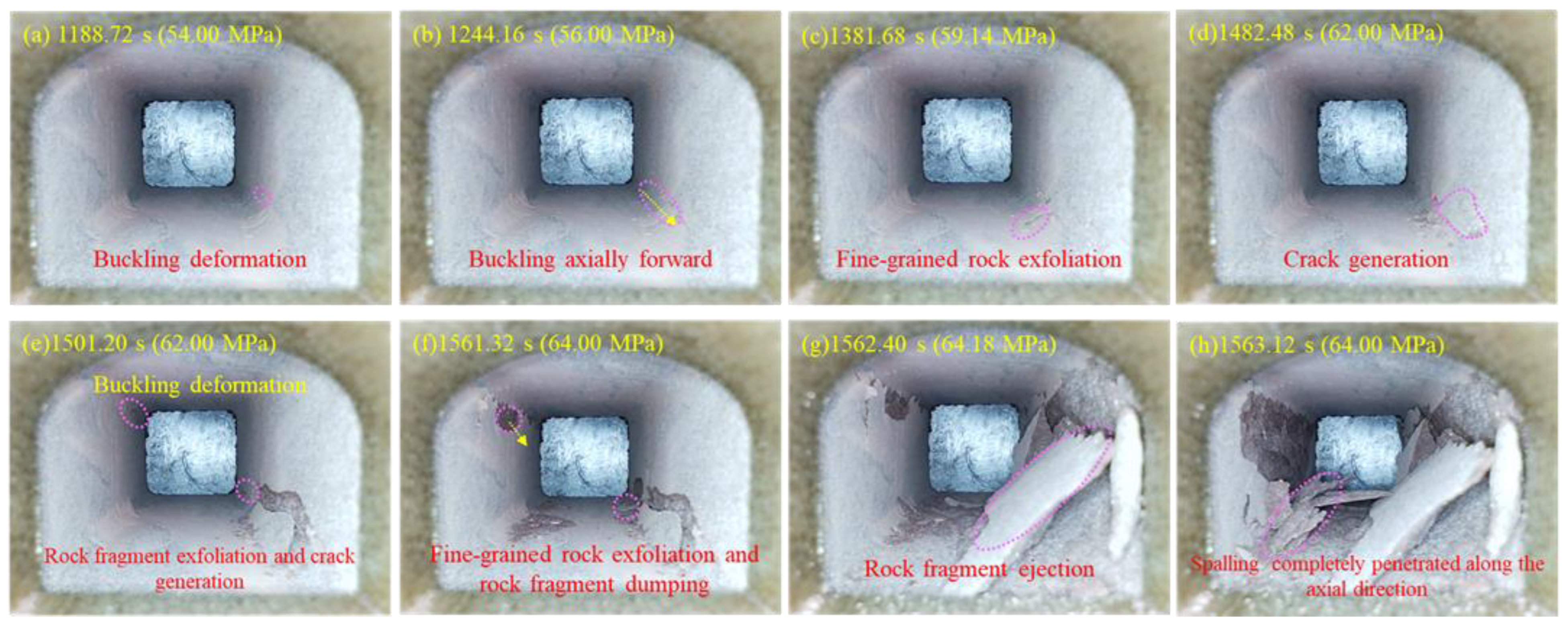
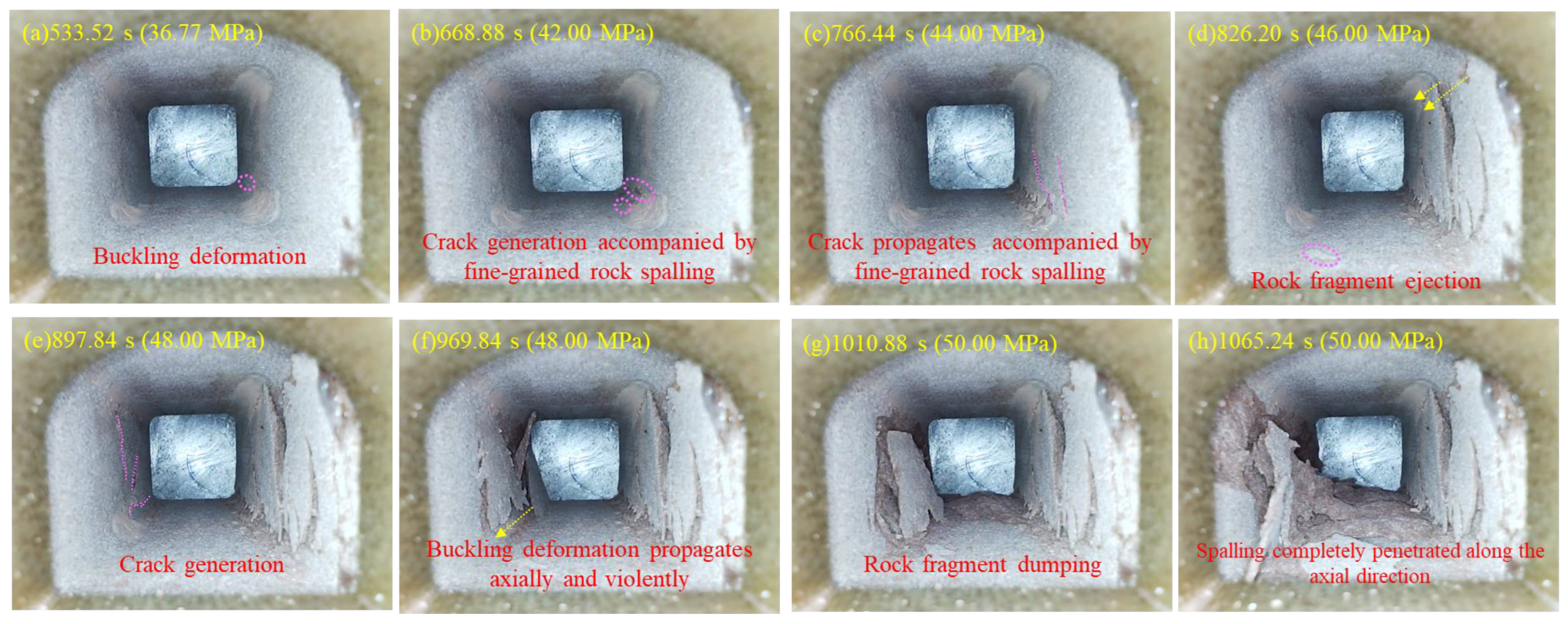
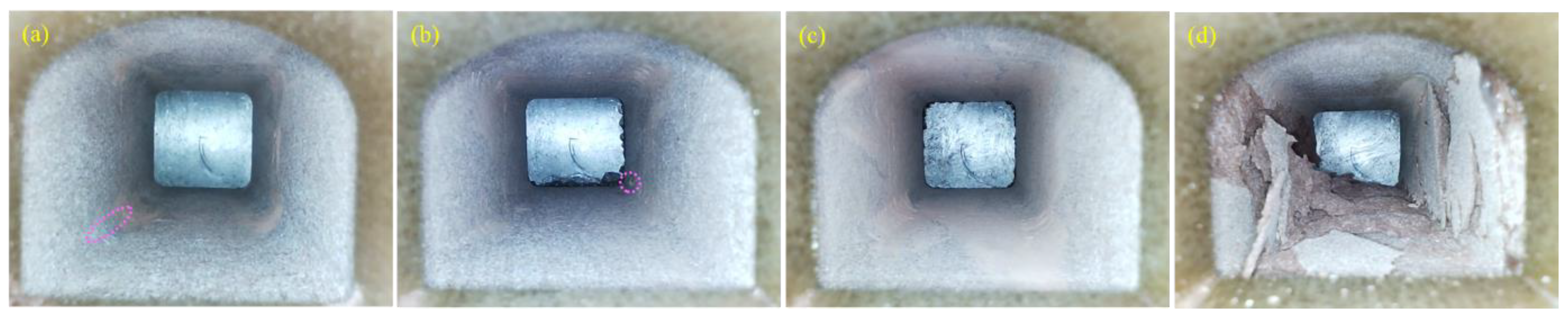
3.3. Spalling Failure Process
4. Discussion
4.1. Effect of Fissure Penetration on Sidewall Failure
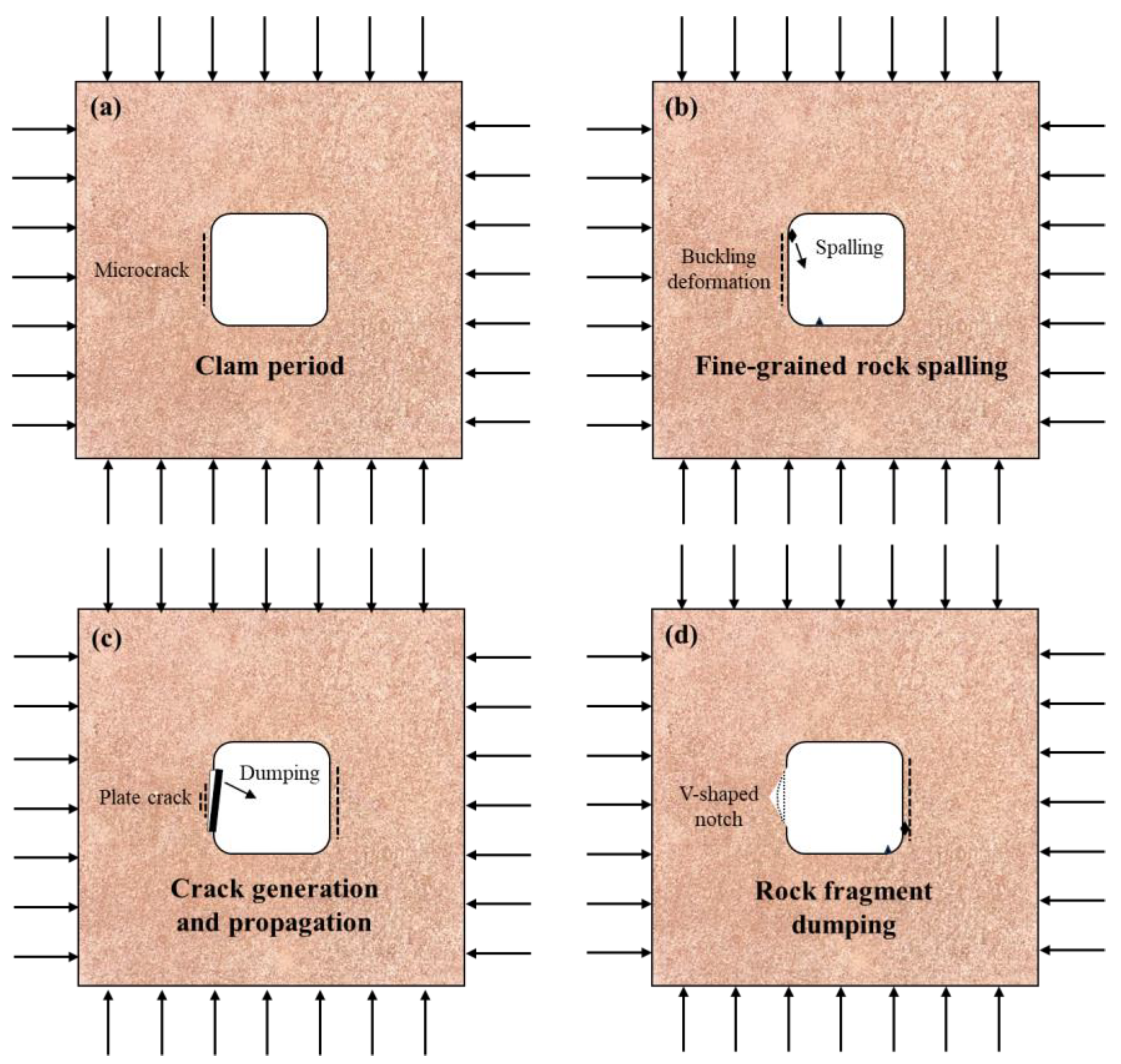
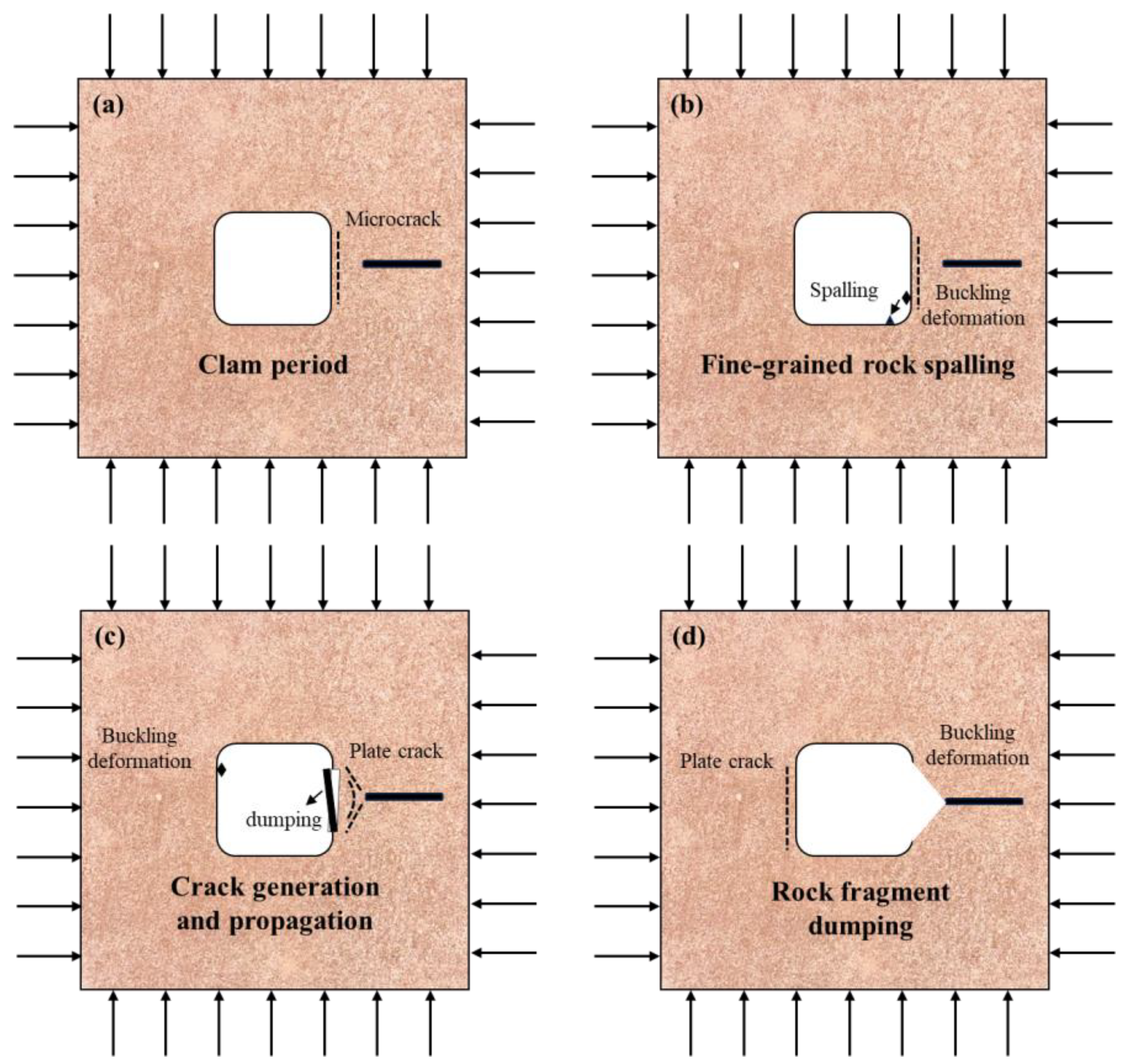
4.1.1. Sidewall Failure Process
4.1.2. Influence of Fissure Penetration on Tunnel Stability
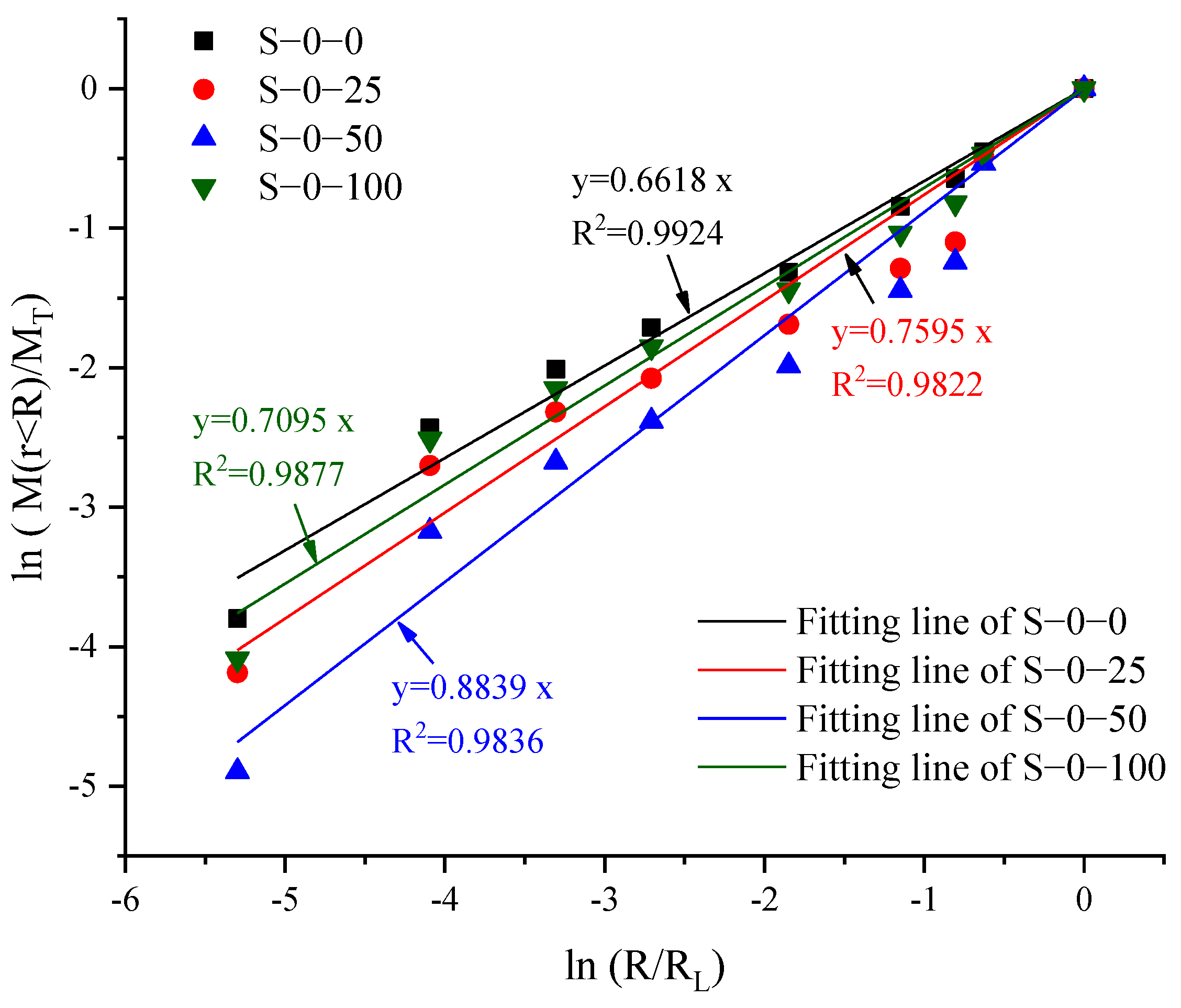
4.2. Effect of Fissure Penetration on Rock Fragment Characteristics
4.3. Guidance for Deep Underground Engineering
5. Conclusions
- (1)
- The failure of the sidewall of the non-fissured sandstone test can be divided into four stages: the calm stage, the spalling stage of fine rock particles, the generation and expansion stage of the sidewall cracks, and the buckling deformation and failure stage of the sidewall rock chips. As fissure penetration increased, the initial failure location of the tunnel sidewall shifted toward the fissured side, and the number of rock slabs resulting from slab cracking decreased.
- (2)
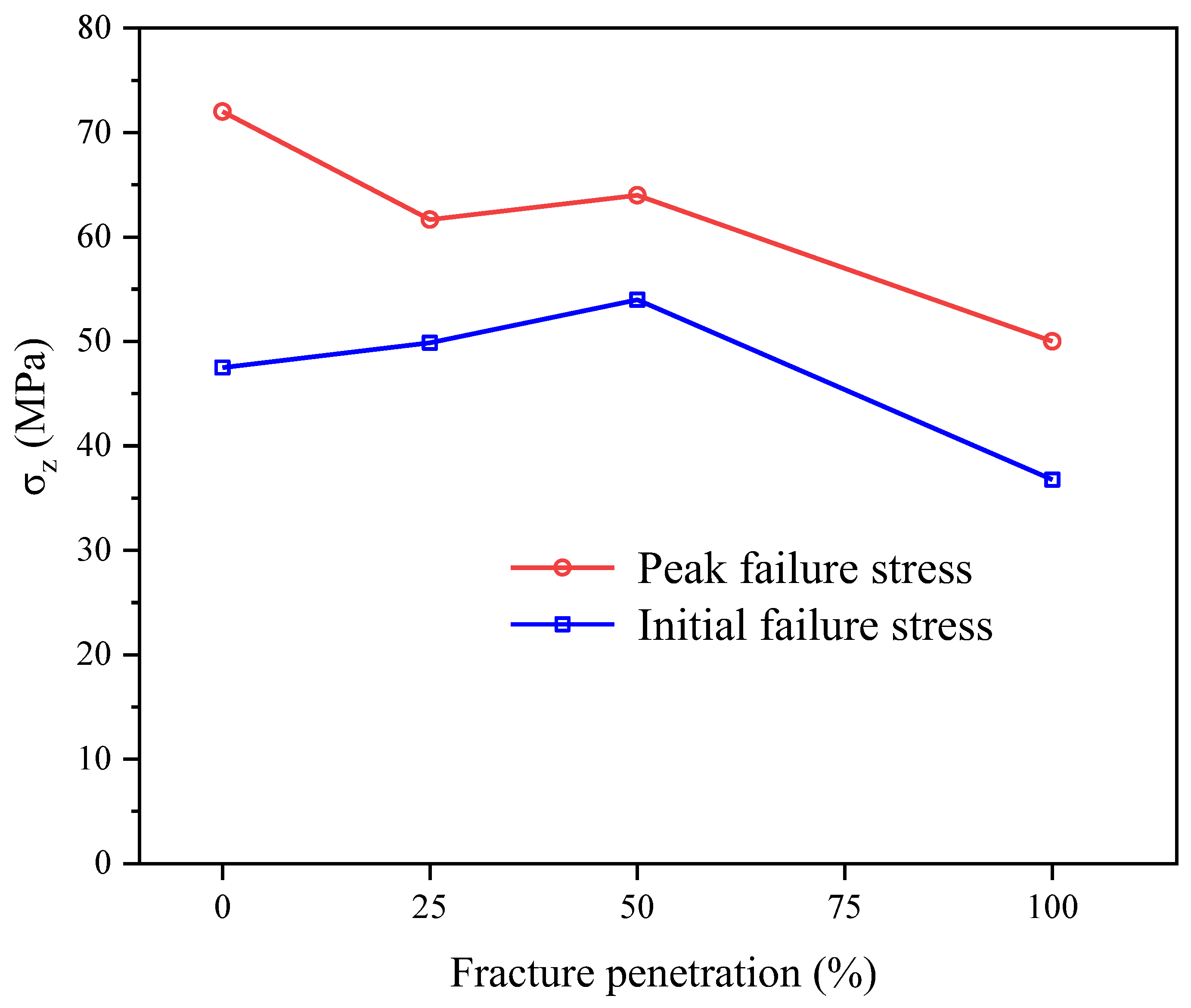
- As fissure penetration increased, the initial failure stress σzi first rose and then decreased, while the peak failure stress σzp consistently decreased, indicating that fissures weakened the surrounding rock’s strength. This also made it more difficult for the surrounding rock to experience initial failure under medium fissure penetration.
- (3)
- The presence of fractures altered the particle size distribution of rock fragments. As fissure penetration increased, the number of larger rock blocks decreased, and the distribution became more block-like. Additionally, fracture penetration significantly impacted the fractal dimension of the rock, with lower penetration resulting in a higher fractal dimension in the rock fragments. When fissure penetration is high, it is necessary to reinforce support and control excavation boundaries to reduce stress concentration.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ortlepp, W.D.; Stacey, T.R. Rockburst mechanisms in tunnels and shafts. Tunn. Undergr. Space Technol. 1994, 9, 59–65. [Google Scholar] [CrossRef]
- Fairhurst, C.; Cook, N.G.W. The phenomenon of rock splitting parallel to the direction of maximum compression in the neighborhood of a surface. In Proceedings of the First Congress on International Society of Rock Mechanics, Lisbon, Portugal, 25 September–1 October 1966; Volume 1, pp. 687–692. [Google Scholar]
- Peng, K.; Zhang, Y.; Wang, Y.; Luo, S. Static compression behavior and strength weakening mechanism of dynamically damaged granite after water soaking. Eng. Fail. Anal. 2024, 156, 107760. [Google Scholar] [CrossRef]
- Peng, K.; Ren, J.; Wang, Y.; Luo, S.; Long, K. Mechanical and damage evolution characteristics of granite after heating-cooling cycles. J. Cent. South Univ. 2023, 30, 4082–4096. [Google Scholar] [CrossRef]
- Shirani Faradonbeh, R.; Taheri, A.; Ribeiro ESousa, L.; Karakus, M. Rockburst assessment in deep geotechnical conditions using true-triaxial tests and data-driven approaches. Int. J. Rock Mech. Min. Sci. 2020, 128, 104279. [Google Scholar] [CrossRef]
- Luo, Y.; Gong, F.; Liu, D.; Wang, S.; Si, X. Experimental simulation analysis of the process and failure characteristics of spalling in D-shaped tunnels under true-triaxial loading conditions. Tunn. Undergr. Space Technol. 2019, 90, 42–61. [Google Scholar] [CrossRef]
- Dai, J.; Gong, F.; Qi, S.; Xu, L. Quantitative evaluation of rockburst proneness for surrounding rocks considering combined effects of the structural plane and excavation disturbance. Tunn. Undergr. Space Technol. 2023, 140, 105335. [Google Scholar] [CrossRef]
- Ortlepp, W.D. The behaviour of tunnels at great depth under large static and dynamic pressures. Tunn. Undergr. Space Technol. 2001, 16, 41–48. [Google Scholar] [CrossRef]
- Carter, B.J. Size and stress gradient effects on fracture around cavities. Rock Mech. Rock Eng. 1992, 25, 167–186. [Google Scholar] [CrossRef]
- Peng, K.; Ren, J.; Wu, T.; Yi, G.; Luo, S.; Luo, K. Influences of arch height and stress sate on tunnel failure: Insights from orthogonal true-triaxial experiment. Theor. Appl. Fract. Mech. 2025, 136, 104824. [Google Scholar] [CrossRef]
- Gong, F.; Wu, W.; Li, T. Simulation test of spalling failure of surrounding rock in rectangular tunnels with different height-to-width ratios. Bull. Eng. Geol. Environ. 2020, 79, 3207–3219. [Google Scholar] [CrossRef]
- Peng, K.; Yi, G.; Wang, Y.; Luo, S.; Wu, H. Experimental and theoretical analysis of spalling in deep hard rock tunnels with different arch structures. Theor. Appl. Fract. Mech. 2023, 127, 104054. [Google Scholar] [CrossRef]
- Kang, P.; Lai, R.Z.; Song, L.; Li, X.B. Influence of axial stress on failure characteristics of deep arch tunnel. Trans. Nonferrous Met. Soc. China 2024, 34, 4032–4048. [Google Scholar]
- Hao, X.; Yuan, L.; Sun, Z.; Zhao, Y.; Ren, B.; Zhao, D.; Xue, J.; Zhang, X.; Yu, G.; Zhang, Q. An integrated study of physical and numerical modelling on the stability of underground tunnel influenced by unloading rate. Tunn. Undergr. Space Technol. 2022, 129, 104602. [Google Scholar] [CrossRef]
- Feng, F.; Li, X.; Luo, L.; Zhao, X.; Chen, S.; Jiang, N.; Huang, W.; Wang, Y. Rockburst response in hard rock owing to excavation unloading of twin tunnels at great depth. Bull. Eng. Geol. Environ. 2021, 80, 7613–7631. [Google Scholar] [CrossRef]
- Su, G.; Jiang, J.; Feng, X.; Jiang, Q.; Chen, Z.; Mo, J. Influence of loading rate on strainburst: An experimental study. Bull. Eng. Geol. Environ. 2019, 78, 3559–3573. [Google Scholar] [CrossRef]
- Gong, F.; Ni, Y.; Ren, L. Effects of Loading Rate on Rockburst Proneness of Granite from Energy Storage and Surplus Perspectives. Rock. Mech. Rock Eng. 2022, 55, 6495–6516. [Google Scholar] [CrossRef]
- Xu, J.; Ma, L.; Xiao, X.; Wu, D. Experimental study of the formation process and behaviors of spalling in rock materials. Eng. Fail. Anal. 2023, 143, 106873. [Google Scholar] [CrossRef]
- Pan, P.-Z.; Miao, S.; Wu, Z.; Feng, X.-T.; Shao, C. Laboratory Observation of Spalling Process Induced by Tangential Stress Concentration in Hard Rock Tunnel. Int. J. Geomech. 2020, 20, 04020011. [Google Scholar] [CrossRef]
- Manouchehrian, A.; Cai, M. Numerical modeling of rockburst near fault zones in deep tunnels. Tunn. Undergr. Space Technol. 2018, 80, 164–180. [Google Scholar] [CrossRef]
- Feng, F.; Li, X.; Rostami, J.; Li, D. Modeling hard rock failure induced by structural planes around deep circular tunnels. Eng. Fract. Mech. 2019, 205, 152–174. [Google Scholar] [CrossRef]
- Fakhimi, A.; Hemami, B. Axial splitting of rocks under uniaxial compression. Int. J. Rock Mech. Min. Sci. 2015, 79, 124–134. [Google Scholar] [CrossRef]
- Huang, L.; Wang, J.; Momeni, A.; Wang, S. Spalling fracture mechanism of granite subjected to dynamic tensile loading. Trans. Nonferrous Met. Soc. China 2021, 31, 2116–2127. [Google Scholar] [CrossRef]
- Zhao, H.; Liu, C.; Huang, G.; Yu, B.; Liu, Y.; Song, Z. Experimental investigation on rockburst process and failure characteristics in trapezoidal tunnel under different lateral stresses. Constr. Build. Mater. 2020, 259, 119530. [Google Scholar] [CrossRef]
- Zhu, W.C.; Liu, J.; Tang, C.A.; Zhao, X.D.; Brady, B.H. Simulation of progressive fracturing processes around underground excavations under biaxial compression. Tunn. Undergr. Space Technol. 2005, 20, 231–247. [Google Scholar] [CrossRef]
- Cheng, T.; He, M.; Li, H.; Liu, D.; Qiao, Y.; Hu, J. Experimental investigation on the influence of a single structural plane on rockburst. Tunn. Undergr. Space Technol. 2023, 132, 104914. [Google Scholar] [CrossRef]
- Kaiser, P.K.; Cai, M. Design of rock support system under rockburst condition. J. Rock Mech. Geotech. Eng. 2012, 4, 215–227. [Google Scholar] [CrossRef]
- Jiang, Q.; Feng, X.; Fan, Y.; Fan, Q.; Liu, G.; Pei, S.; Duan, S. In situ experimental investigation of basalt spalling in a large underground powerhouse cavern. Tunn. Undergr. Space Technol. 2017, 68, 82–94. [Google Scholar] [CrossRef]
- Liu, G.; Feng, X.-T.; Jiang, Q.; Yao, Z.; Li, S. In situ observation of spalling process of intact rock mass at large cavern excavation. Eng. Geol. 2017, 226, 52–69. [Google Scholar] [CrossRef]
- Li, D.; Li, C.C.; Li, X. Influence of Sample Height-to-Width Ratios on Failure Mode for Rectangular Prism Samples of Hard Rock Loaded In Uniaxial Compression. Rock Mech. Rock Eng. 2011, 44, 253–267. [Google Scholar] [CrossRef]
- Zhu, G.-Q.; Feng, X.-T.; Zhou, Y.-Y.; Li, Z.-W.; Fu, L.-J.; Xiong, Y.-R. Physical Model Experimental Study on Spalling Failure Around a Tunnel in Synthetic Marble. Rock Mech. Rock Eng. 2020, 53, 909–926. [Google Scholar] [CrossRef]
- Gong, F.; Si, X.; Li, X.; Wang, S. Experimental Investigation of Strain Rockburst in Circular Caverns Under Deep Three-Dimensional High-Stress Conditions. Rock Mech. Rock Eng. 2019, 52, 1459–1474. [Google Scholar] [CrossRef]
- Gong, F.; Luo, Y.; Li, X.; Si, X.; Tao, M. Experimental simulation investigation on rockburst induced by spalling failure in deep circular tunnels. Tunn. Undergr. Space Technol. 2018, 81, 413–427. [Google Scholar] [CrossRef]
- Gong, F.Q.; Luo, Y.; Si, X.F.; Li, X.B. Experimental modelling on rockburst in deep hard rock circular tunnels. Chin. J. Rock Mech. Eng. 2017, 36, 1634–1648. [Google Scholar] [CrossRef]
- Si, X.; Li, X.; Gong, F.; Huang, L.; Liu, X. Experimental investigation of failure process and characteristics in circular tunnels under different stress states and internal unloading conditions. Int. J. Rock Mech. Min. Sci. 2022, 154, 105116. [Google Scholar] [CrossRef]
- Si, X.; Peng, K.; Luo, S. Experimental Investigation on the Influence of Depth on Rockburst Characteristics in Circular Tunnels. Sensors 2022, 22, 3679. [Google Scholar] [CrossRef]
- Si, X.; Huang, L.; Gong, F.; Li, X. Failure process and characteristics of three-dimensional high-stress circular tunnel under saturated water content. Trans. Nonferrous Met. Soc. China 2022, 32, 2696–2708. [Google Scholar] [CrossRef]
- Si, X.F.; Gong, F.Q. Rockburst simulation tests and strength-weakening effect of circular tunnels under deep high stresses and internal unloading conditions. Chin. J. Rock Mech. Eng. 2021, 40, 276–289. [Google Scholar] [CrossRef]
- Bai, Q.; Zhang, C.; Paul Young, R. Using true-triaxial stress path to simulate excavation-induced rock damage: A case study. Int J. Coal Sci. Technol. 2022, 9, 49. [Google Scholar] [CrossRef]
- Yang, S.Q.; Dai, Y.; Han, L.; He, Y.; Li, Y. Uniaxial Compression Experimental Research On Deformation And Failure Properties Of Brittle Marble Specimen With Pre-Existing Fissures. Chin. J. Rock Mech. Eng. 2009, 28, 2391–2404. [Google Scholar]
- Tyler, S.W.; Wheatcraft, S.W. Fractal Scaling of Soil Particle-Size Distributions: Analysis and Limitations. Soil Sci. Soc. Am. J. 1992, 56, 362–369. [Google Scholar] [CrossRef]
- Yu, L.; Peng, K.; Luo, S.; Wang, Y.; Luo, K. Failure process and characteristics of deep concrete-supported arch tunnel under true-triaxial stress. Theor. Appl. Fract. Mech. 2024, 130, 104295. [Google Scholar] [CrossRef]
- Zhou, H.; Xu, R.C.; Lu, J.J.; Zhang, C.Q.; Meng, F.Z.; Shen, Z. Experimental Investigations And Mechanism Analysis Of Anchoring Effect Of Pre-Stressed Bolts For Slabbing Surrounding Rock In Deep Tunnel. Chin. J. Rock Mech. Eng. 2015, 34, 1081–1090. [Google Scholar] [CrossRef]
- Culshaw, M.G.; Ulusay, R. (Eds.) The ISRM Suggested Methods for Rock Characterization, Testing and Monitoring: 2007–2014; Springer: Berlin/Heidelberg, Germany, 2015. [Google Scholar]
- Sun, Y.; Tan, C.X. An analysis of present-day regional tectonic stress field and crustal movement trend in China. J. Geom. 1995, 1, 1–12. [Google Scholar]
- Chen, L.; Guo, W.; Zhang, D.; Zhao, T. Experimental study on the influence of prefabricated fissure size on the directional propagation law of rock type-I crack. Int. J. Rock Mech. Min. Sci. 2022, 160, 105274. [Google Scholar] [CrossRef]
- Wang, B.; Ning, Y.; Feng, T.; Zneg, Z.M.; Guo, Z.Y. Meso-Mechanism Of Rock Failure Influenced By Bolt Anchorage Under Uniaxial Compression Loading. Chin. J. Geotech. Eng. 2018, 40, 1593–1600. [Google Scholar] [CrossRef]







| Density (kg∙m−3) | P-Wave Propagation Velocity (m/s) | Uniaxial Compressive Strength (MPa) | Poisson’s Ratio | Young’s Modulus (GPa) | Rockburst Tendency |
|---|---|---|---|---|---|
| 2451 | 3034 | 98.39 | 0.21 | 18.60 | moderate |
| Specimen Number | Hole Area (cm2) | Contact Area (cm2) | Loading Rate (N/s) |
|---|---|---|---|
| S-0-0 | 9 | 91 | 910 |
| S-0-25, S-0-50, S-0-100 | 9.4 | 90.6 | 906 |
| Fissure Penetration/% | 0 | 25 | 50 | 100 |
|---|---|---|---|---|
| Clam stage/s | 890.64 | 978.12 | 1188.72 | 533.52 |
| Fine particle spalling stage/s | 766.8 | 183.6 | 55.44 | 96.84 |
| Crack generation and propagation stage/s | 67.32 | 161.64 | 238.32 | 178.92 |
| Spalling and failure stage/s | 76.32 | 92.16 | 18.72 | 31.32 |
| Total/s | 910.44 | 437.4 | 312.48 | 307.08 |
| Specimen | Initial Failure Stress/MPa | Peak Stress/MPa |
|---|---|---|
| S-0-0 | 47.50 | 72.00 |
| S-0-25 | 49.86 | 61.66 |
| S-0-50 | 54.00 | 64.00 |
| S-0-100 | 36.77 | 50.00 |
| R (mm) | RL (mm) | S-0-0 | S-0-25 | S-0-50 | S-0-100 | ||||
|---|---|---|---|---|---|---|---|---|---|
| M (r < R) (g) | MT (g) | M (r < R) (g) | MT (g) | M (r < R) (g) | MT (g) | M (r < R) (g) | MT (g) | ||
| 0.075 | 15 | 0.89 | 39.73 | 0.48 | 31.57 | 0.15 | 20.03 | 0.17 | 10.12 |
| 0.25 | 15 | 3.49 | 39.73 | 2.12 | 31.57 | 0.84 | 20.03 | 0.82 | 10.12 |
| 0.55 | 15 | 5.31 | 39.73 | 3.11 | 31.57 | 1.38 | 20.03 | 1.18 | 10.12 |
| 1 | 15 | 7.17 | 39.73 | 3.96 | 31.57 | 1.85 | 20.03 | 1.59 | 10.12 |
| 2.36 | 15 | 10.65 | 39.73 | 5.83 | 31.57 | 2.75 | 20.03 | 2.39 | 10.12 |
| 4.75 | 15 | 17.07 | 39.73 | 8.71 | 31.57 | 4.72 | 20.03 | 3.59 | 10.12 |
| 6.7 | 15 | 20.84 | 39.73 | 10.51 | 31.57 | 5.78 | 20.03 | 4.46 | 10.12 |
| 8 | 15 | 25.24 | 39.73 | 19.39 | 31.57 | 11.73 | 20.03 | 6.32 | 10.12 |
| 15 | 15 | 39.73 | 39.73 | 31.57 | 31.57 | 20.03 | 20.03 | 10.12 | 10.12 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, J.; Peng, K.; Luo, K.; Luo, S.; Jing, M. Effect of Fissure Penetration Degrees on Spalling Process and Feature of Surrounding Rock in Rectangular Tunnel Under True Triaxial Loading. Appl. Sci. 2025, 15, 2780. https://doi.org/10.3390/app15052780
Zhang J, Peng K, Luo K, Luo S, Jing M. Effect of Fissure Penetration Degrees on Spalling Process and Feature of Surrounding Rock in Rectangular Tunnel Under True Triaxial Loading. Applied Sciences. 2025; 15(5):2780. https://doi.org/10.3390/app15052780
Chicago/Turabian StyleZhang, Jie, Kang Peng, Kun Luo, Song Luo, and Mao Jing. 2025. "Effect of Fissure Penetration Degrees on Spalling Process and Feature of Surrounding Rock in Rectangular Tunnel Under True Triaxial Loading" Applied Sciences 15, no. 5: 2780. https://doi.org/10.3390/app15052780
APA StyleZhang, J., Peng, K., Luo, K., Luo, S., & Jing, M. (2025). Effect of Fissure Penetration Degrees on Spalling Process and Feature of Surrounding Rock in Rectangular Tunnel Under True Triaxial Loading. Applied Sciences, 15(5), 2780. https://doi.org/10.3390/app15052780







