Study of Reasonable Roof Cutting Parameters of Dense-Drilling Roof Cutting and Pressure Relief Self-Forming Roadway in Non-Pillar Mining
Abstract
1. Introduction
2. Engineering Background
3. Determination of Key Parameters
3.1. The Control Mechanism of Roof Cutting Height on Roof Breaking
3.2. The Control Mechanism of Roof Cutting Angle on Roof Breaking
3.3. Control Mechanism of Aperture on Roof Breaking
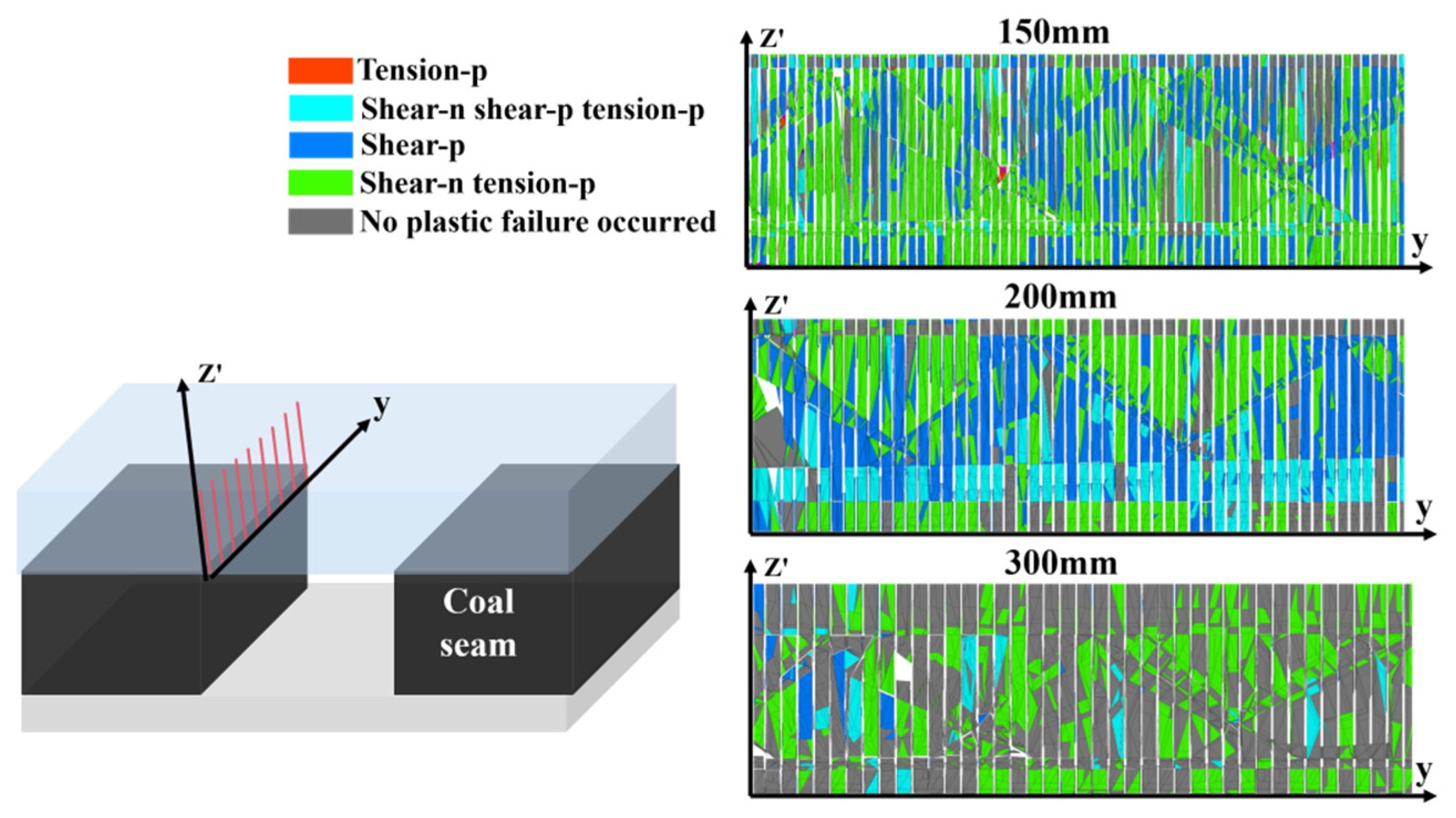
3.4. The Influence Mechanism of Hole Spacing on the Plastic Zone of Round Hole
4. Numerical Simulation of Roof Cutting Parameters
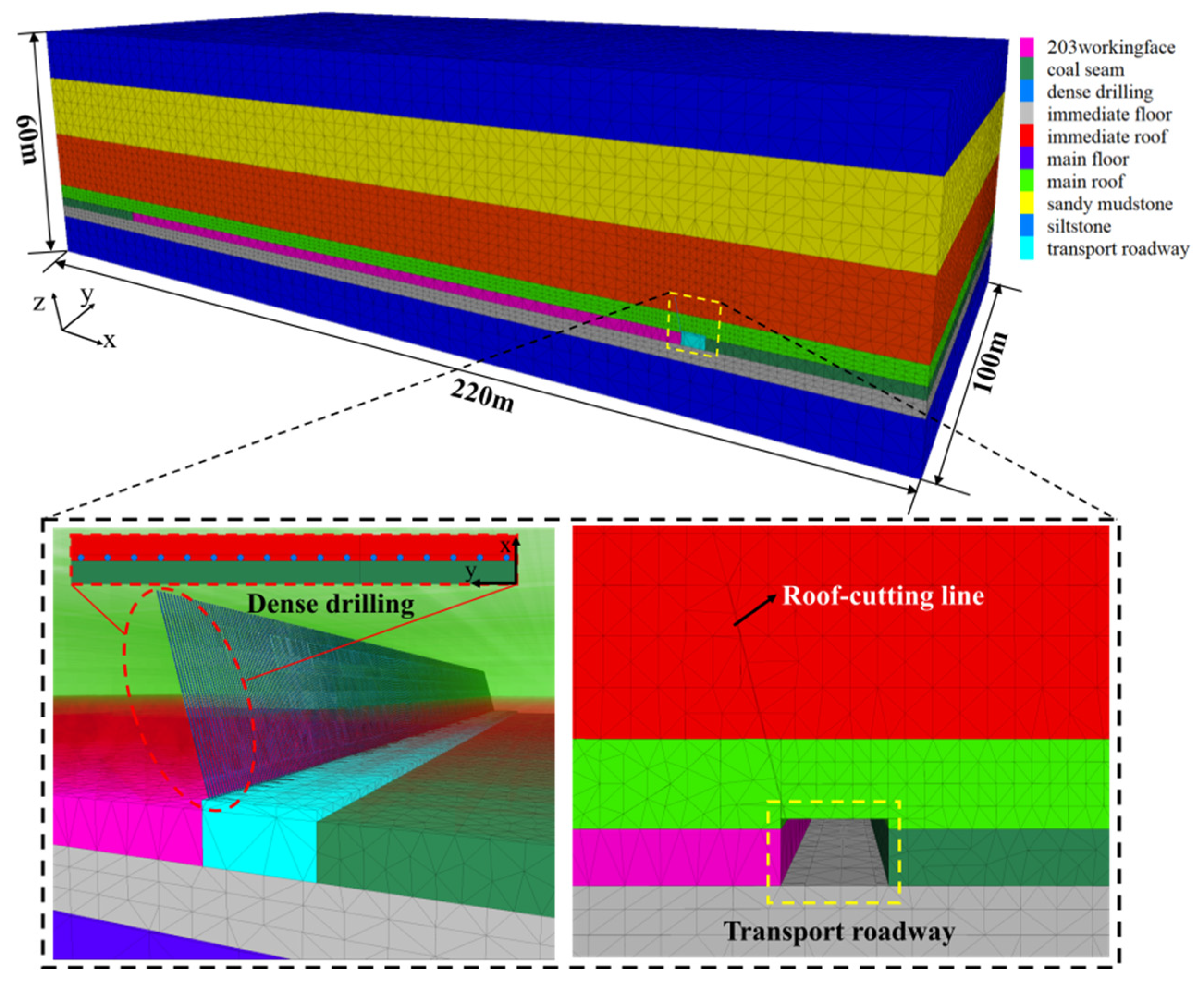
4.1. Establishment of Numerical Model
4.2. Numerical Simulation Experiment Scheme
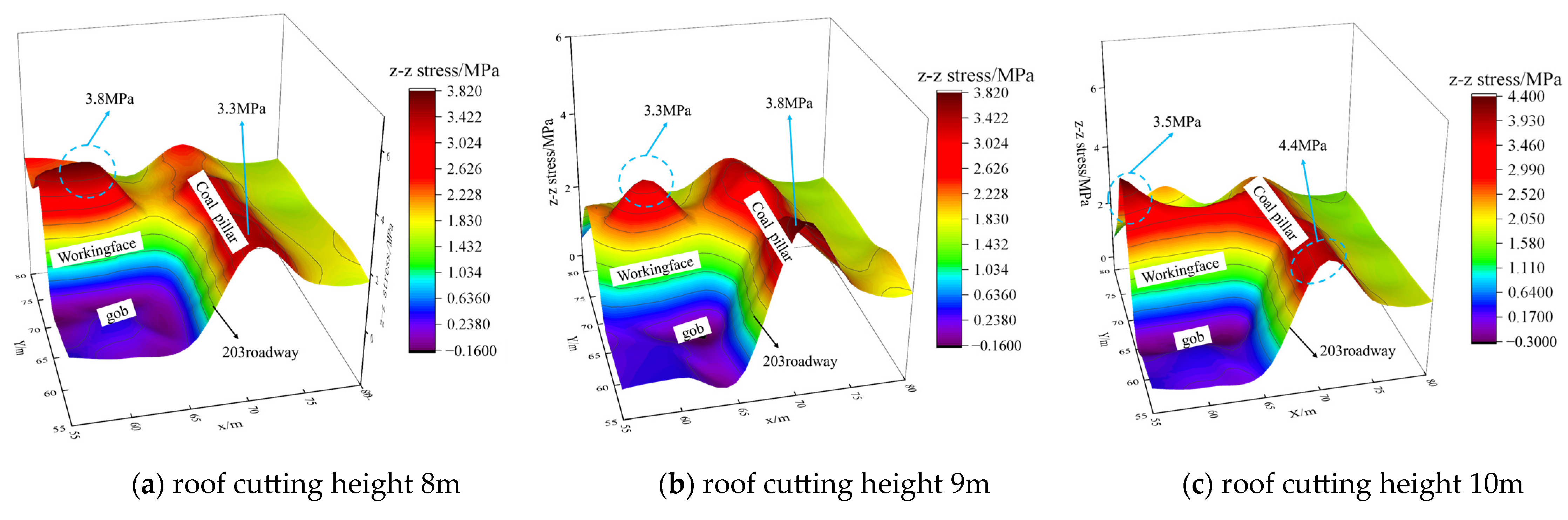
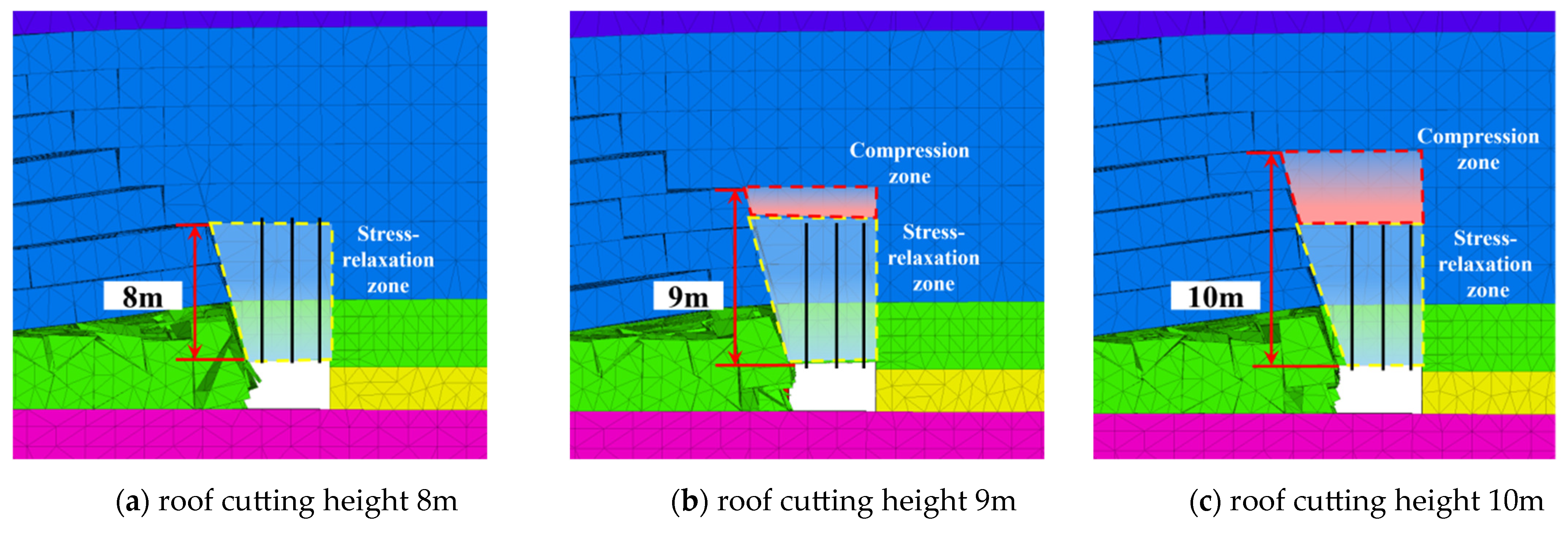
4.3. Numerical Simulation of Cutting Height
4.3.1. Vertical Stress Distribution Law of Surrounding Rock of Roadway with Different Roof Cutting Heights
4.3.2. Load-Bearing Characteristics of Roof Cutting ‘Key Structure’
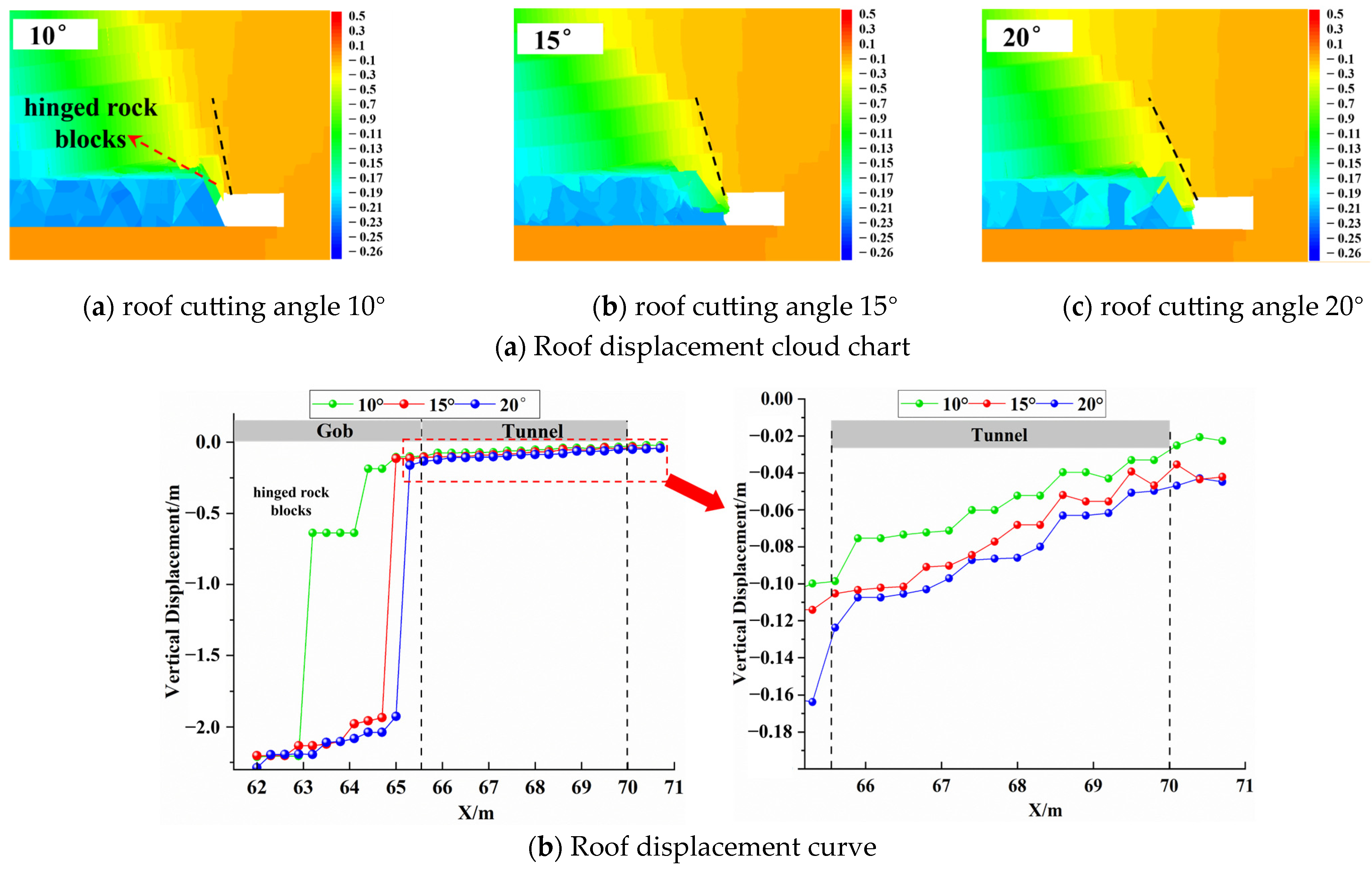
4.4. Numerical Simulation of Cutting Angle
4.4.1. Vertical Stress Distribution Law of Surrounding Rock of Roadway with Different Roof Cutting Angles
4.4.2. Roof Displacement Distribution Law of Different Roof Cutting Angles
4.5. Numerical Simulation of Borehole Spacing
5. Field Engineering Practice
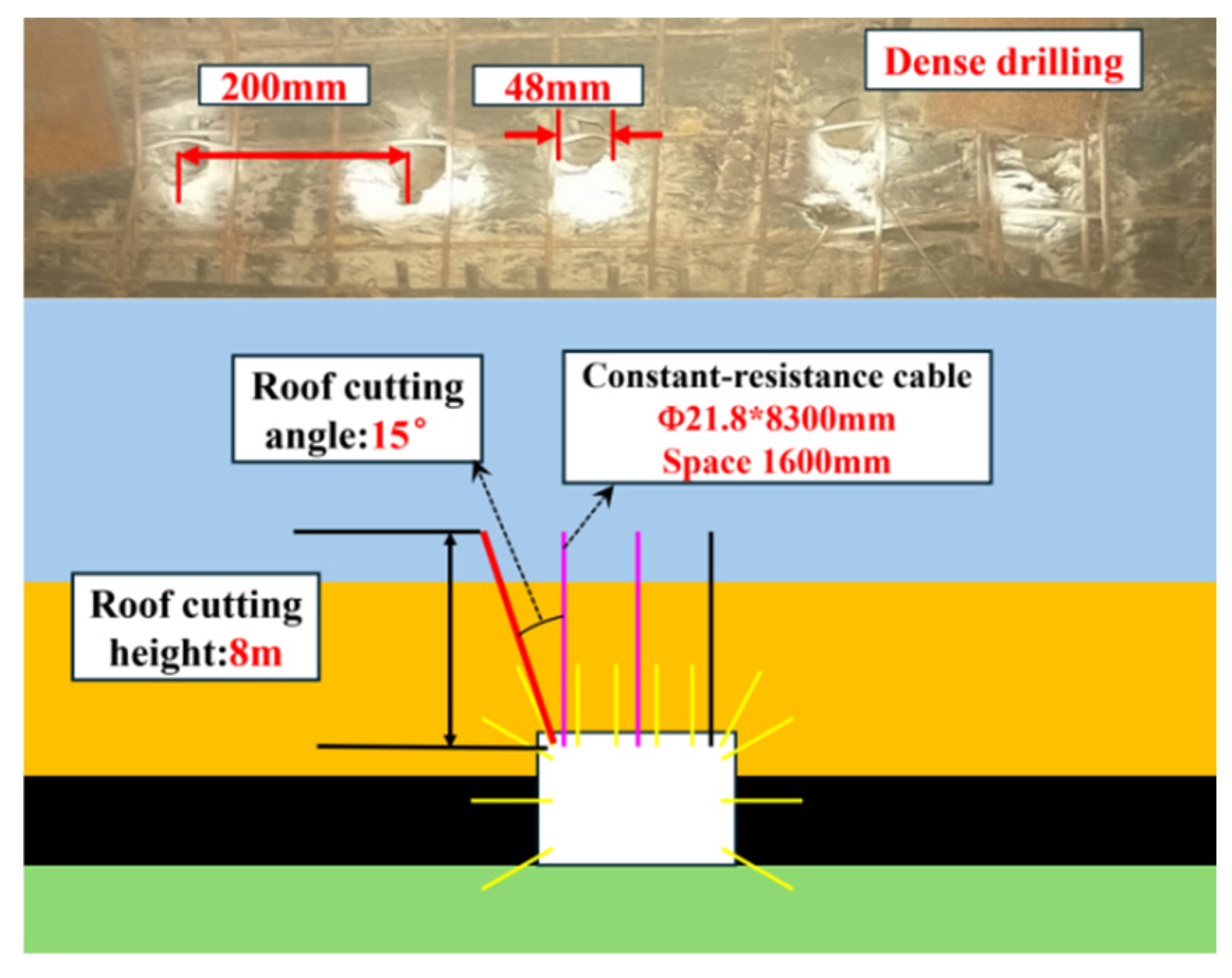
5.1. Dense-Drilling Roof Cutting Layout Scheme
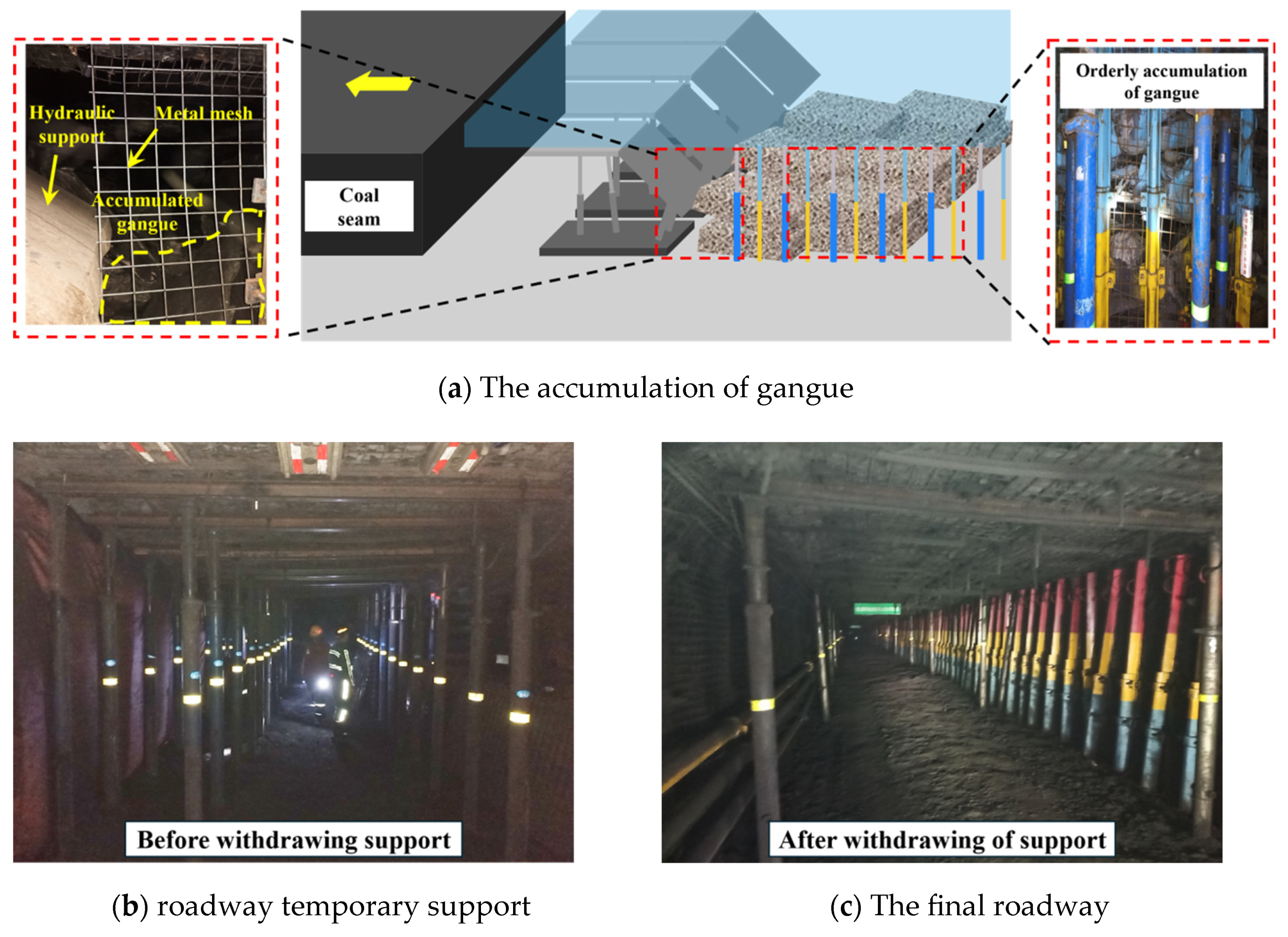
5.2. Engineering Practice Effect
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hua, X. Development status and improvement suggestions of gob-side entry retaining support technology in China. Coal Sci. Technol. 2006, 12, 78–81. [Google Scholar]
- Hua, X.; Li, C.; Liu, X.; Guo, Y.; Qi, Y.; Chen, D. Review the development status and improvement suggestions of gob side entry retaining technology in China. Coal Sci. Technol. 2013, 51, 128–145. [Google Scholar]
- He, M.; Song, Z.; Wang, A.; Yang, H.; Qi, H.; Guo, Z. Longwall mining roof cutting short wall beam theory and its 110 method-the third mining science and technology change. Coal Sci. Technol. 2017, 11, 1–9. [Google Scholar]
- He, M.; Gong, W.; Wang, J.; Qi, P.; Tao, Z.; Du, S.; Peng, Y. Development of a novel energy-absorbing bolt with extraordinarily large elongation and constant resistance. Int. J. Rock Mech. Min. Sci. 2014, 67, 29–42. [Google Scholar] [CrossRef]
- Zhang, G.; He, M.; Yu, X.; Huang, Z. Study on the technology of non-pillar mining in the protective layer of Baijiao Mine. Min. Saf. Eng. 2011, 28, 511–516. [Google Scholar]
- Sun, X.; Liu, X.; Liang, G.; Wang, D.; Jiang, Y. Study on key parameters of gob-side entry retaining with roof cutting and pressure relief in thin coal seam. J. Rock Mech. Eng. 2014, 33, 1449–1456. [Google Scholar]
- He, M.; Chen, S.; Guo, Z.; Yang, J.; Gao, Y. Structural control of surrounding rock of gob-side entry retaining with roof cutting and pressure relief and its engineering application. J. China Univ. Min. Technol. 2017, 46, 959–969. [Google Scholar]
- He, M.; Gao, Y.; Yang, J.; Guo, Z.; Wang, E.; Wang, Y. Study on energy-gathering cutting technology of self-forming roadway without coal pillar and its influence on stress evolution of surrounding rock. J. Rock Mech. Eng. 2017, 36, 1314–1325. [Google Scholar]
- Lin, J.; Guo, K.; Sun, Z.; Wang, T. Study on the timing of hydraulic fracturing roof cutting and pressure relief fracturing in strong dynamic pressure roadway. J. Coal 2021, 46, 140–148. [Google Scholar]
- Sun, Z.; Zhang, Z.; Wang, Z.; Wang, T.; Fu, Y. Application of hydraulic fracturing roof cutting and pressure relief technology in large mining height roadway. Coal Sci. Technol. 2019, 47, 190–197. [Google Scholar]
- Wu, W.; Wang, T.; Bai, J.; Liu, J.; Wang, X.; Xu, H.; Feng, G. Failure Characteristics and Cooperative Control Strategies for Gob-Side Entry Driving near an Advancing Working Face: A Case Study. Processes 2024, 12, 1398. [Google Scholar] [CrossRef]
- Sun, X.; Qi, Z.; Zhang, Y.; Li, Z.; Xie, C.; Yang, J.; Ding, J.; He, L. Characteristics and Control Measures of Deep and Shallow Dense Drilling in Roadway for Pressure Relieving by Cutting Roof. Min. Metall. Explor. 2024, 41, 787–803. [Google Scholar] [CrossRef]
- Liu, Y.; Kang, T.; Zhang, L.; Zhu, W.; Zhang, G.; Wang, J. Research on roof cutting mechanism and parameter optimization of non-blasting dense drilling. Min. Res. Dev. 2023, 43, 34–39. [Google Scholar]
- Zhao, Y.; Xie, H.; Yao, Q. Research on roof cutting and pressure relief roadway protection technology of dense drilling in Songxinzhuang. Coal Mine. Coal Eng. 2023, 55, 45–51. [Google Scholar]
- Wang, M.; Zheng, D.; Wang, X.; Xiao, T.; Shen, W.; Song, Z. Weakening deformation characteristics and creep control of surrounding rock in deep roadway drilling pressure relief. J. Min. Saf. Eng. 2019, 36, 437–445. [Google Scholar]
- Ma, B.; Deng, Z.; Zhao, S.; Li, S. Analysis of mechanism and influencing factors of rock burst prevention and control by borehole pressure relief. Coal Sci. Technol. 2020, 48, 35–40. [Google Scholar]
- Jia, C.; Jiang, Y.; Zhang, X.; Wang, D.; Luan, H.; Changsheng, W. Indoor and numerical experimental study on pressure relief mechanism of large diameter borehole. J. Geotech. Eng. 2017, 39, 1115–1122. [Google Scholar]
- Ding, K.; Zhang, H.; Wang, L.; Guo, J.; Meng, Y. Damage evolution characteristics of coal body and strength weakening mechanism of borehole surrounding rock in deep roadway. Coal J. 2024, 49, 4798–4821. [Google Scholar]
- Ma, J.-Q.; Li, X.-H.; Yao, Q.-L.; Xia, Z.; Xu, Q.; Shan, C.-H.; Sidorenko, A.; Aparin, A. Numerical simulation on mechanisms of dense drilling for weakening roofs and its application in roof control. J. Cent. South Univ. 2023, 30, 1865–1886. [Google Scholar] [CrossRef]
- Wang, M.; Wang, X.; Xiao, T. Deep roadway drilling pressure relief mechanism and key parameter determination method and application. Coal J. 2017, 42, 1138–1145. [Google Scholar]
- Li, D.; Zhang, J.; Zheng, L.; Wang, S.; Wang, W.; Huang, Z. Dense drilling weakening bottom layered roof gob-side entry retaining technology. Coal Mine Saf. 2022, 53, 111–118. [Google Scholar]
- Sun, B.-J.; Hua, X.-Z.; Zhang, Y.; Yin, J.; He, K.; Zhao, C.; Li, Y.; Sun, Y. Analysis of Roof Deformation Mechanism and Control Measures with Roof Cutting and Pressure Releasing in Gob-Side Entry Retaining. Shock Vib. 2021, 2021, 6677407. [Google Scholar] [CrossRef]
- Wang, Q.; Jiang, Z.; Jiang, B.; He, M.; Yang, J.; Xue, H. Ground Control Method of Using Roof Cutting Pressure Release and Energy-Absorbing Reinforcement for Roadway with Extra-Thick Hard Roof. Rock Mech. Rock Eng. 2023, 56, 7197–7215. [Google Scholar] [CrossRef]
- Han, Z.; Dou, L.; Gong, S.; Kan, J.; Chen, S.; He, X. Mechanism of rock burst in deep gob-side entry based on dynamic and static stress: A case study. Geomat. Nat. Hazards Risk 2023, 14, 2271636. [Google Scholar] [CrossRef]
- Li, X.; Liu, S.; Fu, M.; Peng, B.; He, Y. Research and Application of Key Parameters Influencing Factors of Dense Drilling Roof Cutting Pressure Relief. Coal Sci. Technol. 2023, 51, 243–253. [Google Scholar]
- Liu, S.; Li, X.; Zhu, W.; Fu, M.; Zhang, D.; Peng, B. Roof cutting mechanism and key parameter determination method of dense drilling in gob-side entry retaining. Coal Sci. Technol. 2024, 52, 23–33. [Google Scholar]
- Wang, Q.; Wei, H.; Jiang, B.; Wang, X.; Sun, L.; He, M. High pre-tension reinforcing technology and design for ultra-shallow buried large-span urban tunnels. Int. J. Rock Mech. Min. Sci. 2024, 182, 105891. [Google Scholar] [CrossRef]
- Liang, S.; Zhang, L.; Ge, D.; Wang, Q.; Feng, G. Study on Pressure Relief Effect and Rock Failure Characteristics with Different Borehole Diameters. Shock Vib. 2021, 2021, 3565344. [Google Scholar] [CrossRef]
- Jiang, B.; Ma, F.; Gao, H.; Wang, Q.; Cai, S.; Zhang, C.; Bian, Z.; Liu, G. The response mechanism and testing method of the rock elastic modulus while drilling. Geomat. Nat. Hazards Risk 2024, 15, 2354498. [Google Scholar] [CrossRef]
- MT/T 984-2006; Coal Industry Standard of the People’s Republic of China MT/T984-2006, Diamond Composite Anchor Bit for Coal Mine. Xi’an Branch of CCRI: Xi’an, China, 2006.
- Guo, Z.; Zhao, Y.; Yang, D.; Guo Ji Yin, S.; Qiu, X. Research on roof deformation mechanism and control technology of cross-fault roof cutting and pressure relief self-forming roadway. Coal Sci. Technol. 2024, 52, 14–28. [Google Scholar]

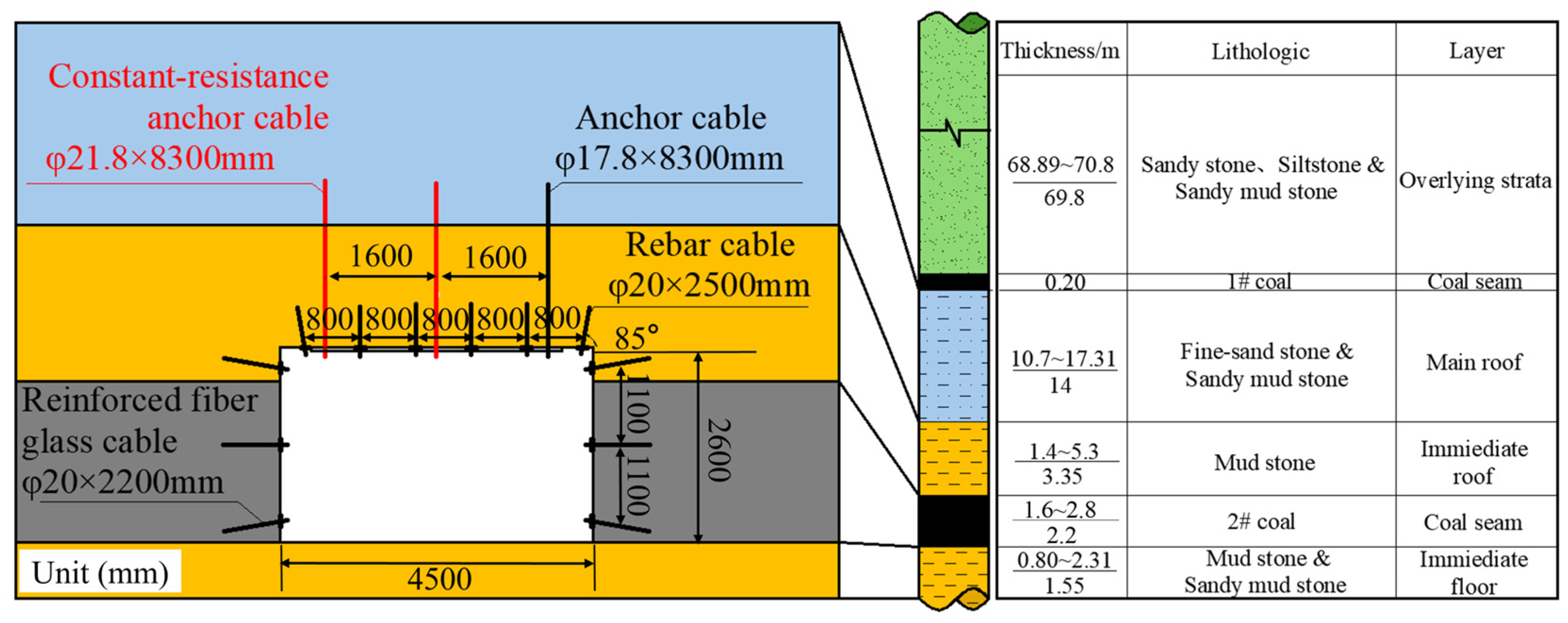
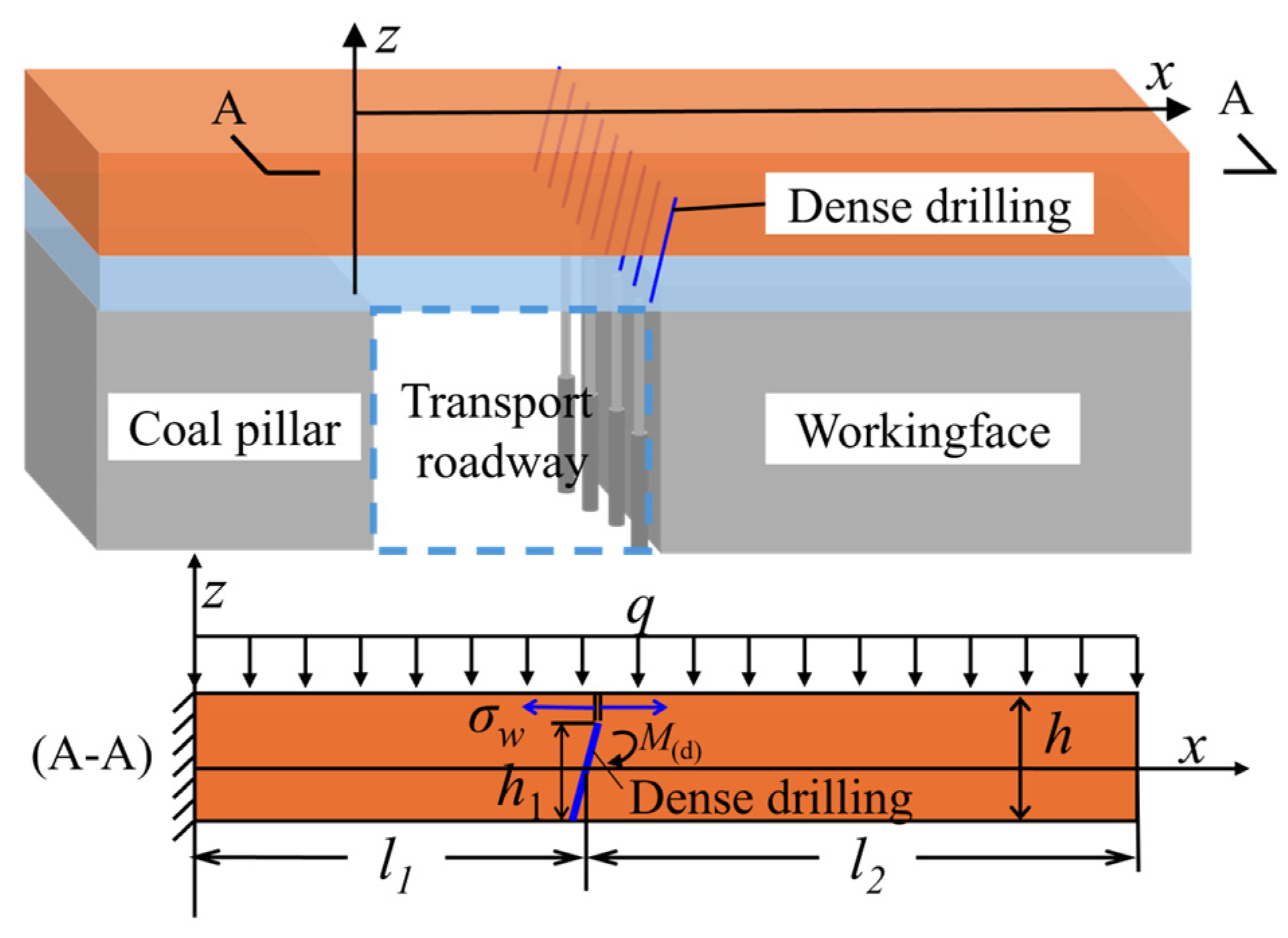
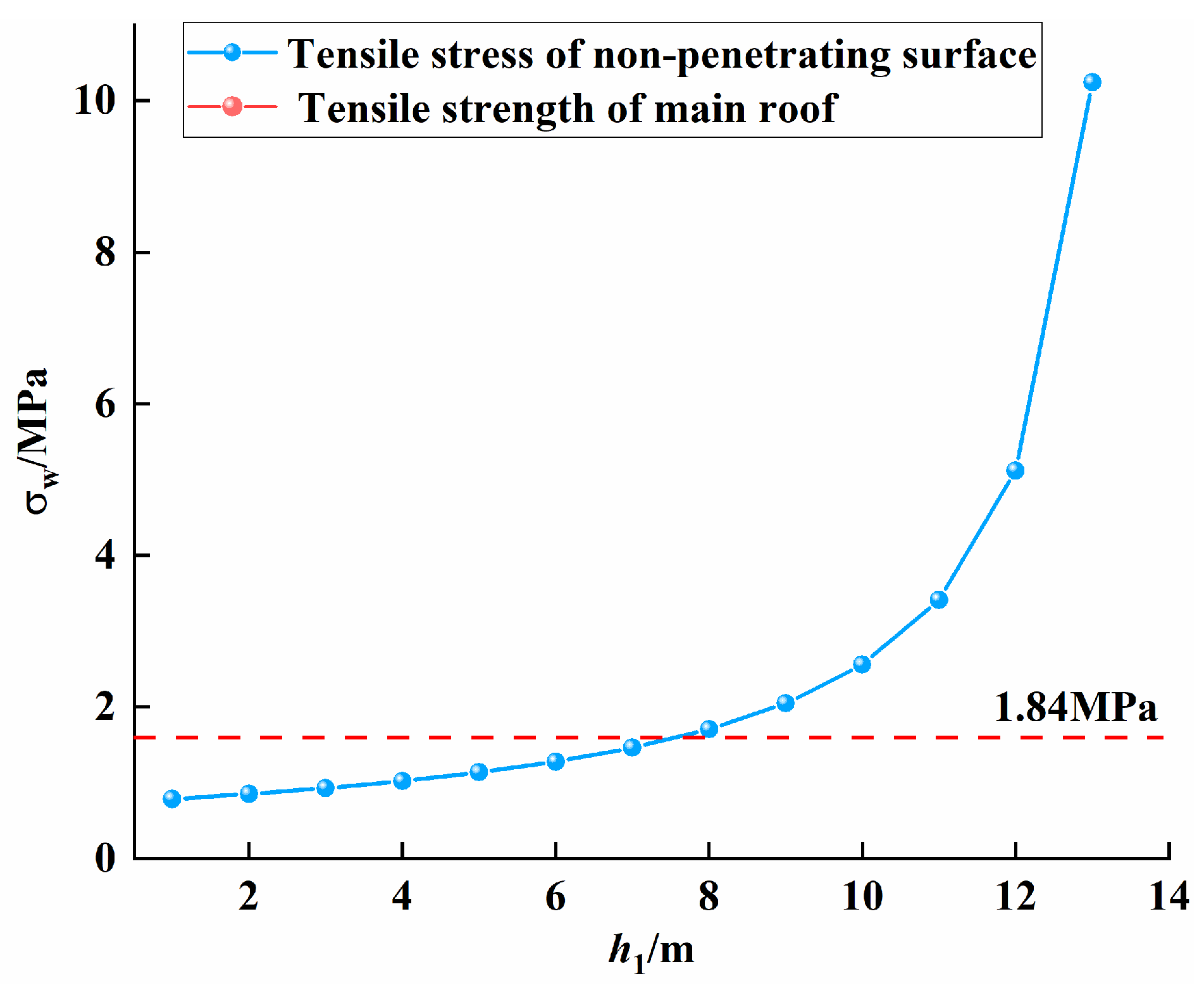

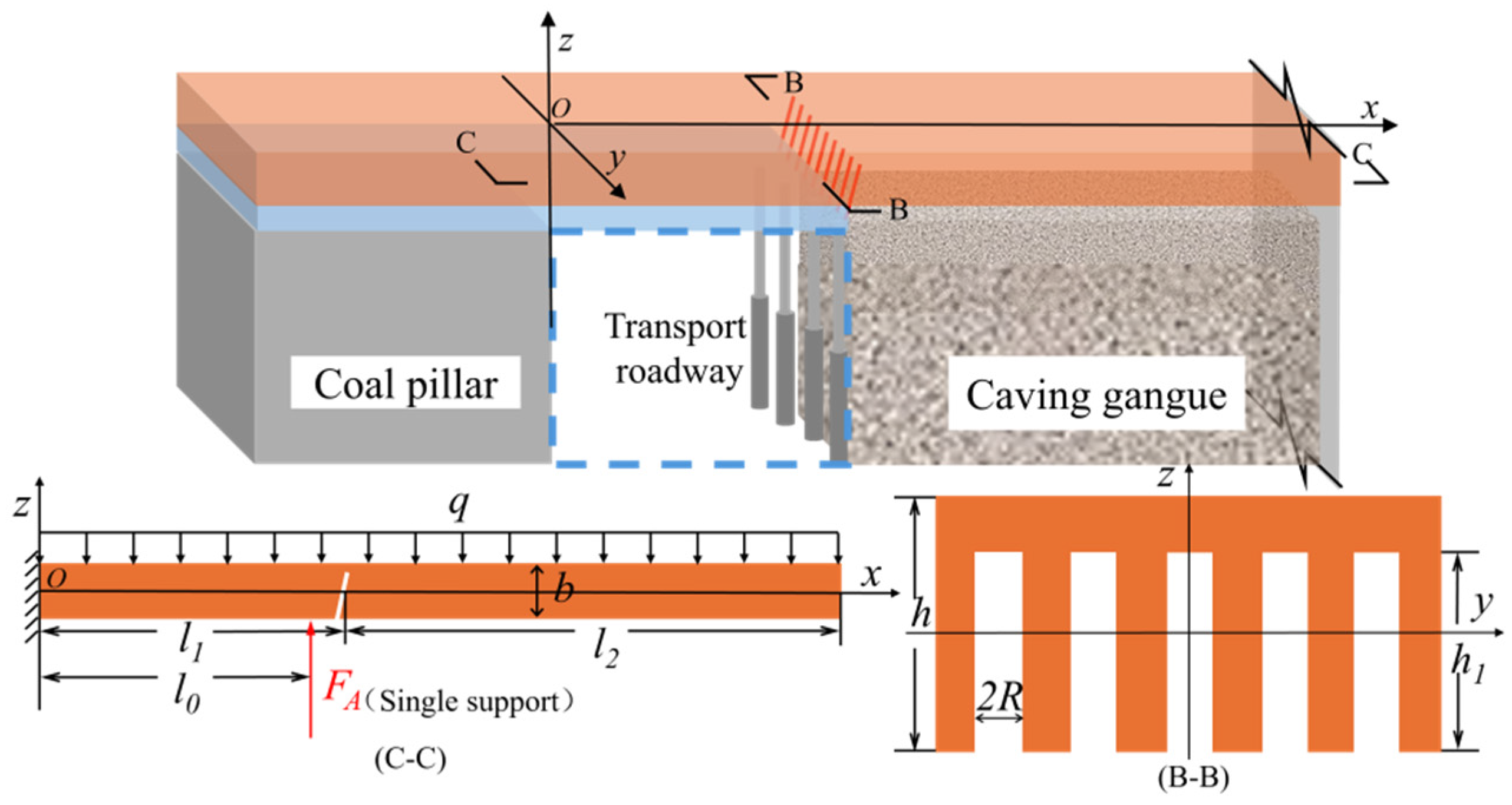




| Name | Lithologic Characters | Volumetric Weight/(kg/m3) | Bulk Modulus/(GPa) | Shear Modulus/(GPa) | Internal Friction Angle/(°) | Cohesion/(MPa) |
|---|---|---|---|---|---|---|
| Overlying strata | Siltstone | 2460 | 10.83 | 8.13 | 38 | 2.75 |
| Main roof | Fine sandstone | 2873 | 21.01 | 13.52 | 42 | 3.20 |
| Immediate roof | Mudstone | 2463 | 5.74 | 3.44 | 30 | 1.25 |
| Coal seam | 2# Coal | 1380 | 4.91 | 2.01 | 32 | 1.25 |
| Immediate floor | Mudstone | 2461 | 6.08 | 3.47 | 30 | 1.20 |
| Main floor | Sandy mudstone | 2510 | 2.56 | 2.36 | 36 | 2.16 |
| 2R | 48 | 55 | 65 | 75 | 94 |
|---|---|---|---|---|---|
| R0/mm | 83 | 95 | 112 | 129 | 162 |
| D/mm | 166 | 190 | 224 | 258 | 324 |
| Lithologic Character | Cohesion/MPa | Angle of Internal Friction/(°) | Joint Normal Stiffness/(Gpa·m−1) | Joint Shear Stiffness/(Gpa·m−1) |
|---|---|---|---|---|
| Siltstone | 2.7 | 15 | 1.05 | 1.35 |
| Fine sandstone | 1.8 | 12 | 0.73 | 0.48 |
| Mudstone | 1.1 | 20 | 0.65 | 0.25 |
| 2# Coal seam | 0.6 | 10 | 0.33 | 0.12 |
| Mudstone | 1.1 | 20 | 0.65 | 0.25 |
| Sandy mudstone | 1.3 | 16 | 0.75 | 0.72 |
| Serial Number | Scheme | Variable | Invariant |
|---|---|---|---|
| I | Change the roof cutting height | 8 m | Roof cutting angle 15° Diameter of borehole 48 mm Hole spacing 200 mm |
| 9 m | |||
| 10 m | |||
| II | Change the roof cutting angle | 10° | Roof cutting height 8 m Diameter of borehole 48 mm Hole spacing 200 mm |
| 15° | |||
| 20° | |||
| III | Change the hole spacing | 150 mm | Roof cutting height 8 m Diameter of borehole 48 mm Roof cutting angle 15° |
| 200 mm | |||
| 300 mm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lang, D.; Chen, S.; Yuan, H.; Yu, J.; Yu, Y.; Luo, S.; Hu, B.; Xie, P. Study of Reasonable Roof Cutting Parameters of Dense-Drilling Roof Cutting and Pressure Relief Self-Forming Roadway in Non-Pillar Mining. Appl. Sci. 2025, 15, 2685. https://doi.org/10.3390/app15052685
Lang D, Chen S, Yuan H, Yu J, Yu Y, Luo S, Hu B, Xie P. Study of Reasonable Roof Cutting Parameters of Dense-Drilling Roof Cutting and Pressure Relief Self-Forming Roadway in Non-Pillar Mining. Applied Sciences. 2025; 15(5):2685. https://doi.org/10.3390/app15052685
Chicago/Turabian StyleLang, Ding, Shuaiming Chen, Hongping Yuan, Jiandong Yu, Yang Yu, Shenghu Luo, Bosheng Hu, and Panshi Xie. 2025. "Study of Reasonable Roof Cutting Parameters of Dense-Drilling Roof Cutting and Pressure Relief Self-Forming Roadway in Non-Pillar Mining" Applied Sciences 15, no. 5: 2685. https://doi.org/10.3390/app15052685
APA StyleLang, D., Chen, S., Yuan, H., Yu, J., Yu, Y., Luo, S., Hu, B., & Xie, P. (2025). Study of Reasonable Roof Cutting Parameters of Dense-Drilling Roof Cutting and Pressure Relief Self-Forming Roadway in Non-Pillar Mining. Applied Sciences, 15(5), 2685. https://doi.org/10.3390/app15052685





