Research on the Reciprocating Friction and Installation Force of Seals in Deep-Sea Samplers
Abstract
1. Introduction
2. Methods
2.1. Numerical Simulation
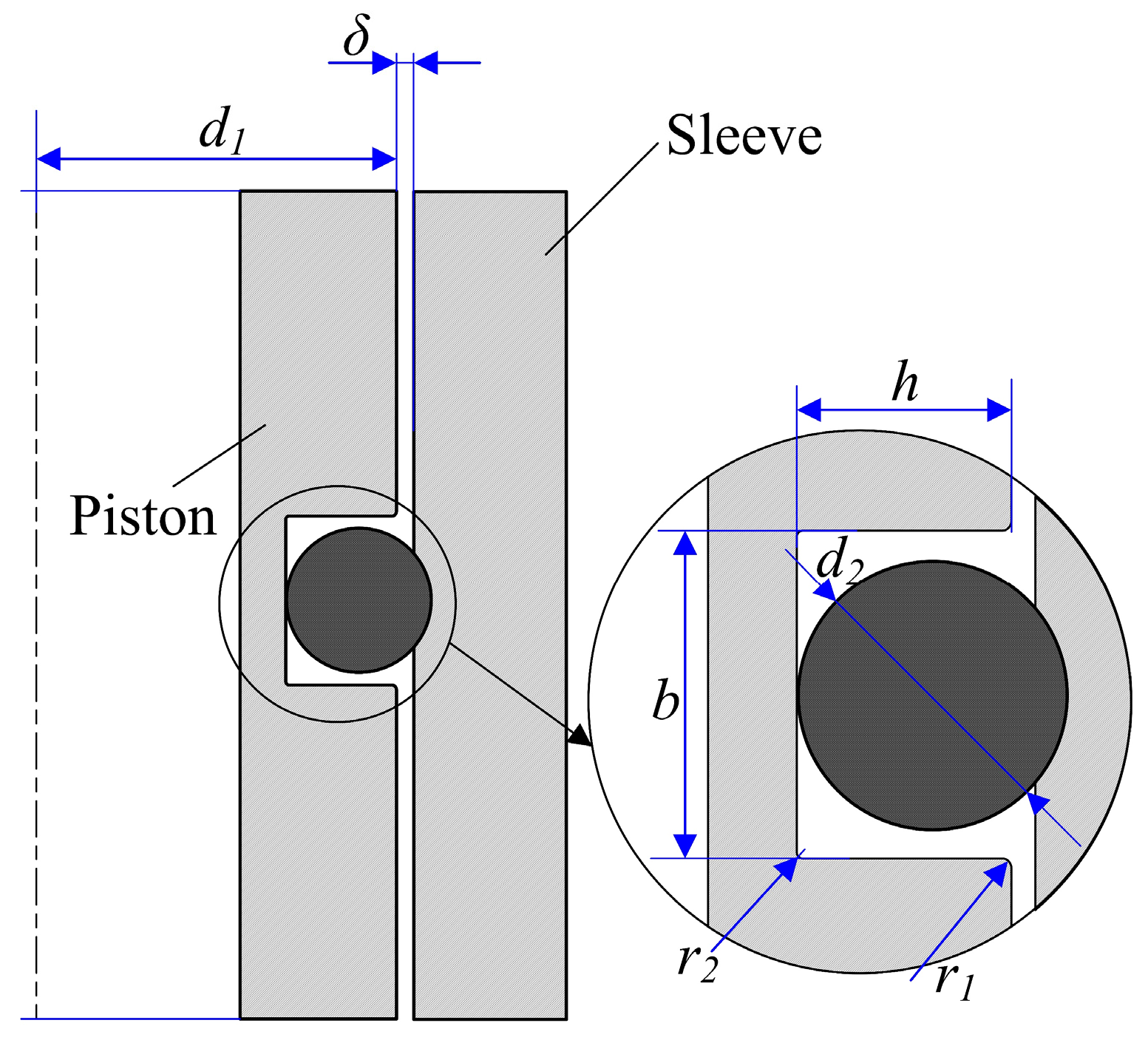
2.1.1. Geometry and Materials
2.1.2. Parameter Setting
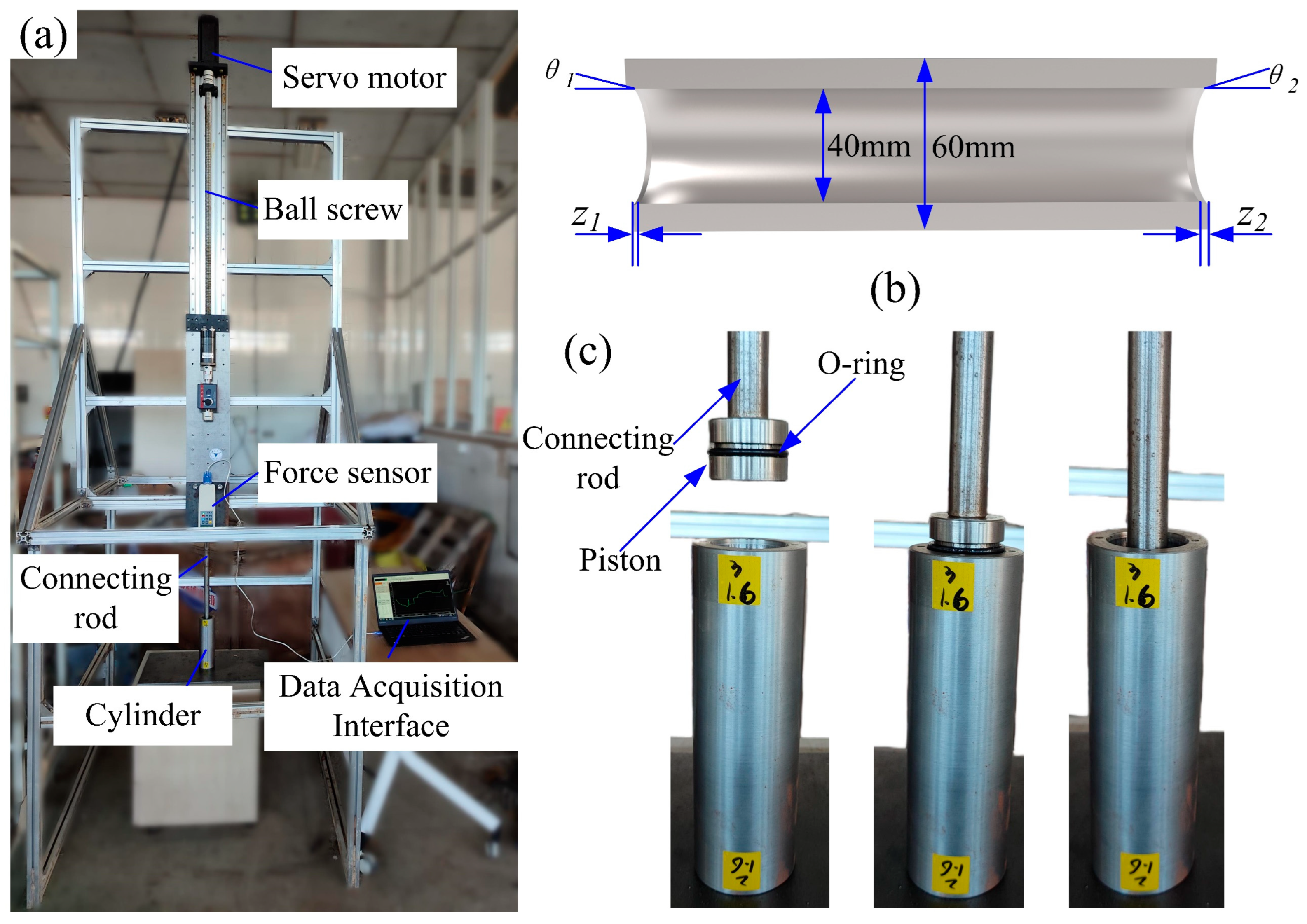
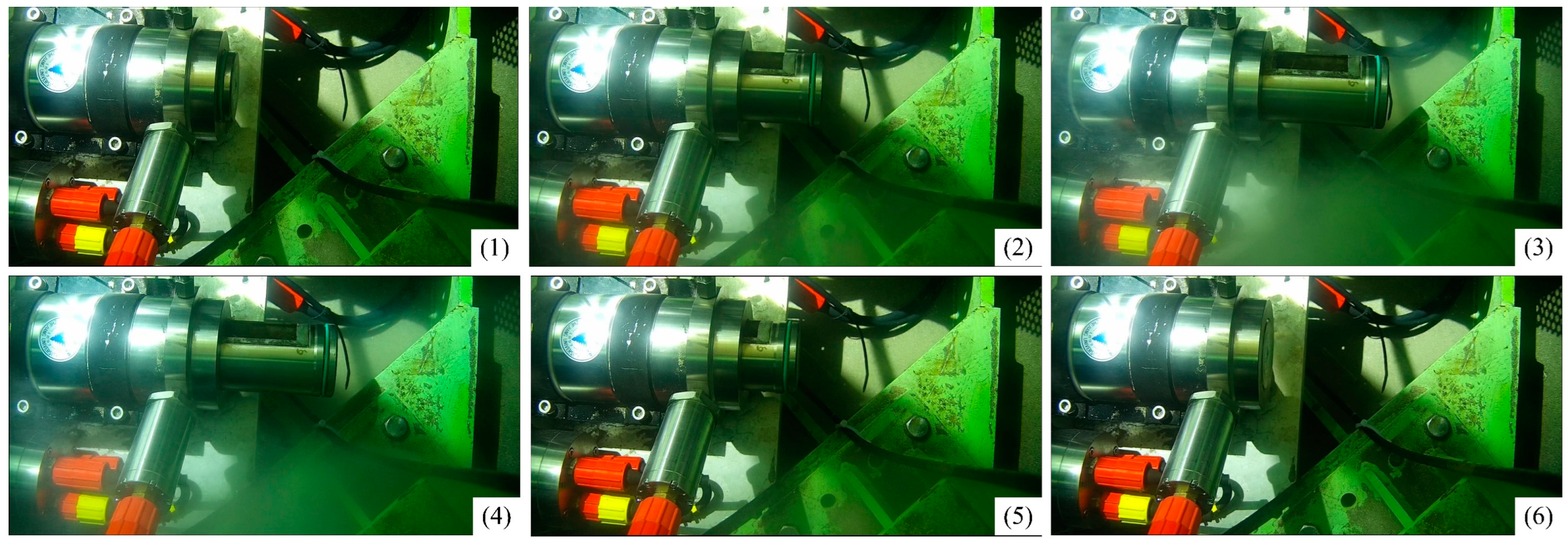
2.2. Experimental Study
2.2.1. Experiment at Room Temperature and Atmospheric Pressure
2.2.2. Experiment Under High Pressure and Low Temperature
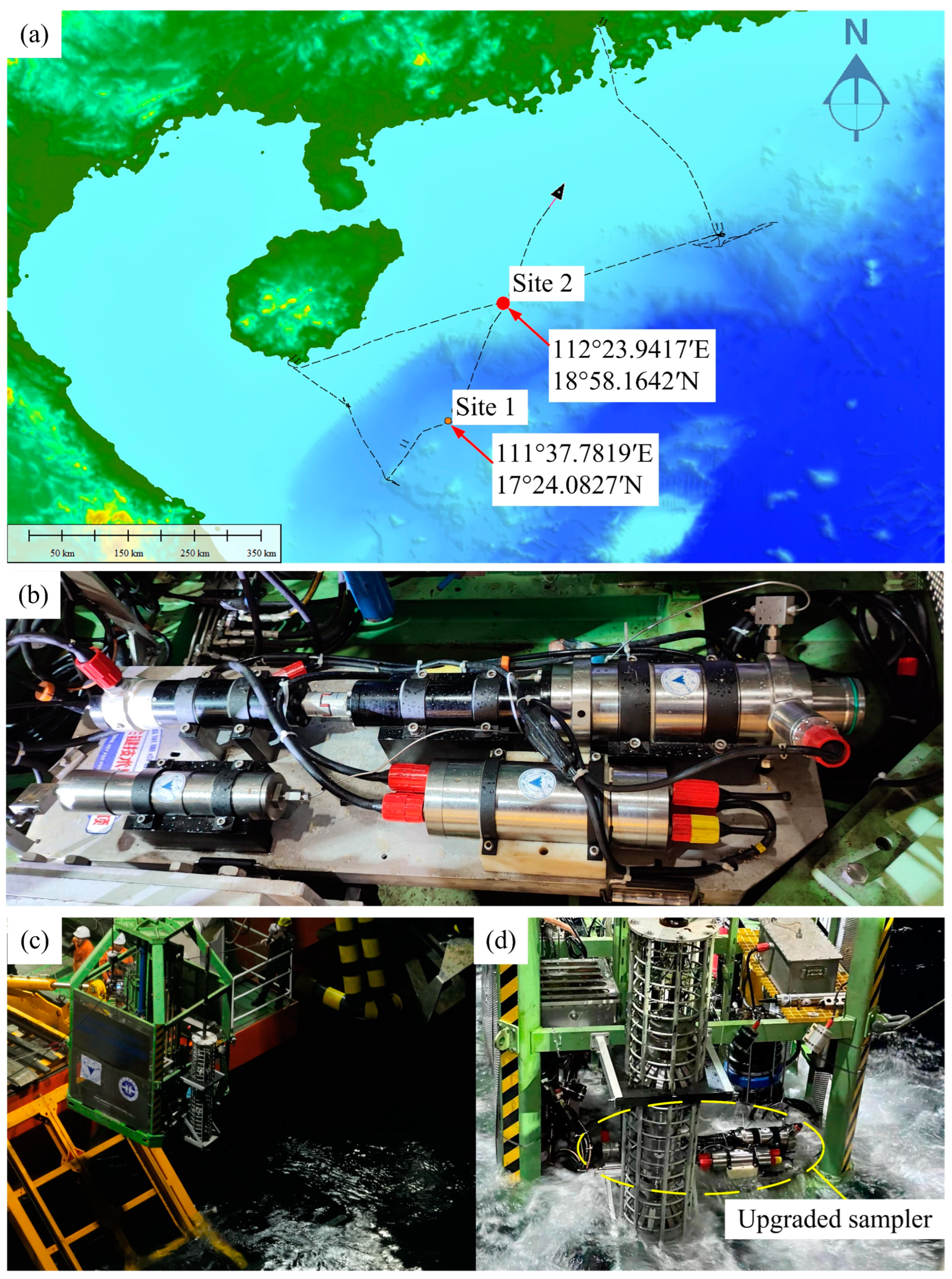
2.3. Field Test of the Upgraded Sampler
3. Results
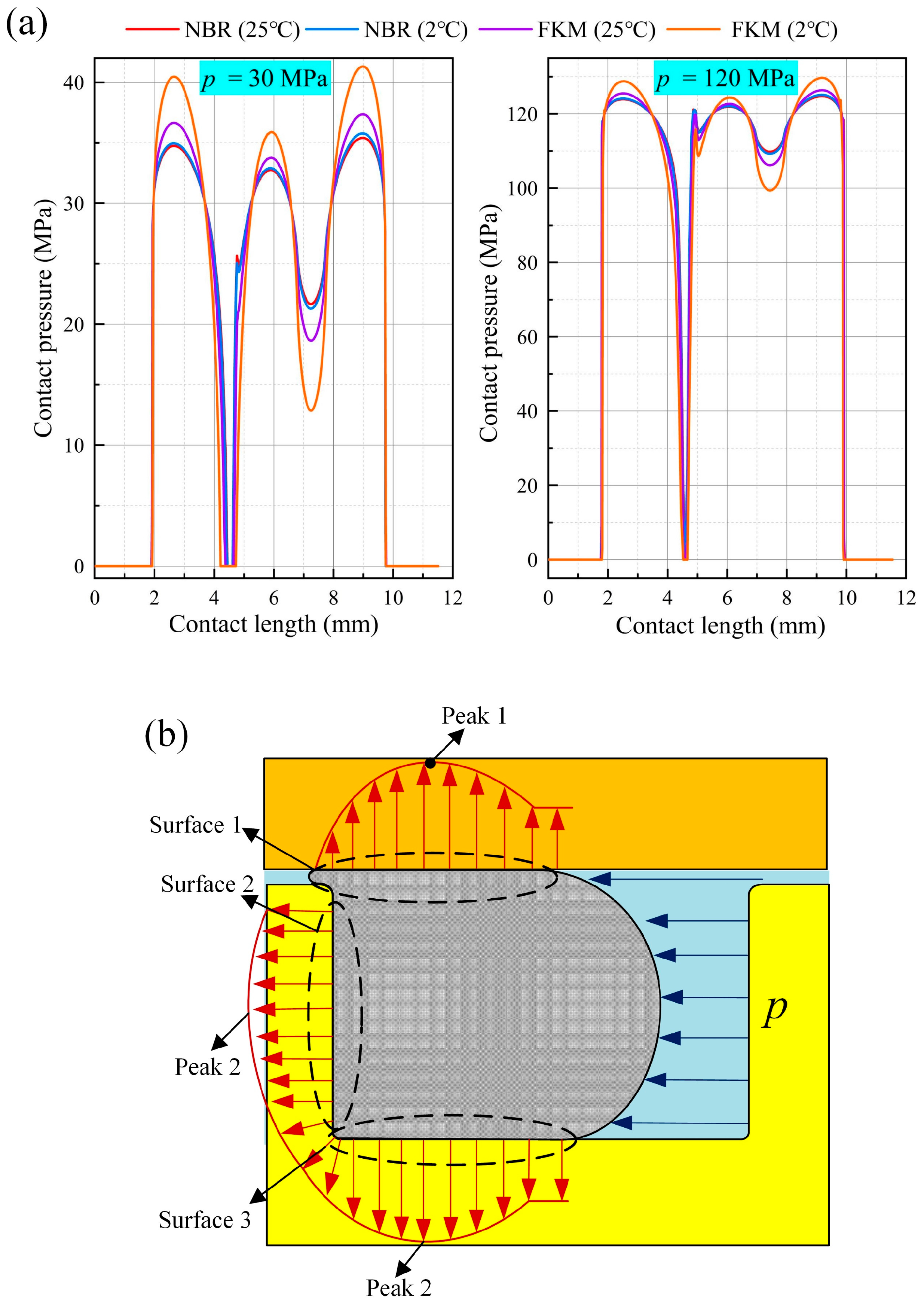
3.1. Results of Numerical Simulation
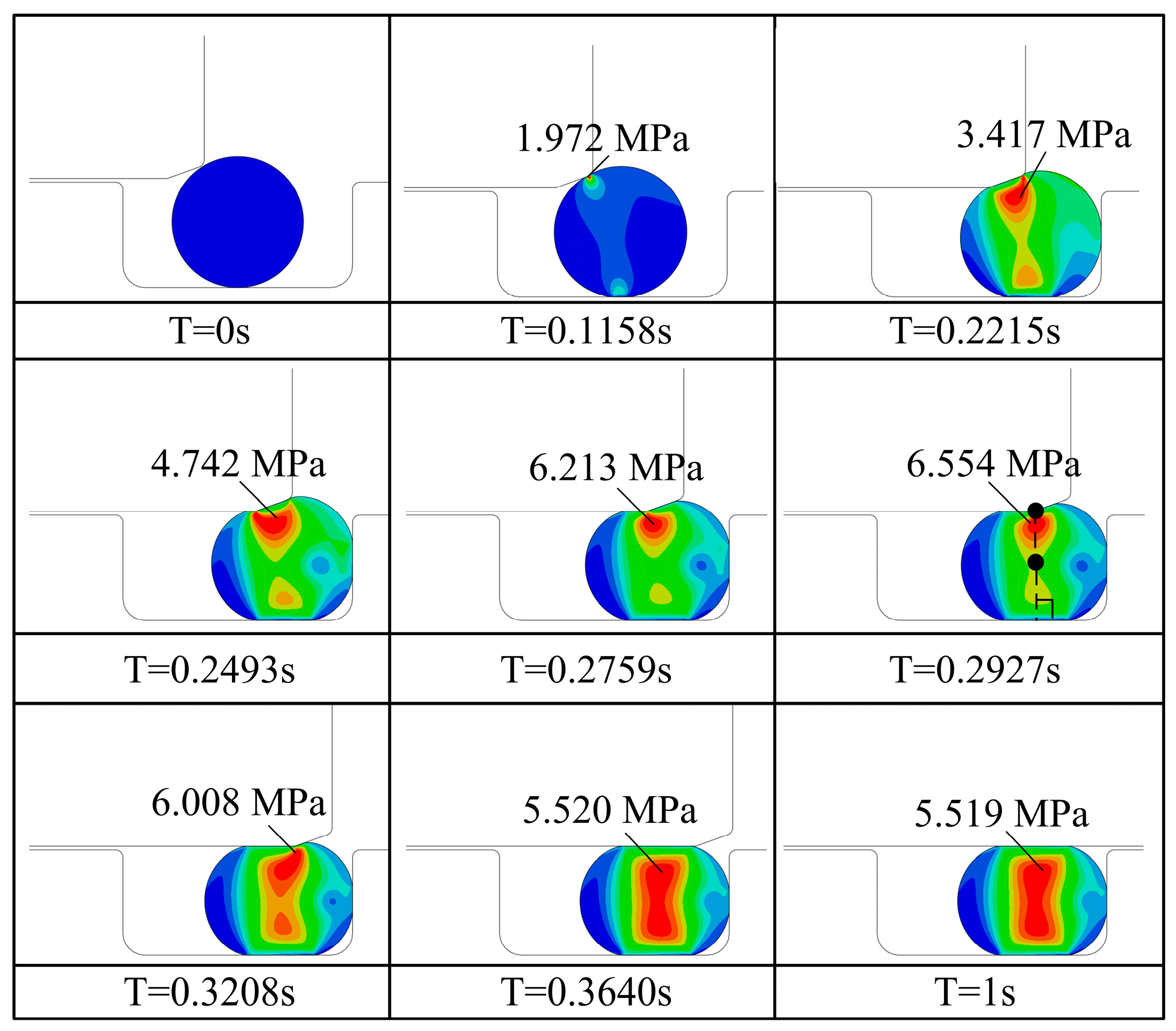
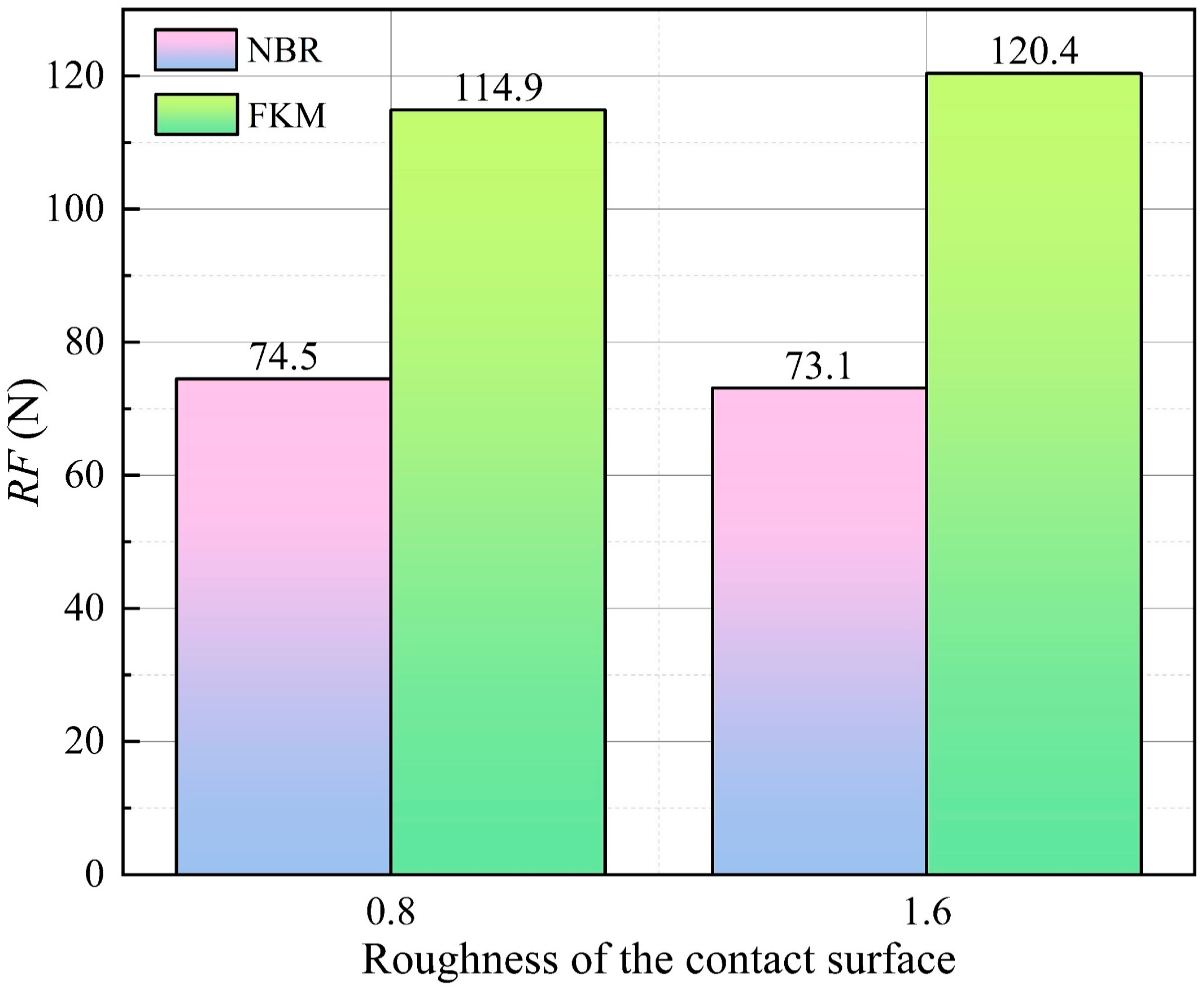
3.1.1. The Installation Force
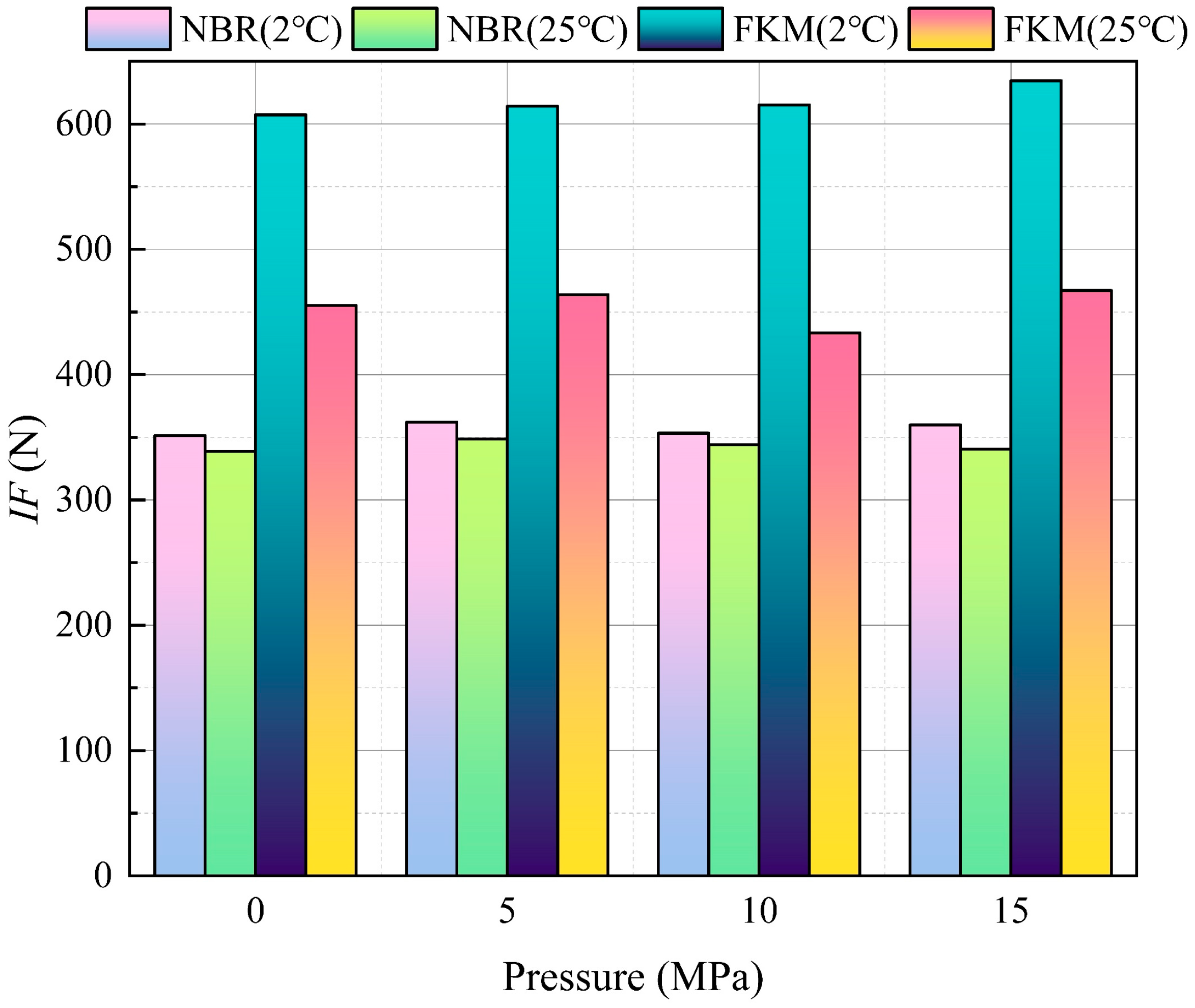
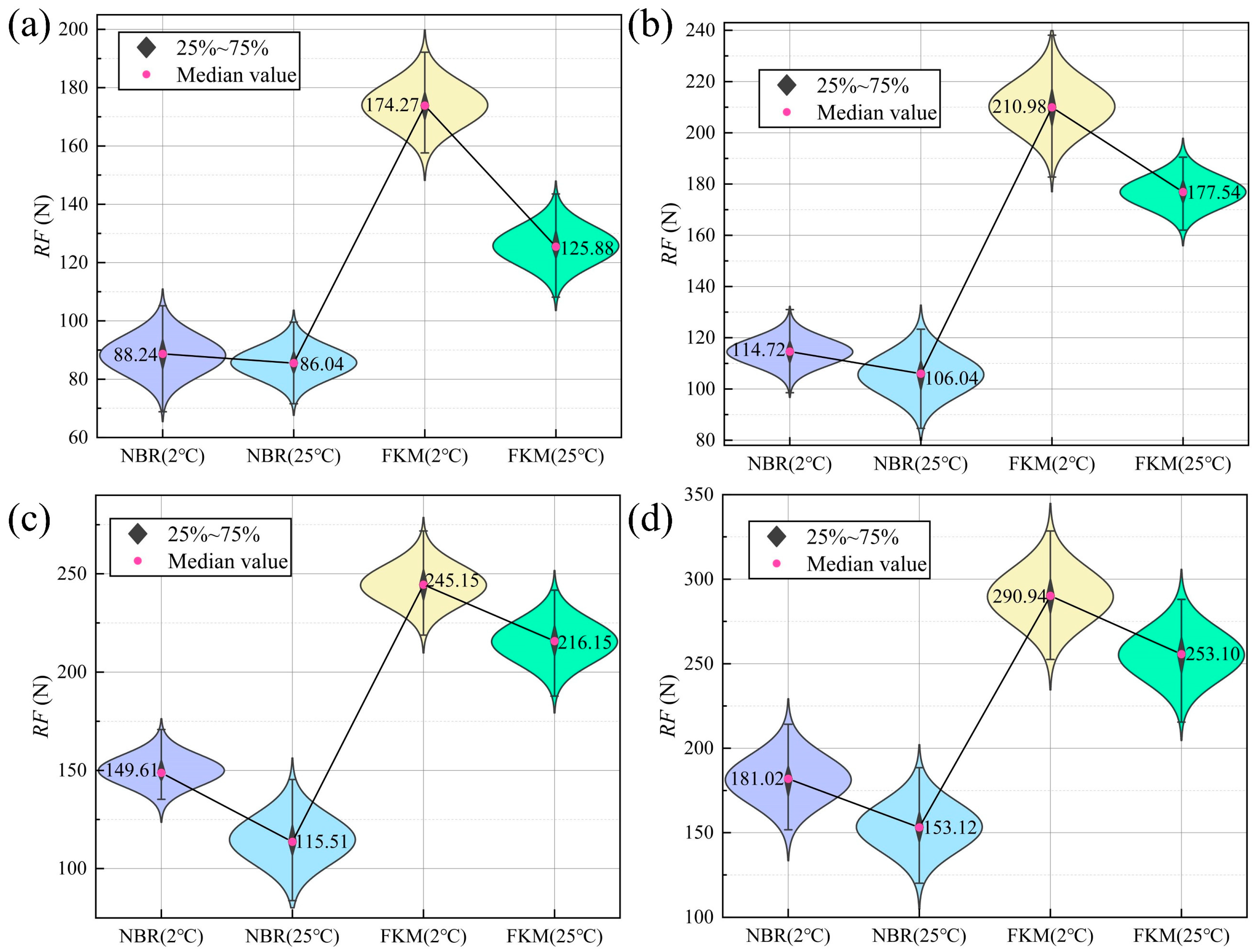
3.1.2. The Reciprocation Friction
3.2. Results of Experimental Study
3.2.1. Results of Experiment at Room Temperature and Atmospheric Pressure
3.2.2. Results of Experiment Under High Pressure and Low Temperature
3.3. Results of Experimental Study
4. Discussion
4.1. Advice for Selection of Seal Materials for Moving Parts of Deep-Sea Equipment
4.2. Methods of Reducing the Installation Force of Sealing Ring
4.3. Limitations of This Study
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Liang, J.; Feng, J.-C.; Zhang, S.; Cai, Y.; Yang, Z.; Ni, T.; Yang, H.-Y. Role of deep-sea equipment in promoting the forefront of studies on life in extreme environments. Iscience 2021, 24, 103299. [Google Scholar]
- Wang, H.; Cao, C.; Guo, J.; Wang, W.; Zhou, P.; Chen, J.-W. Design and friction loss study of full-ocean depth oil-filled direct current motor. J. Zhejiang Univ. Sci. A 2022, 23, 587–598. [Google Scholar]
- Wu, S.; Chen, Z.; Wang, S.; Zhang, J.; Yang, C. A review of deep-seawater samplers: Principles, applications, performance, and trends. Deep Sea Res. Part I Oceanogr. Res. Pap. 2024, 213, 104401. [Google Scholar]
- Elsyad, M.A.; Elhaddad, A.A.; Khirallah, A.S. Retentive properties of O-ring and locator attachments for implant-retained maxillary overdentures: An in vitro study. J. Prosthodont. 2018, 27, 568–576. [Google Scholar] [PubMed]
- Faulhaber, C.; Borland, C.; Boehm, R.; Heyne, J. Measurements of Nitrile Rubber Absorption of Hydrocarbons: Trends for Sustainable Aviation Fuel Compatibility. Energy Fuels 2023, 37, 9207–9219. [Google Scholar]
- Schaefer, R.J. Mechanical properties of rubber. In Harris’ Shock and Vibration Handbook; McGraw Hill: New York, NY, USA, 2010; Volume 6, pp. 33.18–33.31. [Google Scholar]
- Liao, C.; Huang, W.; Wang, Y.; Suo, S.; Liu, Y. Fluid-solid interaction model for hydraulic reciprocating O-ring seals. Chin. J. Mech. Eng. 2013, 26, 85–94. [Google Scholar]
- Wang, B.; Meng, X.; Peng, X.; Chen, Y. Experimental investigations on the effect of rod surface roughness on lubrication characteristics of a hydraulic O-ring seal. Tribol. Int. 2021, 156, 106791. [Google Scholar]
- Yanes Nunez, E. Frictional Behaviour of Elastomer Seals. Ph.D. Thesis, Queen Mary University of London, London, UK, 2023. [Google Scholar]
- Qin, Z.; Wu, Y.-T.; He, L.; Gao, X.; Lyu, S.-K. Empirical research on the friction behavior of O-rings in hydraulic cylinders. PLoS ONE 2023, 18, e0280815. [Google Scholar]
- Huang, H.C.; Ye, Y.Y.; Yang, C.J.; Leng, J.X.; Chen, Y. Study of the sealing characteristic of polytetrafluoroethylene-coated O-ring applied in gas-tight deep-sea water sampler. Adv. Mater. Res. 2011, 295, 3–10. [Google Scholar] [CrossRef]
- He, S.; Peng, Y.; Qiu, S.; Du, X.; Jin, Y. Sealing performance of sealing structure in a sediment sampler under ultra-high pressure. Sci. Rep. 2023, 13, 18548. [Google Scholar]
- Wu, J.-B.; Li, L. Influence of ambient pressure on sealing performance of O-ring in deep-sea hydraulic system. Ocean Eng. 2022, 245, 110440. [Google Scholar] [CrossRef]
- Liu, D.; Yun, F.; Jiao, K.; Wang, L.; Yan, Z.; Jia, P.; Wang, X.; Liu, W.; Hao, X.; Xu, X. Structural analysis and experimental study on the spherical seal of a subsea connector based on a non-standard O-ring seal. J. Mar. Sci. Eng. 2022, 10, 404. [Google Scholar] [CrossRef]
- Sun, S.; Zou, Y.; Zhang, Z.; Yang, Y.; Che, J.; Gong, Y. Simulation and Experiments on Sealing and Extrusion Failure on Application of Deep-Sea Adsorption by Differential Pressure. J. Press. Vessel. Technol. 2024, 146, 041701. [Google Scholar] [CrossRef]
- Han, Q.; Chen, H.; Yang, W.; Zhang, Y.; Yang, J.; Chen, Y. Analysis of reciprocating O-ring seal in the pressure-balanced oil-filled wet-mate electrical connectors for underwater applications. Lubr. Sci. 2019, 31, 335–345. [Google Scholar] [CrossRef]
- Li, Y.; Su, H.; Wang, Y.; Ma, Q.; Sun, H.; Lin, Z. Analysis of the factors influencing the sealing performance in subsea electrical connectors. Ships Offshore Struct. 2024, 20, 1–11. [Google Scholar] [CrossRef]
- Davies, P.; Le Gac, P.Y.; Ciausu, V.; Gallois, H. Testing of nitrile rubber joints for a deep submergence vehicle. Polym. Test. 2020, 90, 106630. [Google Scholar] [CrossRef]
- Wang, H.; Chen, J.-W.; Cao, C.; Ge, Y.; Fang, J.-S.; Zhou, P.; Lin, P.-W. Capturing amphipods in the Mariana Trench with a novel pressure retaining sampler. Deep Sea Res. Part I Oceanogr. Res. Pap. 2022, 184, 103772. [Google Scholar] [CrossRef]
- Yang, Y.; Zhao, W.; Xiao, X. The upper temperature limit of life under high hydrostatic pressure in the deep biosphere. Deep Sea Res. Part I Oceanogr. Res. Pap. 2021, 176, 103604. [Google Scholar] [CrossRef]
- Wang, H.; Ruan, D.-R.; Cao, C.; Fang, J.-S.; Zhou, P.; Fang, Y.-P.; Chen, J.-W. Collection sediment from Mariana Trench with a novel pressure-retaining sampler. Deep Sea Res. Part I Oceanogr. Res. Pap. 2022, 183, 103740. [Google Scholar] [CrossRef]
- Guo, J.; Chen, J.; Ren, Z.; Wang, H.; Zhang, P.; Wang, W.; Wang, Y.; Zhou, P.; Gao, Q.; Ren, X. Pressure-retaining sampler for sediment and overlying seawater based on heavy duty ROV-Jellyfish. Deep Sea Res. Part I Oceanogr. Res. Pap. 2023, 196, 104007. [Google Scholar] [CrossRef]
- He, S.; Qiu, S.; Tang, W.; Peng, Y.; Jin, Y.-P. A novel submersible-mounted sediment pressure-retaining sampler at full ocean depth. Front. Mar. Sci. 2023, 10, 1154269. [Google Scholar] [CrossRef]
- Rivlin, R.S.; Saunders, D. Large elastic deformations of isotropic materials VII. Experiments on the deformation of rubber. Philos. Trans. R. Soc. Lond. Ser. A Math. Phys. Sci. 1951, 243, 251–288. [Google Scholar]
- Zhou, C.; Zheng, J.; Gu, C.; Zhao, Y.; Liu, P. Sealing performance analysis of rubber O-ring in high-pressure gaseous hydrogen based on finite element method. Int. J. Hydrogen Energy 2017, 42, 11996–12004. [Google Scholar] [CrossRef]
- Nair, A.B.; Kurian, P.; Joseph, R. Ethylene–propylene–diene terpolymer/hexa fluoropropylene–vinylidinefluoride dipolymer rubber blends: Thermal and mechanical properties. Mater. Des. 2012, 36, 767–778. [Google Scholar] [CrossRef]
- Kumari, P.; Unnikrishnan, G. Thermal properties of compatibilized and filled natural rubber/acrylonitrile butadiene rubber blends. J. Therm. Anal. Calorim. 2013, 114, 67–75. [Google Scholar] [CrossRef]







| Symbol | Definition | Setting Value |
|---|---|---|
| d1 | Out diameter of piston | 40 mm |
| d2 | Cross-section diameter of O-ring | 3.55 mm |
| δ | Clearance between piston and cylinder | 0.1 mm–0.3 mm |
| h | Depth of the O-ring housing | 2.75 mm |
| b | width of the O-ring housing | 4.2 mm |
| r1 | Filet radius of at housing top | 0.4 mm |
| r2 | Filet radius of at housing bottom | 0.2 mm |
| θ | Angel of the lead-in chamfer | 10°, 15°, 20°, 25° |
| z | Length of the lead-in chamfer | 2 mm |
| Material Type | C10 | C01 | C20 | C11 | C02 |
|---|---|---|---|---|---|
| NBR (25 °C) | 1.5099 | −0.8510 | 0.6193 | −1.0989 | −0.2663 |
| NBR (2 °C) | 5.8453 | −5.2729 | −2.2978 | 9.3654 | −10.8957 |
| FKM (25 °C) | 4.9274 | −4.4537 | −1.0643 | 4.5278 | −6.3517 |
| FKM (25 °C) | 5.4255 | −4.8963 | −1.2351 | 4.9735 | −6.4289 |
| No. | Chamfer Angle (°) | Chamfer Length (mm) | Roughness of the Contact Surface (μm) |
|---|---|---|---|
| 1 | 20 | 2, 3 | 1.6 |
| 2 | 25 | 2, 3 | 1.6 |
| 3 | 15 | 1, 2 | 0.8 |
| Part Name | Design Parameters |
|---|---|
| Pressure vessel | 40 mm in ID, 90 mm in OD, and 170 mm in length |
| Piston | 40 mm in diameter, 80 mm in length, and weighs 0.634 kg |
| Connecting rod | 24 mm in diameter, 110 mm in length, and weighs 0.291 kg |
| Limit plate | 70 mm in diameter, 5 mm in height, and weighs 0.129 kg |
| O-ring | 33.5 mm in ID, 3.55 mm in cross-section diameter |
| Test Site | Depth (m) | In Situ/Final Pressure (MPa) | In Situ Temperature (°C) | Pressure-Retaining Rate |
|---|---|---|---|---|
| 1 | 1288 | 13.3/13.5 | 3.1 | 101.5% |
| 2 | 256 | 2.7/2.6 | 5.3 | 96.3% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, H.; Wang, Y.; Chen, J.; Sun, Y.; Shen, Y.; Wang, H. Research on the Reciprocating Friction and Installation Force of Seals in Deep-Sea Samplers. Appl. Sci. 2025, 15, 1867. https://doi.org/10.3390/app15041867
Zhang H, Wang Y, Chen J, Sun Y, Shen Y, Wang H. Research on the Reciprocating Friction and Installation Force of Seals in Deep-Sea Samplers. Applied Sciences. 2025; 15(4):1867. https://doi.org/10.3390/app15041867
Chicago/Turabian StyleZhang, Hao, Yongjun Wang, Jiawang Chen, Yanjie Sun, Yongchun Shen, and Hao Wang. 2025. "Research on the Reciprocating Friction and Installation Force of Seals in Deep-Sea Samplers" Applied Sciences 15, no. 4: 1867. https://doi.org/10.3390/app15041867
APA StyleZhang, H., Wang, Y., Chen, J., Sun, Y., Shen, Y., & Wang, H. (2025). Research on the Reciprocating Friction and Installation Force of Seals in Deep-Sea Samplers. Applied Sciences, 15(4), 1867. https://doi.org/10.3390/app15041867








