Lateral Load-Bearing Performance of a Long Pile in Layered Soils Based on the Modified Vlasov Foundation Model
Abstract
1. Introduction
2. Computation Model
3. Solution Process
4. Numerical Calculation
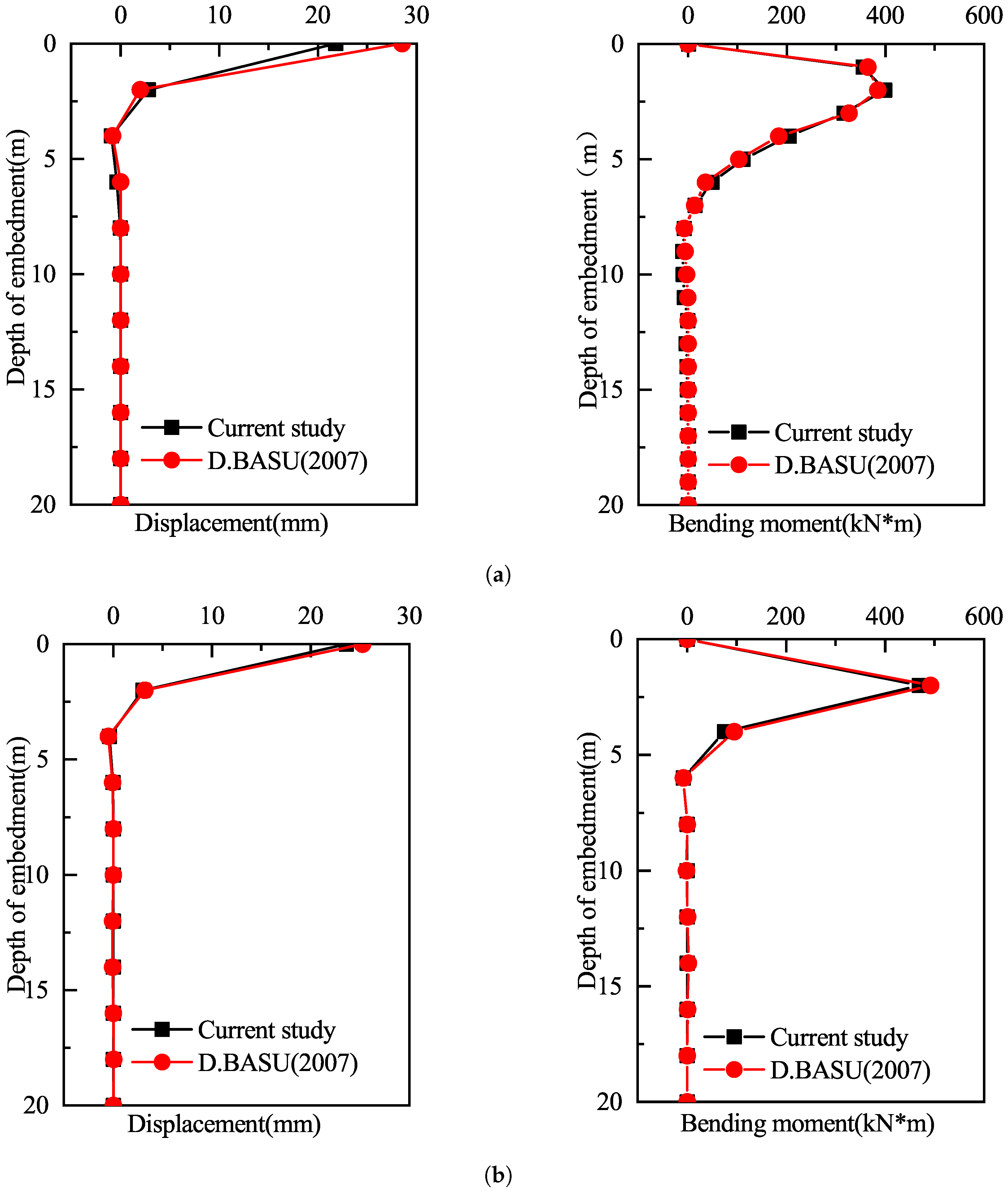
4.1. The Solution Process Verification
4.2. Influence of Slenderness Ratio
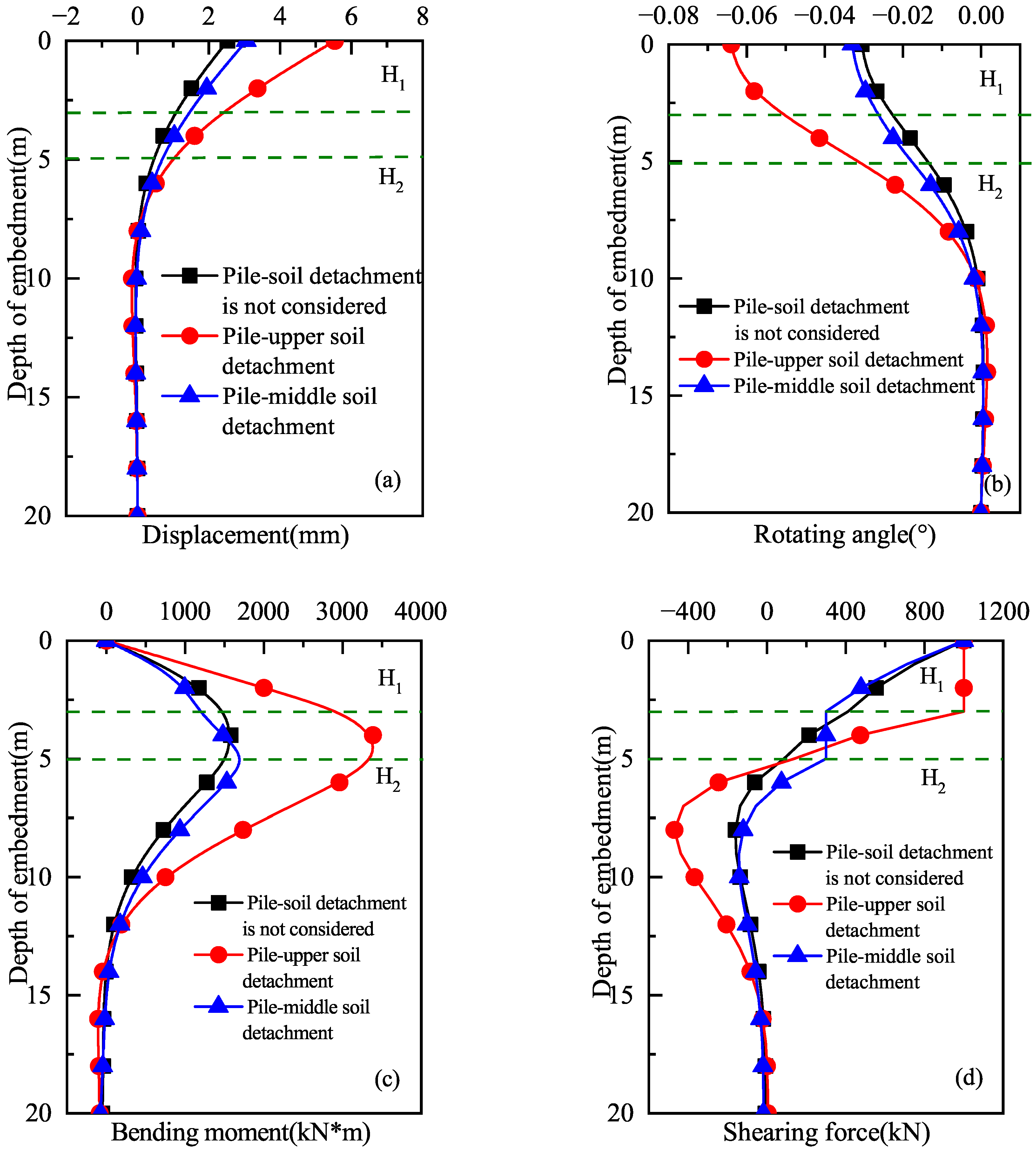
4.3. Influence of Pile–Soil Contact Surface Detachment
5. Conclusions
- (1)
- As the slenderness ratio of the pile increases, the flexibility of the pile also increases, resulting in significant growth in lateral deformation, while the maximum bending moment and maximum shear force decrease. In this study, quantitative calculations were performed to obtain the variation patterns of pile head displacement, rotation angle, and internal forces at different slenderness ratios. The results can provide a reference for pile foundation design under similar conditions.
- (2)
- When detachment occurs at the interface between the upper soil layer and the pile, the pile head displacement and rotation angle increase by approximately 2 times, and the maximum bending moment of the pile significantly increases, with the increase in negative shear force reaching approximately 3 times. Therefore, the support provided by the upper soil layer is crucial for the rigidity of the pile foundation. Special attention should be paid to this factor during design, and measures such as improving the soil layer can be taken to mitigate the adverse effects caused by detachment.
- (3)
- When detachment occurs at the interface between the middle soil layer and the pile, the increase in the pile head displacement and maximum bending moment is less than 25 %, while the maximum negative shear force decreases significantly. Compared to detachment at the upper soil layer, detachment at the middle soil layer has a smaller impact on the performance of the pile foundation.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kong, D.; Deng, M.; Zhao, Z. Seismic Interaction Characteristics of an Inclined Straight Alternating Pile Group-Soil in Liquefied Ground. Int. J. Geomech. 2019, 2019, 3758286. [Google Scholar] [CrossRef]
- Hemel, M.J.; Korff, M.; Peters, D.J. Analytical Model for Laterally Loaded Pile Groups in Layered Sloping Soil. Int. J. Geomech. 2022, 22, 123–145. [Google Scholar] [CrossRef]
- Heidari, B.; Garakani, A.A.; Jozani, S.M.; Tari, P.H. Energy Piles under Lateral Loading: Analytical and Numerical Investigations. Renew. Energy 2022, 16, 234–249. [Google Scholar] [CrossRef]
- Zhang, C.; Zhang, X.; Huang, M.; Tang, H. Responses of Caisson-Piles Foundations to Long-Term Cyclic Lateral Load and Scouring. Soil Dyn. Earthq. Eng. 2019, 119, 62–74. [Google Scholar] [CrossRef]
- McCarron, W.O. Efficient Analysis of Cyclic Laterally Loaded Piles. Results Eng. 2021, 10, 100213. [Google Scholar] [CrossRef]
- Pesicka, B.; Stone, L.J.; O’Neill, T. Case Study: Lateral Pile Testing for Seismic Design in Unexpected Soil Conditions. Proc. Int. Conf. Geotech. 2022, 11, 439–451. [Google Scholar] [CrossRef]
- Basack, S.; Karami, M.; Karakouzian, M. Pile-Soil Interaction under Cyclic Lateral Load in Loose Sand: Experimental and Numerical Evaluations. Soil Dyn. Earthq. Eng. 2022, 162, 107439. [Google Scholar] [CrossRef]
- Keawsawasvong, S.; Ukritchon, B. Failure Modes of Laterally Loaded Piles under Combined Horizontal Load and Moment Considering Overburden Stress Factors. Geotech. Geol. Eng. 2020, 38, 4253–4267. [Google Scholar] [CrossRef]
- Houda, G.; Tayeb, B.; Yahiaoui, D. Key Parameters Influencing Performance and Failure Modes for Interaction Soil–Pile–Structure System under Lateral Loading. Asian J. Civ. Eng. 2018, 19, 355–373. [Google Scholar] [CrossRef]
- Poulos, H.G. Pile behaviour—Theory and application. Géotechnique 1989, 39, 365–415. [Google Scholar] [CrossRef]
- Dai, M.; Zhou, Y.D.; Zhang, T. Recent researches on soil-pile interaction. J. Hohai Univ. (Nat. Sci.) 2006, 34, 568–571. [Google Scholar]
- Yang, Y.; Gao, X.; Wu, W.; Xing, K. A simplified elastoplastic method for laterally loaded single pile with large displacement. Rock Soil Mech. 2020, 41, 95–102. [Google Scholar] [CrossRef]
- Das, Y.C.; Sargand, S.M. Forced vibrations of laterally loaded piles. Int. J. Solids Struct. 1999, 36, 4975–4989. [Google Scholar] [CrossRef]
- Liu, H.L. Numerical solution of laterally loaded cast-in-place concrete large diameter pipe pile installed in multi-layered soil. Rock Soil Mech. 2010, 31, 1638–1644. [Google Scholar] [CrossRef]
- Fang, T.; Huang, M. Deformation and Load-Bearing Characteristics of Step-Tapered Piles in Clay under Lateral Load. Int. J. Geomech. 2019, 18, 456–478. [Google Scholar] [CrossRef]
- Dahal, B.K.; Regmi, S.; Paudyal, K.; Dahal, D.; KC, D. Enhancing Deep Excavation Optimization: Selection of an Appropriate Constitutive Model. CivilEng 2024, 5, 785–800. [Google Scholar] [CrossRef]
- Luan, L.; Liu, Y.; Li, Y. Numerical Simulation for the Soil-Pile-Structure Interaction under Seismic Loading. Int. J. Geomech. 2015, 2015, 959581. [Google Scholar] [CrossRef]
- Nguyen, Q.V.; Fatahi, B. Influence of Size and Load-Bearing Mechanism of Piles on Seismic Performance of Buildings Considering Soil–Pile–Structure Interaction. Int. J. Geomech. 2017, 17, 04017007. [Google Scholar] [CrossRef]
- Ma, J.; Han, S.; Gao, X.; Li, D.; Guo, Y.; Liu, Q. Dynamic Lateral Response of the Partially-Embedded Single Piles in Layered Soil. Appl. Sci. 2022, 12, 1504. [Google Scholar] [CrossRef]
- Zhan-Fang, H.; Bai, X.H.; Yin, C.; Liu, Y.Q. Numerical analysis for the vertical bearing capacity of composite pile foundation system in liquefiable soil under sine wave vibration. PLoS ONE 2021, 16, e0248502. [Google Scholar] [CrossRef]
- Chandaluri, V.K.; Sawant, V. Influence of sloping ground on lateral load capacity of single piles in clayey soil. Geomech. Geoeng. 2020, 15, 312–324. [Google Scholar] [CrossRef]
- Weaver, T.; Chittoori, B. Influence of Limited Soil Improvement on Lateral Pile Stiffness. Proc. Int. Conf. Geotech. 2007, 9, 409–416. [Google Scholar] [CrossRef]
- Lukpanov, R.E.; Yenkebayev, S.B.; Tsigulyov, D.V. Assessment of the bearing capacity of piles in soil, determined by static and dynamic load tests. Eng. J. Satbayev Univ. 2021, 143, 252–260. [Google Scholar] [CrossRef]
- Tiutkin, O.; Radkevych, A.; Dubinchyk, O.; Kharchenko, V. Parametric analysis of a strain state of a soil base strengthened with vertical elements. Min. Miner. Depos. 2024, 18, 104–112. [Google Scholar] [CrossRef]
- Arshad, M.; O’Kelly, B.C. Model Studies on Monopile Behavior under Long-Term Repeated Lateral Loading. J. Geotech. Geoenviron. Eng. 2022, 148, 039. [Google Scholar] [CrossRef]
- Zhang, L.; Jiao, D. Response analysis for slightly inclined single pile undergoing vertical and lateral loads with restraint of pile head. Chin. J. Appl. Mech. 2018, 35, 583–589. [Google Scholar]
- Xiong, H.; Jiang, Y.F.; Yu, R.X. Lateral vibration impedance of piles embedded in layered soil based on Laplace transform. Rock Soil Mech. 2018, 39, 1091–1097. [Google Scholar] [CrossRef]
- Su, A.; Zhang, M.; Shang, W.; Wang, Q. Transient Horizontal Response of a Pipe Pile in Saturated Soil with a Flexible Support at the Pile Head. Appl. Sci. 2025, 15, 682. [Google Scholar] [CrossRef]
- Li, H.; Liu, S.; Tong, L.; Xu, X. Investigating the Resonance Compaction Effect on Laterally Loaded Piles in Layered Soil. Eng. Geol. 2018, 246, 1–11. [Google Scholar] [CrossRef]
- Kavitha, P.E.; Beena, K.S.; Narayanan, K.P. A Review on Soil–Structure Interaction Analysis of Laterally Loaded Piles. Innov. Infrastruct. Solut. 2016, 1, 14. [Google Scholar] [CrossRef]
- Kim, Y.; Jeong, S. Analysis of Soil Resistance on Laterally Loaded Piles Based on 3D Soil–Pile Interaction. Comput. Geotech. 2011, 38, 248–257. [Google Scholar] [CrossRef]
- Zhu, M.X.; Gong, W.M.; He, X.Y. Transfer matrix solutions for responses of laterally loaded piles in multilayered soil deposits. Chin. J. Geotech. Eng. 2015, 37 (Suppl. 2), 46–50. [Google Scholar]
- Gupta, B.K.; Basu, D. Analysis of laterally loaded short and long piles in multilayered heterogeneous elastic soil. Soils Found. 2017, 57, 92–110. [Google Scholar] [CrossRef]
- Zhang, L.; Ou, Q.; Zhou, S.; Zhao, M. Semi-analytical solutions for vertically and laterally loaded piles in multilayered soil deposits. Environ. Earth Sci. 2018, 77, 51–64. [Google Scholar] [CrossRef]
- Dai, Z.H.; Chen, L.J. Two numerical solutions of laterally loaded piles installed in multi-layered soils by m method. Chin. J. Geotech. Eng. 2007, 29, 690–696. [Google Scholar]
- Zhang, L. Nonlinear analysis of laterally loaded rigid piles in cohesionless soil. Comput. Geotech. 2009, 36, 718–724. [Google Scholar] [CrossRef]
- Basar, E.E.; Celik, D.; Uzundurukan, S.; Findik, M. Investigation of structural behavior of piles in liquefiable cohesionless soils. Geomechanics 2022, 23, 89–98. [Google Scholar] [CrossRef]
- Yang, J.B.; Zhong, Z.X. Research on load transfer mechanism of super-long pile. Chin. J. Geotech. Eng. 1998, 20, 111–115. [Google Scholar]
- Jiang, J.P.; Chen, W.J.; Yang, S. Impacts of local scour on lateral bearing behavior of partially embedded single piles. Hydro-Sci. Eng. 2017, 3, 64–70. [Google Scholar] [CrossRef]
- Bao, T.; Liu, Z. Evaluation of Winkler Model and Pasternak Model for Dynamic Soil-Structure Interaction Analysis of Structures Partially Embedded in Soils. Int. J. Geomech. 2020, 20, 04019167. [Google Scholar] [CrossRef]
- Wang, Y.; Orense, R.P. Numerical investigation of inclined piles under liquefaction-induced lateral spreading. Geotechnics 2023, 3, 19. [Google Scholar] [CrossRef]
- Richter, S.; Cudmani, R.; Slominski, C. The behavior of a spread footing over reinforced ground with gravel interface during a strong earthquake. Geotech. Res. 2011, 8, 96–104. [Google Scholar] [CrossRef]
- Das, Y.C.; Vallabhan, C.V.G. Parametric study of beams on elastic foundations. J. Struct. Eng. 1988, 114, 2072–2088. [Google Scholar] [CrossRef]
- Reddy, J.N. Energy and Variational Methods in Applied Mechanics; Wiley: Hoboken, NJ, USA, 1984. [Google Scholar]
- Sun, K. Laterally loaded pile in elastic media. J. Geotech. Eng. 1994, 120, 1324–1344. [Google Scholar] [CrossRef]
- Ma, J.J.; Liu, Q.J.; Zhao, Y.Y. Stability of beams on modified Vlasov foundation subjected to lateral loads acting on the ends. Chin. J. Geotech. Eng. 2008, 30, 850–854. [Google Scholar]
- Basu, D.; Salgado, R. Elastic analysis of laterally loaded pile in multi-layered soil. Geomech. Geoeng. 2007, 2, 183–196. [Google Scholar] [CrossRef]
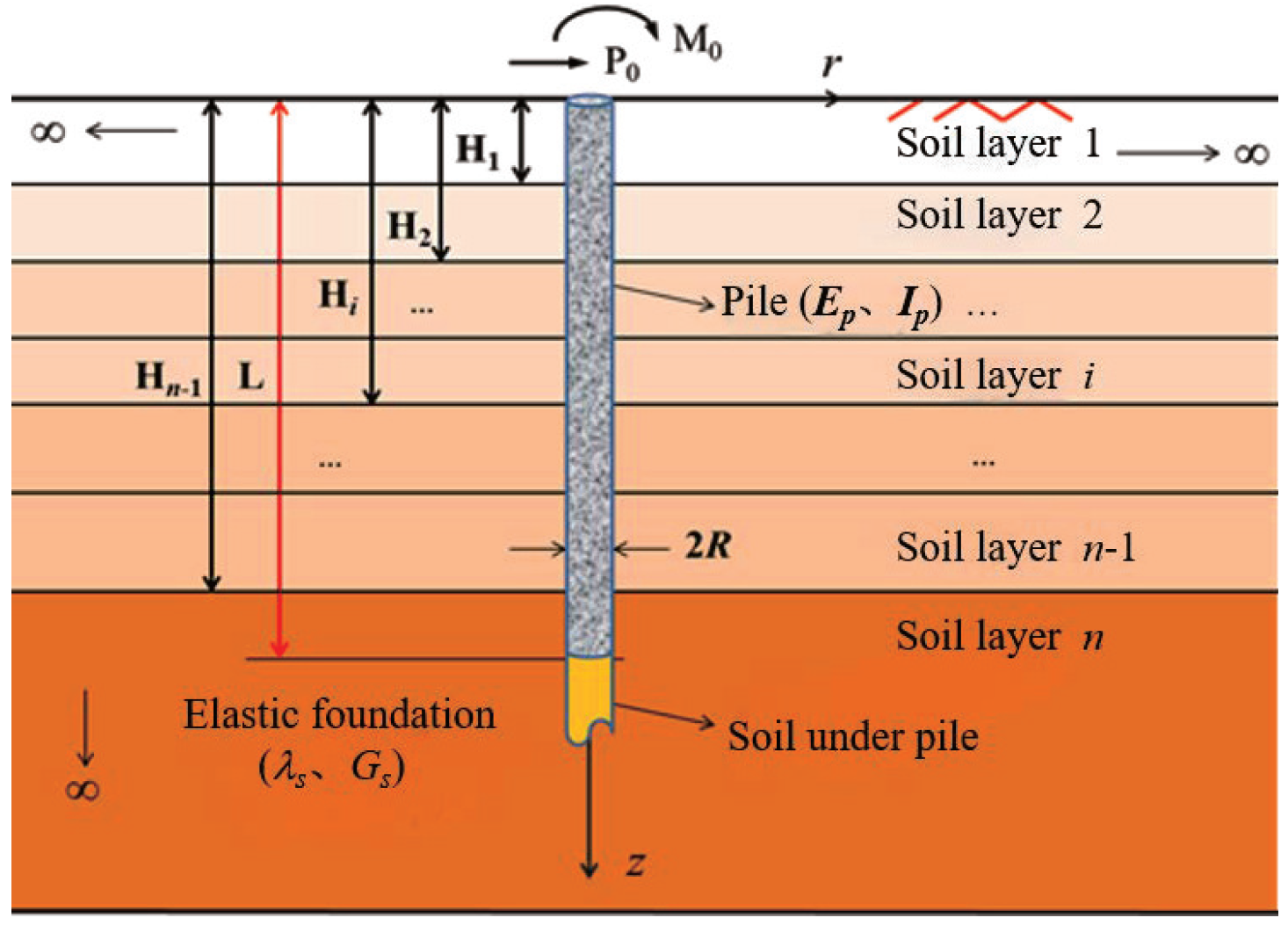
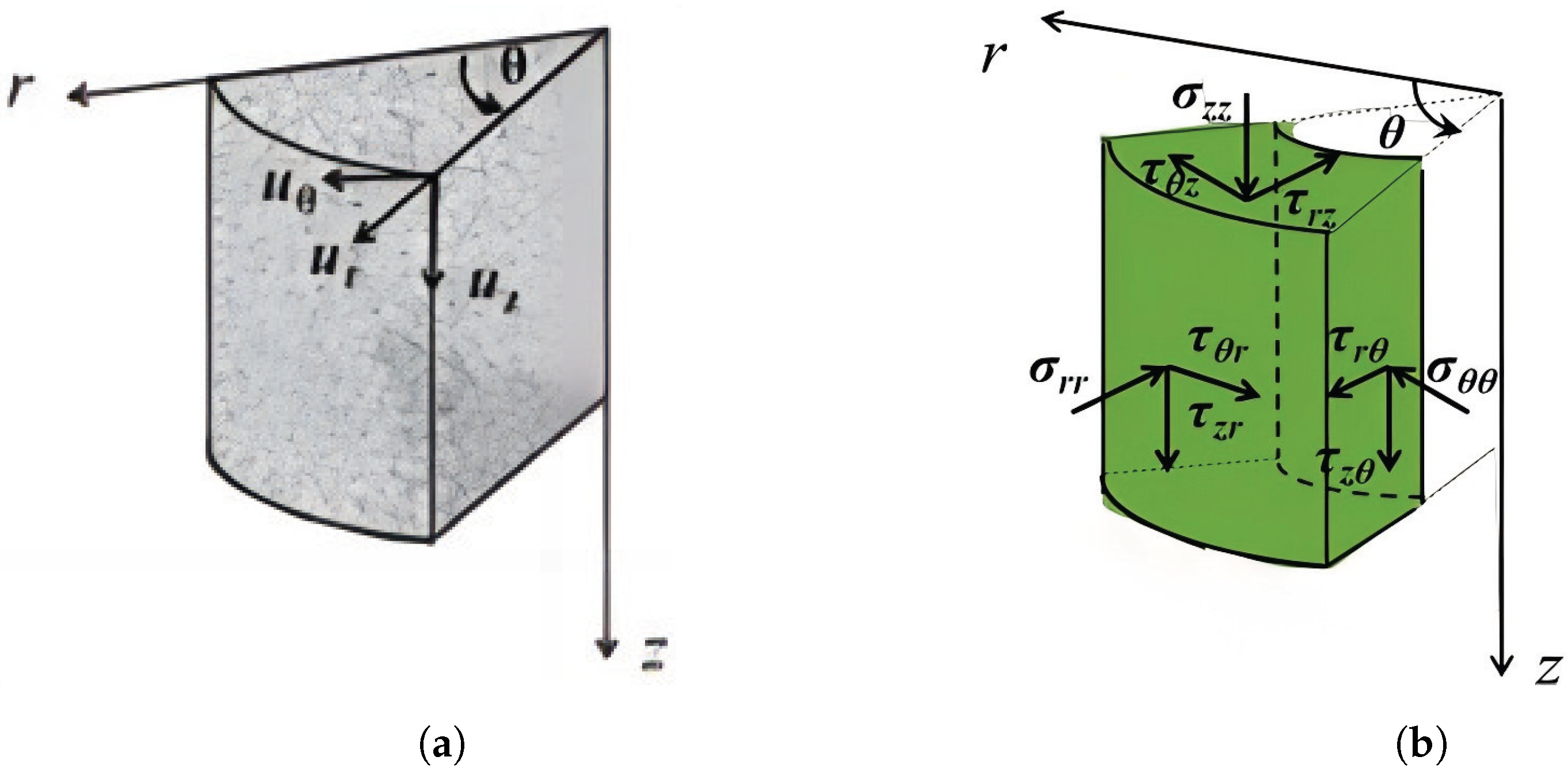


| Parameter | ||
|---|---|---|
| Parameter | Physical Meaning | Value | Unit |
|---|---|---|---|
| Elastic modulus of pile | 2.5 × 104 | MPa | |
| L | Pile length | 20 | m |
| Poisson’s ratio of elastic foundation | 0.25 | — | |
| Depth of foundation | 3, 5 | m | |
| Elastic modulus of the first layer foundation | 50 | MPa | |
| Elastic modulus of the second layer foundation | 100 | MPa | |
| Elastic modulus of the third layer foundation | 200 | MPa |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, F.; Ma, J.; Li, D. Lateral Load-Bearing Performance of a Long Pile in Layered Soils Based on the Modified Vlasov Foundation Model. Appl. Sci. 2025, 15, 1759. https://doi.org/10.3390/app15041759
Liu F, Ma J, Li D. Lateral Load-Bearing Performance of a Long Pile in Layered Soils Based on the Modified Vlasov Foundation Model. Applied Sciences. 2025; 15(4):1759. https://doi.org/10.3390/app15041759
Chicago/Turabian StyleLiu, Fengjun, Jianjun Ma, and Da Li. 2025. "Lateral Load-Bearing Performance of a Long Pile in Layered Soils Based on the Modified Vlasov Foundation Model" Applied Sciences 15, no. 4: 1759. https://doi.org/10.3390/app15041759
APA StyleLiu, F., Ma, J., & Li, D. (2025). Lateral Load-Bearing Performance of a Long Pile in Layered Soils Based on the Modified Vlasov Foundation Model. Applied Sciences, 15(4), 1759. https://doi.org/10.3390/app15041759







