Calculation of the Main Parameters of the Two-Line Helical Traction Transmission of an Electric Locomotive Based on Diagnostic Parameters ^†

Abstract

Gearboxes used in electric locomotives are a critical unit, especially in freight rolling stock. The article presents the calculation of the main parameters of the two-way oblique traction transmission of the VL-80s electric locomotive (which is operated on the railways of Uzbekistan) based on a comprehensive analysis of the diagnostic parameters obtained using the Poisson normal distribution method for the identified failures according to the Uzbekistan depot data. Also, a Pareto diagram was constructed for the chassis of 3VL-80s electric locomotives based on the data of the Locomotive Operation Department of JSC Uzbekistan Temir Yollari, and probabilistic and statistical analyses of the failures and breakdowns of the wheel pair and the large gear wheel of the traction gearbox of the VL-80s electric locomotives were carried out. An algorithm and methodology for assessing the reliability of the large gear wheel of the traction gearbox of an electric locomotive are presented. As a result of a numerical calculation of the coefficients of the empirical regression equations using approximation and spline interpolation methods, a regression equation was obtained for the dependence of the standard deviation of the wear of the tooth of the large gear wheel of the traction reducer of the VL80s electric locomotive on the mileage.

1. Introduction

In the modern foreign patent and sci-tech literature, the problems of increasing the reliability and durability of locomotive gear wheels of complex configurations in the process of their design, operation, and modernization are widely investigated [1,2,3,4,5,6,7,8]. It is obvious that the overall stress state of the wheel-motor block (WMB) elements of locomotives, as well as their supporting equivalent frames, will depend significantly on longitudinal, transverse, and torsional dynamic components of traction forces arising in frames of complex configuration, as well as contact stresses arising from sharp temperature differences. These factors cause a 1.2–1.5 times decrease in the total service life of the locomotive wheel-engine unit elements.

Research has been conducted and is being conducted on this topic by leading scientists worldwide such as S.A. Brebbia (Wessex Institute of Technology, Southampton, UK), G.M. Carlomagno (University of Naples di Napoli, Naples, Italy), A. Varvani-Farahani (Ryerson University, Toronto, ON, Canada), S.K. Chakrabarti (USA), S. Hernandez (University of La Coruna, A Coruña, Spain), S.-H. Nishida (Saga University, Saga, Japan). Authoritative scientific schools and prominent scientists in the CIS countries from MIIT, PGUPS, MAI, VNIIZhT, JSC VNIKTI, JSC Russian Railways, etc., worked on these issues. A significant contribution to solving many complex problems and checking theoretical conclusions related to the study of the processes of oscillations of the elastic self-aligning gear of the locomotive of the rolling stock was made by the Russian Research Institute of Railway Transport (CNII MPS) and the Russian Research Institute of Railcar Building (NIIV), where, along with theoretical studies, a large number of experimental studies (bench and full-scale ones) were conducted [1,2,3,4,5,6,7,8,9,10,11]. In Uzbekistan, the academician of the Academy of Sciences of the Republic of Uzbekistan, professor, doctor of technical sciences Glushchenko A.D., and professors Fayzibaev Sh.S., Khromova G.A., Mukhamedova Z.G. and their students dealt with the problems of optimizing the systems of elastic self-aligning gear of rolling stock [8,12,13,14,15,16,17,18,19,20].

The processes of formation and the growth of defects threaten the possibility of the accident-free operation of rolling stock. Ensuring traffic safety by the timely detection of factory and operational fatigue defects in critical elements of the track and rolling stock brings considerable economic benefits and serves to save human lives. The solution to this problem is increasingly achieved today by physical methods of non-destructive testing.

In the interpretation of theory, non-destructive testing is an independent and intensively developing branch of science and technology at the junction of physical materials science and technology, which is widely used in various areas of production and especially in transport. Practice shows that the correct organization of testing, as well as the skillful use of one or another control method, and a reasonable combination of these methods, allow us to assess the presence of defects in the products under control with great reliability [3,4,5].

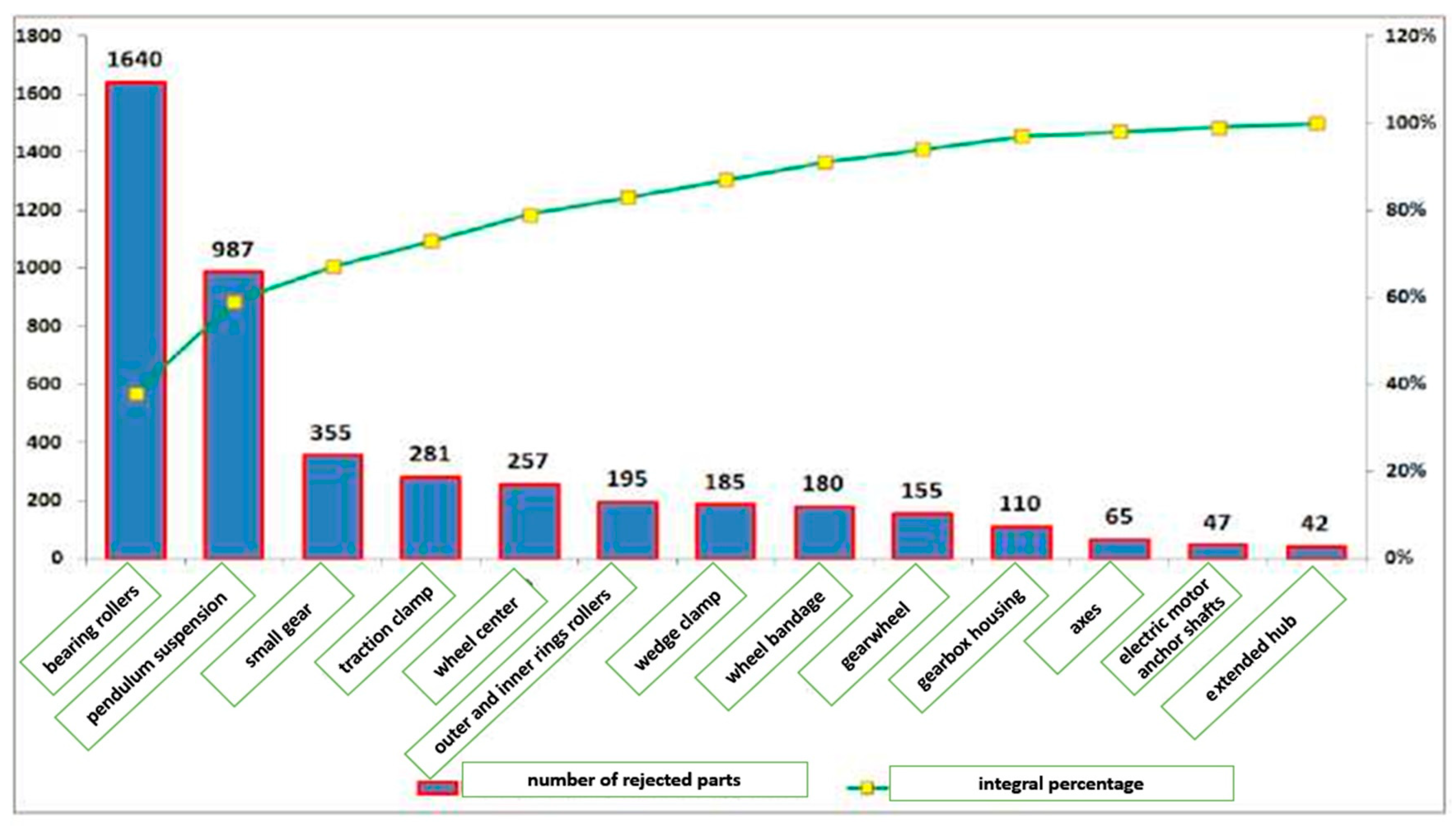
One of the options for assessing the technical condition of locomotive units and assemblies based on the results of non-destructive testing is to use Pareto analysis [9,18]. Analysis of the obtained Pareto diagram makes it possible to determine that the most prevalent components in the rejection of electric locomotive parts of the 3VL-80s series are the bearing rollers of the axle box assembly, pendulum suspensions, and small gears of the traction transmission (Figure 1).

After this, statistical material is collected and analyzed for each factor in order to determine which of them can be considered predominant in solving problems.

Non-destructive testing allows us to detect defective products, but in modern, cost-effective production, it is important not only to detect a defective product, but also to determine the causes of the defect and prevent the occurrence of defective products in the future. One of the ways to solve this problem is to use statistical methods of quality control.

During the operation of the traction rolling stock, the parameters of the parts change as a result of their interaction or the impact of external factors, and during technical inspection or repair, they are monitored. All technically monitored parameters must be within certain tolerances, i.e., have a minimum $X_{min}$ and maximum $X_{max}$ value. The value that can be between the parameters during operation: $∆=X_{max}-X_{min}$—tolerance field [3,4,5].

When all parameters characterizing the ability of an object to perform specified functions are within the tolerance field established by the requirements of the design (project) and normative-technical documentation, then this object is in working condition. If at least one (or several) parameters go beyond the established tolerances, then this object goes into an inoperative state—it fails. Monitoring of the parameters of parts, as a rule, cannot be carried out continuously. During the next repair or inspection of the traction rolling stock, the controlled parameter is measured. The implementation of the controlled parameter occurs from the moment of its nominal value, factory or restored, to the limit value in the period of several consecutive measurements [2,3]. As the controlled parameter changes, after a certain operating time, it goes beyond the set limit value $X_{ext}$, i.e., the part fails. For example, wear of the gear tooth surface or the tire thickness leads to the failure of the wheel pair if their value exceeds the minimum (gear tooth thickness 11 mm, tire thickness 45 mm). In this case, the controlled parameter is considered “decreasing”.

The operating time before failure is determined by a number of random factors—the quality of the component’s manufacture, operating conditions, the quality of the maintenance, repairs and materials used, the level of preparation of the equipment and personnel, all of which are random variables. Any relationship establishing a connection between the possible values of a random variable and the probabilities corresponding to them is the law of distribution of a random variable [3,4,5,9].

When measuring the controlled parameters of the wheel pair, such as wheel rolling, flange thickness, and tire thickness, the measurements were made in accordance with [4,5,6,7] and the values were measured at least once every 30 days. In the time interval between the two measurements, the locomotive mileage may be different, which causes a shift in the realizations of the controlled parameter along the mileage axis. Nevertheless, the spread of mileage values for different locomotives can be neglected [8] and it can be assumed that the technical condition of the locomotive is monitored at equal mileage intervals, and the results of measuring the controlled parameters form an equally spaced series of observations.

Since the values of the controlled parameters and the mean time between failures are random, to obtain reliable results, the samples of the initial data must be sufficiently representative. The minimum required sample size, the number of measurement results of the controlled parameter, according to [5,6,7], is $N=41$.

The methodology and algorithm for determining the compliance of samples of controlled parameters with the assumed distribution laws were developed and described in [9,10,11,19,20]. To check the compliance of a sample with the assumed theoretical distribution law, one can use one of the known criteria, for example, the Pearson goodness-of-fit criterion. It allows one to determine the probability that, due to random causes, the measure of divergence between the theoretical and statistical distributions will be greater than the actually observed one [19]. The samples of the controlled parameters of wear parts correspond to the normal distribution law. The distribution density for this law is as follows:

$$f\left(x\right)={\displaystyle \frac{1}{{\sigma }_x·\sqrt{2\pi }}}·e^{-{\displaystyle \frac{{\left(x-m_x\right)}^2}{2{{\sigma }_x}^2}}},$$

(1)

where $m_x$ is the mathematical expectation of the value of the controlled parameter; ${\sigma }_x$—is the standard deviation of the value of the controlled parameter; $x$—is the current value of the controlled parameter.

With the normal distribution law, the following relationships are imposed on a random variable:

$\sum _{j=1}^k{P}_j^{\mathrm{*}}=1$—the sum of frequencies over all intervals is equal to 1 (k is the number of intervals); $m_x=m_x^{\mathrm{*}}$; $D_x=D_x^{\mathrm{*}}$—parameters of statistical and theoretical distributions.

Since the numerical characteristics of the normal distribution law can be expressed through the mathematical expectation and variance of a random variable, the corresponding estimates are calculated: the average value $m_x^{\mathrm{*}}$ is equal to

$$m_x^{\mathrm{*}}={\displaystyle \frac{1}{N}}·{\sum }_{i=1}^N{x}_i$$

(2)

and the estimate of the dispersion (standard deviation ${\sigma }_x^{\mathrm{*}}$) is

$${\sigma }_x^{\mathrm{*}}=\sqrt{{\displaystyle \frac{1}{N-1}}·{\sum }_{i=1}^N{\left(\left(x_i-m_x^{\mathrm{*}}\right)\right)}^2},$$

(3)

where N is the sample size of the controlled parameter; $x_i$—is the value of the controlled parameter.

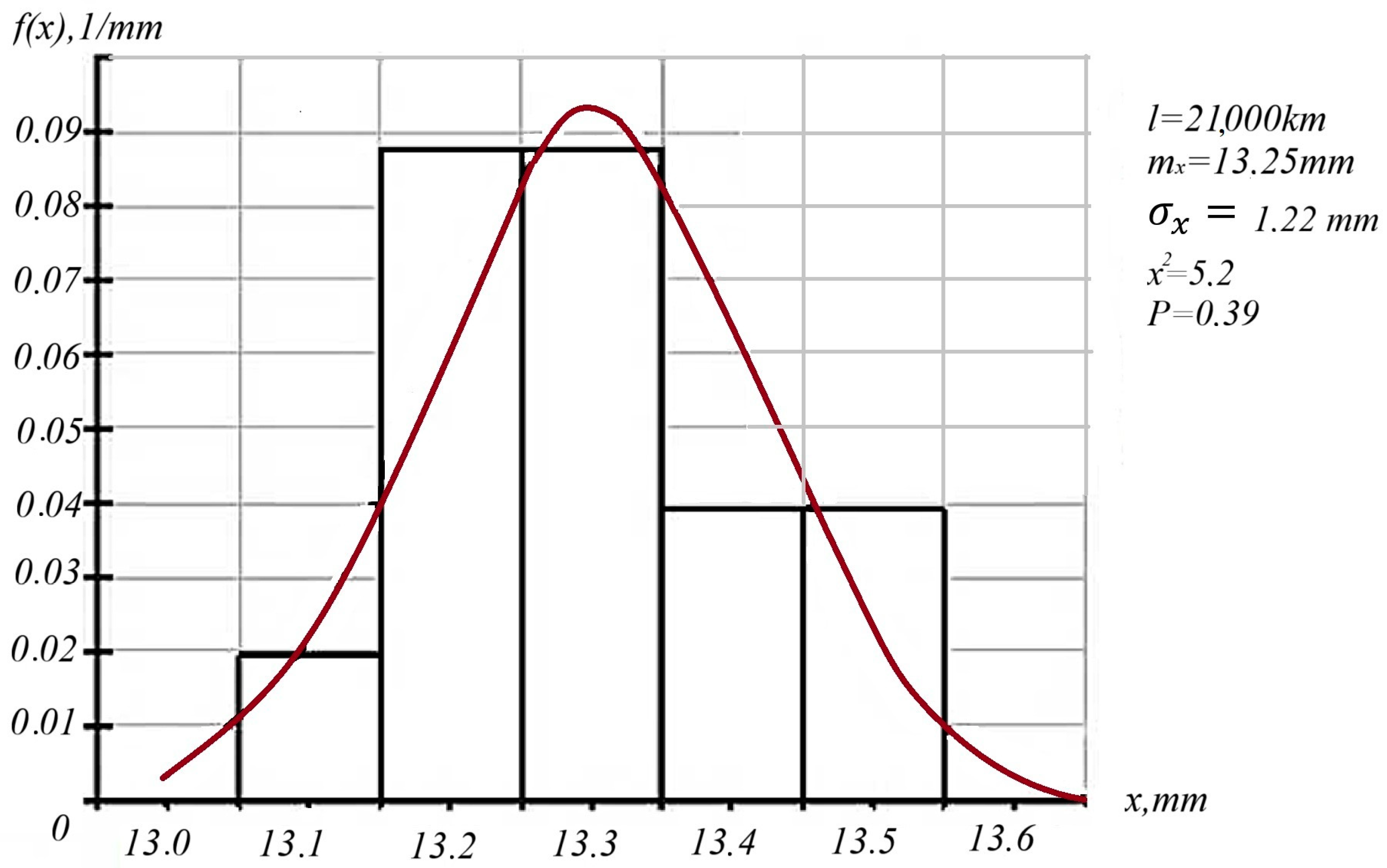
The distribution density functions of the tooth thickness of the large gear wheel of the traction reducer with fixed operating times of the 3VL-80c series electric locomotive in the locomotive repair depot of Uzbekistan are presented in Figure 1. The values of the numerical characteristics of the distribution law of the controlled parameter make it possible to predict their changes at the greatest operating times, which in turn determines the resource, and for this purpose, analytical dependencies of the average values $m_x$ and standard deviations ${\sigma }_x$ on the mileage are found.

The analytical dependence can be represented as a certain nonlinear function $y=f(a_1,a_2,\dots ,a_S,{\mathcal{l}}_i)$ of one argument ${\mathcal{l}}_i,$ the expression of which includes $S$ parameters $a_1,a_2,\dots ,a_S.$ Using this function, it is necessary to approximate the empirical regression specified in the form of n points ${(\mathcal{l}}_i,y_i)$ for $i=1,2,\dots ,n$, where y is understood as one of the parameters of the distribution law under consideration.

The parameters of the function y are found by the least squares method, the condition of which is written as

$$Z\left(a_1,a_2,\dots ,a_S\right)={\sum }_{i=1}^n{\left[f\left(a_1,a_2,\dots ,a_S,{\mathcal{l}}_i\right)-y_i\right]}^2=>min$$

(4)

The values of the controlled parameters and the empirical dependencies $m_x^{\mathrm{*}}\left(\mathcal{l}\right)$ and ${\sigma }_x^{\mathrm{*}}\left(\mathcal{l}\right)$ of the wearing parts of traction rolling stock obtained in practice can be described by linear functions [2,3,4,9,10,11,18,19,20], that is, the approximating function has the form

$$y=a\mathcal{l}+b.$$

(5)

The criterion for the conformity of the approximating function to the empirical dependencies is the minimum sum of the squares of the deviations of the empirical and theoretical functions

$${\sum }_{i=1}^n\left({\left[y_i-(a{\mathcal{l}}_i+b)\right]}^2\right)\to min.$$

(6)

Here

$y_i=\left\{\begin{array}{c}{m}_{xi}\\ {\sigma }_{xi}\end{array}\right.$ when approximating dependencies $\left\{\begin{array}{c}m\left(\mathcal{l}\right)\\ \sigma \left(\mathcal{l}\right)\end{array}\right.$;

${\mathcal{l}}_i$—operating time. In this case, the coefficient $a$ of the linear function is determined by the “least squares” method using the MATHCAD 15 programming environment according to the methods of works [18,19,20] and according to the formula

$$a=r_{y\mathcal{l}}·{\displaystyle \frac{{\sigma }_y}{{\sigma }_{\mathcal{l}}}},$$

(7)

where $r_{y\mathcal{l}}$—is the correlation coefficient between random numbers $y$ and $\mathcal{l}$;

${\sigma }_y,{\sigma }_{\mathcal{l}}$—standard deviations of random numbers $y$ and $\mathcal{l}$, respectively.

The coefficient b for the regression equation is determined by the formula

$$b=m_y-a{m}_{\mathcal{l}},$$

(8)

where $m_y$—is the average value of $y$; $m_{\mathcal{l}}$—is the average value of the operating time.

The correlation coefficient $r_{y\mathcal{l}}$ characterizes the tightness of the linear relationship between random variables $y$ and $\mathcal{l}$, and is determined by the formula

$$r_{y\mathcal{l}}={\displaystyle \frac{k_y\mathcal{l}}{{\sigma }_y{\sigma }_{\mathcal{l}}}}={\displaystyle \frac{{\alpha }_{11}\left(y,\mathcal{l}\right)-m_y{m}_{\mathcal{l}}}{{\sigma }_y{\sigma }_{\mathcal{l}}}},$$

(9)

where ${\alpha }_{11}\left(y,\mathcal{l}\right)={\displaystyle \frac{1}{N}}\sum _{i=1}^N{y}_i{\mathcal{l}}_i$—is the second mixed initial moment for random variables $y$ and $\mathcal{l}$.

For 3VL80s electric locomotives operated by JSC Uzbekistan Temir Yollari (Tashkent, Uzbekistan) (according to the data of the Department of Operation of Locomotives for the Uzbekistan Depot), calculations were carried out on controlled parameters such as the thickness of the wheel pair tread and the thickness of the large-toothed wheel of the traction reducer (Figure 2). As a result of the numerical calculation of the coefficients of the empirical regression equations in the MATHCAD 15 programming environment using approximation and spline interpolation methods, a regression equation was obtained for the dependence on the mileage of the standard deviation of the wear of the tooth of the pinion of the large-toothed wheel of the traction reducer of the VL80s electric locomotive.

$${\sigma }_x\left(\mathcal{l}\right)=0.0000171\mathrm{x}+0.2582241,$$

(10)

where the correlation coefficient r = 0.195.

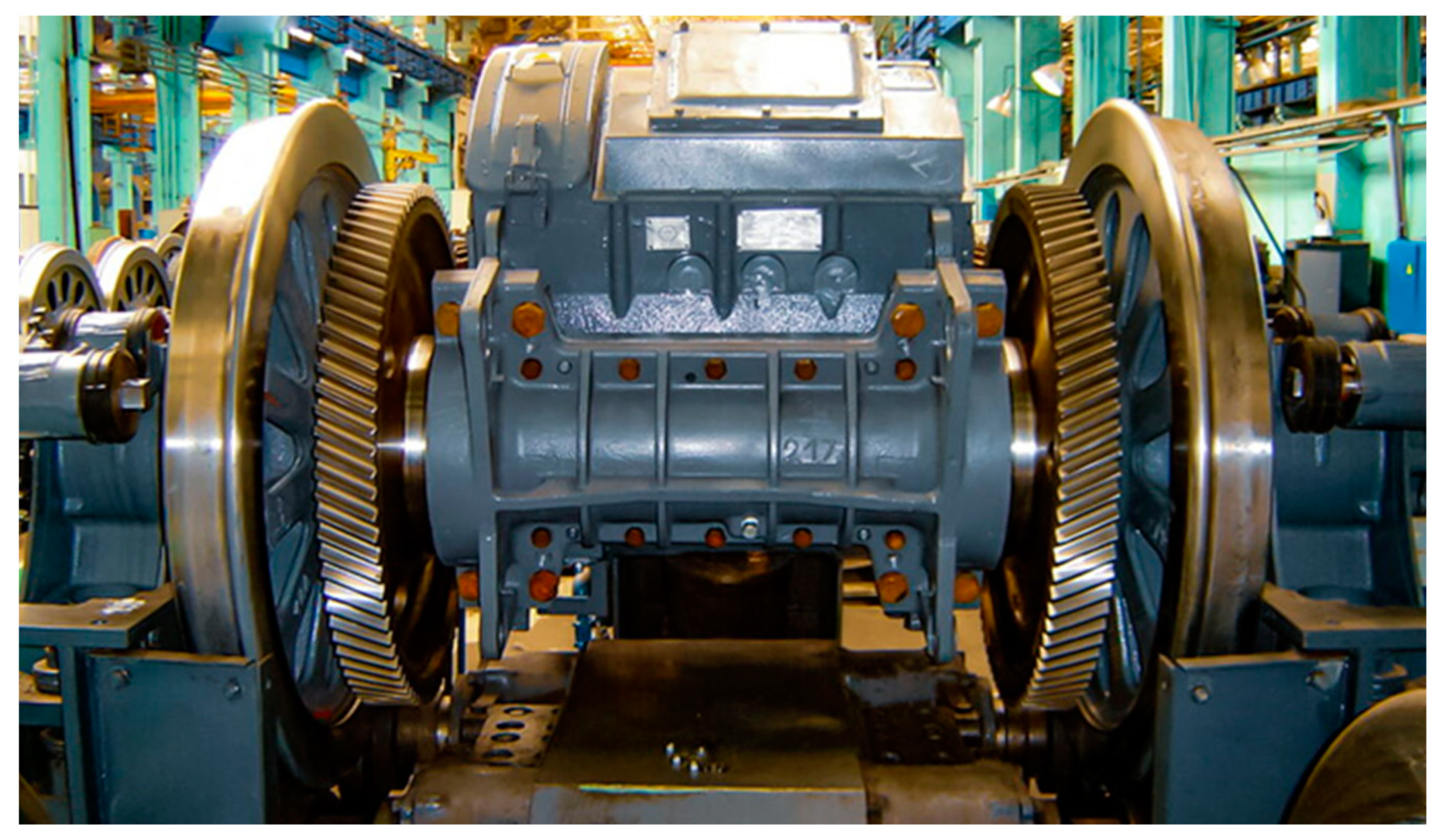
We have conducted surveys of the traction transmission of VL-80s electric locomotives on emerging breakdowns and failures from 2017 to 2023, according to Uzbekistan depot data. The gearing of VL80-c electric locomotives is rigid, double-sided, helical, and consists of two gears and two pinions enclosed in pairs in a protective casing. Figure 3 shows the wheel-motor block (WMB) of a VL-80s electric locomotive with a double-sided helical gear with an all-roller gear.

The advantages of helical gearing are the following:

−

The helical teeth, located at an angle of 24°37′12″, ensure simultaneous gearing of pinion and gear teeth on both sides (due to axial displacement of the TEE armature with two pinions in roller bearings by 6 ÷ 8 mm);

−

The high smoothness of gearing, because the wheel teeth do not enter and exit the gear at once with their entire width b, but gradually;

−

A reduction in noise and additional internal dynamic load.

The disadvantage of helical gears is the axial force tending to move the wheel with the shaft and the required axial fixation of the shaft. Therefore, the tine angle is limited and is recommended to be taken as $\beta =8\dots 25°$.

According to the data of the works, the reject dimensions of the gear are as follows [4,5,6,7]:

• Tooth thickness wear is allowed but not more than 3.5 mm, measured at a height of 10 mm from the top of the tooth;
• Lateral (axial) clearance between the teeth of the gear and the gear wheel in gearing is allowed but not more than 5.5 mm;
• The radial clearance between the gear and gear teeth should be 2.5 + 5.3 mm and depends on the wear of the babbitt in the motor axle bearing shells (“MABs”);
• The pinion may not hang down more than 6 mm from the gear wheel;
• Cracks in the teeth are not permitted;
• Dents, gouges, and splinters on the teeth are allowed: on the gear, not more than 15% (depth not more than 3 mm); on the gear wheel, not more than 25% of the tooth surface (the number of such teeth is not limited).

It has been established that the most dangerous type of failure leading to failure of traction gear and damage to other parts is tooth breakage, which can be caused by large overloads of impact and static action or fatigue of the material from repeatedly flowing loads. Possible causes may be the concentration of dynamic loads along the length of the teeth due to manufacturing errors or large elastic deformations of the shafts; wear of the teeth resulting in weakening and increase in dynamic loads; or insertion of moving gears into the gearing on the move. Cracks usually appear at the base of the teeth on the side of the stretched fibers.

In fatigue damage, the fracture is concave on the gear body and in overload damage it is convex. The teeth of wide helical gears usually break out along the oblique section (from the base to the top of the opposite face), so the teeth must be calculated for bending and contact stiffness to prevent breakage.

Let us consider two types of tooth damage subject to analytical calculation for their prevention—fatigue pitting of the tooth surface and tooth breakage. Fatigue pitting from contact stresses and friction forces are the main type of tooth surface damage. Fatigue pitting of the tooth surface (i.e., falling out of pieces of metal from the surface of the wheel tooth) is preceded by the nucleation of cracks on its surface, which is possible if the contact stress in the areas of cracks σ_H > σ_H0, (contact endurance limit of the tooth material). Therefore, the main criterion for the operability of the teeth of such wheels is fatigue contact strength. Tooth failure is most commonly of a fatigue nature from the prolonged action of alternating bending stresses σ_F in the tooth stems.

Fatigue cracking is also favored by stress concentrations, and the resulting crack develops, leading to sudden tooth failure. Teeth with high surface hardness (at HB > 350) are particularly prone to breakage. Therefore, the main criterion for the operability of such wheels is the bending fatigue strength of the teeth.

2. Materials and Methods

The scope of the research in this article is the determination of the kinematic characteristics of the gear wheel, the resulting transfer functions from dynamic loads, as well as the dynamic calculation of the bending and contact rigidity of the teeth of the pinion and wheel of a double-sided helical traction transmission of electric locomotives.

A great contribution to the solution of this problem was made by the works of Bolotovskaya T.P., Bolotovsky I.A., Bulgakov E.B., Dorofeev V.L., Kudryavtsev V.N., Nezhurin I.P., and other authors.

An analysis is performed on the main methods of designing gears currently used in railway transport on the basis of the standards OST 1 00258-77 [21] “Evolute cylindrical spur gears with external engagement. Calculation of geometric parameters” and OST 1 00480-83 [22] “Evolute cylindrical gears with external engagement. Calculation of geometry in generalizing parameters”. A certain disadvantage of the design method according to OST 1 00258-77 is the dependence of the geometry of the teeth of gear wheels on the geometry of the standard tool. For example, a positive offset coefficient can be used to increase the bending strength by increasing the thickness of the tooth root. On the other hand, an increase in the positive offset coefficient for both gear wheels also leads to a decrease in the tooth height by the amount of the equalizing offset, which leads to a decrease in the overlap coefficient and a decrease in the contact strength of the teeth [3,4].

The design method in generalized parameters according to OST 1 00480-83 allows an increase in the overlap coefficient and improves the smoothness of operation, the contacts, and the bending strength. The disadvantage of this design method in generalized parameters is the complexity of the selection of the initial data for obtaining the required tooth shape. Even an insignificant change in the generalized parameter—the profile angle at the top of the teeth (included in the initial data), for example—leads to a significant change in the shape of the teeth, which significantly complicates the design. In both listed methods, blocking contours are used, providing an initial selection of gear parameters. The blocking contour method is one of the varieties of the simplified calculations of gear transmissions [5,6]. The possibility of the existence of gearing is determined by a set of “blocking contour” lines that limit the choice of tool displacement coefficients according to various geometric factors. The transmission cannot exist outside the boundaries of the blocking contour. A number of indicators are applied to the blocking contour area, presented in the form of isolines of “quality indicators”. Varying the tool offset allows changing the “quality indicators” of the gear engagement without changing the gearing parameters. However, at each point of the blocking contour, the “quality indicators” of the gear engagement change, and, therefore, the information obtained using isolines is insufficient for practical application in aviation technology [3,4].

The methods used to construct blocking contours do not allow the full potential of this method to be revealed. This is due to the lack of the necessary set of blocking contours for designing aircraft gear wheels and the lack of the ability to design an assessment of the “quality” and strength indicators of gear engagement for each point of the blocking contour. In recent years, spur gears with an increased overlap coefficient have been used in railway transport to transmit high powers at low relative mass. However, for such transmissions, when using strictly involute geometry, problems arise during operation under load, for example, when teeth enter engagement, concentrators of high contact stresses arise on the edges of the teeth [1,2,5,6]. The use of a profile modification allows eliminating peak stress values that arise in highly loaded gear transmissions at the entry and exit of teeth on engagement, reducing the dimensions and weight of gear wheels.

A certain disadvantage of the existing methods of profile modification is that the shape of the profile modification line is selected for both wheels independently of each other. For this reason, solving this problem is a complex procedure that requires multiple calculations and bench experiments, and this approach does not lead to a consistent improvement in the quality of engagement, since the elimination of stress peaks for one section of the gear profile can lead to peak loads in other sections of the profile. Errors in determining the shape of the profile modification line can also lead to increased vibration due to a violation of the conjugacy of the engagement of gears under load. When two modified teeth contact, a position error occurs, the contact point shifts from the theoretical engagement line, which leads to a deviation from the specified gear ratio. This error is characterized as the difference in the positions of the ideal driven link (without errors) and the real one (with an error). The magnitude of contact and bending stresses, as well as the vibration activity of the gear transmission, is also determined by the reduced radii of curvature at each point of contact of the moving tooth profiles [5,6]. To determine the position error and the reduced radii of curvature, it is necessary to solve the inverse problem of the theory of gear engagement, i.e., to determine the law of their motion based on the given shape of the tooth profiles.

The currently existing methods allow finding a solution only for profiles specified by a theoretical function, and they allow finding only one contact point. This solution is not sufficient for practical purposes, as when the profile shape is specified using the results of gear measurement, it can lead to a solution error, especially for the double-sided helical traction transmission of electric locomotives [7].

The analysis showed that in order to improve the quality of designing gears with the required strength and vibration indicators, it is necessary to develop and apply a comprehensive method for calculating and selecting the geometric parameters of double-sided helical traction transmission of electric locomotives, ensuring the required stress state of the teeth.

The initial data in the new design method are module, number of teeth, angle of the original contour, thickness or thickness coefficient of the tooth at the top, radial clearance coefficient, offset coefficient, and angle of inclination of the teeth. Although the design is independent of the tool, and various gear cutting methods can be used to obtain the required profile shape, in each individual case, it is necessary to determine the parameters of the tool selected for shaping.

In previous works and methods [1,2,3,4,5,6,7], the tooth thickness was not included in the initial parameters of gear wheels, although this parameter determines many design and technological indicators of gear transmissions and the kinematic characteristics of the gear wheel, the resulting transfer functions from dynamic loads were not taken into account, and a dynamic calculation of the bending and contact rigidity of the teeth of the gear and wheel of the double-sided helical traction transmission of the VL-80s electric locomotive was not carried out, as well as the development of a new method for calculating the strength of teeth based on bending and torsional stresses, taking into account diagnostic parameters according to operating data.

In this regard, in this article proposed by us, the subject of the study is the dynamic calculations of the bending and contact rigidity of the teeth of the pinion and wheel of the double-sided helical traction transmission of the VL-80s electric locomotive, as well as the development of a new method for calculating the strength of the teeth based on bending and torsion stresses, taking into account the diagnostic parameters according to the operating data. The paper uses the Gaussian system and matrix analysis methods and applies numerical methods: Fourier method, piecewise linear approximation method, iteration method, and boundary element method (the Boundary Element Technology). The numerical studies were carried out in C# language and MATHCAD 15 programming environment for software products that have received a certificate of official registration of the computer program of the Republic of Uzbekistan No. DGU 02322,07664,10286 [12,13,14].

3. Results

During the dynamic calculation of the bending and contact stiffness of the teeth of the pinion gear and the wheel of the double-sided helical traction gear of the electric locomotive VL-80s, the first stage is the calculation of contact shear stresses of helical gear, taking into account diagnostic parameters according to operation data.

The gear is forged from chromium-nickel alloy steel 20XNZA with subsequent carburizing and hardening of tooth surfaces along the entire contour. Inside there is a tapered hole with a slope of 1:10 for pressing onto the shaft of the TEE armature. There is a 15 mm wide notch on the side of the hole for the nut. The gear is cut into 21 helical teeth at an angle of 24°. The gear wheel is forged from 55X carbon steel and consists of a hub and a middle part in the form of a disk with holes and a crown with teeth. The forged gear is ground on all sides, 16 relief holes are drilled in the center section and 88 oblique teeth are cut on the crown at an angle of 24°. The teeth are cemented and hardened.

I. Let us calculate the contact fatigue resistance of the active surfaces of wheel teeth.

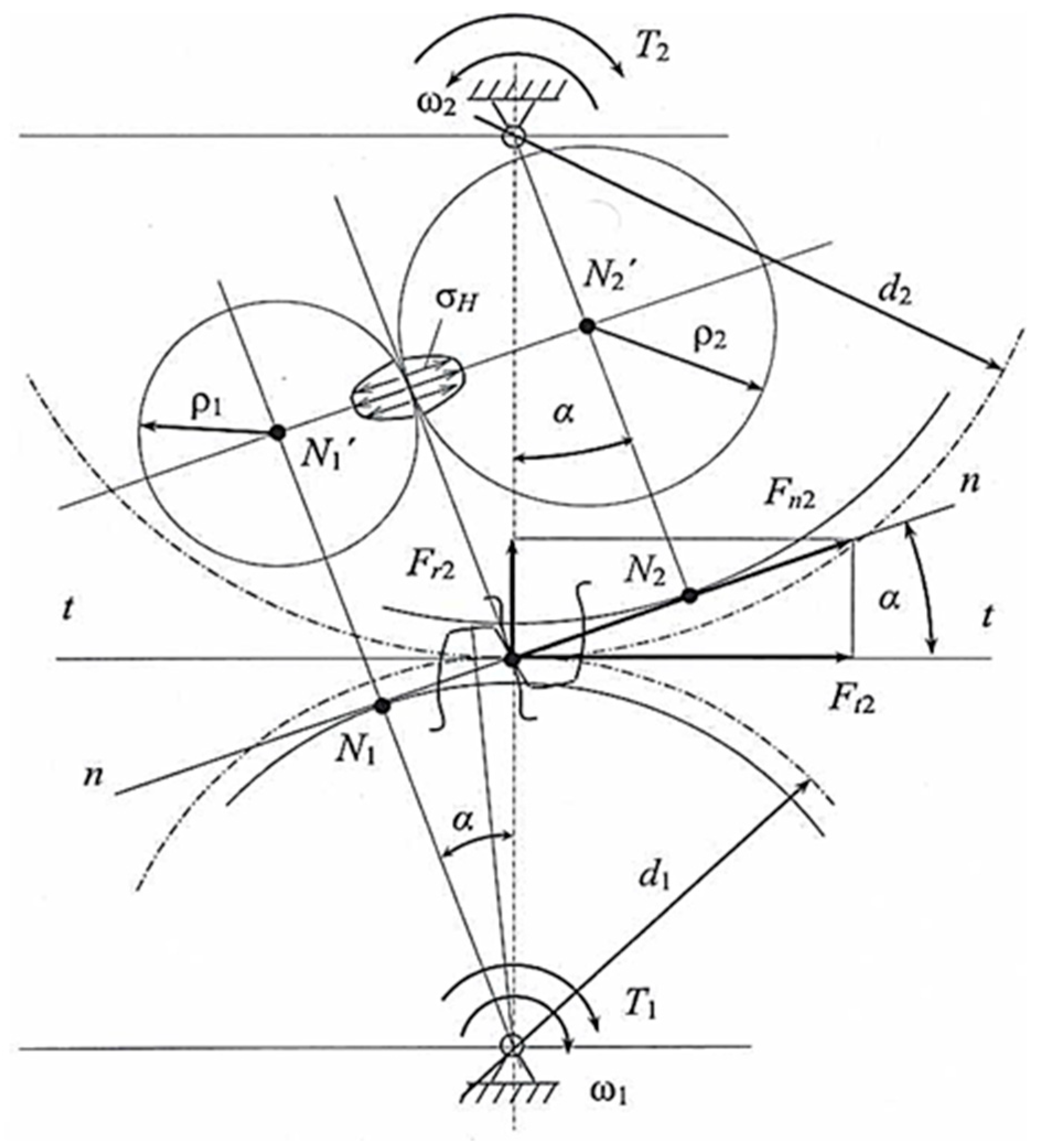
The purpose of calculation is to establish the dependence of serviceability index (contact stress σ_H) on the external load and geometric dimensions of helical gear wheels. The calculation scheme for the calculation of the wheel tooth surface for contact strength is shown in Figure 4.

Based on the Hertz formula [2,3] for the case of compression along the formations of two cylinders, we take the calculation formula as

$${\sigma }_H=\sqrt{{\displaystyle \frac{q_n{E}_{pr}}{2\pi {\rho }_{red}\left(1-{\mu }^2\right)}}}$$

(11)

where $q_n={\displaystyle \frac{F_n}{\mathcal{l}}}$—specific normal stress (intensity of interaction between cylinders of length $\mathcal{l}$, compressed by force $F_n$);

${\rho }_{red}={\displaystyle \frac{{\rho }_1 {\rho }_2}{\left({\rho }_2 \pm {\rho }_1\right)}}$—reduced radius of curvature of cylinders;

$E_{red}={\displaystyle \frac{2 E_1 E_2}{\left(E_1+E_2\right)}}$—the reduced modulus of longitudinal elasticity of the gear and gear cylinder material;

$E_1$и $E_2$—longitudinal elastic modulus of the material of the first and second cylinder, respectively; $\mu$—Poisson’s coefficient.

It has been experimentally established that the lowest contact strength has the near-pole zone of the working surfaces of the teeth, in which the highest loads act on the teeth (the full load is transmitted by one pair of teeth) and the sliding speed of the teeth V_ck is not equal to zero [7].

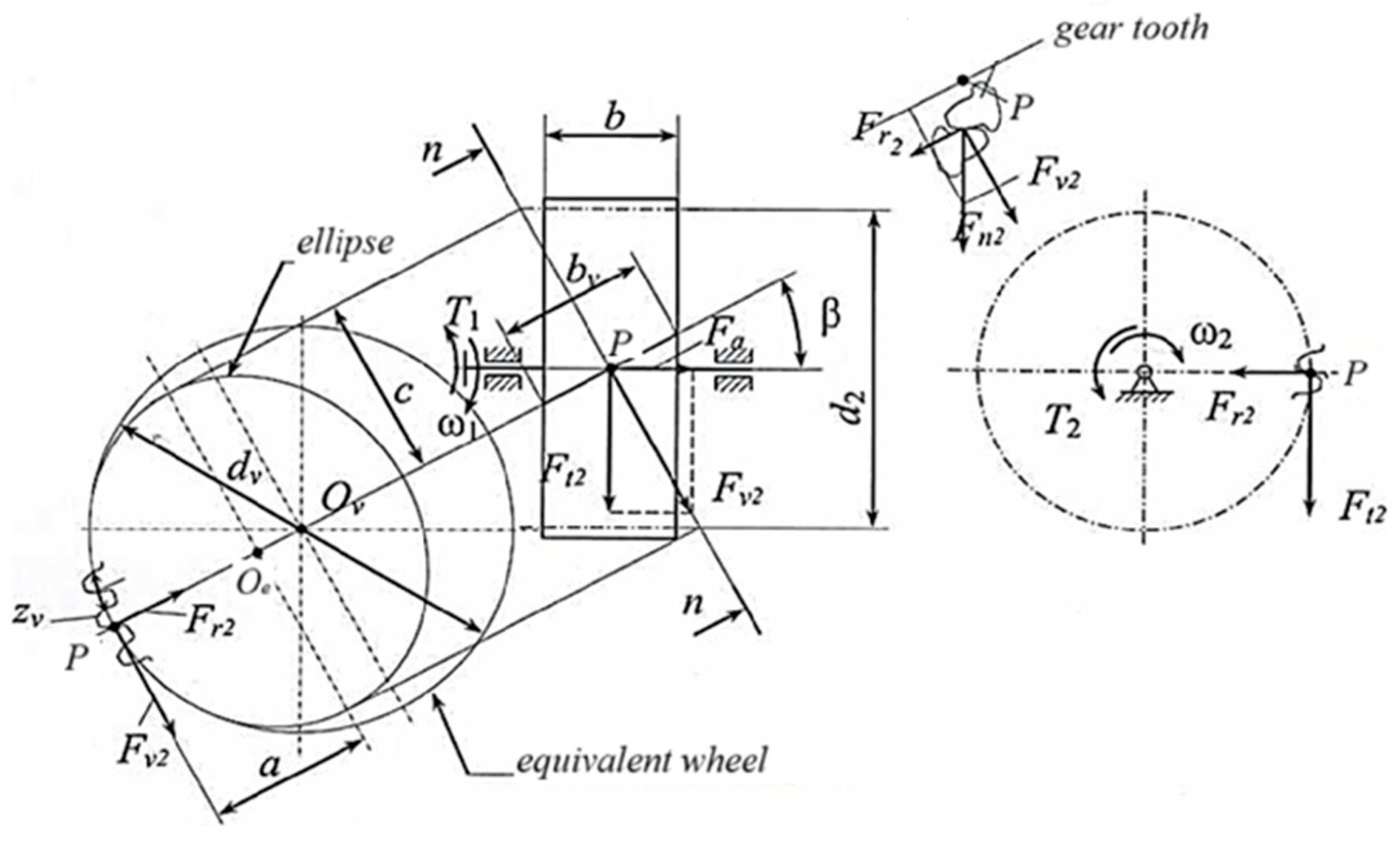
The teeth are regarded as two cylinders of length b (gear ring width) with a radius equal to the radius of curvature of the involutes of the gear and wheel tooth profiles at the meshing pole, i.e., ${\rho }_1$, ${\rho }_2$. From the triangles O₁ N₁ P and O₂ N₂ P (see Figure 5) the radiuses of curvature can be written down ${\rho }_1=N_1\mathrm{P}={\displaystyle \frac{d_1}{2}} \sin \alpha ; {\rho }_2=N_2\mathrm{P}={\displaystyle \frac{d_2}{2}} \sin \alpha$ and through them the reduced radius of curvature

$${\rho }_{red}={\displaystyle \frac{2 d_1{d}_2{\left(\sin \alpha \right)}^2}{4 \left(d_2\pm d_1\right)\sin \alpha }}={\displaystyle \frac{d_{2 }\sin \alpha }{2 \left(u \pm 1\right)}}={\displaystyle \frac{d_{1 }u\sin \alpha }{2 \left(u \pm 1\right)}}$$

(12)

here the sign “plus” is for external, “minus” for internal gearing.

The specific load on the contacting teeth can be calculated as

$$q_n={\displaystyle \frac{F_n}{{\mathcal{l}}_{\mathsf{\Sigma }}}}={\displaystyle \frac{F_n}{b{\epsilon }_a}}={\displaystyle \frac{F_t}{b{\epsilon }_a\cos \alpha }}$$

(13)

where ${\mathcal{l}}_{\mathsf{\Sigma }}=b{\epsilon }_a$—total length of contact lines at the boundary of one- and two-pair gearing.

By substituting the values ${\rho }_{pr}$ and $q_n$ into the Hertz Formula (11) and replacing $\sin \alpha \cos \alpha ={\displaystyle \frac{1}{2 }} \sin 2\alpha$, we obtain

$${\sigma }_H=\sqrt{{\displaystyle \frac{2E_{red}2F_t \left(u \pm 1\right)}{2\pi \sin 2\alpha \left(1-{\mu }^2\right)b{\epsilon }_a{d}_1}}}$$

(14)

Denoting:

$Z_H=\sqrt{{\displaystyle \frac{2}{\sin 2\alpha }}}$—coefficient, taking into account the shape of contacting tooth surfaces (for normal wheels at $\alpha =20°$, $Z_H=$1.76); $Z_M=\sqrt{{\displaystyle \frac{E_{red}}{2\pi \left(1-{\mu }^2\right)}}}$—coefficient, taking into account mechanical properties of materials of contacting wheels (for steel wheels $Z_M=$275 MPa^1/2 ); $Z_{\epsilon }=\sqrt{{\displaystyle \frac{1}{{\epsilon }_a}}}$—coefficient taking into account the total length of the contact lines (for spur gearing $Z_{\epsilon }\approx 1$).

Then, we obtain the calculation dependence in the form recommended by GOST for verification calculation

$${\sigma }_{H1}=Z_H{Z}_M{Z}_{\epsilon }\sqrt{{\displaystyle \frac{F_t \left(u \pm 1\right) K_{load\beta }{K}_{loadV}}{bu{d}_1}} } \le \left[{\sigma }_{d1}\right]$$

(15)

where $K_{load\beta }$—coefficient of non-uniformity of load along the length of the contact lines of gearing (along the tooth length for spur wheels), due to misalignment of wheel teeth caused by shaft deflection, deformations of the housing, bearings, or assembly error;

$K_{loadV}$—dynamic load factor, which takes into account the occurrence of additional dynamic loads in the gearing, i.e., takes into account the internal dynamics of the transmission caused by inaccuracies in the manufacturing of wheel teeth in terms of circumferential pitch (usually $p_1 \ne p_2$).

The specified coefficients are selected according to pre-designed tables based on diagnostic parameters from operation data [23].

It should be noted that the verification calculation is carried out on the wheel because the wheel material is assumed to be of lower strength than the gear due to the fact that the tooth of the wheel is less likely to gear (by a factor of one) than the tooth of the gear. Therefore, Formula (4) can be modified. For this purpose, we input the following substitutions

$$F_t={\displaystyle \frac{2T_2}{d_2}} ·{10}^3={\displaystyle \frac{2T_2}{d_{1}u}} ·{10}^3; d_1={\displaystyle \frac{2 a_{\mathcal{W}}}{\left(u \pm 1\right)}}$$

Then

$${\sigma }_{H2}={\displaystyle \frac{Z_H{Z}_M{Z}_{\epsilon }}{au}}\sqrt{{\displaystyle \frac{T_2 {10}^3 {\left(u \pm 1\right)}^3 K_{load\beta }{K}_{loadV}}{2b_2}} } \le \left[{\sigma }_{d2}\right]$$

(16)

In Formula (6), the torque $T_2$ is given in N∙m, a = $a_{\mathcal{W}}$—in mm, contact stresses ${\sigma }_{H2}$ in MPa. The numerical coefficient 10³ is used to harmonize the dimensions of these quantities.

In the planning calculation, $a$ or $d_1$ must be determined from the given torque $T_2$ and transmission ratio u.

To obtain the calculated formula for the center-to-center distance, we introduce in Expression (6) $b={\psi }_{ba}·a$, where ${\psi }_{ba}$—wheel width coefficient with respect to center distance. Then, expressing a from this formula we obtain

$$a_{\mathcal{W}}=a=\left(u \pm 1\right) \sqrt[3]{0.5 {\left(Z_H{Z}_M{Z}_{\epsilon }\right)}^2{K}_{loadV}}\sqrt[3]{{\displaystyle \frac{{10}^3{T}_2{K}_{load\beta }}{{\psi }_{ba}{u}^2{\left[{\sigma }_{d2}\right]}^2}}}$$

Denoted by $K_a=\sqrt[3]{0.5 {\left(Z_H{Z}_M{Z}_{\epsilon }\right)}^2{K}_{loadV}}$—auxiliary factor; for spur gears it is recommended to $K_a$ = 49.5 MPa^1/3 (at $K_{loadV}$ = 1).

Final formula for the design calculation of the center-to-center spacing of closed helical spur steel gears

$$a_{\mathcal{W}}=a=K_a \left(u \pm 1\right) \sqrt[3]{{\displaystyle \frac{{10}^3{T}_2{K}_{load\beta }}{{\psi }_{ba}{u}^2{\left[{\sigma }_{d2}\right]}^2}}}$$

(17)

If it is necessary to determine d₁ at the initial stage of design calculation, we introduce the gear ring width coefficient into Formula (4) ${\psi }_{bd}={\displaystyle \frac{b}{d_1}}$, [24] and expression

$${\displaystyle \frac{F_t}{d_{1}b}}={\displaystyle \frac{2·{10}^3{ T}_1}{d_1^3 {\psi }_{bd}}}={\displaystyle \frac{2·{10}^3{ T}_2}{d_1^3 {\psi }_{bd}u}}$$

whereupon we obtain

$${\sigma }_{H2}=Z_H{Z}_M{Z}_{\epsilon }\sqrt{{\displaystyle \frac{{2·T}_2 {10}^3 {\left(u \pm 1\right)}^3 K_{load\beta }{K}_{loadV}}{d_1^3 {\psi }_{bd} u^2}} } \le \left[{\sigma }_{d2}\right]$$

(18)

From where the gear dividing diameter

$$d_1=K_{d }\sqrt[3]{{\displaystyle \frac{{10}^3{T}_2{K}_{load\beta }\left(u \pm 1\right)}{{\psi }_{bd}{u}^2{\left[{\sigma }_{d2}\right]}^2}}}$$

(19)

where $K_d=\sqrt[3]{2{\left(Z_H{Z}_M{Z}_{\epsilon }\right)}^2{K}_{loadV} }$—auxiliary coefficient; for steel spur wheels is recommended to $K_d$ = 78 MPa^1/2. In Formulas (7) and (8), T₂ is given in N∙m, contact stresses ${\sigma }_{H2}$ in MPa, $d_1$ and $a$—in mm. Values of ${\psi }_{bd}$ selected according to the recommendations in

Table 1. Values of recommended coefficients according to diagnostic parameters.

Position of Wheels Relative to the Supports	Hardness of Active Tooth Surfaces Title 3
	${\mathbf{HB}}_{\mathbf{1}} \mathbf{\le }$ $350 \mathbf{or} {\mathbf{HB}}_1 \mathbf{and} {\mathbf{HB}}_2 \le$ 350	${\mathbf{HB}}_1 \mathbf{and} {\mathbf{HB}}_2 >$ 350
Symmetrical	0.8…1.4	0.4…0.9
Unsymmetrical	0.6…1.2	0.3…0.6
Cantilever	0.3…0.4	0.2…0.25

. By choosing ${\psi }_{bd}$, determine ${\psi }_{ba}$ by formula ${\psi }_{ba}={\displaystyle \frac{2{\psi }_{bd}}{\left(u+1\right)}}$.

$${\psi }_{bd}={\displaystyle \frac{b}{d_1}}$$

Formulas (5), (6) and (8) follow the trend that the value of contact stresses ${\sigma }_H$ does not depend separately on the modulus or on the number of teeth but is determined only by their product or wheel diameters. According to the conditions of contact strength for given $d_1$ or a, the transmission modulus can be as small as desired or correspond to the equality $mz=d_1$ and $m \left(z_1+z_2\right)=2a$.

The value of m is selected based on practical recommendations and diagnostic parameters, and then the tooth is tested for bending fatigue. When checked, it is possible to obtain ${\sigma }_F$ much smaller than [${\sigma }_F$] because the load capacity at tooth surface hardness H < 350 HB is limited by contact fatigue rather than bending fatigue.

If the calculated value exceeds the permissible value ${\sigma }_F$, then at the accepted values of d and m increase m. This means that bending fatigue, rather than contact fatigue, is decisive in a given transmission of the selected materials. In practice, such cases occur in the case of wheels with high-hardness teeth at the H > 50 HRC.

The following should be considered when selecting a module.

Fine-modular wheels with a large number of teeth are better for smooth running conditions (${\epsilon }_a$ increases) and for economic reasons.

However, for power transmissions, it is recommended to take $m\ge$ 2 MM. In high-speed gears, it is recommended to use the following for noise reduction $z_{1 }\ge$ 26.

Large-modular wheels can operate for a long time after the onset of pitting and are less sensitive to overloading.

Given these diagnostic parameters, the following recommendations should be used in the orientated evaluation of the module.

1. By choosing ${\psi }_m$ from

Table 2. Selection of wheel tooth modulus.

Construction Characteristic	ψ_m = b/m, No More than
Highly loaded precision gears, shafts, supports and housings with increased rigidity at HB:
up to 350	45…30
over 350	30…20
Conventional gearbox-type transmissions in a separate housing with sufficiently rigid shafts and supports at the HB:
up to 350	30…25
over 350	20…15
Rough gears (including those with cantilever shafts)
Rough gears (including those with cantilever shafts)	15…10

, determine $m$ = ${\displaystyle \frac{b}{{\psi }_m}}$, where $b={\psi }_{bd}{d}_1$; $d_1$ is obtained from the contact fatigue calculation. If a is obtained from the calculation, then we determine $d_1={\displaystyle \frac{2 a}{\left(u \pm 1\right)}}$, then ${\psi }_{bd}={\displaystyle \frac{{\psi }_{ba}\left(u \pm 1\right)}{2}}$, $b={\psi }_{bd}{d}_1$ and further $m$.

2. The modulus is chosen according to empirical dependencies:

$m$ = (0.01…0.02) $a_{\mathcal{W}}$—at hardness of gear and wheel teeth H $\le$ 350 HB;

$m$ = (0.01…0.02)$a_{\mathcal{W}}$—at hardness of gear and wheel teeth H $\ge$ 45 HRC.

The obtained modulus value is rounded to the values of GOST 9563.

Row 1: 1; 1.25; 1.5; 2; 2.5; 3; 4; 5; 6; 8; 10; 12; 16; 20…

Row 2: 1.125; 1.375; 1.75; 2.25; 2.75; 3.5; 4.5; 5.5; 7; 9; 11; 14; 18; 22… When assigning modules to Row 1, you should give preference to Row 2.

When selecting ${\psi }_{ba}$, it is recommended to use a number of:

0.1; 0.125; 0.16; 0.2; 0.25; 0.315; 0.4; 0.5; 0.63; 0.8; 1; 1.25.

II. Next, we calculate the bending fatigue resistance of the wheel teeth.

This calculation is the basic calculation for gears with high hardness of the tooth surface (at H $\ge$ 350 HB). The purpose of the calculation is to establish the dependence of the operability index (stress ${\sigma }_F$ in the tooth foot section) on external loading and geometric dimensions of the teeth.

Initial bases for the calculation:

(1) The calculation is made for the moment of force application at the tooth apex (Figure 6). This corresponds to the start of gearing for a wheel tooth and the end of gearing for a gear tooth. Although there is theoretically another pair of teeth in gear at this point, it is assumed that the entire load is transferred by one pair of teeth. Only for precisely manufactured gears (above 6th degree of accuracy) can it be considered that the load is transmitted by two pairs of teeth

Let us transfer the force $F_n$ along its line of action on the symmetry axis of the tooth to point C and decompose it into two force components: circumferential $F_t^{\prime }$ and radial $F_r^{\prime }$;

Then

$$F_t^{\prime }=F_n \cos {\alpha }_{\alpha }=F_t \cos {\alpha }_{\alpha }/\cos \alpha$$

$$F_r^{\prime }=F_n \sin {\alpha }_{\alpha }=F_t \sin {\alpha }_{\alpha }/\cos \alpha$$

Here ${\alpha }_{\alpha }$ is the pressure angle at the tooth apex, which is greater than the pressure (gearing) angle a on the dividing circle.

(2) Having plotted the normal stresses (Figure 4), we obtain that the highest stresses occur in the indicated dangerous section. The concentration of stress is also observed here.

The calculated stress is the stress on the tensile side of the tooth, i.e.,

$${\sigma }_{F_n }={\sigma }_u-{\sigma }_c$$

(20)

fatigue fracture cracks occur on the tensile side of the tooth. This is not random, because the surface layers of the tooth material, as experiments show, offer less resistance to alternating tensile stresses than to compressive stresses.

(3) For the dangerous section D-K, located near the chord of the basic circle, we write (taking into account the stress concentration)

$${\sigma }_F={\sigma }_{F_{nom}}{K}_T$$

(21)

where ${\sigma }_{F_{nom}}$—calculated nominal stress;

$K_T$—theoretical stress concentration coefficient.

$${\sigma }_F=\left({\displaystyle \frac{M_x}{W_x}}-{\displaystyle \frac{N_z}{A}}\right)·K_T=\left({\displaystyle \frac{F_t^{\prime }{h}_p}{W_x}}-{\displaystyle \frac{F_r^{\prime }}{A}}\right)·K_T$$

(22)

where $W_x={\displaystyle \frac{b S_p^2}{6}}$—axial moment of resistance of the dangerous section of the tooth stem;

${ A=S}_{p}b$—tooth stem cross-sectional area;

$h_p$,$S_p$—design tooth height and thickness, respectively;

b—Tooth length (width of the gear wheel ring).

Values $h_p$ and $S_p$ can be expressed in fractions of the tooth modulus: $h_p={\gamma }_{h}m$; $S_p={\gamma }_{S}m$;

Where ${\gamma }_h$, ${\gamma }_S$—coefficients according to design height and design tooth thickness.

Figure 4 shows that the force $F_t^{\prime }$ causes transverse bending of the tooth and the force $F_r^{\prime }$ causes compression of the tooth. Thus, in the dangerous cross-section of the tooth (in the stem at the transition point of the involute to the tooth flange) there are three internal force factors: bending moment M_x, transverse force Q_y and longitudinal force N_z.

Substituting in expression (12) the values included in it, we obtain

$${\sigma }_F={\displaystyle \frac{F_t}{bm}} {\displaystyle \frac{1}{\cos \alpha }} \left({\displaystyle \frac{6{\gamma }_h\cos {\alpha }_{\alpha }}{{\gamma }_S^2}}-{\displaystyle \frac{\sin {\alpha }_{\alpha }}{{\gamma }_S}}\right) K_T$$

(23)

Denoted by

$Y_F={\displaystyle \frac{1}{\cos \alpha }}\left({\displaystyle \frac{6{\gamma }_h\cos {\alpha }_{\alpha }}{{\gamma }_S^2}}-{\displaystyle \frac{\sin {\alpha }_{\alpha }}{{\gamma }_S}}\right)K_T$—tooth shape coefficient, and introducing design load coefficients $K_{F\beta }$ and $K_{FV}$, we obtain a formula for the verification calculation of straight wheel teeth for bending fatigue resistance

$${\sigma }_F={\displaystyle \frac{F_t{Y}_F}{bm}} K_{F\beta }{K}_{FV}\le \left[{\sigma }_F\right]$$

(24)

Substituting in the form (14) $F_t={\displaystyle \frac{2T}{d}}={\displaystyle \frac{2T}{mz}}$, we obtain

$${\sigma }_F={\displaystyle \frac{2T{Y}_F}{b{m}^{2}z}} K_{F\beta }{K}_{FV}\le \left[{\sigma }_F\right]$$

(25)

To obtain the formula for the design calculation it is necessary to substitute in expression (15) $b={\psi }_{bd}{d}_1={\psi }_{bd}m{z}_1$ and solving it with respect to the tooth modulus $m$

$$m\ge \sqrt[3]{2K_{FV}} \sqrt[3]{{\displaystyle \frac{{10}^3{T}_1{Y}_{F_1}{K}_{F\beta }}{{\psi }_{bd}{z}_1^2\left[{\sigma }_{F_1}\right]}}}$$

(26)

Denoting by $K_m=\sqrt[3]{2K_{FV}}$ = 1.4—auxiliary coefficient

We finally obtain

$$m\ge K_m \sqrt[3]{{\displaystyle \frac{{10}^3{T}_1{Y}_{F_1}{K}_{F\beta }}{{\psi }_{bd}{z}_1^2\left[{\sigma }_{F_1}\right]}}}$$

(27)

where $T_1$—torque on the gear shaft, N∙m; $z_1$—number of gear teeth;

$\left[{\sigma }_{F_1}\right]$—permissible bending stress for gear material, MPa;

${\psi }_{bd}$—gear width coefficient relative to the diameter of the dividing circle (taken from the data in Table 1).

Tooth shape coefficient $Y_F$ ($Y_{F_1}>Y_{F_2}$).

To ensure approximately equal operability of the gear and wheel teeth, the gear is made stronger than the wheel.

Gear and wheel teeth have equal resistance to bending fatigue under the condition

$${\displaystyle \frac{\left[{\sigma }_{F_1}\right]}{Y_{F_1}}} \approx {\displaystyle \frac{\left[{\sigma }_{F_2}\right]}{Y_{F_2}}}$$

III. Next, we calculate the contact fatigue resistance of the active surfaces of the teeth of helical gears.

The derivation of the formula for the check calculation is based on the replacement of helical wheels by equivalent spur wheels [4,5,6,7].

The calculation scheme for the contact fatigue resistance calculation of the active surfaces of the teeth of helical gear wheel teeth is shown in Figure 6. Figure 6 also shows the forces acting on the tooth of helical wheel and the formation of an equivalent spur wheel. The calculation of helical gear teeth in the simplest form can be reduced to the calculation of spur wheels (see paragraphs I and II of this article), the strengths of which are mutually equivalent to each other [4,5,6,7].

In this case, the initial formula for determining contact stresses is as follows

$${\sigma }_H=\sqrt{{\displaystyle \frac{q_{n }{E}_{red}}{{\rho }_{red}2\pi \left(1-{\mu }^2\right)}} K_{load\beta }{ K}_{loadV}{K}_{H\alpha }}\le \left[{\sigma }_H\right]$$

(28)

The reduced radius of curvature of tooth profiles of equivalent spur wheels is determined with regard to Formula (2) by the formula

$${\rho }_{red}={\displaystyle \frac{{\rho }_{\nu 2}{\rho }_{\nu 1}}{{\rho }_{\nu 2}\pm {\rho }_{\nu 1}}}={\displaystyle \frac{d_{\nu 2}\sin {\alpha }_n}{2 \left(u\pm 1\right)}}={\displaystyle \frac{d_{\nu 1}u\sin {\alpha }_n}{2 \left(u\pm 1\right) {\left(\cos \beta \right)}^2}}$$

(29)

Taking into account Expressions (5) and (19), we obtain Formula (18) in the form

$${\sigma }_H=\sqrt{{\displaystyle \frac{F_{t }{E}_{red}{\left(\cos \beta \right)}^2}{{b{\epsilon }_{a}K}_{\epsilon } \cos {\alpha }_n\sin {\alpha }_n}}·{\displaystyle \frac{u\pm 1}{u}}·{\displaystyle \frac{2}{d_{1}2\pi \left(1-{\mu }^2\right)}} K_{load\beta }{ K}_{loadV}{K}_{H\alpha }}\le \left[{\sigma }_H\right]$$

(30)

Denoted by:

$Z_H=\sqrt{{\displaystyle \frac{2{\left(\cos \beta \right)}^2}{\sin 2{\alpha }_n}}}=1.76\cos \beta$—coefficient that takes into account the shape of the mating tooth surfaces;

$Z_M=\sqrt{{\displaystyle \frac{E_{red}}{\pi \left(1-{\mu }^2\right)}}}$—coefficient, taking into account mechanical properties of materials of contacting wheels (for steel wheels $Z_M=$275 MPa^1/2);

$Z_{\epsilon }=\sqrt{{\displaystyle \frac{1}{{\epsilon }_a{K}_{\epsilon }}}}$—coefficient taking into account the total length of contact lines depending on

${\epsilon }_a=\left(1.88-3.2\left({\displaystyle \frac{1}{z_1}}\pm {\displaystyle \frac{1}{z_2}}\right)\right)\cos \beta$ and $K_{\epsilon }=0.9\dots 0.95$.

Then, the Formula (20) for the verification calculation will have the form

$${\sigma }_H=Z_M{Z}_H{Z}_{\epsilon } \sqrt{{\displaystyle \frac{F_{t }}{b{d}_1}}·{\displaystyle \frac{u\pm 1}{u}} K_{load\beta }{ K}_{loadV}{K}_{H\alpha }}\le \left[{\sigma }_H\right]$$

(31)

IV. Next, let us calculate the teeth of helical gears for fatigue resistance.

Helical gears are calculated using the formulas of equivalent spur gears with the introduction of correction factors [4,5,6,7]. Similarly, to the calculation of spur gears, the bending stresses in the teeth of helical gears can be calculated by the formula

$${\sigma }_F=Y_F{Y}_{\epsilon }{Y}_{\beta }{\displaystyle \frac{F_V}{b_{\nu }{m}_{\nu }}}{K}_{F\beta }{ K}_{FV}{K}_{F\alpha } \le \left[{\sigma }_F\right]$$

(32)

where $F_V={\displaystyle \frac{F_t}{\cos \beta }}$; $b_{\nu }$=${\displaystyle \frac{b}{\cos \beta }}$. Then, we obtain

$${\sigma }_F=Y_F{Y}_{\epsilon }{Y}_{\beta }{\displaystyle \frac{F_t}{b{m}_n}}$$

(33)

where $Y_{\epsilon }={\displaystyle \frac{1}{{{\epsilon }_{a}K}_{\epsilon }}}$—tooth overlap coefficient. According to GOST 21354 for helical wheels $K_{\epsilon }=1;$$Y_{\beta }=1-{\displaystyle \frac{\beta }{140}}$—coefficient that takes into account the inclination of the teeth.

According to the presented mathematical model by Formulas (1)–(32) the numerical calculation of bending and contact stiffness of teeth of pinion and wheel of double-sided helical traction gear of electric locomotive VL-80s was carried out, and also the development of new method of calculation of strength of teeth by bending and torsion stresses taking into account diagnostic parameters according to operation data in programming environment MATHCAD 15 was carried out.

4. Conclusions

Based on the theoretical and numerical studies carried out, the following general conclusions can be drawn:

First. On the basis of probabilistic-statistical processing of data on long-term experience of operation of double-sided helical traction gear of electric locomotive VL-80s on the Uzbekistan depot, it is established that their application allowed us to sufficiently provide operability of the whole traction gear, and, at the same time, its application had a positive influence on the reliability of operation of other units of traction drive.

Second. The article presents a Pareto diagram constructed for the chassis of 3VL-80s electric locomotives based on data from the Locomotive Operation Department of JSC Uzbekistan Temir Yollari, and also provides a probabilistic statistical analysis of the failures and breakdowns of the wheel pair and the large gear wheel of the traction reducer for VL-80c electric locomotives.

Third. The article presents an algorithm and methodology for assessing the reliability of a large gear wheel of a traction gearbox for electric locomotive. Numerical studies were performed in programming environment, using approximation and spline interpolation methods. As a result of numerical calculation of the coefficients of empirical regression equations using approximation and spline interpolation methods, a regression equation was obtained for the dependence of the mean square deviation of the wear of the tooth of the large gear wheel of the traction reducer of the VL80s electric locomotive on the mileage.

Fourth. On the basis of computational studies carried out in MATHCAD 15 programming environment, it is established that the double-sided helical traction gear used on the electric locomotive VL-80s has a higher carrying capacity because at least two pairs of teeth and more are in operation at the same time;

Fifth. The use of helical gearing reduces tooth wear by 25%, (as the area of gearing is increased, and the teeth enter the gear smoothly, without impact and with less noise than in spur gearing).