On the Correlation of Cymbals’ Vibrational Behavior and Manufacturing Processes
Abstract
1. Introduction
2. Theoretical Background
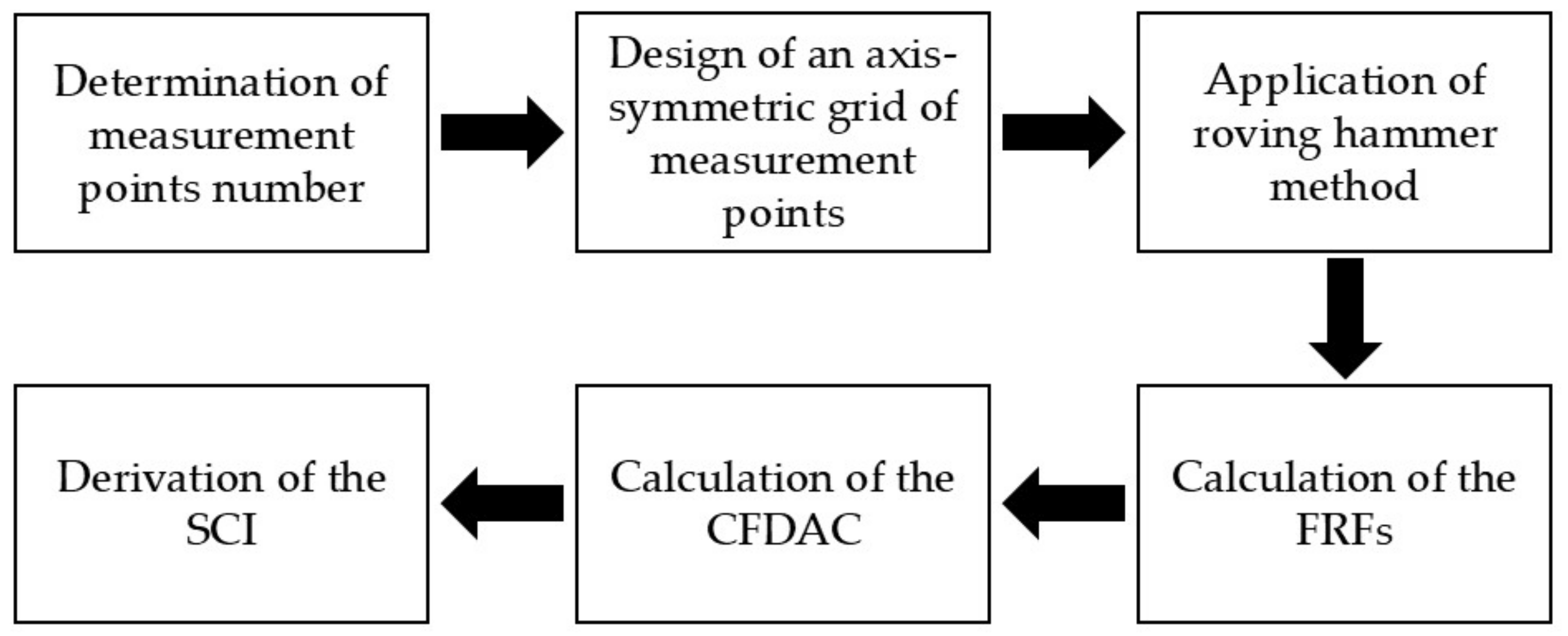
2.1. Complex Frequency Domain Assurance Criterion
2.2. Spectral Correlation Index
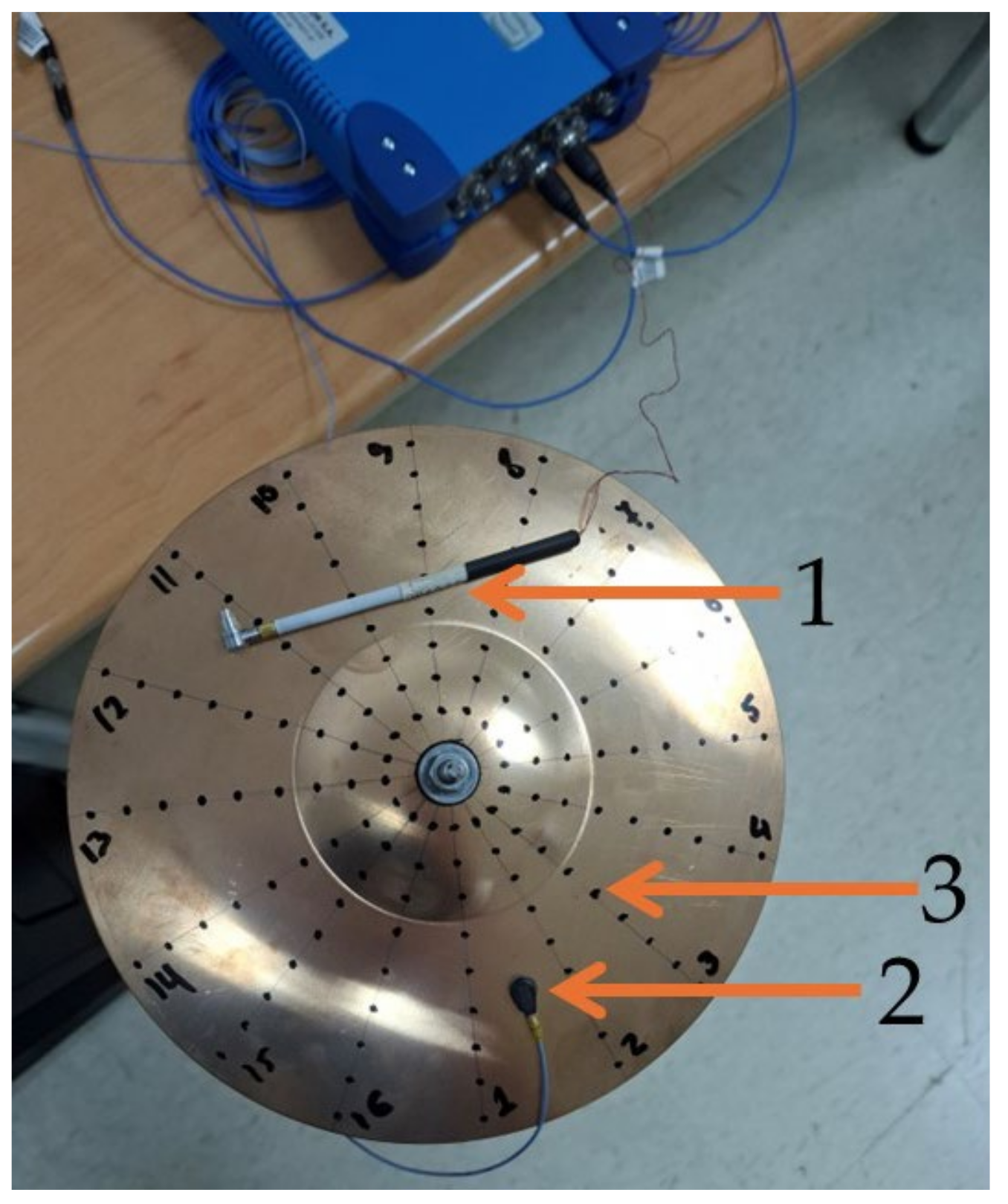
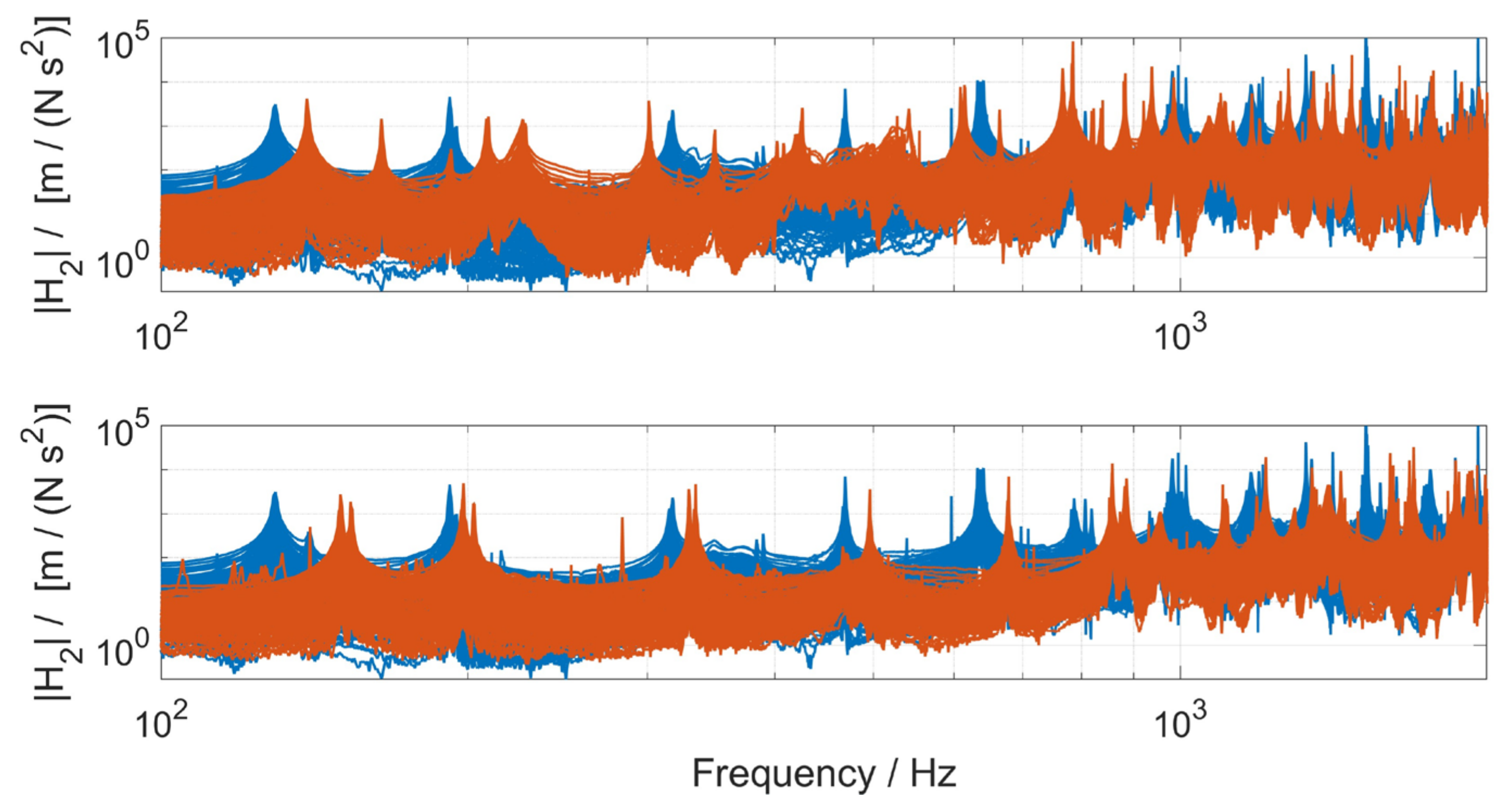
3. Experimental Measurement Setup
4. Results
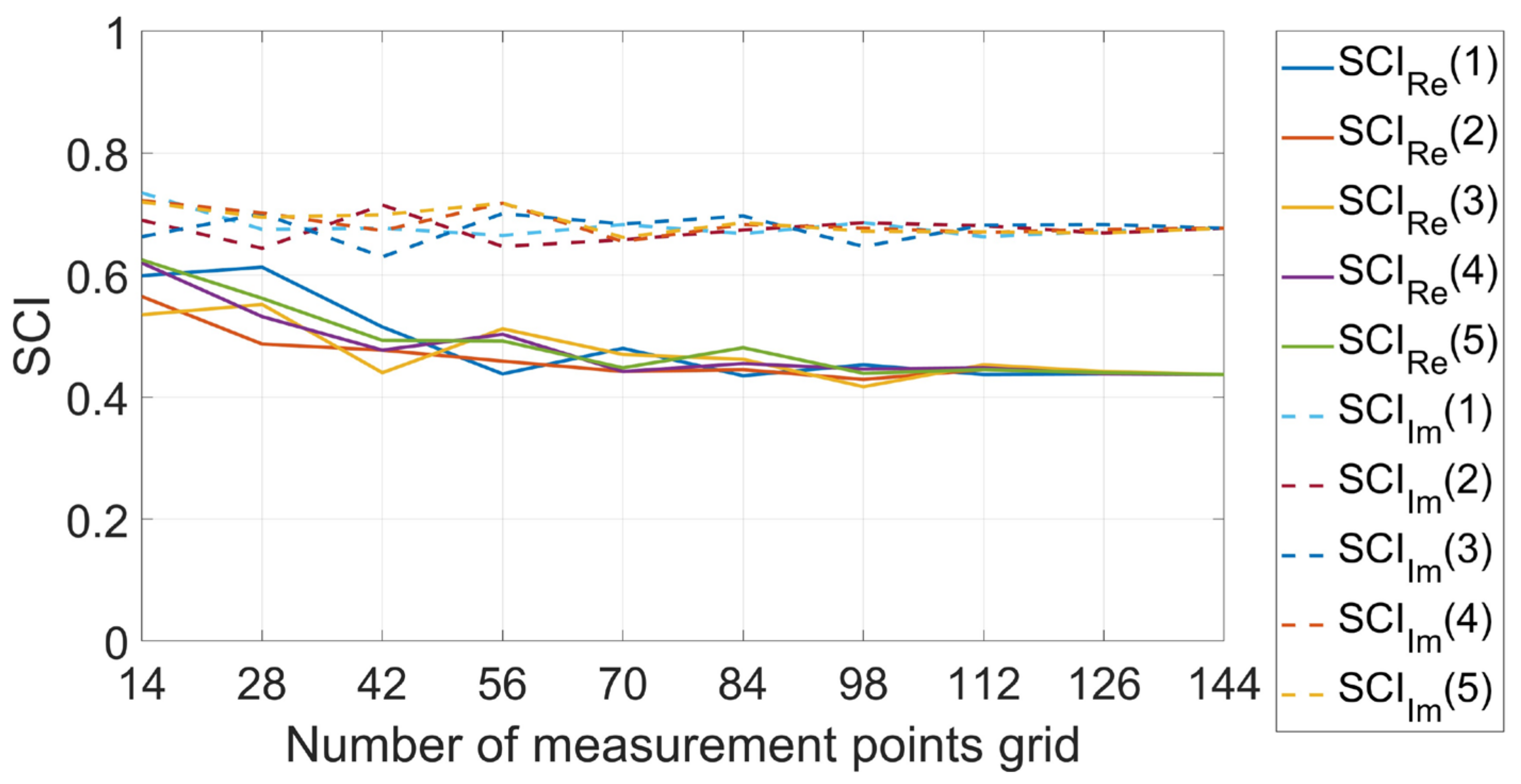
4.1. Stability Calculations
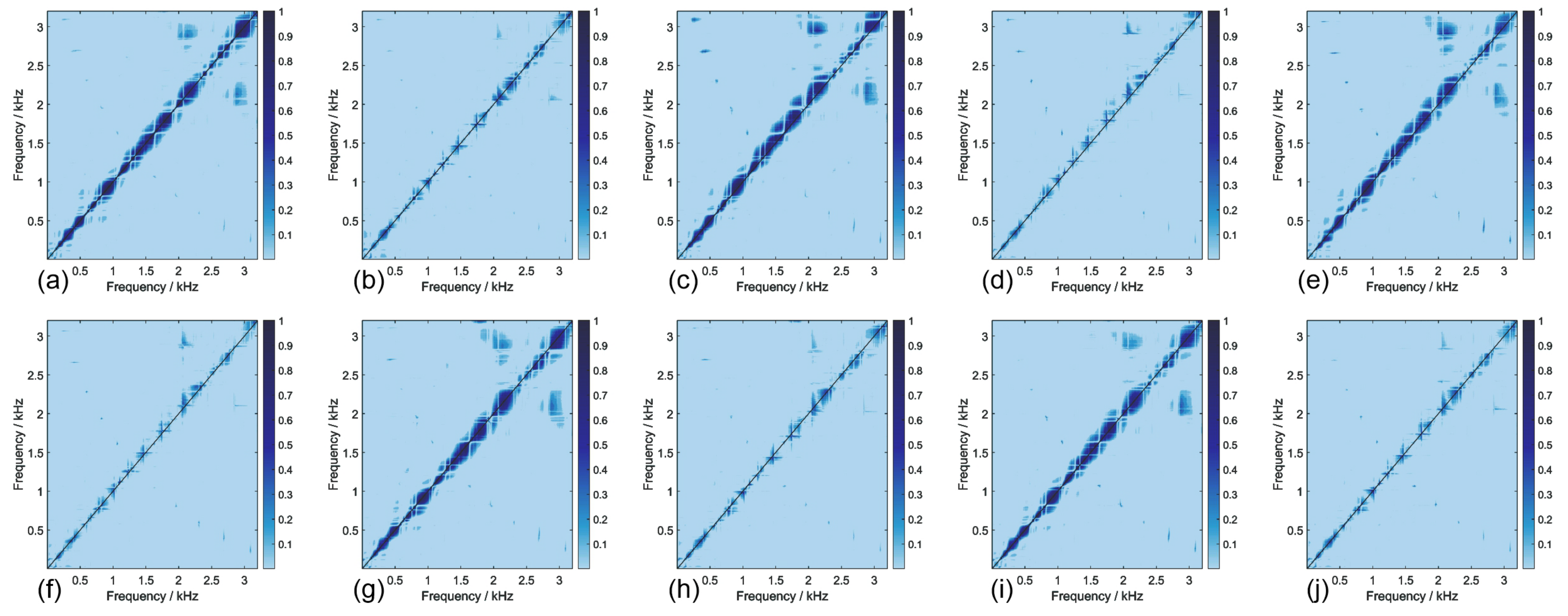
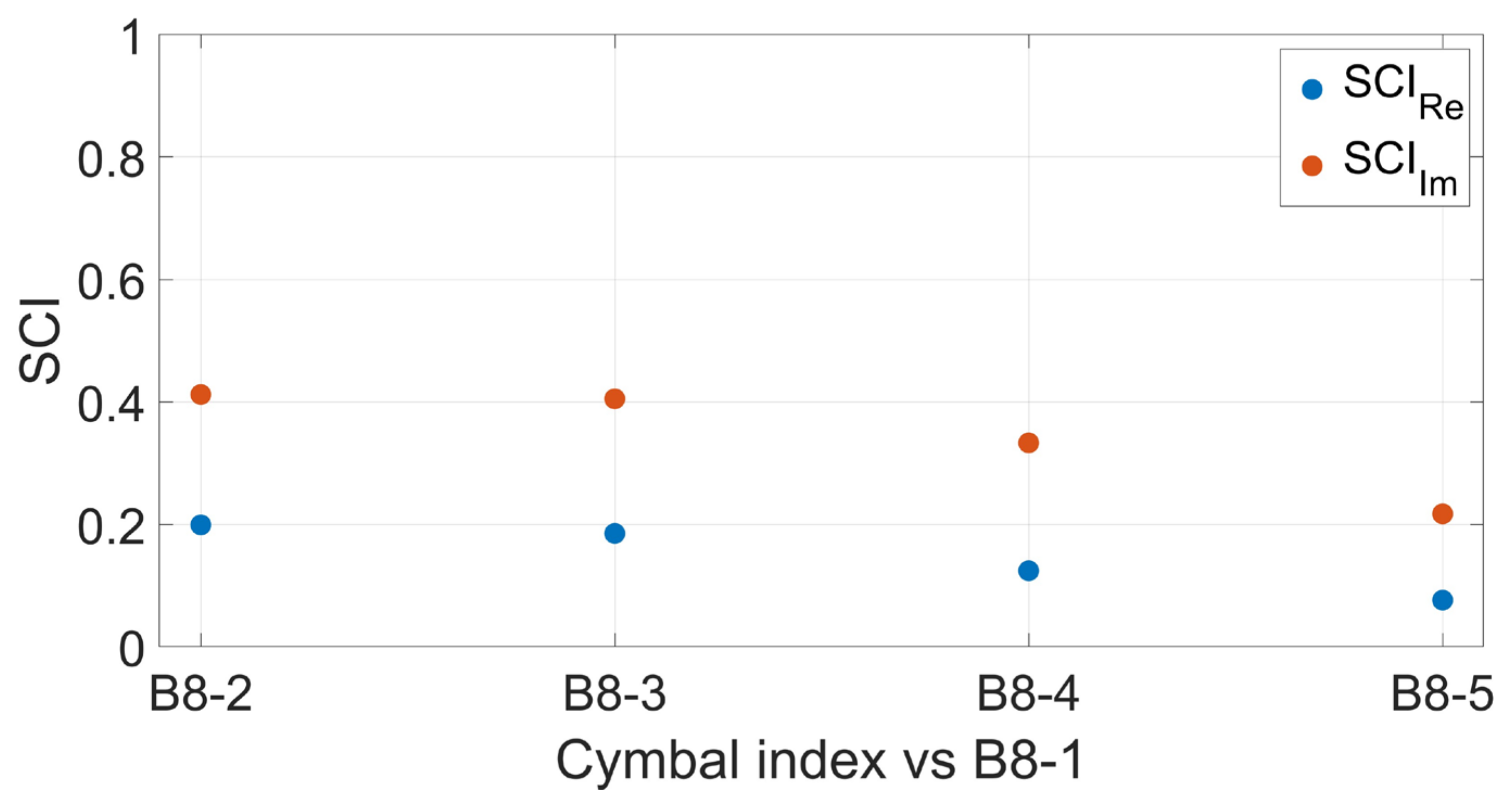
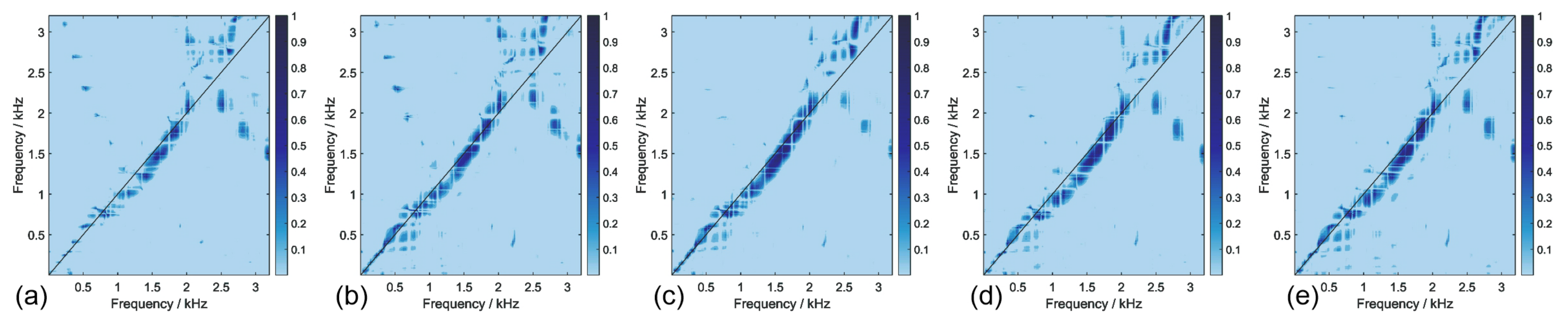
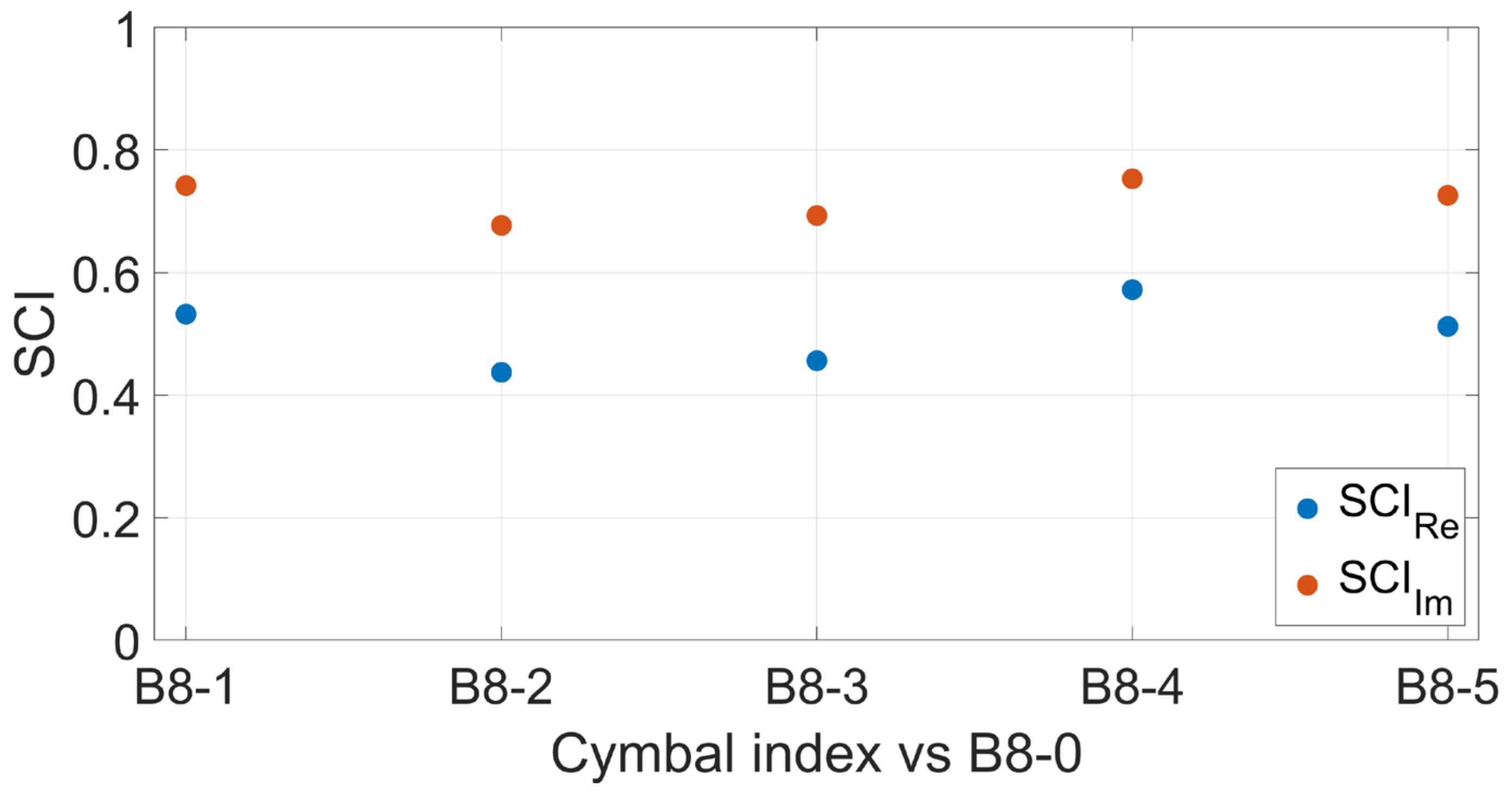
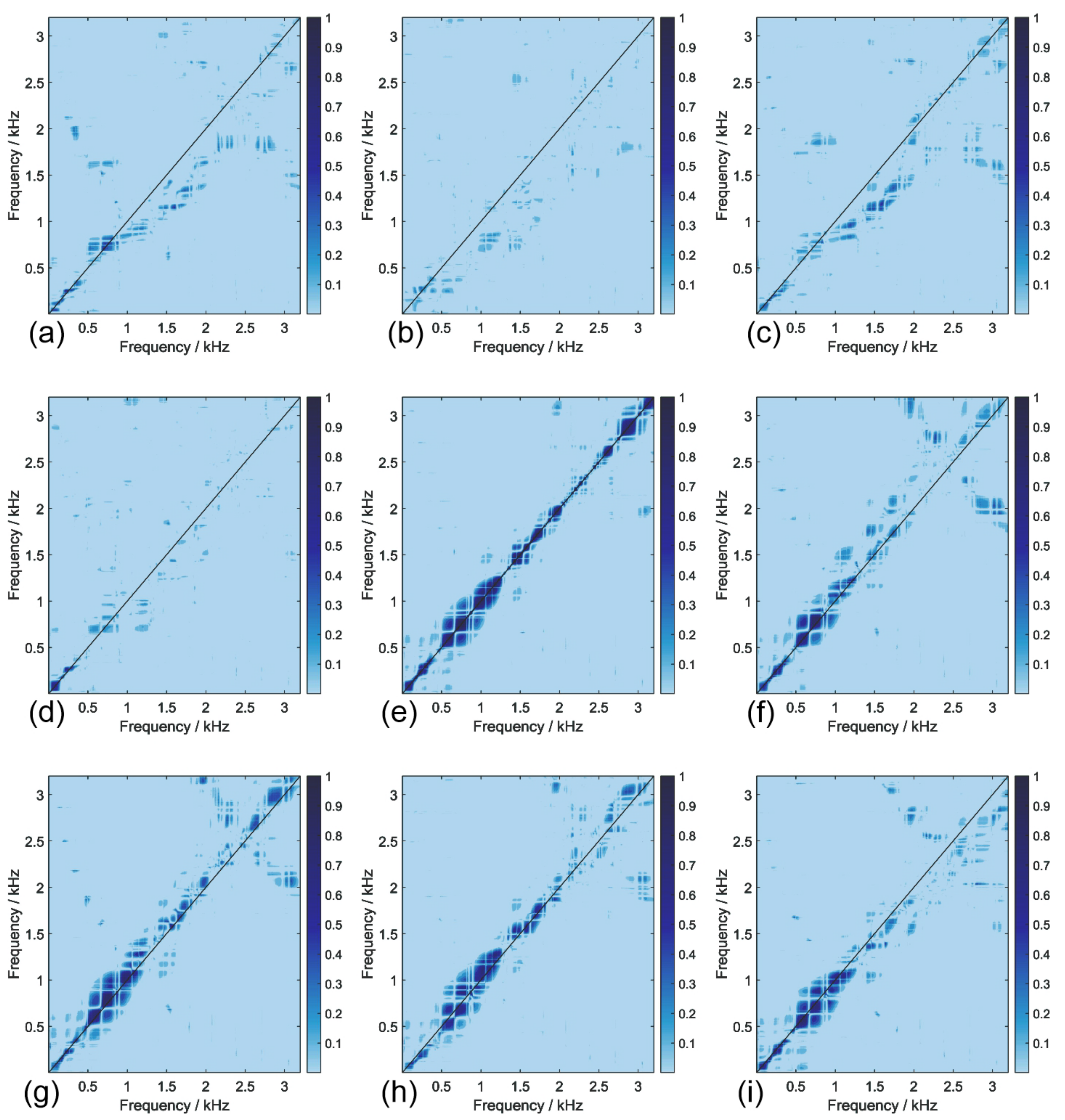
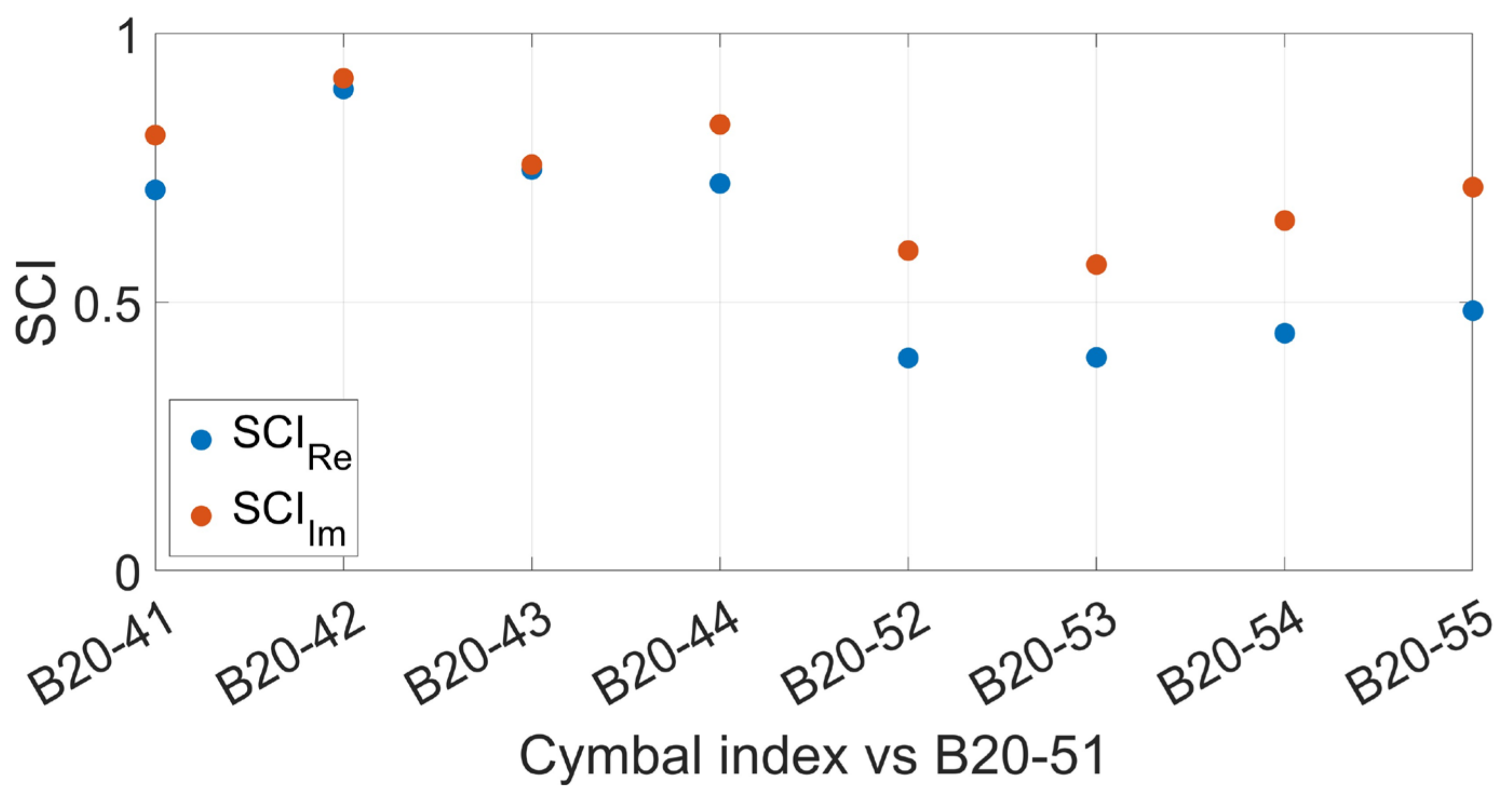
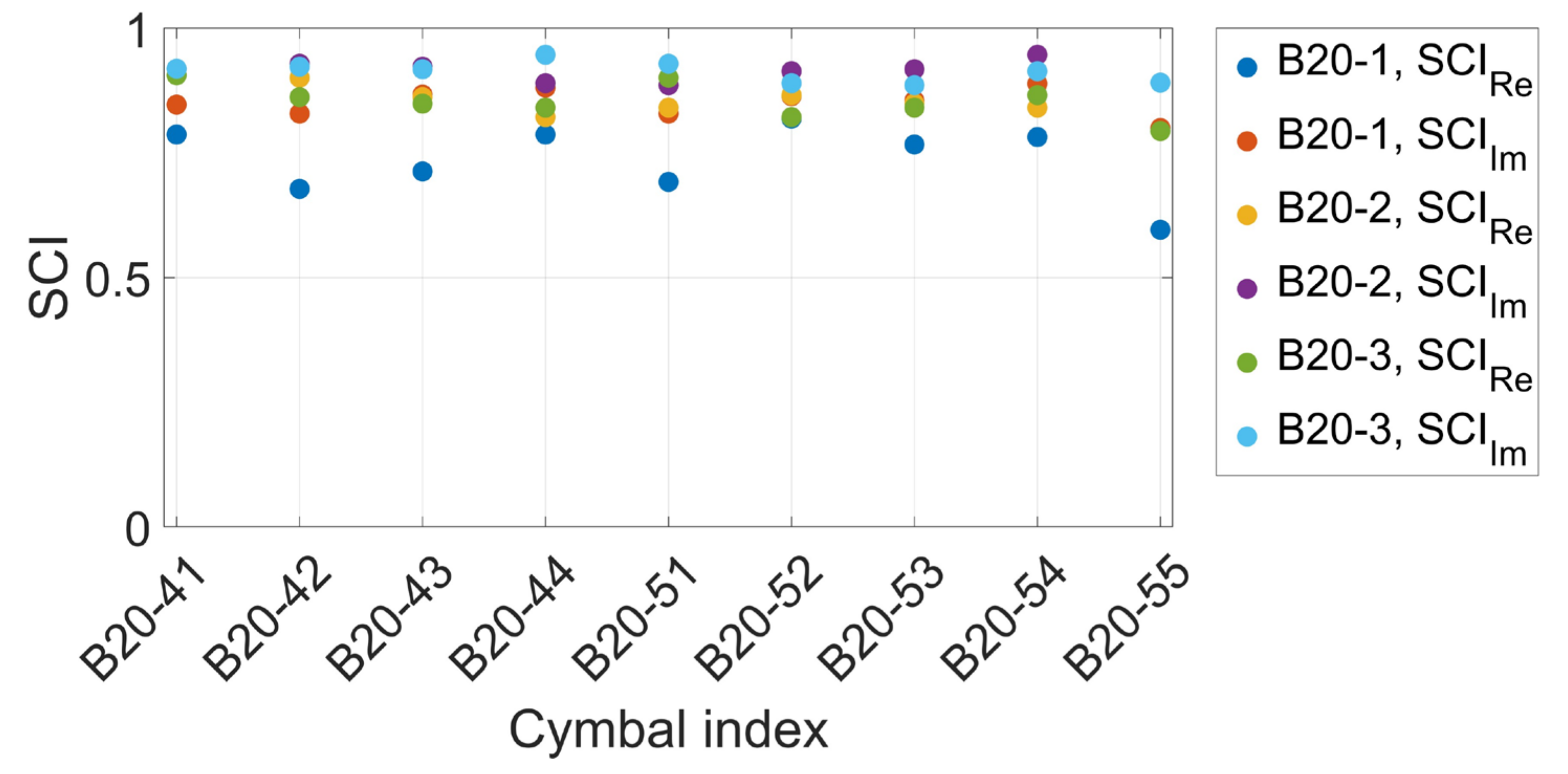
4.2. Correlation Between Blanks
4.3. Correlation Between Blanks and Finished Cymbals
4.4. Correlation Between Blanks and Semi-Finished Cymbals
4.5. Correlation Between Blanks and Finished Cymbals and Between Semi-Finished and Finished Cymbals
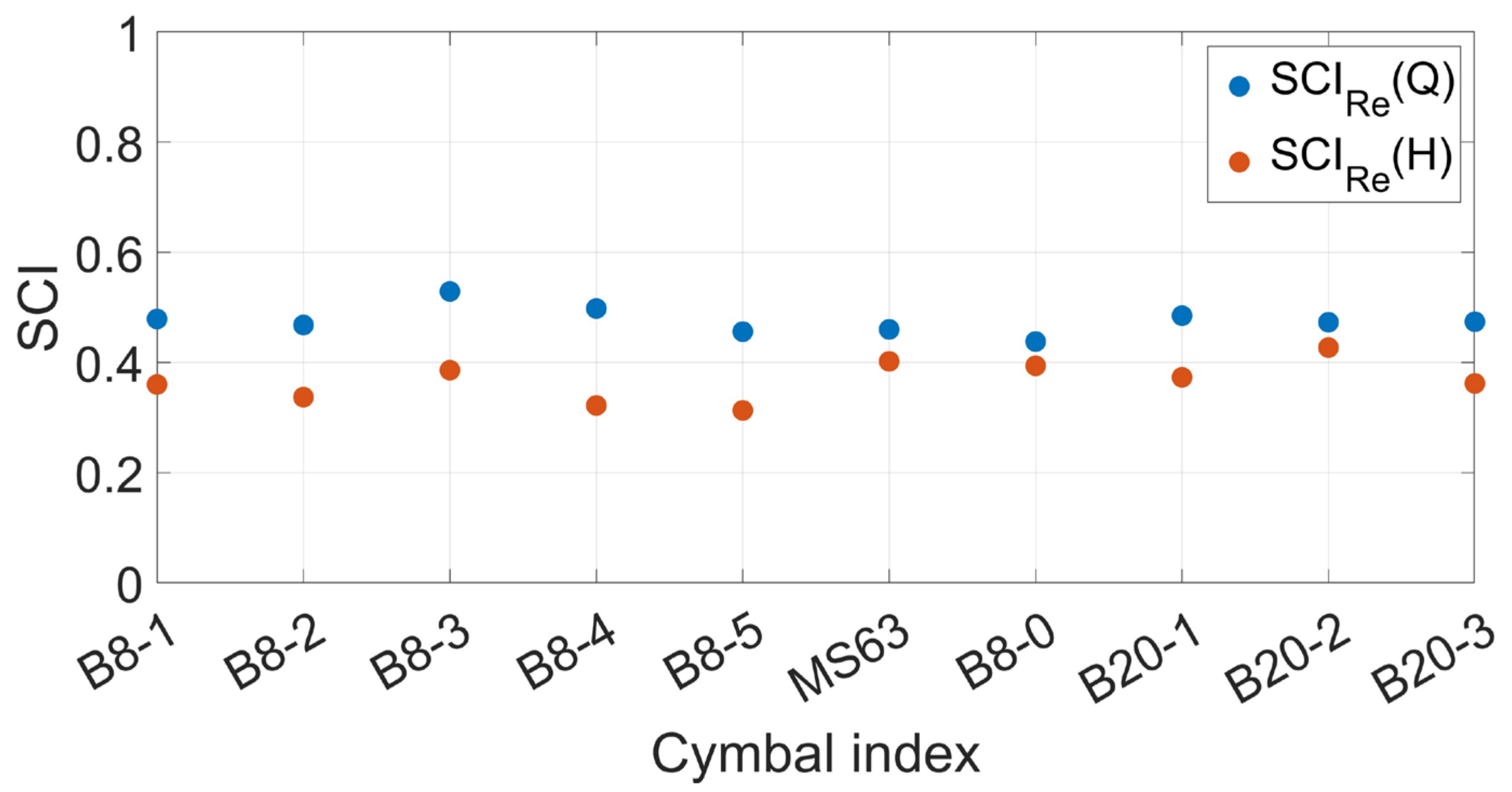
4.6. Vibration Symmetry of Cymbals
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Rossing, T.D. Science of Percussion Instruments, 1st ed.; World Scientific: Singapore, 2000. [Google Scholar]
- Perrin, R.; Swallowe, G.M.; Moore, T.R.; Zietlow, S.A. Normal modes of an 18 inch crash cymbal. Proc. Inst. Acoust. 2006, 28 Pt 1, 653–662. [Google Scholar]
- Bucur, V. Struck Idiophones Played with Mallets: Gongs, Cymbals, Chimes, Sound Plates, Triangle. In Handbook of Materials for Percussion Musical Instruments; Springer: Cham, Switzerland, 2022; pp. 401–481. [Google Scholar]
- Jossic, M.; Mamou-Mani, A.; Chomette, B.; Roze, D.; Ollivier, F.; Josserand, C. Modal active control of Chinese gongs. J. Acoust. Soc. Am. 2017, 141, 4567–4578. [Google Scholar] [CrossRef] [PubMed]
- Fletcher, N.H.; Rossing, T.D. Physics of Musical Instruments, 2nd ed.; Springer: New York, NY, USA, 2010; pp. 640–674. [Google Scholar]
- Schad, C.R.; Frik, G. Plattenglocken. Acustica 1996, 82, 158–168. [Google Scholar]
- Bestle, P.; Hanss, M.; Eberhard, P. Experimental and numerical analysis of the musical behaviour of triangle instruments. In Proceedings of the 5th European Conference of Computational Mechanics (ECCM V), Barcelona, Spain, 20–25 July 2014; pp. 3104–3114. [Google Scholar]
- Perrin, R.; Swallowe, G.M.; Zietlow, S.A.; Moore, T.R. The normal model of cymbals. Proc. Inst. Acoust. 2008, 30 Pt 2, 460–467. [Google Scholar]
- Ducceschi, M.; Touzé, C. Modal approach for nonlinear vibrations of damped impacted plates: Application to sound synthesis of gongs and cymbals. J. Sound Vib. 2015, 344, 313–331. [Google Scholar] [CrossRef]
- Chaigne, A.; Touzé, C.; Thomas, O. Mechanical models of musical instruments and sound synthesis: The case of gongs and cymbals. In Proceedings of the International Symposium on Musical Acoustics, Nara, Japan, 31 March–3 April 2004. hal-03179390. [Google Scholar]
- Nguyen, Q.B.; Touzé, C. Nonlinear vibrations of thin plates with variable thickness: Application to sound synthesis of cymbals. J. Acoust. Soc. Am. 2019, 145, 977–988. [Google Scholar] [CrossRef] [PubMed]
- Kaselouris, E.; Alexandraki, C.; Bakarezos, M.; Tatarakis, M.; Papadogiannis, N.A.; Dimitriou, V. A detailed FEM Study on the Vibro-acoustic Behaviour of Crash and Splash Musical Cymbals. Int. J. Circuits Syst. Signal Process. 2022, 16, 948–955. [Google Scholar] [CrossRef]
- Brezas, S.; Kaselouris, E.; Orphanos, Y.; Tatarakis, M.; Bakarezos, M.; Papadogiannis, N.A.; Dimitriou, V. Vibrational Analysis of a Splash Cymbal by Experimental Measurements and Parametric CAD-FEM Simulations. Vibration 2024, 7, 146–160. [Google Scholar] [CrossRef]
- Nakanishi, T.; Aihara, T.; Iwahar, M.; Sakai, T.; Minorikawa, G. Sound quality analysis of cymbals. In Proceedings of the INTER-NOISE15, San Fransisco, CA, USA, 9–12 August 2015; pp. 4353–4361. [Google Scholar]
- Kaselouris, E.; Paschalidou, S.; Alexandraki, C.; Dimitriou, V. FEM-BEM Vibroacoustic Simulations of Motion Driven Cymbal-Drumstick Interactions. Acoustics 2023, 5, 165–176. [Google Scholar] [CrossRef]
- Osamura, K.; Kuratani, F.; Koide, T.; Ogawa, W.; Taniguchi, H.; Monju, Y.; Mizuta, T.; Shobu, T. The correlation between the percussive sound and the residual stress/strain distributions in a cymbal. J. Mater. Eng. Perform. 2016, 25, 5323–5329. [Google Scholar] [CrossRef]
- Cymbal Wiki. Available online: https://www.cymbal.wiki/wiki/Main_Page (accessed on 13 November 2024).
- Kuratani, F.; Yoshida, T.; Koide, T.; Mizuta, T.; Osamura, K. Understanding the effect of hammering process on the vibration characteristics of cymbals. J. Phys. Conf. Ser. 2016, 744, 012110. [Google Scholar] [CrossRef]
- Zildjian FX Raw Crash Small Bell. Available online: https://www.thomann.de/gr/zildjian_fx_raw_crash_small_bell.htm?glp=1&gad_source=1 (accessed on 17 January 2025).
- Meinl. Byzance Vintage Cymbals. Available online: https://meinlcymbals.com/en/cymbals/ByzanceVintage (accessed on 17 January 2025).
- Pérez, M.A.; Serra-López, R. A frequency domain-based correlation approach for structural assessment and damage identification. Mech. Syst. Signal Process. 2019, 119, 432–456. [Google Scholar] [CrossRef]
- Pérez, M.A.; Font-Moré, J.; Fernández-Esmerats, J. Structural damage assessment in lattice towers based on a novel frequency domain-based correlation approach. Eng. Struct. 2021, 226, 111329. [Google Scholar] [CrossRef]
- Viala, R.; Pérez, M.A.; Placet, V.; Manjón, A.; Foltête, E.; Cogan, S. Towards model-based approaches for musical instruments making: Validation of the model of a Spanish guitar soundboard and characterization features proposal. Appl. Acoust. 2021, 172, 107591. [Google Scholar] [CrossRef]
- Giro, Y.; Le Carrou, J.L.; Vincenti, A.; Dartois, S.; Viala, R.; Navarret, B. Predicting the effects of bracing pattern modifications on acoustic guitar soundboards. In Proceedings of the Forum Acusticum, Turin, Italy, 11–15 September 2023; pp. 3419–3422. [Google Scholar]
- Brezas, S.; Kaselouris, E.; Grigoriou, D.; Papadaki, H.; Orphanos, Y.; Bakarezos, M.; Papadogiannis, N.A.; Dimitriou, V. Vibration analysis of 3D printed cymbals and Tzouras top plates. In Proceedings of the INTER-NOISE24, Nantes, France, 25–29 August 2024; pp. 6741–6749. [Google Scholar]
- Pérez, M.A.; Pernas-Sánchez, J.; Artero-Guerrero, J.A.; Serra-López, R. High-velocity ice impact damage quantification in composite laminates using a frequency domain-based correlation. Mech. Syst. Signal Process. 2021, 147, 107124. [Google Scholar] [CrossRef]
- Product Specifications. Available online: https://www.pcb.com/contentStore/docs/pcb_corporate/vibration/products/specsheets/086e80_c.pdf (accessed on 17 January 2025).
- Product Specifications. Available online: https://www.pcb.com/contentStore/docs/pcb_corporate/vibration/products/specsheets/tld352a56_e.pdf (accessed on 17 January 2025).
- Mc Connell, K.G.; Varoto, P.S. Vibration Testing: Theory and Practice; John Wiley & Sons: New York, NY, USA, 2008. [Google Scholar]

| Alloy | Quantity | Index | Diameter (in) | Mass (g) | Manufacturing Stage | Cymbal | |||
|---|---|---|---|---|---|---|---|---|---|
| Blank | Semi-Finished | Finished/ Commercial | Front View | Side View | |||||
| MS63 | 1 | MS63 | 8 | 380 | ✓ |  |  | ||
| B8 | 1 | B8-0 | 8 | 195 | ✓ |  |  | ||
| B8 | 5 | B8-1,2,3,4,5 | 8 | 222 | ✓ |  |  | ||
| B20 | 1 | B20-1 | 8 | 151 | ✓ |  |  | ||
| B20 | 1 | B20-2 | 8 | 150 | ✓ |  |  | ||
| B20 | 1 | B20-3 | 8 | 157 | ✓ |  |  | ||
| B20 | 4 | B20-41,42,43,44 | 8 | 241 | ✓ |  |  | ||
| B20 | 5 | B20-51,52,53,54,55 | 8 | 247 | ✓ |  |  | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brezas, S.; Kaselouris, E.; Orphanos, Y.; Bakarezos, M.; Papadogiannis, N.A.; Dimitriou, V. On the Correlation of Cymbals’ Vibrational Behavior and Manufacturing Processes. Appl. Sci. 2025, 15, 1425. https://doi.org/10.3390/app15031425
Brezas S, Kaselouris E, Orphanos Y, Bakarezos M, Papadogiannis NA, Dimitriou V. On the Correlation of Cymbals’ Vibrational Behavior and Manufacturing Processes. Applied Sciences. 2025; 15(3):1425. https://doi.org/10.3390/app15031425
Chicago/Turabian StyleBrezas, Spyros, Evaggelos Kaselouris, Yannis Orphanos, Makis Bakarezos, Nektarios A. Papadogiannis, and Vasilis Dimitriou. 2025. "On the Correlation of Cymbals’ Vibrational Behavior and Manufacturing Processes" Applied Sciences 15, no. 3: 1425. https://doi.org/10.3390/app15031425
APA StyleBrezas, S., Kaselouris, E., Orphanos, Y., Bakarezos, M., Papadogiannis, N. A., & Dimitriou, V. (2025). On the Correlation of Cymbals’ Vibrational Behavior and Manufacturing Processes. Applied Sciences, 15(3), 1425. https://doi.org/10.3390/app15031425








