Optimization of Anatase TiO2 Photocatalyst for Diclofenac Degradation by Using Response Surface Methodology
Abstract
1. Introduction
2. Materials and Methods
2.1. Chemicals
2.2. TiO2 Synthesis
2.3. Characterization Methods
2.4. Photocatalytic Degradation Experiments
2.5. Mathematical Modeling
3. Result and Discussion
3.1. Characterization
3.1.1. Phase Composition and Morphology
3.1.2. Textural Properties and Light Absorption
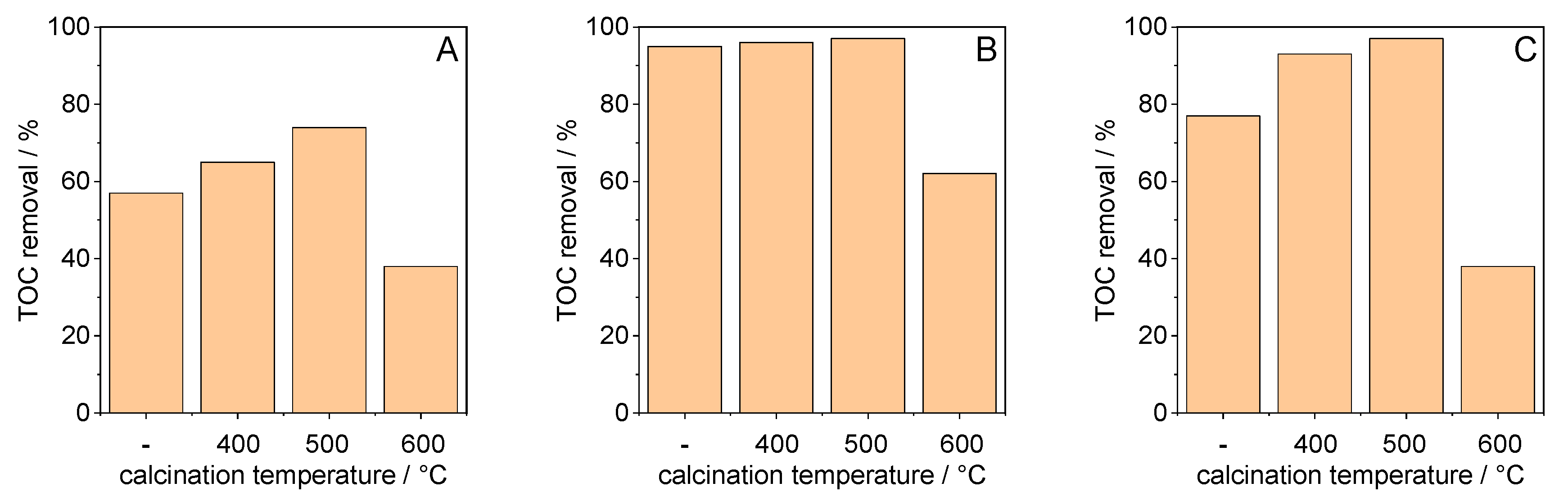
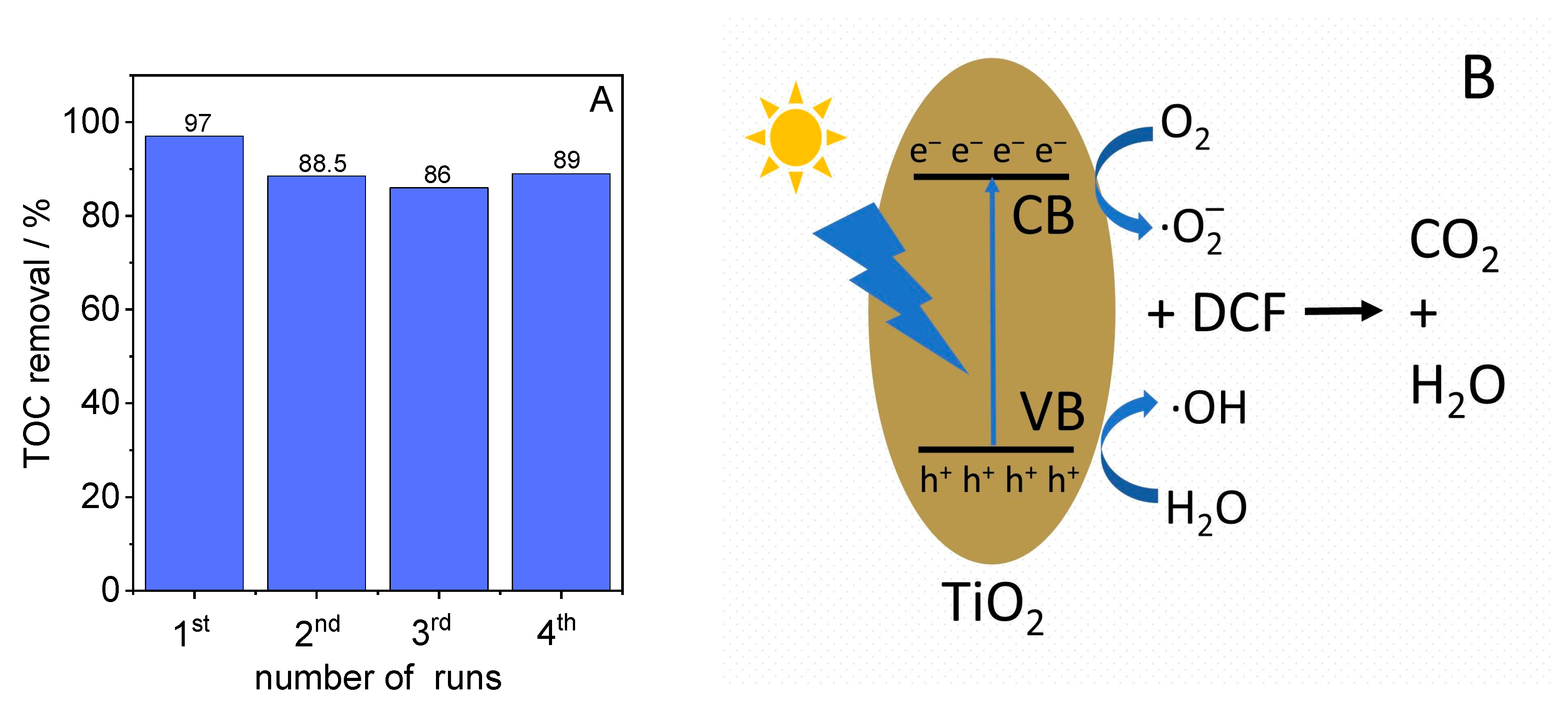
3.2. Photocatalytic Results
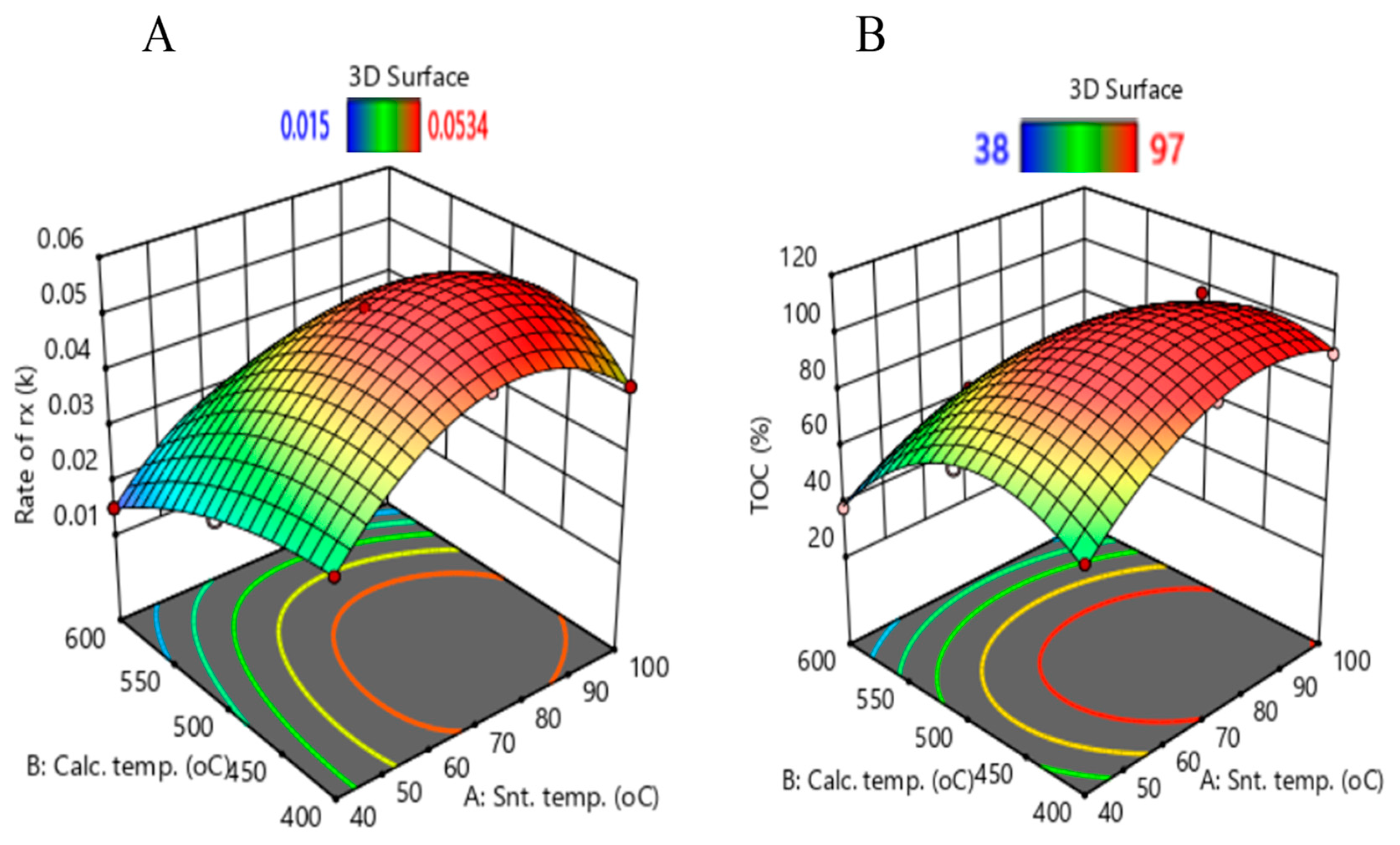
3.3. Results of the RSM Model
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Boretti, A.; Rosa, L. Reassessing the projections of the world water development report. NPJ Clean Water 2019, 2, 15. [Google Scholar] [CrossRef]
- Dandesa, B.; Akuma, D.A.; Alemayehu, E. Water purification improvement using moringa oleifera seed extract pastes for coagulation follow scoria filtration. Heliyon 2023, 9, e17420. [Google Scholar] [CrossRef]
- Pratyusha, K.; Gaikwad, N.M.; Phatak, A.; Chaudhari, P. Review on: Waste material management in pharmaceutical industry. Int. J. Pharm. Sci. Rev. Res. 2012, 16, 121–129. [Google Scholar]
- Ahammad, N.A.; Ahmad, M.A.; Hameed, B.H.; Mohd Din, A.T. A mini review of recent progress in the removal of emerging contaminants from pharmaceutical waste using various adsorbents. Environ. Sci. Pollut. Res. 2023, 30, 124459–124473. [Google Scholar] [CrossRef]
- Younas, F.; Mustafa, A.; Farooqi, Z.U.R.; Wang, X.; Younas, S.; Mohy-Ud-Din, W.; Ashir Hameed, M.; Mohsin Abrar, M.; Maitlo, A.A.; Noreen, S. Current and emerging adsorbent technologies for wastewater treatment: Trends, limitations, and environmental implications. Water 2021, 13, 215. [Google Scholar] [CrossRef]
- Saravanan, A.; Kumar, P.S.; Jeevanantham, S.; Karishma, S.; Tajsabreen, B.; Yaashikaa, P.; Reshma, B. Effective water/wastewater treatment methodologies for toxic pollutants removal: Processes and applications towards sustainable development. Chemosphere 2021, 280, 130595. [Google Scholar] [CrossRef]
- Deegan, A.; Shaik, B.; Nolan, K.; Urell, K.; Oelgemöller, M.; Tobin, J.; Morrissey, A. Treatment options for wastewater effluents from pharmaceutical companies. Int. J. Environ. Sci. Technol. 2011, 8, 649–666. [Google Scholar] [CrossRef]
- Malato, S.; Fernández-Ibáñez, P.; Maldonado, M.I.; Blanco, J.; Gernjak, W. Decontamination and disinfection of water by solar photocatalysis: Recent overview and trends. Catal. Today 2009, 147, 1–59. [Google Scholar] [CrossRef]
- Pavel, M.; Anastasescu, C.; State, R.-N.; Vasile, A.; Papa, F.; Balint, I. Photocatalytic degradation of organic and inorganic pollutants to harmless end products: Assessment of practical application potential for water and air cleaning. Catalysts 2023, 13, 380. [Google Scholar] [CrossRef]
- Vinayagam, V.; Palani, K.N.; Ganesh, S.; Rajesh, S.; Akula, V.V.; Avoodaiappan, R.; Kushwaha, O.S.; Pugazhendhi, A. Recent developments on advanced oxidation processes for degradation of pollutants from wastewater with focus on antibiotics and organic dyes. Environ. Res. 2024, 240, 117500. [Google Scholar] [CrossRef]
- Pandis, P.K.; Kalogirou, C.; Kanellou, E.; Vaitsis, C.; Savvidou, M.G.; Sourkouni, G.; Zorpas, A.A.; Argirusis, C. Key points of advanced oxidation processes (AOPs) for wastewater, organic pollutants and pharmaceutical waste treatment: A mini review. ChemEngineering 2022, 6, 8. [Google Scholar] [CrossRef]
- Saravanan, A.; Kumar, P.S.; Jeevanantham, S.; Anubha, M.; Jayashree, S. Degradation of toxic agrochemicals and pharmaceutical pollutants: Effective and alternative approaches toward photocatalysis. Environ. Pollut. 2022, 298, 118844. [Google Scholar] [CrossRef] [PubMed]
- Velempini, T.; Prabakaran, E.; Pillay, K. Recent developments in the use of metal oxides for photocatalytic degradation of pharmaceutical pollutants in water—A review. Mater. Today Chem. 2021, 19, 100380. [Google Scholar] [CrossRef]
- Madkhali, N.; Prasad, C.; Malkappa, K.; Choi, H.Y.; Govinda, V.; Bahadur, I.; Abumousa, R. Recent update on photocatalytic degradation of pollutants in waste water using TiO2-based heterostructured materials. Results Eng. 2023, 17, 100920. [Google Scholar] [CrossRef]
- Fujishima, A.; Zhang, X.; Tryk, D.A. TiO2 photocatalysis and related surface phenomena. Surf. Sci. Rep. 2008, 63, 515–582. [Google Scholar] [CrossRef]
- Dambournet, D.; Belharouak, I.; Amine, K. Tailored preparation methods of TiO2 anatase, rutile, brookite: Mechanism of formation and electrochemical properties. Chem. Mater. 2010, 22, 1173–1179. [Google Scholar] [CrossRef]
- Katal, R.; Masudy-Panah, S.; Tanhaei, M.; Farahani, M.H.D.A.; Jiangyong, H. A review on the synthesis of the various types of anatase TiO2 facets and their applications for photocatalysis. Chem. Eng. J. 2020, 384, 123384. [Google Scholar] [CrossRef]
- Lekphet, W.; Ke, T.-C.; Su, C.; Kathirvel, S.; Sireesha, P.; Akula, S.B.; Li, W.-R. Morphology control studies of TiO2 microstructures via surfactant-assisted hydrothermal process for dye-sensitized solar cell applications. Appl. Surf. Sci. 2016, 382, 15–26. [Google Scholar] [CrossRef]
- You, Y.; Xu, C.; Xu, S.; Cao, S.; Wang, J.; Huang, Y.; Shi, S.-Q. Structural characterization and optical property of TiO2 powders prepared by the sol–gel method. Ceram. Int. 2014, 40, 8659–8666. [Google Scholar] [CrossRef]
- Han, H.; Jang, J.U.; Oh, D.; Na, K.-H.; Choi, W.-Y.; Jayakrishnan, N.; Nayak, A.K. Advances and Perspectives of Titanium-Based Nanocomposites for Energy Generation and Environmental Remediation Applications: A Review. Energy Fuels 2023, 37, 17708–17735. [Google Scholar] [CrossRef]
- Quintero, Y.; Mosquera, E.; Diosa, J.; García, A. Ultrasonic-assisted sol–gel synthesis of TiO2 nanostructures: Influence of synthesis parameters on morphology, crystallinity, and photocatalytic performance. J. Sol.-Gel Sci. Technol. 2020, 94, 477–485. [Google Scholar] [CrossRef]
- Leyva-Porras, C.; Toxqui-Teran, A.; Vega-Becerra, O.; Miki-Yoshida, M.; Rojas-Villalobos, M.; García-Guaderrama, M.; Aguilar-Martínez, J. Low-temperature synthesis and characterization of anatase TiO2 nanoparticles by an acid assisted sol–gel method. J. Alloys Compd. 2015, 647, 627–636. [Google Scholar] [CrossRef]
- Sebek, M.; Krake, E.F.; Baumann, W.; Strunk, J.; Steinfeldt, N. Effect of atmosphere and relative humidity on photodegradation of clopidogrel under artificial solar and indoor light irradiation. J. Pharm. Biomed. Anal. 2023, 234, 115506. [Google Scholar] [CrossRef]
- Acar, Z.Y.; Asiltürk, M. An investigation on the influence of hydrolysis ratio and base type on the characterization of synthesised nano-TiO2 by using sol–gel method. J. Nanopart. Res. 2022, 24, 162. [Google Scholar] [CrossRef]
- Yoldas, B.E. Hydrolysis of titanium alkoxide and effects of hydrolytic polycondensation parameters. J. Mater. Sci. 1986, 21, 1087–1092. [Google Scholar] [CrossRef]
- Li, C.; Wang, J.; Feng, S.; Yang, Z.; Ding, S. Low-temperature synthesis of heterogeneous crystalline TiO2–halloysite nanotubes and their visible light photocatalytic activity. J. Mater. Chem. A 2013, 1, 8045–8054. [Google Scholar] [CrossRef]
- Sing, K.S. Reporting physisorption data for gas/solid systems with special reference to the determination of surface area and porosity (Recommendations 1984). Pure Appl. Chem. 1985, 57, 603–619. [Google Scholar] [CrossRef]
- Vijayan, B.; Dimitrijevic, N.M.; Rajh, T.; Gray, K. Effect of calcination temperature on the photocatalytic reduction and oxidation processes of hydrothermally synthesized titania nanotubes. J. Phys. Chem. C 2010, 114, 12994–13002. [Google Scholar] [CrossRef]
- Rajput, R.B.; Jamble, S.N.; Kale, R.B. Solvothermal synthesis of anatase TiO2 for the detoxification of methyl orange dye with improved photodegradation efficiency. Eng. Sci. 2021, 17, 176–184. [Google Scholar] [CrossRef]
- Athikaphan, P.; Neramittagapong, S.; Assawasaengrat, P.; Neramittagapong, A. Methanol production from CO2 reduction over Ni/TiO2 catalyst. Energy Rep. 2020, 6, 1162–1166. [Google Scholar] [CrossRef]
- Chan, C.K.; Porter, J.F.; Li, Y.G.; Guo, W.; Chan, C.M. Effects of calcination on the microstructures and photocatalytic properties of nanosized titanium dioxide powders prepared by vapor hydrolysis. J. Am. Ceram. Soc. 1999, 82, 566–572. [Google Scholar] [CrossRef]
- Dette, C.; Pérez-Osorio, M.A.; Kley, C.S.; Punke, P.; Patrick, C.E.; Jacobson, P.; Giustino, F.; Jung, S.J.; Kern, K. TiO2 anatase with a bandgap in the visible region. Nano Lett. 2014, 14, 6533–6538. [Google Scholar] [CrossRef]
- Torralvo-Fernández, M.J.; Enciso, E.; Martinez, S.; Sobrados, I.; Sanz, J.; Tonti, D.; Soria, J.; Yurdakal, S.; Palmisano, G.; Augugliaro, V. Influence of the preparation temperature on the photocatalytic activity of 3d-ordered macroporous anatase formed with an opal polymer template. ACS Appl. Nano Mater. 2018, 1, 2567–2578. [Google Scholar] [CrossRef]
- Luís, A.; Neves, M.; Mendonça, M.; Monteiro, O. Influence of calcination parameters on the TiO2 photocatalytic properties. Mater. Chem. Phys. 2011, 125, 20–25. [Google Scholar] [CrossRef]
- Henderson, M.A. A surface science perspective on TiO2 photocatalysis. Surf. Sci. Rep. 2011, 66, 185–297. [Google Scholar]
- Lukong, V.; Ukoba, K.; Jen, T. Heat-assisted sol–gel synthesis of TiO2 nanoparticles structural, morphological and optical analysis for self-cleaning application. J. King Saud Univ.-Sci. 2022, 34, 101746. [Google Scholar] [CrossRef]
- Arumbu, P.; Srinivasalu, S. Sustainable model for high signal to noise ratio to measure underwater acoustic signal using Acoustic Doppler Velocimeter. Comput. Electr. Eng. 2018, 68, 262–270. [Google Scholar] [CrossRef]
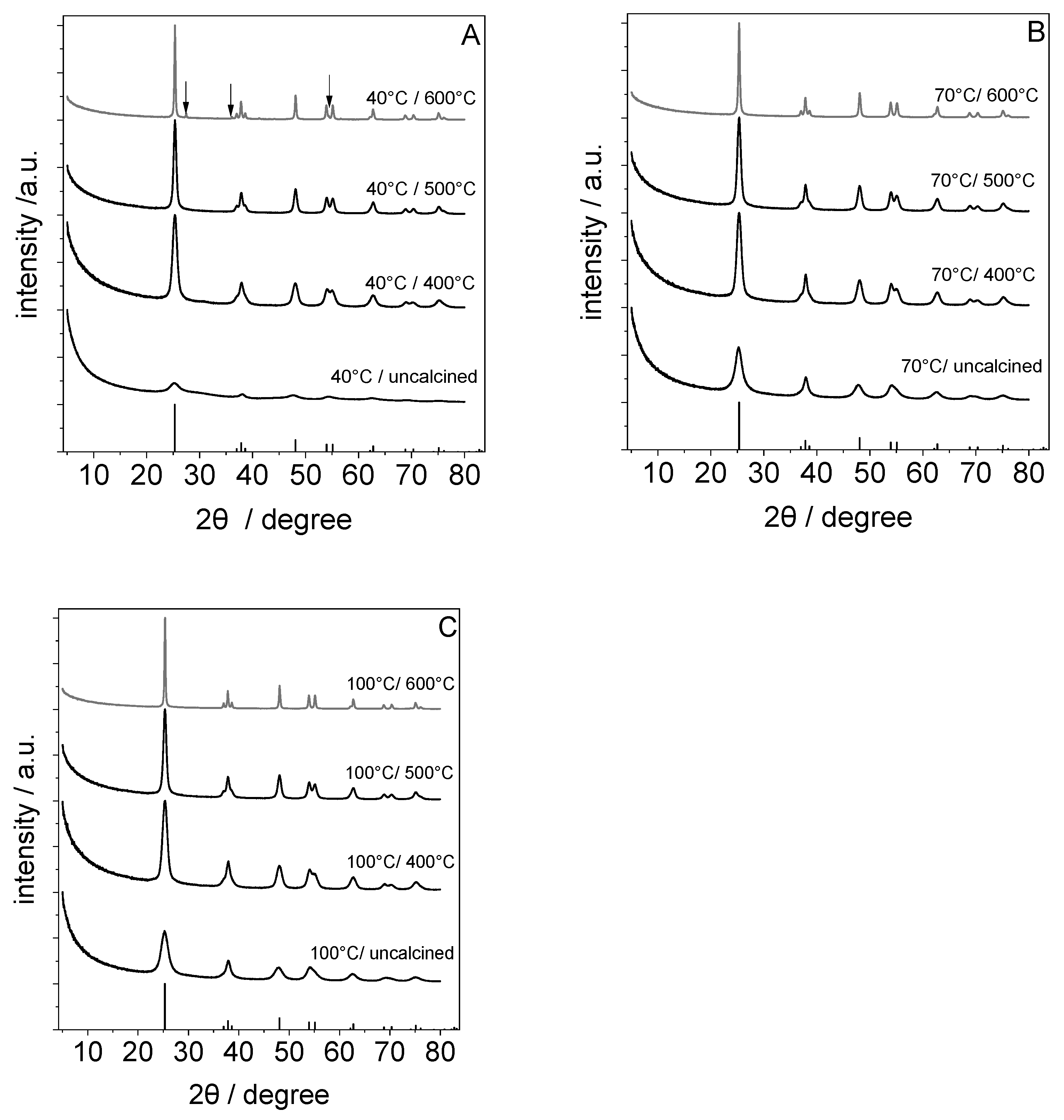

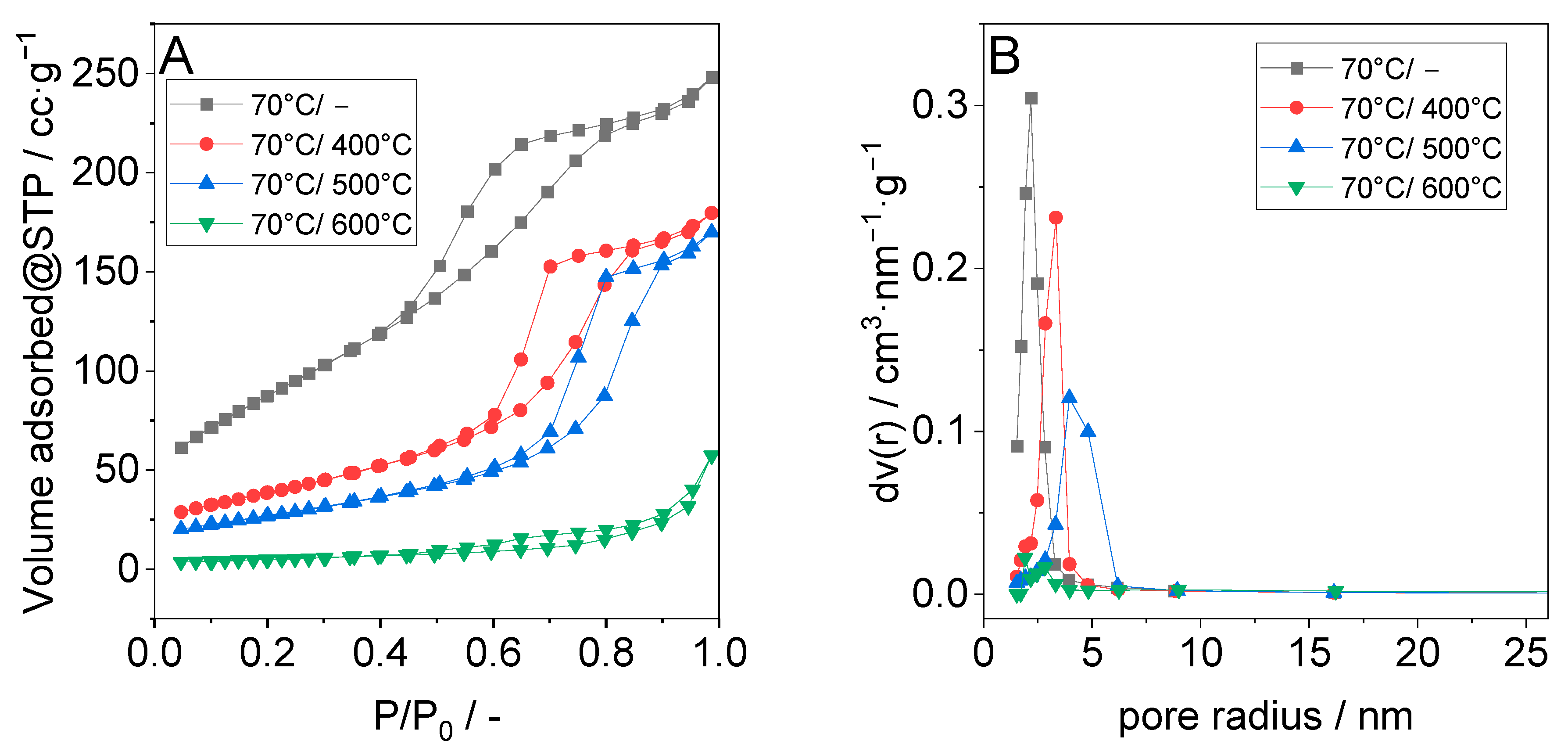
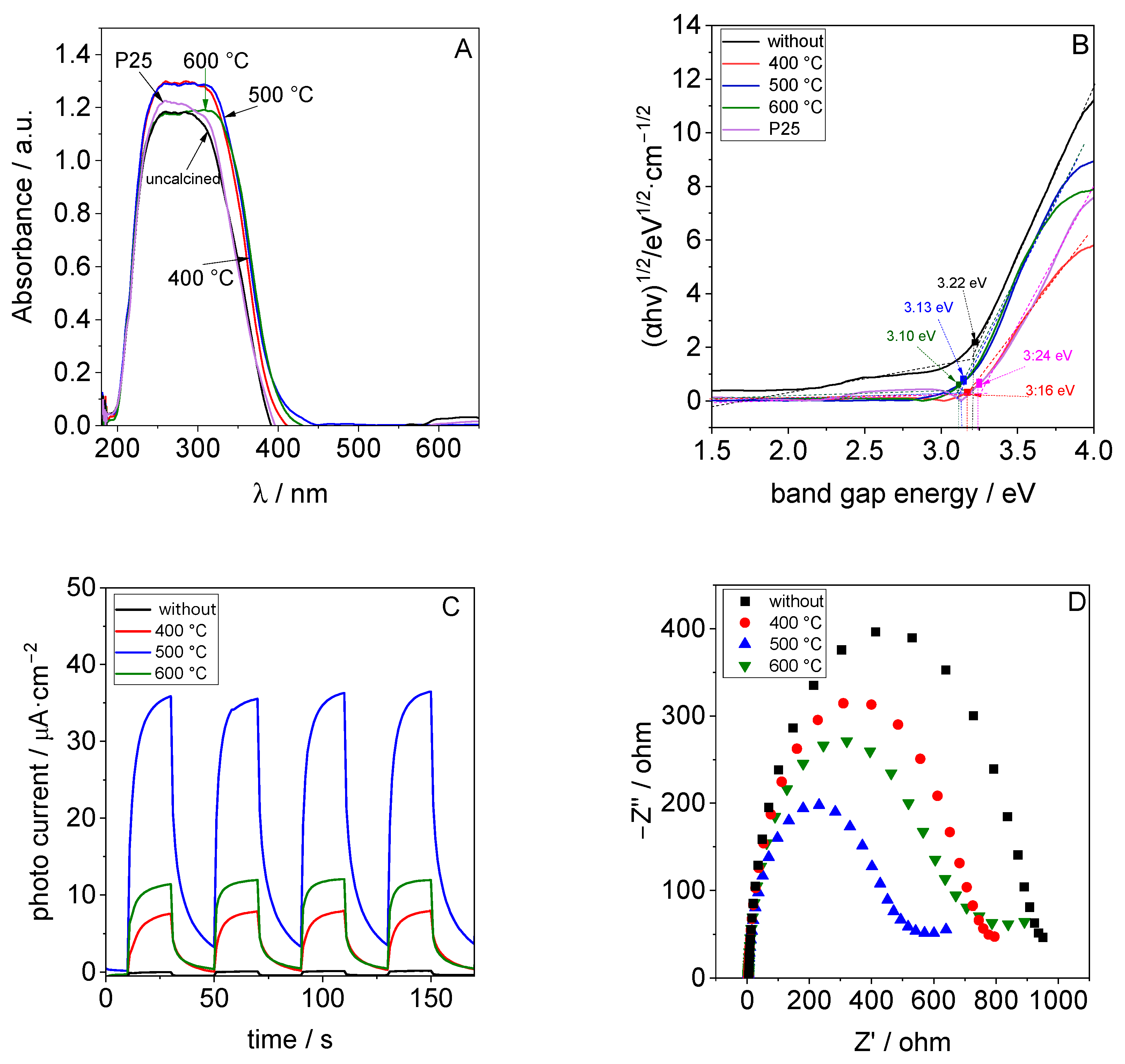
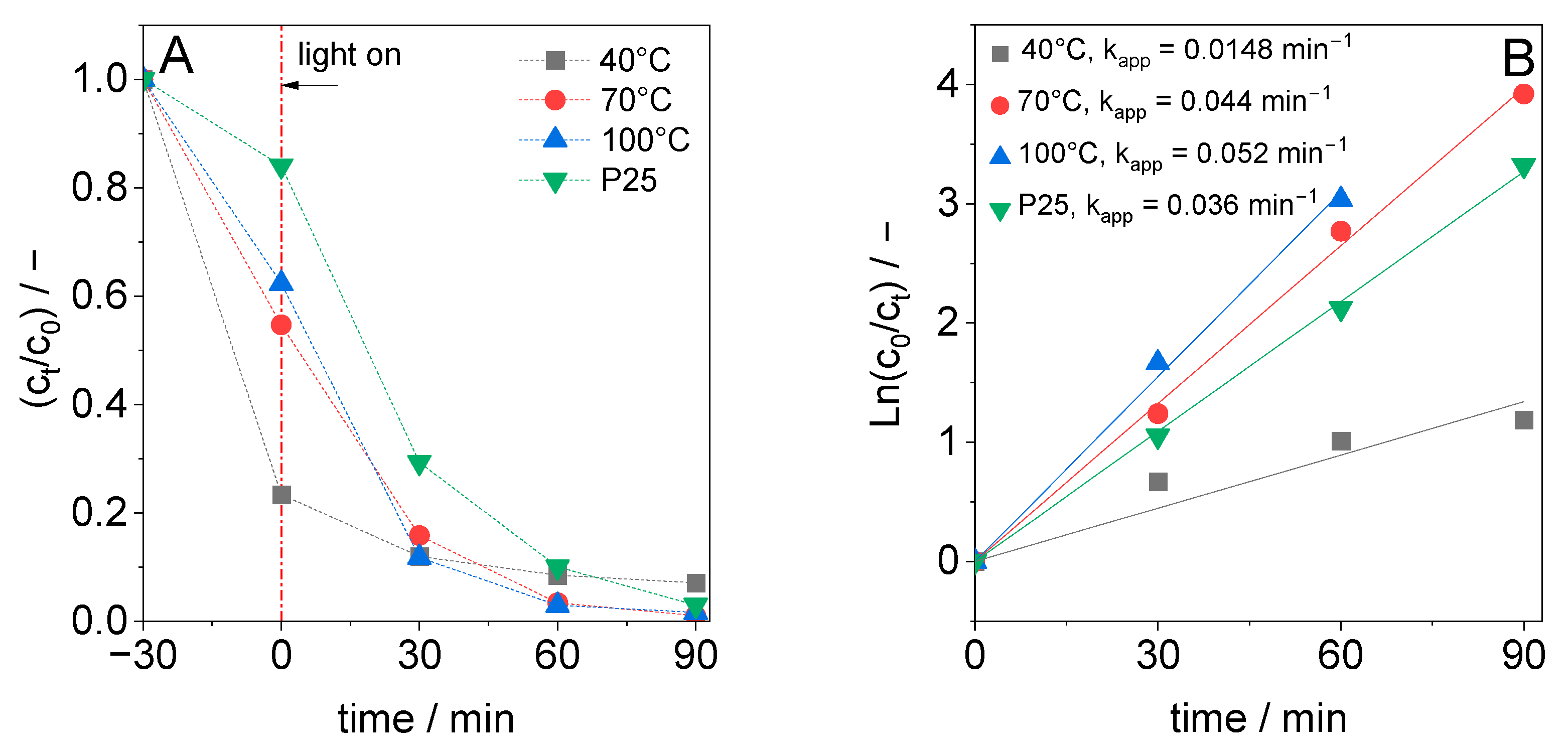



| Conditions | Synth. at 40 °C | Synth. at 70 °C | Synth. at 100 °C |
|---|---|---|---|
| Crystallite Size (nm) | Crystallite Size (nm) | Crystallite Size (nm) | |
| Uncalcined | 7 | 6 | 5 |
| Calcined at 400 °C | 8 | 8 | 7 |
| Calcined at 500 °C | 14 | 11 | 12 |
| Calcined at 600 °C | 33 | 26 | 34 |
| Samples | Tcalc °C | ABET m2 g−1 | Vp cm3 g−1 | Sp nm | C-Content wt% | Eg eV |
|---|---|---|---|---|---|---|
| TiO2 | - | 325.4 | 3.8 | 2.4 | 0.66 | 3.22 |
| TiO2 | 400 | 140.7 | 2.8 | 4.0 | 0.05 | 3.16 |
| TiO2 | 500 | 97.4 | 2.6 | 5.4 | 0.04 | 3.13 |
| TiO2 | 600 | 17.9 | 0.9 | - | 0.04 | 3.10 |
| Statistic Parameters | Kapp | TOC Removal |
|---|---|---|
| R2 | 0.954 | 0.983 |
| Adjusted R2 | 0.877 | 0.955 |
| Predicted R2 | 0.440 | 0.831 |
| Adeq Precision | 10.101 | 14.846 |
| TSynth °C | TCalcin °C | Experimental Results | Fitting Results | ||
|---|---|---|---|---|---|
| Kapp min−1 | TOC Removal % | Kapp min−1 | TOC Removal % | ||
| 40 | 400 | 0.033 | 65 | 0.028 | 61 |
| 500 | 0.027 | 74 | 0.032 | 72 | |
| 600 | 0.015 | 38 | 0.012 | 36 | |
| 70 | 400 | 0.051 | 96 | 0.050 | 93 |
| 500 | 0.053 | 97 | 0.051 | 97 | |
| 600 | 0.031 | 62 | 0.029 | 54 | |
| 100 | 400 | 0.042 | 93 | 0.041 | 89 |
| 500 | 0.049 | 97 | 0.040 | 86 | |
| 600 | 0.016 | 38 | 0.015 | 36 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Akuma, D.A.; Lund, H.; Hoa Duong, T.T.; Fufa, F.; Strunk, J.; Steinfeldt, N. Optimization of Anatase TiO2 Photocatalyst for Diclofenac Degradation by Using Response Surface Methodology. Appl. Sci. 2025, 15, 1401. https://doi.org/10.3390/app15031401
Akuma DA, Lund H, Hoa Duong TT, Fufa F, Strunk J, Steinfeldt N. Optimization of Anatase TiO2 Photocatalyst for Diclofenac Degradation by Using Response Surface Methodology. Applied Sciences. 2025; 15(3):1401. https://doi.org/10.3390/app15031401
Chicago/Turabian StyleAkuma, Desalegn Abdissa, Henrik Lund, Thi Thanh Hoa Duong, Fekadu Fufa, Jennifer Strunk, and Norbert Steinfeldt. 2025. "Optimization of Anatase TiO2 Photocatalyst for Diclofenac Degradation by Using Response Surface Methodology" Applied Sciences 15, no. 3: 1401. https://doi.org/10.3390/app15031401
APA StyleAkuma, D. A., Lund, H., Hoa Duong, T. T., Fufa, F., Strunk, J., & Steinfeldt, N. (2025). Optimization of Anatase TiO2 Photocatalyst for Diclofenac Degradation by Using Response Surface Methodology. Applied Sciences, 15(3), 1401. https://doi.org/10.3390/app15031401






