Analysis of Tunnel Lining Damage Characteristics Under the Combined Actions of Fault Dislocation and Seismic Action
Abstract
1. Introduction
2. Numerical Modeling
2.1. Model Introduction
2.2. Materials and Parameters
2.3. Ground Motion Processing
2.4. Analysis Procedure
3. Results and Discussion
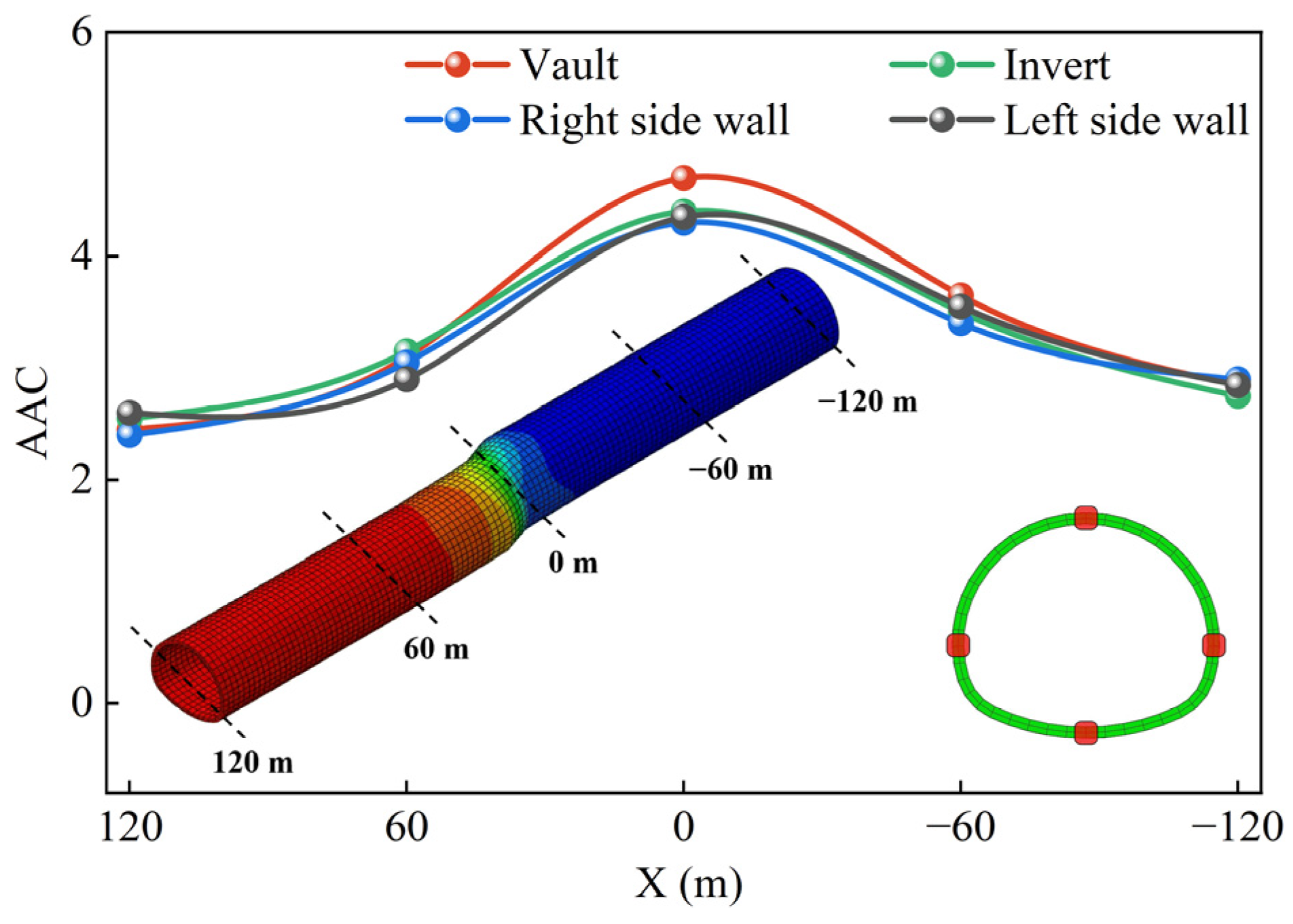
3.1. Acceleration Response
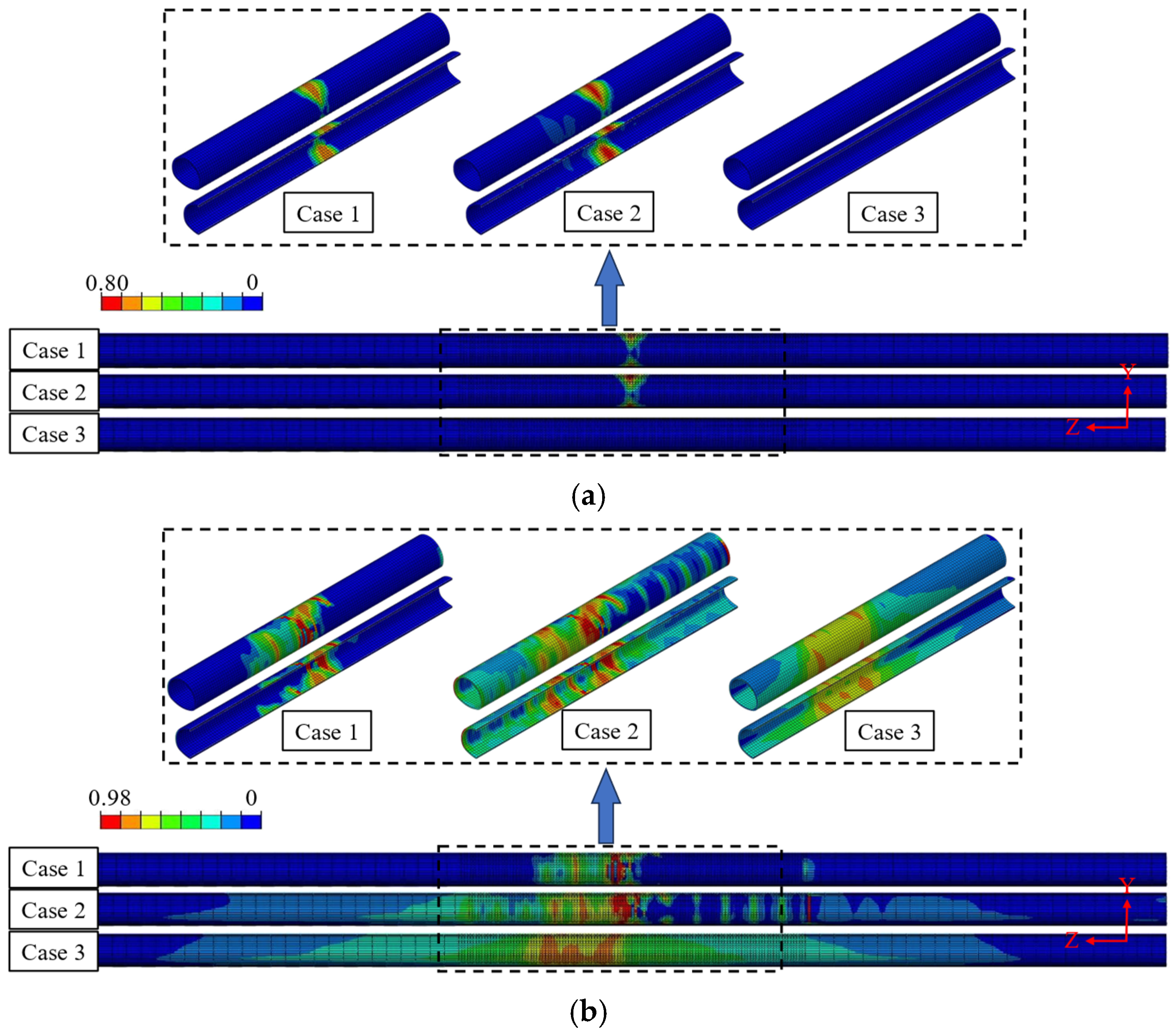
3.2. Description of the Damage
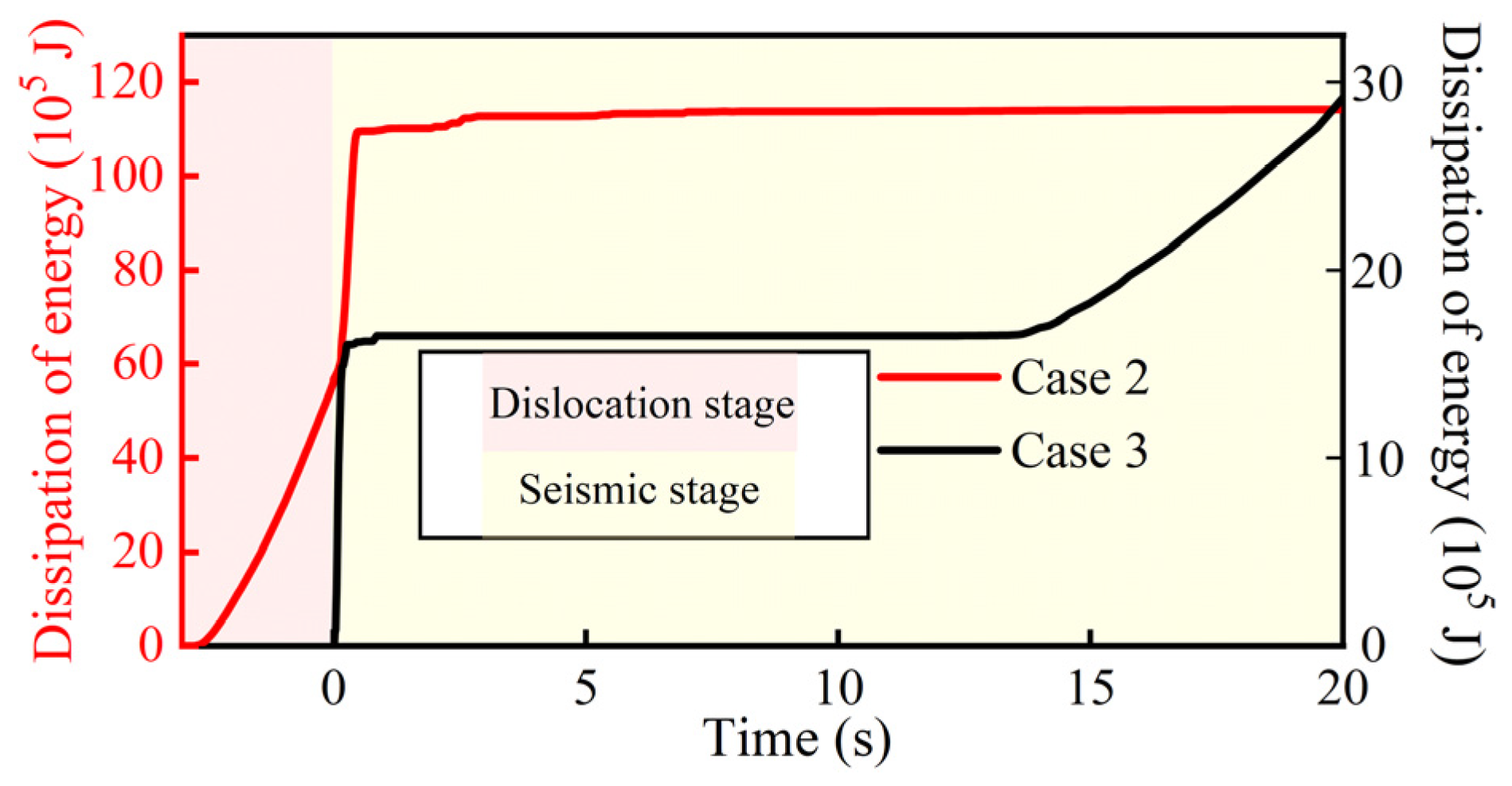
3.3. Description of Energy Dissipation
3.4. Damage Assessment of the Tunnel Lining
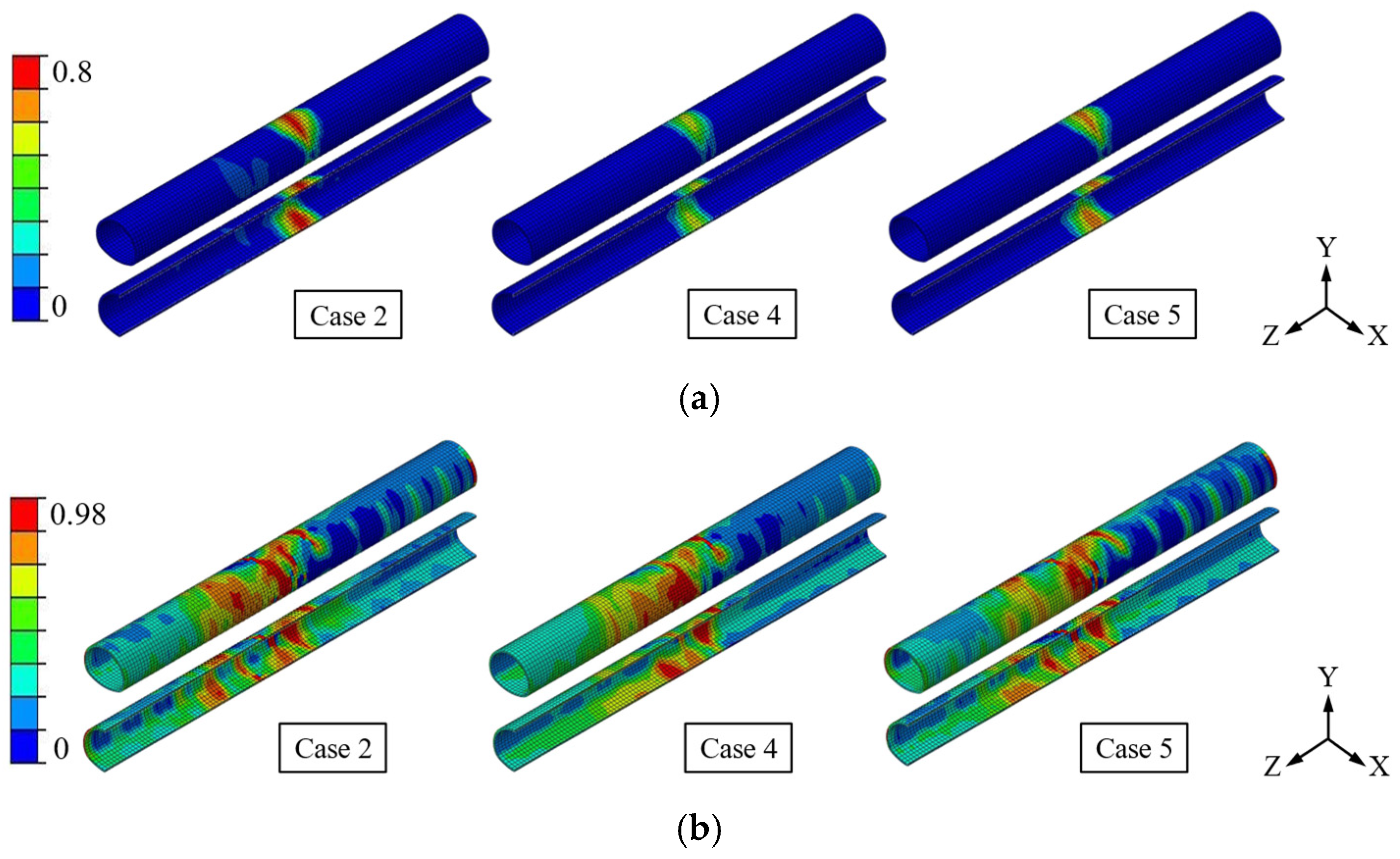
3.5. Lining Damage Under Different Initial Fault Displacements
3.6. Lining Damage at Different Peak Seismic Accelerations
4. Conclusions
- (1)
- The peak acceleration observed at monitoring points throughout the tunnel demonstrates an amplification effect due to the combined impacts of fault dislocation and an earthquake. This effect is particularly pronounced at the tunnel vault located at the fault plane, with an acceleration amplification coefficient of 4.7.
- (2)
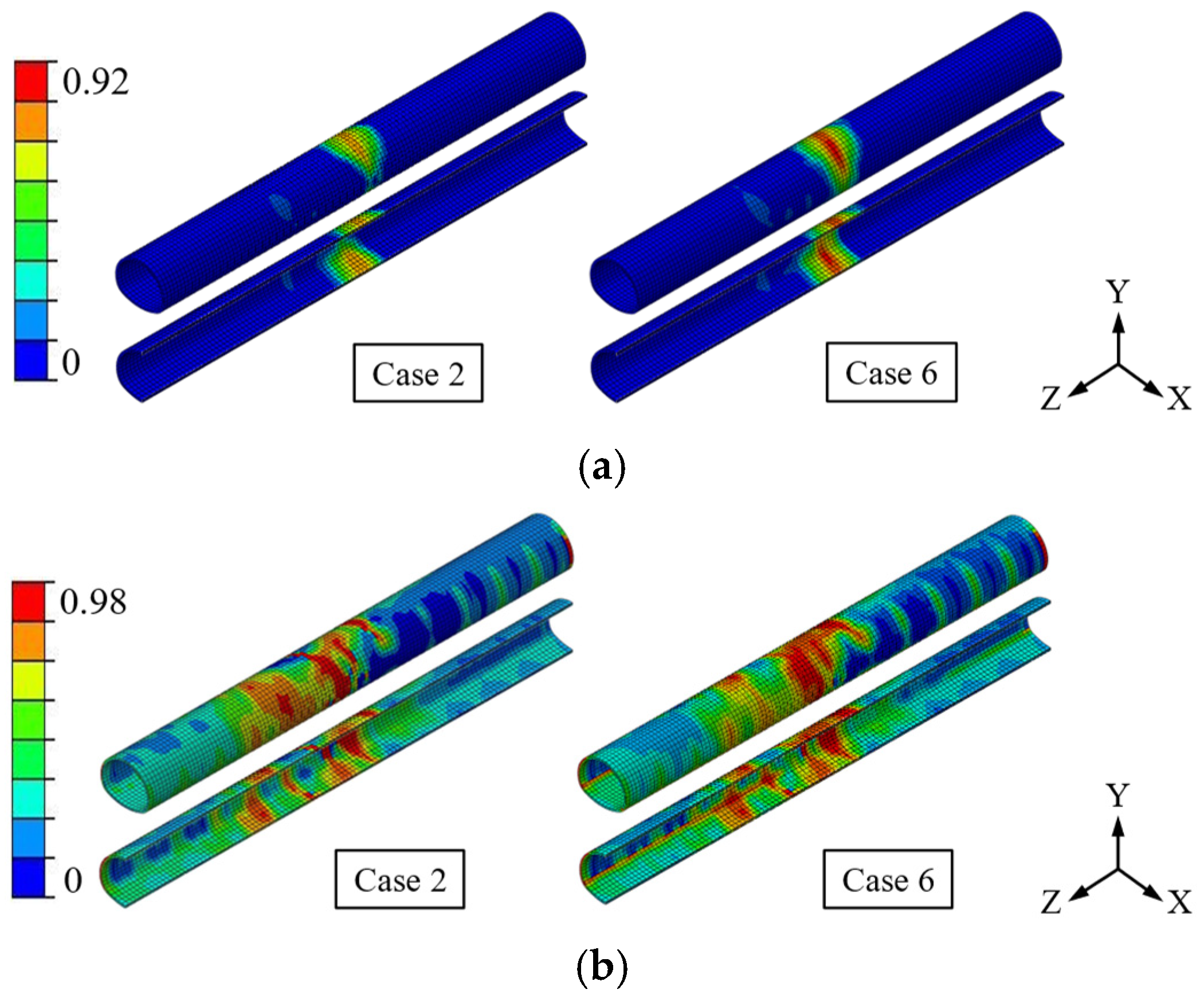
- The energy dissipation of the tunnel lining in Case 3 is about 3000 kJ; in Case 2, the energy dissipation of the tunnel lining in the seismic phase is about 5500 kJ, which is an increase of 85% compared with that in Case 3. This shows that the damage to the tunnel caused by the combined effects of fault dislocation and seismicity is not a simple superposition, and the initial damage caused by fault dislocation will aggravate the damage of the tunnel during the seismic action stage.
- (3)
- The lining damage is mainly concentrated in the fault fracture zone area under fault dislocation, and after superimposed seismic action, the scope and degree of lining damage increase, and the increase in tensile damage is more significant compared with compression damage, indicating that seismic action is the main cause of tensile damage in the tunnel.
- (4)
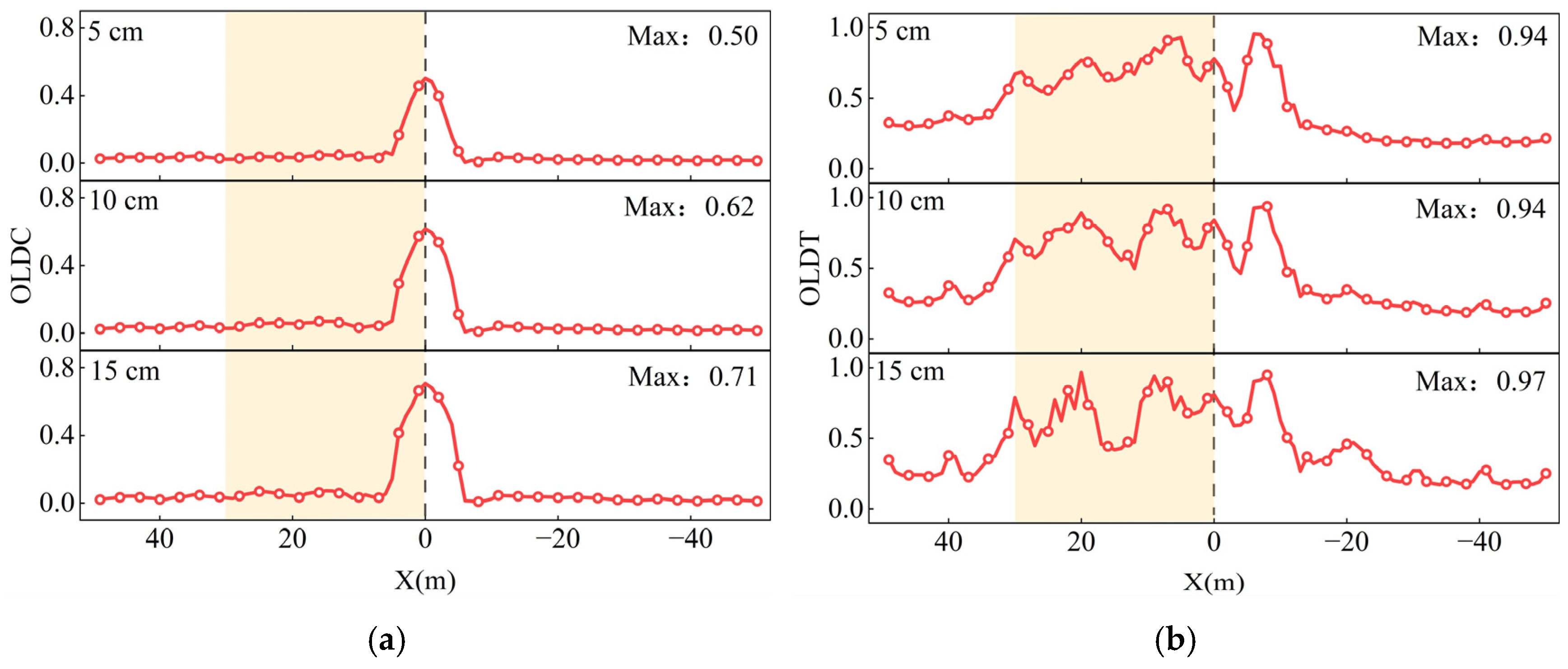
- Both the initial fault dislocation and an increase in seismic intensity elevate the damage level of the tunnel. The OLDC index is symmetrically distributed along the fault plane in an inverted V-shape, with damage primarily concentrated within 14 m on either side of the fault plane. Notably, the compressive damage to the lining intensifies under stronger seismic effects. The OLDT index follows a similar distribution pattern, with complete damage (DS4) concentrated within −20 m to 30 m on both sides of the fault plane.
- (5)
- Due to the limitations of the research methodology, this study only analyzes the tunnel damage characteristics under different fault initial displacements and various peak seismic accelerations. In future work, we will conduct a sensitivity analysis of key parameters under more conditions. Additionally, due to the lack of seismic damage records under fault dislocation-coupled seismic loading, model tests are needed to validate the theoretical results.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Yu, H.; Chen, J.; Bobet, A.; Yuan, Y. Damage Observation and Assessment of the Longxi Tunnel During the Wenchuan Earthquake. Tunn. Undergr. Space Technol. 2016, 54, 102–116. [Google Scholar] [CrossRef]
- Dimitrios, L.; Bouckovalas, G.; Papadimitriou, A. Analysis of Fault Rupture Propagation through Uniform Soil Cover. Soil Dyn. Earthq. Eng. 2009, 29, 1389–1404. [Google Scholar] [CrossRef]
- FHWA (Federal Highway Administration). Technical Manual for Design and Construction of Road Tunnels-Civil Elements; Publication No. FHWA-NHI-10-034; Department of Transportation, Federal Highway Administration: Washington, DC, USA, 2019.
- ISO 23469:2005; Bases for Design of Structures—Seismic Actions for Designing Geotechnical Works. International Organization for Standardization: Geneva, Switzerland, 2005.
- ITA (International Tunneling and Underground Space Association). Tunnel Market Survey. 2016. Available online: https://www.tunnel-online.info/en/artikel/tunnel_Tunnel_Market_Survey_2016_3051818.html (accessed on 8 December 2019).
- Bao, L.; Wei, F. Study on the Response of Tunnel Lining under Fault Dislocation. Sustainability 2023, 15, 5150. [Google Scholar] [CrossRef]
- Ahmadi, M.; Moosavi, M.; Jafari, M.K. Experimental investigation of reverse fault rupture propagation through cohesive granular soils. Geomech. Energy Environ. 2018, 14, 61–65. [Google Scholar] [CrossRef]
- Yang, H.; Wang, M.; Luo, X.; Yu, L.; Zhang, X.; Tang, L. Longitudinal and cross-sectional partitioned failure mechanism of tunnels subjected to stick-slip action of strike-slip faults. J. Cent. South Univ. 2024, 31, 250–271. [Google Scholar] [CrossRef]
- Zhu, H.; Yu, H.; Han, F.; Wei, Y.; Yuan, Y. Seismic Resilience Design Principles and Key Issues for Tunnels Crossing Active Faults. Chin. J. Highw. Transp. 2023, 36, 193–204. [Google Scholar]
- Sabagh, M.; Ghalandarzadeh, A. Numerical modelings of continuous shallow tunnels subject to reverse faulting and its verification through a centrifuge. Comput. Geotech. 2020, 128, 103813. [Google Scholar] [CrossRef]
- Yao, C.; Zhang, Y.; He, C.; Kang, X.; Yang, W.; Geng, P.; Wang, T. An anti-rotation shelf to mitigate the rotation of shield tunnels subjected to normal faulting. Comput. Geotech. 2023, 156, 105297. [Google Scholar] [CrossRef]
- Liu, X.; Lin, L. Research on model experiment of effect of thrust fault with 75° dip angle stick-slip dislocation on highway tunnel. Chin. J. Rock Mech. Eng. 2011, 30, 2523–2530. [Google Scholar]
- Liu, X.; Wang, X.; Lin, L. Model experiment on effect of normal fault with 75° dip angle stick-slip dislocation on highway tunnel. Chin. J. Rock Mech. Eng. 2013, 32, 1714. [Google Scholar]
- Liu, X.; Wang, X.; Lin, L. Model experimental study on influence of normal fault with 60° dip angle stick-slip dislocation on mountain tunnel. Chin. Civil Eng. J. 2014, 47, 121–128. [Google Scholar] [CrossRef]
- Tang, J.; He, M.; Bian, H.; Qiao, Y. A novel numerical framework to simulate mechanical response and damage characteristics of mountain tunnels under faulting. Eng. Fail. Anal. 2024, 158, 108030. [Google Scholar] [CrossRef]
- Yuan, R.; Qiu, Y.; Cheng, Y. A Framework of Probabilistic Seismic Risk Analysis of Urban Shallow-Buried Tunnels Subjected to Active Fault Dislocations. J. Earthq. Eng. 2023, 28, 1312–1330. [Google Scholar] [CrossRef]
- Baziar, M.H.; Nabizadeh, A.; Lee, J.; Huang, W. Centrifuge modeling of interaction between reverse faulting and tunnel. Soil Dyn. Earthq. Eng. 2014, 65, 151–164. [Google Scholar] [CrossRef]
- Cui, G.; Ji, L.; Jing, H. Damping Shake Technology of the Shock Absorption Layer of Fault-Crossing Tunnels in a Dangerous Mountainous Area with High-Intensity Earthquakes. Chin. Earthq. Eng. J. 2019, 44, 226–291. [Google Scholar]
- Geng, P.; Wu, C.; Tang, J.; Li, L. Analysis of Dynamic Response Properties for Tunnel Through Fault Fracture Zone. Chin. J. Rock Mech. Eng. 2012, 31, 1406–1413. [Google Scholar]
- Zang, X.; Shen, Y.; Zhou, P.; Qiu, J. Partitions of Seismic Affected Zone and Characteristics of Segmental Lining Based on Energy Principle. J. Cen. South Univ. 2023, 30, 227–242. [Google Scholar] [CrossRef]
- Yu, H.; Zhang, Z.; Chen, J.; Bobet, A.; Zhao, M.; Yuan, Y. Analytical solution for longitudinal seismic response of tunnel liners with sharp stiffness transition. Tunn. Undergr. Space Technol. 2018, 77, 103–144. [Google Scholar] [CrossRef]
- Zhang, Y.; Yuan, K.; Zhao, W.; Fan, J. Study on structural deformation characteristics and surface crack distribution of girder tunnel across Lenglongling fault caused by Menyuan earthquake. Chin. J. Rock Mech. Eng. 2023, 42, 1055–1069. [Google Scholar]
- Zhao, Y.; Guo, E.; Liu, Z.; Gao, L. Damage analysis of urban metro tunnel under strike-slip fault. Rock Soil Mech. 2014, 35, 467–473. [Google Scholar]
- GB/T50218-2014; Standard for Engineering Classification of Rock Masses. China Planning Press: Beijing, China, 2014.
- Du, J.; Yan, S.; Sun, W.; Cao, M.; Li, Y.; Yang, K. Study on Damage Evolution of Tunnel Lining under Strike-Slip Fault Movements. Geofluids 2023, 2023, 7106729. [Google Scholar] [CrossRef]
- Hou, R. ABAQUS Development of Free Field Boundary based on ABAQUS and Its Application of Dynamic Analysis on Tunnel Structure. Ph.D. Thesis, Southwest Jiaotong University, Chengdu, China, 2014. [Google Scholar]
- GB 50010-2010; Design Code for Concrete Structures. China Architecture & Building Press: Beijing, China, 2015.
- Zhuang, H.; Hu, Z.; Wang, X.; Chen, G. Seismic responses of a large underground structure in liquefied soils by FEM numerical modelling. Bull. Eng. Geol. Environ. 2015, 13, 3645–3668. [Google Scholar] [CrossRef]
- Vazouras, P.; Karamanos, S.A. Structural behavior of buried pipe bends and their effect on pipeline response in fault crossing areas. Bull. Earthq. Eng. 2015, 15, 4999–5024. [Google Scholar] [CrossRef]
- Demirci, H.E.; Bhattacharya, S.; Karamitros, D.; Alexander, N. Experimental and numerical modelling of buried pipelines crossing reverse faults. Soil Dyn. Earthq. Eng. 2018, 114, 198–214. [Google Scholar] [CrossRef]
- GB 50011-2010; Code for Seismic Design of Buildings. Standards Press of China: Beijing, China, 2010.
- Xie, H.; Ju, Y.; Li, L. Criteria for Strength and Structural Failure of Rocks Based on Energy Dissipation and Energy Release Principles. Chin. J. Rock Mech. Eng. 2005, 24, 3003–3010. [Google Scholar]
- Qiao, Y.; Tang, J.; Liu, G.; He, M. Longitudinal mechanical response of tunnels under active normal faulting. Undergr. Space 2022, 7, 662–679. [Google Scholar] [CrossRef]
- Shen, Y.; Gao, B.; Yang, X.; Tao, S. Seismic damage mechanism and dynamic deformation characteristic analysis of mountain tunnel after Wenchuan earthquake. Eng. Geol. 2014, 180, 85–98. [Google Scholar] [CrossRef]
- Liu, G.; Xiao, M.; Chen, J. Seismic performance assessment of tunnel structure based on incremental dynamic analysis. Adv. Eng. Sci. 2019, 51, 92–100. [Google Scholar] [CrossRef]
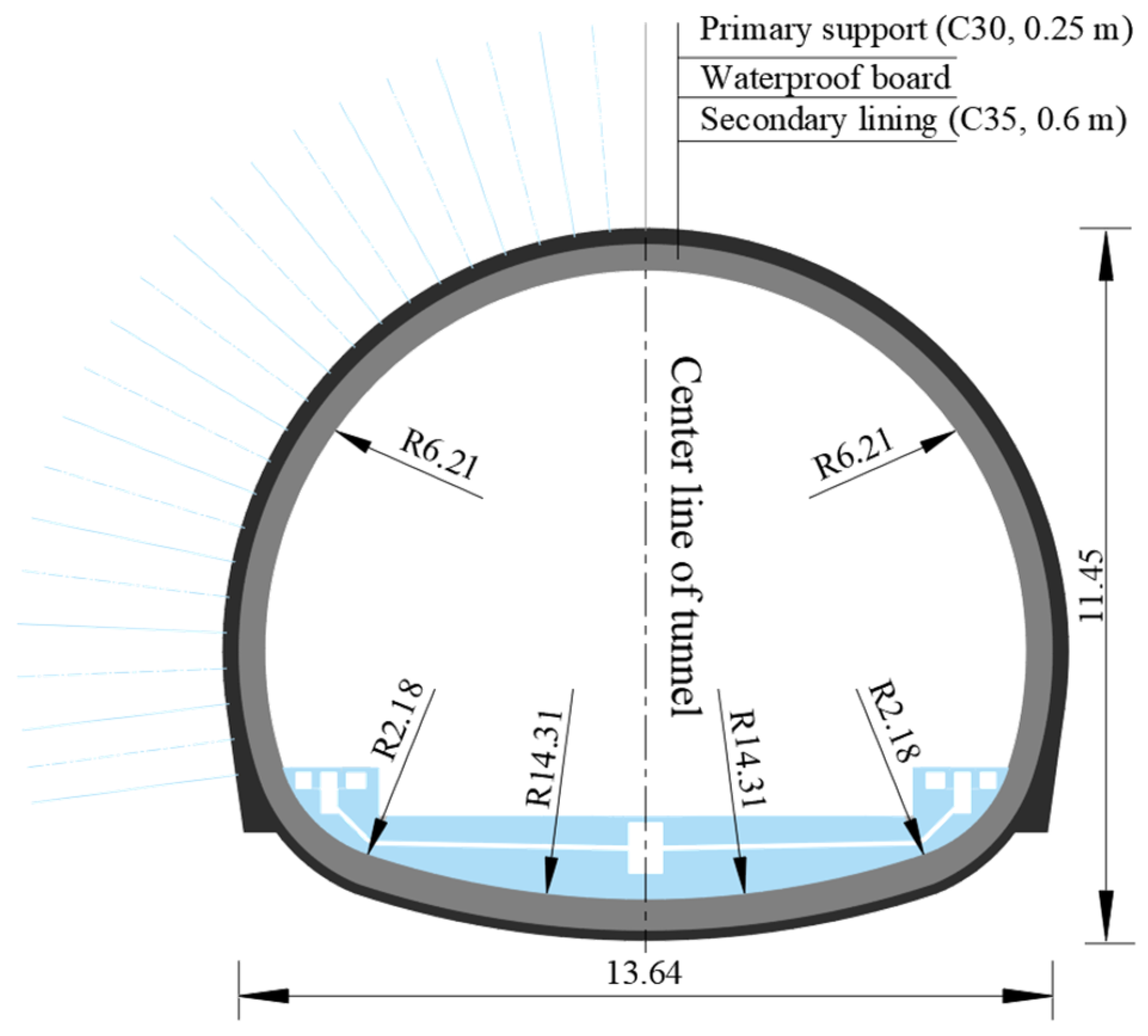

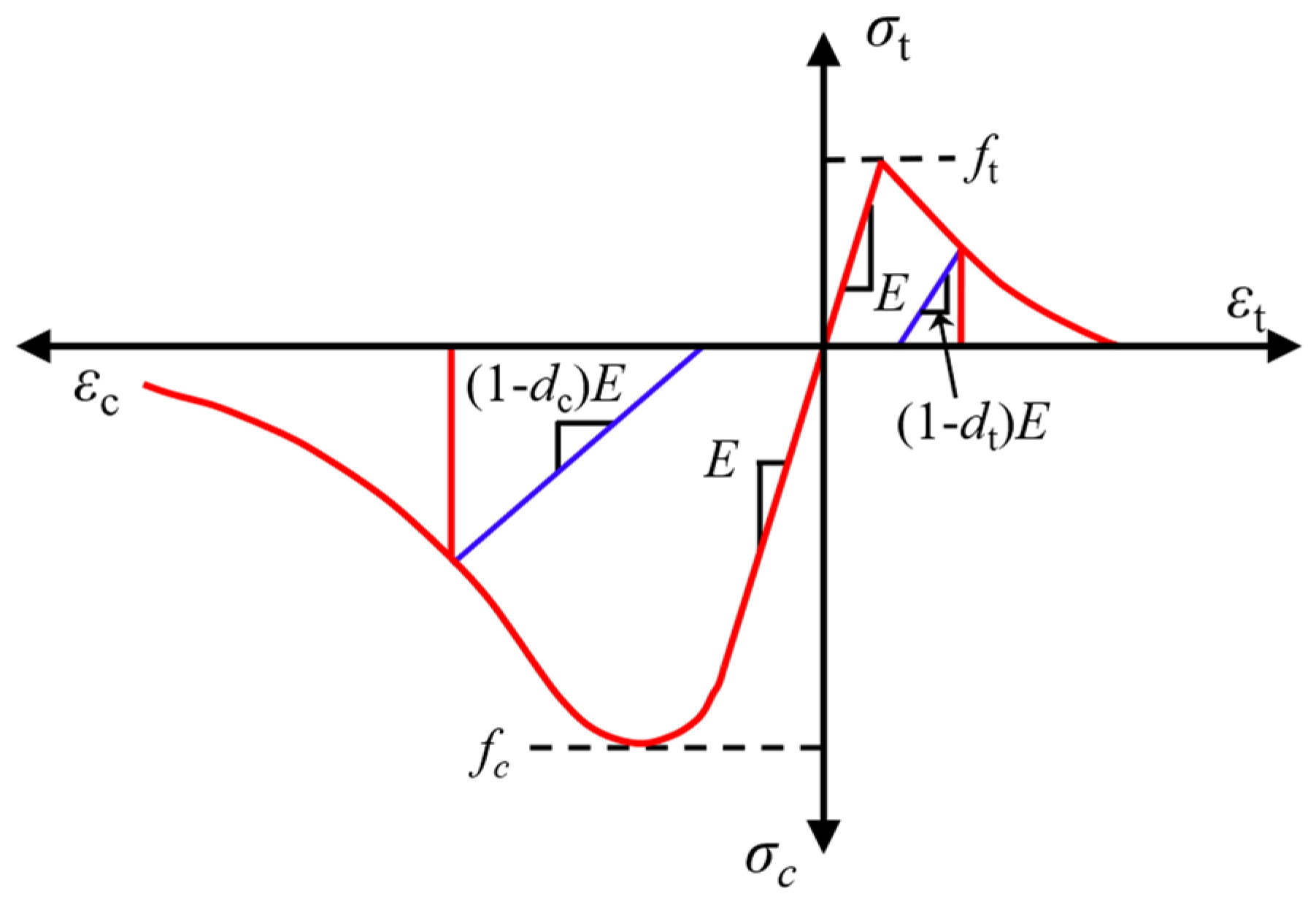

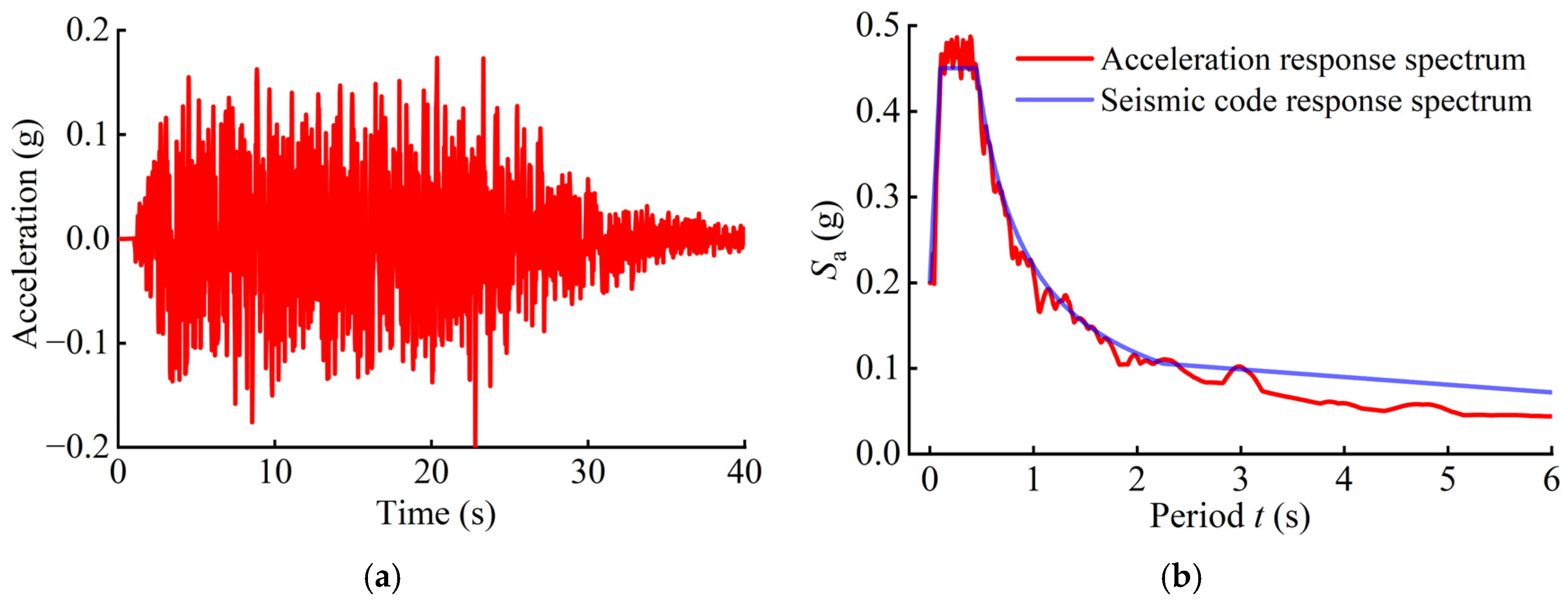

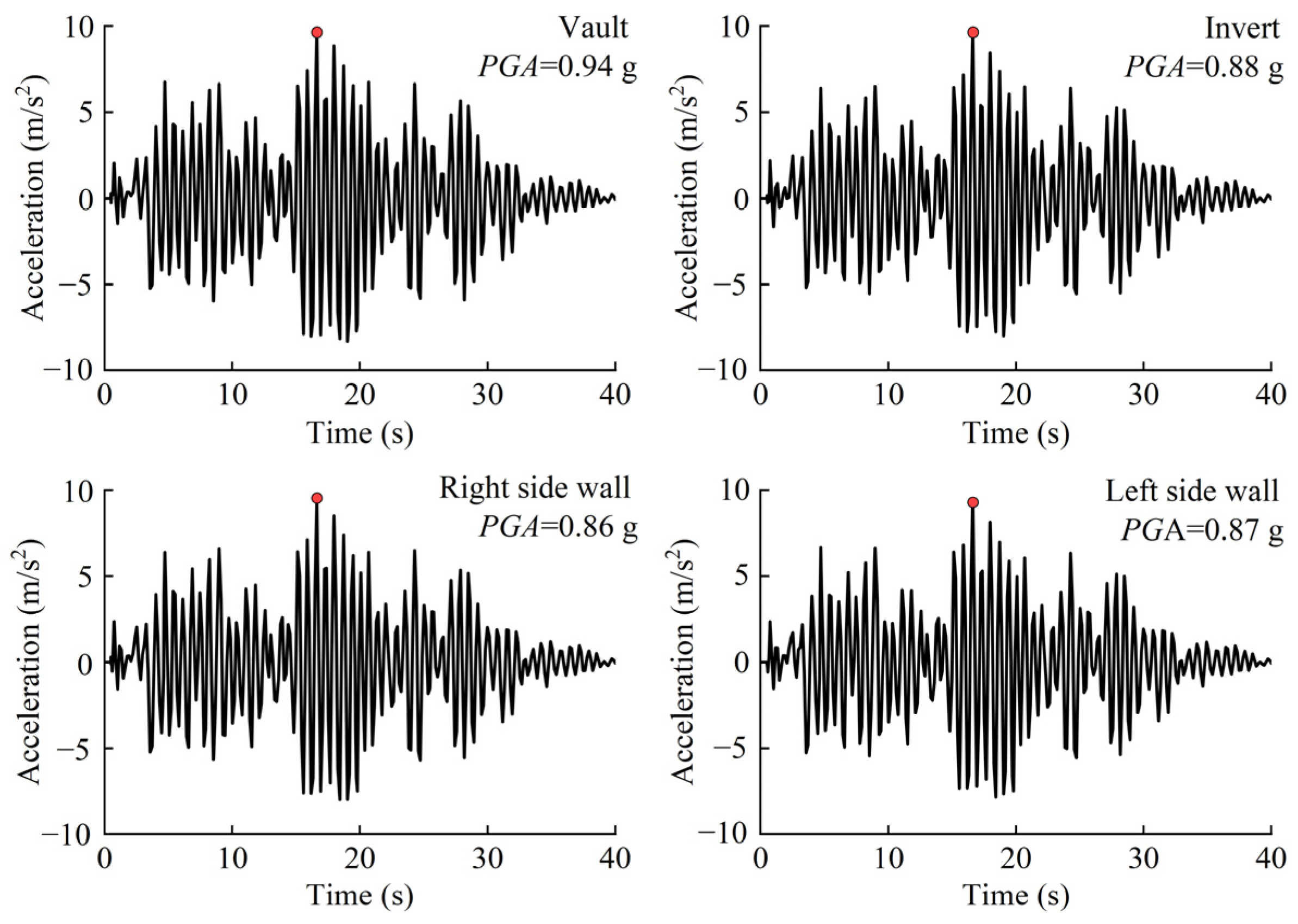


| Name | Density/(kg/m3) | Elastic Modulus/GPa | Poisson Ratio | Internal Friction Angle/(°) | Cohesion/(MPa) |
|---|---|---|---|---|---|
| The complete rock mass | 2100 | 3.0 | 0.33 | 31 | 0.40 |
| Fault fracture zone | 1700 | 1.1 | 0.41 | 21 | 0.07 |
| Name | Density/(kg/m3) | Elastic Modulus/MPa | Poisson Ratio | Compressive Yield Stress/MPa | Tensile Yield Stress/MPa |
|---|---|---|---|---|---|
| Primary support | 2400 | 30,000 | 0.2 | 20.1 | 2.01 |
| Secondary lining | 2500 | 31,500 | 0.2 | 23.4 | 2.20 |
| Location | 120 | 60 | 0 | −60 | −120 | |
|---|---|---|---|---|---|---|
| Name | ||||||
| Vault | 0.49 | 0.62 | 0.94 | 0.73 | 0.57 | |
| Invert | 0.51 | 0.63 | 0.88 | 0.7 | 0.55 | |
| Right side wall | 0.48 | 0.61 | 0.86 | 0.68 | 0.58 | |
| Left side wall | 0.52 | 0.58 | 0.87 | 0.71 | 0.57 | |
| Damage Level | Description of Damage Characteristics | Overall Lining Damage Index |
|---|---|---|
| DS1 | No damage or minor cracks (no repair required). | OLDC, OLDT < 0.3 |
| DS2 | Visible cracking in the lining (surface repair). | 0.3 < OLDC, OLDT < 0.6 |
| DS3 | Cracks are widely distributed in the tunnel lining, and some linings are crushed and spalling (need to suspend operations). | 0.6 < OLDC, OLDT < 0.8 |
| DS4 | Significant and numerous cracks in the tunnel lining; the lining is sheared off; vault collapse (loss of the operational function of the tunnel). | 0.8 < OLDC, OLDT |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Du, J.; Yan, S.; Sun, W.; Li, Y.; Cao, M. Analysis of Tunnel Lining Damage Characteristics Under the Combined Actions of Fault Dislocation and Seismic Action. Appl. Sci. 2025, 15, 1150. https://doi.org/10.3390/app15031150
Du J, Yan S, Sun W, Li Y, Cao M. Analysis of Tunnel Lining Damage Characteristics Under the Combined Actions of Fault Dislocation and Seismic Action. Applied Sciences. 2025; 15(3):1150. https://doi.org/10.3390/app15031150
Chicago/Turabian StyleDu, Jiaxuan, Songhong Yan, Weiyu Sun, Yuxiang Li, and Mingxing Cao. 2025. "Analysis of Tunnel Lining Damage Characteristics Under the Combined Actions of Fault Dislocation and Seismic Action" Applied Sciences 15, no. 3: 1150. https://doi.org/10.3390/app15031150
APA StyleDu, J., Yan, S., Sun, W., Li, Y., & Cao, M. (2025). Analysis of Tunnel Lining Damage Characteristics Under the Combined Actions of Fault Dislocation and Seismic Action. Applied Sciences, 15(3), 1150. https://doi.org/10.3390/app15031150





