Simulation Research on the Effects of Air Gaps and Ambient Temperature on a 27.5 kV Power Cable
Abstract
1. Introduction
2. Cable Model Construction
2.1. Model Structure and Geometry
2.2. Boundary Conditions and Formulas
3. Cable Thermal Field Research
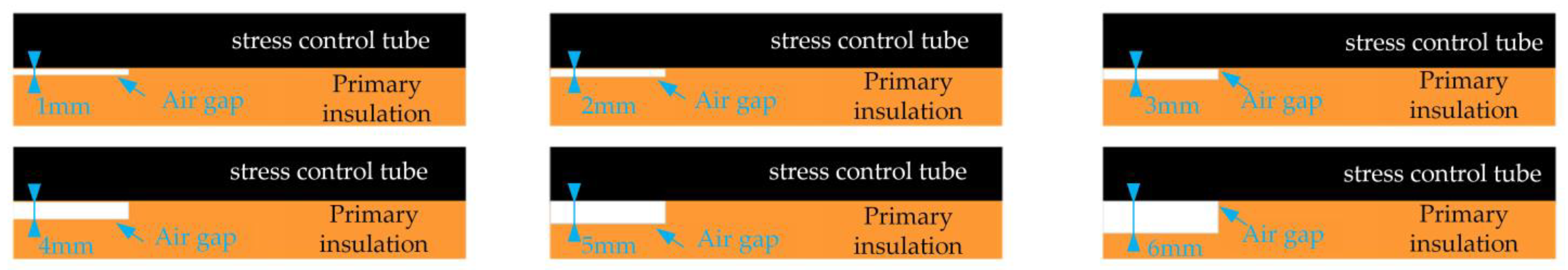
4. Cable Electrical Field Research
5. Conclusions
- (1)
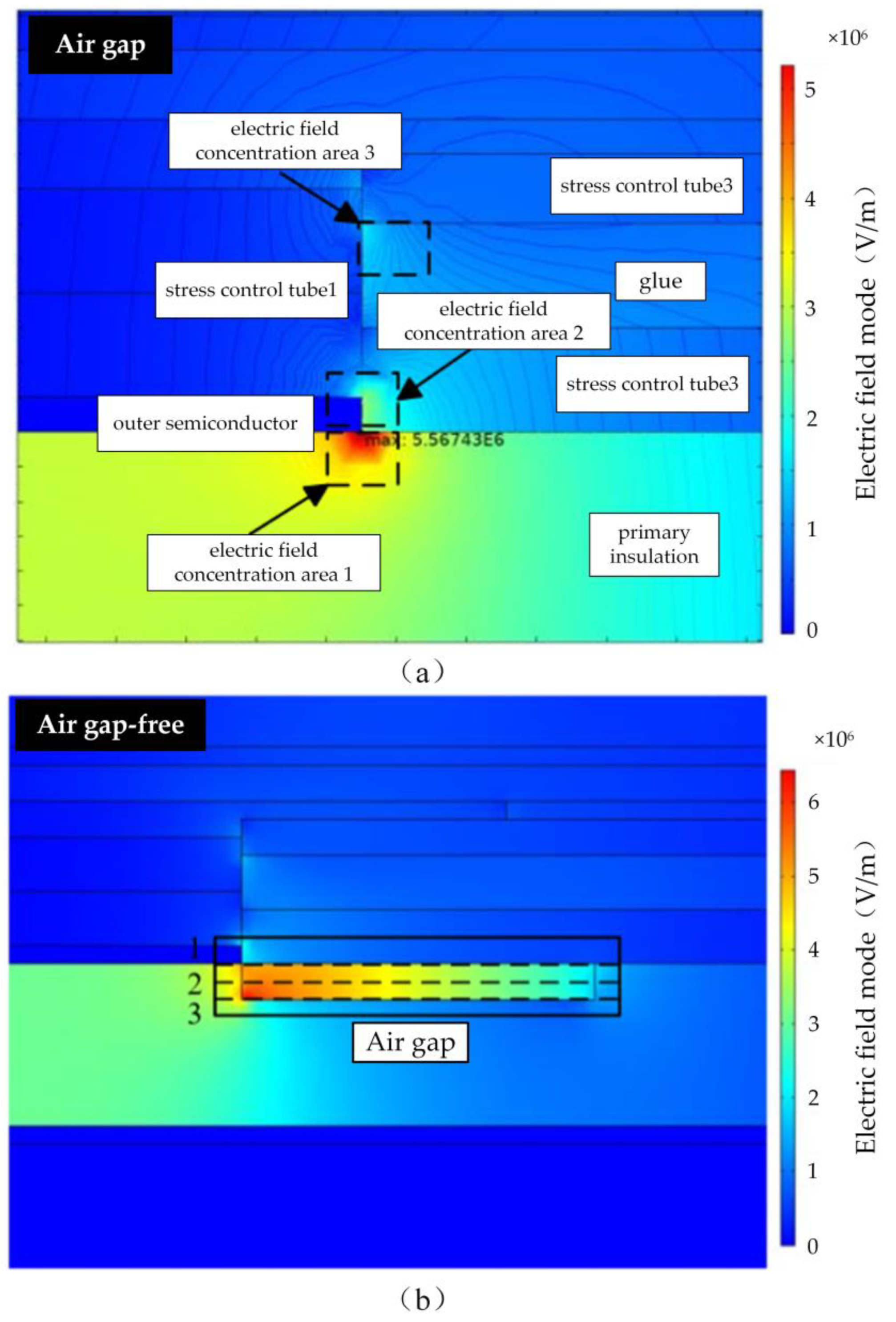
- The temperature distribution of the cable under different conditions was explored. It was found that the temperature difference at the junction of the outer semiconductor, insulating layer and stress control tube of the cable is the largest, and the distribution at the semiconductor layer and stress control tube is the most uneven. which is morelikely to produce an air gap to aggravate the structural stratification, resulting in cable degradation.
- (2)
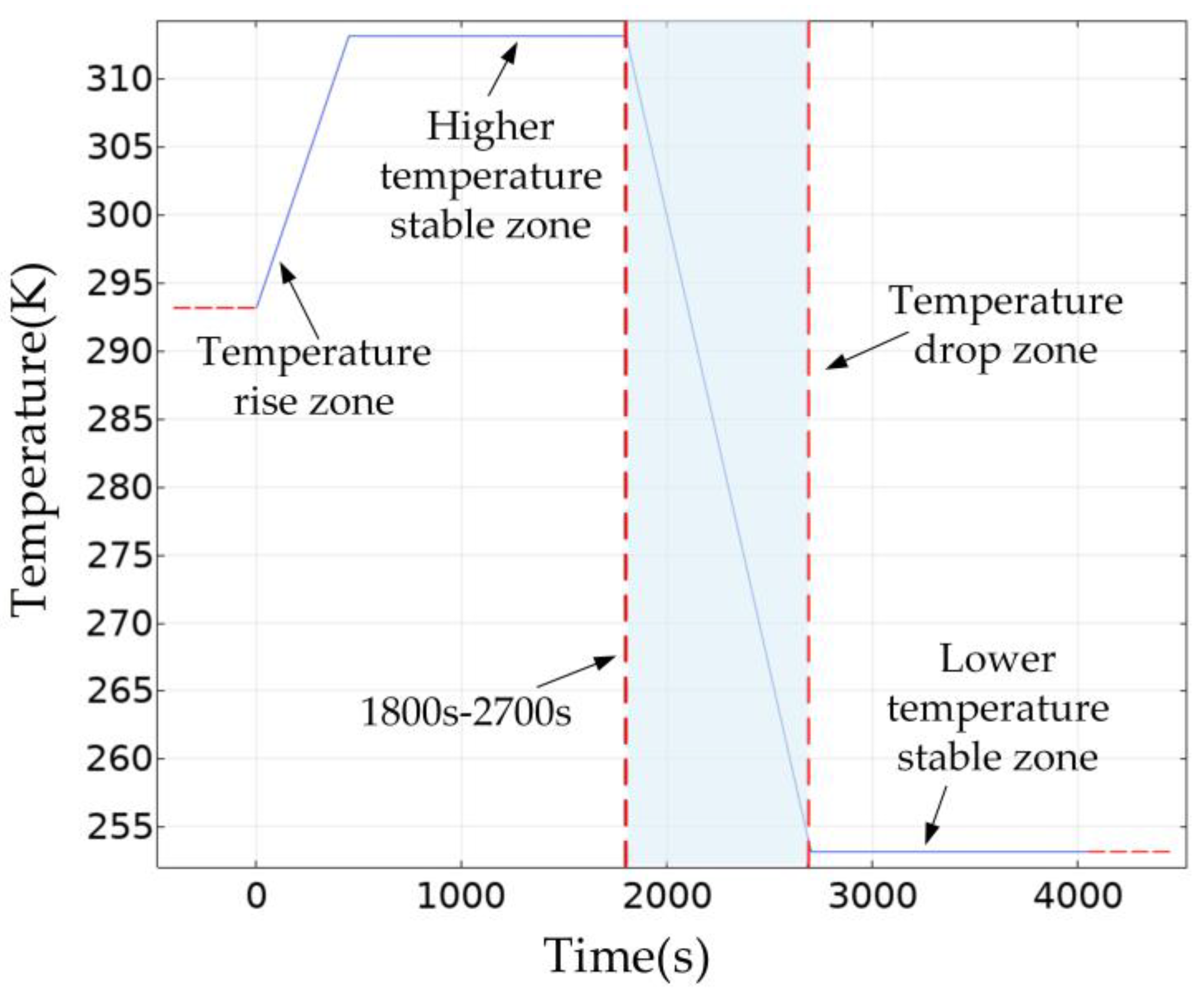
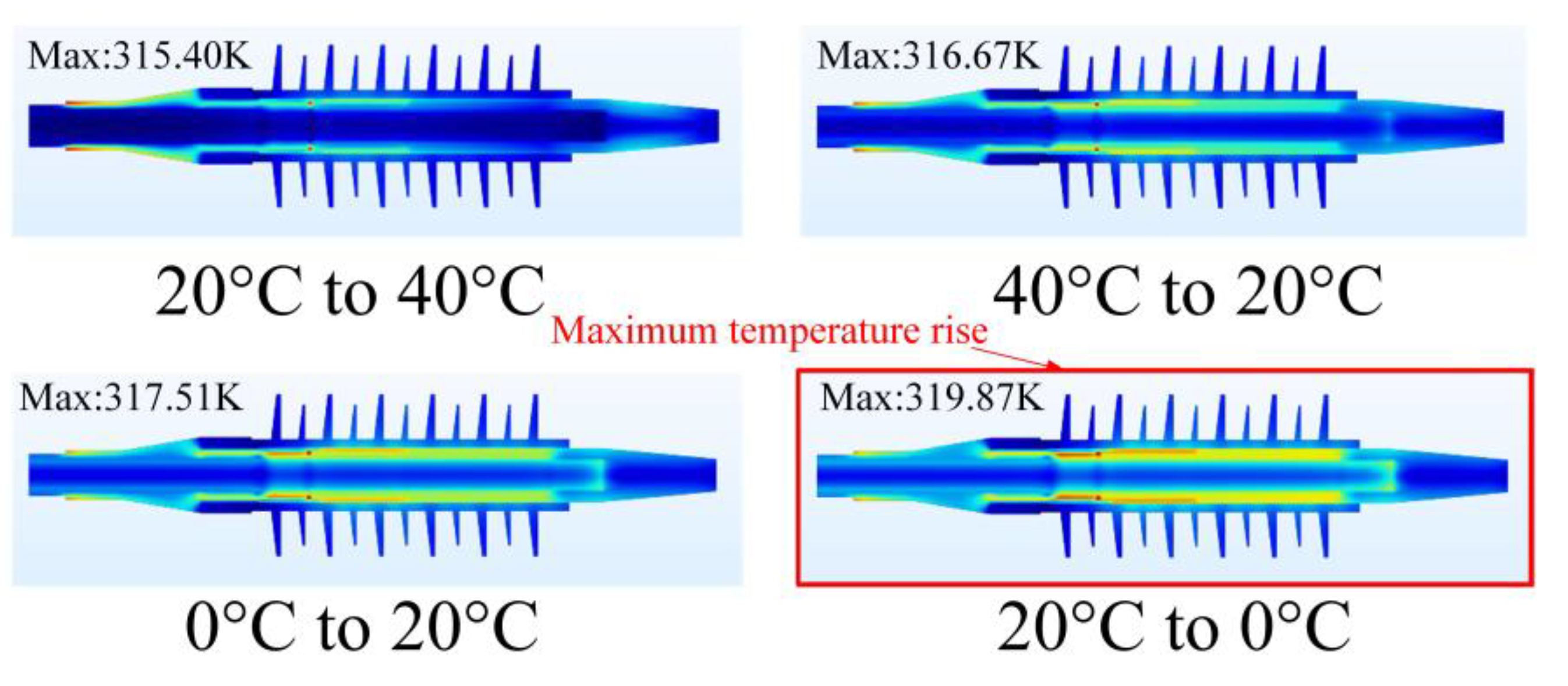
- The influence of different temperature differences on the temperature of a cable air gap was studied. It was found that a decrease in the ambient temperature has a greater influence on the temperature in the air gap than an increase in the ambient temperature. When the ambient temperature decreased from 20° to 0°, the air gap temperature was the highest, reaching 319.87 K.
- (3)
- The electrothermal field distribution law of cable air gaps with different thicknesses was found. When air gap thickness is smaller, the electric field strength in the air gap is higher, and partial discharge is more likely to occur, resulting in a greater cold and heat alternating effect and a further increase in the air gap.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| Abbreviation/Symbol | Significance |
| PE | Polyethylene |
| PVC | Polyvinyl chloride |
| XLPE | Cross-linked Polyethylene |
| EPDM | Ethylene Propylene Diene Monomer |
| Time | |
| / | Temperature, in K |
| Density of the material at a temperature of | |
| Specific heat capacity of the material at constant pressure | |
| Thermal conductivity of the material at temperature of | |
| Joule heat source | |
| Current density of contact | |
| Electric field inside contact | |
| Conductivity of contact material at the temperature of | |
| Electric potential. When solving electric field control equation |
References
- Liu, C.; Yang, S.; Cui, Y.; Chu, S.; Xiong, Q. An improved quantitative assessment method on hazardous interference of power lines to the signal cable in high-speed railway. IET Electr. Syst. Transp. 2022, 12, 65–78. [Google Scholar] [CrossRef]
- Zhou, J.; Qiu, G. China’s high-speed rail network construction and planning over time: A network analysis. J. Transp. Geogr. 2018, 70, 40–54. [Google Scholar]
- Hao, Y.; Chen, Y.; Chen, Y.; Liu, Y.; Cheng, Y.; Yang, L.; Xiao, J. Partial discharge detection using the fiber-optic Mach-Zehnder interferometer system for XLPE cables. Electr. Eng. 2022, 104, 2133–2140. [Google Scholar] [CrossRef]
- Guo, L.; Cao, W.; Bai, L.; Zhang, J.; Xing, L.; Xiang, E.; Zhou, L. Fault diagnosis based on multiscale texture features of cable terminal on EMU of high-speed railway. IEEE Trans. Instrum. Meas. 2021, 70, 3502612. [Google Scholar] [CrossRef]
- Dai, G.; Su, M.; Yan, B. Case study of twin cable-stayed bridges for high-speed railway in China: Design, analysis and construction. Struct. Eng. Int. 2014, 24, 396–401. [Google Scholar] [CrossRef]
- Qin, S.; Gao, Z. Developments and prospects of long-span high-speed railway bridge technologies in China. Engineering 2017, 3, 787–794. [Google Scholar] [CrossRef]
- Li, S.; Cao, B.; Cui, Y.; Gao, S.; Kang, Y.; Gao, S.; Dong, H. Terahertz-based insulation delamination defect inspection of vehicle cable terminals. IEEE Trans. Transport. Electrif. 2023, 9, 1765–1773. [Google Scholar] [CrossRef]
- Tang, Y.; Gao, G.; Liu, K.; Chen, K.; Xie, Y.L.; Xin, D.L.; Zhou, S.Y.; Wu, G. Electric field distribution and performance optimization of high-speed train cable terminal with internal defects. Eng. Fail. Anal. 2023, 150, 107360. [Google Scholar] [CrossRef]
- Sun, J.; Lv, Y.; Ding, F.; Liu, J.; Zhi, Q.; Song, S.; Wen, H. A testing method with steep impact voltage inspecting the internal defects in emu roof cable termination. IET Sci. Meas. Technol. 2022, 16, 149–159. [Google Scholar] [CrossRef]
- Liu, K.; Yang, Z.; Wei, W.; Gao, B.; Xin, D.; Sun, C.; Gao, G.; Wu, G. Novel detection approach for thermal defects: Study on its feasibility and application to vehicle cables. High Volt. 2022, 8, 358–367. [Google Scholar] [CrossRef]
- Gao, G.; Tang, Y.; Cao, H.; Xin, D.; Xie, Y.; Chen, K.; Liu, K.; Wu, G. Research on electric field characteristics under different length interface air gap defects in cable terminals of high-speed train. Measurement 2024, 228, 114397. [Google Scholar] [CrossRef]
- Wang, X.; Tu, D.; Tanaka, Y.; Muronaka, T.; Takada, T.; Shinoda, C.; Hashizumi, T. Space charge in XLPE power cable under dc electrical stress and heat treatment. IEEE Trans. Dielectr. Electr. Insul. 1995, 2, 467–474. [Google Scholar] [CrossRef]
- Lee, W.-K. A study of electric stress enhancement. Part 1. Implication in power cable design. IEEE Trans. Dielectr. Electr. Insul. 2004, 11, 976–982. [Google Scholar]
- Bostrom, J.-O.; Marsden, E.; Hampton, R.N.; Nilsson, U. Electrical stress enhancement of contaminants in XLPE insulation used for power cables. IEEE Electr. Insul. Mag. 2003, 19, 6–12. [Google Scholar] [CrossRef]
- Malik, N.H.; Al-Arainy, A.A. Electrical stress distribution in three-core belted power cables. IEEE Trans. Power Deliv. 1987, 2, 589–595. [Google Scholar]
- Kim, C.; Jiang, P.; Liu, F.; Hyon, S.; Ri, M.; Yu, Y.; Ho, M. Investigation on dielectric breakdown behavior of thermally aged cross-linked polyethylene cable insulatiol. Polym. Test. 2019, 80, 106045. [Google Scholar] [CrossRef]
- Degefa, M.Z.; Lehtonen, M.; Millar, R.J. Comparison of Air-Gap Thermal Models for MV Power Cables Inside Unfilled Conduit. IEEE Trans. Power Deliv. 2012, 27, 1662–1669. [Google Scholar] [CrossRef]
- Grzybowski, S.; Cao, F.L. Accelerated aging study on 15 kV XLPE and EPR cables insulation caused by switching impulses. IEEE Trans. Dielectr. Electr. Insul. 2015, 22, 2809–2817. [Google Scholar]
- Yun, J.; Ming, H.; Xia, R.; Li, W. Capacitive coupling detection and characteristic analysis of partial discharge in high-voltage XPLE power cable joints. High. Volt. Engine 2010, 36, 90–97. [Google Scholar]
- Li, Q.; Yang, X.; Zhang, C.; Fu, Q.; Li, D.; Zhou, W. The impact of different air gap defects in polypropylene coaxial cables on electric field distortion. J. Phys. Conf. Ser. 2024, 2741, 012058. [Google Scholar]
- Liu, J.; Xin, X.; Gao, Y.; Yu, X.; Li, Z.; Liu, Y.; Wang, J.; Zhang, Z. Analysis of electric field distribution of cable insulation defects. J. Phys. Conf. Ser. 2018, Volume 1087, 042040. [Google Scholar]
- Xu, N.; Liu, Y.; Wang, Z.; Wu, M.; Ahmed, M.; Liu, Y.; Gao, J.; Zhong, L. Anomalous attenuation phenomenon of terahertz wave through the micron air gap defects in XLPE insulation material. Polym. Test. 2024, 136, 108486. [Google Scholar] [CrossRef]
- Gao, G.; Li, B.; He, M.; Liu, K.; Tang, Y.; Xin, D. Evaluation of partial discharge severity for air gap defects in vehicle cable terminals. In Proceedings of the 2022 IEEE International Conference on High Voltage Engineering and Applications (ICHVE), Chongqing, China, 25–29 September 2022; pp. 1–4. [Google Scholar]
- Ge, X.; Fan, F.; Given, M.J.; Stewart, B.G. Insulation resistance degradation models of extruded power cables under thermal ageing. Energies 2024, 17, 1062. [Google Scholar] [CrossRef]
- Yang, J.; Li, R.; Hu, L.; Wang, W. Influence of Thermal Aging on Space Charge Characteristics and Electrical Conduction Behavior of Cross-Linked Polyethylene Cable Insulation. Polymers 2024, 16, 1600. [Google Scholar] [CrossRef]
- Mecheri, Y.; Bouazabia, S.; Boubakeur, A.; Lallouani, M. Effect of thermal ageing on the properties of XLPE as an insulating material for HV cables. In Proceedings of the International Electrical Insulation Conference, Quebec City, QC, Canada, 18–21 June 2023; pp. 29–31. [Google Scholar]
- Pandey, P.; Reddy, C. Effect of Inhomogeneous Ageing of Insulation on Electric Field Distribution of a 220 kV HVAC in-Service Cable. IEEE Trans. Dielectr. Electr. Insul. 2024, 31, 3285–3293. [Google Scholar] [CrossRef]
- Li, S.; Cao, B.; Li, J.; Cui, Y.; Kang, Y.; Wu, G. Review of condition monitoring and defect inspection methods for composited cable terminals. High Volt. 2023, 8, 431–444. [Google Scholar] [CrossRef]
- Zhao, X.; Pu, L.; Xu, Z.; Chen, G.; Duan, W.; Sun, H.; Ju, Z. Interfacial space charge characteristic of PPLP insulation for HVDC cables. High Volt. 2020, 5, 628–635. [Google Scholar] [CrossRef]
- Ocłoń, P.; Cisek, P.; Pilarczyk, M.; Taler, D. Numerical simulation of heat dissipation processes in underground power cable system situated in thermal backfill and buried in a multilayered soil. Energy Convers. Manag. 2015, 95, 352–370. [Google Scholar] [CrossRef]
- Dubitsky, S.; Greshnyakov, G.; Korovkin, N. Refinement of Underground Power Cable Ampacity by Multiphysics FEA Simulation. Int. J. Energy 2015, 9, 1998–4316. [Google Scholar]
- EN 50264; Railway Applications-Railway Rolling Stock Power and Control Cables Having Special Fire Performance. British Standards Institution: London, UK, 2008.
- Choudhary, M.; Shafiq, M.; Kiitam, I.; Hussain, A.; Palu, I.; Taklaja, P. A review of aging models for electrical insulation in power cables. Energies 2022, 15, 3408. [Google Scholar] [CrossRef]
- Jongen, R.A.; Morshuis, P.H.F.; Smit, J.J.; Janssen, A.L.J. Influence of ambient temperature on the failure behavior of cable joints. In Proceedings of the 2007 Annual Report-Conference on Electrical Insulation and Dielectric Phenomena, Vancouver, BC, Canada, 14–17 October 2007; pp. 643–646. [Google Scholar]
- Williams, G.M.; Lewin, P.L.; LeBlanc, M. Accurate determination of ambient temperature at burial depth for high voltage cable ratings. In Proceedings of the Conference Record of the 2004 IEEE International Symposium on Electrical Insulation, Indianapolis, IN, USA, 19–22 September 2004; pp. 458–461. [Google Scholar]
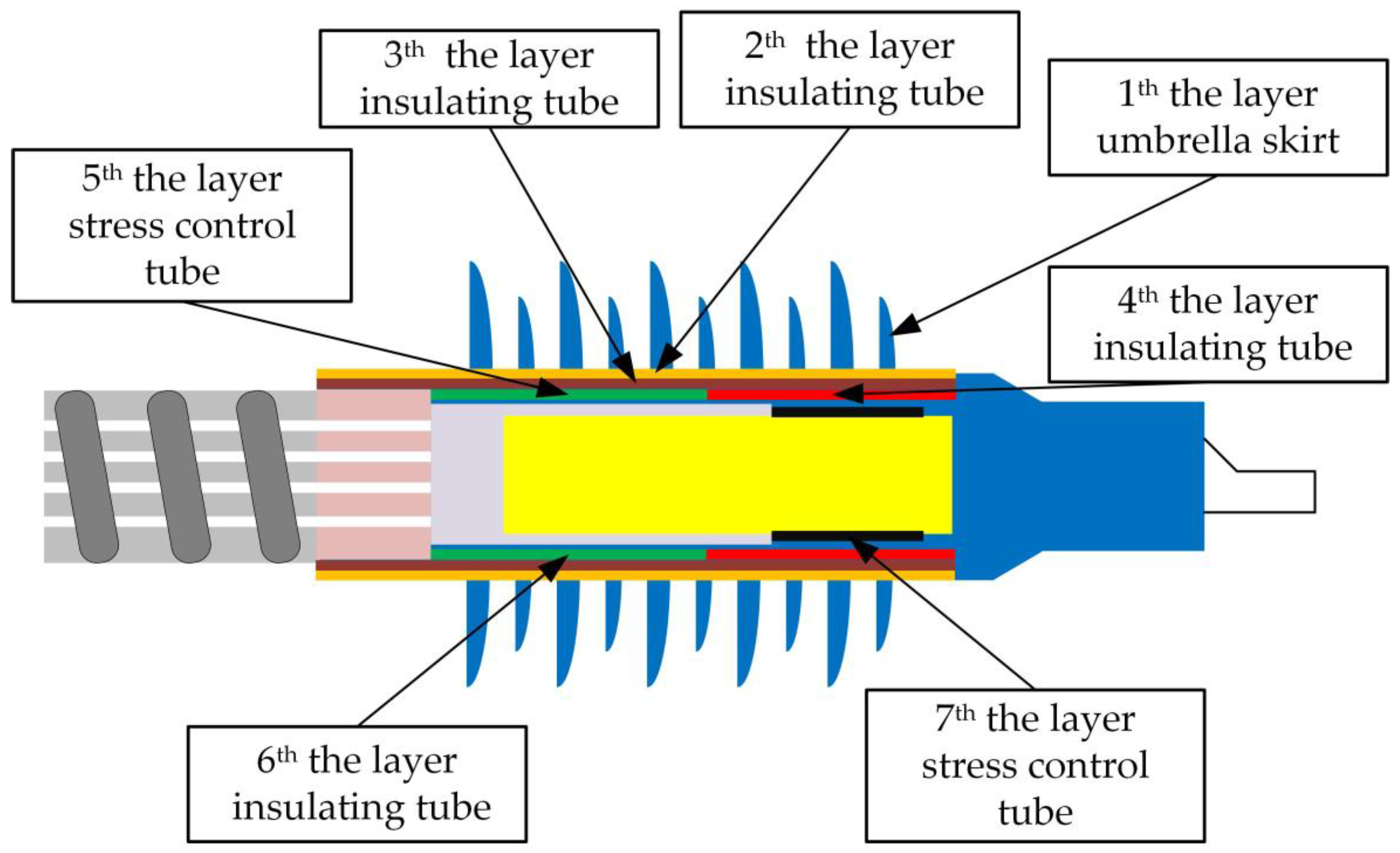






| Structure | Materials | Outside Radius /(mm) |
|---|---|---|
| Cable core | Copper-aluminum alloy | 9.77 |
| Semiconductor layer | Semiconductor polymer | 19.57 |
| stress control tube | Polymer materials | 18.27 |
| Glue | Hot melt glue | / |
| heat shrink tube | PE | 23.77 |
| Sheathing layer | PVC | 23.92 |
| Umbrella skirt | Rubber | 27.12 |
| Structure | Electric Conductivity /(S/m) | Relative Dielectric Constant /(1) | Thermal Conductivity /(W/(m·k)) | Poisson Ratio /(P) |
|---|---|---|---|---|
| Cable core | 5.998 × 107 | 1 | 400 | 0.35 |
| Semiconductor layer | 2 | 100 | 10 | 0.25 |
| stress control tube | 1.3 × 10−9 | 25.3 | 35.3 | 0.44 |
| Glue | 7.7 | 0.5 × 10−8 | 10 | 0.38 |
| heat shrink tube | 0.25 × 10−10 | 3.34 | 0.25 | 0.22 |
| Sheathing layer | 1 × 10−18 | 2.6 | 0.46 | 0.38 |
| Umbrella skirt | 0.8 × 10−18 | 0.85 | 2.25 | 0.26 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pan, L.; Luo, Y.; Wang, X.; Lei, D.; Wang, J.; Zhang, H.; Yang, Z.; Wei, W. Simulation Research on the Effects of Air Gaps and Ambient Temperature on a 27.5 kV Power Cable. Appl. Sci. 2025, 15, 1028. https://doi.org/10.3390/app15031028
Pan L, Luo Y, Wang X, Lei D, Wang J, Zhang H, Yang Z, Wei W. Simulation Research on the Effects of Air Gaps and Ambient Temperature on a 27.5 kV Power Cable. Applied Sciences. 2025; 15(3):1028. https://doi.org/10.3390/app15031028
Chicago/Turabian StylePan, Like, Yunfeng Luo, Xinwei Wang, Dong Lei, Jiawei Wang, Huan Zhang, Zefeng Yang, and Wenfu Wei. 2025. "Simulation Research on the Effects of Air Gaps and Ambient Temperature on a 27.5 kV Power Cable" Applied Sciences 15, no. 3: 1028. https://doi.org/10.3390/app15031028
APA StylePan, L., Luo, Y., Wang, X., Lei, D., Wang, J., Zhang, H., Yang, Z., & Wei, W. (2025). Simulation Research on the Effects of Air Gaps and Ambient Temperature on a 27.5 kV Power Cable. Applied Sciences, 15(3), 1028. https://doi.org/10.3390/app15031028






