Plate Size Effects in Gravelly Soil Based on In Situ Plate Load Tests and Finite Element Analysis
Abstract
1. Introduction
2. Geotechnical Profile and Soil Parameter
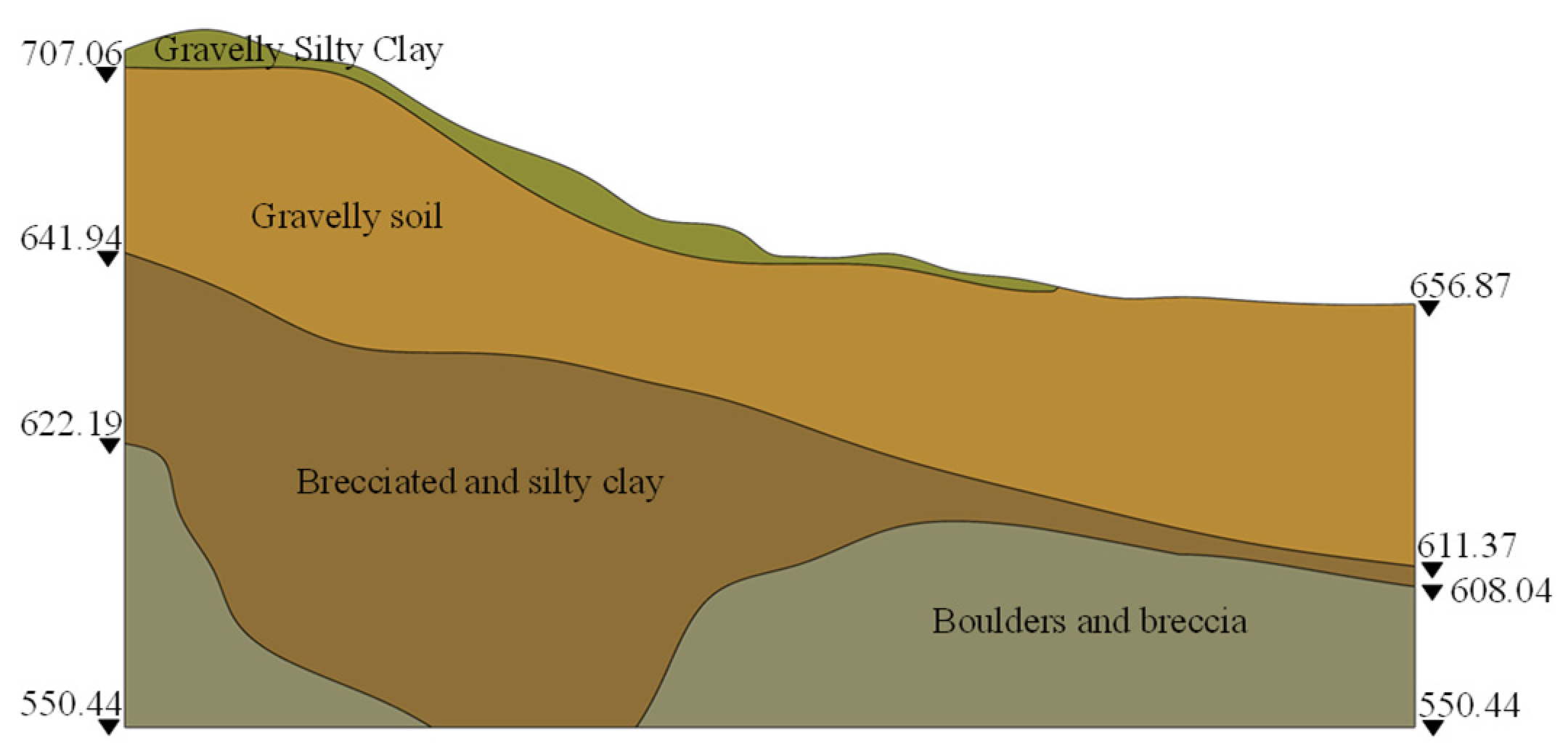
2.1. Geotechnical Profile
2.2. Soil Parameter
3. In Situ Plate Load Test
3.1. Experimental Setup
3.2. Field PLT Results
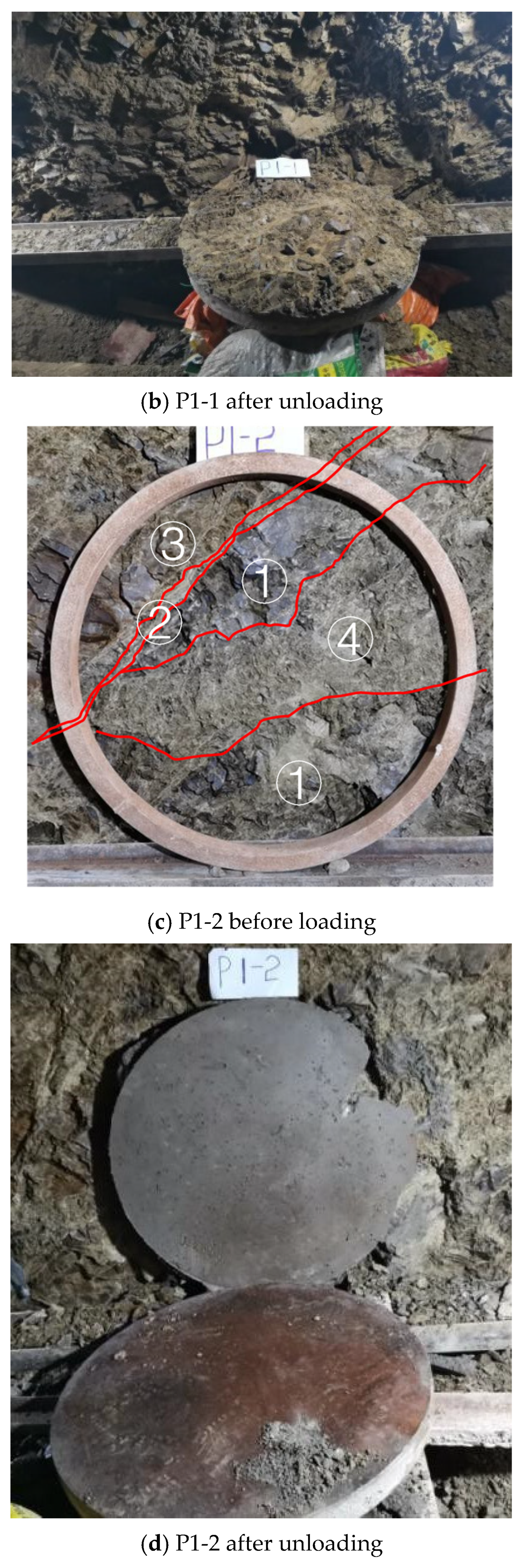
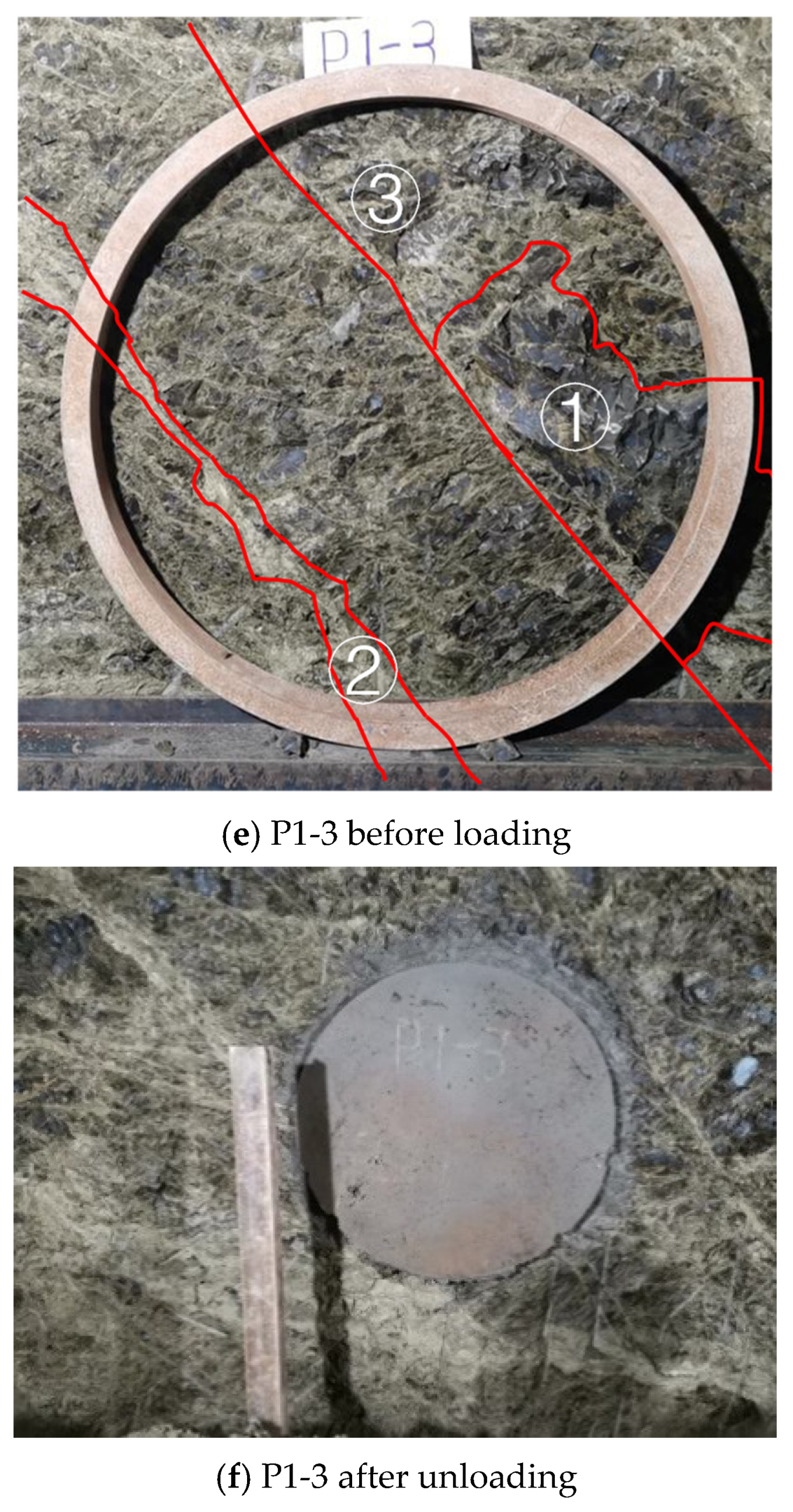
3.2.1. Geological Description of PLT Test Sites
3.2.2. Average Contact Pressure Settlement
4. Finite Element Analysis
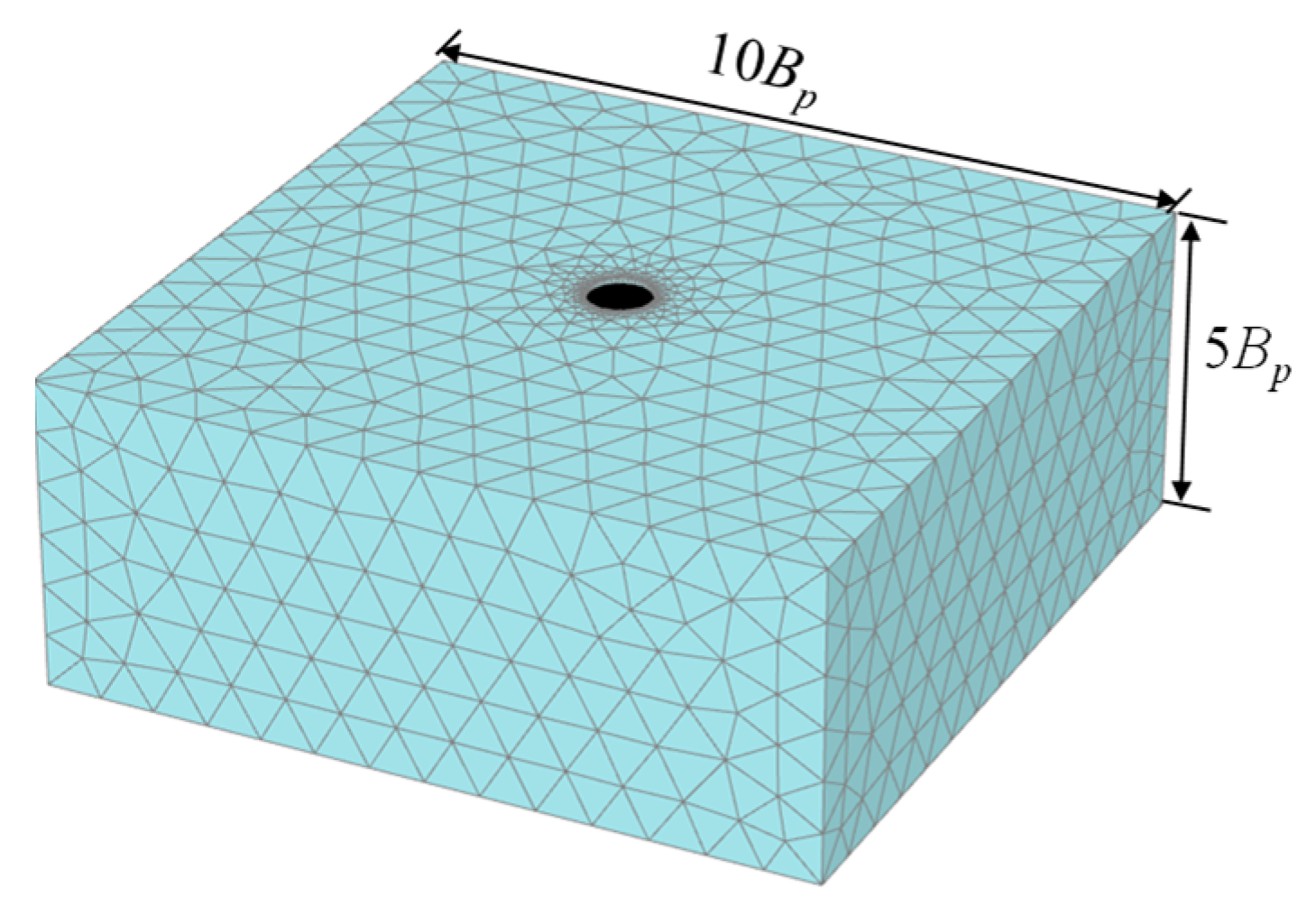
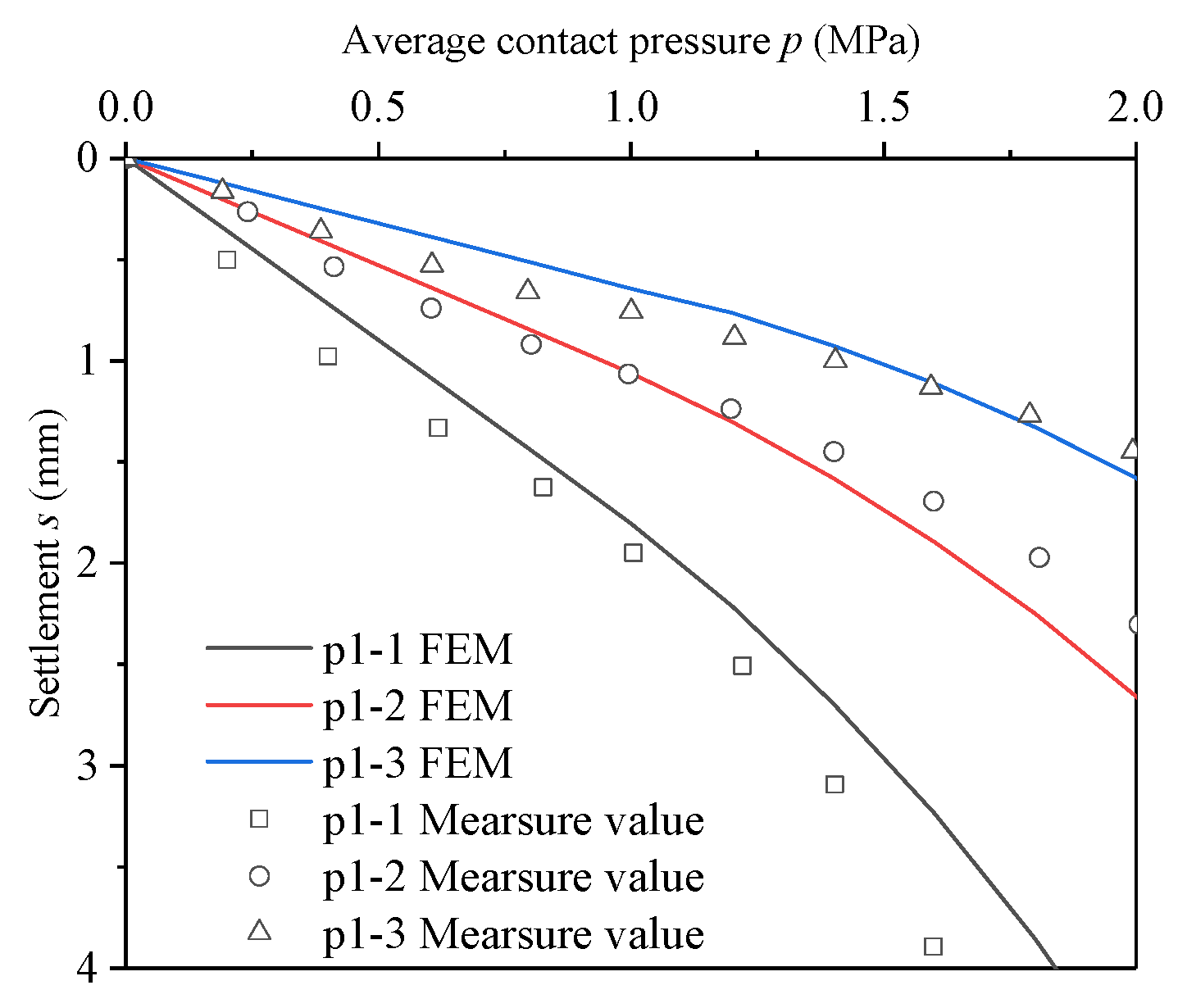
4.1. Calibration of the Model
4.2. Results and Analysis
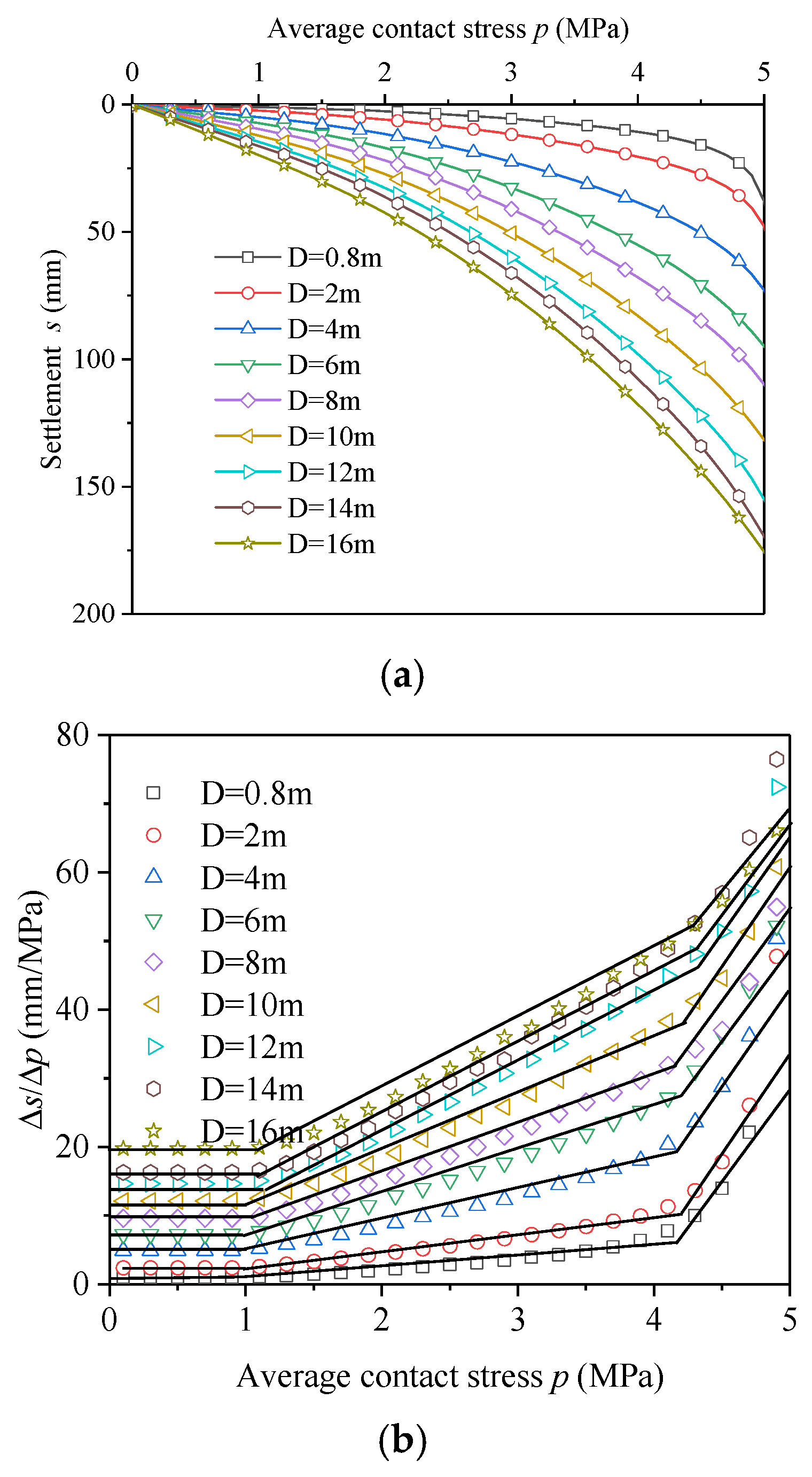
4.2.1. Average Contact Pressure–Settlement Curves
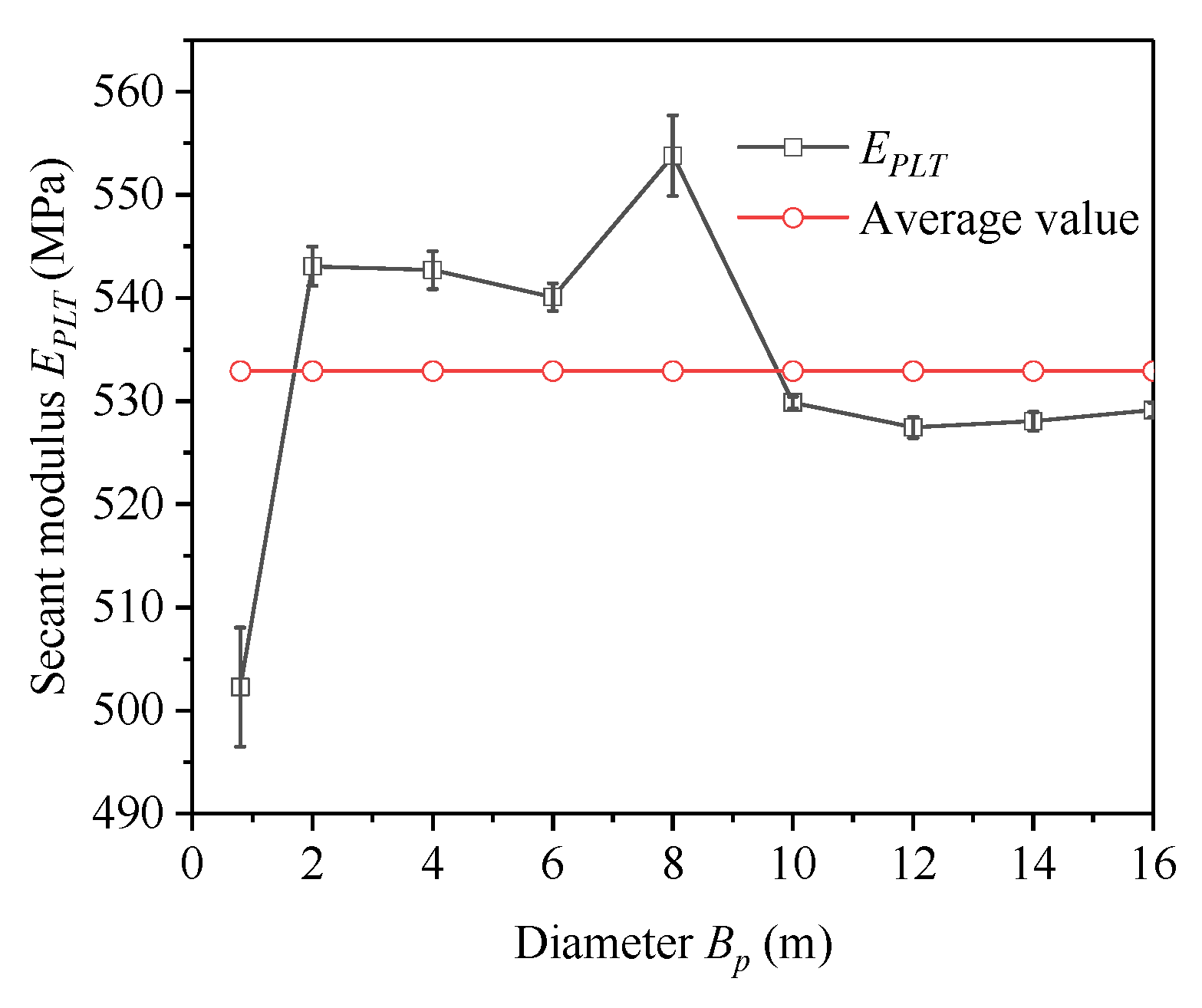
4.2.2. Secant Modulus EPLT
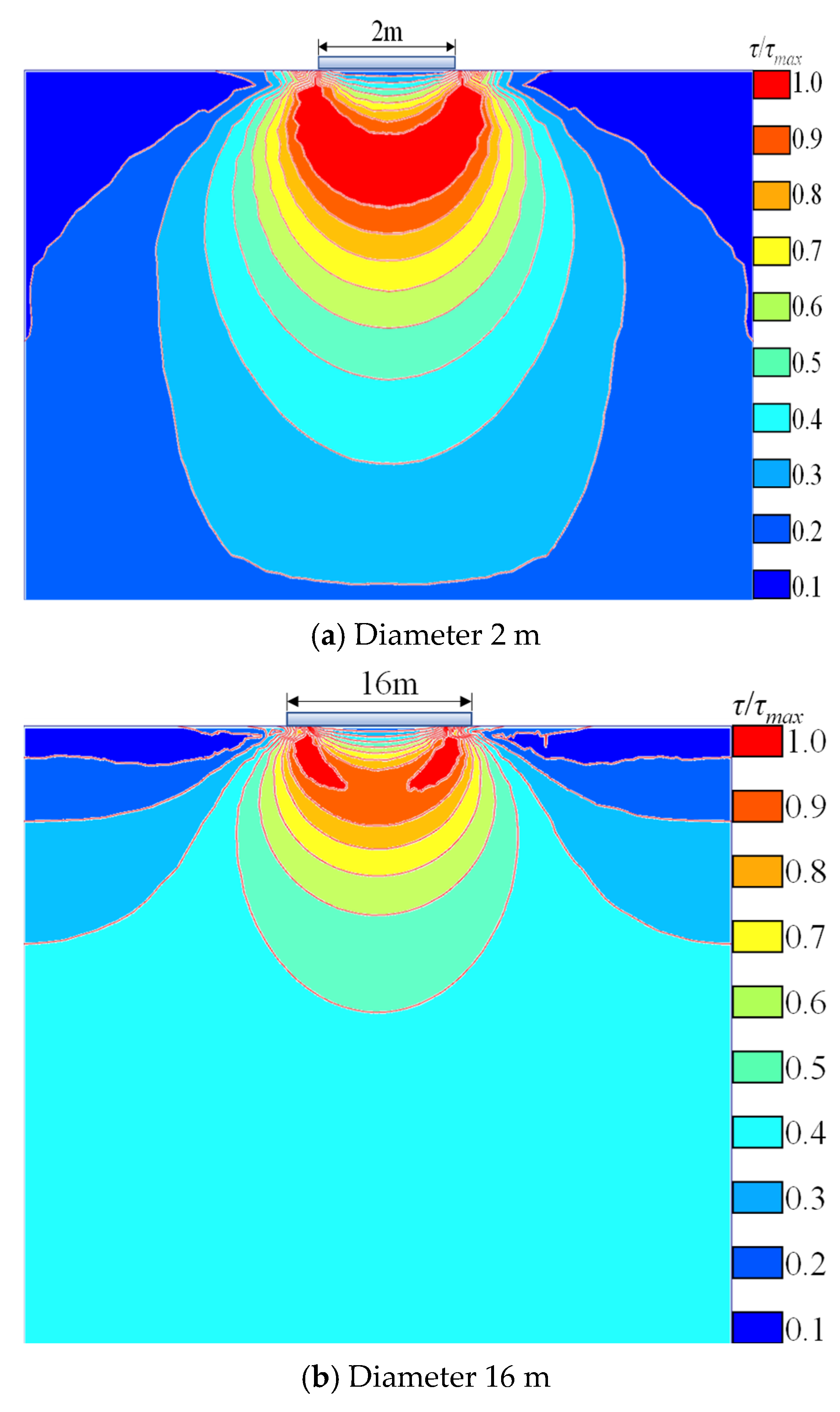
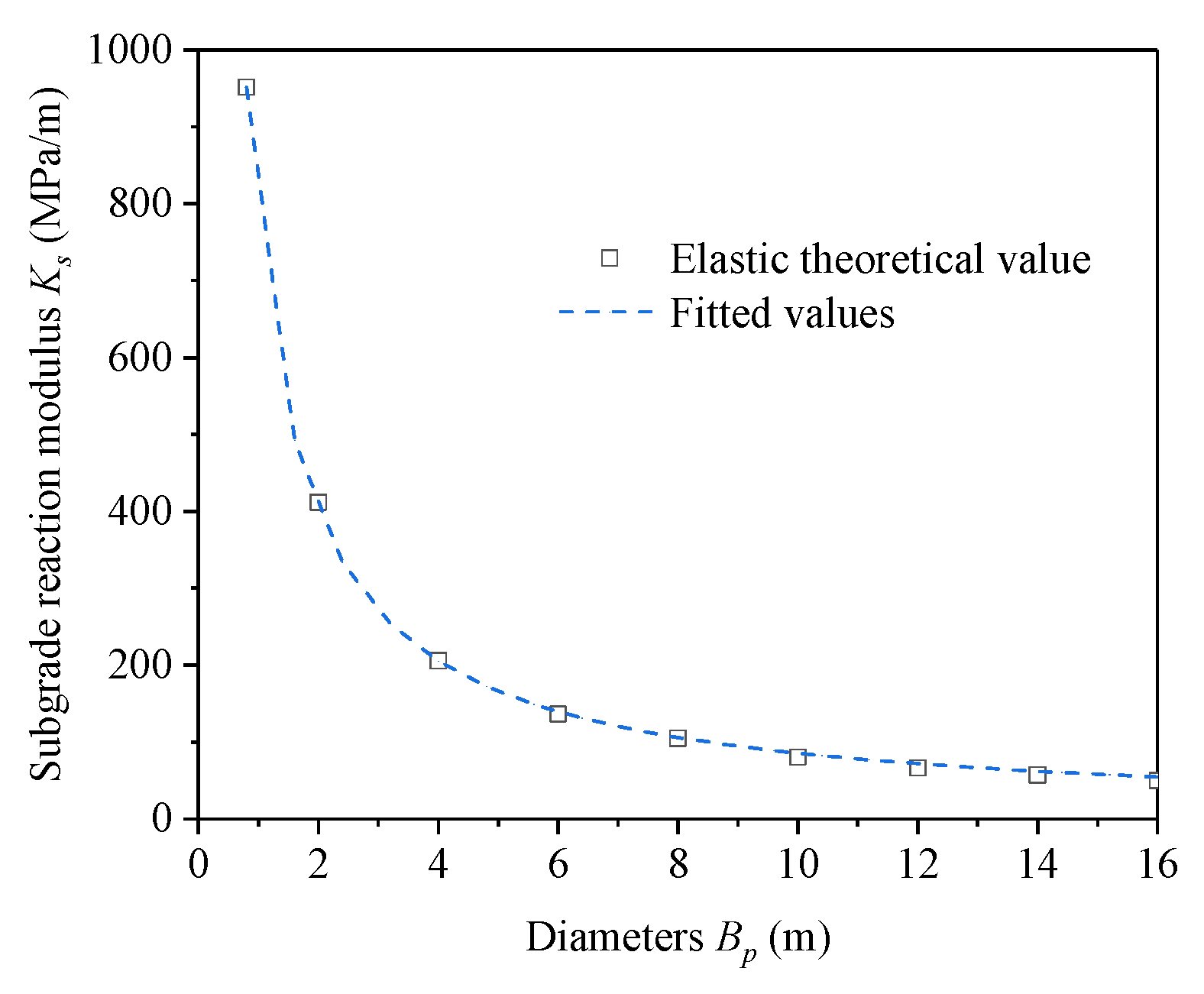
4.2.3. Coefficient of Subgrade Reaction
5. Discussions
6. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Akbas, S.O.; Kulhawy, F.H. Axial compression of footings in cohesionless soils. II: Load-settlement behavior. J. Geotech. Geoenviron. Eng. 2009, 135, 1575–1582. [Google Scholar] [CrossRef]
- Oh, W.T.; Vanapalli, S.K. Infuence of poisson’s ratio on the stress vs. settlement behavior of shallow foundations in unsaturated fne-grained soils. Soils Rocks 2016, 39, 71–79. [Google Scholar] [CrossRef]
- Consoli, N.C.; Rosa, F.D.; Fonini, A. Plate load tests on cemented soil layers overlaying weaker soil. J. Geotech. Geoenviron. Eng. 2009, 135, 1846–1856. [Google Scholar] [CrossRef]
- Nwokediuko, N.M.; Ogirigbo, O.R.; Inerhunwa, I. Load-settlement characteristics of tropical red soils of southern nigeria. Eur. J. Eng. Res. Sci. 2019, 4, 107–113. [Google Scholar] [CrossRef]
- Demir, A.; Yildiz, A.; Laman, M.; Ornek, M. Experimental and numerical analyses of circular footing on geogrid-reinforced granular fll underlain by soft clay. Acta Geotech. 2014, 9, 711–723. [Google Scholar] [CrossRef]
- Ebid, A.M.; Onyelowe, K.C.; Salah, M. Load-settlement curve and subgrade reaction of strip footing on bi-layered soil using constitutive fem-ai coupled techniques. Designs 2022, 6, 104. [Google Scholar] [CrossRef]
- Tang, Y.; Vo, T.; Taiebat, H.A.; Russell, A.R. Influences of suction on plate load tests on unsaturated silty sands. J. Geotech. Geoenviron. Eng. 2018, 144, 04018043. [Google Scholar] [CrossRef]
- EN 1997-2:2007; Eurocode 7: Geotechnical Design—Part 2: Design Assisted by Field Testing. European Committee for Standardization: Brussels, Belgium, 2004.
- Bowles, J.E. Foundation Analysis and Design, 5th ed.; McGraw-Hill: New York, NY, USA, 1997; pp. 318–319. [Google Scholar]
- Cerato, A.B.; Lutenegger, A.J. Scale effects of shallow foundation bearing capacity on granular material. J. Geotech. Geoenviron. Eng. 2007, 133, 1192–1202. [Google Scholar] [CrossRef]
- Zhu, F.; Clark, J.I.; Phillips, R. Scale effect of strip and circular footings resting on dense sand. J. Geotech. Geoenviron. Eng. 2001, 127, 613–621. [Google Scholar] [CrossRef]
- Araujo, D.A.M.; Costa, C.M.L.; Costa, Y.D.J. Dimension effect on plate load test results. In Proceedings of the 2nd World Congress on Civil, Structural, and Environmental Engineering, Barcelona, Spain, 2–4 April 2017; pp. 2–4. [Google Scholar] [CrossRef]
- Briaud, J.L.; Gibbens, R. Predicted and measured behavior of five large spread footings on sand. In Proceedings of the Prediction Symposium, ASCE, GSP41, Texas City, TX, USA, 16–18 June 1994. [Google Scholar]
- Winkler, E. Die Lehre von der Elastizität und Festigkeit (on Elasticity and Fixity); Dominicius: Prague, Czech Republic, 1867; p. 182. Available online: https://books.google.co.th/books?id=25E5AAAAcAAJ&printsec=frontcover&hl=th&source=gbs_ge_summary_r&cad=0#v=onepage&q&f=false (accessed on 23 October 2024).
- Li, W.; Yuan, W.; Tao, Q.; Gong, W.; Dai, G. The influence of pile shaft distributed grouting on the horizontal load response of rectangular piles. KSCE J. Civ. Eng. 2024, 28, 5516–5523. [Google Scholar] [CrossRef]
- DL/T 5356-2006; Code for Coarse-Grained Soil Tests for Hydropower and Water Conservancy Engineering. National Development and Reform Commission (NDRC) of the People’s Republic of China, China Electric Power Press: Beijing, China, 2004.
- Timoshenko, S.; Goodier, J.N. Theory of Elasticity, 2nd ed.; McGraw-Hill: New York, NY, USA, 1951; pp. 368–372. [Google Scholar]
- Fu, Z.; Chen, S.; Liu, S. Discrete element simulations of shallow plate-load tests. Int. J. Geomech. 2016, 16, 04015077. [Google Scholar] [CrossRef]
- Sadeghi-Chahardeh, A.; Gumiere, S.J. Application of discrete element method simulation in environmental modeling. In Soil Health and Environmental Sustainability: Application of Geospatial Technology; Springer International Publishing: Cham, Switzerland, 2022; pp. 43–59. [Google Scholar]
- Bahrami, M.; Naderi-Boldaji, M.; Ghanbarian, D.; Keller, T. Simulation of soil stress under plate sinkage loading: A comparison of finite element and discrete element methods. Soil Tillage Res. 2022, 223, 105463. [Google Scholar] [CrossRef]
- Ping, W.V.; Ge, L. Field verification of laboratory resilient modulus measurements on subgrade soils. Transp. Res. Rec. 1997, 1557, 53–61. [Google Scholar] [CrossRef]
- Japan Road Association. Specifications for Highway Bridges, 5th ed.; Japan Road Association: Tokyo, Japan, 2017. [Google Scholar]
- Lin, P.S.; Yang, L.W.; Juang, C.H. Subgrade reaction and load-settlement characteristics of gravelly cobble deposits by plate-load tests. Can. Geotech. J. 1998, 35, 801–810. [Google Scholar] [CrossRef]
- Bentley Systems. Plaxis 3D Reference Manual (Version 2022). Available online: https://www.plaxis.com/manuals (accessed on 23 October 2024).
- Terzaghi, K. Evaluation of coefficients of subgrade reaction. Geotechnique. 1955, 5, 297–326. [Google Scholar] [CrossRef]
- Construction Civil. Plate Load Test: Determining the Bearing-Capacity of Soils. Available online: https://www.theconstructioncivil.org/ (accessed on 23 October 2024).
- IS 1888: 1982; Method of Load Test on Soils. Bureau of Indian Standards: New Delhi, India, 1982.
- Nishioka, H.; Anzai, A.; Koda, M.; Tateyama, M. Estimation of deformation modulus of ground and coefficient of subgrade reaction depending on ground investigation method. RTRI Report. J. Railw. Tech. Res. Inst. 2010, 24, 11–16. [Google Scholar]
- Fukushima, H.; Nishimoto, S.; Tomizawa, K. Scale Effect of Spread Foundation Using Various Size Plates Loading Tests. In Proceedings of the 39th Geotechnical Engineering Research Presentation Conference, Niigata, Japan, 7–9 July 2004; pp. 1395–1396. [Google Scholar] [CrossRef]
- Yoshida, I.; Yoshinaka, R. A method to estimate modulus of horizontal subgrade reaction for a pile. Soils Found. 1972, 12, 1–17. [Google Scholar] [CrossRef] [PubMed]





| Parameters | γ/(kN/m3) | w/(%) | Es/(MPa) | φ/(°) | c/(kPa) | ||
|---|---|---|---|---|---|---|---|
| Es1 | Es2 | Es3 | |||||
| Value | 24 | 5.54 | 338.15 | 579.69 | 886.58 | 26° | 128 |
| Parameters | P1-1 | P1-2 | P1-3 |
|---|---|---|---|
| EPLT/(kN/m2) | 275.4 | 485.1 | 761.4 |
| Ks/(kN/m3) | 522.1 | 919.6 | 1443.3 |
| Diameter/(m) | 0.8 | 2 | 4 | 6 | 8 | 10 | 12 | 14 | 16 |
| Elastic limit load/(MPa) | 1 | ||||||||
| Displacement/(mm) | 1.0459 | 2.4242 | 4.8511 | 7.3169 | 9.5136 | 12.4125 | 14.9525 | 17.4559 | 19.8946 |
| Yield limit load/(MPa) | 3.8 | 4 | 4.1 | 4.1 | 4.5 | 4.6 | 4.6 | 4.6 | 4.6 |
| Displacement/(mm) | 9.37 | 20.4239 | 40.4610 | 57.7655 | 84.8532 | 108.2208 | 127.3327 | 140.0661 | 149.7152 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, W.; Tao, Q.; Gu, R.; Li, C.; Dai, G.; Gong, W. Plate Size Effects in Gravelly Soil Based on In Situ Plate Load Tests and Finite Element Analysis. Appl. Sci. 2025, 15, 760. https://doi.org/10.3390/app15020760
Li W, Tao Q, Gu R, Li C, Dai G, Gong W. Plate Size Effects in Gravelly Soil Based on In Situ Plate Load Tests and Finite Element Analysis. Applied Sciences. 2025; 15(2):760. https://doi.org/10.3390/app15020760
Chicago/Turabian StyleLi, Wenshuai, Qiyu Tao, Rui Gu, Chao Li, Guoliang Dai, and Weiming Gong. 2025. "Plate Size Effects in Gravelly Soil Based on In Situ Plate Load Tests and Finite Element Analysis" Applied Sciences 15, no. 2: 760. https://doi.org/10.3390/app15020760
APA StyleLi, W., Tao, Q., Gu, R., Li, C., Dai, G., & Gong, W. (2025). Plate Size Effects in Gravelly Soil Based on In Situ Plate Load Tests and Finite Element Analysis. Applied Sciences, 15(2), 760. https://doi.org/10.3390/app15020760





