Generating Input Ground Motions for Seismic Risk Assessment Using Recorded Ground Motions from the Moderate Magnitude Earthquake
Abstract
1. Introduction
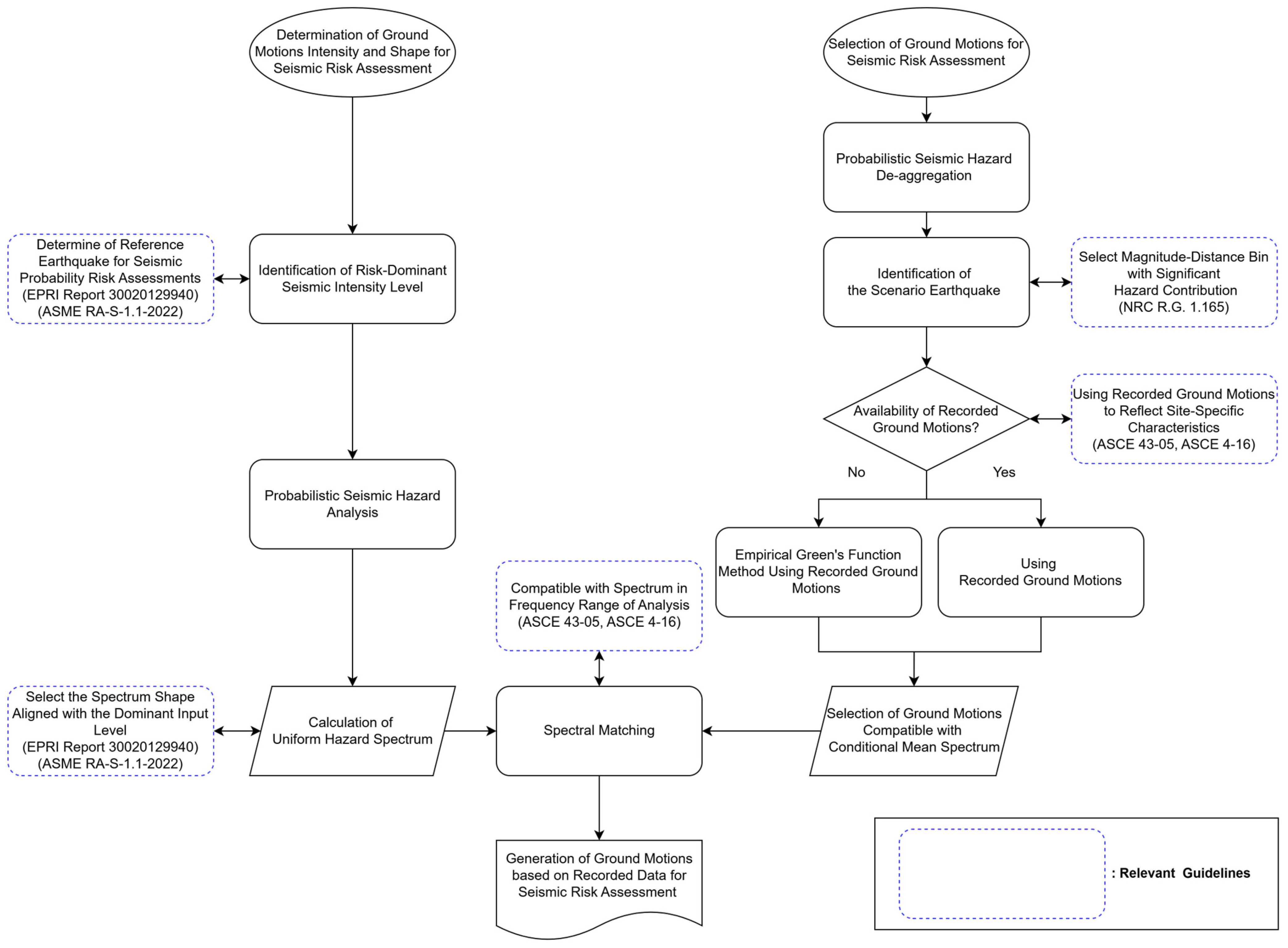
2. Guidelines for Using Recorded Ground Motions in Response History Analysis
2.1. Seismic Intensity and Spectrum Shape
2.2. Selection of Ground Motions
3. Procedure Considering Guidelines for Generating Ground Motions
4. Probabilistic Seismic Hazard Analysis and Evaluation of Example Scenario Earthquake
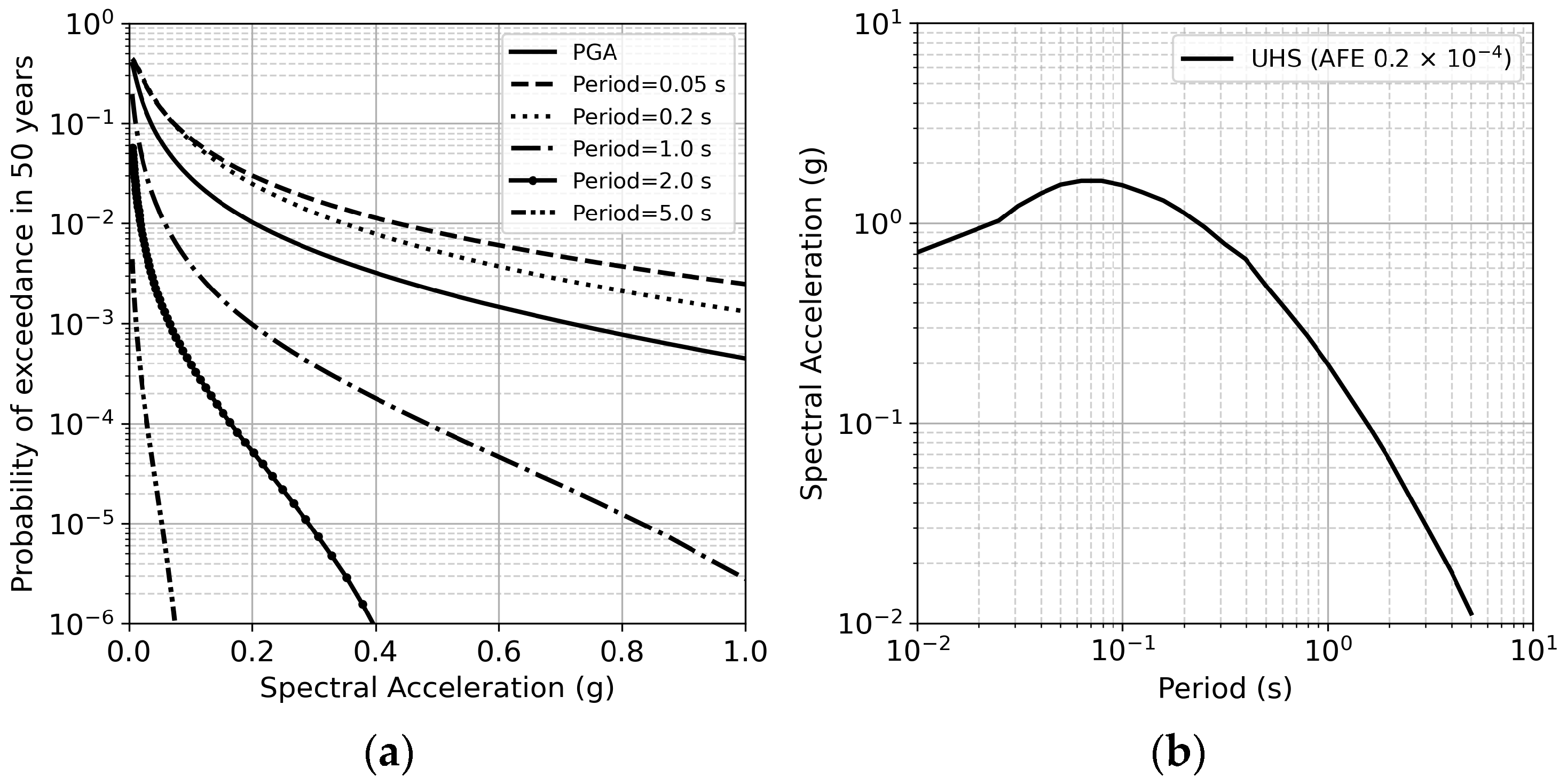
4.1. Development of a Uniform Hazard Spectrum as the Risk Assessment Spectrum
| Parameter | Value | |
|---|---|---|
| Seismic Source Model | 100 km 100 km single area source | |
| Gutenberg-Richter | value | 2.843 |
| value | 0.95 | |
| Min Magnitude | 5.0 Mw | |
| Max Magnitude | 7.0 Mw | |
| Ground Motion Prediction Equation | Atkinson and Boore (2006) [30] | |
| Annual Frequency of Exceedance | 0.2 × 10−4 (/year) | |
4.2. Seismic Hazard De-Aggregation for Selection of Scenario Earthquake
4.3. Target Spectrum for Selecting Seed Ground Motions in Risk Assessment
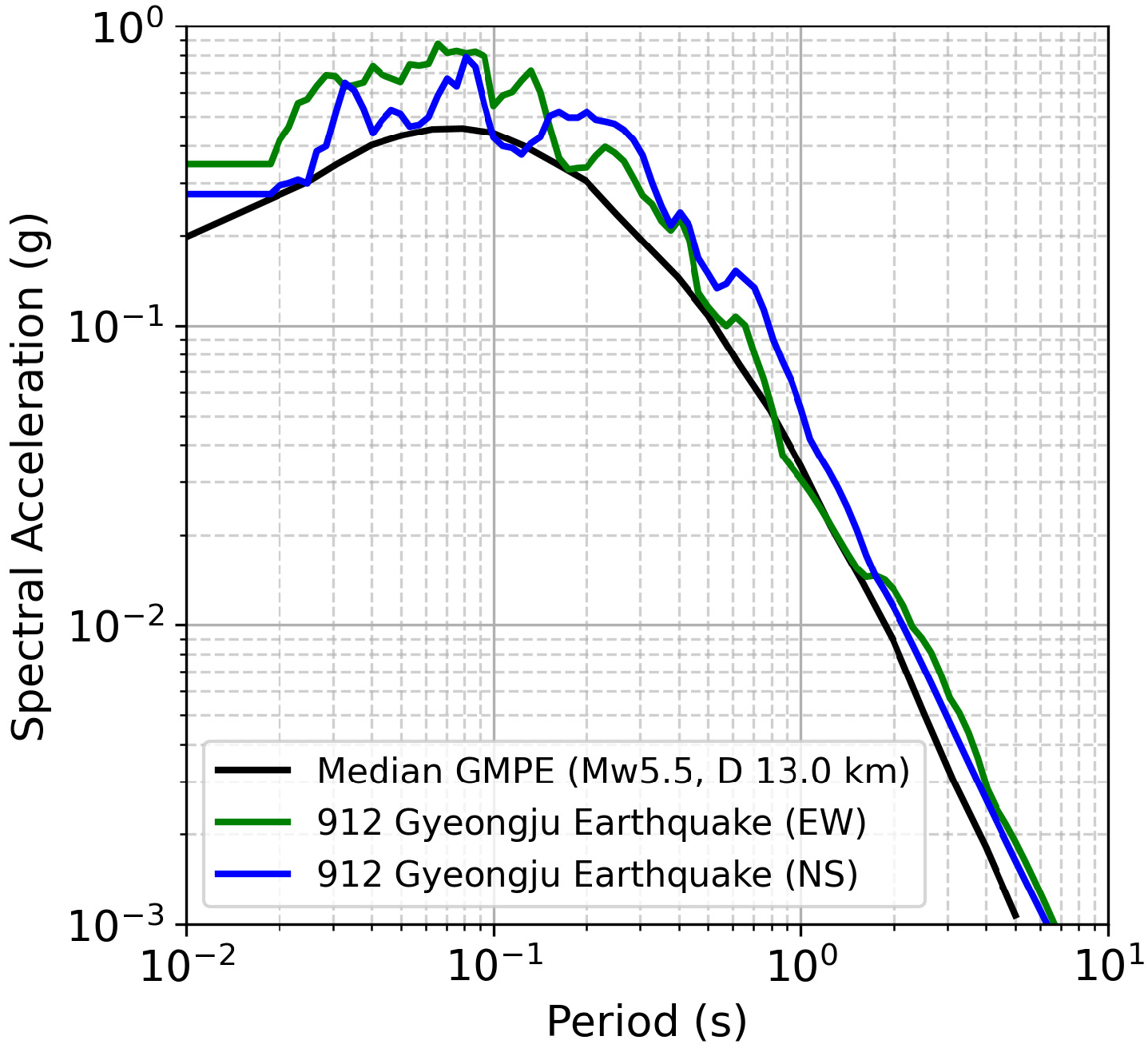
5. Finite-Fault Modeling for Scenario Earthquake Utilizing Empirical Green’s Function Method
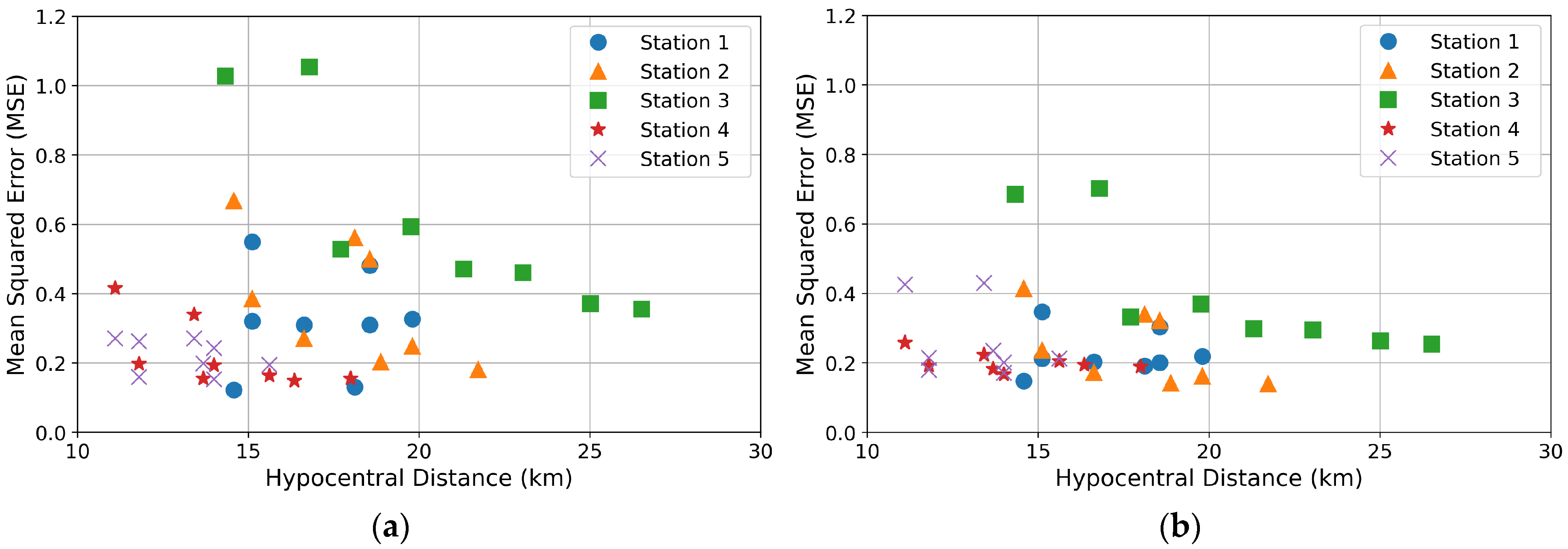
6. Selection of Seed Ground Motions for UHS and CMS
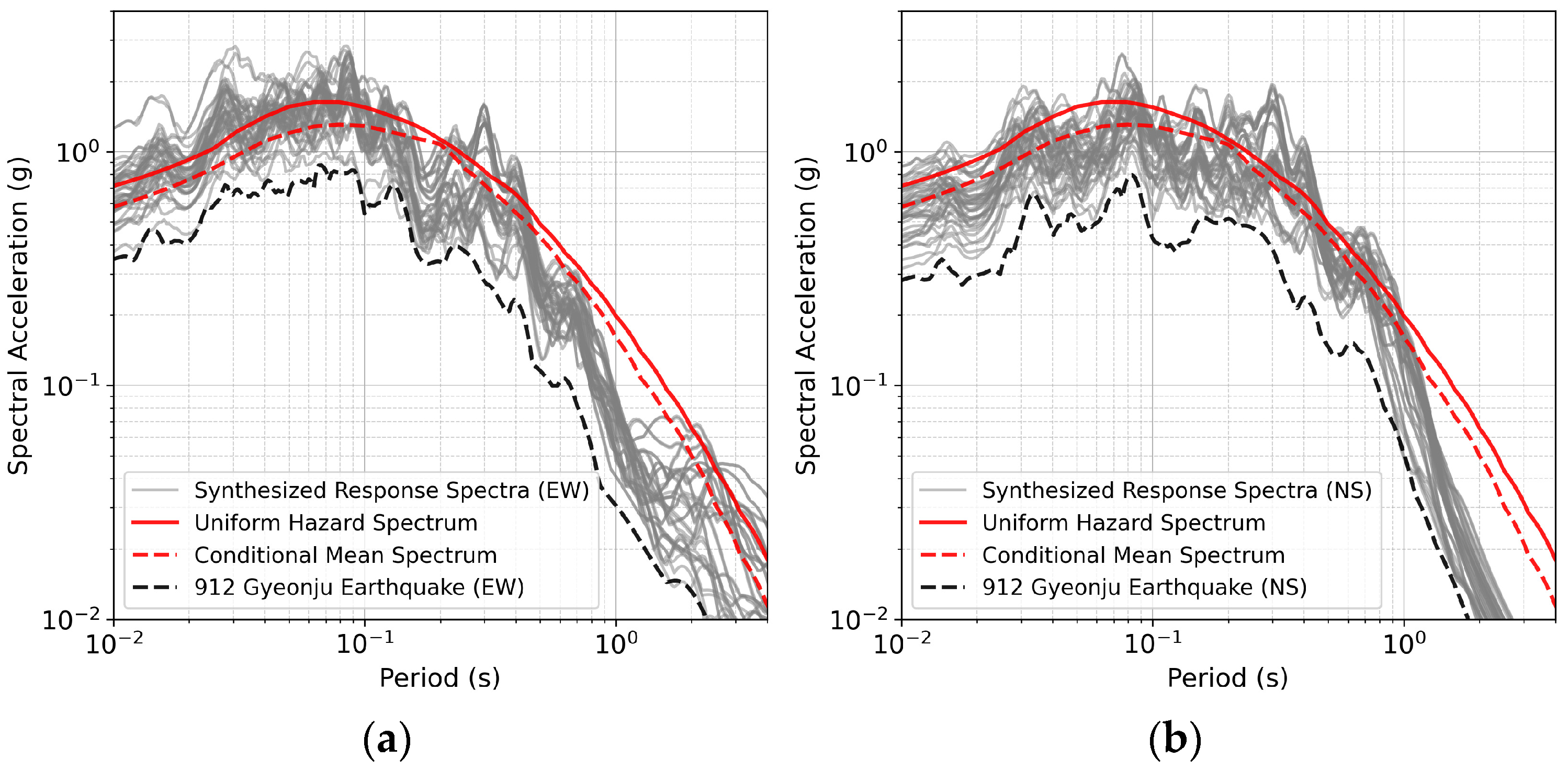
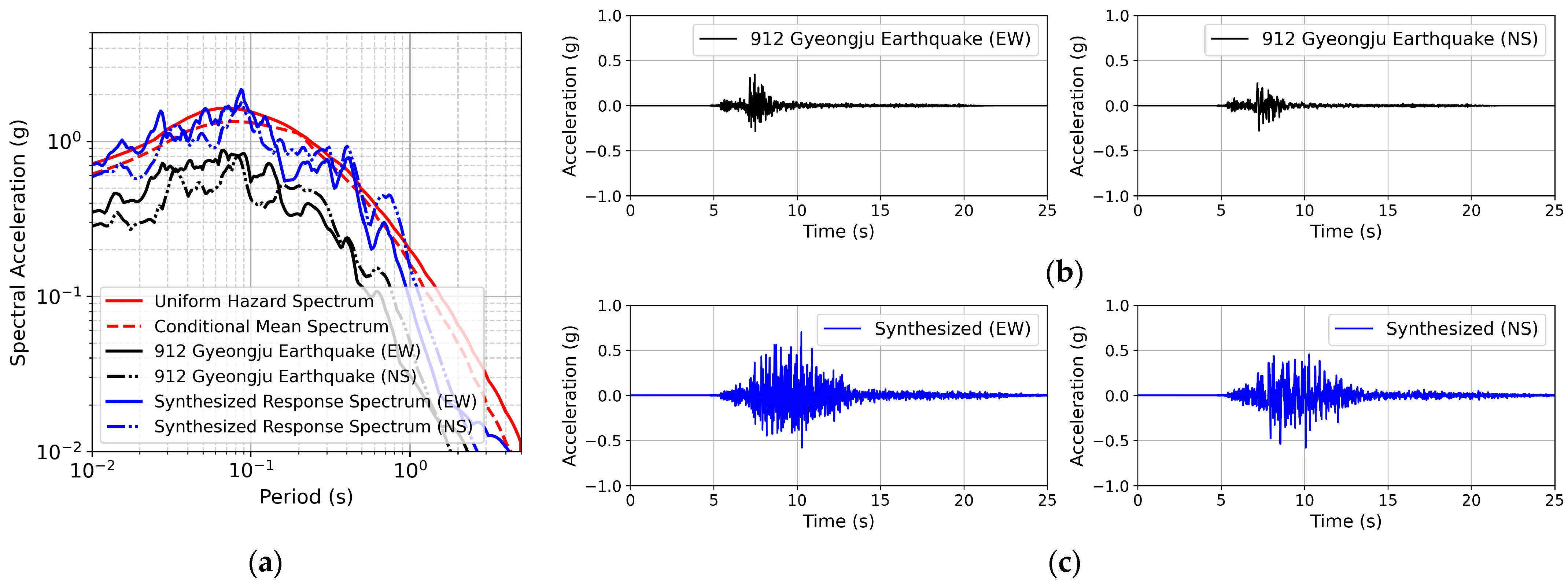
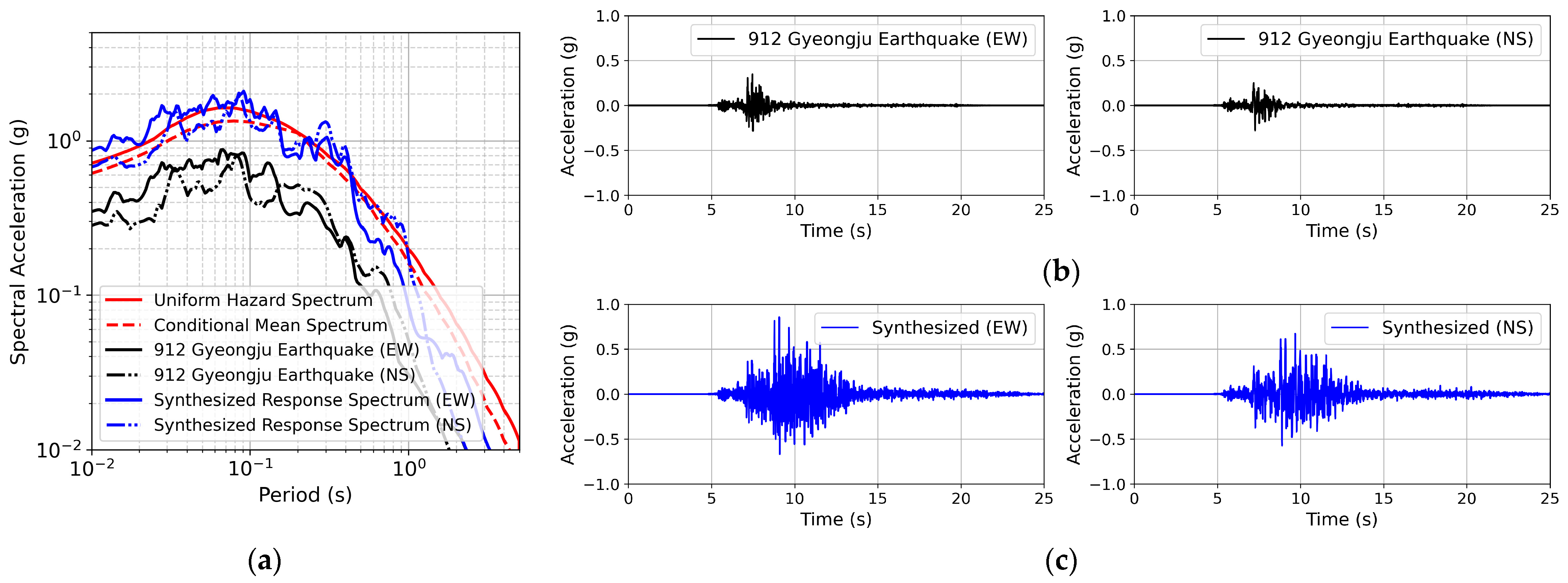
7. Spectral Matching of Seed Ground Motions to Risk Assessment Spectrum
8. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kappos, A. Dynamic Loading and Design of Structures, 1st ed.; CRC Press: London, UK, 2002. [Google Scholar]
- Somerville, P.G.; Smith, N.F.; Graves, R.W.; Abrahamson, N.A. Modification of empirical strong ground motion attenuation relations to include the amplitude and duration effects of rupture directivity. Seismol. Res. Lett. 1997, 68, 199–222. [Google Scholar] [CrossRef]
- Hutchings, L.; Viegas, G. Application of empirical Green’s functions in earthquake source, wave propagation and strong ground motion studies. In Earthquake Research and Analysis-New Frontiers in Seismology; IntechOpen: London, UK, 2012; pp. 87–140. [Google Scholar] [CrossRef]
- Bradley, B.A. Strong ground motion characteristics observed in the 4 September 2010 Darfield, New Zealand earthquake. Soil Dyn. Earthq. Eng. 2012, 42, 32–46. [Google Scholar] [CrossRef]
- Chen, Z.X.; Chen, G.; Liu, Y. Effects of topographic irregularity on seismic site amplification considering input signal frequency: A case study. Eng. Struct. 2024, 304, 117667. [Google Scholar] [CrossRef]
- Gasparini, D.; Vanmarcke, E.H. SIMQKE: A Program for Artificial Motion Generation; Department of Civil Engineering, Massachusetts Institute of Technology: Cambridge, MA, USA, 1976. [Google Scholar]
- Conte, J.P.; Pister, K.S.; Mahin, S.A. Nonstationary ARMA modeling of seismic motions. Soil Dyn. Earthq. Eng. 1992, 11, 411–426. [Google Scholar] [CrossRef]
- Genovese, F.; Palmeri, A. Wavelet-based generation of fully non-stationary random processes with application to seismic ground motions. Mech. Syst. Signal Process. 2025, 223, 111833. [Google Scholar] [CrossRef]
- Genovese, F.; Muscolino, G.; Palmeri, A. Effects of stochastic generation on the elastic and inelastic spectra of fully non-stationary accelerograms. Probabilistic Eng. Mech. 2023, 71, 103377. [Google Scholar] [CrossRef]
- Huang, D.; Wang, Z. Wavelet-based stochastic model for jointly simulating three-component ground motions. Bull. Seismol. Soc. Am. 2022, 112, 1483–1501. [Google Scholar] [CrossRef]
- ASCE/SEI 4-16; Seismic Analysis of Safety-Related Nuclear Structures. American Society of Civil Engineers (ASCE): New York, NY, USA, 2017. [CrossRef]
- ASCE/SEI 7-10; Minimum Design Loads for Buildings and Other Structures. American Society of Civil Engineers (ASCE): New York, NY, USA, 2013. [CrossRef]
- United States Nuclear Regulatory Commission (USNRC). Regulatory Guide 1.208: A Performance-Based Approach to Define the Site-Specific Earthquake Ground Motion; United States Nuclear Regulatory Commission: Washington, DC, USA, 2007.
- Yanni, H.; Fragiadakis, M.; Mitseas, I.P. Probabilistic generation of hazard-consistent suites of fully non-stationary seismic records. Earthq. Eng. Struct. Dyn. 2024, 53, 3140–3164. [Google Scholar] [CrossRef]
- Chandramohan, R.; Baker, J.W.; Deierlein, G.G. Impact of hazard-consistent ground motion duration in structural collapse risk assessment. Earthq. Eng. Struct. Dyn. 2016, 45, 1357–1379. [Google Scholar] [CrossRef]
- Lu, D.; Yu, X.; Jia, M.; Wang, G. Seismic risk assessment for a reinforced concrete frame designed according to Chinese codes. Struct. Infrastruct. Eng. 2014, 10, 1295–1310. [Google Scholar] [CrossRef]
- Johnson, J.J.; Gürpinar, A.; Campbell, R.D.; Kammerer, A. Seismic Design Standards and Calculational Methods in the United States and Japan; United States Nuclear Regulatory Commission, Office of Nuclear Regulatory Research: Washington, DC, USA, 2017.
- Federal Emergency Management Agency. NEHRP Recommended Seismic Provisions: Design Examples; Federal Emergency Management Agency: Washington, DC, USA, 2016.
- ASCE/SEI 7-16; Minimum Design Loads and Associated Criteria for Buildings and Other Structures. American Society of Civil Engineers (ASCE): New York, NY, USA, 2017. [CrossRef]
- ASCE/SEI 43-05; Seismic Design Criteria for Structures, Systems, and Components in Nuclear Facilities. American Society of Civil Engineers (ASCE): New York, NY, USA, 2005. [CrossRef]
- Grant, F.; Hardy, G.; Short, S. Seismic Fragility and Seismic Margin Guidance for Seismic Probabilistic Risk Assessments; Electric Power Research Institute: Palo Alto, CA, USA, 2018; p. 3002012994. [Google Scholar]
- Son, M.; Cho, C.S.; Shin, J.S.; Rhee, H.M.; Sheen, D.H. Spatiotemporal distribution of events during the first three months of the 2016 Gyeongju, Korea, earthquake sequence. Bull. Seismol. Soc. Am. 2018, 108, 210–217. [Google Scholar] [CrossRef]
- Cornell, C.A. Engineering seismic risk analysis. Bull. Seismol. Soc. Am. 1968, 58, 1583–1606. [Google Scholar] [CrossRef]
- Lin, T.; Baker, J. Probabilistic seismic hazard deaggregation of ground motion prediction models. In Proceedings of the 5th International Conference on Earthquake Geotechnical Engineering, Santiago, Chile, 10–13 January 2014; p. 12. [Google Scholar]
- Baker, J.W. An Introduction to Probabilistic Seismic Hazard Analysis (PSHA), version 1.3; White Paper: Washington, DC, USA, 2008; p. 72. [Google Scholar]
- SECY-93-087; Policy, Technical, and Licensing Issues Pertaining to Evolutionary and Advanced Light-Water Reactor (ALWR) Designs. United States Nuclear Regulatory Commission (USNRC): Washington, DC, USA, 1993.
- Pagani, M.; Monelli, D.; Weatherill, G.; Danciu, L.; Crowley, H.; Silva, V.; Henshaw, P.; Butler, L.; Nastasi, M.; Panzeri, L.; et al. OpenQuake engine: An open hazard (and risk) software for the global earthquake model. Seismol. Res. Lett. 2014, 85, 692–702. [Google Scholar] [CrossRef]
- Seo, J.M.; Rhee, H.M.; Hahm, D.G.; Kim, J.H.; Choi, I.K.; Kim, I.K. Development of Ground Motion Response Spectra Considering Site Amplification Effect; KAERI/TR-5373; Korea Atomic Energy Research Institute: Daejeon, Republic of Korea, 2013. [Google Scholar]
- Seo, J.M.; Rhee, H.M.; Choi, I.K. A study on the best estimate of seismicity parameter b-value and its application for the seismic hazard analysis of the Korean Peninsula. J. Earthq. Eng. Soc. Korea 2010, 14, 35–42. [Google Scholar] [CrossRef]
- Atkinson, G.M.; Boore, D.M. Earthquake ground-motion prediction equations for eastern North America. Bull. Seismol. Soc. Am. 2006, 96, 2181–2205. [Google Scholar] [CrossRef]
- Korea Hydro and Nuclear Power. Stress Test Report for Wolsung Nuclear Power Plant Unit 1; Final Report; Korea Hydro and Nuclear Power: Gyeongju, Republic of Korea, 2013. [Google Scholar]
- American Society of Mechanical Engineers; American National Standards Institute. Standard for Level 1/Large Early Release Frequency Probabilistic Risk Assessment for Nuclear Power Plant Applications; American Society of Mechanical Engineers: New York, NY, USA, 2008. [Google Scholar]
- Bazzurro, P.; Allin Cornell, C. Disaggregation of seismic hazard. Bull. Seismol. Soc. Am. 1999, 89, 501–520. [Google Scholar] [CrossRef]
- Kameda, H.; Ishikawa, Y.; Okumura, T.; Nakajima, M. Probabilistic Scenario earthquakes definition and engineering applications. Doboku Gakkai Ronbunshu 1997, 1997, 5–87. [Google Scholar] [CrossRef]
- United States Nuclear Regulatory Commission (USNRC). Regulatory Guide 1.165: Identification and Characterization of Seismic Sources and Determination of Safe Shutdown Earthquake Ground Motion; United States Nuclear Regulatory Commission: Washington, DC, USA, 1997.
- Baker, J.W. Conditional mean spectrum: Tool for ground-motion selection. J. Struct. Eng. 2011, 137, 322–331. [Google Scholar] [CrossRef]
- Hartzell, S.H. Earthquake aftershocks as Green’s functions. Geophys. Res. Lett. 1978, 5, 1–4. [Google Scholar] [CrossRef]
- Irikura, K. Prediction of strong acceleration motion using empirical Green’s function. In Proceedings of the 7th Japan Earthquake Engineering Symposium, Tokyo, Japan, 10–12 December 1986; Volume 151, pp. 151–156. [Google Scholar]
- Aki, K. Scaling law of seismic spectrum. J. Geophys. Res. 1967, 72, 1217–1231. [Google Scholar] [CrossRef]
- Kamae, K.; Irikura, K.; Pitarka, A. A technique for simulating strong ground motion using hybrid Green’s function. Bull. Seismol. Soc. Am. 1998, 88, 357–367. [Google Scholar] [CrossRef]
- Somerville, P. Scaling relations between seismic moment and rupture area of earthquakes in stable continental regions. Earthq. Spectra 2021, 37, 1534–1549. [Google Scholar] [CrossRef]
- Al Atik, L.; Abrahamson, N. An improved method for nonstationary spectral matching. Earthq. Spectra 2010, 26, 601–617. [Google Scholar] [CrossRef]
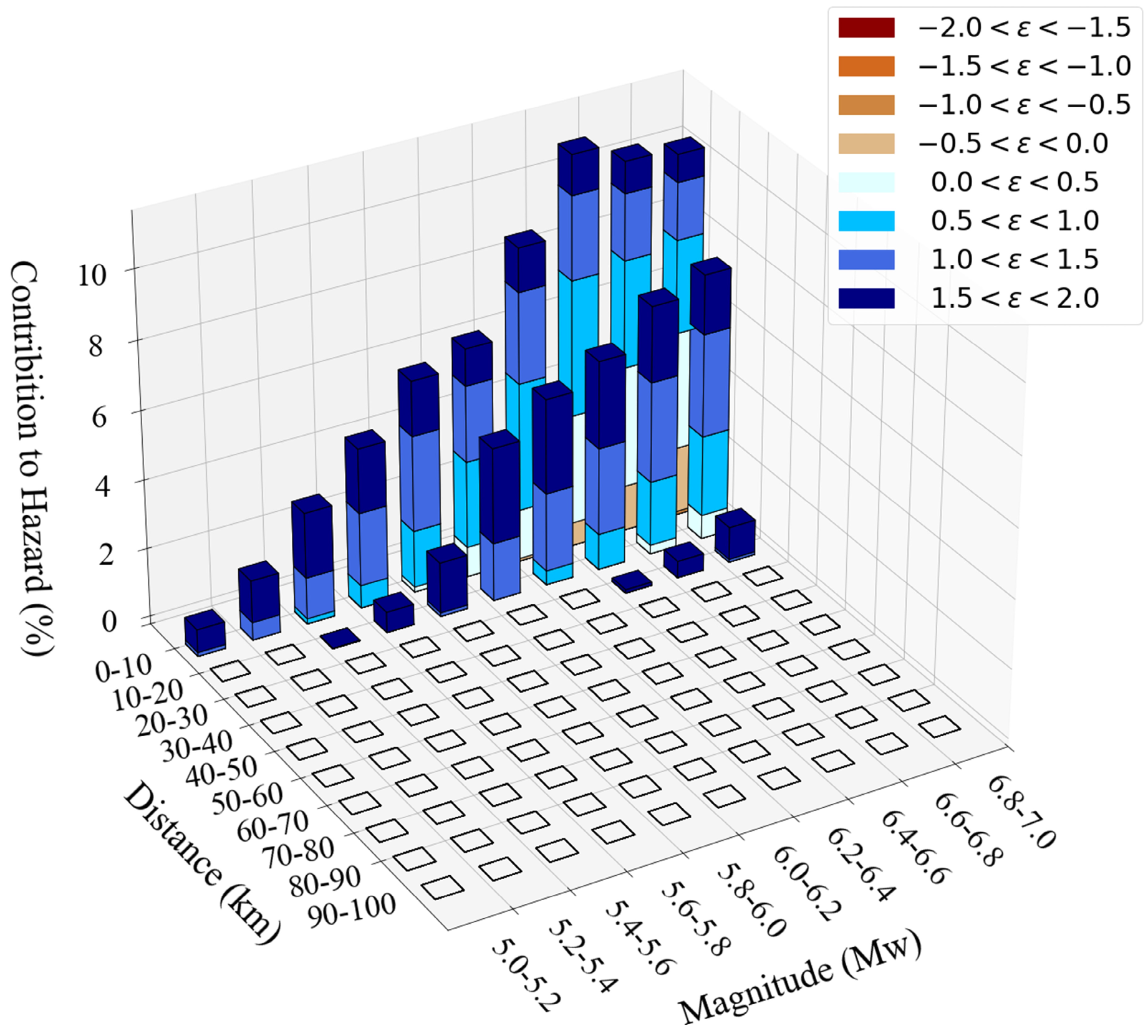



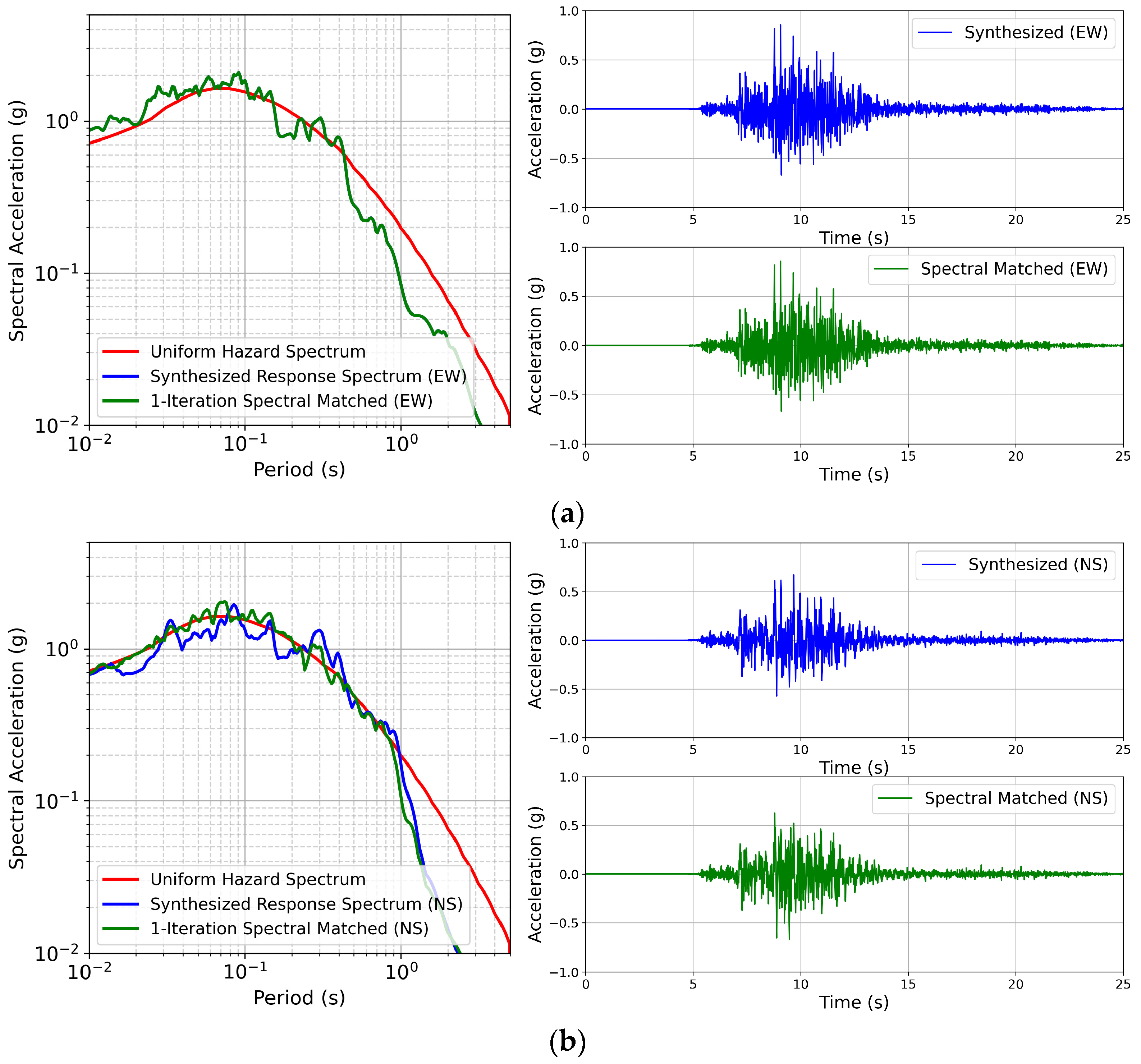

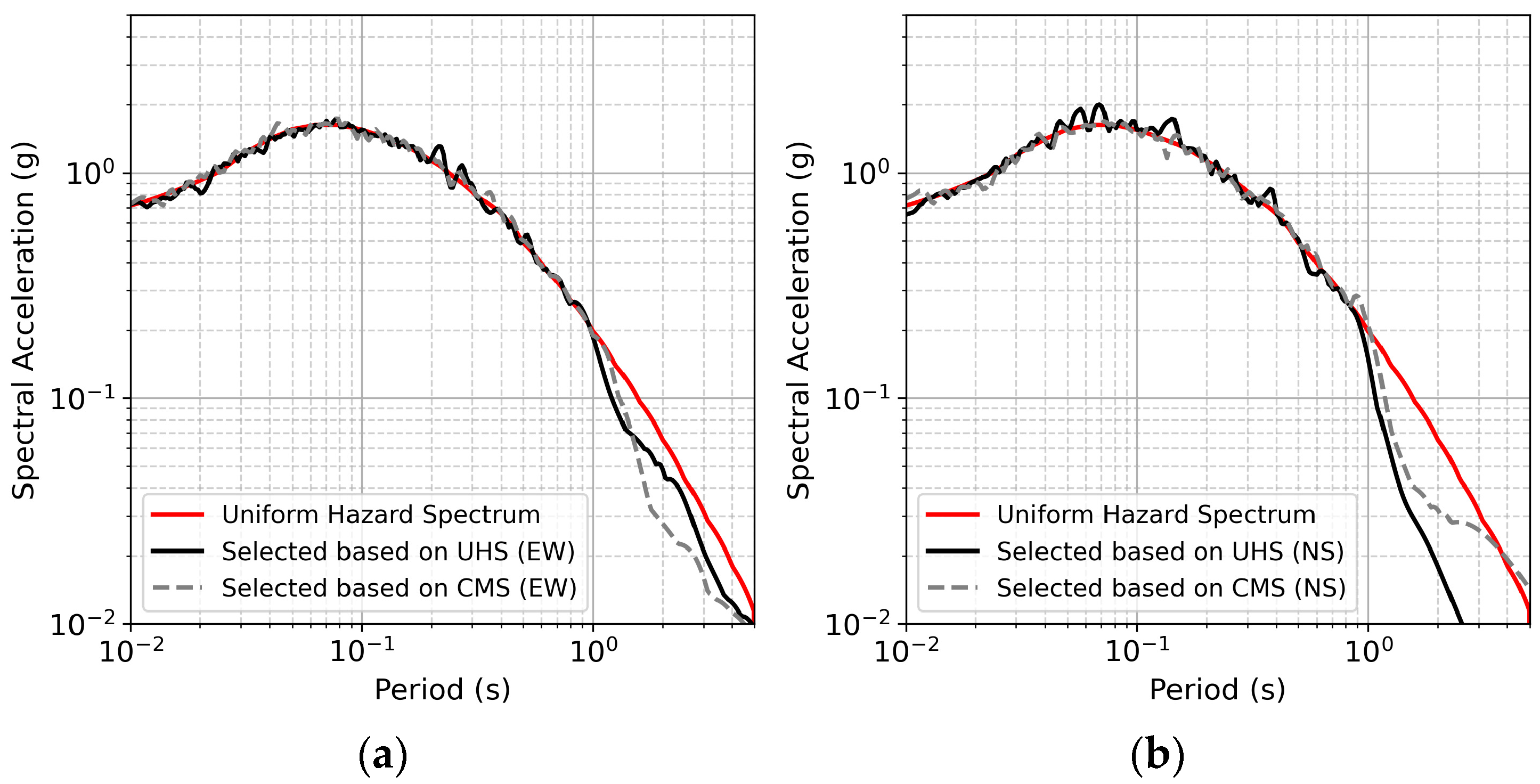
| Parameter | Value |
|---|---|
| Magnitude bin (Mw) | 6.4–6.6 |
| Distance * bin (km) | 0–10 |
| bin | 0.5–1.0 |
| Contribution to Hazard (%) | 3.93 |
| Parameter | 912 Gyeongju Earthquake | EGFM Finite Fault Model | |
|---|---|---|---|
| Magnitude | 5.5 Mw | 6.5 Mw | |
| Focal Mechanism | Strike | 26° | 26° |
| Dip | 67° | 67° | |
| Rake | 175° | 175° | |
| Fault dimension | Width | 4.0 km | 12.0 km |
| Length | 4.0 km | 16.0 km | |
| Hypocentral Depth | 12.0 km | (Min) 8.5 km, (Max) 12.2 km | |
| S-wave Velocity | 3.5 km/s | 3.5 km/s | |
| Rupture Velocity | 2.8 km/s | 2.8 km/s | |
| Rupture Starting Point | Horizontal Direction Components | Virtual Seismic Station Number | ||||
|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | ||
| 1 | EW | 0.2048 | 0.1083 | 0.2539 | 0.0842 | 0.138 |
| NS | 0.1048 | 0.094 | 0.1175 | 0.0648 | 0.0604 | |
| 2 | EW | 0.1787 | 0.1291 | 0.2327 | 0.1002 | 0.0903 |
| NS | 0.1413 | 0.1412 | 0.2382 | 0.0548 | 0.0686 | |
| 3 | EW | 0.0837 | 0.2154 | 0.2976 | 0.125 | 0.2029 |
| NS | 0.0381 | 0.1698 | 0.2304 | 0.072 | 0.0672 | |
| 4 | EW | 0.2806 | 0.3341 | 0.5171 | 0.2514 | 0.1657 |
| NS | 0.2685 | 0.3338 | 0.5095 | 0.1642 | 0.0962 | |
| 5 | EW | 0.2082 | 0.0998 | 0.2416 | 0.0833 | 0.1183 |
| NS | 0.1179 | 0.0801 | 0.1139 | 0.0715 | 0.0756 | |
| 6 | EW | 0.1604 | 0.128 | 0.244 | 0.1056 | 0.0954 |
| NS | 0.1490 | 0.1198 | 0.2159 | 0.058 | 0.0574 | |
| 7 | EW | 0.1012 | 0.2263 | 0.3249 | 0.1149 | 0.1990 |
| NS | 0.0294 | 0.2725 | 0.2681 | 0.0782 | 0.0715 | |
| 8 | EW | 0.2753 | 0.3003 | 0.5439 | 0.2181 | 0.1645 |
| NS | 0.2056 | 0.2602 | 0.5095 | 0.1211 | 0.0788 | |
| Rupture Starting Point | Horizontal Direction Components | Virtual Seismic Station Number | ||||
|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | ||
| 1 | EW | 0.1514 | 0.0857 | 0.1932 | 0.1122 | 0.1605 |
| NS | 0.0515 | 0.0552 | 0.0697 | 0.0823 | 0.0738 | |
| 2 | EW | 0.1469 | 0.0973 | 0.1661 | 0.1209 | 0.1202 |
| NS | 0.0654 | 0.0743 | 0.132 | 0.0619 | 0.0588 | |
| 3 | EW | 0.1036 | 0.1554 | 0.2099 | 0.1441 | 0.2932 |
| NS | 0.0434 | 0.0801 | 0.1222 | 0.0476 | 0.1317 | |
| 4 | EW | 0.2004 | 0.2161 | 0.3568 | 0.1777 | 0.1586 |
| NS | 0.1466 | 0.1974 | 0.3274 | 0.0803 | 0.0558 | |
| 5 | EW | 0.1613 | 0.0837 | 0.1849 | 0.1021 | 0.1272 |
| NS | 0.0569 | 0.055 | 0.0689 | 0.0869 | 0.085 | |
| 6 | EW | 0.1283 | 0.0997 | 0.1734 | 0.1305 | 0.1147 |
| NS | 0.0717 | 0.0612 | 0.1212 | 0.0743 | 0.0565 | |
| 7 | EW | 0.1406 | 0.154 | 0.2185 | 0.1168 | 0.2883 |
| NS | 0.0501 | 0.1671 | 0.15 | 0.0496 | 0.1411 | |
| 8 | EW | 0.1953 | 0.1952 | 0.3766 | 0.1695 | 0.1555 |
| NS | 0.1075 | 0.1436 | 0.3254 | 0.0532 | 0.0452 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ji, H.Y.; Kim, J.H. Generating Input Ground Motions for Seismic Risk Assessment Using Recorded Ground Motions from the Moderate Magnitude Earthquake. Appl. Sci. 2025, 15, 640. https://doi.org/10.3390/app15020640
Ji HY, Kim JH. Generating Input Ground Motions for Seismic Risk Assessment Using Recorded Ground Motions from the Moderate Magnitude Earthquake. Applied Sciences. 2025; 15(2):640. https://doi.org/10.3390/app15020640
Chicago/Turabian StyleJi, Hae Yeon, and Jung Han Kim. 2025. "Generating Input Ground Motions for Seismic Risk Assessment Using Recorded Ground Motions from the Moderate Magnitude Earthquake" Applied Sciences 15, no. 2: 640. https://doi.org/10.3390/app15020640
APA StyleJi, H. Y., & Kim, J. H. (2025). Generating Input Ground Motions for Seismic Risk Assessment Using Recorded Ground Motions from the Moderate Magnitude Earthquake. Applied Sciences, 15(2), 640. https://doi.org/10.3390/app15020640







