Analysis of a Tracked In-Pipe Robot’s Obstacle-Crossing Performance
Abstract
1. Introduction
2. Materials and Methods
3. Results
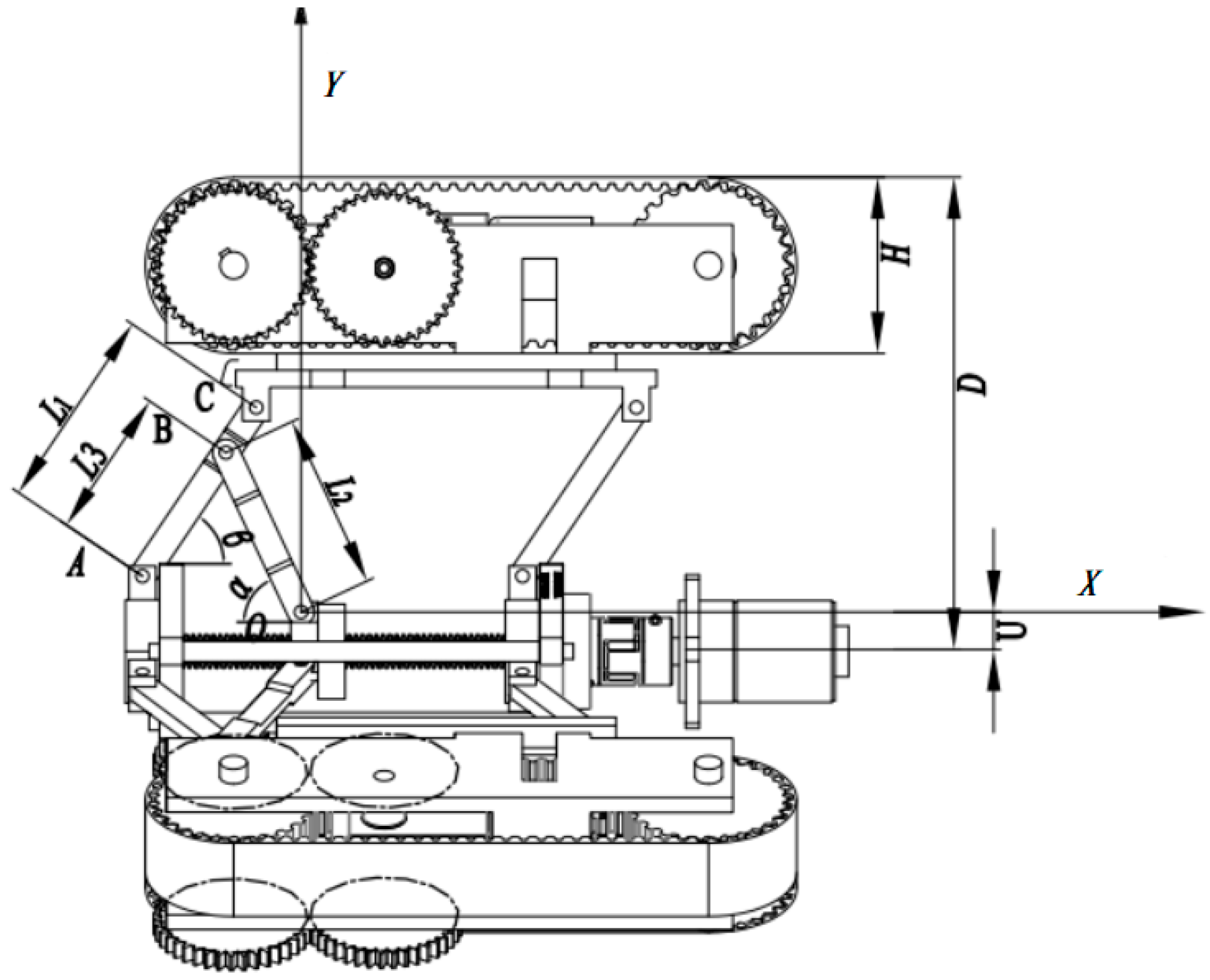
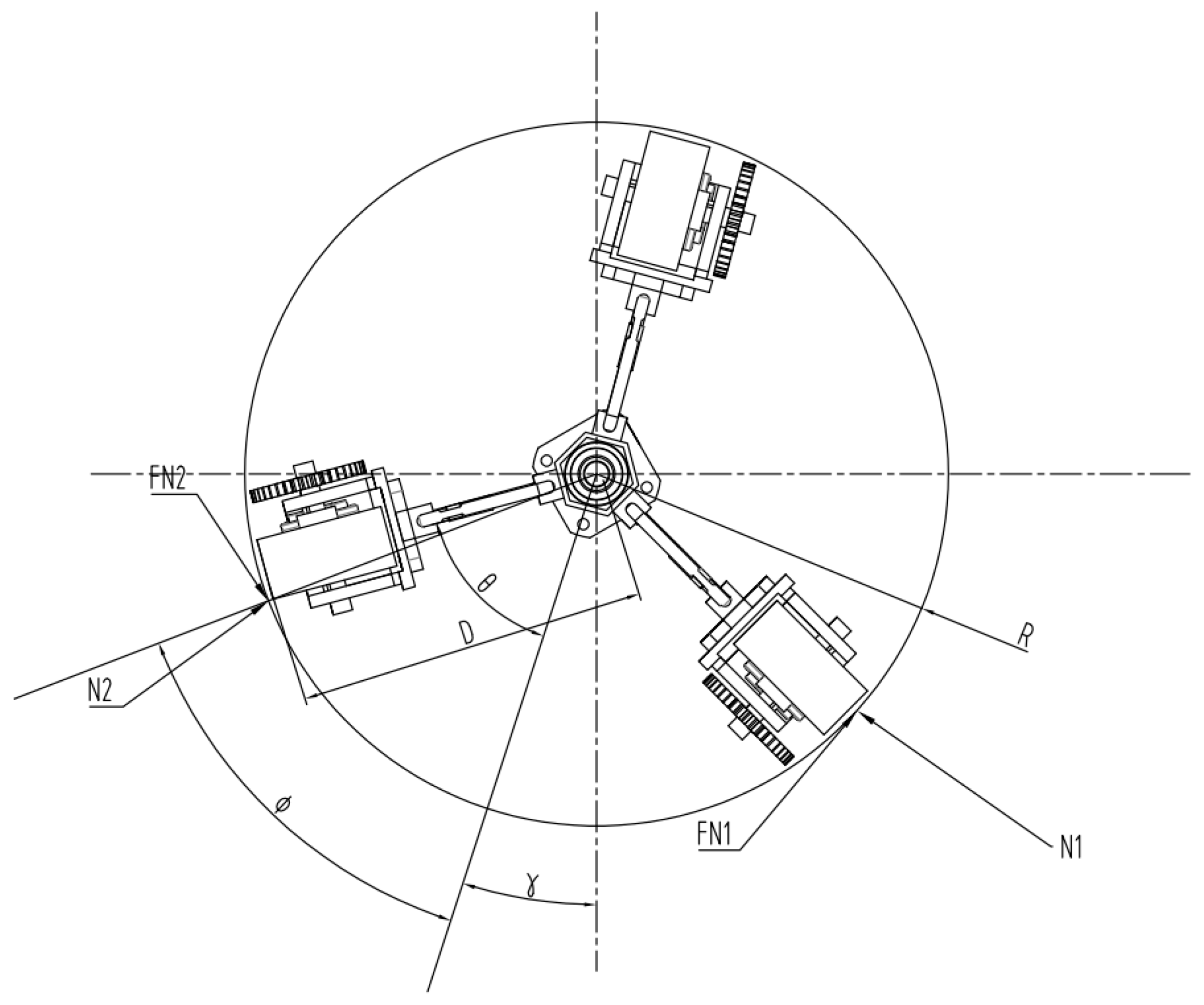
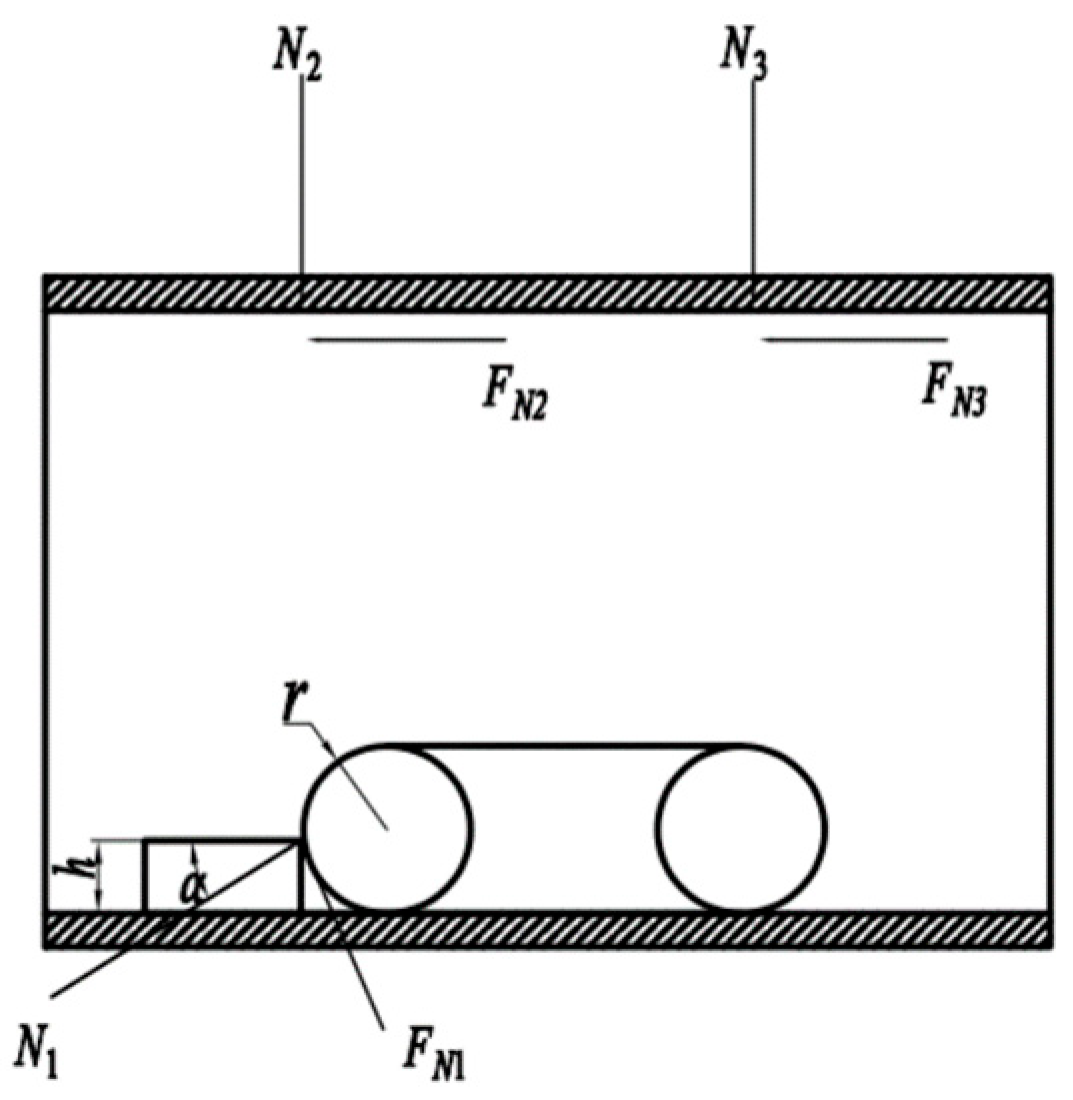
3.1. Force Analysis of Pipe Diameter Adaptation Mechanism
3.2. Analysis of the Robot’s Ability to Pass Obstacles
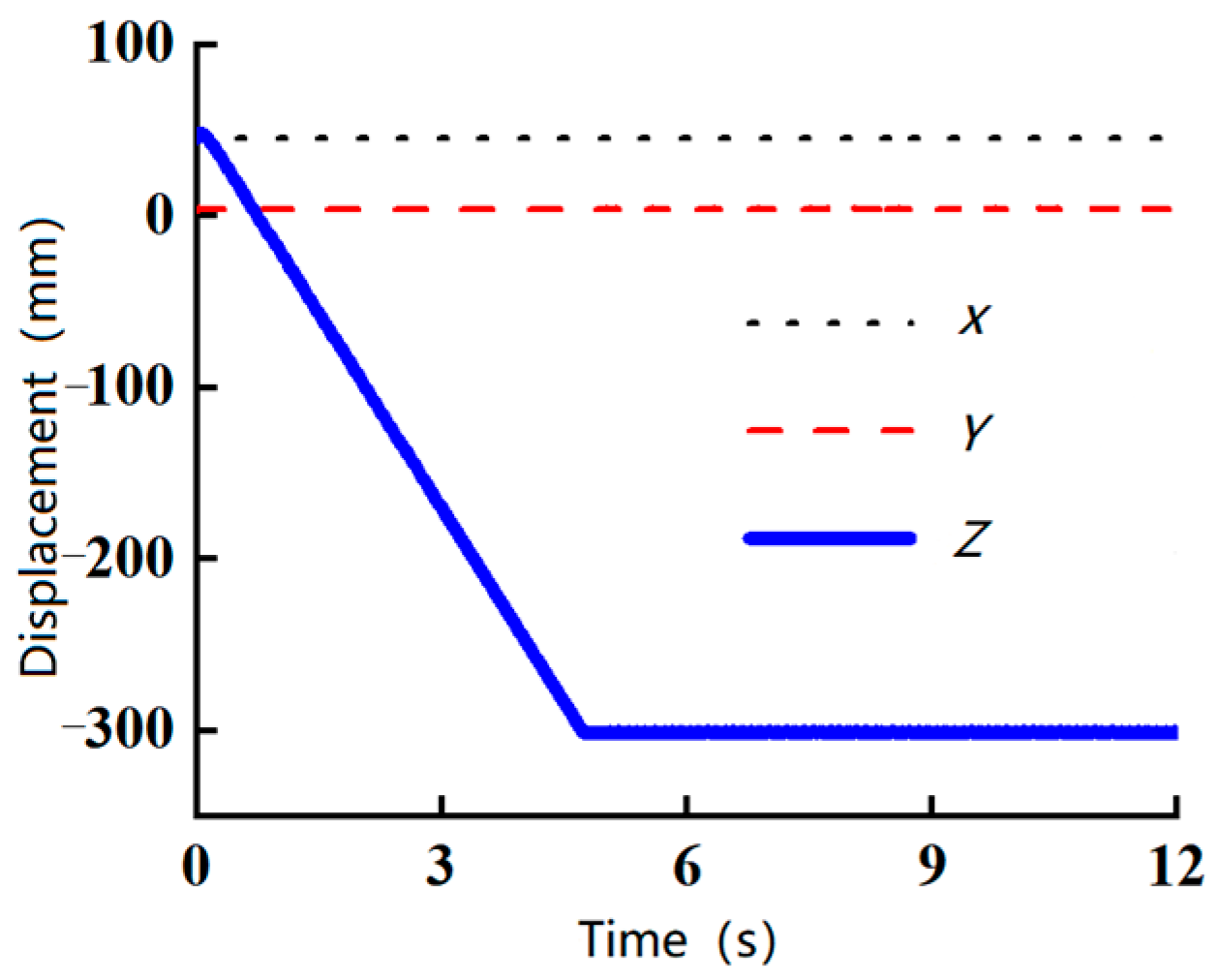
3.3. Simulation Analysis of Passing an Obstacle
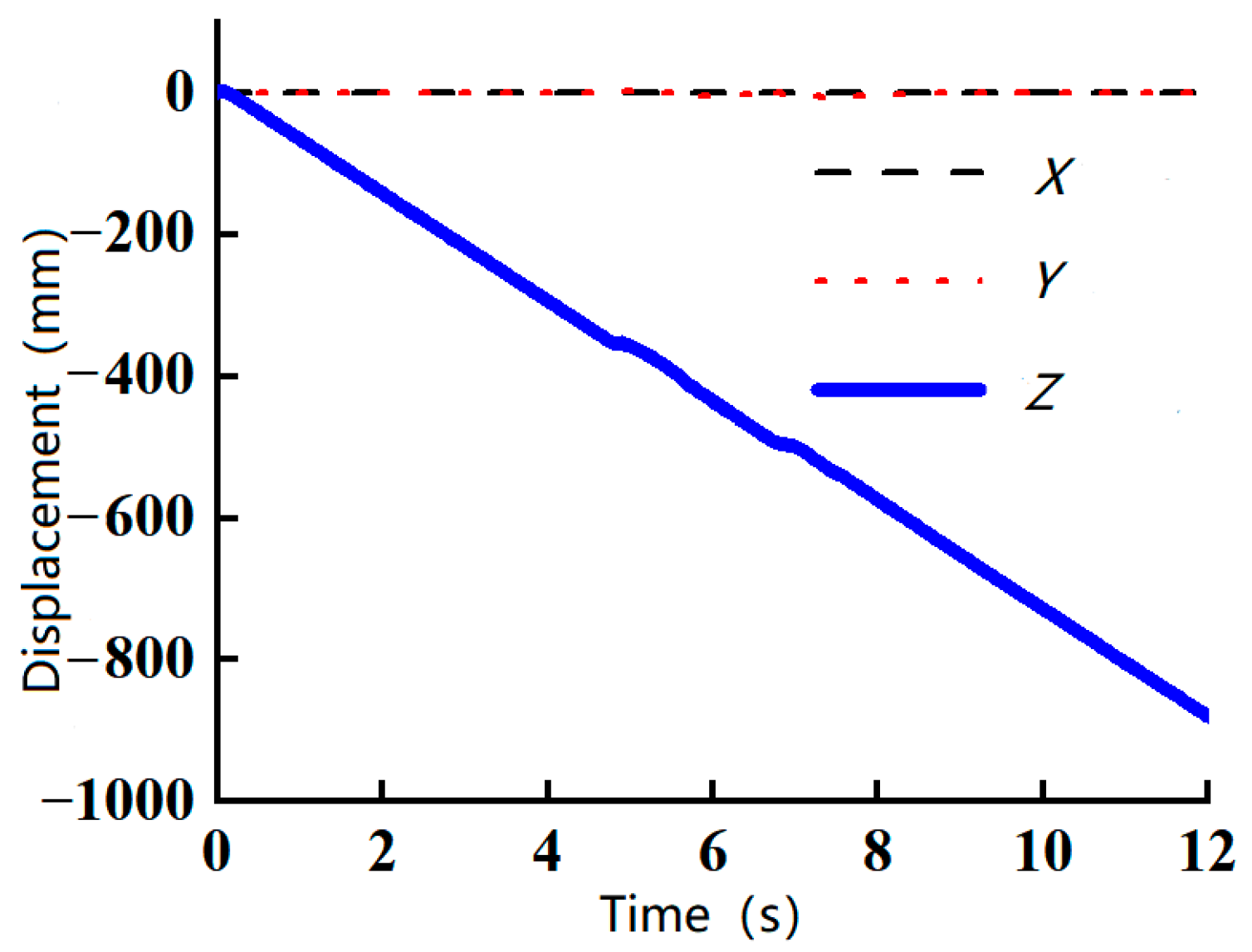
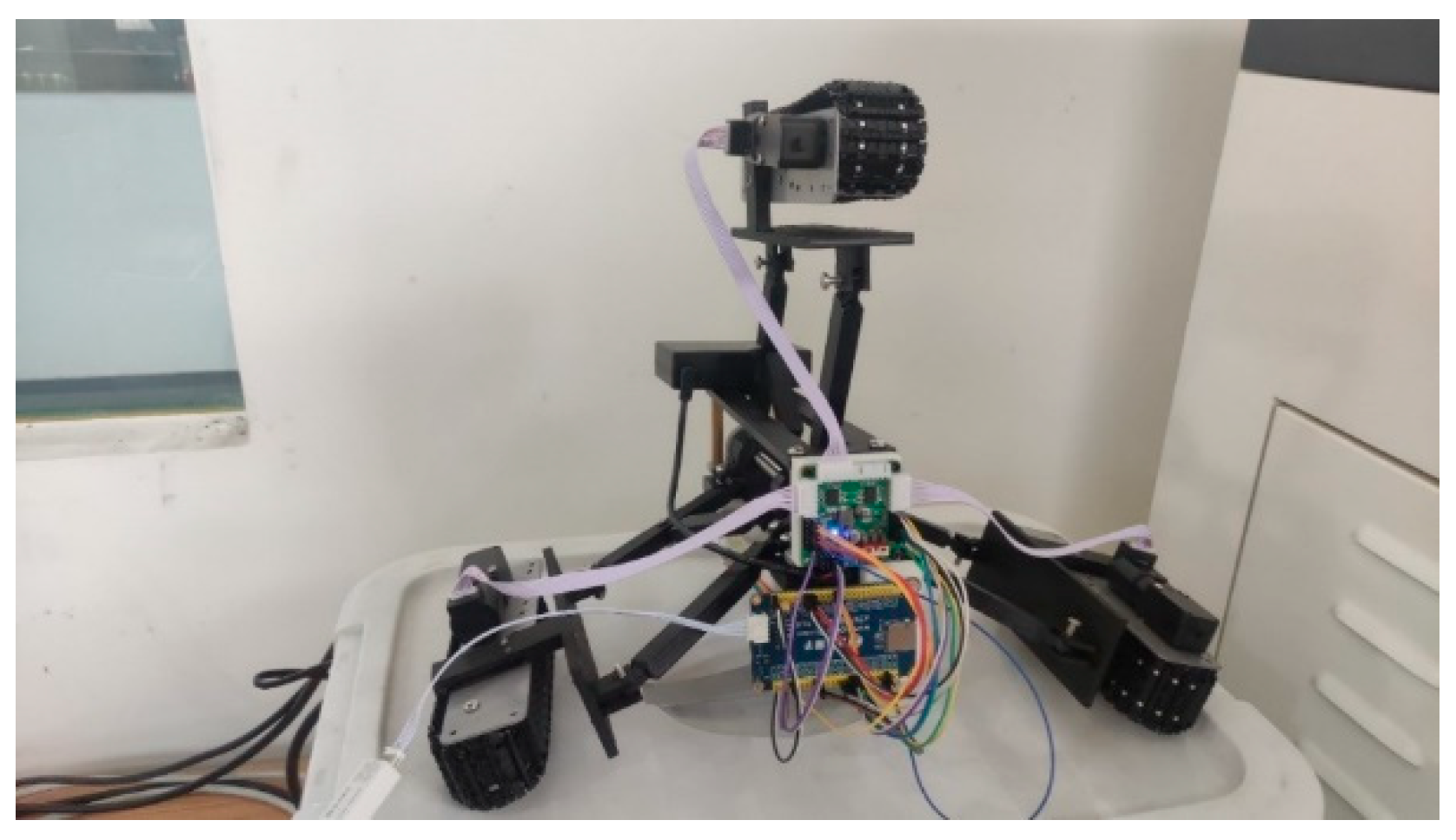
3.4. Prototype Testing and Analysis of the Robot
3.4.1. Experimental Purpose
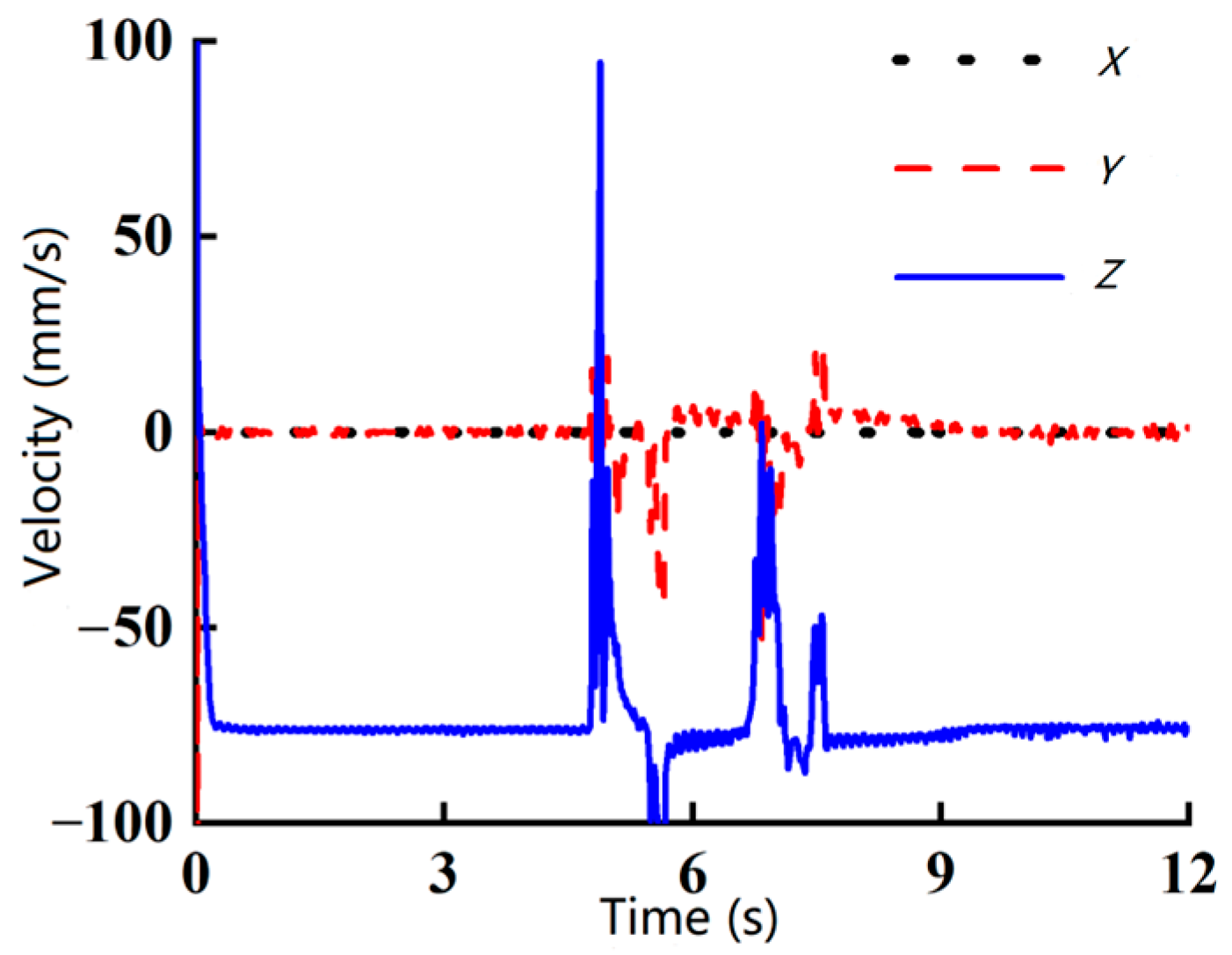
3.4.2. Results of Prototype Testing
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ma, S.; Lyu, S.; Zhan, Q. Research on the Explosive Characteristics of Oil and Gas Mixture in Urban Drainage Pipeline. Chem. Eng. Trans. 2017, 62, 1399–1404. [Google Scholar] [CrossRef]
- Gao, Z.; Zhang, H.; Gao, P. New Progress in the Construction of Oil and Gas Pipelines in China in 2022. Int. Pet. Econ. 2023, 31, 16–23. [Google Scholar]
- Zhang, L. The “13th Five-Year” Energy Plan Requires Optimization in Three Aspects. Min. Mach. 2016, 44, 112–113. [Google Scholar]
- Gan, X.; Xu, B.; Dong, S. Development Status Quo of Pipeline Robot. Technol. Appl. Robot. 2003, 6, 2–5. [Google Scholar]
- Qi, A. Analysis of the Current Situation and Problems of Oil and Gas Pipeline Transportation Development in China. Int. Pet. Econ. 2009, 17, 1–2. [Google Scholar]
- Li, H.; Li, R.; Zhang, J.; Zhang, P. Development of a Pipeline Inspection Robot for the Standard Oil Pipeline of China National Petroleum Corporation. Appl. Sci. 2020, 10, 2853. [Google Scholar] [CrossRef]
- Tavakoli, M.; Marques, L.; Almeida, A.T.D. Development of an Industrial Pipeline Inspection Robot. Ind. Robot. 2010, 37, 309–322. [Google Scholar] [CrossRef]
- Chattopadhyay, P.; Ghoshal, S.; Majumder, A.; Dikshit, H. Locomotion Methods of Pipe Climbing Robots: A Review. J. Eng. Sci. Technol. Rev. 2018, 11, 154–165. [Google Scholar] [CrossRef]
- Xu, Z.; Yang, Z.; Zu, J. Impedance matching circuit for synchronous switch harvesting on inductor interface. In Proceedings of the 2015 IEEE International Conference on Mechatronics and Automation (ICMA), Beijing, China, 2–5 August 2015; IEEE: New York City, NY, USA, 2015. ISBN 9781479970988. [Google Scholar]
- Roslin, N.S.; Anuar, A.; Jalal, M.F.A.; Sahari, K.S.M. A Review: Hybrid Locomotion of in-Pipe Inspection Robot. Procedia Eng. 2012, 41, 1456–1462. [Google Scholar] [CrossRef]
- Wang, W.; Dong, W.; Su, Y.; Wu, D.; Du, Z. Development of Search-and-Rescue Robots for Underground Coal Mine Applications. J. Field Robot. 2014, 31, 386–407. [Google Scholar] [CrossRef]
- Yan, H.; Wang, L.; Li, P.; Wang, Z.; Yang, X.; Hou, X. Research on Passing Ability and Climbing Performance of Pipeline Plugging Robots in Curved Pipelines. IEEE Access 2020, 8, 173666–173680. [Google Scholar] [CrossRef]
- Wang, W.; Wang, X.; Zheng, G.; Chen, R.; Yuan, Z.; Huang, J.; Wu, K.; Bao, G. A Modular Soft Pipe-Climbing Robot with High Maneuverability. In IEEE/ASME Transactions on Mechatronics; IEEE: New York, NY, USA, 2024; pp. 4734–4743. [Google Scholar] [CrossRef]
- Zhao, Q.; Jiang, Z.; Chu, H.K. A Soft-Rigid Air-Propelled Pipe-Climbing Robot. In Proceedings of the IEEE International Conference on Robotics and Automation, Hong Kong, 21–23 April 2021; Institute of Electrical and Electronics Engineers Inc.: New York City, NY, USA, 2021; Volume 2021, pp. 11850–11855. [Google Scholar]
- Kim, J.H.; Lee, J.C.; Choi, Y.R. PiROB: Vision-Based Pipe-Climbing Robot for Spray-Pipe Inspection in Nuclear Plants. Int. J. Adv. Robot. Syst. 2018, 15, 1729881418817974. [Google Scholar] [CrossRef]
- Li, J.; Huang, F.; Tu, C.; Tian, M.; Wang, X. Elastic Obstacle-Surmounting Pipeline-Climbing Robot with Composite Wheels. Machines 2022, 10, 874. [Google Scholar] [CrossRef]
- Han, S.; Ahn, J.; Moon, H. Remotely Controlled Prehensile Locomotion of a Two-Module 3D Pipe-Climbing Robot. J. Mech. Sci. Technol. 2016, 30, 1875–1882. [Google Scholar] [CrossRef]
- Lee, S.H. Design of the Out-Pipe Type Pipe Climbing Robot. Int. J. Precis. Eng. Manuf. 2013, 14, 1559–1563. [Google Scholar] [CrossRef]
- Tu, Q.; Liu, Q.; Ren, T.; Li, Y. Obstacle Crossing and Traction Performance of Active and Passive Screw Pipeline Robots. J. Mech. Sci. Technol. 2019, 33, 2417–2427. [Google Scholar] [CrossRef]
- Ylikorpi, T.J.; Halme, A.J.; Forsman, P.J. Dynamic Modeling and Obstacle-Crossing Capability of Flexible Pendulum-Driven Ball-Shaped Robots. Robot. Auton. Syst. 2017, 87, 269–280. [Google Scholar] [CrossRef]
- Toussaint, K.; Pouliot, N.; Montambault, S. Transmission Line Maintenance Robots Capable of Crossing Obstacles: State-of-the-Art Review and Challenges Ahead. J. Field Robot. 2009, 26, 477–499. [Google Scholar] [CrossRef]
- Yu, J.; Zhang, D.; Dai, Y. Analysis of the Climbing Characteristics of a Crawler-Type Oil and Gas Pipeline Inspection Robot. Tools Hydraul. 2023, 51, 57–61. [Google Scholar]
- Chen, P.; Gao, F. Mechanism Design and Motion Analysis of Circular Pipeline Robot. Heavy Mach. 2023, 2, 103–108. [Google Scholar] [CrossRef]
- Zhang, X.; Fu, K.; Chen, M. Dynamic Evolution Law and Vibration Reduction Analysis of a Differential Pressure Type Multi-Section Series Pipeline Robot When Crossing Obstacles. J. Mech. Eng. 2024, 60, 1–8. [Google Scholar]
- Zhang, Y.; Dong, L.; Huan, R. Design and Kinematic Analysis of Crawler-Type Pipeline Endoscope Robots for Wind Turbine Blades. China Mech. Eng. 2021, 32, 1884–1889. [Google Scholar]
- Liu, D.; Liu, J. Asymmetric Inertia Driving System for In-Pipe Robots and Its Dynamics Characteristics. China Mech. Eng. 2020, 31, 2189–2195+2205. [Google Scholar]
- Yue, M.; Deng, Z. Dynamic Modeling and Optimal Controller Design of a Spherical Robot in Climbing State. Jixie Gongcheng Xuebao/J. Mech. Eng. 2009, 45, 46–51. [Google Scholar] [CrossRef]
- Tang, D.; Li, Q.; Liang, T.; Jiang, S.; Deng, Z. Mechanical Self-Adaptive Drive Technology of Triaxial Differential Pipe-Robot. J. Mech. Eng. 2008, 30, 128–133. [Google Scholar] [CrossRef]
- Qi, L.; Zhang, T.; Xu, K.; Pan, H.; Zhang, Z.; Yuan, Y. A Novel Terrain Adaptive Omni-Directional Unmanned Ground Vehicle for Underground Space Emergency: Design, Modeling and Tests. Sustain. Cities Soc. 2021, 65, 102621. [Google Scholar] [CrossRef]
- Verma, M.S.; Ainla, A.; Yang, D.; Harburg, D.; Whitesides, G.M. A Soft Tube-Climbing Robot. Soft Robot. 2018, 5, 133–137. [Google Scholar] [CrossRef] [PubMed]
- Kanada, A.; Giardina, F.; Howison, T.; Mashimo, T.; Iida, F. Reachability Improvement of a Climbing Robot Based on Large Deformations Induced by Tri-Tube Soft Actuators. Soft Robot. 2019, 6, 483–494. [Google Scholar] [CrossRef]
- Aracil, R.; Saltarén, R.; Reinoso, O. Parallel Robots for Autonomous Climbing along Tubular Structures. Robot. Auton. Syst. 2003, 42, 125–134. [Google Scholar] [CrossRef]
- Ling, Z.; Chen, Y.; Tang, J.; Zheng, M. Development and Design of Self-Adaptive Variable Diameter Robot for In-Pipe Inspection. In Proceedings of the IOP Conference Series: Materials Science and Engineering, Chennai, India, 16–17 September 2020; Institute of Physics Publishing: Bristol, UK, 2020; Volume 782. [Google Scholar]

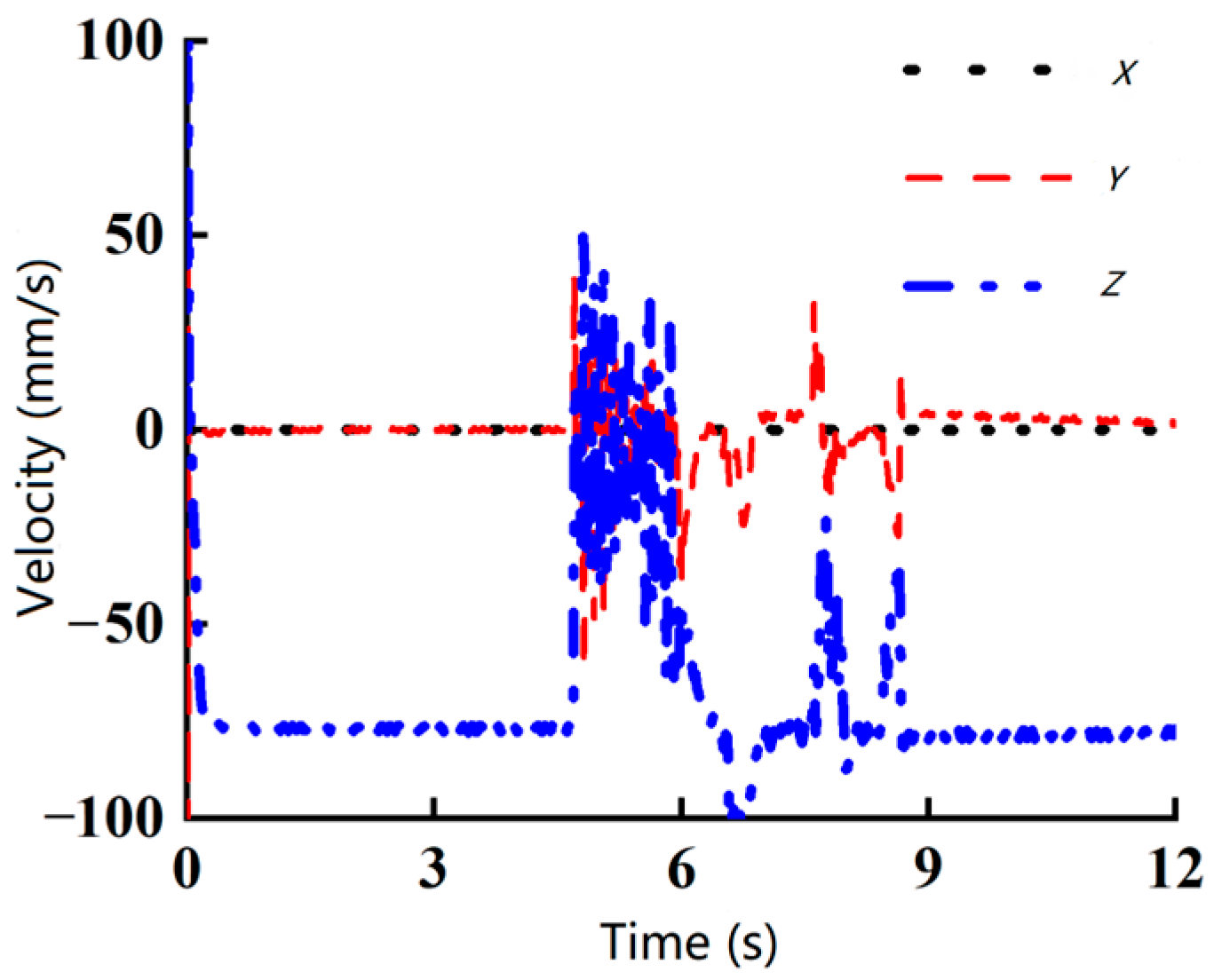
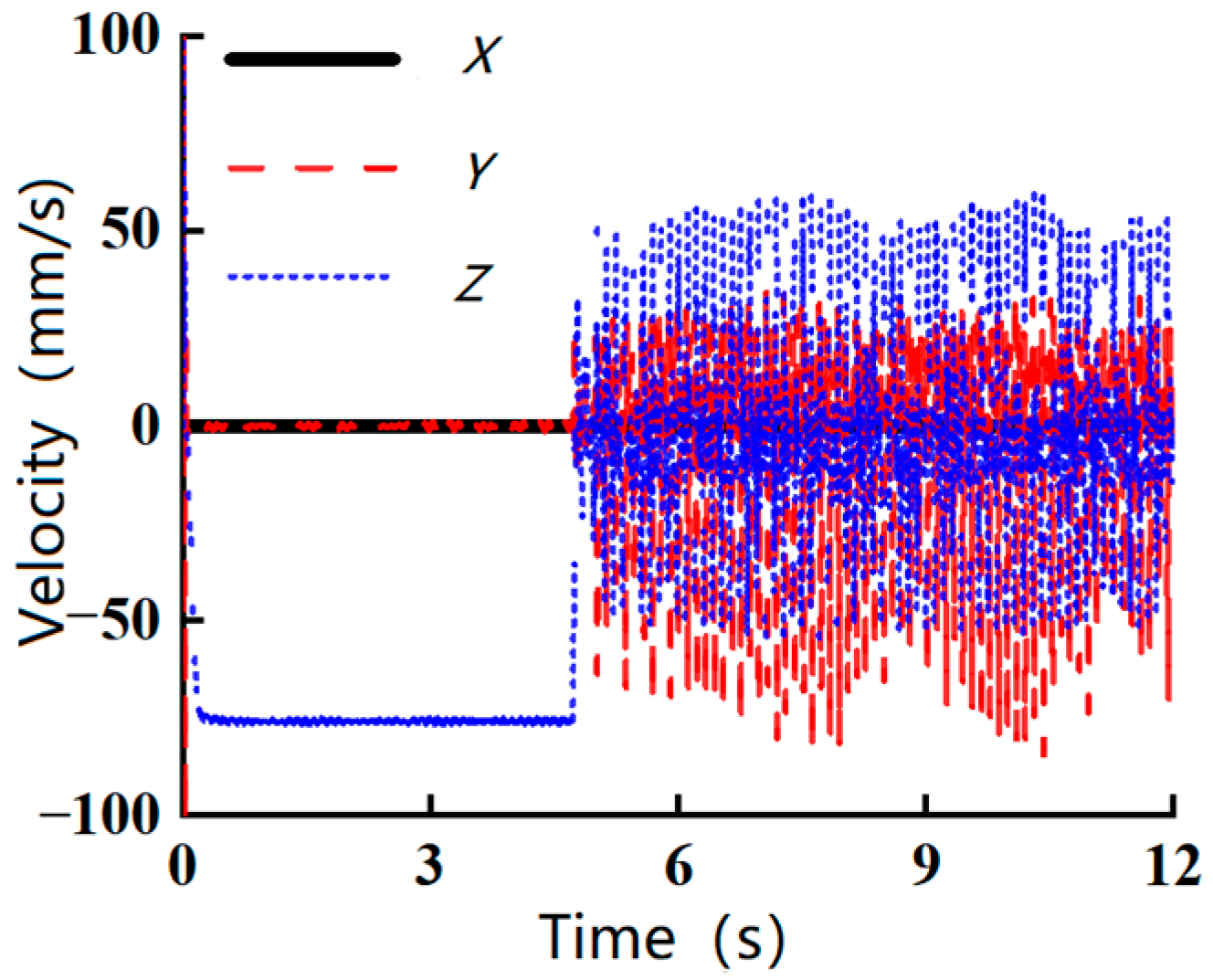
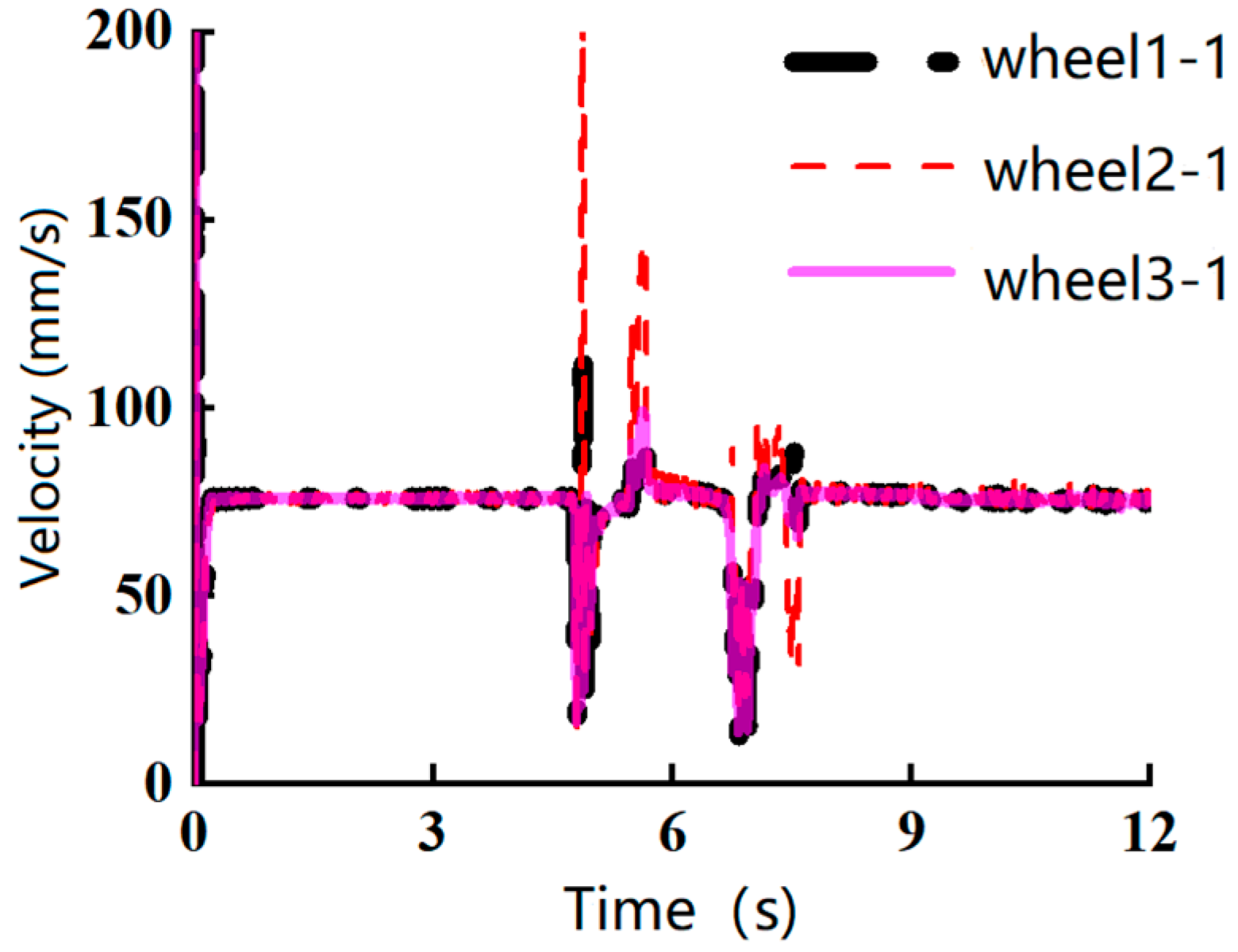

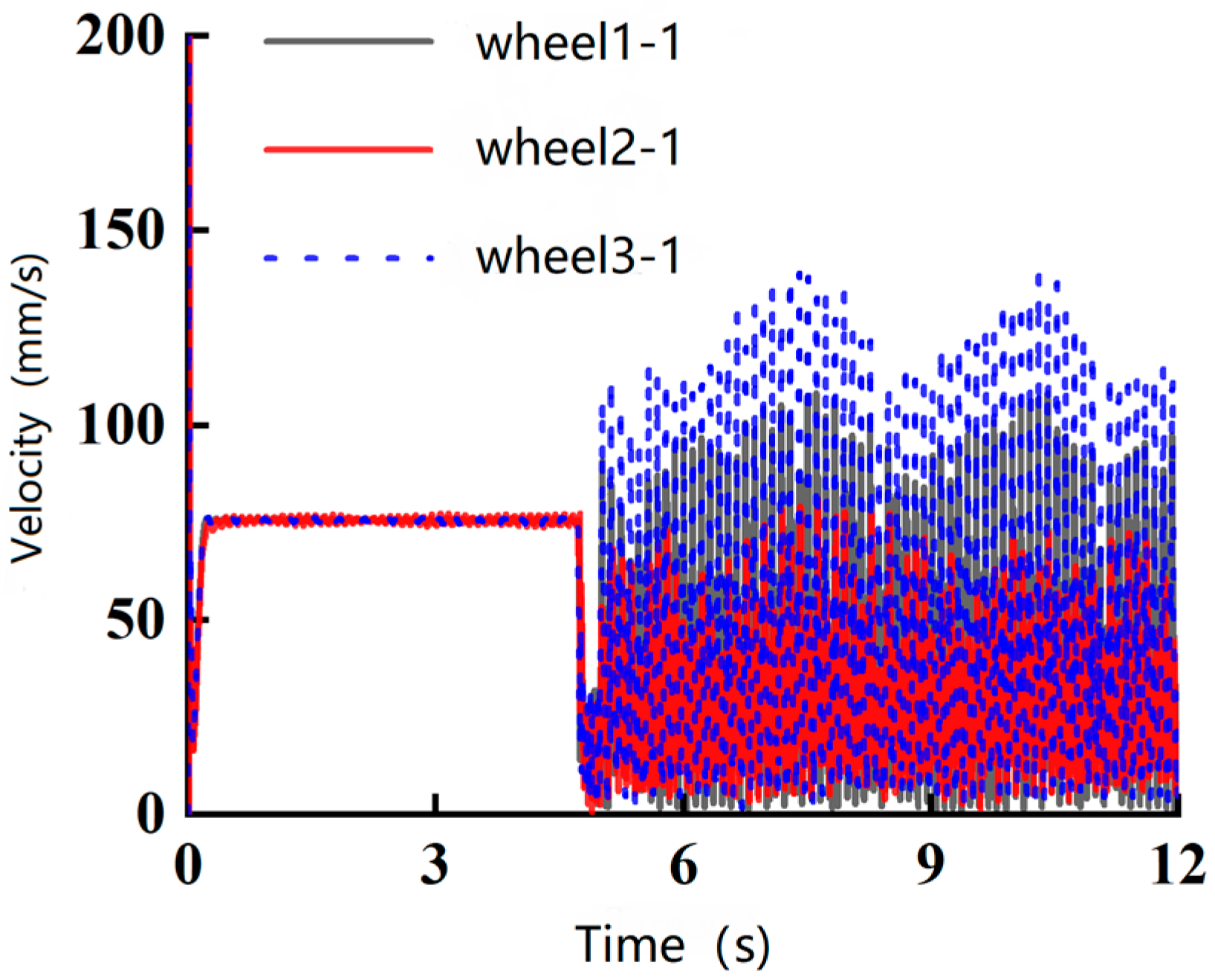

| Serial Number | Height (mm) | Passing Period (s) | Velocity (m/min) | Growth Rate (%) |
|---|---|---|---|---|
| 1 | 0 | 4 | 4.2 | \ |
| 2 | 5 | 5 | 0.24 | 25 |
| 3 | 7.5 | 7 | 0.15 | 40 |
| 4 | 7.8 | 15 | 0.045 | 114.29 |
| 5 | 7.6 | 7.5 | 0.12 | 7.14 |
| 6 | 7.7 | 8.5 | 0.08 | 13.33 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, G.; Ye, L.; Liu, P.; Li, F.; Zhu, X. Analysis of a Tracked In-Pipe Robot’s Obstacle-Crossing Performance. Appl. Sci. 2025, 15, 5905. https://doi.org/10.3390/app15115905
Liu G, Ye L, Liu P, Li F, Zhu X. Analysis of a Tracked In-Pipe Robot’s Obstacle-Crossing Performance. Applied Sciences. 2025; 15(11):5905. https://doi.org/10.3390/app15115905
Chicago/Turabian StyleLiu, Guodong, Linzheng Ye, Peide Liu, Fei Li, and Xijing Zhu. 2025. "Analysis of a Tracked In-Pipe Robot’s Obstacle-Crossing Performance" Applied Sciences 15, no. 11: 5905. https://doi.org/10.3390/app15115905
APA StyleLiu, G., Ye, L., Liu, P., Li, F., & Zhu, X. (2025). Analysis of a Tracked In-Pipe Robot’s Obstacle-Crossing Performance. Applied Sciences, 15(11), 5905. https://doi.org/10.3390/app15115905






