Flexural Buckling Failure of Rock Slopes: A Review of Influencing Factors and Analysis Methods

Abstract

The flexural buckling failure is a relatively common instability phenomenon in rock slopes both at the small and large scale. It poses a serious threat to the normal operation and maintenance of nearby infrastructure and the life and property safety of surrounding residents. A profound understanding of the deformation and failure mechanism of flexural buckling, as well as the development of quantitative approaches for buckling stability analyses, are of great significance for the risk identification and control of buckling landslides. This study combines the literature relevant to flexural buckling of rock slopes by systematically reviewing the research progress and trends from 1970 to 2023, following the research path of “triggering mechanism → analysis methods”. Based on this proposition, the intrinsic and triggering factors that influence the deformation process of flexural buckling failure in dip rock slopes are detailed and clarified. Then, the main progress achieved in physical, analytical, and numerical modelling regarding the stability and run-out analysis of buckling landslides is comprehensively introduced. Finally, this study provides some outlooks for future research and practice in the field of buckling landslides.

1. Introduction

Under internal and external forces, layered rock masses in the upper portion of a dip slope may slide along weak structural planes, thus compressing the stratified rock masses in the lower portion and causing them to bend and bulge. When bending proceeds, the breaking of rock layers due to shearing or tension may occur at the slope toe, resulting successive failure [1,2,3,4,5]. The deformation and failure mechanism of flexural buckling is quite complex, involving the action of interlayer sliding in the active zone and bending uplift in the passive zone, as well as the dynamic adjustment of the boundary between the active and passive zones. Although such landslides usually experience a long period of time-dependent deformation before failure, they may cause significant casualties and economic damage if timely countermeasures are not adopted [2,6,7,8,9,10]. For example, the Athytos highway landslide in the Central Macedonia region of Greece cut off the main road connecting the Chalkidiki peninsula with the mainland [6]. The Yokawatashi landslide in Niigata, Japan, resulted in three people missing and severe damage to several railway lines [11,12]. The Shiaolin ancient landslide in southern Taiwan led to 397 fatalities and hundreds of millions in economic losses [4]. The largest Dhampu–Chhoya landslide occurred along a 20 km stretch of the Kali Gandaki River (one of the largest rivers traversing southward from Tibet to India through the High Himalayas), temporarily damming the river and causing dramatic morphological changes [13]. With an estimated debris volume of 4.9 × 10⁷ m³, the Xiaguiwa landslide impacted the Jinsha River, SE Tibetan Plateau, forming a dam over 60 m high that temporarily blocked the river [14,15,16]. Thus, the research on the deformation and failure mechanism of buckling landslides under the coupled action of internal and external forces, as well as the corresponding methods for stability evaluation and run-out analysis, holds significant scientific and practical value. This is particularly true for the identification, monitoring, early warning, and risk prevention and control of such landslides.

However, the refinement and advancement of theoretical knowledge on the flexural buckling failure of dip rock slopes are often constrained by existing analysis methods. For flexural buckling failure, since the potential sliding surface does not daylight on the slope face but dips into the slope toe, the formation of a rupture surface involves complex geological and mechanical processes. Assessing the stability condition and changing trend of such a landslide is intricate and challenging. Consequently, existing quantitative methods for the stability analysis of buckling landslides need to simplify this aforementioned complex process equivalently, which leads to the applicability and consistency of a variety of approaches that usually cannot achieve satisfactory results and are worth further discussion.

For this purpose, this study comprehensively reviews the current research achievements related to buckling landslides, following the research path of “triggering mechanism → analysis methods”. This study re-examines the intrinsic and triggering factors influencing the deformation process of flexural buckling failure, as well as the existing physical, analytical, and numerical methods. Finally, this study summarizes the primary deficiencies in the research field of buckling failure and outlines the future research directions.

2. Analysis of Previous Studies on Flexural Buckling of Dip Slopes

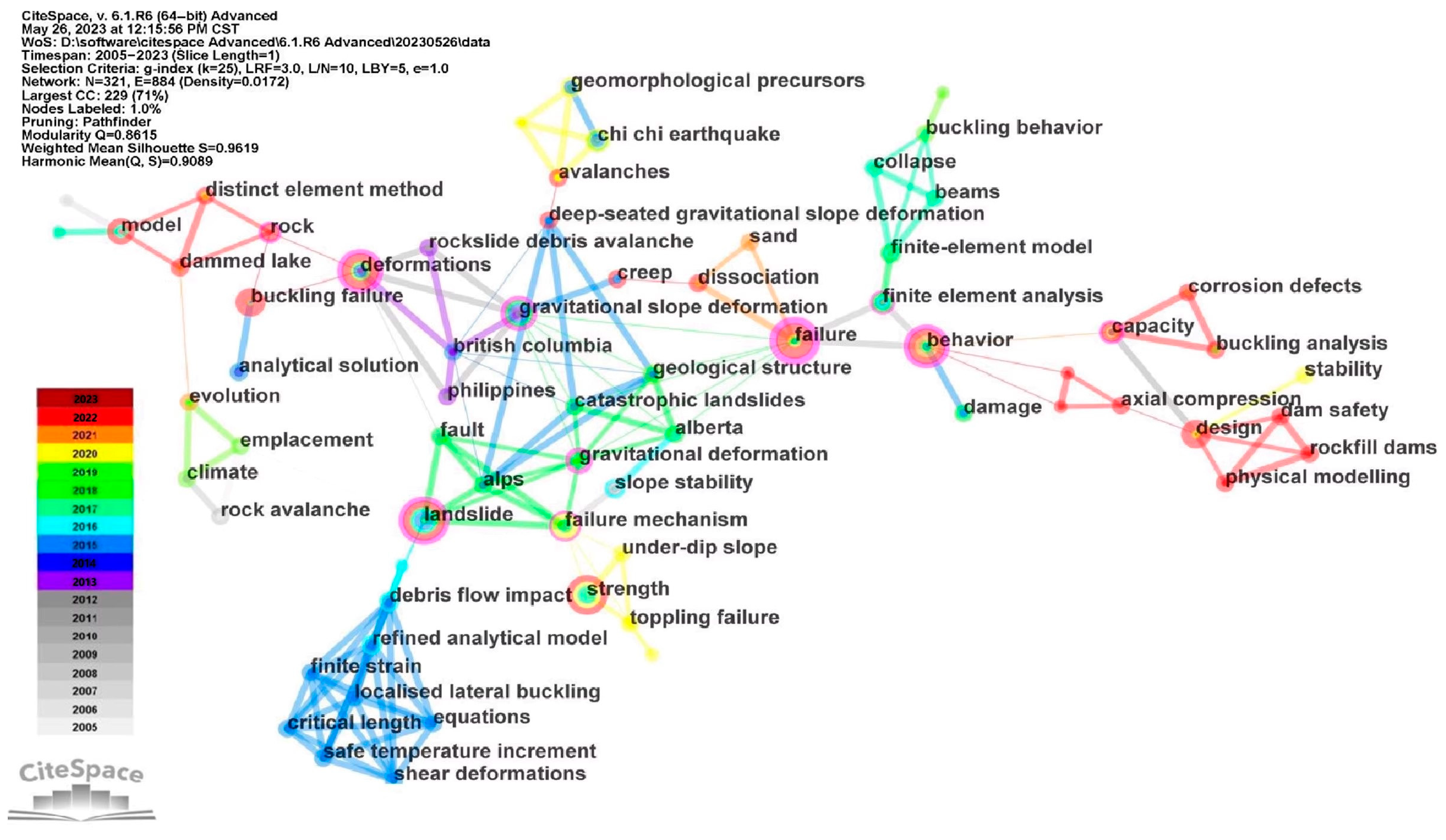
2.1. Bibliometric Analysis

A bibliometric analysis was conducted on the core literature database of the Web of Science related to buckling failure of rock slopes using the visualization analysis tool Citespace 6.1.R6. The search terms “buckling landslide” and “buckling failure & slope” were used to retrieve literature, with a time span from 1 January 1970 to 31 December 2023. A collection of documents that included the aforementioned search terms in their titles, abstracts, or keywords were obtained, resulting in a total of 133 valid documents.

From Figure 1, it can be seen that the number of papers related to buckling failure in the past 15 years is more than five times that of the previous period, and the number of papers has shown a significant increasing trend since 2015. The reason may lie in the fact that during this period, disaster cases captured the attention of both the academic and engineering communities, thereby effectively promoting the development of research on buckling landslides. For instance, the Xinmo landslide, which occurred in Xinmo Village, Maoxian County, Aba Prefecture, Sichuan Province, blocked a 2 km stretch of the river and resulted in over 100 casualties, causing significant loss of life and property [3]. In terms of document types, there are a total of 131 research papers (accounting for 98.5%), which make up the vast majority; there are only two review papers (accounting for 1.5%), which mainly focus on the research methods for the buckling of engineering components, and there is no systematic review on the research on buckling landslides.

By analyzing the keywords of the obtained literature related to buckling landslides, a total of 316 keywords were identified, with the top 100 high-frequency keywords retained for co-occurrence analysis. The analysis results are shown in Figure 2, where the larger the node size, the higher the frequency of the keyword appearing in different papers. The node connection lines represent the correlation between different keywords appearing in the same paper, and the color of the connection lines represents the temporal relevance of this correlation. It can be found that the keywords with higher frequency include “deformations”, “gravitational slope deformation”, “landslide”, “failure”, “behavior”, and “strength”, indicating that there is relatively more research around the topics of deformation and failure mechanisms of buckling landslides, as well as their evolution laws from deformation to instability. In addition, high-frequency keywords related to modelling methods such as “finite-element analysis”, “distinct element method”, and “physical modelling” also appear, indicating that the above methods are commonly used in the research on buckling landslides. Regarding the connections between keywords, some keywords, such as “landslide” and “gravitational slope deformation”, “failure”, and “refined analytical model”, are often mentioned together, indicating that the trends and progress of buckling landslide research mainly follow the path of “triggering mechanism → analysis methods”. In short, the results of bibliometric analysis show that instability mechanisms, evolution laws, and quantitative evaluation methods are hot topics in the research field of buckling landslides.

2.2. Brief Historical Retrospect of the Buckling Failure Phenomenon in the Literature

In terms of buckling failure, Kutter [17] introduced this phenomenon that occurred in British open pit coal mines and discussed the underlying mechanism and the corresponding recognition criterion. Later, Cavers [18] summarized three modes of buckling failure for stratified rock slopes (flexural buckling of plane slopes, three hinge buckling of plane slopes, and three hinge buckling of curved slopes) and proposed corresponding quantitative evaluation methods for buckling stability. The aforementioned study laid the foundation for the subsequent development of analytical approaches for buckling stability assessment in the twentieth century [19,20,21,22,23]. After that, with the progress and innovation of monitoring and modelling technologies [24,25,26,27,28,29,30,31,32,33,34], the academic and engineering communities have conducted in-depth studies on buckling failure through in situ investigations and modelling (analytical, numerical, and physical), mainly focusing on the deformation and failure mechanisms, as well as the stability analyses of buckling landslides.

3. Deformation Process of Flexural Buckling Failure and Its Influencing Factors

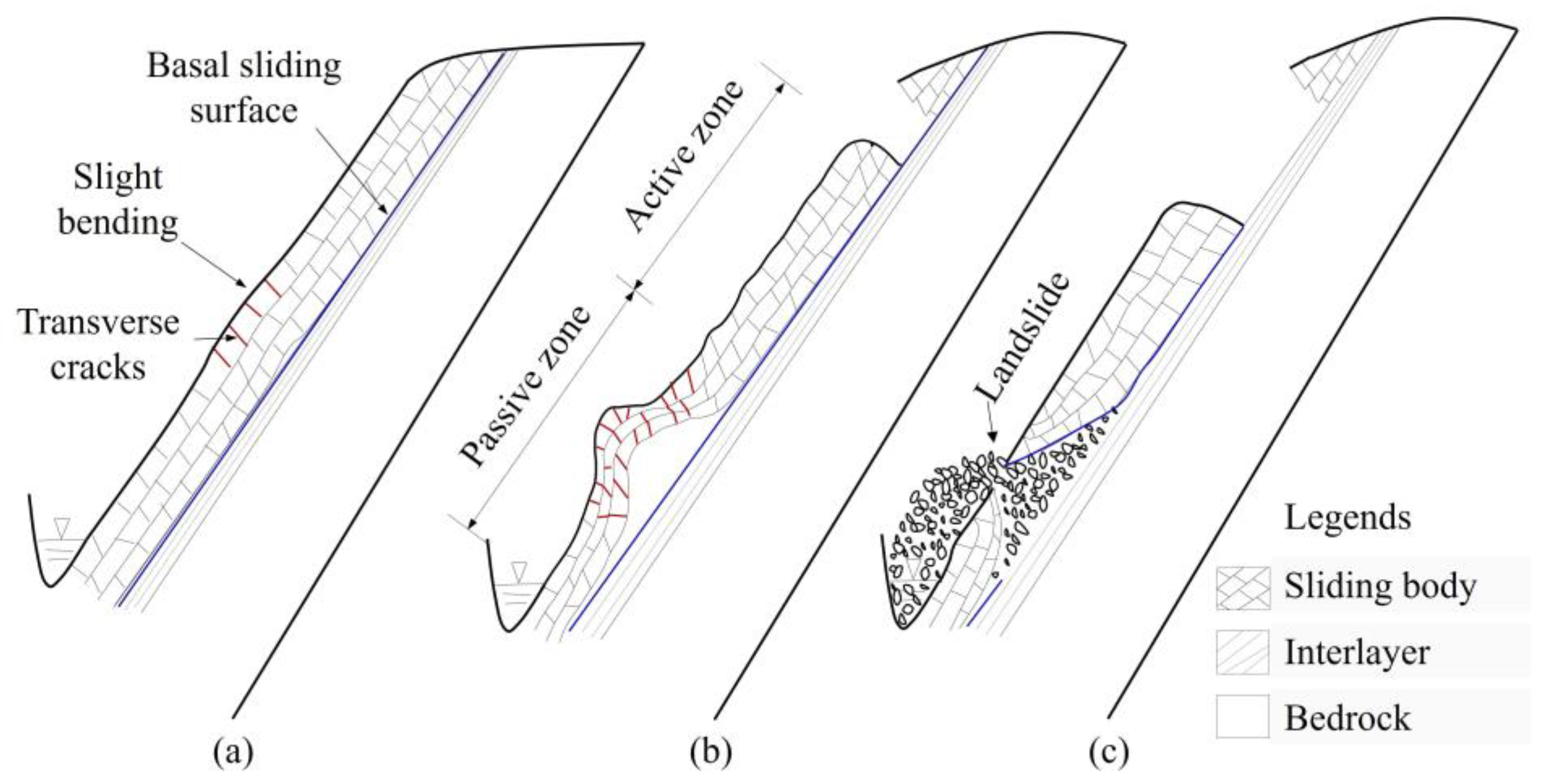
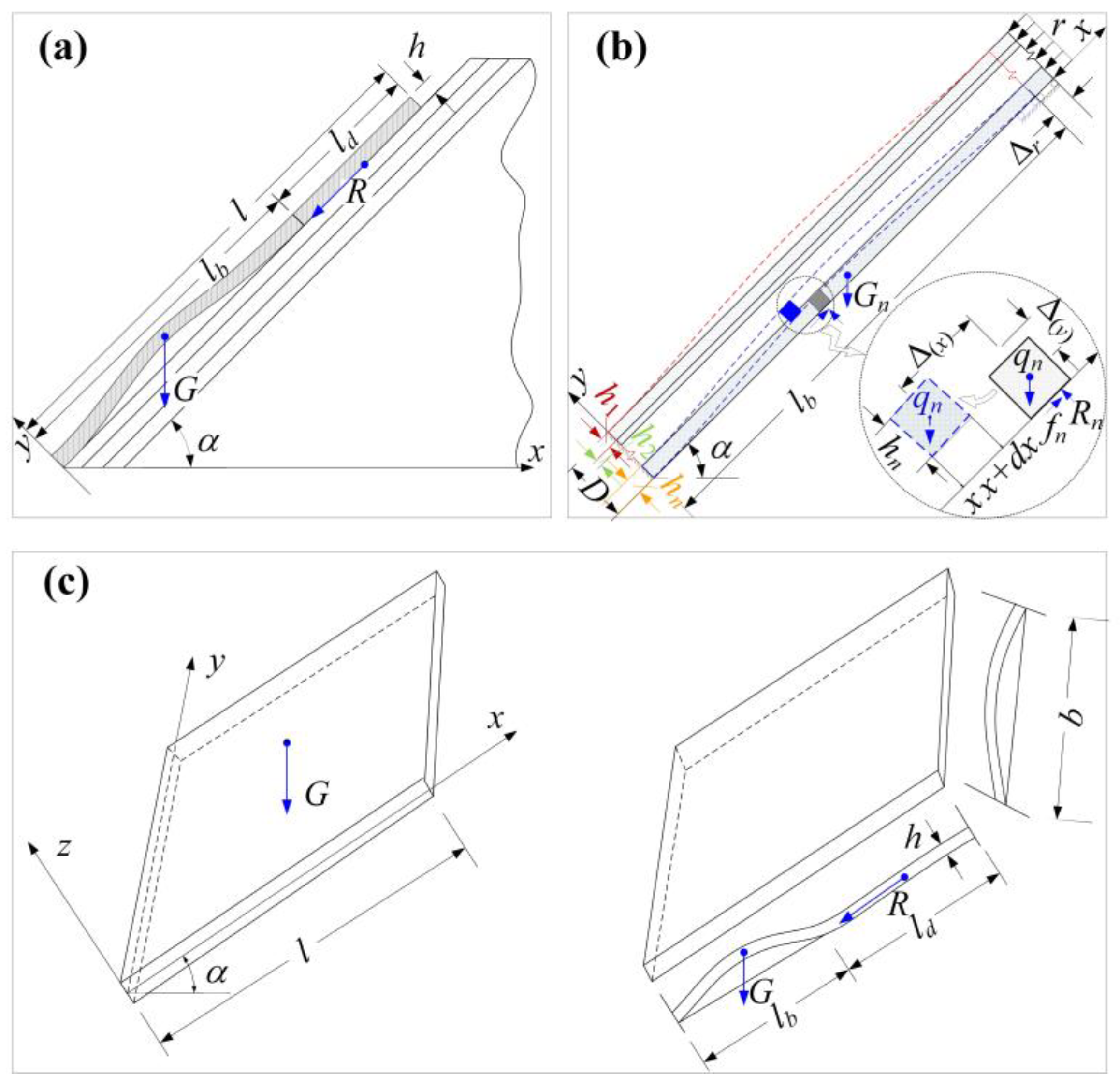
3.1. Deformation Process of Flexural Buckling Failure

According to previous studies [2,35,36,37,38], the deformation process of flexural buckling failure in rock slopes can be divided into three stages, as shown in Figure 3. In the slight bending stage (Figure 3a), under the downslope driving force, the middle and rear portions of a rock slope slide along the non-daylighting basal sliding surface and the rock layers at the front edge of the slope show slight bending due to compression. In addition, tension cracks, local crushing, and loosening can be observed in the bending segment. During the intense bending and uplift stage (Figure 3b), enhanced bending of rock layers leads to strong dilatancy of rock mass within the bending segment, with a significantly uplifted slope surface, and intense loosening and fracturing of rock mass can be found in the front edge of the rock slope. In the shearing failure and landslide stage (Figure 3c), a through-going sliding surface is formed due to the shearing failure of rock mass within the bending segment, and then a landslide is initiated. It is notable that Yan et al. [39] believed that there is a fracturing and disintegration stage between the intense bending and uplift stage and the shearing failure and landslide stage, but from the perspective of deformation and failure characteristics, this intermediate stage can be regarded as one part of the intense bending and uplift stage.

3.2. Factors Affecting the Deformation Process of Flexural Buckling

The deformation process of flexural buckling failure of dip rock slopes is typically affected by the coupled actions of intrinsic and triggering factors. The intrinsic factors mainly refer to the structural and geomechanical features of a dip rock slope, including the thickness of the rock layer, the dip angle of the rock layer, the shear strength parameters of the basal sliding surface (cohesion and internal friction angle), the deformation parameters of the rock mass, and others. Conversely, the triggering factors include natural factors such as earthquakes and rainfall, as well as human engineering activities such as reservoir water level fluctuations, blasting vibrations, excavation of slopes, and loading at the slope crest. The aforementioned factors from both aspects jointly influence each stage of flexural buckling of rock slopes, such as the buckling depth, and, in the case of failure, the location of the tension cracks at the rear edge of the rock slope, the location of the rupture surface near the slope toe, and the degree of fragmentation and disintegration of rock mass after the landslide.

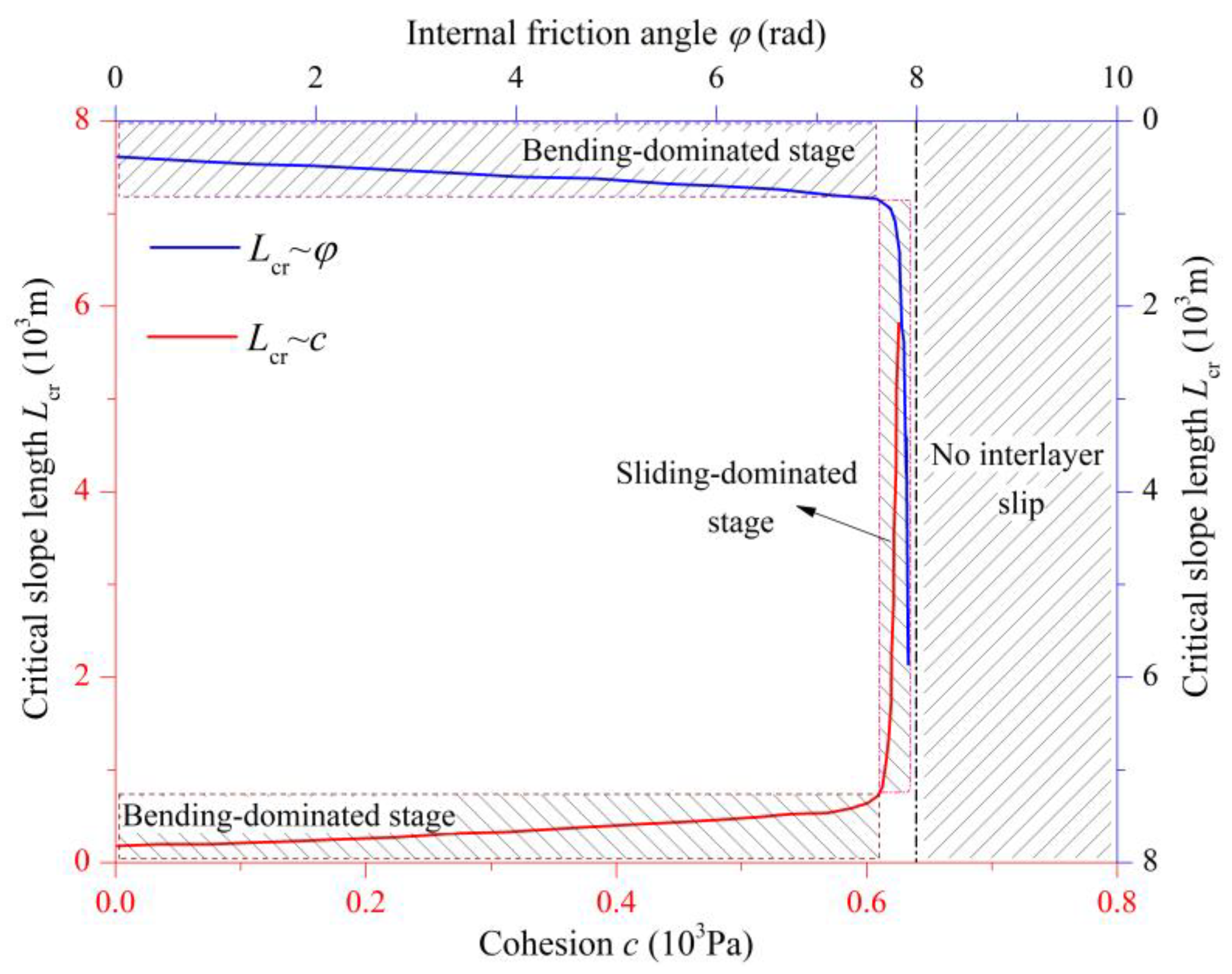
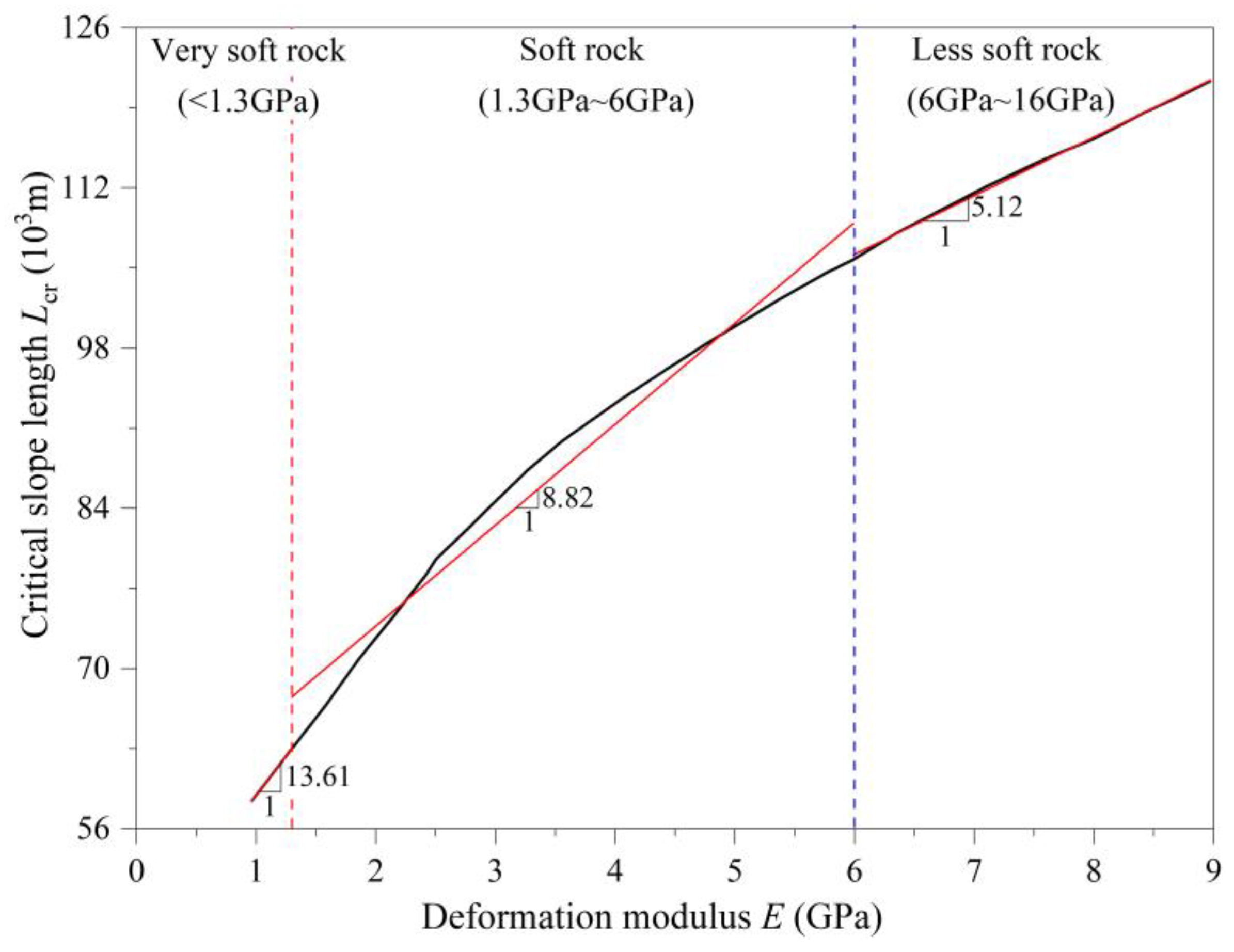
3.2.1. Intrinsic Factors

(1) Thickness of rock layer

From the perspective of material mechanics, the thin-layer rock mass with lower cross-sectional bending stiffness is prone to buckling instability. Tang et al. [29] believed that the influence of rock layer thickness on the buckling stability is mainly reflected in the buckling depth (buckling depth equals the number of bending rock layers multiplied by the single-layer thickness of rock); the thicker the single rock layer, the more pronounced its decisive role in the overall bending stiffness and the final buckling state. Through the experimental investigation of the deformation and failure features in cataclinal slopes, Lo and Weng [30] indicated a negative relationship between the buckling stability and the single-layer thickness. Later, the aforementioned negative relationship, in other words, the positive relationship between the critical slope length/height and the single-layer thickness, has also been demonstrated through sensitivity analyses using various analytical approaches [36,40,41].

Table 1. Historical events of flexural buckling failure and their associated information.

Event Name	Slope Length (m)	Rock Layer				Literature Sources
		Dip Angle (°)	Thickness (m)	Thickness Classification	Lithology
Bawang Mountain landslide	1462	40	3.0~4.0	Very thick	Limestone	Qin et al. [42]
Malvern Hills coal mine flexural failure	21	45	2.0~3.0	Very thick	Laminated mudstone with multiple coal seams	Seale [43]
Lavini di Marco flexural buckling	192	25~29	0.1~0.2	Medium-thick	Limestones with marly clayey interbeds	Tommasi et al. [44]
Outang landslide	1800	24~29	0.5~3.2	Medium-thick	Silty mudstone, shale, and argillaceous siltstone	Dai et al. [45]
Muyubao landslide	1200	27	0.1~0.3	Medium-thick	Quartz sandstone and mudstone	Zhou et al. [31]; Huang et al. [46]
Xinmo landslide	250	48	0.3~1.2	Medium-thick to thick	Psammitic schist	Zhao et al. [3]
Xiaguiwa landslide	1030	41	1.2~1.4	Very thick	Amphibolite and mica schist	Li et al. [14]
Hejia landslide	1150	44~56	0.3~0.5	Medium-thick	Metamorphic tuffaceous sandstone, silty slate	Jin et al. [40]
Tangjiashan landslide	905	45	0.08~0.3	Thin to medium-thick	Marlstone, limestone, and siltstone	Qi et al. [47]; Jin et al. [40]

presents data on the geometry (including layer thickness) of typical events of flexural buckling failure [3,14,31,40,42,43,44,45,46,47]. According to the Chinese Code for Investigation of Geotechnical Engineering (GB50021-2001, 2009), the thickness of a single rock layer can be classified into four categories: very thick (>1.0 m), thick (0.5 m ~ 1.0 m), medium-thick (0.1 m ~ 0.5 m), and thin (<0.1 m). The data indicate that the majority of flexural buckling failures occurred in the thin and medium-thick rock layers, with occurrences also noted in very thick rock layers. The reason for that feature is that the ratio of singe-layer thickness to slope length or height remains relatively small, leading to buckling deformation of the rock layer with high flexibility.

(2) Strength parameters of basal sliding surface

Based on the deformation process of flexural buckling failure, the shear strength parameters of basal sliding surface (cohesion and internal friction angle) directly affect the residual sliding force of rock masses in the active zone, which finally induces the bending of layered rock masses in the passive zone, altering the buckling stability of rock slope accordingly. At present, the effect of shear strength parameters of basal sliding surface on the flexural buckling stability is mainly investigated using analytical approaches. Referring to the Zhu et al. [48] and Jin et al. [40], the relationship curves between the buckling stability indicators and the shear strength parameters of basal sliding surface generally follow the same pattern of change, as shown in Figure 4. From the figure, it can be seen that as the cohesion or internal friction angle of basal sliding surface increases, the critical slope length for buckling failure first shows a linear and gentle growth trend in the bending-dominated stage. However, when the cohesion or internal friction angle of basal sliding surface exceeds a certain critical value, the critical slope length for buckling failure increases sharply and abruptly during the sliding-dominated stage. The reason lies in the fact that when the shear strength parameters of basal sliding surface increase to a certain extent, the anti-sliding force acting on the upper-middle part of the slope is greater than the sliding force, then the interlayer sliding in the upper-middle part of the slope will cease and the compression effect exerting on the layered rock mass of the lower portion will disappear, hence the critical slope length for buckling instability increases sharply.

According to above analyses, it is theoretically feasible to increase the slope stability against flexural buckling by enhancing the shear strength of basal sliding surface. In engineering practices, reinforcements such as anchor rods, anchor cables, and anti-sliding piles can be selected according to specific situations, as well as preventions of water-induced softening of basal sliding surface.

(3) Dip angle of rock layer

In general, the slope stability against flexural buckling gradually decreases with the increase of the dip angle of rock layer [23,29,36,40,48,49]. The main reason is that an increased dip angle leads to high bending moments and shear forces in the stratified rock mass, and the self-weight component parallel to basal sliding surface also increases, intensifying the risk of flexural buckling failure. For dip rock slopes consisting of medium-soft rocks, such as marl, slate, phyllite, shale, Tang et al. [29] conducted a systematic and in-depth study on the influence of dip angle combing model testing and numerical simulation. By monitoring the deformation of slope model, it was found that when the dip angle of the rock layer was 20°, the slope model only underwent slight deformation; with the increase of the dip angle, the rock layers exhibited creeping deformation, and the rock layers of the lower part of the slope model showed bending phenomena; when the dip angle of the rock layer was less than or equal to 20°, the front edge of the slope model was usually relatively stable and did not uplift. Based on the above phenomena, Tang et al. [29] believed that a dip angle of rock layer greater than 20° is an important geological condition for the buckling instability of dip slopes with medium-soft rocks. Recently, Chen et al. [50] statistically investigated the relationship between the instability deformation of buckling landslides and the dip angle of the strata, pointing out that the dip angles of rock layers with buckling deformation are mostly distributed between 20° and 50°. The main reason is that rock layers with a gentler dip angle generally undergo bedding sliding–tension cracking, while rock layers with a steeper dip angle are mostly dominated by toppling deformation. It should be noted that buckling failure can also occur in very steep rock layers when the boundary condition of the layer toe is pinned, owing to the fracturing and loosening [1].

Table 1 also lists the dip angle information of nine typical buckling landslides. As can be seen from the table, the dip angles of the above buckling landslide cases generally vary between 20° and 50°, fully corroborating the research conclusions of Chen et al. [50]. Therefore, extra caution should be exercised when encountering this dip angle range in the site.

(4) Deformation parameters of rock mass

At present, there is relatively little research on how deformation parameters of rock mass influence the buckling stability of rock slopes. In this respect, most studies use theoretical analysis to explore these relationships [36,41,49,51]. The relationship between critical length and the deformation modulus is often nonlinear positive, as shown in Figure 5. The reason is that intact rock with high elastic moduli has strong sectional bending performance and is not prone to plastic deformation, thus exhibiting better buckling stability. From the perspective of lithology, the elastic modulus of intact rock can indirectly reflect the strength of the rock. Soft rocks with lower elastic moduli are more prone to buckling failure due to their poor bending and shear resistance. For instance, in the field investigation of the deformation and failure modes of rock slopes in soft rocks, Sun [21] found that buckling dominates when the boundary conditions of the rock layer are fixed.

3.2.2. Triggering Factors

(1) Rainfall

Rainfall, especially continuous heavy rainfall, could trigger buckling landslides. For example, in 2017, continuous rainfall in Xinmo, Sichuan, for as long as two months, resulted in the reactivation of old landslides in the Xinmo area, blocking the river for 2 km and burying more than 100 people [3]. The mechanism of rainfall affecting the stability of buckling landslides is mainly manifested in the following aspects. First, the rainfall-induced softening weakens the strength of the basal sliding surface, which significantly affects the buckling stability adversely. Second, the seepage field formed by rainfall infiltration generates static water pressure within the slope body, exerting a buoyant force and reducing the resisting force along the base of the sliding mass, thereby reducing the buckling stability. Finally, the rainfall infiltration will gradually reduce the matrix suction in unsaturated soil-like infillings or interbeds between layers, along which sliding occurs, which also leads to a reduction in buckling stability. The instability and failure of buckling landslides induced by rainfall is usually a relatively slow process, and current research mainly focuses on the impact of long-term heavy rainfall on buckling landslides investigated by using numerical methods, or the impact of static and dynamic water pressure formed by rainfall on buckling landslides through theoretical calculations. Due to the complicated mechanism of rainfall-induced buckling landslides, it remains a key and challenging issue in this research field.

(2) Earthquakes

Seismic landslides undergo the process of initiation, run-out, and accumulation in an extremely short period of time, releasing tremendous energy that often causes incalculable losses to the surrounding ecological environment and the safety of lives and property. The Tangjiashan coseismic landslide triggered by the Wenchuan earthquake in 2008 resulted in 84 deaths, and at the same time blocked the Jianjiang River to form a barrier lake, causing huge economic losses [47]. Under normal circumstances, the structural loosening of the slope body, the formation of internal fracture surfaces, and the sharp rise in pore water pressure within the slope body caused by earthquakes can create favorable conditions for the rapid initiation of buckling landslides.

At present, the stability assessment of buckling landslides under earthquakes still poses a great challenge, and there is a relative scarcity of relevant research literature, which mainly focuses on theoretical analysis. Based on the principle of energy balance, Qi et al. [47] proposed an analytical approach for evaluating the stability of buckling landslides considering the action of earthquakes and pore water pressure and verified the effectiveness of the developed analytical method with the Tangjiashan landslide case. By simplifying the seismic action as horizontal or vertical inertial forces using the pseudo-static method, Liao et al. [52] derived analytical expressions for the stability assessment of dip slopes against flexural buckling and three hinge buckling under earthquakes and pointed out that the buckling instability of dip slopes is closely related to the direction of the horizontal seismic force. Utilizing the parameter sensitivity analysis method, Yang et al. [53] investigated the impact of the horizontal seismic coefficient on the buckling stability of dip slopes, and the study showed that as the horizontal seismic coefficient increased, the critical buckling length decreased, and the buckling stability decreased. Recently, Pei et al. [54] adopted a shaking table test to analyze the response of buckling landslides under continuous seismic loads, pointing out that the stress amplification of the bedding structure under earthquakes is an important reason for triggering buckling instability.

(3) Human engineering activities

Among human engineering activities, reservoir water level fluctuation is a special factor influencing the buckling stability of bank slopes of reservoirs, such as the Three Gorges, Lijiaxia on the Yellow River, Yalong River, and Chihaxia [31,45,55]. The direct effect of reservoir water level fluctuations on buckling stability is mainly manifested in the changes in static and dynamic water pressure, buoyancy, and seepage field within the bank slope, as well as the water–rock interaction in the drawdown zone. By analyzing the monitoring data of the Muyubao landslide, Feng et al. [56] believed that its deformation is mainly affected by buoyancy and dynamic water pressure, and when the rate of reservoir water level fluctuation is small, buoyancy controls the deformation of the landslide; when the rate of reservoir water level fluctuation increases, dynamic water pressure becomes the main controlling factor of landslide deformation. Through field investigations, Yan et al. [57] found that the deterioration and damage of rock mass near the front edge of dip slopes induced by long-term water–rock interaction is an important factor triggering buckling failure of reservoir bank, and they proposed an approach for evaluating the buckling stability of bank slopes considering the effect of rock mass deterioration. In addition, excavations of dip slopes in road and mining projects can cause changes in the original stress state and boundary conditions of dip slopes, triggering the buckling deformation and failure of rock layers [1,22,43].

4. Analysis Methods for Flexural Buckling Failure

4.1. Physical Model Tests

By comprehensively and realistically reproducing the complex geological conditions of a landslide, physical model tests can intuitively simulate the entire process of landslide deformation, instability, run-out, and accumulation, revealing the effects of key parameters on the whole landslide process, and further provide a scientific basis for the construction of new theoretical and numerical models.

At present, common physical model tests of buckling landslides include conventional indoor model tests, base friction tests, centrifugal model tests, and shaking table tests. Utilizing resin-bonded spherical artificial rock particles to assembly rock slabs and small-scale indoor models of stratified rock slopes, Weng et al. [58] and Lo and Weng [30] reproduced the evolution process of flexural buckling and systematically studied the effects of slope angle, dip angle, layer thickness, and material deterioration caused by rainfall on the buckling deformation and failure of dip slopes. By experimentally modelling the dynamic excavation processes in a model of open pit mines, Wen et al. [59] found that rocks on the upper part of the slope slipped along the bedding planes, while slip-buckling failure occurred near the foot of the slope. Taking the generalized model of the Qingshi bank slope in the Three Gorges Reservoir area as an example, Yan et al. [57] studied the effect of rock mass deterioration in the hydro-fluctuation belt on the buckling stability of bank slopes, and they found that the thrusting of the rear edge is the premise of buckling failure of the bank slope, and that the continuous deterioration of rock mass in the hydro-fluctuation belt is the leading factor of buckling failure. Specifically, the rock mass deterioration in the hydro-fluctuation belt involves the weakening of the mechanical parameters, the separation of interlayers, and the increasing fragmentation of the rock mass [55,60,61,62]. As to the base friction tests for the modelling of the buckling landslide evolution process, Du et al. [27] discussed and analyzed the main influencing factors of critical slope length and the uplift height. Subsequently, Tang et al. [29] also explored the relationship between the buckling mechanism and controlling factors. Based on centrifugal model tests, Weng et al. [35] reproduced the entire process of buckling instability of stratified rock slopes and revealed the impact of scale effects on the buckling instability and failure mechanism of stratified rock slopes. The scale effect refers to the phenomenon where the size of a slope influences its deformation and failure [63,64]. Recently, based on shaking table tests, Li et al. [65] physically modeled the failure process and acceleration responses of two interbedded dip slopes under different seismic intensities, and they found that buckling failure tends to occur when the dip of rock layer is less than 65°. Combining shaking table tests with a digital image correlation technique, Pei et al. [54] analyzed the dynamic response and deformation characteristics of the Xinmo landslide under accumulated seismic actions, revealing the contribution of stress amplification on the discontinuities to the buckling failure. Using the Xiaguiwa landslide as a case study, Wang et al. [66] experimentally investigated the dynamic damage mechanism of dip slopes under multiple earthquakes and found that damage in the bedding rock slope first appeared at one-quarter of the elevation due to buckling. Based on the large-scale centrifugal shaking table test platform, Li [67] observed that the seismic failure pattern in layered rock slopes with steep strata is significantly influenced by lithological assemblies. Specifically, a slope with an interbedded rock stratum is prone to seismic buckling failure.

In the physical model tests of buckling failure, the preparation of similar materials for the geological body and the set-up of the reduced-scale landslide models are costly and complicated, coupled with the fact that the deformation, instability, and failure process of buckling landslides generally span a longer time, leading to high resource investment, long time consumption, and limited parameter acquisition in model tests. The number of existing physical modelling studies on the buckling failure is still relatively small. In the future, it is necessary to develop more rational and reliable equipment for modelling internal and external forces, as well as more refined monitoring instruments for stress, kinematic parameters, and water pressure, to further promote the development and application of physical model tests in the research field of buckling landslides.

4.2. Analytical Methods

At present, analytical methods for evaluating flexural buckling stability of dip slopes mainly adopt three theoretical mechanical schemes, namely, the single beam (column) model, the multi-layer beam model, and the elastic (plastic) slab model, as illustrated in Figure 6. By simplifying the flexural buckling failure of dip slopes under various loads into the instability problems of the previous mechanical models, the deflection equations of the column, beam, or slab models are assumed according to the boundary constraint conditions, and then the explicit or implicit solutions to the critical slope length or critical load are determined based on methods such as the Euler buckling theory, the energy balance principle, the catastrophe theory, or the force balance conditions. Subsequently, the critical slope length or critical load can be adopted to evaluate the flexural buckling stability of dip rock slopes, that is, when the longitudinal load at the slope crest is greater than the critical buckling load (P_cr) or the actual slope length is greater than the critical slope length (L_cr), the slope will undergo buckling.

(1) Single beam (column) model

The instability and evolution process of the flexural buckling of dip slopes may involve a single rock layer or multiple rock layers. The single beam (column) model simplifies the single buckled rock layer or the pack of buckled rock layers as a single beam (column) with assumed end conditions, and the beam (column) stability theory is capable of analyzing the flexural buckling of the dip slope. Adopting the model of column with pinned ends, Cavers [18] derived the critical slope length against flexural buckling based on the Euler buckling theory. Then, by solving the eigenvalue of the basic differential equation of beam deflection under the combined action of transverse load, axial concentrated load, and distributed load, Sun and Zhang [19] proposed the analytical solution to critical buckling load considering the self-weight of rock layer and the hydrostatic pressure. Based on the cusp catastrophe theory, Qin et al. [42] determined the necessary and sufficient conditions for the flexural buckling failure of stratified rock slopes. By considering the effects of earthquakes and hydrostatic pressure along the basal sliding surface, Qi et al. [47] developed an analytical approach in the frame of energy equilibrium theory and analyzed the typical buckling failure of the Tangjiashan landslide. In order to investigate the flexural buckling failure of stratified rock slopes under top loading, Liu et al. [49] presented an explicit analytical approach by combining Euler’s method and the superposition principle. Under equilibrium conditions, Garzon [69] evaluated the flexural buckling stability of stratified rock slopes using the tangent-modulus theory and classified the buckling failure modes into three types: brittle, elastic, and inelastic failure. Recently, accounting for the longitudinal and transverse bending of layered rock mass under the combined action of the self-weight of rock layer, the hydrostatic pressure, and the seismic load, Yang et al. [53] presented the conditional equation for the length of the buckling segment based on the elastic column stability theory and energy principles. Representative analytical approaches for the stability evaluation of flexural buckling based on the single beam (column) model are shown in

Table 2. Typical analytical approaches based on the single beam (column) model.

No.	Analytical Approaches	Literature Sources
1	$l_{cr}^3={\displaystyle \frac{{\pi }^{2}E{h}^2}{2.25(\gamma \sin \alpha -\gamma \cos \alpha \tan \phi -\frac{c}{h})}}$	Cavers [18]
2	$\begin{array}{c}{\displaystyle \frac{1}{l_{cr}^2}}={\displaystyle \frac{1}{l_{cr1}^2}}+{\displaystyle \frac{1}{l_{cr2}^2}};\\ where l_{cr1}=\sqrt[3]{{\displaystyle \frac{{\pi }^{2}E{h}^3}{1.44[\gamma h(\sin \alpha -\cos \alpha \tan \phi )-c]}}},l_{cr2}=\sqrt{{\displaystyle \frac{{\pi }^{2}E{h}^2}{5.88q}}}\end{array}$	Liu et al. [49]
3	$\begin{array}{c}{l}_{bcr}=\sqrt{C_1^2+2C_{1}l}-C_1;\\ where C_1={\displaystyle \frac{2.4(\gamma h\sin \alpha -c-\gamma h\cos \alpha \tan \phi )}{\gamma \cos \alpha }}\end{array}$	Garzon [69]
4	$\begin{array}{c}if{l}_w<l_{bcr},{\displaystyle \frac{{(\gamma h)}^{2}E{h}^3}{3l_{bcr}^2}}-0.5l_{bcr}\gamma h(\sin \alpha +{\beta }_s{K}_s\cos \alpha )=\\ \left[\gamma h\sin \alpha -\gamma h(\cos \alpha -{\beta }_s{K}_s\sin \alpha )\tan \phi -c\right](l-l_{bcr});\\ if{l}_{bcr}<l_w\le l,{\displaystyle \frac{{(\gamma h)}^{2}E{h}^3}{3l_{cr}^2}}-0.5l_{bcr}\gamma h(\sin \alpha +{\beta }_s{K}_s\cos \alpha )=\\ \left\{\gamma h\sin \alpha -\left[\gamma h\cos \alpha -\gamma h{\beta }_s{K}_s\sin \alpha -0.5{\gamma }_w{(l_w-l_{bcr})}^2\cos \alpha \right]\tan \phi -c\right\}(l-l_{bcr});\end{array}$	Yang et al. [53]

.

(2) Multi-layer beam model

This model regards the pack of buckled rock layers as a multi-layer beam with fixed ends, assuming that multiple rock layers bend in the same way under the combined action of self-weight and external loads, and no relative slip occurs in the buckled rock layers. Based on the multi-layer beam model, Li et al. [23] derived the analytical solutions for the critical buckling load and the critical buckling length of dip slopes using the energy equilibrium principle. Considering the effects of water pressure and earthquakes, Zhu et al. [48] proposed theoretical buckling curves for each rock layer based on special function theory and deeply discussed the buckling failure characteristics of stratified rock slopes. Zhang et al. [36] derived analytical solutions for the critical buckling length and critical buckling depth of stratified rock slopes using the energy equilibrium principle and introduced the generalized Hoek–Brown criterion to account for the impact of scale effect on buckling stability. Similarly, utilizing the energy equilibrium principle, Wang et al. [32] provided a calculation formula for the critical buckling length of dip slopes, and they verified it in combination with numerical simulation and field data. Later, Wang et al. [70] suggested that critical sliding displacement is more suitable for quantitatively analyzing the progressive evolution process of buckling landslides. They proposed a calculation method for determining critical sliding displacement and established corresponding criterion for assessing flexural buckling stability, taking into account both the tensile and compressive strengths of rock. Based on the mechanical analysis, Jin et al. [40] established the buckling curve equation of multi-layered rock masses under the action of earthquake and top loading and presented the analytical formula of critical slope length for buckling failure using the energy method, as well as the method for determining the position of failure surface according to the maximum deflection point. Then, this approach was successfully applied to typical landslide cases. Typical methods for the stability evaluation of flexural buckling based on the multi-layer beam model are given in

Table 3. Typical analytical approaches based on the multi-layer beam model.

No.	Analytical Approaches	Literature Sources
1	$\begin{array}{c}{P}_{cr}={\displaystyle \frac{{\pi }^{2}nE{h}^3}{6l^2}}-{\displaystyle \frac{2Q}{\sqrt[3]{4}}}\\ 6(\gamma nh\sin \alpha -\gamma nh\cos \alpha \tan \phi -c)l_{cr}^3+3Q{l}_{cr}^2-{\pi }^{2}nE{h}^3=0\end{array}$	Li et al. [23]
2	$\begin{array}{l}\min D_{cr}={\displaystyle \sum _{i=1}^n{h}_i},l_{bcr}\\ \mathrm{s}.\mathrm{t}.3\left[\right(\sin \alpha -\cos \alpha \tan {\phi }_n){\displaystyle \sum _{i=1}^n{\gamma }_i{h}_i}-c_n]l_{bcr}^3+6r{\displaystyle \sum _{i=1}^n{h}_i}{l}_{bcr}^2-2{\pi }^2{\displaystyle \sum _{i=1}^n{E}_i{h}_i^3}=0\\ r{\displaystyle \sum _{i=1}^n{h}_i}+l_{bcr}{\displaystyle \sum _{i=1}^n{\gamma }_i{h}_i}\sin \alpha -l_{bcr}({\displaystyle \sum _{i=1}^n{\gamma }_i{h}_i}\cos \alpha \tan {\phi }_n+c_n)>0\end{array}$	Zhang et al. [36]
3	$\begin{array}{c}{l}_{bcr}^3+k_1{l}_{bcr}^2-k_2=0,U_{cr}=l_{bcr}+U_1-{\displaystyle \frac{(l_{bcr}+U_1)\tan \alpha }{\sqrt{1+{\tan }^2\alpha }}}\\ where{k}_1={\displaystyle \frac{2l(n\gamma h\sin \alpha -n\gamma h\cos \alpha \tan \phi -c)}{-n\gamma h\sin \alpha +2n\gamma h\cos \alpha \tan \phi +2c}},\\ k_2={\displaystyle \frac{nE{\pi }^2{h}^3}{6(-n\gamma h\sin \alpha +2n\gamma h\cos \alpha \tan \phi +2c)(1-{\mu }^2)}},\\ U_1={\displaystyle \frac{l_{bcr}^3}{{(nEd\pi )}^2}}{[{\sigma }_t+0.5\gamma l_{bcr}\sin \alpha +(l-l_{bcr})(\gamma \sin \alpha -\gamma \cos \alpha \tan \phi -{\displaystyle \frac{c}{nd}})]}^2\end{array}$	Wang et al. [70]
4	$\begin{array}{c}{\displaystyle {\int }_0^{l_{cr}}\left[EI{(-\frac{Ry-M}{EI})}^2-\frac{1}{2}R{(y^{\prime })}^2+Ny+M{y}^{\prime }\right]}dx=0,\\ where R=\left[\gamma h\sin \alpha +\gamma h{K}_s\cos \alpha -{\displaystyle \frac{N}{x}}\tan \phi \right]x,\\ M={\displaystyle \frac{h}{2}}\left[(2n-1){\displaystyle \frac{N}{x}}\tan \phi +2c\right]x+{\displaystyle \frac{1}{2}}Nx,\\ N=(\gamma h\cos \alpha +{\displaystyle \frac{qh}{l}}\cos \alpha -\gamma h{K}_s\sin \alpha )x\end{array}$	Jin et al. [40]

.

(3) Elastic (plastic) slab model

Considering the presence of joints, fissures, and fractures in dip rock slopes, the longitudinal and transverse lengths of the buckled rock layers are usually limited. Therefore, simplifying the flexural buckling of dip slopes as thin plate instability problems is more in line with the actual situation. This model regards the rock layer as a plate with a hinged bottom, peripheral roller supports, and finite length, and it can be analyzed according to the stability theory of elastic (plastic) compressed plates. In combination with the elastic plate model and the energy equilibrium principle, Liu and Zhou [71] systematically investigated the effect of the self-weight component of the rock layer in the direction parallel to the strata, interlayer friction, and cohesion on the buckling stability of stratified rock slopes. Considering that the rock mass will exhibit plastic deformation when the compressive stress exceeds its elastic limit, Feng et al. [68] regarded the rock layer as an elastic-plastic plate in the analysis of flexural buckling. Recently, in response to the buckling deformation caused by the rock mass deterioration in the hydro-fluctuation belt of the Wuxia Gorge, Yan et al. [41] proposed a formula for the critical length of buckling segment considering the dynamic deterioration effect of the rock mass based on the elastic–plastic plate model. According to the energy equilibrium of the elastic–plastic plate model, Wu et al. [72] and Zhang et al. [73] presented a criterion to judge whether the dip slope is sliding or structurally unstable under the combined effects of self-weight, hydrostatic pressure, and earthquakes, and they subsequently applied this method to analyze three practical buckling cases. Some typical methods for the stability evaluation of flexural buckling developed on the elastic (plastic) slab model are shown in

Table 4. Typical analytical approaches based on the elastic (plastic) slab model.

No.	Analytical Approaches	Literature Sources
1	$\begin{array}{c}{\displaystyle \frac{{\pi }^{2}D}{b^4}}{l}_{bcr}^4+(H-{\displaystyle \frac{\gamma h}{2}}\sin \alpha )l_{bcr}^3+({\displaystyle \frac{2{\pi }^{2}D\sqrt{{\psi }_{\mathrm{t}}}}{b}}-lH)l_{bcr}^2+{\pi }^{2}D{\psi }_{\mathrm{t}}=0\\ whereH=\gamma h(\sin \alpha -\cos \alpha \tan \phi )-c,D={\displaystyle \frac{E{h}^3}{12(1-{\mu }^2)}};{\psi }_{\mathrm{t}}=E_{\mathrm{t}}/E\end{array}$	Feng et al. [68]
2	$\begin{array}{c}{\displaystyle \frac{{\pi }^{2}K(t)}{b^4}}{l}_{bcr}^4+\left[H-{\displaystyle \frac{\gamma h\sin \alpha }{2}}\right]l_{bcr}^3+{\psi }_{\mathrm{t}}{\pi }^{2}K(t)\\ +\left\{{\displaystyle \frac{\left[\mu (1+{\psi }_{\mathrm{t}})+2\sqrt{{\psi }_{\mathrm{t}}}(1-\mu )\right]}{b^2}}{\pi }^{2}K(t)-Hl\right\}{l}_{bcr}^2=0\\ whereK(t)={\displaystyle \sum _{i=1}^n\frac{E(t)h_i^3}{12(1-{\mu }^2)}},H=\gamma h(\sin \alpha -\cos \alpha \tan \phi )-c,{\psi }_{\mathrm{t}}=E(t)/E\end{array}$	Yan et al. [41]
3	$\begin{array}{c}({\displaystyle \frac{{\pi }^{2}D}{b^4}}-{\displaystyle \frac{{\gamma }_w\sin \alpha \tan \varphi }{2}})l_{bcr}^4+(Q+{\gamma }_{w}l\sin \alpha \tan \varphi -{\displaystyle \frac{\gamma h(\sin \alpha +{\beta }_s{K}_s\cos \alpha )}{2}})l_{bcr}^3\\ +\left\{{\displaystyle \frac{{\pi }^{2}D}{b^2}}\left[2\mu {\psi }_t+2(1-\mu )\sqrt{{\psi }_t}\right]-lQ-{\displaystyle \frac{{\gamma }_w{l}^2\sin \alpha \tan \varphi }{2}}\right\}{l}_{bcr}^2+{\pi }^{2}D{\psi }_t=0\\ where D={\displaystyle \frac{E{h}^3}{12(1-{\mu }^2)}},Q=\gamma h(\sin \alpha -\cos \alpha \tan \varphi )+{\beta }_s{K}_s\gamma h(\cos \alpha +\sin \alpha \tan \varphi ),\\ {\psi }_t=E_t/E\end{array}$	Wu et al. [72]

.

4.3. Numerical Simulations

Currently, numerical research on the flexural buckling of dip rock slopes can be mainly classified into the stability analysis and the run-out analysis. The stability analysis generally accounts for the small deformation of slope, such as the formation of sliding surface and the local bending of rock layers. In terms of run-out analysis, more attention is paid to large deformation and complex motions, such as the fracturing of rock layers and the run-out and accumulation of landslides. Accordingly, there are various numerical methods that are suitable for analyzing the different phases of flexural buckling of dip rock slopes.

Mostly, the finite difference method (FDM) and the finite element method (FEM) are capable of modelling the instability process of the flexural buckling of dip slopes. For instance, combining the FDM with the Cosserat continuum model, Pant and Adhikary [74] deeply investigated the buckling deformation process and failure mechanism of a stratified rock slope, and they found that the explicit joint model would overestimate the critical buckling coefficient of the rock masses with fewer layers. Subsequently, Adhikary et al. [24] introduced the large deformation Cosserat continuum model into the AFENA code and numerically recreated the entire process of buckling instability of a stratified rock slope. Pereira and Lana [26], and Silva and Lana [28] used the finite element software Phase2 to simulate the buckling deformation process of phyllite rock layers, focusing on the influences of the shear and normal stiffness of discontinuities, cohesion, and in situ stress on the buckling failure process of a stratified slope. Recently, Ridl et al. [75] regarded the inherent anisotropic characteristics of the rock mass as a discrete crack network, and they implemented numerical analysis of the buckling deformation of the stratified rock mass using the FEM.

In terms of numerical modelling of the entire evolution of flexural buckling failure, the Universal Distinct Element Code (UDEC), Particle Flow Code (PFC), Discontinuous Deformation Analysis (DDA), and Numerical Manifold Method (NMM) are more suitable. For example, by using the UDEC program, the buckling instability and failure process of the slope at the Westfield open-pit coal mine was detailed analyzed [76,77]. Alzo’ubi et al. [78] investigated the effect of long-term weathering on buckling deformation and found that changes in the in situ stress state of a slope and the tensile strength of rock significantly affect the flexural buckling stability of the slope. According to the UDEC simulation results, Tang et al. [29] proposed some insightful views on the geological and mechanical conditions, the determination of critical failure state, and early signs for the buckling instability of a stratified rock slope. Later, taking the Xiaguiwa landslide in the upper Jinsha River, southeastern Tibetan Plateau, as an example, Li et al. [15] simulated the entire process from buckling deformation and failure to the formation of a landslide dam using the UDEC. Through discrete element modeling that accounted for the rheological properties of anisotropic gneissic rocks, Huber et al. [79] identified the critical orientation configuration between isotropy planes and slope faces that leads to buckling failure. Based on the PFC simulations of the buckling instability process of the Xinglangpo landslide, Miao et al. [33] conducted an in-depth study of the micro-mechanical mechanism of the landslide. Wu [80] employed the DDA method to simulate buckling deformation and ground subsidence phenomena in the lower slope area of the Chu-fen-er-shan landslide. By combining DEM and DDA, Tommasi et al. [25,44] conducted a back-analysis of the buckling phenomenon of the Lavini di Marco area of Trento, Italy, and deeply investigated the effect of rock mass properties, slope geometry, and hydrological conditions on the buckling deformation behavior of stratified rock slopes. Zhang [81] proposed a stiffness reduction method to evaluate the flexural buckling stability of a stratified rock slope and simulated the entire process of buckling deformation and instability of a single rock layer using the DDA. Recently, by simulating the instability and evolution process of Malvern Hills in New Zealand using the NMM, Wang et al. [32,69] explored the effect of soft interlayers and staggered joints on the buckling deformation and failure process of the stratified rock slope, confirming that NMM simulation can well reproduce the entire process from the progressive instability and failure to the run-out and accumulation of buckling landslides.

5. Concluding Remarks and Future Prospects

To fully understand the behavior of buckling failure of dip rock slopes, a bibliometric analysis was conducted to synthesize relevant literature and systematically review research hotspots and development trends in this field. Although significant advancements have been made in studying the deformation process, influencing factors and analysis methods related to the flexural buckling failure of dip slopes, challenges remain in both theoretical research and engineering practice.

Existing theoretical studies on instability mechanisms and stability evaluation of flexural buckling in dip rock slopes have primarily focused on exploring the influence of either intrinsic or triggering factors through numerical and analytical modelling, while rarely considering the coupled effects of multiple factors. The application of physical model tests, particularly centrifugal modeling which can reproduce prototype stress states while accounting for time-dependent effects, remains at an early stage. In future research, refined numerical methods should be combined with novel physical modelling techniques to systematically investigate the influence mechanisms of multi-factor coupling on buckling failures of dip slopes.

Based on case reviews, flexural buckling failures predominantly develop in soft–hard interbedded dip rock slopes with layer inclination of 20–50° and thin-to-medium thickness characteristics. For rapid assessment of dip slope buckling, analytical approaches and corresponding assessment indicators must be carefully selected. Regarding stiff and fragile rock layers, force-equilibrium or energy-based analytical methods at precise limit states are preferable. The assessment index of the elastic critical load is particularly useful here, as rock mass strength drops sharply once this threshold is reached, leading to destructive landslides. For soft rock layers, critical-displacement-based methods are more appropriate, although their application across different scenarios remains challenging. Civil engineering applications require strict displacement limits to protect structures and maintain serviceability. In contrast, mining engineering often permits larger displacements and operates with marginal safety conditions when short-term bench stability is prioritized.