Implementation of a Breast Phantom with Acoustic Properties for Ultrasonic Thermometry
Abstract
1. Introduction
2. Theoretical Framework
2.1. Thermometry as a Method of Diagnosis and Detection of Breast Anomalies
2.2. Breast Thermal and Ultrasonic Properties of Interest
2.3. Review of Ultrasonic Phantoms: Properties of the Materials and Protocols Used for the Construction of Phantoms, Oriented Towards Thermal Applications
2.4. Method for Determining Temperature in Phantoms
3. Methodology
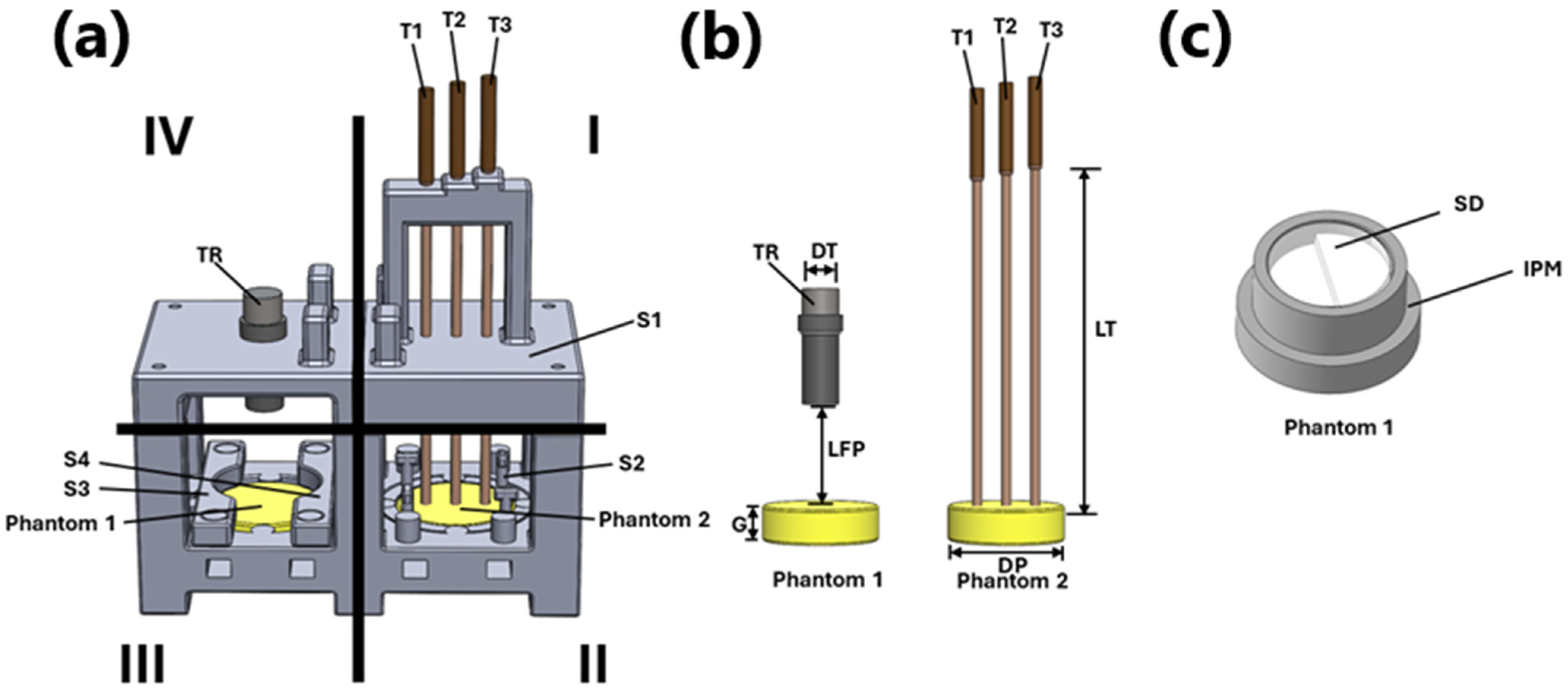
3.1. Protocol for the Elaboration of the Thermometry Phantom
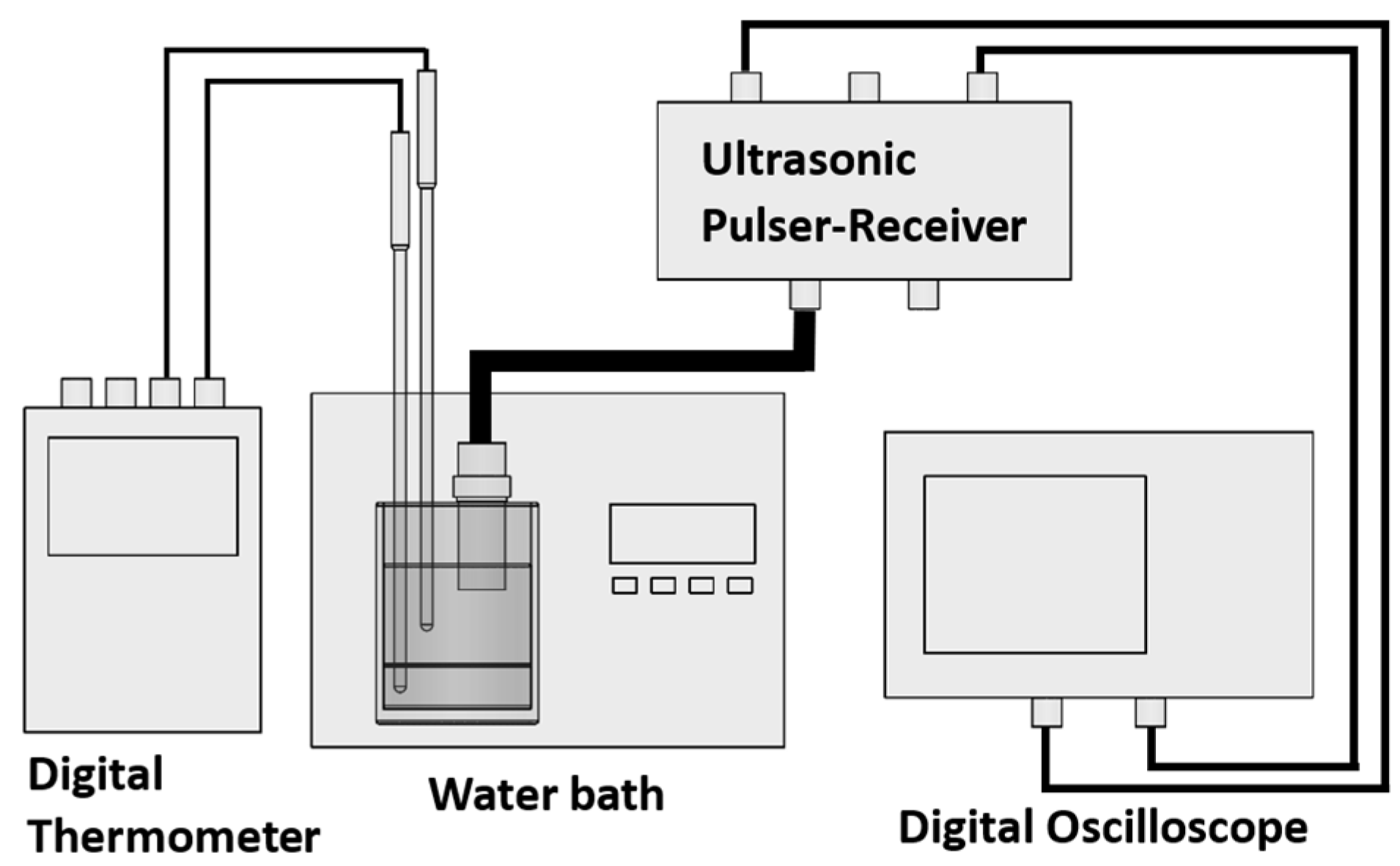
3.2. System Setup and Ultrasonic Signal Acquisition
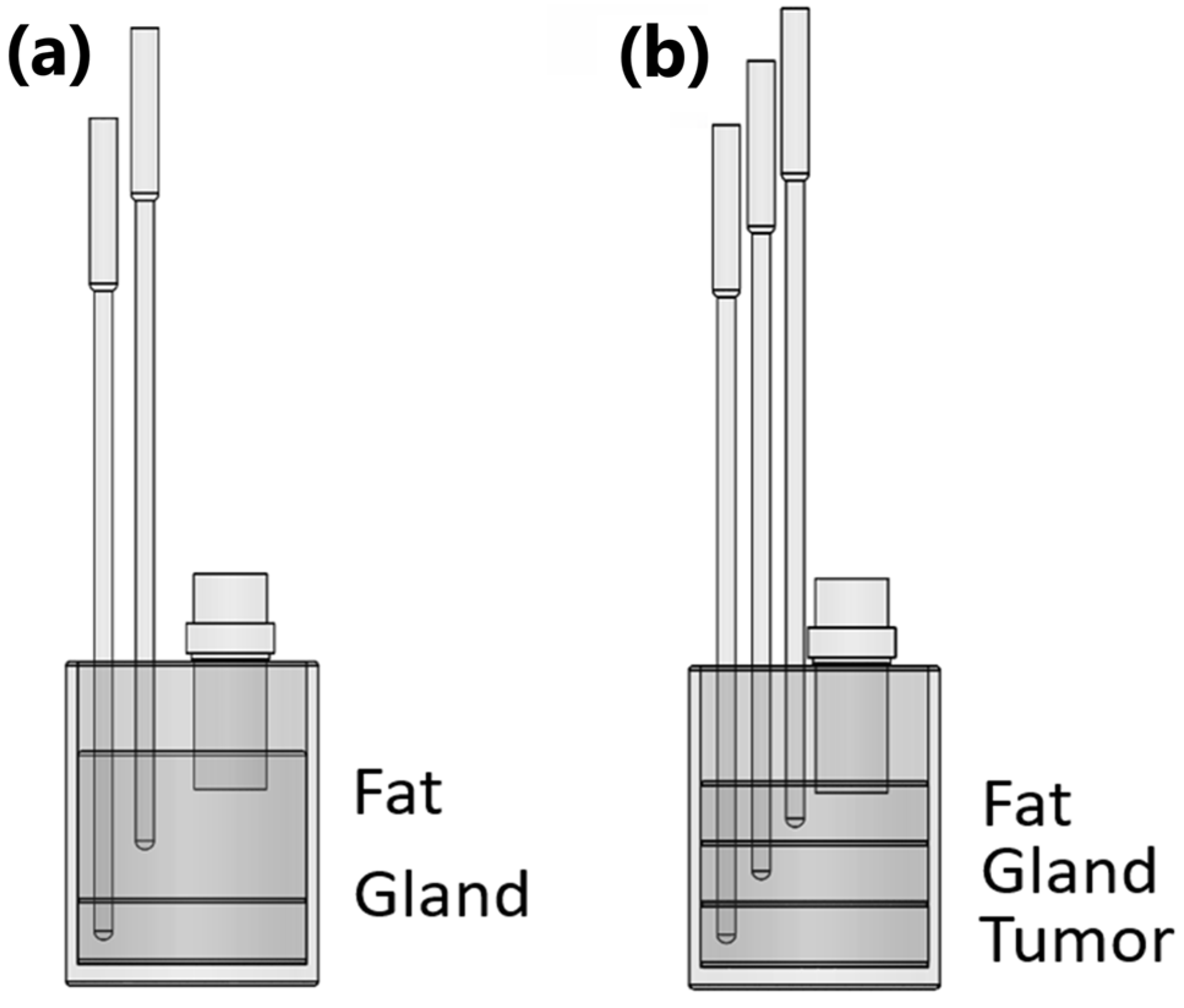
3.2.1. Design of Experiment: Single-Layer Phantom
3.2.2. Design of Experiment: Bilayer and Tri-Layer Phantoms
3.2.3. Signal Acquisition Protocol
3.2.4. Signal Conditioning
3.3. Methods for Determining the Speed of Sound in Phantoms
3.3.1. Single-Layer SOS Characterization
3.3.2. Bilayer SOS Characterization
3.3.3. Tri-Layer SOS Characterization
4. Results
4.1. Results of Phantom Elaboration
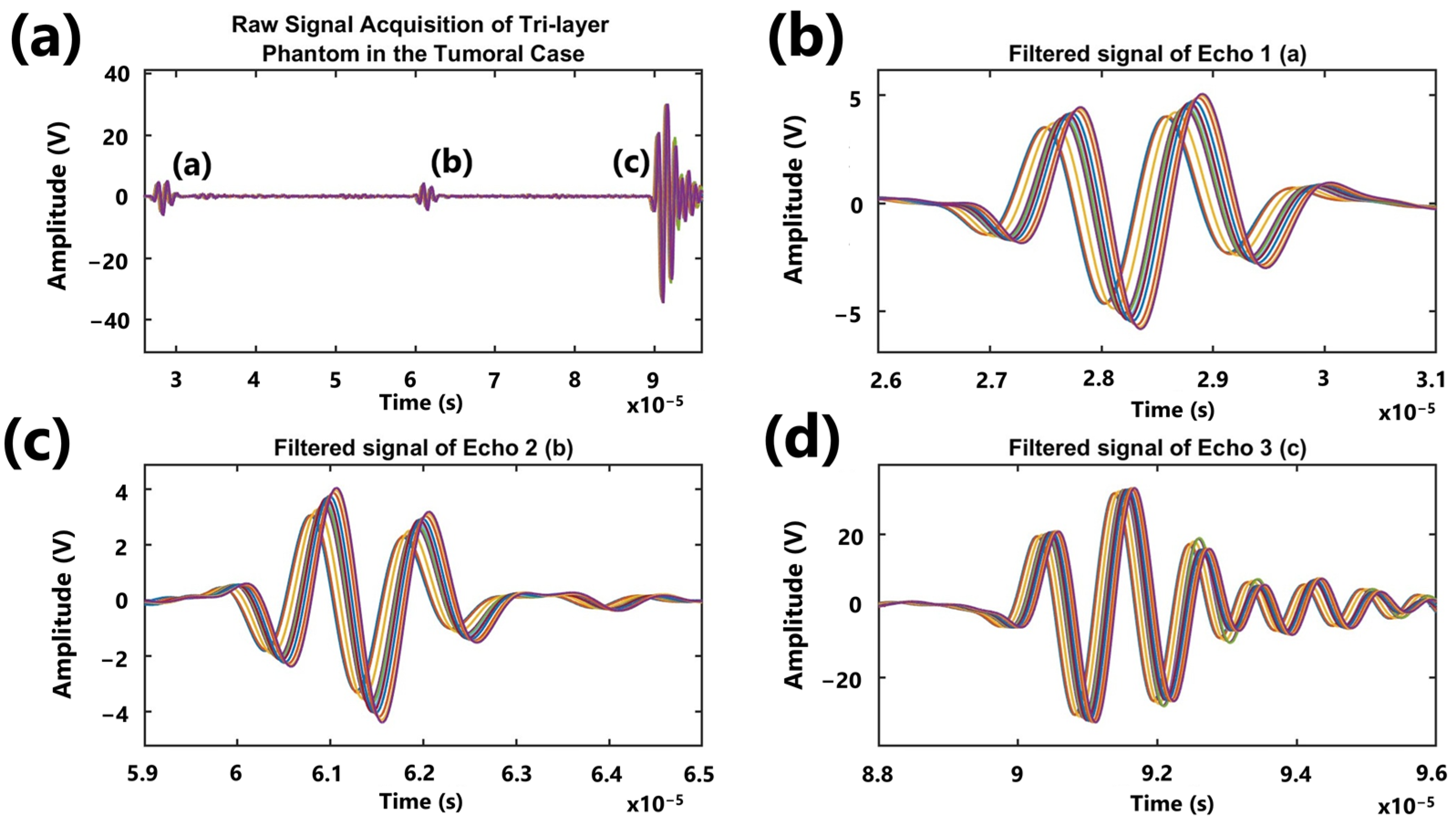
4.2. Results of Signal Acquisition
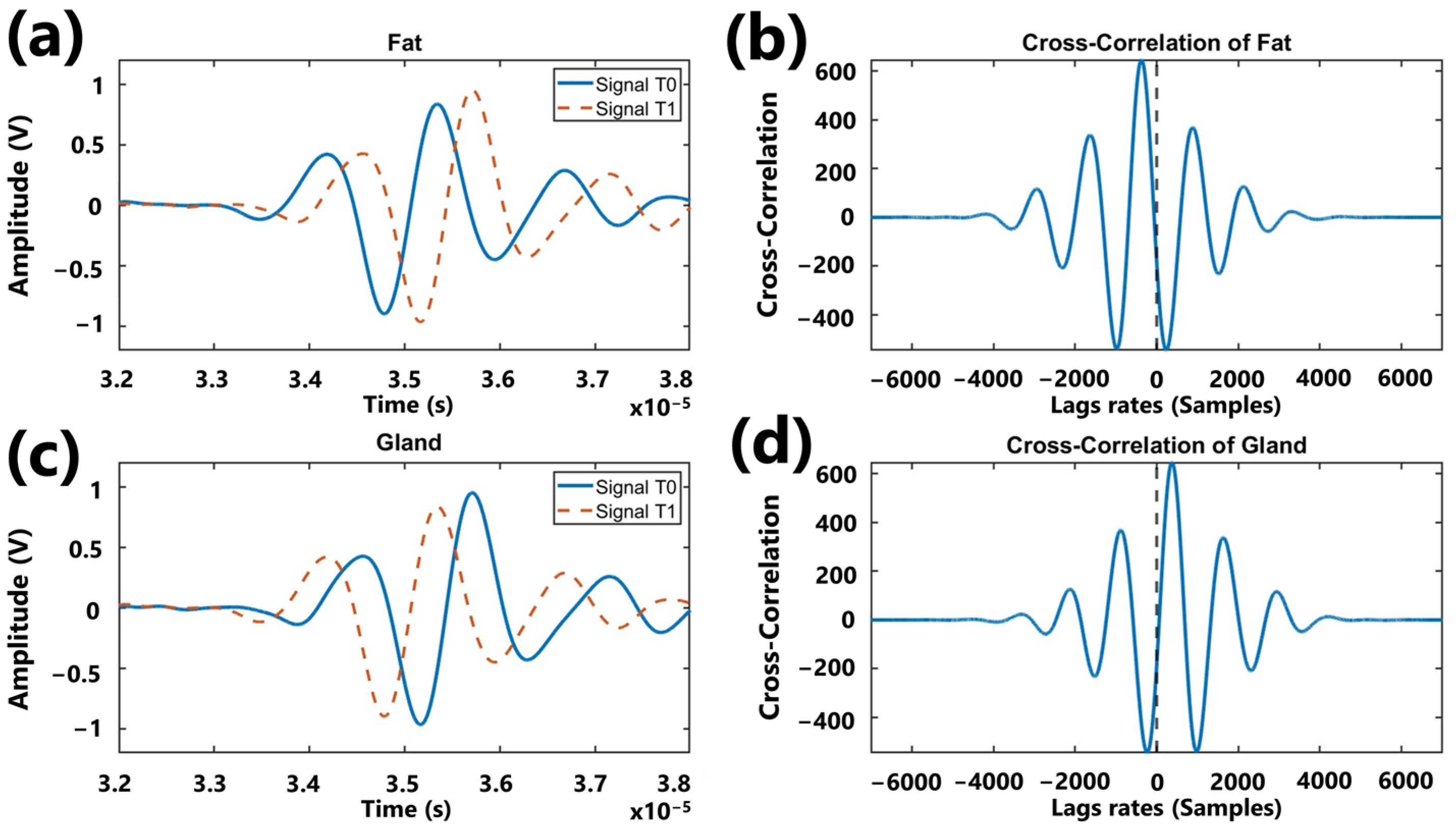
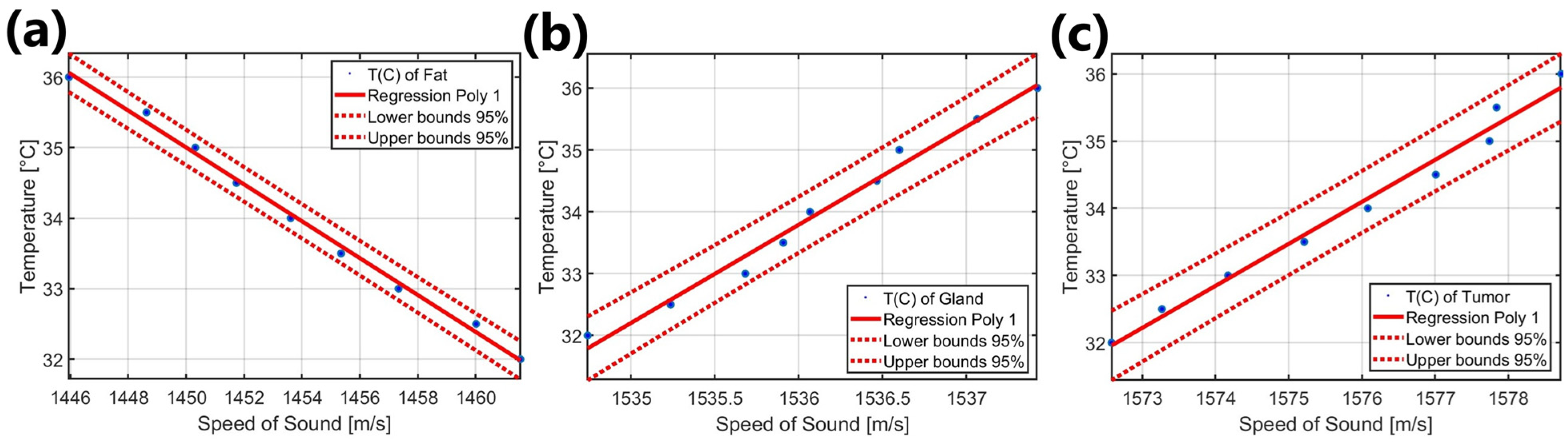
4.3. Results of Signal Processing
5. Discussion
Comparative Analysis with Commercial Brands
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Sung, H.; Ferlay, J.; Siegel, R.L.; Laversanne, M.; Soerjomataram, I.; Jemal, A.; Bray, F. Global Cancer Statistics 2020: GLOBOCAN Estimates of Incidence and Mortality Worldwide for 36 Cancers in 185 Countries. CA Cancer J. Clin. 2021, 71, 209–249. [Google Scholar] [CrossRef]
- Yuan, Y.; Wang, Q.; Tan, Y.; Rong, L.; Tai, S.S.; Ye, L.J.; Liu, Z. Analysis of Breast Diseases Examination with Thermal Texture Mapping, Mammography and Ultrasound. In Proceedings of the 26th Annual International Conference of the IEEE Engineering in Medicine and Biology Society, San Francisco, CA, USA, 1–5 September 2004; Volume 3, pp. 1166–1169. [Google Scholar]
- Mambou, S.J.; Maresova, P.; Krejcar, O.; Selamat, A.; Kuca, K. Breast Cancer Detection Using Infrared Thermal Imaging and a Deep Learning Model. Sensors 2018, 18, 2799. [Google Scholar] [CrossRef] [PubMed]
- Kontos, M.; Wilson, R.; Fentiman, I. Digital Infrared Thermal Imaging (DITI) of Breast Lesions: Sensitivity and Specificity of Detection of Primary Breast Cancers. Clin. Radiol. 2011, 66, 536–539. [Google Scholar] [CrossRef] [PubMed]
- Head, J.F.; Wang, F.; Lipari, C.A.; Elliott, R.L. The Important Role of Infrared Imaging in Breast Cancer. IEEE Eng. Med. Biol. Mag. 2000, 19, 52–57. [Google Scholar] [CrossRef] [PubMed]
- Han, F.; Shi, G.; Liang, C.; Wang, L.; Li, K. A Simple and Efficient Method for Breast Cancer Diagnosis Based on Infrared Thermal Imaging. Cell Biochem. Biophys. 2015, 71, 491–498. [Google Scholar] [CrossRef]
- Qi, H.; Diakides, N.A. Thermal Infrared Imaging in Early Breast Cancer Detection-a Survey of Recent Research. In Proceedings of the 25th Annual International Conference of the IEEE Engineering in Medicine and Biology Society (IEEE Cat. No.03CH37439), Cancun, Mexico, 17–21 September 2003; Volume 2, pp. 1109–1112. [Google Scholar]
- Paulsen, K.D.; Moskowitz, M.J.; Ryan, T.P.; Mitchell, S.E.; Hoopes, P.J. Initial in Vivo Experience with EIT as a Thermal Estimator during Hyperthermia. Int. J. Hyperth. 1996, 12, 573–591. [Google Scholar] [CrossRef]
- Meaney, P.M.; Paulsen, K.D.; Hartov, A.; Crane, R.K. Microwave Imaging for Tissue Assessment: Initial Evaluation in Multitarget Tissue-Equivalent Phantoms. IEEE Trans. Biomed. Eng. 1996, 43, 878–890. [Google Scholar] [CrossRef]
- Jolesz, F.A. MRI-Guided Focused Ultrasound Surgery. Annu. Rev. Med. 2009, 60, 417–430. [Google Scholar] [CrossRef]
- Burfeindt, M.J.; Colgan, T.J.; Mays, R.O.; Shea, J.D.; Behdad, N.; Van Veen, B.D.; Hagness, S.C. MRI-Derived 3-D-Printed Breast Phantom for Microwave Breast Imaging Validation. IEEE Antennas Wirel. Propag. Lett. 2012, 11, 1610–1613. [Google Scholar] [CrossRef]
- Merckel, L.G.; Knuttel, F.M.; Deckers, R.; van Dalen, T.; Schubert, G.; Peters, N.H.G.M.; Weits, T.; van Diest, P.J.; Mali, W.P.T.M.; Vaessen, P.H.H.B.; et al. First Clinical Experience with a Dedicated MRI-Guided High-Intensity Focused Ultrasound System for Breast Cancer Ablation. Eur. Radiol. 2016, 26, 4037–4046. [Google Scholar] [CrossRef]
- Keenan, K.E.; Wilmes, L.J.; Aliu, S.O.; Newitt, D.C.; Jones, E.F.; Boss, M.A.; Stupic, K.F.; Russek, S.E.; Hylton, N.M. Design of a Breast Phantom for Quantitative MRI. J. Magn. Reson. Imaging 2016, 44, 610–619. [Google Scholar] [CrossRef] [PubMed]
- Liney, G.P.; Tozer, D.J.; Turnbull, L.W. A Simple and Realistic Tissue-Equivalent Breast Phantom for MRI. J. Magn. Reson. Imaging 1999, 10, 968–971. [Google Scholar] [CrossRef]
- Lutz, N.W.; Bernard, M. Contactless Thermometry by MRI and MRS: Advanced Methods for Thermotherapy and Biomaterials. iScience 2020, 23, 101561. [Google Scholar] [CrossRef]
- Ruschin, M.; Davidson, S.R.H.; Phounsy, W.; Yoo, T.S.; Chin, L.; Pignol, J.-P.; Ravi, A.; McCann, C. Technical Note: Multipurpose CT, Ultrasound, and MRI Breast Phantom for Use in Radiotherapy and Minimally Invasive Interventions. Med. Phys. 2016, 43, 2508–2514. [Google Scholar] [CrossRef]
- Pernot, M.; Tanter, M.; Bercoff, J.; Waters, K.R.; Fink, M. Temperature Estimation Using Ultrasonic Spatial Compound Imaging. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2004, 51, 606–615. [Google Scholar] [CrossRef] [PubMed]
- Daschner, R.; Hewener, H.; Bost, W.; Weber, S.; Tretbar, S.; Fournelle, M. Ultrasound Thermometry for HIFU-Therapy. Curr. Dir. Biomed. Eng. 2021, 7, 554–557. [Google Scholar] [CrossRef]
- Van Dongen, K.W.A.; Verweij, M.D. A Feasibility Study for Non-Invasive Thermometry Using Non-Linear Ultrasound. Int. J. Hyperth. 2011, 27, 612–624. [Google Scholar] [CrossRef]
- Lai, C.-Y.; Kruse, D.E.; Caskey, C.F.; Stephens, D.N.; Sutcliffe, P.L.; Ferrara, K.W. Noninvasive Thermometry Assisted by a Dual-Function Ultrasound Transducer for Mild Hyperthermia. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2010, 57, 2671–2684. [Google Scholar] [CrossRef]
- Wolf, M.; Rath, K.; Ruiz, A.E.R.; Kühnicke, E. Ultrasound Thermometry for Optimizing Heat Supply During a Hyperthermia Therapy of Cancer Tissue. Phys. Procedia 2015, 70, 888–891. [Google Scholar] [CrossRef]
- Sethuraman, S.; Anand, A.; Li, J. Integrated Ultrasound Thermometry and Multiphysics Modeling for Liver RF Ablation Monitoring: Ex Vivo Studies. In Proceedings of the 2014 IEEE International Ultrasonics Symposium, Chicago, IL, USA, 3–6 September 2014; pp. 1650–1653. [Google Scholar]
- Arthur, R.M.; Straube, W.L.; Starman, J.D.; Moros, E.G. Noninvasive Temperature Estimation Based on the Energy of Backscattered Ultrasound. Med. Phys. 2003, 30, 1021–1029. [Google Scholar] [CrossRef]
- Arthur, R.M.; Trobaugh, J.W.; Straube, W.L.; Moros, E.G. Temperature Dependence of Ultrasonic Backscattered Energy in Motion-Compensated Images. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2005, 52, 1644–1652. [Google Scholar] [CrossRef]
- Georg, O.; Wilkens, V. Non-Invasive Estimation of Temperature Using Diagnostic Ultrasound during HIFU Therapy. In Proceedings of the 13th International Symposium on Therapeutic Ultrasound, Shanghai, China, 12–15 May 2013; American Institute of Physics: Melville, NY, USA, 2017; Volume 1816, pp. 040002-1–040002-5. [Google Scholar]
- Li, S.; Zhou, Z.; Wu, S.; Wu, W. A Review of Quantitative Ultrasound-Based Approaches to Thermometry and Ablation Zone Identification Over the Past Decade. Ultrason. Imaging 2022, 44, 213–228. [Google Scholar] [CrossRef] [PubMed]
- Arthur, R.M.; Straube, W.L.; Trobaugh, J.W.; Moros, E.G. Non-Invasive Estimation of Hyperthermia Temperatures with Ultrasound. Int. J. Hyperth. 2005, 21, 589–600. [Google Scholar] [CrossRef] [PubMed]
- Ng, S.Y.; Kuo, Y.-L.; Lin, C.-L. Low-Cost and Easily Fabricated Ultrasound-Guided Breast Phantom for Breast Biopsy Training. Appl. Sci. 2021, 11, 7728. [Google Scholar] [CrossRef]
- Jawli, A.; Aldehani, W.; Nabi, G.; Huang, Z. Tissue-Mimicking Material Fabrication and Properties for Multiparametric Ultrasound Phantoms: A Systematic Review. Bioengineering 2024, 11, 620. [Google Scholar] [CrossRef]
- Geoghegan, R.; Santamaria, A.; Priester, A.; Zhang, L.; Wu, H.; Grundfest, W.; Marks, L.; Natarajan, S. A Tissue-Mimicking Prostate Phantom for 980 Nm Laser Interstitial Thermal Therapy. Int. J. Hyperth. 2019, 36, 992–1001. [Google Scholar] [CrossRef]
- Negussie, A.H.; Partanen, A.; Mikhail, A.S.; Xu, S.; Abi-Jaoudeh, N.; Maruvada, S.; Wood, B.J. Thermochromic Tissue-Mimicking Phantom for Optimisation of Thermal Tumour Ablation. Int. J. Hyperth. 2016, 32, 239–243. [Google Scholar] [CrossRef]
- Jawli, A.; Nabi, G.; Huang, Z. A Polyvinyl Alcohol (PVA)-Based Phantom for Prostate Cancer Detection Using Multiparametric Ultrasound: A Validation Study. Bioengineering 2024, 11, 1052. [Google Scholar] [CrossRef]
- Li, S.; Fear, E.; Curiel, L. Breast Tissue Mimicking Phantoms for Combined Ultrasound and Microwave Imaging. Phys. Med. Biol. 2021, 66, 245011. [Google Scholar] [CrossRef]
- Polyakov, M.V.; Sirotin, D.S. A Realistic Breast Phantom for Investigating the Features of the Microwave Radiometry Method Using Mathematical and Physical Modelling. Technologies 2025, 13, 106. [Google Scholar] [CrossRef]
- Särestöniemi, M.; Singh, D.; Dessai, R.; Heredia, C.; Myllymäki, S.; Myllylä, T. Realistic 3D Phantoms for Validation of Microwave Sensing in Health Monitoring Applications. Sensors 2024, 24, 1975. [Google Scholar] [CrossRef]
- Slanina, T.; Nguyen, D.H.; Moll, J.; Krozer, V. Temperature Dependence Studies of Tissue-Mimicking Phantoms for Ultra-Wideband Microwave Breast Tumor Detection. Biomed. Phys. Eng. Express 2022, 8, 055017. [Google Scholar] [CrossRef]
- Antoniou, A.; Evripidou, N.; Georgiou, L.; Chrysanthou, A.; Ioannides, C.; Damianou, C. Tumor Phantom Model for MRI-Guided Focused Ultrasound Ablation Studies. Med. Phys. 2023, 50, 5956–5968. [Google Scholar] [CrossRef] [PubMed]
- Filippou, A.; Damianou, C. Agar-Based Phantom for Evaluating Targeting of High-Intensity Focused Ultrasound Systems for Breast Ablation. J. Med. Phys. 2024, 49, 343–355. [Google Scholar] [CrossRef] [PubMed]
- Daniels, M.J.; Varghese, T.; Madsen, E.L.; Zagzebski, J.A. Non-Invasive Ultrasound-Based Temperature Imaging for Monitoring Radiofrequency Heating—Phantom Results. Phys. Med. Biol. 2007, 52, 4827–4843. [Google Scholar] [CrossRef] [PubMed]
- Lai, C.-Y.; Kruse, D.E.; Ferrara, K.W.; Caskey, C.F. Creation and Characterization of an Ultrasound and CT Phantom for Noninvasive Ultrasound Thermometry Calibration. IEEE Trans. Biomed. Eng. 2014, 61, 502–512. [Google Scholar] [CrossRef]
- Mansfel’d, A.D. Acoustothermometry: Current Status and Prospects. Acoust. Phys. 2009, 55, 556–566. [Google Scholar] [CrossRef]
- Amiri, H.; Makkiabadi, B. A Review of Ultrasound Thermometry Techniques. Front. Biomed. Technol. 2020, 7, 82–91. [Google Scholar] [CrossRef]
- Maass-Moreno, R.; Damianou, C.A.; Sanghvi, N.T. Noninvasive Temperature Estimation in Tissue via Ultrasound Echo-Shifts. Part II. In Vitro Study. J. Acoust. Soc. Am. 1996, 100, 2522–2530. [Google Scholar] [CrossRef]
- Abolhassani, M.D.; Norouzy, A.; Takavar, A.; Ghanaati, H. Noninvasive Temperature Estimation Using Sonographic Digital Images. J. Ultrasound Med. 2007, 26, 215–222. [Google Scholar] [CrossRef]
- Liu, H.-L.; Li, M.-L.; Shih, T.-C.; Huang, S.-M.; Lu, I.-Y.; Lin, D.-Y.; Lin, S.-M.; Ju, K.-C. Instantaneous Frequency-Based Ultrasonic Temperature Estimation During Focused Ultrasound Thermal Therapy. Ultrasound Med. Biol. 2009, 35, 1647–1661. [Google Scholar] [CrossRef] [PubMed]
- Pouch, A.M.; Cary, T.W.; Schultz, S.M.; Sehgal, C.M. In Vivo Noninvasive Temperature Measurement by B-Mode Ultrasound Imaging. J. Ultrasound Med. 2010, 29, 1595–1606. [Google Scholar] [CrossRef]
- Huang, C.-W.; Lien, D.-H.; Chen, B.-T.; Shieh, J.; Tsui, P.-H.; Chen, C.-S.; Chen, W.-S. Ultrasound Thermal Mapping Based on a Hybrid Method Combining Cross-Correlation and Zero-Crossing Tracking. J. Acoust. Soc. Am. 2013, 134, 1530–1540. [Google Scholar] [CrossRef]
- Geng, X.; Zhou, Z.; Li, Q.; Wu, S.; Wang, C.-Y.; Liu, H.-L.; Chuang, C.-C.; Tsui, P.-H. Comparison of Ultrasound Temperature Imaging with Infrared Thermometry during Radio Frequency Ablation. Jpn. J. Appl. Phys. 2014, 53, 047001. [Google Scholar] [CrossRef]
- Bayat, M.; Ballard, J.R.; Ebbini, E.S. Ultrasound Thermography: A New Temperature Reconstruction Model and in Vivo Results. In Proceedings of the 14th International Symposium on Therapeutic Ultrasound, Las Vegas, NV, USA, 2–5 April 2014; American Institute of Physics Inc.: Melville, NY, USA, 2017; Volume 1821, pp. 060004-1–060004-5. [Google Scholar]
- Simon, C.; VanBaren, P.; Ebbini, E.S. Two-Dimensional Temperature Estimation Using Diagnostic Ultrasound. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 1998, 45, 1088–1099. [Google Scholar] [CrossRef]
- Arthur, R.M.; Basu, D.; Guo, Y.; Trobaugh, J.W.; Moros, E.G. 3-D in Vitro Estimation of Temperature Using the Change in Backscattered Ultrasonic Energy. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2010, 57, 1724–1733. [Google Scholar] [CrossRef] [PubMed]
- Gambin, B.; Kruglenko, E. Temperature Measurement by Statistical Parameters of Ultrasound Signal Backscattered from Tissue Samples. Acta Phys. Pol. A 2015, 128, A-72–A-78. [Google Scholar] [CrossRef]
- Byra, M.; Gambin, B. Temperature Detection Based on Nonparametric Statistics of Ultrasound Echoes. Hydroacoustics 2015, 18, 17–23. [Google Scholar]
- Katz-Hanani, I.; Rothstein, T.; Gaitini, D.; Gallimidi, Z.; Azhari, H. Age-Related Ultrasonic Properties of Breast Tissue In Vivo. Ultrasound Med. Biol. 2014, 40, 2265–2271. [Google Scholar] [CrossRef]
- Li, C.; Duric, N.; Littrup, P.; Huang, L. In Vivo Breast Sound-Speed Imaging with Ultrasound Tomography. Ultrasound Med. Biol. 2009, 35, 1615–1628. [Google Scholar] [CrossRef]
- Said Camilleri, J.; Farrugia, L.; Curto, S.; Rodrigues, D.B.; Farina, L.; Caruana Dingli, G.; Bonello, J.; Farhat, I.; Sammut, C.V. Review of Thermal and Physiological Properties of Human Breast Tissue. Sensors 2022, 22, 3894. [Google Scholar] [CrossRef] [PubMed]
- Miaskowski, A.; Sawicki, B. Magnetic Fluid Hyperthermia Modeling Based on Phantom Measurements and Realistic Breast Model. IEEE Trans. Biomed. Eng. 2013, 60, 1806–1813. [Google Scholar] [CrossRef] [PubMed]
- Madsen, E.L.; Zagzebski, J.A.; Banjavie, R.A.; Jutila, R.E. Tissue Mimicking Materials for Ultrasound Phantoms. Med. Phys. 1978, 5, 391–394. [Google Scholar] [CrossRef]
- Burlew, M.M.; Madsen, E.L.; Zagzebski, J.A.; Banjavic, R.A.; Sum, S.W. A New Ultrasound Tissue-Equivalent Material. Radiology 1980, 134, 517–520. [Google Scholar] [CrossRef]
- Huang, J.; Holt, R.G.; Cleveland, R.O.; Roy, R.A. Experimental Validation of a Tractable Numerical Model for Focused Ultrasound Heating in Flow-through Tissue Phantoms. J. Acoust. Soc. Am. 2004, 116, 2451–2458. [Google Scholar] [CrossRef] [PubMed]
- Dang, J.; Frisch, B.; Lasaygues, P.; Zhang, D.; Tavernier, S.; Felix, N.; Lecoq, P.; Auffray, E.; Varela, J.; Mensah, S.; et al. Development of an Anthropomorphic Breast Phantom for Combined PET, B-Mode Ultrasound and Elastographic Imaging. IEEE Trans. Nucl. Sci. 2011, 58, 660–667. [Google Scholar] [CrossRef]
- Nguyen, P.T.; Abbosh, A.M.; Crozier, S. Thermo-Dielectric Breast Phantom for Experimental Studies of Microwave Hyperthermia. IEEE Antennas Wirel. Propag. Lett. 2016, 15, 476–479. [Google Scholar] [CrossRef]
- Chen, R.K.; Shih, A.J. Multi-Modality Gellan Gum-Based Tissue-Mimicking Phantom with Targeted Mechanical, Electrical, and Thermal Properties. Phys. Med. Biol. 2013, 58, 5511–5525. [Google Scholar] [CrossRef]
- Menikou, G.; Yiannakou, M.; Yiallouras, C.; Ioannides, C.; Damianou, C. MRI-compatible Breast/Rib Phantom for Evaluating Ultrasonic Thermal Exposures. Int. J. Med. Robot. Comput. Assist. Surg. 2018, 14, e1849. [Google Scholar] [CrossRef]
- He, Y.; Liu, Y.; Dyer, B.A.; Boone, J.M.; Liu, S.; Chen, T.; Zheng, F.; Zhu, Y.; Sun, Y.; Rong, Y.; et al. 3D-Printed Breast Phantom for Multi-Purpose and Multi-Modality Imaging. Quant. Imaging Med. Surg. 2019, 9, 63–74. [Google Scholar] [CrossRef]
- Suzuki, A.; Tsubota, Y.; Wu, W.; Yamanaka, K.; Terada, T.; Otake, Y.; Kawabata, K. Oil Gel-Based Phantom for Evaluating Quantitative Accuracy of Speed of Sound Measured in Ultrasound Computed Tomography. Ultrasound Med. Biol. 2019, 45, 2554–2567. [Google Scholar] [CrossRef]
- Ruvio, G.; Solimene, R.; Cuccaro, A.; Fiaschetti, G.; Fagan, A.J.; Cournane, S.; Cooke, J.; Ammann, M.J.; Tobon, J.; Browne, J.E. Multimodal Breast Phantoms for Microwave, Ultrasound, Mammography, Magnetic Resonance and Computed Tomography Imaging. Sensors 2020, 20, 2400. [Google Scholar] [CrossRef] [PubMed]
- Li, S.; Fear, E.; Curiel, L. Breast Tissue Mimicking Phantoms for Combined Microwave and Ultrasound Imaging. In Proceedings of the 2020 IEEE International Ultrasonics Symposium (IUS), Las Vegas, NV, USA, 7–11 September 2020; pp. 1–4. [Google Scholar]
- Hariyanto, A.P.; Budiarti, N.T.; Suprijanto; Ng, K.H.; Haryanto, F.; Endarko. Evaluation of Physical Properties and Image of Polyvinyl Chloride as Breast Tissue Equivalence for Dual-Modality (Mammography and Ultrasound). Phys. Eng. Sci. Med. 2023, 46, 1175–1185. [Google Scholar] [CrossRef] [PubMed]
- Maass-Moreno, R.; Damianou, C.A. Noninvasive Temperature Estimation in Tissue via Ultrasound Echo-Shifts. Part I. Analytical Model. J. Acoust. Soc. Am. 1996, 100, 2514–2521. [Google Scholar] [CrossRef] [PubMed]
- Corach, J.; Sorichetti, P.A.; Romano, S.D. Electrical and Ultrasonic Properties of Vegetable Oils and Biodiesel. Fuel 2015, 139, 466–471. [Google Scholar] [CrossRef]
- Corach, J.; Sorichetti, P.A.; Romano, S.D. Electrical Properties of Vegetable Oils between 20 Hz and 2 MHz. Int. J. Hydrog. Energy 2014, 39, 8754–8758. [Google Scholar] [CrossRef]
- Javanaud, C.; Rahalkar, R.R. Velocity of Sound in Vegetable Oils. Lipid/Fett 1988, 90, 73–75. [Google Scholar] [CrossRef]
- Menikou, G.; Damianou, C. Acoustic and Thermal Characterization of Agar Based Phantoms Used for Evaluating Focused Ultrasound Exposures. J. Ther. Ultrasound 2017, 5, 14. [Google Scholar] [CrossRef]
- Sofokleous, P.; Damianou, C. High-Quality Agar and Polyacrylamide Tumor-Mimicking Phantom Models for Magnetic Resonance-Guided Focused Ultrasound Applications. J. Med. Ultrasound 2024, 32, 121–133. [Google Scholar] [CrossRef]
- Koch, T.; Lakshmanan, S.; Brand, S.; Wicke, M.; Raum, K.; Mörlein, D. Ultrasound Velocity and Attenuation of Porcine Soft Tissues with Respect to Structure and Composition: II. Skin and Backfat. Meat Sci. 2011, 88, 67–74. [Google Scholar] [CrossRef]
- Zhang, S.; Gao, J. Studying on the Thermal Conductivity Measurement of Biological Tissues. In Emerging Computation and Information Technologies for Education; Mao, E., Xu, L., Tian, W., Eds.; Springer: Berlin/Heidelberg, Germany, 2012; pp. 743–749. [Google Scholar]
- Acero Mendoza, R.V.; Bazán, I.; Ramírez-García, A. Analyzing Temperature Distributions and Gradient Behaviors for Early-Stage Tumor Lesions in 3D Computational Model of Breast. Appl. Sci. 2024, 14, 4538. [Google Scholar] [CrossRef]
- Sun Nuclear Zerdine Ultrasound Phantoms. Available online: https://www.sunnuclear.com/products/zerdine-ultrasound-phantom (accessed on 24 April 2025).
- Sun Nuclear Urethane Ultrasound Phantoms. Available online: https://www.sunnuclear.com/products/urethane-ultrasound-phantom (accessed on 24 April 2025).
- Sun Nuclear Sun Nuclear Breast Biopsy Phantoms. Available online: https://www.sunnuclear.com/products/breast-biopsy-phantoms (accessed on 24 April 2025).
- Techforhealth The AdvantageTM Phantom. Available online: https://techforhealth.com/product/the-advantage-phantom/ (accessed on 24 April 2025).
- Elevate Healthcare Blue Phantom. Available online: https://elevatehealth.net/solutions/brands/blue-phantom (accessed on 24 April 2025).
- Kyoto Kagaku Breast Ultrasound Examination Phantom “BREAST FAN”. Available online: https://www.kyotokagaku.com/en/products_data/us-6/ (accessed on 24 April 2025).
- GTSimulators Breast Biopsy Ultrasound Training Model. Available online: https://www.gtsimulators.com/collections/cae-blue-phantom-cae-healthcare/products/breast-biopsy-ultrasound-training-model-bpb170 (accessed on 24 April 2025).
- GTSimulators Breast Biopsy Training Model. Available online: https://www.gtsimulators.com/collections/kyoto-kagaku-ultrasound-phantoms/products/breast-biopsy-training-model-duo-set-kkus-9 (accessed on 24 April 2025).



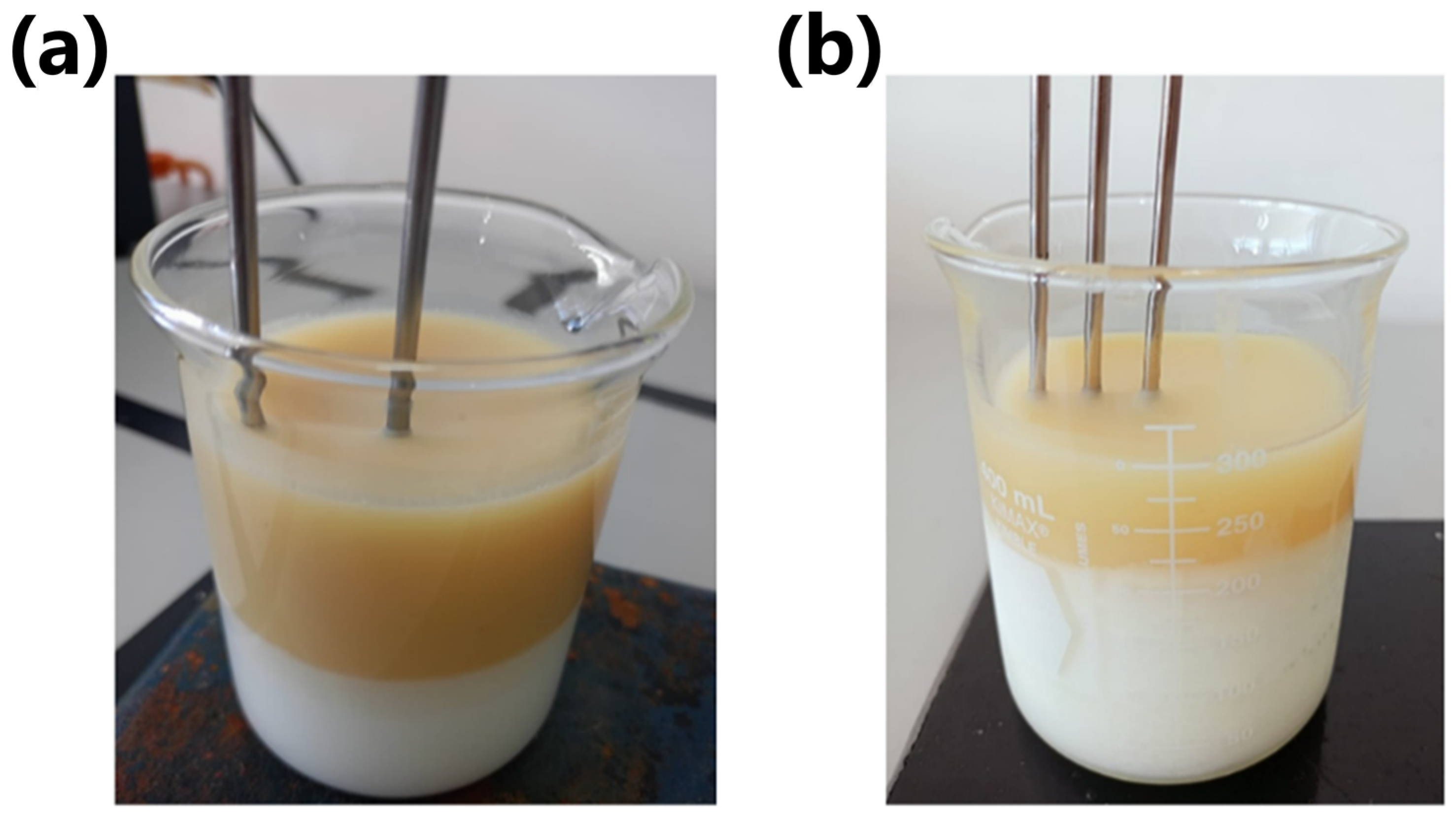
| Reagents | Gland | Tumor |
|---|---|---|
| Water (gr) | 100 | 150 |
| Agar (gr) | 2 | 3 |
| Oil (gr) | 15 | 26.78 |
| Salt (gr) | 0 | 5 |
| Detergent (drops) | 3–4 | 5–6 |
| Temperature (°C) | Fat | Gland | Tumor | |||
|---|---|---|---|---|---|---|
| Mean | STD | Mean | STD | Mean | STD | |
| 32.0 | 1461.55 | 14.78 | 1534.74 | 23.22 | 1572.58 | 21.48 |
| 32.5 | 1460.02 | 14.22 | 1535.24 | 22.78 | 1573.27 | 20.63 |
| 33.0 | 1457.34 | 14.07 | 1535.68 | 22.75 | 1574.17 | 19.61 |
| 33.5 | 1455.35 | 13.64 | 1535.91 | 22.86 | 1575.21 | 18.21 |
| 34.0 | 1453.61 | 12.23 | 1536.07 | 22.93 | 1576.08 | 17.02 |
| 34.5 | 1451.74 | 11.40 | 1536.47 | 22.68 | 1577.01 | 16.02 |
| 35.0 | 1450.32 | 10.33 | 1536.60 | 22.80 | 1577.74 | 14.86 |
| 35.5 | 1448.63 | 9.75 | 1537.07 | 22.54 | 1577.84 | 14.77 |
| 36.0 | 1445.95 | 7.84 | 1537.42 | 22.32 | 1578.72 | 13.47 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Acero Mendoza, R.V.; Bazán, I.; Ramírez-García, A. Implementation of a Breast Phantom with Acoustic Properties for Ultrasonic Thermometry. Appl. Sci. 2025, 15, 5275. https://doi.org/10.3390/app15105275
Acero Mendoza RV, Bazán I, Ramírez-García A. Implementation of a Breast Phantom with Acoustic Properties for Ultrasonic Thermometry. Applied Sciences. 2025; 15(10):5275. https://doi.org/10.3390/app15105275
Chicago/Turabian StyleAcero Mendoza, Ruth Valeria, Ivonne Bazán, and Alfredo Ramírez-García. 2025. "Implementation of a Breast Phantom with Acoustic Properties for Ultrasonic Thermometry" Applied Sciences 15, no. 10: 5275. https://doi.org/10.3390/app15105275
APA StyleAcero Mendoza, R. V., Bazán, I., & Ramírez-García, A. (2025). Implementation of a Breast Phantom with Acoustic Properties for Ultrasonic Thermometry. Applied Sciences, 15(10), 5275. https://doi.org/10.3390/app15105275






