Next Article in Journal
Applications of Linear and Ensemble-Based Machine Learning for Predicting Winning Teams in League of Legends
Previous Article in Journal
Different Approaches to Artificial Intelligence–Based Predictive Maintenance on an Axle Test Bench with Highly Varying Tests
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Applied Sciences
Volume 15
Issue 10
10.3390/app15105238
applsci-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Mechanical Response Mechanism and Yield Characteristics of Coal Under Quasi-Static and Dynamic Loading

by
Liupeng Huo
1,
Feng Gao
1,* and
Yan Xing
2
1
State Key Laboratory of Intelligent Construction and Healthy Operation and Maintenance of Deep Underground Engineering, China University of Mining and Technology, Xuzhou 221116, China
2
School of Mechanics and Civil Engineering, China University of Mining and Technology, Xuzhou 221116, China
*
Author to whom correspondence should be addressed.
Appl. Sci. 2025, 15(10), 5238; https://doi.org/10.3390/app15105238
Submission received: 17 March 2025 / Revised: 27 April 2025 / Accepted: 29 April 2025 / Published: 8 May 2025
Browse Figures
Versions Notes

Abstract

:
During deep mining engineering, coal bodies are subjected to complex geological stresses such as periodic roof pressure and blasting impacts, which may induce mechanical property deterioration and trigger severe rock burst accidents. This study systematically investigated the mechanical characteristics and failure mechanisms of coal under strain rates on two orders of magnitude through quasi-static cyclic loading–unloading experiments and split Hopkinson pressure bar (SHPB) tests, combined with acoustic emission (AE) localization and crack characteristic stress analysis. The research focused on the differential mechanical responses of coal-rock masses under distinct stress environments in deep mining. The results demonstrated that under quasi-static loading, the stress–strain curve exhibited four characteristic stages: compaction (I), linear elasticity (II), nonlinear crack propagation (III), and post-peak softening (IV). The peak strain displayed linear growth with increasing cycle, accompanied by a failure mode characterized by oblique shear failure that induced a transition from gradual to abrupt increases in the AE counts. In contrast, under the dynamic loading conditions, there was a bifurcated post-peak phase consisting of two unloading stages due to elastic rebound effects, with nonlinear growth of the peak strain and an interlaced failure pattern combining lateral tensile cracks and axial compressive fractures. The two loading conditions exhibited similar evolutionary trends in crack damage stress, though a slight reduction in stress occurred during the final dynamic loading phase due to accumulated damage. Notably, the crack closure stress under quasi-static loading followed a decrease–increase pattern with cycle progression, whereas the dynamic loading conditions presented the inverse increase–decrease tendency. These findings provide theoretical foundations for stability control in underground engineering and prevention of dynamic hazards.

1. Introduction

As the depth of coal resource extraction has increased, the complex geological environment and high-intensity mining disturbances in deep underground operations have significantly elevated the risks of dynamic hazards such as rock burst and roof collapses [1,2,3,4,5,6]. Influenced by advancing working faces, roof fracturing, and blasting activities, deep coal-rock masses undergo continuous loading–unloading effects [7,8], leading to cumulative internal damage and mechanical property degradation of the coal, which serves as a critical trigger for dynamic hazards [9,10,11]. Consequently, elucidating the mechanical responses and failure characteristics of coal rock under cyclic loading–unloading conditions is of paramount theoretical significance for stability control in deep mining goafs and hazard prevention.
Cyclic loading–unloading under varying conditions exerts markedly distinct influences on the mechanical properties and failure behaviors of coal [12,13,14,15]. The current research is predominantly focused on coal mechanics under quasi-static cyclic loading, encompassing uniaxial, biaxial, and true triaxial servo-controlled experiments [16,17,18]. However, the strain rate is typically under 0 s−1 in those studies. But in deep mining, dynamic disturbances like blasting or roof impacts can cause instantaneous strain rates of up to 103 s−1. The strain rates from different disturbances vary widely in magnitude. As a result, the mechanical properties and failure characteristics of coal and rock masses show remarkable differences under different strain rates [19,20,21,22]. Studies have revealed that coal rock under quasi-static loading exhibits progressive damage evolution, whereas dynamic impact loads trigger instantaneous energy accumulation and crack propagation through stress wave transmission, resulting in a transition from simple splitting to pulverizing failure mode [21,22]. For instance, SHPB experiments have demonstrated that increased dynamic loading rates substantially enhance energy dissipation rates in coal and induce brittle fractures dominated by axial tensile stresses [23,24]. Furthermore, the fatigue damage characteristics of coal rock under cyclic dynamic loads (e.g., frequency dependence) fundamentally differ from those under quasi-static loading: low-frequency cycles amplify internal crack network propagation through prolonged energy accumulation, while high-frequency disturbances accelerate localized stress concentration via stress wave superposition [25].
Although current studies have explored coal-rock mechanics under quasi-static and dynamic loading conditions, most studies have focused on a single loading condition. In practice, coal and rock may experience both quasi-static and dynamic loading. Yet, few studies have compared the mechanical properties under these two conditions. Moreover, for deep mining “three-high” environments (high geostress, gas pressure, and temperature), the situation becomes even more complicated [26,27]. Thus, a comparative investigation of the mechanical behaviors and failure characteristics under quasi-static and dynamic loading is imperative, especially a comparative study of the crack characteristic stress and failure modes under the two conditions.
This study investigated coal specimens through quasi-static cyclic loading–unloading experiments under different confining pressures and SHPB dynamic impact tests, systematically analyzing the influence of cyclic loading and unloading cycles on the mechanical properties of coal under these two loading conditions. By contrasting the failure characteristics under quasi-static loading (characterized by AE signals) with those under dynamic loading (evaluated through crack distribution patterns), combined with crack characteristic stress analysis, the failure mechanisms of coal rock under both loading conditions were comprehensively elucidated. The findings provide theoretical insights for the early warning and prevention of dynamic hazards in deep mining operations.

2. Materials and Methods

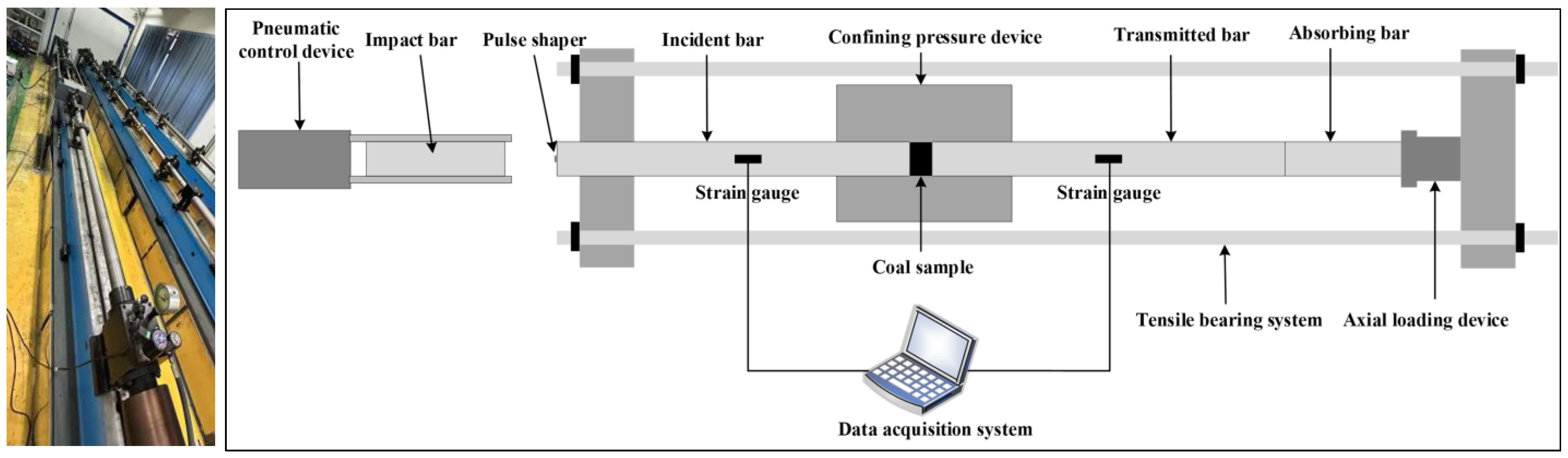
2.1. Materials and Equipment

The anthracite specimens used in the quasi-static loading and dynamic loading experiments were collected from the Da Ling Coal Mine in Ordos City, Inner Mongolia, China. The primary mineral composition of the anthracite was 39.08% quartz, 31.66% albite, 23.3% kaolinite, and 5.96% calcite. Cylindrical specimens with heights of 100 mm and 25 mm, both with a diameter of 50 mm, were prepared for the quasi-static and dynamic tests, respectively. In accordance with the International Society for Rock Mechanics (ISRM)’s recommended standards [28], the samples used for quasi-static loading were processed to dimensions of φ50 mm × 100 mm, while the dynamic samples were φ50 mm × 25 mm, with their non-parallelism and flatness strictly controlled within 0.01 mm and 0.05 mm, respectively. Table 1 shows the dimensions and tolerances of the specimens used in this experiment.
The quasi-static loading experiments were conducted using the TAW-2000 triaxial shear rheometer (Figure 1) developed by Chaoyang Instruments Co., Ltd., (Changchun, China), whereas the dynamic impact tests were performed using the 50 mm split Hopkinson pressure bar (SHPB) system (Figure 2) manufactured by Liwei Technology Co., Ltd. (Luoyang, China).

2.2. Test Methods

Figure 1 and Figure 2 illustrate the experimental setup and key procedures for the quasi-static cyclic loading and dynamic impact tests, respectively. The methodologies are summarized below.
(1)
Quasi-static cyclic loading experiment
Prior to quasi-static cyclic loading, the displacement increment between cycles was determined. Conventional uniaxial compression tests were first conducted to determine the peak stress and displacement of the coal specimens needed, and the peak values of 120.31 kN and 1.603 mm are obtained, respectively. To balance experimental efficiency and cycle number, a displacement increment of 0.2 mm was adopted. The loading protocol employed displacement-controlled loading at a rate of 0.005 mm/s.
(2)
Dynamic loading experiment
As coal strain during impact is governed by gas pressure in the experimental apparatus, gas pressure was selected as the control parameter for the cyclic impacts. Given the gas pressure control precision of 0.1 MPa in the system shown in Figure 2, a pressure increment of 0.3 MPa was implemented to optimize the experiment duration.
Additionally, different confining pressures (0, 10, and 20 MPa) were selected to simulate different depths ranging from approximately 400 m to 800 m. The depth was calculated based on the data in the literature [29,30].

3. Results

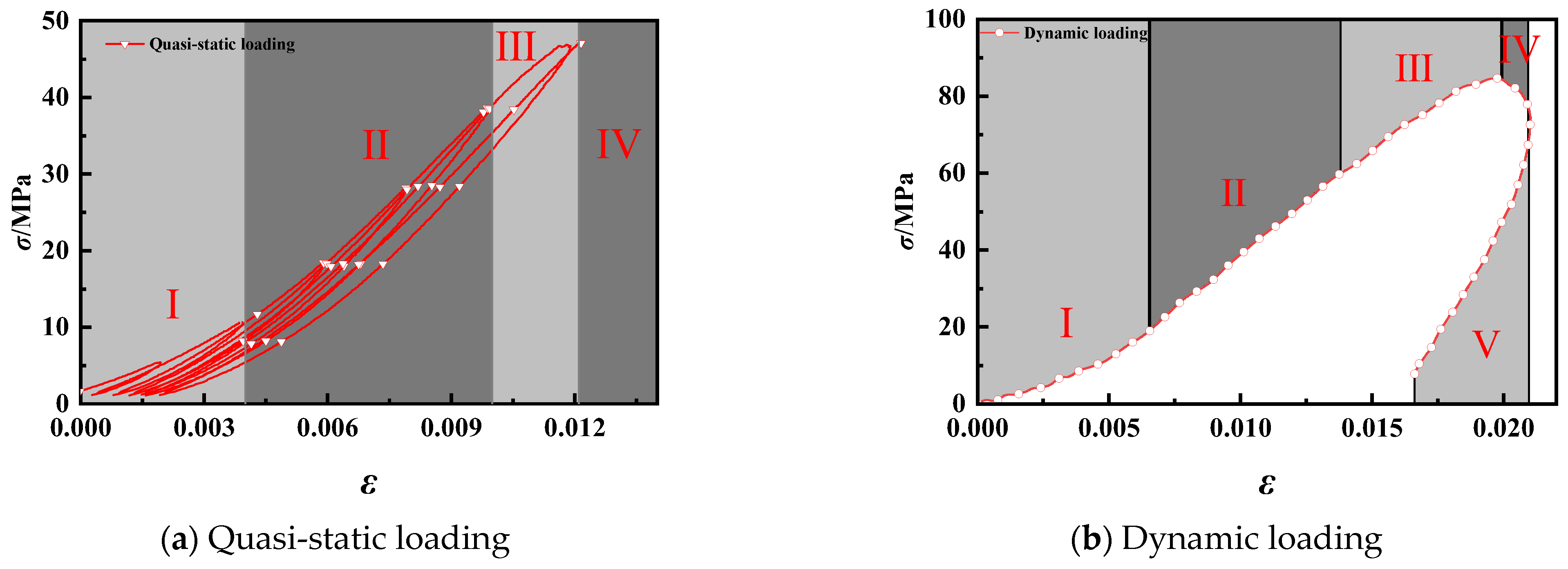
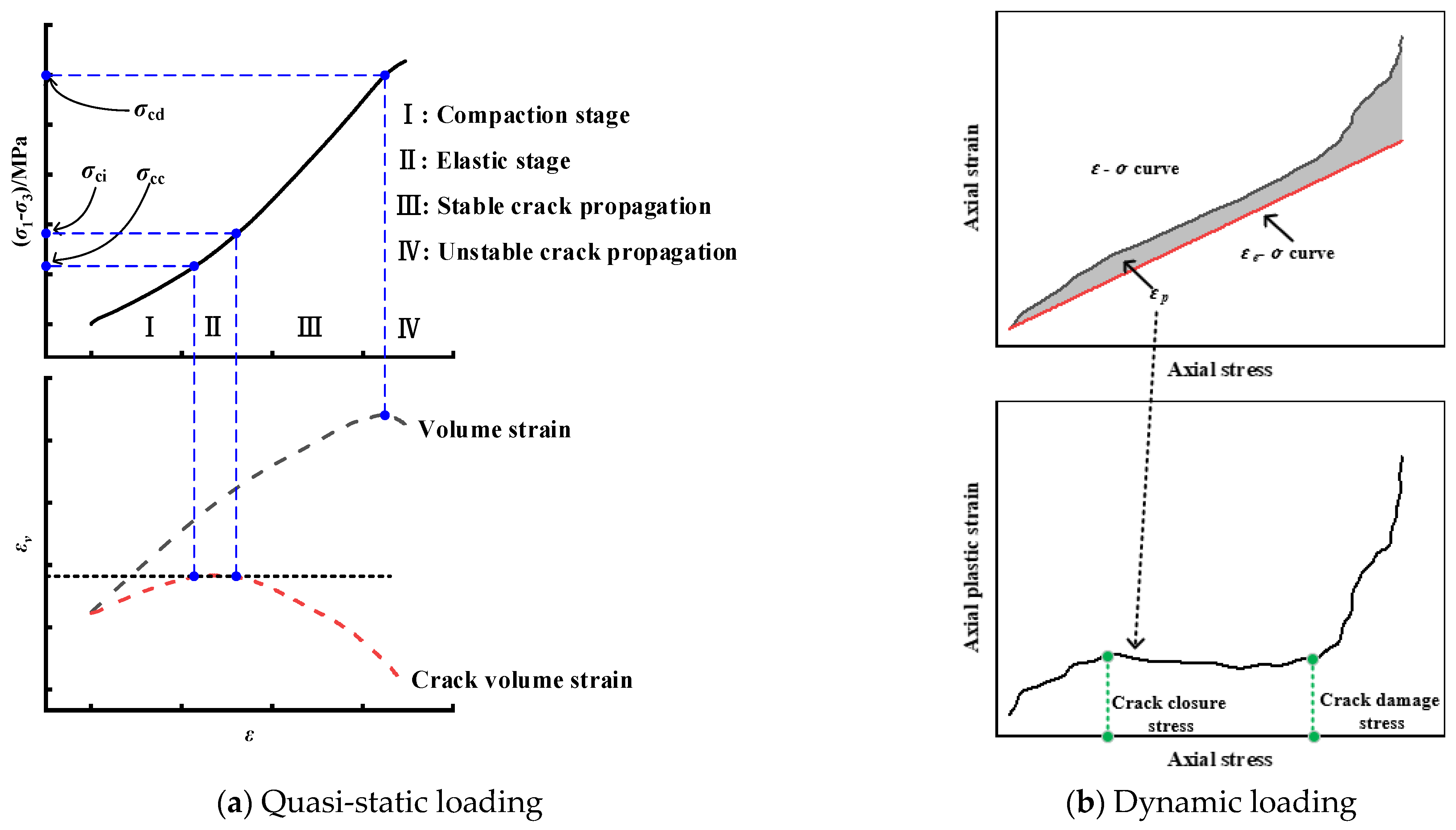
3.1. Comparison of Stress–Strain Curves

Figure 3 shows the stress–strain (σ-ε) curves of the coal specimens under the varying cyclic loading-unloading cycles and confining pressures, with representative curves illustrated in Figure 4. As shown in Figure 3 and Figure 4, under quasi-static cyclic loading-unloading conditions, the axial stress–strain curves can be broadly categorized into four stages: compaction (I), linear elasticity (II), nonlinear crack propagation (III), and post-peak softening (IV). In the quasi-static loading curve of coal (Figure 4a), only compaction stage I was observed during the first two cycles. As the number of cycles increased, the peak stress gradually rose, and the stress–strain curves progressively extended into stage II, as showed in cycles 3–5 in Figure 4a. At the sixth cycle, the σ-ε curve reached stage III, approaching the maximum axial stress the material could sustain. During the seventh loading cycle, brittle failure occurred, marked by a sharp stress drop, corresponding to stage IV. Notably, the number of cycles in stage I remained consistent across the different confining pressures. In contrast, stage II exhibited an increasing number of cycles, manifested by denser σ-ε curves. The cycle count in stage III showed a gradual reduction with increasing confining pressure, indicating the prolongation effect of confining pressure on the failure phase (stage III).
As shown in Figure 4b, under dynamic loading conditions, the axial stress–strain curves differed significantly from those under quasi-static conditions, which were divided into five stages: compaction (I), near-linear elasticity (II), nonlinear crack propagation (III), first unloading process (IV), and second unloading process (V) [31]. The critical distinction lay in the post-peak phase bifurcation into stages IV and V. Stage IV corresponds to the growth of plastic strain, with confining pressure exerting a negligible influence. Stage V represents the rebound deformation phase, characterized by elastic strain release. Elevated confining pressures enhanced the magnitude of stage V, demonstrating stronger rebound effects, which suggests that confining pressure promotes the accumulation of internal elastic strain in coal, thereby inducing greater post-peak elastic energy release [32].

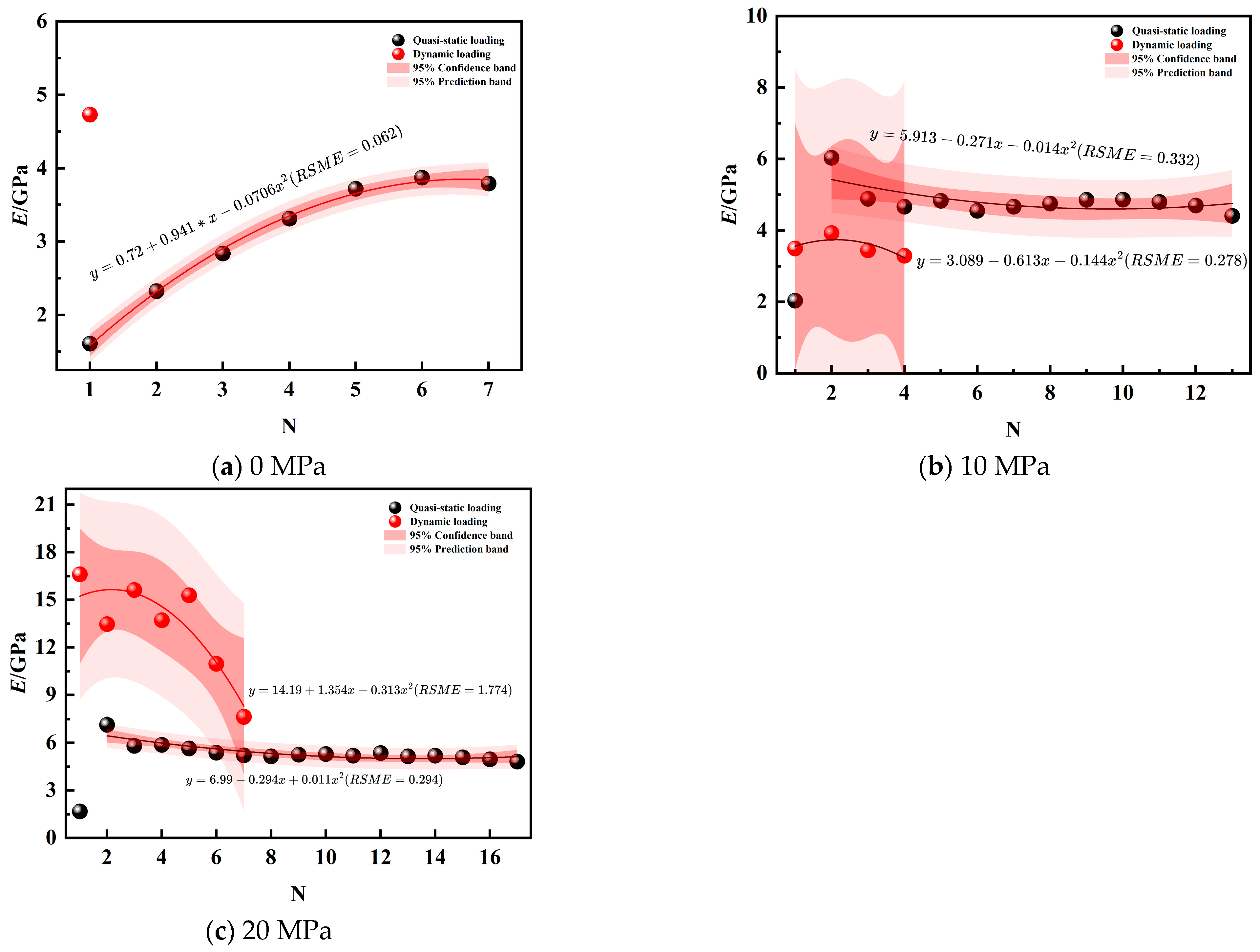
3.2. Comparison of Young’s Modulus Variations Under Quasi-Static and Dynamic Loading

In this study, based on the methodology established by Gong et al. [33], the secant modulus of the second category was adopted to represent Young’s modulus, calculated as the slope of the line connecting the peak stress point and the half-peak stress point. Figure 5 compares the variations in Young’s modulus of coal samples under quasi-static and dynamic loading conditions at different confining pressures. The results indicated that the dynamic Young’s modulus under most confining pressures exceeded the quasi-static modulus, with shorter loading durations under dynamic conditions enhancing material stiffness, consistent with prior studies [34]. However, at the confining pressure of 10 MPa, the dynamic Young’s modulus decreased below the quasi-static value, likely due to extensive internal fissures within the sample that compromised the coal rigidity [35].
For both loading regimes, the Young’s modulus exhibited a similar trend with increasing cycle number N: an initial increase and then decrease. This reduction in deformation resistance at higher N values stems from progressive internal damage under cyclic loading. However, critical transition points (peak modulus) differ between quasi-static and dynamic conditions. Under quasi-static loading, the gradual compaction of internal cracks over extended durations allows for the full mobilization of matrix stiffness, delaying modulus degradation. In contrast, dynamic loading’s transient nature prevents crack closure, limiting stiffness contributions to partial matrix activation. Consequently, the modulus decline initiates earlier under dynamic loading, even before significant damage accumulation [33]. For example, at a confining pressure of 10 MPa, the critical point occurs at N = 4 for quasi-static loading but shifts to N = 2 for dynamic loading. This discrepancy arises from differences in loading increments and elastic strain accumulation rates: dynamic loading accelerates elastic strain storage, advancing the critical transition, while confining pressure amplifies plasticity-dominated responses in later stages.
To analyze the fitting curve and experimental data fitting more thoroughly, this study used RMSE (Root Mean Square Error), 95% confidence bands, and prediction bands to evaluate the fitting curve. Figure 5 shows wider confidence bands under dynamic loading. This is mainly due to the fluctuation in the experimental data under dynamic loading. Various factors like impact-air-pressure control precision, impact-speed fluctuation, and shaper and coupling-agent thickness affected the shock waves, causing experimental data fluctuation in cyclic impacts on the same sample. This leads to a larger confidence interval and greater fitting curve error under dynamic loading compared to quasi-static loading.
Also, the limited number of dynamic loading data samples resulted in broader confidence intervals and an abnormal confidence band distribution. However, the mechanical property fluctuation with impact time under dynamic loading is common and aligns with previous studies. The experimental results showed that the mechanical properties first increased and then decreased with impact time. So, this study only used the fitting curve for the qualitative trend analysis.

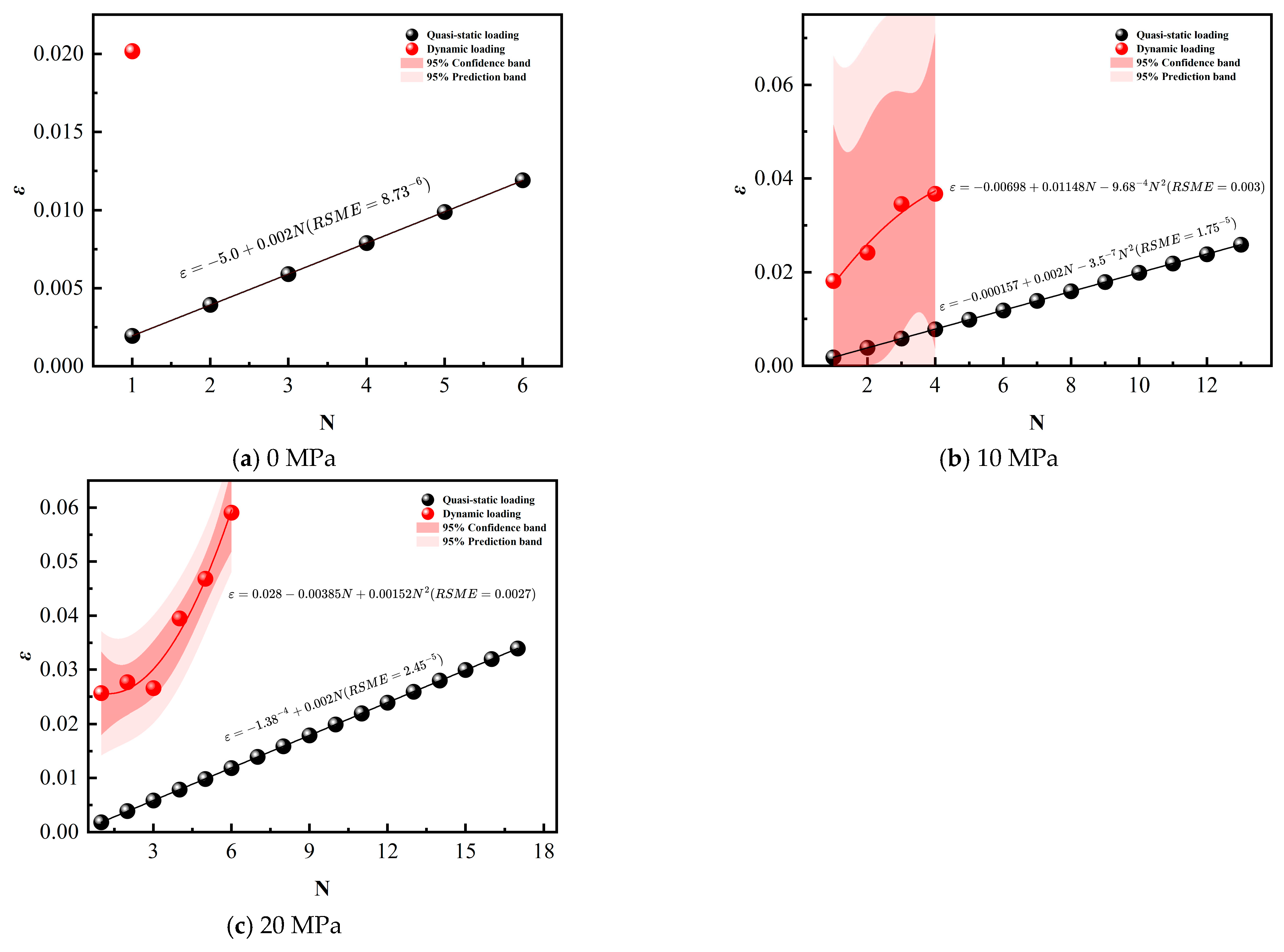
3.3. Comparison of Cyclic Peak Stress and Strain Variations

As shown in Figure 6 and Figure 7, the peak strain and peak stress under quasi-static and dynamic loading conditions were compared across varying confining pressures. With increasing cycle number N, the peak strain of the coal specimens under both loading regimes demonstrated a gradual upward trend. Notably, dynamic loading consistently produced higher peak strains than quasi-static loading, highlighting its pronounced strain rate effects. For instance, under initial dynamic impact (the first cycle), the peak strain increased from 0.020 at a confining pressure of 0 MPa to 0.026 at 20 MPa, indicating enhanced plasticity with elevated confining pressures. Furthermore, while the peak strain under quasi-static loading increased linearly with N, dynamic loading induced a nonlinear growth pattern.
As shown in Figure 6, the 95% confidence and prediction bands decreased with increasing confining pressure. Similar to 3.2, this was due to the dynamic loading experimental data fluctuation and the increased dynamic impact cycles (data points) with rising confining pressure [36]. Under quasi-static loading, there were no obvious 95% confidence and prediction bands. This means the quasi-static experimental data fit the curve well at the 95% confidence level (with R2 = 0.999).
Similar to peak strain evolution, Figure 7 shows that peak stress also rose with increasing N. However, unlike peak strain, peak stress cannot increase indefinitely due to material strength limitations, resulting in a nonlinear trend characterized by a gradually decreasing slope, followed by abrupt post-peak stress reduction. Notably, dynamic loading exhibited a more distinct “rise-then-decline” peak stress trajectory compared to quasi-static loading. This phenomenon likely stemmed from the larger loading increments in dynamic protocols and the accelerated failure processes inherent to high-strain-rate regimes [22].
Figure 7 also shows how increasing the confining pressure affected the 95% confidence and prediction bands. Under dynamic loading, although the samples at 20 MPa were subjected to more impact cycles and thus a larger sample scale, the peak stress fluctuation was greater. This resulted in wider confidence and prediction bands than at 10 MPa. For quasi-static loading, the 95% confidence and prediction bands tended to increase with confining pressure. This was mainly because a higher confining pressure causes more significant stress changes in coal specimens, especially during the yielding and failure stage. The effect of these changes on increasing the confidence interval width outweighs the impact of a larger sample scale on reducing it.

4. Failure Characteristics and Mechanisms

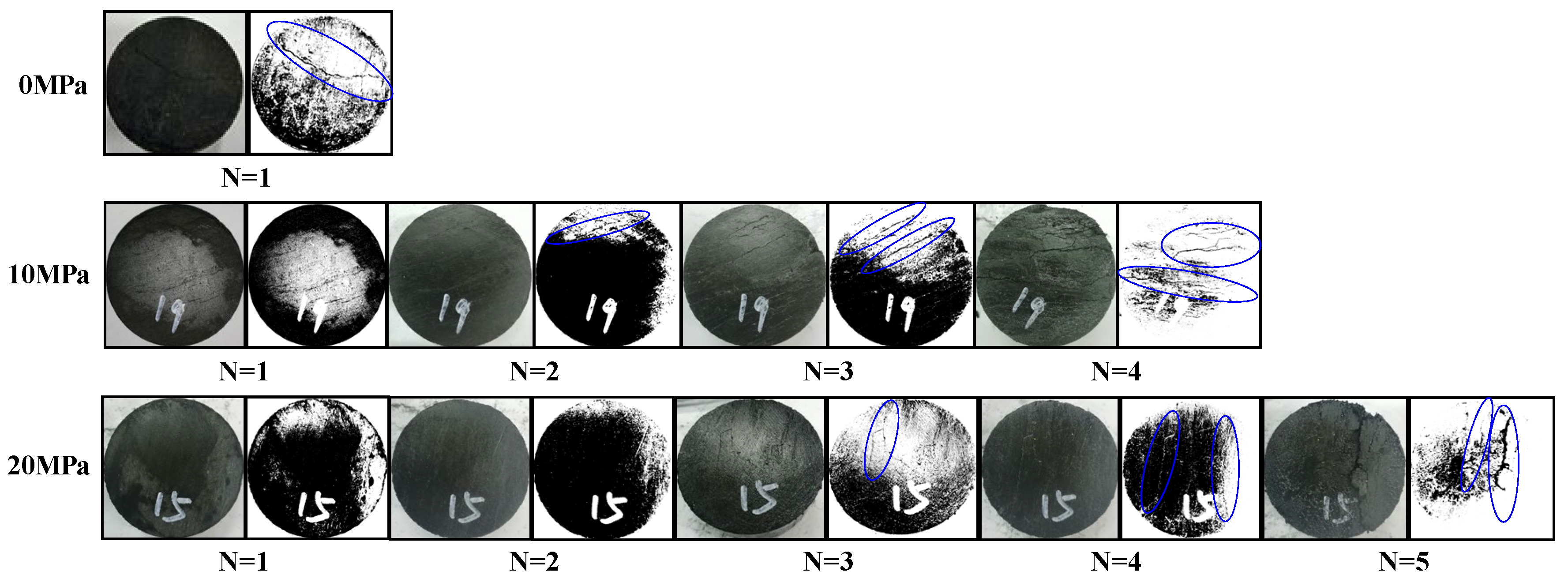
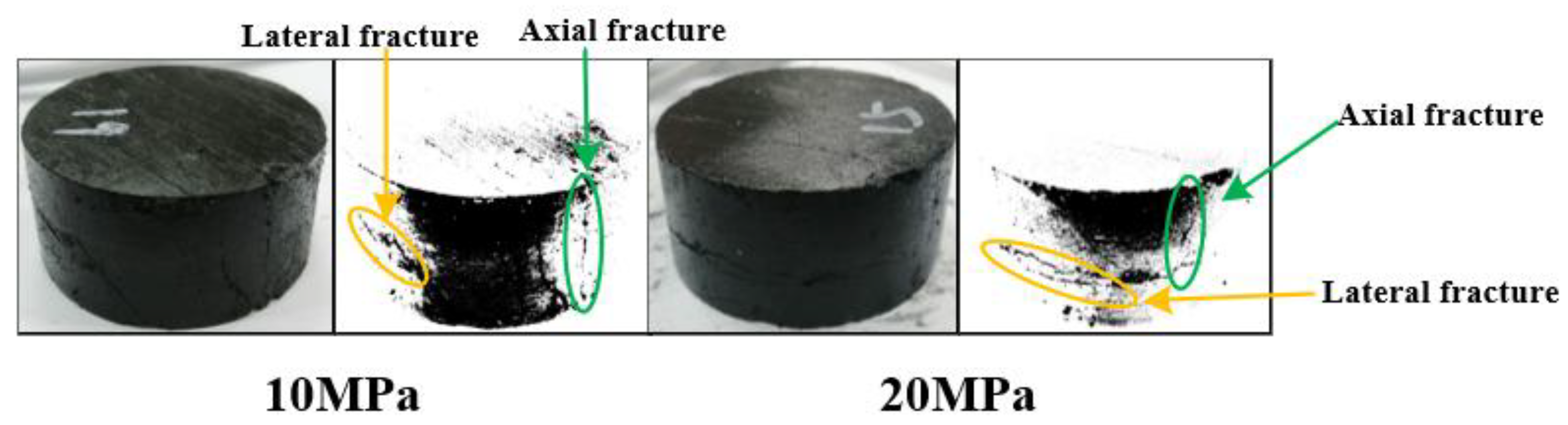
4.1. Comparative Analysis of Failure Modes

To investigate the failure process and characteristics of coal under quasi-static loading, acoustic emission (AE) equipment was employed to localize internal crack propagation during the experiment. Figure 8 illustrates the evolution of the AE signals during quasi-static loading. The results demonstrated that coal samples under quasi-static loading predominantly failed via diagonal shear or conjugate shear modes. In the initial loading phase (low cycle numbers), no AE signals were detected. AE activity only initiated when N reached 10, indicating the onset of internal crack propagation, with initial cracks occurring near the sample end regions. As N increased further, the AE signals near the original end regions gradually proliferated, and new AE sources propagated toward the sample interior. At N = 10, cracks began to form, yet AE signal growth remained slow. For example, under a confining pressure of 20 MPa, the AE counts only increased by 46 as N rose from 10 to 16, corresponding to the early phase of unstable crack propagation. A dramatic surge in AE activity (455 counts) occurred between N = 16 and N = 17, marking the advanced phase of unstable crack propagation and subsequent macroscopic fracture formation. This AE evolution aligns with the characteristic trend of “gradual increase–rapid increase–abrupt surge” in AE counts [37].
Under dynamic loading, AE signal acquisition was not feasible due to the opaque confining pressure system (see Figure 2). Consequently, surface crack evolution was analyzed to characterize dynamic failure. Figure 9 presents the surface crack progression in coal specimens under varying confining pressures. Under unconfined dynamic impacts, a single transverse penetrating crack dominated the surface. At confining pressures of 10 MPa and 20 MPa, multiple interconnected cracks emerged, exhibiting tensile splitting failure modes [38]. This behavior arises from the synergistic effects of (1) the continuous propagation of pre-existing fractures, (2) new crack generation under cyclic impacts, and (3) the coalescence of original and induced fractures, ultimately forming interconnected fracture networks and macroscopic failure surfaces.

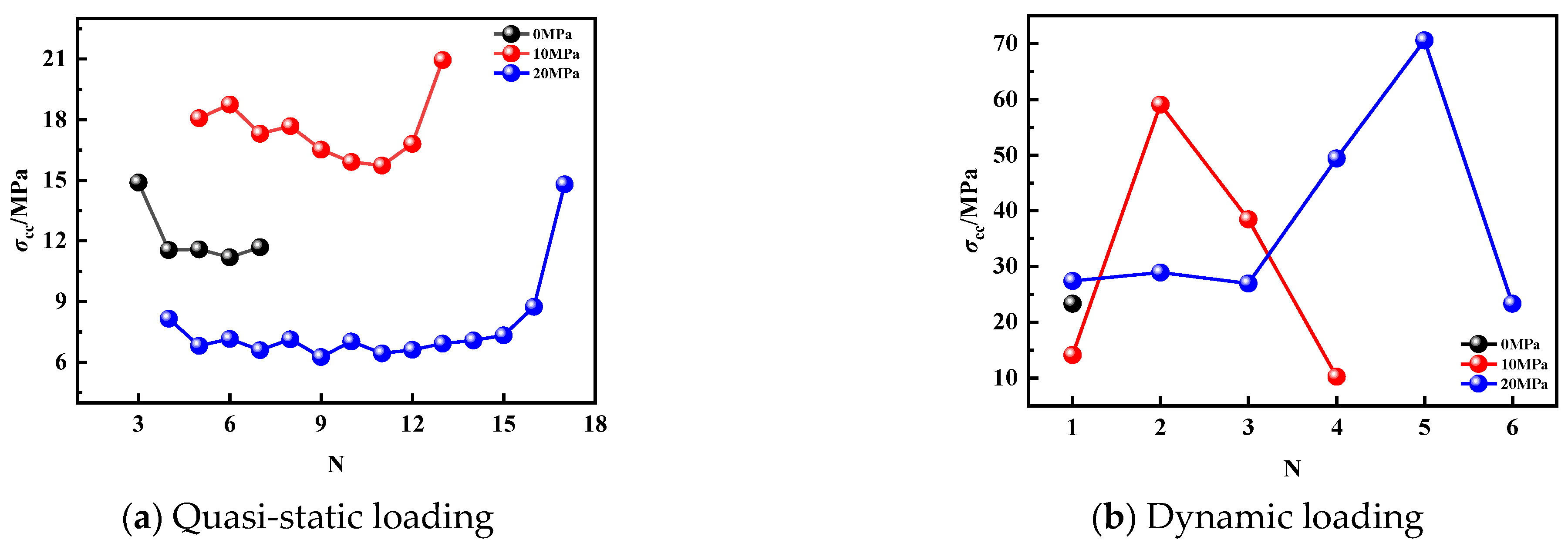
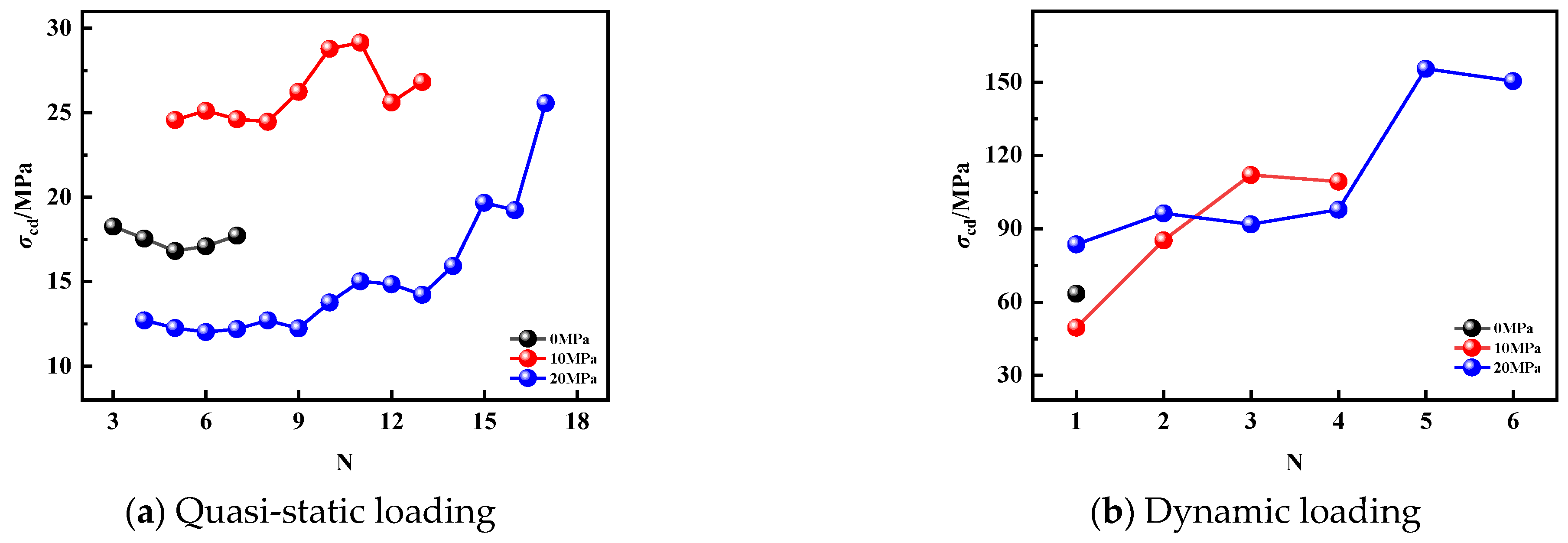
4.2. Evolution of Crack Closure Stress and Damage Stress Under Dynamic Cyclic Loading–Unloading

In the progressive failure of coal, crack closure stress (σcc) and crack damage stress (σcd) play critical roles. σcc represents the stress at which pre-existing cracks within the material fully close, while σcd denotes the onset of unstable crack propagation and corresponds to the long-term strength threshold of the material [39]. Figure 10 illustrates the determination methodologies for σcc and σcd under quasi-static and dynamic loading conditions.
ε v = ε 1 + ε 2 + ε 3
ε v c = ε v − ( 1 − 2 μ ) ( σ 1 − σ 3 ) E
where ε1, ε2, and ε3 are the principal strains; E and μ denote Young’s modulus and Poisson’s ratio, respectively; and (σ1–σ3) is the deviatoric stress.
For dynamic loading condition, lateral strains (ε2, ε3) are experimentally inaccessible. Following Zhou et al. [40], σcc and σcd were determined using axial stress–strain data, with elastic volumetric strain (εve) calculated via the tangent modulus at half of the peak stress (Figure 10b).
Figure 11 shows that under quasi-static loading, σcc initially decreased and then increased with N. This trend arose because σcc reflects the minimum stress required to close internal cracks. Early cycles generate new cracks, reducing σcc. However, at higher N and confining pressures, crack closure is mechanically constrained, elevating the scale of σcc [41]. In contrast, under dynamic loading, σcc first increased (due to confinement-restricted crack closure) and then decreased (post-critical damage overwhelmed the confinement effects).
Figure 12 shows that σcd increased with N under both loading regimes. Confining pressure suppresses new crack initiation, necessitating higher deviatoric stresses to propagate cracks (quasi-static) [42]. Under dynamic loading, cyclic impacts elevate the crack damage stress threshold [43]. However, Figure 12b shows a slight σcd reduction in the final cycle, which was attributed to severe cumulative damage impairing the load-bearing capacity.

5. Discussion

This study systematically compared the mechanical properties and failure characteristics of coal under quasi-static and dynamic loading regimes. The variations in cyclic peak stress, strain, and Young’s modulus reflected the fundamental differences in coal’s mechanical responses to these distinct loading conditions. The AE signal evolution and surface crack patterns further delineated the failure mechanisms: AE localization captured the progressive damage under quasi-static loading, while surface crack networks illustrate the dynamic failure’s sudden energy release. The comparative analyses of σcc and σcd further demonstrated how loading regimes govern internal crack initiation and propagation.
The strain rate disparity between quasi-static (10−5–10−3 s−1) and dynamic (102–103 s−1) loading spanned multiple orders of magnitude, a critical factor that is often overlooked in single-regime studies [18]. To address this gap, experiments were conducted at strain rates of 10−3 s−1 (quasi-static loading) and 102 s−1 (dynamic loading). Figure 3 shows the contrasting stress paths: quasi-static loading showed a continuous trajectory, whereas dynamic loading involves intermittent cycles with partial unloading phases. This divergence in stress paths explains why quasi-static unloading minimally affects coal integrity, whereas dynamic cycles represent single-impact events on progressively damaged specimens [44]. Consequently, dynamic loading produced less pronounced peak stress transitions compared to quasi-static conditions. For instance, as shown in Figure 7c, a sharp stress drop occurred between N = 16 and N = 17 under quasi-static loading, while dynamic loading (N = 4–6) exhibited gradual stress variations.
This stress path dichotomy also governed the Young’s modulus evolution. Dynamic loading induced earlier modulus transition points due to the high strain rates suppressing microcrack closure and propagation [45]. Since coal’s stiffness is primarily derived from an intact matrix, cumulative damage under dynamic loading progressively reduces the undamaged matrix volume, accelerating modulus degradation.
There were significant differences in the failure characteristics between quasi-static and dynamic loading regimes. As shown in Figure 9 and Figure 10, the coal specimens under quasi-static loading failed via oblique shear failure, governed by the coupled effects of confining pressure and axial stress. In contrast, dynamic loading induced composite failure modes: longitudinal splitting fractures and transverse tensile cracks (Figure 13). This duality arose from stress wave interactions within the coal. A portion of the incident compressive stress wave is reflected as a tensile wave at internal boundaries. When the amplitude of the tensile wave exceeds the dynamic tensile strength (σt), lateral tensile failure occurs [23], satisfying the criterion in Equation (3). Conversely, axial compressive fractures develop when the incident compressive stress surpasses the dynamic compressive strength (σc), as defined by Equation (4). During cyclic dynamic loading, the initial impacts generate stresses below σc, producing lateral tensile cracks. As N increases and stresses exceed σc, axial compressive cracks emerge. Consequently, fully failed specimens exhibit hybrid fracture networks: early-stage transverse tensile cracks and late-stage axial compressive fractures (Figure 13).
σ t < σ ( t ) < σ c
σ c < σ t < σ ( t )
where σt, σc, and σ(t) denote the dynamic tensile strength, dynamic compressive strength, and instantaneous internal stress within the coal specimen, respectively.
Previous research [46,47] has shown that under cyclic impacts, rock failure typically splits along the loading direction, as most experiments were conducted without confining pressure. In our study, at low impact air pressures, the lateral deformation of the specimens was restricted by confining pressure. Tensile crack occurred when the axial impact-induced tensile stress agrees with Formula (3), leading to the gradual formation of tensile cracks perpendicular to the loading direction. At high impact air pressures, the internal stress agrees with Formula (4), and the impact-induced stress exceeds the confining pressure. When this stress reaches the dynamic compressive strength of the coal samples, compressive cracks form along the loading direction.
This study only analyzed the mechanical properties and macro failure modes of coal. Future research will use CT, SEM, and DEM to study the meso mechanical properties and failure mechanisms of coal under quasi-static and dynamic loading conditions. In addition, research on coal failure modes and mechanisms under strain rates of 10−5 to 102 will be considered to further explore the strain rate’s impact on failure mechanisms.

6. Conclusions

This study conducted cyclic loading–unloading experiments on coal under quasi-static and dynamic loading conditions and analyzed the mechanical property disparities under the two strain rate regimes. The failure processes and modes were investigated using AE localization and crack characterization methods. The findings provide theoretical foundations for hazard prevention in underground engineering. The main conclusions can be summarized as follows:
(1)
The stress–strain curves of coal under quasi-static loading can be divided into four stages: compaction, linear elasticity, nonlinear crack propagation, and post-peak softening. In contrast, dynamic loading induces bifurcation of the post-peak phase into first and second unloading stages due to rebound effects.
(2)
Under quasi-static loading, peak strain increases linearly with cycle number N, while dynamic loading exhibits nonlinear strain growth. Post-peak stress drops abruptly after quasi-static failure but declines gradually under dynamic loading due to rapid energy dissipation.
(3)
The AE counts during quasi-static loading transition from gradual to abrupt increases, corresponding to oblique shear failure. Under dynamic loading, lateral tensile cracks initiate first, followed by multiple axial compressive fractures.
(4)
The crack closure stress σcc under quasi-static loading initially decreases and then increases with N, whereas it follows an inverse trend (increase then decrease) under dynamic loading. Crack damage stress σcd generally increases with N for both regimes. However, dynamic loading produces a slight σcd reduction in the final cycle due to severe cumulative damage prior to failure.

Author Contributions

L.H.: writing—review and editing; F.G.: methodology; Y.X.: investigation and data curation. All authors have read and agreed to the published version of the manuscript.

Funding

This research was funded by the National Key R&D Program of China (Nos. 2022YFC3004602 and 2022YFC3004600) and National Natural Science Foundation of China (Nos. 51934007, 42030810, 52004268, and 52174091).

Institutional Review Board Statement

Not applicable.

Informed Consent Statement

Not applicable.

Data Availability Statement

The original contributions presented in the study are included in the article, further inquiries can be directed to the corresponding author.

Conflicts of Interest

The authors declare no conflicts of interest.

References

  1. Young, M.; Walton, G.; Holley, E. Investigation of factors influencing roof stability at a Western U.S. longwall coal mine. J. Min. Sci. Technol. 2019, 29, 139–143. [Google Scholar] [CrossRef]
  2. Jia, Z.; Xie, H.; Zhang, R.; Li, C.; Zhang, Z. Acoustic Emission Characteristics and Damage Evolution of Coal at Different Depths Under Triaxial Compression. Rock Mech. Rock Eng. 2020, 53, 1–14. [Google Scholar] [CrossRef]
  3. Gong, F.; Wang, Y.; Wang, Z.; Pan, J.; Luo, S. A new criterion of coal burst proneness based on the residual elastic energy index. J. Min. Sci. Technol. 2021, 31, 553–563. [Google Scholar] [CrossRef]
  4. Siwek, S. Earth tides and seismic activity in deep coal mining. Int. J. Rock Mech. Min. 2021, 148, 104972. [Google Scholar] [CrossRef]
  5. Lyu, C.; Liu, J.; Ren, Y.; Liang, C.; Zeng, Y. Mechanical characteristics and permeability evolution of salt rock under thermal-hydro-mechanical (THM) coupling condition. Eng. Geol. 2022, 302, 106633. [Google Scholar] [CrossRef]
  6. Zhang, A.; Xie, H.; Zhang, R.; Gao, M.; Xie, J.; Jia, Z.; Ren, L.; Zhang, Z. Mechanical properties and energy characteristics of coal at different depths under cyclic triaxial loading and unloading. Int. J. Rock Mech. Min. 2023, 161, 105271. [Google Scholar] [CrossRef]
  7. Wang, X.; Wang, J.; Liu, X.; Zhou, X.; Li, N.; Feng, J.; Yang, H. Microcracking evolution and clustering fractal characteristics in coal failure under multi step and cyclic loading. Eng. Fract. Mech. 2024, 310, 110511. [Google Scholar] [CrossRef]
  8. Wang, Z.; Li, W.; Hu, Y. Experimental study on mechanical behavior, permeability, and damage characteristics of Jurassic sandstone under varying stress paths. Bull. Eng. Geol. Environ. 2021, 80, 4423–4439. [Google Scholar] [CrossRef]
  9. Lawson, H.E.; Tesarik, D.; Larson, M.K.; Abraham, H. Effects of overburden characteristics on dynamic failure in underground coal mining. Int. J. Min. Sci. Technol. 2017, 27, 121–129. [Google Scholar] [CrossRef]
  10. Ai, T.; Wu, S.; Zhang, R.; Gao, M.; Zhou, J.; Xie, J.; Ren, L.; Zhang, Z. Changes in the structure and mechanical properties of a typical coal induced by water immersion. Int. J. Rock Mech. Min. 2021, 138, 104597. [Google Scholar] [CrossRef]
  11. Gao, M.; Xie, J.; Gao, Y.; Wang, W.; Li, C.; Yang, B.; Liu, J.; Xie, H. Mechanical behavior of coal under different mining rates: A case study from laboratory experiments to field testing. Int. J. Min. Sci. Technol. 2021, 31, 825–841. [Google Scholar] [CrossRef]
  12. Zhang, M.; Zhang, S.; Li, C.; Zhang, D.; Wang, C.; Jin, Y. Research on the Mechanical Properties and Energy Evolution of Coal Rock under Triaxial Cyclic Gradient Loading. Solid Fuel Chem. 2025, 59, 10–20. [Google Scholar] [CrossRef]
  13. Tan, T.; Wang, E.; Wang, X.; Ma, L.; Yao, W. Resistivity and damage of coal under cyclic loading and unloading. Eng. Geol. 2023, 323, 107234. [Google Scholar]
  14. Lu, H.; Xia, C.; Xu, C.; Wang, C.; Zhao, H.; Maruvanchery, V.; Li, G. Precursory characteristics of coal-rock failure using acoustic emission and computed tomography under uniaxial monotonic and cyclic compression. Measurement 2025, 247, 116844. [Google Scholar] [CrossRef]
  15. Liang, Y.; Ran, Q.; Zou, Q.; Zhang, B.; Hong, Y. Experimental Study of Mechanical Behaviors and Failure Characteristics of Coal Under True Triaxial Cyclic Loading and Unloading and Stress Rotation. Nat. Resour. Res. 2022, 31, 971–991. [Google Scholar] [CrossRef]
  16. Liu, X.; Dai, F.; Zhang, R.; Liu, J. Static and dynamic uniaxial compression tests on coal rock considering the bedding directivity. Environ. Earth Sci. 2015, 73, 5933–5949. [Google Scholar] [CrossRef]
  17. Zhang, H.; Wan, Z.; Ma, D.; Zhang, Y.; Cheng, J.; Zhang, Q. Experimental Investigation on the Strength and Failure Behavior of Coal and Synthetic Materials under Plane-Strain Biaxial Compression. Energies 2017, 10, 500. [Google Scholar] [CrossRef]
  18. Zhou, X.; Liu, X.; Wang, X.; Liu, Y.; Xie, H.; Du, P. Acoustic Emission Characteristics of Coal Failure Under Triaxial Loading and Unloading Disturbance. Rock Mech. Rock Eng. 2022, 56, 1043–1061. [Google Scholar] [CrossRef]
  19. Li, X.; Zhou, Z.; Lok, T.S.; Hong, L.; Yin, T. Innovative testing technique of rock subjected to coupled static and dynamic loads. Int. J. Rock Mech. Min. Sci. 2008, 45, 739–748. [Google Scholar] [CrossRef]
  20. Zhao, Y.; Zhao, G.F.; Jiang, Y.; Elsworth, D.; Huang, Y. Effects of bedding on the dynamic indirect tensile strength of coal: Laboratory experiments and numerical simulation. Int. J. Coal Geol. 2014, 132, 81–93. [Google Scholar] [CrossRef]
  21. Feng, J.; Wang, E.; Shen, R.; Chen, L.; Li, X.; Xu, Z. Investigation on energy dissipation and its mechanism of coal under dynamic loads. Geomech. Eng. 2016, 11, 657–670. [Google Scholar] [CrossRef]
  22. Gu, Z.; Shen, R.; Liu, Z.; Zhao, E.; Chen, H.; Yuan, Z.; Chu, X.; Tian, J. Dynamic Characteristics of Coal under Triaxial Constraints Based on the Split-Hopkinson Pressure Bar Test System. Nat. Resour. Res. 2023, 32, 587–601. [Google Scholar] [CrossRef]
  23. Feng, J.; Wang, E.; Huang, Q.; Ding, H.; Zhang, X. Experimental and numerical study of failure behavior and mechanism of coal under dynamic compressive loads. J. Min. Sci. Technol. 2020, 30, 613–621. [Google Scholar] [CrossRef]
  24. Li, X.; Gu, H.; Tao, M.; Peng, K.; Cao, W.; Li, Q. Failure characteristics and meso-deterioration mechanism of pre-stressed coal subjected to different dynamic loads. Theor. Appl. Fract. Mec. 2021, 115, 103061. [Google Scholar] [CrossRef]
  25. Bagde, M.N.; Petro, V. The Effect of Machine Behaviour and Mechanical Properties of Intact Sandstone Under Static and Dynamic Uniaxial Cyclic Loading. Rock Mech. Rock Eng. 2005, 38, 59–67. [Google Scholar] [CrossRef]
  26. Kong, X.; Wang, E.; He, X.; Zhao, E.; Zhao, C. Mechanical characteristics and dynamic damage evolution mechanism of coal samples in compressive loading experiments. Eng. Fract. Mech. 2019, 210, 160–169. [Google Scholar] [CrossRef]
  27. Zhang, A.; Zhang, R.; Ai, T.; Ren, L.; Zhang, Z.; Xie, J.; Lou, C.; Xiao, K.; Yang, Z. Fatigue characteristics of deep excavation-disturbed jinping marble. J. Rock Mech. Geotech. Eng. 2025, 17, 341–356. [Google Scholar] [CrossRef]
  28. Ulusay, R. The ISRM Suggested Methods for Rock Characterization, Testing and Monitoring: 2007–2014; Springer International Publishing: Cham, Switzerland, 2014; Volume 15, pp. 47–48. [Google Scholar]
  29. Huo, L.; Gao, F.; Xing, Y.; Gao, L.; Zhou, C. A Novel Rockburst Tendency Index Based on LURR. KSCE J. Civ. Eng. 2024, 28, 5987–6000. [Google Scholar] [CrossRef]
  30. Xie, H.; Gao, F.; Ju, Y.; Gao, M.; Zhang, R.; Gao, Y.; Liu, J.; Xie, L. Quantitative definition and analysis of deep mining. J. China Coal Soc. 2015, 40, 1–10. [Google Scholar]
  31. Jin, J.F. Study on Dynamic Mechanical Characteristics of Rock Under Combined Static Load and Cycllic Impact. Ph.D. Thesis, Central South University, Changsha, China, 2012. [Google Scholar]
  32. Liu, X.; Cai, D.; Gu, Z.; Zhang, S.; Zhou, X.; Gao, A. Analysis of progressive damage and energy consumption characteristics of gas-bearing coal under cyclic dynamic loads. Energy 2024, 306, 132419. [Google Scholar] [CrossRef]
  33. Gong, F.Q.; Li, X.B.; Liu, X.L. Preliminary Experimental Study on Mechanical Characteristics of Rock Under Three-Dimensional Static and Dynamic Combined Loading. Chin. J. Rock Mech. Eng. 2011, 30, 1179–1190. [Google Scholar]
  34. Lu, Z.; Ju, W.; Gao, F.; Yi, K. Influence of Loading Rate on the Failure Characteristics of Composite Coal–Rock Specimens Under Quasi-static Loading Conditions. Rock Mech. Rock Eng. 2022, 55, 909–921. [Google Scholar] [CrossRef]
  35. Zhu, J.; Zhang, B.; Zhang, J.J.Y. Coal pore characteristics in different coal mine dynamic disasters. Arab. J. Geosci. 2018, 11, 499. [Google Scholar] [CrossRef]
  36. Altman, D.G.; Machin, D.; Bryant, T.N.; Gardner, M.J. Statistics with confidence: Confidence intervals and statistical guidelines. Wiley: London, UK, 2000. [Google Scholar]
  37. Liang, Y.; Yang, Y.; Xin, G.; Zou, Q.; Ran, Q.; Qin, D. Mechanical properties and crack propagation characteristics of mudstone under different loading frequencies. Eng. Fract. Mech. 2025, 316, 110863. [Google Scholar] [CrossRef]
  38. Zhao, G.M.; Ma, W.W.; Meng, X.R. Failure Modes and Energy Characteristics of Rock-like Materials Under Dynamic Loading. Rock Soil Mech. 2015, 36. [Google Scholar]
  39. Mandelbrot, B.B. The Fractal Geometry of Nature; Freeman House Publishing: San Francisco, CA, USA, 1982. [Google Scholar]
  40. Zhou, Z.; Wang, P.; Cai, X.; Zhao, Y.; Liu, J.; Yan, Z.; Chen, L. Estimating crack closure and damage stress thresholds of rock during uniaxial compression based on axial plastic strain. J. Cent. South Univ. 2023, 30, 3335–3348. [Google Scholar] [CrossRef]
  41. Duan, Y.; Zhang, G.; Qin, T. Analysis of Crack-Characteristic Stress and Energy Characteristics of Sandstone under Triaxial Unloading Confining Pressure. Appl. Sci. 2023, 13, 2671. [Google Scholar] [CrossRef]
  42. Zhang, M.; Zhang, G.; Sun, W.; Chen, L.; Zheng, X.; Xu, C. Effect of crack density on crack initiation and damage for high confining pressure using wave velocities. Geomech. Geophys. Geo-Energy Geo-Resour. 2024, 10, 92. [Google Scholar] [CrossRef]
  43. Ghasemi, S.; Khamehchiyan, M.; Taheri, A.; Nikudel, M.R.; Zalooli, A. Microcracking Behavior of Gabbro During Monotonic and Cyclic Loading. Rock Mech. Rock Eng. 2021, 54, 2441–2463. [Google Scholar] [CrossRef]
  44. Zhang, Z.Z.; Gao, F. Study on Confining Pressure Effect of Energy Evolution of Loaded Rock. Chin. J. Rock Mech. Eng. 2015, 34, 1–11. [Google Scholar]
  45. Ray, S.K.; Sarkar, M.; Singh, T.N. Effect of cyclic loading and strain rate on the mechanical behaviour of sandstone. Int. J. Rock Mech. Min. 1999, 36, 543–549. [Google Scholar] [CrossRef]
  46. Cao, R.; Wang, H.; Qiu, X.; Wang, C.; Lin, H.; Qiao, Q. Damage characteristics and fracture behaviour of marble after cycle impact loading. Theor. Appl. Fract. Mec. 2023, 125, 103903. [Google Scholar] [CrossRef]
  47. Sun, B.; Yang, P.; Liu, S.; Zeng, S. Impact dynamic characteristics and constitutive model of granite damaged by cyclic loading. J. Mater. Res. Technol. 2023, 24, 333–345. [Google Scholar] [CrossRef]
Applsci 15 05238 g001
Figure 1. Quasi-static cyclic loading experiment.
Figure 1. Quasi-static cyclic loading experiment.
Applsci 15 05238 g001
Applsci 15 05238 g002
Figure 2. Dynamic cyclic loading experiment.
Figure 2. Dynamic cyclic loading experiment.
Applsci 15 05238 g002
Applsci 15 05238 g003
Figure 3. Comparison of stress–strain curves under quasi-static and dynamic loading at varying confining pressures.
Figure 3. Comparison of stress–strain curves under quasi-static and dynamic loading at varying confining pressures.
Applsci 15 05238 g003
Applsci 15 05238 g004
Figure 4. Typical stress–strain curves.
Figure 4. Typical stress–strain curves.
Applsci 15 05238 g004
Applsci 15 05238 g005
Figure 5. Comparison of Young’s modulus under quasi-static and dynamic loading.
Figure 5. Comparison of Young’s modulus under quasi-static and dynamic loading.
Applsci 15 05238 g005
Applsci 15 05238 g006
Figure 6. Comparison of peak strain under quasi-static and dynamic loading.
Figure 6. Comparison of peak strain under quasi-static and dynamic loading.
Applsci 15 05238 g006
Applsci 15 05238 g007
Figure 7. Comparison of peak stress under quasi-static and dynamic loading.
Figure 7. Comparison of peak stress under quasi-static and dynamic loading.
Applsci 15 05238 g007
Applsci 15 05238 g008
Figure 8. Evolution of acoustic emission signals during quasi-static loading.
Figure 8. Evolution of acoustic emission signals during quasi-static loading.
Applsci 15 05238 g008
Applsci 15 05238 g009
Figure 9. Surface crack evolution during dynamic loading.
Figure 9. Surface crack evolution during dynamic loading.
Applsci 15 05238 g009
Applsci 15 05238 g010
Figure 10. Schematic diagram for calculating σcc and σcd under quasi-static and dynamic loading.
Figure 10. Schematic diagram for calculating σcc and σcd under quasi-static and dynamic loading.
Applsci 15 05238 g010
Applsci 15 05238 g011
Figure 11. Crack closure stress under quasi-static and dynamic loading conditions.
Figure 11. Crack closure stress under quasi-static and dynamic loading conditions.
Applsci 15 05238 g011
Applsci 15 05238 g012
Figure 12. Crack damage stress under quasi-static and dynamic loading conditions.
Figure 12. Crack damage stress under quasi-static and dynamic loading conditions.
Applsci 15 05238 g012
Applsci 15 05238 g013
Figure 13. Lateral fractures during dynamic loading.
Figure 13. Lateral fractures during dynamic loading.
Applsci 15 05238 g013
Table 1. Sample parameters.
Table 1. Sample parameters.
No.Confining Pressure
/MPa		Height
/mm		Diameter/
mm		Mass
/g		Wave
Velocity
/km/s		No.Confining Pressure
/MPa		Height
/mm		Diameter/
mm		Mass
/g		Wave
Velocity
/km/s
1025.1549.9982.252.2751099.9449.95324.562.655
2025.5449.9776.442.09620100.3050.00324.962.704
31025.3549.9781.792.389310100.2549.99328.422.666
41025.3550.0069.182.006410100.5149.95336.982.547
52024.9049.9481.932.248520100.0749.97328.122.655
62025.0149.9682.042.389620100.1349.99344.162.732
Tolerance0.640.0613.071.14Tolerance0.570.0519.60.185
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Huo, L.; Gao, F.; Xing, Y. Mechanical Response Mechanism and Yield Characteristics of Coal Under Quasi-Static and Dynamic Loading. Appl. Sci. 2025, 15, 5238. https://doi.org/10.3390/app15105238

AMA Style

Huo L, Gao F, Xing Y. Mechanical Response Mechanism and Yield Characteristics of Coal Under Quasi-Static and Dynamic Loading. Applied Sciences. 2025; 15(10):5238. https://doi.org/10.3390/app15105238

Chicago/Turabian Style

Huo, Liupeng, Feng Gao, and Yan Xing. 2025. "Mechanical Response Mechanism and Yield Characteristics of Coal Under Quasi-Static and Dynamic Loading" Applied Sciences 15, no. 10: 5238. https://doi.org/10.3390/app15105238

APA Style

Huo, L., Gao, F., & Xing, Y. (2025). Mechanical Response Mechanism and Yield Characteristics of Coal Under Quasi-Static and Dynamic Loading. Applied Sciences, 15(10), 5238. https://doi.org/10.3390/app15105238

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Back to TopTop