Linearly Polarized γ Photon Generation from Unpolarized Electron Bunch Interacting with Laser
Abstract
1. Introduction
2. Simulation of the High Energy Photon Production
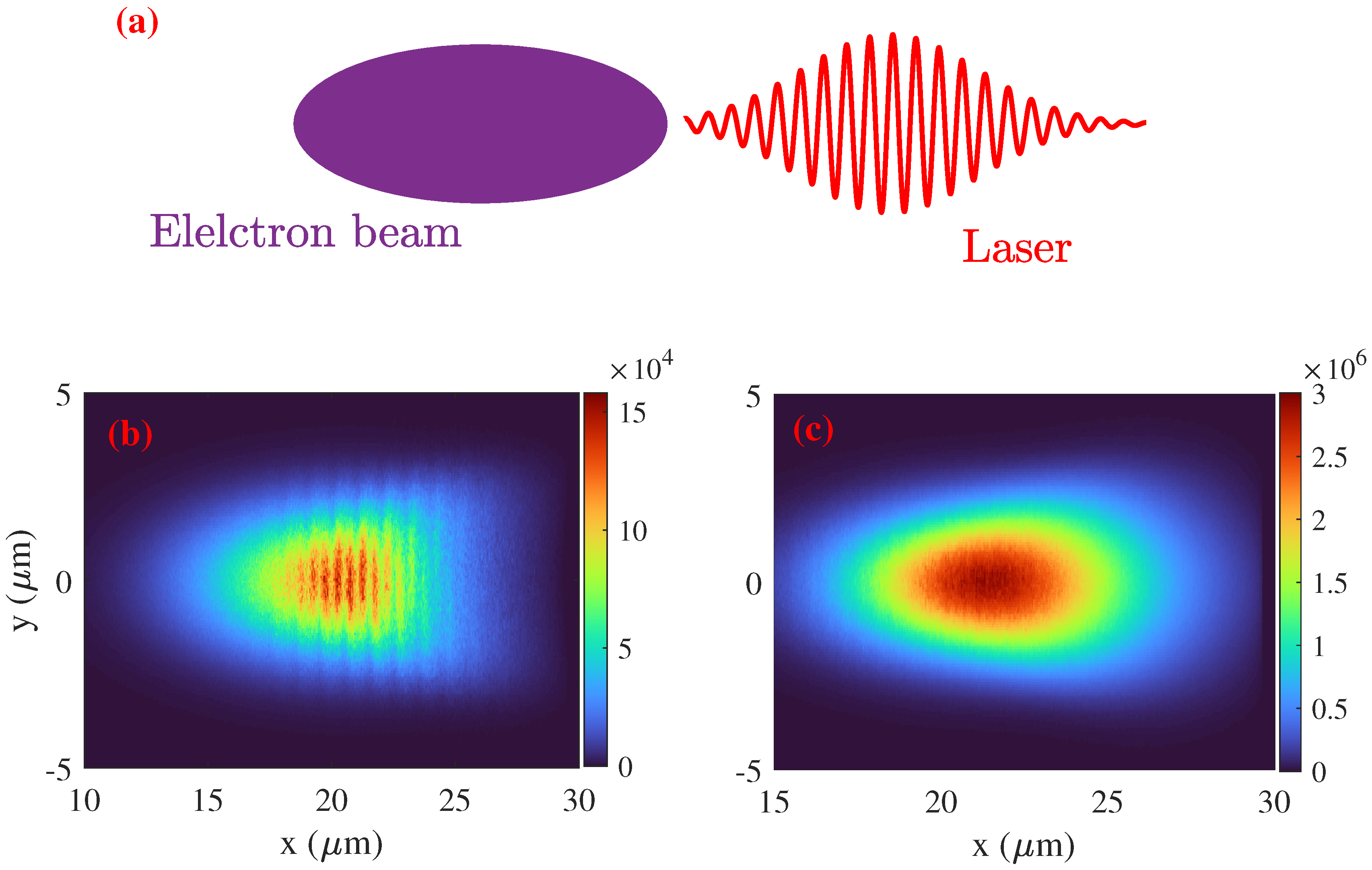
2.1. Model and Simulation Parameters
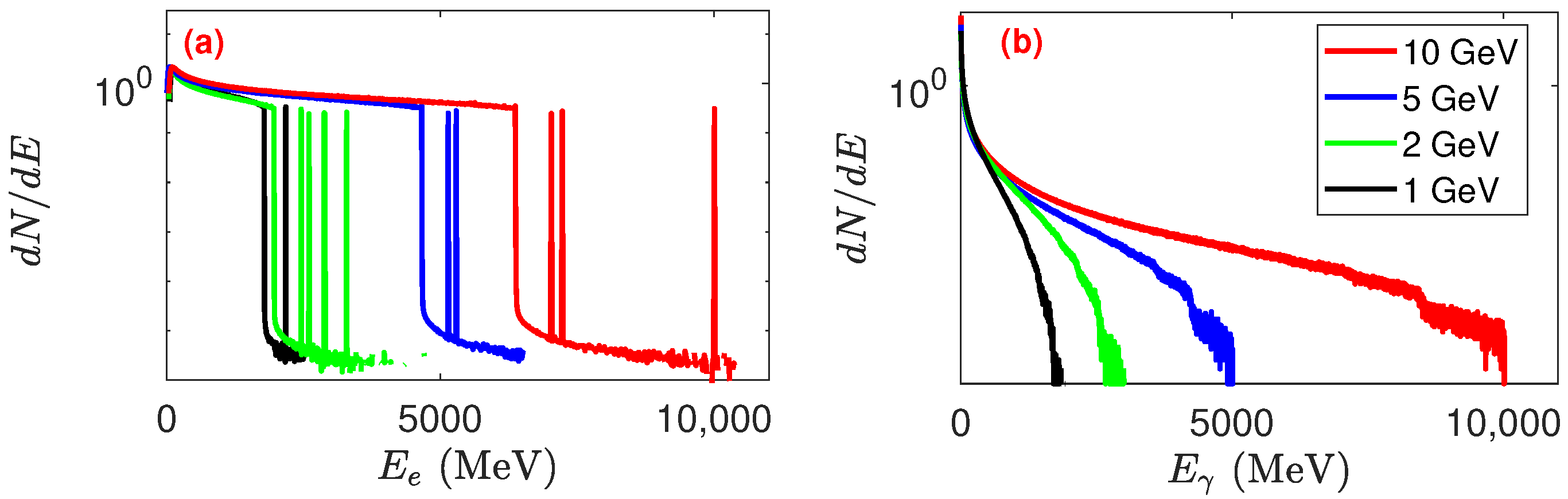
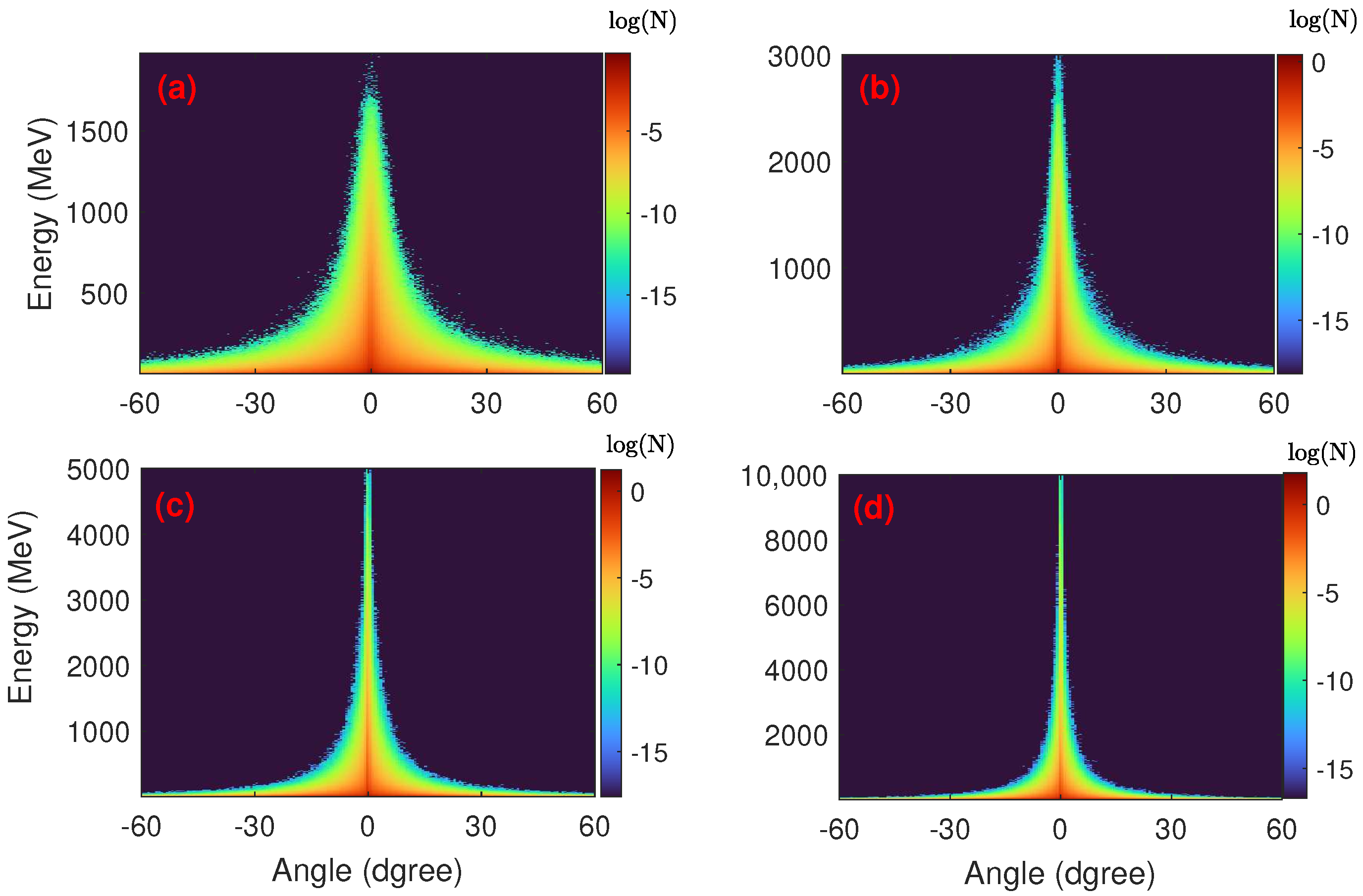
2.2. Simulation Results
3. Photon Polarization
3.1. Theory
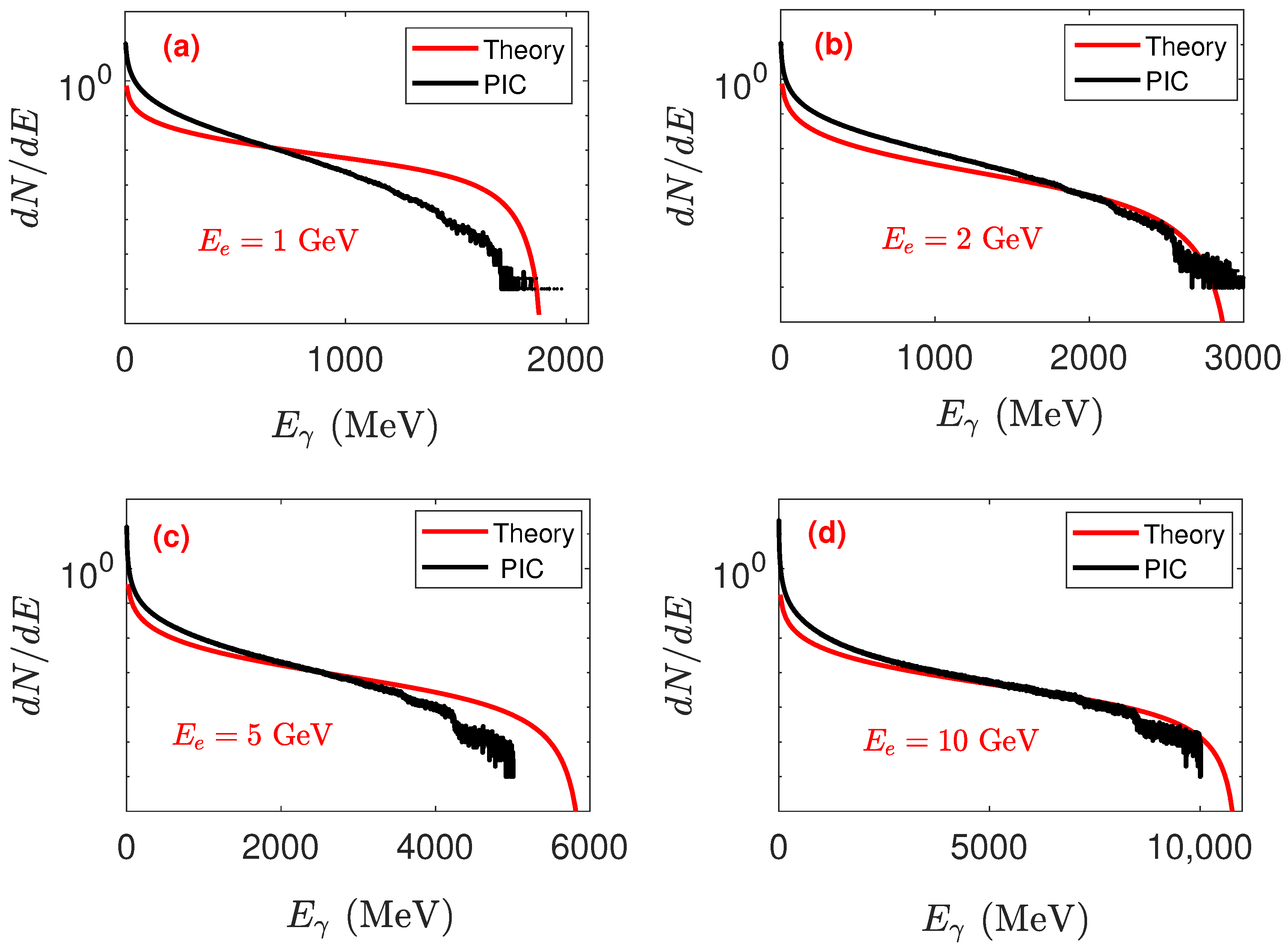
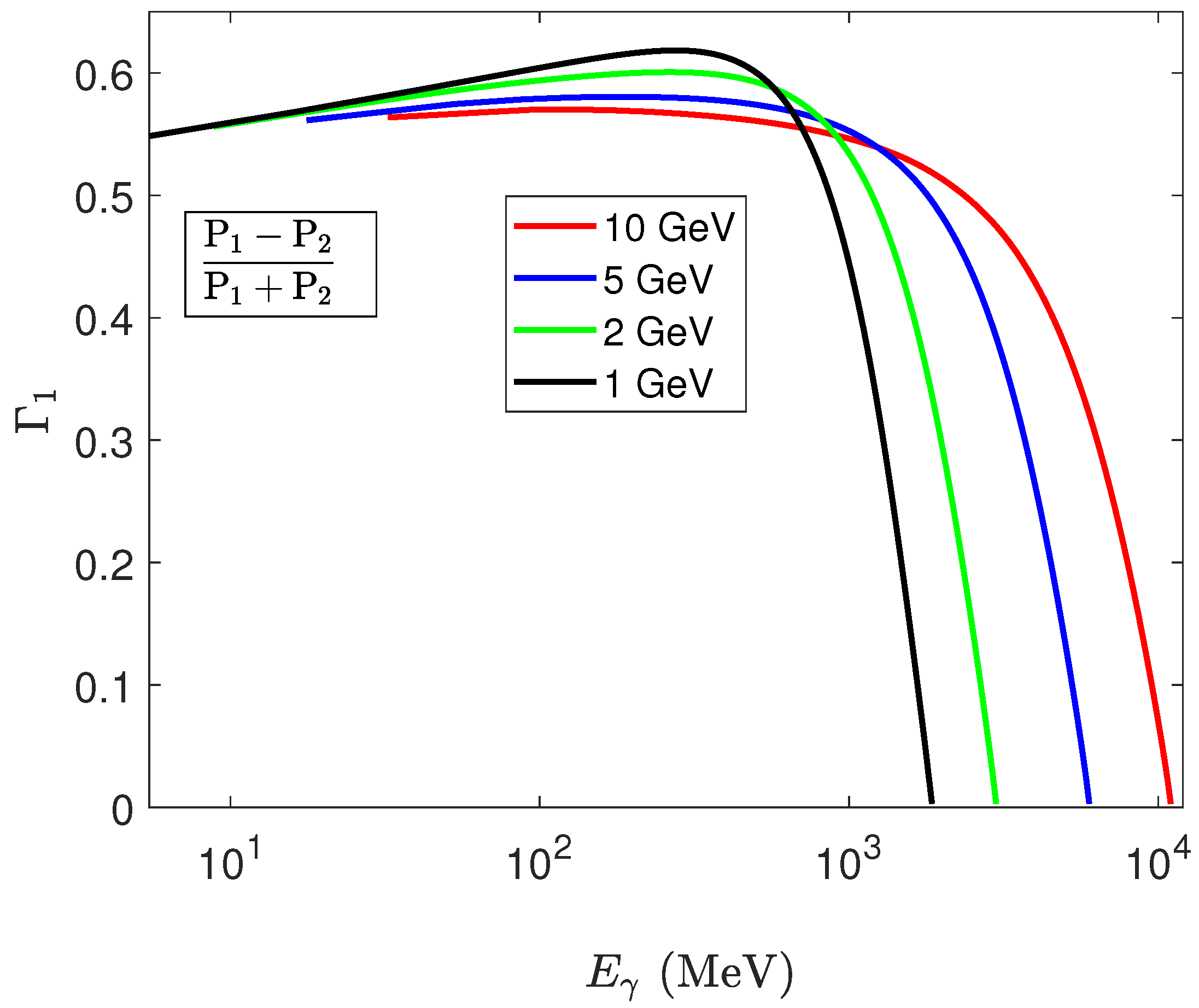
3.2. Calculation Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Yoon, J.W.; Kim, Y.G.; Choi, I.; Sung, J.H.; Lee, H.W.; Lee, S.K.; Nam, C.H. Realization of laser intensity over 1023W/cm2. Optica 2021, 8, 630–635. [Google Scholar] [CrossRef]
- Danson, C.N.; Haefner, C.; Bromage, J.; Butcher, T.; Chanteloup, J.C.F.; Chowdhury, E.A.; Galvanauskas, A.; Gizzi, L.A.; Hein, J.; Hillier, D.I.; et al. Petawatt and exawatt class lasers worldwide. High Power Laser Sci. Eng. 2019, 7, e54. [Google Scholar] [CrossRef]
- Turcu, I.C.E.; Negoita, F.; Jaroszynski, D.A.; Mckenna, P.; Balascuta, S.; Ursescu, D.; Dancus, I.; Cernaianu, M.O.; Tataru, M.V.; Ghenuche, P.; et al. High field physics and QED experiments at ELI-NP. Rom. Rep. Phys. 2016, 68, S145. [Google Scholar]
- Shen, B.; Bu, Z.; Xu, J.; Xu, T.; Ji, L.; Li, R.; Xu, Z. Exploring vacuum birefringence based on a 100 PW laser and an x-ray free electron laser beam. Plasma Phys. Control. Fusion 2018, 60, 044002. [Google Scholar] [CrossRef]
- Bulanov, S.S.; Mur, V.D.; Narozhny, N.B.; Nees, J.; Popov, V.S. Multiple Colliding Electromagnetic Pulses: A Way to Lower the Threshold of e+e− Pair Production from Vacuum. Phys. Rev. Lett. 2010, 104, 220404. [Google Scholar] [CrossRef]
- Di Piazza, A.; Mueller, C.; Hatsagortsyan, K.Z.; Keitel, C.H. Extremely high-intensity laser interactions with fundamental quantum systems. Rev. Mod. Phys. 2012, 84, 1177. [Google Scholar] [CrossRef]
- Gonoskov, A.; Blackburn, G.; Marklund, M.; Bulanov, S.S. Charged particle motion and radiation in strong electromagnetic fields. Rev. Mod. Phys. 2022, 94, 045001. [Google Scholar] [CrossRef]
- Fedotov, A.; Ilderton, A.; Karbstein, F.; King, B.; Seipt, D.; Taya, H.; Torgrimsson, G. Advances in QED with intense background fields. Phys. Rep. 2023, 1010, 1–138. [Google Scholar]
- Gonsalves, A.J.; Nakamura, K.; Daniels, J.; Benedetti, C.; Pieronek, C.; de Raadt, T.C.H.; Steinke, S.; Bin, J.H.; Bulanov, S.S.; van Tilborg, J.; et al. Petawatt laser guiding and electron beam acceleration to 8 GeV in a laser-heated capillary discharge waveguid. Phys. Rev. Lett. 2019, 122, 084801. [Google Scholar] [CrossRef]
- Tajima, T.; Malka, V. Laser plasma accelerators. Plasma Phys. Control. Fusion 2020, 62, 034004. [Google Scholar] [CrossRef]
- Albert, F.; Thomas, A.G.R. Applications of laser wakefield accelerator-based light sources. Plasma Phys. Control. Fusion 2016, 58, 103001. [Google Scholar] [CrossRef]
- Gales, S.; Balabanski, D.L.; Negoita, F.; Tesileanu, O.; Ur, C.A.; Ursescu, D.; Zamfir, N.V. New frontiers in nuclear physics with high-power lasers and brilliant monochromatic gamma beams. Phys. Scr. 2016, 91, 093004. [Google Scholar] [CrossRef]
- Tamburini, F.; Thidé, B.; Molina-Terriza, G.; Anzolin, G. Twisting of light around rotating black holes. Nat. Phys. 2011, 7, 195. [Google Scholar] [CrossRef]
- Schwinger, J.S. On gauge invariance and vacuum polarization. Phys. Rev. 1951, 82, 664. [Google Scholar] [CrossRef]
- Burke, D.L.; Field, R.C.; HortonSmith, G.; Spencer, J.E.; Walz, D.; Berridge, S.C.; Bugg, W.M.; Shmakov, K.; Weidemann, A.W.; Bula, C.; et al. Positron production in multiphoton light-by-light scattering. Phys. Rev. Lett. 1997, 79, 1626. [Google Scholar] [CrossRef]
- Bamber, C.; Boege, S.J.; Koffas, T.; Kotseroglou, T.; Melissinos, A.C.; Meyerhofer, D.D.; Reis, D.A.; Ragg, W.; Bula, C.; McDonald, K.T.; et al. Studies of nonlinear QED in collisions of 46.6 GeV electrons with intense laser pulses. Phys. Rev. D 1999, 60, 092004. [Google Scholar] [CrossRef]
- Mirzaie, M.; Hojbota, C.I.; Kim, D.Y.; Pathak, V.B.; Pak, T.G.; Kim, C.M.; Lee, H.W.; Yoon, J.W.; Lee, S.K.; Rhee, Y.J.; et al. All-optical nonlinear Compton scattering performed with a multi-petawatt laser. Nat. Photonics 2024, 18, 1212. [Google Scholar] [CrossRef]
- Abramowicz, H.; Acosta, U.; Altarelli, M.; Assmann, R.; Bai, Z.; Behnke, T.; Benhammou, Y.; Blackburn, T.; Boogert, S.; Borysov, O.; et al. Conceptual design report for the LUXE experiment. Eur. Phys. J. Spec. Top. 2021, 230, 2445–2560. [Google Scholar] [CrossRef]
- Meuren, S.; Bucksbaum, P.H.; Fisch, N.J.; Fiúza, F.; Glenzer, S.; Hogan, M.J.; Qu, K.; Reis, D.A.; White, G.; Yakimenko, V. On seminal HEDP research opportunities enabled by colocating multi-petawatt laser with high-density electron beams. arXiv 2020, arXiv:2002.10051. [Google Scholar]
- Speth, J.; Vander Woude, A. Giant resonances in nuclei. Rep. Prog. Phys. 1981, 44, 719–786. [Google Scholar] [CrossRef]
- Akbar, Z.; Roy, P.; Park, S.; Crede, V.; Anisovich, A.V.; Denisenko, I.; Klempt, E.; Nikonov, V.A.; Sarantsev, A.V.; Adhikari, K.P.; et al. Measurement of the helicity asymmetry E in ω→π+π−π0 photoproduction. Phys. Rev. C 2017, 96, 065209. [Google Scholar] [CrossRef]
- Moortgat-Pick, G.; Abe, T.; Alexander, G.; Ananthanarayan, B.; Babich, A.A.; Bharadwaj, V.; Barber, D.; Bartl, A.; Brachmann, A.; Chen, S.; et al. Polarized positrons and electrons at the linear collider. Phys. Rep. 2008, 460, 131–243. [Google Scholar] [CrossRef]
- Boehm, C.; Degrande, C.; Mattelaere, O.; Vincent, A.C. Circular polarisation: A new probe of dark matter and neutrinos in the sky. J. Cosmol. Astropart. Phys. 2017, 5, 043. [Google Scholar] [CrossRef]
- Laurent, P.; Rodriguez, J.; Wilms, J.; Bel, M.C.; Pottschmidt, K.; Grinberg, V. Polarized Gamma-Ray Emission from the Galactic Black Hole Cygnus X-1. Science 2011, 332, 438–439. [Google Scholar] [CrossRef]
- Olsen, H.; Maximon, L.C. Photon and Electron Polarization in High-Energy Bremsstrahlung and Pair Production with Screening. Phys. Rev. 1959, 114, 887. [Google Scholar] [CrossRef]
- Abbott, D.; Adderley, P.; Adeyemi, A.; Aguilera, P.; Ali, M.; Areti, H.; Baylac, M.; Benesch, J.; Bosson, G.; Cade, B.; et al. Production of Highly Polarized Positrons Using Polarized Electrons at MeV Energies. Phys. Rev. Lett. 2016, 116, 214801. [Google Scholar] [CrossRef]
- Sokolov, A.A.; Ternov, I.M. On polarization and spin effects in the theory of synchrotron radiation. Sov. Phys. Dokl. 1964, 8, 1203. [Google Scholar]
- Omori, T.; Fukuda, M.; Hirose, T.; Kurihara, Y.; Kuroda, R.; Nomura, M.; Ohashi, A.; Okugi, T.; Sakaue, K.; Saito, T.; et al. Efficient propagation of polarization from laser photons to positrons through Compton scattering and electron-positron pair creation. Phys. Rev. Lett. 2006, 96, 114801. [Google Scholar] [CrossRef]
- Lohmann, D.; Peise, J.; Ahrens, J.; Anthony, I.; Arends, H.J.; Beck, R.; Crawford, R.; Hunger, A.; Kaiser, K.H.; Kellie, J.D.; et al. Linearly polarized photons at mami (mainz). Nucl. Instrum. Methods Phys. Res. Sect. A 1994, 343, 494. [Google Scholar] [CrossRef]
- Li, Y.; Shaisultanov, R.; Chen, Y.; Wan, F.; Hatsagortsyan, K.Z.; Keitel, C.H.; Li, J. Polarized ultrashort brilliant multi-GeV γ rays via single-shot laser-electron interaction. Phys. Rev. Lett. 2020, 124, 014801. [Google Scholar] [CrossRef]
- Bragin, S.; Meuren, S.; Keitel, C.H.; Di Piazza, A. High energy vacuum birefringence and dichroism in an ultrastrong laser field. Phys. Rev. Lett. 2017, 119, 250403. [Google Scholar] [CrossRef] [PubMed]
- Tang, S.; King, B.; Hu, H. Highly polarised gamma photons from electron-laser collisions. Phys. Lett. B 2020, 809, 135701. [Google Scholar] [CrossRef]
- King, B.; Tang, S. Nonlinear Compton scattering of polarized photons in plane-wave backgrounds. Phys. Rev. A 2020, 102, 022809. [Google Scholar] [CrossRef]
- Xue, K.; Dou, Z.; Wan, F.; Yu, T.; Wang, W.; Ren, J.; Zhao, Q.; Zhao, Y.; Xu, Z.; Li, J. Generation of highly-polarized high-energy brilliant γ-rays via laser-plasma interaction. Matter Radiat. Extrem. 2020, 5, 054402. [Google Scholar] [CrossRef]
- Elaji, A.; Bake, M.A.; Tang, S.; Xie, B. Bright attosecond polarized γ-ray emission from the interaction of an intense laser pulse with non-uniform near-critical-density plasma. Chin. J. Phys. 2022, 77, 2751. [Google Scholar] [CrossRef]
- Yan, W.; Fruhling, C.; Golovin, G.; Haden, D.; Luo, J.; Zhang, P.; Zhao, B.; Zhang, J.; Liu, C.; Chen, M.; et al. High-order multiphoton Thomoson scattering. Nat. Photonics 2017, 11, 541. [Google Scholar] [CrossRef]
- Miller, K.G.; Pierce, J.R.; Ambat, M.V.; Shaw, J.L.; Weichman, K.; Mori, W.B.; Froula, D.H.; Palastro, J.P. Dephasingless laser wakefield acceleration in the bubble regime. Sci. Rep. 2023, 13, 21306. [Google Scholar] [CrossRef]
- Burdonov, K.; Fazzini, A.; Lelasseux, V.; Albrecht, J.; Antici, P.; Ayoul, Y.; Beluze, A.; Cavanna, D.; Ceccotti, T.; Chabanis, M.; et al. Characterization and performance of the Apollon short-focal-area facility following its commissioning at 1 PW level. Matter Radiat. Extrem. 2021, 6, 064402. [Google Scholar] [CrossRef]
- Mohammad, R.P.; Mirzaie, M.; Hojbota, C.I.; Pak, T.G.; Kim, S.B.; Lee, G.W.; Massudi, R.; Niknam, A.R.; Lee, S.K.; Kim, K.Y.; et al. Laser wakefield electron acceleration with polarization-dependent ionization injection. Phys. Rev. Apll. 2023, 20, 034026. [Google Scholar]
- Kettle, B.; Hollatz, D.; Gerstmayr, E.; Samarin, G.M.; Alejo, A.; Astbury, S.; Baird, C.; Bohlen, S.; Campbell, M.; Colgan, C.; et al. A laser-plasma platform for photon-photon physics: The two photon Breit-Wheeler process. New J. Phys. 2021, 23, 115006. [Google Scholar] [CrossRef]
- Ilderton, A.; King, B.; Seipt, D. Extended locally constant field approximation for nonlinear compton scattering. Phys. Rev. A 2019, 99, 042121. [Google Scholar] [CrossRef]
- Joshi, C.; Adli, E.; An, W.; Clayton, C.E.; Corde, S.; Gessner, S.; Hogan, M.J.; Litos, M.; Lu, W.; Marsh, K.A.; et al. Plasma wakefield acceleration experiments at FACET II. Plasma Phys. Control. Fusion 2018, 60, 034001. [Google Scholar] [CrossRef]
- Arber, T.D.; Bennett, K.; Brady, C.S.; Lawrence-Douglas, A.; Ramsay, M.G.; Sircombe, N.J.; Gillies, P.; Evans, R.G.; Schmitz, H.; Bell, A.R.; et al. Contemporary particle-in-cell approach to laser-plasma modelling. Plasma Phys. Control. Fusion 2015, 57, 113001. [Google Scholar] [CrossRef]
- Jansen, O.; Wang, T.; Stark, D.J.; d’Humieres, E.; Toncian, T.; Arefiev, A.V. Leveraging extreme laser-driven magnetic fields for gamma-ray generation and pair production. Plasma Phys. Control. Fusion 2018, 60, 054006. [Google Scholar] [CrossRef]
- Belland, A.R.; Kirk, J.G. Possibility of Prolific Pair Production with High-Power Lasers. Phys. Rev. Lett. 2008, 101, 200403. [Google Scholar]
- Bake, M.A.; Aimidula, A.; Zakir, A.; Abdukerim, N.; Ablat, A. Photon and positron generation by ultrahigh intensity laser interaction with electron beams. Front. Phys. 2018, 13, 135202. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
He, Y.; Yakup, B.; Bake, M.A. Linearly Polarized γ Photon Generation from Unpolarized Electron Bunch Interacting with Laser. Appl. Sci. 2025, 15, 481. https://doi.org/10.3390/app15010481
He Y, Yakup B, Bake MA. Linearly Polarized γ Photon Generation from Unpolarized Electron Bunch Interacting with Laser. Applied Sciences. 2025; 15(1):481. https://doi.org/10.3390/app15010481
Chicago/Turabian StyleHe, Yang, Burabigul Yakup, and Mamat Ali Bake. 2025. "Linearly Polarized γ Photon Generation from Unpolarized Electron Bunch Interacting with Laser" Applied Sciences 15, no. 1: 481. https://doi.org/10.3390/app15010481
APA StyleHe, Y., Yakup, B., & Bake, M. A. (2025). Linearly Polarized γ Photon Generation from Unpolarized Electron Bunch Interacting with Laser. Applied Sciences, 15(1), 481. https://doi.org/10.3390/app15010481



