Calculation Model of Drainage Radius of Single-Layer/Multi-Layer Commingled Gas Production Wells in a Closed Constant-Volume Gas Reservoir and Its Application
Abstract
1. Introduction
2. Calculation Model of Drainage Radius of Single-Layer Gas Well
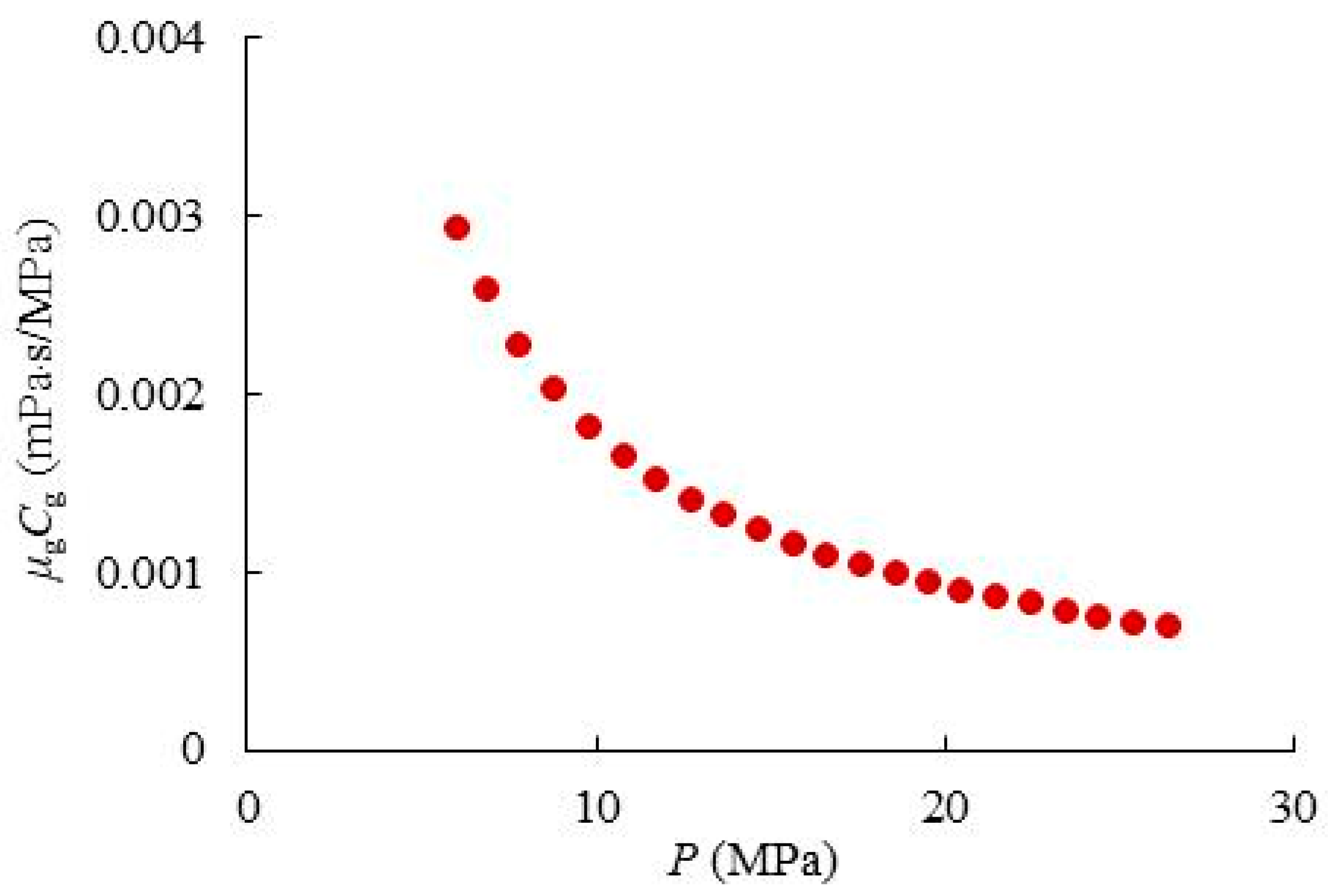
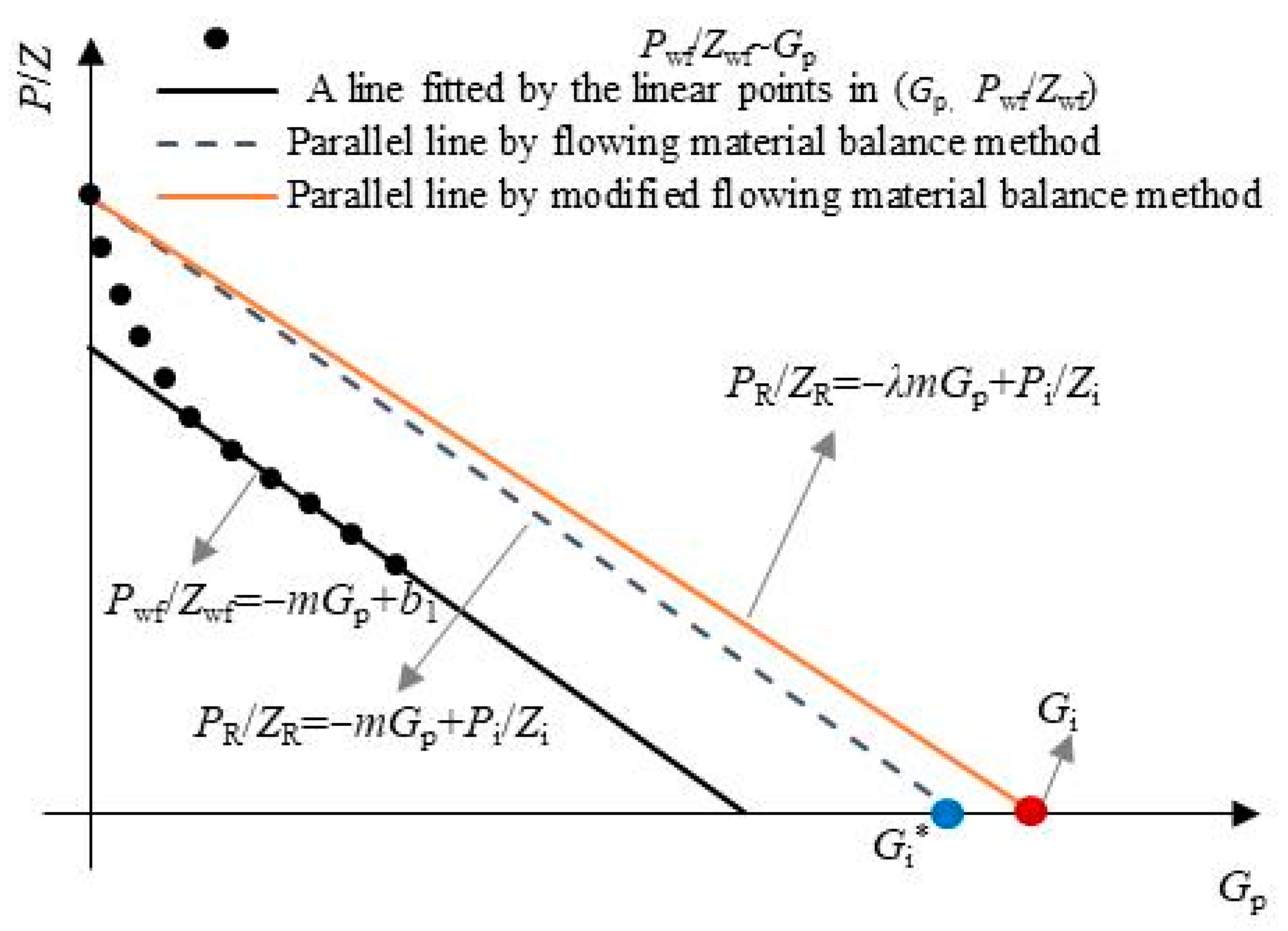
2.1. Modified Flowing Material Balance Method
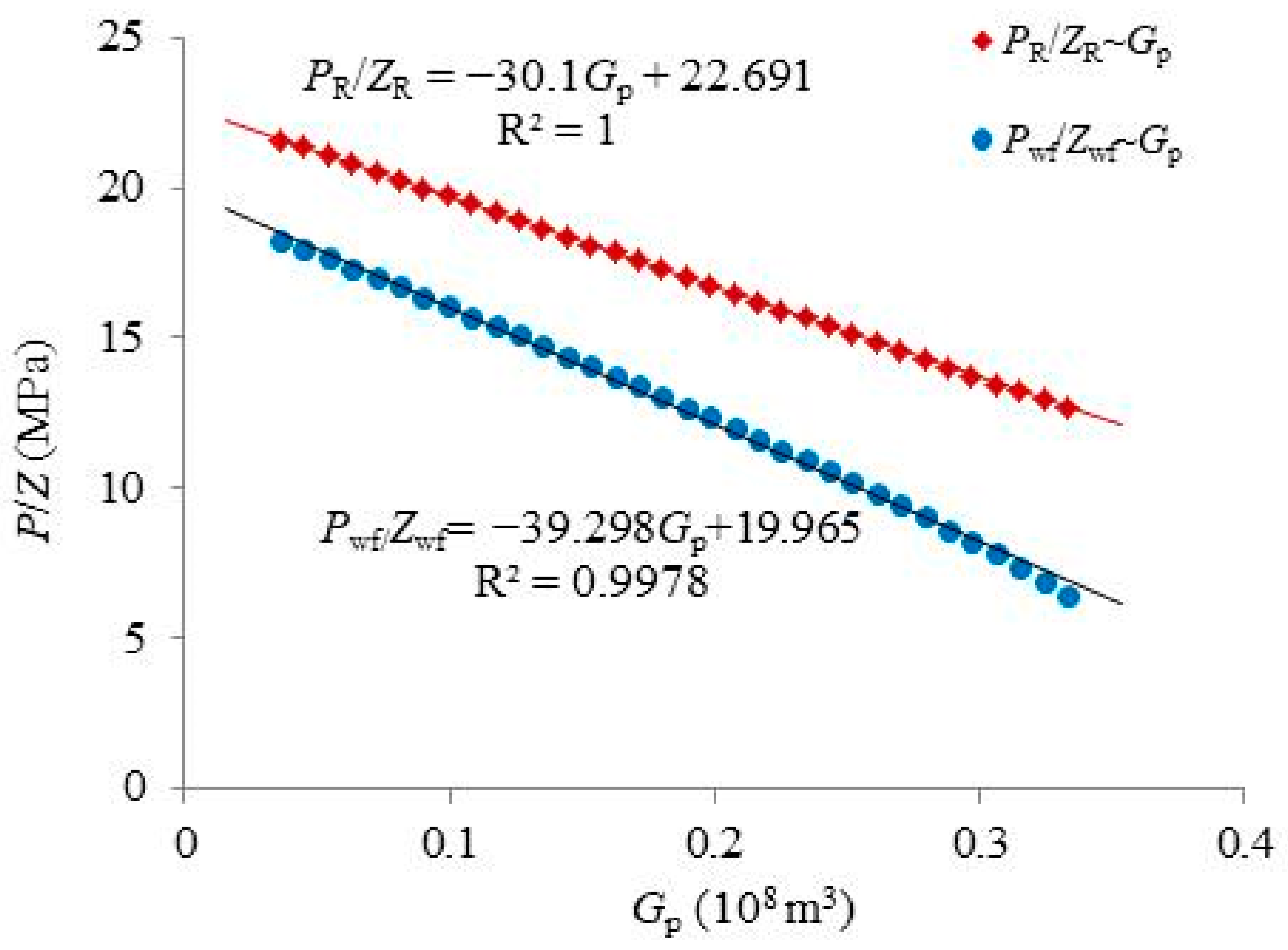
2.2. Method Validation
2.3. Practical Application
3. Calculation Model of Drainage Radius of Multi-Layer Commingled Gas Production Well and Its Application
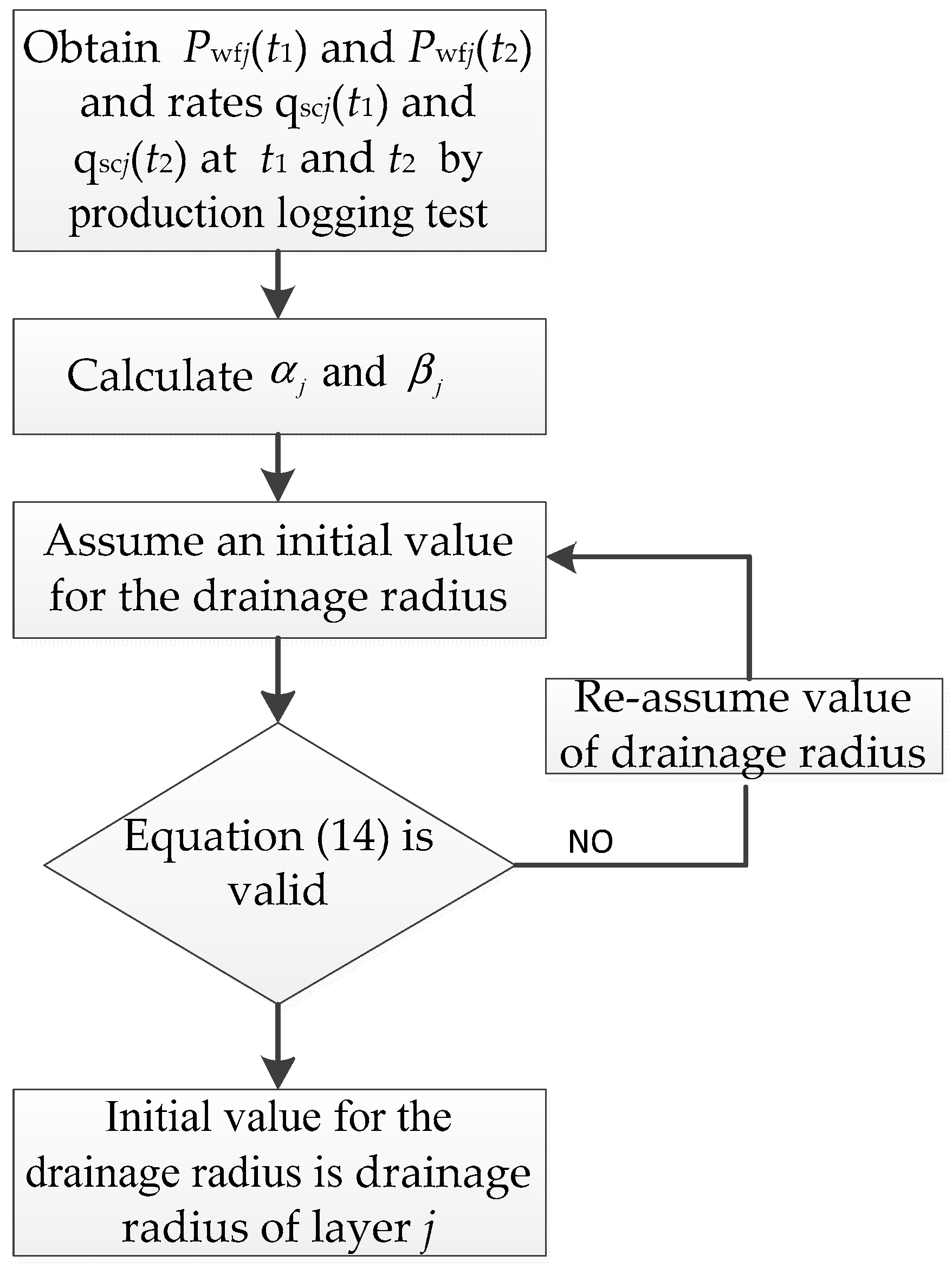
3.1. Calculation Model of Drainage Radius of Multi-Layered Gas Well
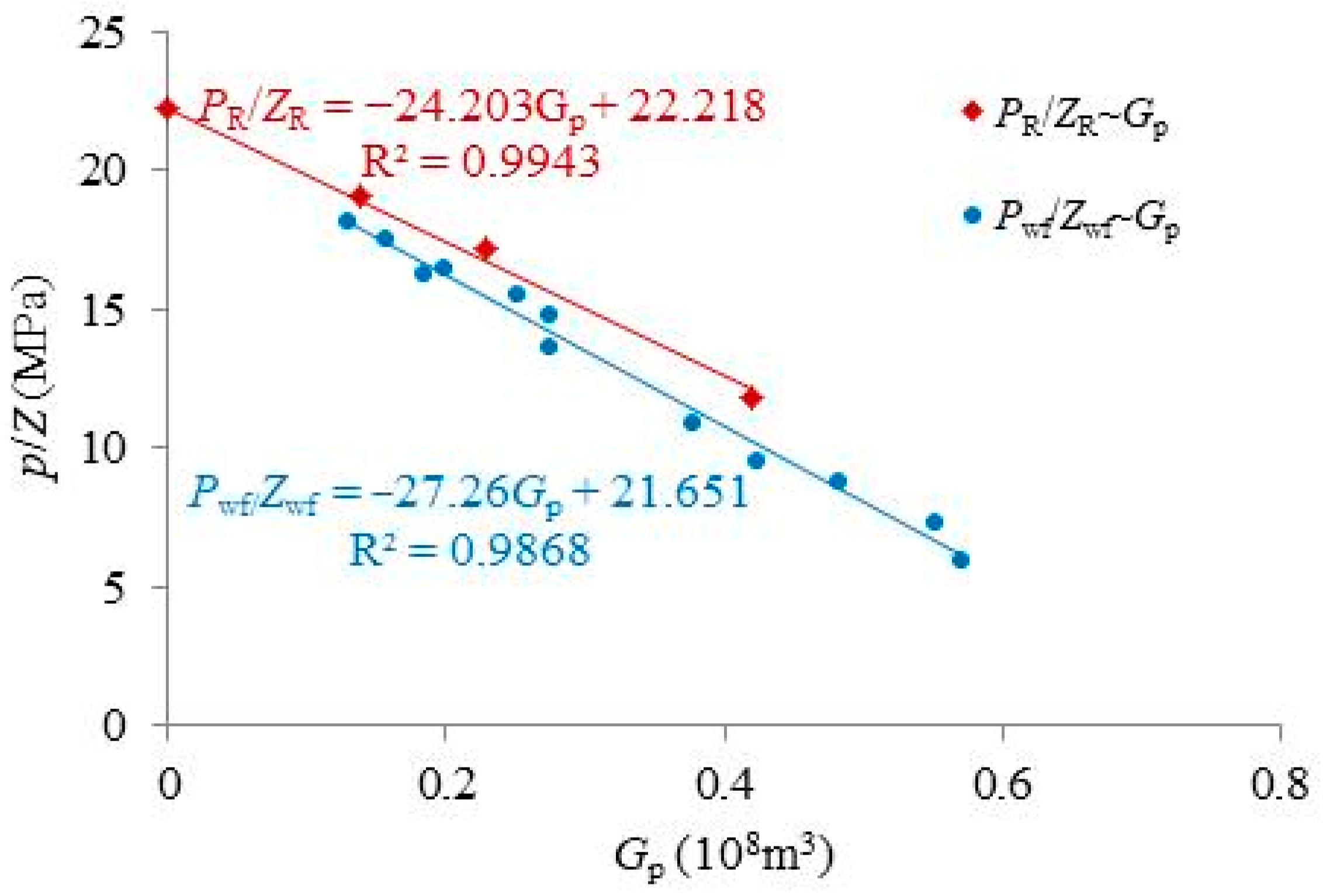
3.2. Method Validation
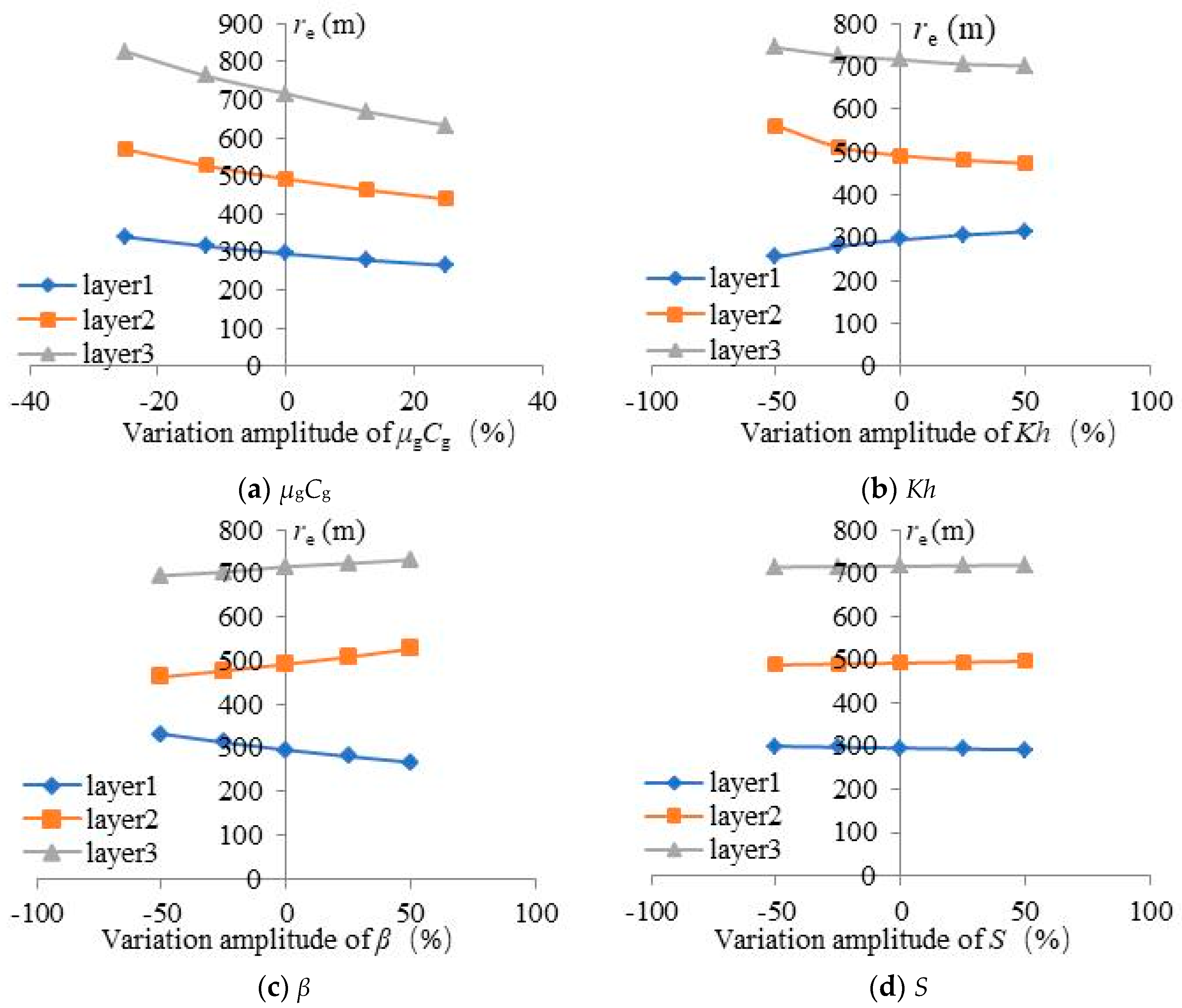
3.3. Sensitivity Analysis
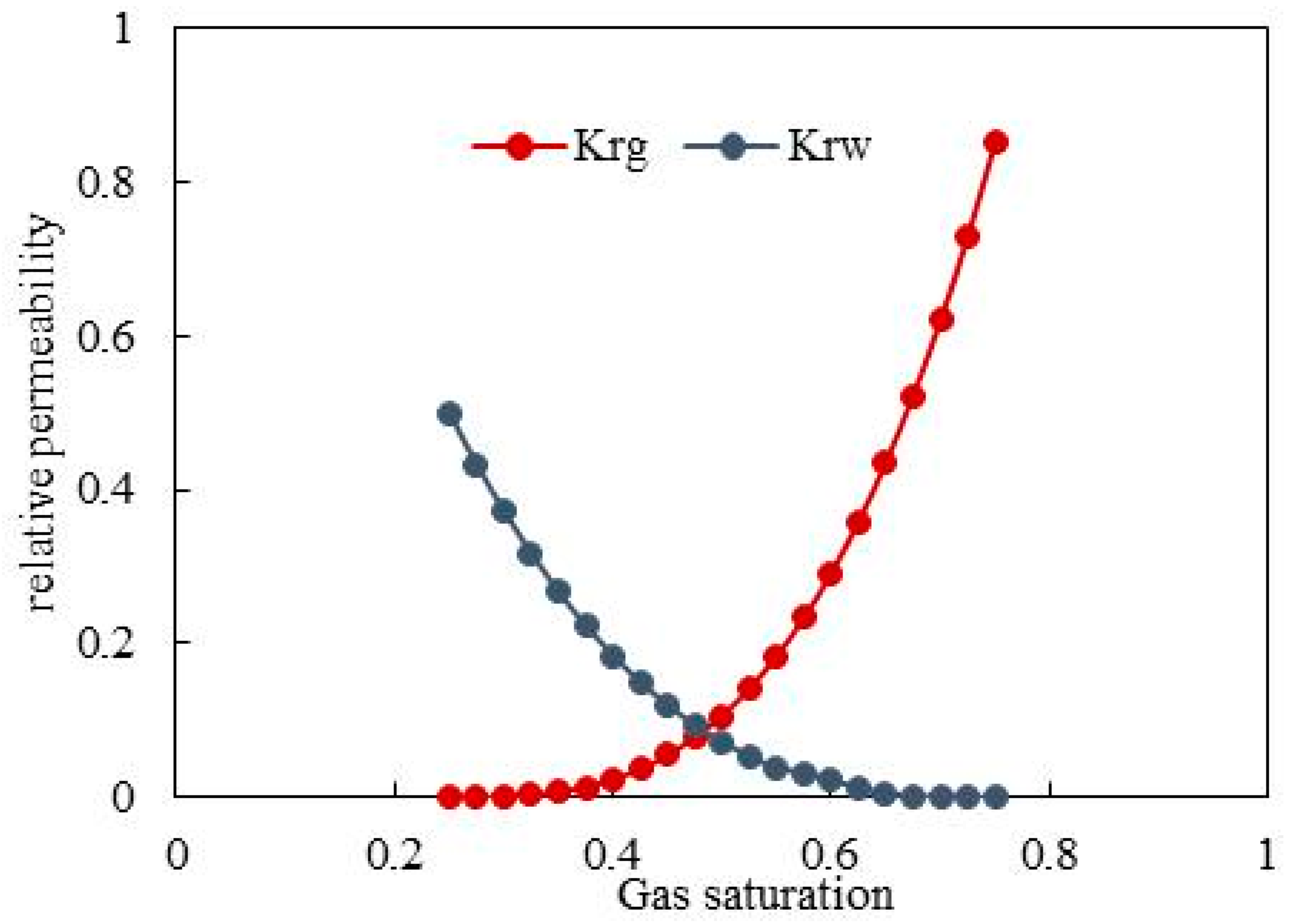
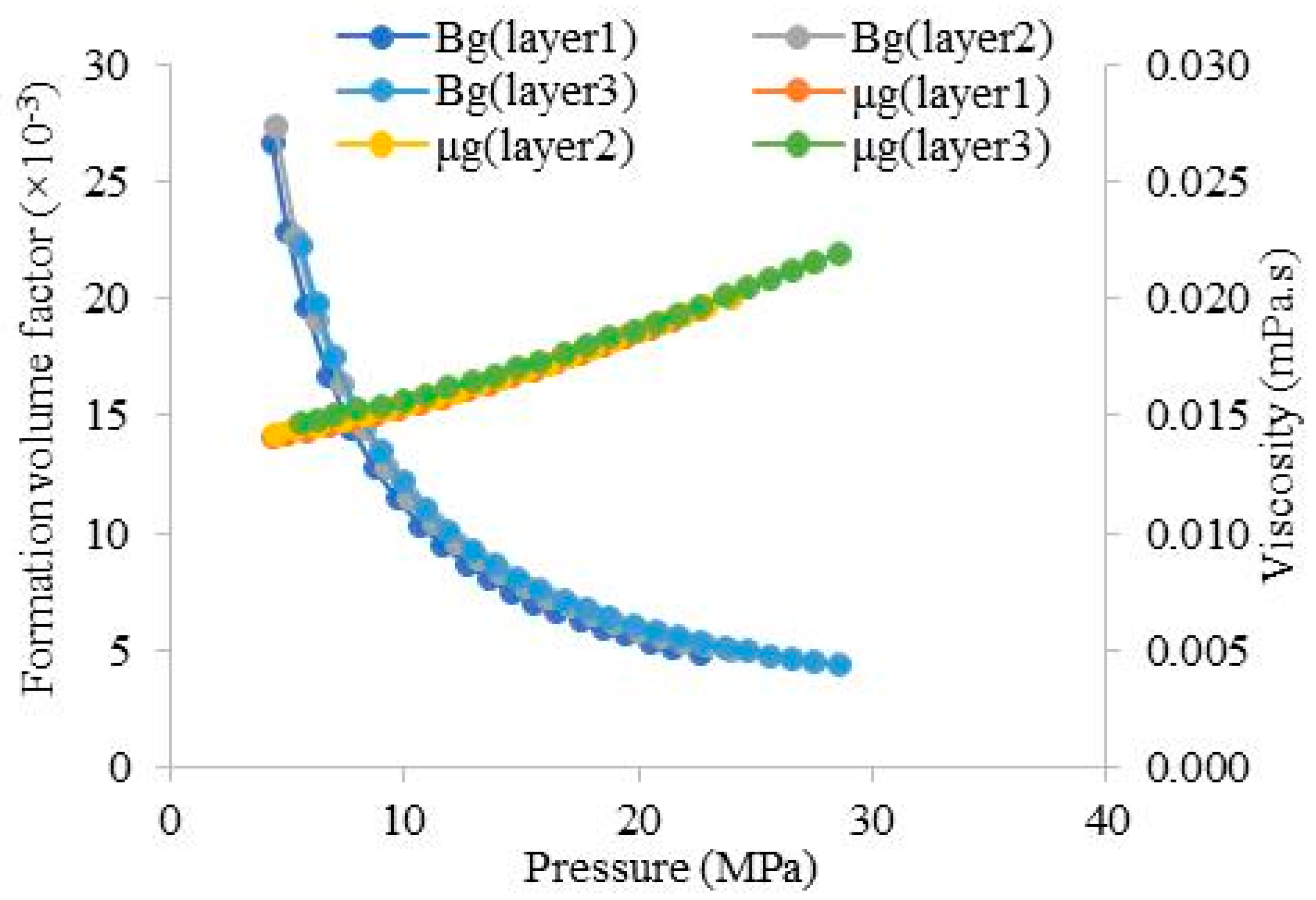
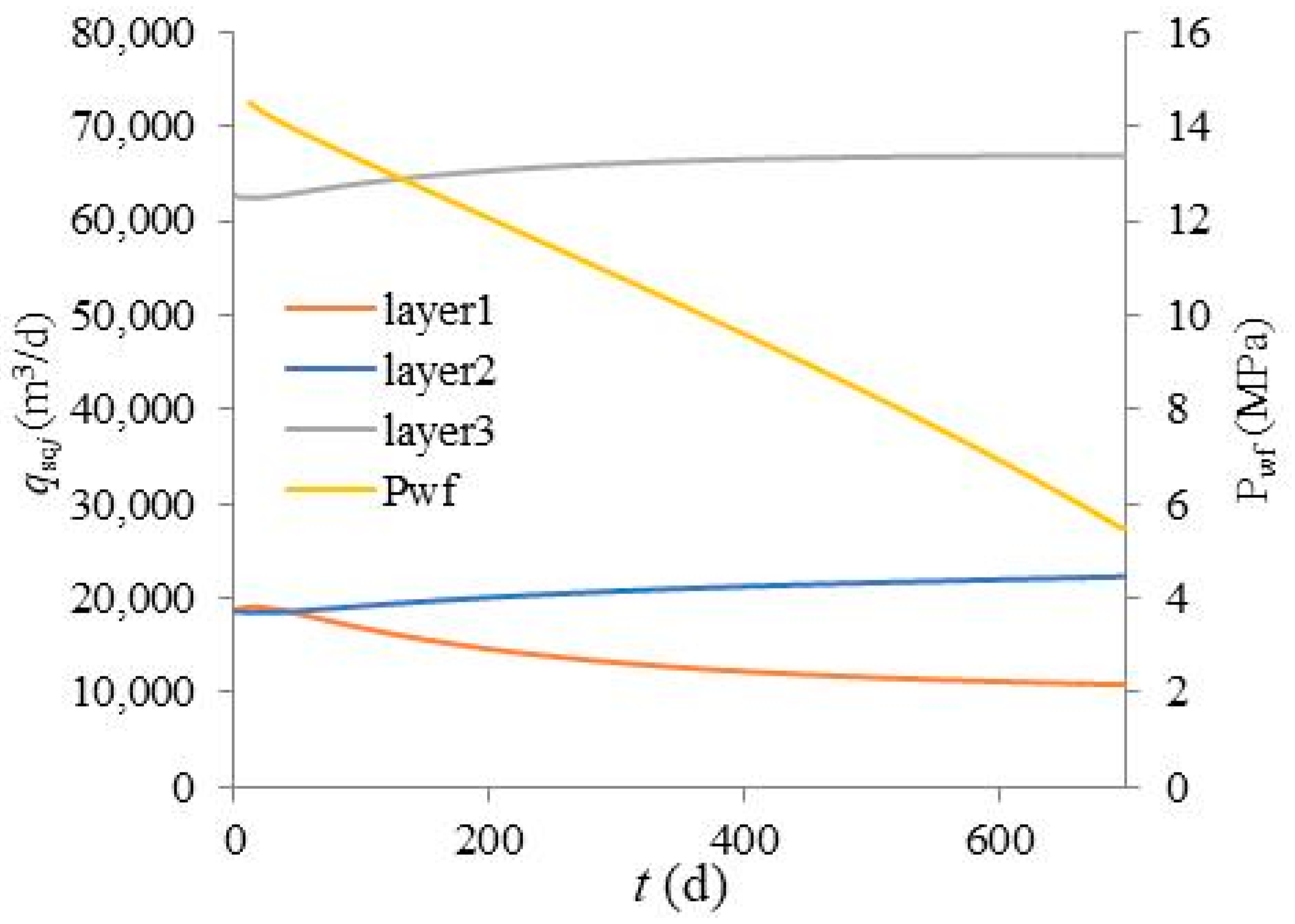
3.4. Case Study of Calculation Model of Drainage Radius
4. Discussion
5. Conclusions
- (1)
- The novel calculation model of the drainage radius of a single-layer gas well is proposed and proven to be effective and accurate. Results from the case study indicate that the error of calculated drainage radius is only 0.73%, significantly lower than that of the flowing material balance method (5.78%).
- (2)
- The newly derived calculation model of the drainage radius of multi-layer commingled gas production well is established by coupling a production capacity equation with the principle of mass balance in the closed constant-volume gas reservoir. This model is significantly concise and efficient, requiring only two gas production profile tests to swiftly calculate the drainage radius for each layer within the gas reservoir.
- (3)
- The proposed calculation model of the drainage radius of the multi-layer commingled gas production well is validated using reservoir numerical simulation. The results indicate that a maximum relative error of this model is only 2.33%, demonstrating that it has good simplicity, significant efficiency and high precision and meets the engineering calculation demands in gas fields.
- (4)
- Sensitivity analysis reveals that the calculation model of drainage radius is most sensitive to the physical properties of natural gas, while the influences of formation coefficient, skin factor and change rate of the gas rate are comparatively small.
- (5)
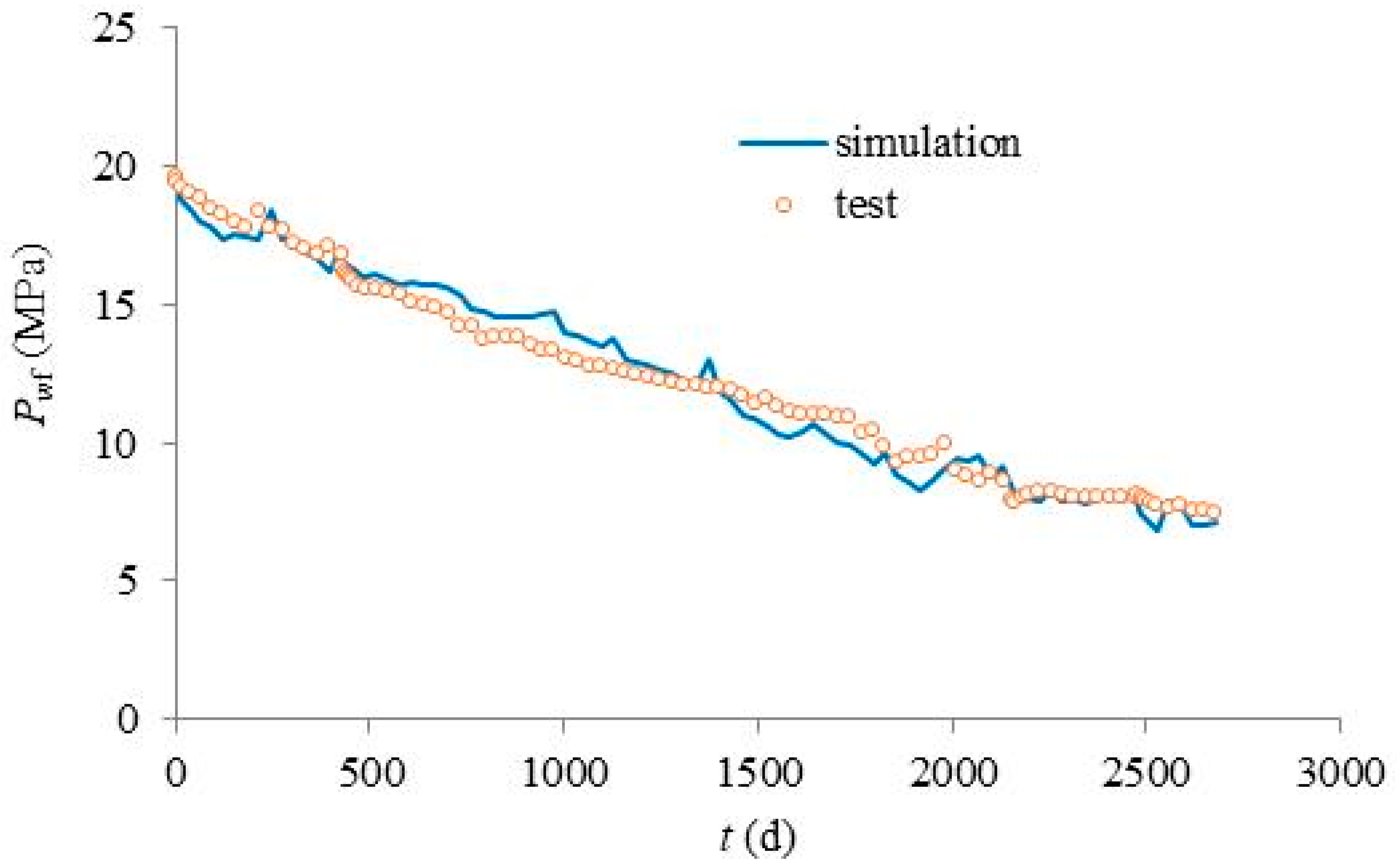
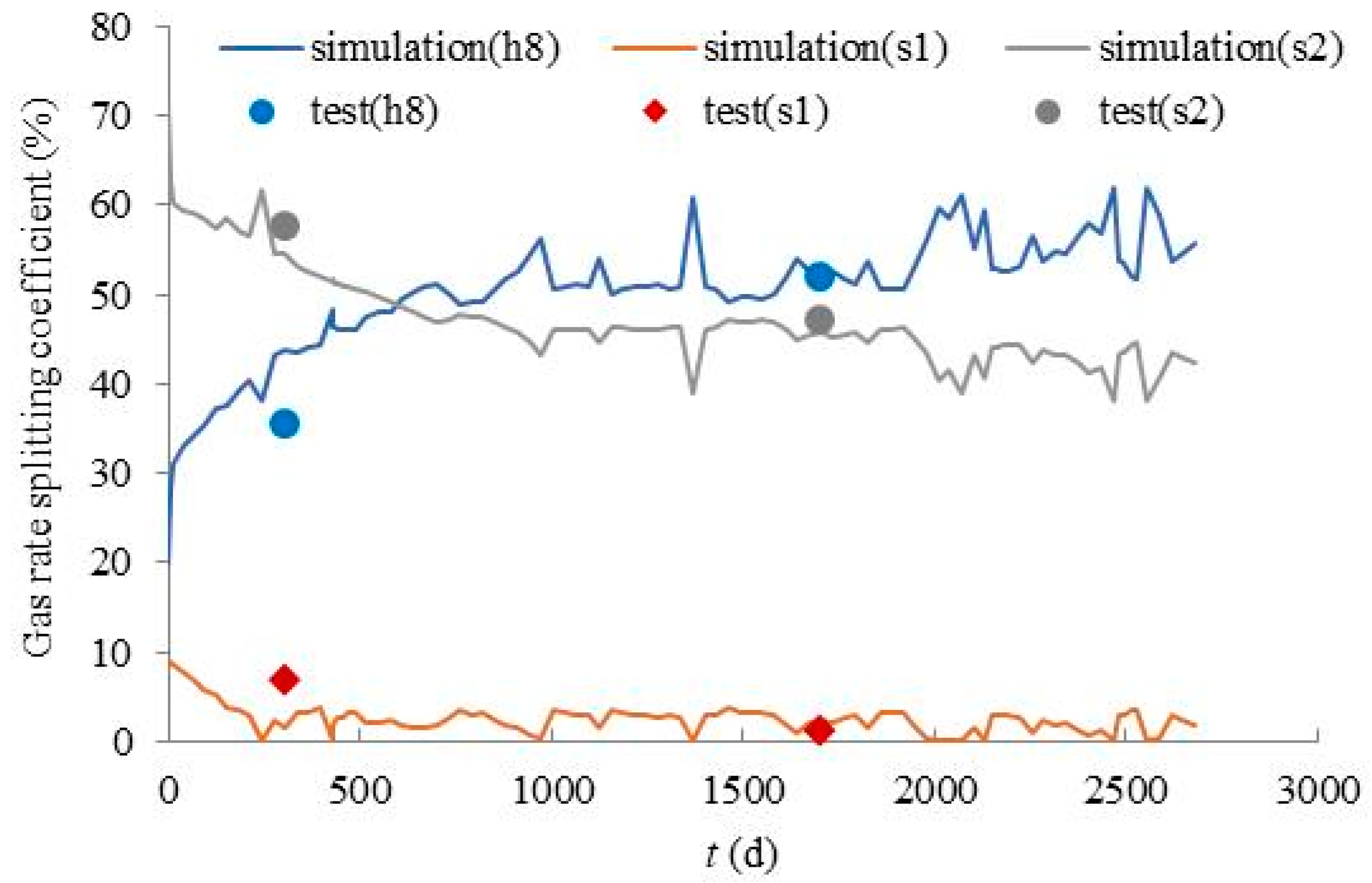
- The case study of a closed constant-volume gas reservoir with multi-layer commingled gas production well demonstrates the proposed calculation model of drainage radius has satisfactory accuracy and reliability. It can achieve a good history-match of the actual measured bottomhole flowing pressure and gas rate splitting coefficients for each layer with the simulated data.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Nie, R.-S.; Zhou, H.; Chen, Z.; Guo, J.-C.; Xiong, Y.; Chen, Y.-Y.; He, W.-F. Investigation radii in multi-zone composite reservoirs. J. Pet. Sci. Eng. 2019, 182, 106262. [Google Scholar] [CrossRef]
- Van Poollen, H.K. Radius of drainage and stabilization-time equations. Oil Gas J. 1964, 62, 138–164. [Google Scholar]
- Earlougher, R.C. Advances in Well Test Analysis, Monograph Series; SPE Press: Richardson, TX, USA, 1977; pp. 5–15. [Google Scholar]
- Daungkaew, S.; Hollaender, F.; Gringarten, A.C. Frequently Asked Questions in Well Test Analysis. In Proceedings of the SPE Annual Technical Conference and Exhibition, Dallas, TX, USA, 1–4 October 2000. [Google Scholar]
- Whittle, T.M.; Gringarten, A.C. The Determination of Minimum Tested Volume from the Deconvolution of Well Test Pressure Transients. In Proceedings of the Society of Petroleum Engineers, Denver, CO, USA, 21–24 September 2008. [Google Scholar]
- Kuchuk, F.J. Radius of Investigation for Reserve Estimation from Pressure Transient Well Tests. In Proceedings of the SPE Middle East Oil and Gas Show and Conference, Manama, Bahrain, 15–18 March 2009. [Google Scholar]
- Aguilera, R. Radius and linear distance of investigation and interconnected pore volume in naturally fractured reservoirs. J. Can. Pet. Technol. 2006, 45, 729–734. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, C.; Chen, T.; Ma, X. Modeling the nonlinear flow for a multiple fractured horizontal well with multiple finite-conductivity fractures in triple media carbonate reservoir. J. Porous Media 2018, 21, 1283–1305. [Google Scholar] [CrossRef]
- Galvao, M.S.C.; Guimaraes, C.S. A New Method for Calculating Individual Layer Permeability and Skin in a Multilayered Reservoir Using Production Logging Data: The Delta Transient Method. In Proceedings of the SPE Latin America and Caribbean Mature Fields Symposium, Salvador, Brazil, 15–16 March 2017. [Google Scholar]
- Guimaraes, C.S.; Galvao, M.S.C. Application of the Delta Transient Method to Multi-Rate Tests: A Method for Calculating Individual Layer Permeability and Skin in a Multilayered Reservoir using Production Logging Data. In Proceedings of the Offshore Technology Conference, Rio de Janeiro, Brazil, 24–26 October 2017. [Google Scholar]
- Bela, R.V.; Pesco, S.; Barreto, A.B.; Onur, M. Assisted history matching and graphical methods for estimating individual layer properties from well testing data in stratified reservoirs with multilateral wells. J. Pet. Sci. Eng. 2022, 208, 109326. [Google Scholar] [CrossRef]
- Sallam, M.A.; El-Banbi, A.H. Analysis of multi-layered commingled and compartmentalized gas reservoirs. J. Pet. Explor. Prod. Technol. 2018, 8, 1573–1586. [Google Scholar] [CrossRef]
- Juell, A.; Whitson, C.H. Backpressure equation for layered gas reservoirs. In Proceedings of the SPE Annual Technical Conference and Exhibition, Denver, CO, USA, 30 October–2 November 2011. [Google Scholar]
- Ramakrishnan, T.S.; Prange, M.D.; Kuchuk, F.J. Radius of investigation in pressure transient testing. Transp. Porous Media 2020, 12, 106–112. [Google Scholar] [CrossRef]
- Wang, Y.; Yi, X. Flow modeling of well test analysis for a multiple-fractured horizontal well in triple media carbonate reservoir. Int. J. Nonlinear Sci. Numer. Simul. 2018, 19, 439–457. [Google Scholar] [CrossRef]
- Wei, M.; Duan, Y.; Dong, M.; Fang, Q.; Dejam, M. Transient production decline behavior analysis for a multi-fractured horizontal well with discrete fracture networks in shale gas reservoirs. J. Porous Media 2019, 22, 343–361. [Google Scholar] [CrossRef]
- Blasingame, T.A.; Johnston, J.L.; Lee, W.J. Type-Curve Analysis Using the Pressure Integral Method. In Proceedings of the SPE California Regional Meeting, Bakersfield, CA, USA, 5–9 April 1989. [Google Scholar]
- Mattar, L.; Mcneil, R. The flowing gas material balance. J. Can. Pet. Technol. 1998, 37, 52–55. [Google Scholar] [CrossRef]
- Shariff, A.J. An Approach for Determining the Drainage Boundary of the Gas Wells Producing from Low-Permeability Gas Reservoirs. In Proceedings of the SPE Unconventional Gas Technology Symposium, Louisville, KY, USA, 18–21 May 1986. [Google Scholar]
- Schilthuis, R.J. Active Oil and Reservoir Energy. Trans. Aime 1936, 118, 33–52. [Google Scholar] [CrossRef]
- Havlena, D.; Odeh, A.S. The Material Balance as an Equation of a Straight Line? Part II, Field Cases. J. Can. Pet. Technol. 1964, 16, 815–822. [Google Scholar] [CrossRef]
- Hirakawa, S. Gas Reservoir Material Balance. J. Jpn. Assoc. Petrol. Technol. 1959, 24, 1–2. [Google Scholar] [CrossRef]
| Property | Value |
|---|---|
| K (mD) | 1 |
| h (m) | 10 |
| (%) | 7 |
| Sgi | 0.25 |
| Pi (MPa) | 20 |
| Ti (°C) | 88.66 |
| Pi (MPa) | 20 |
| rw (m) | 0.1 |
| qsc (m3/d) | 30,000 |
| re (m) | 500 |
| Property | Actual Value of Gas Reservoir Model | Flowing Material Balance Method | Modified Flowing Material Balance Method | ||
|---|---|---|---|---|---|
| Value | Error (%) | Value | Error (%) | ||
| Gi (108 m3) | 0.7581 | 0.5774 | 23.84 | 0.7403 | 2.35 |
| re (m) | 500 | 436.4 | 12.72 | 494.1 | 1.18 |
| Property | Material Balance Method | Flowing Material Balance Method | Modified Flowing Material Balance Method | ||
|---|---|---|---|---|---|
| Value | Error (%) | Value | Error (%) | ||
| Gi (108 m3) | 0.9180 | 0.8150 | 11.22 | 0.9047 | 1.45 |
| re (m) | 617.9 | 582.2 | 5.78 | 613.4 | 0.73 |
| Reservoir Property | Layer 1 | Layer 2 | Layer 3 |
|---|---|---|---|
| Kj (mD) | 1 | 1.5 | 2 |
| hj (m) | 6 | 8 | 10 |
| (%) | 7 | 7 | 7 |
| Sgij | 0.8 | 0.8 | 0.8 |
| Tij (°C) | 88.66 | 90.68 | 92.91 |
| rw (m) | 0.2 | ||
| qsc (m3/d) | 100,000 | ||
| rej (m) | 300 | 500 | 700 |
| Production Time | Production Data | Layer 1 | Layer 2 | Layer 3 |
|---|---|---|---|---|
| 300 d | qscj (m3/d) | 13,216.9 | 20,740.4 | 66,042.7 |
| Pwf (MPa) | 10.9988 | |||
| 600 d | qscj (m3/d) | 11,181.7 | 21,973.9 | 66,844.4 |
| Pwf (MPa) | 7.1236 | |||
| rej-calculated (m) | 295.7 | 492.3 | 716.3 | |
| Relative Error (%) | 1.43 | 1.54 | 2.33 | |
| Time | Property | Layer h8 | Layer s1 | Layer s2 |
|---|---|---|---|---|
| Kj (mD) | 0.2 | 0.17 | 0.276 | |
| hj (m) | 10.4 | 4.6 | 8.6 | |
| (%) | 7.6 | 6.5 | 4.7 | |
| Sgij | 0.69 | 0.71 | 0.75 | |
| Tij (°C) | 90.5 | 91.7 | 93.1 | |
| Sj | −1.5 | −1 | −4 | |
| rw (m) | 0.1 | |||
| 3 December 2015 | Pwfj (MPa) | 16.5336 | 16.6436 | 16.9107 |
| qscj (m3/d) | 4977 | 951 | 8094 | |
| 25 September 2019 | Pwfj (MPa) | 8.4850 | 8.5426 | 8.7133 |
| qscj (m3/d) | 6400 | 133 | 5802 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xin, C.; Zhou, W.; Zhang, L.; Qiao, X.; Wang, Y.; Xiao, Y. Calculation Model of Drainage Radius of Single-Layer/Multi-Layer Commingled Gas Production Wells in a Closed Constant-Volume Gas Reservoir and Its Application. Appl. Sci. 2024, 14, 1873. https://doi.org/10.3390/app14051873
Xin C, Zhou W, Zhang L, Qiao X, Wang Y, Xiao Y. Calculation Model of Drainage Radius of Single-Layer/Multi-Layer Commingled Gas Production Wells in a Closed Constant-Volume Gas Reservoir and Its Application. Applied Sciences. 2024; 14(5):1873. https://doi.org/10.3390/app14051873
Chicago/Turabian StyleXin, Cuiping, Wei Zhou, Lei Zhang, Xiangyang Qiao, Yongke Wang, and Yue Xiao. 2024. "Calculation Model of Drainage Radius of Single-Layer/Multi-Layer Commingled Gas Production Wells in a Closed Constant-Volume Gas Reservoir and Its Application" Applied Sciences 14, no. 5: 1873. https://doi.org/10.3390/app14051873
APA StyleXin, C., Zhou, W., Zhang, L., Qiao, X., Wang, Y., & Xiao, Y. (2024). Calculation Model of Drainage Radius of Single-Layer/Multi-Layer Commingled Gas Production Wells in a Closed Constant-Volume Gas Reservoir and Its Application. Applied Sciences, 14(5), 1873. https://doi.org/10.3390/app14051873







