Study on the Influence of a Rigid Wall on Cavitation in Underwater Explosions Near the Free Surface
Abstract
:1. Introduction
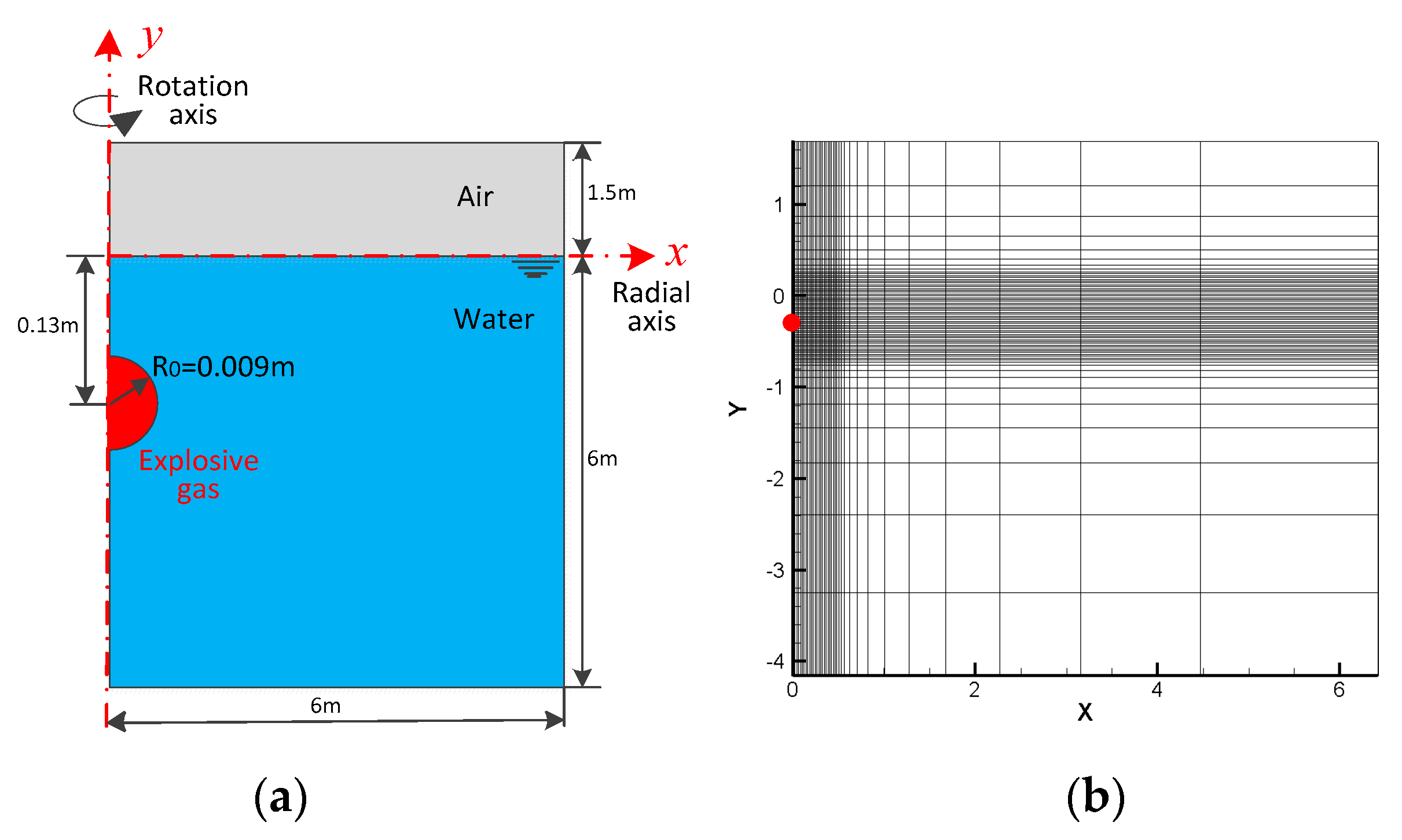
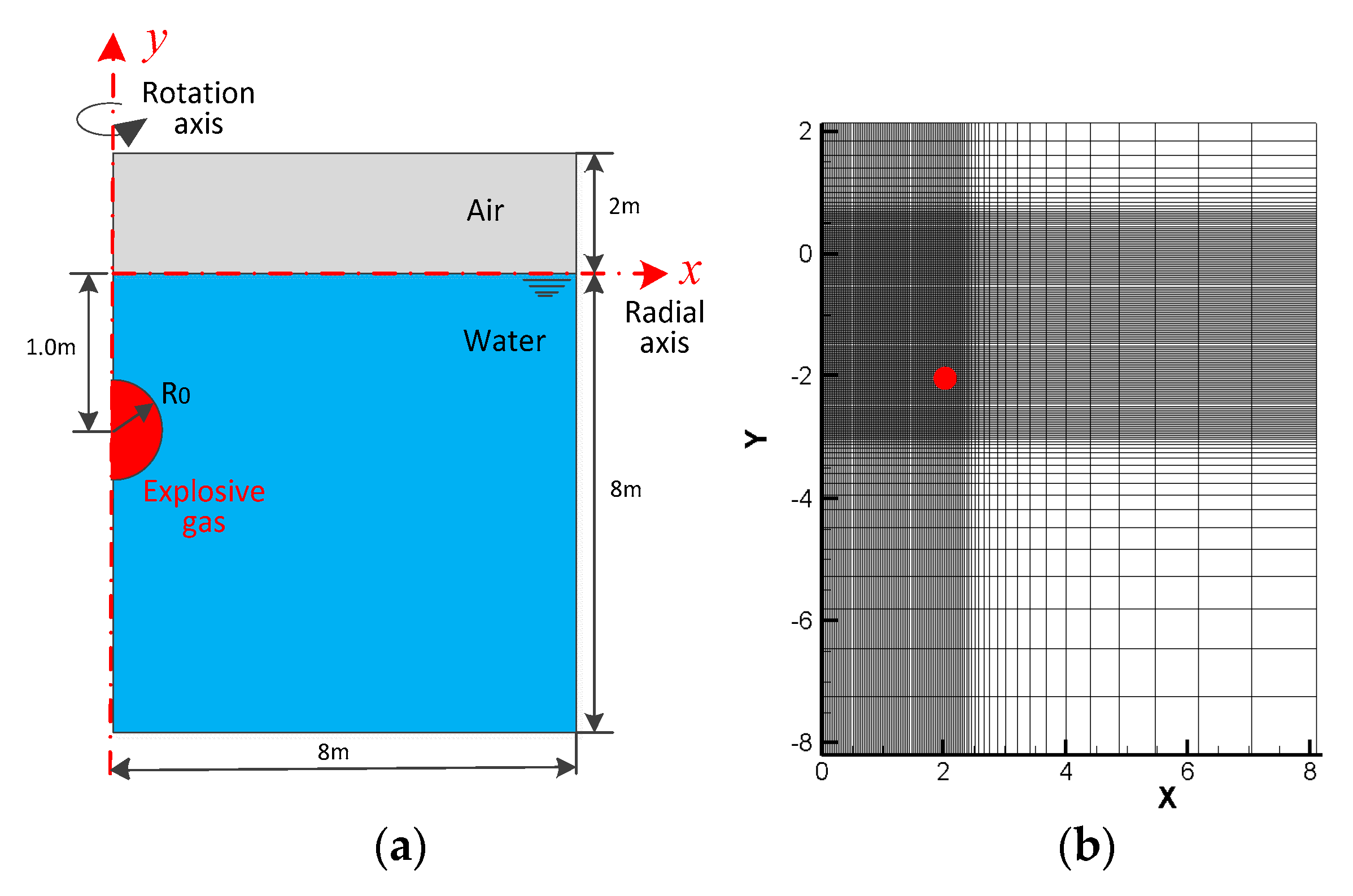
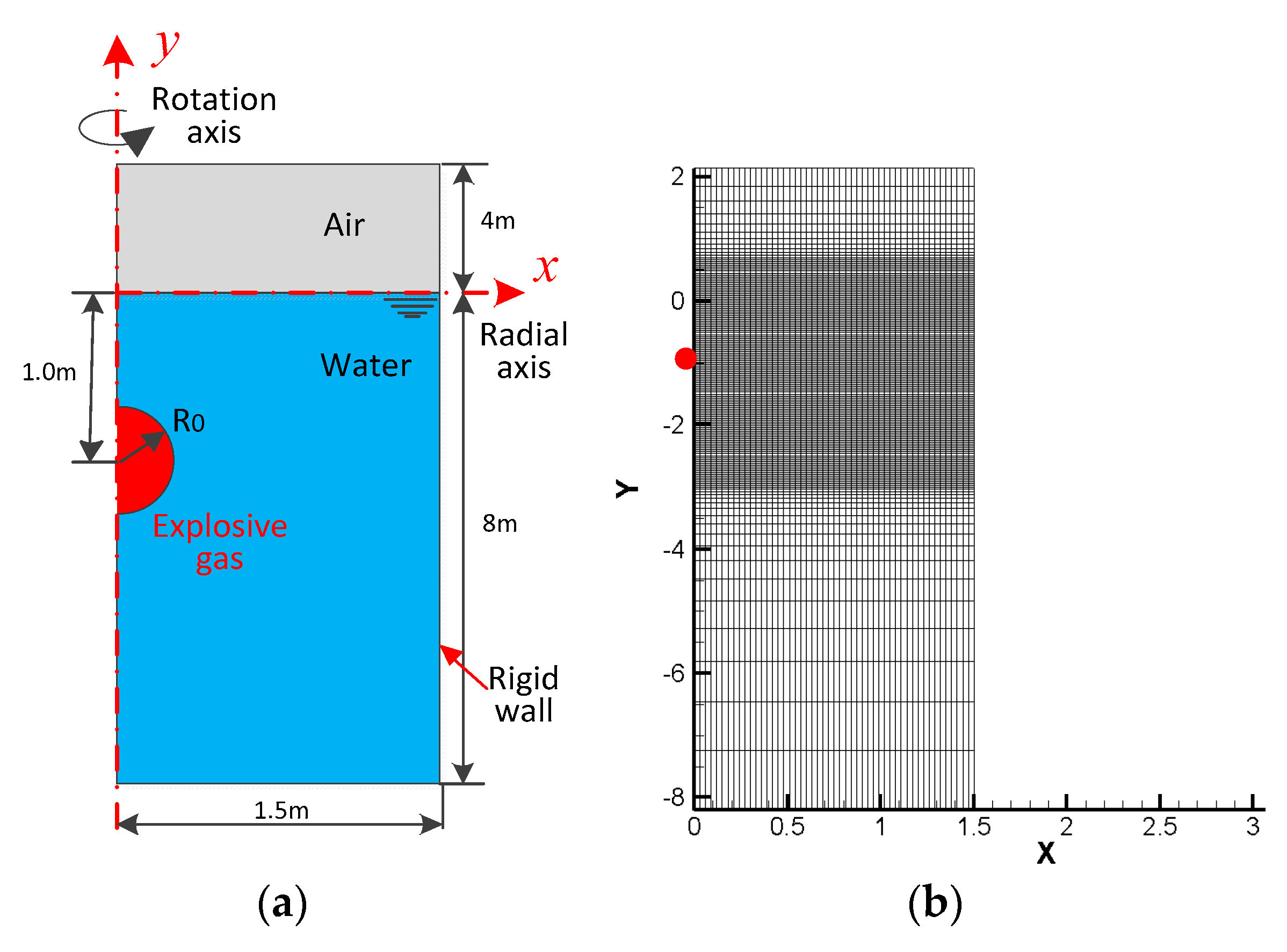
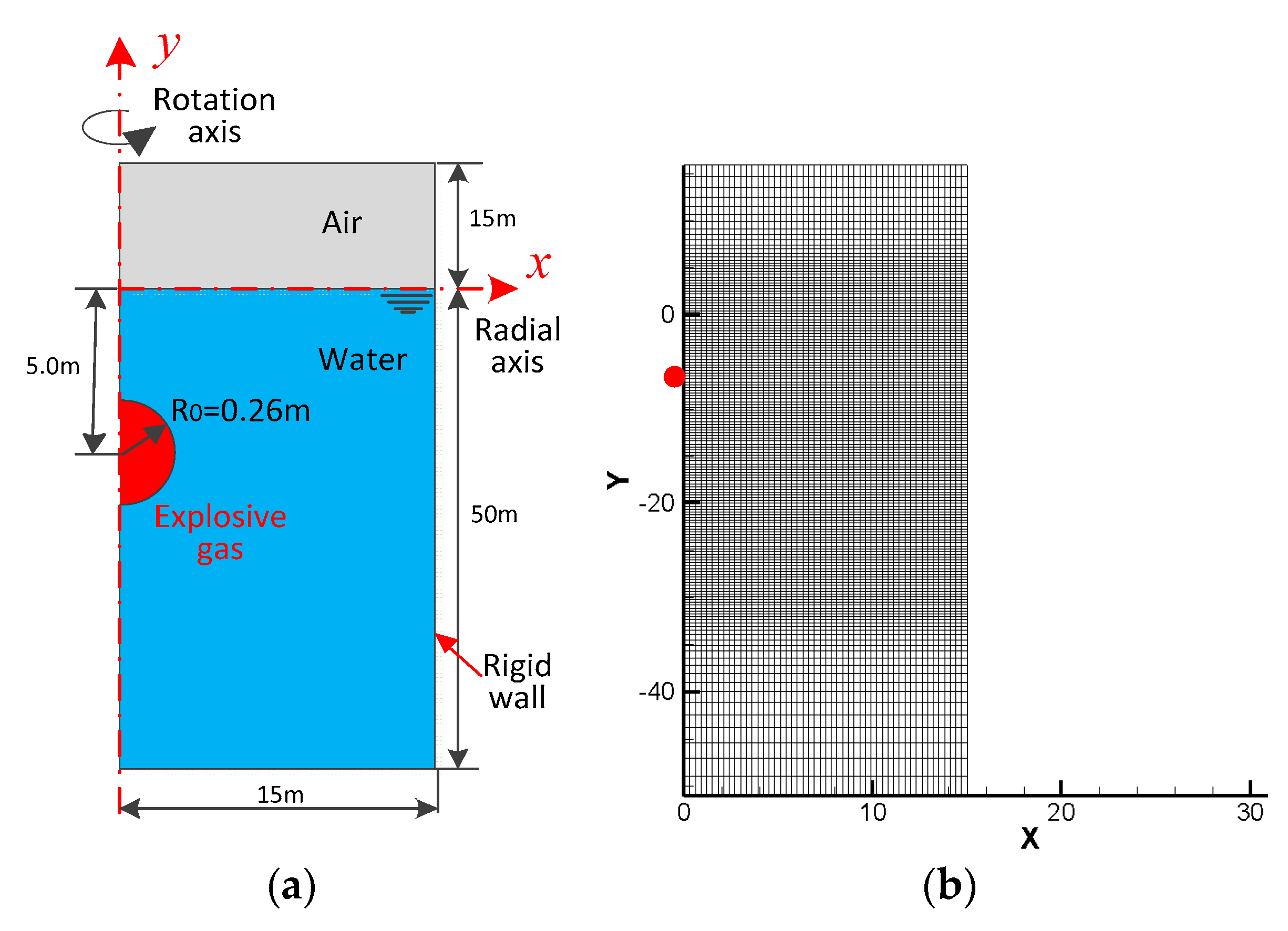
2. Numerical Method
3. Results and Discussions
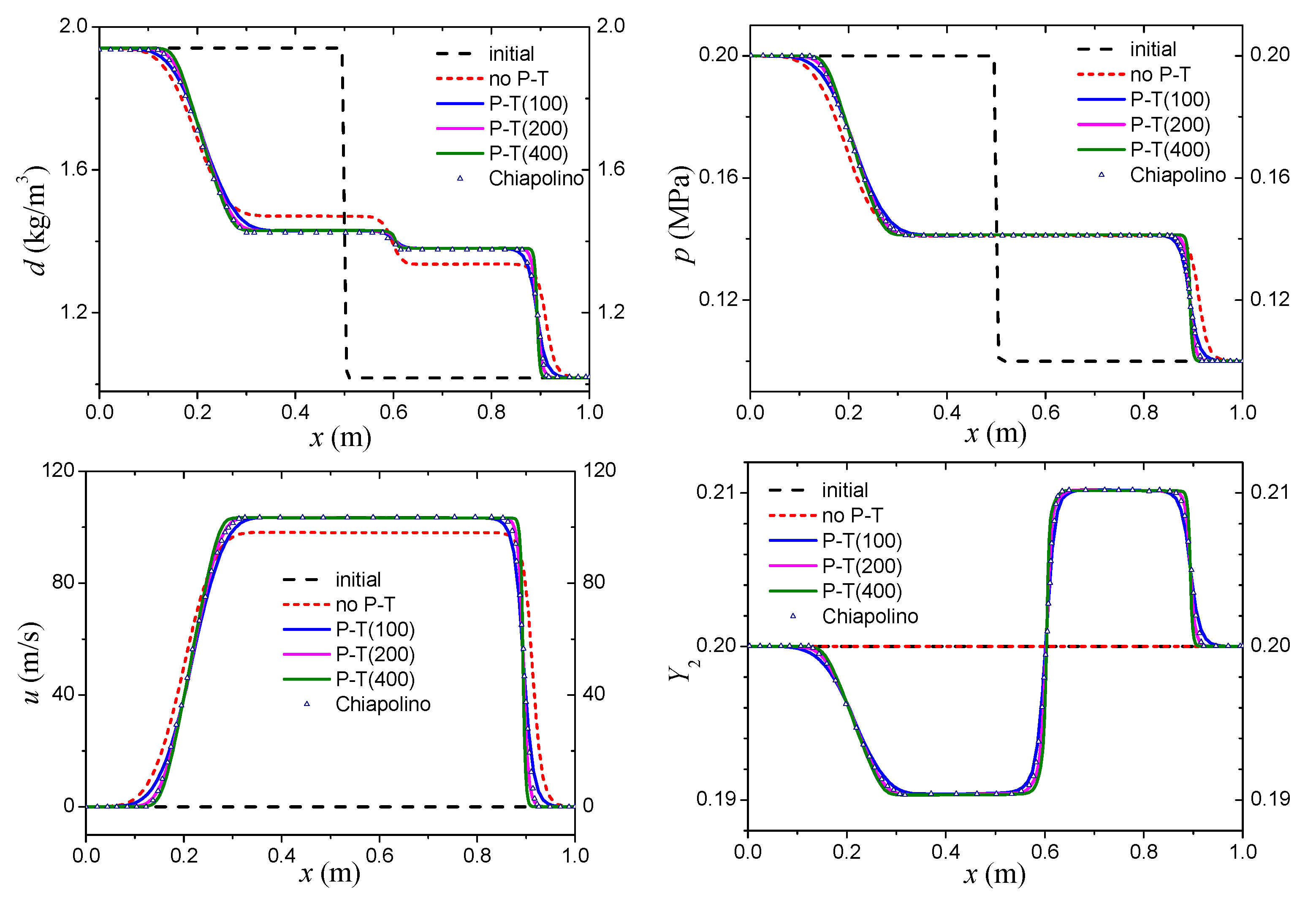
3.1. Testing and Verification
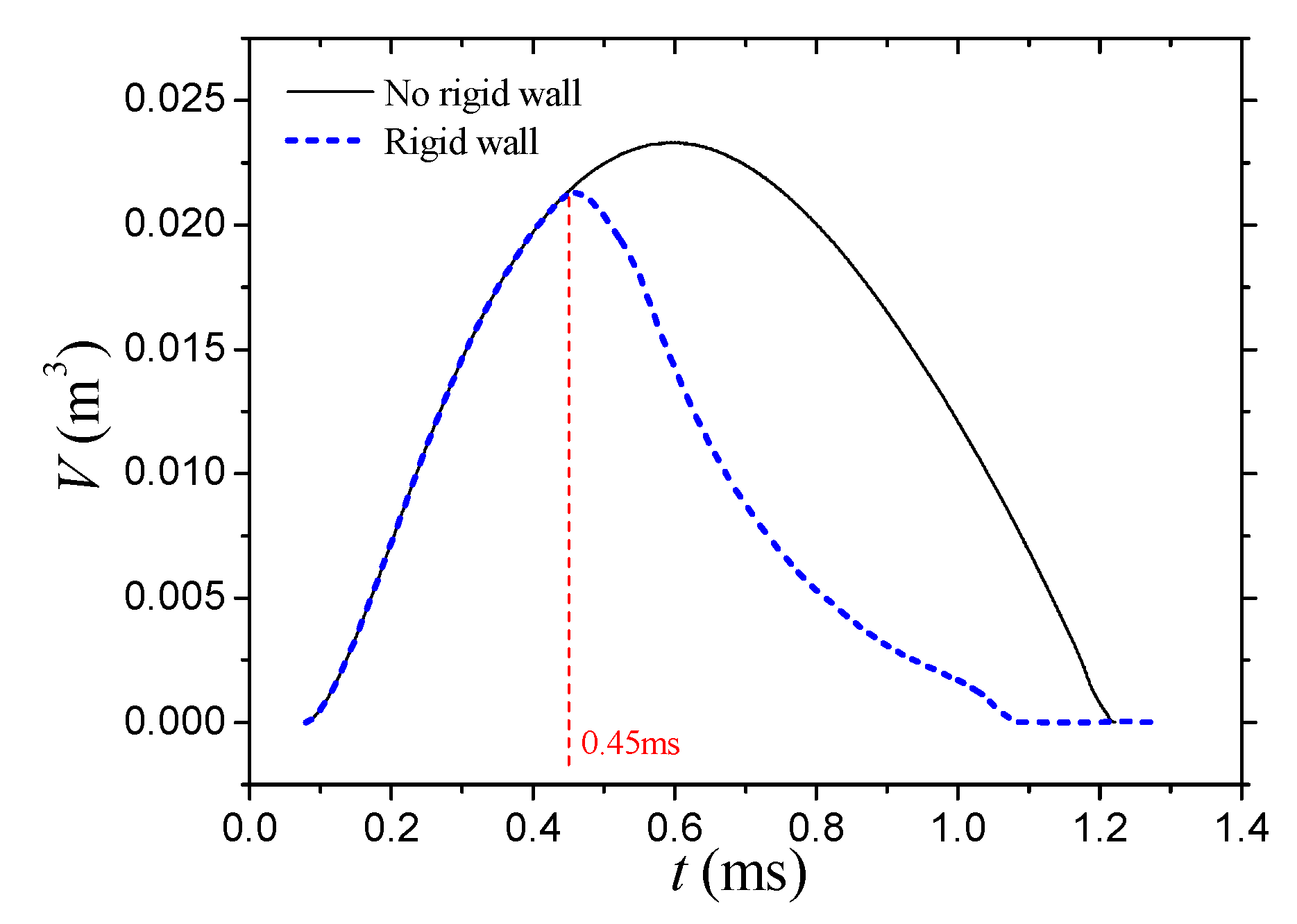
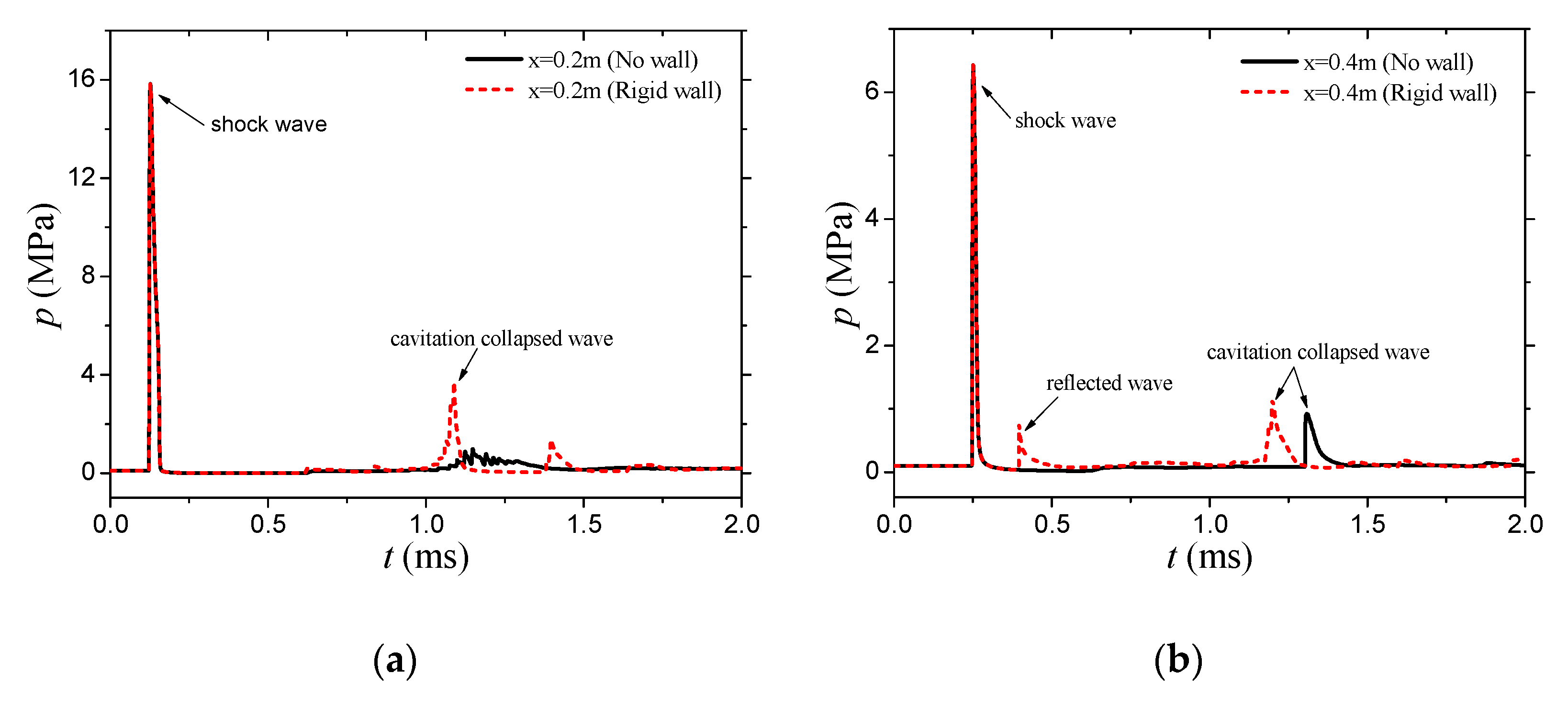
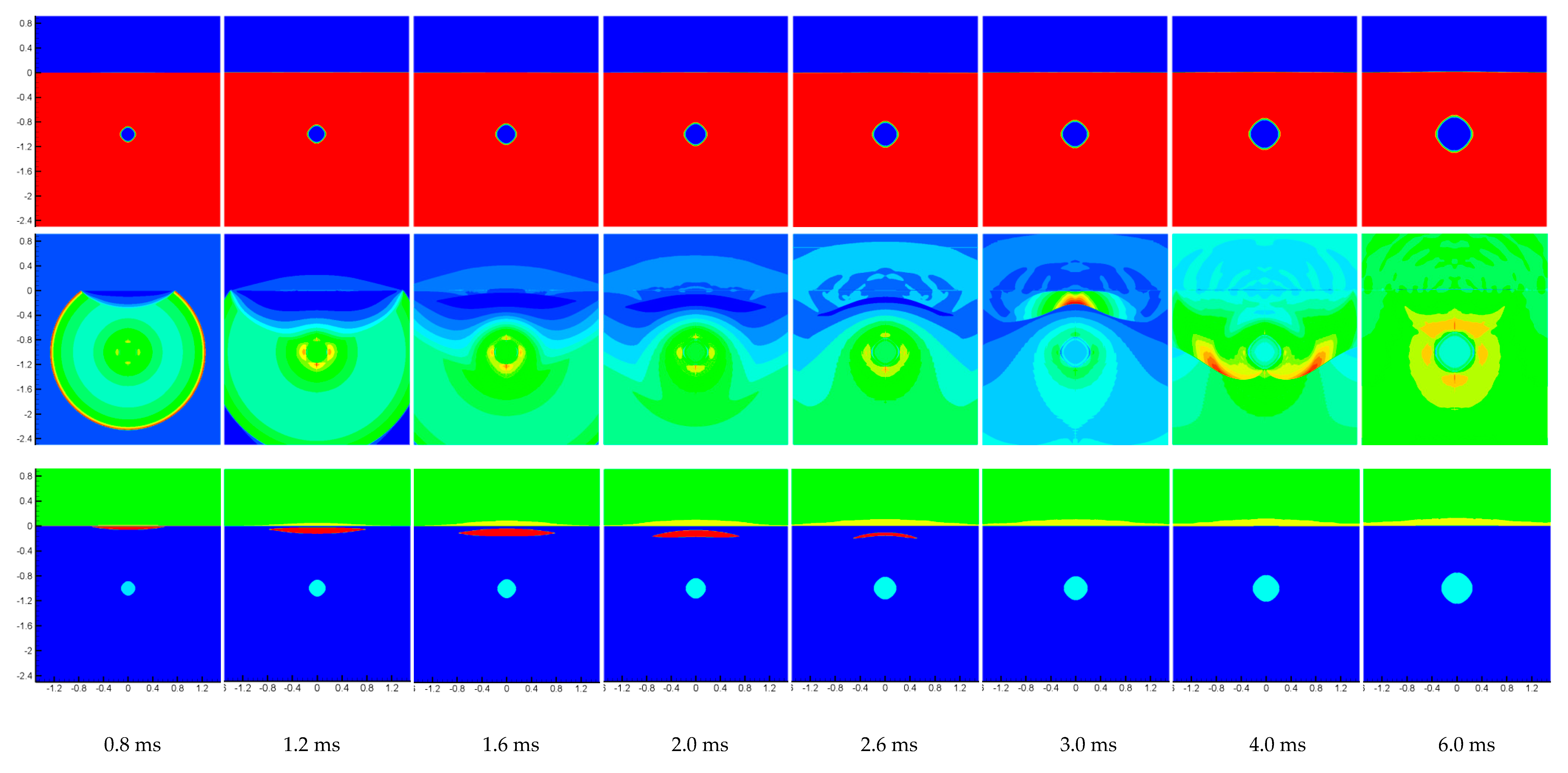
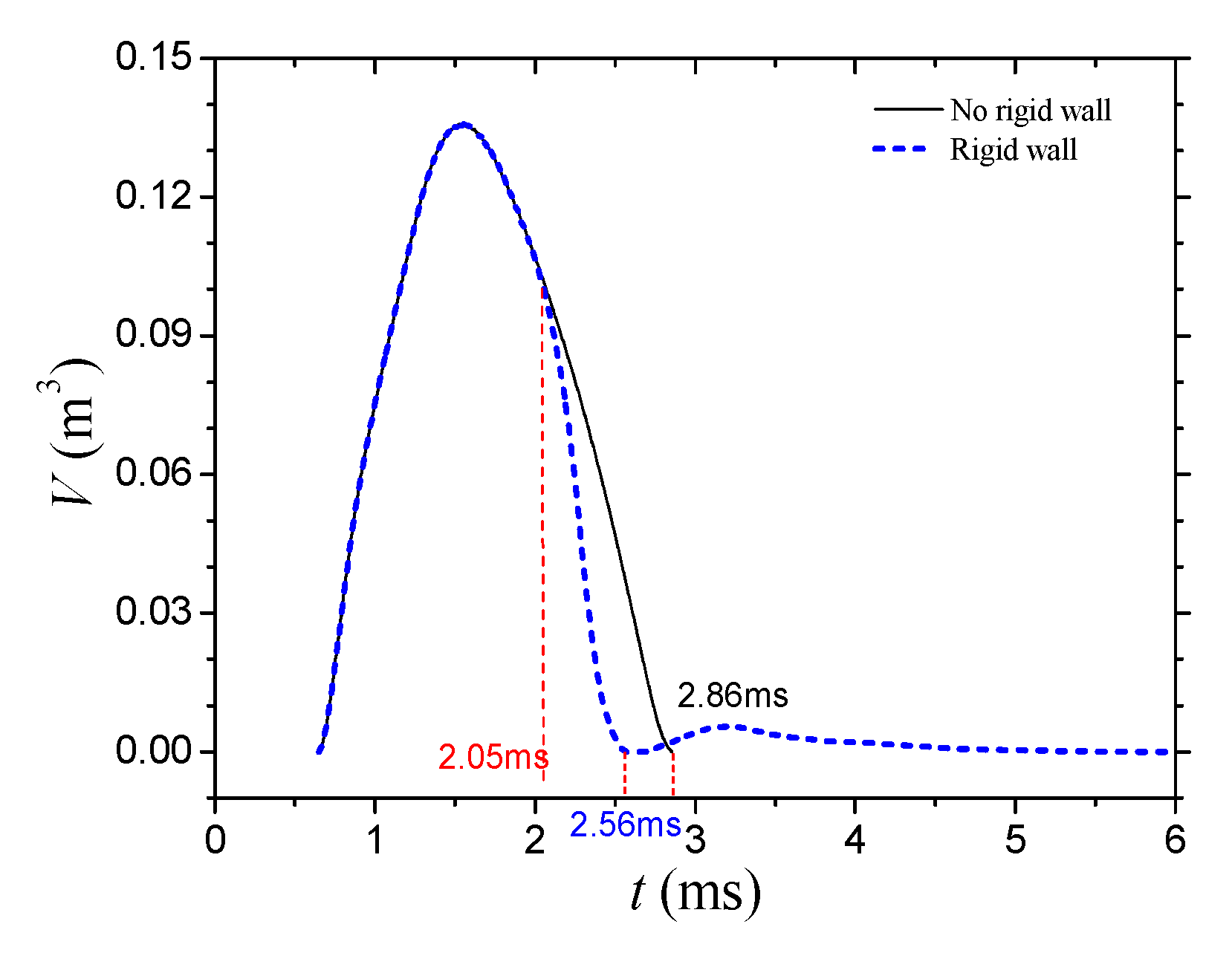
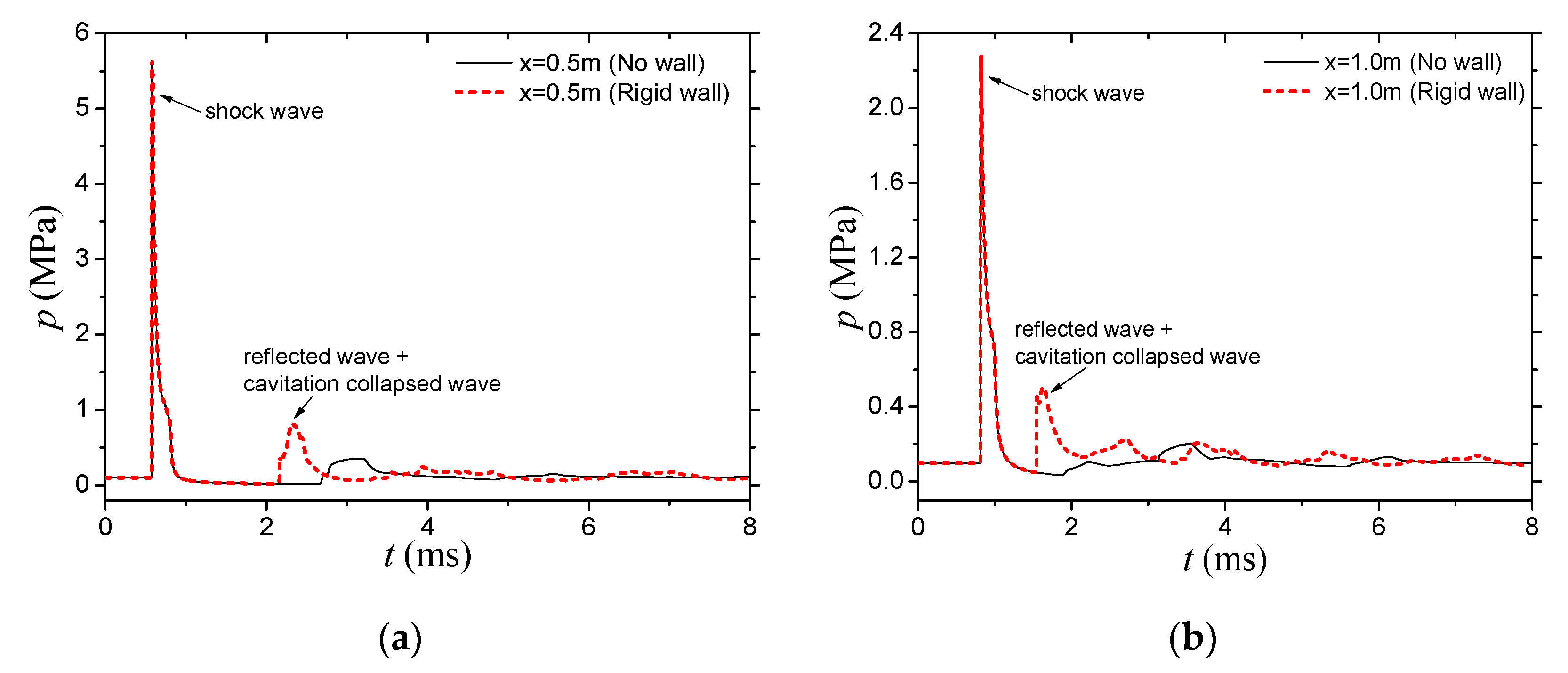
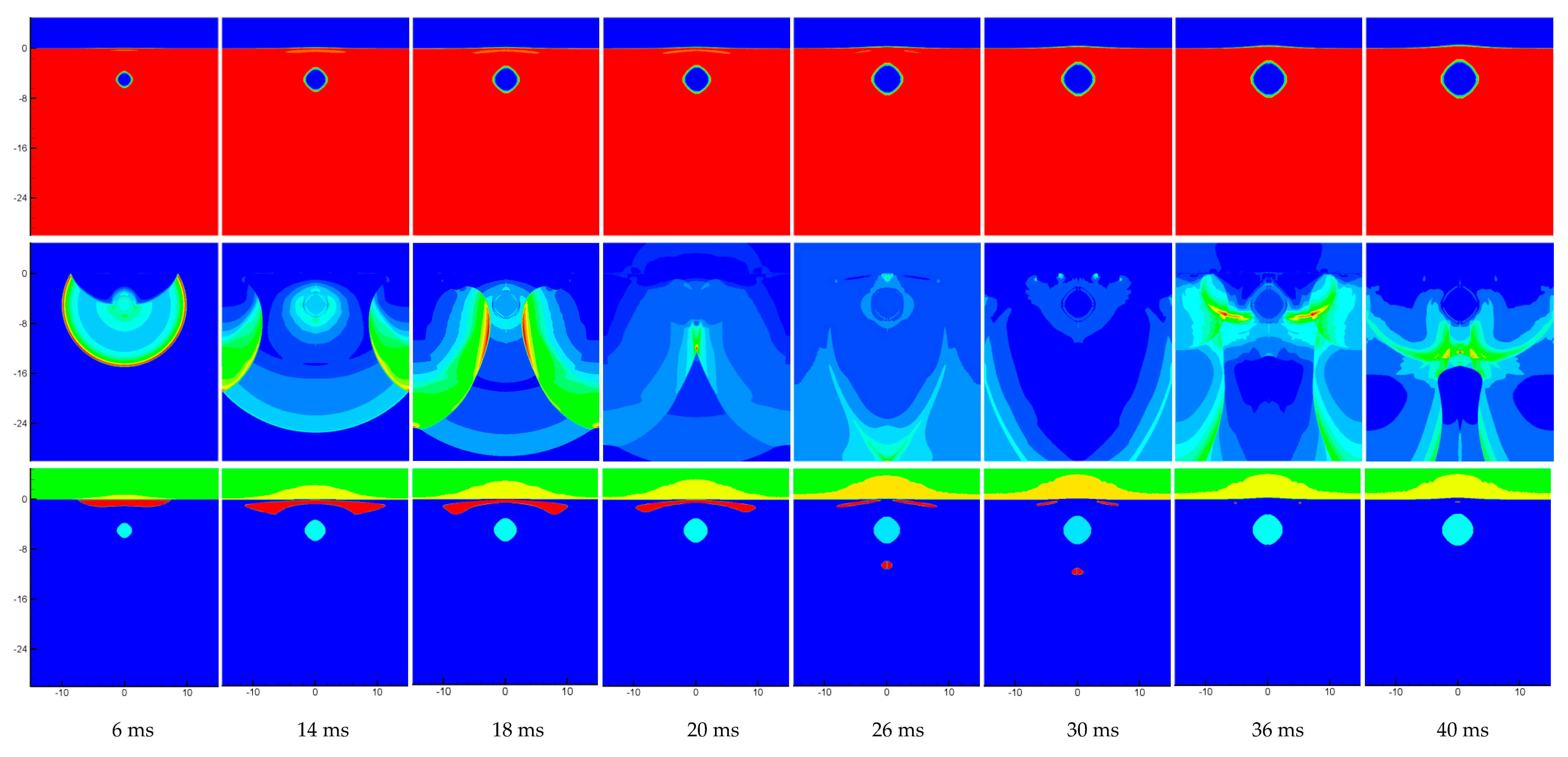
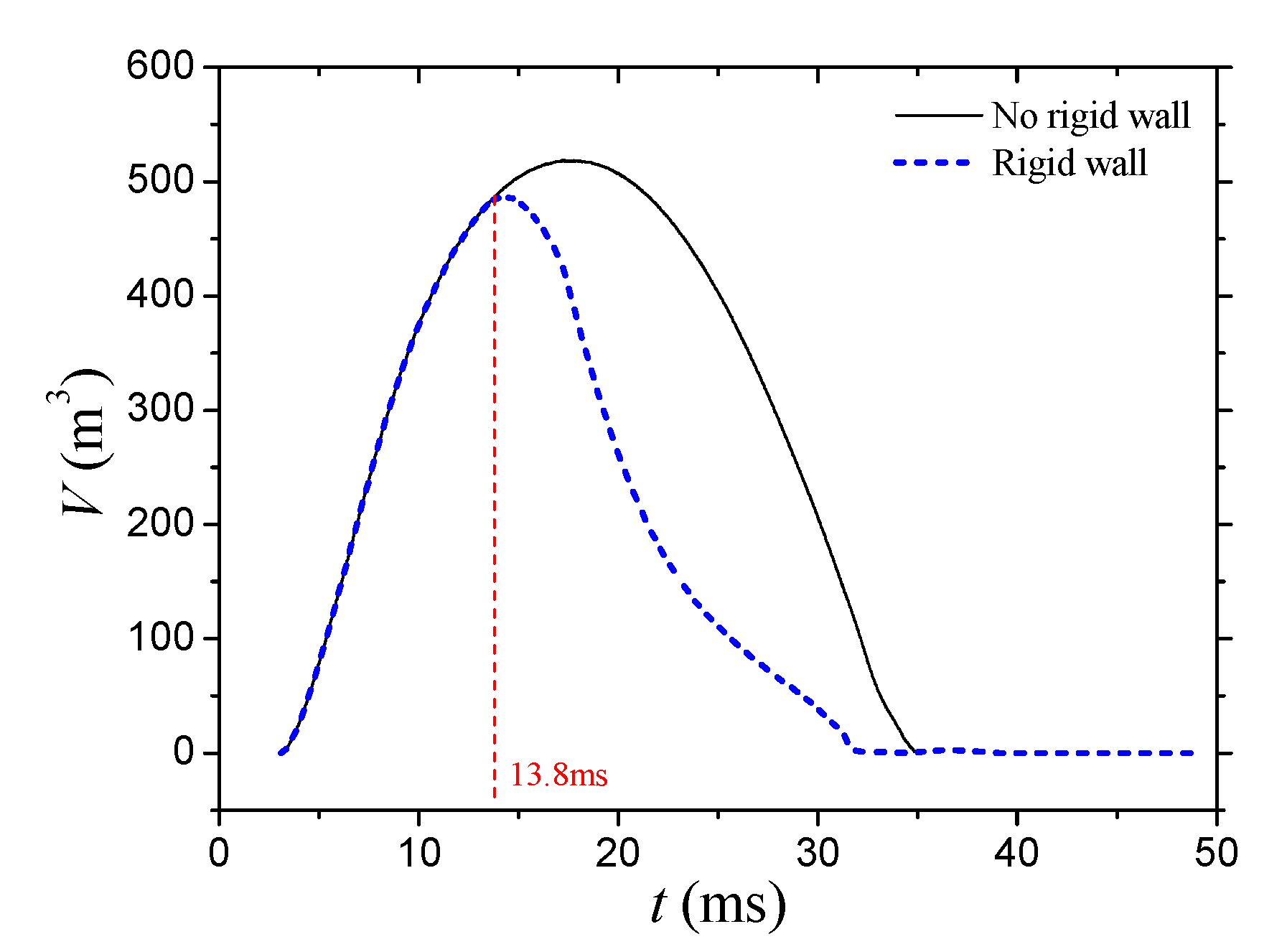
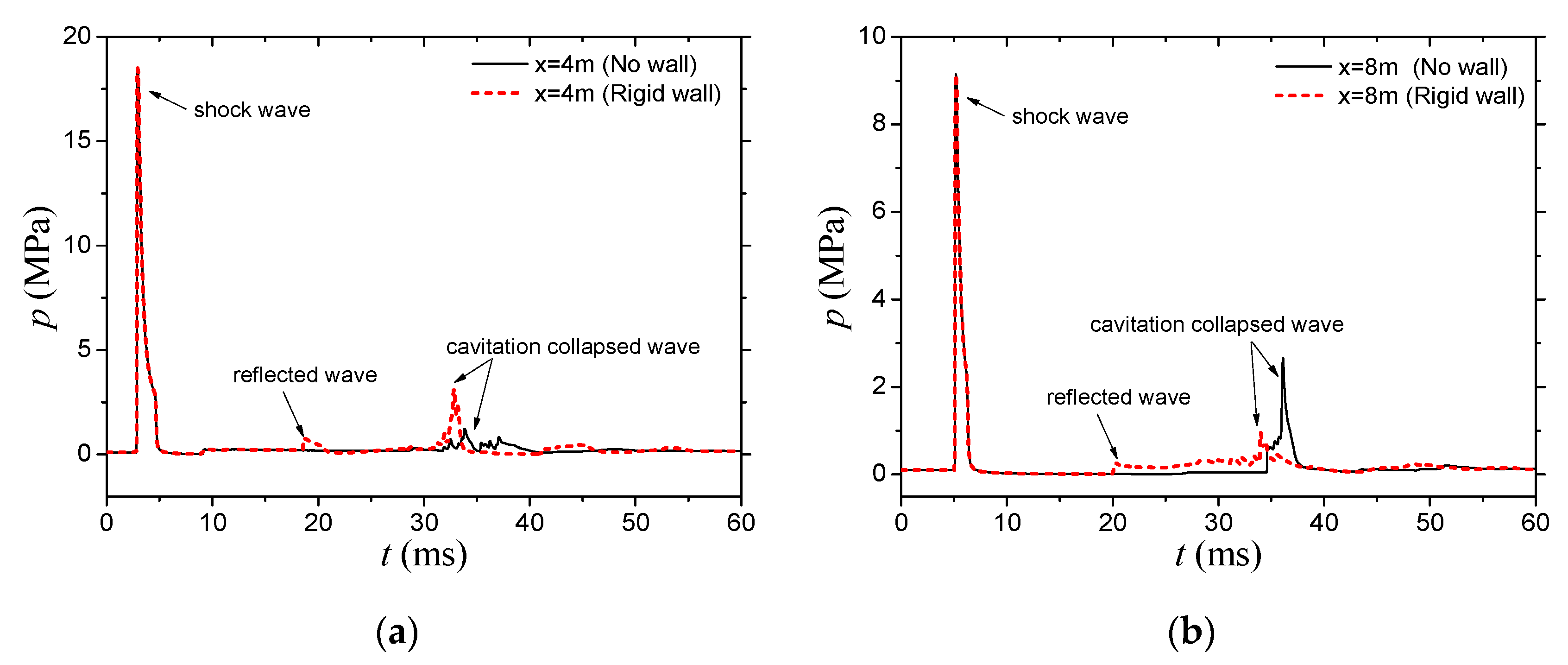
3.2. Effect of Rigid Wall on Small-Charge Underwater Explosion near Free Surface
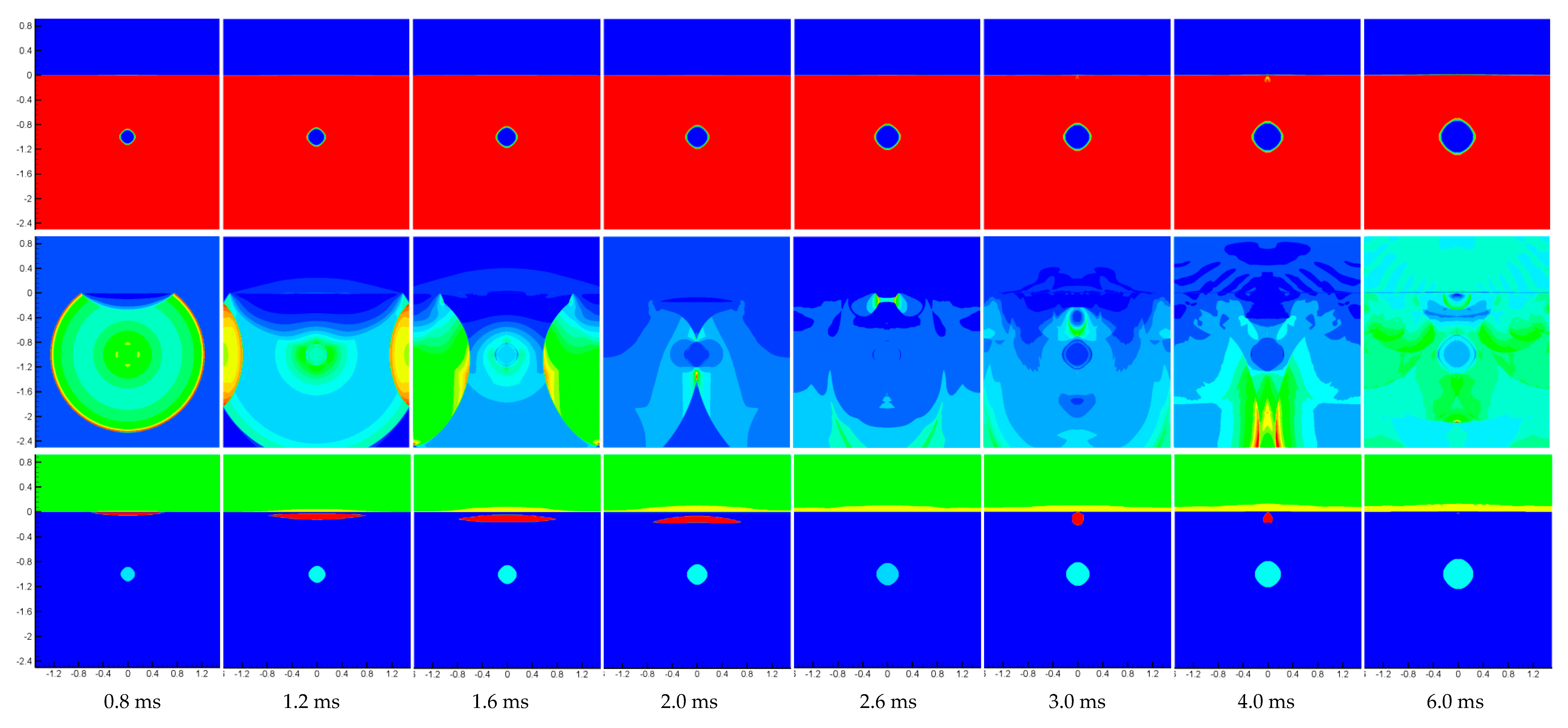
3.3. Effect of Rigid Wall on Medium-Charge Underwater Explosion near Free Surface
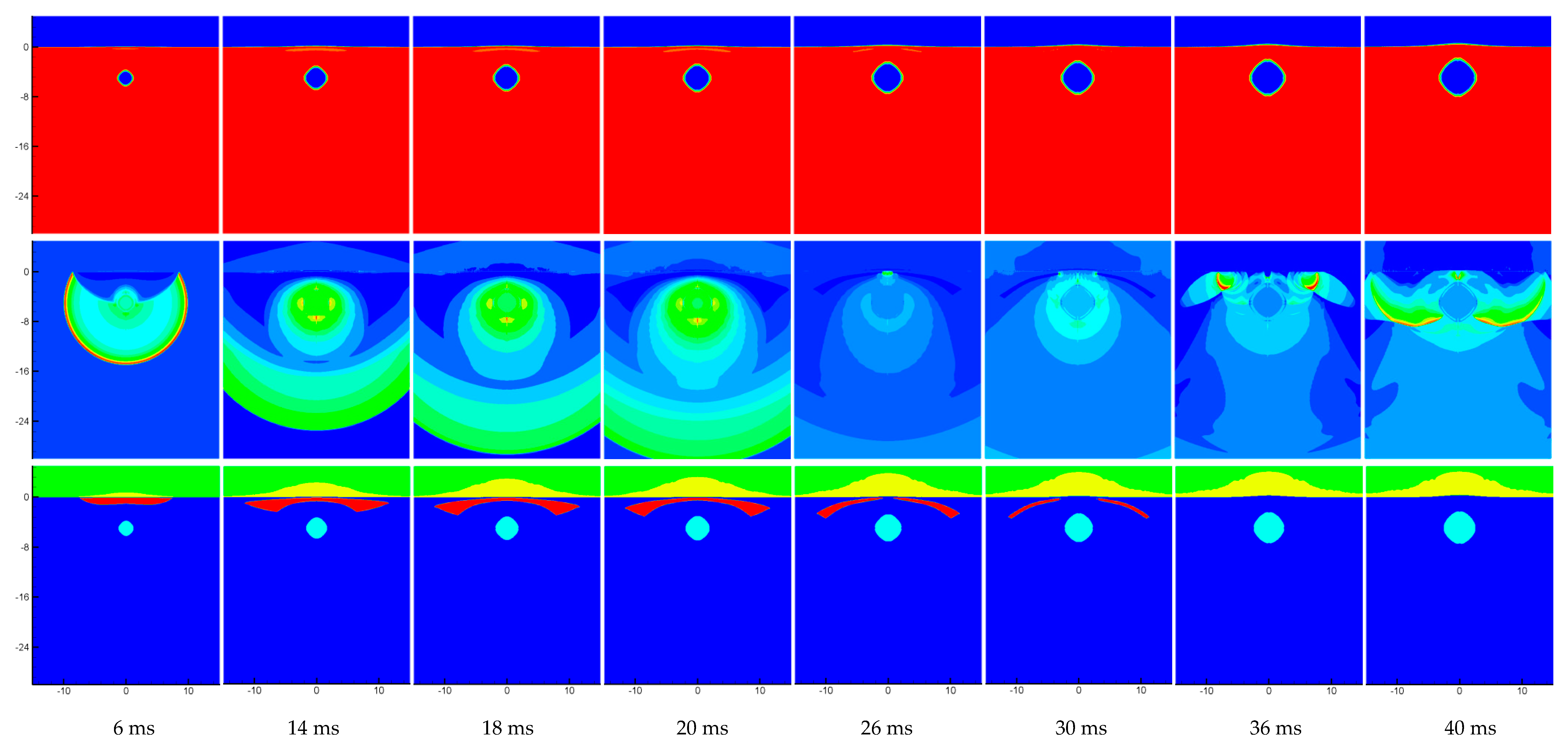
3.4. Effect of Rigid Wall on Large-Charge Underwater Explosion near Free Surface
4. Conclusions
- (1)
- The presence of a rigid wall significantly affects the cavitation evolution process in underwater explosions. It influences the shape, size, and dynamics of the cavitation domain, as well as the interaction between the explosion and the surrounding fluid.
- (2)
- The cavitation collapse load is influenced by the existence of a rigid wall. The collapse time and collapse pressure of the cavitation domain are significantly different compared to the free-field condition. These differences arise from the interaction between the reflected wave on the wall and the cavitation collapse load.
- (3)
- The size and position of the cavitation domain undergo notable changes under different operating conditions. The proximity of the rigid wall leads to distinct patterns in the cavitation domain evolution, resulting in variations in the pressure distribution and the emergence of new cavitation regions.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kedrinskii, V.K. Hydrodynamics of Explosion-Experiments and Models; Springer: Berlin/Heidelberg, Germany, 2005; p. 223. [Google Scholar]
- Chen, Y.; Heister, S.D. A numerical treatment for attached cavitation. J. Fluid Eng. 1994, 116, 613. [Google Scholar] [CrossRef]
- Rachid, F.; Felipe, B. A thermodynamically consistent model for cavitating flows of compressible fluids. Int. J. Nonlin Mech. 2003, 38, 1007. [Google Scholar] [CrossRef]
- Nikita, P.V.; Alexander, A.S. Multiphase phenomena in underwater explosion. Exp. Therm. Fluid Sci. 2015, 60, 367. [Google Scholar]
- Xie, W.F.; Liu, T.G.; Khoo, B.C. Application of a one-fluid model for large scale homogeneous unsteady cavitation: The modified Schmidt model. Comput. Fluids 2006, 35, 1177. [Google Scholar] [CrossRef]
- Phan, T.H.; Nguyen, V.T.; Park, W.G. Numerical study on strong nonlinear interactions between spark- generated underwater explosion bubbles and a free surface. Int. J. Heat Tran. 2020, 163, 120506. [Google Scholar] [CrossRef]
- Yu, J.; Liu, G.Z.; Wang, J.; Wang, H.K. An effective method for modeling the load of bubble jet in underwater explosion near the wall. Ocean Eng. 2021, 220, 108408. [Google Scholar] [CrossRef]
- Felippa, C.A.; DeRuntz, J.A. Finite element analysis of shock-induced hull cavitation. Comput. Methods Appl. Mech. Eng. 1984, 44, 297. [Google Scholar] [CrossRef]
- Sprague, M.A.; Geers, T.L. A spectral-element method for modeling cavitation in transient fluid-structure interaction. J. Comput. Phys. 2003, 184, 149. [Google Scholar] [CrossRef]
- Sprague, M.A. Advanced Computational Techniques for the Analysis of 3-D Fluid-Structure Interaction with Cavitation. Ph.D. Thesis, University of Colorado at Boulder, Boulder, CO, USA, 2002. [Google Scholar]
- Stefan, S.; Austen, A. Fluid structure Interaction-preliminary assessment of LS-DYNA/USA acoustic shock methods. In Proceedings of the 80th Shock and Vibration Symposium, San Diego, CA, USA, 25–29 October 2009. [Google Scholar]
- Ranlet, D.; Daddazio, R.; Weidlinger, A. A submerged shock response problem suitable for use as a benchmark. In Proceedings of the 67th Shock and Vibration Symposium, Arlington, VA, USA, 18–22 November 1996. [Google Scholar]
- Yu, J.; Liu, J.H.; Wang, H.K.; Wang, J.; Zhang, L.P.; Liu, G.Z. Numerical simulation of underwater explosion cavitation characteristics based on phase transition model in compressible multicomponent fluids. Ocean. Eng. 2021, 240, 109934. [Google Scholar] [CrossRef]
- Yu, J.; Liu, J.H.; Wang, H.K.; Wang, J.; Zhou, Z.T.; Mao, H.B. Application of two-phase transition model in underwater explosion cavitation based on compressible multiphase flows. AIP. Advs. 2022, 12, 025209. [Google Scholar] [CrossRef]
- Shukla, R.K.; Pantano, C.; Freund, J.B. An interface capturing method for the simulation of multi-phase compressible flows. J. Comput. Phys. 2010, 229, 7411–7439. [Google Scholar] [CrossRef]
- Wang, G.H.; Zhang, S.R.; Yu, M.; Li, H.B.; Kong, Y. Investigation of the shock wave propagation characteristics and cavitation effects of underwater explosion near boundaries. Appl. Ocean. Res. 2014, 16, 40. [Google Scholar] [CrossRef]
- Deshpande, M.; Feng, J.; Merkle, C.L. Cavity flow predictions based on the Euler equations. J. Fluid. Eng. 1994, 116, 36. [Google Scholar] [CrossRef]
- Deshpande, M.; Feng, J.; Merkle, C.L. Numerical modeling of the thermodynamic effects of cavitation. J. Fluid. Eng. 1997, 119, 420. [Google Scholar] [CrossRef]
- Daramizadeh, A.; Ansari, M.R. Numerical simulation of underwater explosion near air-water free surface using a five-equation reduced model. Ocean. Eng. 2015, 110, 25. [Google Scholar] [CrossRef]
- Qin, J.R.; Yu, S.J.; Lai, M.C. Direct calculations of cavitating flows in fuel delivery pipe by the space-time CE/SE method. SAE Trans. 1999, 108, 1720. [Google Scholar]
- Aanhold, J.E.; Meijer, G.J.; Lemmen, P.P.M. Underwater shock response analysis on a floating vessel. Shock Vib. 1998, 5, 53. [Google Scholar] [CrossRef]
- Schmidt, D.P.; Rutland, C.J.; Corradini, M.L. A fully compressible, two-dimensional model of small, high speed, cavitating nozzles. Atomiz Sprays. 1999, 9, 255. [Google Scholar] [CrossRef]
- Rungsiyaphornrata, S.; Klaseboerb, E.; Khoo, B.C.; Yeo, K.S. The merging of two gaseous bubbles with an application to underwater explosions. Comput. Fluid 2003, 32, 1049. [Google Scholar] [CrossRef]
- Liu, T.G.; Khoo, B.C.; Xie, W.F. Isentropic one-fluid modelling of unsteady cavitating flow. J. Comp. Phys. 2004, 201, 80. [Google Scholar] [CrossRef]
- Zhang, A.M.; Li, S.M.; Cui, P.; Li, S.; Liu, Y.L. A unified theory for bubble dynamics. Phys. Fluids 2023, 35, 033323. [Google Scholar] [CrossRef]
- Pelanti, M.; Shyue, K.M. A mixture-energy-consistent six-equation two-phase numerical model for fluid with interfaces, cavitation and evaporation waves. J. Comput. Phys. 2014, 259, 331. [Google Scholar] [CrossRef]
- Pelanti, M.; Shyue, K.M. A numerical model for multiphase liquid-vapor-gas flows with interfaces and cavitation. Int. J. Multiphase. Flow. 2019, 113, 208. [Google Scholar] [CrossRef]
- Chiapolino, A.; Boivin, P.; Saurel, R. A simple phase transition relaxation solver for liquid–vapor flows. Int. J. Numer. Methods Fluids 2016, 83, 583. [Google Scholar] [CrossRef]
- Chiapolino, A.; Boivin, P.; Saurel, R. A simple and fast phase transition relaxation solver for compressible multicomponent two-phase flows. Comput. Fluids 2017, 150, 31. [Google Scholar] [CrossRef]
- Zhang, J. A simple and effective five-equation two-phase numerical model for liquid-vapor phase transition in cavitating flows. Int. J. Multiph. Flow. 2021, 132, 103417. [Google Scholar] [CrossRef]
- Metayer, L.; Saurel, R. The Noble-Abel Stiffened-Gas equation of state. Phys. Fluids 2016, 28, 046102. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, J.; Zhang, X.; Zhao, Y.; Zhang, L.; Chen, J.; Xu, Y. Study on the Influence of a Rigid Wall on Cavitation in Underwater Explosions Near the Free Surface. Appl. Sci. 2024, 14, 1822. https://doi.org/10.3390/app14051822
Yu J, Zhang X, Zhao Y, Zhang L, Chen J, Xu Y. Study on the Influence of a Rigid Wall on Cavitation in Underwater Explosions Near the Free Surface. Applied Sciences. 2024; 14(5):1822. https://doi.org/10.3390/app14051822
Chicago/Turabian StyleYu, Jun, Xianpi Zhang, Yanjie Zhao, Lunping Zhang, Jiping Chen, and Yuanqing Xu. 2024. "Study on the Influence of a Rigid Wall on Cavitation in Underwater Explosions Near the Free Surface" Applied Sciences 14, no. 5: 1822. https://doi.org/10.3390/app14051822
APA StyleYu, J., Zhang, X., Zhao, Y., Zhang, L., Chen, J., & Xu, Y. (2024). Study on the Influence of a Rigid Wall on Cavitation in Underwater Explosions Near the Free Surface. Applied Sciences, 14(5), 1822. https://doi.org/10.3390/app14051822






