Nonlinear Semi-Numeric and Finite Element Analysis of Three-Point Bending Tests of Notched Polymer Fiber-Reinforced Concrete Prisms
Abstract
1. Introduction
2. Materials and Methods
3. Results
4. Calculation
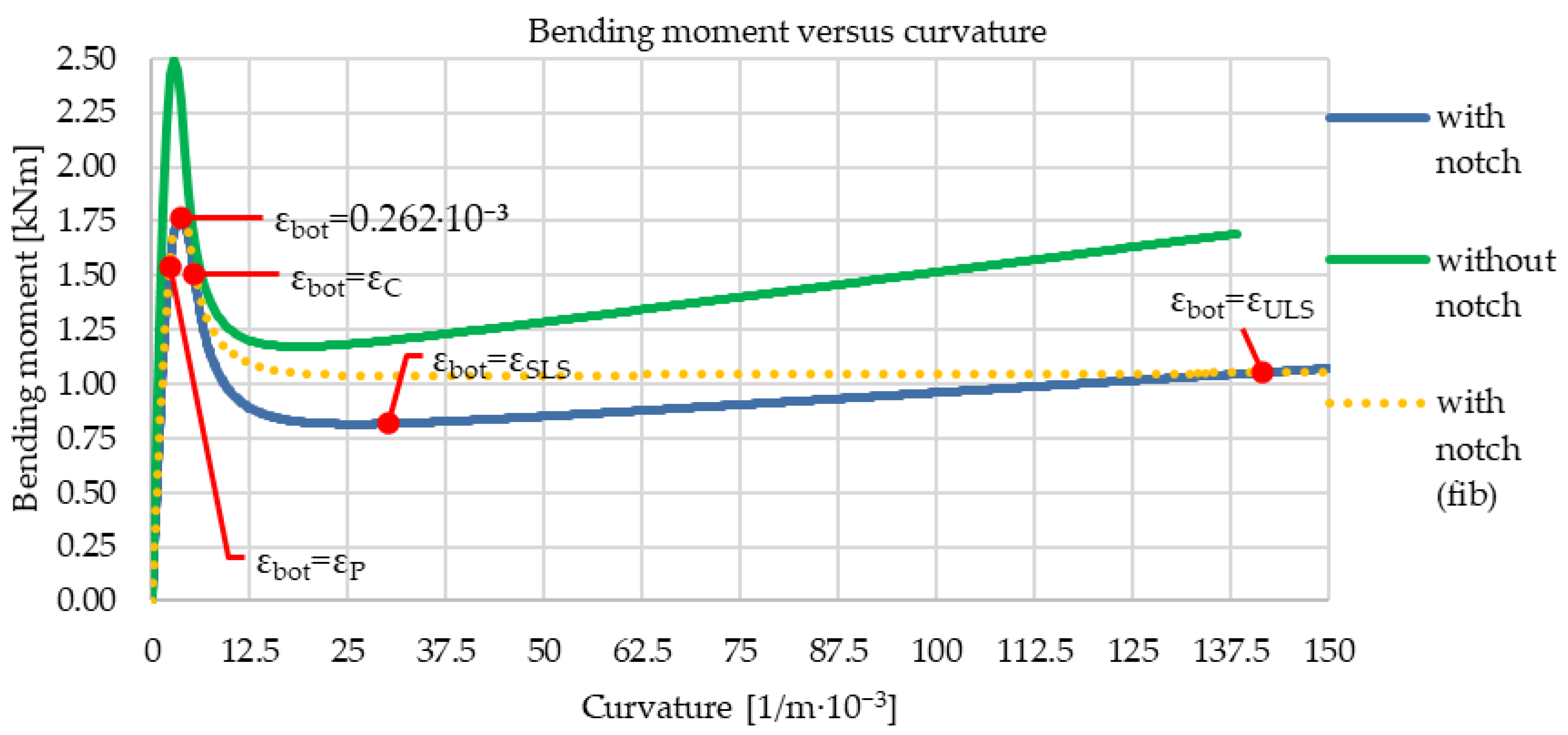
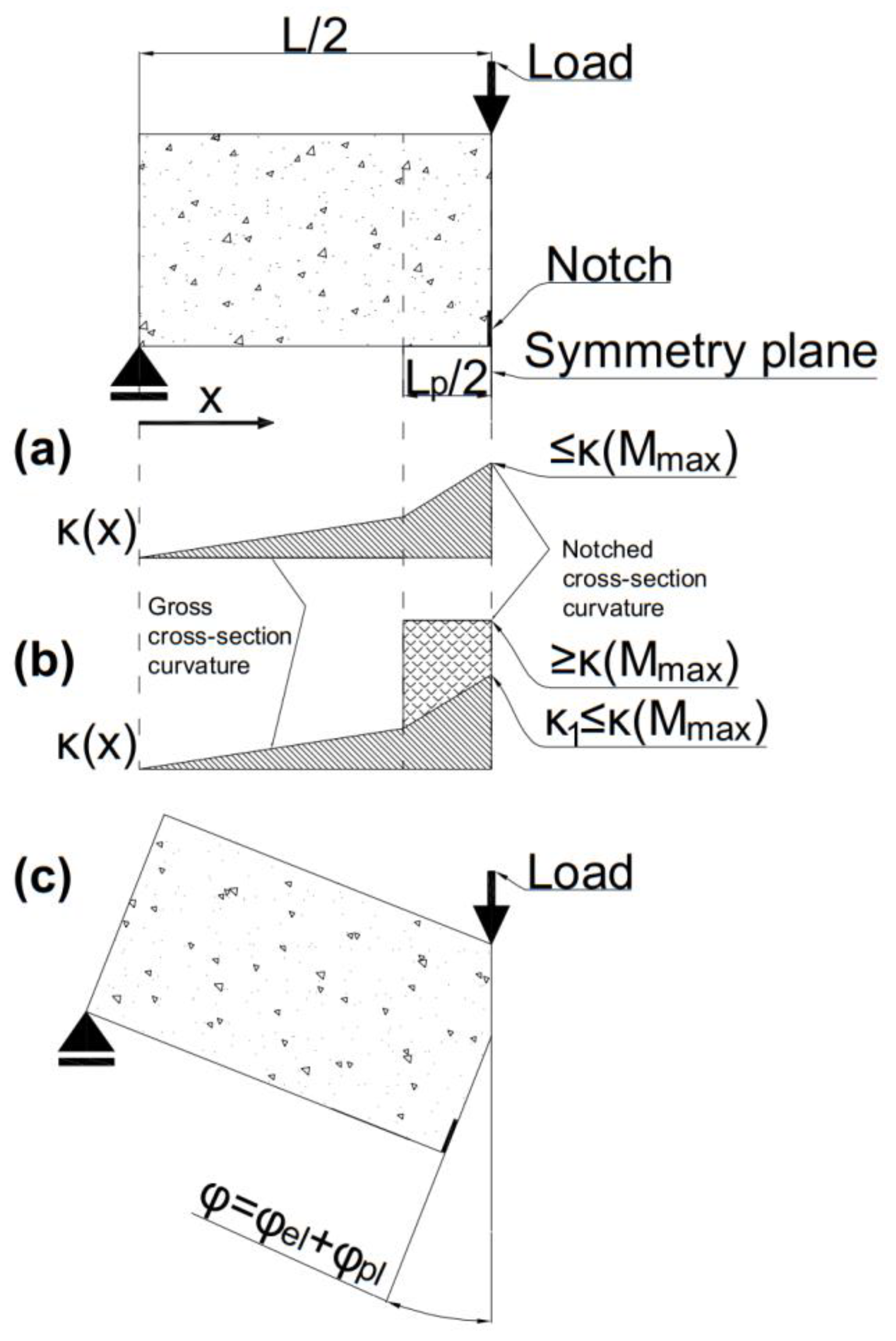
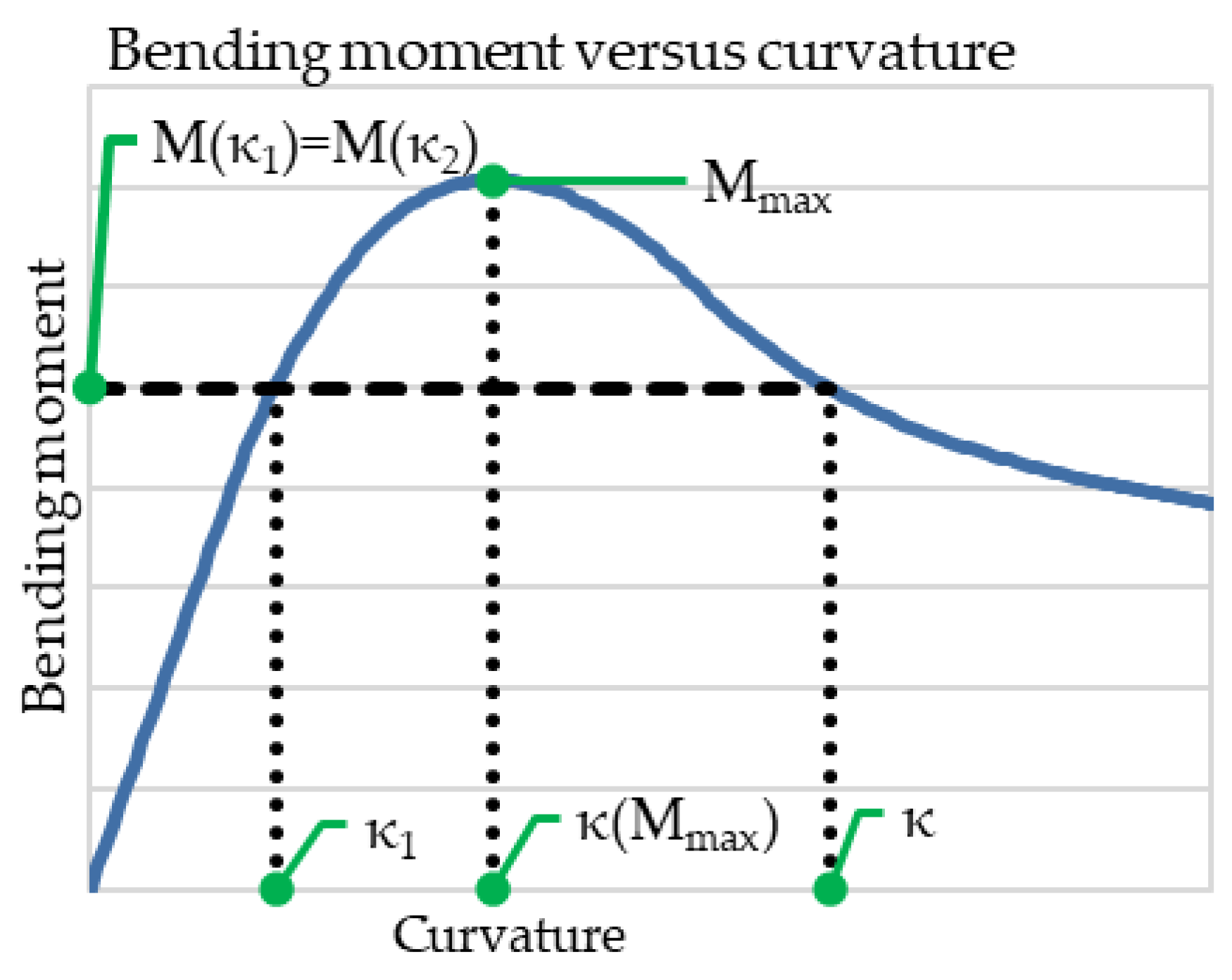
4.1. Moment-Curvature Relation Calculation
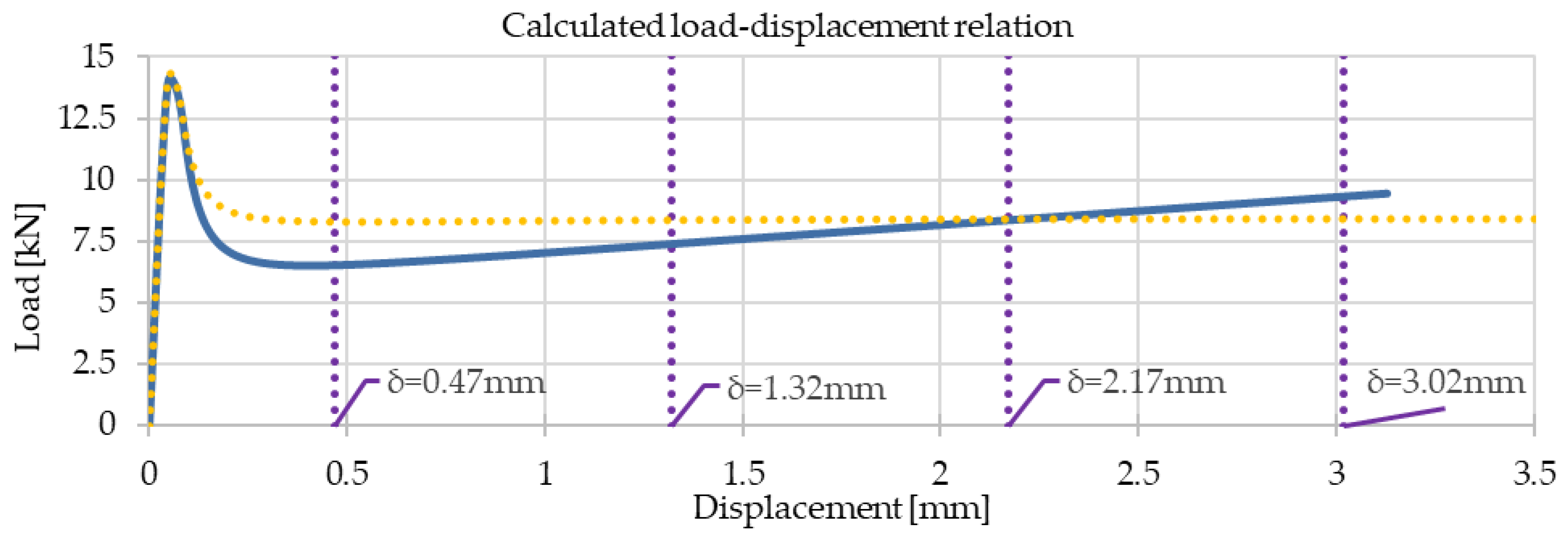
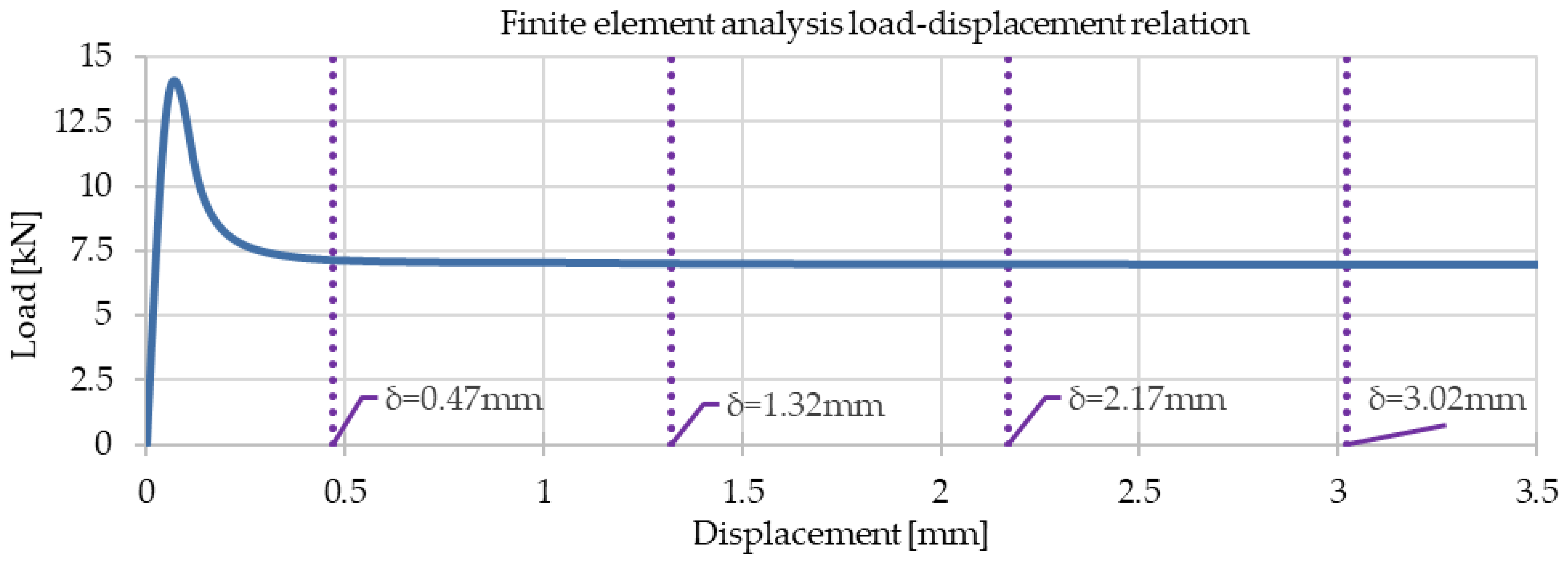
Load-Displacement Calculation
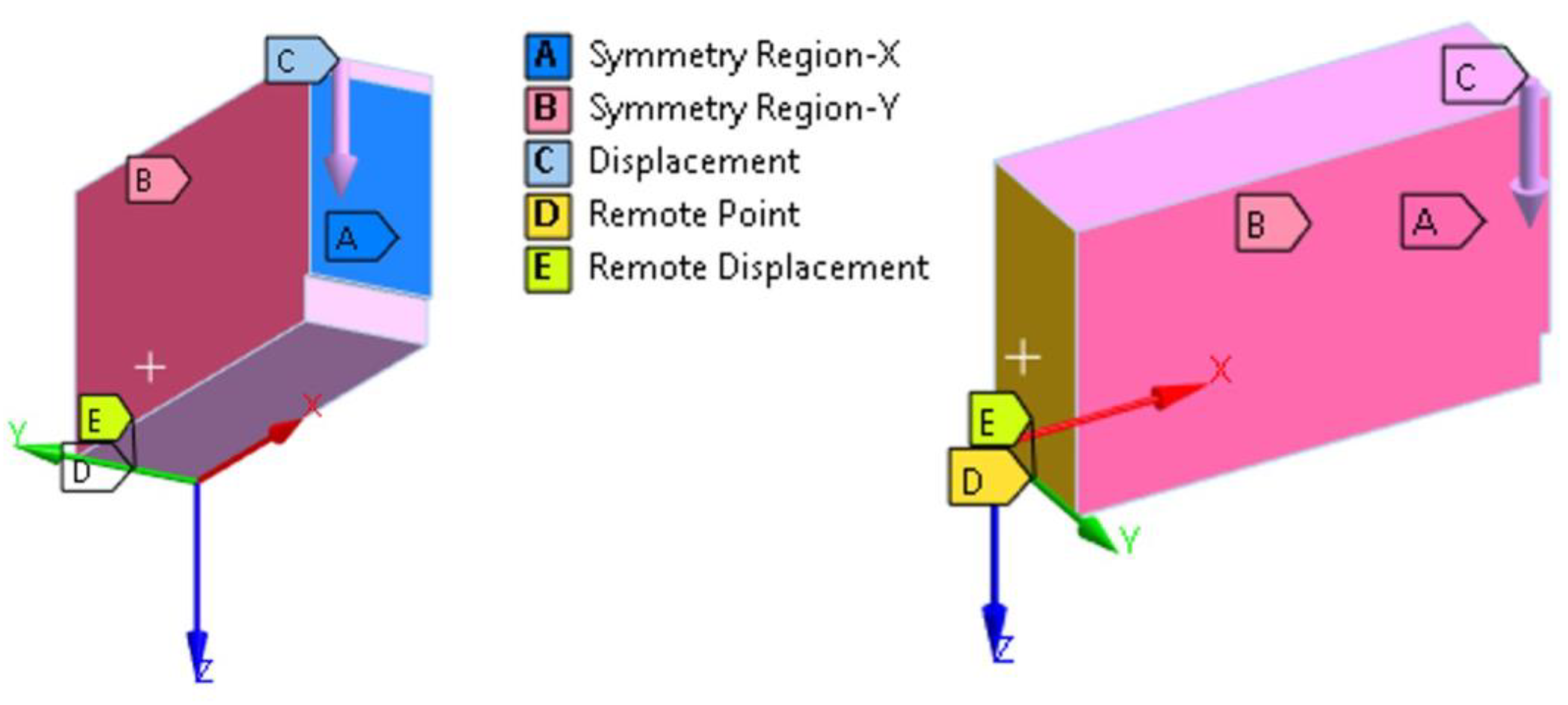
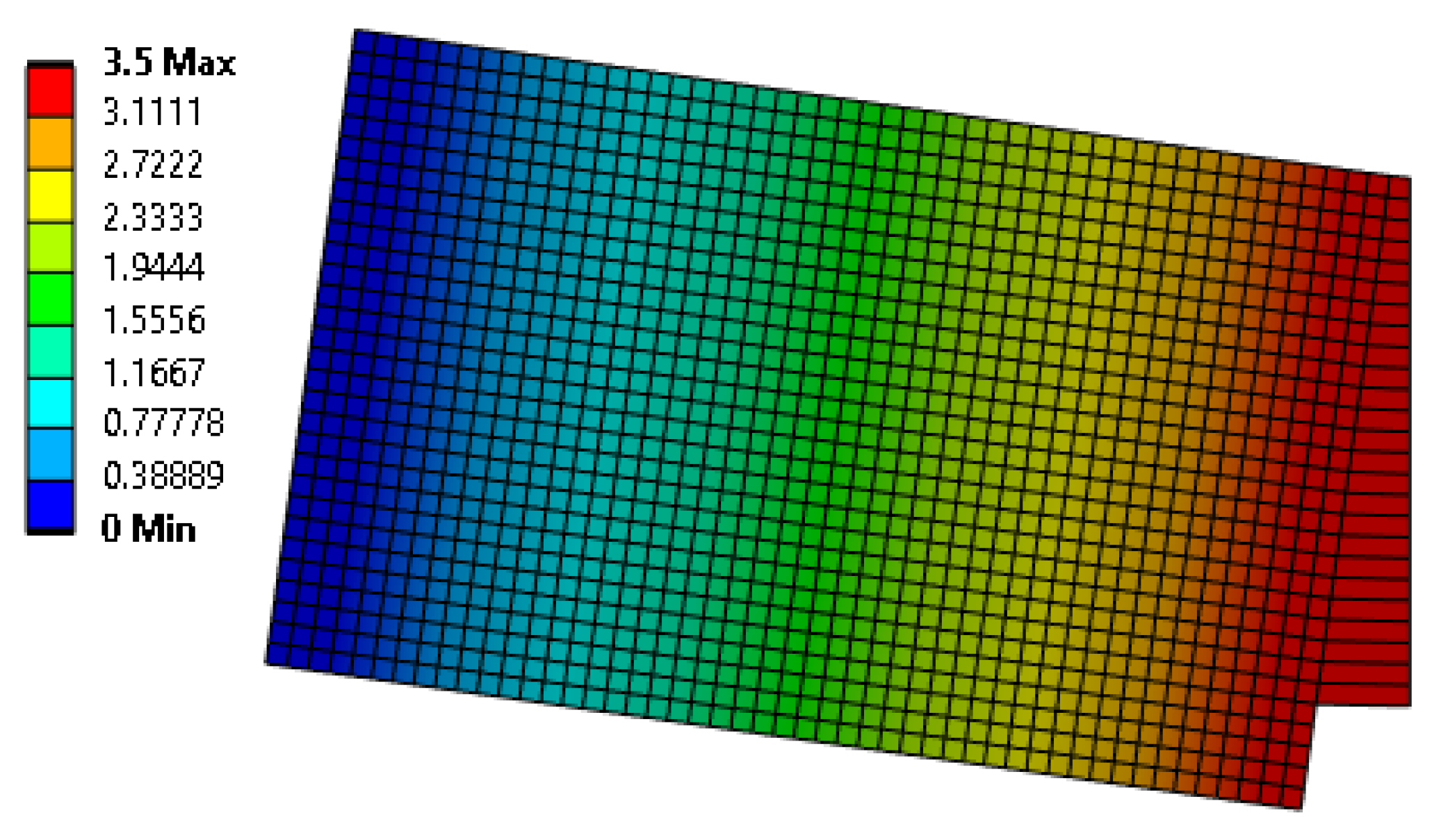
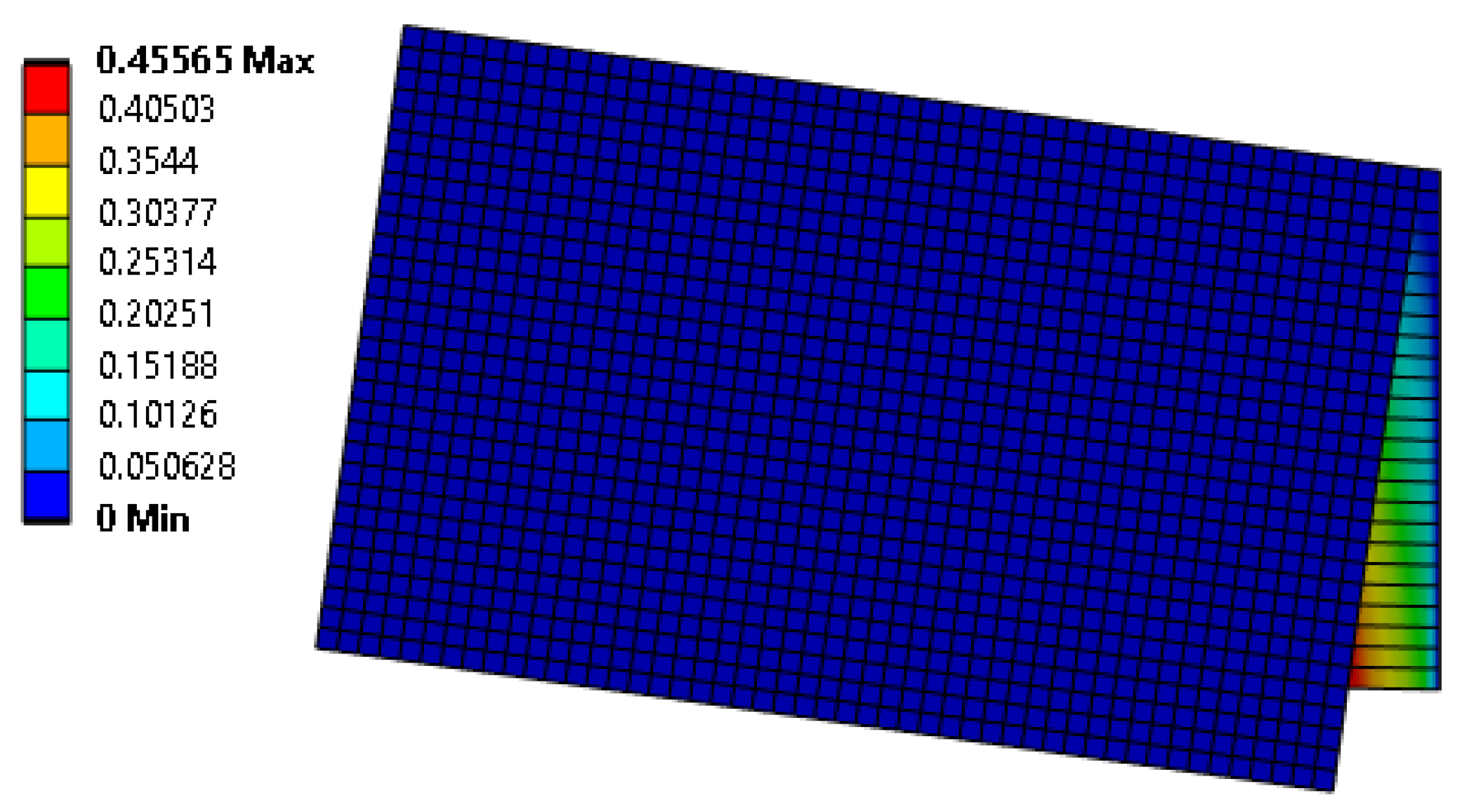
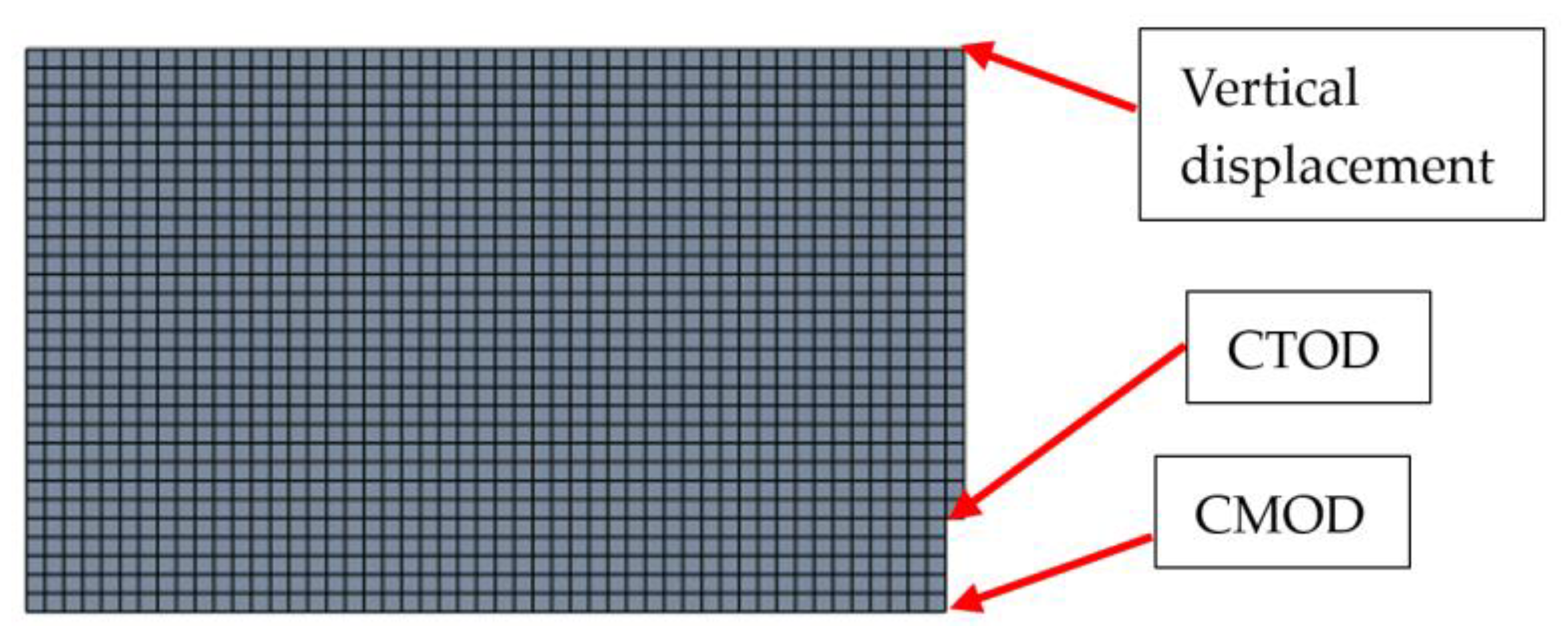
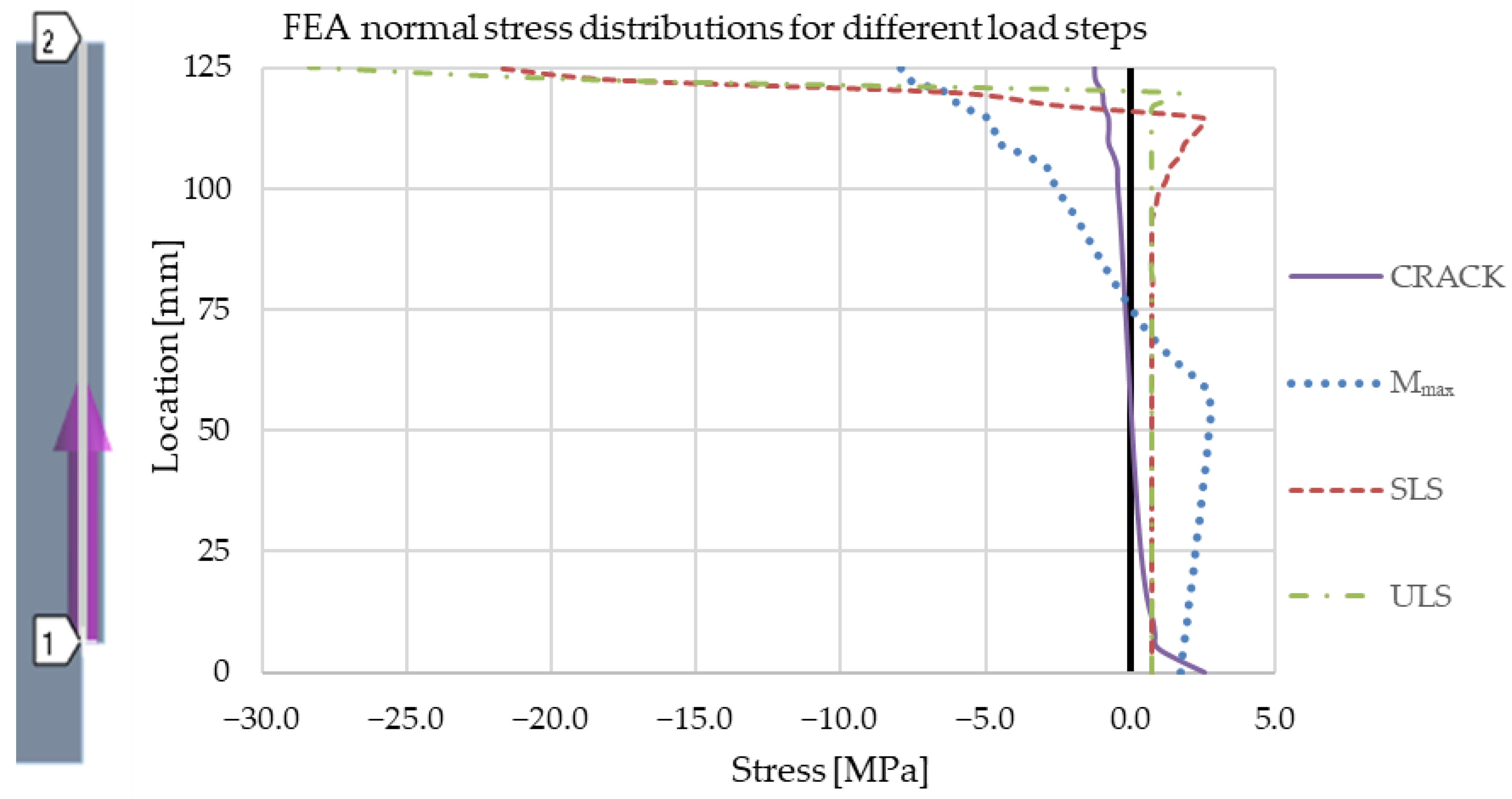
4.2. Finite Element Simulation of the Three-Point Bending Test
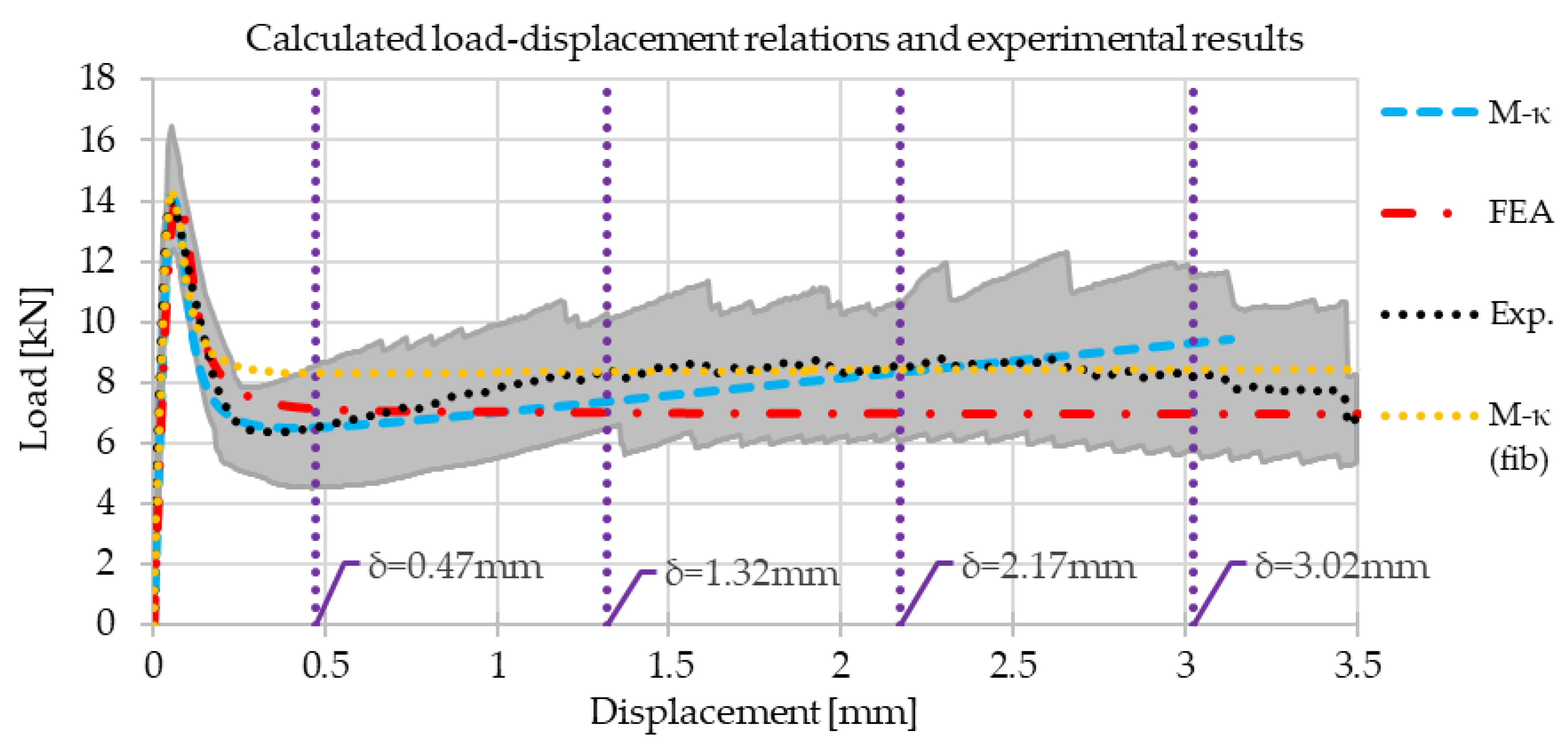
4.3. Comparison of the Experimental, Moment-Curvature-Based Calculation, and Finite Element Analysis Results
5. Conclusions
- All fiber-reinforced prisms showed the same failure mode: a crack occurred at the notched cross-section. The fiber-reinforced prisms showed softening behavior (the residual load-bearing capacity was less than the load at (or shortly after) crack formation). However, the load started to increase again for larger displacements. This behavior can be described as post-crack hardening behavior.
- The compressive strength of the fiber-reinforced concrete cubes was slightly lower than the compressive strength of the plain concrete cubes. This can be attributed to the relatively high fiber dosage.
- The nonlinear semi-numeric computational procedure based on the moment-curvature relation with back-calculated tensile strength properties agreed with the averaged experimental results. The result matching was especially good at the maximum load and CMOD values of 0.5 mm and 2.5 mm. The calculated load-displacement curve matched (accurate to around 0.05 mm) the averaged experimental results at the maximum load and at CMOD values of 0.5 mm and 2.5 mm. This agreement can be attributed to the fact that the tensile strength properties for the calculation were determined by an inverse analysis, where the maximum load and loads at presumed CMOD values of 0.5 mm and 2.5 mm were matched with the experimental results. The load-displacement calculation proves that the back-calculated (by inverse analysis) mechanical properties (tensile strength and residual tensile strengths at CMOD values of 0.5 mm and 2.5 mm) are correct.
- The nonlinear semi-numeric computational procedure based on the moment-curvature relation with tensile strength properties calculated with simplified expressions from the fib Model Code for Concrete Structures 2010 [17] was in relatively lesser agreement with the averaged experimental results, whereas the calculated load-displacement curve closely aligned with the averaged experimental result at the maximum load, with an accuracy of approximately 0.02 mm and 0.19 kN, and at the CMOD value of 2.5 mm, with an accuracy of approximately 0.06 mm and 0.01 kN, the alignment of the load-displacement results demonstrated less than satisfactory alignment for the CMOD value of 0.5 mm, with an accuracy of approximately 0.02 mm and 1.75 kN, indicating a notable discrepancy between the experimental and computational outcomes. The relatively lesser agreement can be attributed to the fact that tensile strength properties were calculated with simplified expressions from the fib Model Code for Concrete Structures 2010 [17], which resulted in a notably higher residual tensile strength at the CMOD value of 0.5 mm and a lower residual tensile strength at the CMOD value of 2.5 mm compared with the back-calculated residual tensile strength values. Contrary to the experimental observations and contrary to the back-calculated residual tensile strength values, the residual tensile strength values calculated with simplified expressions from the fib Model Code for Concrete Structures 2010 [17] give an impression of post-crack softening behavior (with the difference between the residual tensile strength at the CMOD value of 0.5 mm being approximately 0.01 kN lower than the residual tensile strength at the CMOD value of 2.5 mm).
- The finite element analysis with the Menetrey-Willam material model, which used the back-calculated tensile strength and considered a constant residual tensile strength equal to the back-calculated residual tensile strength at the CMOD value of 0.5 mm, was in expectedly lesser agreement with the averaged experimental results compared with the computational procedure based on the moment-curvature relation with back-calculated tensile strength properties. In comparison with the computational procedure relying on the moment-curvature relation, wherein tensile strength properties were determined through simplified expressions drawn from the fib Model Code for Concrete Structures 2010 [17], the correlation between the finite element analysis load-displacement outcomes and the experimental results did not exhibit a degradation in quality: the results at the maximum load were accurate to 0.02 mm and 0.05 kN, at the CMOD value of 0.5 mm the results were accurate to 0.02 mm and 0.61 kN, and the results at the CMOD value of 2.5 mm were accurate to approximately 0.05 mm and 1.44 kN. Considering the straightforward Menetrey-Willam material model with its concise input parameters, performing finite element analyses with this material model and a carefully chosen constant residual tensile strength presents a practical way to analyze structural elements made of fiber-reinforced concrete. In this study, we picked the lowest value of the residual tensile strength within the CMOD range of 0.5 mm to 2.5 mm for the constant residual tensile strength. Consequently, the finite element analysis results present a lower limit of the investigated fiber-reinforced concrete prisms’ behavior within the CMOD range of 0.5 mm to 2.5 mm. Although the force results slightly overestimated the load-bearing capacity around a CMOD value of 0.5 mm, the calculated forces still came closer to the experimentally determined value (at a CMOD of 0.5 mm) compared with the results of the nonlinear semi-numeric computational procedure based on the moment-curvature relation where tensile strength properties were determined using simplified expressions from the fib Model Code for Concrete Structures 2010 [17].
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Balea, A.; Fuente, E.; Monte, M.C.; Blanco, Á.; Negro, C. 20—Fiber Reinforced Cement Based Composites. In Fiber Reinforced Composites; Joseph, K., Oksman, K., George, G., Wilson, R., Appukuttan, S., Eds.; Woodhead Publishing Series in Composites Science and Engineering; Woodhead Publishing: Duxford, UK, 2021; pp. 597–648. ISBN 978-0-12-821090-1. [Google Scholar]
- Amin, A.; Foster, S.J.; Gilbert, R.I.; Kaufmann, W. Material Characterisation of Macro Synthetic Fibre Reinforced Concrete. Cem. Concr. Compos. 2017, 84, 124–133. [Google Scholar] [CrossRef]
- Design of Steel Fibre Reinforced Concrete Using the σ-w Method: Principles and Applications. Mater. Struct. 2002, 35, 262–278. [CrossRef]
- Pedersen, C. New Production Processes, Materials and Calculation Techniques for Fibre Reinforced Pipes. Ph.D. Thesis, Technical University of Denmark, Kongens Lyngby, Denmark, 1996. [Google Scholar]
- Casanova, P.; Rossi, P. Analysis and Design of Steel Fiber Reinforced Concrete Beams. Struct. J. 1997, 94, 595–602. [Google Scholar]
- Stang, H.; Olesen, J.F. On the Interpretation of Bending Tests on FRC-Materials. In Proceedings of the FRAMCOS-3, Gifu, Japan, 12–16 October 1998; Aedificatio Publishers: Freiburg, Germany, 1998; pp. 511–520. [Google Scholar]
- Zhang, J.; Stang, H. Applications of Stress Crack Width Relationship in Predicting the Flexural Behavior of Fibre-Reinforced Concrete. Cem. Concr. Res. 1998, 28, 439–452. [Google Scholar] [CrossRef]
- Tada, H.; Paris, P.C.; Irwin, G.R. The Stress Analysis of Cracks Handbook, 2nd ed.; Paris Productions & (Del Research Corp.): St. Louis, MI, USA, 1985. [Google Scholar]
- Soranakom, C.; Mobasher, B. Closed-Form Solutions for Flexural Response of Fiber-Reinforced Concrete Beams. J. Eng. Mech. 2007, 133, 933–941. [Google Scholar] [CrossRef]
- Volpatti, G.; Martínez, J.A.; Diaz, J.C.; Zampini, D. Advanced Closed-Form Moment-Curvature Formulation for Fiber-Reinforced Concrete Members. Compos. Struct. 2022, 279, 114755. [Google Scholar] [CrossRef]
- Blanco, A.; Pujadas, P.; De la Fuente, A.; Cavalaro, S.; Aguado, A. Application of Constitutive Models in European Codes to RC–FRC. Constr. Build. Mater. 2013, 40, 246–259. [Google Scholar] [CrossRef]
- Unuk, Ž.; Kuhta, M. Full-Scale Test and Load-Bearing Capacity Evaluation of Synthetic-Polymer-Fiber-Reinforced Concrete Tetrapods under Quasi-Static Loading. Buildings 2022, 12, 2143. [Google Scholar] [CrossRef]
- Di Prisco, M.; Colombo, M.; Dozio, D. Fibre-reinforced Concrete in Fib Model Code 2010: Principles, Models and Test Validation. Struct. Concr. 2013, 14, 342–361. [Google Scholar] [CrossRef]
- Enfedaque, A.; Suárez, F.; Alberti, M.G.; Gálvez, J.C. Suitability of Constitutive Models of the Structural Concrete Codes When Applied to Polyolefin Fibre Reinforced Concrete. Materials 2022, 15, 2323. [Google Scholar] [CrossRef] [PubMed]
- Zhang, H.; Huang, Y.J.; Yang, Z.J.; Xu, S.L.; Chen, X.W. A Discrete-Continuum Coupled Finite Element Modelling Approach for Fibre Reinforced Concrete. Cem. Concr. Res. 2018, 106, 130–143. [Google Scholar] [CrossRef]
- Farsi, A.; Bedi, A.; Latham, J.P.; Bowers, K. Simulation of Fracture Propagation in Fibre-Reinforced Concrete Using FDEM: An Application to Tunnel Linings. Comput. Part. Mech. 2020, 7, 961–974. [Google Scholar] [CrossRef]
- Fédération Internationale Du Béton/International Federation for Structural Concrete (fib). fib Model Code for Concrete Structures 2010; Wilhelm Ernst & Sohn, Verlag für Architektur und Technische Wissenschaften GmbH & Co. KG: Berlin, Germany, 2013. [Google Scholar]
- Ansys® Mechanical APDL, Release 2022 R2, Help System, Material Reference; ANSYS, Inc.: Canonsburg, PA, USA, 2022.
- EN 14651: 2005+A1: 2007; Test Method for Metallic Fibre Concrete–Measuring the Flexural Tensile Strength (Limit of Proportionality (LOP), Residual). European Committee for Standardization: Brussels, Belgium, 2007.
- EN 1992-1-1; Eurocode 2: Design of Concrete Structures—Part 1-1: General Rules and Rules for Buildings. European Committee for Standardization: Brussels, Belgium, 2004.
- Leaders in Concrete & Concrete Products: BarChipInc. Available online: https://barchip.com/product/ (accessed on 13 November 2023).
- EN 14889-2:2006; Fibres for Concrete Polymer Fibres. Definitions, Specifications and Conformity. European Committee for Standardization: Brussels, Belgium, 2006.
- EN 12390-3; Testing Hardened Concrete–Part 3: Compressive Strength of Test Specimens. European Committee for Standardization: Brussels, Belgium, 2019.
- Hordijk, D. Local Approach to Fatigue of Concrete. Ph.D. Thesis, Delft University and Technology, Delft, The Netherlands, 1991. [Google Scholar]
- de Montaignac, R.; Massicotte, B.; Charron, J.-P. Design of SFRC Structural Elements: Flexural Behaviour Prediction. Mater. Struct. 2012, 45, 623–636. [Google Scholar] [CrossRef]
- Krastev, R. Consistent Presentation of the Beam Deflection Theory Including Shear Correction. Int. Sci. J. Math. Model. 2021, 5, 120–123. [Google Scholar]
- Ansys® Mechanical, Release 2022 R2, Help System, Mechanical User’s Guide; ANSYS, Inc.: Canonsburg, PA, USA, 2022.
- Ansys® Mechanical APDL, Release 2022 R2, Help System, Element Reference; ANSYS, Inc.: Canonsburg, PA, USA, 2022.
- Park, S.H.; Bang, K.H.; Cho, J.R. Structural Integrity Evaluation of a Reactor Cavity during a Steam Explosion for External Reactor Vessel Cooling. Energies 2021, 14, 3605. [Google Scholar] [CrossRef]
- Pokorska, I.; Poński, M.; Kubissa, W.; Libura, T.; Brodecki, A.; Kowalewski, Z. Computational Fracture Evolution Analysis of Steel-Fiber-Reinforced Concrete Using Concrete Continuous Damage and Fiber Progressive Models. Materials 2023, 16, 5635. [Google Scholar] [CrossRef] [PubMed]
- CEB-FIP MODEL CODE 1990; Thomas Telford Publishing: London, UK, 1993.


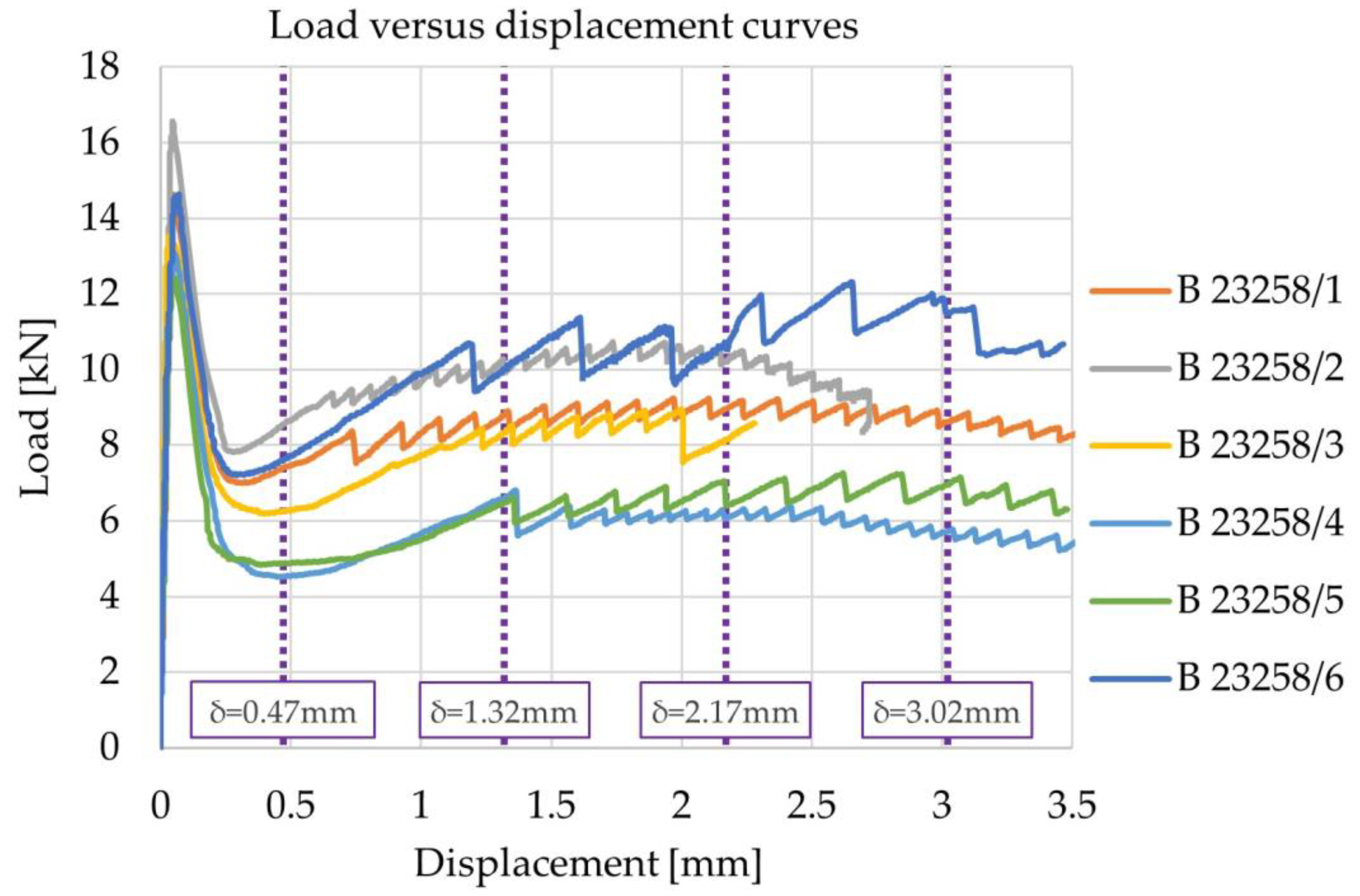




| B23258/1 | B23258/2 | B23258/3 | B23258/4 | B23258/5 | B23258/6 | |
|---|---|---|---|---|---|---|
| B [mm] | 150.33 | 149.57 | 149.62 | 149.21 | 149.87 | 150.96 |
| Hn [mm] | 125.06 | 125.03 | 125.01 | 125 | 125.2 | 125.06 |
| Component | Dosage [kg/m3] |
|---|---|
| Aggregate (maximum grain size: 16 mm) | 711 |
| Cement (CEM II/A-S 52.5 N) | 135 |
| Total water | 71.4 |
| Plasticizer | 0.4 |
| Barchip 48 fibers | 5 |
| Property | Value/Description |
|---|---|
| Tensile strength [MPa] | 640 |
| Young’s modulus [GPa] | 12 |
| Length [mm] | 48 |
| Base material | Virgin polypropylene |
| B23258/1 | B23258/2 | B23258/3 | B23258/4 | B23258/5 | B23258/6 | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| i | Fi [kN] | σi [MPa] | Fi [kN] | σi [MPa] | Fi [kN] | σi [MPa] | Fi [kN] | σi [MPa] | Fi [kN] | σi [MPa] | Fi [kN] | σi [MPa] | Faver.i [kN] | Fsd.i [kN] | σaver.i [MPa] | σsd.i [MPa] |
| Max. | 14.63 | 4.67 | 16.57 | 5.31 | 13.53 | 4.34 | 13.06 | 4.20 | 12.43 | 3.97 | 14.64 | 4.65 | 14.14 | 1.345 | 4.52 | 0.43 |
| 0.47 | 7.40 | 2.36 | 8.56 | 2.75 | 6.27 | 2.01 | 4.53 | 1.46 | 4.88 | 1.56 | 7.59 | 2.41 | 6.54 | 1.462 | 2.09 | 0.47 |
| 1.32 | 8.80 | 2.81 | 10.11 | 3.24 | 8.32 | 2.67 | 6.61 | 2.13 | 6.48 | 2.07 | 10.02 | 3.18 | 8.39 | 1.449 | 2.68 | 0.46 |
| 2.17 | 8.96 | 2.86 | 10.22 | 3.28 | 8.15 | 2.61 | 6.07 | 1.95 | 6.42 | 2.05 | 10.71 | 3.40 | 8.42 | 1.749 | 2.69 | 0.55 |
| 3.02 | 8.62 | 2.75 | / | / | / | / | 5.71 | 1.84 | 6.96 | 2.22 | 11.49 | 3.65 | 8.20 | 2.166 | 2.61 | 0.68 |
| Without Fibers | With Fibers | |||||
|---|---|---|---|---|---|---|
| BV1 | BV2 | BV3 | ZV1 | ZV2 | ZV3 | |
| fc | 48.53 | 48.44 | 47.64 | 45.38 | 44.76 | 45.87 |
| fc.aver. | 48.21 | 45.33 | ||||
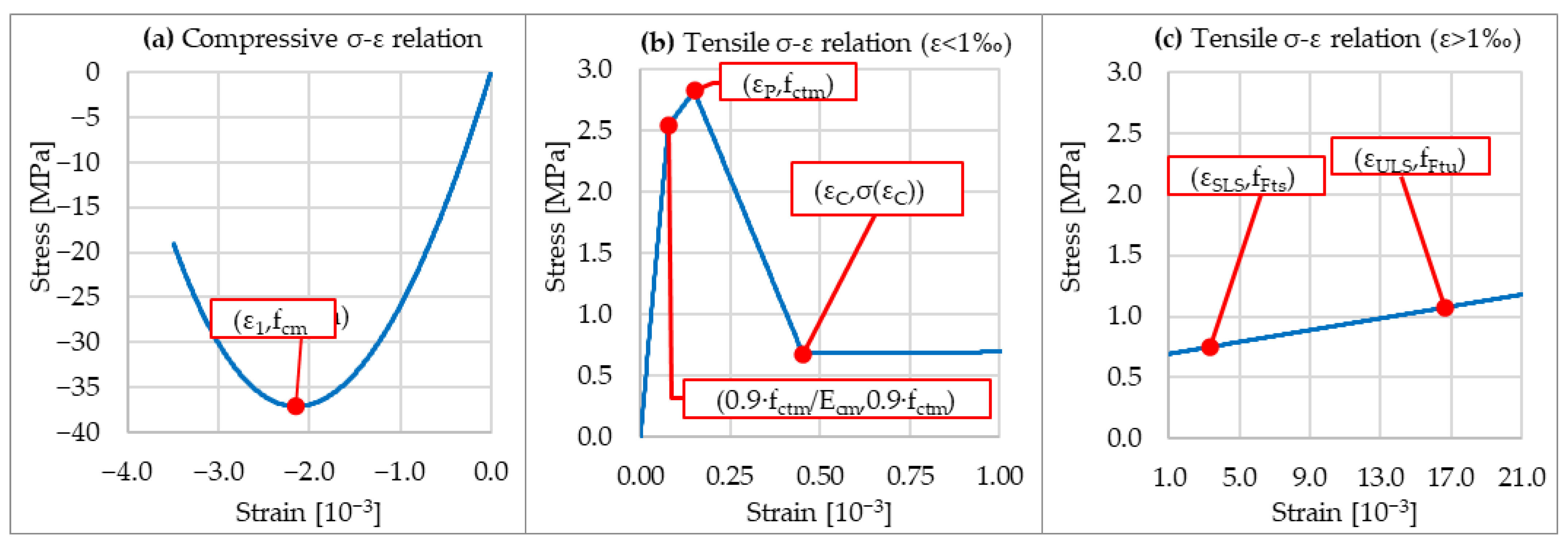
| Property | Unit | Value |
|---|---|---|
| fcm | [MPa] | 37.10 |
| Ecm | [GPa] | 32.60 |
| εc1 | [10−3] | −2.15 |
| GF | [N/m] | 139.90 |
| εP | [10−3] | 0.15 |
| εC | [10−3] | 0.45 |
| εSLS | [10−3] | 3.33 |
| εULS | [10−3] | 16.67 |
| fctm | [MPa] | 2.82 |
| fFts | [MPa] | 0.75 |
| fFtu | [MPa] | 1.07 |
| CMOD [mm] | CTOD [mm] | κ [1/m] | δstandard [mm] | δcalculated [mm] | F [kN] |
|---|---|---|---|---|---|
| 0. | 0 | 2.27 × 10−3 | 0.04 | 0.04 | 12.31 |
| 2.11 × 10−2 | 1.76 × 10−2 | 0.0036 | 0.06 | 0.05 | 14.14 |
| 0.5 | 0.42 | 0.0301 | 0.47 | 0.47 | 6.54 |
| 1.5 | 1.25 | 0.0860 | 1.32 | 1.35 | 7.43 |
| 2.5 | 2.08 | 0.1416 | 2.17 | 2.22 | 8.42 |
| 3.5 | 2.92 | 0.1971 | 3.02 | 3.08 | 9.40 |
| CMOD [mm] | CTOD [mm] | κ [1/m] | δstandard [mm] | δcalculated [mm] | F [kN] |
|---|---|---|---|---|---|
| 0 | 0 | 2.24 × 10−3 | 0.04 | 0.04 | 12.35 |
| 2.09 × 10−2 | 1.74 × 10−2 | 0.0036 | 0.06 | 0.05 | 14.33 |
| 0.5 | 0.42 | 0.0362 | 0.47 | 0.57 | 8.29 |
| 1.5 | 1.25 | 0.1032 | 1.32 | 1.62 | 8.38 |
| 2.5 | 2.08 | 0.1694 | 2.17 | 2.65 | 8.41 |
| 3.5 | 2.92 | 0.2354 | 3.02 | 3.68 | 8.41 |
| Property | Unit | Value |
|---|---|---|
| Young’s modulus | MPa | 3.26 × 104 |
| Poisson’s ratio | / | 2.00 × 10−1 |
| Bulk modulus | MPa | 1.81 × 104 |
| Shear modulus | MPa | 1.36 × 104 |
| Uniaxial compressive strength | MPa | 3.71 × 101 |
| Uniaxial tensile strength | MPa | 2.82 × 100 |
| Biaxial compressive strength | MPa | 4.31 × 101 |
| Plastic strain at uniaxial Compressive strength | / | 1.69 × 10−3 |
| Plastic strain at transition from power law to exponential softening | / | 3.04 × 10−3 |
| Relative stress at start of nonlinear hardening | / | 3.66 × 10−1 |
| Residual relative stress at transition from power law to exponential softening | / | 5.11 × 10−1 |
| Residual compressive relative stress | / | 2.00 × 10−1 |
| Mode 1 area specific fracture energy | N/m | 7.51 × 101 |
| Residual tensile relative stress | / | 2.66 × 10−1 |
| Dilatancy angle | ° | 9 |
| CMOD [mm] | CTOD [mm] | F [kN] | δ [mm] | CMOD/CTOD [/] |
|---|---|---|---|---|
| 0.0032 | 0.0007 | 3.01 | 0.01 | 4.79 |
| 0.0417 | 0.0271 | 14.09 | 0.07 | 1.54 |
| 0.5036 | 0.4113 | 7.15 | 0.45 | 1.22 |
| 2.4978 | 2.0694 | 6.98 | 2.12 | 1.21 |
| Average Experimental Load | Corresponding Experimental Displacement | M-κ Calculated Load | M-κ Calculated Displacement | M-κ (fib) Calculated Load | M-κ (fib) Calculated Displacement | FEA Load | FEA Displacement | |
|---|---|---|---|---|---|---|---|---|
| [kN] | [mm] | [kN] | [mm] | [kN] | [mm] | [kN] | [mm] | |
| Max. load | 14.14 | 0.053 * | 14.14 | 0.051 | 14.33 | 0.051 | 14.09 | 0.067 |
| CMOD = 0.5 mm | 6.54 | 0.465 | 6.54 | 0.473 | 8.29 | 0.480 | 7.15 | 0.452 |
| CMOD = 2.5 mm | 8.42 | 2.165 | 8.42 | 2.216 | 8.41 | 2.220 | 6.98 | 2.117 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Unuk, Ž.; Kuhta, M. Nonlinear Semi-Numeric and Finite Element Analysis of Three-Point Bending Tests of Notched Polymer Fiber-Reinforced Concrete Prisms. Appl. Sci. 2024, 14, 1604. https://doi.org/10.3390/app14041604
Unuk Ž, Kuhta M. Nonlinear Semi-Numeric and Finite Element Analysis of Three-Point Bending Tests of Notched Polymer Fiber-Reinforced Concrete Prisms. Applied Sciences. 2024; 14(4):1604. https://doi.org/10.3390/app14041604
Chicago/Turabian StyleUnuk, Žiga, and Milan Kuhta. 2024. "Nonlinear Semi-Numeric and Finite Element Analysis of Three-Point Bending Tests of Notched Polymer Fiber-Reinforced Concrete Prisms" Applied Sciences 14, no. 4: 1604. https://doi.org/10.3390/app14041604
APA StyleUnuk, Ž., & Kuhta, M. (2024). Nonlinear Semi-Numeric and Finite Element Analysis of Three-Point Bending Tests of Notched Polymer Fiber-Reinforced Concrete Prisms. Applied Sciences, 14(4), 1604. https://doi.org/10.3390/app14041604







